Hysteresis Modeling and Compensation of Piezoelectric Actuators Using Gaussian Process with High-Dimensional Input
Abstract
:1. Introduction
2. Rate-Dependent Hysteresis Nonlinear Modeling of PEA
2.1. Principle of GP-Based Hysteresis Modeling
2.2. Extension of the Input Dimensions
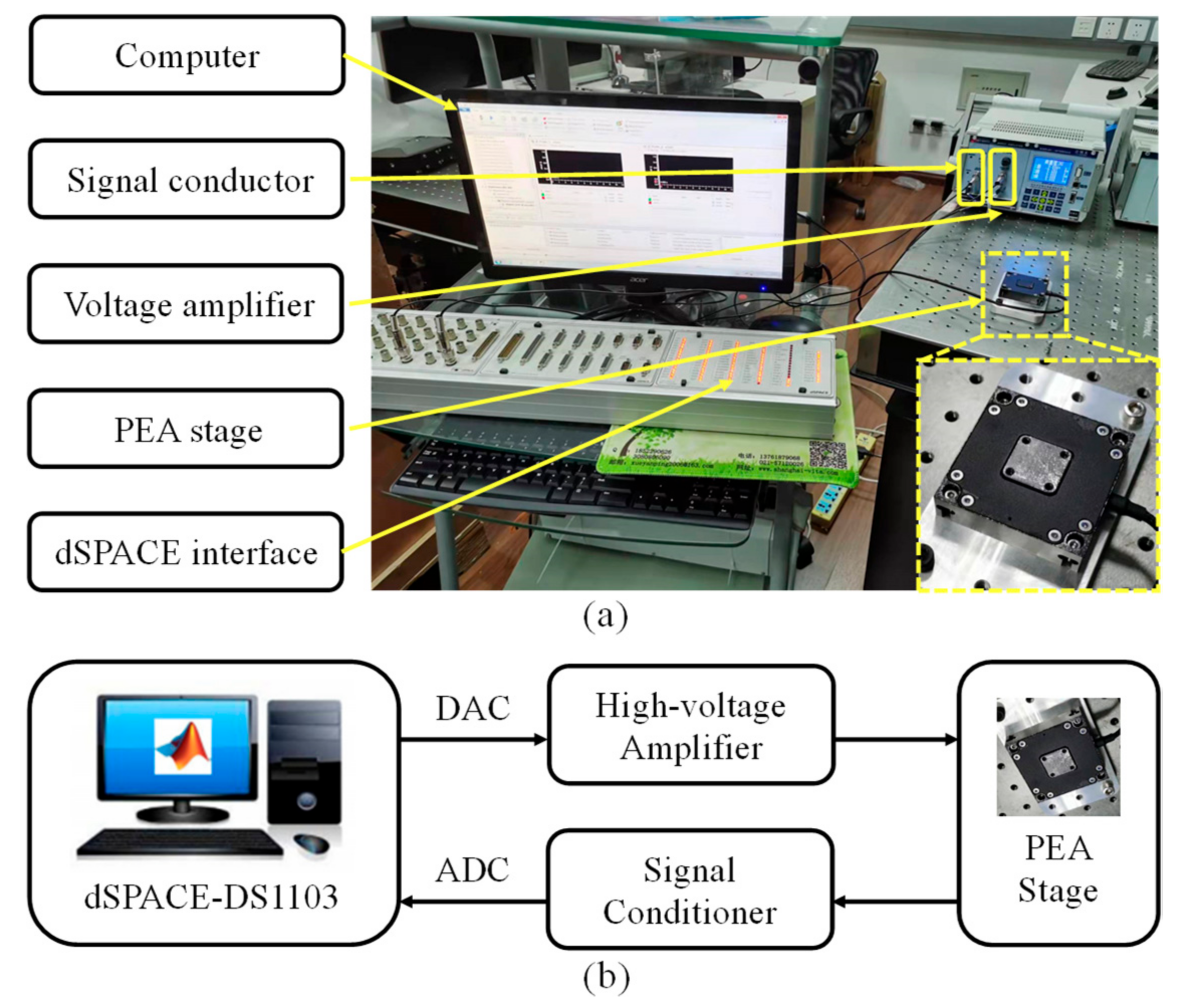
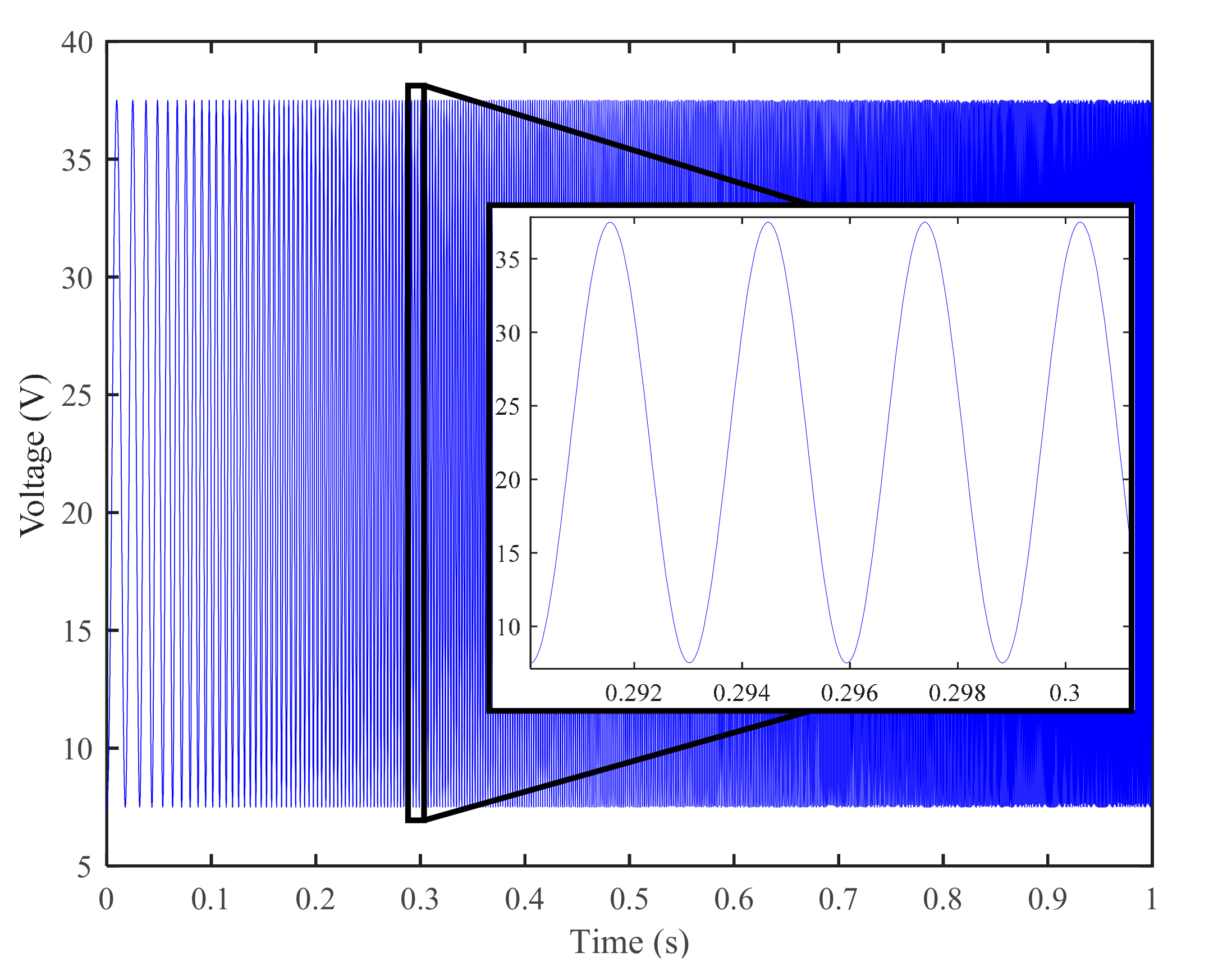
2.3. Experimental Setup
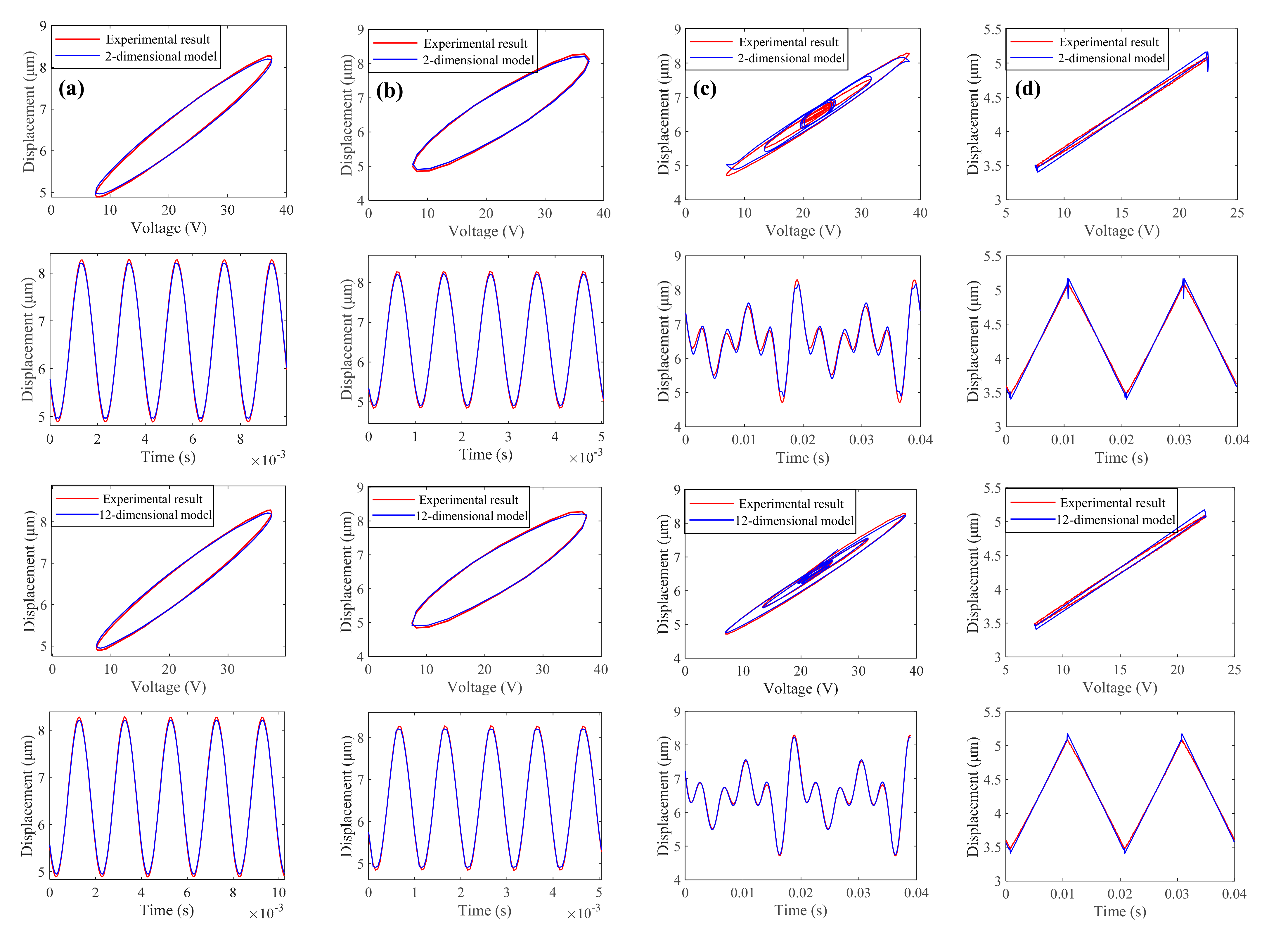
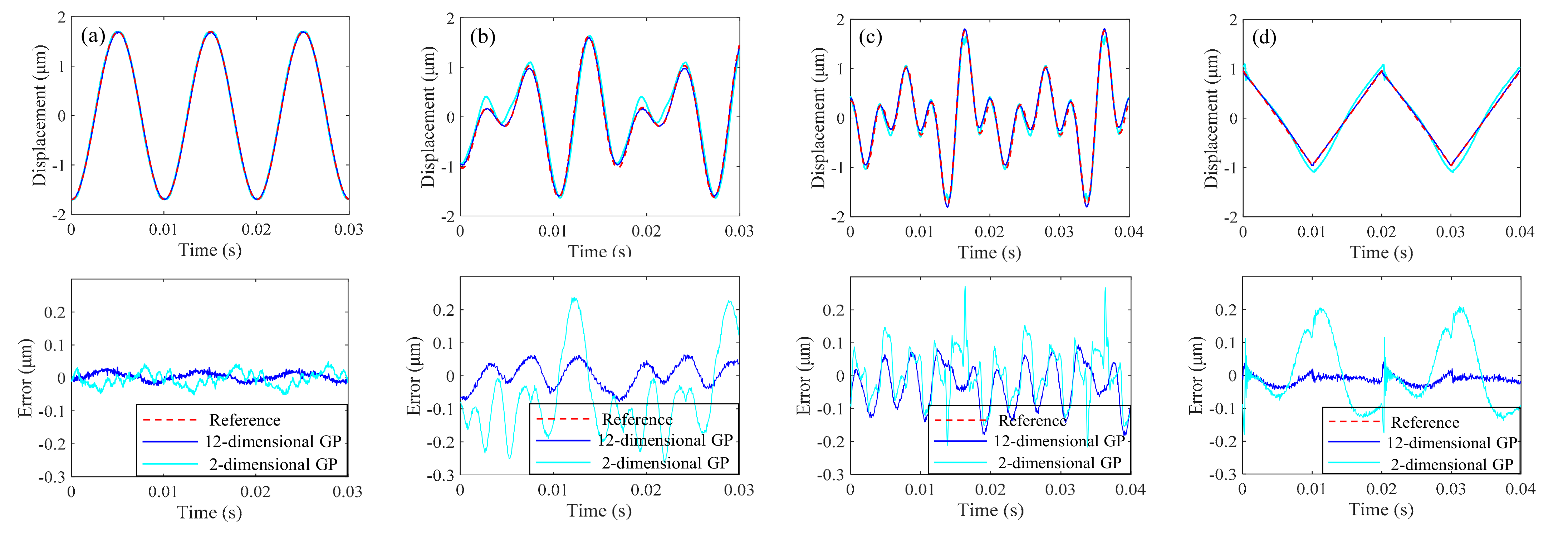
2.4. Modeling Results with Different Input Dimension
3. Controller Design Based on Hysteresis Compensation
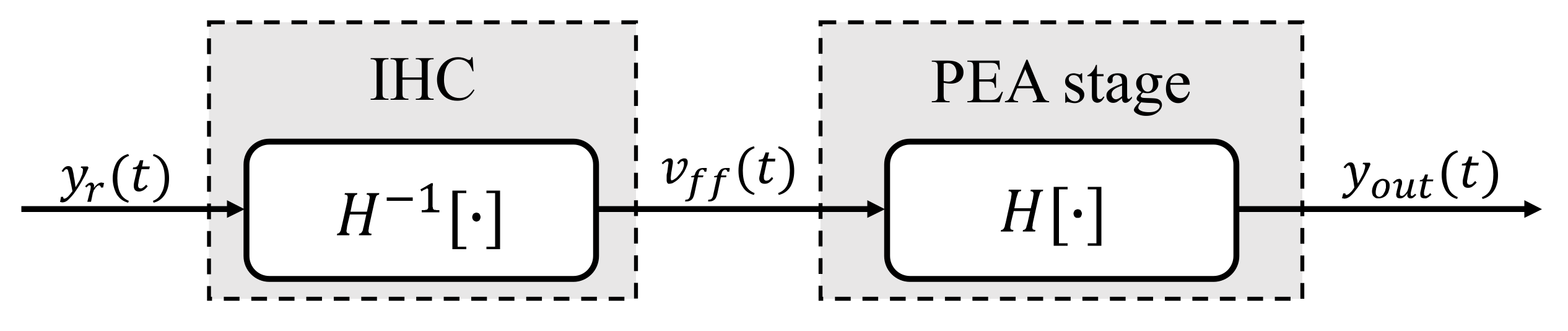
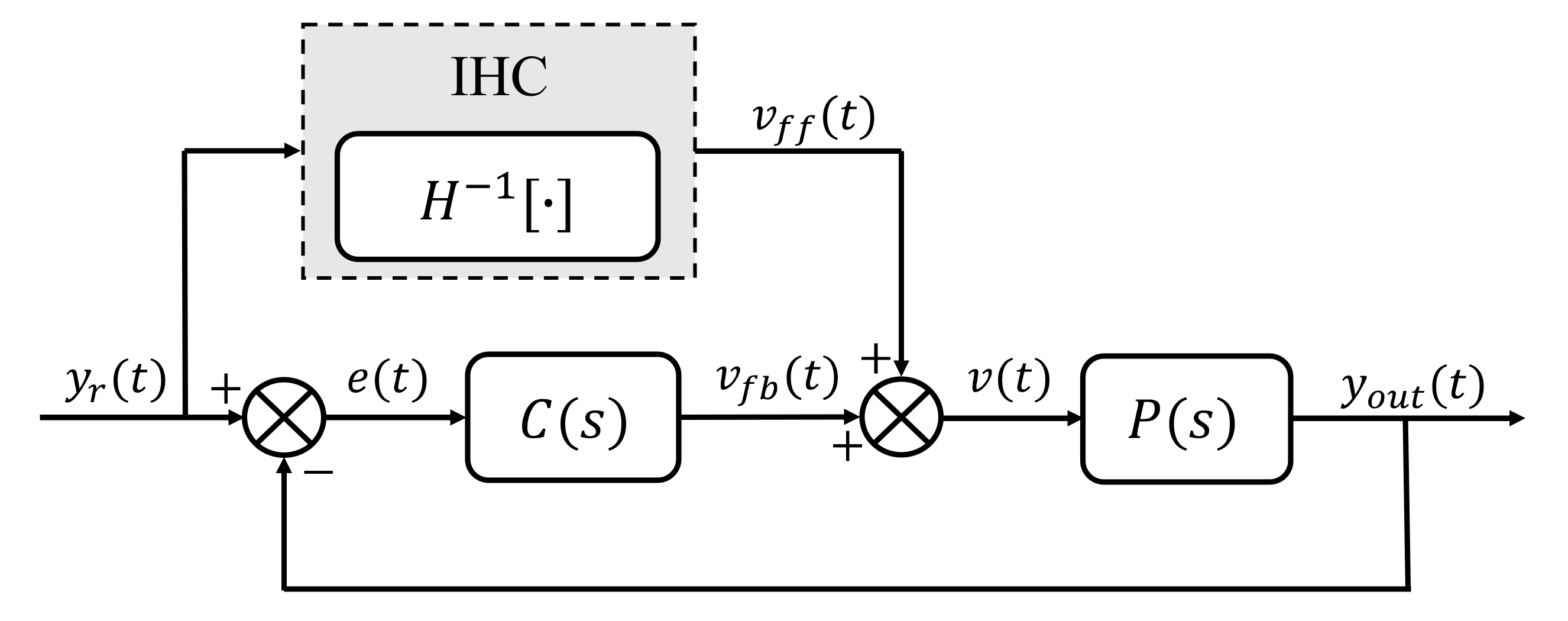
3.1. The Open-Loop Controller Based on IHC
3.2. Closed-Loop Controller
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A. MPI Model for Rate-Dependent Hysteresis
References
- Zhu, Z.; To, S.; Ehmann, K.F.; Zhou, X. Design, analysis, and realization of a novel piezoelectrically actuated rotary spatial vibration system for micro-/nano-machining. IEEE/ASME Trans. Mechatron. 2017, 22, 1227–1237. [Google Scholar] [CrossRef]
- Vagia, M.; Eielsen, A.A.; Gravdahl, J.T.; Pettersen, K.Y. Design of a nonlinear damping control scheme for nanopositioning. In Proceedings of the 2013 IEEE/ASME International Conference on Advanced Intelligent Mechatronics, Wollongong, Australia, 9–12 July 2013; pp. 94–99. [Google Scholar]
- Schitter, G.; Astrom, K.J.; DeMartini, B.E.; Thurner, P.J.; Turner, K.L.; Hansma, P.K. Design and modeling of a high-speed AFM-scanner. IEEE Trans. Control. Syst. Technol. 2007, 15, 906–915. [Google Scholar] [CrossRef]
- Devasia, S.; Eleftheriou, E.; Moheimani, S.O.R. A survey of control issues in Nanopositioning. IEEE Trans. Control Syst. Technol. 2007, 15, 802–823. [Google Scholar] [CrossRef]
- Gu, G.; Zhu, L.; Su, C.; Ding, H.; Fatikow, S. Modeling and Control of Piezo-Actuated Nanopositioning Stages: A Survey. IEEE Trans. Autom. Sci. Eng. 2016, 13, 313–332. [Google Scholar] [CrossRef]
- Shan, Y.; Leang, K. Accounting for hysteresis in repetitive control design: Nanopositioning example. Jpn. J. Appl. Phys. 2002, 41, 4851–4856. [Google Scholar] [CrossRef]
- Yu, Z.; Wu, Y.; Fang, Z.; Sun, H. Modeling and compensation of hysteresis in piezoelectric actuators. Heliyon 2020, 6, e03999. [Google Scholar] [CrossRef]
- Seki, K.; Ruderman, M.; Iwasaki, M. Modeling and compensation for hysteresis properties in piezoelectric actuators. In Proceedings of the 2014 IEEE 13th International Workshop on Advanced Motion Control (AMC), Yokohama, Japan, 14–16 March 2014; pp. 687–692. [Google Scholar]
- Li, K.; Yang, Z.; Lallart, M.; Zhou, S.; Chen, Y.; Liu, H. Hybrid hysteresis modeling and inverse model compensation of piezoelectric actuators. Smart Mater. Struct. 2019, 28, 115038. [Google Scholar] [CrossRef]
- Richter, H.; Misawa, E.; Lucca, D.; Lu, H. Modeling nonlinear behavior in a piezoelectric actuator. Precis. Eng. 2001, 25, 128–137. [Google Scholar] [CrossRef] [Green Version]
- Gu, G.; Zhu, L. Modeling of rate-dependent hysteresis in piezoelectric actuators using a family of ellipses. Sens. Actuators Phys. 2011, 165, 303–309. [Google Scholar] [CrossRef]
- Gawthrop, P.; Bhikkaji, B.; Moheimani, S. Physical-model-based control of a piezoelectric tube for nano-scale positioning applications. Mechatronics 2010, 20, 74–84. [Google Scholar] [CrossRef]
- Zhao, X.; Tan, Y. Neural network based identification of Preisach-type hysteresis in piezoelectric actuator using hysteretic operator. Sens. Actuators Phys. 2006, 126, 306–311. [Google Scholar] [CrossRef]
- Hu, H.; Mrad, R. On the classical Preisach model for hysteresis in piezoceramic actuators. Mechatronics 2002, 13, 85–94. [Google Scholar] [CrossRef]
- Kuhnen, K. Modeling, identifification and compensation of complex hysteretic nonlinearities: A modifified Prandtl-Ishlinskii approach. Eur. J. Control 2003, 9, 407–418. [Google Scholar] [CrossRef]
- Janocha, H.; Kuhnen, K. Real-time compensation of hysteresis and creep in piezoelectric actuators. Sens. Actuators Phys. 2000, 79, 83–89. [Google Scholar] [CrossRef]
- Iamail, M.; Ikhouane, F.; Rodellar, J. The hysteresis Bouc-Wen model, a survey. Arch. Comput. Methods Eng. 2009, 16, 161–188. [Google Scholar]
- Charalampakis, A.; Dimou, C. Identifification of Bouc-Wen hysteretic systems using particle swarm optimization. Comput. Struct. 2010, 88, 1197–1205. [Google Scholar] [CrossRef]
- Kwok, N.; Ha, Q.; Nguyen, M.; Li, J.; Samali, B. Bouc-Wen model parameter identifification for a MR flfluid damper using computationally effificient GA. ISA Trans. 2007, 46, 167–179. [Google Scholar] [CrossRef]
- Xu, Q.; Li, Y. Dahl model-based hysteresis compensation and precise positioning control of an XY parallel micromanipulator with piezoelectric actuation. ASME Trans. J. Dyn. Syst. Meas. Control 2010, 132, 041011. [Google Scholar] [CrossRef]
- Lin, C.; Lin, P. Tracking control of a biaxial piezo-actuated positioning stage using generalized Duhem model. Comput. Math. Appl. 2012, 64, 766–787. [Google Scholar] [CrossRef] [Green Version]
- Mayergoyz, I.D. Dynamic Preisach models of hysteresis. IEEE Trans. Magn. 1988, 24, 2925–2927. [Google Scholar] [CrossRef]
- Tan, U.X.; Win, T.L.; Ang, W.T. Modeling piezoelectric actuator hysteresis with singularity Free Prandtl-Ishlinskii model. In Proceedings of the 2006 IEEE International Conference on Robotics and Biomimetics, Kunming, China, 17–20 December 2006; pp. 251–256. [Google Scholar]
- Janaideh, M.A.; Rakheja, S.; Su, C.Y. Experimental characterization and modeling of rate-dependent hysteresis of a piezoceramic actuator. Mechatronics 2009, 19, 656–670. [Google Scholar] [CrossRef]
- Qin, Y.; Shirinzadeh, B.; Tian, Y.; Zhang, D. Design issues in a decoupled XY stage: Static and dynamics modeling, hysteresis compensation, and tracking control. Sens. Actuators Phys. 2013, 194, 95–105. [Google Scholar] [CrossRef]
- Al Janaideh, M.; Krejci, P. Inverse rate-dependent prandtl–Ishlinskii model for feedforward compensation of hysteresis in a piezomicropositioning actuator. IEEE/ASME Trans. Mechatron. 2013, 18, 1498–1507. [Google Scholar] [CrossRef]
- Yang, M.J.; Li, C.X.; Gu, G.Y.; Zhu, L.M. Modeling and compensating the dynamic hysteresis of piezoelectric actuators via a modifified rate-dependent Prandtl–Ishlinskii model. Smart Mater. Struct. 2015, 24, 125006. [Google Scholar] [CrossRef]
- Raj, R.A.; Samikannu, R.; Yahya, A.; Mosalaosi, M. Performance evaluation of natural esters and dielectric correlation assessment using artificial neural network (ANN). J. Adv. Dielectr. 2020, 10, 2050025. [Google Scholar] [CrossRef]
- Haggag, S.; Nasrat, L.; Ismail, H. ANN approaches to determine the dielectric strength improvement of MgO based low density polyethylene nanocomposite. J. Adv. Dielectr. 2021, 11, 2150016-48727. [Google Scholar] [CrossRef]
- Dong, R.; Tan, Y.; Chen, H.; Xie, Y. A neural networks based model for rate-dependent hysteresis for piezoceramic actuators. Sens. Actuators Phys. 2008, 143, 370–376. [Google Scholar] [CrossRef]
- Li, P.; Yan, F.; Ge, C.; Wang, X.; Xu, L.; Guo, J.; Li, P. A simple fuzzy system for modelling of both rate-independent and rate-dependent hysteresis in piezoelectric actuators. Mech. Syst. Signal Process. 2013, 36, 182–192. [Google Scholar] [CrossRef]
- Mao, X.; Wang, Y.; Liu, X.; Guo, Y. A hybrid feedforward-feedback hysteresis compensator in piezoelectric actuators based on least-squares support vector machine. IEEE Trans. Ind. Electron. 2018, 65, 5704–5711. [Google Scholar] [CrossRef] [Green Version]
- Li, P.; Li, P.; Sui, Y. Adaptive Fuzzy Hysteresis Internal Model Tracking Control of Piezoelectric Actuators with Nanoscale Application. IEEE Trans. Fuzzy Syst. 2016, 24, 1246–1254. [Google Scholar] [CrossRef]
- Tao, Y.D.; Li, H.X.; Zhu, L.M. Rate-dependent hysteresis modeling and compensation of piezoelectric actuators using Gaussian process. Sens. Actuators Phys. 2019, 295, 357–365. [Google Scholar] [CrossRef]
- Hu, C.X.; Ou, T.S.; Zhu, Y.; Zhu, L.M. GRU-Type LARC Strategy for Precision Motion Control with Accurate Tracking Error Prediction. IEEE Trans. Ind. Electron. 2021, 68, 812–820. [Google Scholar] [CrossRef]
- Sun, Z.; Song, B.; Xi, N.; Yang, R.; Hao, L.; Yang, Y.; Chen, L. Asymmetric hysteresis modeling and compensation approach for nanomanipulation system motion control considering working-range effect. IEEE Trans. Ind. Electron. 2017, 64, 5513–5523. [Google Scholar] [CrossRef]

| The Type of Input Signals | MPI Model (NRMSE/RME, %) | GP-Based Model (NRMSE/RME, %) | ||||
|---|---|---|---|---|---|---|
| 2-Dimension | 6-Dimension | 9-Dimension | 12-Dimension | |||
| Sinusoid signal | 100 Hz | 0.6497/2.01 | 0.4034/0.95 | 0.1908/0.75 | 0.1812/0.66 | 0.1766/0.69 |
| 200 Hz | 1.1673/2.93 | 0.2165/0.96 | 0.1712/0.56 | 0.1691/0.55 | 0.1673/0.56 | |
| 300 Hz | 2.9334/5.68 | 0.9519/1.93 | 0.9223/1.65 | 0.9187/1.65 | 0.9196/1.58 | |
| 500 Hz | 3.2692/6.33 | 1.3732/2.74 | 1.2974/2.31 | 1.3116/2.40 | 1.2749/2.29 | |
| 1000 Hz | 4.9547/8.96 | 1.3183/2.77 | 1.2875/2.32 | 1.2874/2.32 | 1.2855/2.31 | |
| Mixed-frequency signal | 120 + 180 Hz | 2.1251/4.97 | 2.1670/4.65 | 2.1462/4.28 | 1.9929/3.83 | 1.6752/3.62 |
| 100 + 150 + 200 + 250 Hz | 2.8872/6.69 | 3.0209/7.28 | 2.7079/6.19 | 1.6165/4.03 | 1.0759/2.76 | |
| Triangular wave | 50 Hz | 2.1725/7.29 | 2.2333/13.8 | 2.1070/7.18 | 2.0444/7.41 | 2.0192/6.24 |
| The Type of Reference Trajectories | MPI-Based Compensator (NRMSE/RME, %) | GP-Based Compensator (NRMSE/RME, %) | ||||
|---|---|---|---|---|---|---|
| 2-Dimension | 6-Dimension | 9-Dimension | 12-Dimension | |||
| Sinusoid signal | 100 Hz | 0.8204/1.80 | 0.5118/1.44 | 0.3565/1.01 | 0.5098/1.33 | 0.3178/0.96 |
| 200 Hz | 1.5220/2.61 | 0.5622/1.50 | 0.5177/1.39 | 0.4199/1.25 | 0.5028/1.29 | |
| 300 Hz | 2.8829/4.67 | 1.7609/5.02 | 1.7623/3.60 | 1.3508/4.51 | 0.9646/2.05 | |
| 400 Hz | 3.1589/7.46 | 2.8196/8.65 | 1.9965/4.07 | 1.7018/3.84 | 1.0976/2.50 | |
| 500 Hz | 3.5382/12.1 | 2.7784/6.83 | 2.6411/5.74 | 2.0716/4.66 | 1.9783/4.85 | |
| 600 Hz | 5.4530/13.2 | 3.1666/7.58 | 2.8752/7.02 | 2.8545/6.14 | 1.4955/4.98 | |
| 700 Hz | 6.8551/19.0 | 3.5210/7.36 | 2.8407/8.30 | 2.5233/7.33 | 1.6366/5.48 | |
| 800 Hz | 7.9379/19.9 | 4.4801/10.0 | 3.5891/8.19 | 3.1453/7.77 | 2.7030/6.87 | |
| 900 Hz | 6.0733/16.1 | 5.5373/12.7 | 3.6697/8.93 | 3.7629/9.53 | 2.6164/6.67 | |
| 1000 Hz | 9.3307/19.6 | 4.6383/10.5 | 4.1660/9.26 | 4.0557/10.5 | 3.2564/8.85 | |
| Mixed-frequency signal | 120 + 180 Hz | 1.6816/3.85 | 2.2292/7.72 | 1.2907/3.30 | 1.1217/2.83 | 1.1096/2.51 |
| 100 + 150 + 200 + 250 Hz | 2.5270/8.65 | 3.5872/9.85 | 2.3128/5.79 | 1.9443/5.19 | 1.8159/4.86 | |
| Triangular wave | 50 Hz | 2.0806/4.21 | 3.8415/9.04 | 1.0245/4.74 | 0.9673/2.78 | 0.8783/2.27 |
| The Type of Reference Trajectories | Pure PI Controller (NRMSE/RME, %) | MPI-Based Method (NRMSE/RME, %) | GP-Based Method (NRMSE/RME, %) | ||||
|---|---|---|---|---|---|---|---|
| 2-Dimension | 6-Dimension | 9-Dimension | 12-Dimension | ||||
| Sinusoid signal | 100 Hz | 1.1033/2.30 | 0.8094/2.15 | 0.7000/1.48 | 0.6940/1.62 | 0.6672/1.42 | 0.6407/1.38 |
| 200 Hz | 2.2465/3.74 | 1.4492/2.73 | 1.4192/2.38 | 1.4005/2.43 | 1.3979/2.53 | 1.3883/2.48 | |
| 300 Hz | 3.1921/5.34 | 2.5918/6.57 | 3.0714/7.57 | 2.2458/4.17 | 2.1828/3.82 | 2.1721/3.86 | |
| 400 Hz | 4.2390/6.53 | 4.3373/12.4 | 4.2570/12.0 | 3.3804/5.70 | 3.2419/5.57 | 3.2052/5.44 | |
| 500 Hz | 5.3924/8.86 | 7.5462/17.6 | 5.1274/11.0 | 4.7590/8.32 | 4.2756/6.76 | 3.5501/6.86 | |
| 600 Hz | 6.5219/10.6 | 10.362/24.4 | 6.5440/12.4 | 5.8782/12.0 | 5.0000/9.19 | 4.9335/9.40 | |
| 700 Hz | 7.7353/12.3 | 10.020/23.3 | 7.2384/18.5 | 6.9444/14.1 | 6.6222/12.7 | 6.2461/10.8 | |
| 800 Hz | 8.9472/13.9 | 11.016/30.3 | 8.3979/19.4 | 7.5350/15.8 | 6.9765/12.7 | 6.6185/12.8 | |
| 900 Hz | 10.1254/16.2 | 11.556/32.6 | 8.8955/23.1 | 9.2630/19.1 | 7.7958/13.3 | 7.3941/11.9 | |
| 1000 Hz | 11.1463/18.2 | 14.633/31.5 | 9.4682/17.1 | 10.4491/20.4 | 9.05513/16.3 | 8.3210/14.6 | |
| Mixed-frequency signal | 120 + 180 Hz | 1.3174/2.91 | 0.8590/2.09 | 0.8139/2.03 | 0.8012/2.05 | 0.7527/2.00 | 0.7497/1.84 |
| 100 + 150 + 200 + 250 Hz | 2.2445/3.90 | 0.9088/2.87 | 1.1093/6.28 | 0.8894/2.64 | 0.8787/2.69 | 0.8065/2.43 | |
| Triangular wave | 50 Hz | 1.3380/3.19 | 0.8782/2.84 | 0.9745/4.42 | 0.5786/3.22 | 0.4682/2.55 | 0.4405/2.41 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Meng, Y.; Wang, X.; Li, L.; Huang, W.; Zhu, L. Hysteresis Modeling and Compensation of Piezoelectric Actuators Using Gaussian Process with High-Dimensional Input. Actuators 2022, 11, 115. https://doi.org/10.3390/act11050115
Meng Y, Wang X, Li L, Huang W, Zhu L. Hysteresis Modeling and Compensation of Piezoelectric Actuators Using Gaussian Process with High-Dimensional Input. Actuators. 2022; 11(5):115. https://doi.org/10.3390/act11050115
Chicago/Turabian StyleMeng, Yixuan, Xiangyuan Wang, Linlin Li, Weiwei Huang, and Limin Zhu. 2022. "Hysteresis Modeling and Compensation of Piezoelectric Actuators Using Gaussian Process with High-Dimensional Input" Actuators 11, no. 5: 115. https://doi.org/10.3390/act11050115
APA StyleMeng, Y., Wang, X., Li, L., Huang, W., & Zhu, L. (2022). Hysteresis Modeling and Compensation of Piezoelectric Actuators Using Gaussian Process with High-Dimensional Input. Actuators, 11(5), 115. https://doi.org/10.3390/act11050115







