Design and Test of an Active Pneumatic Soft Wrist for Soft Grippers
Abstract
:1. Introduction
2. Structure Design
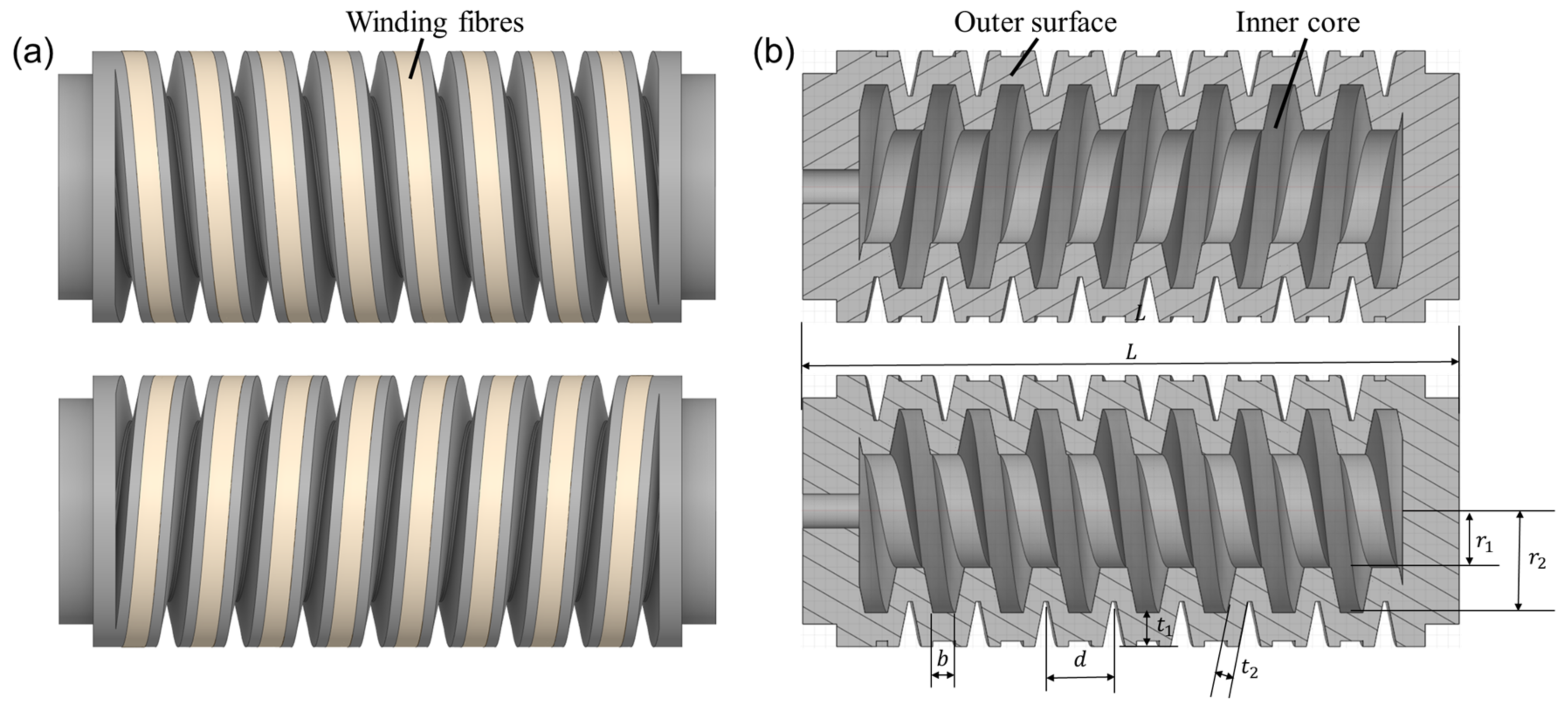
2.1. Structure of Pneumatic Helical Actuators
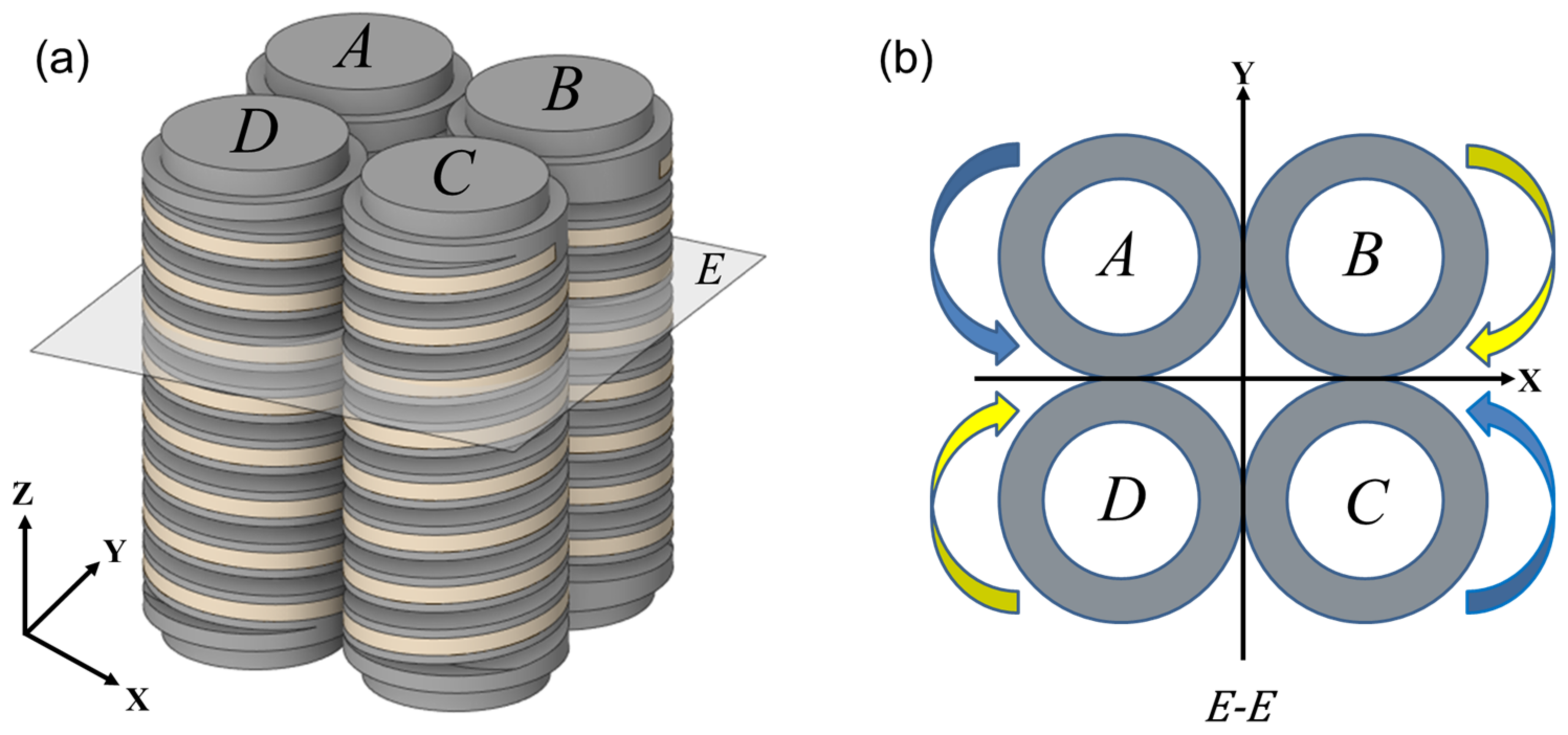
2.2. Structure Design of the Pneumatic Soft Wrist
3. Kinematic Analysis
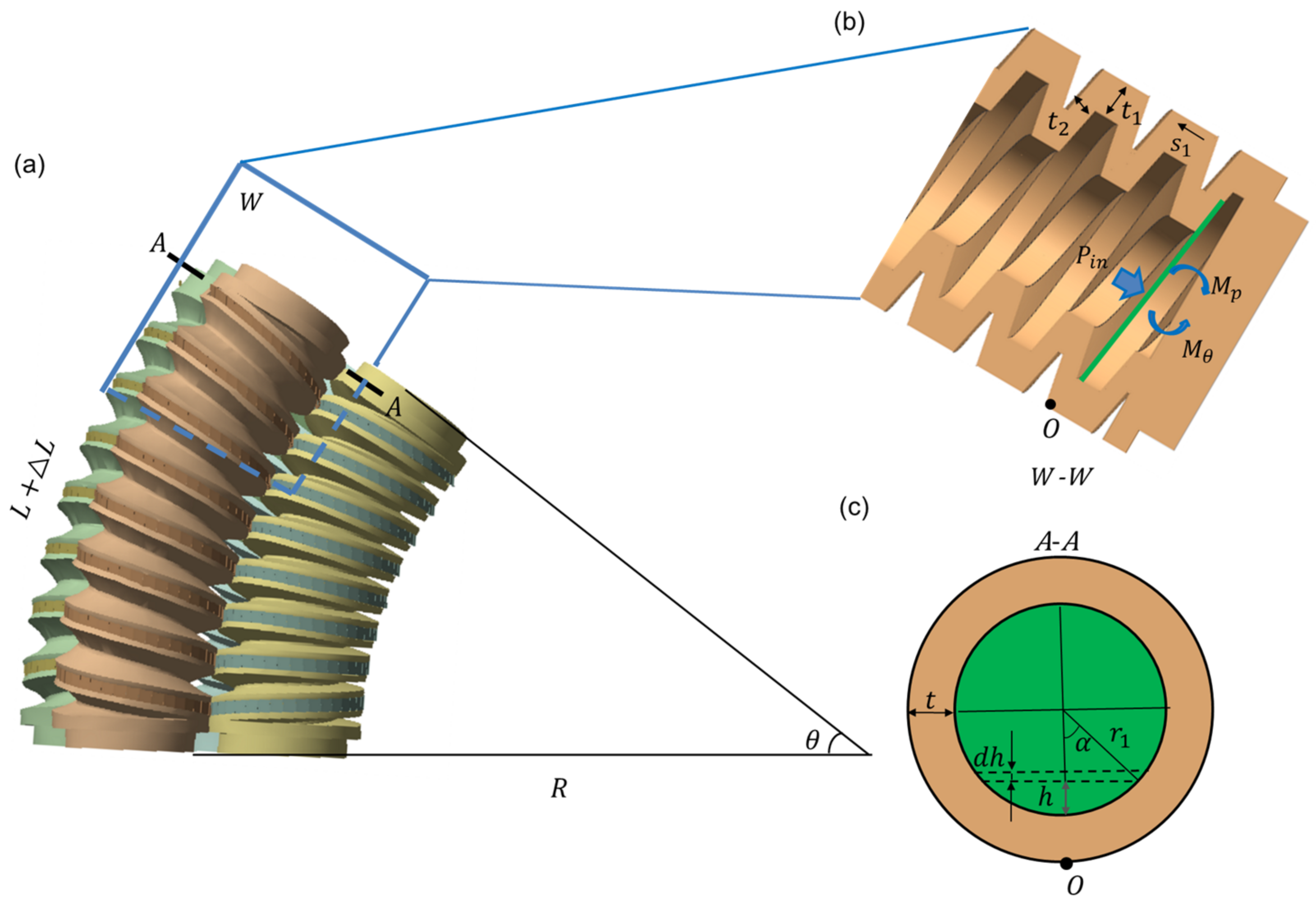
3.1. Bending Model
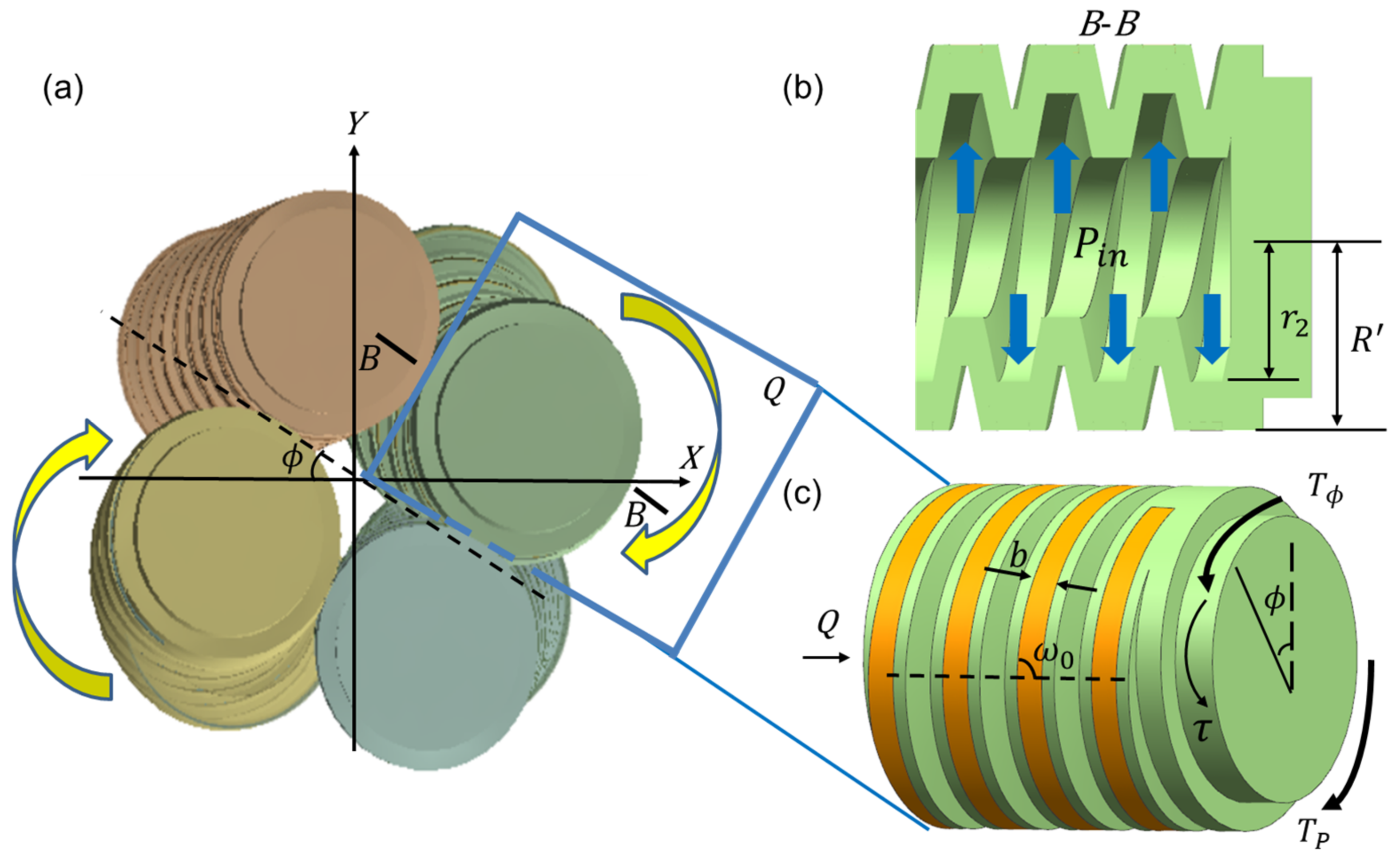
3.2. Twisting Model
4. Numerical Modeling
4.1. Simulation Parameters
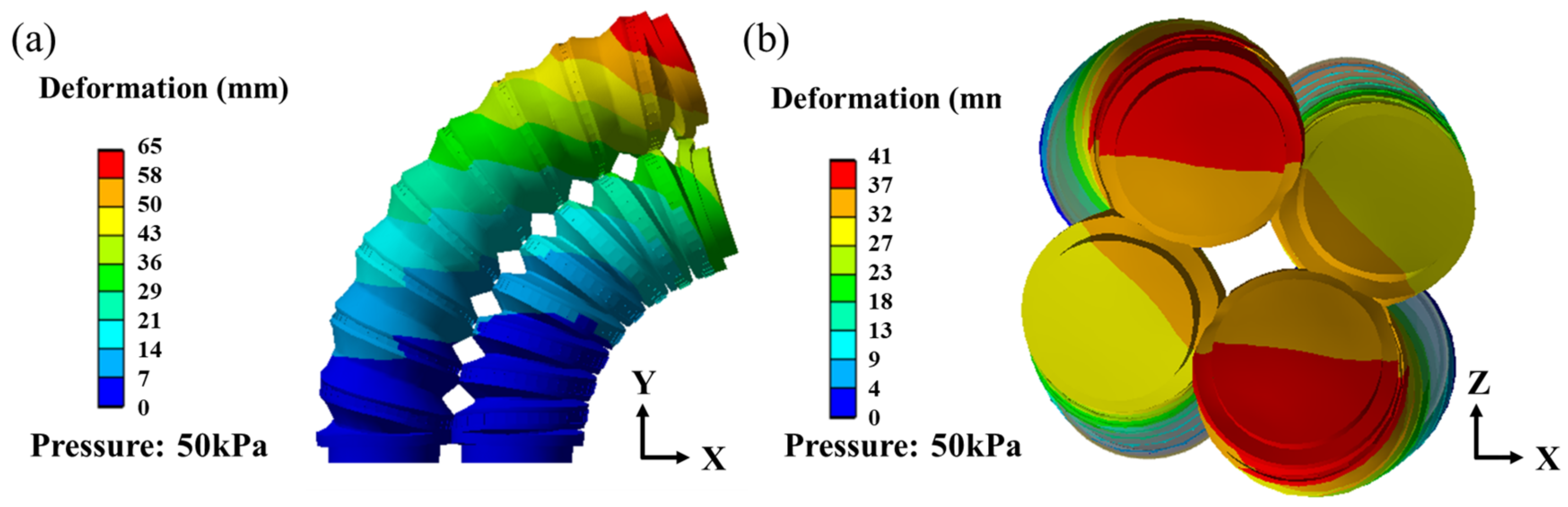
4.2. Simulation Results
5. Experimental Tests
5.1. Sample Fabrication
5.2. Experimental Results
6. Application for a Soft Hand
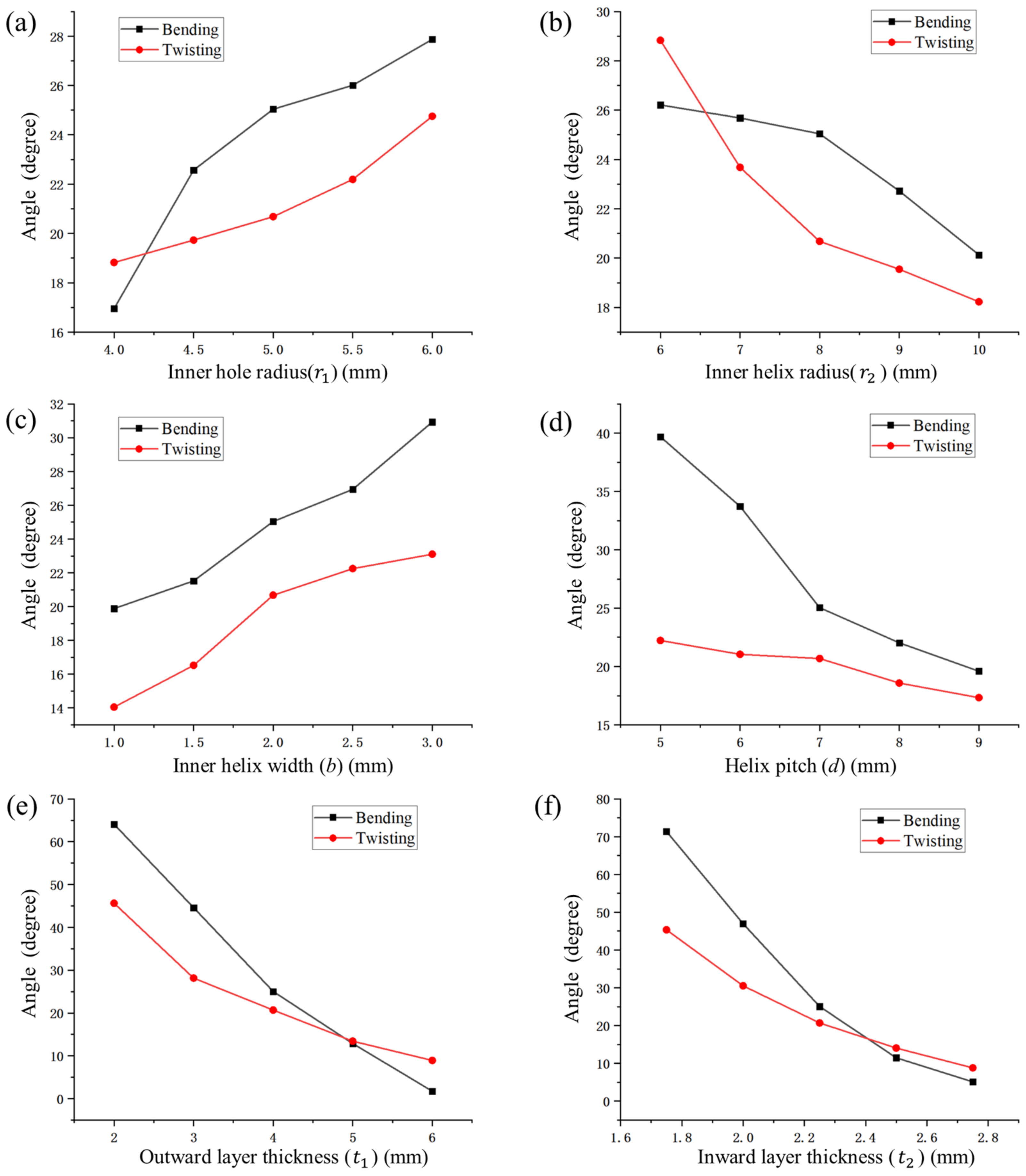
6.1. Sensitivity Studies of Geometric Parameters
6.2. Verfication and Validation of the Soft Wrist
7. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Nomenclature
| A | Force integral area () |
| b | Width of inner helix surface (m) |
| c | Length of the chamber internal structure (m) |
| d | Pitch (m) |
| Material constant (Pa) | |
| Silicone rubber material parameter (Pa) | |
| Fiber material parameter (Pa) | |
| Actuator diameter (m) | |
| Incompressibility parameter (Pa−1) | |
| e | Thickness of fiber layer (m) |
| Force generated by air pressure (N) | |
| Initial shear modulus (Pa) | |
| Distance from bottom edge (m) | |
| Parameter in tangential stress calculation (None) | |
| The first invariant (None) | |
| Volume variation ratio (None) | |
| Actuator length (m) | |
| Moment generated by pressure (bending) (N) | |
| Moment generated by material (bending) (N) | |
| Moment generated by the material (N) | |
| Chamber pressure (pa) | |
| Actuator chamber radius (m) | |
| Internal radius (m) | |
| Outer radius (m) | |
| Curvature radius | |
| Actuator radius (m) | |
| Average equivalent thickness (m) | |
| Outer layer thickness (m) | |
| Inner layer thickness (m) | |
| Moment generated by pressure (twisting) (N) | |
| Moment generated by material (twisting) (N) | |
| Helix angle (rad) | |
| Bending angle (rad) | |
| Stretch ratio in bending model (none) | |
| Stretch ratio in twisting model (none) | |
| Shear stress (Pa) | |
| Angle of fiber cloth (rad) | |
| Helix angle of fiber cloth (rad) | |
| Twist angle (rad) |
References
- Li, M.; Pal, A.; Aghakhani, A.; Pena-Francesch, A.; Sitti, M. Soft Actuators for Real-World Applications. In Nature Reviews Materials; Springer: New York, NY, USA, 2022; pp. 235–249. [Google Scholar]
- Wang, D.; Wu, X.; Zhang, J.; Du, Y. A Pneumatic Novel Combined Soft Robotic Gripper with High Load Capacity and Large Grasping Range. Actuators 2022, 11, 3. [Google Scholar] [CrossRef]
- Grazioso, S.; Di Gironimo, G.; Siciliano, B. A Geometrically Exact Model for Soft Continuum Robots: The Finite Element Deformation Space Formulation. Soft Robot. 2019, 6, 790–811. [Google Scholar] [CrossRef] [PubMed]
- Lu, M.; Chen, G.; He, Q.; Zong, W.; Yu, Z.; Dai, Z. Development of a Hydraulic Driven Bionic Soft Gecko Toe. J. Mech. Robot. 2021, 13, 051005. [Google Scholar] [CrossRef]
- Zhang, Z.; Ni, X.; Wu, H.; Sun, M.; Bao, G.; Wu, H.; Jiang, S. Pneumatically Actuated Soft Gripper with Bistable Structres. Soft Robot. 2022, 9, 57–71. [Google Scholar] [CrossRef] [PubMed]
- Zhang, Z.; Ni, X.; Gao, W.; Shen, H.; Sun, M.; Guo, G.; Wu, H.; Jiang, S. Pneumatically Controlled Reconfigurable Bistable Bionic Flower for Robotic Gripper. Soft Robot. 2022, 9, 657–669. [Google Scholar] [CrossRef] [PubMed]
- Gloumakov, Y.; Spiers, A.J.; Dollar, A.M. Dimensionality Reduction and Motion Clustering during Activities of Daily Living: Decoupling Hand Location and Orientation. IEEE Trans. Neural Syst. Rehabil. Eng. 2020, 28, 2955–2965. [Google Scholar] [CrossRef]
- El-Atab, N.; Mishra, R.B.; Al-Modaf, F.; Joharji, L.; Alsharif, A.A.; Alamoudi, H.; Diaz, M.; Qaiser, N.; Hussain, M.M. Soft Actuators for Soft Robotic Applications: A Review. Adv. Intell. Syst. 2020, 2, 2000128. [Google Scholar] [CrossRef]
- Abondance, S.; Teeple, C.B.; Wood, R.J. A Dexterous Soft Robotic Hand for Delicate In-Hand Manipulation. IEEE Robot. Autom. Lett. 2020, 5, 5502–5509. [Google Scholar] [CrossRef]
- Subramaniam, V.; Jain, S.; Agarwal, J.; Valdivia y Alvarado, P. Design and Characterization of a Hybrid Soft Gripper with Active Palm Pose Control. Int. J. Rob. Res. 2020, 39, 1668–1685. [Google Scholar] [CrossRef]
- Zhong, G.; Hou, Y.; Dou, W. A Soft Pneumatic Dexterous Gripper with Convertible Grasping Modes. Int. J. Mech. Sci. 2019, 153–154, 445–456. [Google Scholar]
- Zhang, H.; Kumar, A.S.; Fuh, J.Y.H.; Wang, M.Y. Design and Development of a Topology-Optimized Three-Dimensional Printed Soft Gripper. Soft Robot. 2018, 5, 650–661. [Google Scholar] [CrossRef]
- Gong, Z.; Fang, X.; Chen, X.; Cheng, J.; Xie, Z.; Liu, J.; Chen, B.; Yang, H.; Kong, S.; Hao, Y.; et al. A Soft Manipulator for Efficient Delicate Grasping in Shallow Water: Modeling, Control, and Real-World Experiments. Int. J. Rob. Res. 2021, 40, 449–469. [Google Scholar] [CrossRef]
- Xiao, W.; Hu, D.; Chen, W.; Yang, G.; Han, X. Modeling and Analysis of Bending Pneumatic Artificial Muscle with Multi-Degree of Freedom. Smart Mater. Struct. 2021, 30, 095018. [Google Scholar] [CrossRef]
- Alici, G.; Canty, T.; Mutlu, R.; Hu, W.; Sencadas, V. Modeling and Experimental Evaluation of Bending Behavior of Soft Pneumatic Actuators Made of Discrete Actuation Chambers. Soft Robot. 2018, 5, 24–35. [Google Scholar] [CrossRef] [Green Version]
- Sanan, S.; Lynn, P.S.; Griffith, S.T. Pneumatic Torsional Actuators for Inflatable Robots. J. Mech. Robot. 2014, 6, 031003. [Google Scholar] [CrossRef]
- Chatterjee, A.; Chahare, N.R.; Kondaiah, P.; Gundiah, N. Role of Fiber Orientations in the Mechanics of Bioinspired Fiber-Reinforced Elastomers. Soft Robot. 2021, 8, 640–650. [Google Scholar] [CrossRef]
- Chandler, J.H.; Chauhan, M.; Garbin, N.; Obstein, K.L.; Valdastri, P. Parallel Helix Actuators for Soft Robotic Applications. Front. Robot. AI 2020, 7, 119. [Google Scholar] [CrossRef]
- Jiang, C.; Wang, D.; Zhao, B.; Liao, Z.; Gu, G. Modeling and Inverse Design of Bio-Inspired Multi-Segment Pneu-Net Soft Manipulators for 3D Trajectory Motion. Appl. Phys. Rev. 2021, 8, 041416. [Google Scholar] [CrossRef]
- Nassour, J. Marionette-Based Programming of a Soft Textile Inflatable Actuator. Sensors Actuators, A Phys. 2019, 291, 93–98. [Google Scholar] [CrossRef]
- Xavier, M.S.; Tawk, C.D.; Zolfagharian, A.; Pinskier, J.; Howard, D.; Young, T.; Lai, J.; Harrison, S.M.; Yong, Y.K.; Bodaghi, M.; et al. Soft Pneumatic Actuators: A Review of Design, Fabrication, Modeling, Sensing, Control and Applications. IEEE Access 2022, 10, 59442–59485. [Google Scholar] [CrossRef]
- Kurumaya, S.; Phillips, B.T.; Becker, K.P.; Rosen, M.H.; Gruber, D.F.; Galloway, K.C.; Suzumori, K.; Wood, R.J. A Modular Soft Robotic Wrist for Underwater Manipulation. Soft Robot. 2018, 5, 399–409. [Google Scholar] [CrossRef]
- Fei, Y.; Wang, J.; Pang, W. A Novel Fabric-Based Versatile and Stiffness-Tunable Soft Gripper Integrating Soft Pneumatic Fingers and Wrist. Soft Robot. 2019, 6, 1–20. [Google Scholar] [CrossRef] [PubMed]
- Shen, Z.; Zhong, H.; Xu, E.; Zhang, R.; Yip, K.C.; Chan, L.L.; Chan, L.L.; Pan, J.; Wang, W.; Wang, Z. An Underwater Robotic Manipulator with Soft Bladders and Compact Depth-Independent Actuation. Soft Robot. 2020, 7, 535–549. [Google Scholar] [CrossRef] [PubMed]
- Wang, T.; Ge, L.; Gu, G. Programmable Design of Soft Pneu-Net Actuators with Oblique Chambers Can Generate Coupled Bending and Twisting Motions. Sens. Actuators A Phys. 2018, 271, 131–138. [Google Scholar] [CrossRef]
- Haddad, F.S. Automatic Design of Fiber-Reinforced Soft Actuators. Proc. Natl. Acad. Sci. USA 2017, 114, 51–56. [Google Scholar]
- Sui, D.; Zhao, S.; Wang, T.; Liu, Y.; Zhu, Y.; Zhao, J. Design of a Bio-Inspired Extensible Continuum Manipulator with Variable Stiffness. J. Bionic Eng. 2022. [Google Scholar] [CrossRef]
- Tian, Y.; Zhang, Q.; Cai, D.; Chen, C.; Zhang, J.; Duan, W. Theoretical Modelling of Soft Robotic Gripper with Bioinspired Fibrillar Adhesives. Mech. Adv. Mater. Struct. 2020, 29, 2250–2266. [Google Scholar] [CrossRef]
- Wang, Z.; Polygerinos, P.; Overvelde, J.T.B.; Galloway, K.C.; Bertoldi, K.; Walsh, C.J. Interaction Forces of Soft Fiber Reinforced Bending Actuators. IEEE/ASME Trans. Mechatronics 2017, 22, 717–727. [Google Scholar] [CrossRef]
- Sedal, A.; Bruder, D.; Bishop-Moser, J.; Vasudevan, R.; Kota, S. A Continuum Model for Fiber-Reinforced Soft Robot Actuators. J. Mech. Robot. 2018, 10, 024501. [Google Scholar] [CrossRef]
- Xavier, M.S.; Fleming, A.J.; Yong, Y.K. Finite Element Modeling of Soft Fluidic Actuators: Overview and Recent Developments. Adv. Intell. Syst. 2021, 3, 2000187. [Google Scholar] [CrossRef]
- ANSYS® Inc. ANSYS® Mechanical User’s Guide; ANSYS®: Canonsburg, PA, USA, 2021. [Google Scholar]
- Chen, L.; Yang, C.; Wang, H.; Branson, D.T.; Dai, J.S.; Kang, R. Design and Modeling of a Soft Robotic Surface with Hyperelastic Material. Mech. Mach. Theory 2018, 130, 109–122. [Google Scholar] [CrossRef]
- Marechal, L.; Balland, P.; Lindenroth, L.; Petrou, F.; Kontovounisios, C.; Bello, F. Toward a Common Framework and Database of Materials for Soft Robotics. Soft Robot. 2021, 8, 284–297. [Google Scholar] [CrossRef]
- Rus, D.; Tolley, M.T. Design, Fabrication and Control of Soft Robots. Nature 2015, 521, 467–475. [Google Scholar] [CrossRef] [Green Version]
- Cacucciolo, V.; Renda, F.; Poccia, E.; Laschi, C.; Cianchetti, M. Modelling the Nonlinear Response of Fibre-Reinforced Bending Fluidic Actuators. Smart Mater. Struct. 2016, 25, 105020. [Google Scholar] [CrossRef] [Green Version]
- Guo, D.; Kang, Z. Chamber Layout Design Optimization of Soft Pneumatic Robots. Smart Mater. Struct. 2020, 29, 025017. [Google Scholar] [CrossRef]
- Webster, R.J.; Jones, B.A. Design and Kinematic Modeling of Constant Curvature Continuum Robots: A Review. Int. J. Rob. Res. 2010, 29, 1661–1683. [Google Scholar] [CrossRef]
- Xiao, W.; Hu, D.; Yang, G.; Jiang, C. Modeling and Analysis of Soft Robotic Surfaces Actuated by Pneumatic Network Bending Actuators. Smart Mater. Struct. 2022, 31, 055001. [Google Scholar] [CrossRef]
- Chen, F.; Miao, Y.; Gu, G.; Zhu, X. Soft Twisting Pneumatic Actuators Enabled by Freeform Surface Design. IEEE Robot. Autom. Lett. 2021, 6, 5253–5260. [Google Scholar] [CrossRef]
- Polygerinos, P.; Wang, Z.; Overvelde, J.T.B.; Galloway, K.C.; Wood, R.J.; Bertoldi, K.; Walsh, C.J. Modeling of Soft Fiber-Reinforced Bending Actuators. IEEE Trans. Robot. 2015, 31, 778–789. [Google Scholar] [CrossRef] [Green Version]
- Zhang, X.; Oseyemi, A.E. A Herringbone Soft Pneu-Net Actuator for Enhanced Conformal Gripping. Robotica 2022, 40, 1345–1360. [Google Scholar] [CrossRef]



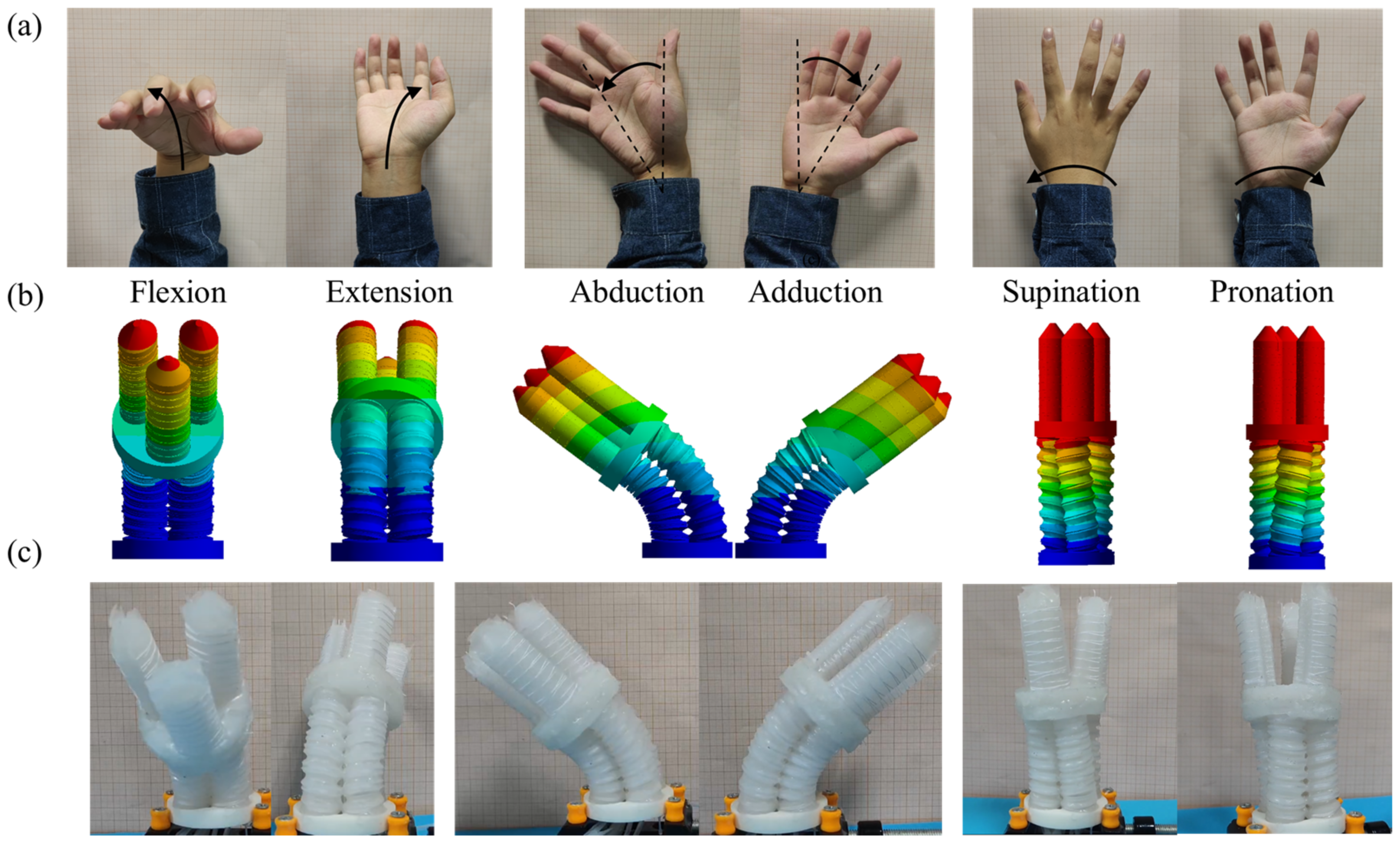
| Motions | Pressured Chambers | Directions of Motions |
|---|---|---|
| Bending forward (flexion) |  | 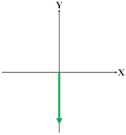 |
| Bending backward (extension) | 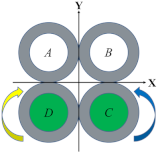 |  |
| Bending left (abduction) |  | 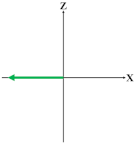 |
| Bending right (adduction) | 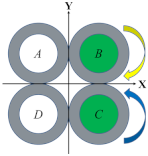 |  |
| Twisting left (supination) | 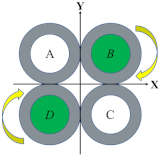 | 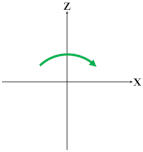 |
| Twisting right (pronation) | 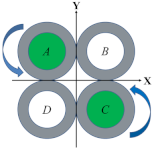 | 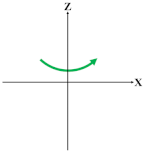 |
| Materials | Parameters | Values |
|---|---|---|
| Silicone chamber | Material constant C1 | 0.1 MPa [30] |
| Material constant C2 | 0.013 MPa [30] | |
| Material constant C3 | 0.00002 MPa [30] | |
| Kevlar fiber | Young’s modulus | 28 GPa |
| Poisson’s ratio | 0.37 |
| Inner Hole Radius r1 | Inner Helix Radius r2 | Helix Width b | Helix Pitch d | Outward Layer Thickness t1 | Inward Layer Thickness t2 |
|---|---|---|---|---|---|
| 4 | 6 | 1 | 5 | 2 | 1.75 |
| 4.5 | 7 | 1.5 | 6 | 3 | 2 |
| 5 | 8 | 2 | 7 | 4 | 2.25 |
| 5.5 | 9 | 2.5 | 8 | 5 | 2.5 |
| 6 | 10 | 3 | 9 | 6 | 2.75 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chen, G.; Lin, T.; Ding, S.; Chen, S.; Ji, A.; Lodewijks, G. Design and Test of an Active Pneumatic Soft Wrist for Soft Grippers. Actuators 2022, 11, 311. https://doi.org/10.3390/act11110311
Chen G, Lin T, Ding S, Chen S, Ji A, Lodewijks G. Design and Test of an Active Pneumatic Soft Wrist for Soft Grippers. Actuators. 2022; 11(11):311. https://doi.org/10.3390/act11110311
Chicago/Turabian StyleChen, Guangming, Tao Lin, Shi Ding, Shuang Chen, Aihong Ji, and Gabriel Lodewijks. 2022. "Design and Test of an Active Pneumatic Soft Wrist for Soft Grippers" Actuators 11, no. 11: 311. https://doi.org/10.3390/act11110311








