Using the Nonlinear Duffing Effect of Piezoelectric Micro-Oscillators for Wide-Range Pressure Sensing
Abstract
:1. Introduction
2. Theory
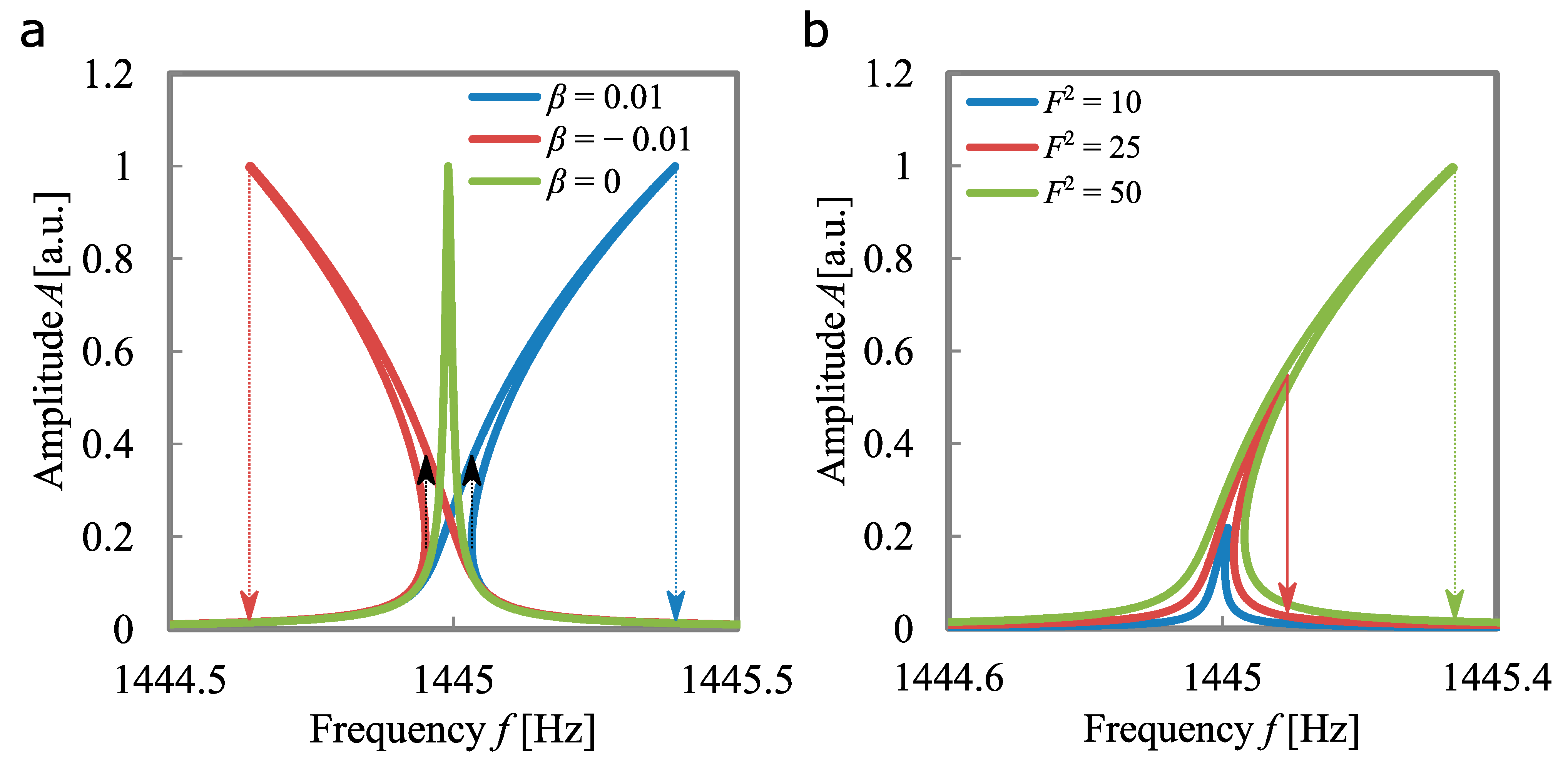
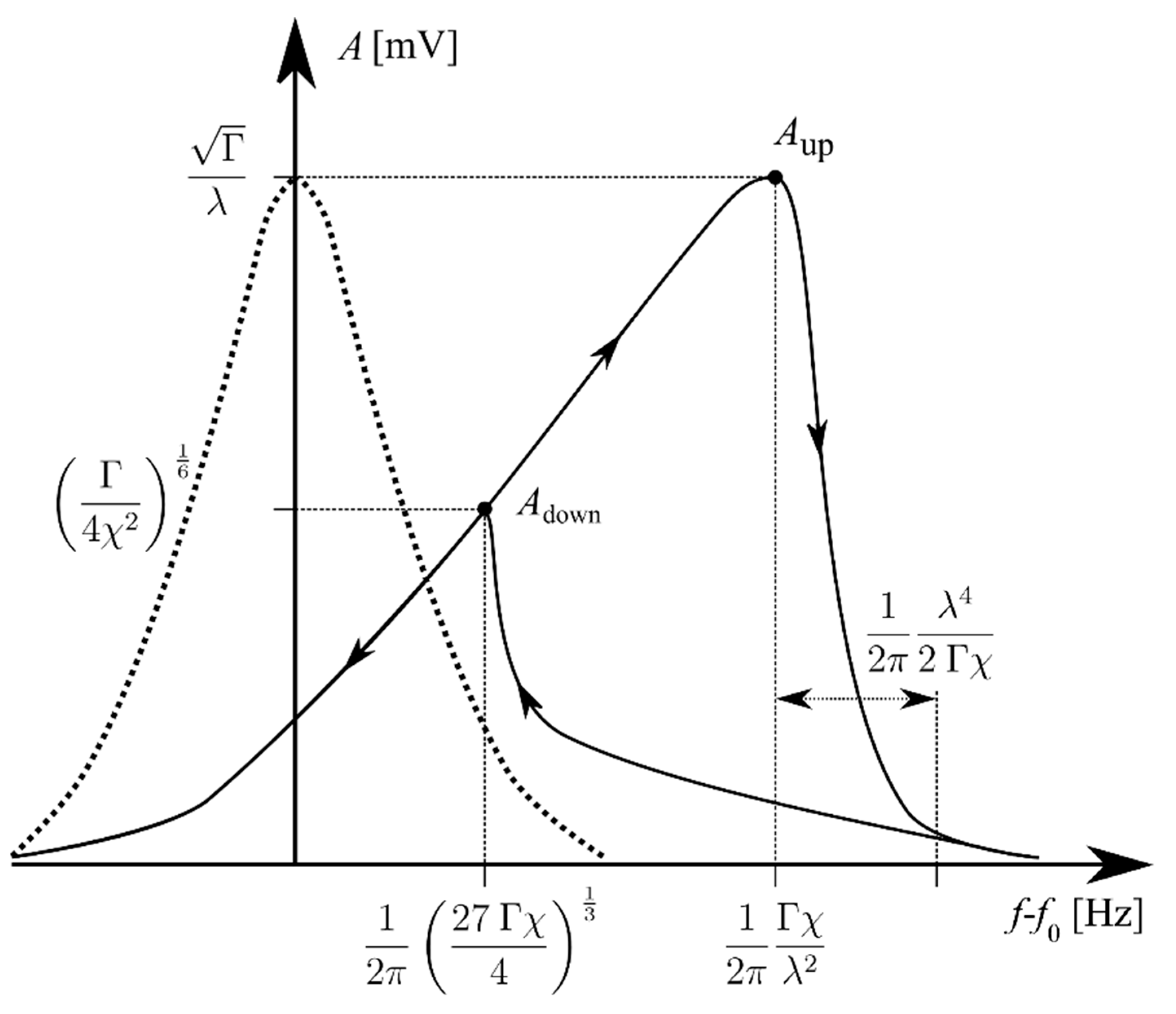
2.1. Nonlinear Duffing Oscillator
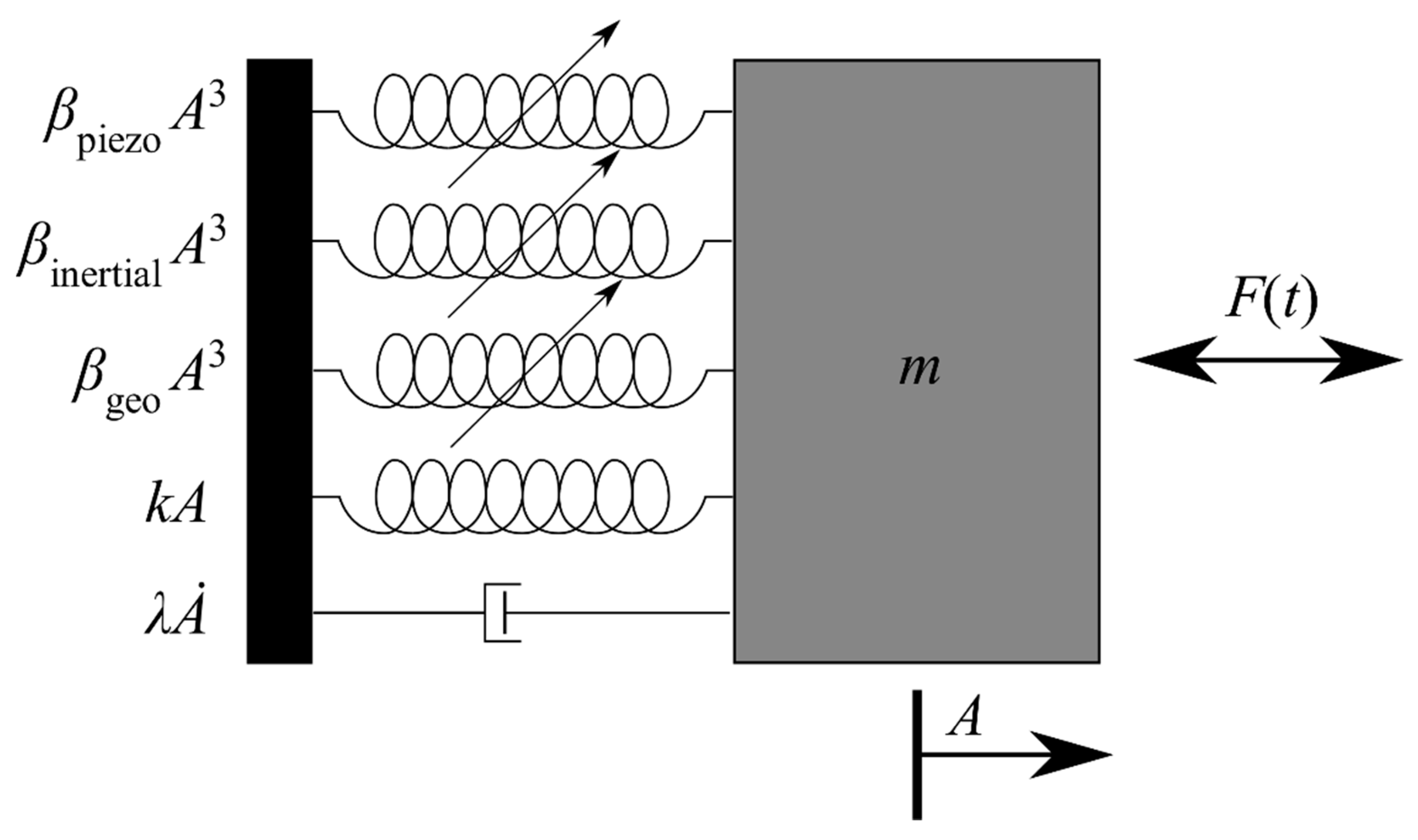
2.2. Ayela’s Model
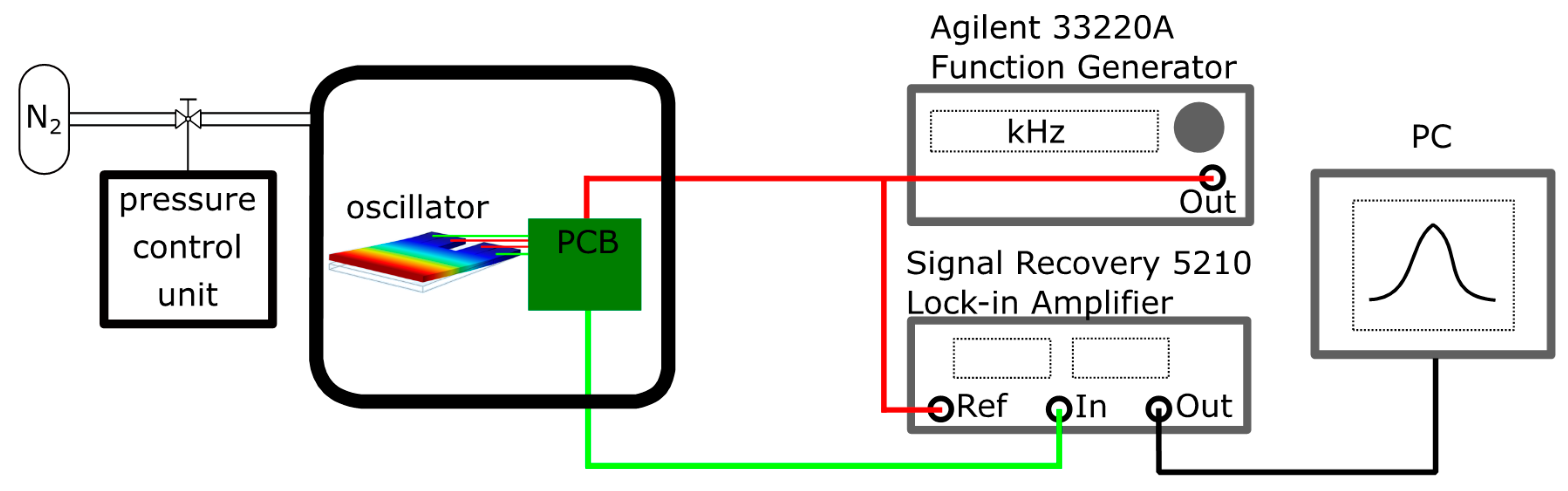
3. Experimental Section
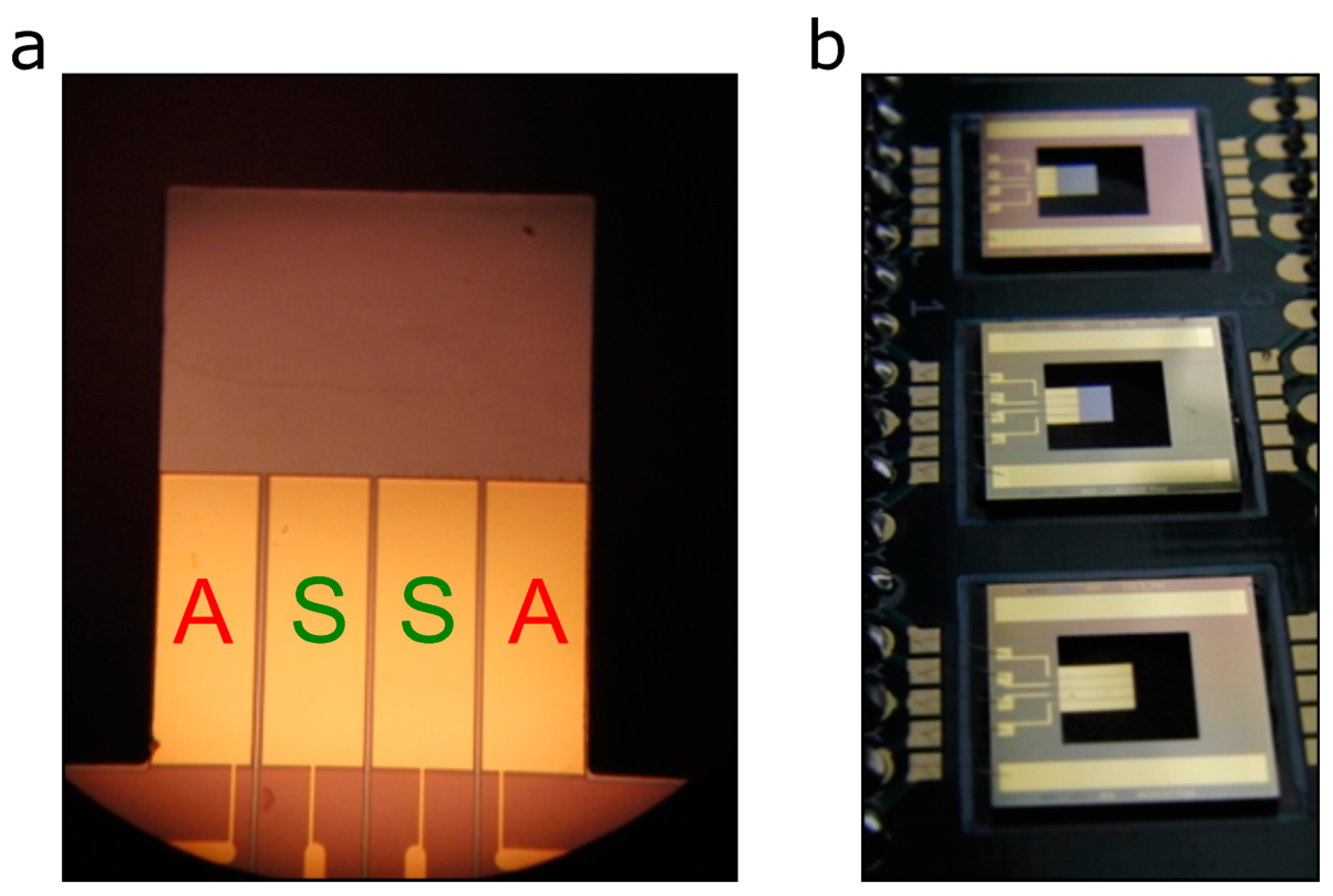
3.1. Manufacturing
3.2. Experimental Setup
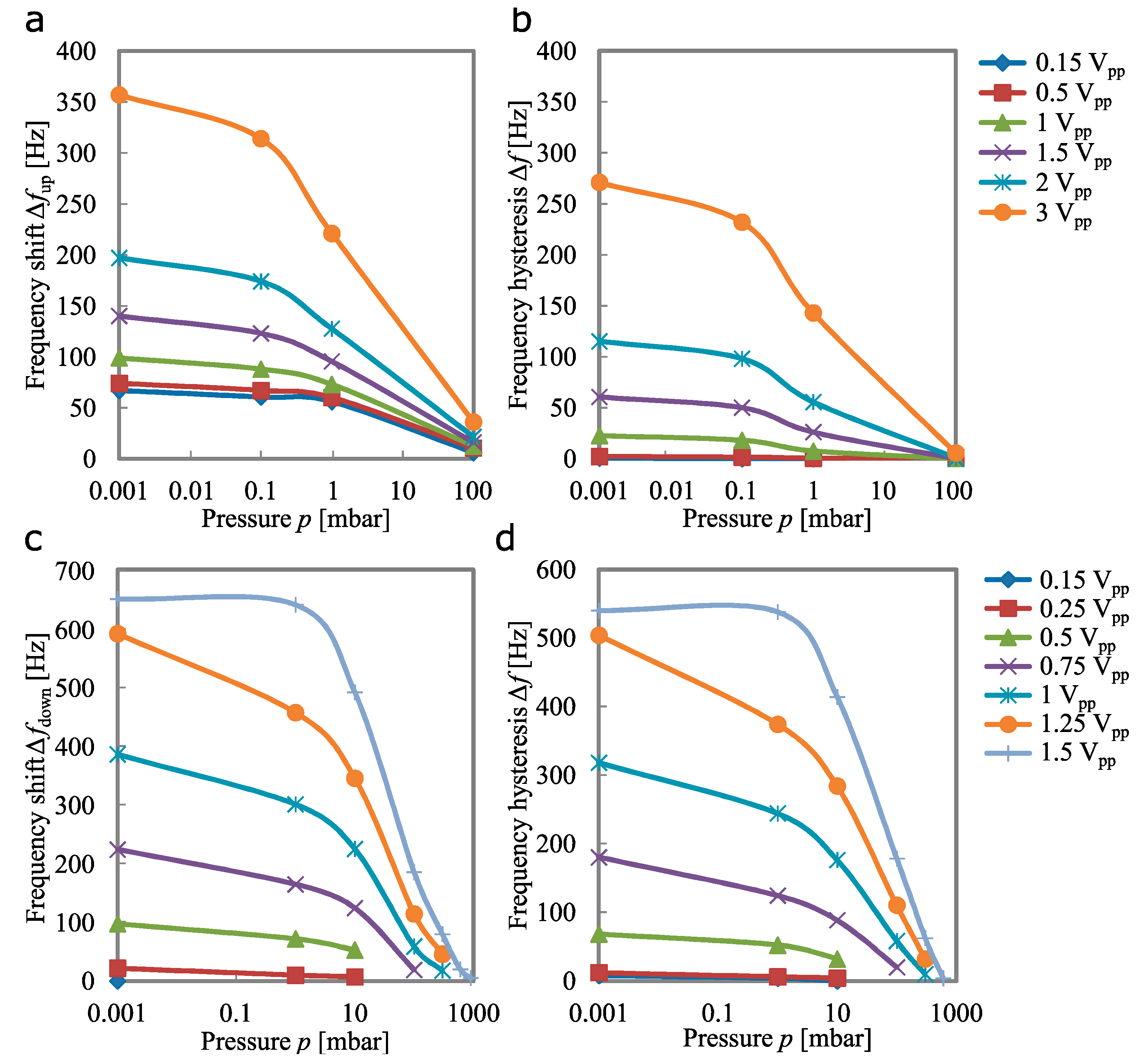
4. Results
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Aikele, M.; Bauer, K.; Ficker, W.; Neubauer, F.; Prechtel, U.; Schalk, J.; Seidel, H. Resonant accelerometer with self-test. Sens. Actuator A Phys. 2001, 92, 161–167. [Google Scholar] [CrossRef]
- Binnig, G.; Quate, C.F.; Gerber, C. Atomic force microscope. Phys. Rev. Lett. 1986, 56, 930–934. [Google Scholar] [CrossRef] [Green Version]
- Chen, S.T.; Du, S.; Arroyo, E.; Jia, Y.; Seshia, A. Utilising Nonlinear air damping as a soft mechanical stopper for MEMS vibration energy harvesting. J. Phys. Conf. Ser. 2016, 773, 012098. [Google Scholar] [CrossRef]
- Almog, R.; Zaitsev, S.; Shtempluck, O.; Buks, E. Signal amplification in a nanomechanical Duffing resonator via stochastic resonance. Appl. Phys. Lett. 2007, 90, 013508. [Google Scholar] [CrossRef] [Green Version]
- Sharma, M.; Sarraf, E.H.; Baskaran, R.; Cretu, E. Parametric resonance: Amplification and damping in MEMS gyroscopes. Sens. Actuator A Phys. 2012, 177, 79–86. [Google Scholar] [CrossRef]
- Li, Y.; Li, H.; Xiao, Y.; Cao, L.; Guo, Z. A compensation method for nonlinear vibration of silicon-micro resonant sensor. Sensors 2021, 21, 2545. [Google Scholar] [CrossRef]
- Hasan, M.; Alsaleem, F.; Oukad, H. Novel threshold pressure sensors based on nonlinear dynamics of MEMS resonators. J. Micromech. Microeng. 2018, 28, 065007. [Google Scholar] [CrossRef]
- Keskar, G.; Elliott, B.; Gaillard, J.; Skove, M.J.; Rao, A.M. Using electric actuation and detection of oscillations in microcantilevers for pressure measurements. Sens. Actuator A Phys. 2008, 147, 203–209. [Google Scholar] [CrossRef]
- Kacem, N.; Arcamone, J.; Perez-Murano, F.; Hentz, S. Dynamic range enhancement of nonlinear nanomechanical resonant cantilevers for highly sensitive NEMS gas/mass sensor applications. J. Micromech. Microeng. 2010, 20, 045023. [Google Scholar] [CrossRef]
- Green, P.L.; Worden, K.; Atallah, K.; Sims, N.D. The effect of Duffing-type non-linearities and Coulomb damping on the response of an energy harvester to random excitations. J. Intell. Mater. Syst. Struct. 2012, 23, 2039–2054. [Google Scholar] [CrossRef] [Green Version]
- Millar, S.; Desmulliez, M. MEMS ultra low leak detection methods: A review. Sens. Rev. 2009, 29, 339–344. [Google Scholar] [CrossRef]
- Martínez Rojas, J.A.; Fernández, J.L.; Sánchez Montero, R.; López Espí, P.L.; Diez-Jimenez, E. Model-based systems engineering applied to trade-off analysis of wireless power transfer technologies for implanted biomedical microdevices. Sensors 2021, 21, 3201. [Google Scholar] [CrossRef]
- Ayela, F.; Fournier, T. An experimental study of anharmonic micromachined silicon resonators. Meas. Sci. Technol. 1998, 9, 1821–1830. [Google Scholar] [CrossRef]
- Nabholz, U.; Heinzelmann, W.; Mehner, J.E.; Degenfeld-Schonburg, P. Amplitude- and gas pressure-dependent nonlinear damping of high-Q oscillatory MEMS micro mirrors. J. Microelectromech. Syst. 2018, 27, 383–391. [Google Scholar] [CrossRef] [Green Version]
- Lifshitz, R.; Cross, M.C. Nonlinear Dynamics of Nanomechanical and Micromechanical Resonators. In Review of Nonlinear Dynamics and Complexity; John Wiley & Sons: Hoboken, NJ, USA, 2008. [Google Scholar]
- Duffing, G. Erzwungene Schwingungen bei Veränderlicher Eigenfrequenz und ihre Technische Bedeutung; Vieweg & Sohn: Braunschweig, Germany, 1918. [Google Scholar]
- Tajaddodianfar, F.; Yazdi, M.R.H.; Pishkenari, H.N. Nonlinear dynamics of MEMS/NEMS resonators: Analytical solution by the homotopy analysis method. Microsyst. Technol. 2017, 23, 1913–1926. [Google Scholar] [CrossRef]
- Mahmoodi, S.N.; Jalili, N.; Daqaq, M.F. Modeling, nonlinear dynamics, and identification of a piezoelectrically actuated microcantilever sensor. IEEE ASME Trans. Mechatron. 2008, 13, 58–65. [Google Scholar] [CrossRef]
- McHugh, K.; Dowell, E. Nonlinear responses of inextensible cantilever and free–free beams undergoing large deflections. J. Appl. Mech. 2018, 85, 051008. [Google Scholar] [CrossRef] [Green Version]
- Villanueva, L.G.; Karabalin, R.B.; Matheny, M.H.; Chi, D.; Sader, J.E.; Roukes, M.L. Nonlinearity in nanomechanical cantilevers. Phys. Rev. B 2013, 87, 024304. [Google Scholar] [CrossRef]
- Ababneh, A.; Schmid, U.; Hernando, J.; Sánchez-Rojas, J.L.; Seidel, H. The influence of sputter deposition parameters on piezoelectric and mechanical properties of AlN thin films. Mater. Sci. Eng. B Solid State Mater Adv. Technol. 2010, 172, 253–258. [Google Scholar] [CrossRef]
- Davis, W.O. Measuring quality factor from a nonlinear frequency response with jump discontinuities. J. Microelectromech. Syst. 2011, 20, 968–975. [Google Scholar] [CrossRef]
- Qiu, H.; Schwarz, P.; Völlm, H.; Feili, D.; Wu, X.; Seidel, H. Electrical crosstalk in two-port piezoelectric resonators and compensation solutions. J. Micromech. Microeng. 2013, 23, 045007. [Google Scholar] [CrossRef]
- Kucera, M.; Wistrela, E.; Pfusterschmied, G.; Ruiz-Díez, V.; Manzaneque, T.; Sánchez-Rojas, J.L.; Schalk, J.; Bittner, A.; Schmid, U. Characterization of a roof tile-shaped out-of-plane vibrational mode in aluminum-nitride-actuated self-sensing micro-resonators for liquid monitoring purposes. Appl. Phys. Lett. 2014, 104, 233501. [Google Scholar] [CrossRef]
- Sanchez-Rojas, J.L.; Hernando, J.; Donoso, A.; Bellido, J.; Manzaneque, T.; Ababneh, A.; Seidel, H.; Schmid, U. Modal optimization and filtering in piezoelectric microplate resonators. J. Micromech. Microeng. 2010, 20, 055027. [Google Scholar] [CrossRef]
- Kim, B.; Candler, R.N.; Hopcroft, M.A.; Agarwal, M.; Park, W.T.; Kenny, T.W. Frequency stability of wafer-scale encapsulated MEMS resonators. Sens. Actuator A Phys. 2007, 136, 125–131. [Google Scholar] [CrossRef]
- Prikhodko, I.; Trusov, A.; Shkel, A. Compensation of drifts in high-Q MEMS gyroscopes using temperature self-sensing. Sens. Actuator A Phys. 2013, 201, 517–524. [Google Scholar] [CrossRef] [Green Version]
- Tanner, D.; Olsson, R., III; Parson, T.; Crouch, S.; Walraven, J.; Ohlhausen, J. Stability experiments on MEMS aluminum nitride RF resonators. Proc. SPIE 2010, 7592, 759209. [Google Scholar]
- Defoort, M.; Taheri-Tehrani, P.; Horsley, D.A. Exploiting nonlinear amplitude-frequency dependence for temperature compensation in silicon micromechanical resonators. Appl. Phys. Lett. 2016, 109, 153502. [Google Scholar] [CrossRef]
- Zuo, J.; Zhang, H.; Chang, Y.; Liang, J.; Pang, W.; Duan, X. Highly sensitive AlN contour-mode resonator-based pressure sensor for in-line monitoring of chemical reactions. In Proceedings of the 20th International Conference on Solid-State Sensors, Actuators and Microsystems & Eurosensors XXXIII (TRANSDUCERS & EUROSENSORS XXXIII), Berlin, Germany, 23–27 June 2019; pp. 1373–1376. [Google Scholar]
- Wang, T.; Tang, Z.; Lin, H.; Zhan, K.; Wan, J.; Wu, S.; Gu, Y.; Luo, W.; Zhang, W. A low temperature drifting acoustic wave pressure sensor with an integrated vacuum cavity for absolute pressure sensing. Sensors 2020, 20, 1788. [Google Scholar] [CrossRef] [Green Version]
- Anderås, E.; Katardjiev, I.; Yantchev, V. Lamb wave resonant pressure micro-sensor utilizing a thin-film aluminium nitride membrane. J. Micromech. Microeng. 2011, 21, 085010. [Google Scholar] [CrossRef]
- Rodríguez-Madrid, J.G.; Iriarte, G.F.; Williams, O.A.; Calle, F. High precision pressure sensors based on SAW devices in the GHz range. Sens. Actuator A Phys. 2013, 189, 364–369. [Google Scholar] [CrossRef] [Green Version]
- Han, X.; Mao, Q.; Zhao, L.; Li, X.; Li, W.; Yang, P.; Wang, Y.; Yan, X.; Wang, S.; Zhu, N.; et al. Novel resonant pressure sensor based on piezoresistive detection and symmetrical in-plane mode vibration. Microsyst. Nanoeng. 2020, 6, 95. [Google Scholar] [CrossRef]
- Shi, X.; Lu, Y.; Xie, B.; Li, Y.; Wang, J.; Chen, D.; Chen, J. A resonant pressure microsensor based on double-ended tuning fork and electrostatic excitation/piezoresistive detection. Sensors 2018, 18, 2494. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Zengerle, T.; Stopp, M.; Ababneh, A.; Seidel, H. Investigations on nonlinearities of roof-tile shape modes for pressure measurement applications. In Proceedings of the 21th International Conference on Solid-State Sensors, Actuators and Microsystems, TRANSDUCERS 21, Online, 20–25 June 2021. [Google Scholar]
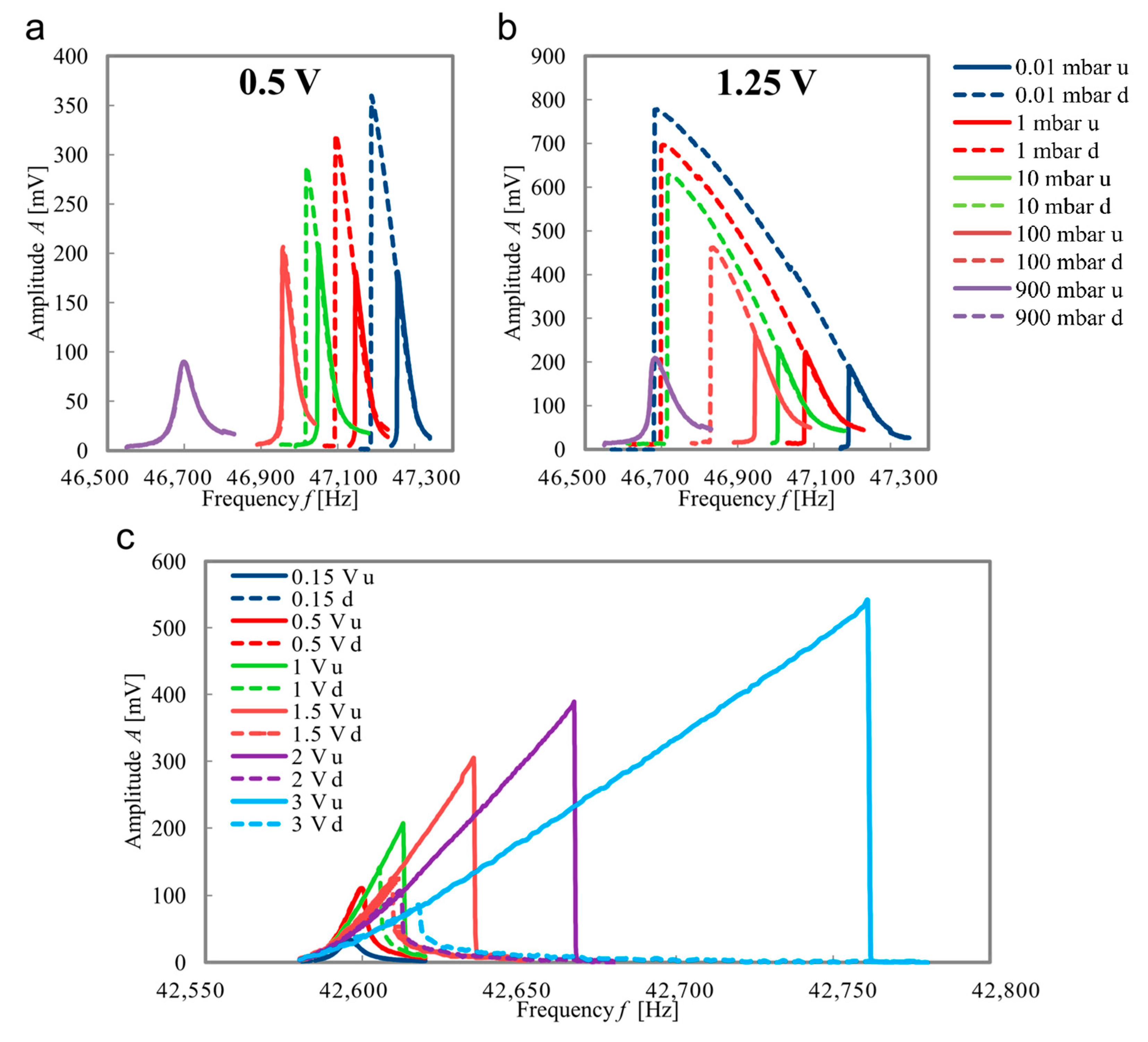
| Ayela’s Model [9] | Amplitude [mV] | Frequency [Hz] |
|---|---|---|
| Upward Sweep | ||
| Downward Sweep |
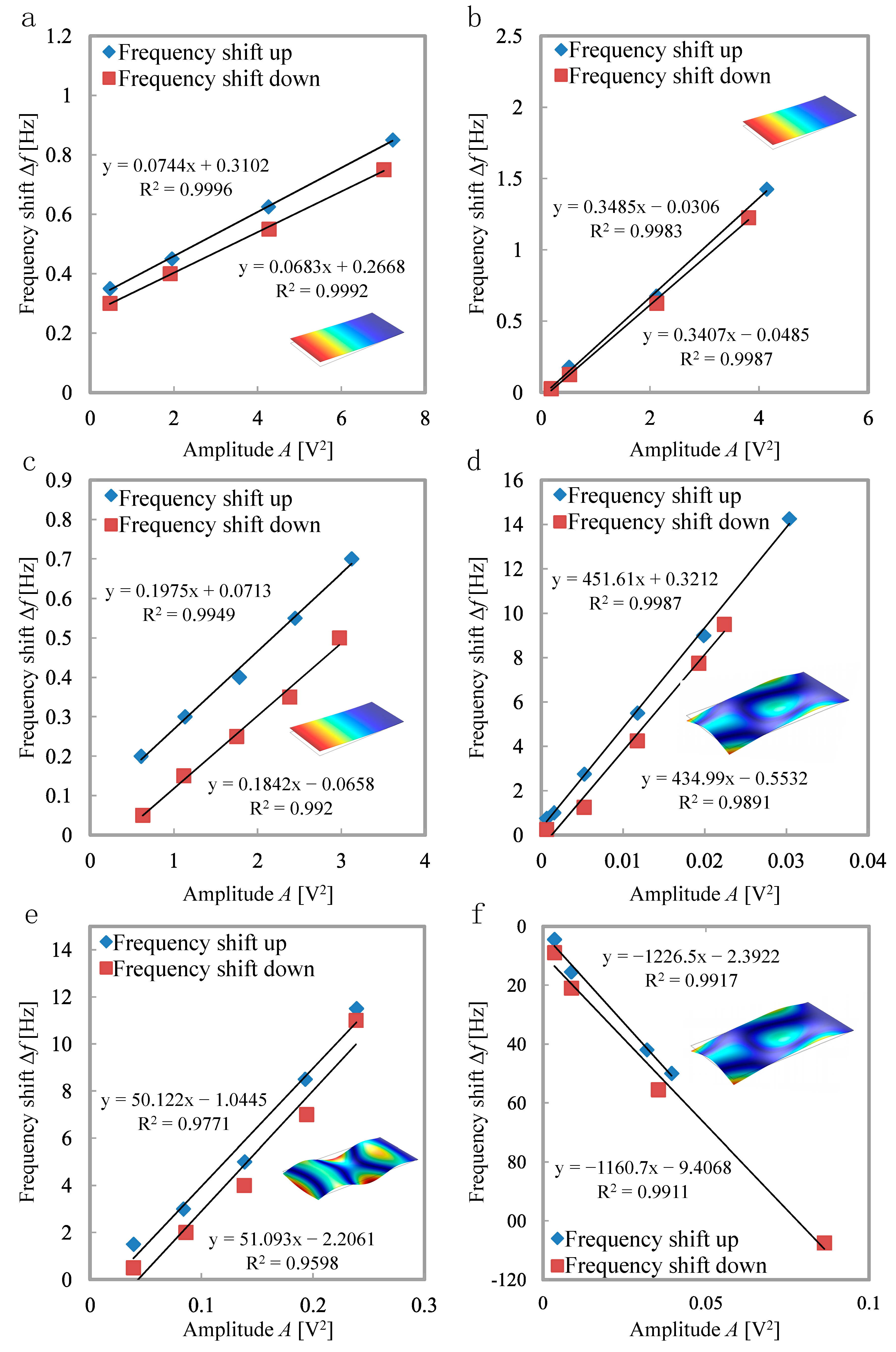
| Sensor | Mode | Resonance Frequency fr [Hz] | Sweep Direction | χ [m−2s−1] | β [m−2s−2] |
|---|---|---|---|---|---|
| C33 | 1st bending | 1396.6 | up | 0.0744 | 1741 |
| down | 0.0683 | 1598 | |||
| C50 | 1st bending | 1448.3 | up | 0.3485 | 8457 |
| down | 0.3307 | 8025 | |||
| C100 | 1st bending | 967.9 | up | 0.1975 | 3203 |
| down | 0.1842 | 2987 | |||
| C100 | 4th bending | 35543 | up | 50.122 | 29.8 × 106 |
| down | 51.093 | 30.4 × 106 | |||
| C33 | 2nd roof-tile-shape | 42704.5 | up | 435.61 | 31.2 × 107 |
| down | 434.99 | 31.1 × 107 | |||
| C100 | 2nd roof-tile-shape | 47355.5 | up | −1226.5 | −97.3 × 107 |
| down | −1160.7 | −92.1 × 107 |
| Sensor | Measurement Range [mbar] | Sensitivity [Hz/mbar] | Sensitivity [ppm/mbar] | Measurement Principle | Resonance Frequency |
|---|---|---|---|---|---|
| Zuo [30] | 50–2000 | 16.5 | 0.1 | AlN Contour mode resonator | 140 MHz |
| Wang [31] | 100–4000 | 221 | 0.27 | AlN Contour mode resonator | 820 MHz |
| Anderas [32] | 0.1–500 | 360 | 0.4 | AlN Contour mode resonator | 900 MHz |
| Rodriguez-Madrid [33] | 1000–4000 | 330 | 0.03 | AlN Surface acoustic wave resonator | 10.8 GHz |
| Han [34] | 0.01–2000 | 1.9 | 35 | Capacitive oscillator with piezoelectric read out | 53 kHz |
| Shi [35] | 100–1500 | 8 | 94 | Capacitive oscillator with piezoelectric read out | 85 kHz |
| This work C_100 U = 1.5 Vpp | 1–10 10–100 100–900 | 17 3.4 0.23 | 362 72 4.9 | Duffing nonlinearity | 47 kHz |
| This work C_100 U = 1.25 Vpp | 0.001–1 1–10 10–100 100–300 | 136 13 2.6 0.35 | 2894 277 55 7.4 | Duffing nonlinearity | 47 kHz |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zengerle, T.; Stopp, M.; Ababneh, A.; Seidel, H. Using the Nonlinear Duffing Effect of Piezoelectric Micro-Oscillators for Wide-Range Pressure Sensing. Actuators 2021, 10, 172. https://doi.org/10.3390/act10080172
Zengerle T, Stopp M, Ababneh A, Seidel H. Using the Nonlinear Duffing Effect of Piezoelectric Micro-Oscillators for Wide-Range Pressure Sensing. Actuators. 2021; 10(8):172. https://doi.org/10.3390/act10080172
Chicago/Turabian StyleZengerle, Tobias, Michael Stopp, Abdallah Ababneh, and Helmut Seidel. 2021. "Using the Nonlinear Duffing Effect of Piezoelectric Micro-Oscillators for Wide-Range Pressure Sensing" Actuators 10, no. 8: 172. https://doi.org/10.3390/act10080172
APA StyleZengerle, T., Stopp, M., Ababneh, A., & Seidel, H. (2021). Using the Nonlinear Duffing Effect of Piezoelectric Micro-Oscillators for Wide-Range Pressure Sensing. Actuators, 10(8), 172. https://doi.org/10.3390/act10080172






