Torque Measurement Technology by Using a Magnetostrictive Ring and Multiple Magnets
Abstract
1. Introduction
2. Materials and Methods
2.1. Torque Sensing Material
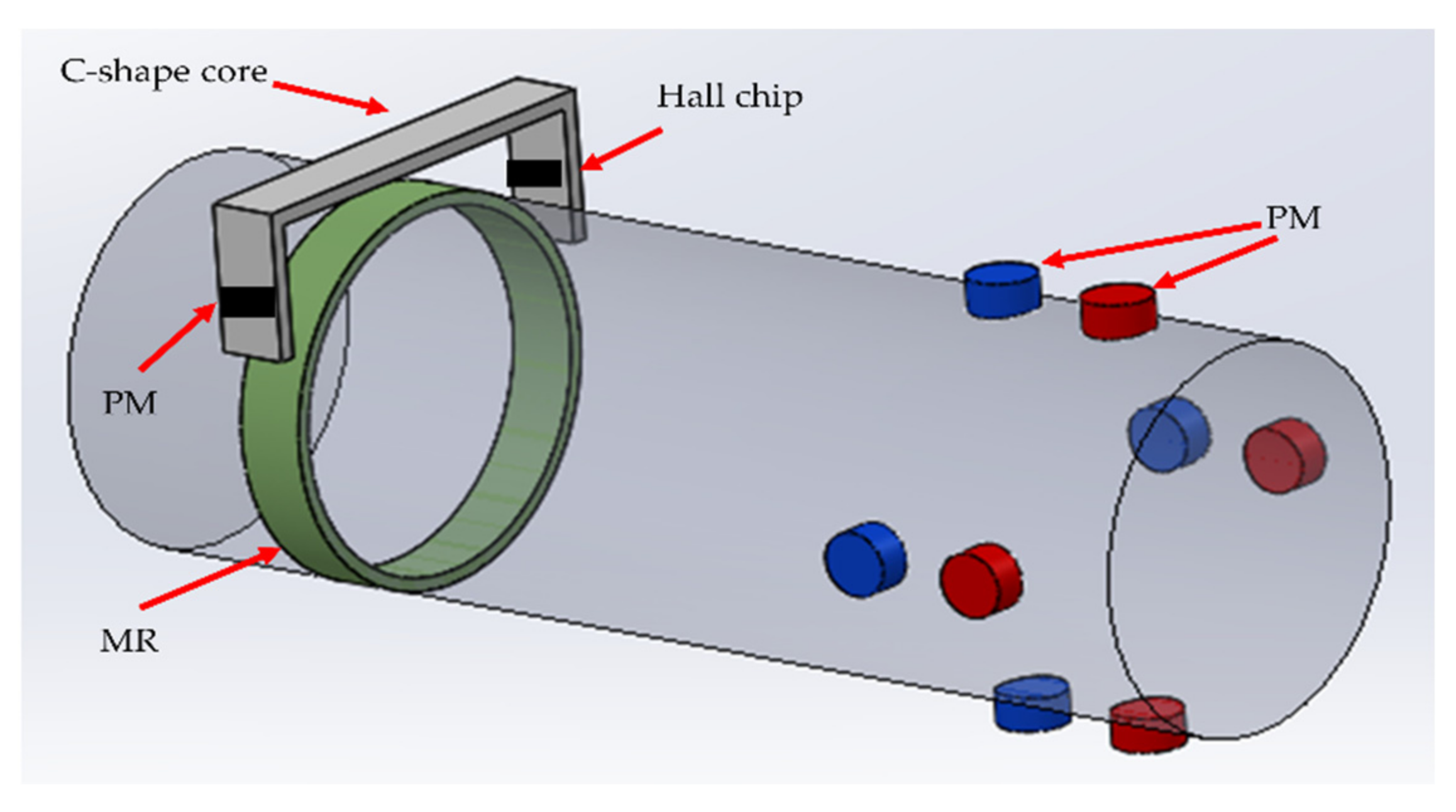
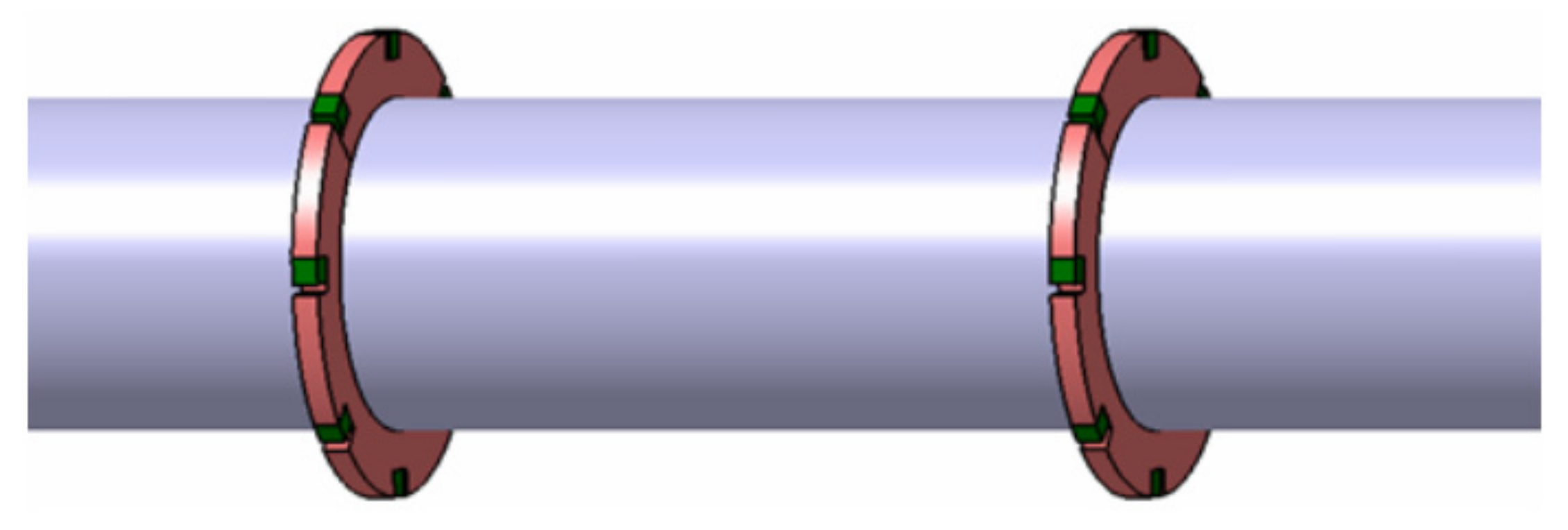
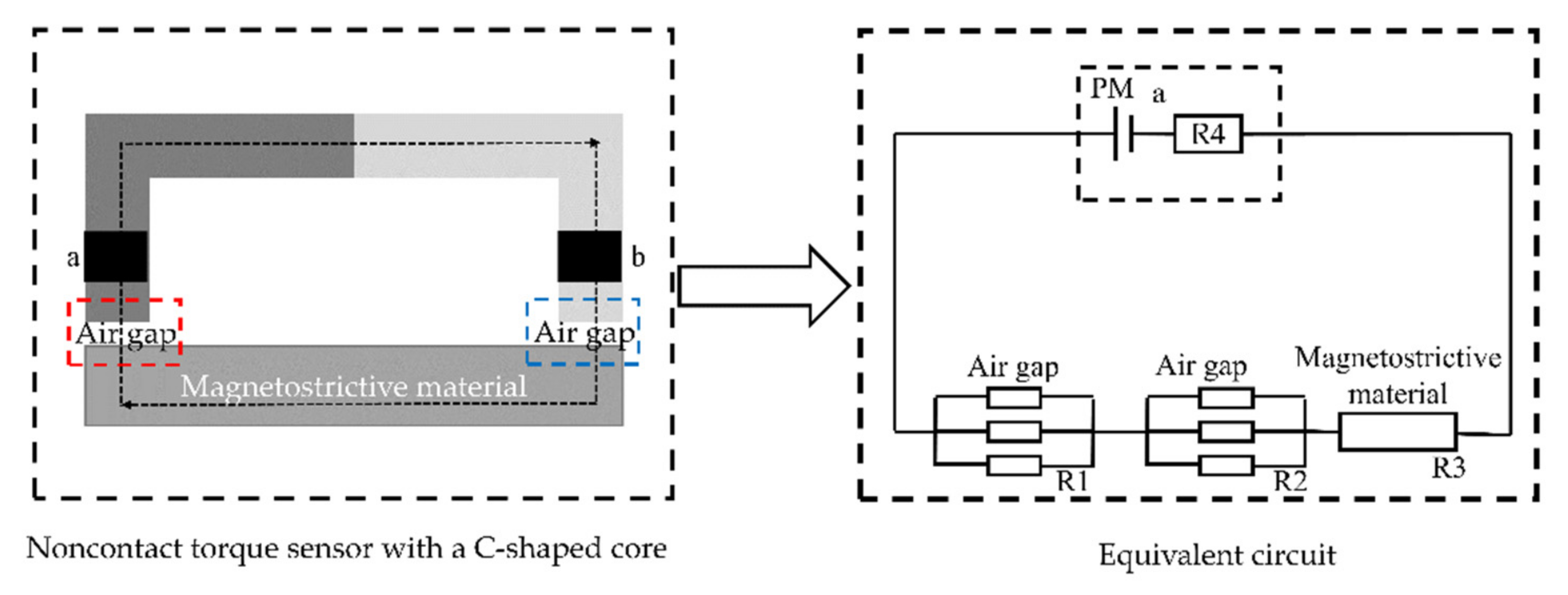
2.2. Structure of the Proposed Sensor
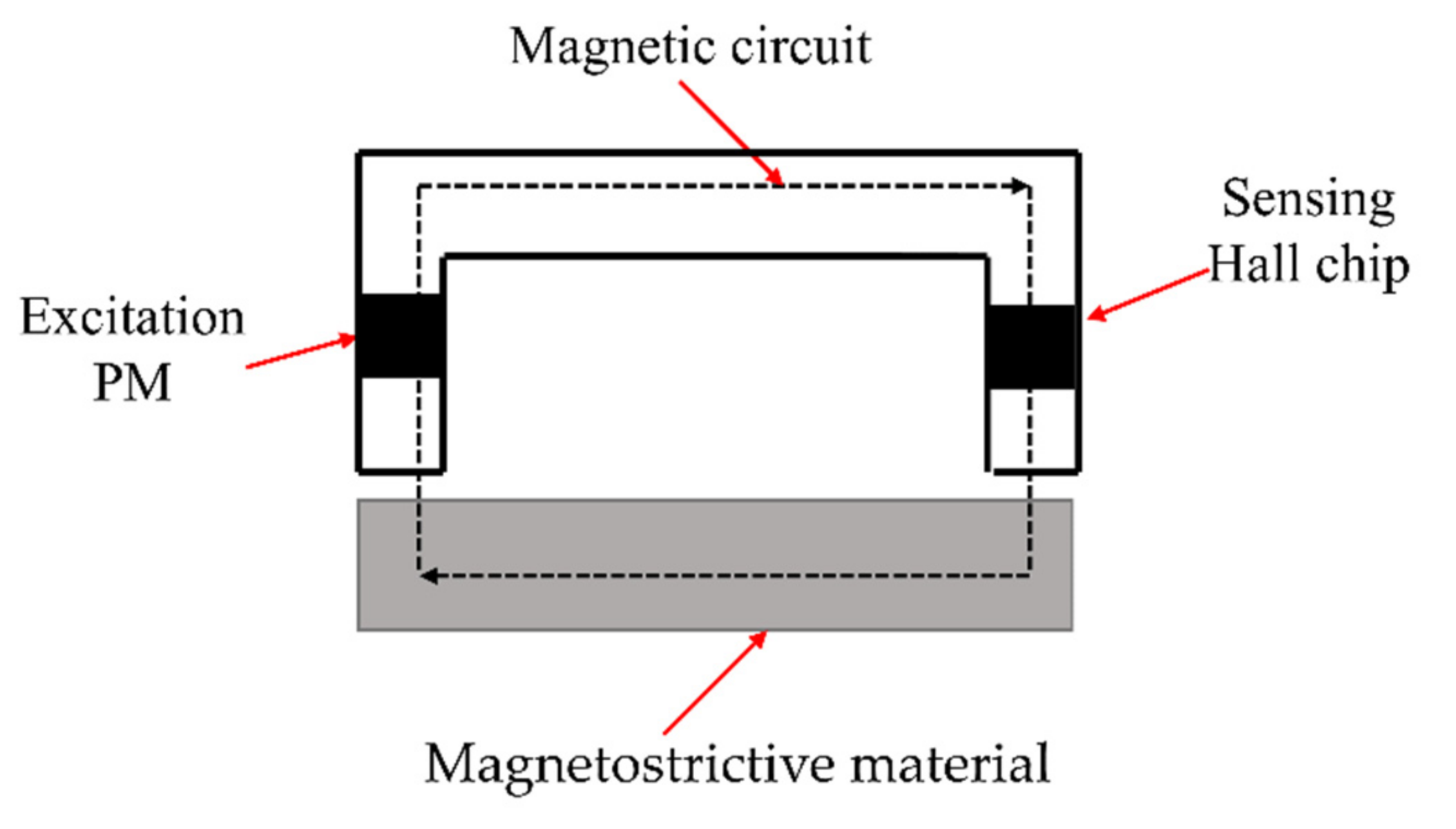
2.3. Working Principle
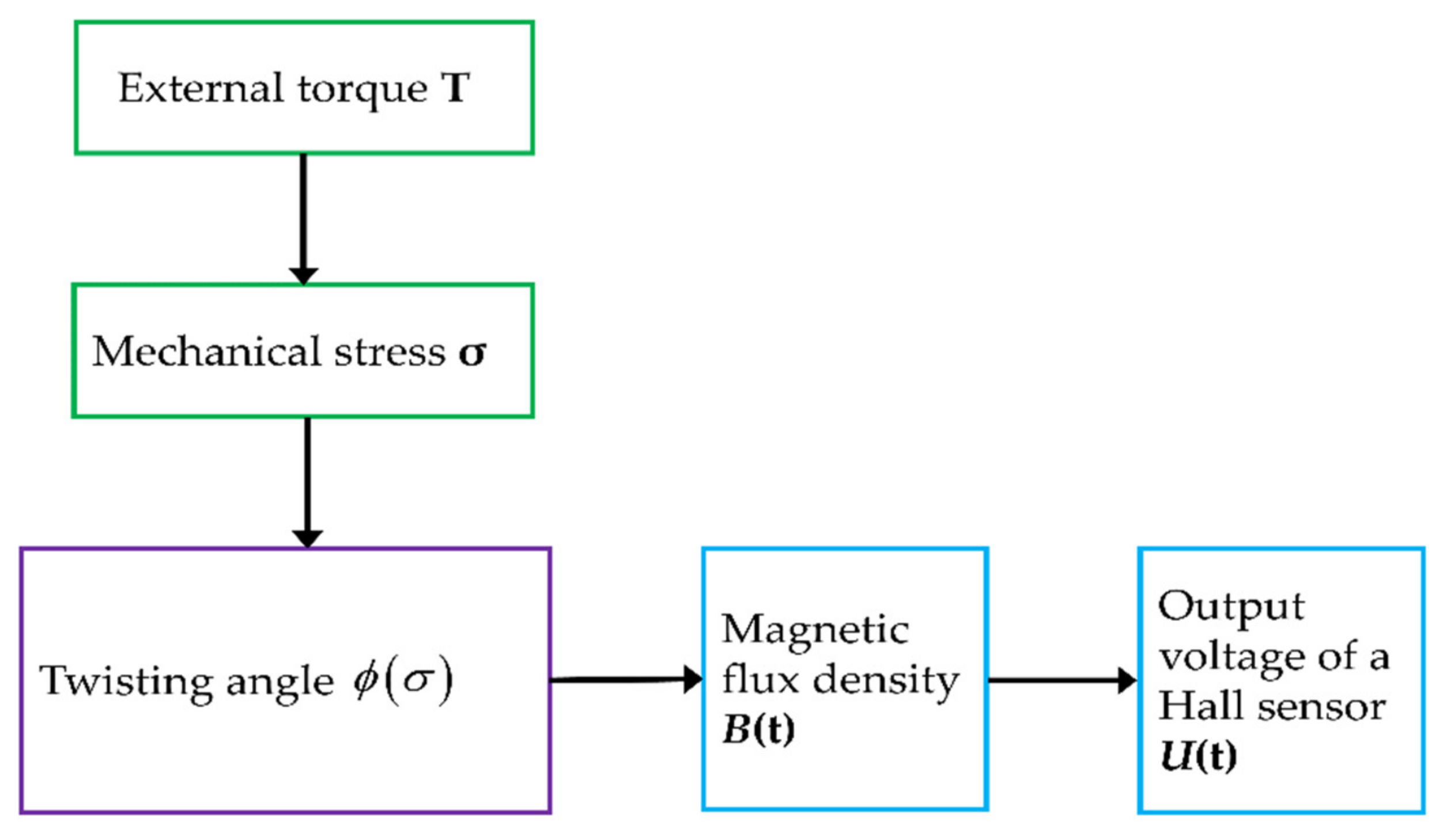
2.3.1. The Method Based on Twist Measurement
2.3.2. The Method Based on the Inverse Magnetostrictive Effect
3. Results and Discussion
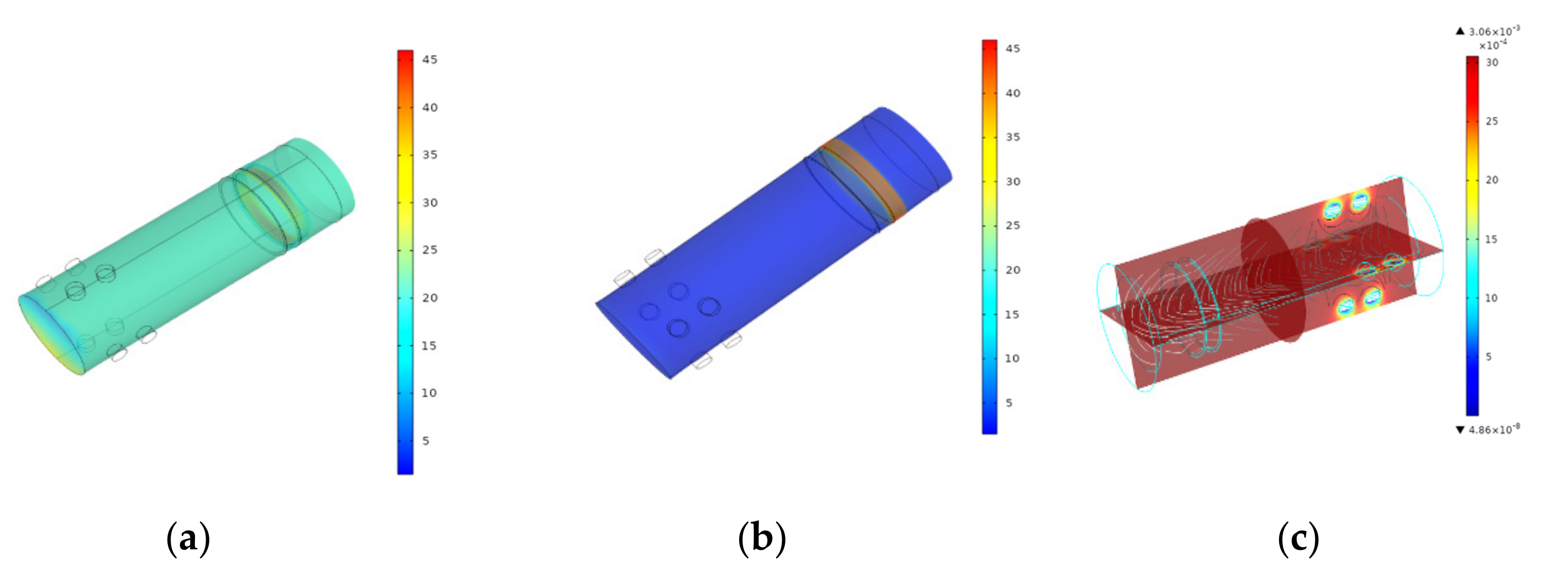
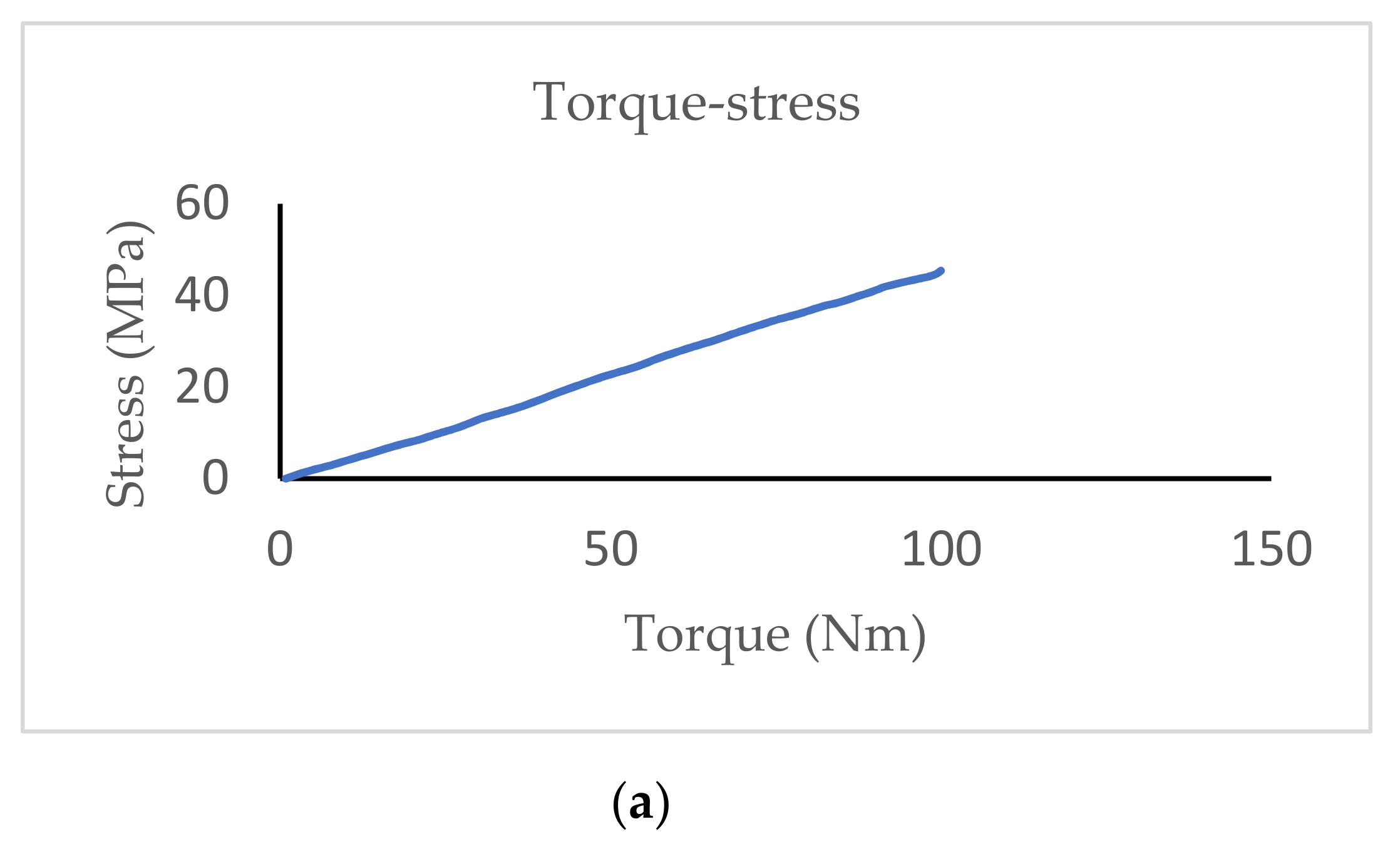
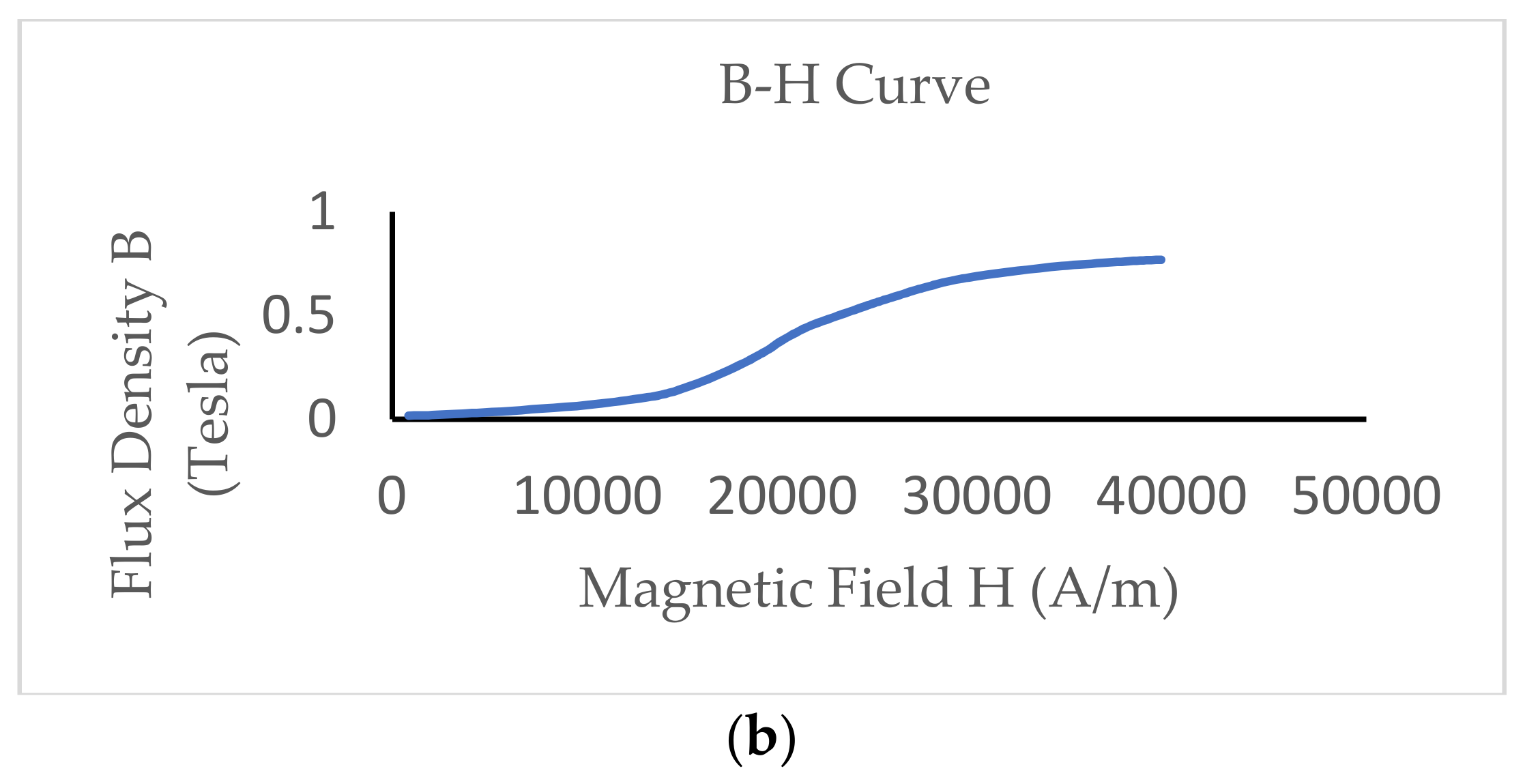
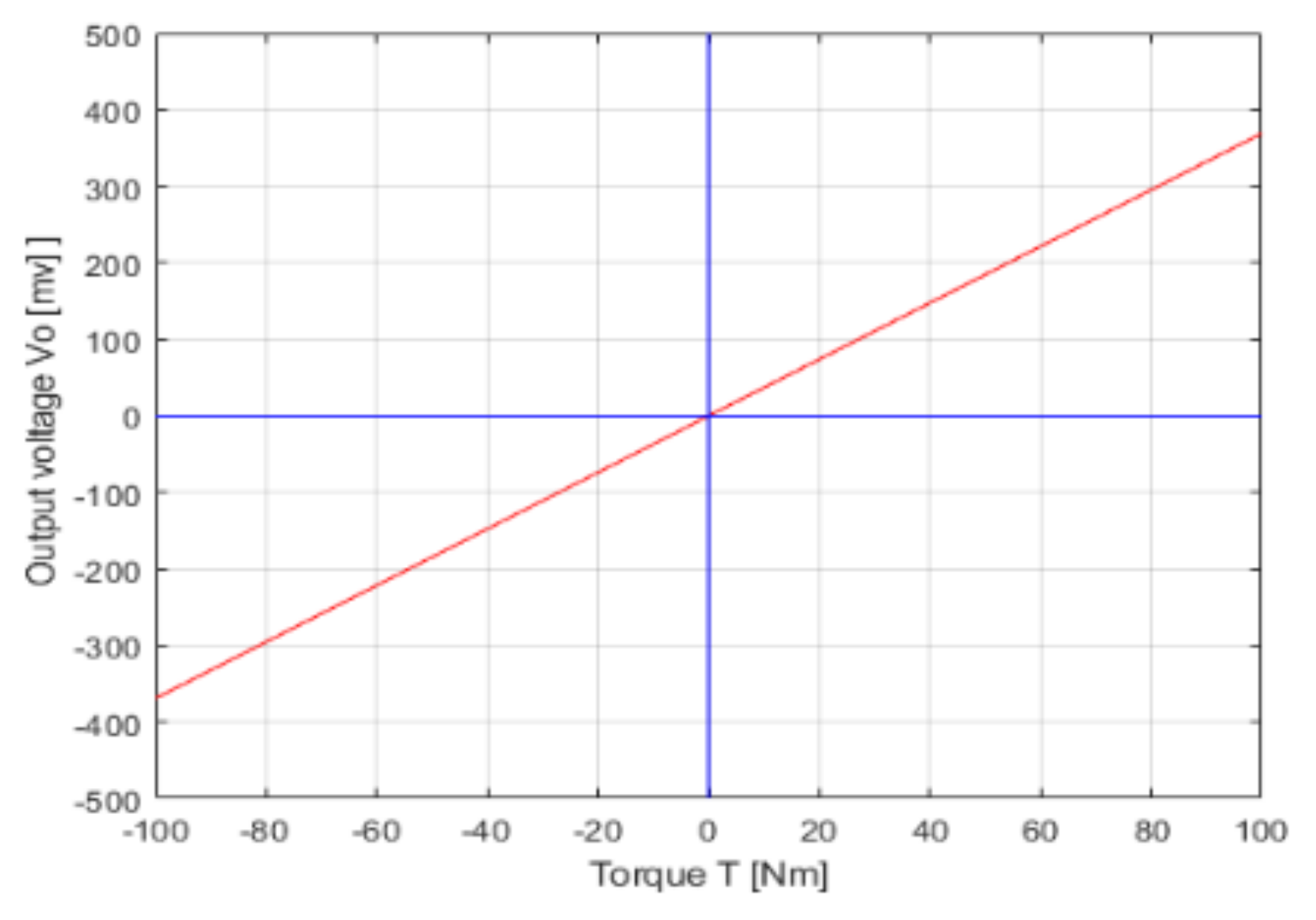
3.1. Modelling and Simulation
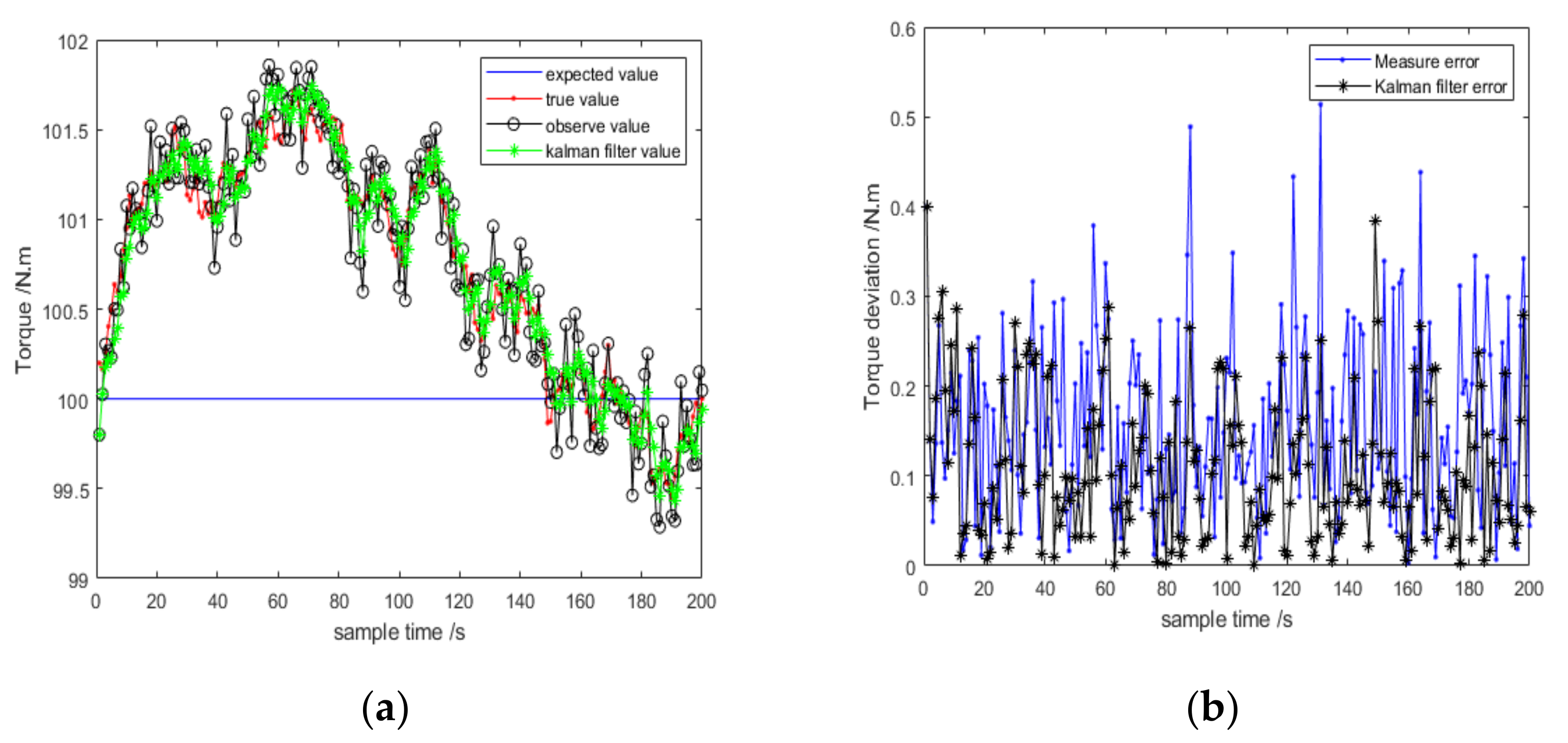
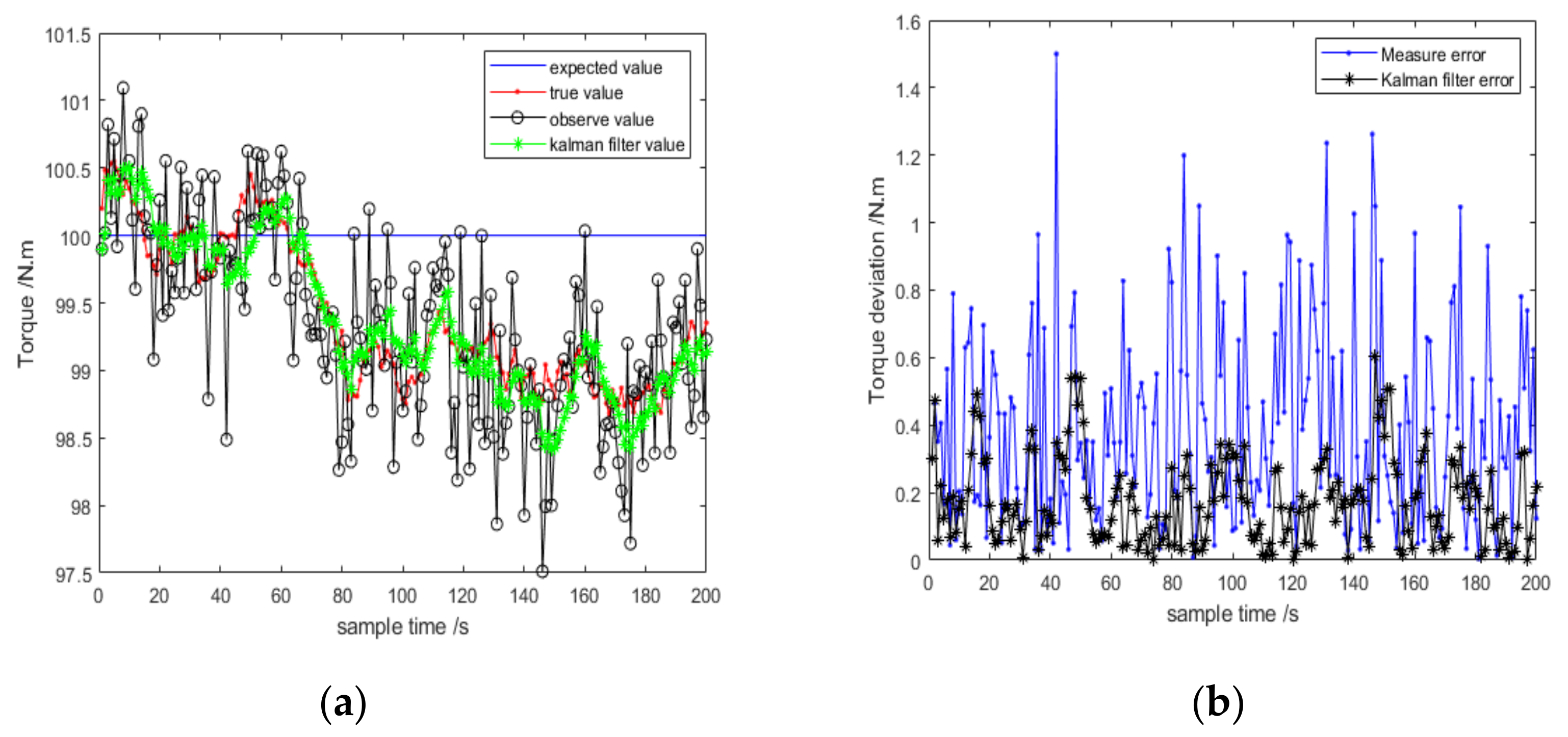
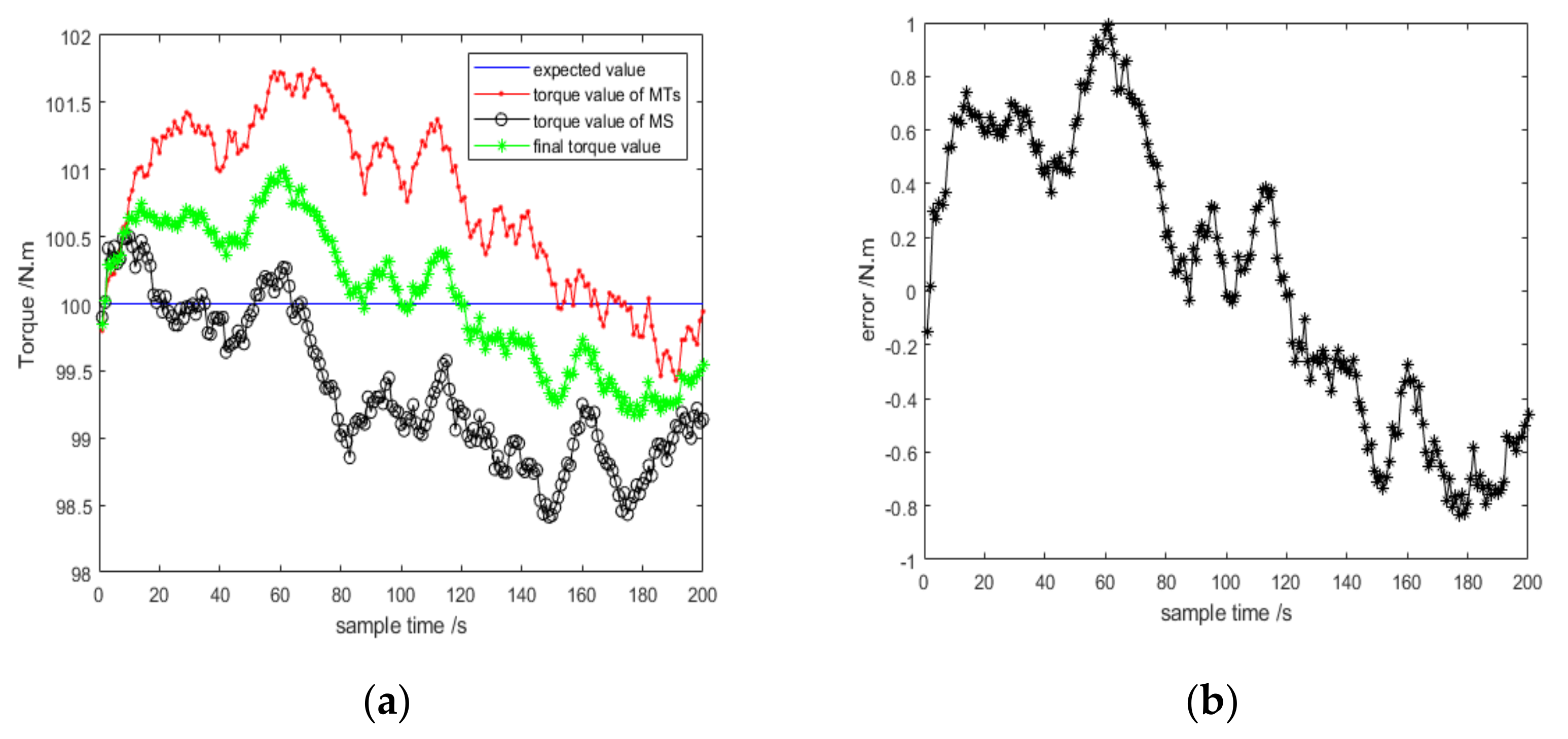
3.2. Data Processing
- no perfect mathematic model;
- the disturbances in the system are uncontrollable and difficult to model;
- errors exist in the measurement sensor.
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Vetelino, J.; Reghu, A. Introduction to Sensors; CRC press: Boca Raton, FL, USA, 2017. [Google Scholar]
- Tan, Y.; Wang, X.; Ren, L. Design and experiment of a cardan-type self-decoupled and self-powered bending moment and torque sensor. IEEE Trans. Ind. Electron. 2020. [Google Scholar] [CrossRef]
- Morris, A.S.; Langari, R. Measurement and Instrumentation: Theory and Application; Academic Press: New York, NY, USA, 2012. [Google Scholar]
- Fleming, W.J. Overview of automotive sensors. IEEE Sens. J. 2001, 1, 296–308. [Google Scholar] [CrossRef]
- Goszczak, J. Torque measurement issues. In Proceedings of the IOP Conference Series: Materials Science and Engineering, Bali, Indonesia, 19–20 March 2016; p. 012041. [Google Scholar]
- Lee, K.; Cho, C. Study of noncontact torque measurement method with magnetic sensor band. J. Mech. Sci. Technol. 2015, 29, 3897–3903. [Google Scholar] [CrossRef]
- Zappalá, D.; Bezziccheri, M.; Crabtree, C.J.; Paone, N. Non-intrusive torque measurement for rotating shafts using optical sensing of zebra-tapes. Meas. Sci. Technol. 2018, 29. [Google Scholar] [CrossRef]
- Muro, H.; Saito, C.; Shimada, M.; Furuya, Y. Magnetostrictive-ring type torque sensor using two Hall ICs with differential magnetic field detection. In Proceedings of the SENSORS, 2014 IEEE, Valencia, Spain, 2–5 November 2014; pp. 412–415. [Google Scholar]
- Garshelis, I.J. A torque transducer utilizing a circularly polarized ring. IEEE Trans. Magn. 1992, 28, 2202–2204. [Google Scholar] [CrossRef]
- Tsujisawa, T.; Yamakawa, K. Improvement of the angular-dependent noise in a magneto-striction type torque sensor. Int. J. Automot. Eng. 2011, 2, 75–80. [Google Scholar] [CrossRef][Green Version]
- Ruser, H.; Troltzsch, U.; Horn, M. Low-cost magnetic torque sensor principle. In Proceedings of the SENSORS, 2002 IEEE, Orlando, FL, USA, 12–14 June 2002; pp. 901–904. [Google Scholar]
- Fleming, W.J. Magnetostrictive torque sensors—Derivation of transducer model. SAE Trans. 1989, 98, 519–538. [Google Scholar]
- Moffett, M.B.; Clark, A.E.; Wun-Fogle, M.; Linberg, J.; Teter, J.P.; McLaughlin, E.A. Characterization of Terfenol-D for magnetostrictive transducers. J. Acoust. Soc. Am. 1991, 89, 1448–1455. [Google Scholar] [CrossRef]
- Fraden, J. Handbook of Modern Sensors: Physics, Designs, and Applications; Springer: Berlin/Heidelberg, Germany, 1998. [Google Scholar]
- Skarpetis, M.G.; Tsiantos, V.; Karagiannis, V.; Ktena, A.; Manasis, C.; Ladoukakis, O.; Elias, C.; Hristoforou, E.; Vourna, P. Modelling of a Magnetostrictive Torque Sensor. MATEC Web Conf. 2016, 41. [Google Scholar] [CrossRef]
- Apicella, V.; Clemente, C.S.; Davino, D.; Leone, D.; Visone, C. Review of modeling and control of magnetostrictive actuators. Actuators 2019, 8, 45. [Google Scholar] [CrossRef]
- Wu, G.; Zhang, Y.; Liu, K.; Cheng, M.; Xiao, K. Design Method of Magnetic Circuit for Permanent Electromagnetic Bearing. CNKI J. 2004. [Google Scholar] [CrossRef]
- Wang, S.L.; Wang, W.; Su, S.Q.; Zhang, S.F. A magneto-mechanical model on differential permeability and stress of ferromagnetic material. J. Xi’an Univ. Sci. Technol. 2005. [Google Scholar] [CrossRef]
- Oberkampf, W.L.; Helton, J.C.; Joslyn, C.A.; Wojtkiewicz, S.F.; Ferson, S. Challenge problems: Uncertainty in system response given uncertain parameters. Reliab. Eng. Syst. Saf. 2004, 85, 11–19. [Google Scholar] [CrossRef]
- Galanis, G.; Anadranistakis, M. A one-dimensional Kalman filter for the correction of near surface temperature forecasts. Meteorol. Appl. A J. Forecast. Pract. Appl. Train. Tech. Model. 2002, 9, 437–441. [Google Scholar] [CrossRef]
- Bishop, G.; Welch, G. An introduction to the kalman filter. In Proceedings of the SIGGRAPH, Los Angeles, CA, USA, 12–17 August 2001; Volume 8, p. 41. [Google Scholar]
- Dobre, C.; Xhafa, F. Pervasive Computing: Next Generation Platforms for Intelligent Data Collection; Morgan Kaufmann: San Francisco, CA, USA, 2016. [Google Scholar]
- Higgins, W.T. A Comparison of Complementary and Kalman Filtering. IEEE Trans. Aerosp. Electron. Syst. 1975, AES-11, 321–325. [Google Scholar] [CrossRef]
- Islam, T.; Islam, M.S.; Shajid-Ul-Mahmud, M.; Hossam-E-Haider, M. Comparison of complementary and Kalman filter based data fusion for attitude heading reference system. In AIP Conference Proceedings; AIP Publishing LLC.: Melville, NY, USA, 2017; p. 020002. [Google Scholar]





| Physical Properties | Magnetic Properties | ||
|---|---|---|---|
| Density (g/cm3) | 7.87 | Curie temperature (°C) | 380 |
| Crystallization Temperature (°C) | >400 | Electrical resistivity (μΩ/cm) | 58 |
| Saturation magnetostriction coefficient(ppm) | 1400 | Saturation magnetostriction strain (room temperature) | 10−3 |
| Lower operating temperature limit (°C) | 15 | Saturation magnetization(A/m3) | 1.5 × 106 |
| Material Properties | Value |
|---|---|
| Density (g/cm3) | 7.4–7.6 |
| Curie temperature (°C) | 310–370 |
| Maximum operating temperature (°C) | 80–200 |
| Material Properties | Value |
|---|---|
| E (Young’s modulus) | 60–109 Pa |
| ν (Poisson’s ratio) | 0.45 |
| σ (Electric conductivity) | 5.96·106 S/m |
| εr (Relative permittivity) | 1 |
| λs (Saturation magnetostriction) | 2·10−4 |
| Temperature sensitivity | 20% loss at 80 °C |
| Robustness | Very Brittle |
| Magnetic permeability | 3–10 |
| Material Properties | Value |
|---|---|
| Density | 2700 |
| Youngs modulus | 70 GPa |
| Magnetic susceptibility | 6.0 e−7 m3/kg |
| Poisson ratio | 0.36 |
| Electrical resistivity | 0.0000027 ohm-cm |
| Parameters | Value (Kalman Filter 1) | Value (Kalman Filter 2) |
|---|---|---|
| 100.2 | 100.2 | |
| 4 | 4 | |
| 0.01 | 0.01 | |
| 0.25 | 0.25 | |
| 99.8 | 99.9 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Xu, F.; Dhimole, V.K.; Cho, C. Torque Measurement Technology by Using a Magnetostrictive Ring and Multiple Magnets. Actuators 2021, 10, 124. https://doi.org/10.3390/act10060124
Xu F, Dhimole VK, Cho C. Torque Measurement Technology by Using a Magnetostrictive Ring and Multiple Magnets. Actuators. 2021; 10(6):124. https://doi.org/10.3390/act10060124
Chicago/Turabian StyleXu, Feng, Vivek Kumar Dhimole, and Chongdu Cho. 2021. "Torque Measurement Technology by Using a Magnetostrictive Ring and Multiple Magnets" Actuators 10, no. 6: 124. https://doi.org/10.3390/act10060124
APA StyleXu, F., Dhimole, V. K., & Cho, C. (2021). Torque Measurement Technology by Using a Magnetostrictive Ring and Multiple Magnets. Actuators, 10(6), 124. https://doi.org/10.3390/act10060124







