Optimized Voltage-Induced Control of Magnetic Domain-Wall Propagation in Hybrid Piezoelectric/Magnetostrictive Devices
Abstract
1. Introduction
2. Materials and Methods
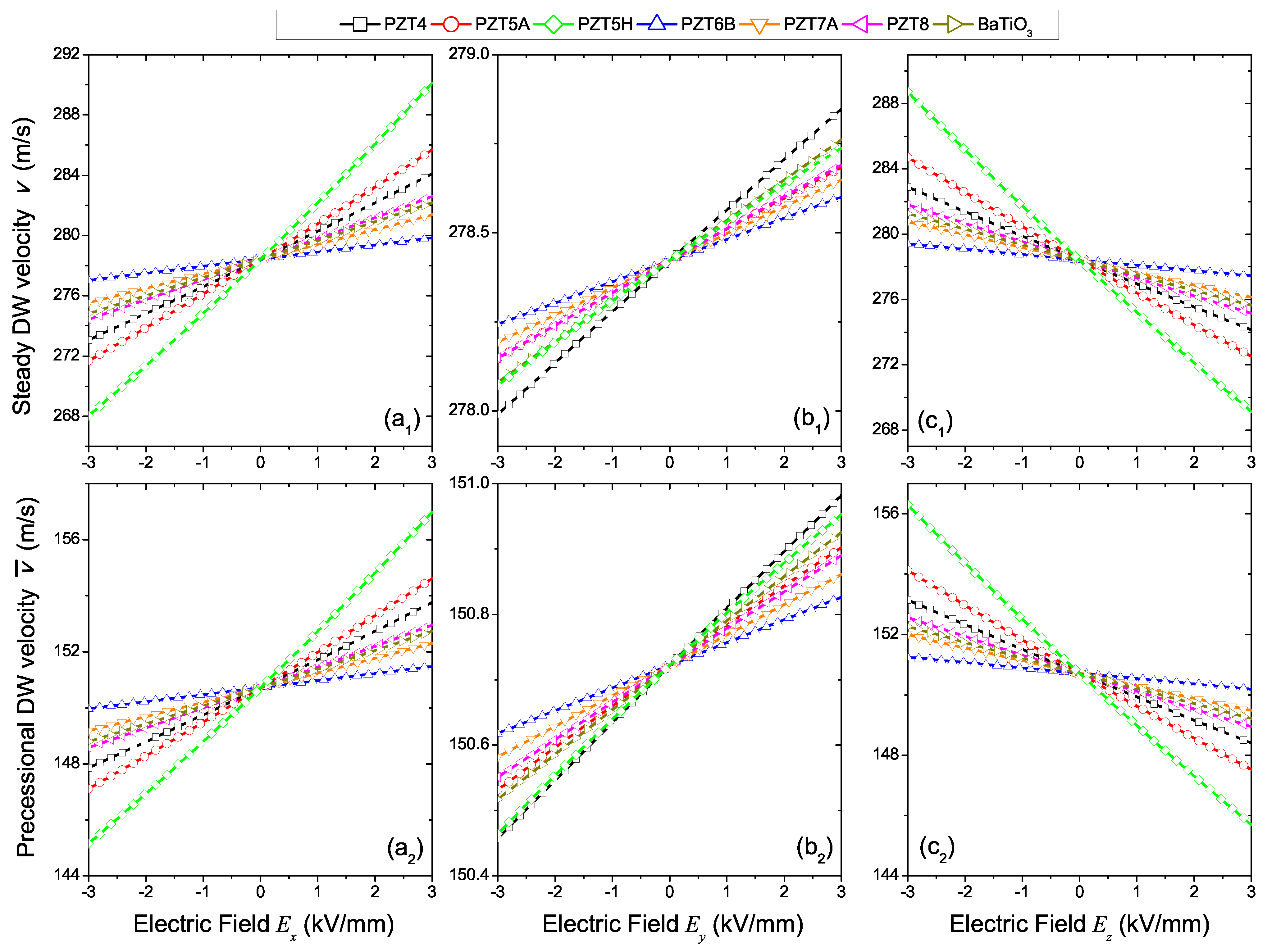
3. Results and Discussion
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Conflicts of Interest
Abbreviations
| PZ | Piezoelectric |
| MS | Magnetostrictive |
| ME | Magnetoelastic |
| DW | Domain-Wall |
| LLG | Landau-Lifshitz-Gilbert equation |
| WB | Walker Breakdown |
References
- Hubert, A.; Schäfer, R. Magnetic Domains: The Analysis of Magnetic Microstructures; Springer: Berlin/Heidelberg, Germany, 2008. [Google Scholar]
- Chikazumi, S.; Graham, C.D. Physics of Ferromagnetism; Oxford University Press: Oxford, UK, 2009. [Google Scholar]
- Cullity, B.D.; Graham, C.D. Introduction to Magnetic Materials; Wiley: New York, NY, USA, 2009. [Google Scholar]
- Atkinson, D.; Eastwood, D.S.; Bogart, L.K. Controlling domain wall pinning in planar nanowires by selecting domain wall type and its application in a memory concept. Appl. Phys. Lett. 2008, 92, 022510. [Google Scholar] [CrossRef]
- Ono, T.; Nakatani, Y. Magnetic domain wall oscillator. Appl. Phys. Exp. 2008, 1, 061301. [Google Scholar] [CrossRef]
- Allwood, D.; Xiong, G.; Cooke, M.D.; Faulkner, C.C.; Atkinson, D.; Vernier, N.; Cowburn, R.P. Submicrometer ferromagnetic NOT gate and shift register. Science 2002, 296, 2003–2006. [Google Scholar] [CrossRef] [PubMed]
- Allwood, D.A.; Xiong, G.; Faulkner, C.C.; Atkinson, D.; Petit, D.; Cowburn, R.P. Magnetic domain-wall logic. Science 2005, 309, 1688–1692. [Google Scholar] [CrossRef] [PubMed]
- Xu, P.; Xia, K.; Gu, C.; Tang, L.; Yang, H.; Li, J. An all-metallic logic gate based on current-driven domain wall motion. Nat. Nanotechnol. 2008, 3, 97–100. [Google Scholar] [CrossRef]
- Eerenstein, W.; Mathur, N.D.; Scott, J.F. Multiferroic and magnetoelectric materials. Nature 2006, 442, 759–765. [Google Scholar] [CrossRef]
- Vaz, C.A.; Hoffman, J.; Ahn, C.H.; Ramesh, R. Magnetoelectric coupling effects in multiferroic complex oxide. Adv. Mater. 2010, 22, 2900–2918. [Google Scholar] [CrossRef]
- Balinskiy, M.; Chavez, A.C.; Barra, A.; Chiang, H.; Carman, G.P.; Khitun, A. Magnetoelectric spin wave modulator based on synthetic multiferroic structure. Sci. Rep. 2018, 8, 10867. [Google Scholar] [CrossRef]
- Lei, N.; Devolder, T.; Agnus, G.; Aubert, P.; Daniel, L.; Kim, J.V.; Zhao, W.; Trypiniotis, T.; Cowburn, R.P.; Chappert, C.; et al. Strain-controlled magnetic domain wall propagation in hybrid piezoelectric/ferromagnetic structures. Nat. Commun. 2013, 4, 1378. [Google Scholar] [CrossRef]
- De Ranieri, E.; Roy, P.E.; Fang, D.; Vehsthedt, E.K.; Irvine, A.C.; Heiss, D.; Casiraghi, A.; Campion, R.P.; Gallagher, B.L.; Jungwirth, T.; et al. Piezoelectric control of the mobility of a domain wall driven by adiabatic and non-adiabatic torques. Nat. Mater. 2013, 12, 808–814. [Google Scholar] [CrossRef]
- Shepley, P.M.; Rushforth, A.W.; Wang, M.; Burnell, G.; Moore, T.A. Modification of perpendicular magnetic anisotropy and domain wall velocity in Pt/Co/Pt by voltage-induced strain. Sci. Rep. 2015, 5, 7921. [Google Scholar] [CrossRef]
- Hu, J.M.; Yang, T.; Momeni, K.; Cheng, X.; Chen, L.; Lei, S.; Zhang, S.; Trolier-McKinstry, S.; Gopalan, V.; Carman, G.P.; et al. Fast magnetic domain-wall motion in a ring-shaped nanowire driven by a voltage. Nano Lett. 2016, 16, 2341–2348. [Google Scholar] [CrossRef] [PubMed]
- Consolo, G.; Valenti, G. Analytical solution of the strain-controlled magnetic domain wall motion in bilayer piezoelectric/magnetostrictive nanostructures. J. Appl. Phys. 2017, 121, 043903. [Google Scholar] [CrossRef]
- Consolo, G.; Valenti, G. Strain-mediated propagation of magnetic domain-walls in cubic magnetostrictive materials. Ric. Matem. 2021, 70, 81–97. [Google Scholar] [CrossRef]
- Consolo, G.; Federico, S.; Valenti, G. Magnetostriction in transversely isotropic hexagonal crystals. Phys. Rev. B 2020, 101, 014405. [Google Scholar] [CrossRef]
- Zhang, S.; Li, Z. Roles of nonequilibrium conduction electrons on the magnetization dynamics of ferromagnets. Phys. Rev. Lett. 2004, 93, 127204. [Google Scholar] [CrossRef]
- Schryer, N.L.; Walker, L.R. The motion of 180deg domain walls in uniform dc magnetic fields. J. Appl. Phys. 1974, 45, 5406–5421. [Google Scholar] [CrossRef]
- Consolo, G.; Currò, C.; Martinez, E.; Valenti, G. Mathematical modeling and numerical simulation of domain wall motion in magnetic nanostrips with crystallographic defects. Appl. Mathem. Modell. 2012, 36, 4876–4886. [Google Scholar] [CrossRef]
- Consolo, G.; Valenti, G. Traveling wave solutions of the one-dimensional extended Landau-Lifshitz-Gilbert equation with nonlinear dry and viscous dissipations. Acta Appl. Mathem. 2012, 122, 141–152. [Google Scholar] [CrossRef]
- Landau, L.D.; Lifshitz, E.M. On the theory of the dispersion of magnetic permeability in ferromagnetic bodies. Phys. Zeits. Sowjetun. 1935, 8, 153–169. [Google Scholar]
- Gilbert, T.L. A lagrangian formulation of the gyromagnetic equation of the magnetization field. Phys. Rev. 1955, 100, 1243. [Google Scholar]
- Tiberkevich, V.; Slavin, A. Nonlinear phenomenological model of magnetic dissipation for large precession angles: Generalization of the gilbert model. Phys. Rev. B 2007, 75, 014440. [Google Scholar] [CrossRef]
- Shu, Y.C.; Lin, M.P.; Wu, K.C. Micromagnetic modeling of magnetostrictive materials under intrinsic stress. Mech. Mater. 2004, 36, 975–997. [Google Scholar] [CrossRef]
- Baňas, L. A numerical method for the Landau–Lifshitz equation with magnetostriction. Math. Meth. Appl. Sci. 2005, 28, 1939–1954. [Google Scholar] [CrossRef]
- Liang, C.Y.; Keller, S.M.; Sepulveda, A.E.; Bur, A.; Sun, W.Y.; Wetzlar, K.; Carman, G.P. Modeling of magnetoelastic nanostructures with a fully coupled mechanical-micromagnetic model. Nanotechnology 2014, 25, 435701. [Google Scholar] [CrossRef]
- Mougin, A.; Cormier, M.; Adam, J.P.; Metaxas, P.J.; Ferre’, J. Domain wall mobility, stability and Walker breakdown in magnetic nanowires. Eur. Phys. Lett. 2007, 78, 57007. [Google Scholar] [CrossRef]
- Federico, S.; Consolo, G.; Valenti, G. Tensor representation of magnetostriction for all crystal classes. Math. Mech. Solids 2019, 24, 2814–2843. [Google Scholar] [CrossRef]
- Zhang, J.X.; Chen, L.Q. Phase-field microelasticity theory and micromagnetic simulations of domain structures in giant magnetostrictive materials. Acta Mater. 2005, 53, 2845–2855. [Google Scholar] [CrossRef]
- Mballa-Mballa, F.S.; Hubert, O.; He, S.; Depeyre, S.; Meilland, P. Micromagnetic modeling of magneto-mechanical behavior. IEEE Trans. Magn. 2014, 50, 1–4. [Google Scholar] [CrossRef]
- Yang, J. An Introduction to the Theory of Piezoelectricity; Springer: Boston, MA, USA, 2006. [Google Scholar]
- Baltensperger, W.; Helman, J.S. A model that gives rise to effective dry friction in micromagnetics. J. Appl. Phys. 1993, 73, 6516–6518. [Google Scholar] [CrossRef]
- Min, H.; McMichael, R.D.; Donahue, M.J.; Miltat, J.; Stiles, M.D. Effects of disorder and internal dynamics on vortex wall propagation. Phys. Rev. Lett. 2010, 104, 217201. [Google Scholar] [CrossRef]
- Metaxas, P.J.; Jamet, J.P.; Mougin, A.; Cormier, M.; Ferré, J.; Baltz, V.; Rodmacq, B.; Dieny, B.; Stamps, R.L. Creep and flow regimes of magnetic domain-wall motion in ultrathin Pt/Co/Pt films with perpendicular anisotropy. Phys. Rev. Lett. 2007, 99, 217208. [Google Scholar] [CrossRef]
- Gopman, D.B.; Sampath, V.; Ahmad, H.; Bandyopadhyay, S.; Atulasimha, J. Static and dynamic magnetic properties of sputtered Fe-Ga thin films. IEEE Trans. Magn. 2017, 53, 1–4. [Google Scholar] [CrossRef]
- Clark, A.E.; Hathaway, K.B.; Wun-Fogle, M.; Restorff, J.B.; Lograsso, T.A.; Keppens, V.M.; Petculescu, G.; Taylor, R.A. Extraordinary magnetoelasticity and lattice softening in bcc fe-ga alloys. J. Appl. Phys. 2003, 93, 8621–8623. [Google Scholar] [CrossRef]
- Wuttig, M.; Dai, L.; Cullen, J.R. Elasticity and magnetoelasticity of Fe-Ga solid solutions. Appl. Phys. Lett. 2002, 80, 1135–1137. [Google Scholar] [CrossRef]
- Rafique, S.; Cullen, J.R.; Wuttig, M.; Cui, J. Magnetic anisotropy of FeGa alloys. J. Appl. Phys. 2004, 95, 6939–6941. [Google Scholar] [CrossRef]
- Esteves, G.; Fanchera, C.M.; Röhrig, S.; Maierc, G.A.; Jones, J.L.; Deluca, M. Electric-field-induced structural changes in multilayer piezoelectric actuators during electrical and mechanical loading. Acta Mater. 2017, 132, 96–105. [Google Scholar] [CrossRef]
- Bruno, B.P.; Fahmy, A.R.; Stürmer, M.; Wallrabe, U.; Wapler, M.C. Properties of piezoceramic materials in high electric field actuator applications. Smart Mater. Struct. 2019, 28, 015029. [Google Scholar] [CrossRef]
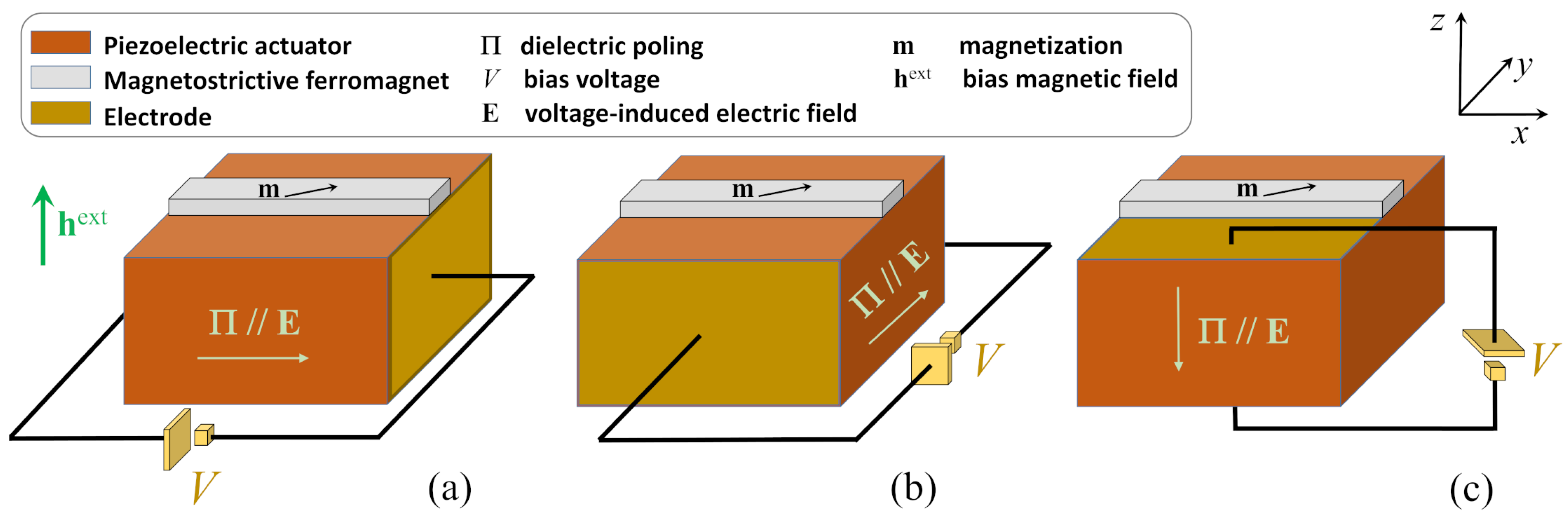

| Quantity | Unit | Value | Ref. |
|---|---|---|---|
| T | 1.5 | [37] | |
| pJ/m | 14 | [37] | |
| – | 0.04 | [37] | |
| – | [38,39] | ||
| – | [38,39] | ||
| GPa | 200 | [38,39] | |
| GPa | 160 | [38,39] | |
| GPa | 130 | [38,39] | |
| [40] | |||
| – | 0.6424 | assumed | |
| – | 0.0092 | assumed | |
| – | 0.3484 | assumed | |
| deg | 10 | assumed | |
| – | 0.1 | assumed |
| Material | d1 | d2 |
|---|---|---|
| PZT4 | +2.89 | −1.23 |
| PZT5A | +3.74 | −1.71 |
| PZT5H | +5.92 | −2.74 |
| PZT6B | +0.72 | −0.27 |
| PZT7A | +1.53 | −0.65 |
| PZT8 | +2.15 | −0.93 |
| BaTiO3 | +1.91 | −0.79 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Consolo, G.; Valenti, G. Optimized Voltage-Induced Control of Magnetic Domain-Wall Propagation in Hybrid Piezoelectric/Magnetostrictive Devices. Actuators 2021, 10, 134. https://doi.org/10.3390/act10060134
Consolo G, Valenti G. Optimized Voltage-Induced Control of Magnetic Domain-Wall Propagation in Hybrid Piezoelectric/Magnetostrictive Devices. Actuators. 2021; 10(6):134. https://doi.org/10.3390/act10060134
Chicago/Turabian StyleConsolo, Giancarlo, and Giovanna Valenti. 2021. "Optimized Voltage-Induced Control of Magnetic Domain-Wall Propagation in Hybrid Piezoelectric/Magnetostrictive Devices" Actuators 10, no. 6: 134. https://doi.org/10.3390/act10060134
APA StyleConsolo, G., & Valenti, G. (2021). Optimized Voltage-Induced Control of Magnetic Domain-Wall Propagation in Hybrid Piezoelectric/Magnetostrictive Devices. Actuators, 10(6), 134. https://doi.org/10.3390/act10060134







