Figure 1.
(a) Untrained twisted coiled actuator (TCA). (b) Trained TCA.
Figure 1.
(a) Untrained twisted coiled actuator (TCA). (b) Trained TCA.
Figure 2.
Thermocouples after having been attached to the TCAs, with thermal paste applied.
Figure 2.
Thermocouples after having been attached to the TCAs, with thermal paste applied.
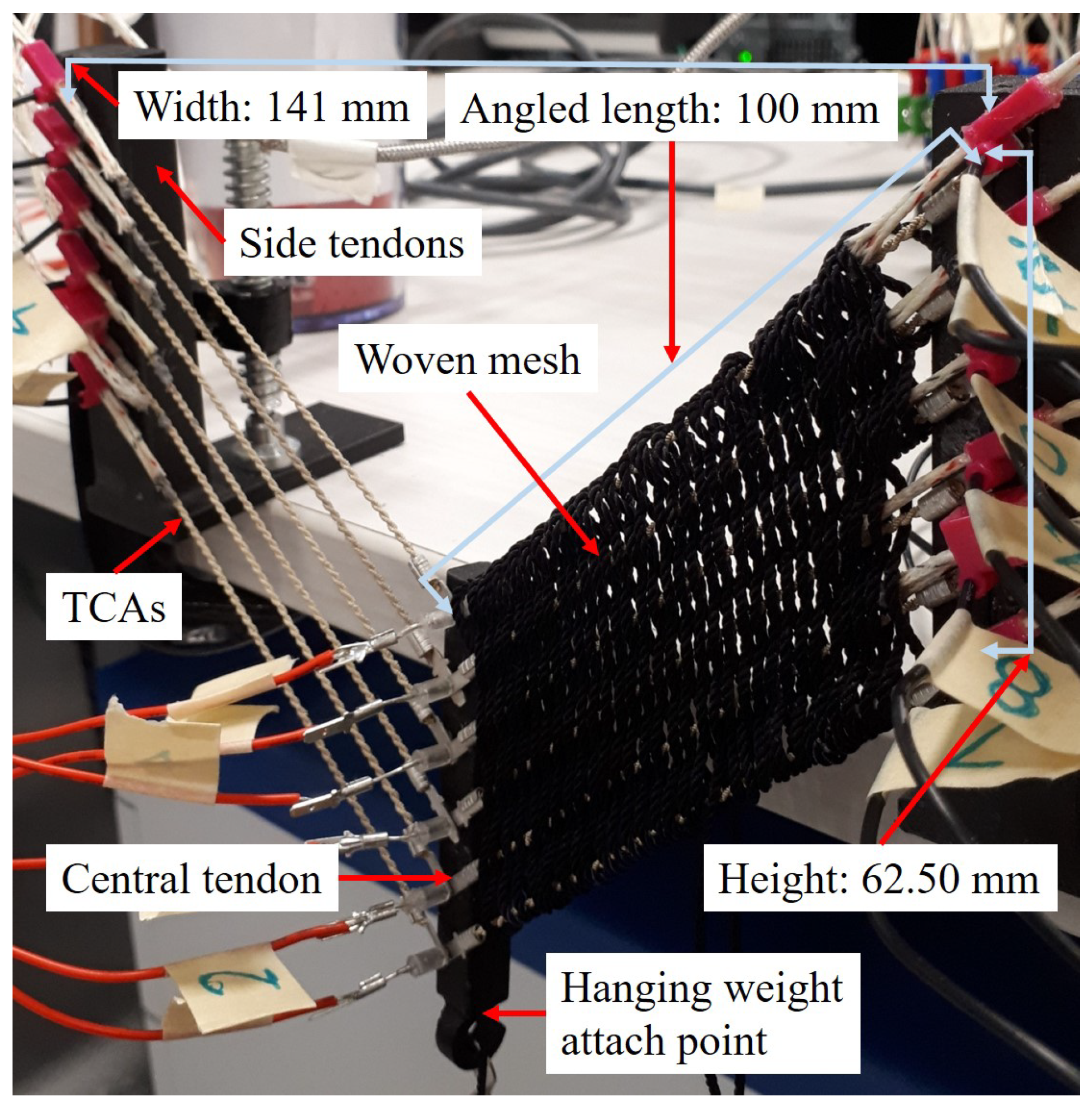
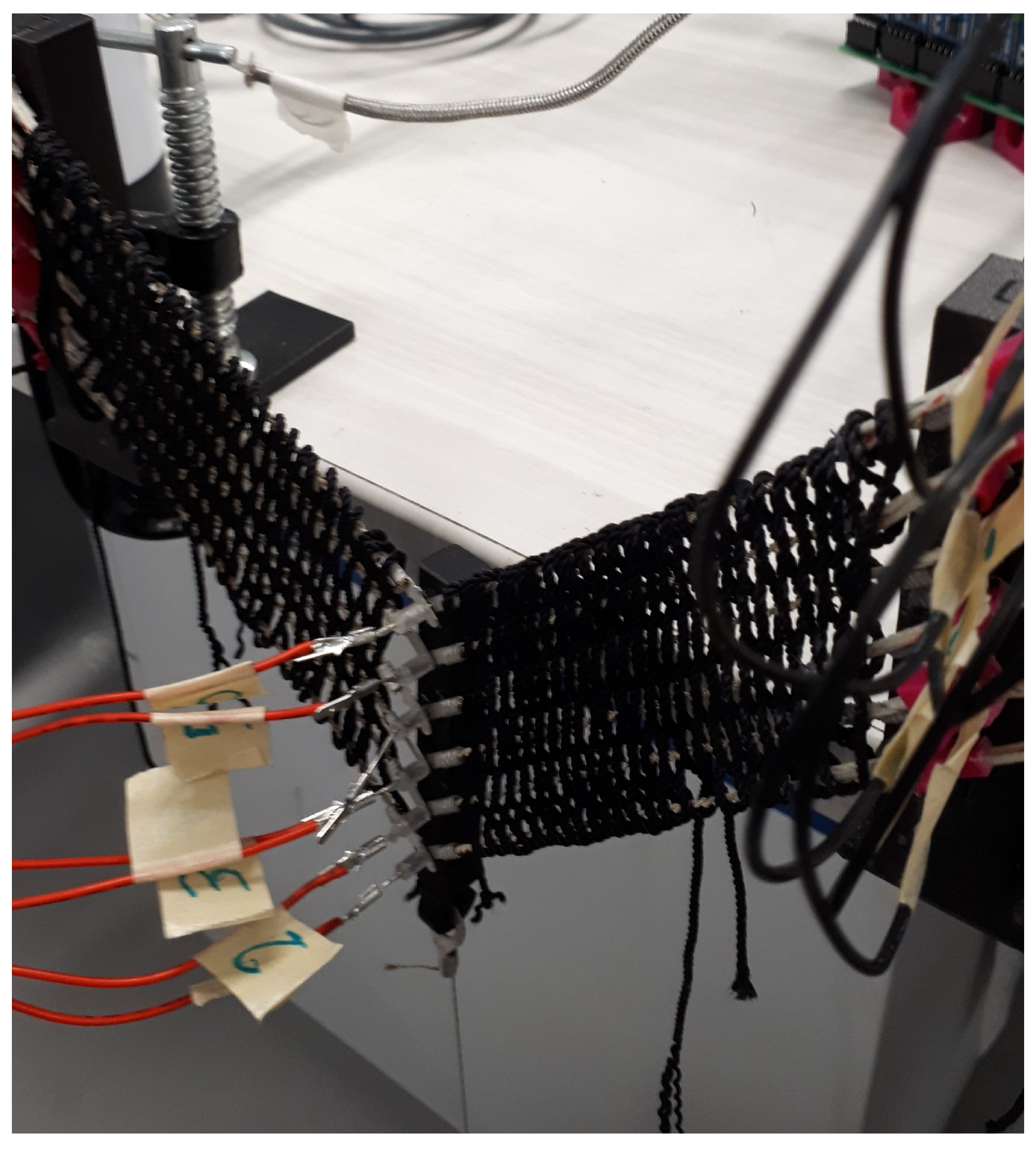
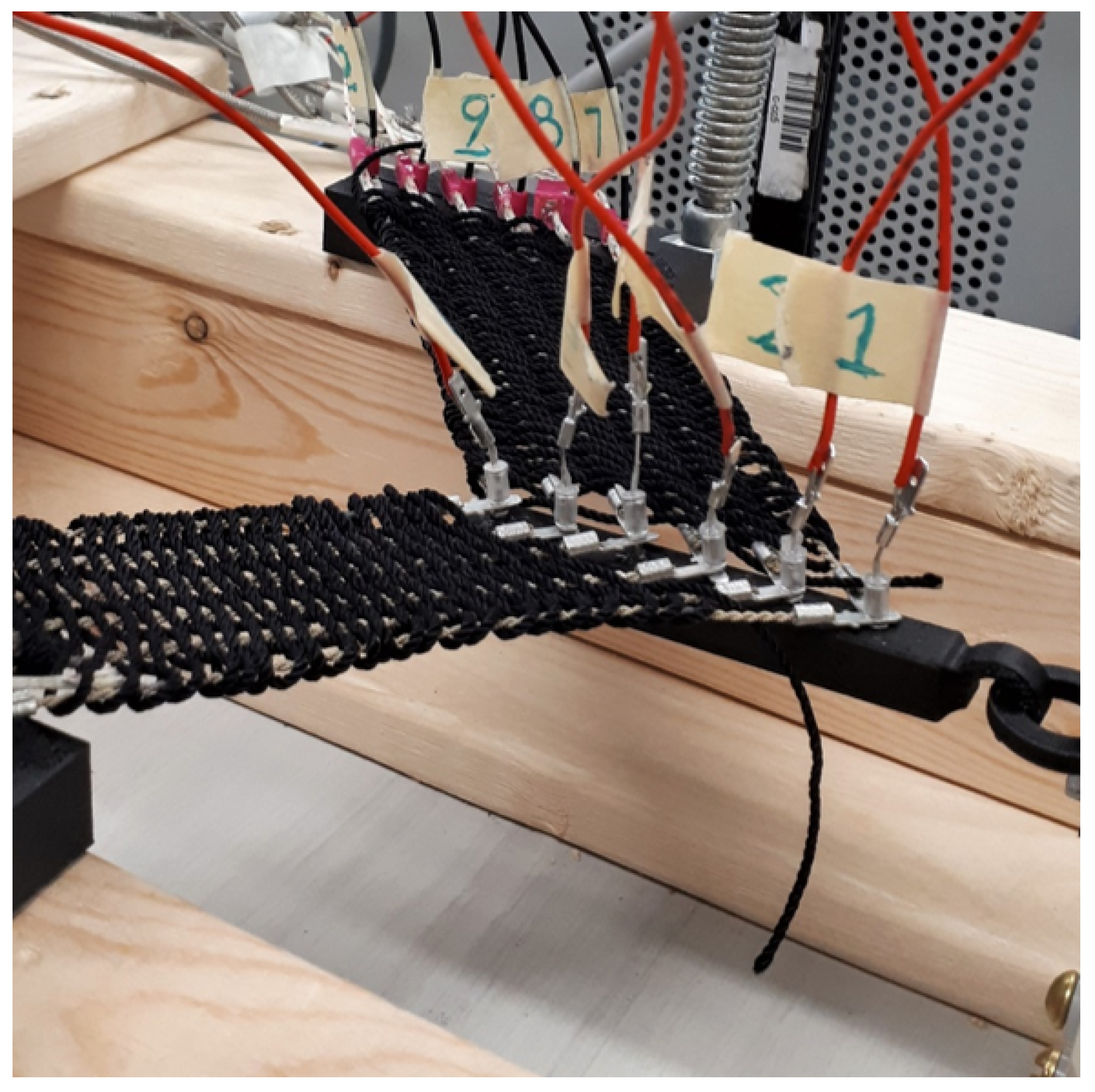
Figure 3.
Actuator in the process of being woven. The right side has been completely woven, while the left has yet to be woven.
Figure 3.
Actuator in the process of being woven. The right side has been completely woven, while the left has yet to be woven.
Figure 4.
Actuator configuration for the stroke characterisation experiment.
Figure 4.
Actuator configuration for the stroke characterisation experiment.
Figure 5.
Actuator configuration for the force characterisation experiment.
Figure 5.
Actuator configuration for the force characterisation experiment.
Figure 6.
Actuator configuration for the contraction period characterisation experiments.
Figure 6.
Actuator configuration for the contraction period characterisation experiments.
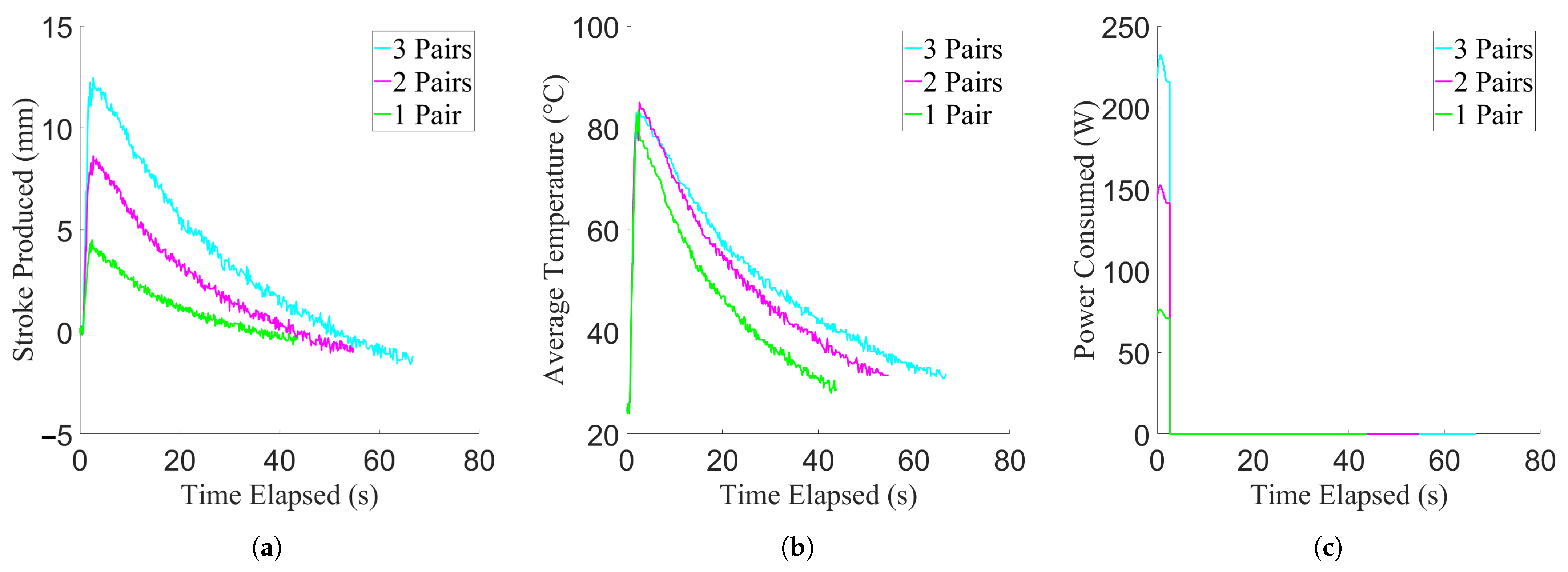
Figure 7.
Results of the stroke characterisation experiment, showing data from example trials. For the purpose of clarity, example curves were used, due to low variance between trials. (a) Stroke production of the actuator. (b) Temperature achieved by the actuator. (c) Power consumed by the actuator.
Figure 7.
Results of the stroke characterisation experiment, showing data from example trials. For the purpose of clarity, example curves were used, due to low variance between trials. (a) Stroke production of the actuator. (b) Temperature achieved by the actuator. (c) Power consumed by the actuator.
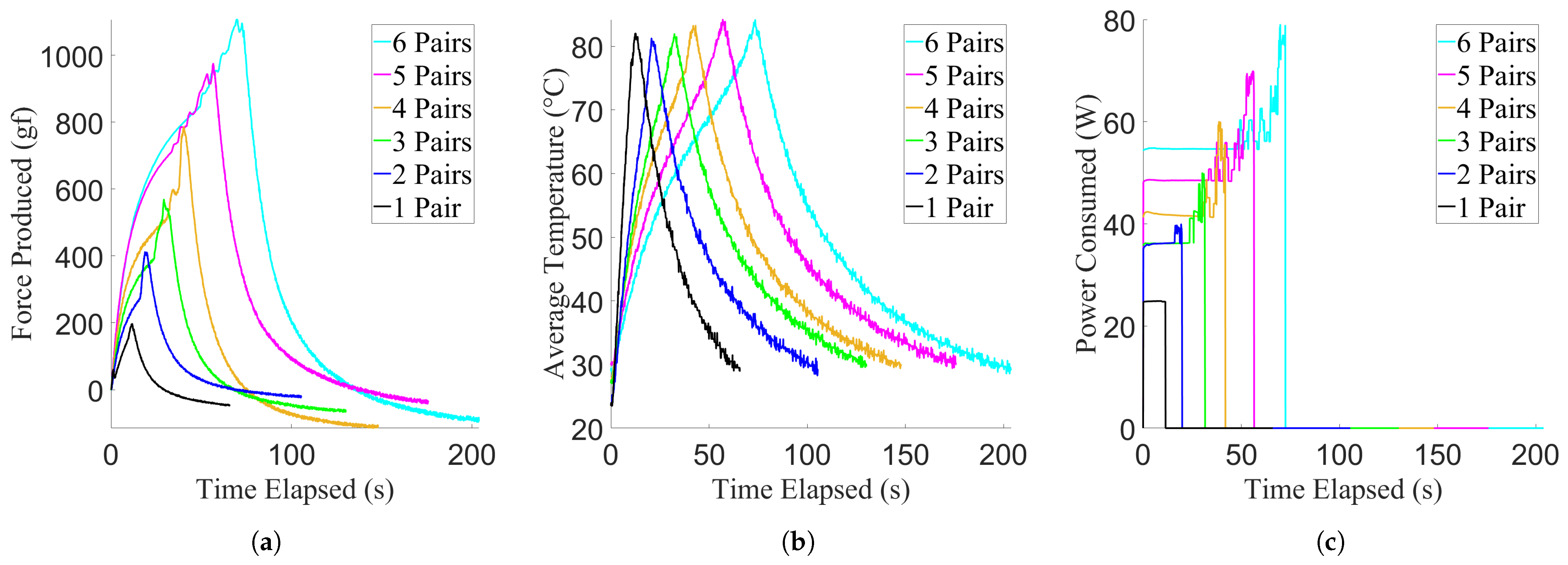
Figure 8.
Results of the force characterisation experiment, showing data from example trials. For the purpose of clarity, example curves were used due to low variance between trials. (a) Force production of the actuator. (b) Temperature achieved by the actuator. (c) Power consumed by the actuator.
Figure 8.
Results of the force characterisation experiment, showing data from example trials. For the purpose of clarity, example curves were used due to low variance between trials. (a) Force production of the actuator. (b) Temperature achieved by the actuator. (c) Power consumed by the actuator.
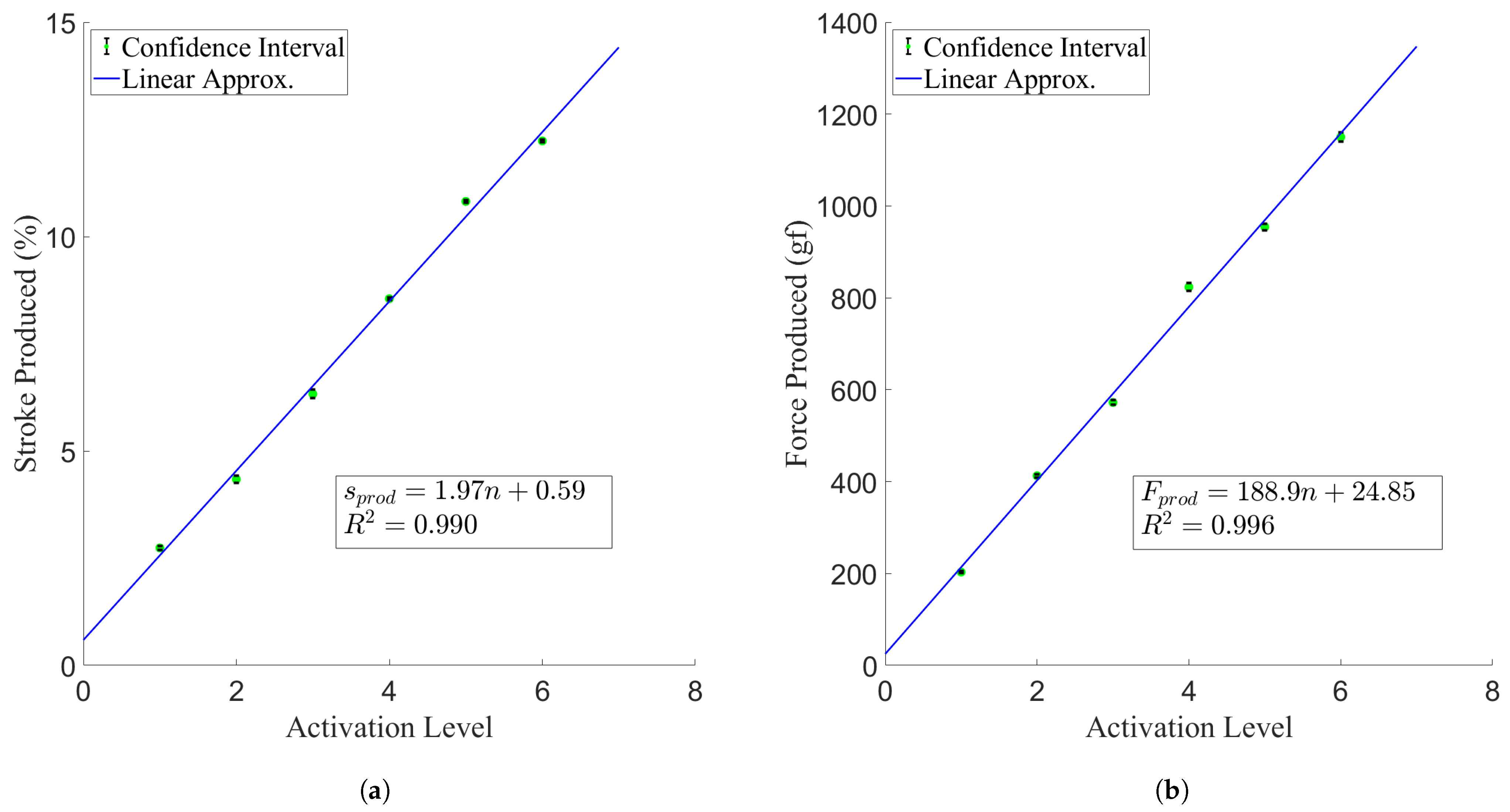
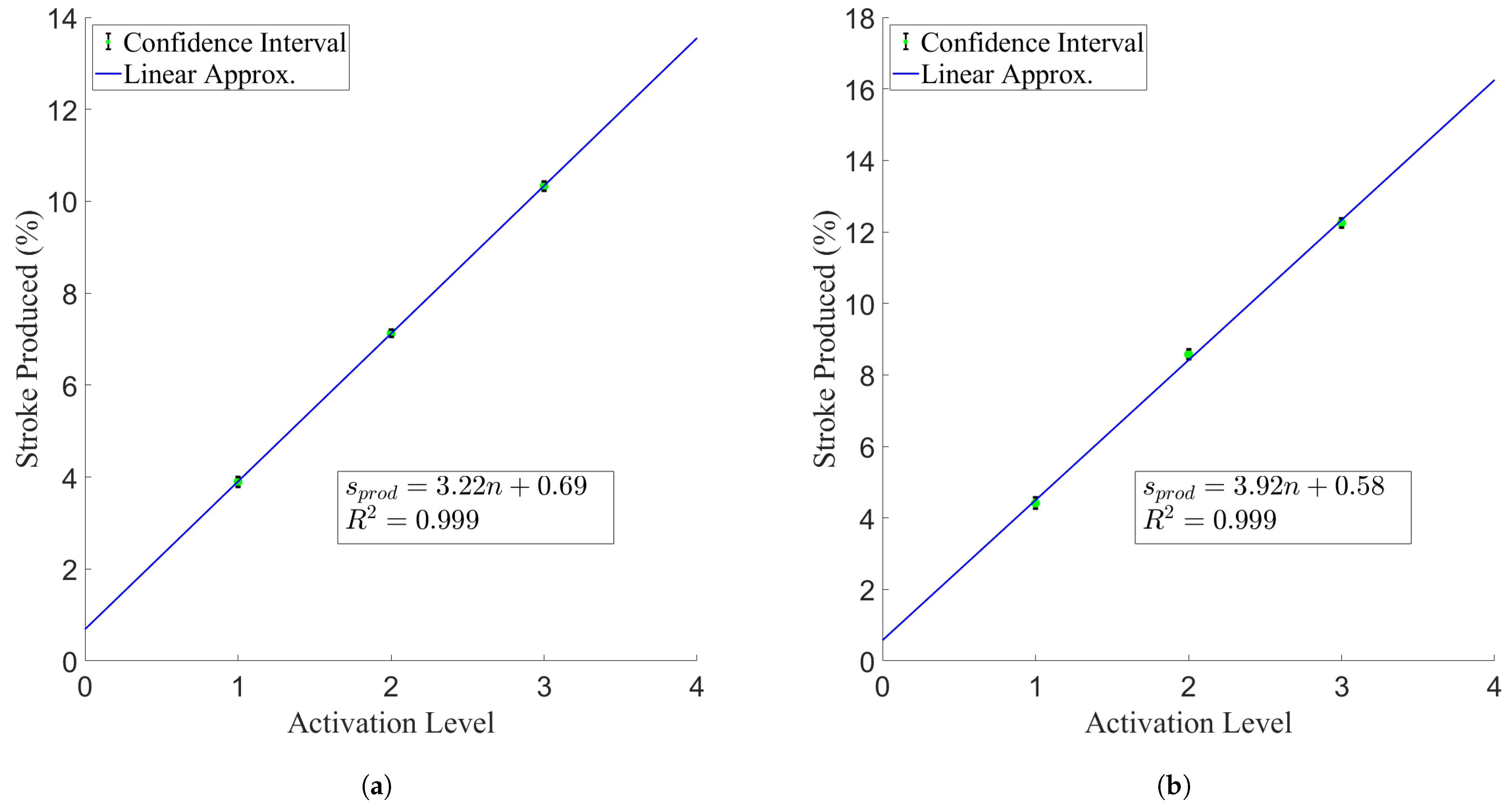
Figure 9.
Maximum actuator production per active pairs of TCAs, with overlaid linear regressions. The variable n denotes the number of activated pairs of TCAs. (a) Stroke production results. (b) Force production results.
Figure 9.
Maximum actuator production per active pairs of TCAs, with overlaid linear regressions. The variable n denotes the number of activated pairs of TCAs. (a) Stroke production results. (b) Force production results.
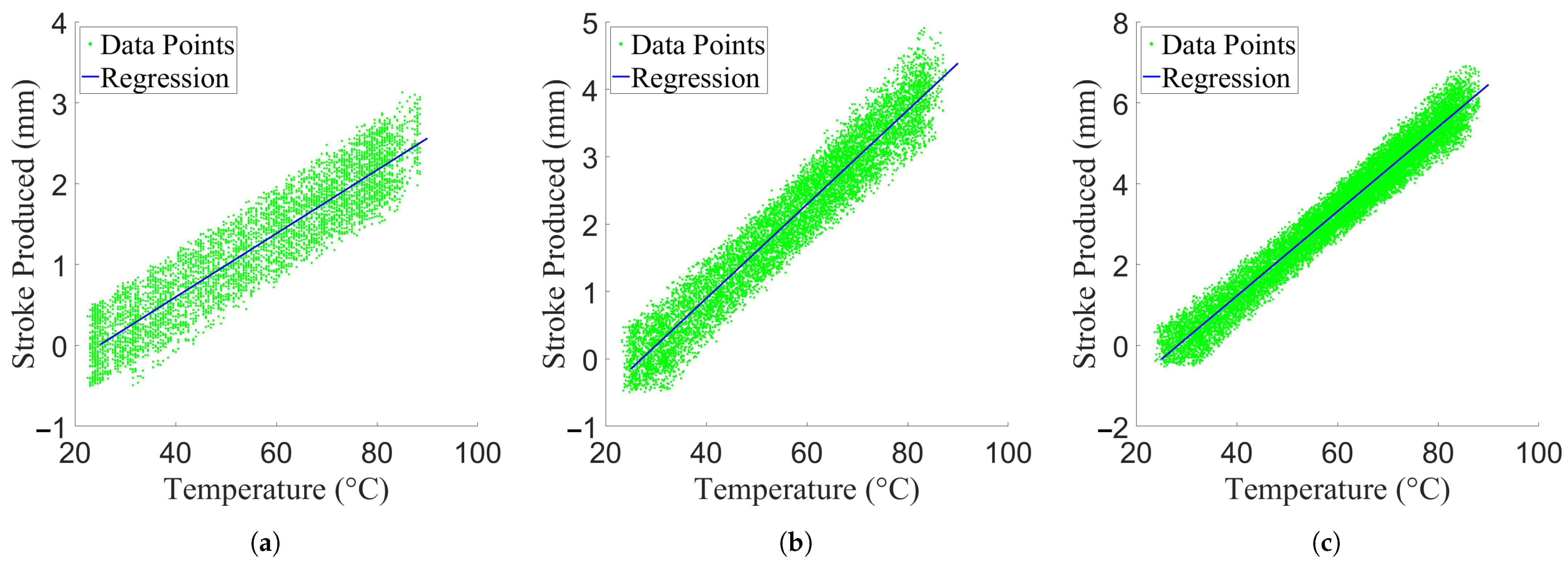
Figure 10.
Regressed temperature–stroke relationships overlaid over experimental data, separated by activation level. (a) One activated pair. (b) Two activated pairs. (c) Three activated pairs.
Figure 10.
Regressed temperature–stroke relationships overlaid over experimental data, separated by activation level. (a) One activated pair. (b) Two activated pairs. (c) Three activated pairs.
Figure 11.
Regressed temperature–force relationships overlaid over experimental data, separated by activation level. (a) One activated pair. (b) Two activated pairs. (c) Three activated pairs.
Figure 11.
Regressed temperature–force relationships overlaid over experimental data, separated by activation level. (a) One activated pair. (b) Two activated pairs. (c) Three activated pairs.
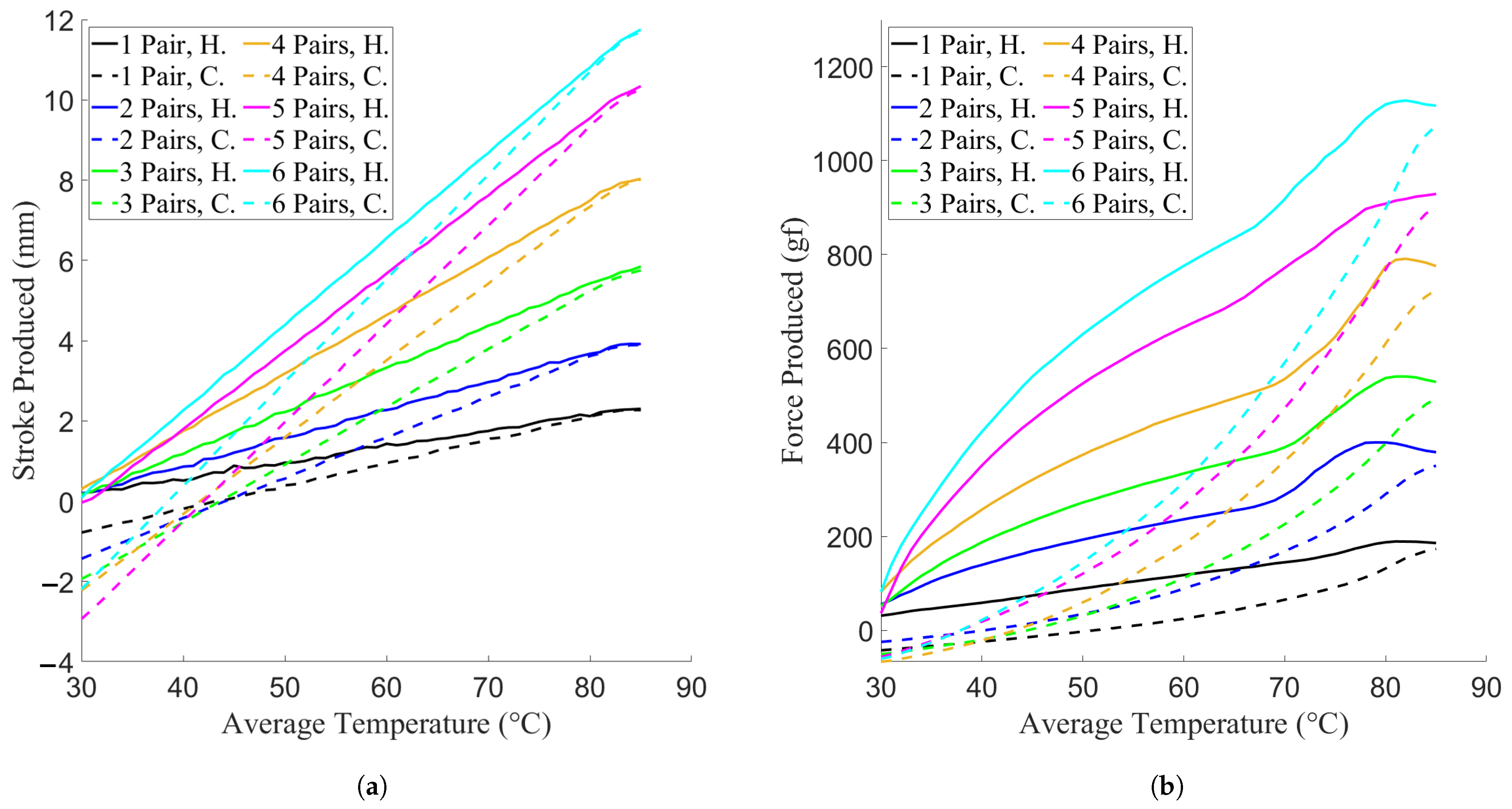
Figure 12.
Average hysteresis at each activation level during the characterisation experiments. The abbreviation “H.” denotes the production of the actuator while it is heating, and the abbreviation “C.” denotes the production of the actuator while it is cooling. (a) Stroke production hysteresis curves. (b) Force production hysteresis curves.
Figure 12.
Average hysteresis at each activation level during the characterisation experiments. The abbreviation “H.” denotes the production of the actuator while it is heating, and the abbreviation “C.” denotes the production of the actuator while it is cooling. (a) Stroke production hysteresis curves. (b) Force production hysteresis curves.
Figure 13.
Results of the constant contraction period experiment, showing data from example trials. For the purpose of clarity, example curves were used, due to low variance between trials. (a) Stroke production of the actuator. (b) Temperature achieved by the actuator. (c) Power consumed by the actuator.
Figure 13.
Results of the constant contraction period experiment, showing data from example trials. For the purpose of clarity, example curves were used, due to low variance between trials. (a) Stroke production of the actuator. (b) Temperature achieved by the actuator. (c) Power consumed by the actuator.
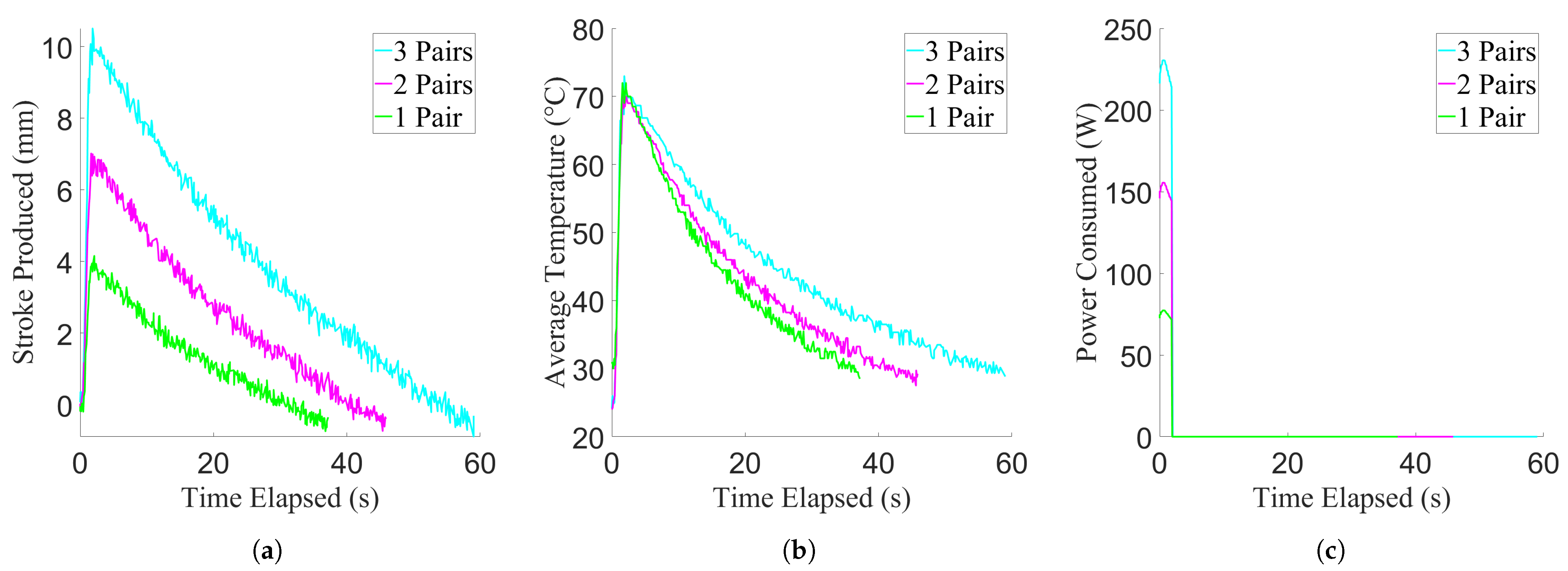
Figure 14.
Results of the minimum contraction period experiment, showing data from example trials. For the purpose of clarity, example curves were used due to low variance between trials. (a) Stroke production of the actuator. (b) Temperature achieved by the actuator. (c) Power consumed by the actuator.
Figure 14.
Results of the minimum contraction period experiment, showing data from example trials. For the purpose of clarity, example curves were used due to low variance between trials. (a) Stroke production of the actuator. (b) Temperature achieved by the actuator. (c) Power consumed by the actuator.
Figure 15.
Maximum stroke production per active pairs of TCAs, with overlaid linear regressions. The variable n denotes number of active pairs of TCAs. (a) Constant contraction period experiment. (b) Minimum contraction period experiment.
Figure 15.
Maximum stroke production per active pairs of TCAs, with overlaid linear regressions. The variable n denotes number of active pairs of TCAs. (a) Constant contraction period experiment. (b) Minimum contraction period experiment.
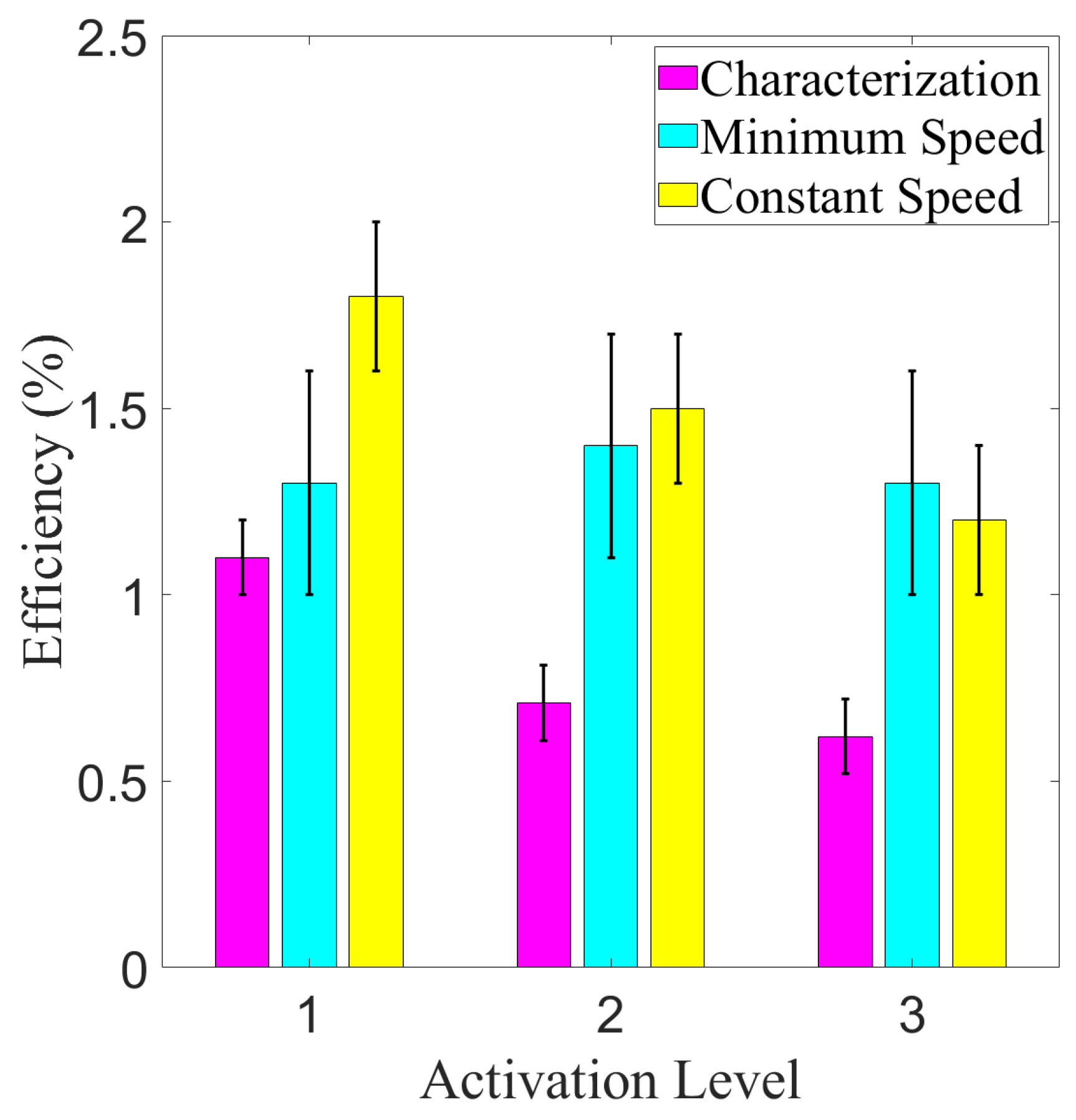
Figure 16.
Efficiency of the actuator during isotonic contractions compared to activation level, for the stroke characterisation experiment, minimum contraction period experiment, and constant contraction period experiment. Significance in differences not displayed due to inapplicability.
Figure 16.
Efficiency of the actuator during isotonic contractions compared to activation level, for the stroke characterisation experiment, minimum contraction period experiment, and constant contraction period experiment. Significance in differences not displayed due to inapplicability.
Figure 17.
Layout of the control flow for the PID controller.
Figure 17.
Layout of the control flow for the PID controller.
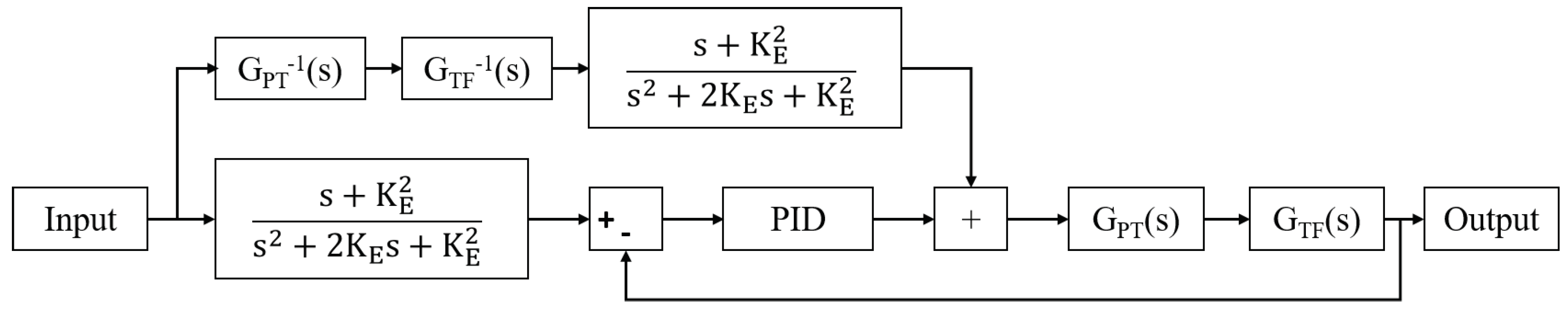
Figure 18.
Layout of the control flow for the 2 DOF controller. The values of , , and were taken from the tuned PID controllers for each activation level.
Figure 18.
Layout of the control flow for the 2 DOF controller. The values of , , and were taken from the tuned PID controllers for each activation level.
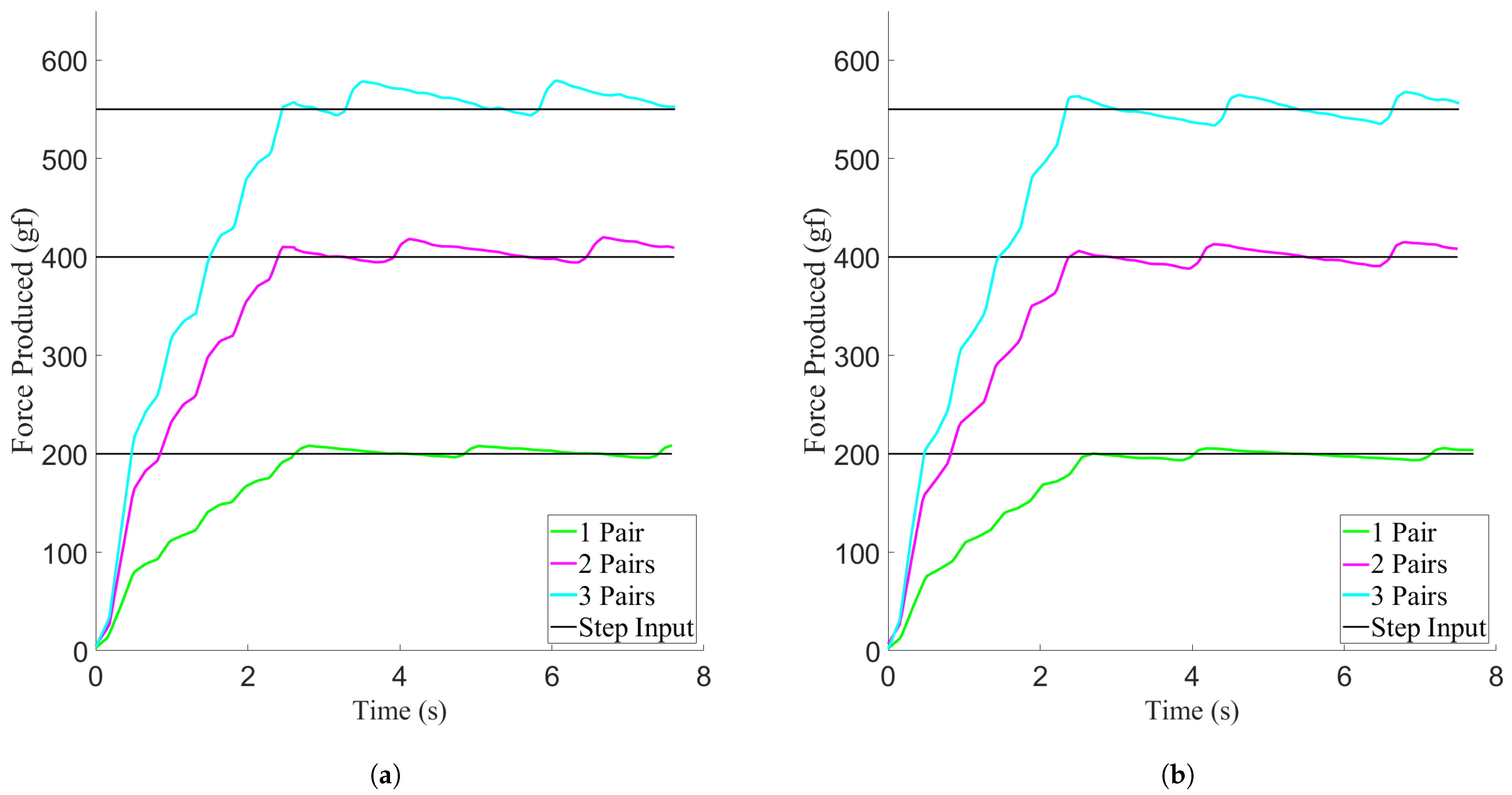
Figure 19.
Force responses of the force control implementation experiments, showing data from example trials. For the purpose of clarity, example curves were used due to low variance between trials. (a) PID controller force response. (b) 2 DOF controller force response.
Figure 19.
Force responses of the force control implementation experiments, showing data from example trials. For the purpose of clarity, example curves were used due to low variance between trials. (a) PID controller force response. (b) 2 DOF controller force response.
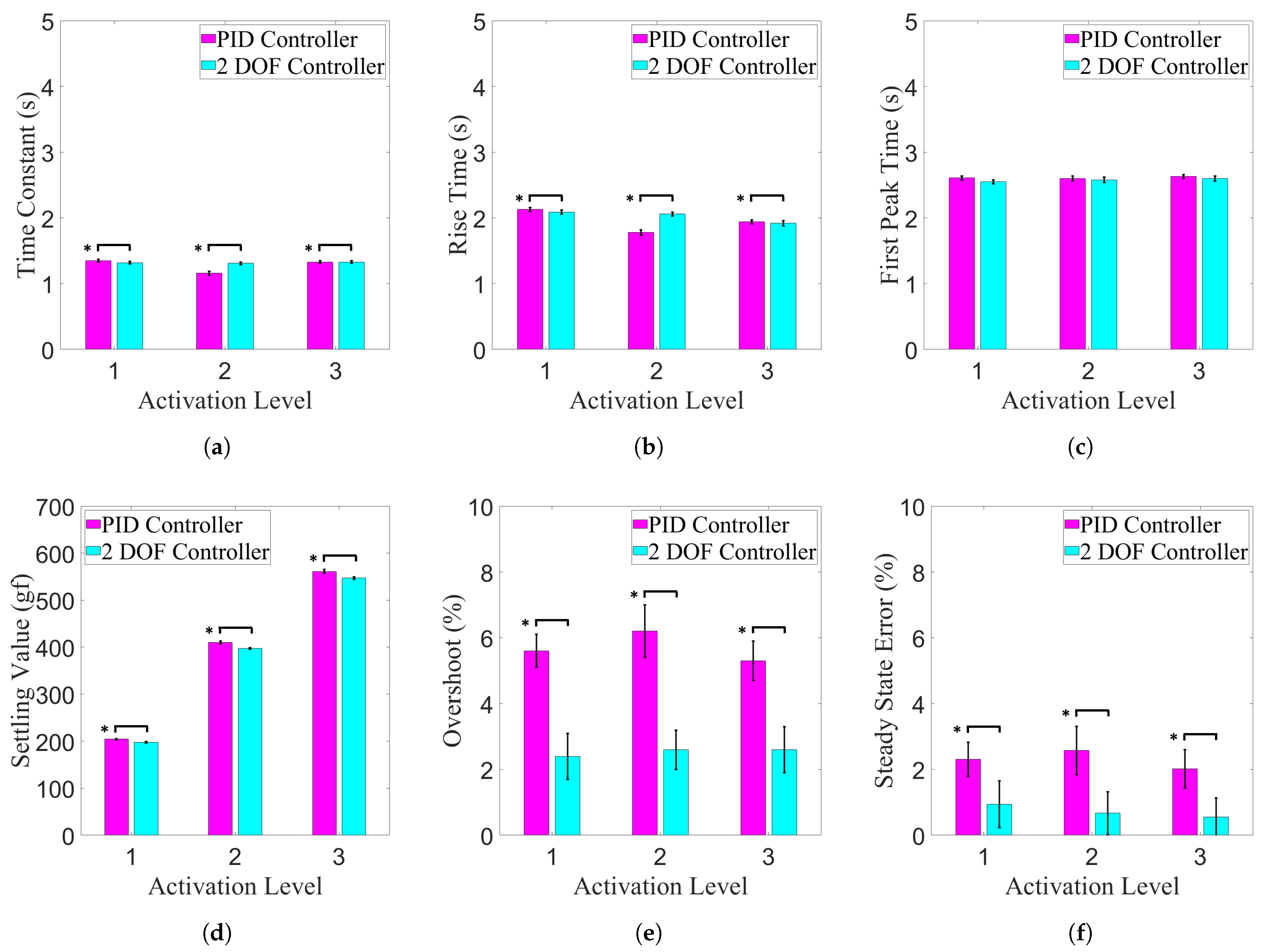
Figure 20.
Calculated control performance values for the PID and 2 DOF controller implementation experiments, compared to activation level. A ∗ denotes a grouping with a significant difference. All p values < 0.01. (a) Time constant values. (b) Rise time values. (c) First peak time values. (d) Settling values. (e) Percent overshoot values. (f) Percent steady state error values.
Figure 20.
Calculated control performance values for the PID and 2 DOF controller implementation experiments, compared to activation level. A ∗ denotes a grouping with a significant difference. All p values < 0.01. (a) Time constant values. (b) Rise time values. (c) First peak time values. (d) Settling values. (e) Percent overshoot values. (f) Percent steady state error values.
Table 1.
Regressed temperature–stroke relationships, as described in Equation (
2), by activation level.
Table 1.
Regressed temperature–stroke relationships, as described in Equation (
2), by activation level.
| Activation Level | | |
|---|
| 1 | 0.039 | −0.97 |
| 2 | 0.067 | −1.89 |
| 3 | 0.104 | −2.95 |
Table 2.
Regressed temperature–force relationships, as described in Equation (
3), by activation level.
Table 2.
Regressed temperature–force relationships, as described in Equation (
3), by activation level.
| Activation Level | | | | | | |
|---|
| 1 | −9.11 | 2.39 | −0.024 | 1.21 | −26.96 | 245.17 |
| 2 | −9.43 | 2.51 | −0.26 | 12.29 | −272.94 | 2266.01 |
| 3 | −1.19 | 3.29 | −0.35 | 17.60 | −410.85 | 3584.51 |
Table 3.
Calculated peak and RMS hysteresis per activation level for the stroke characterisation experiment.
Table 3.
Calculated peak and RMS hysteresis per activation level for the stroke characterisation experiment.
| Activation Level | Peak Hysteresis Offset (mm) | RMS Hysteresis Offset (mm) |
|---|
| 1 | 0.98 | 0.54 |
| 2 | 1.62 | 0.91 |
| 3 | 2.10 | 1.21 |
| 4 | 2.53 | 1.45 |
| 5 | 2.90 | 1.63 |
| 6 | 2.29 | 1.31 |
Table 4.
Calculated peak and RMS hysteresis per activation level for the force characterisation experiment.
Table 4.
Calculated peak and RMS hysteresis per activation level for the force characterisation experiment.
| Activation Level | Peak Hysteresis Offset (gf) | RMS Hysteresis Offset (gf) |
|---|
| 1 | 94.36 | 80.38 |
| 2 | 159.06 | 133.27 |
| 3 | 240.94 | 190.36 |
| 4 | 314.30 | 239.17 |
| 5 | 407.22 | 310.43 |
| 6 | 485.84 | 376.26 |
Table 5.
Average stroke and contraction period by activation level in the minimum contraction period experiment.
Table 5.
Average stroke and contraction period by activation level in the minimum contraction period experiment.
| Activation Level | Mean Stroke Production (mm) | Mean Contraction Period (s) |
|---|
| 1 | 4.42 | 2.43 |
| 2 | 8.57 | 2.43 |
| 3 | 12.25 | 2.36 |
Table 6.
Stroke and contraction period comparisons between the constant and minimum contraction period experiments, by activation level. All values expressed as percentages.
Table 6.
Stroke and contraction period comparisons between the constant and minimum contraction period experiments, by activation level. All values expressed as percentages.
| Activation Level | Mean Stroke Comparison | Mean Contraction Period Comparison |
|---|
| 1 | 0.90 | 0.82 |
| 2 | 0.84 | 0.82 |
| 3 | 0.84 | 0.85 |
Table 7.
Thermal resistances and time constants of the system for each activation level, calculated from experimental data. With each additional pair of TCAs, the thermal resistance decreased due the additional surface area, which increased the active portion of the actuator’s heat loss capabilities.
Table 7.
Thermal resistances and time constants of the system for each activation level, calculated from experimental data. With each additional pair of TCAs, the thermal resistance decreased due the additional surface area, which increased the active portion of the actuator’s heat loss capabilities.
| Activation Level | Thermal Resistance (°C/W) | Time Constant (s) |
|---|
| 1 | 2.26 | 7.48 |
| 2 | 1.47 | 13.14 |
| 3 | 1.26 | 17.07 |
| 4 | 1.15 | 22.32 |
| 5 | 0.89 | 31.25 |
| 6 | 0.82 | 38.00 |
Table 8.
Frequency domain transfer functions for the power–temperature relationships and the temperature–force relationships for each activation level of the actuator.
Table 8.
Frequency domain transfer functions for the power–temperature relationships and the temperature–force relationships for each activation level of the actuator.
| Activation Level | | |
|---|
| 1 | | |
| 2 | | |
| 3 | | |
Table 9.
Force setpoints used for controller tuning and testing purposes, based on the results of the force characterisation experiment, available in
Figure 8.
Table 9.
Force setpoints used for controller tuning and testing purposes, based on the results of the force characterisation experiment, available in
Figure 8.
| Activation Level | Force Setpoint (g Force Equivalent) |
|---|
| 1 | 200 |
| 2 | 400 |
| 3 | 550 |
Table 10.
Tuned PID coefficients.
Table 10.
Tuned PID coefficients.
| Activation Level | | | |
|---|
| 1 | 30.0 | 0.05 | 1.50 |
| 2 | 3.5 | 0.10 | 0.90 |
| 3 | 4.0 | 0.10 | 0.06 |
Table 11.
Tuned 2 DOF controller coefficients.
Table 11.
Tuned 2 DOF controller coefficients.
| Activation Level | |
|---|
| 1 | 2.0 |
| 2 | 3.5 |
| 3 | 6.0 |