Figure 1.
Photo of bucket arc and connection of upper and lower column.
Figure 1.
Photo of bucket arc and connection of upper and lower column.
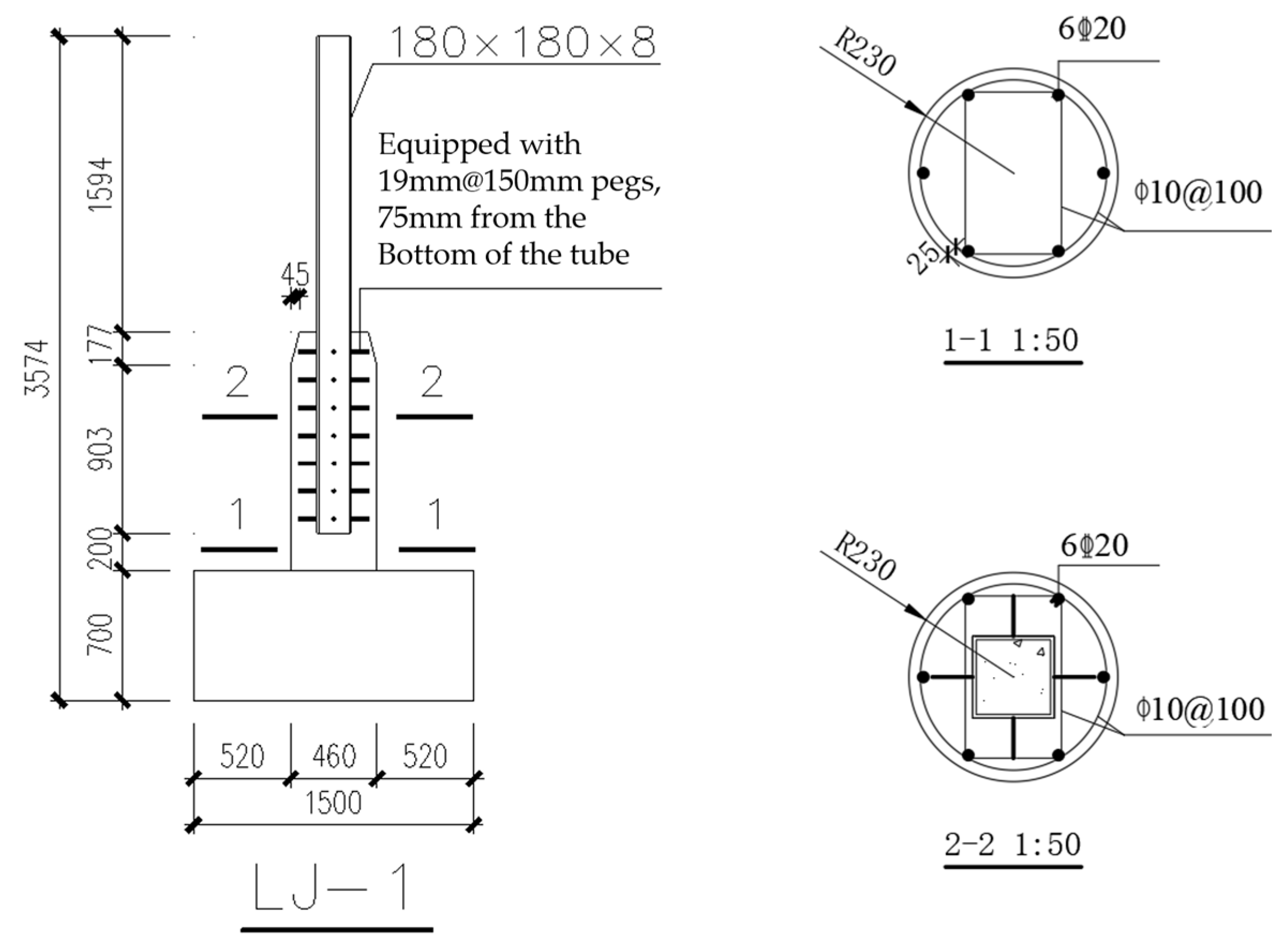
Figure 2.
Dimensions and reinforcement of LJ-1 specimen.
Figure 2.
Dimensions and reinforcement of LJ-1 specimen.
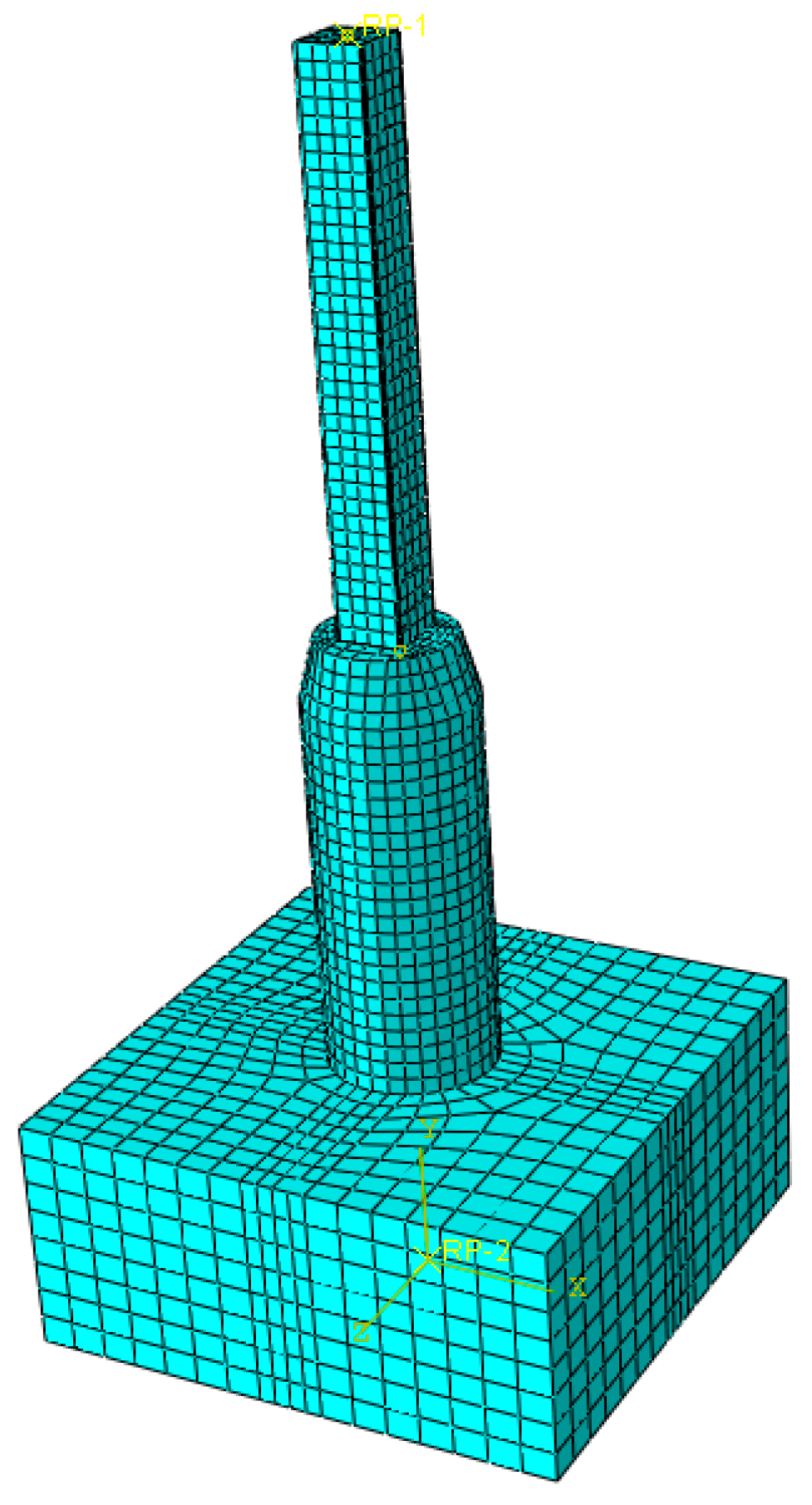
Figure 3.
Overall model and mesh division.
Figure 3.
Overall model and mesh division.
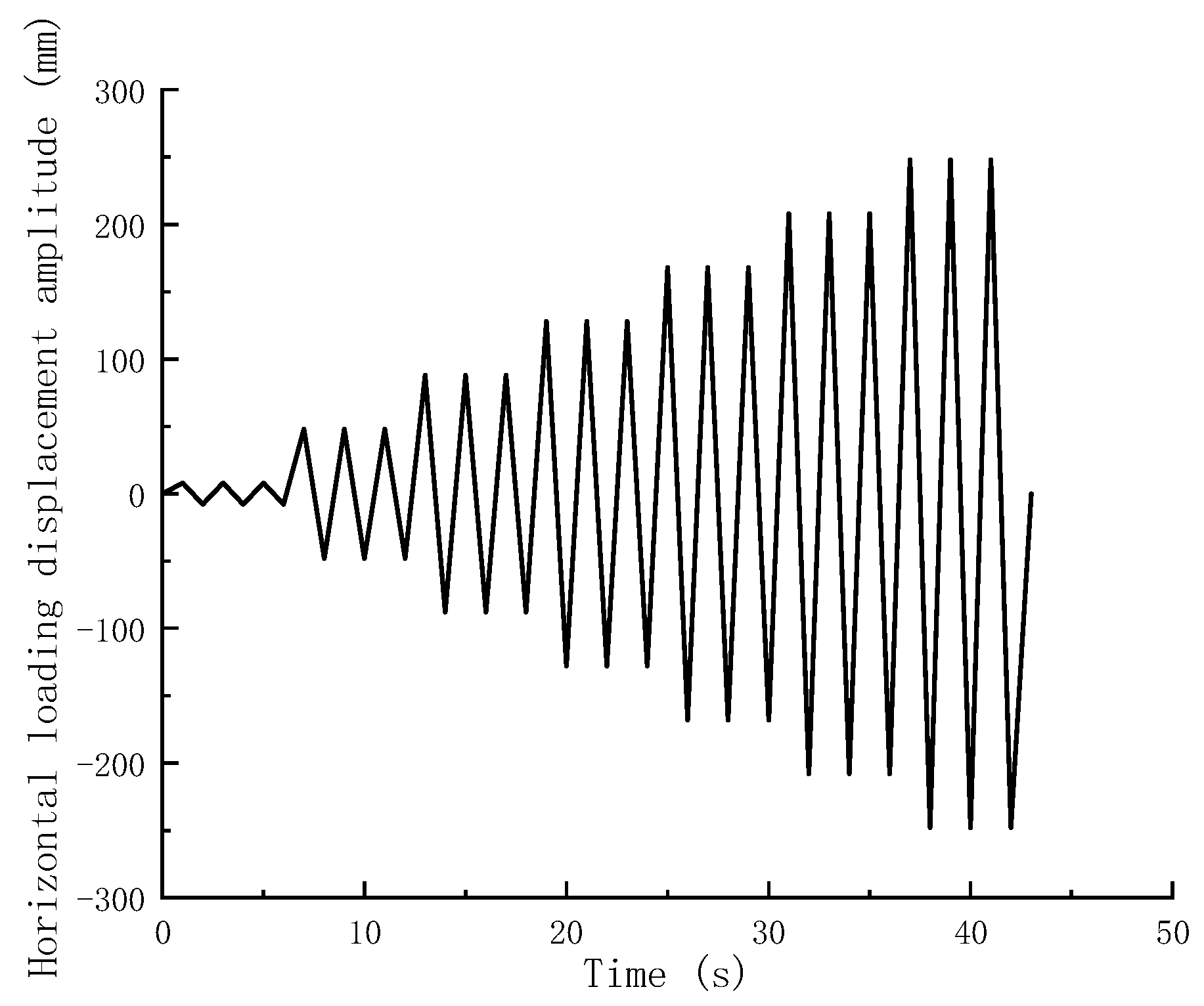
Figure 4.
Horizontal loading regime.
Figure 4.
Horizontal loading regime.
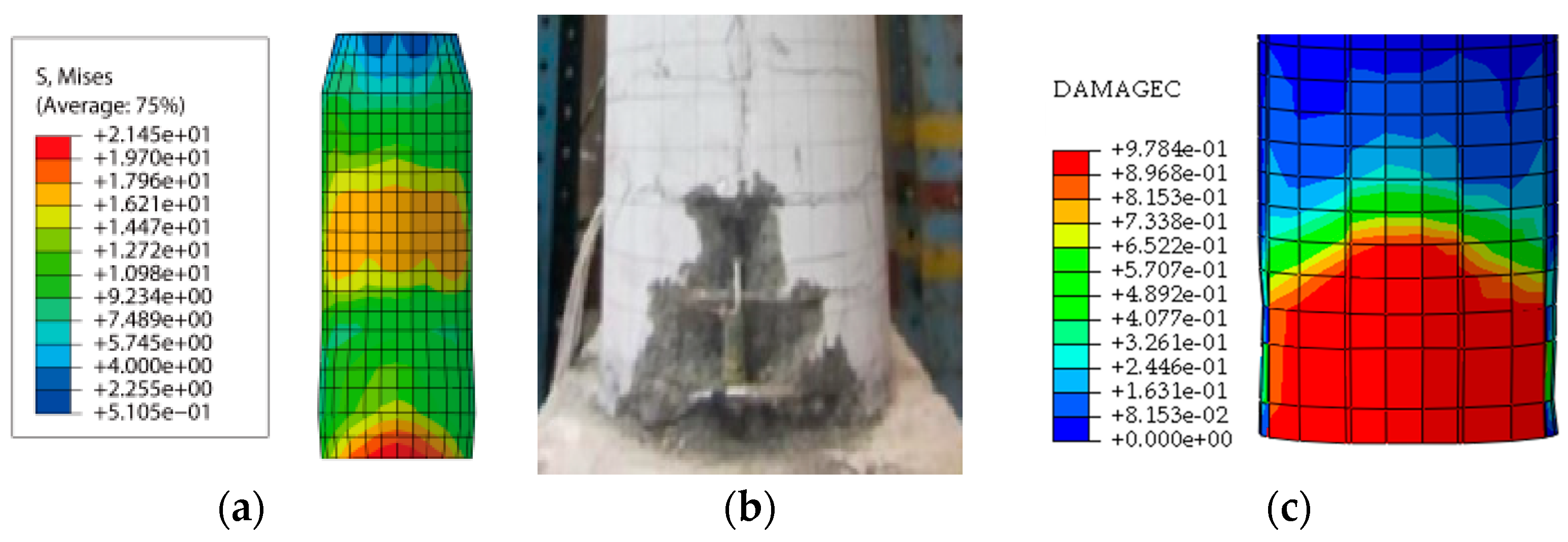
Figure 5.
Simulation and test results comparison of Specimen LJ-1: (a) finite element stress cloud; (b) test damage pattern; (c) damage stress cloud.
Figure 5.
Simulation and test results comparison of Specimen LJ-1: (a) finite element stress cloud; (b) test damage pattern; (c) damage stress cloud.
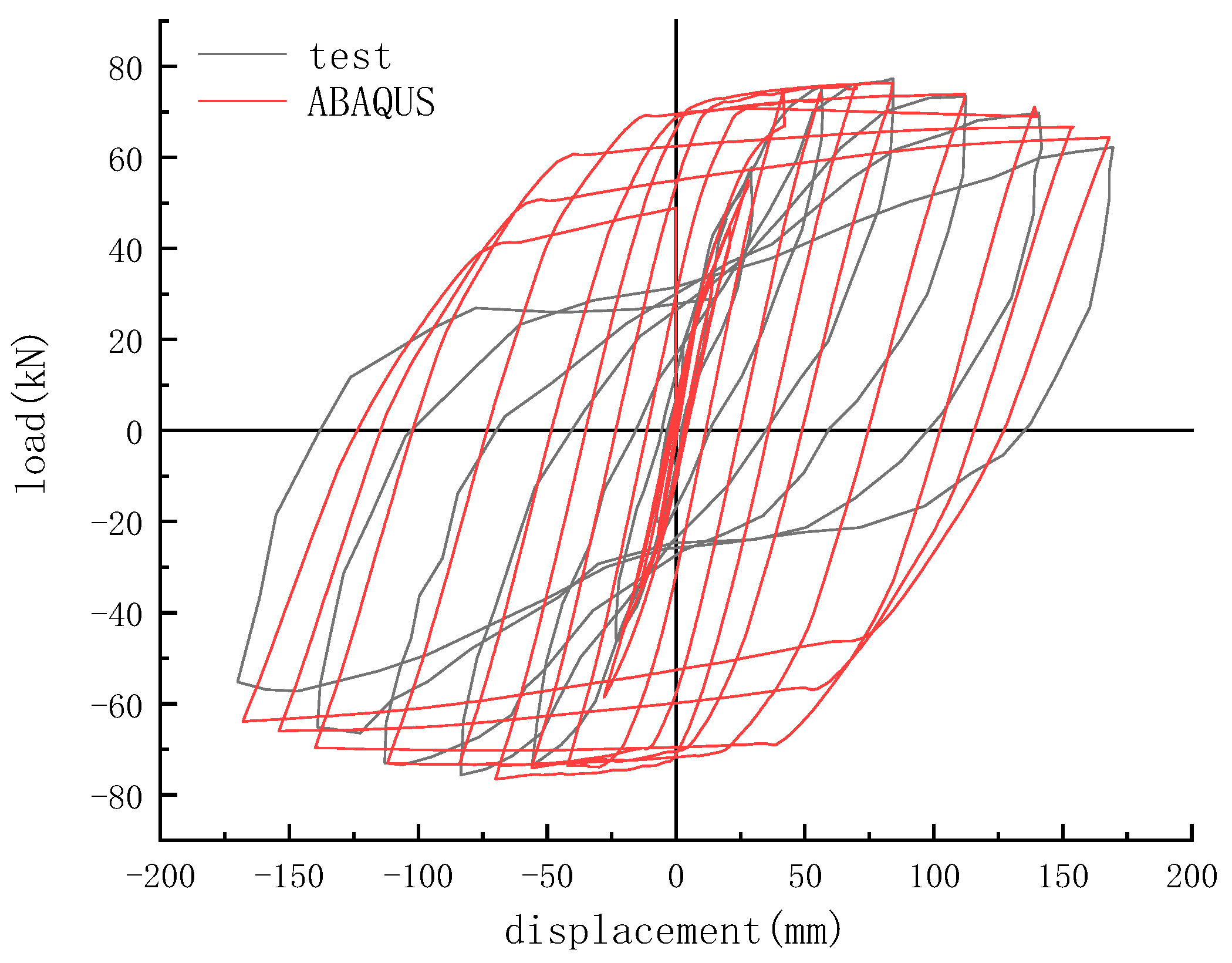
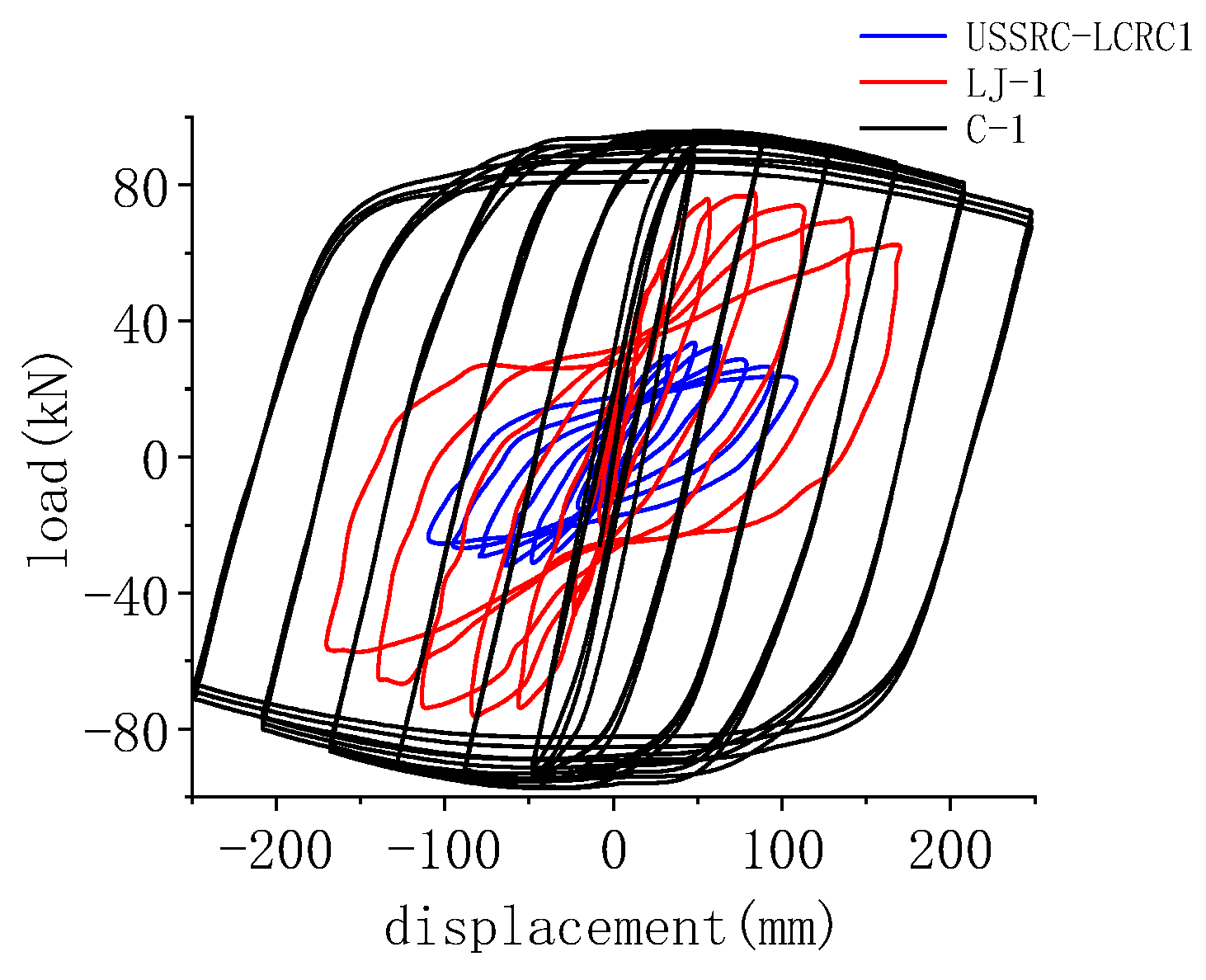
Figure 6.
Hysteresis curve of LJ-1 specimens.
Figure 6.
Hysteresis curve of LJ-1 specimens.
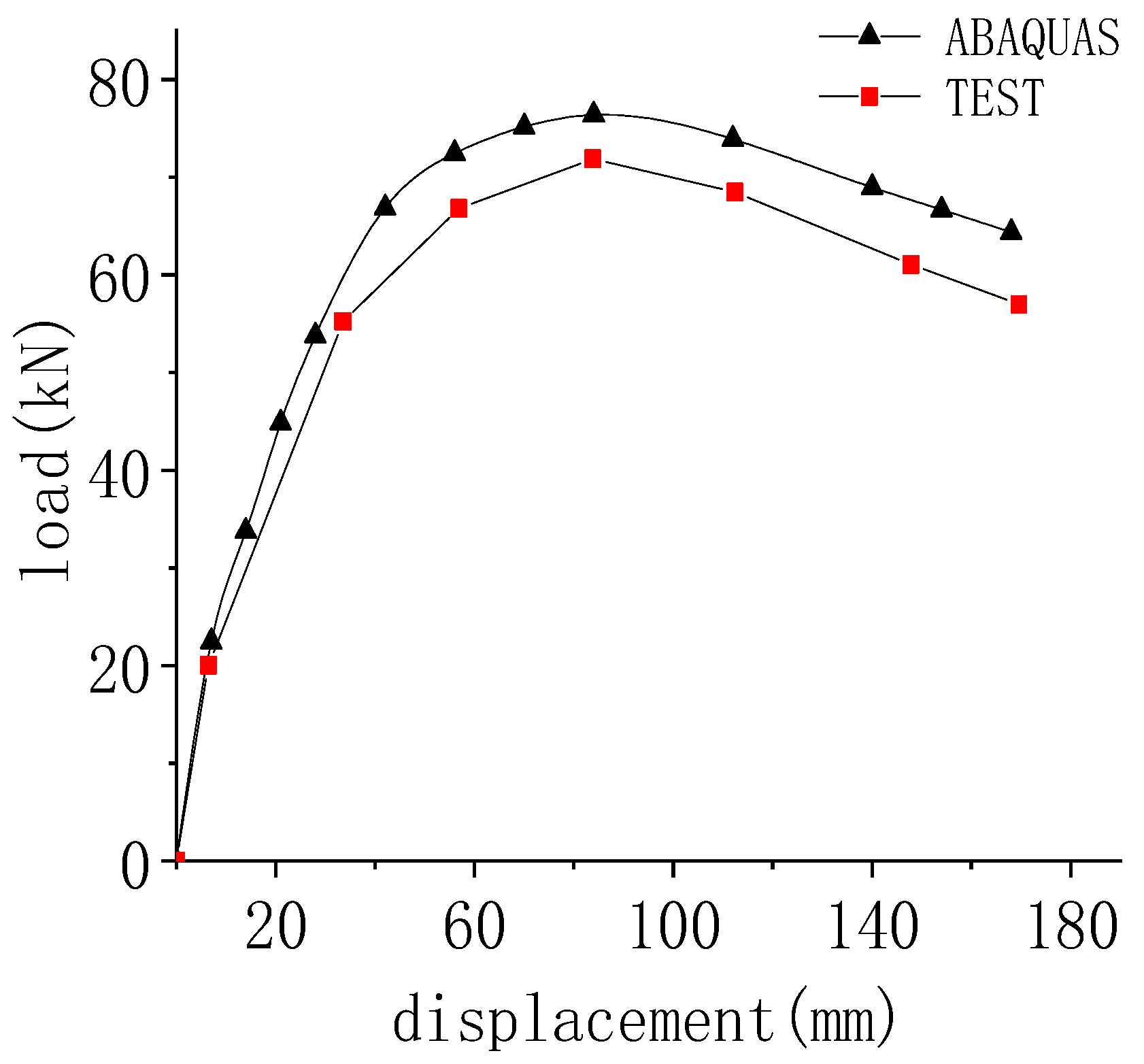
Figure 7.
Comparison of skeleton curves of LJ-1 specimens.
Figure 7.
Comparison of skeleton curves of LJ-1 specimens.
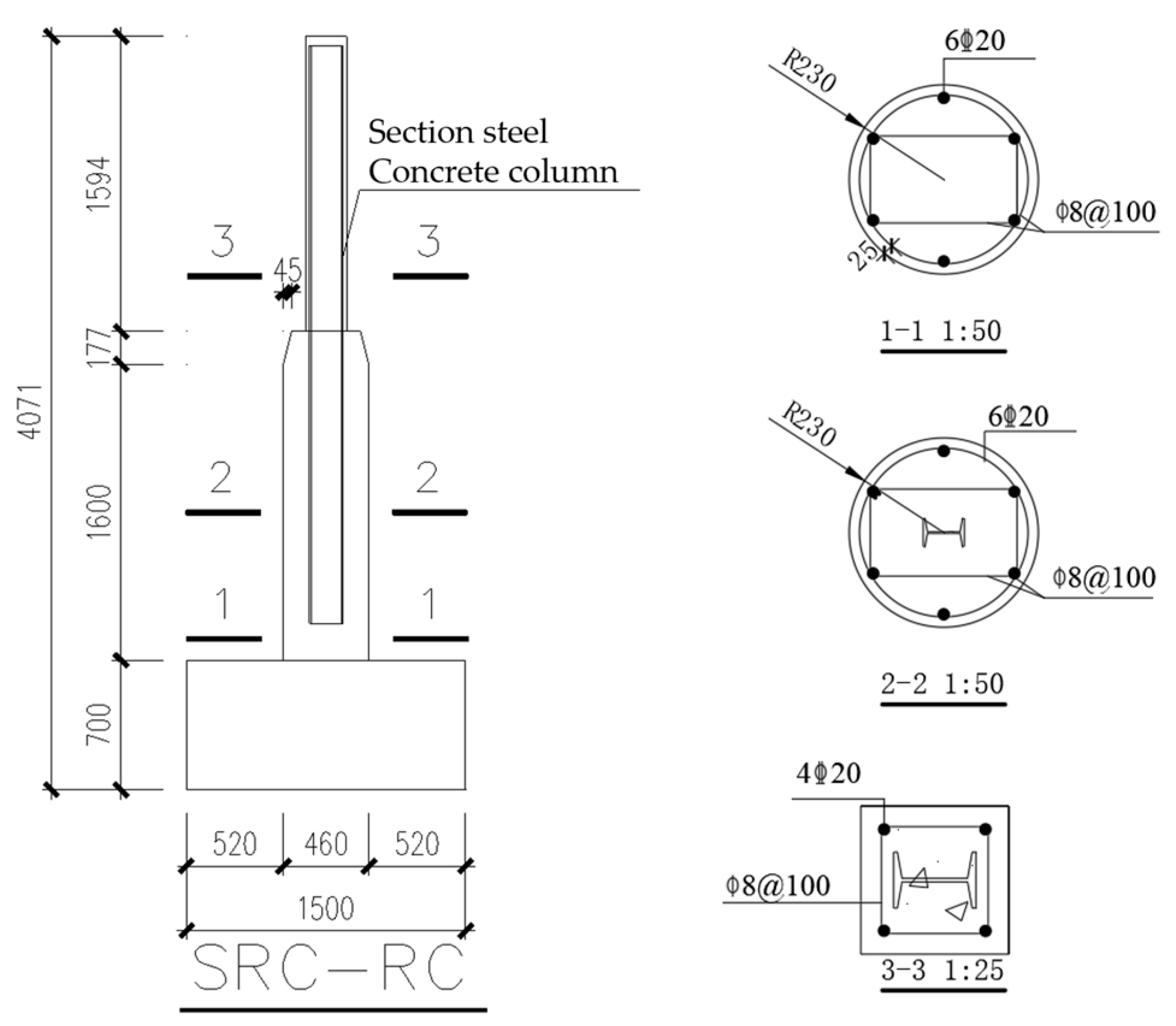
Figure 8.
Dimensions and reinforcement of USSRC-LCRC1 specimens.
Figure 8.
Dimensions and reinforcement of USSRC-LCRC1 specimens.
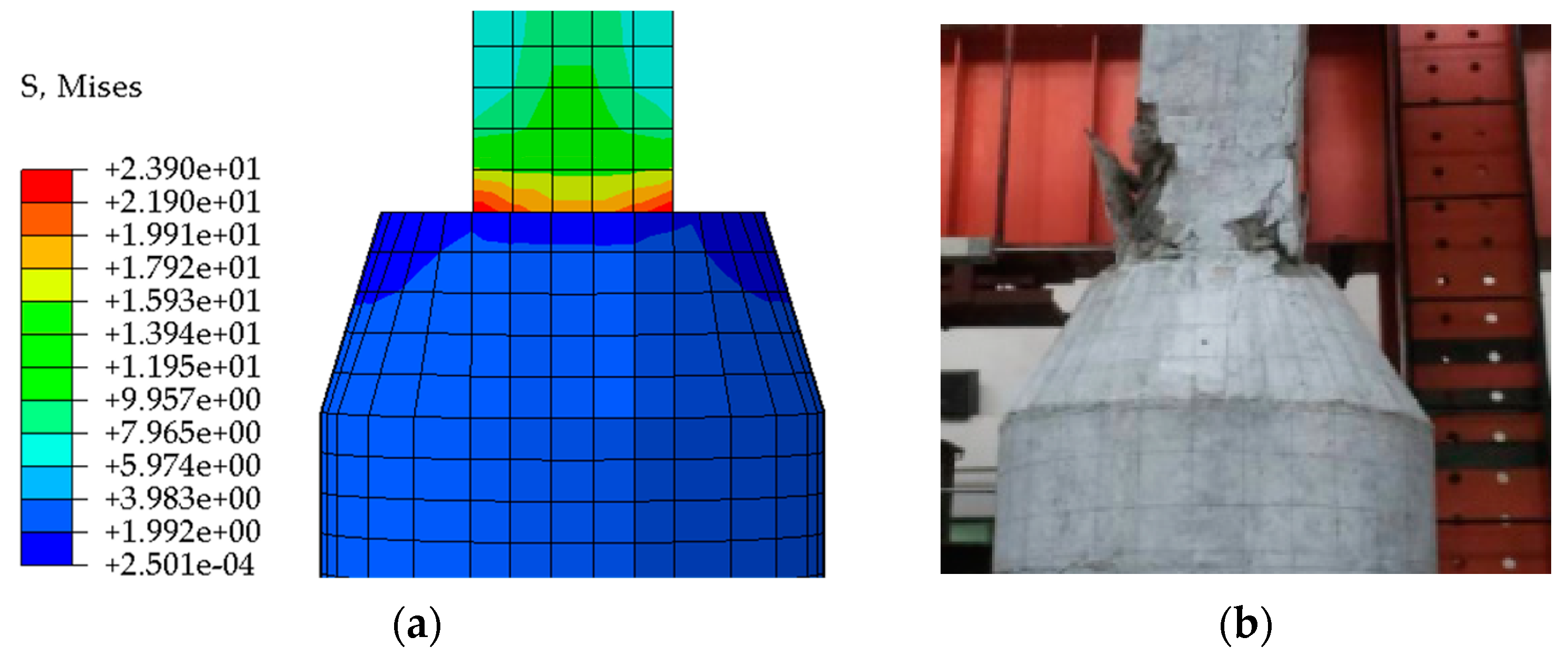
Figure 9.
Comparison of simulation and experimental results of USSRC-LCRC1: (a) Finite element stress cloud diagram; (b) Test damage pattern.
Figure 9.
Comparison of simulation and experimental results of USSRC-LCRC1: (a) Finite element stress cloud diagram; (b) Test damage pattern.
Figure 10.
Comparison of skeleton curves of SRC-RC1 specimen.
Figure 10.
Comparison of skeleton curves of SRC-RC1 specimen.
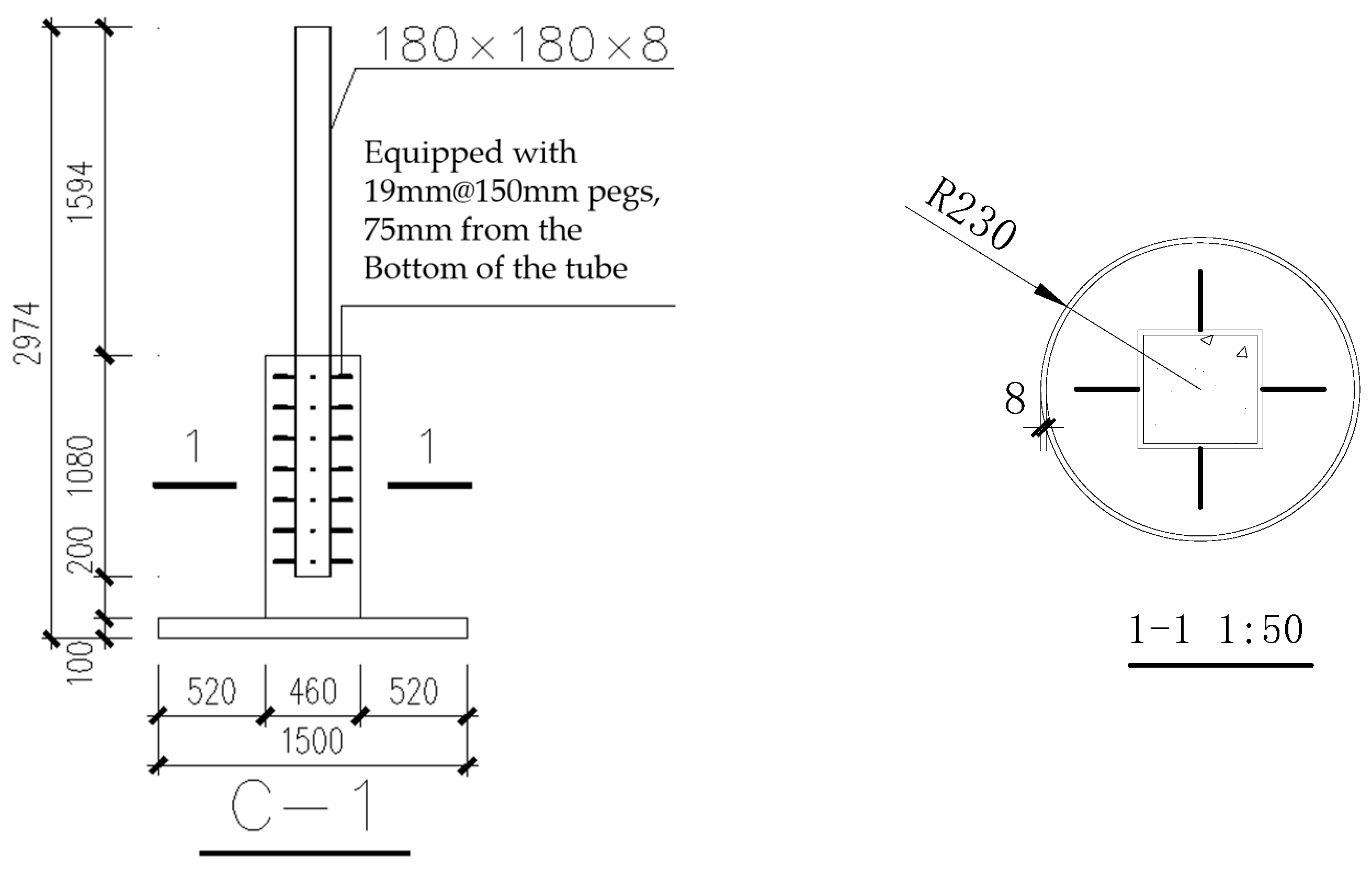
Figure 11.
Dimensions of C-1 specimen.
Figure 11.
Dimensions of C-1 specimen.
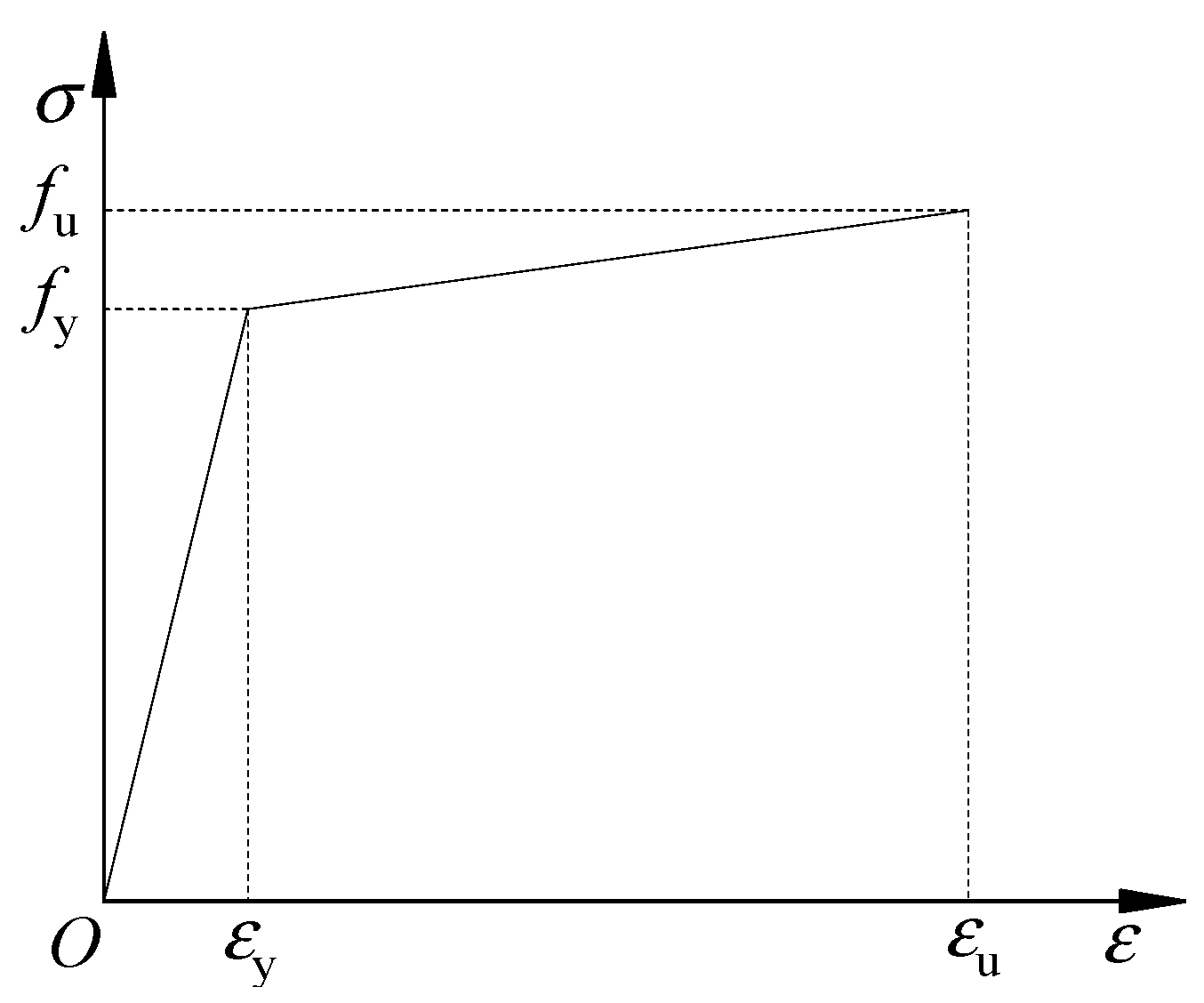
Figure 12.
The stress-strain relationship of steel.
Figure 12.
The stress-strain relationship of steel.
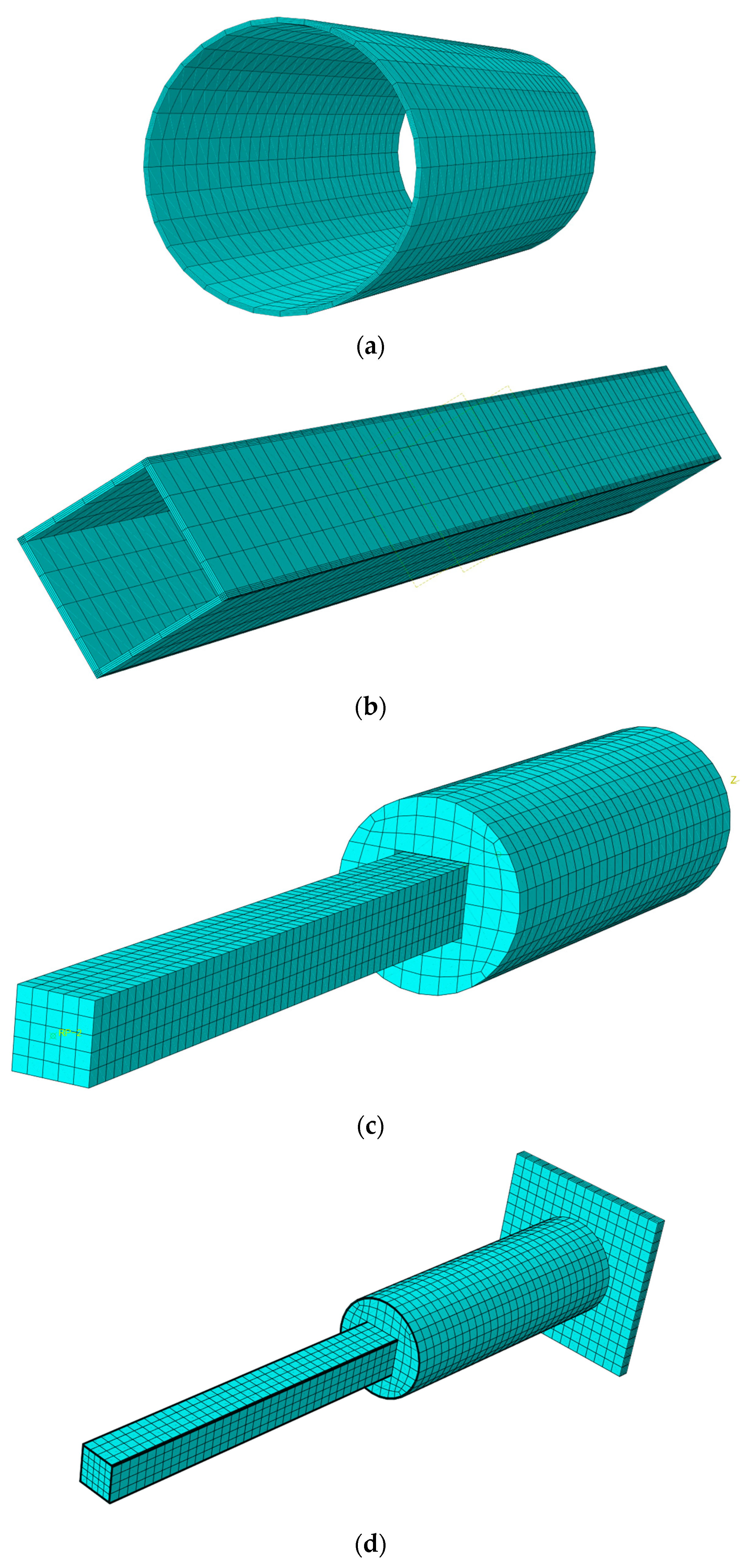
Figure 13.
Meshing diagram of each component: (a) circular steel tube meshing; (b) square steel tube meshing; (c) concrete meshing; (d) overall model and meshing.
Figure 13.
Meshing diagram of each component: (a) circular steel tube meshing; (b) square steel tube meshing; (c) concrete meshing; (d) overall model and meshing.
Figure 14.
Comparison of the skeleton curves of the three types of components.
Figure 14.
Comparison of the skeleton curves of the three types of components.
Figure 15.
Comparison of the hysteresis curves of the three types of components.
Figure 15.
Comparison of the hysteresis curves of the three types of components.
Figure 16.
Comparison of stiffness degradation curves of the three types of column connection components.
Figure 16.
Comparison of stiffness degradation curves of the three types of column connection components.
Figure 17.
Horizontal displacement loading system.
Figure 17.
Horizontal displacement loading system.
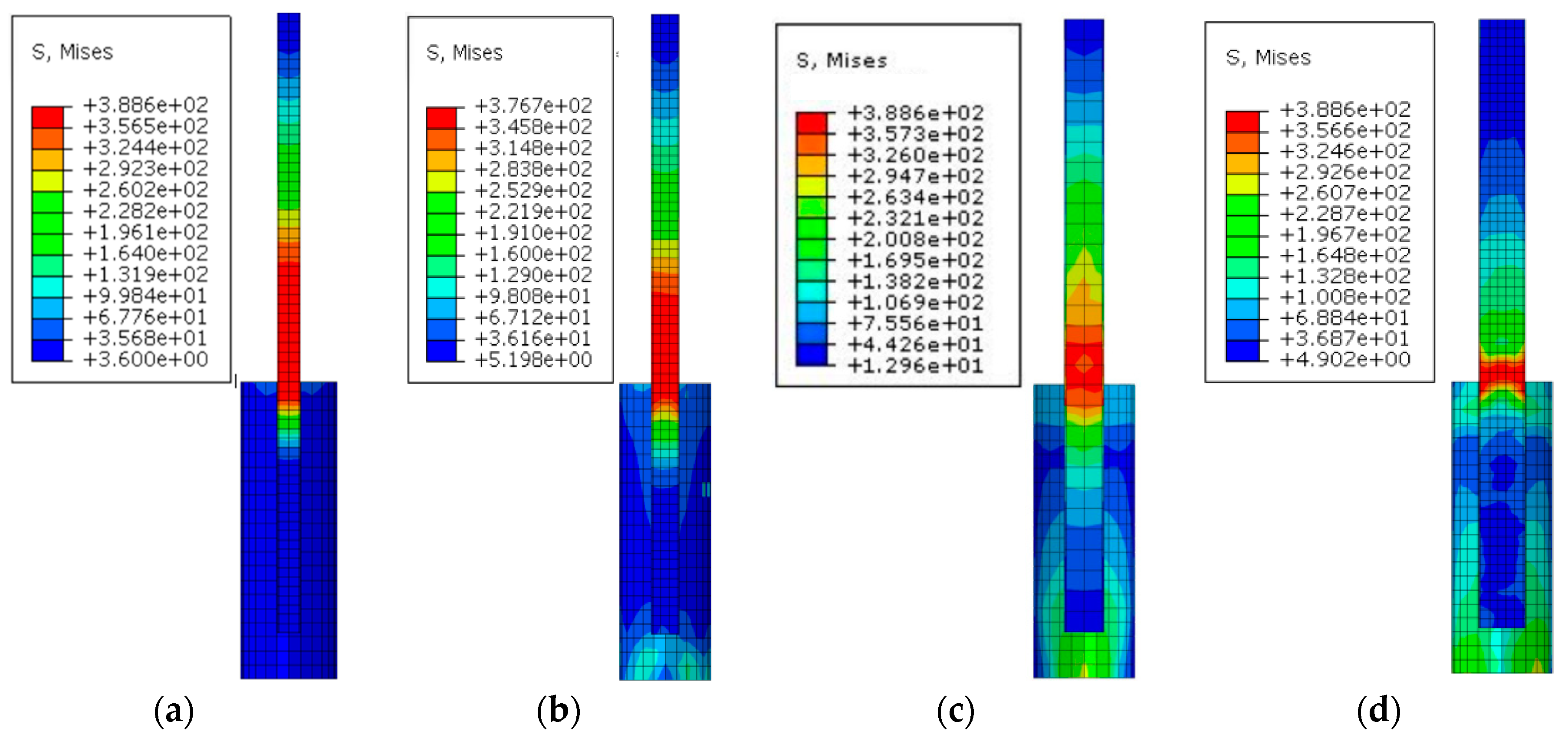
Figure 18.
The stress cloud of the steel tube of TY specimen at failure: (a) TY-1; (b) TY-2; (c) TY-3; (d) TY-4.
Figure 18.
The stress cloud of the steel tube of TY specimen at failure: (a) TY-1; (b) TY-2; (c) TY-3; (d) TY-4.
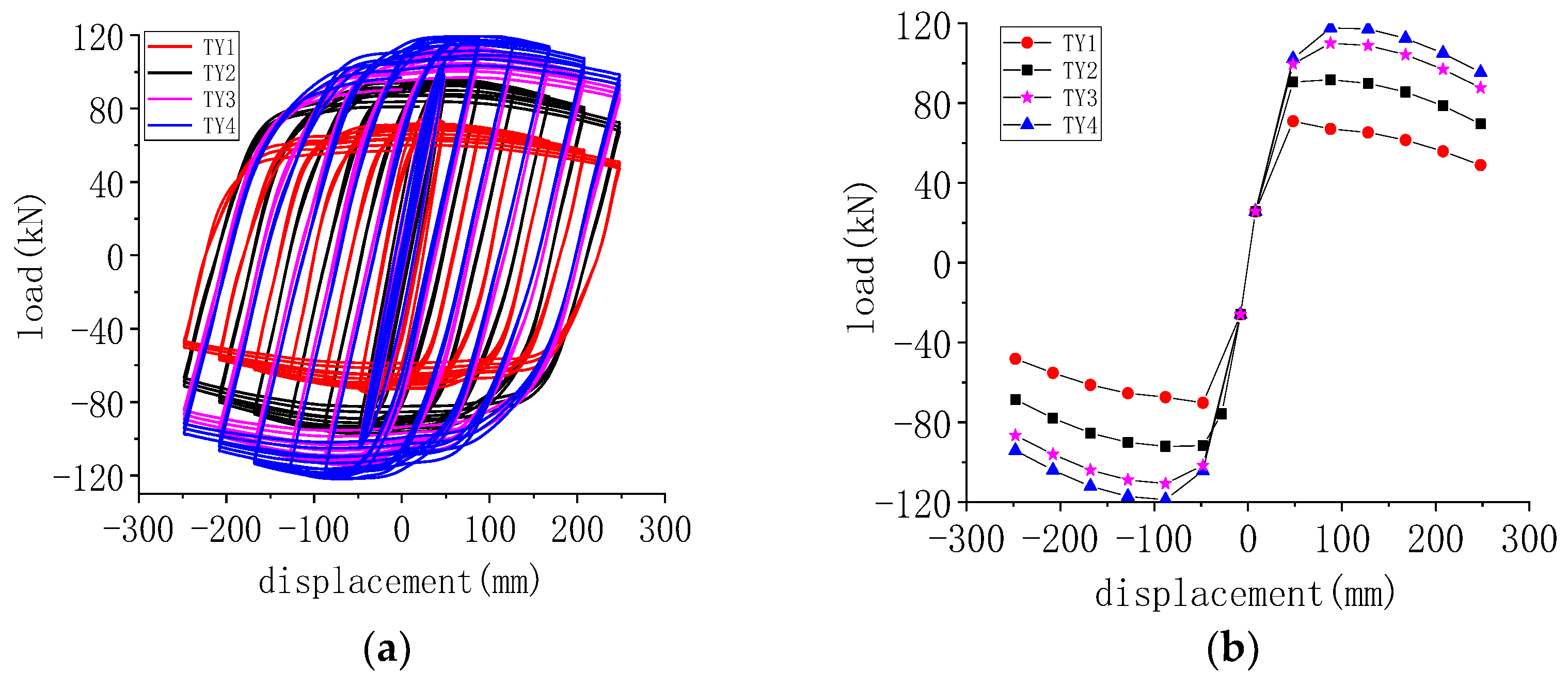
Figure 19.
Hysteresis curve and skeleton curve of TY specimen: (a) hysteresis curve; (b) skeleton curve.
Figure 19.
Hysteresis curve and skeleton curve of TY specimen: (a) hysteresis curve; (b) skeleton curve.
Figure 20.
Stiffness degradation curve of TY specimen.
Figure 20.
Stiffness degradation curve of TY specimen.
Figure 21.
The stress cloud of steel tube for specimen LS at failure: (a) LS-1; (b) LS-2; (c) LS-3; (d) LS-4.
Figure 21.
The stress cloud of steel tube for specimen LS at failure: (a) LS-1; (b) LS-2; (c) LS-3; (d) LS-4.
Figure 22.
Hysteresis curve and skeleton curve of specimen LS: (a) hysteresis curve; (b) skeleton curve.
Figure 22.
Hysteresis curve and skeleton curve of specimen LS: (a) hysteresis curve; (b) skeleton curve.
Figure 23.
Stiffness degradation curve of LS specimens.
Figure 23.
Stiffness degradation curve of LS specimens.
Table 1.
Parameters of the LJ-1 specimen.
Table 1.
Parameters of the LJ-1 specimen.
| Specimen | Steel Tube Cross-Sectional Size (mm) |
|---|
| LJ-1 | 180 × 180 × 8 |
Table 2.
Comparison of test and simulation parameters of LJ-1 specimen.
Table 2.
Comparison of test and simulation parameters of LJ-1 specimen.
| Phase | Yield Point | Peak Point | Failure Point |
|---|
| | | | | |
|---|
| Test | 55.25 | 33.54 | 71.86 | 83.82 | 61.08 | 147.84 |
| Simulation | 58.64 | 35.78 | 76.38 | 84.00 | 64.923 | 135.89 |
| Error (%) | 6.14 | 6.68 | 6.29 | 0.21 | 6.29 | 8.08 |
Table 3.
Parameters of the USSRC-LCRC1 specimen.
Table 3.
Parameters of the USSRC-LCRC1 specimen.
| Specimen | Metal Type | Ratio of Axial Compression | Longitudinal Reinforcement in the Upper | Longitudinal Support at the Lower | Stirrup Configuration |
|---|
| USSRC-LCRC1 | Q235b
(100 × 68 × 4.5) | 0.3 | 4![Buildings 13 00916 i001]() 20 20 | 6![Buildings 13 00916 i001]() 20 20 | ϕ8@100 |
Table 4.
Comparison of test and simulation parameters for SRC-RC1 specimens.
Table 4.
Comparison of test and simulation parameters for SRC-RC1 specimens.
| Phase | Yield Point | Peak Point | Failure Point |
|---|
| | | | | |
|---|
| Test | 24.97 | 23.30 | 32.94 | 55.49 | 28.00 | 87.39 |
| Simulation | 24.18 | 25.14 | 31.28 | 57.90 | 26.59 | 96.05 |
| Error (%) | 3.17 | 7.90 | 5.04 | 4.34 | 5.05 | 9.91 |
Table 5.
Parameters of C-1 specimen.
Table 5.
Parameters of C-1 specimen.
| Specimen | Steel Tube Size (mm) | Axial Compression Ratio | Length to Slenderness Ratio | Steel Tube | Concrete Strength |
|---|
| C-1 | 180 × 180 × 8 | 0.25 | 61.35 | Q355 | C30 |
Table 6.
Concrete basic parameters.
Table 6.
Concrete basic parameters.
Density
t/mm3 | Young’s Modulus | Poisson’s Ratio | Dilation Angle | Eccentricity | fb0/fc0 | K | Viscosity Parameter |
|---|
| 2.4 × 10−9 | 33,683.3 | 0.2 | 38 | 0.1 | 1.16 | 0.66667 | 0.01 |
Table 7.
Concrete compression damage.
Table 7.
Concrete compression damage.
| Yield Stress | Plastic Strain | Damage Parameters | Inelastic Strain |
|---|
| 15.88461008 | 0 | 0 | 0 |
| 22.97324533 | 0.000703841 | 0.447155833 | 0.000703841 |
| 26.50630226 | 0.001296779 | 0.563619489 | 0.001296779 |
| 28.42775095 | 0.001938553 | 0.64289235 | 0.001938553 |
| 29.52847376 | 0.002605198 | 0.69962601 | 0.002605198 |
| 30.17329051 | 0.003285658 | 0.74192038 | 0.003285658 |
| 30.5480548 | 0.003974301 | 0.779448462 | 0.003974301 |
| 30.75498954 | 0.004668031 | 0.800280601 | 0.004668031 |
| 30.85379147 | 0.005365037 | 0.821127858 | 0.005365037 |
| 30.88102445 | 0.006064211 | 0.838299099 | 0.006064211 |
| 29.27111322 | 0.007512997 | 0.871400333 | 0.007512997 |
| 25.98163115 | 0.009012678 | 0.901553528 | 0.009012678 |
| 22.520346 | 0.010517565 | 0.924978211 | 0.010517565 |
| 19.46283054 | 0.012010217 | 0.942166576 | 0.012010217 |
| 16.92110929 | 0.013487239 | 0.954633177 | 0.013487239 |
Table 8.
Concrete tensile damage.
Table 8.
Concrete tensile damage.
| Yield Stress | Cracking Strain | Damage Parameters | Cracking Strain |
|---|
| 3.067031 | 0 | 0 | 0 |
| | | 0.9 | 0.1 |
Table 9.
Bifold model parameters of steel.
Table 9.
Bifold model parameters of steel.
Density
t/mm3 | Young’s Modulus | Poisson’s Ratio | Yield Stress | Plastic Strain |
|---|
| 7.85 × 10−9 | 2.72 × 105 | 0.3 | 323.8 | 0 |
| 388.56 | 0.0235 |
Table 10.
Load-bearing performance and ductility index of the three types of components.
Table 10.
Load-bearing performance and ductility index of the three types of components.
| Member | | | | | | |
|---|
| USCFST-LCCFST | 44.4 | 211.0 | 83.7 | 91.7 | 77.9 | 4.75 |
| USCFST-LCRC | 33.5 | 147.8 | 55.2 | 71.9 | 61.1 | 4.41 |
| USSRC-LCRC | 23.3 | 87.4 | 25.0 | 32.9 | 28.0 | 3.75 |
Table 11.
Comparison of equivalent viscous damping coefficients of the three types of column connection components.
Table 11.
Comparison of equivalent viscous damping coefficients of the three types of column connection components.
| Member | Equivalent Viscous Damping Coefficients |
|---|
| Yield Phase | Peak Phase | Failure Phase |
|---|
| USCFST-LCCFST | 0.092 | 0.300 | 0.550 |
| USCFST-LCRC | 0.093 | 0.183 | 0.281 |
| USSRC-LCRC | 0.115 | 0.161 | 0.289 |
Table 12.
Specific settings of the variable parameters of the USCFST-LCCFSE column connection components.
Table 12.
Specific settings of the variable parameters of the USCFST-LCCFSE column connection components.
| Specimen No. | | | | | | |
|---|
| TY-1 | 235 | 30 | 0.063 | 0.3 | 8 | 1080 |
| TY-2 | 355 |
| TY-3 | 390 |
| TY-4 | 420 |
| LS-1 | 355 | 30 | 0.012 | 0.3 | 8 | 1080 |
| LS-2 | 0.027 |
| LS-3 | 0.063 |
| LS-4 | 0.159 |
Table 13.
Load-bearing performance and ductility index of TY specimens.
Table 13.
Load-bearing performance and ductility index of TY specimens.
| Specimen | | | | | | | |
|---|
| TY-1 | 37.38 | 48.00 | 177.73 | 58.67 | 70.57 | 59.98 | 4.75 |
| TY-2 | 44.38 | 87.99 | 211.00 | 84.71 | 91.70 | 77.95 | 4.75 |
| TY-3 | 50.49 | 87.99 | 219.42 | 101.24 | 110.40 | 93.84 | 4.35 |
| TY-4 | 53.32 | 87.99 | 224.44 | 105.29 | 118.26 | 100.52 | 4.21 |
Table 14.
Equivalent viscous damping coefficient of TY specimens.
Table 14.
Equivalent viscous damping coefficient of TY specimens.
| Specimen No. | Equivalent Viscous Damping Coefficient |
|---|
| Yield Stage | Peak Stage | Failure Stage |
|---|
| TY-1 | 0.259 | 0.415 | 0.554 |
| TY-2 | 0.092 | 0.300 | 0.550 |
| TY-3 | 0.116 | 0.230 | 0.492 |
| TY-4 | 0.105 | 0.200 | 0.469 |
Table 15.
Load-bearing performance and ductility index of LS specimens.
Table 15.
Load-bearing performance and ductility index of LS specimens.
| Specimen | | | | | | | |
|---|
| LS-1 | 47.35 | 87.99 | 107.30 | 22.91 | 23.21 | 19.73 | 2.27 |
| LS-2 | 45.99 | 87.99 | 119.07 | 47.29 | 49.16 | 41.78 | 2.59 |
| LS-3 | 44.38 | 87.99 | 211.00 | 84.71 | 91.70 | 77.95 | 4.75 |
| LS-4 | 42.83 | 88.00 | 247.15 | 115.98 | 128.86 | 109.53 | 5.77 |
Table 16.
Equivalent viscous damping coefficients of LS specimens.
Table 16.
Equivalent viscous damping coefficients of LS specimens.
| Specimen | Equivalent Viscous Damping Coefficients |
|---|
| Yield Stage | Peak Stage | Failure Stage |
|---|
| LS-1 | 0.081 | 0.106 | 0.372 |
| LS-2 | 0.142 | 0.144 | 0.355 |
| LS-3 | 0.092 | 0.300 | 0.550 |
| LS-4 | 0.180 | 0.253 | 0.508 |









 20
20 20
20




