Computational Comparison of Performance of Different Steel Plate Shear Yielding Dampers
Abstract
1. Introduction
2. Materials and Methods
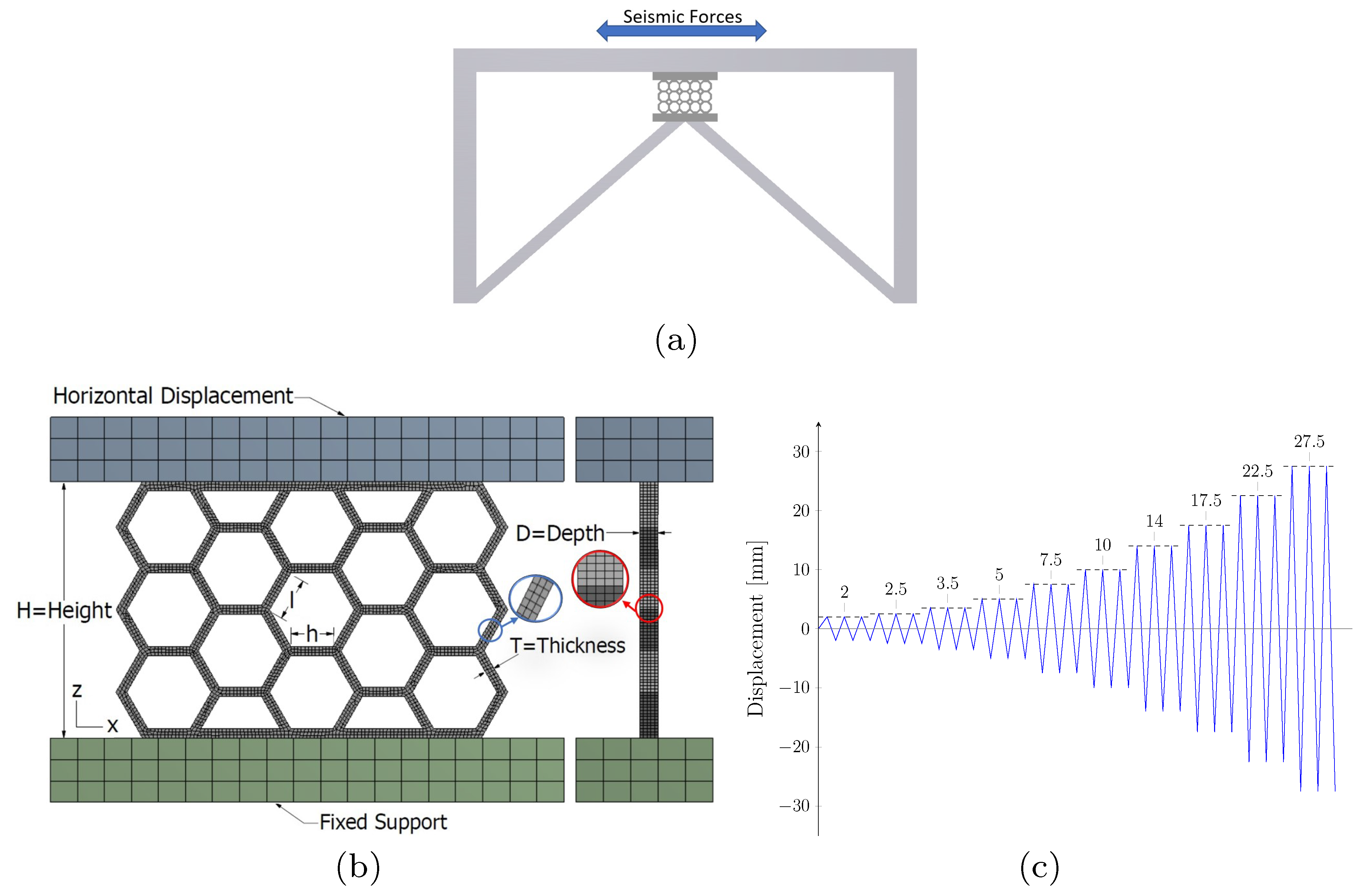
2.1. Finite Element Model
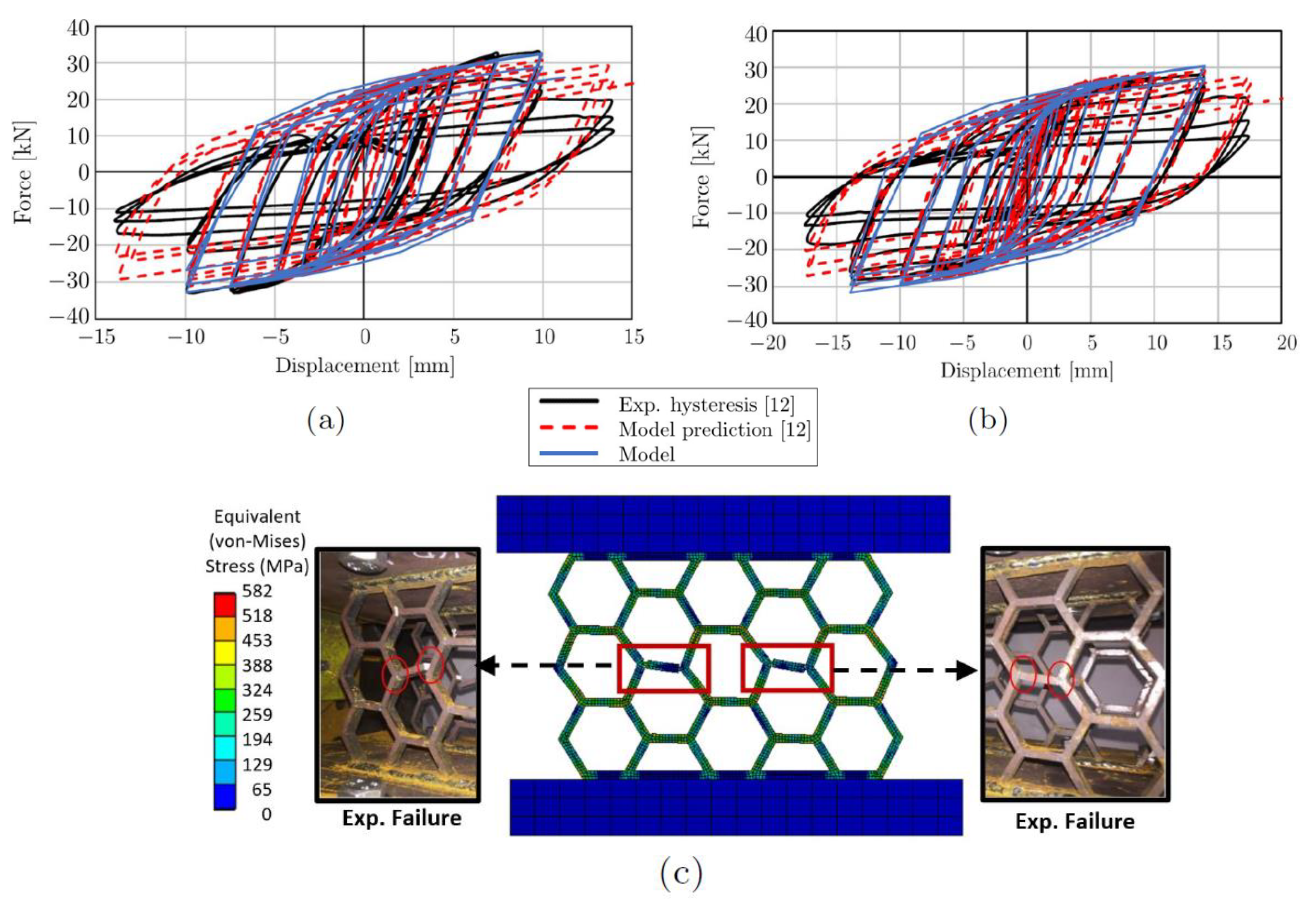
2.2. FE Model Calibration and Validation
3. Results
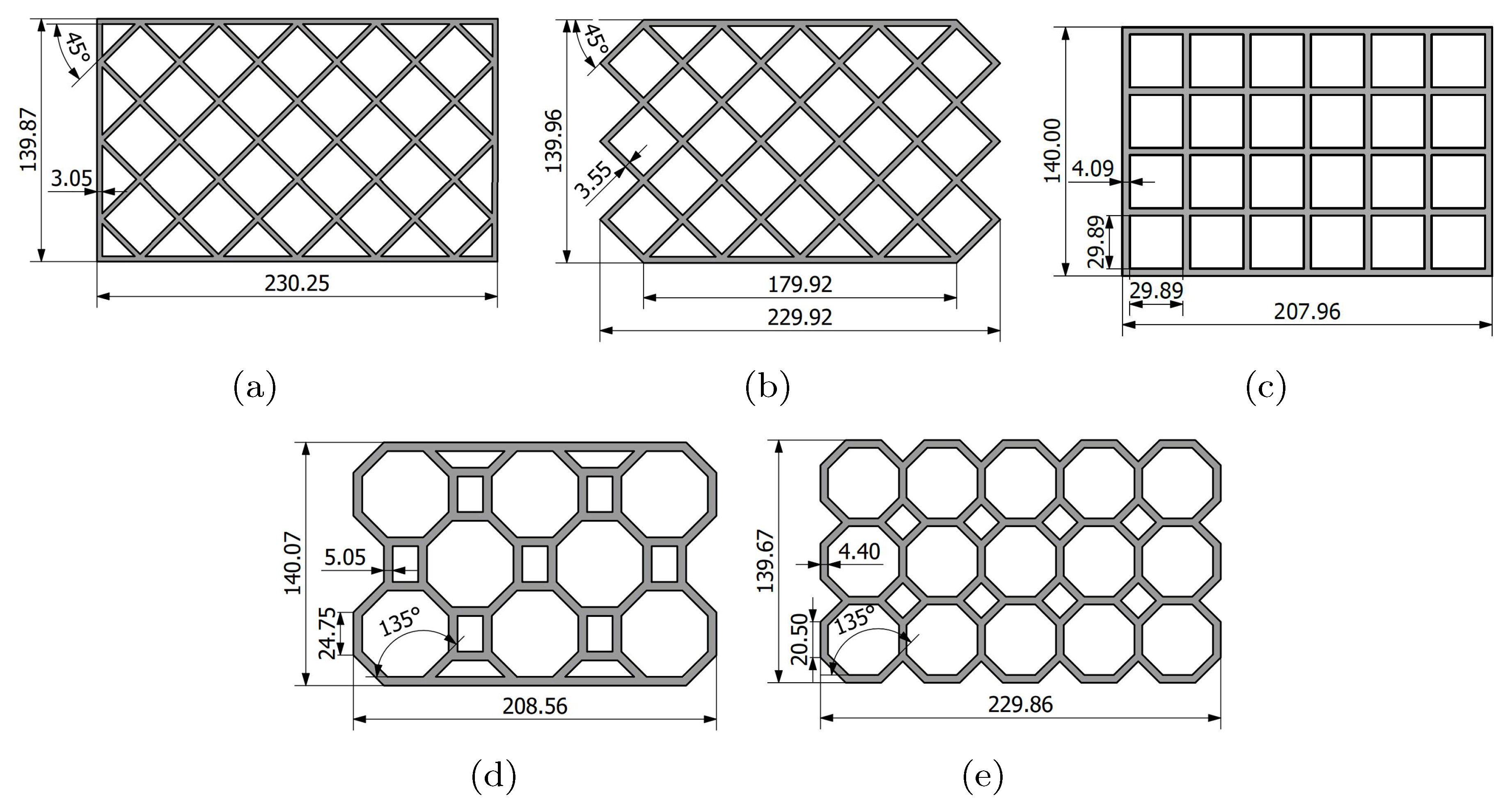
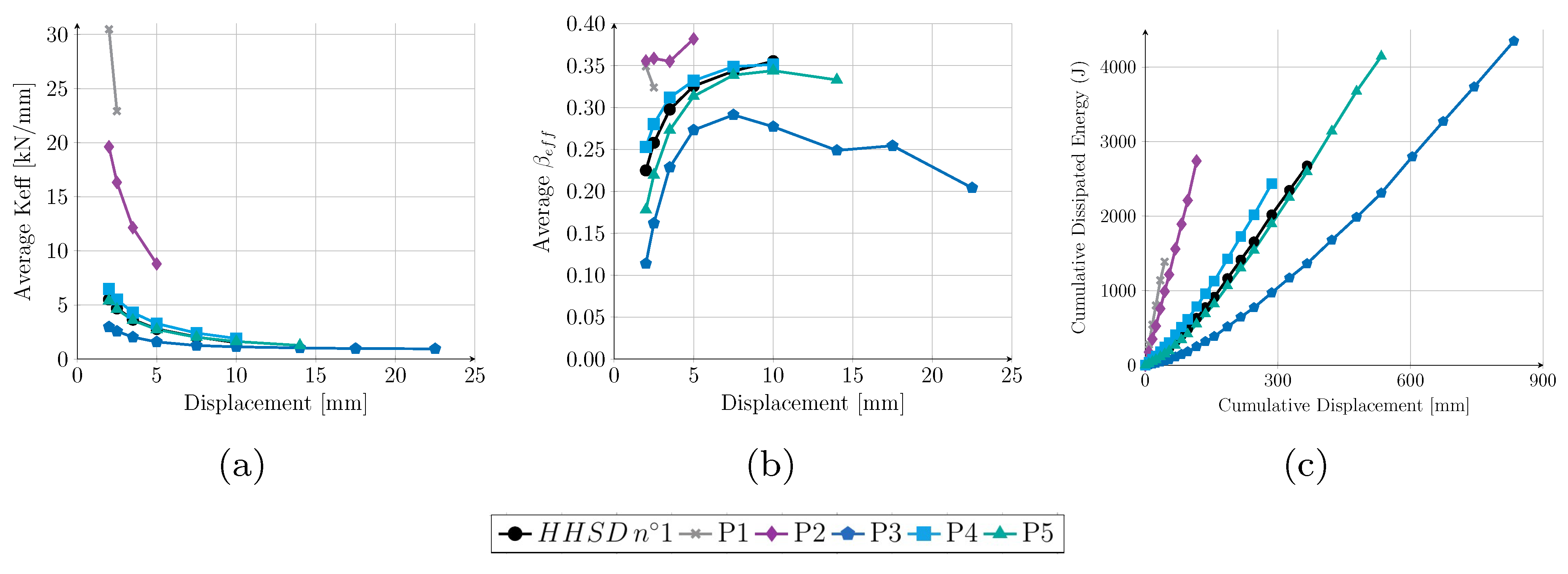
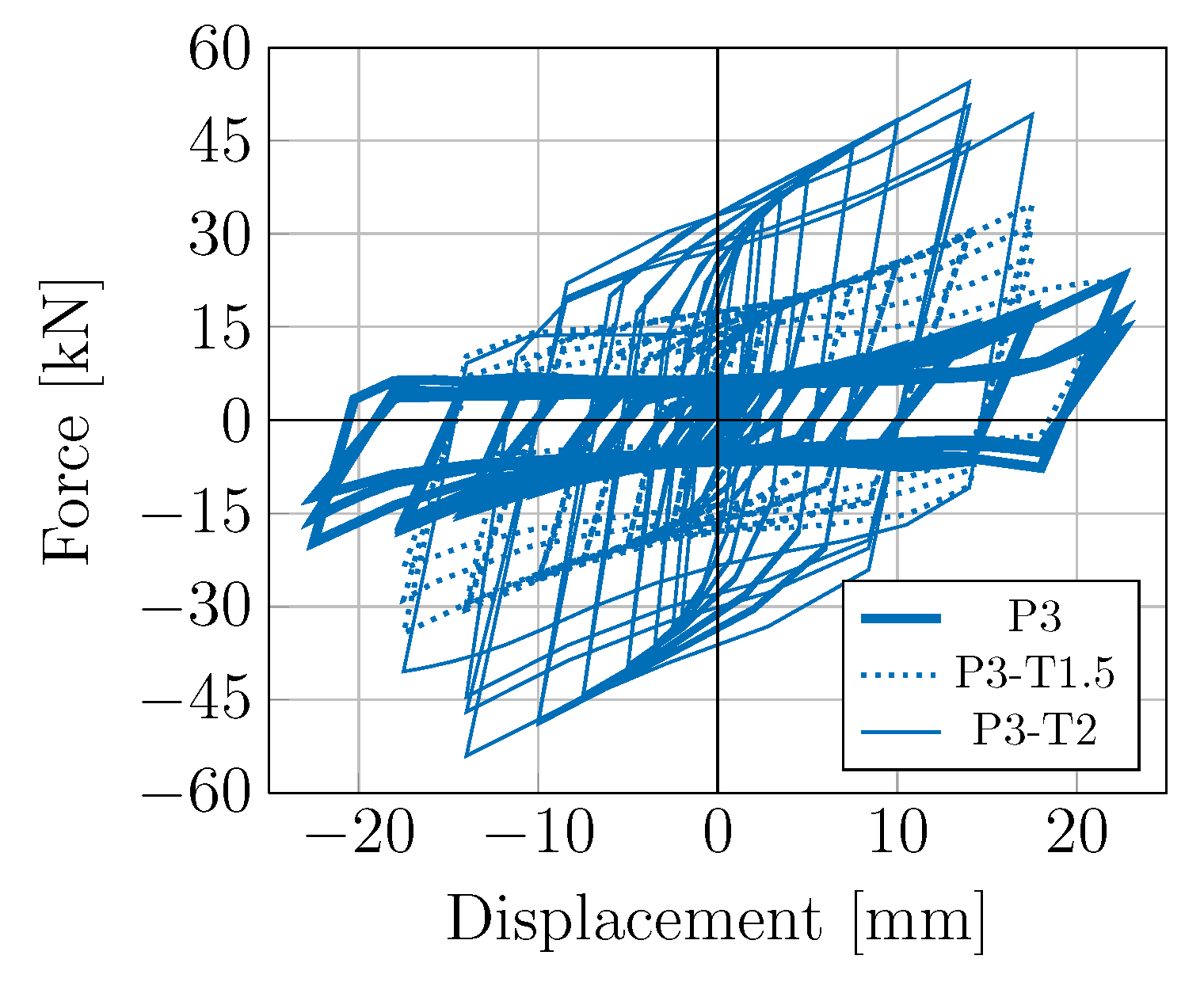
3.1. Simulation Results of SPSYDs
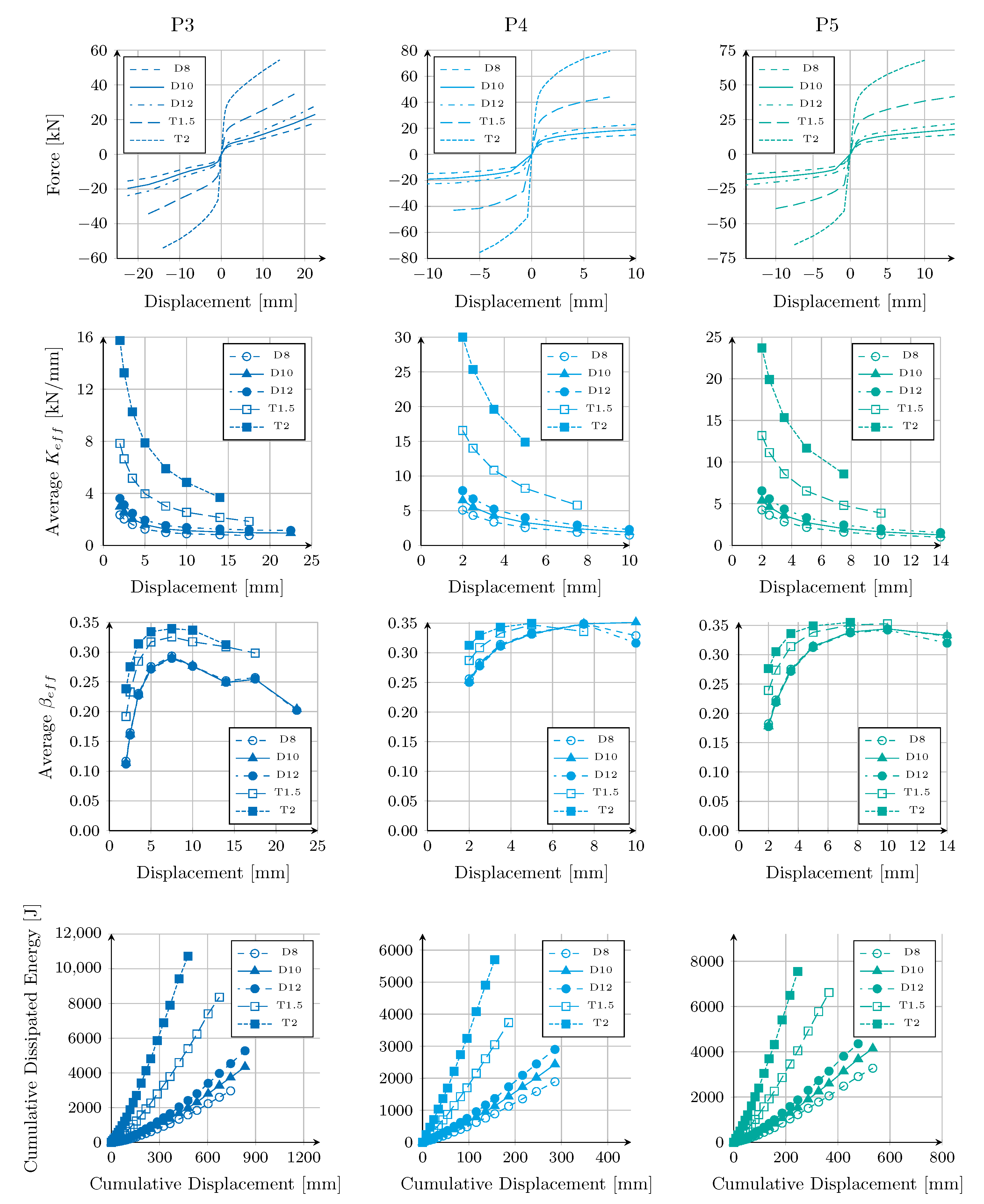
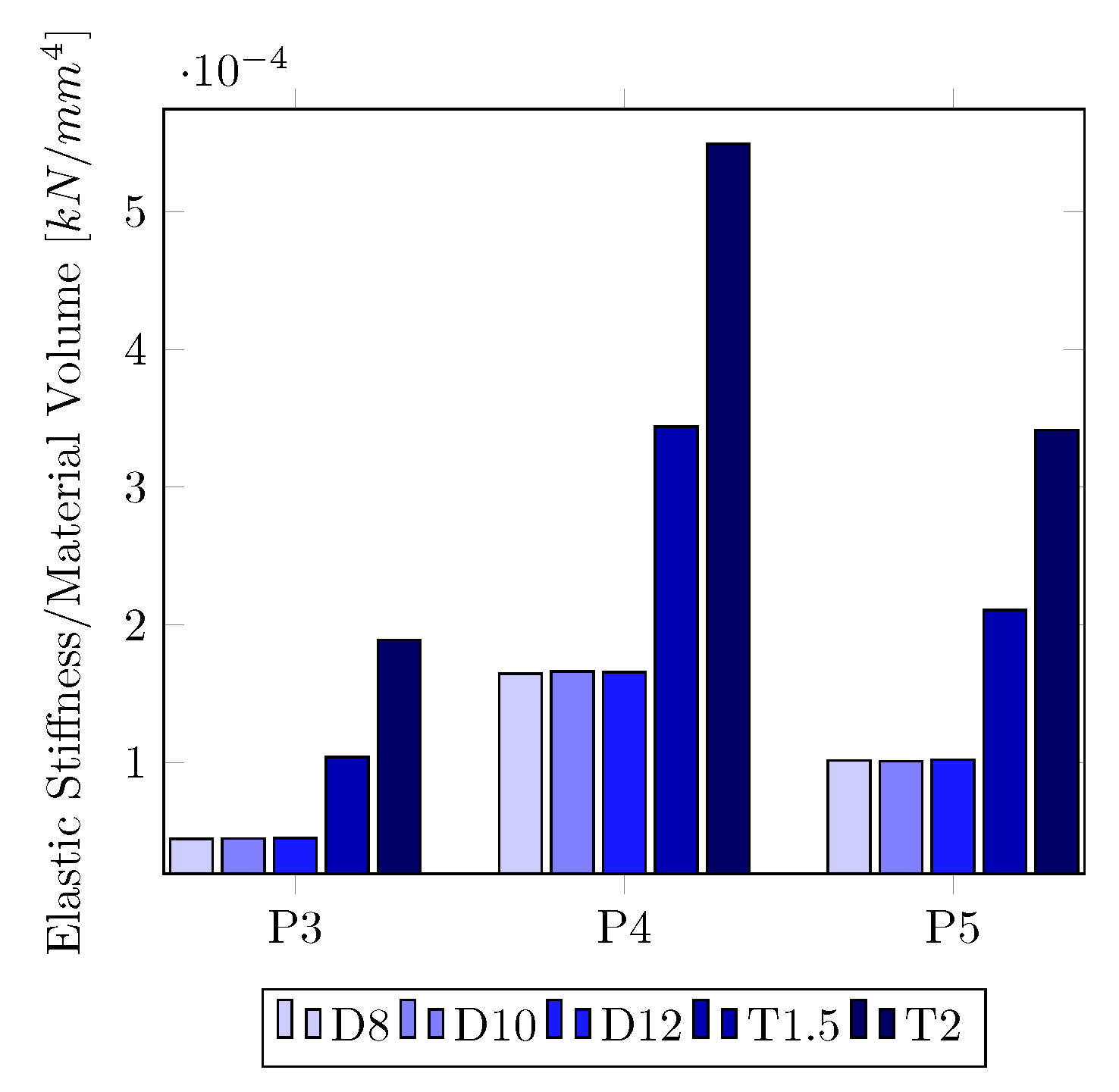
3.2. Influence of Depth and Thickness on the Behavior of Dampers
3.3. Influence of the Number of Diagonals on the Behavior of Dampers
4. Discussion
5. Conclusions
- The performance of a damper can be significantly affected in terms of flexibility and dissipation capacity through modification of its geometry and by maintaining a constant amount of material.
- The presence of infill material patterns oriented at 45° and generating diagonals of connected material between the support plates increases the rigidity of the damper under shear stresses. To avoid premature fracture, interruptions of these diagonals using bars or material configuration with orientation changes should be used to increase flexibility and maintain uniform flow stress distribution.
- Considering the damper designs resulting in adequate seismic performance (P3, P4, P5), a change in plate depth does not affect the effective damping. Stiffness is indeed directly proportional to this dimension.
- A variation in the thickness of the plate causes a significant change in the damper flexibility, since it modifies the resistive amount of material in the shear plane.
- The number of infill material diagonals influences the stiffness and the dissipated energy capacity, without affecting the maximum number of cycles that the damper withstands prior to failure.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Teruna, D.R.; Majid, T.A.; Budiono, B. Experimental study of hysteretic steel damper for energy dissipation capacity. Adv. Civ. Eng. 2015, 2015, 631726. [Google Scholar] [CrossRef]
- Sahoo, D.R.; Singhal, T.; Taraithia, S.S.; Saini, A. Cyclic behavior of shear-and-flexural yielding metallic dampers. J. Constr. Steel Res. 2015, 114, 247–257. [Google Scholar] [CrossRef]
- de Chile, G. Propuestas Plan de Acción de Restauración y Reconstrucción Patrimonial Debido al Terremoto y Maremoto del 27 de Febrero del 2010. 2010. Available online: https://www.google.com.hk/url?sa=t&rct=j&q=&esrc=s&source=web&cd=&ved=2ahUKEwi0hcqH6eH9AhWqTmwGHcI9AcMQFnoECAkQAQ&url=https%3A%2F%2Fwww.desarrollosocialyfamilia.gob.cl%2Fpdf%2Fplan-reconstruccion-resumen-ejecutivo.pdf&usg=AOvVaw11j3ne0X5iaWvuhdk3_D4N (accessed on 13 March 2023).
- Saaed, T.E.; Nikolakopoulos, G.; Jonasson, J.E.; Hedlund, H. A state-of-the-art review of structural control systems. J. Vib. Control. 2015, 21, 919–937. [Google Scholar] [CrossRef]
- Javanmardi, A.; Ibrahim, Z.; Ghaedi, K.; Benisi Ghadim, H.; Hanif, M.U. State-of-the-Art Review of Metallic Dampers: Testing, Development and Implementation. Arch. Comput. Methods Eng. 2020, 27, 455–478. [Google Scholar] [CrossRef]
- Teruna, D.R.; Wijaya, H. Seismic fragility assessment of building with metallic hysteretic damper in consideration of stiffness ratio. Key Eng. Mater. 2018, 765, 377–382. [Google Scholar] [CrossRef]
- Ranaei, O.; Aghakouchak, A.A. A new hybrid energy dissipation system with viscoelastic and flexural yielding strips dampers for multi-level vibration control. Arch. Civ. Mech. Eng. 2019, 19, 584–597. [Google Scholar] [CrossRef]
- Francavilla, A.B.; Latour, M.; Piluso, V.; Rizzano, G. Design criteria for beam-to-column connections equipped with friction devices. J. Constr. Steel Res. 2020, 172, 106240. [Google Scholar] [CrossRef]
- Zhai, Z.; Guo, W.; Yu, Z.; He, C.; Zeng, Z. Experimental and numerical study of S-shaped steel plate damper for seismic resilient application. Eng. Struct. 2020, 221, 111006. [Google Scholar] [CrossRef]
- Jarrah, M.; Khezrzadeh, H.; Mofid, M.; Jafari, K. Experimental and numerical evaluation of piston metallic damper (PMD). J. Constr. Steel Res. 2019, 154, 99–109. [Google Scholar] [CrossRef]
- Xiao, L.; Li, Y.; Hui, C.; Zhou, Z.; Deng, F. Experimental Study on Mechanical Properties of Shear Square Section Steel Tube Dampers. Metals 2022, 12, 418. [Google Scholar] [CrossRef]
- Javanmardi, A.; Ghaedi, K.; Ibrahim, Z.; Huang, F.; Xu, P. Development of a new hexagonal honeycomb steel damper. Arch. Civ. Mech. Eng. 2020, 20. [Google Scholar] [CrossRef]
- Motamedi, M.; Nateghi-A, F. Study on mechanical characteristics of accordion metallic damper. J. Constr. Steel Res. 2018, 142, 68–77. [Google Scholar] [CrossRef]
- Chan, R.W.K.; Albermani, F. Experimental study of steel slit damper for passive energy dissipation. Eng. Struct. 2008, 30, 1058–1066. [Google Scholar] [CrossRef]
- Naeem, A.; Kim, J. Seismic performance evaluation of a multi-slit damper. Eng. Struct. 2019, 189, 332–346. [Google Scholar] [CrossRef]
- Zongjing, L.; Ganping, S. Test and evaluation of modified TADAS devices with different grades of steel. Earthq. Eng. Eng. Vib. 2020, 19, 451–464. [Google Scholar] [CrossRef]
- Eskandari, M.; Najafabadi, E.P. Experimental and analytical study of telescopic lead yielding damper. J. Constr. Steel Res. 2018, 150, 371–383. [Google Scholar] [CrossRef]
- Nuzzo, I.; Losanno, D.; Cilento, F.; Caterino, N. Analytical and numerical modelling of shear-link device for seismic energy dissipation in frame structures. Eng. Struct. 2020, 214, 110630. [Google Scholar] [CrossRef]
- Zhang, C.; Zhu, T.; Wang, L.; Wu, M. Ultra-low cycle fatigue performance evaluation of the miniaturized low yield strength steel shear panel damper. J. Constr. Steel Res. 2017, 135, 277–284. [Google Scholar] [CrossRef]
- Guo, L.; Wang, J.; Wang, W.; Wang, H. Experimental, numerical and analytical study on seismic performance of shear-bending yielding coupling dampers. Eng. Struct. 2021, 244, 112724. [Google Scholar] [CrossRef]
- Park, H.Y.; Kim, J.; Kuwahara, S. Cyclic behavior of shear-type hysteretic dampers with different cross-sectional shapes. J. Constr. Steel Res. 2021, 187, 106964. [Google Scholar] [CrossRef]
- Nuzzo, I.; Losanno, D.; Caterino, N.; Serino, G.; Bozzo Rotondo, L.M. Experimental and analytical characterization of steel shear links for seismic energy dissipation. Eng. Struct. 2018, 172, 405–418. [Google Scholar] [CrossRef]
- Yadav, D.; Sahoo, D.R. Validation of hysteretic behavior and prediction of energy dissipation potential of aluminium shear yielding devices. Int. J. Mech. Sci. 2021, 194, 106204. [Google Scholar] [CrossRef]
- Wang, W.; Song, J.l.; Su, S.Q.; Cai, H.l.; Zhang, R.F. Experimental and numerical studies of an axial tension-compression corrugated steel plate damper. Thin-Walled Struct. 2021, 163, 107498. [Google Scholar] [CrossRef]
- Bai, J.; Huang, J.; Duan, L.; Cui, G.; Li, F.; Zhu, L. Seismic performance of a shear-type energy dissipation brace: Experimental investigation and numerical analysis. Eng. Struct. 2022, 273, 115011. [Google Scholar] [CrossRef]
- Kim, J.; Eom, T.S.; Kim, S.B.; Park, H.Y. Mechanical characteristics of H-shaped shear panel damper fabricated by SPHC steel according to stiffener reinforcement and stud support installation. Structures 2022, 42, 563–574. [Google Scholar] [CrossRef]
- Montazeri, E.; Kazemi, S.M.; Askariani, S.S. Finite element parametric study on the cyclic response of steel frames equipped with comb-teeth dampers. Structures 2021, 31, 111–126. [Google Scholar] [CrossRef]
- Huynh, N.T.; Nguyen, T.V.T.; Tam, N.T.; Nguyen, Q.M. Optimizing Magnification Ratio for the Flexible Hinge Displacement Amplifier Mechanism Design. In Lecture Notes in Mechanical Engineering. Proceedings of the 2nd Annual International Conference on Material, Machines and Methods for Sustainable Development (MMMS2020); Long, B.T., Kim, H.S., Ishizaki, K., Toan, N.D., Parinov, I.A., Kim, Y.H., Eds.; Springer International Publishing: Cham, Switzerland, 2021; Volume 2021, pp. 769–778. [Google Scholar] [CrossRef]
- Chau, N.L.; Dao, T.P.; Nguyen, V.T.T. An Efficient Hybrid Approach of Finite Element Method, Artificial Neural Network-Based Multiobjective Genetic Algorithm for Computational Optimization of a Linear Compliant Mechanism of Nanoindentation Tester. Math. Probl. Eng. 2018, 2018, 7070868. [Google Scholar] [CrossRef]
- Chen, Y.; Chen, C.; Jiang, H.; Liu, T.; Wan, Z. Study of an innovative graded yield metal damper. J. Constr. Steel Res. 2019, 160, 240–254. [Google Scholar] [CrossRef]
- Keykhosro Kiani, B.; Hosseini Hashemi, B. Development of a double-stage yielding damper with vertical shear links. Eng. Struct. 2021, 246, 112959. [Google Scholar] [CrossRef]
- Pan, P.; Cao, Y.; Wang, H.; Sun, J. Development of double-stage yielding coupling beam damper. J. Constr. Steel Res. 2020, 172, 106147. [Google Scholar] [CrossRef]
- Ahmadi, Z.; Aghakouchak, A.A.; Mirghaderi, S.R. Cyclic behavior of moment frames with conventional or slit Infills. J. Constr. Steel Res. 2021, 178, 106494. [Google Scholar] [CrossRef]
- Quan, C.; Wang, W.; Li, Y. Hysteretic model and resilient application of corrugated shear panel dampers. Thin-Walled Struct. 2022, 178, 109477. [Google Scholar] [CrossRef]
- Ahmadie Amiri, H.; Najafabadi, E.P.; Estekanchi, H.E. Experimental and analytical study of Block Slit Damper. J. Constr. Steel Res. 2018, 141, 167–178. [Google Scholar] [CrossRef]
- Ghandil, M.; Riahi, H.T.; Behnamfar, F. Introduction of a new metallic-yielding pistonic damper for seismic control of structures. J. Constr. Steel Res. 2022, 194, 107299. [Google Scholar] [CrossRef]
- Lie, W.; Wu, C.; Luo, W.; Wu, C.; Li, C.; Li, D.; Wu, C. Cyclic behaviour of a novel torsional steel-tube damper. J. Constr. Steel Res. 2022, 188, 107010. [Google Scholar] [CrossRef]
- Giannuzzi, D.; Ballarini, R.; Huckelbridge, A.; Pollino, M.; Valente, M. Braced Ductile Shear Panel: New Seismic-Resistant Framing System. J. Struct. Eng. 2014, 140, 04013050. [Google Scholar] [CrossRef]
- Ye, J.; Yan, G.; Liu, R.; Xue, P.; Wang, D. Hysteretic behavior of replaceable steel plate damper for prefabricated joint with earthquake resilience. J. Build. Eng. 2022, 46, 103714. [Google Scholar] [CrossRef]
- Wang, J.; Men, J.; Zhang, Q.; Fan, D.; Zhang, Z.; Huang, C.H. Seismic performance evaluation of a novel shape-optimized composite metallic yielding damper. Eng. Struct. 2022, 268, 114714. [Google Scholar] [CrossRef]
- Valente, M.; Castiglioni, C.A.; Kanyilmaz, A. Welded fuses for dissipative beam-to-column connections of composite steel frames: Numerical analyses. J. Constr. Steel Res. 2017, 128, 498–511. [Google Scholar] [CrossRef]
- Xu, L.Y.; Fan, J.S.; Yang, Y.; Wang, C. Ratcheting assessment of low yield point steel BLY160: Experimental analysis and constitutive modelling. Mech. Mater. 2020, 148, 103460. [Google Scholar] [CrossRef]
- Gao, Y.; Shi, G.; Wang, X. Mechanical properties of low yield point steels subjected to low-cycle structural damage. J. Constr. Steel Res. 2021, 183, 106733. [Google Scholar] [CrossRef]
- Ghaedi, K.; Ibrahim, Z.; Javanmardi, A.; Rupakhety, R. Experimental Study of a New Bar Damper Device for Vibration Control of Structures Subjected to Earthquake Loads. J. Earthq. Eng. 2021, 25, 300–318. [Google Scholar] [CrossRef]
- Benavent-Climent, A.; Escolano-Margarit, D.; Arcos-Espada, J.; Ponce-Parra, H. New metallic damper with multiphase behavior for seismic protection of structures. Metals 2021, 11, 183. [Google Scholar] [CrossRef]
- Rahnavard, R.; Hassanipour, A.; Mounesi, A. Numerical study on important parameters of composite steel-concrete shear walls. J. Constr. Steel Res. 2016, 121, 441–456. [Google Scholar] [CrossRef]
- Morch, H.; Duchêne, L.; Harzallah, R.; Tuninetti, V.; Habraken, A.M. Efficient temperature dependence of parameters for thermo-mechanical finite element modeling of alloy 230. Eur. J. Mech. A/Solids 2021, 85, 104116. [Google Scholar] [CrossRef]
- Chaboche, J.L. Constitutive equations for cyclic plasticity and cyclic viscoplasticity. Int. J. Plast. 1989, 5, 247–302. [Google Scholar] [CrossRef]
- ABAQUS Inc. Chapter Models for metals Subjected to Cyclic Loading. In ABAQUS Theory Manual Version 6.7. Available online: http://130.149.89.49:2080/v6.7/books/usb/default.htm?startat=pt05ch18s02abm16.html (accessed on 13 March 2023).
- ANSYS Inc. ANSYS Mechanical APDL Material Reference, Release 15.0; ANSYS Inc.: Canonsburg, PA, USA, 2013. [Google Scholar]
- Briones, B.; de la Llera, J.C. Analysis, design and testing of an hourglass-shaped copper energy dissipation device. Eng. Struct. 2014, 79, 309–321. [Google Scholar] [CrossRef]
- ANSYS Inc. ANSYS Mechanical Help System, Release 19.3, Rate-Independet Plasticity; ANSYS Inc.: Canonsburg, PA, USA, 2019. [Google Scholar]
- ANSYS Inc. ANSYS Mechanical APDL Command Reference, Release 13.0; ANSYS Inc.: Canonsburg, PA, USA, 2010. [Google Scholar]
- Keykhosro Kiani, B.; Hosseini Hashemi, B.; Torabian, S. Optimization of slit dampers to improve energy dissipation capacity and low-cycle-fatigue performance. Eng. Struct. 2020, 214, 110609. [Google Scholar] [CrossRef]
- Chopra, A.K. Dynamics of Structures: Theory and Applications to Earthquake Engineering; Prentice-Hall: Hoboken, NJ, USA, 2001. [Google Scholar]
- Zhao, B.; Lu, B.; Zeng, X.; Gu, Q. Experimental and numerical study of hysteretic performance of new brace type damper. J. Constr. Steel Res. 2021, 183, 106717. [Google Scholar] [CrossRef]
- Lin, X.; Li, H.; He, L.; Zhang, L. Experimental study on seismic behavior of the damage-control steel plate fuses for beam-to-column connection. Eng. Struct. 2022, 270, 114862. [Google Scholar] [CrossRef]








| Shear Damper | Material | Dimensions of the Damper (mm) * | Cyclic Loading Protocol | Effective Damping | Max. Force (kN) | Max. Displacement or Strain | |
|---|---|---|---|---|---|---|---|
| Type | (MPa) | ||||||
| HHSD [12] | Steel | 301 | H = 95–185, L = 207–228, D = 8–12, and thickness in the plane of 5–10 | Incremental protocol with 3 cycles at each displacement value | Value | 20–100 | 10–17 (mm) |
| Shear Square Section Steel Tube Damper [11] | Q235 Steel | 238–243 | Square tubes with side of 150–200, length of 150–250 and thickness of 3–5 | Value | 64–339 | 2.6–17.5 (mm) | |
| SL [18,22] | A36 Steel | 342 | H = 310, L = 300–500, D = 19 | Graph | 396–1030 | 19–25.8 (mm) | |
| Al-SYD [23] | Al-6063/ Al-1100 | 33.5/27 | H = 100, L = 152.4, D = 4.5–7.6 | Incremental protocol, with 3 cycles at each shear strain value | Value | 60–259 | 15% shear strain |
| TLYD [17] | Lead | ND | Each lead ring has a length of 60, a radius of 35, and thickness between 8 and 16 | Incremental protocol, with 2 cycles at each displacement value | Graph | 250–500 | 70 (mm) |
| ATCCSPD [24] | Q235 Steel | 260–283 | dimensions of each plate H = 260, L = 130–234, D = 3–5 | Starts with load control and then incremental displacement, with 3 cycles at each displacement value | Graph | 344–579 | 29–36 (mm) |
| SEDB [25] | Q235B Steel | 215 | Flat steel plate H = 120, L = 100, D = 5; Corrugated steel plate H = 264, L = 100, D = 4 mm | Graph | 500–750 | 10–17 (mm) | |
| LYSPD [19] | Steel | 100 | H = 120, L = 120, D = 6–12 mm | 2 protocols: incremental and constant strain amplitud | ND ** | 180–360 | 60% shear strain |
| MSD [15] | Steel | 242 | H = 3000, L = 800, D = 300 | Incremental protocol with a variable quantity of cycles at each displacement value | ND ** | 430 | 120 (mm) |
| HSPD [26] | SPHC Steel | 212 | H = 210, dimensions of cross-sectional H-shape 222 × 50 × 4 × 6 | Constant amplitude cyclic loading of = 5 % rad | ND ** | 113–125 | = 0.01 rad |
| CTD [27] | A36 Steel | 273 | H = 300, L = 800, D = 6–25 | Incremental displacement | Graph | 70–500 | 25–45 (mm) |
| Element Size (mm) | Nodes | Elements | (kN) | (kN) | Time (s) | Error of (%) | Error of (%) |
|---|---|---|---|---|---|---|---|
| 4 | 14,756 | 2175 | 13.16 | 13.42 | 56 | 10.67 | 9.85 |
| 3 | 26,787 | 4196 | 12.27 | 12.58 | 103 | 3.16 | 2.92 |
| 2 | 67,637 | 11,985 | 12.09 | 12.42 | 251 | 1.67 | 1.60 |
| 1 | 409,785 | 84,430 | 11.89 | 12.22 | 1939 | - | - |
| Plate | Nodes | Elements | () | (mm) | (kN) | (kN) | (mm) | (kN) | (mm) | Cycles until Degradation | |
|---|---|---|---|---|---|---|---|---|---|---|---|
| HHSD n°1 | 67,637 | 11,985 | 8.924 | 1.00 | 8.639 | 16.135 | 10.0 | −16.373 | −10.0 | 10.0 | 18 |
| P1 | 80,410 | 13,070 | 112.240 | 0.38 | 40.513 | 60.904 | 2.5 | −63.000 | −2.0 | 6.5 | 5 |
| P2 | 69,941 | 11,370 | 68.057 | 0.40 | 25.726 | 43.841 | 5.0 | −44.113 | −5.0 | 12.5 | 10 |
| P3 | 58,185 | 9400 | 3.435 | 1.75 | 5.659 | 22.974 | 22.5 | −19.647 | −22.5 | 12.8 | 25 |
| P4 | 68,269 | 12,190 | 12.760 | 0.75 | 9.220 | 18.953 | 10.0 | −19.103 | −10.0 | 13.4 | 16 |
| P5 | 66,395 | 11,260 | 7.742 | 1.15 | 8.641 | 17.961 | 14.0 | −18.168 | −14.0 | 12.1 | 21 |
| Plate | Nodes | Elements | () | (mm) | (kN) | (kN) | (mm) | (kN) | (mm) | Cycles until Degradation | |
|---|---|---|---|---|---|---|---|---|---|---|---|
| P3-D8 | 48,029 | 7520 | 2.728 | 1.74 | 4.453 | 18.054 | 22.5 | −15.380 | −22.5 | 12.9 | 24 |
| P3 | 58,185 | 9400 | 3.435 | 1.75 | 5.659 | 22.974 | 22.5 | −19.647 | −22.5 | 12.8 | 25 |
| P3-D12 | 68,341 | 11,280 | 4.148 | 1.77 | 6.884 | 27.839 | 22.5 | −23.789 | −22.5 | 12.7 | 25 |
| P3-T1.5 | 77,666 | 13,805 | 11.678 | 1.17 | 12.983 | 34.669 | 17.5 | −34.297 | −17.5 | 15.0 | 23 |
| P3-T2 | 96,342 | 18,035 | 27.570 | 0.94 | 24.845 | 54.387 | 14.0 | −53.979 | −14.0 | 14.9 | 20 |
| P4-D8 | 56,332 | 9736 | 10.103 | 0.75 | 7.310 | 14.824 | 10.0 | −14.773 | −10.0 | 13.3 | 16 |
| P4 | 68,269 | 12,190 | 12.760 | 0.75 | 9.220 | 18.953 | 10.0 | −19.103 | −10.0 | 13.4 | 16 |
| P4-D12 | 80,299 | 14,634 | 15.264 | 0.77 | 11.318 | 23.001 | 10.0 | −22.668 | −10.0 | 13.0 | 16 |
| P4-T1.5 | 83,999 | 15,835 | 38.335 | 0.61 | 22.642 | 44.066 | 7.5 | −43.007 | −7.5 | 12.3 | 13 |
| P4-T2 | 107,547 | 21,065 | 78.641 | 0.55 | 41.643 | 79.421 | 7.5 | −75.436 | −5.0 | 13.7 | 12 |
| P5-D8 | 55,007 | 9052 | 6.230 | 1.13 | 6.767 | 14.142 | 14.0 | −14.302 | −14.0 | 12.4 | 21 |
| P5 | 66,395 | 11,260 | 7.742 | 1.15 | 8.641 | 17.961 | 14.0 | −18.168 | −14.0 | 12.1 | 21 |
| P5-D12 | 78,396 | 13,596 | 9.385 | 1.16 | 10.551 | 21.914 | 14.0 | −22.127 | −14.0 | 12.0 | 20 |
| P5-T1.5 | 97,680 | 18,390 | 23.620 | 0.87 | 19.812 | 41.616 | 14.0 | −39.082 | −10.0 | 16.2 | 18 |
| P5-T2 | 113,558 | 21,960 | 49.678 | 0.72 | 34.751 | 67.683 | 10.0 | −65.182 | −7.5 | 13.8 | 15 |
| Plate | Nodes | Elements | () | (mm) | (kN) | (kN) | (mm) | (kN) | (mm) | Dissipation Rate | |
|---|---|---|---|---|---|---|---|---|---|---|---|
| P4-1d * | 68,269 | 12,190 | 12.760 | 0.75 | 9.220 | 18.953 | 10.0 | −19.103 | −10.0 | 13.4 | 0.03173 |
| P4-2d | 93,938 | 16,810 | 19.455 | 0.73 | 13.670 | 27.874 | 10.0 | −27.855 | −10.0 | 13.8 | 0.03440 |
| P4-3d | 118,934 | 21,280 | 26.301 | 0.71 | 18.142 | 36.927 | 10.0 | −37.069 | −10.0 | 14.0 | 0.03600 |
| P5-1d | 53,208 | 8980 | 5.802 | 1.18 | 6.589 | 13.714 | 14.0 | −13.884 | −14.0 | 11.9 | 0.05049 |
| P5-2d * | 66,395 | 11,260 | 7.742 | 1.15 | 8.641 | 17.961 | 14.0 | −18.168 | −14.0 | 12.1 | 0.05407 |
| P5-3d | 79,069 | 13,410 | 9.713 | 1.14 | 10.696 | 22.297 | 14.0 | −22.530 | −14.0 | 12.3 | 0.05515 |
| HHSD-0d * | 67,637 | 11,985 | 8.924 | 1.00 | 8.639 | 16.135 | 10.0 | −16.373 | −10.0 | 10.0 | 0.03484 |
| HHSD-1d | 92,465 | 16,405 | 14.572 | 0.81 | 11.355 | 23.228 | 10.0 | −23.291 | −10.0 | 12.4 | 0.03201 |
| HHSD-2d | 117,460 | 20,865 | 19.521 | 0.78 | 14.785 | 30.408 | 10.0 | −30.479 | −10.0 | 12.8 | 0.03312 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Bustos, F.; Hinojosa, J.; Tuninetti, V. Computational Comparison of Performance of Different Steel Plate Shear Yielding Dampers. Buildings 2023, 13, 793. https://doi.org/10.3390/buildings13030793
Bustos F, Hinojosa J, Tuninetti V. Computational Comparison of Performance of Different Steel Plate Shear Yielding Dampers. Buildings. 2023; 13(3):793. https://doi.org/10.3390/buildings13030793
Chicago/Turabian StyleBustos, Flavia, Jorge Hinojosa, and Víctor Tuninetti. 2023. "Computational Comparison of Performance of Different Steel Plate Shear Yielding Dampers" Buildings 13, no. 3: 793. https://doi.org/10.3390/buildings13030793
APA StyleBustos, F., Hinojosa, J., & Tuninetti, V. (2023). Computational Comparison of Performance of Different Steel Plate Shear Yielding Dampers. Buildings, 13(3), 793. https://doi.org/10.3390/buildings13030793







