Mechanical Performance of Concrete Segment Lining Structure of Shield Tunneling in Different Strata
Abstract
:1. Introduction
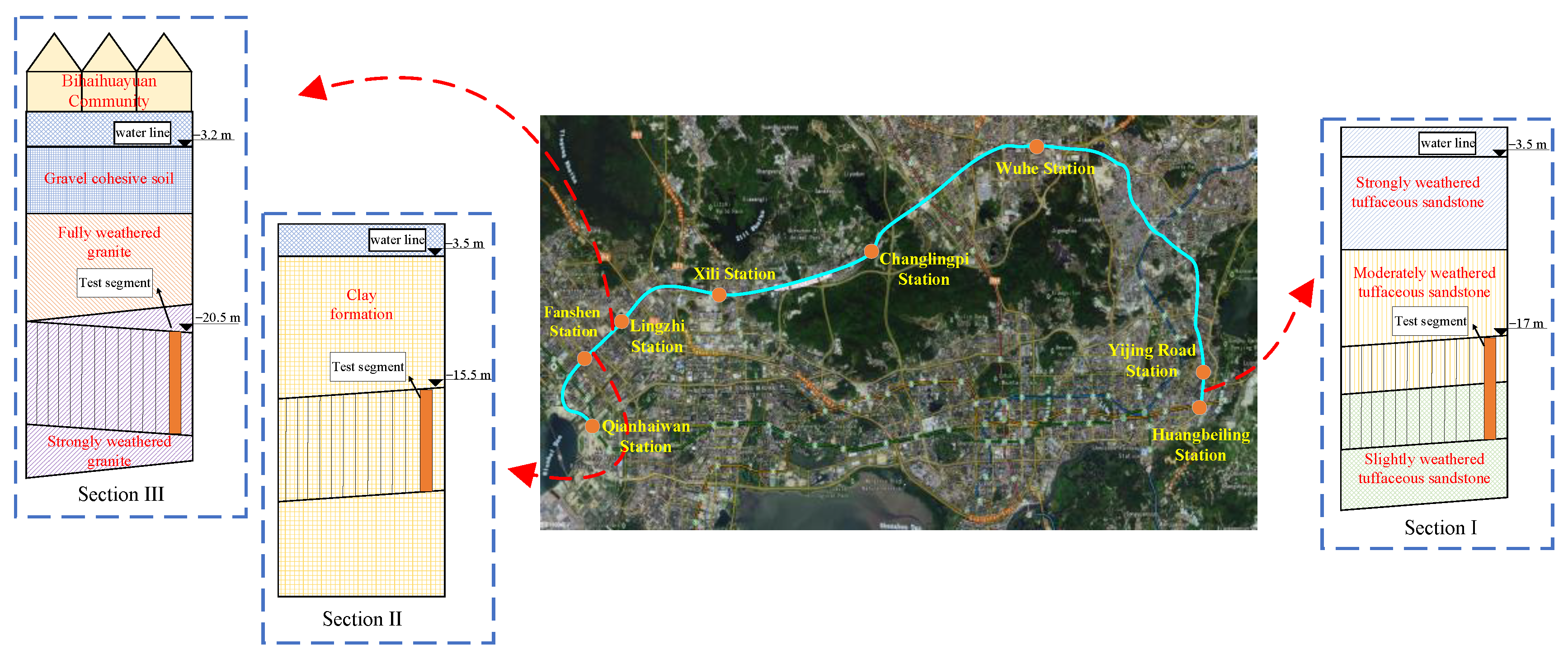
2. Project Overview
3. Test Preparation, Component Installation and Data Collection
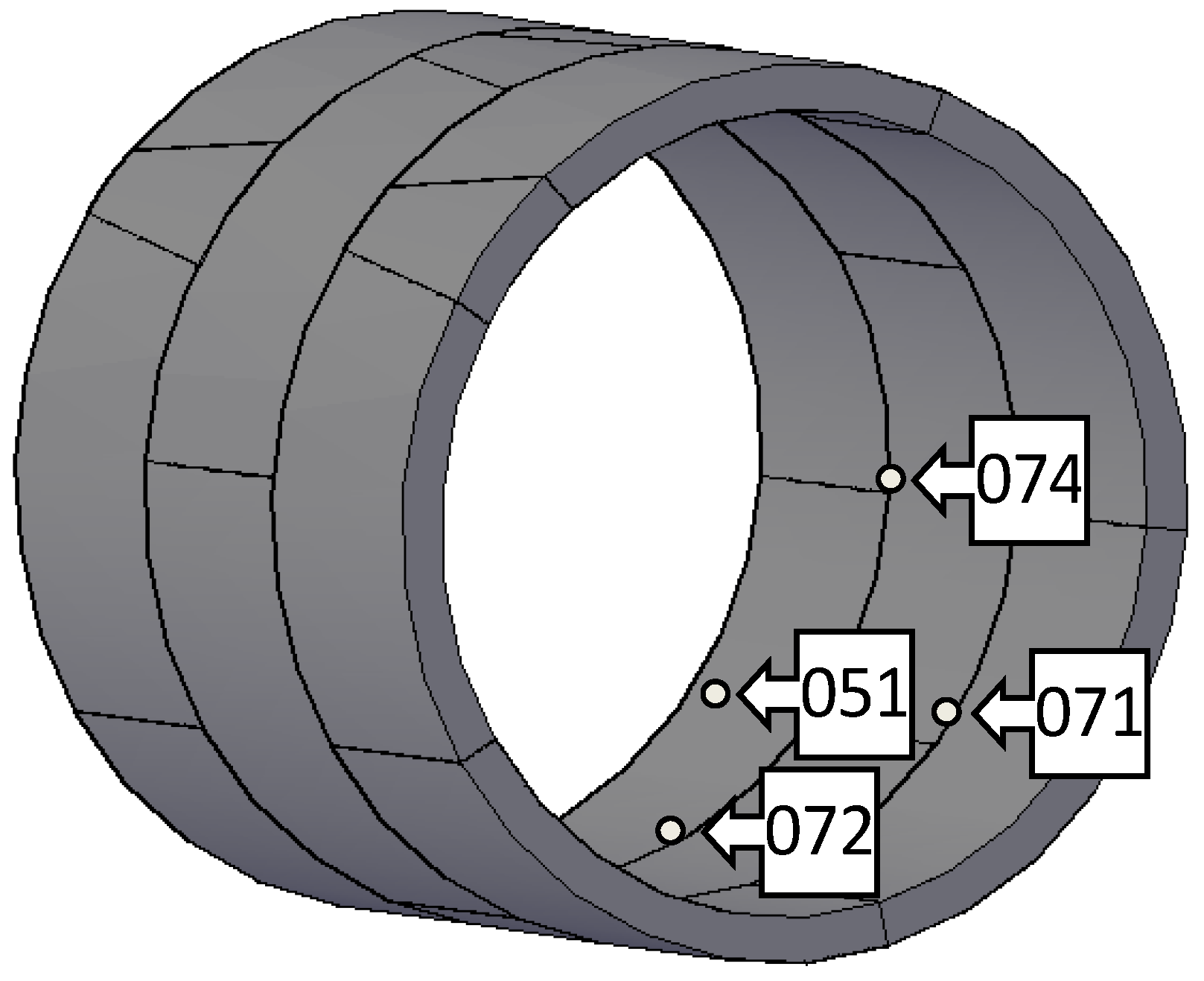
3.1. Selection of Test Sections
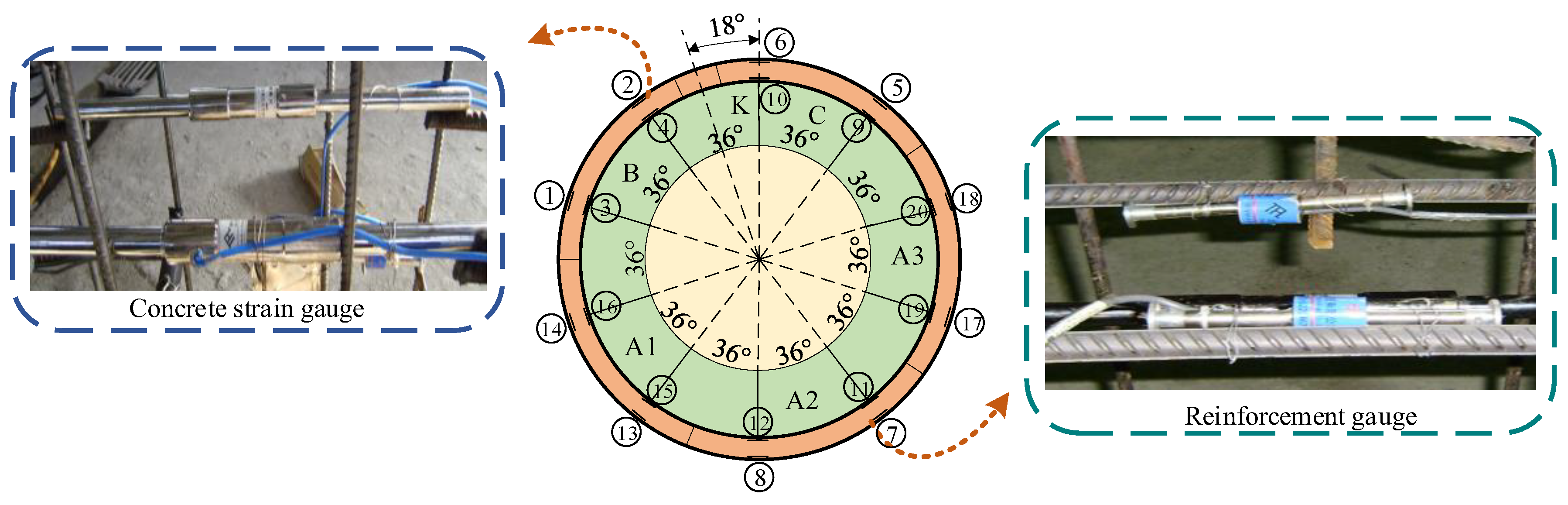
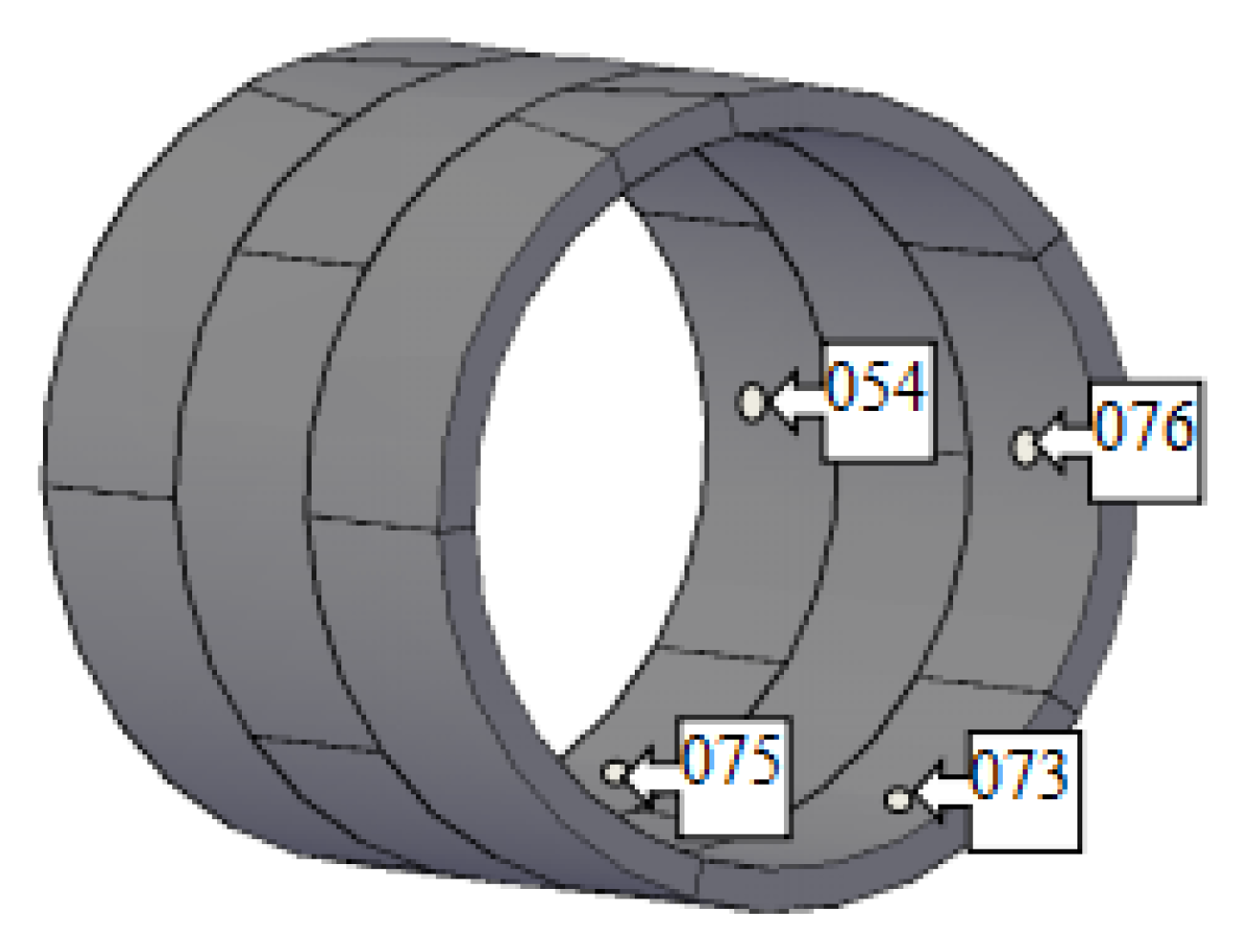
3.2. Installation of Test Components
3.3. Test Segment Data Collection
- (1)
- It is assumed that the circumferential joints of the segment have no influence on the force of the segment, and the force between the segments is continuous;
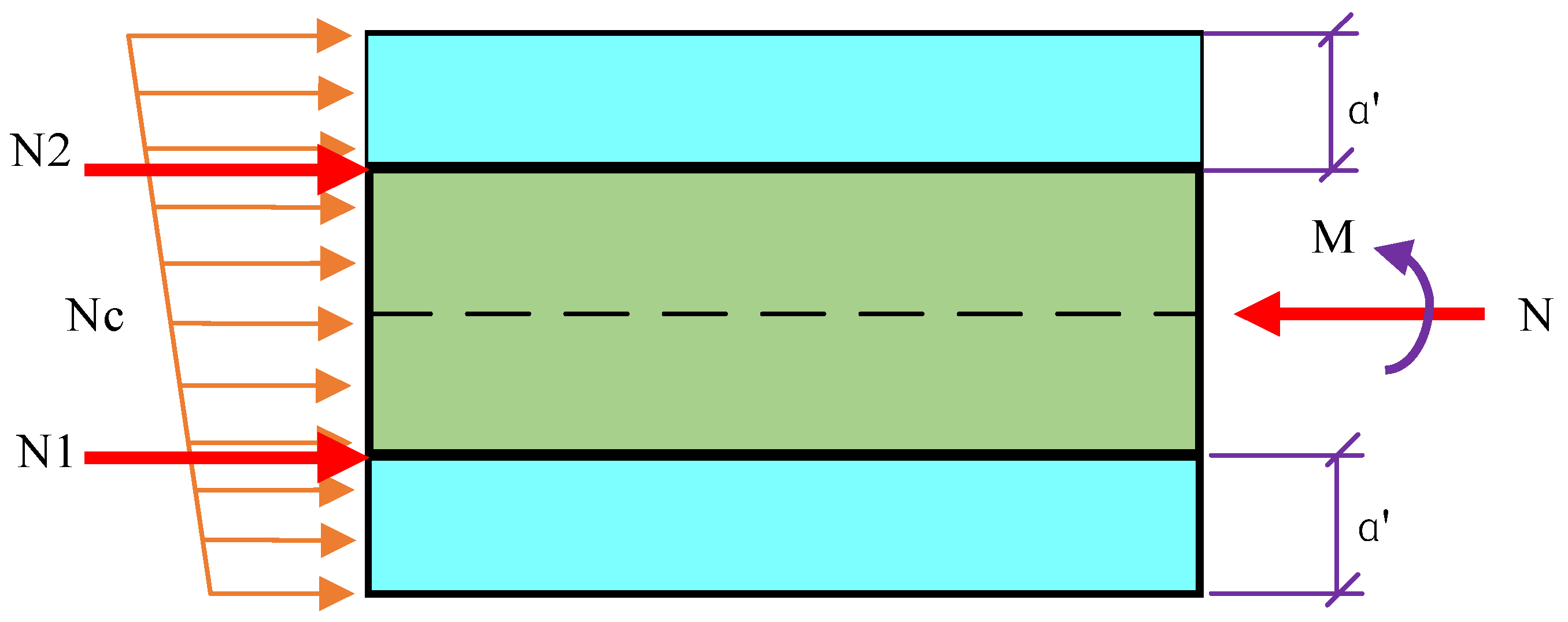
- (2)
- Take a calculation unit in the circumferential direction of the segment and simplify the arc segment into a rectangular unit.
4. Test Results and Analysis
4.1. Soft and Hard Uneven Formation
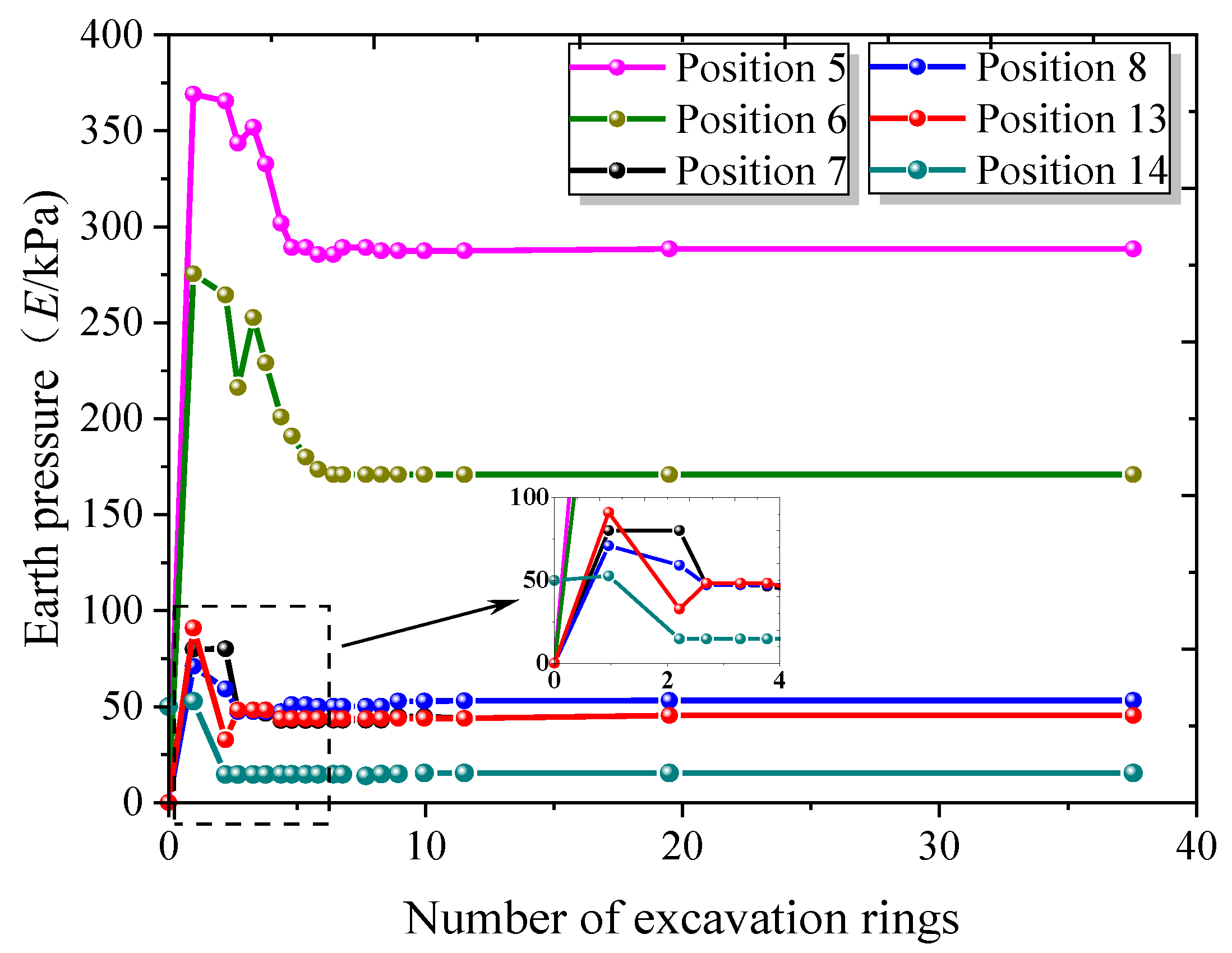
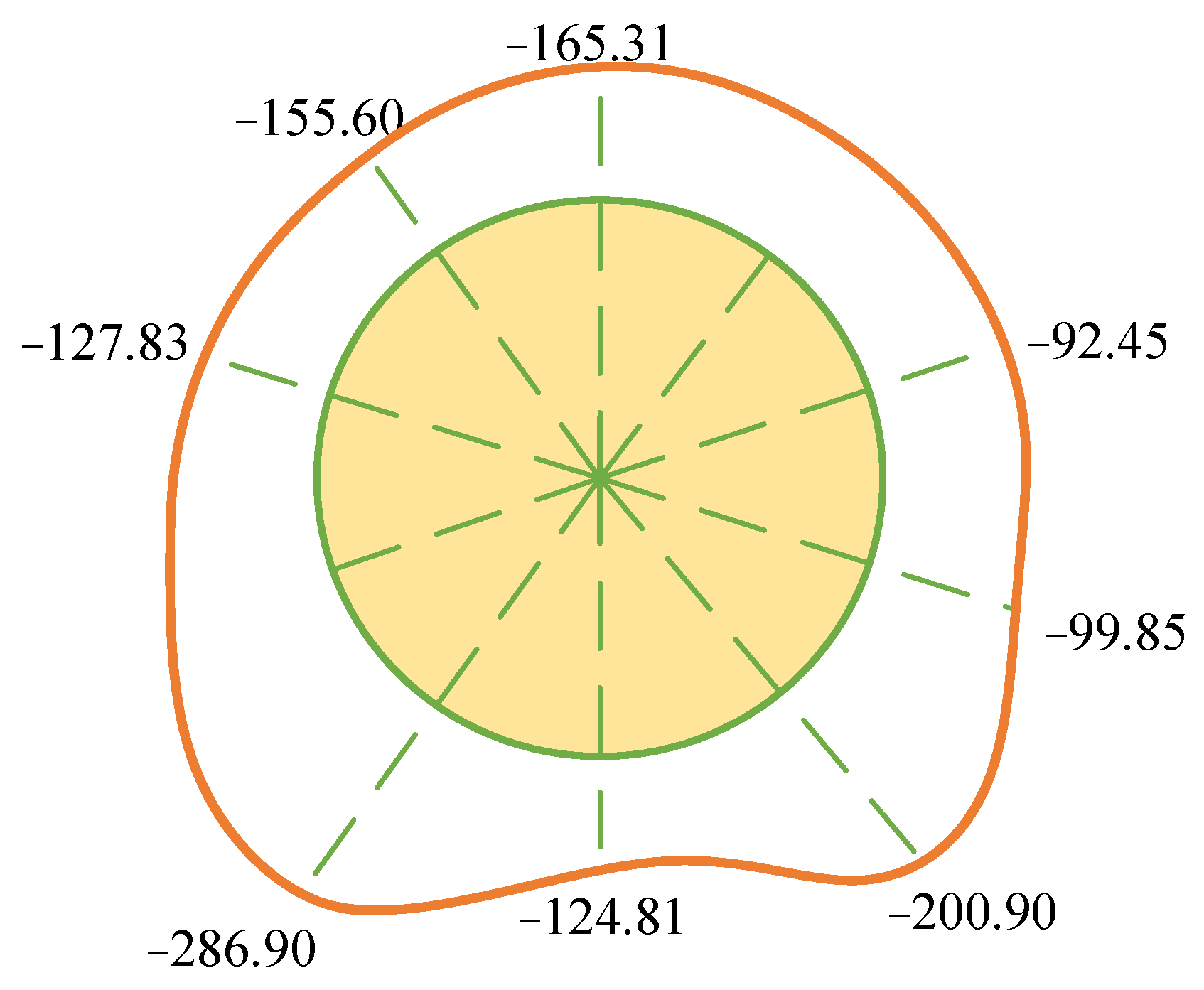
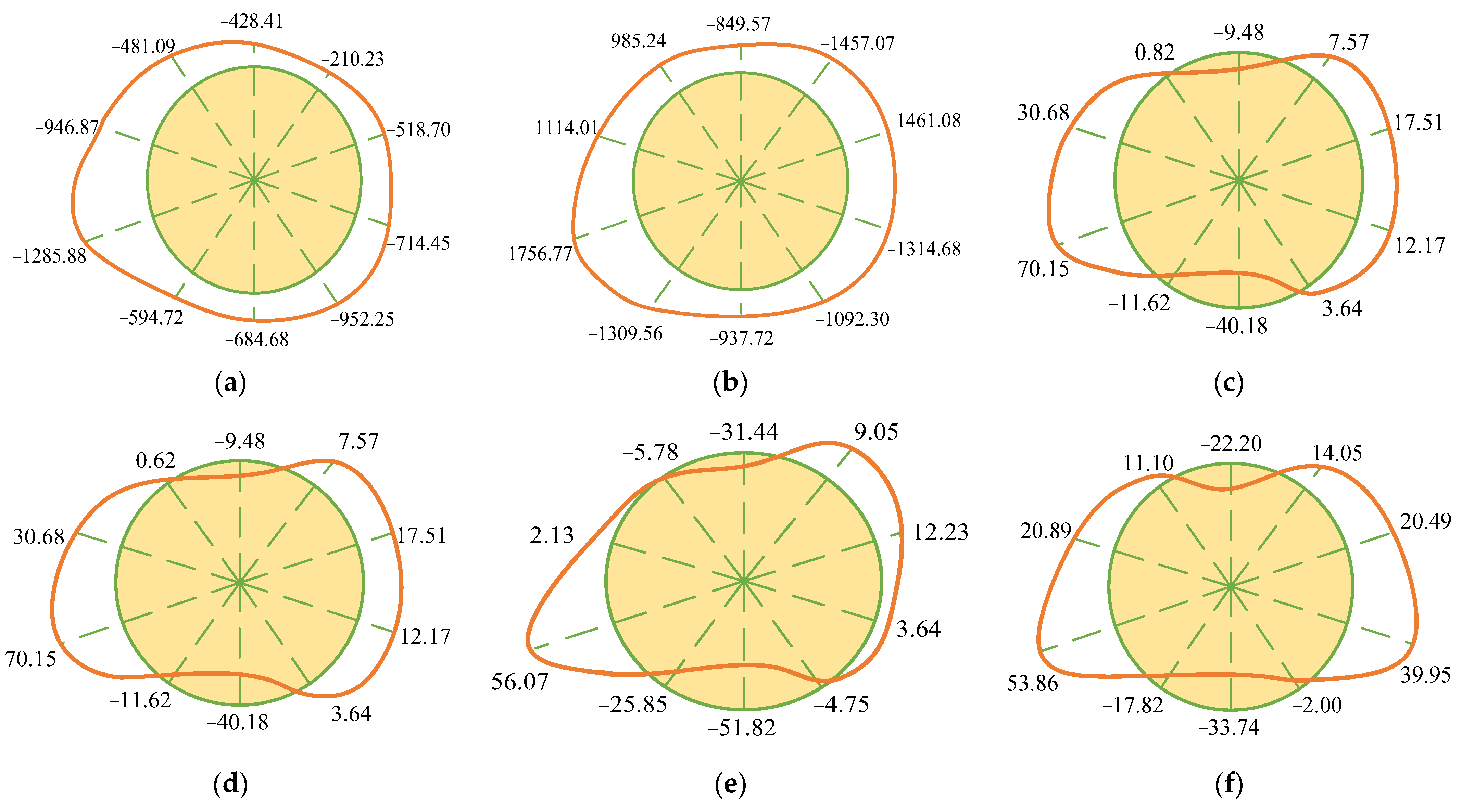
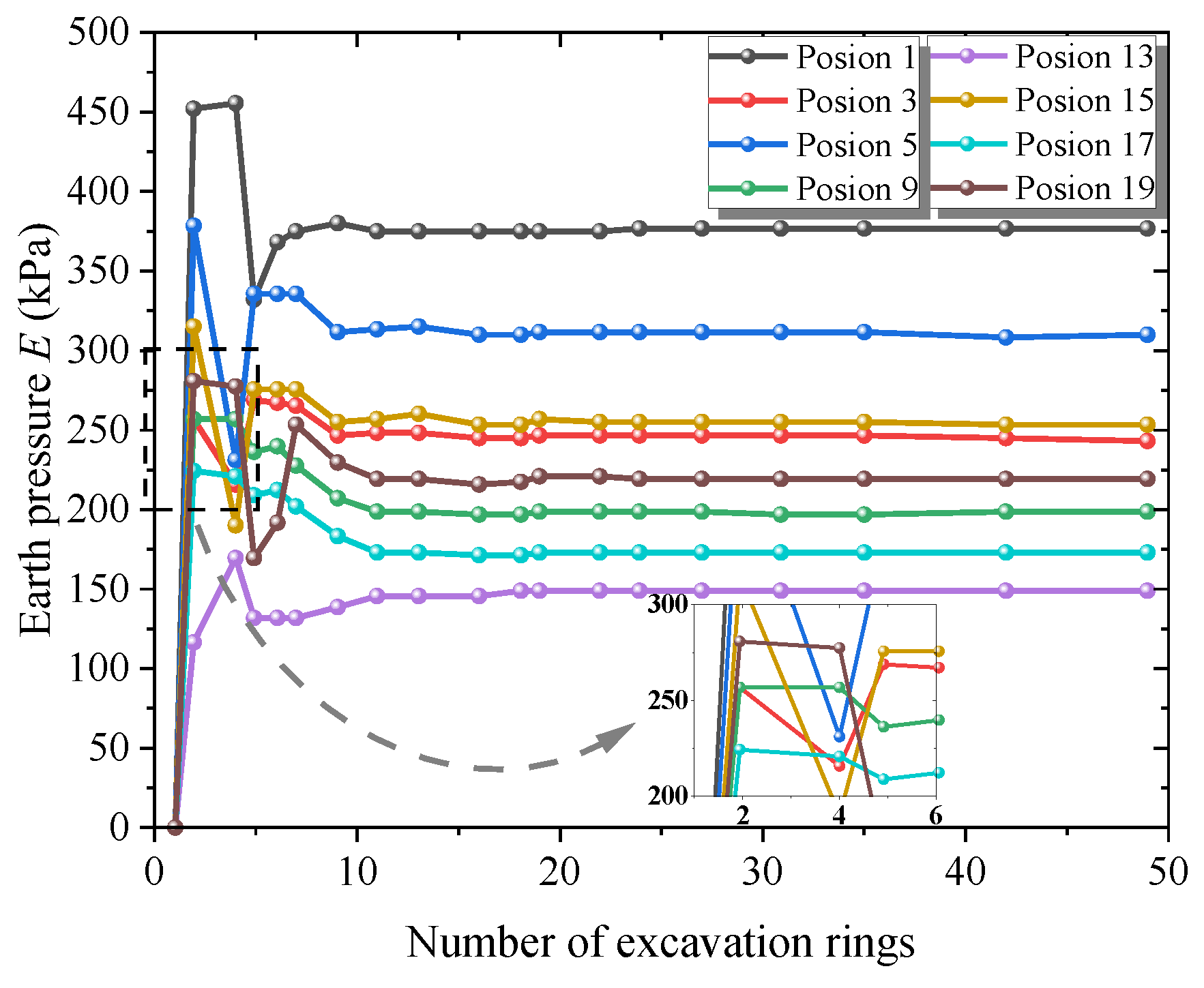
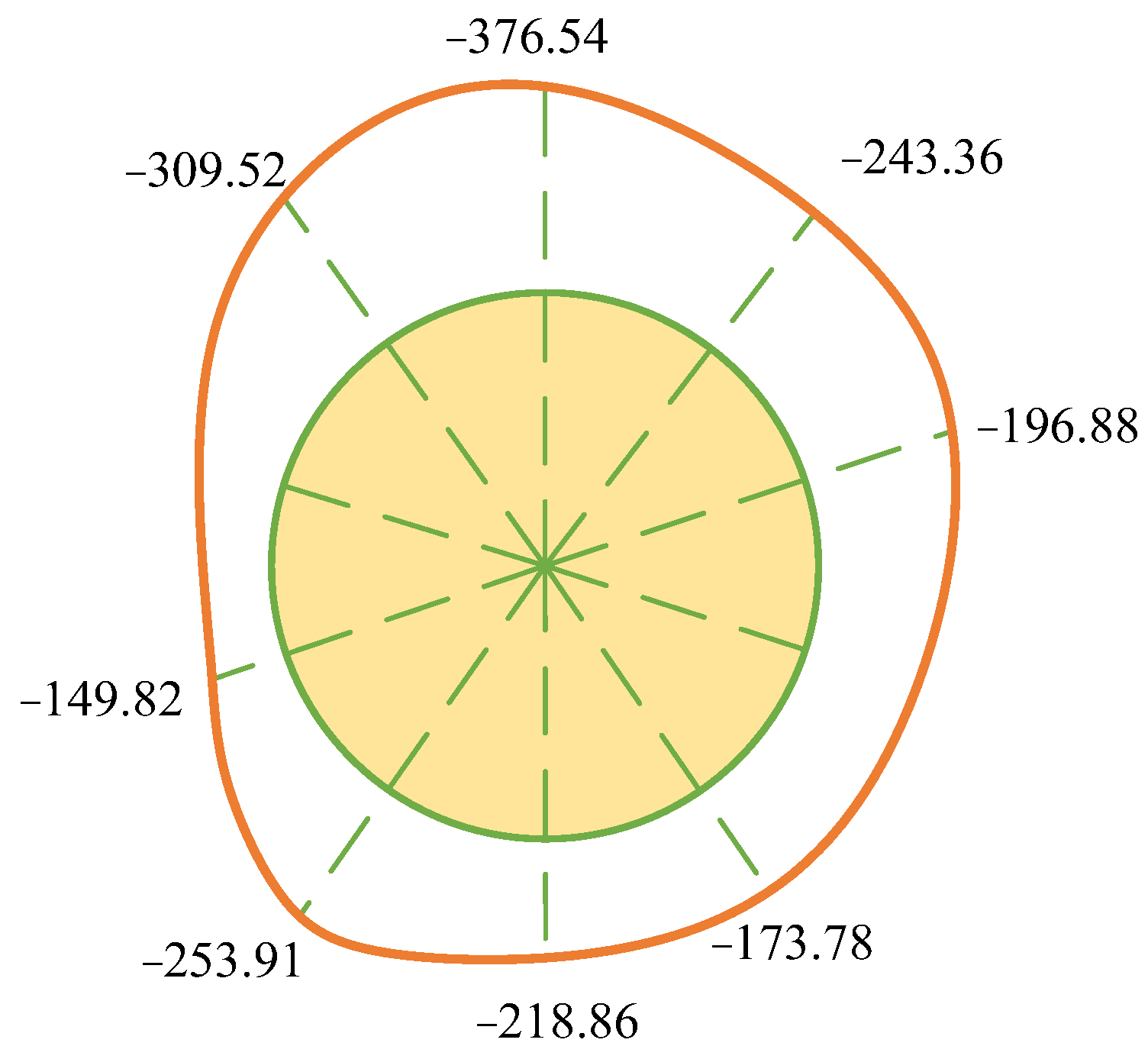
4.1.1. Distribution Law of Soil Pressure on Pipe Segments
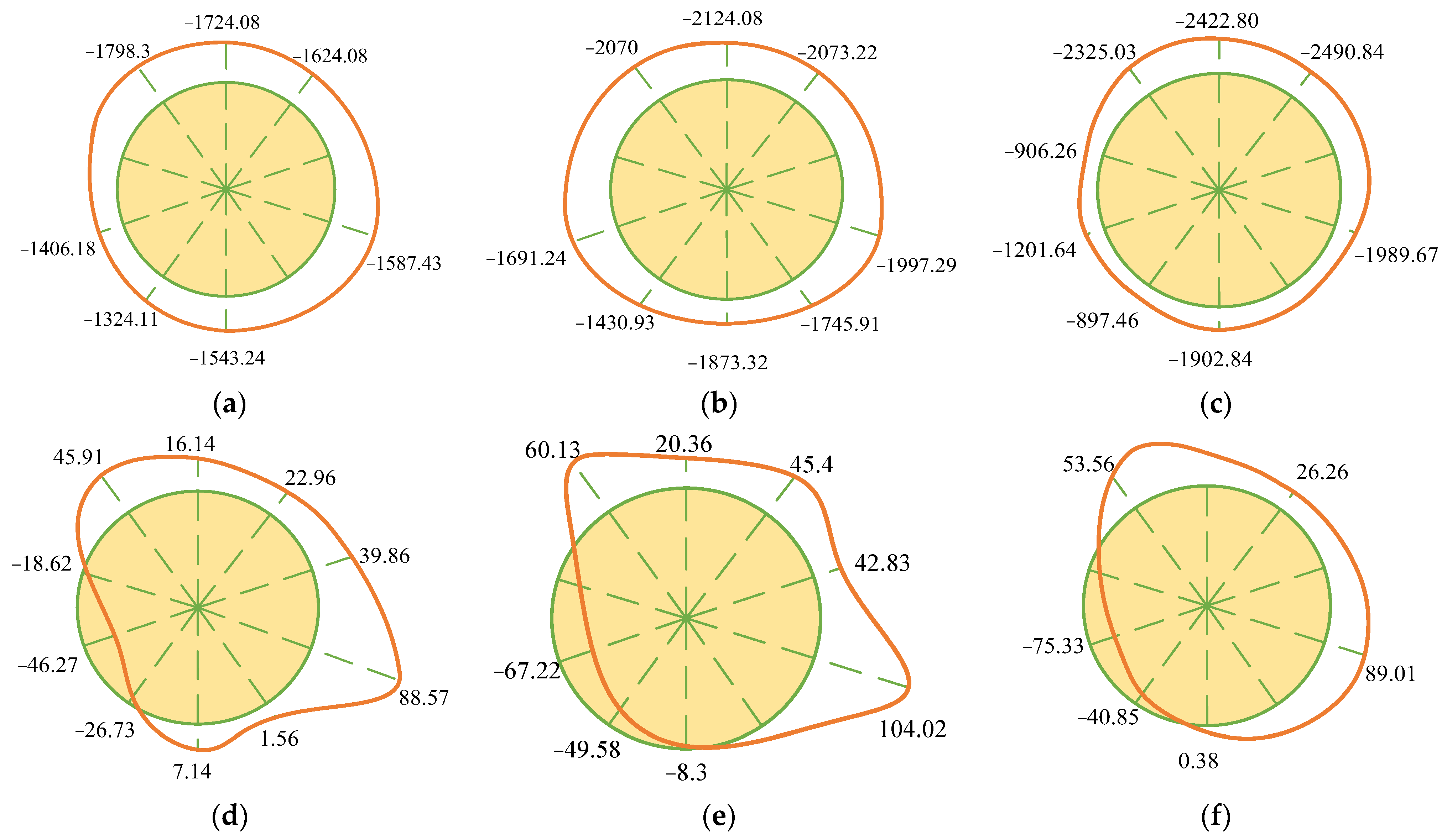
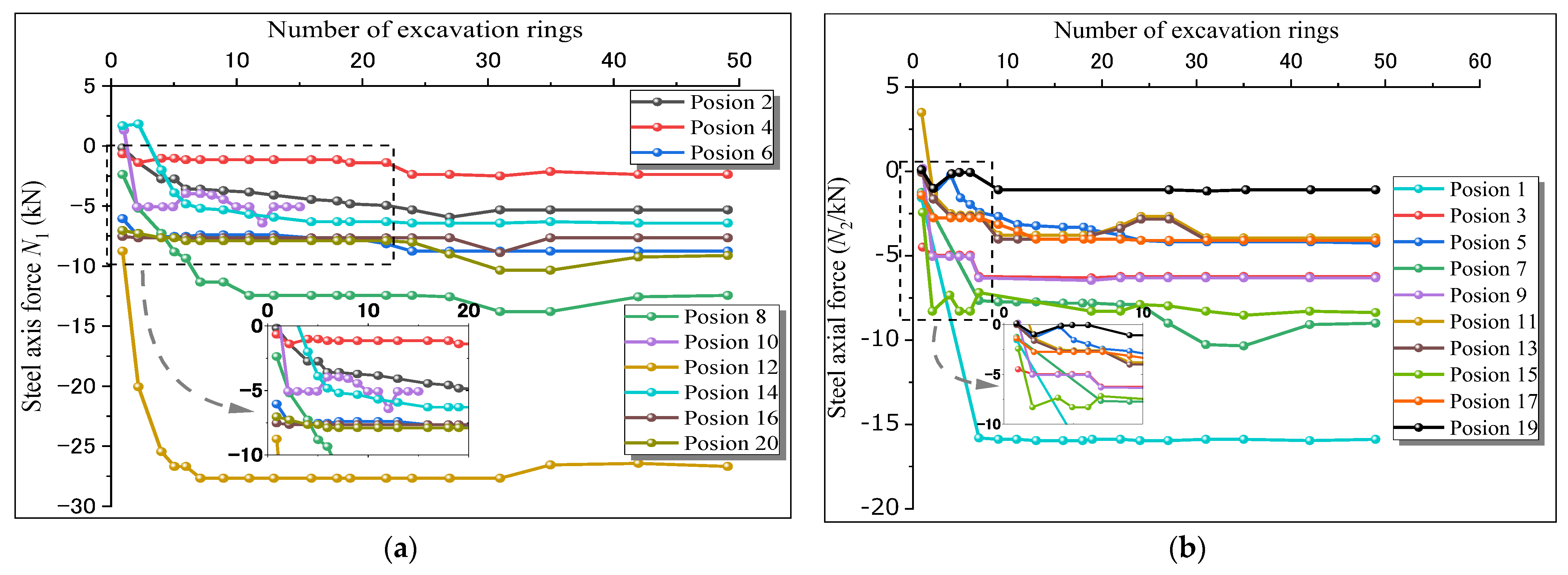
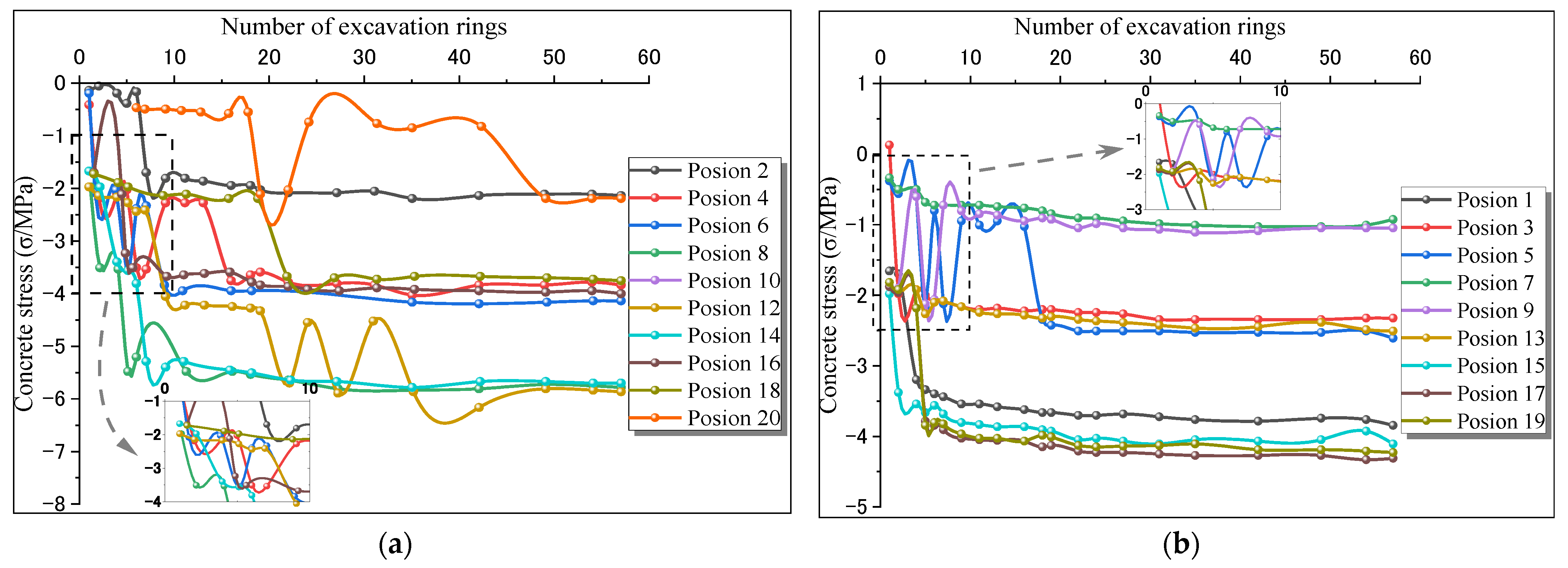
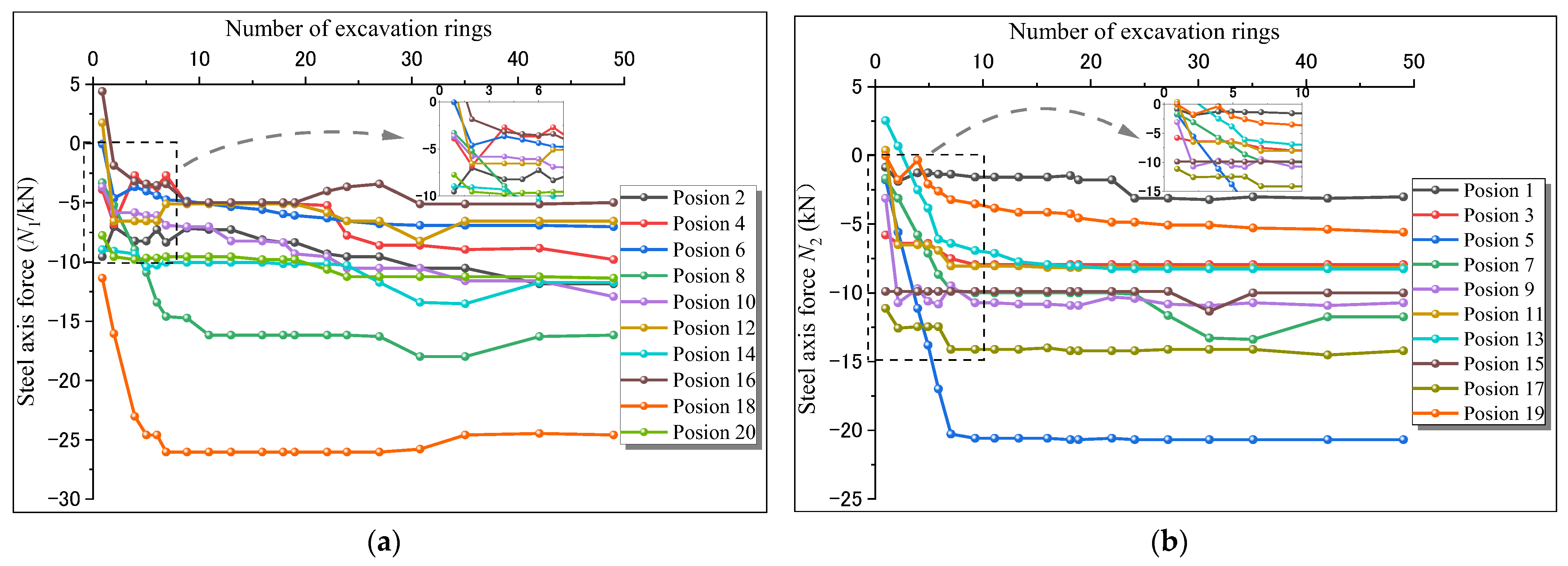
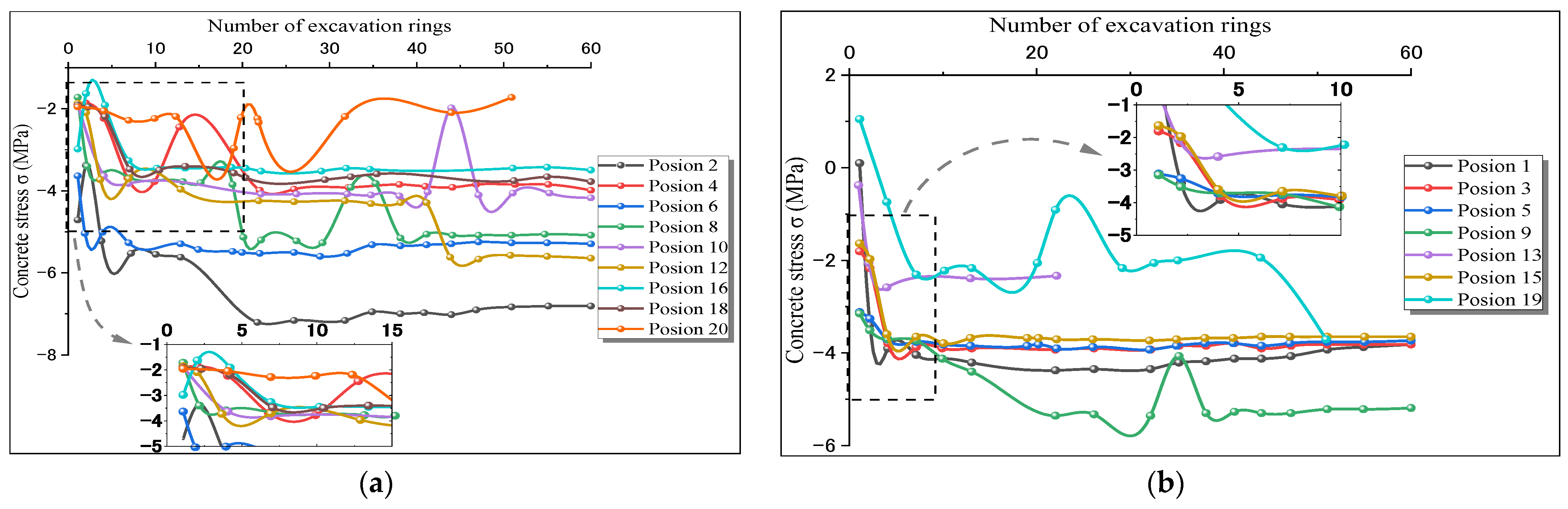
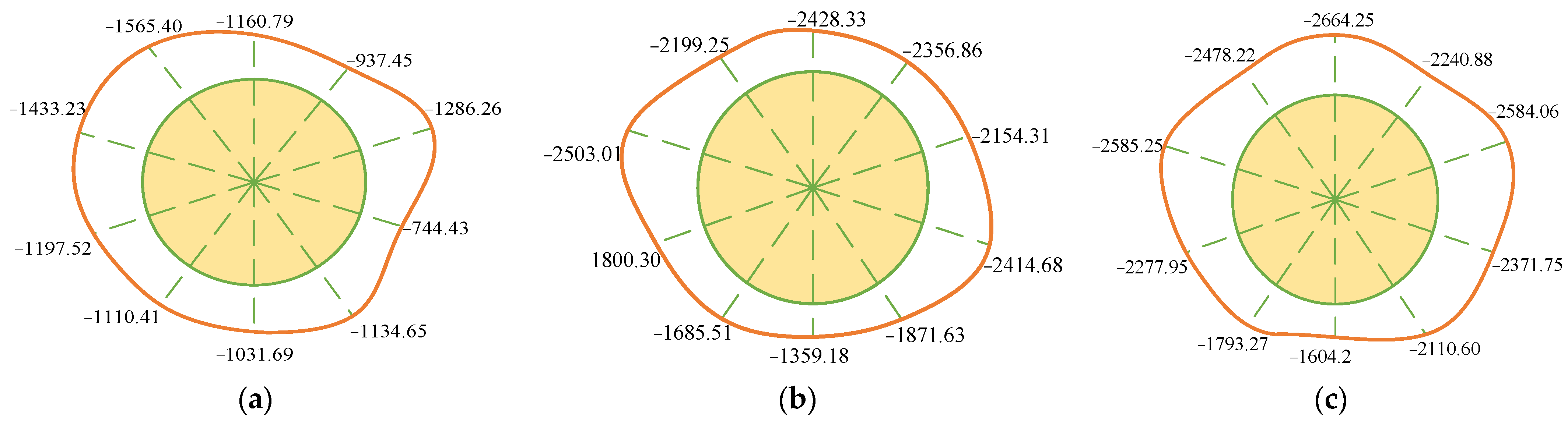
4.1.2. Distribution Rules of Internal Forces in Segments
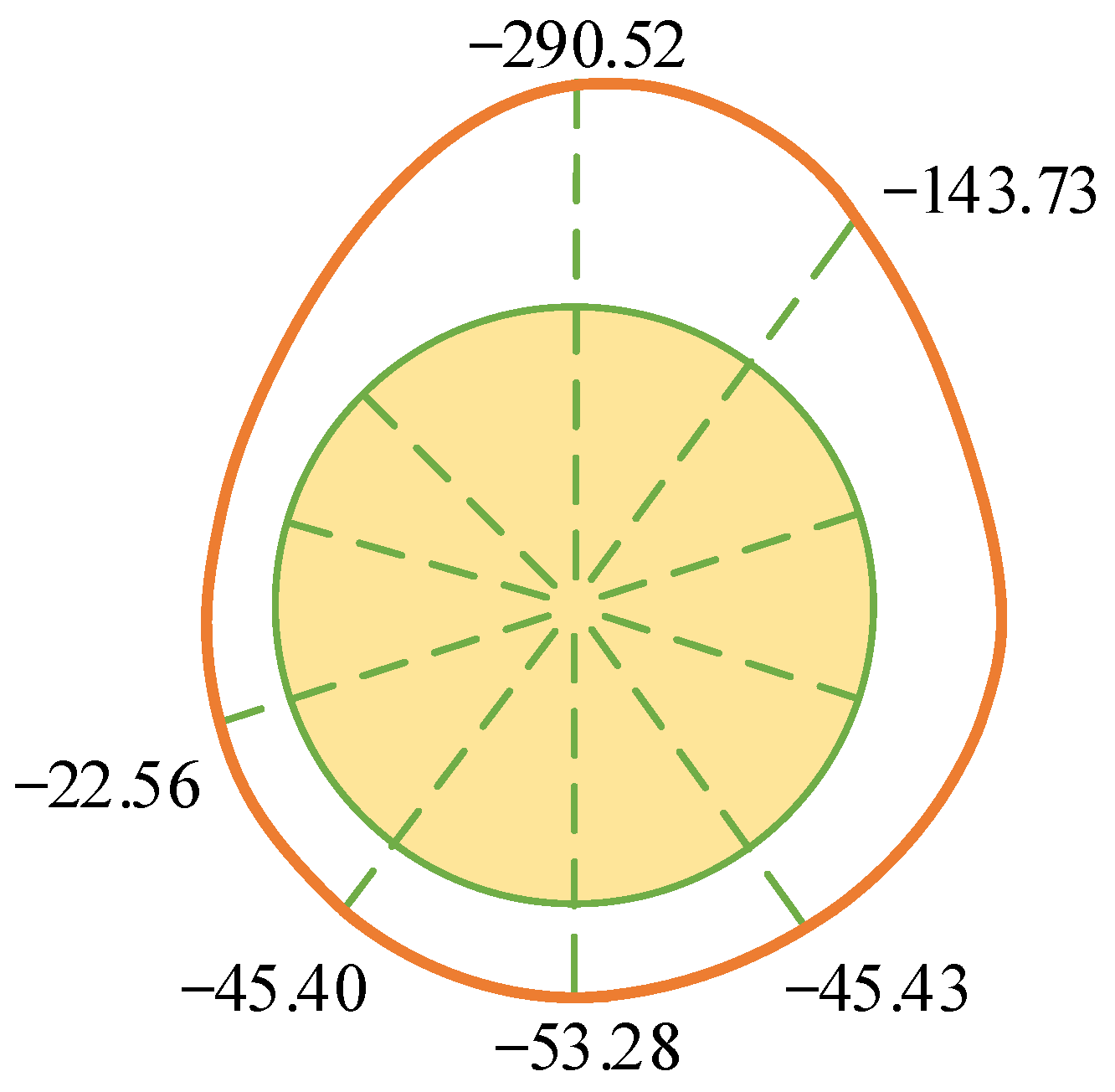
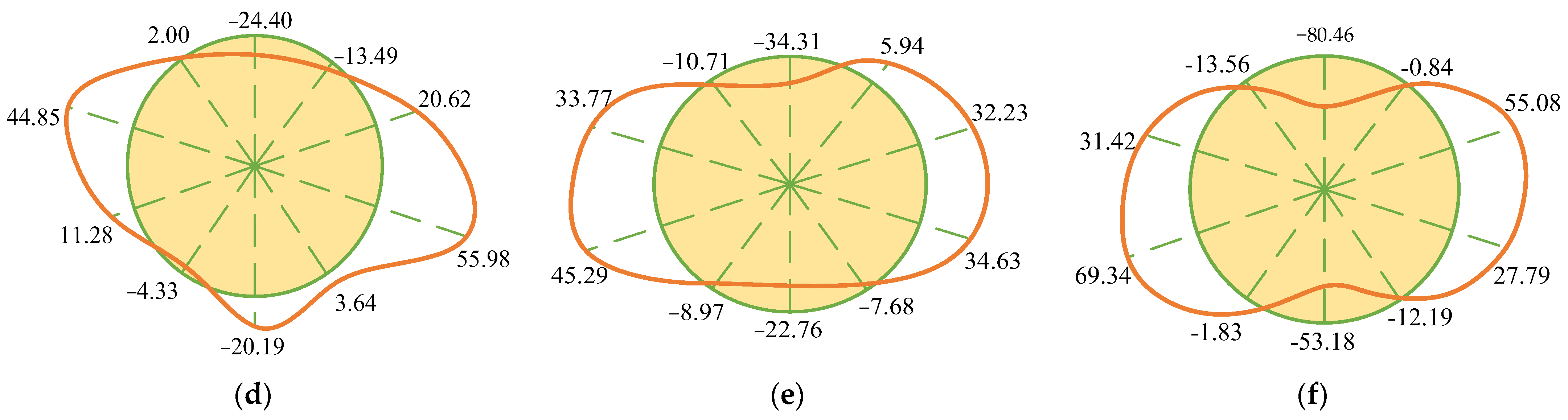
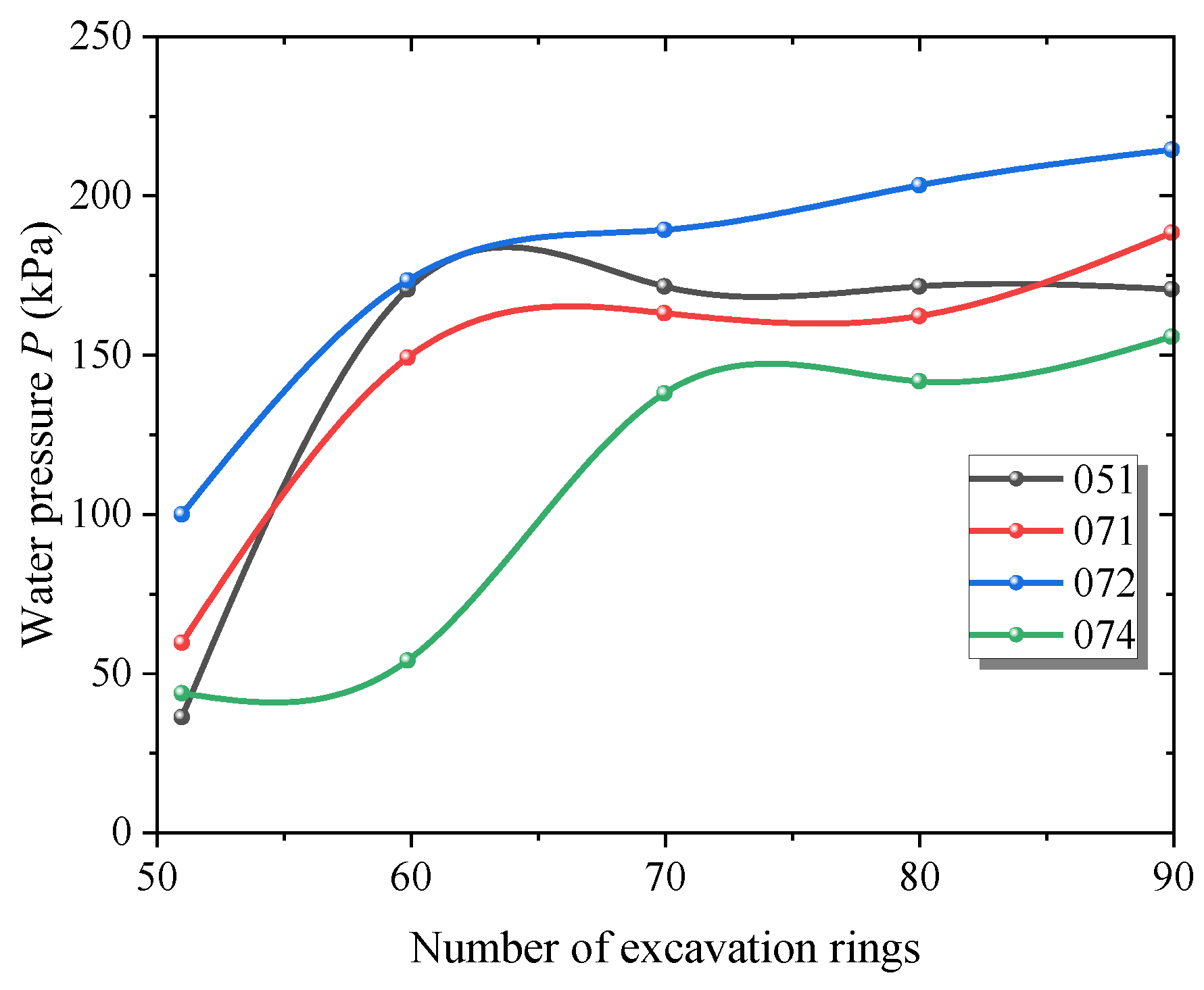
4.1.3. Distribution Rules of Pore Water Pressure
4.2. Clay Formation
4.2.1. Distribution Law of Soil Pressure on Pipe Segments
4.2.2. Distribution Rules of Internal Forces in Segments
4.2.3. Distribution Law of Pore Water Pressure
4.3. Proximity Pile Foundation
4.3.1. Distribution Law of Soil Pressure on Pipe Segments
4.3.2. Distribution Law of Internal Force in Pipe Segments
4.3.3. Distribution Law of Pore Water Pressure
5. Conclusions
- (1)
- In the stratum with uneven hardness, the hardness of soil varies, the earth pressure on the left and right sides of the test ring is asymmetric, and the vault pressure is greater than the arch bottom pressure. There are water passages and bedrock fissure water in the stratum, and the surrounding groundwater will continuously replenish the groundwater loss here because of the head difference until the water pressure reaches a stable equilibrium state; in clay stratum, the soil is gravelly cohesive soil with a single property and low permeability coefficient, and the earth pressure distribution at each position of the segment ring is relatively balanced. There is no effective flow channel in the gravel cohesive soil layer, and the water pressure can not be completely transmitted downwards, which leads to the actual water pressure difference between the arch bottom and the arch waist being smaller than the theoretical difference; in the fully weathered granite layer of the overlying building, the segment of the test ring is subjected to greater additional stress, which is much greater than the internal force of the segment without the overlying building. A boundary surface for groundwater circulation is formed between rock strata, which can store a large amount of fissure water and pore water. Groundwater in other places can supply groundwater loss here at a faster speed, and finally reach a state of hydraulic balance.
- (2)
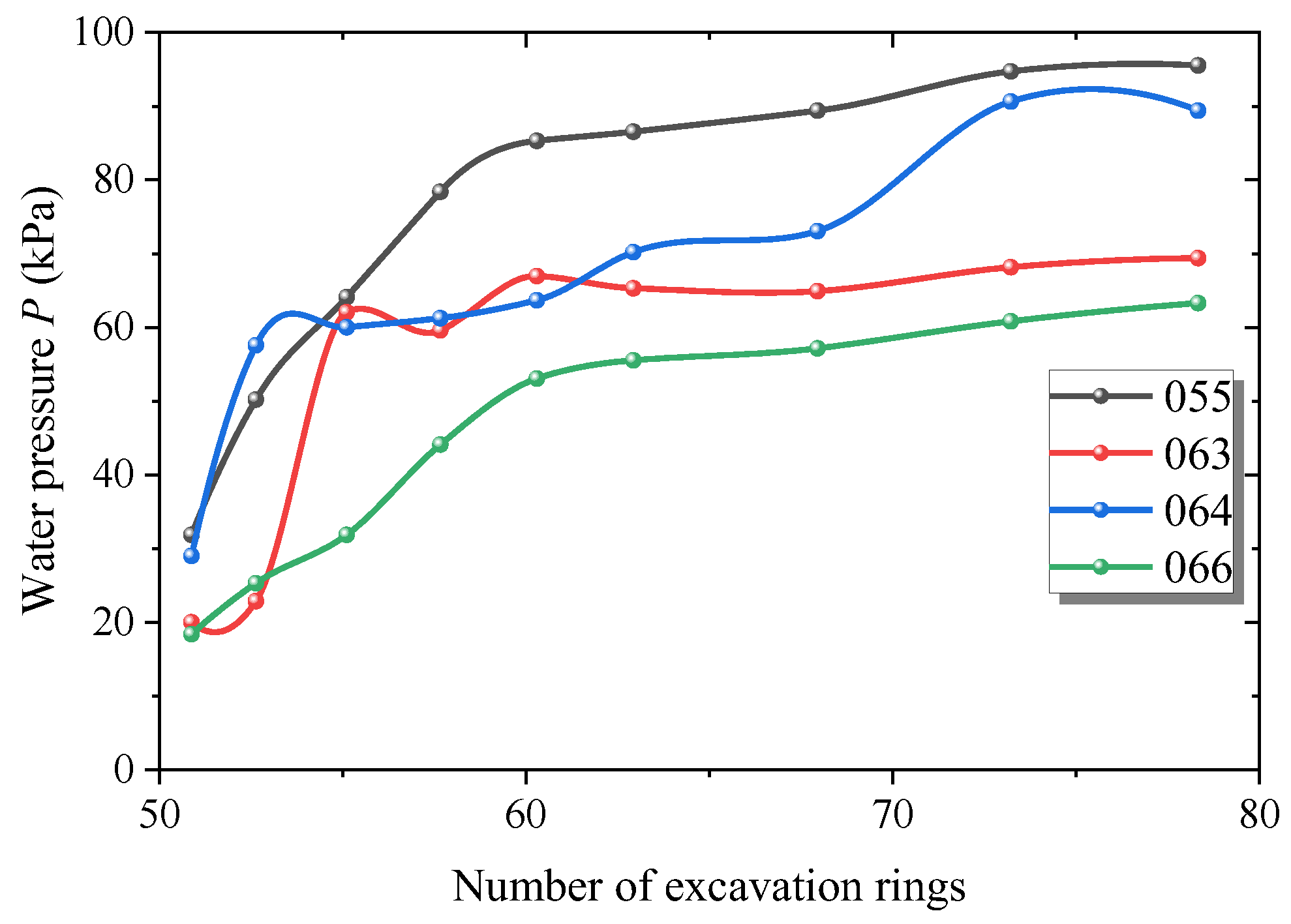
- The mechanical properties of shield tunnel segments in different strata are quite different, but their mechanical properties change stages are consistent. That is, when the segment ring is just assembled, under the protection of the shield shell, the internal force is small, and when the segment comes out of the shield tail, the internal force of the segment reaches the maximum peak. When the segment is assembled for a certain period of time, the internal force of the segment tends to be stable, and the internal force of the stabilized segment is generally smaller than when it just comes out of the shield tail.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Qin, B.Y.; Li, H.Y.; Wang, Z.J.; Jiang, Y.; Lu, D.C.; Du, X.; Qian, Q.L. New Framework of Low-Carbon City Development of China: Underground Space Based Integrated Energy Systems. Undergr. Space 2024, 14, 300–318. [Google Scholar] [CrossRef]
- Zhang, H.; Chen, L.; Zhu, Y.M.; Zhou, Z.L.; Chen, S.G. Stress Field Distribution and Deformation Law of Large Deformation Tunnel Excavation in Soft Rock Mass. Appl. Sci. 2019, 9, 865. [Google Scholar] [CrossRef]
- Zhu, Y.M.; Chen, L.; Zhang, H.; Zhou, Z.L.; Chen, S.G. Physical and Mechanical Characteristics of Soft Rock Tunnel and the Effect of Excavation on Supporting Structure. Appl. Sci. 2019, 9, 1517. [Google Scholar] [CrossRef]
- Zeng, Y.H.; Liu, K.L.; Fang, Y.; Zhang, X.F.; Bai, Y. Fire Model Test on Temperature Field in the Rescue Station of an Extralong Railway Tunnel. Adv. Civ. Eng. 2019, 2019, e1732572. [Google Scholar] [CrossRef]
- Zhang, H.; Chen, L.; Chen, S.G.; Sun, J.C.; Yang, J.S. The Spatiotemporal Distribution Law of Microseismic Events and Rockburst Characteristics of the Deeply Buried Tunnel Group. Energies 2018, 11, 3257. [Google Scholar] [CrossRef]
- Zhang, S.F.; Gao, Z.H.; Wang, S.; Chen, B.; Mao, C.Z. Study on the Seismic Response Characteristics of Shield Tunnels with Different General Segment Assembly Methods and Widths. Buildings 2023, 13, 2039. [Google Scholar] [CrossRef]
- Xu, X.D.; He, M.C.; Zhu, C.; Lin, Y.; Cao, C. A New Calculation Model of Blasting Damage Degree—Based on Fractal and Tie Rod Damage Theory. Eng. Fract. Mech. 2019, 220, 106619. [Google Scholar] [CrossRef]
- Sazid, M.; Singh, T.N. Two-Dimensional Dynamic Finite Element Simulation of Rock Blasting. Arab J. Geosci. 2013, 6, 3703–3708. [Google Scholar] [CrossRef]
- Sazid, M. Effect of Underground Blasting on Surface Slope Stability: A Numerical Approach. Am. J. Min. Metall. 2017, 4, 32–36. [Google Scholar]
- Lin, F.; Zhang, H.; Chen, S.G. Study on Type Selection of Shield Equipment in Different Geological Conditions. In Green Building, Materials and Civil Engineering; CRC Press: Boca Raton, FL, USA, 2014; ISBN 978-0-429-22702-8. [Google Scholar]
- Zou, J.F.; Qian, Z.H.; Xiang, X.H.; Chen, G.H. Face Stability of a Tunnel Excavated in Saturated Nonhomogeneous Soils. Tunn. Undergr. Space Technol. 2019, 83, 1–17. [Google Scholar] [CrossRef]
- Zhang, H.; Chen, S.G.; Deng, X.F. Analysis of the influence of shield driving parameters on ground settlements. Mod. Tunn. Technol. 2010, 47, 48–53. [Google Scholar]
- Liao, S.M.; Liu, J.H.; Wang, R.L.; Li, Z.M. Shield Tunneling and Environment Protection in Shanghai Soft Ground. Tunn. Undergr. Space Technol. 2009, 24, 454–465. [Google Scholar] [CrossRef]
- Zhang, J.L.; Gao, Y.M.; Liu, X.; Zhang, Z.A.; Yuan, Y.; Mang, H.A. A Shield Tunneling Method for Enlarging the Diameter of Existing Tunnels: Experimental Investigations. Tunn. Undergr. Space Technol. 2022, 128, 104605. [Google Scholar] [CrossRef]
- Xie, X.Y.; Yang, Y.B.; Ji, M. Analysis of Ground Surface Settlement Induced by the Construction of a Large-Diameter Shield-Driven Tunnel in Shanghai, China. Tunn. Undergr. Space Technol. 2016, 51, 120–132. [Google Scholar] [CrossRef]
- Zhang, H.; Chen, S.G.; Tan, X.R. Research on the mechanical behavior of a segment of a shield tunnel adjacent to a pile foundation. Mod. Tunn. Technol. 2012, 49, 101–107. [Google Scholar]
- Zhang, H.; Chen, S.G.; Deng, X.F. Analysis on influence of shield tunneling on ground and bridge pile. Tunn. Undergr. Space Technol. 2011, 7, 552–557. [Google Scholar]
- Xu, Z.W.; Zhou, S.H.; Zhang, C.; Yang, M.H.; Jiang, M.Y. A Bayesian Network Model for Suitability Evaluation of Underground Space Development in Urban Areas: The Case of Changsha, China. J. Clean. Prod. 2023, 418, 138135. [Google Scholar] [CrossRef]
- Yan, Z.G.; Li, J.T.; Shen, Y.; Xiao, Z.Q.; Ai, Q.; Zhu, H.H. Damage Identification Method on Shield Tunnel Based on PLSR and Equivalent Damage Analysis. Tunn. Undergr. Space Technol. 2023, 137, 105127. [Google Scholar] [CrossRef]
- Sazid, M.; Hussein, K.; Abudurman, K. Rock Stress Measurement Methods in Rock Mechanics—A Brief Overview. World J. Eng. Technol. 2023, 11, 252–272. [Google Scholar] [CrossRef]
- Zhu, W.B.; Ju, S.J. Shield Tunneling Technology in Mixed Face Ground Conditions; China Science and Technology Press: Beijing, China, 2006; ISBN 978-7-5046-4327-8. [Google Scholar]
- Fang, Y.; Fu, Y.P.; Qi, C.; He, C. Structural load and internal force characteristics of shield tunnel segment in expansive rock and soil strata. China Railw. Sci. 2014, 35, 56–63. [Google Scholar]
- Zhang, H.; Chen, S.G.; Tan, X.R.; Zhao, Y.B. Research on Mechanical Behaviour of Segmental Structure of Shield Tunnel in Different Strata. Chin. J. Undergr. Space Eng. 2015, 11, 845–851. [Google Scholar]
- Zhang, X.H.; Zhou, S.H.; Di, H.G.; Wang, P.X.; Wang, J.T. Semi–Analytical Solution for Ultimate Bearing Capacity of Straight–Jointed Segmental Tunnel Lining. Tunn. Undergr. Space Technol. 2023, 138, 105160. [Google Scholar]
- Song, K.Z.; Yuan, D.J.; Wang, M.S. Segmental mechanical analysis of shield tunnel during construction stage. Rock Soil Mech. 2008, 29, 619–623+628. [Google Scholar]
- Huang, D.W.; Jiang, H.; Xu, C.J.; Li, X.; Zhan, T. Analytical Algorithm of Longitudinal Bending Stiffness of Shield Tunnel Considering Longitudinal Residual Jacking Force. Tunn. Undergr. Space Technol. 2023, 137, 105146. [Google Scholar] [CrossRef]
- Liu, Y.B.; Liao, S.M.; Liu, M.B.; Chen, L.S. A Bending-Shear-Torsion Resistant Beam Foundation Model for Segmental Tunnels in Longitudinal Direction. Tunn. Undergr. Space Technol. 2023, 140, 105296. [Google Scholar] [CrossRef]
- Chen, J.S.; Mo, H.H. Three-dimensional finite element analysis of mechanical behaviors of shield tunnel segment during construction. Chin. J. Rock Mech. Eng. 2006, 25, 3482–3489. [Google Scholar]
- Zhu, W.B.; Ju, S.J. Causes andcountermeasures for segment cracking in shield-driven tunnel. Mod. Tunn. Technol. 2003, 40, 21–25. [Google Scholar] [CrossRef]
- Zhu, H.H.; Cui, M.Y.; Yang, J.S. Design model for shield lining segments and distribution of load. Chin. J. Geotech. Eng. 2000, 22, 190–194. [Google Scholar]
- Zhu, H.H.; Tao, L.B. Study on two beam-spring models for the numerical analysis of segments in shield tunnel. Rock Soil Mech. 1998, 19, 26–32. [Google Scholar]
- Chen, W.; Peng, Z.B.; Tang, M.X. Testing study on work property of shield segment. Chin. J. Rock Mech. Eng. 2004, 23, 959–963. [Google Scholar]
- Hu, Z.P.; Luo, L.J.; Cai, Z.Y. Study on flatshell-elastic hinge-foundation model in shield tunnel. Rock Soil Mech. 2005, 26, 1403–1408. [Google Scholar]
- Kong, X.X.; Tang, L.; Ling, X.Z.; Li, H. Development of Shield Model Test System for Studying the Bias Load of Shield in Soil-Rock Compound Strata. Tunn. Undergr. Space Technol. 2024, 143, 105464. [Google Scholar] [CrossRef]
- Zhu, Y.T.; Zhu, Y.F.; Chen, E.J.; Zhai, Y.X.; Min, R.; Tang, B.; Huang, X. Synchronous Shield Tunnelling Technology Combining Advancement and Segment Fabrication: Principle, Verification and Application. Undergr. Space 2023, 13, 23–47. [Google Scholar] [CrossRef]
- Sazid, M.; Ahmed, H.A. Stability Analysis of Shallow Depth Tunnel in Weak Rock Mass: 3D Numerical Modeling Approach. J. City Dev. 2019, 1, 18–22. [Google Scholar]
- Verma, A.K.; Singh, T.N. Assessment of Tunnel Instability—A Numerical Approach. Arab. J. Geosci. 2010, 3, 181–192. [Google Scholar] [CrossRef]
- GB 50010-2010; Code for Design of Concrete Structures. China Building Industry Press: Beijing, China, 2015.






Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hu, H.; Xue, T.; Li, J.; Liu, P.; Wang, B.; Liu, Y. Mechanical Performance of Concrete Segment Lining Structure of Shield Tunneling in Different Strata. Buildings 2023, 13, 3118. https://doi.org/10.3390/buildings13123118
Hu H, Xue T, Li J, Liu P, Wang B, Liu Y. Mechanical Performance of Concrete Segment Lining Structure of Shield Tunneling in Different Strata. Buildings. 2023; 13(12):3118. https://doi.org/10.3390/buildings13123118
Chicago/Turabian StyleHu, Hui, Tao Xue, Jianjun Li, Peisi Liu, Bo Wang, and Yun Liu. 2023. "Mechanical Performance of Concrete Segment Lining Structure of Shield Tunneling in Different Strata" Buildings 13, no. 12: 3118. https://doi.org/10.3390/buildings13123118
APA StyleHu, H., Xue, T., Li, J., Liu, P., Wang, B., & Liu, Y. (2023). Mechanical Performance of Concrete Segment Lining Structure of Shield Tunneling in Different Strata. Buildings, 13(12), 3118. https://doi.org/10.3390/buildings13123118




