Moisture Diffusion Coefficient of Concrete under Different Conditions
Abstract
:1. Introduction
2. Theoretical Modeling
3. Experimental Design
3.1. Concrete Mixing Ratio
3.2. Basic Concrete Performance Tests
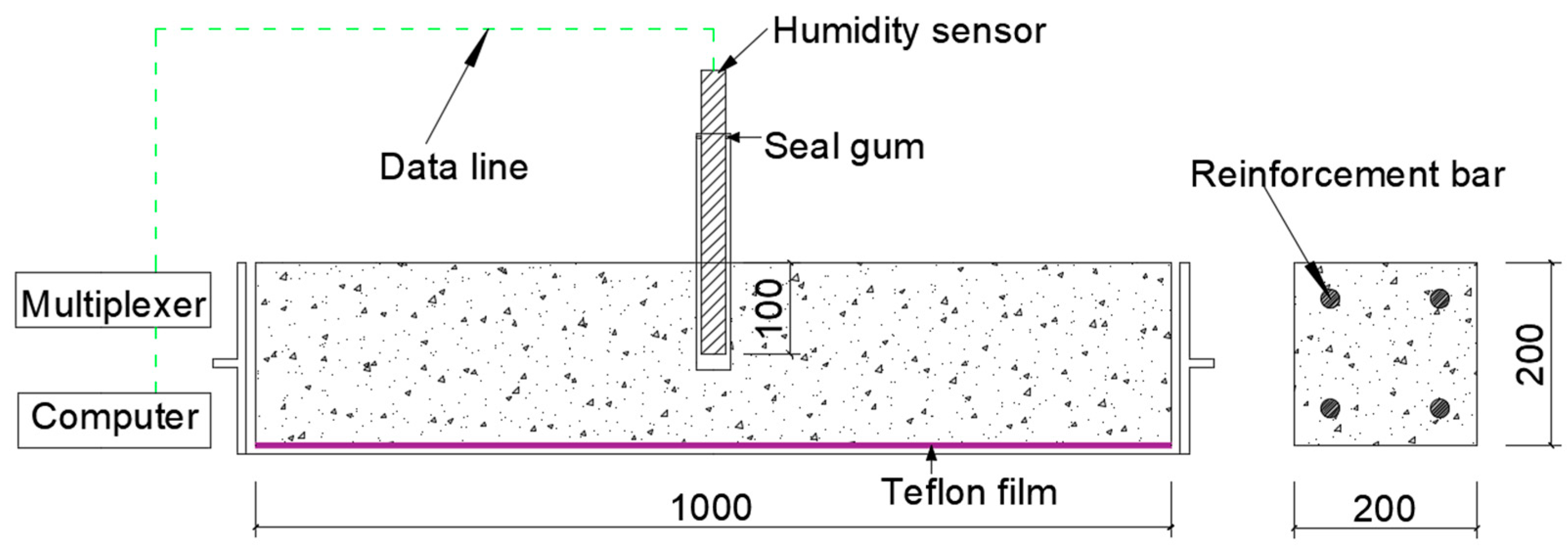
3.3. Concrete Internal Humidity and Shrinkage
3.4. Pore Structure Test
4. Results and Discussion
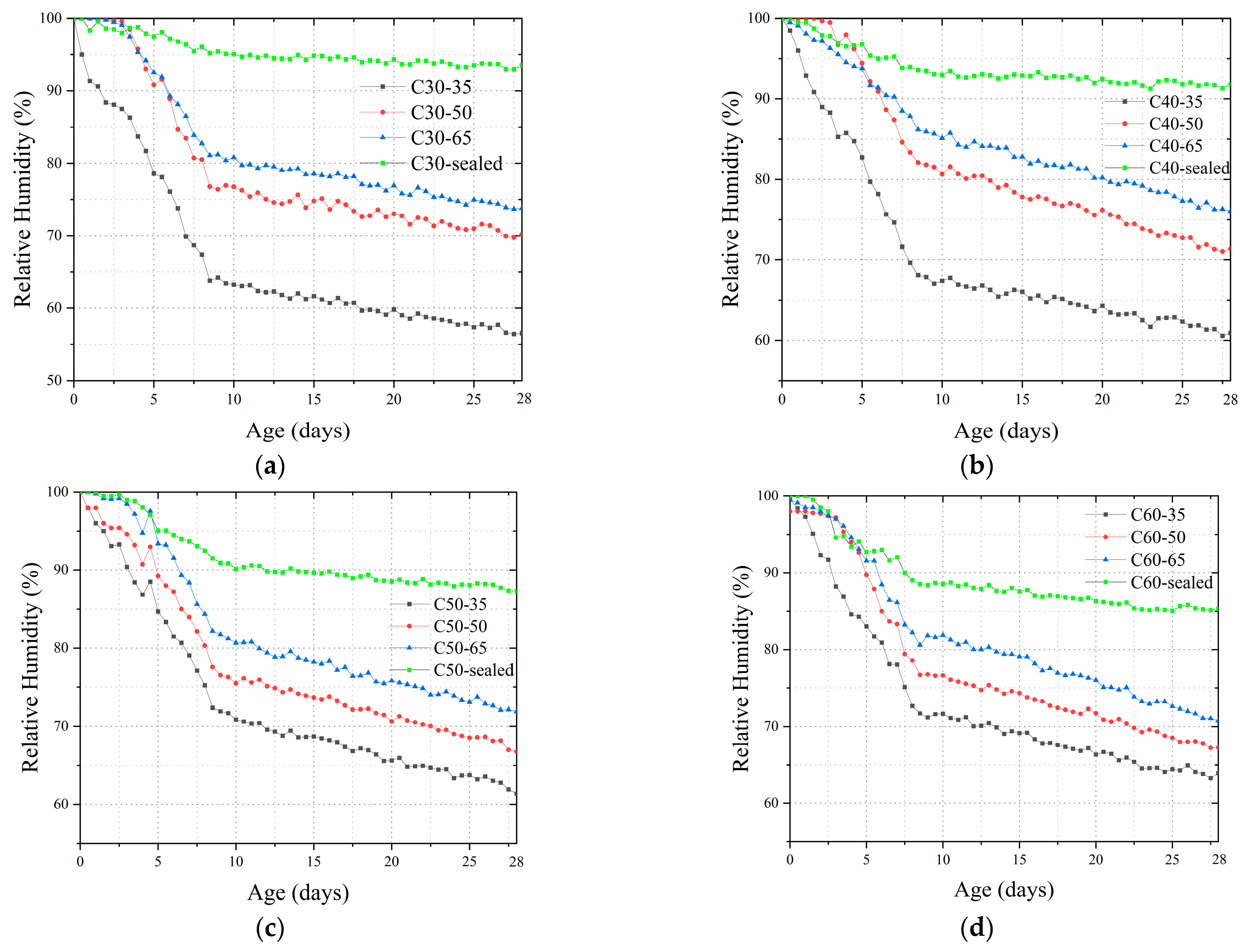
4.1. Concrete Internal Humidity
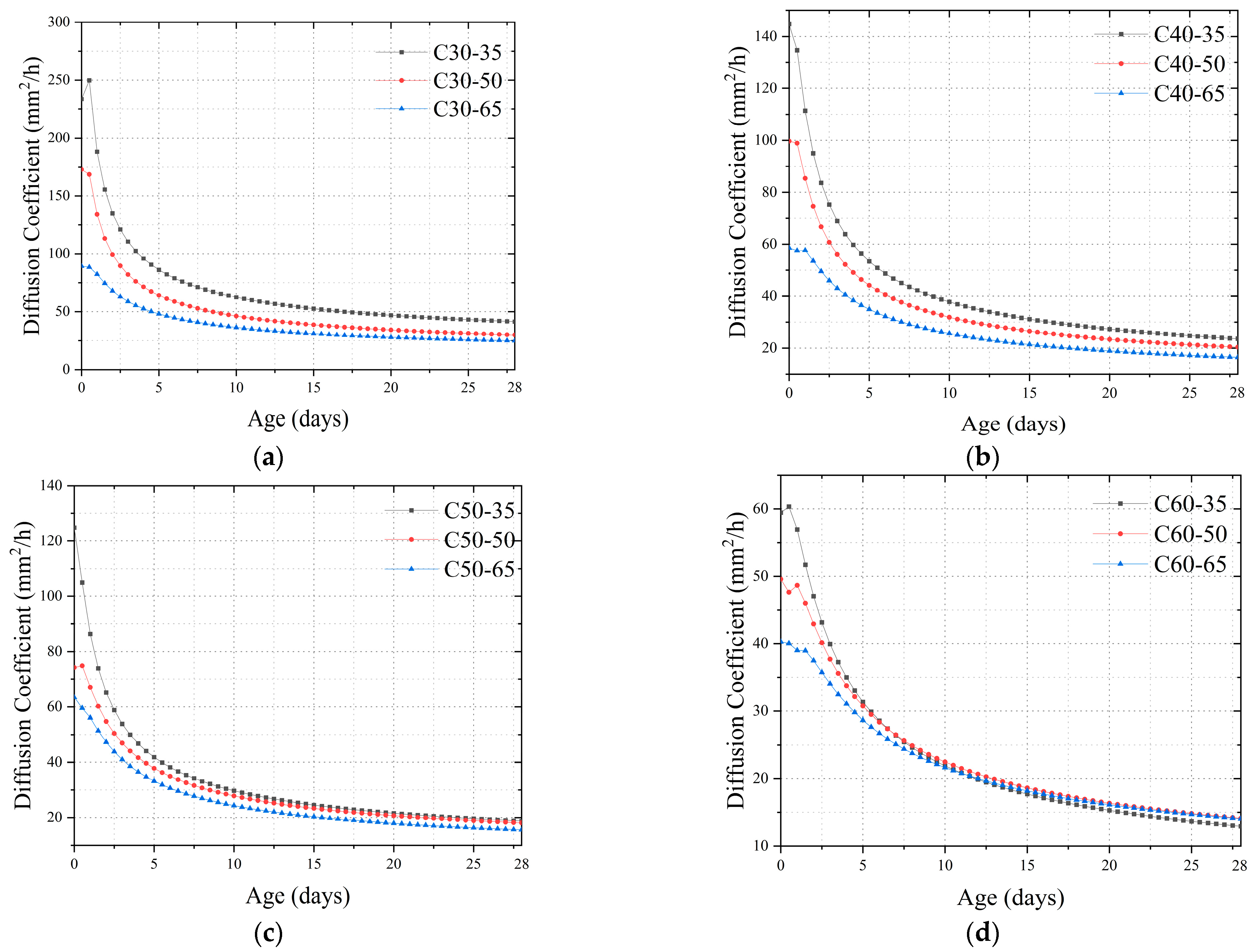
4.2. Concrete Moisture Diffusion Coefficient
4.3. Effect of Concrete Strength on Moisture Diffusion Coefficient
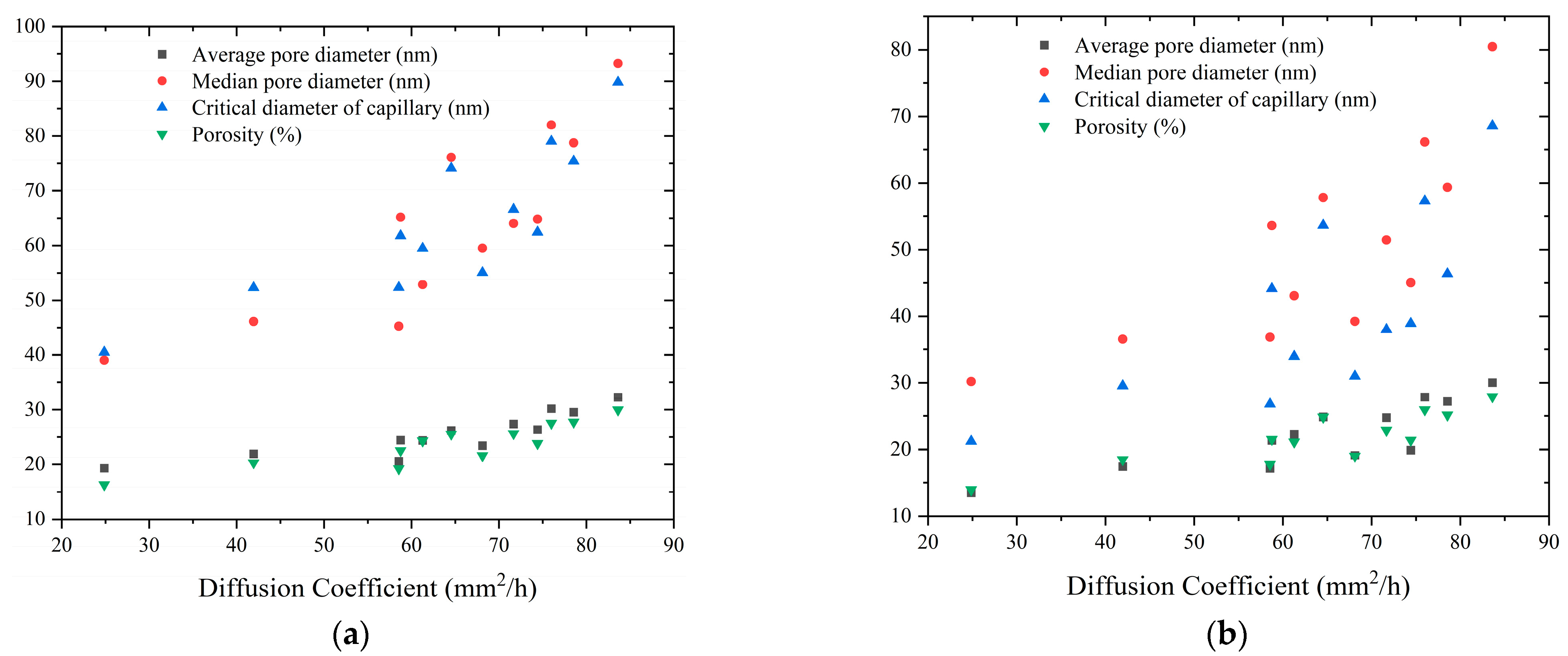
4.4. Effect of Pore Structure on the Moisture Diffusion Coefficient of Concrete
5. Conclusions
- The internal humidity of concrete varies with age. As the age increases, the internal humidity of concrete decreases and gradually stabilizes. Due to more intense hydration, the internal humidity of high-strength concrete decreases more rapidly under sealed conditions. The internal humidity of specimens under dry conditions decreases faster than that of sealed specimens due to the drying effect. For the same concrete strength class, the lower the external humidity and the higher the reinforcement rate, the faster the internal humidity of the concrete decreases.
- The moisture diffusion coefficient of concrete can be divided into a rapid decline phase (0–1 day), a slow decline phase (1–8 days), and a stabilization phase (after 8 days) as the age increases. Due to the existence of a humidity gradient, the lower the external environmental humidity, the higher the humidity diffusion coefficient of concrete for the same concrete strength class and reinforcement ratio, and the two exhibit a linear relationship. With the increase in age and concrete strength, the influence of external humidity on the humidity diffusion coefficient of concrete weakens. Meanwhile, along with the gradual decrease in the internal humidity of concrete, the humidity diffusion coefficient of concrete also gradually decreases, and it is more difficult for the water in concrete to be transmitted to the external environment.
- At the same age, high-strength concrete has smaller pore structure parameters, which makes transporting water in high-strength concrete difficult. As a result, the moisture diffusion coefficient of concrete decreases as the concrete strength class increases for the same external humidity and reinforcement ratio. The method proposed in this study for calculating the internal humidity of concrete using the humidity diffusion coefficient is accurate.
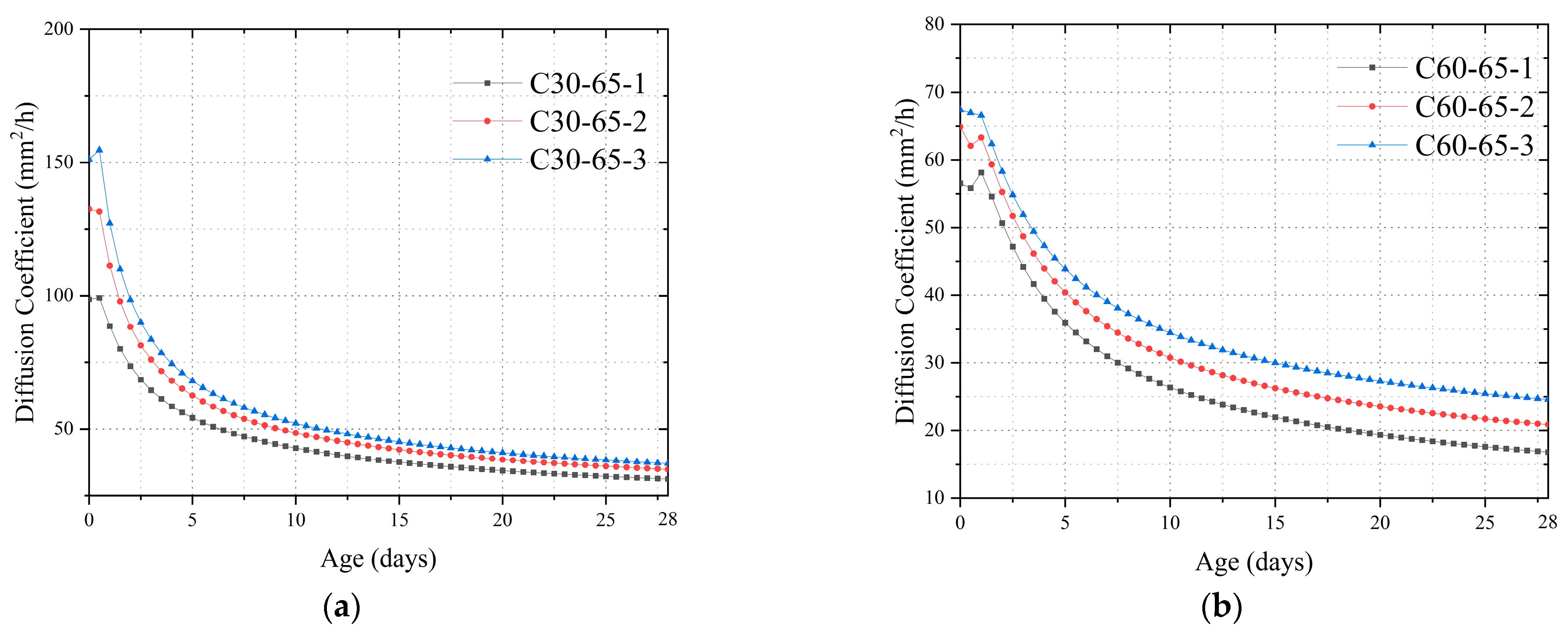
- Reinforcing bars create confining tensile stresses inside the concrete, which changes the pore structure inside the concrete. For the same concrete strength, the higher the reinforcement rate, the larger the pore structure parameters of concrete (average pore diameter, median pore diameter, critical diameter of capillary, and porosity). Because the pore structure is the main transport channel for water in concrete, the higher the reinforcement ratio, the greater the moisture diffusion coefficient of the concrete and the faster the moisture in the concrete decreases for the same external humidity and concrete strength.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Krauss, P.D.; Rogalla, E.A. Transverse Cracking in Newly Constructed Bridge Decks; National Academy of Sciences: Washington, DC, USA, 1996. [Google Scholar]
- Chen, L.; Huang, L.; Hua, J.; Chen, Z.; Wei, L.; Osman, A.I.; Fawzy, S.; Rooney, D.W.; Dong, L.; Yap, P.-S. Green construction for low-carbon cities: A review. Environ. Chem. Lett. 2023, 21, 1627–1657. [Google Scholar] [CrossRef]
- Zhou, F.; Jiang, H.; Huang, L.; Hu, Y.; Xie, Z.; Zeng, Z.; Liu, M.; Wang, B.; Zhou, X. Early Shrinkage Modeling of Complex Internally Confined Concrete Based on Capillary Tension Theory. Buildings 2023, 13, 2201. [Google Scholar] [CrossRef]
- Huang, L.; Hua, J.; Kang, M.; Zhou, F.; Luo, Q. Capillary tension theory for predicting shrinkage of concrete restrained by reinforcement bar in early age. Constr. Build. Mater. 2019, 210, 63–70. [Google Scholar] [CrossRef]
- Tran, N.P.; Gunasekara, C.; Law, D.W.; Houshyar, S.; Setunge, S.; Cwirzen, A. A critical review on drying shrinkage mitigation strategies in cement-based materials. J. Build. Eng. 2021, 38, 102210. [Google Scholar] [CrossRef]
- Wang, X.; Song, P.; Yu, H.; Taylor, P.; Sadati, S.; Freeseman, K.; Ning, Y. Extended life concrete bridge decks utilizing internal curing to reduce cracking–Materials characterization and engineering demonstration. Constr. Build. Mater. 2021, 275, 122163. [Google Scholar] [CrossRef]
- Miao, Y.; Lu, Z.; Wang, F.; Wang, H.; Li, Y.; Lin, J.; Jiang, J. Shrinkage cracking evolvement in concrete cured under low relative humidity and its relationship with mechanical development. J. Build. Eng. 2023, 72, 106670. [Google Scholar] [CrossRef]
- Yu, S.; Sun, Z.; Qian, W.; Yu, J.; Yang, J. A meshless method for modeling the microscopic drying shrinkage cracking processes of concrete and its applications. Eng. Fract. Mech. 2023, 277, 109014. [Google Scholar] [CrossRef]
- Shen, D.; Kang, J.; Jiao, Y.; Li, M.; Li, C. Effects of different silica fume dosages on early-age behavior and cracking resistance of high strength concrete under restrained condition. Constr. Build. Mater. 2020, 263, 120218. [Google Scholar] [CrossRef]
- Klemczak, B.; Knoppik-Wróbel, A. Reinforced concrete tank walls and bridge abutments: Early-age behaviour, analytic approaches and numerical models. Eng. Struct. 2015, 84, 233–251. [Google Scholar] [CrossRef]
- Chen, L.; Chen, Z.; Zhang, Y.; Liu, Y.; Osman, A.I.; Farghali, M.; Hua, J.; Al-Fatesh, A.; Ihara, I.; Rooney, D.W. Artificial intelligence-based solutions for climate change: A review. Environ. Chem. Lett. 2023, 21, 2525–2557. [Google Scholar] [CrossRef]
- Chen, L.; Msigwa, G.; Yang, M.; Osman, A.I.; Fawzy, S.; Rooney, D.W.; Yap, P.-S. Strategies to achieve a carbon neutral society: A review. Environ. Chem. Lett. 2022, 20, 2277–2310. [Google Scholar] [CrossRef]
- Farghali, M.; Osman, A.I.; Mohamed, I.M.; Chen, Z.; Chen, L.; Ihara, I.; Yap, P.-S.; Rooney, D.W. Strategies to save energy in the context of the energy crisis: A review. Environ. Chem. Lett. 2023, 21, 2003–2039. [Google Scholar] [CrossRef] [PubMed]
- Deysel, R.C.; Boshoff, W.P.; Smit, M.S. Implementing capillary pressure control measures to prevent plastic shrinkage cracking in concrete. Constr. Build. Mater. 2023, 397, 132407. [Google Scholar] [CrossRef]
- Liang, S.; Liu, Y.; Song, G.; Yan, H.; Song, B.; Liu, J. Effect of sulfoaluminate expansive additive on mechanical properties, internal relative humidity, and shrinkage of early-age mortar. Case Stud. Constr. Mater. 2023, 19, e02226. [Google Scholar] [CrossRef]
- Wang, Y.; Zhu, J.; Guo, Y.; Wang, C. Early shrinkage experiment of concrete and the development law of its temperature and humidity field in natural environment. J. Build. Eng. 2023, 63, 105528. [Google Scholar] [CrossRef]
- Persson, B. Moisture in concrete subjected to different kinds of curing. Mater. Struct. 1997, 30, 533–544. [Google Scholar] [CrossRef]
- Kim, J.-K.; Lee, C.-S. Moisture diffusion of concrete considering self-desiccation at early ages. Cem. Concr. Res. 1999, 29, 1921–1927. [Google Scholar] [CrossRef]
- Nilsson, L.-O. Long-term moisture transport in high performance concrete. Mater. Struct. 2002, 35, 641–649. [Google Scholar] [CrossRef]
- Andrade, C.; Sarría, J.; Alonso, C. Relative humidity in the interior of concrete exposed to natural and artificial weathering. Cem. Concr. Res. 1999, 29, 1249–1259. [Google Scholar] [CrossRef]
- Parrott, L. Some effects of cement and curing upon carbonation and reinforcement corrosion in concrete. Mater. Struct. 1996, 29, 164–173. [Google Scholar] [CrossRef]
- Wang, J.; Li, H.; Wang, Z.; Yi, Z.; Huang, F. Humidity field and moisture transfer of concrete with different pre-saturated recycled sand. Constr. Build. Mater. 2023, 382, 131338. [Google Scholar] [CrossRef]
- Shen, D.; Liu, C.; Wang, M.; Jin, X.; Tang, H. Prediction model for internal relative humidity in early-age concrete under different curing humidity conditions. Constr. Build. Mater. 2020, 265, 119987. [Google Scholar] [CrossRef]
- Tazawa, E.-I.; Miyazawa, S. Influence of cement and admixture on autogenous shrinkage of cement paste. Cem. Concr. Res. 1995, 25, 281–287. [Google Scholar] [CrossRef]
- Tazawa, E.-I.; Miyazawa, S. Experimental study on mechanism of autogenous shrinkage of concrete. Cem. Concr. Res. 1995, 25, 1633–1638. [Google Scholar] [CrossRef]
- Aitcin, P.-C. Demystifying autogenous shrinkage. Concr. Int. 1999, 21, 54–56. [Google Scholar]
- Ni, W.-M. The Mathematics of Diffusion; SIAM: Philadelphie, PA, USA, 2011. [Google Scholar]
- Park, S.-S.; Kwon, S.-J.; Jung, S.H.; Lee, S.-W. Modeling of water permeability in early aged concrete with cracks based on micro pore structure. Constr. Build. Mater. 2012, 27, 597–604. [Google Scholar] [CrossRef]
- Linderoth, O.; Johansson, P.; Wadsö, L. Development of pore structure, moisture sorption and transport properties in fly ash blended cement-based materials. Constr. Build. Mater. 2020, 261, 120007. [Google Scholar] [CrossRef]
- Huang, L.M.; Tang, L.P.; Lofgren, I.; Olsson, N.; Yang, Z.H.; Li, Y.Q. Moisture and ion transport properties in blended pastes and their relation to the refined pore structure. Cem. Concr. Res. 2022, 161, 106949. [Google Scholar] [CrossRef]
- Bažant, Z.; Najjar, L. Nonlinear water diffusion in nonsaturated concrete. Matériaux Constr. 1972, 5, 3–20. [Google Scholar] [CrossRef]
- Ayano, T.; Wittmann, F.H. Drying, moisture distribution, and shrinkage of cement-based materials. Mater. Struct. 2002, 35, 134–140. [Google Scholar] [CrossRef]
- Sakata, K. A study on moisture diffusion in drying and drying shrinkage of concrete. Cem. Concr. Res. 1983, 13, 216–224. [Google Scholar] [CrossRef]
- Akita, H.; Fujiwara, T.; Ozaka, Y. A practical procedure for the analysis of moisture transfer within concrete due to drying. Mag. Concr. Res. 1997, 49, 129–137. [Google Scholar] [CrossRef]
- Ba, M.F.; Qian, C.X.; Gao, G.B. Nonlinear calculation of moisture transport in underground concrete. Comput. Concr. 2014, 13, 361–375. [Google Scholar] [CrossRef]
- Rahimi-Aghdam, S.; Rasoolinejad, M.; Bažant, Z.P. Moisture diffusion in unsaturated self-desiccating concrete with humidity-dependent permeability and nonlinear sorption isotherm. J. Eng. Mech. 2019, 145, 04019032. [Google Scholar] [CrossRef]
- Lin, G.; Liu, Y.; Xiang, Z. Numerical modeling for predicting service life of reinforced concrete structures exposed to chloride environments. Cem. Concr. Compos. 2010, 32, 571–579. [Google Scholar] [CrossRef]
- Wang, A.; Sun, D.; Deng, M.; Chen, X.; Zhang, F. Influence of restraint on pore structures and air permeability of concrete containing MgO-type expansive agent. Asian J. Chem. 2013, 25, 5532. [Google Scholar] [CrossRef]
- Zhang, R.; Wang, Q.; Ma, L. Relations between pore structure and creep characteristics of concrete under micro-expanding and lateral restraints. J. Chin. Ceram. Soc. 2015, 7, 585–593. [Google Scholar]
- Yoo, D.-Y.; Park, J.-J.; Kim, S.-W.; Yoon, Y.-S. Influence of reinforcing bar type on autogenous shrinkage stress and bond behavior of ultra high performance fiber reinforced concrete. Cem. Concr. Compos. 2014, 48, 150–161. [Google Scholar] [CrossRef]
- Sule, M.; van Breugel, K. The effect of reinforcement on early-age cracking due to autogenous shrinkage and thermal effects. Cem. Concr. Compos. 2004, 26, 581–587. [Google Scholar] [CrossRef]
- Huang, L.; Hua, J.; Kang, M.; Zhang, A. Influence of reinforcement configuration on the shrinkage and cracking potential of high-performance concrete. Constr. Build. Mater. 2017, 140, 20–30. [Google Scholar] [CrossRef]
- Yoo, D.-Y.; Park, J.-J.; Kim, S.-W.; Yoon, Y.-S. Early age setting, shrinkage and tensile characteristics of ultra high performance fiber reinforced concrete. Constr. Build. Mater. 2013, 41, 427–438. [Google Scholar] [CrossRef]
- Ming, K. Research on Restrained Deformation Properties of Reinforced Concrete Members due to Early Shrinkage during Construction. Ph.D. Thesis, Chongqing University, Chongqing, China, 2010. [Google Scholar]
- Radlinska, A.; Weiss, J. Quantifying variability in assessing the risk of early-age cracking in restrained concrete elements. In Brittle Matrix Composites 8; Elsevier: Amsterdam, The Netherlands, 2006; pp. 331–342. [Google Scholar]
- Zhang, J.; Huang, Y.; Qi, K.; Gao, Y. Interior relative humidity of normal-and high-strength concrete at early age. J. Mater. Civ. Eng. 2012, 24, 615–622. [Google Scholar] [CrossRef]
- Zhang, J.; Qi, K.; Huang, Y. Calculation of moisture distribution in early-age concrete. J. Eng. Mech. 2009, 135, 871–880. [Google Scholar] [CrossRef]
- Deboodt, T.; Fu, T.; Ideker, J.H. Evaluation of FLWA and SRAs on autogenous deformation and long-term drying shrinkage of high performance concrete. Constr. Build. Mater. 2016, 119, 53–60. [Google Scholar] [CrossRef]
- Shen, D.; Liu, K.; Ji, Y.; Shi, H.; Zhang, J. Early-age residual stress and stress relaxation of high-performance concrete containing fly ash. Mag. Concr. Res. 2018, 70, 726–738. [Google Scholar] [CrossRef]
- GB/T50081-2002; Standard for Test Method of Mechanical Properties on Ordinary Concrete. Ministry of Construction of the People’s Republic of China and General Administration of Quality Supervision. Inspection and Quarantine of the People’s Republic of China: Beijing, China, 2003.









| Concrete | Cement (kg/m3) | Water (kg/m3) | Fly Ash (kg/m3) | Sand (kg/m3) | Coarse Aggregate (kg/m3) | Water Reducing Agent (kg/m3) |
|---|---|---|---|---|---|---|
| C30 | 336 | 195 | 69 | 705 | 1094 | 9.9 |
| C40 | 365 | 185 | 65 | 685 | 1090 | 10.4 |
| C50 | 394 | 175 | 61 | 665 | 1086 | 10.9 |
| C60 | 423 | 166 | 58 | 645 | 1083 | 11.4 |
| Composition (%) | Cement | Fly Ash |
|---|---|---|
| SiO2 | 21.47 | 49.47 |
| CaO | 65.77 | 4.45 |
| Al2O3 | 5.47 | 20.67 |
| Fe2O3 | 4.28 | 14.32 |
| MgO | 1.44 | 1.17 |
| SO3 | 0.52 | 1.40 |
| Basic Properties | Concrete Strength | Curing Condition | Age (Days) | ||
|---|---|---|---|---|---|
| 3 | 7 | 28 | |||
| Cube compressive strength (MPa) | C30 | Dry | 15.3 | 21.3 | 34.5 |
| Sealed | 17.1 | 21.4 | 39.5 | ||
| C40 | Dry | 25.1 | 31.0 | 45.4 | |
| Sealed | 27.0 | 32.0 | 48.5 | ||
| C50 | Dry | 34.0 | 40.6 | 55.0 | |
| Sealed | 36.5 | 42.0 | 56.5 | ||
| C60 | Dry | 44.0 | 53.0 | 63.0 | |
| Sealed | 46.5 | 59.4 | 68.0 | ||
| Splitting tensile strength (MPa) | C30 | Dry | 2.2 | 3.7 | 4.3 |
| Sealed | 2.4 | 4.1 | 4.4 | ||
| C40 | Dry | 2.5 | 4.1 | 4.7 | |
| Sealed | 2.7 | 4.2 | 4.7 | ||
| C50 | Dry | 2.9 | 4.4 | 5.0 | |
| Sealed | 3.1 | 4.5 | 5.1 | ||
| C60 | Dry | 3.4 | 4.7 | 5.4 | |
| Sealed | 3.5 | 4.9 | 5.6 | ||
| Static modulus of elasticity (×104 MPa) | C30 | Dry | 1.6 | 1.7 | 2.9 |
| Sealed | 1.7 | 2.0 | 2.9 | ||
| C40 | Dry | 1.8 | 2.1 | 3.0 | |
| Sealed | 1.6 | 2.2 | 3.0 | ||
| C50 | Dry | 2.0 | 2.3 | 3.1 | |
| Sealed | 2.1 | 2.4 | 3.2 | ||
| C60 | Dry | 2.5 | 2.5 | 3.2 | |
| Sealed | 2.4 | 2.6 | 3.4 | ||
| No. | Concrete Strength | External Humidity (%) | Curing Condition |
|---|---|---|---|
| C30-35 | C30 | 35 | Dry |
| C30-50 | C30 | 50 | Dry |
| C30-65 | C30 | 65 | Dry |
| C30-Sealed | C30 | - | Sealed |
| C40-35 | C40 | 35 | Dry |
| C40-50 | C40 | 50 | Dry |
| C40-65 | C40 | 65 | Dry |
| C40-Sealed | C40 | - | Sealed |
| C50-35 | C50 | 35 | Dry |
| C50-50 | C50 | 50 | Dry |
| C50-65 | C50 | 65 | Dry |
| C50-Sealed | C50 | - | Sealed |
| C60-35 | C60 | 35 | Dry |
| C60-50 | C60 | 50 | Dry |
| C60-65 | C60 | 65 | Dry |
| C60-Sealed | C60 | - | Sealed |
| No. | Concrete Strength | External Humidity (%) | Reinforcement Ratio (%) | Curing Condition | Reinforcement Bar Diameter (mm) |
|---|---|---|---|---|---|
| C30-65-1 | C30 | 65 | 1.14 | Dry | 12 |
| C30-65-3 | C30 | 65 | 3.24 | Dry | 20 |
| C30-65-6 | C30 | 65 | 6.56 | Dry | 28 |
| C60-65-1 | C60 | 65 | 1.14 | Dry | 12 |
| C60-65-3 | C60 | 65 | 3.24 | Dry | 20 |
| C60-65-6 | C60 | 65 | 6.56 | Dry | 28 |
| No. | H0 | a | b | c | d | Average Fitting Error |
|---|---|---|---|---|---|---|
| C30-35 | 98.7 | 0.020 | 8.1 | 3.8 | 1.7 | 1.1% |
| C30-50 | 98.5 | 0.014 | 7.6 | 3.5 | 1.9 | 0.3% |
| C30-65 | 99.0 | 0.015 | 16 | 4.5 | 2.3 | 2.1% |
| C40-35 | 98.5 | 0.015 | 8.5 | 3.0 | 2.0 | 0.9% |
| C40-50 | 98.5 | 0.010 | 16.0 | 3.6 | 2.4 | 4.6% |
| C40-65 | 98.6 | 0.013 | 21.0 | 3.5 | 2.6 | 6.5% |
| C50-35 | 99.1 | 0.005 | 40.0 | 4.0 | 2.8 | 1.4% |
| C50-50 | 98.5 | 0.008 | 18.0 | 3.8 | 2.6 | 0.7% |
| C50-65 | 98.6 | 0.009 | 20.0 | 3.5 | 2.7 | 4.1% |
| C60-35 | 98.8 | 0.010 | 15.0 | 2.5 | 2.6 | 2.9% |
| C60-50 | 99.4 | 0.012 | 15.0 | 3.0 | 2.6 | 3.1% |
| C60-65 | 99.6 | 0.015 | 15.0 | 3.2 | 2.6 | 5.2% |
| C30-65-1 | 98.5 | 0.011 | 15.0 | 6.0 | 2.3 | 6.2% |
| C30-65-3 | 99.4 | 0.010 | 19.0 | 6.0 | 2.3 | 4.2% |
| C30-65-6 | 99.5 | 0.011 | 25.0 | 6.0 | 2.3 | 2.2% |
| C60-65-1 | 98.5 | 0.016 | 10.0 | 3.0 | 2.3 | 0.05% |
| C60-65-3 | 99.0 | 0.016 | 12.0 | 4.0 | 2.3 | 2.9% |
| C60-65-6 | 99.0 | 0.015 | 13.0 | 5.0 | 2.3 | 5.6% |
| Age (Days) | Average Pore Diameter (nm) | Median Pore Diameter (nm) | Critical Diameter of Capillary (nm) | Porosity (%) | D (mm2/h) | |
|---|---|---|---|---|---|---|
| C30-65 | 3 | 24.45 | 65.17 | 61.79 | 22.52 | 13.21 |
| 7 | 21.94 | 46.13 | 52.30 | 20.32 | 10.24 | |
| 28 | 19.30 | 39.04 | 40.50 | 16.32 | 14.92 | |
| C30-65-1 | 3 | 26.20 | 76.10 | 74.12 | 25.54 | 12.96 |
| 7 | 24.39 | 52.88 | 59.49 | 24.31 | 11.75 | |
| 28 | 20.58 | 45.25 | 52.34 | 19.21 | 13.19 | |
| C30-65-3 | 3 | 30.17 | 82.02 | 79.03 | 27.56 | 11.22 |
| 7 | 27.41 | 64.04 | 66.60 | 25.64 | 11.34 | |
| 28 | 23.42 | 59.51 | 55.07 | 21.61 | 15.45 | |
| C30-65-6 | 3 | 32.26 | 93.27 | 89.82 | 29.97 | 8.88 |
| 7 | 29.53 | 78.76 | 75.43 | 27.75 | 13.72 | |
| 28 | 26.35 | 64.83 | 62.46 | 23.84 | 16.31 | |
| C60-65 | 3 | 21.35 | 53.61 | 44.13 | 21.52 | 10.08 |
| 7 | 17.41 | 36.53 | 29.55 | 18.47 | 17.80 | |
| 28 | 13.50 | 30.19 | 21.23 | 13.94 | 28.67 | |
| C60-65-1 | 3 | 24.87 | 57.81 | 53.65 | 24.83 | 13.30 |
| 7 | 22.28 | 43.07 | 33.95 | 21.13 | 20.58 | |
| 28 | 17.14 | 36.83 | 26.78 | 17.74 | 17.25 | |
| C60-65-3 | 3 | 27.85 | 66.13 | 57.29 | 25.94 | 11.89 |
| 7 | 24.75 | 51.46 | 37.99 | 22.88 | 17.49 | |
| 28 | 19.10 | 39.23 | 31.00 | 19.01 | 19.84 | |
| C60-65-6 | 3 | 30.01 | 80.45 | 68.58 | 27.92 | 7.88 |
| 7 | 27.20 | 59.36 | 46.34 | 25.15 | 8.59 | |
| 28 | 19.90 | 45.04 | 38.91 | 21.41 | 12.69 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhou, F.; Li, W.; Hu, Y.; Huang, L.; Xie, Z.; Yang, J.; Wu, D.; Chen, Z. Moisture Diffusion Coefficient of Concrete under Different Conditions. Buildings 2023, 13, 2421. https://doi.org/10.3390/buildings13102421
Zhou F, Li W, Hu Y, Huang L, Xie Z, Yang J, Wu D, Chen Z. Moisture Diffusion Coefficient of Concrete under Different Conditions. Buildings. 2023; 13(10):2421. https://doi.org/10.3390/buildings13102421
Chicago/Turabian StyleZhou, Fengbin, Wenhao Li, Ying Hu, Lepeng Huang, Zhuolin Xie, Jun Yang, Daifeng Wu, and Zhonghao Chen. 2023. "Moisture Diffusion Coefficient of Concrete under Different Conditions" Buildings 13, no. 10: 2421. https://doi.org/10.3390/buildings13102421
APA StyleZhou, F., Li, W., Hu, Y., Huang, L., Xie, Z., Yang, J., Wu, D., & Chen, Z. (2023). Moisture Diffusion Coefficient of Concrete under Different Conditions. Buildings, 13(10), 2421. https://doi.org/10.3390/buildings13102421






