Structural Health Monitoring of a Brazilian Concrete Bridge for Estimating Specific Dynamic Responses
Abstract
:1. Introduction
2. Case Study
2.1. Brazilian Concrete Bridge
2.2. Vehicle Loadings
2.3. Structural Health Monitoring (SHM)
2.4. Definition of the Equivalent Beam
3. VBI System
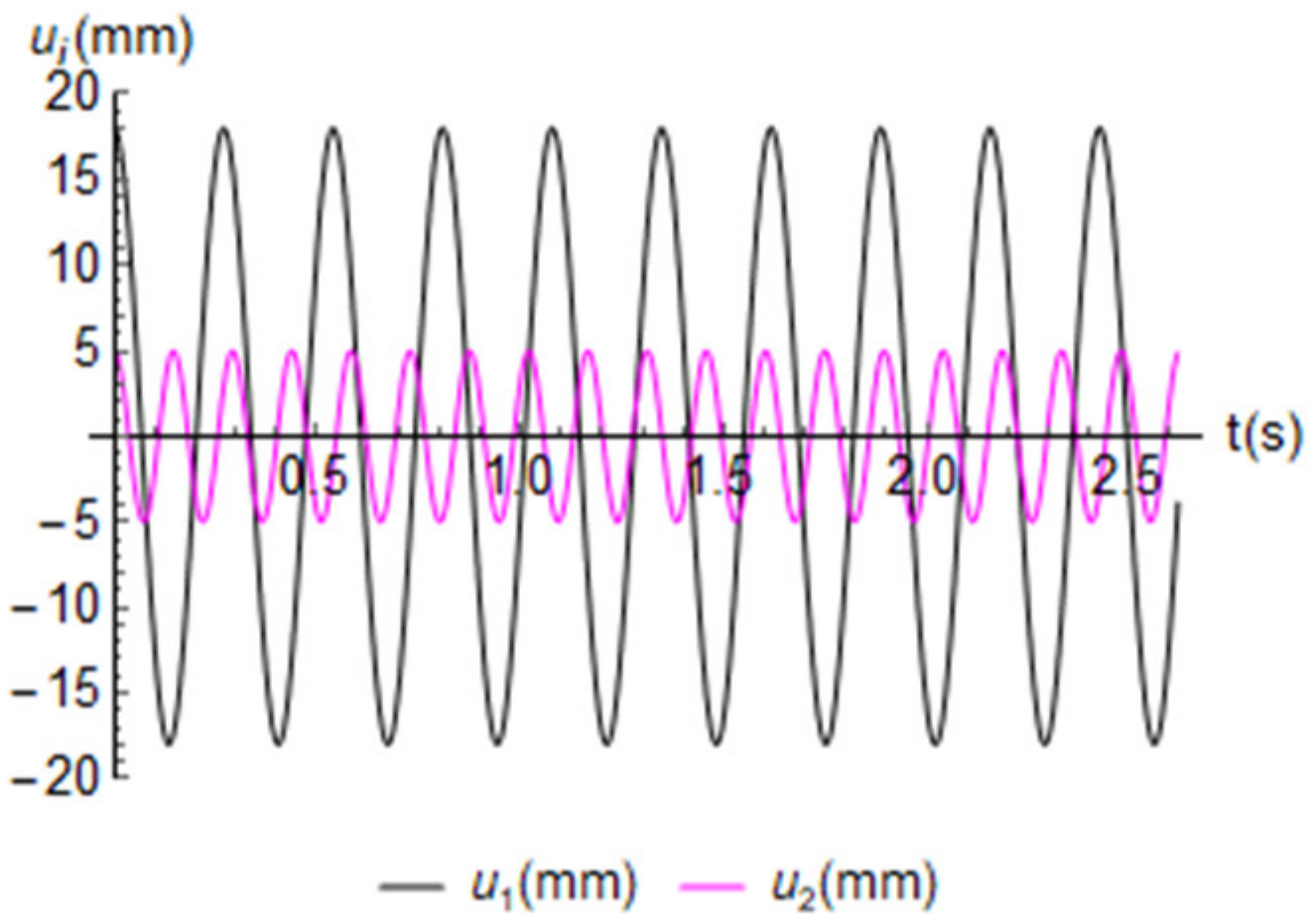
3.1. Modified Suspended Rigid BEAM Model
3.1.1. Bridge Response
3.1.2. Vehicle Response
3.2. Sprung Mass Model
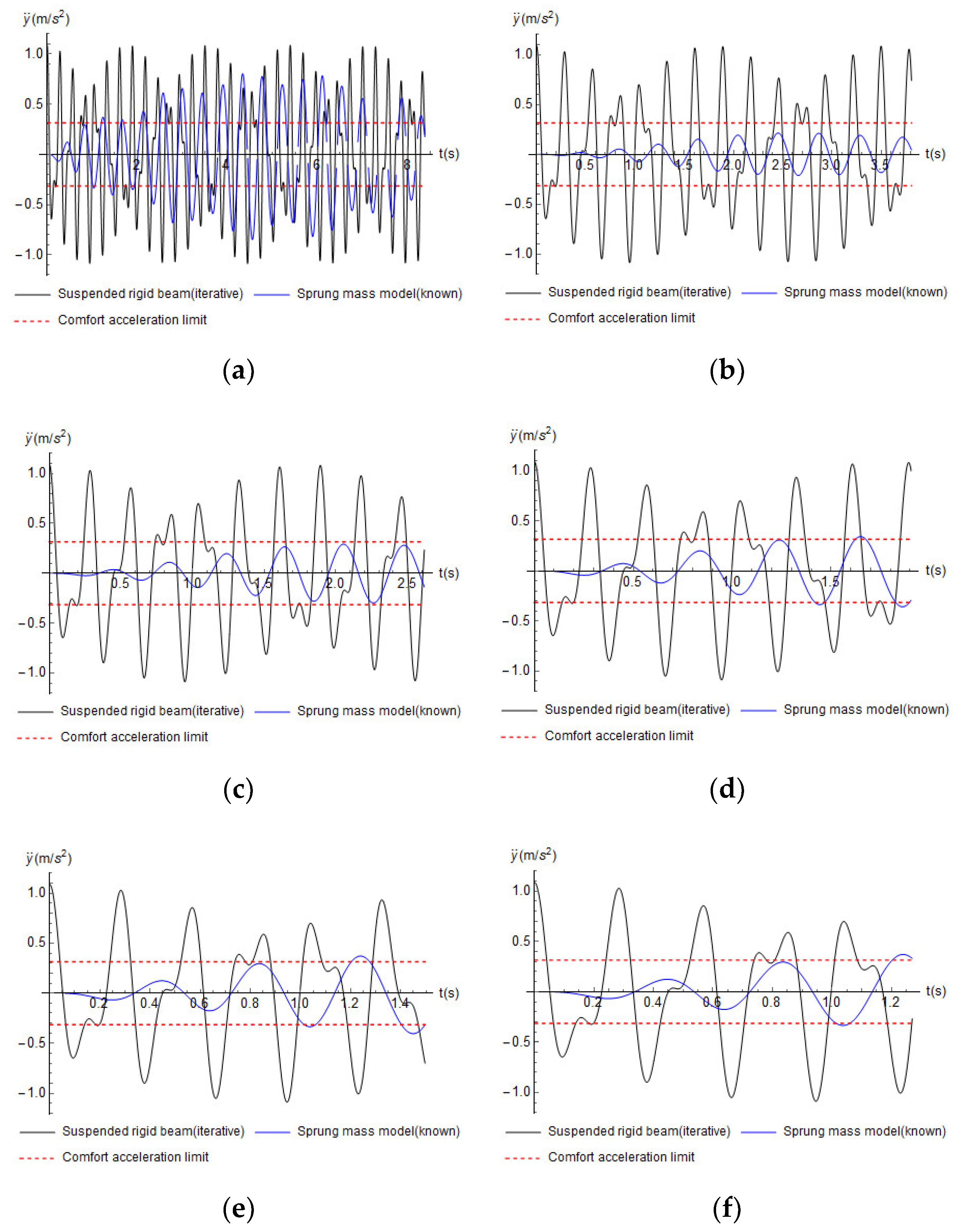
3.3. Additional Effects for VBI
3.3.1. Vehicle Vertical Accelerations
3.3.2. Pavement Irregularities
- (1)
- The contribution of the IM factors due to irregularities could be low. When considering three imperfections, in [70], it was shown as the total 2D irregularities are about ±4.0 mm. In [11], a more accurate analysis by considering 3D pavement irregularities was carried out, estimating an amplitude of about ±5.0 mm. In [38,39], it was shown that the irregularities affect the vertical bridge displacements little; in fact, the response obtained accounting for the irregularities is very similar to the analytical solutions without irregularities. However, for larger amplitude (e.g., > ±15.0 mm), the irregularity effects should not be neglected, as shown in [12]. In [34], road surface irregularities in the lateral directions can quite amplify the IM factor;
- (2)
- Most codes do not explicitly account for the irregularities; therefore, they are not considered in design practice for bridges. Codes provide equations and methods for new bridges where the pavement is considered of a very good quality and under standard maintenance (i.e., class A [72]). The irregularities are considered in, e.g., Spanish code [28] and European code [54] using a factor between 0.50 and 1.0, and in the literature [30], where is proposed a factor between 0.70 and 6.0. However, in [28,54], the irregularity contribution on the IM factors is very small, e.g., for the studied bridge, it is 0.42%.
3.4. IM Factor
4. Analyses and Results
4.1. Calibration and Some Structural Responses
4.2. Other Structural Responses
4.3. IM Factors
5. Conclusions
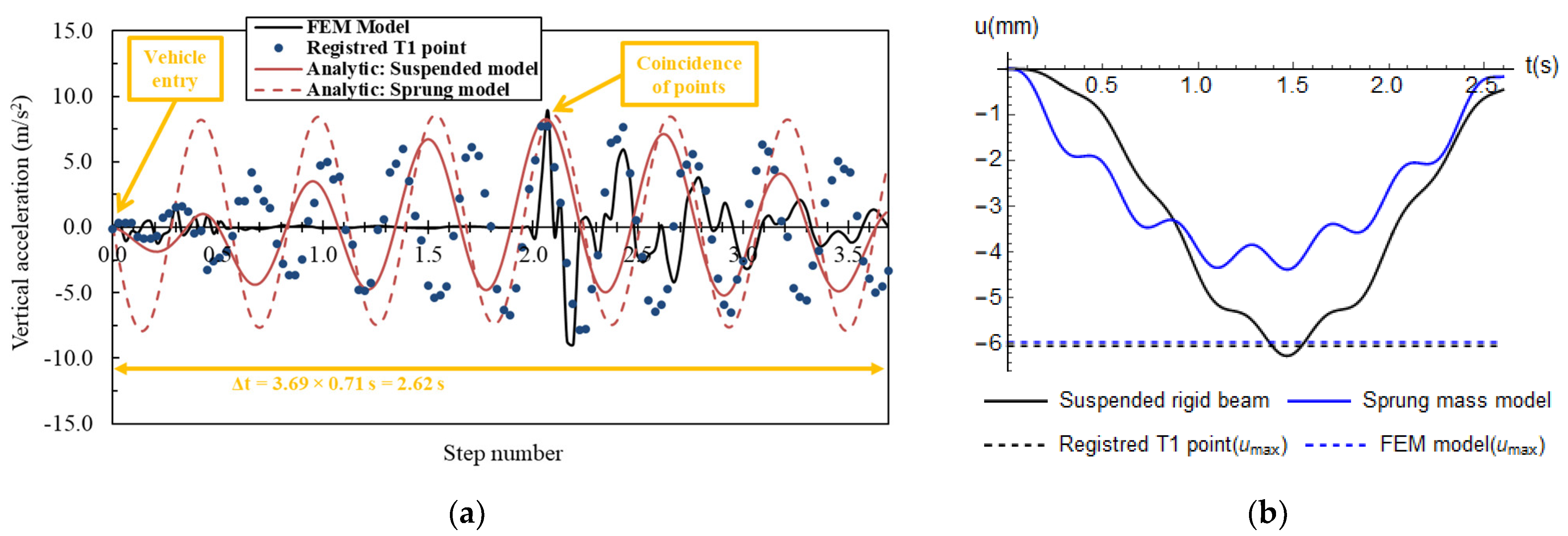
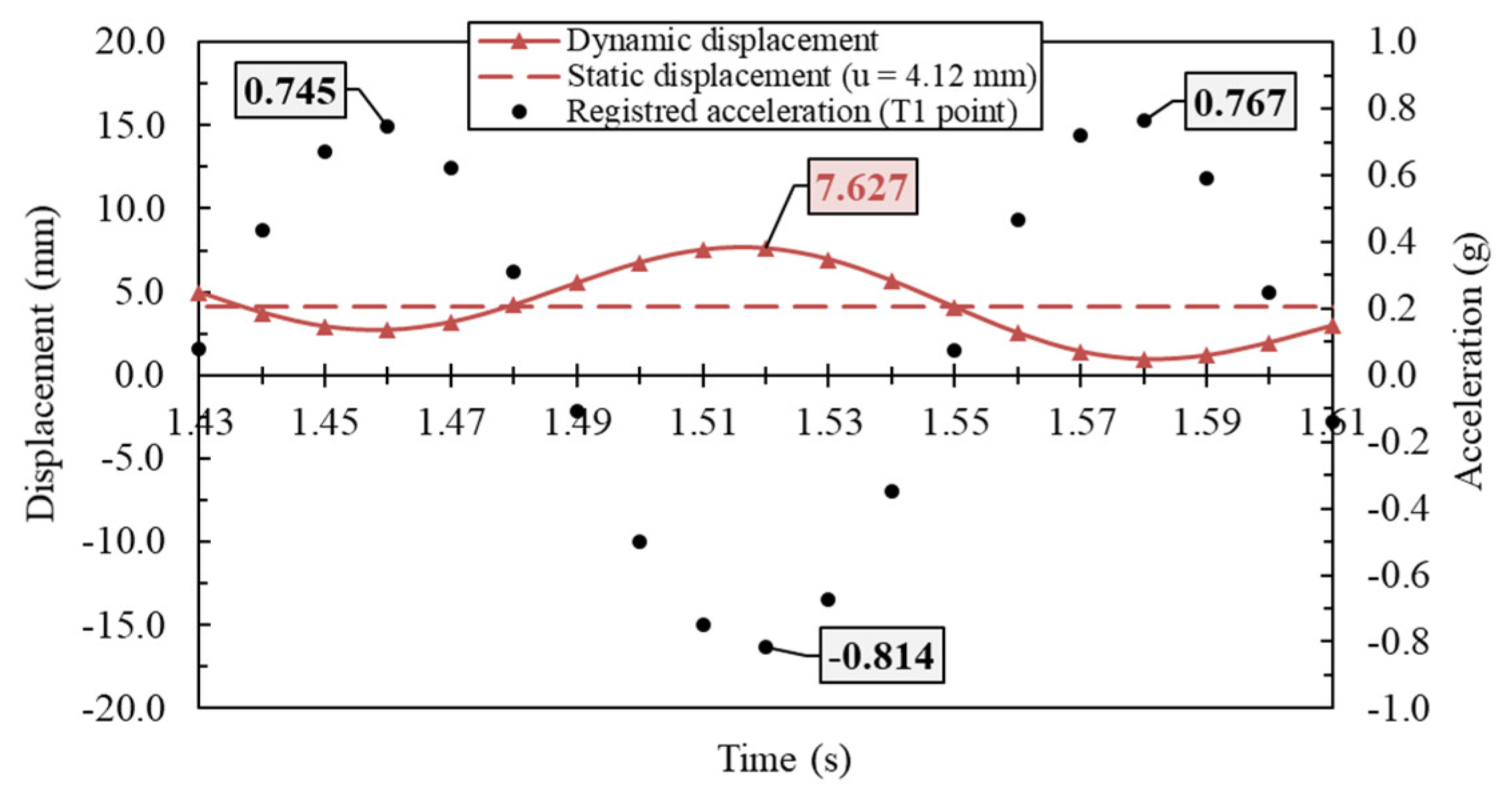
- The real test measurements of the Brazilian bridge for 8 months consisted of three phases: registration and elaboration of accelerations from T1 and T2 sensors and visualisation of collected data by using a 4G mobile phone each ~2.0 min to a predefined cloud [66]. Data were filtered and corrected to eliminate noise interferences for obtaining reliable responses. The two-axle 2C vehicle with v = 16.0 m/s was used for dynamic test. The monitoring provided a maximum acceleration and displacement of the bridge of about 8.0 m/s2 and 0.60 cm, respectively;
- The FEM and modified analytical models were carried out to simulate the bridge and the vehicle response. These models accounted for the geometrical and mechanical characteristics of the system. From these models, not only the bridge displacements were obtained but also the vehicle accelerations; for this, proposed equations provided more conservative vertical accelerations with values up to about three times the standard value of 0.315 m/s2. With respect to the registered value of 6.0 mm, other models provide a difference of about 1.0% (FEM), 20% (sprung model) and 5% (suspended model), indicating that the FEM and modified suspended models well estimate the bridge response;
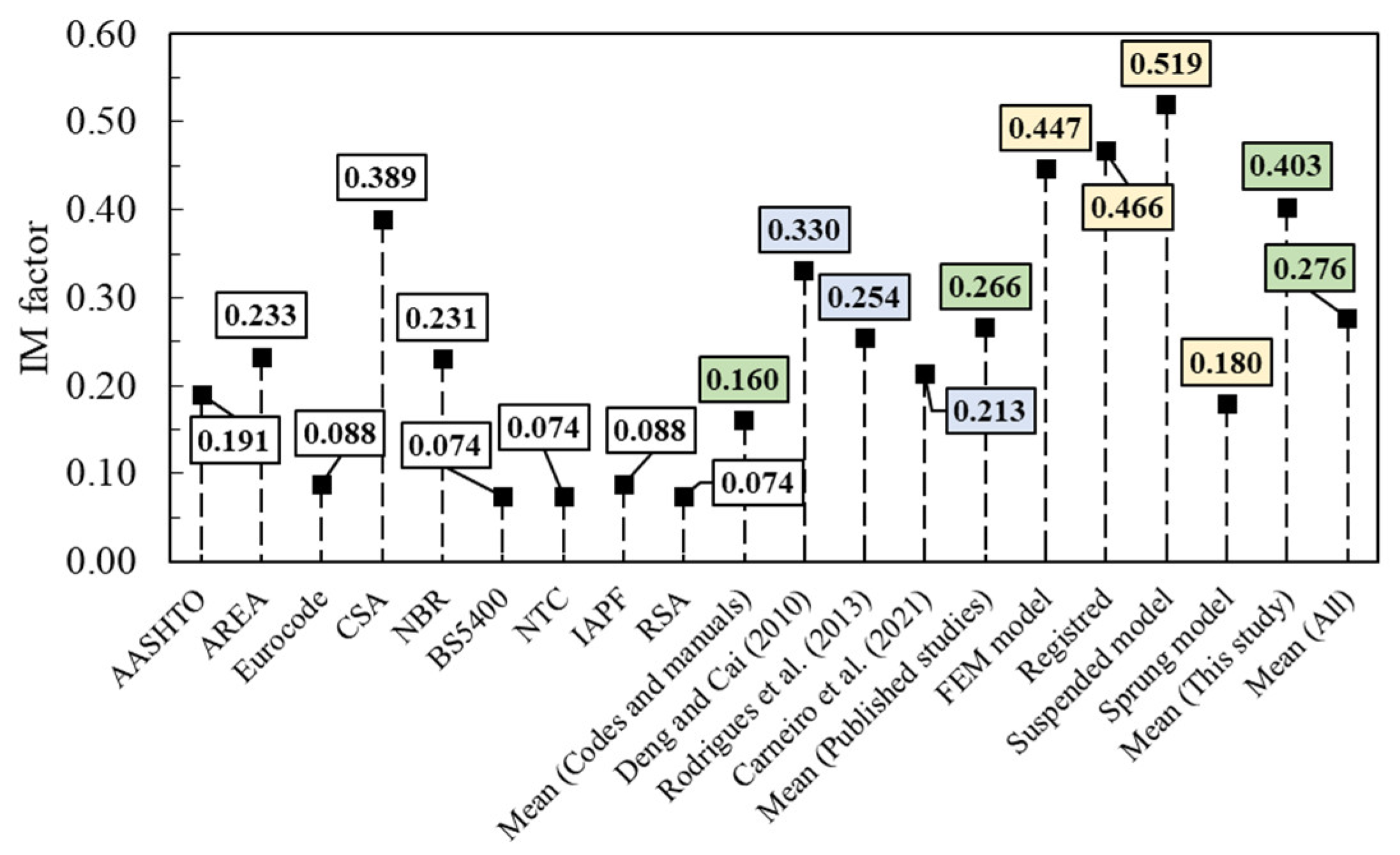
- From the codes and literature, several IM factors were calculated for the studied bridge. The results show that a monitored IM factor (IM = 0.403) is 2.5 greater than IM from codes (i.e., 2.5 × 0.16). Moreover, the results show that a unique way to estimate IMs does not exist; therefore, more accurate research should be developed, and “in-situ” studies for bridges are necessary.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Thierry, J.A. The Bailey bridge. Mil. Eng. 1946, 38, 96–103. [Google Scholar]
- Alexander, N.A.; Kashani, M.M. Exploring bridge dynamics for ultra-high-speed, Hyperloop, trains. Structures 2018, 14, 69–74. [Google Scholar] [CrossRef] [Green Version]
- Tadeu, A.; Romero, A.; Bandeira, F.; Pedro, F.; Dias, S.; Serra, M.; Brett, M.; Galvin, P. Theoretical and experimental analysis of the quasi-static and dynamic behaviour of the world’s longest suspension footbridge in 2020. Eng. Struct. 2022, 253, 1–15. [Google Scholar] [CrossRef]
- Tadeu, A.; da Silva, F.M.; Ramezani, B.; Romero, A.; Skerget, L.; Bandeira, F. Experimental and numerical evaluation of the wind load on the 516 Arouca pedestrian suspension bridge. J. Wind Eng. Ind. Aerodyn. 2022, 220, 1–13. [Google Scholar] [CrossRef]
- Liu, K.; Zhou, H.; Wang, Y.Q.; Shi, Y.J.; De Roeck, G. Fatigue assessment of a composite railway bridge for high speed trains. Part II: Conditions for which a dynamic analysis is needed. J. Constr. Steel Res. 2013, 82, 246–254. [Google Scholar] [CrossRef]
- Xia, H.; Zhang, N. Dynamic analysis of railway bridge under high-speed trains. Comput. Struct. 2005, 83, 1891–1901. [Google Scholar] [CrossRef]
- Galvín, P.; Romero, A.; Moliner, E.; Martínez-Rodrigo, M.D. Two FE models to analyse the dynamic response of short span simply-supported oblique high-speed railway bridges: Comparison and experimental validation. Eng. Struct. 2018, 167, 48–64. [Google Scholar] [CrossRef]
- Xia, H.; Zhang, N.; De Roeck, G. Dynamic analysis of high speed railway bridge under articulated trains. Comput. Struct. 2003, 81, 2467–2478. [Google Scholar] [CrossRef]
- Firus, A.; Schneider, J.; Berthold, H.; Albinger, M.; Seyfarth, A. Parameter identification of a biodynamic walking model for human-structure interaction. In Proceedings of the 9th International Conference on Bridge Maintenance, Safety and Management, IABMAS, Melbourne, Australia, 9–13 July 2018. [Google Scholar]
- Cai, C.; He, Q.; Zhu, S.; Zhai, W.; Wang, M. Dynamic interaction of suspension-type monorail vehicle and bridge: Numerical simulation and experiment. Mech. Syst. Signal Process. 2019, 118, 388–407. [Google Scholar] [CrossRef]
- Camara, A.; Kavrakov, I.; Nguyen, K.; Morgenthal, G. Complete framework of wind-vehicle-bridge interaction with random real surfaces. J. Sound Vib. 2019, 458, 197–217. [Google Scholar] [CrossRef]
- Agostinacchio, M.; Ciampa, D.; Olita, S. The vibrations induced by surface irregularities in road pavements—A Matlab approach. Eur. Transp. Res. Rev. 2014, 6, 267–275. [Google Scholar] [CrossRef] [Green Version]
- Huseynov, F.; Kim, C.; Obrien, E.J.; Brownjohn, J.M.W.; Hester, D.; Chang, K.C. Bridge damage detection using rotation measurements—Experimental validation. Mech. Syst. Signal Process. 2020, 135, 106380. [Google Scholar] [CrossRef]
- Lantsoght, E.O.L.; Veen, C.V.D.; Boer, D.A.; Hordijk, D.A. State-of-the-art in load testing of concrete bridges. Eng. Struct. 2017, 150, 231–241. [Google Scholar] [CrossRef] [Green Version]
- Leitão, F.N.; Da Silva, J.G.S.; Vellasco, P.C.G.S.; De Andrade, S.A.L.; De Lima, L.R.O. Composite (stell-concrete) highway bridge fatigue assessment. J. Constr. Steel Res. 2011, 67, 14–24. [Google Scholar] [CrossRef]
- Carneiro, A.L.; Portela, E.L.; Bittencourt, T.N.; Beck, A.T. Fatigue safety level provided by Brazilian design standards for a prestressed girder highway bridge. Ibracon Struct. Mater. J. 2021, 14, 1–24. [Google Scholar] [CrossRef]
- Wang, T.L.; Shahawy, M.; Huang, D.Z. Impact in highway prestressed concrete bridges. Comput. Struct. 1992, 44, 525–534. [Google Scholar] [CrossRef]
- Liu, C.; Huang, D.; Wang, T.L. Analytical dynamic impact study based on correlated road roughness. Comput. Struct. 2002, 80, 1639–1650. [Google Scholar] [CrossRef]
- Cai, C.S.; Shi, X.M.; Araujo, M.; Chen, S.R. Effect of approach span condition on vehicle-induced dynamic response of slab-on-girder road bridges. Eng. Struct. 2007, 29, 3210–3226. [Google Scholar] [CrossRef]
- Brady, S.P.; O’Brien, E.J.; Znidaric, A. Effect of vehicle velocity on the dynamic amplification of a vehicle crossing a simply supported bridge. J. Bridge Eng. 2006, 11, 241–249. [Google Scholar] [CrossRef]
- Siwowski, T. Fatigue assessment of existing riveted truss bridges: Case study. Bull. Pol. Acad. Sci. 2015, 63, 125–133. [Google Scholar] [CrossRef] [Green Version]
- Svendsen, B.T.; Gunnstein, T.F.; Ronnquist, A. Damage detection applied to a full-scale steel bridge using temporal moments. Shock. Vib. 2020, 2020, 3083752. [Google Scholar] [CrossRef]
- Siriwardane, S.; Ohga, M.; Dissanayake, R.; Taniwaki, K. Application of new damage indicator-based sequential law for remaining fatigue life estimation of railway bridges. J. Constr. Steel Res. 2008, 64, 228–237. [Google Scholar] [CrossRef]
- André, A.; Fernandes, J.; Ferraz, I.; Pacheco, P. New modular bridges solutions. Mater. Sci. Eng. 2018, 419, 012021. [Google Scholar] [CrossRef] [Green Version]
- Mousavi, A.A.; Zhang, C.; Masri, S.F.; Gholipour, G. Structural damage localization and quantification based on a CEEMDAN Hilbert transform neural network approach: A model steel truss bridge case study. Sensors 2020, 20, 1271. [Google Scholar] [CrossRef] [Green Version]
- Yang, Y.B.; Yau, G.B.; Wu, Y.S. Vehicle-Bridge Interaction Dynamics, with Applications to High-Speed Railways; World Scientific Publishing Co. Pte, Ltd.: Singapore, 2004; p. 565. [Google Scholar]
- Yang, Y.B.; Wu, Y.S. A versatile element for analyzing vehicle-bridge interaction response. Eng. Struct. 2001, 23, 452–469. [Google Scholar] [CrossRef]
- Yang, Y.B.; Zhang, B.; Wang, T.; Xu, H.; Wu, Y. Two-axle test vehicle for bridges: Theory and applications. Int. J. Mech. Sci. 2019, 152, 51–62. [Google Scholar] [CrossRef]
- Yang, Y.B.; Wang, Z.L.; Shi, K.; Xu, H.; Mo, X.Q.; Wu, Y.T. Two-axle test vehicle for damage detection for railway tracks modelled as simply supported beams with elastic foundation. Eng. Struct. 2020, 219, 1–13. [Google Scholar] [CrossRef]
- Deng, L.; Cai, C.S. Development of dynamic impact factor for performance evaluation of existing multi-girder concrete bridges. Eng. Struct. 2010, 32, 21–31. [Google Scholar] [CrossRef]
- Li, H.; Wu, G. Fatigue evaluation of steel bridge details integrating multi-scale dynamic analysis of coupled train-track-bridge system and fracture mechanics. Appl. Sci. 2020, 10, 3261. [Google Scholar] [CrossRef]
- Henchi, K.; Fafard, M.; Talbot, M. Dhatt, An efficient algorithm for dynamic analysis of bridges under moving vehicles using a coupled modal and physical components approach. J. Sound Vib. 1998, 212, 663–683. [Google Scholar] [CrossRef]
- Fish, J.; Belytschko, T. A First Course in Finite Elements; John Wiley & Sons, Ltd.: New York, NY, USA, 2007; p. 344. [Google Scholar]
- Oliva, J.; Goicolea, J.M.; Antolin, P.; Astiz, M.A. Relevance of a complete road surface description in vehicle-bridge interaction dynamics. Eng. Struct. 2013, 56, 466–476. [Google Scholar] [CrossRef]
- Araujo, A.O.; Pfeil, M.S.; Mota, H.C. Modelos analitico-numericos para interação dinâmica veiculo-pavimento-estrutura. In Proceedings of the XXXVII Iberian Latin American Congress on Computational Methods in Engineering, Cilamce 2016, Brasilia, Brazil, 6–9 November 2016. [Google Scholar]
- Dormand, J.; Prince, P. A family of embedded runge-kutta formulae. J. Comput. Appl. Math. 1980, 6, 19–26. [Google Scholar] [CrossRef] [Green Version]
- Shampine, L.; Reichelt, M. The matlab ode suite. SIAM J. Sci. Comput. 1997, 18, 1–22. [Google Scholar] [CrossRef] [Green Version]
- Koc, M.A.; Esen, I. Modelling and analysis of vehicle-structure-road coupled interaction considering structural flexibility. vehicle parameters and road roughness. J. Mech. Sci. Technol. 2017, 31, 2057–2074. [Google Scholar] [CrossRef]
- Greco, F.; Leonetti, P. Numerical formulation based on moving mesh method for vehicle-bridge interaction. Adv. Eng. Softw. 2018, 121, 75–83. [Google Scholar] [CrossRef]
- Montenegro, P.A.; Barbosa, D.; Carvalho, H.; Calçada, R. Dynamic effects on a train-bridge system caused by stochastically generated turbulent wind fields. Eng. Struct. 2020, 211, 1–16. [Google Scholar] [CrossRef]
- CAN/CSA-S6-06; Canadian Highway Bridge Design Code. Canadian Standards Association (CSA): Toronto, ON, Canada, 2010.
- Ma, L.; Zhang, W.; Han, W.S.; Liu, J.X. Determining the dynamic amplification factor of multi-span continuous box girder bridges in highways using vehicle-bridge interactions analyses. Eng. Struct. 2019, 181, 47–59. [Google Scholar] [CrossRef]
- Zacchei, E.; Lyra, P.; Stucchi, F. Pushover analysis for flexible and semi-flexible pile-supported wharf structures accounting the dynamic magnification factors due to torsional effects. Struct. Concr. 2020, 2020, 1–20. [Google Scholar] [CrossRef]
- Marrana, J.R.M.S.S. Analise Comparativa e Regulamentação Internacional em Ações de Trafego Rodoviário. Master’s Thesis, University of Porto, Porto, Portugal, 2016; p. 114. [Google Scholar]
- Nouri, M.; Mohammadzadeh, S. Probabilistic estimation of dynamic impact factor for masonry arch bridges using health monitoring data and new finite element method. Struct Control Health Monit. 2020, 27, 1–19. [Google Scholar] [CrossRef]
- American Association of State Highway and Transportation Officials (ASHTOO). AASHTO LRFD Bridge—Design Specifications; American Association of State Highway and Transportation Officials (ASHTOO): Washington, DC, USA, 2012; p. 1661. [Google Scholar]
- American Association of State Highway and Transportation Officials (ASHTOO). AASHTO: Standard Specifications for Highway Bridges; American Association of State Highway and Transportation Officials (ASHTOO): Washington, DC, USA, 2012; p. 740. [Google Scholar]
- BS 5400-2:1978; Steel, Concrete and Composite Bridges—Part 2: Specification for Loads. British Standard (BSI): London, UK, 1978.
- ABNT NBR 7188; Road and Pedestrian Live Load on Bridges, Viaducts, Footbridges and other Structures. Brazilian Association of Technical Standards (ABNT): Brasilia, Brazil, 2013.
- Minister of Infrastructure and Transport. Norme Tecniche per le Costruzioni (NTC), NTC 2008; Minister of Infrastructure and Transport: Rome, Italy, 2008. [Google Scholar]
- Ministério da Habilitação. Obras Públicas e Transportes, Regulamento de Solicitações em Edifícios e Pontes (RSA); Ministério da Habilitação: Lisbon, Portugal, 1983. [Google Scholar]
- Jung, H.; Kim, G.; Cheolwoo, P. Impact factors of bridges based on natural frequency for various superstructure types. KSCE J. Civ. Eng. 2013, 17, 458–464. [Google Scholar] [CrossRef]
- Mohseni, I.; Khalim, A.R.; Nikbakht, E. Effectiveness of skewness in dynamic impact factor of concrete multicell box-girder bridges subjected to truck loads. Arab. J. Sci. Eng. 2014, 39, 6083–6097. [Google Scholar] [CrossRef]
- EN 1991-3:1995; Eurocode 1: Actions on Structures—Part 2: Traffic Loads on Bridges. European Committee for standardization (CEN): Brussels, Belgium, 2003.
- Ministry of Development. Instrucción de Acciones a Considerer en Puentes de Ferrocarril (IAPF); Ministry of Development: Madrid, Spain, 2010. [Google Scholar]
- Rodrigues, J.F.S.; Casas, J.R.; Almeida, P.A.O. Fatigue-safety assessment of reinforced concrete (RC) bridges: Application to the Brazilian highway network. Struct. Infrastruct. Eng. 2013, 9, 601–616. [Google Scholar] [CrossRef]
- Chang, D.; Lee, H. Impact factors for simple-span highway girder bridges. J. Struct. Eng. 1992, 120, 1–12. [Google Scholar] [CrossRef]
- Commander, B. Evolution of bridge diagnostic load testing in the USA. Front. Built Environ. 2019, 5, 1–11. [Google Scholar] [CrossRef]
- Junior, A.B.S.; Lage, G.E.; Caruso, N.C. Desenvolvimento de um Sistema de Baixo Custo Para Monitoramento de Obras de Arte Especiais; Dissertation, Mauá Institute of Technology (MIT): São Caetano do Sul, Brazil, 2020; p. 121. [Google Scholar]
- Sap2000, version 15.0.0; Computers and Structures, Inc.: Walnut Creek, CA, USA, 2013.
- Autodesk InfraWorks, version 20 (student); Autodesk, Inc.: San Rafael, CA, USA, 2020.
- AutoCAD, version 2010; Autodesk, Inc.: San Rafael, CA, USA, 2010.
- González, A. Vehicle-bridge dynamic interaction using finite element modelling. In Finite Element Analysis; Moratal, D., Ed.; IntechOpen: London, UK, 2010; pp. 1–26. [Google Scholar]
- Pedro, R.L.; Demarche, J.; Miguel, L.F.F.; Lopez, R.H. An efficient approach for the optimization of simply supported steel-concrete composite I-girder bridges. Adv. Eng. Softw. 2017, 112, 31–45. [Google Scholar] [CrossRef]
- Ftool Software, Version 4.00.00 Basic. 2017. Available online: https://www.ftool.com.br/Ftool/ (accessed on 1 January 2021).
- Cloud. Available online: https://smartcampus.maua.br/node/dash/#!/30?socketid=22aIKS8ebiLaf2lzAA0J (accessed on 1 January 2021).
- PRISM Software, Earthquake Engineering Research Group, Department of Architectural Engineering, INHA University, South Korea. Available online: http://sem.inha.ac.kr/prism/ (accessed on 1 January 2021).
- Ghindea, C.L.; Cruciat, R.I.; Racanel, I.R. Dynamic test of a bridge over the Danube—Black Sea Channel at Agigea. Mater. Tools Proc. 2019, 12, 491–498. [Google Scholar] [CrossRef]
- Gonzalez, A.; Rattigan, P.; Obrien, E.J.; Caprani, C. Determination of bridge lifetime dynamic amplification factor using finite element analysis of critical loading scenarios. Eng. Struct. 2008, 30, 2330–2337. [Google Scholar] [CrossRef]
- Calçada, R.; Montenegro, P.; Castro, M. Numerical Evaluation of the Dynamic Load Allowance Factor in from ASTHOO in Steel Modular Bridges from the Peru Provias Project: Probabilistic Approach; Technical Report for Faculdade de Engenharia da Universidade do Porto: Porto, Portugal, 2019. [Google Scholar]
- Clough, R.W.; Penzien, J. Dynamics of Structures, 3rd ed.; McGraw-Hill: New York, NY, USA, 2003; p. 752. [Google Scholar]
- ISO-8608:2016; Mechanical Vibration—Road Surface Profile—Reporting of Measured Data. International Organization for Standardization (ISO): Geneva, Switzerland, 2016; p. 44.
- Neves, S.G.M.; Azevedo, A.F.M.; Calçada, R. A direct method for analyzing the vertical vehicle-structures interaction. Eng. Struct. 2012, 34, 414–420. [Google Scholar] [CrossRef] [Green Version]
- Wolfram Mathematica 12, version number 12.0; Wolfram Research, Inc.: Champaign, IL, USA, 2019.
- Yang, Y.B.; Yau, J.D.; Hsu, L.C. Vibration of simple beams due to trains moving as high speeds. Eng. Struct. 1997, 19, 936–944. [Google Scholar] [CrossRef]

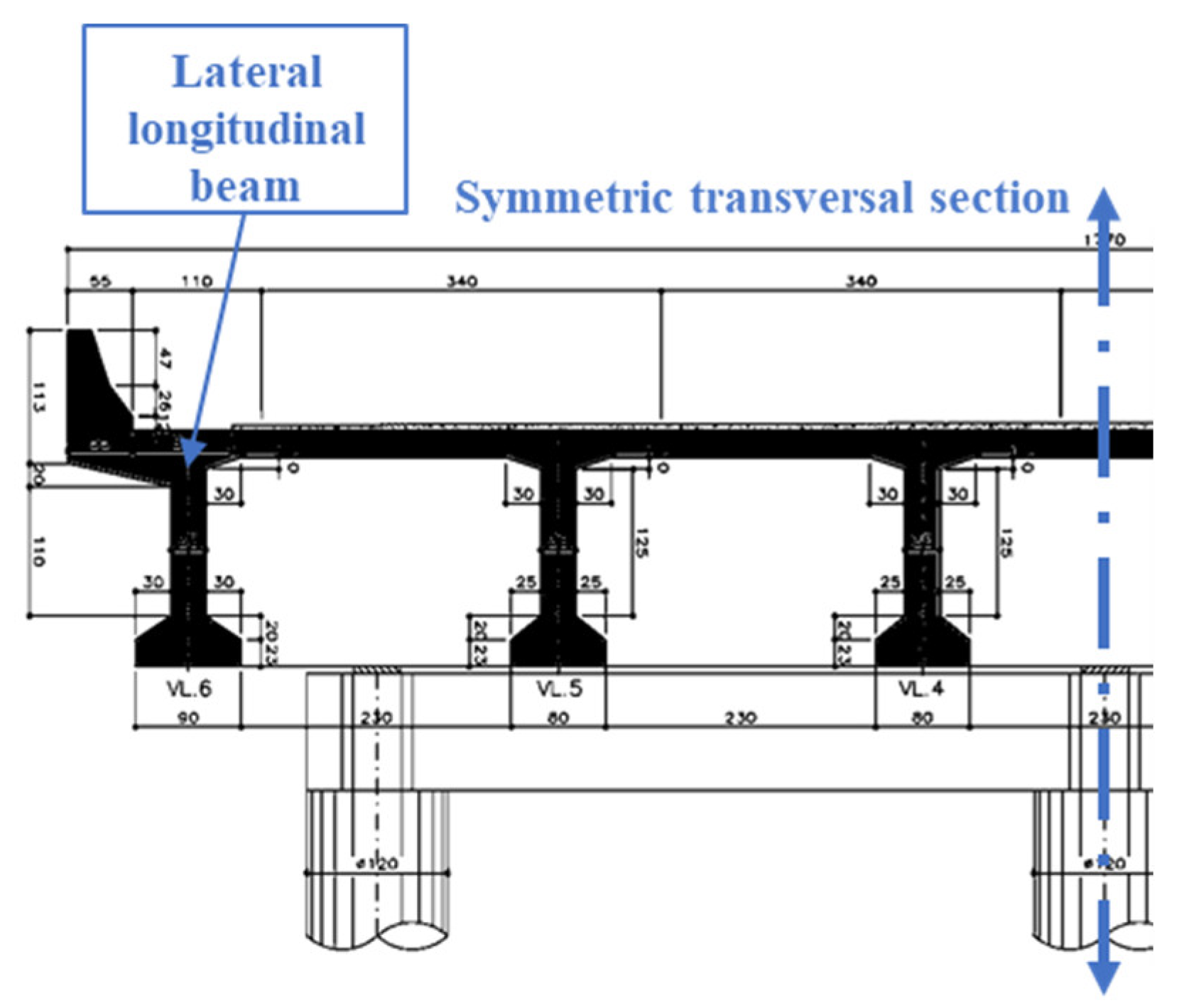



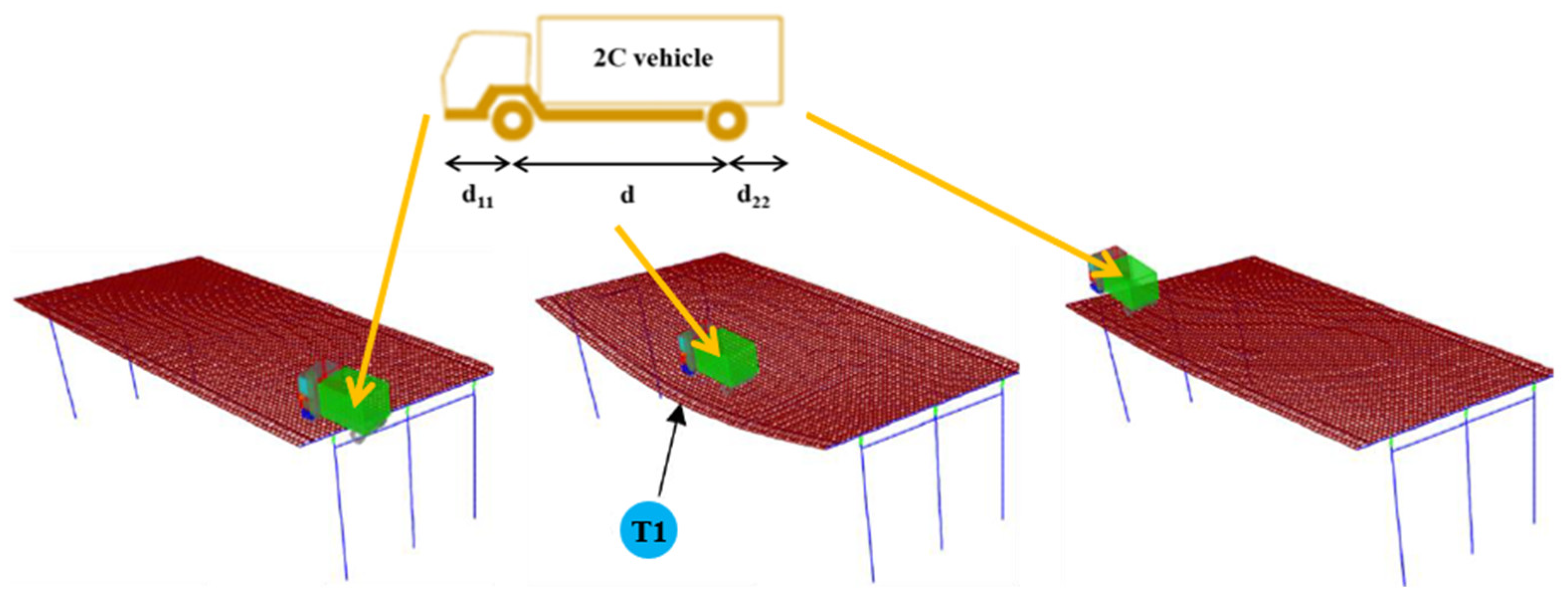
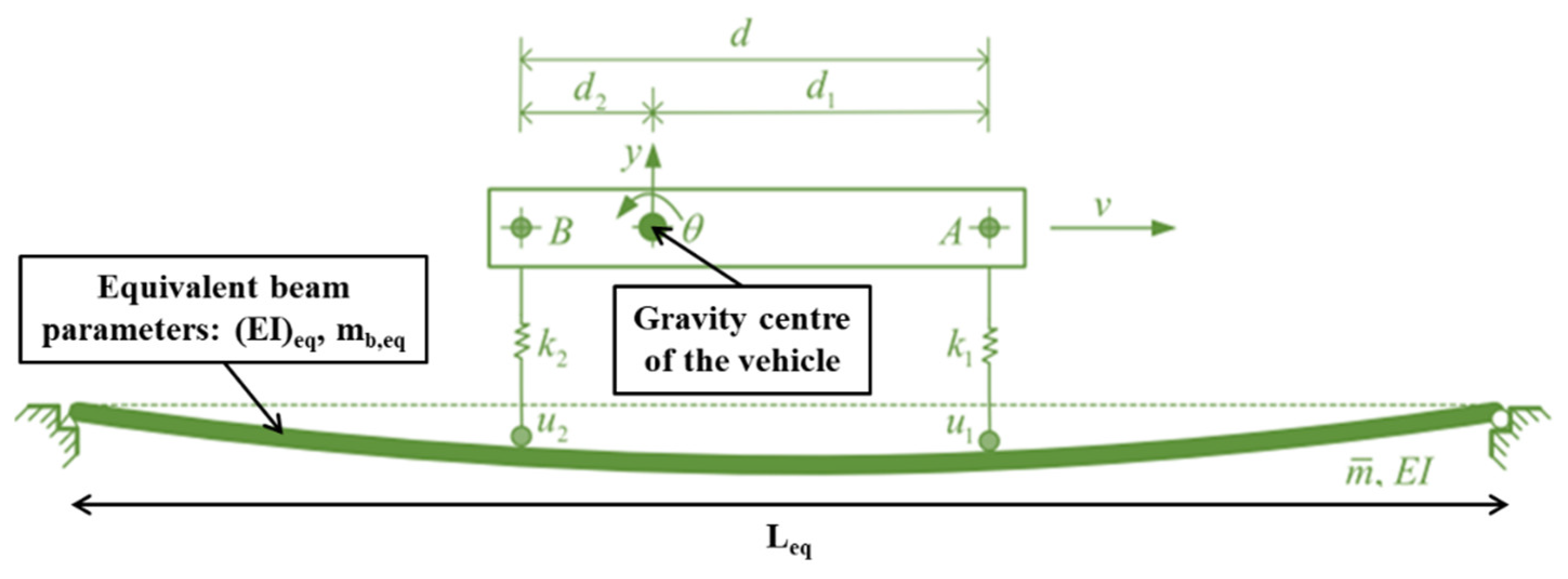
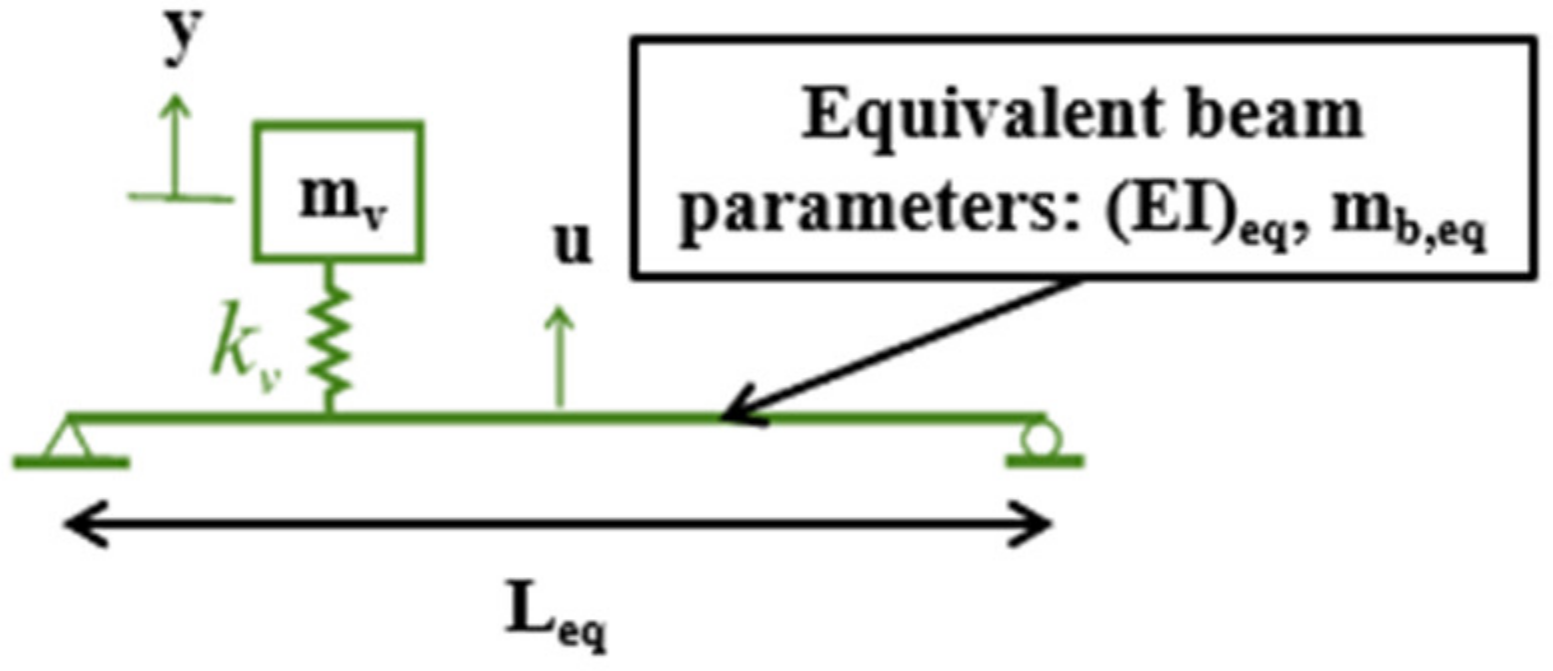

| Parameter | Value |
|---|---|
| Longitudinal length, L | 41.90 m |
| Transversal length, D | 17.70 m |
| Pile length | 15.0 m |
| Pavement weight | 24.0 kN/m3 a |
| Wheel-guard weight | 7.85 kN/m3 b |
| Bridge mass, mb | 8500.95 kN |
| Bridge frequency, fb | 2.421 Hz c |
| Bridge damping ratio | 5.0% [49] |
| Horizontal stiffness of one elastomeric pad | 24,000.0 kN/m d |
| Concrete elastic modulus, E | 50.0 GPa d |
| Concrete transversal modulus, G | 23.0 GPa |
| Parameter | Value |
|---|---|
| Vehicle mass, mv | 15,000.0 kg a |
| Front suspended mass, mv,1 | 772.0 kg a |
| Rear suspended mass, mv,2 | 1066.0 kg a |
| Length d11 | 0.50 m [56] |
| Length d | 4.50 m [56] |
| Length d22 | 2.0 m [56] |
| Front suspended frequency, fv,1 | 3.71 Hz b |
| Rear suspended frequency, fv,2 | 6.86 Hz b |
| Front suspended stiffness, k1 | 580.0 kN/m [35] |
| Rear suspended stiffness, k2 | 1180.0 kN/m [35] |
| Vehicle frequency, fv | 2.41 Hz c |
| Mass moment of inertia, Jc | ~25,000.0 kg m2 |
| Equivalent Parameter | Value |
|---|---|
| Concrete elastic modulus × inertia, (EI)eq | 1.94 × (50.0 GPa × 0.7255 m4) = 70.37 GN m2 a |
| Mass, mb,eq | 6714.34 kg/m b |
| Longitudinal length, Leq | 42.0 m c |
| Frequency, fb,eq | 2.40 Hz c |
| Reference | Specification for IM Factors |
|---|---|
| Codes and manuals | |
| American (AASHTO) [47] | It amplifies static live load stresses. It is estimated by L. IM < 0.30–0.33 a |
| American (AREA) [5] | It is estimated by L and D |
| Brazilian (NBR) [49] | It amplifies static vertical moving loads. It is estimated by L. IM < 0.35 b |
| English (BS5400) [48] | It amplifies static bending moments and shears. It is estimated by L. IM < 1.0 |
| Italian (NTC) [50] | It amplifies static stresses and displacements. It is estimated by L. IM < 1.0 |
| Portuguese (RSA) [51] | It amplifies static loads and transversal forces. It is estimated by L. IM < 1.0 |
| Spanish (IAPF) [55] | It amplifies static forces. It is estimated by L, v, fb |
| European (Eurocode) [54] | It amplifies static forces and moments. It is estimated by L, v, fb |
| Canadian (CSA) [41] | It amplifies static live loads. It is estimated by fb and the number of axles. IM < 0.40 |
| Published studies | |
| Deng and Cai (2010) [30] | It is estimated by L and pavement irregularities. IM < 0.33 a |
| Rodrigues et al. (2013) [56] | It is estimated by L, v, fb |
| Carneiro et al. (2021) [16] | It is estimated by L, v, fb |
| This study | It was defined by “in-situ” experimental tests, FEM and analytic VBI models |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zacchei, E.; Lyra, P.H.C.; Lage, G.E.; Antonine, E.; Soares, A.B., Jr.; Caruso, N.C.; de Assis, C.S. Structural Health Monitoring of a Brazilian Concrete Bridge for Estimating Specific Dynamic Responses. Buildings 2022, 12, 785. https://doi.org/10.3390/buildings12060785
Zacchei E, Lyra PHC, Lage GE, Antonine E, Soares AB Jr., Caruso NC, de Assis CS. Structural Health Monitoring of a Brazilian Concrete Bridge for Estimating Specific Dynamic Responses. Buildings. 2022; 12(6):785. https://doi.org/10.3390/buildings12060785
Chicago/Turabian StyleZacchei, Enrico, Pedro H. C. Lyra, Gabriel E. Lage, Epaminondas Antonine, Airton B. Soares, Jr., Natalia C. Caruso, and Cassia S. de Assis. 2022. "Structural Health Monitoring of a Brazilian Concrete Bridge for Estimating Specific Dynamic Responses" Buildings 12, no. 6: 785. https://doi.org/10.3390/buildings12060785
APA StyleZacchei, E., Lyra, P. H. C., Lage, G. E., Antonine, E., Soares, A. B., Jr., Caruso, N. C., & de Assis, C. S. (2022). Structural Health Monitoring of a Brazilian Concrete Bridge for Estimating Specific Dynamic Responses. Buildings, 12(6), 785. https://doi.org/10.3390/buildings12060785







