Springback Prediction of a Hot Stamping Component Based on the Area Fractions of Phases
Abstract
1. Introduction
2. Experimental Study
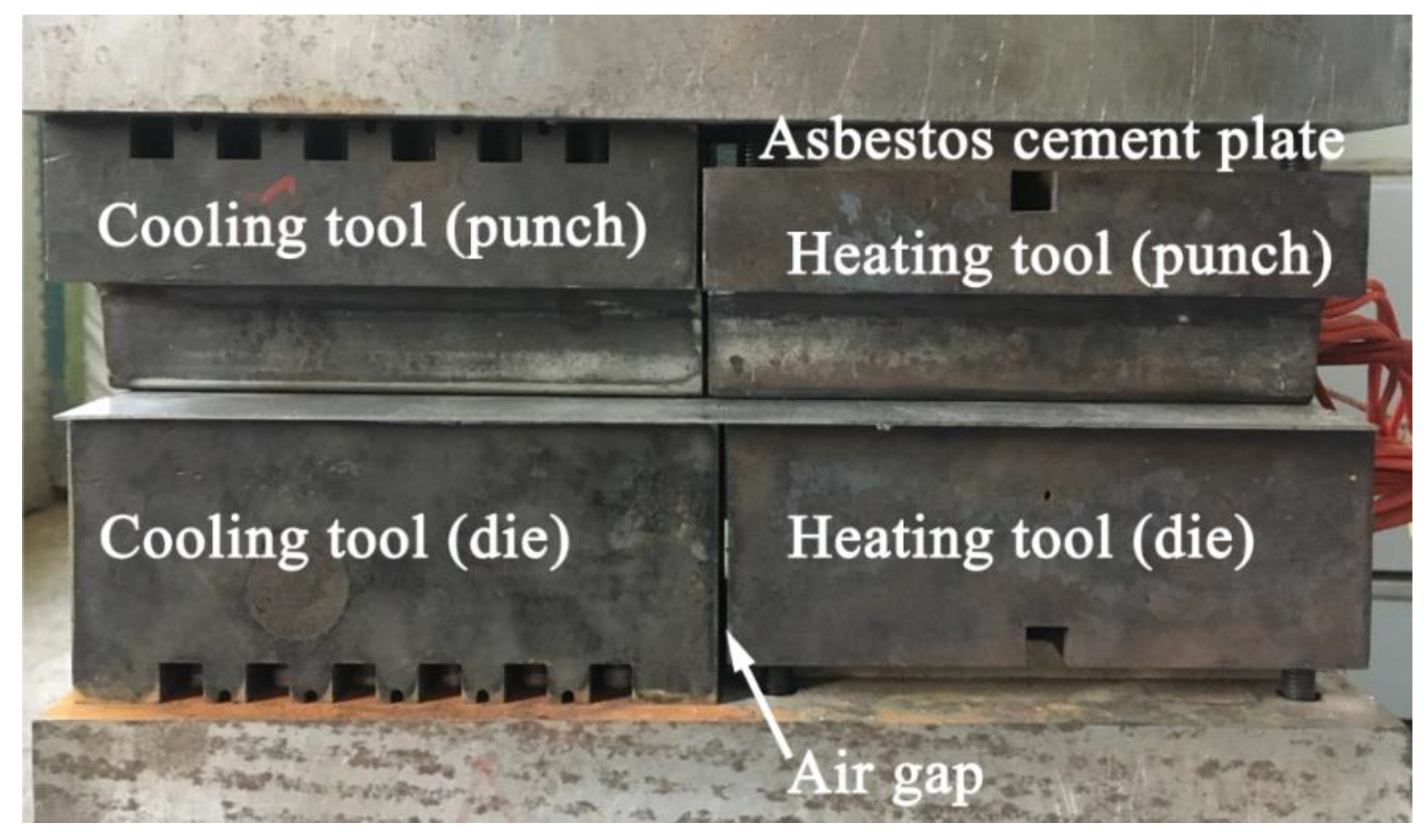
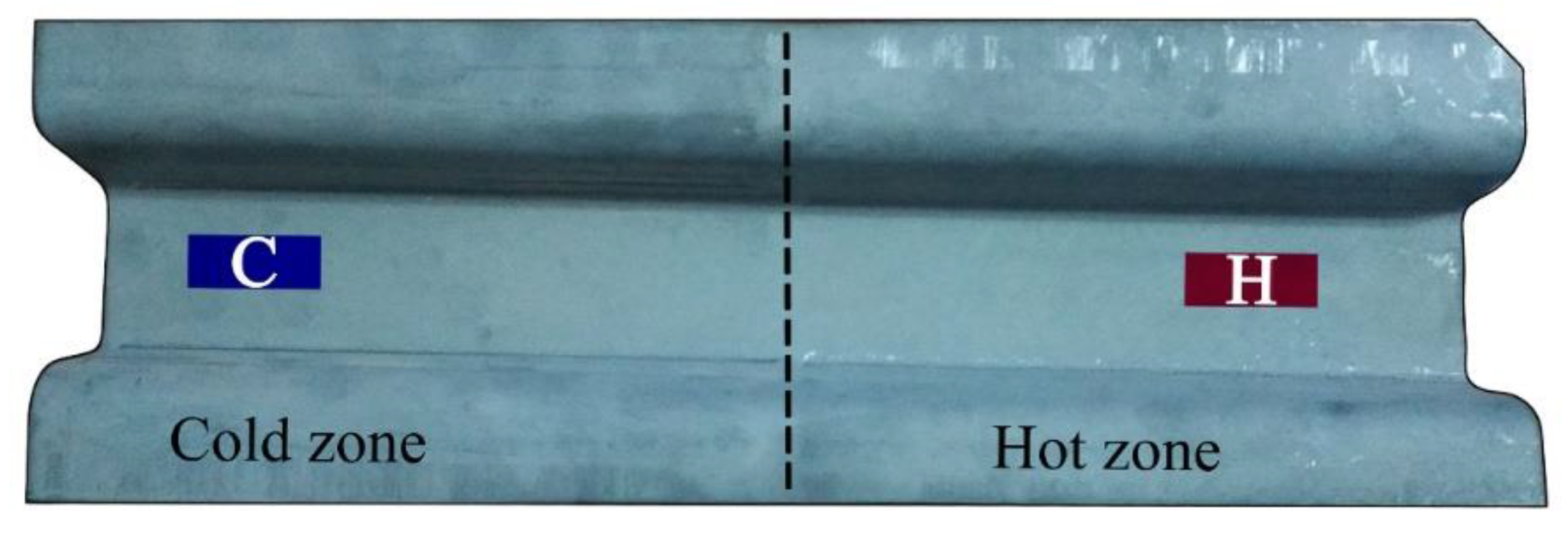
2.1. THS Experiments
2.2. Metallography
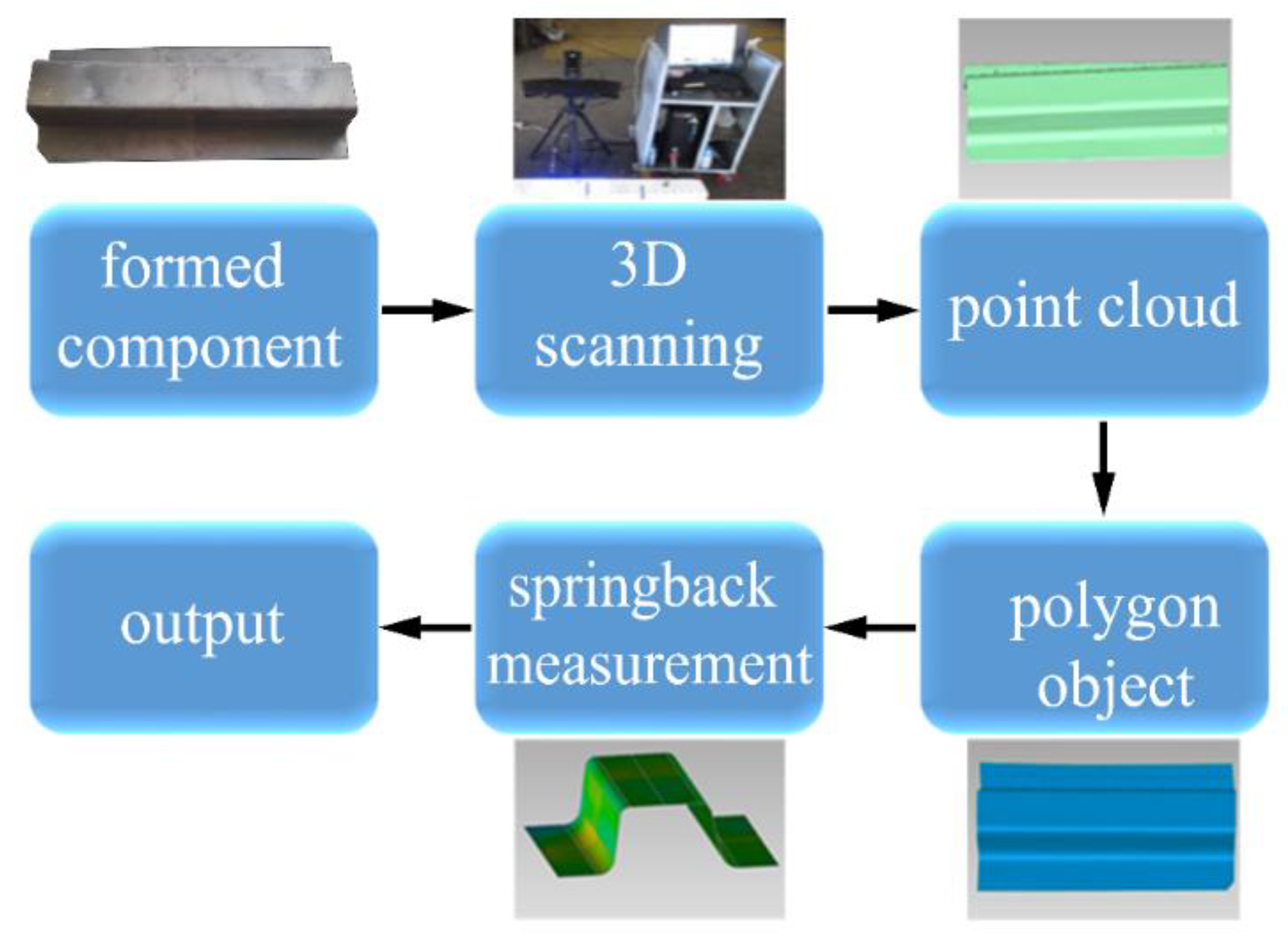
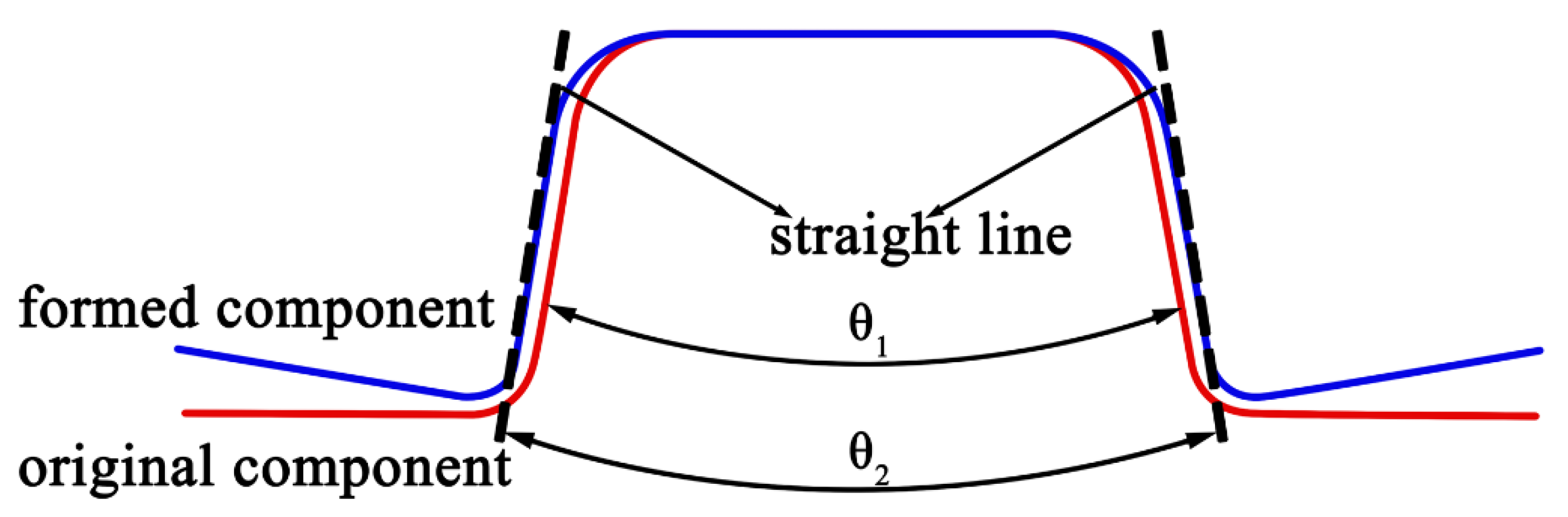
2.3. Springback Measurement
3. Results and Discussion
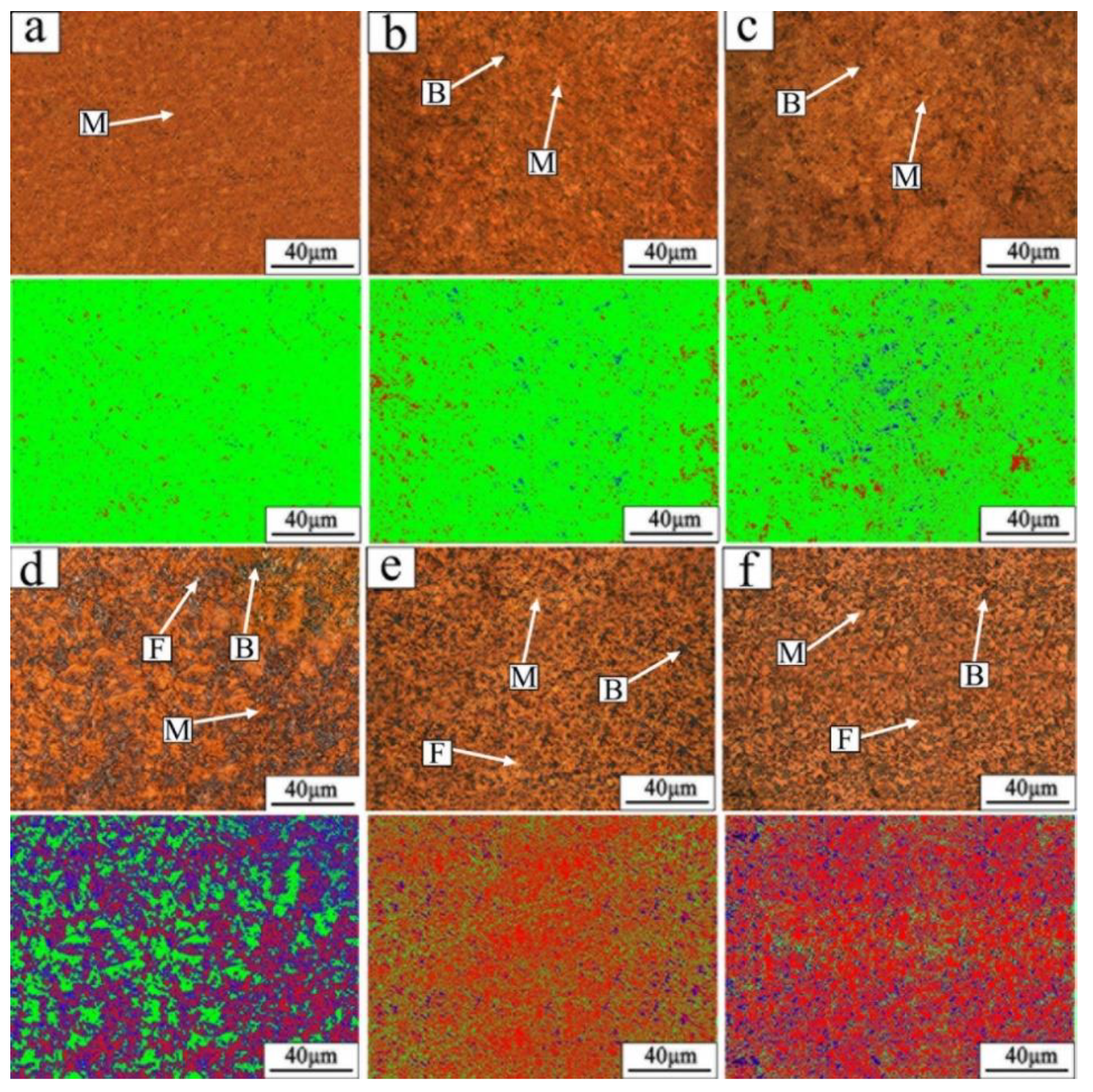
3.1. Quenched Phases Analysis
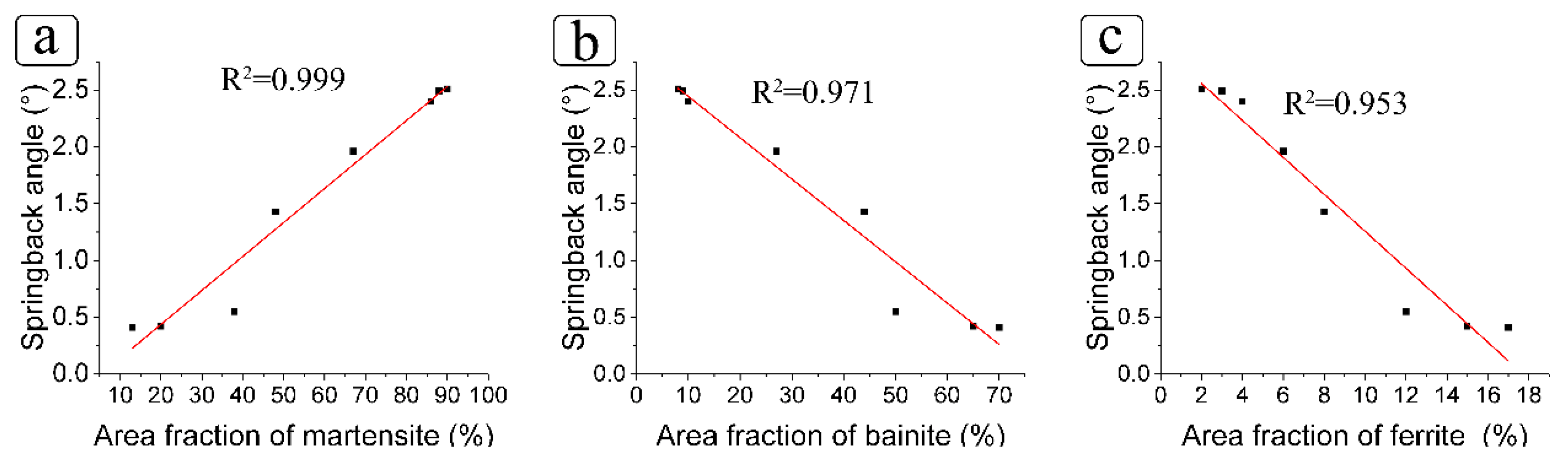
3.2. Springback Results
4. Conclusions
- (1)
- The quenched microstructure of the cold zone was almost full martensite. The quenched microstructure of the hot zone changed greatly with increasing heating tool temperature. The area fraction of martensite dropped dramatically at heating tool temperatures higher than 200 °C. When the heating tool temperature was 600 °C, martensite dropped to 13% and bainite increased to 70%. Ferrite gradually increased at temperatures ranging from 25 °C to 600 °C and its maximum was close to 17%.
- (2)
- The springback angle of the cold zone was large and remained unchanged with the increase of the heating tool temperature. When the heating tool temperature was higher than 300 °C, the springback angle of the hot zone decreased significantly. The springback angle was minimum and the change of it tended to be stable with the heating tool temperature over 550 °C.
- (3)
- The relationship between the area fractions of quenched phases and the springback angle was established by the optimization method. A relative error analysis revealed that the relationship could be used to accurately predict the springback of a tailored formed hot stamped component.
Author Contributions
Funding
Conflicts of Interest
References
- Mori, K.; Bariani, P.F.; Behrens, B.A.; Brosius, A.; Bruschi, S.; Maeno, T.; Merklein, M.; Yanagimoto, J. Hot stamping of ultra-high strength steel parts. Cirp. Ann-Manuf. Technol. 2017, 66, 755–777. [Google Scholar] [CrossRef]
- Karbasian, H.; Tekkaya, A.E. A review on hot stamping. J. Mater. Process. Technol. 2010, 210, 2103–2118. [Google Scholar] [CrossRef]
- Ying, L.; Zhao, X.; Dai, M.H.; Zhang, S.Z.; Hu, P. Crashworthiness design of quenched boron steel thin-walled structures with functionally graded strength. Int. J. Impact Eng. 2016, 95, 72–88. [Google Scholar] [CrossRef]
- Merklein, M.; Wieland, M.; Lechner, M.; Bruschi, S.; Ghiotti, A. Hot stamping of boron steel sheets with tailored properties. J. Mater. Process Technol. 2016, 228, 11–24. [Google Scholar] [CrossRef]
- Omer, K.; George, R.; Bardelcik, A.; Worswick, M.; Malcolm, S.; Detwiler, D. Development of a hot stamped channel section with axially tailored properties–experiments and models. Int. J. Mater. 2018, 11, 149–164. [Google Scholar] [CrossRef]
- Vrolijk, M.; Lorenz, D.; Porzner, H.; Holecek, M. Supporting lightweight design: Virtual modeling of hot stamping with tailored properties and warm and hot formed aluminium. Procedia Eng. 2017, 183, 336–342. [Google Scholar] [CrossRef]
- Chokshi, P.; Dashwood, R.; Hughes, D.J. Artificial neural network (ANN) based microstructural prediction model for 22MnB5 boron steel during tailored hot stamping. Comput. Struct. 2017, 190, 162–172. [Google Scholar] [CrossRef]
- Mu, Y.; Wang, B.; Zhou, J.; Huang, X.; Li, X. Hot stamping of boron steel using partition heating for tailored properties: Experimental trials and numerical analysis. Metall. Mater. Trans. A 2017, 48, 5467–5479. [Google Scholar] [CrossRef]
- Bardelcik, A.; Worswick, M.J.; Winkler, S.; Wells, M.A. A strain rate sensitive constitutive model for quenched boron steel with tailored properties. Int. J. Impact Eng. 2012, 50, 49–62. [Google Scholar] [CrossRef]
- Li, F.F.; Fu, M.W.; Lin, J.P. Effect of cooling path on the phase transformation of boron steel 22mnb5 in hot stamping process. Int. J. Adv. Manuf. Technol. 2015, 81, 1391–1402. [Google Scholar] [CrossRef]
- Omer, K.; Kortenaar, L.; Butcher, C.; Worswick, M.; Malcolm, S.; Detwiler, D. Testing of a hot stamped axial crush member with tailored properties experiments and models. Int. J. Impact Eng. 2017, 103, 12–28. [Google Scholar] [CrossRef]
- Zhou, M.B.; Tang, J.L.; Yang, J.; Wang, C.Y. Tailored properties of a novelly designed press hardened 22MnMoB steel. J. Iron Steel Res. Int. 2017, 24, 508–512. [Google Scholar] [CrossRef]
- Mu, Y.H.; Wang, B.Y.; Zhou, J.; Kang, Y.; Li, X.T. Heating parameters optimization of hot stamping by partition heating for tailored properties. ISIJ. Int. 2017, 57, 1442–1450. [Google Scholar] [CrossRef]
- Nakagawa, Y.; Mori, K.I.; Maeno, T. Springback-free mechanism in hot stamping of ultra-high-strength steel parts and deformation behaviour and quenchability for thin sheet. Int. J. Adv. Manuf. Techol. 2018, 95, 459–467. [Google Scholar] [CrossRef]
- Krinninger, M.; Opritescu, D.; Golle, R.; Volk, W. Experimental investigation of the influence of punch velocity on the springback behavior and the flat length in free bending. Procedia CIRP 2016, 41, 1066–1071. [Google Scholar] [CrossRef][Green Version]
- Bao, J.; Liu, H.; Xing, Z.; Song, B.; Yang, Y. Springback of hot stamping and die quenching with ultra-high-strength boron steel. Eng. Rev. 2013, 33, 151–156. [Google Scholar]
- Barcellona, A.; Palmeri, D.; Worswick, M. Hot forming of boron steels using heated and cooled tooling for tailored properties. Met. Mater. Trans. A 2009, 40, 1160–1174. [Google Scholar] [CrossRef]
- Merklein, M.; Svec, T. Effect of plastic hot deformation on the hardness and continuous cooling transformations of 22MnB5 microalloyed boron steel. In Proceedings of the 29th IDDRG International Conference, Graz, Austria, 31 May–2 June 2010. [Google Scholar]
- Nikravesh, M.; Naderi, M.; Akbari, G.H. Transformation kinetics of the hot stamping steel 22MnB5 in dependency of the applied deformation on the austenitic microstructure. Mater. Sci. Eng. A Struct. 2012, 540, 24–29. [Google Scholar] [CrossRef]
- Shipway, P.H.; Bhadeshia, H.K. The effect of small stresses on the kinetics of the bainite transformation. Mater. Sci. Eng. A Struct. 1995, 201, 143–149. [Google Scholar] [CrossRef]
- George, R.; Bardelcik, A.; Worswick, M.J. Hot forming of boron steels using heated and cooled tooling for tailored properties. J. Mater. Process Technol. 2012, 212, 2386–2399. [Google Scholar] [CrossRef]
- Bardelcik, A.; Salisbury, C.P.; Winkler, S.; Wells, M.A.; Worswick, M.J. Effect of cooling rate on the high strain rate properties of boron steel. Int. J. Impact Eng. 2010, 37, 694–702. [Google Scholar] [CrossRef]
- Åkerström, P.; Oldenburg, M. Austenite decomposition during press hardening of a boron steel-computer simulation and test. J. Mater. Process Technol. 2006, 174, 399–406. [Google Scholar] [CrossRef]

| C | Mn | B | Si | P | Cu | Ni | Cr | Al | Ti | Mo |
|---|---|---|---|---|---|---|---|---|---|---|
| 0.22 | 1.23 | 0.004 | 0.25 | 0.008 | 0.03 | 0.02 | 0.20 | 0.03 | 0.037 | <0.02 |
| Heating Tool Temperature (°C) | Measured Value (°) | Calculated Value (°) |
|---|---|---|
| 25 | 2.51 | 2.65 |
| 100 | 2.49 | 2.49 |
| 200 | 2.40 | 2.33 |
| 300 | 1.96 | 1.86 |
| 400 | 1.43 | 1.40 |
| 500 | 0.55 | 0.59 |
| 550 | 0.42 | 0.45 |
| 600 | 0.41 | 0.41 |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, X.; Yan, X.; Zhang, Z. Springback Prediction of a Hot Stamping Component Based on the Area Fractions of Phases. Metals 2019, 9, 694. https://doi.org/10.3390/met9060694
Li X, Yan X, Zhang Z. Springback Prediction of a Hot Stamping Component Based on the Area Fractions of Phases. Metals. 2019; 9(6):694. https://doi.org/10.3390/met9060694
Chicago/Turabian StyleLi, Xiangji, Xu Yan, and Zhiqiang Zhang. 2019. "Springback Prediction of a Hot Stamping Component Based on the Area Fractions of Phases" Metals 9, no. 6: 694. https://doi.org/10.3390/met9060694
APA StyleLi, X., Yan, X., & Zhang, Z. (2019). Springback Prediction of a Hot Stamping Component Based on the Area Fractions of Phases. Metals, 9(6), 694. https://doi.org/10.3390/met9060694




