Material and Damage Characterization of the Elastoplastic Response of the EK4 Deep Drawing Steel
Abstract
:1. Introduction
2. Materials and Methods
2.1. Material
2.2. Experimental Procedure
2.2.1. Tensile Tests
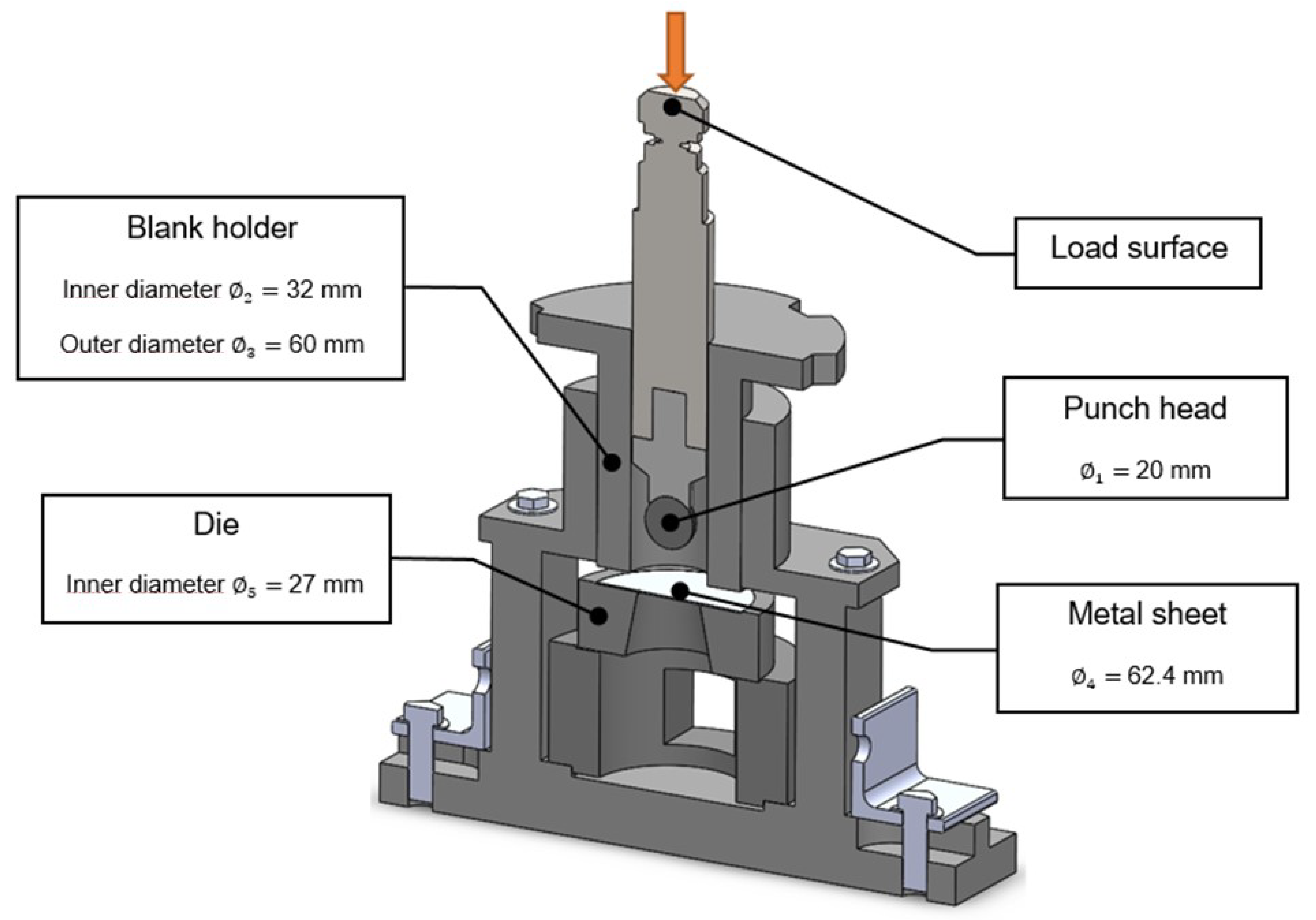
2.2.2. Erichsen Test
2.3. Constitutive Modelling
2.4. Damage Index
3. Results and Discussion
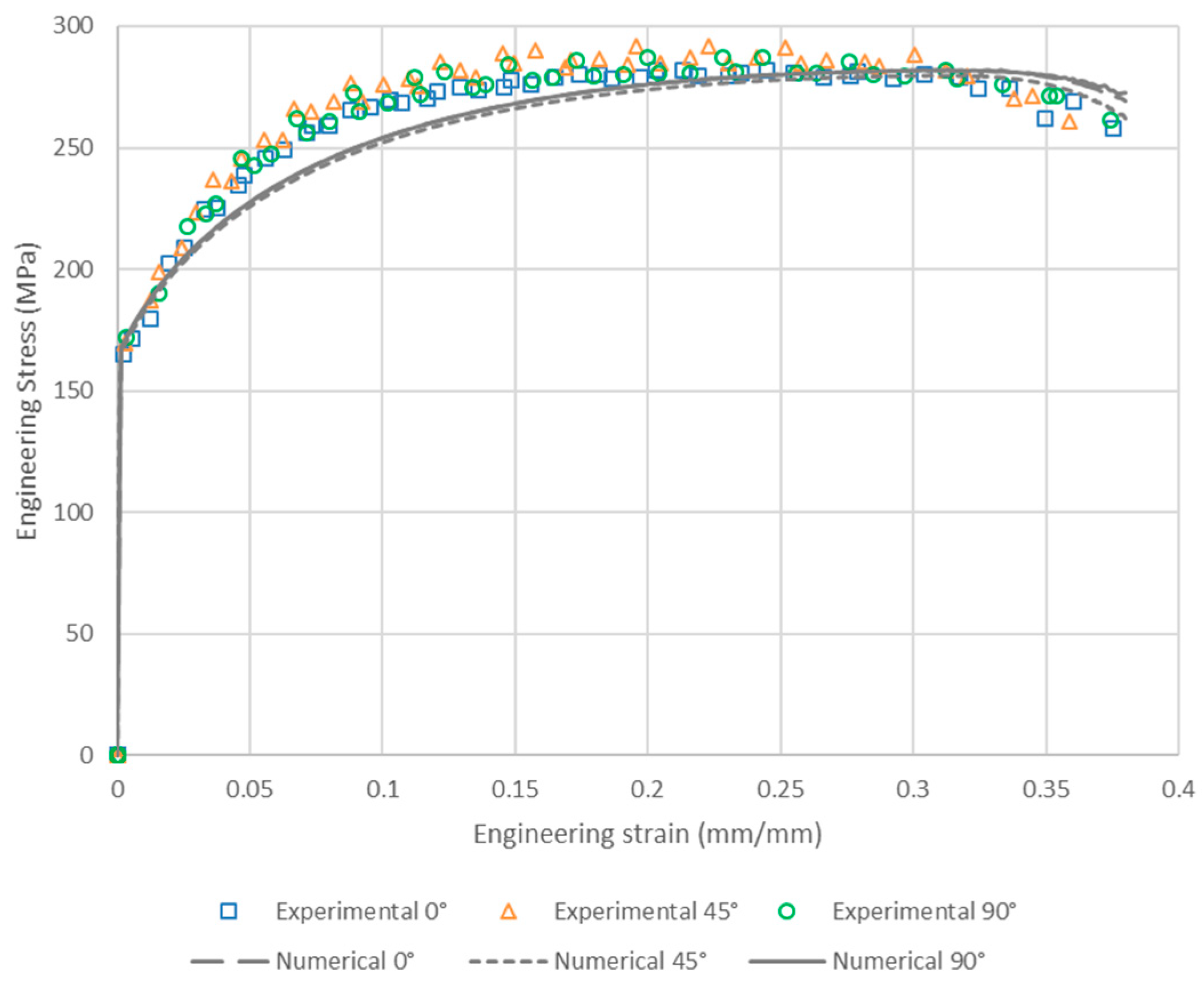
3.1. Tensile Tests
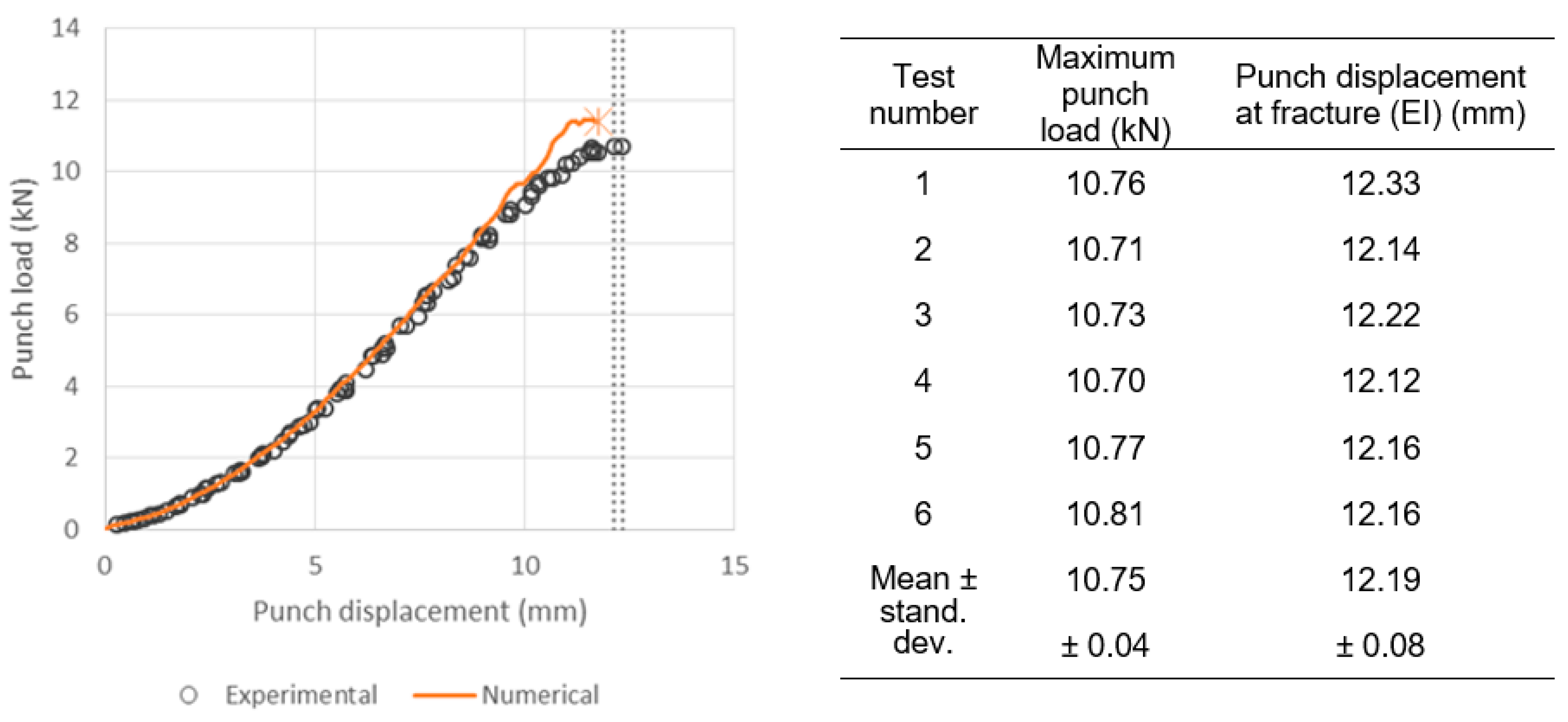
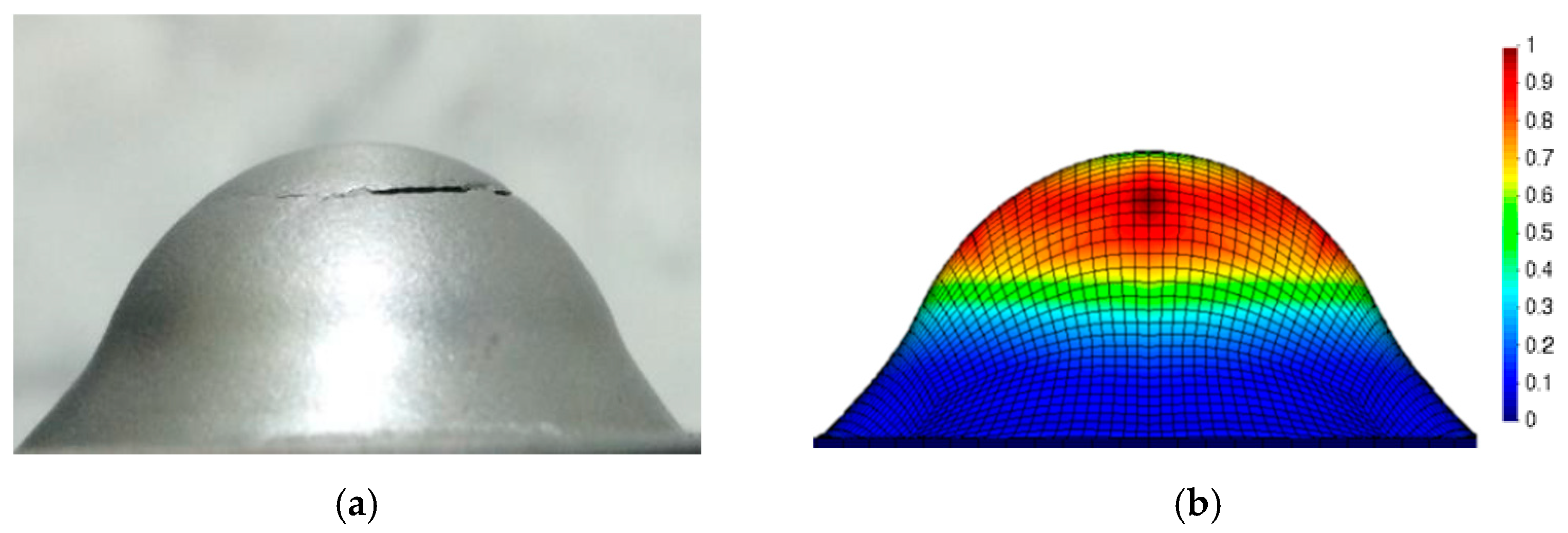
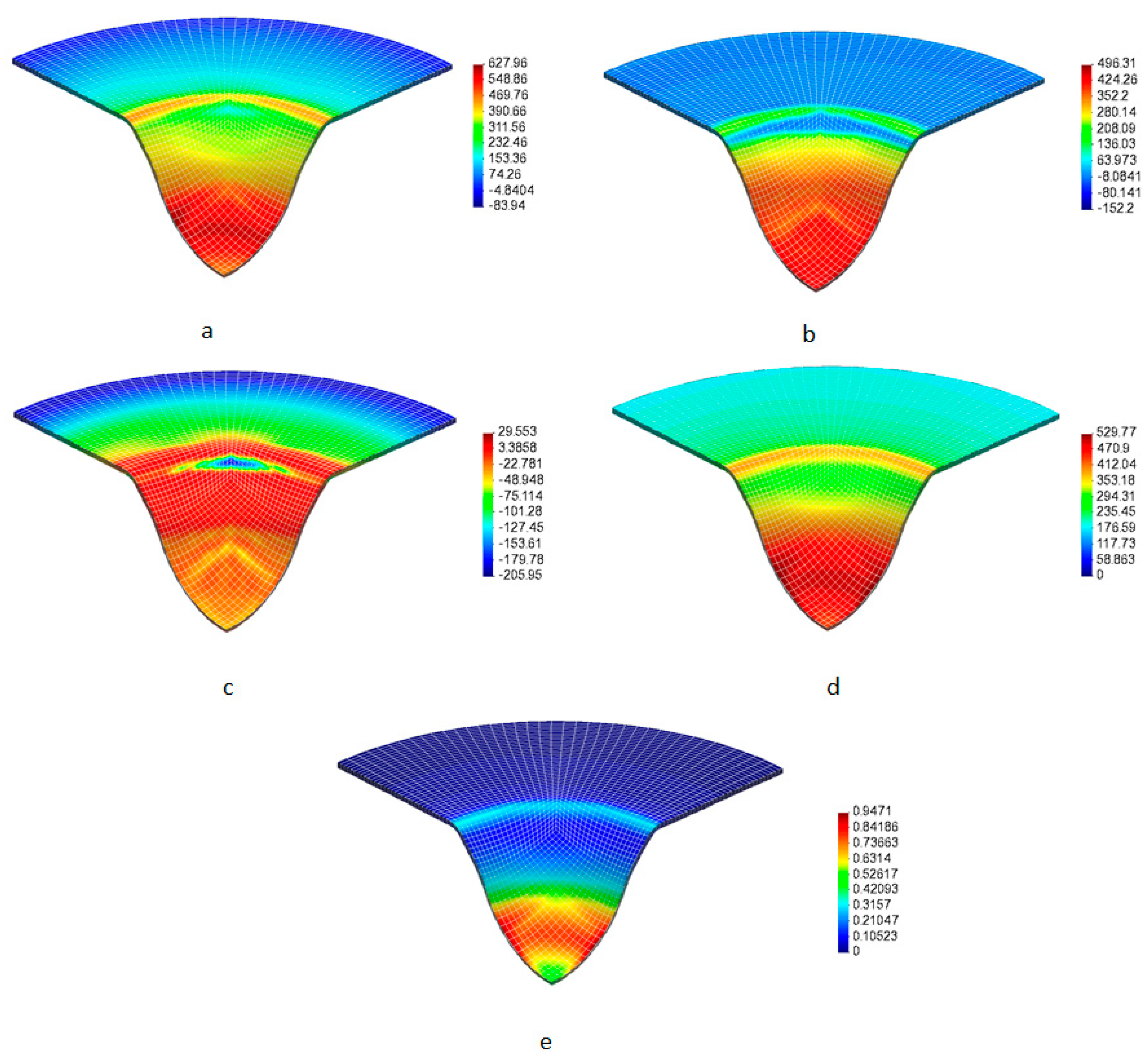
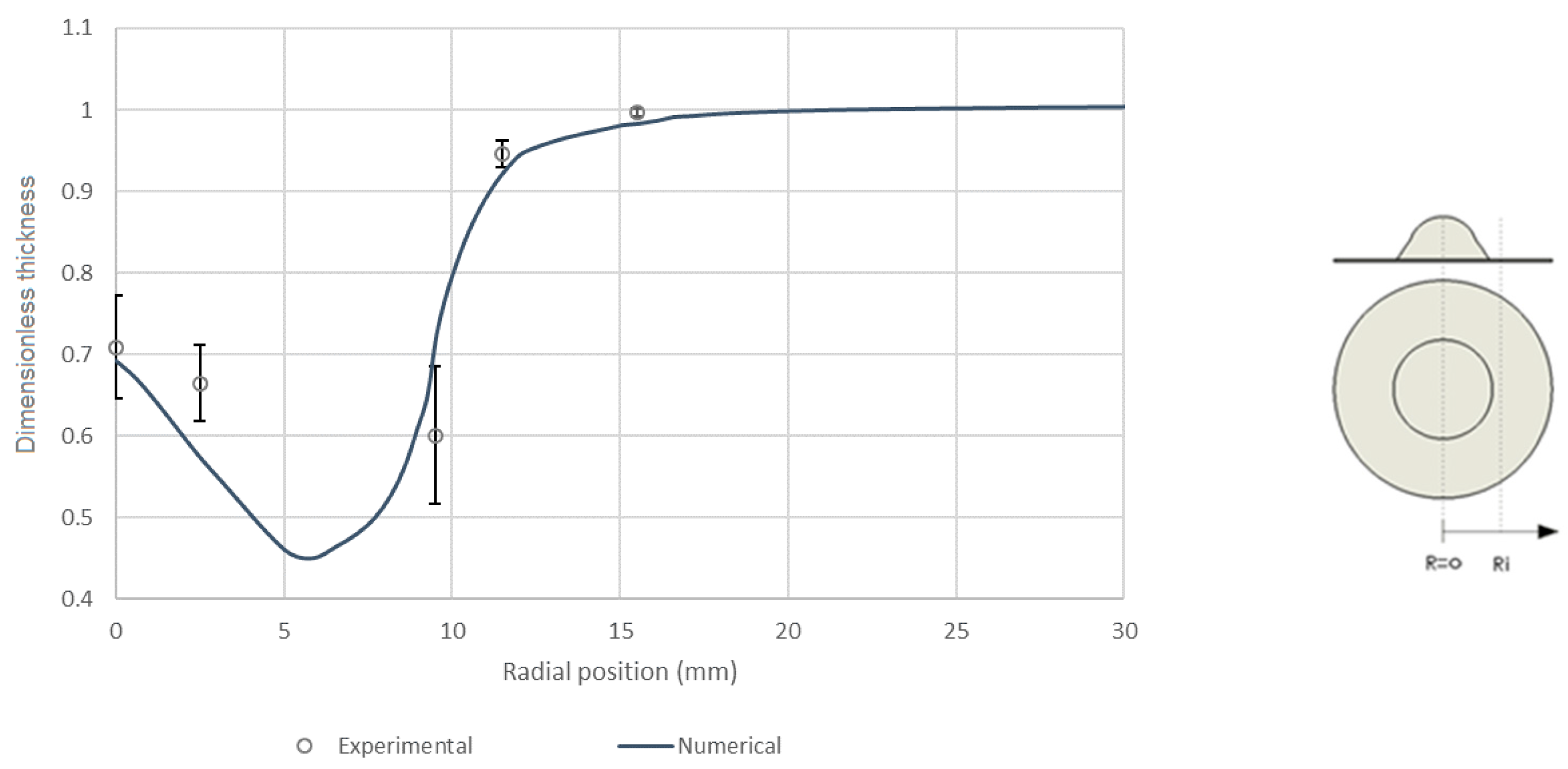
3.2. Erichsen Test
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Ponthot, J.-P.; Feulvarch, E.; Drapier, S.; Bergheau, J.-M. Editorial for thematic issues: Computational methods in manufacturing. Int. J. Mater. Form. 2017, 10, 1–2. [Google Scholar] [CrossRef] [Green Version]
- Banabic, D.; Bunge, H.-J.; Pöhlandt, K.; Tekkaya, A.E. Formability of Metallic Materials; Springer: Berlin/Heidelberg, Germany; New York, NY, USA, 2000. [Google Scholar] [CrossRef]
- Hill, R. A Theory of the Yielding and Plastic Flow of Anisotropic Metals. Proc. R. Soc. Lond. Ser. A Math. Phys. Sci. 1948, 193, 281–297. [Google Scholar] [CrossRef] [Green Version]
- García, C.; Celentano, D.; Flores, F.; Ponthot, J.-P. Numerical modelling and experimental validation of steel deep drawing processes: Part I. Material characterization. J. Mater. Process. Technol. 2006, 172, 451–460. [Google Scholar] [CrossRef]
- García, C.; Celentano, D.; Flores, F.; Ponthot, J.-P.; Oliva, O. Numerical Modelling and Experimental Validation of Steel Deep Drawing Processes. Part II: Aplications. J. Mater. Process. Technol. 2006, 172, 461–471. [Google Scholar] [CrossRef]
- Pacheco, M.; Celentano, D.; Garcia-Herrera, C.; Méndez, J.; Flores, F. Numerical simulation and experimental validation of a multi-step deep drawing process. Int. J. Mater. Form. 2015, 10, 15–27. [Google Scholar] [CrossRef]
- Salehinia, I.; Shahani, A. Effect of sheet anisotropy on the wear in deep-drawing process of a cylindrical cup. Int. J. Mech. Sci. 2009, 51, 856–868. [Google Scholar] [CrossRef]
- Cogun, F.; Darendeliler, H. Comparison of different yield criteria in various deep drawn cups. Int. J. Mater. Form. 2017, 10, 85–98. [Google Scholar] [CrossRef]
- Hosford, W.F. On yield loci of anisotropic cubic metals. In Proceedings of the 7th North American Metalworking Conference, Dearborn, MI, USA, 15–18 April 1979; SME: Dearborn, MI, USA; pp. 191–197. [Google Scholar]
- Hu, W. Characterized behaviors and corresponding yield criterion of anisotropic sheet metals. Mater. Sci. Eng. A 2003, 345, 139–144. [Google Scholar] [CrossRef]
- Aretz, H. A non-quadratic plane stress yield function for orthotropic sheet metals. J. Mater. Process. Technol. 2005, 168, 1–9. [Google Scholar] [CrossRef]
- Banabic, D.S.; Comsa, D. Plane-stress yield criterion for highly-anisotropic sheet metals. In Proceedings of the 7th International Conference and Workshop on Numerical Simulation of 3D Sheet Metal Forming Processes, Numisheet, Interlaken, Switzerland, 1–5 September 2008; pp. 43–48. [Google Scholar]
- Neto, D.M.; Oliveira, M.; Dick, R.E.; Barros, P.D.; Alves, J.L.C.M.; Menezes, L.F. Numerical and experimental analysis of wrinkling during the cup drawing of an AA5042 aluminium alloy. Int. J. Mater. Form. 2015, 10, 125–138. [Google Scholar] [CrossRef]
- Cazacu, O.; Barlat, F. Generalization of Drucker’s Yield Criterion to Orthotropy. Math. Mech. Solids 2001, 6, 613–630. [Google Scholar] [CrossRef]
- Alister, F.; Celentano, D.; Signorelli, J.; Bouchard, P.-O.; Pino, D.; Cruchaga, M. Pino Characterization of the Elastoplastic Response of Low Zn-Cu-Ti Alloy Sheets Using the CPB-06 Criterion. Materials 2019, 12, 3072. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Alister, F.; Celentano, D.; Nicoletti, E.; Signorelli, J.; Bouchard, P.-O.; Pino, D.; Pradille, C.; Cruchaga, M. Elastoplastic Characterization of Zn-Cu-Ti Alloy Sheets: Experiments, Modeling, and Simulation. J. Mater. Eng. Perform. 2022, 31, 1512–1529. [Google Scholar] [CrossRef]
- Han, H.N.; Kim, K.-H. A ductile fracture criterion in sheet metal forming process. J. Mater. Process. Technol. 2003, 142, 231–238. [Google Scholar] [CrossRef]
- Beese, A.; Luo, M.; Li, Y.; Bai, Y.; Wierzbicki, T. Partially coupled anisotropic fracture model for aluminum sheets. Eng. Fract. Mech. 2010, 77, 1128–1152. [Google Scholar] [CrossRef]
- Mohr, D.; Gu, G. Anisotropic Hosford–Coulomb fracture initiation model: Theory and application. Eng. Fract. Mech. 2015, 147, 480–497. [Google Scholar] [CrossRef]
- DIN EN 10209; Cold Rolled Low Carbon Steel Flat Products for Vitreous Enamelling-Technical Delivery Conditions. European Committee For Standardization: Pilsen, Czech Republic, 2013.
- ASTM International. Standard Test Methods for Tension Testing of Metallic Materials; E8/E8m15-a.[s.l.]; ASTM International: West Conshehoken, PA, USA, 2015. [Google Scholar]
- ASTM International. Standard Test Method for Tensile Strain-Hardening Exponents (n-Values) of Metallic Sheet Materials; E646-07e1.[s.l.]; ASTM International: West Conshehoken, PA, USA, 2014. [Google Scholar]
- ASTM International. Standard Test Method for Plastic Strain Ratio R for Sheet Metal; E517-00. [s.l.] ; ASTM International: West Conshehoken, PA, USA, 2010. [Google Scholar]
- ASTM International. Standard Test Method for Ball Punch Deformation of Metallic Sheet Material; E643-09. [s.l.]; ASTM International: West Conshehoken, PA, USA, 2009. [Google Scholar]
- Yan, Y.; Wang, H.; Li, Q. The inverse parameter identification of Hill 48 yield criterion and its verification in press bending and roll forming process simulations. J. Manuf. Process. 2015, 20, 46–53. [Google Scholar] [CrossRef]
- Celentano, D.J.; Guelorget, B.; François, M.; A Cruchaga, M.; Slimane, A. Numerical simulation and experimental validation of the microindentation test applied to bulk elastoplastic materials. Model. Simul. Mater. Sci. Eng. 2012, 20, 045007. [Google Scholar] [CrossRef]


| Sample Direction | Young Modulus (GPa) | Yield Stress (MPa) | Maximum Force (kN) | UTS (MPa) | Fracture Engineering Strain (%) | Lankford Coefficient |
|---|---|---|---|---|---|---|
| 0° | 161 | 166 | 2.107 | 281 | 37.5 | 1.406 |
| 45° | 193 | 172 | 2.162 | 288 | 35.9 | 1.208 |
| 90° | 182 | 172 | 2.115 | 282 | 37.7 | 1.610 |
| Isotropic Hardening | Yield Criterion | ||||||
| Ap | np | F | G | H | L | M | N |
| 535 MPa | 0.30 | 0.389 | 0.389 | 0.611 | 1.278 | 1.278 | 1.610 |
| Plastic flow potential | |||||||
| F | G | H | L | M | N | ||
| 0.362 | 0.415 | 0.585 | 1.225 | 1.304 | 1.330 | ||
| a | b | c | M12 | M22 |
|---|---|---|---|---|
| 2.0 | 0.70 | 0.005 | 0.10 | 1.02 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Barrera, C.; García-Herrera, C.; Celentano, D.J.; Signorelli, J.W. Material and Damage Characterization of the Elastoplastic Response of the EK4 Deep Drawing Steel. Metals 2022, 12, 720. https://doi.org/10.3390/met12050720
Barrera C, García-Herrera C, Celentano DJ, Signorelli JW. Material and Damage Characterization of the Elastoplastic Response of the EK4 Deep Drawing Steel. Metals. 2022; 12(5):720. https://doi.org/10.3390/met12050720
Chicago/Turabian StyleBarrera, Carlos, Claudio García-Herrera, Diego J. Celentano, and Javier W. Signorelli. 2022. "Material and Damage Characterization of the Elastoplastic Response of the EK4 Deep Drawing Steel" Metals 12, no. 5: 720. https://doi.org/10.3390/met12050720
APA StyleBarrera, C., García-Herrera, C., Celentano, D. J., & Signorelli, J. W. (2022). Material and Damage Characterization of the Elastoplastic Response of the EK4 Deep Drawing Steel. Metals, 12(5), 720. https://doi.org/10.3390/met12050720









