Elastoplastic Fracture Analysis of the P91 Steel Welded Joint under Repair Welding Thermal Shock Based on XFEM
Abstract
:1. Introduction
2. FE Model and Simulation Method
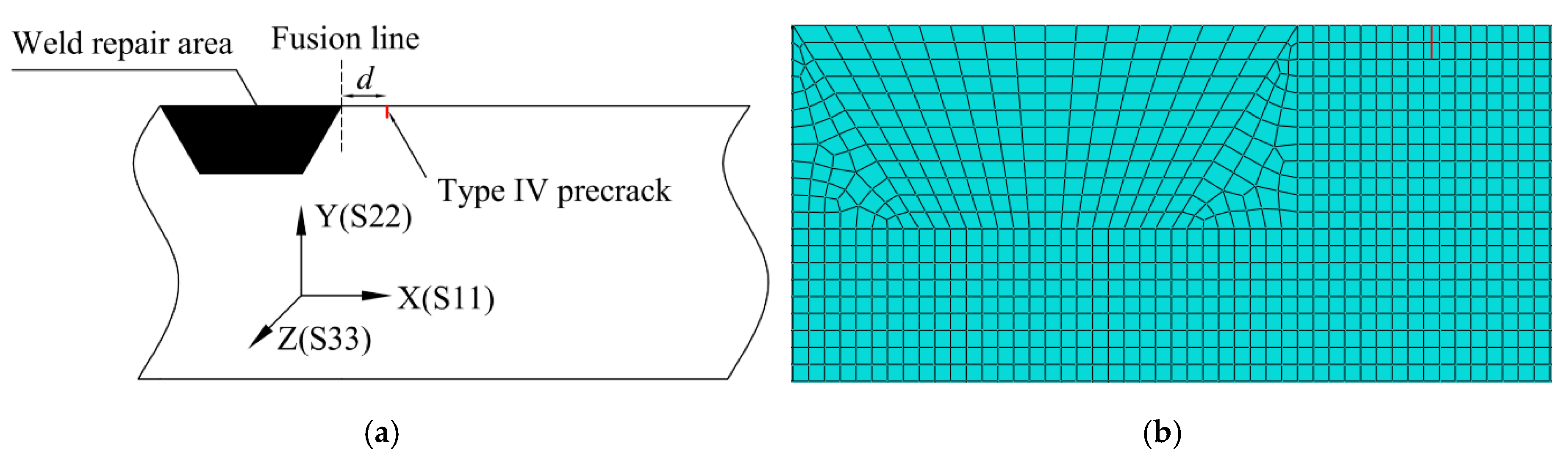
2.1. Model Geometry
2.2. Material Properties and Heat Source Model
2.2.1. Material Property
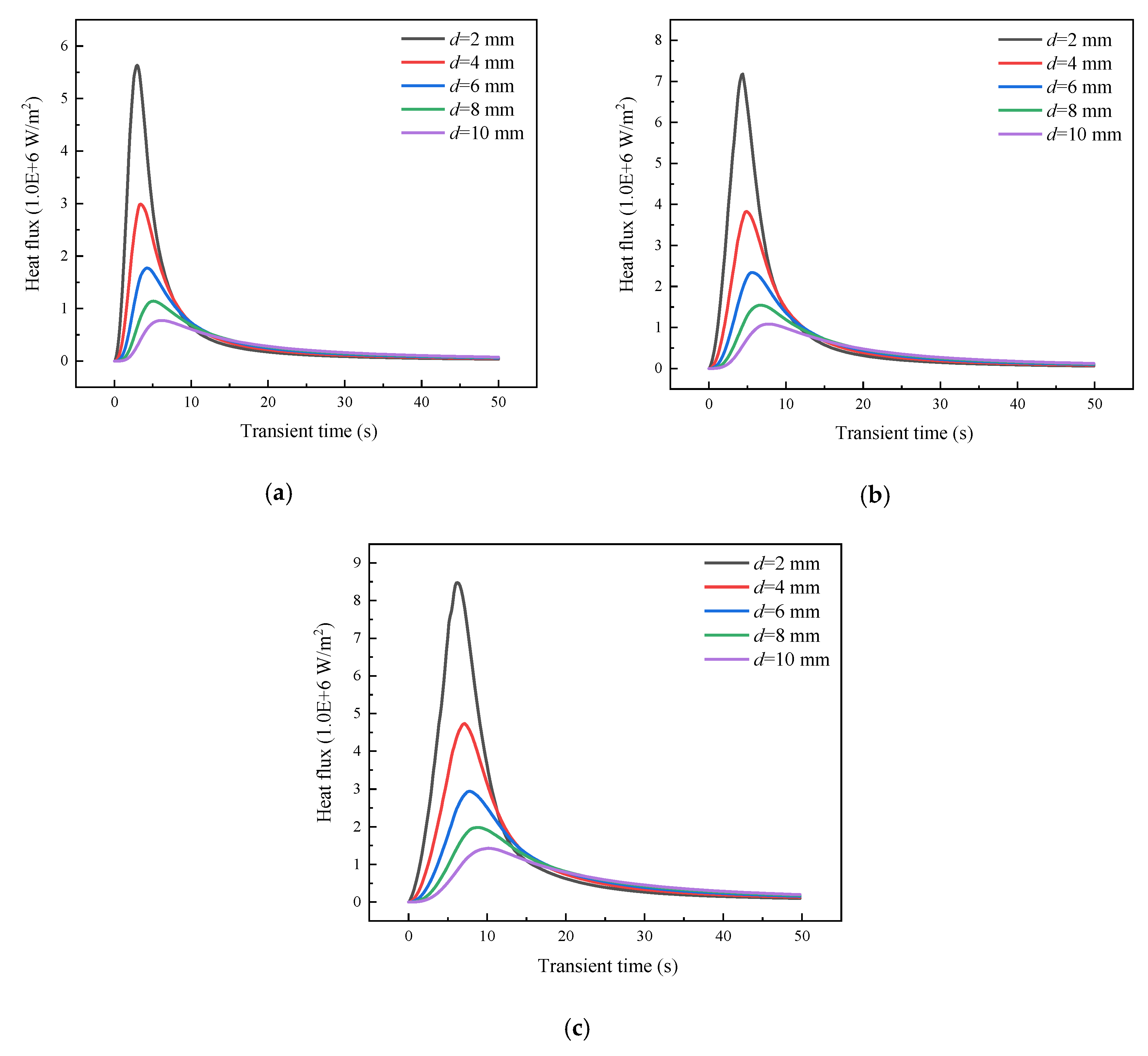
2.2.2. Heat Source Definition
- R is the heat flux (W/m2);
- λ is the thermal conductivity (W/m·k);
- T is the distribution function of temperature field (K);
- ρ is the density (kg/m3);
- c is the specific heat (J/kg·K);
- t is the transient time (s);
- Q is the intensity of thermal energy (W), including the thermal energy generated by the heat source and the thermal energy generated by the solid-liquid phase change.
2.3. Boundary Conditions
2.4. Thermal Loading Patterns
2.5. Damage Model
3. Weld Repair Experiments
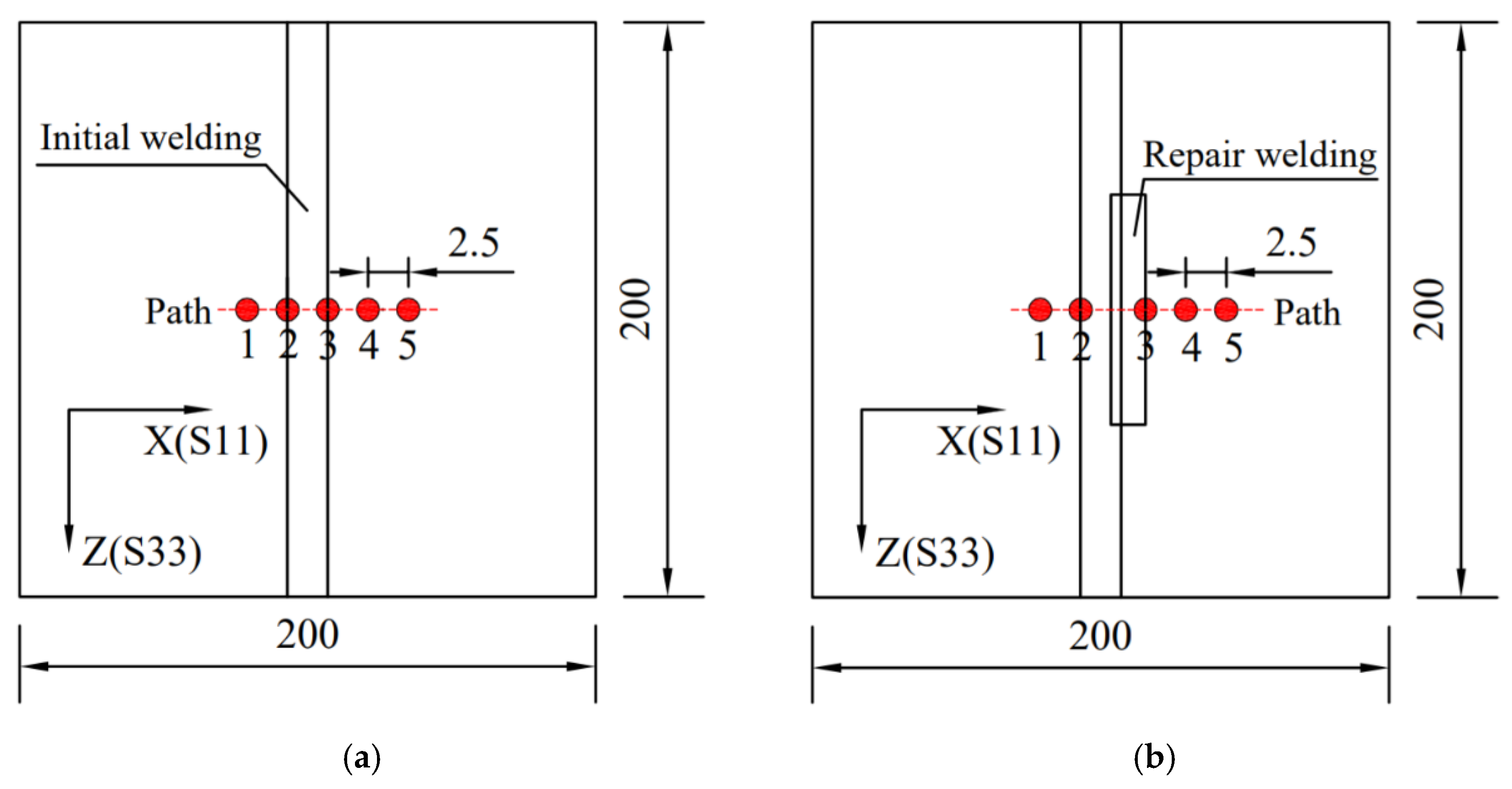
3.1. Weld Repair Specimens
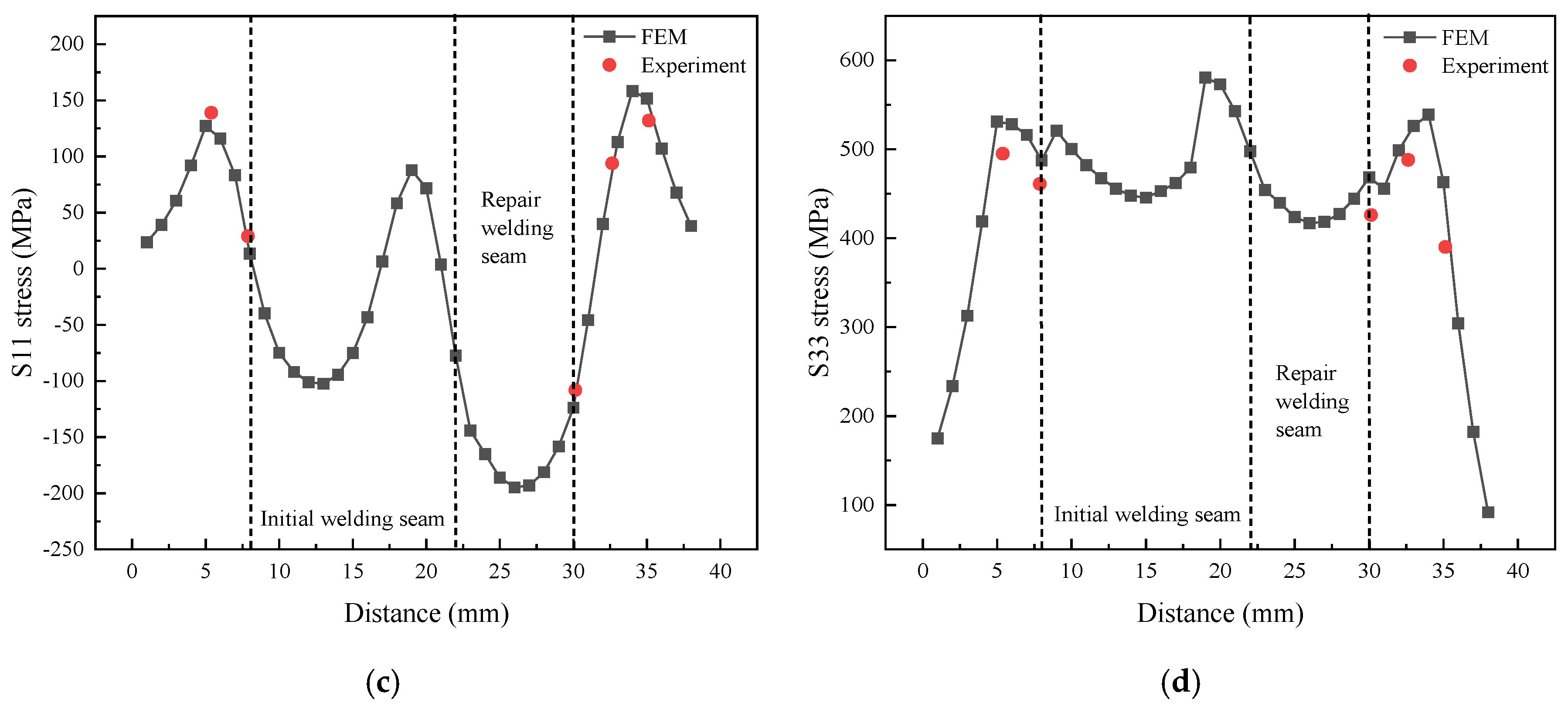
3.2. Verification of the FE Model
4. Results and Discussion
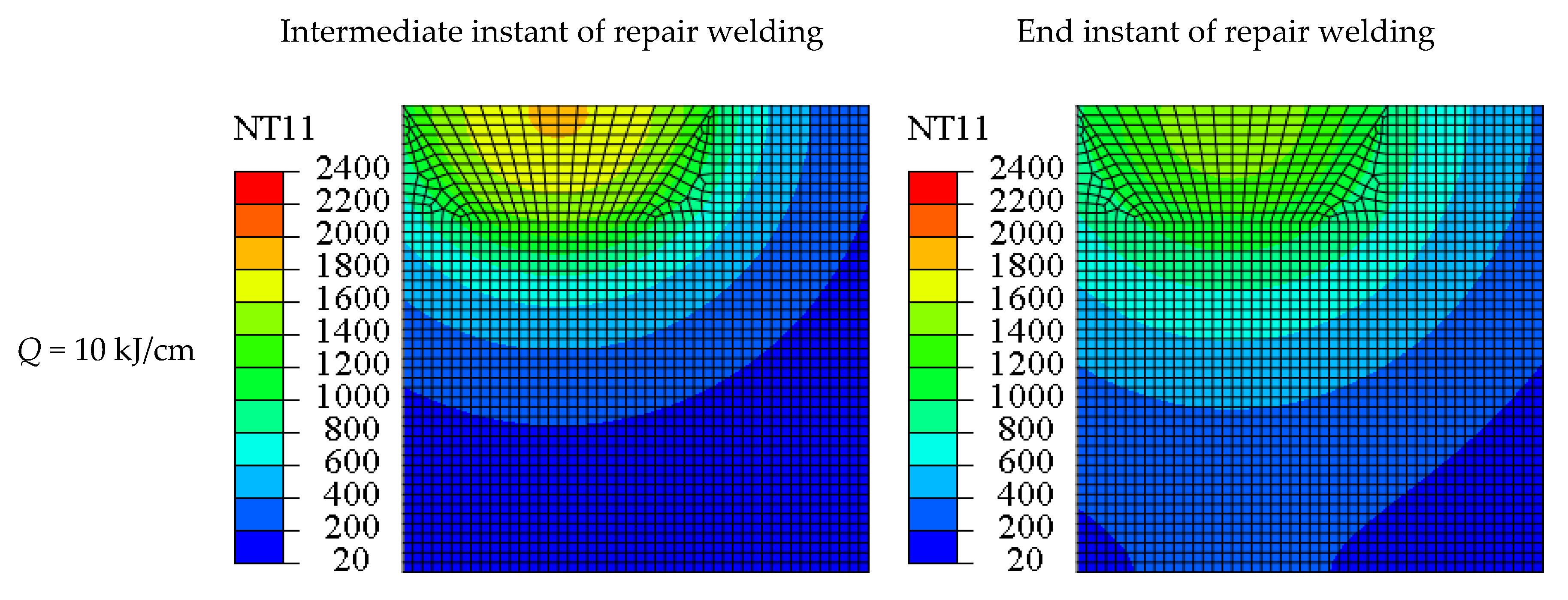
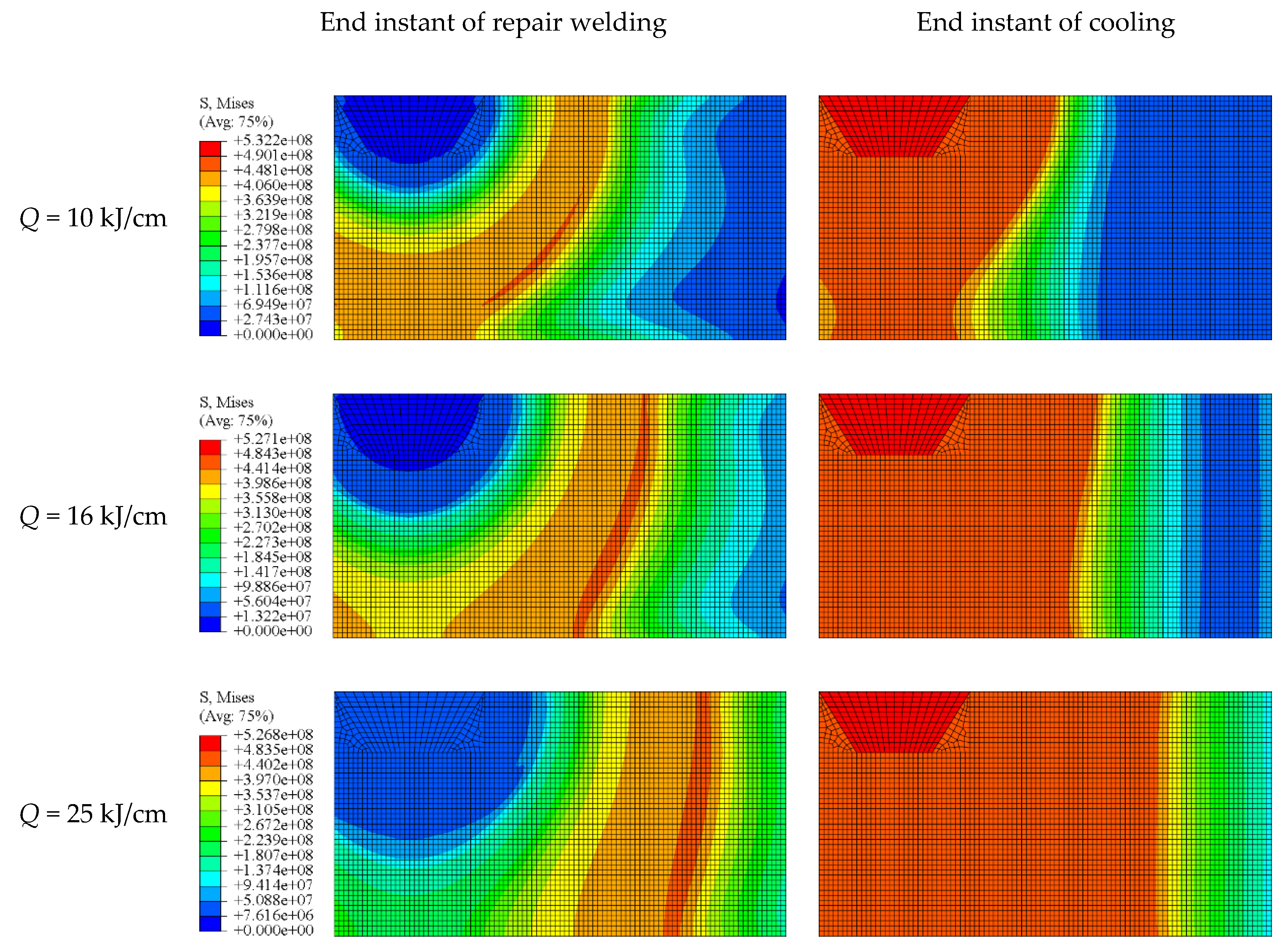
4.1. Thermal Analysis
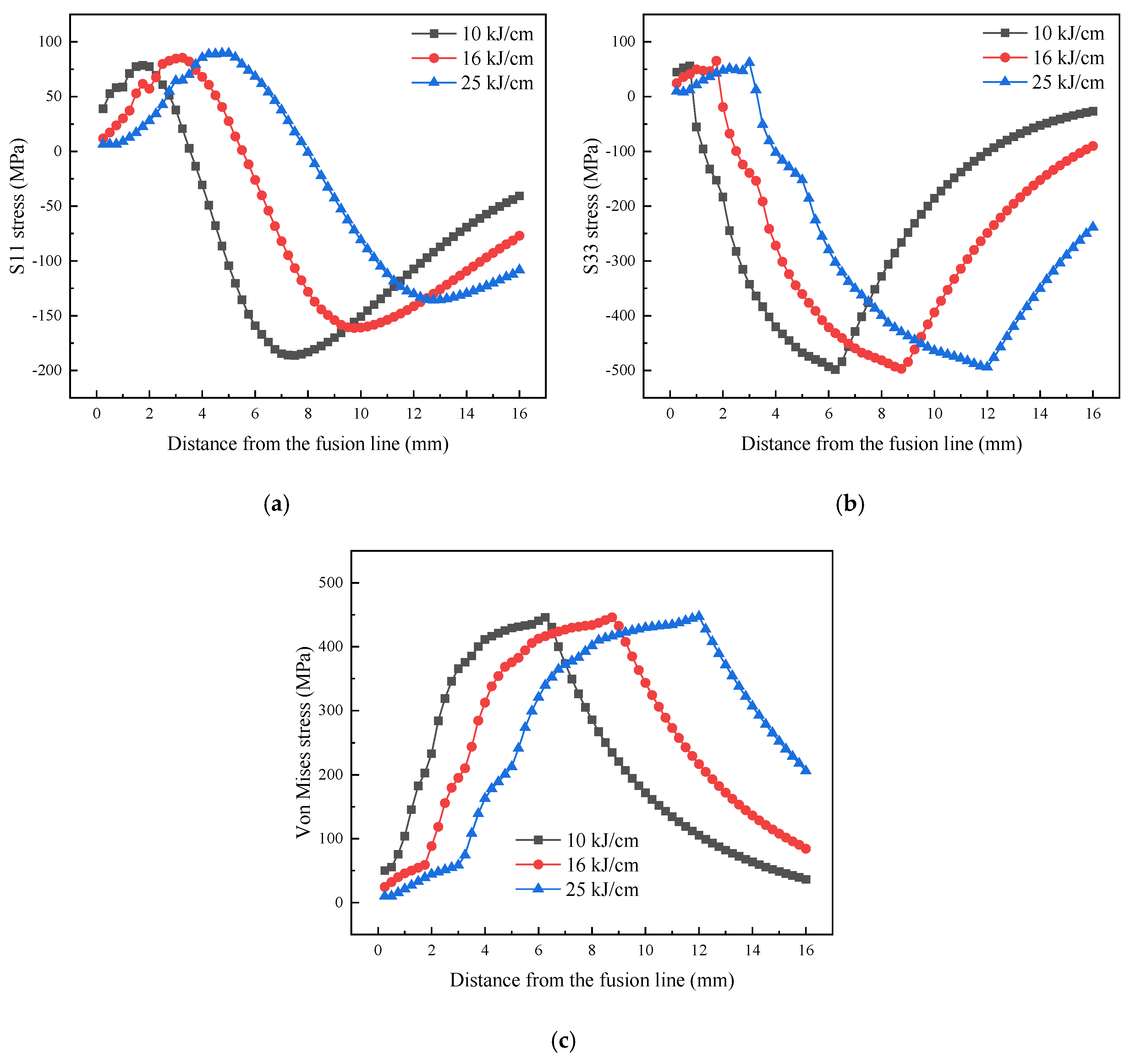
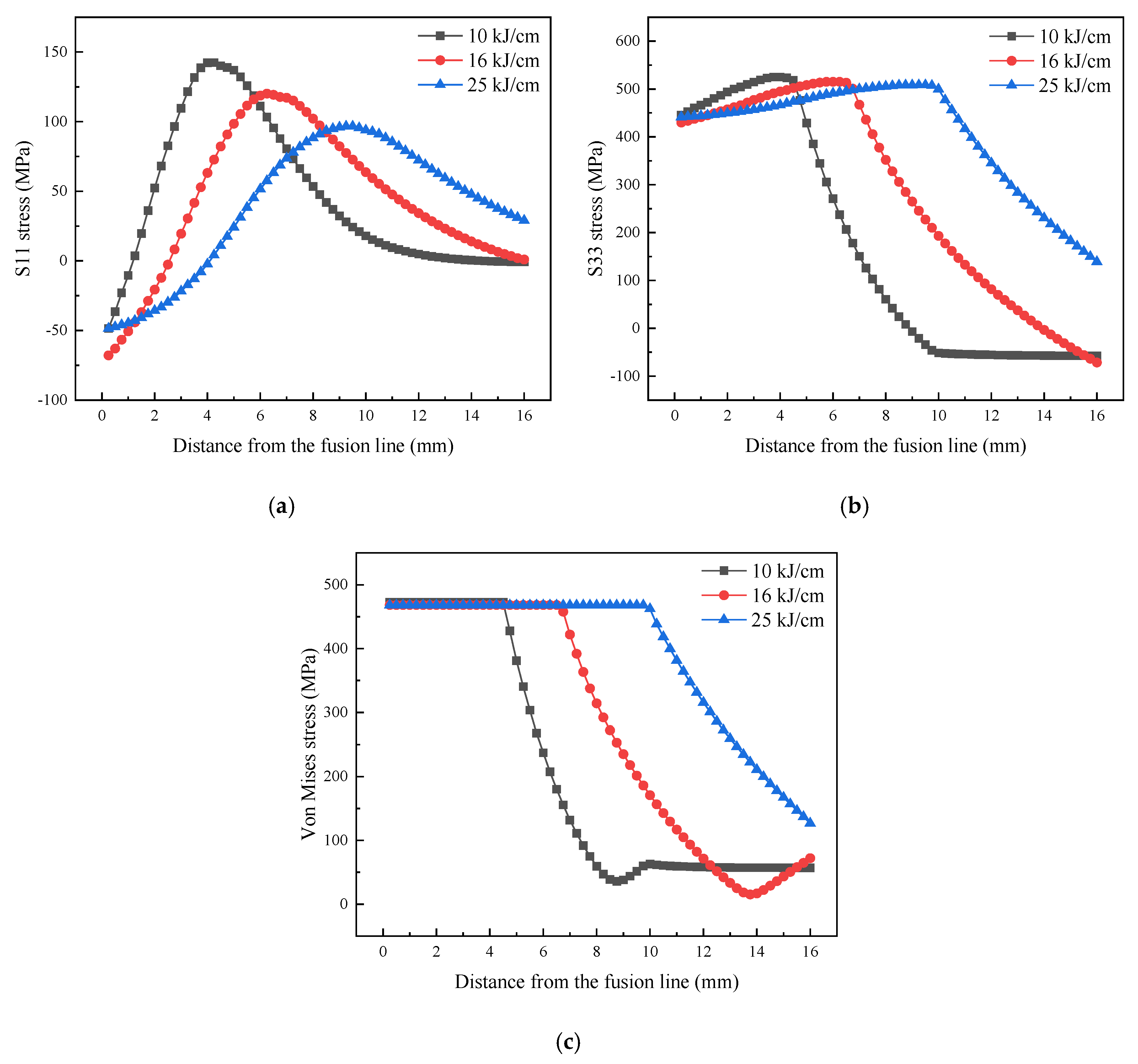
4.2. Mechanical Analysis
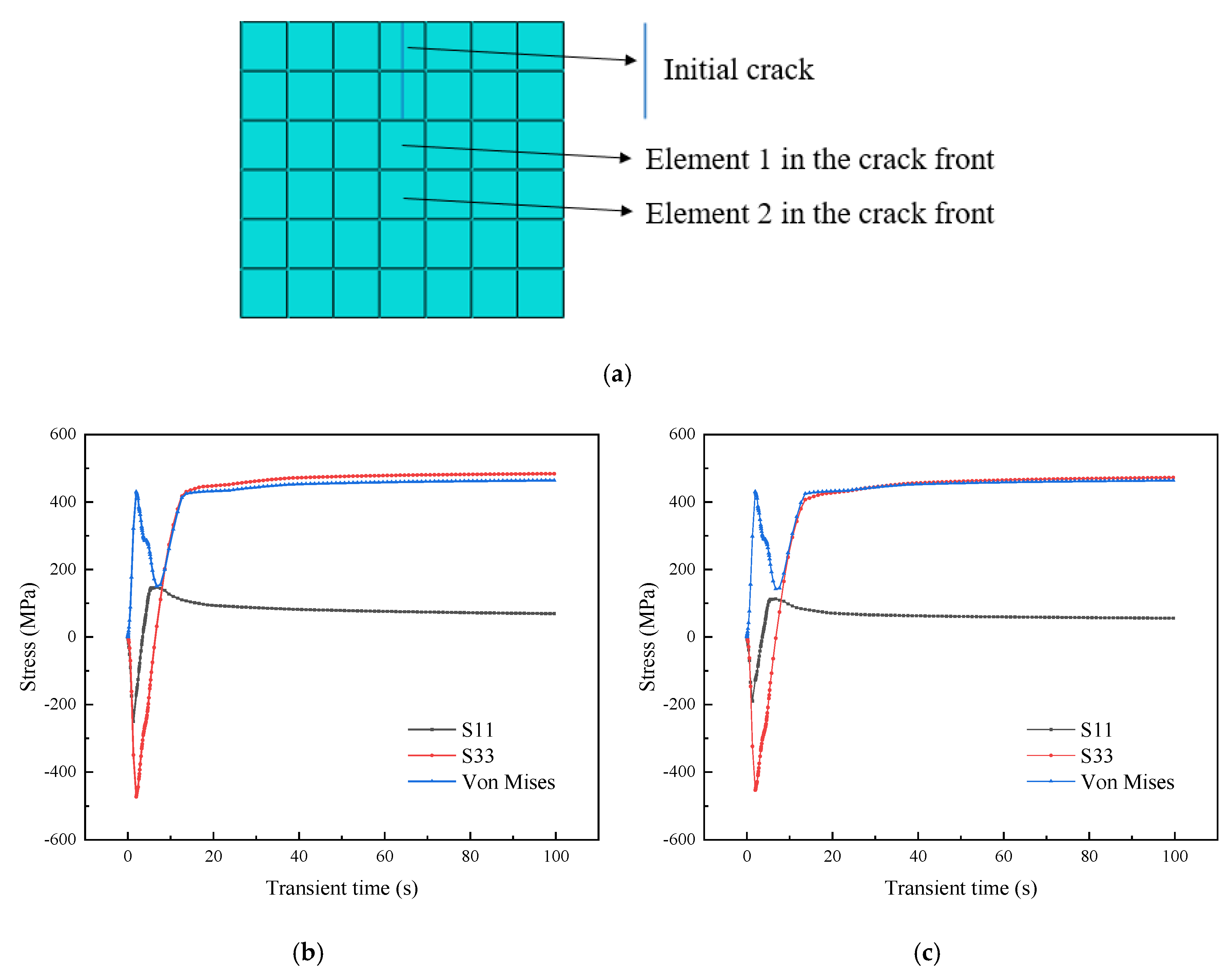
4.3. XFEM Analysis
5. Conclusions
- (1)
- With the increase of linear energy, the range of the HAZ expands and the overall temperature of the HAZ rises. With the increase of linear energy, the range of S11, S33 and Von Mises high stress area expands.
- (2)
- In the repair welding and cooling process, the material in the HAZ undergoes a change from compressed state to tensioned state due to the expansion and shrinkage of the welding seam. It is the direct cause of crack propagation in the HAZ.
- (3)
- Crack propagation occurs in the early stage of cooling. The crack propagation direction is roughly perpendicular to the upper surface and has a tendency to deflect to the welding seam.
- (4)
- For cracks at the same position, with the increase of linear energy, the crack propagation length increases. For the same linear energy, with the distance from the fusion line increases, the crack propagation length decreases.
- (5)
- After a certain distance from the fusion line, the cracks in the HAZ do not extend. This distance is the critical distance for the crack propagation. With the increase of linear energy, the critical distance of crack propagation increases. The critical distance of crack propagation is consistent with the high stress area after repair welding. Therefore, for repair welding, low linear energy is preferred to constrain the cracking behavior in the HAZ.
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Viswanathan, R.; Coleman, K.; Rao, U. Materials for ultra-supercritical coal-fired power plant boilers. Int. J. Pres. Vessel Pip. 2006, 83, 778–783. [Google Scholar] [CrossRef]
- Klueh, R.L. Elevated temperature ferritic and martensitic steels and their application to future nuclear reactors. Int. Mater. Rev. 2005, 50, 287–310. [Google Scholar] [CrossRef] [Green Version]
- Chu, Q.L.; Zhang, M.; Li, J.H.; Chen, Y.N.; Luo, H.L.; Wang, Q. Failure analysis of a steam pipe weld used in power generation plant. Eng. Fail. Anal. 2014, 44, 363–370. [Google Scholar] [CrossRef]
- Hu, Z.X.; Zhao, J.P. Effects of martensitic transformation on residual stress of P91 welded joint. Mater. Res. Express 2018, 5, 096528. [Google Scholar] [CrossRef]
- Abson, D.J.; Rothwell, J.S. Review of type IV cracking of weldments in 9–12%Cr creep strength enhanced ferritic steels. Int. Mater. Rev. 2013, 58, 437–473. [Google Scholar] [CrossRef]
- Viswanathan, R.; Gandy, D. Weld Repair of Aged Cr-Mo Steel Piping—A Review of Literature. J. Press. Vessel Technol. ASME 2000, 122, 76–85. [Google Scholar] [CrossRef]
- Parker, J.D.; Siefert, J.A. Weld repair of Grade 91 piping and components in power generation applications, creep performance of repair welds. Mater. High. Temp. 2016, 33, 58–67. [Google Scholar] [CrossRef]
- Weglowski, M. Determination of GTA and GMA welding arc temperatures. Weld. Int. 2005, 19, 186–192. [Google Scholar] [CrossRef]
- Alvarez, P.; Vázquez, L.; Ruiz, N.; Rodríguez, P.; Magaña, A.; Niklas, A.; Santos, F. Comparison of hot cracking susceptibility of TIG and laser beam welded alloy 718 by varestraint testing. Metals 2019, 9, 985. [Google Scholar] [CrossRef] [Green Version]
- Chelladurai, A.M.; Gopal, K.A.; Murugan, S.; Albert, S.K.; Venugopal, S.; Jayakumar, T. Effect of energy transfer modes on solidification cracking in pulsed laser welding. Sci. Technol. Weld. Join. 2015, 20, 578–584. [Google Scholar] [CrossRef]
- Hosseini, S.A.; Abdollah-zadeh, A.; Naffakh-Moosavy, H.; Mehri, A. Elimination of hot cracking in the electron beam welding of AA2024-T351 by controlling the welding speed and heat input. J. Manuf. Process. 2019, 46, 147–158. [Google Scholar] [CrossRef]
- Coniglio, N.; Cross, C.E. Mechanisms for solidification crack initiation and growth in aluminum welding. Metall. Mater. Trans. A 2009, 40, 2718–2728. [Google Scholar] [CrossRef]
- Wei, Y.H.; Liu, R.P.; Dong, Z.J. Software package for simulation and prediction of welding solidification cracks. Sci. Technol. Weld. Join. 2003, 8, 325–333. [Google Scholar] [CrossRef]
- Bordreuil, C.; Niel, A. Modelling of hot cracking in welding with a cellular automaton combined with an intergranular fluid flow model. Comp. Mater. Sci. 2014, 82, 442–450. [Google Scholar] [CrossRef] [Green Version]
- Agarwal, G.; Kumar, A.; Richardson, I.M.; Hermans, M.J.M. Evaluation of solidification cracking susceptibility during laser welding in advanced high strength automotive steels. Mater. Des. 2019, 183, 108104. [Google Scholar] [CrossRef]
- Jiang, W.C.; Liu, Z.B.; Gong, J.M.; Tu, S.T. Numerical simulation to study the effect of repair width on residual stresses of a stainless steel clad plate. Int. J. Pres. Ves. Pip. 2010, 87, 457–463. [Google Scholar] [CrossRef]
- Hyde, T.H.; Saber, M.; Sun, W. Creep crack growth data and prediction for a P91 weld at 650 °C. Int. J. Pres. Ves. Pip. 2010, 87, 721–729. [Google Scholar] [CrossRef]
- Pandey, C.; Saini, N.; Mahapatra, M.M.; Kumar, P. Hydrogen induced cold cracking of creep resistant ferritic P91 steel for different diffusible hydrogen levels in deposited metal. Int. J. Hydrogen. Energy 2016, 41, 17695–17712. [Google Scholar] [CrossRef]
- Zhang, Y.J.; Yang, K.; Zhao, J.P. Experimental research and numerical simulation of weld repair with high energy spark deposition method. Metals 2020, 10, 980. [Google Scholar] [CrossRef]
- He, K.F.; Yang, Q.; Xiao, D.M.; Li, X.J. Analysis of thermo-elastic fracture problem during aluminium alloy MIG welding using the extended finite element method. Appl. Sci. 2017, 7, 69. [Google Scholar] [CrossRef] [Green Version]
- Li, H.; Li, J.S.; Yuan, H. A review of the extended finite element method on macrocrack and microcrack growth simulations. Theor. Appl. Fract. Mech. 2018, 97, 236–249. [Google Scholar] [CrossRef]
- Belytschko, T.; Black, T. Elastic crack growth in finite elements with minimal remeshing. Int. J. Numer. Meth. Eng. 1999, 45, 601–620. [Google Scholar] [CrossRef]
- Moës, N.; Dolbow, J.; Belytschko, T. A finite element method for crack growth without remeshing. Int. J. Numer. Meth. Eng. 1999, 46, 131–150. [Google Scholar] [CrossRef]
- Daux, C.; Moës, N.; Dolbow, J.; Sukumar, N.; Belytschko, T. Arbitrary branched and intersecting cracks with the extended finite element method. Int. J. Numer. Meth. Eng. 2000, 48, 1741–1760. [Google Scholar] [CrossRef]
- Chessa, J.; Wang, H.W.; Belytschko, T. On the construction of blending elements for local partition of unity enriched finite elements. Int. J. Numer. Meth. Eng. 2003, 57, 1015–1038. [Google Scholar] [CrossRef]
- Stolarska, M.; Chopp, D.L.; Moës, N.; Belytschko, T. Modelling crack growth by level sets in the extended finite element method. Int. J. Numer. Meth. Eng. 2001, 51, 943–960. [Google Scholar] [CrossRef]
- Song, J.-H.; Areias, P.M.A.; Belytschko, T. A method for dynamic crack and shear band propagation with phantom nodes. Int. J. Numer. Meth. Eng. 2006, 67, 868–893. [Google Scholar] [CrossRef]
- Yang, J.P.; Guo, J.; Qiao, Y.X. DL/T 869-2012 The Code of Welding for Power Plant; China Electric Power Press: Beijing, China, 2012. (In Chinese) [Google Scholar]
- Yaghi, A.H.; Hyde, T.H.; Becker, A.A.; Williams, J.A.; Sun, W. Residual stress simulation in welded sections of P91 pipes. J. Mater. Process. Tech. 2005, 167, 480–487. [Google Scholar] [CrossRef]
- Arora, H.; Singh, R.; Brar, G.S. Thermal and structural modelling of arc welding processes: A literature review. Meas. Control. 2019, 52, 955–969. [Google Scholar] [CrossRef]
- Zhang, W.Y. Principle and Technology of Metal; Fusion Welding; China Machine Press: Beijing, China, 1980. (In Chinese) [Google Scholar]
- Zhang, J.X.; Liu, C. Finite Element Calculation of Welding Stress and Deformation and Its Engineering Application; Science Press: Beijing, China, 2015. (In Chinese) [Google Scholar]
- Asferg, J.L.; Poulsen, P.N.; Nielsen, L.O. A direct XFEM formulation for modeling of cohesive crack growth in concrete. Comput. Concr. 2007, 4, 83–100. [Google Scholar] [CrossRef]
- Benzeggagh, M.L.; Kenane, M. Measurement of mixed-mode delamination fracture toughness of unidirectional glass/epoxy composites with mixed-mode bending apparatus. Compos. Sci. Technol. 1996, 56, 439–449. [Google Scholar] [CrossRef]
- Pan, J.Z. Practical Manual for Pressure Vessel Materials—Carbon Steel and Alloy Steel; Chemical Industry Press: Beijing, China, 2000. (In Chinese) [Google Scholar]
- Dutt, B.S.; Babu, M.N.; Shanthi, G.; Moitra, A.; Sasikala, G. Investigation on fracture behavior of Grade 91 steel at 300–550 °C. J. Mater. Eng. Perform. 2018, 27, 6577–6584. [Google Scholar] [CrossRef]
- ASME. ASME Boiler & Pressure Vessel Code, Section II, Part A; American Society of Mechanical Engineers: New York, NY, USA, 2019. [Google Scholar]
- ASME. ASME Boiler & Pressure Vessel Code, Section II, Part C; American Society of Mechanical Engineers: New York, NY, USA, 2019. [Google Scholar]
- Chen, H.N.; Li, R.F.; Chen, J. GB/T 24179-2009 Metallic Materials-Residual Stress Determination—The Indentation Strain-Gage Method; China Standard Press: Beijing, China, 2009. (In Chinese) [Google Scholar]
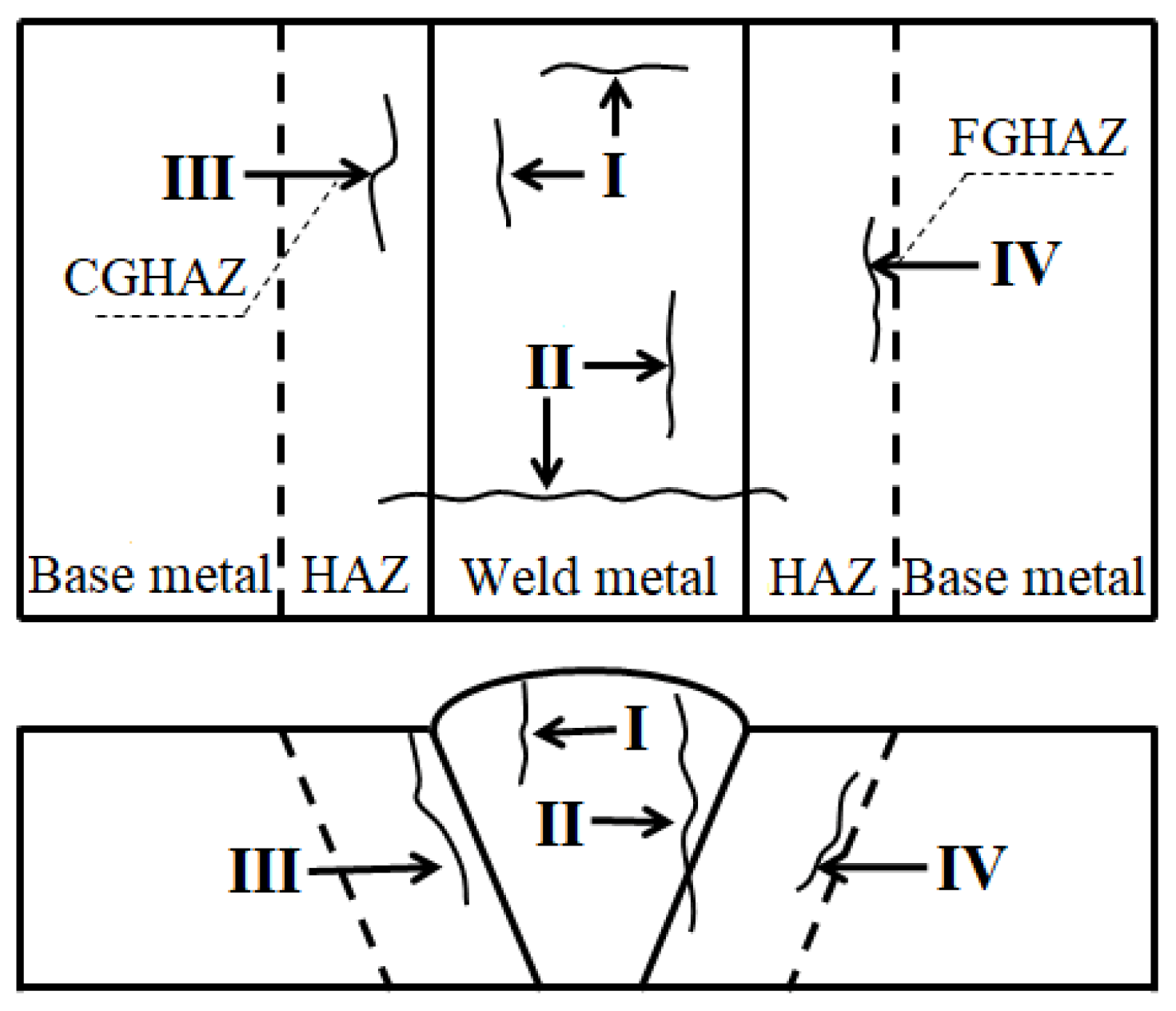
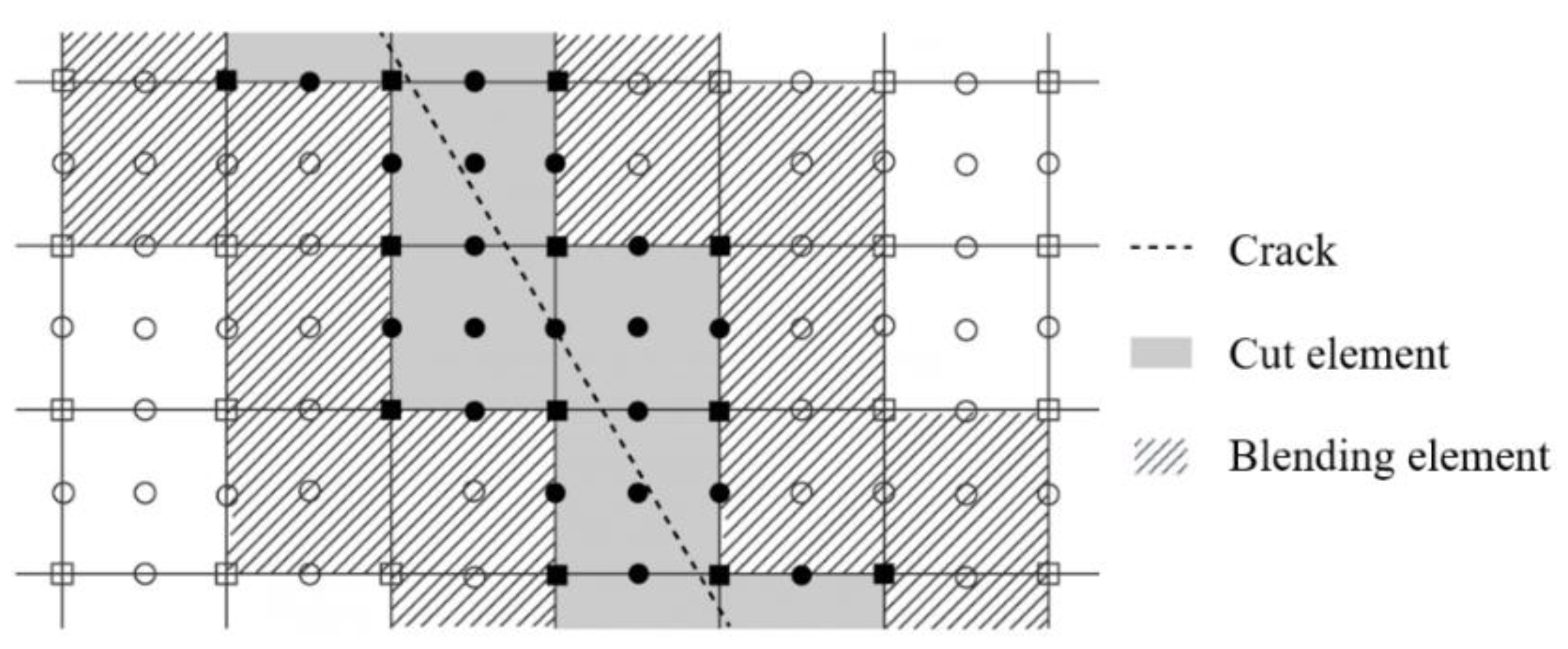
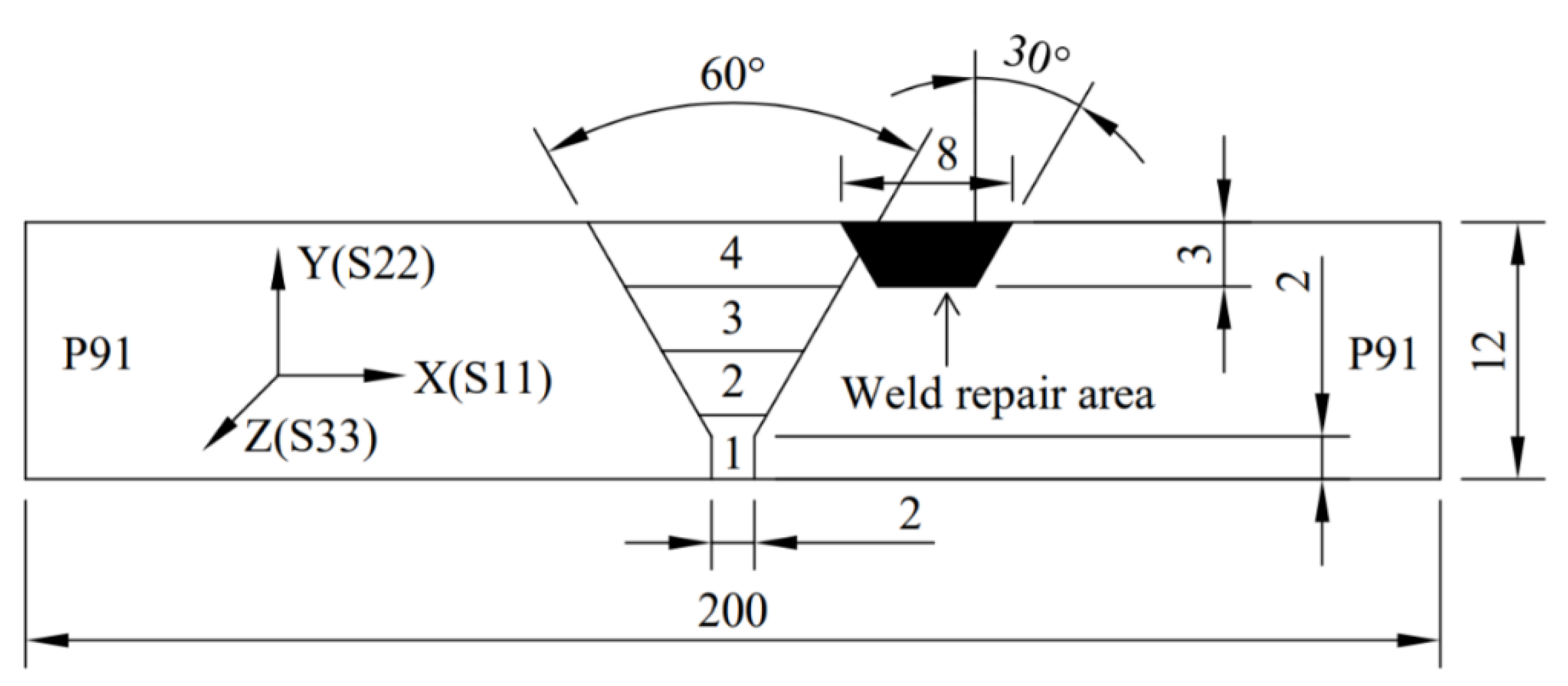





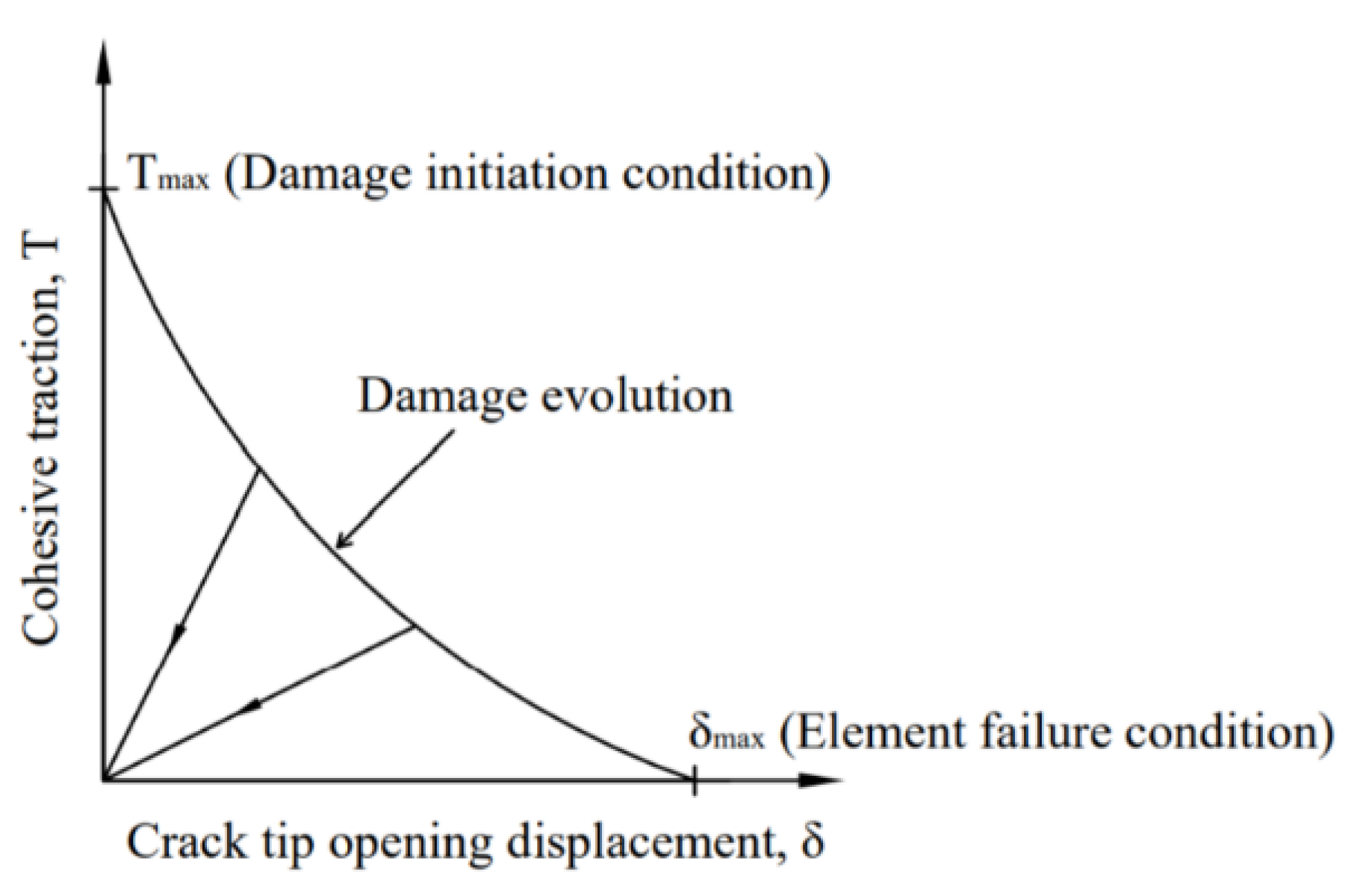









| C | Si | Mn | S | P | Cr | Ni | Mo | V | Nb | N | Al |
|---|---|---|---|---|---|---|---|---|---|---|---|
| 0.08 | 0.27 | 0.6 | 0.006 | 0.007 | 8.86 | 0.38 | 0.98 | 0.19 | 0.06 | 0.06 | 0.04 |
| Material Grade | C | Si | Mn | Cr | Ni | Mo | V | Nb |
|---|---|---|---|---|---|---|---|---|
| ER90S-B9 | 0.1 | 0.3 | 0.5 | 9.0 | 0.7 | 1.0 | 0.2 | 0.06 |
| E9015-B9 | 0.09 | 0.2 | 0.6 | 9.0 | 0.8 | 1.1 | 0.2 | 0.05 |
| Layer Number | Welding Method | Electrode Grade | Electrode Diameter (mm) | Welding Current (A) | Arc Voltage (V) | Welding Speed (cm/min) |
|---|---|---|---|---|---|---|
| 1 | GTAW | ER90S-B9 | 2.4 | 100 | 12 | 6 |
| 2 | GTAW | ER90S-B9 | 2.4 | 110 | 14 | 6 |
| 3 | SMAW | E9015-B9 | 3.2 | 110 | 20 | 12 |
| 4 | SMAW | E9015-B9 | 3.2 | 120 | 22 | 12 |
| Repair | SMAW | E9015-B9 | 3.2 | 120 | 22 | 12 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yang, K.; Zhang, Y.; Zhao, J. Elastoplastic Fracture Analysis of the P91 Steel Welded Joint under Repair Welding Thermal Shock Based on XFEM. Metals 2020, 10, 1285. https://doi.org/10.3390/met10101285
Yang K, Zhang Y, Zhao J. Elastoplastic Fracture Analysis of the P91 Steel Welded Joint under Repair Welding Thermal Shock Based on XFEM. Metals. 2020; 10(10):1285. https://doi.org/10.3390/met10101285
Chicago/Turabian StyleYang, Kai, Yingjie Zhang, and Jianping Zhao. 2020. "Elastoplastic Fracture Analysis of the P91 Steel Welded Joint under Repair Welding Thermal Shock Based on XFEM" Metals 10, no. 10: 1285. https://doi.org/10.3390/met10101285
APA StyleYang, K., Zhang, Y., & Zhao, J. (2020). Elastoplastic Fracture Analysis of the P91 Steel Welded Joint under Repair Welding Thermal Shock Based on XFEM. Metals, 10(10), 1285. https://doi.org/10.3390/met10101285






