New Developments in Understanding Harper–Dorn, Five-Power Law Creep and Power-Law Breakdown

Abstract

This paper discusses recent developments in creep, over a wide range of temperature, that may change our understanding of creep. The five-power law creep exponent (3.5–7) has never been explained in fundamental terms. The best the scientific community has done is to develop a natural three power-law creep equation that falls short of rationalizing the higher stress exponents that are typically five. This inability has persisted for many decades. Computational work examining the stress-dependence of the climb rate of edge dislocations may rationalize the phenomenological creep equations. Harper–Dorn creep, “discovered” over 60 years ago, has been immersed in controversy. Some investigators have insisted that a stress exponent of one is reasonable. Others believe that the observation of a stress exponent of one is a consequence of dislocation network frustration. Others believe the stress exponent is artificial due to the inclusion of restoration mechanisms, such as dynamic recrystallization or grain growth that is not of any consequence in the five power-law regime. Also, the experiments in the Harper–Dorn regime, which accumulate strain very slowly (sometimes over a year), may not have attained a true steady state. New theories suggest that the absence or presence of Harper–Dorn may be a consequence of the initial dislocation density. Novel experimental work suggests that power-law breakdown may be a consequence of a supersaturation of vacancies which increase self-diffusion.

1. Introduction

This paper will review recent work that appears to allow a better understanding of the basis of elevated temperature creep in single phase ceramics, minerals, metals, and class M (pure metal behavior) alloys. This paper does not directly address multiphase materials such as superalloys and dispersion-strengthened alloys. Elevated temperature creep can be described by an examination of Figure 1 for high purity aluminum. This data was compiled by Blum [1] and represents trends in steady-state creep behavior that are widely accepted. This plot describes steady-state creep where hardening processes are believed to be exclusively balanced by dynamic recovery. Figure 1 shows three regions of steady-state creep behavior ranging from:

(1)

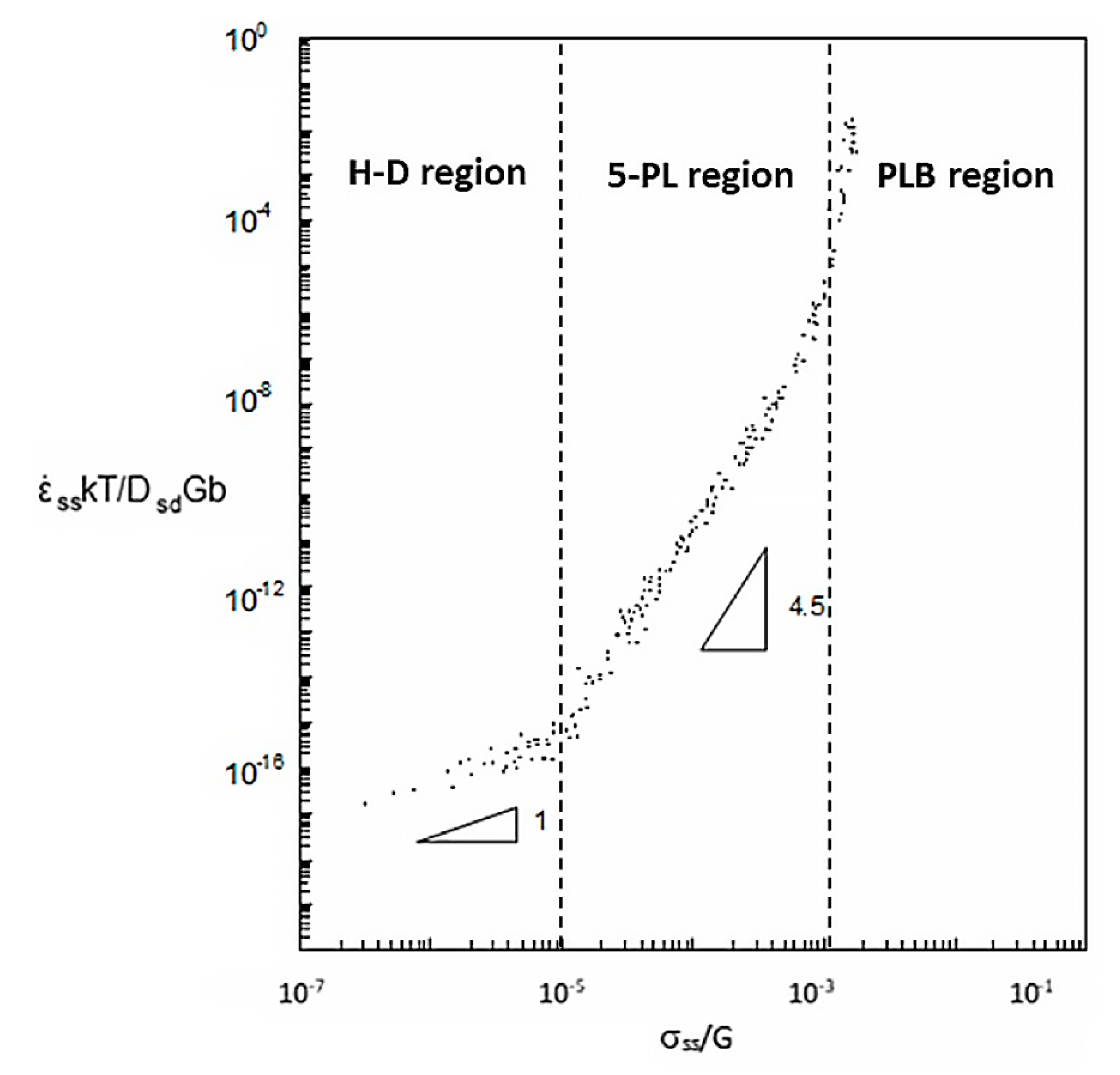
Temperatures near the melting point with a slope of 1 (stress exponent of 1), (HD).

(2)

Temperatures above approximately 0.6T_m where the constant slopes for crystalline materials vary from 3.5–7 (5-PL).

(3)

Below about 0.6T_m, the stress exponent is no longer constant and power-law breakdown is observed (PLB) This is sometime referred to as intermediate temperature creep that extends to roughly 0.3T_m.

The point here is that each of these regions have been poorly understood in the past. More recent work appears to offer clarification of the creep mechanisms for each of these regions. Each section will be described separately.

2. General Creep Plasticity Considerations and Five Power-Law Creep

Before each section is described, there are some general features that Harper–Dorn and five power-law creep (and perhaps PLB) have in common. First, there are three microstructural dislocation features that are often evident in the three creep regimes. These are the Frank network dislocation density, subgrains that may or may not be present, and the misorientation across boundaries (related to the spacing of the dislocations in the subgrain boundaries). Work by the author [2,3,4,5] showed that the rate-controlling process for plasticity in these regimes involved the Frank networks. Creep in both regimes can be described without consideration of the presence of subgrains. Several other investigators, e.g., Evans and Knowles [6], Kumar et al. [7], Northwood et al. [8], Ardell [9], Lagneborg et al. [10], Burton [11], and many others also considered Frank networks to be the microstructural feature associated with the rate-controlling process for elevated-temperature creep. The author further described a generalized equation based on the network model of Evans and Knowles [6]. This will turn out to be relevant to both the Harper–Dorn regime and the five power-law regime and possibly the power-law breakdown (PLB). The author wrote a detailed derivation of the network model [7]. The derivation is lengthy and only the highlights will be presented here:

First the climb velocity, v_c, must be calculated and the original expression comes from Weertman [12]. v_c is determined from the vacancy concentration gradient influenced by the fact that the formation energy for a vacancy is altered by the climb (applied) stress.

v_c = 2πD_L·[(Ωσ/kT)]·ln(R_o/b)

(1)

where Ω is the atomic volume, D_L is the lattice self-diffusion coefficient, and R_o is the diffusion distance. The activation energy for both five-power-law creep (5PL) and low stress creep (so-called Harper–Dorn (HD) creep) is that for lattice self-diffusion suggesting that in both regimes, edge dislocation climb is rate-controlling (in PLB, the activation energy appears to decrease from that of the 5-PL and HD regimes [13,14]). Note in particular that the stress exponent = 1 in Equation (1).

Weertman suggests that the approximate average dislocation velocity is:

v’ ~ v_cx_g/x_c

(2)

where x_c is the climb distance and x_g is the glide distance. Also,

$${\dot{\epsilon }}_{ss} ={\rho }_{\mathrm{m}}{bv}^{\prime }$$

(3)

where ρ_m is the mobile dislocation density. It has been phenomenologically observed that frequently in the five-power regime,

ρ = (σ_ss/Gb)^p

(4)

where ρ is the total dislocation density. This is not the Taylor equation although it is frequently claimed such since many believe that p is about 2.0. Actually, the proper strengthening equation is:

$${{\sigma }_y|}_{\dot{\epsilon },T}={\sigma }_0{}^{\prime }+\alpha MGb{\left(\rho \right)}^{\frac{1}{2}}$$

(5)

where σ_o′ is the strengthening effect of solutes/impurities, Peierls stress, etc., and α is a constant and varies from 0.2 to 0.4 among metals. It should be pointed out that, even in high purity Al (99.999% pure), σ_o′ can be a significant (e.g., over half) contributor to elevated temperature strength. That is, the strength at a fixed temperature and strain rate is the sum of an athermal (dislocation hardening) and the thermally activated term σ_o′.

Weertman suggested that the total dislocation density is equal to the mobile dislocation density (probably a bit unrealistic) which leads to:

$${\dot{\epsilon }}_{ss}=K·D_{\mathrm{sd}}/bG\mathsf{\Omega }/k{\rm T}\left(x_{\mathrm{g}}/x_{\mathrm{c}}\right)·{\left(\sigma /G\right)}^3$$

(6)

which is often referred to as the “natural three power law” equation. The problem is that the stress exponents in the “five-power law regime” are 3.5–7 and typically five. The exponent for high purity aluminum in Figure 1 is about 4.5. The creep plasticity community has not been able to derive an equation for five-power law creep and this has been a deficiency for a very long time. There have been mathematical gymnastics performed to derive a higher stress exponent (including Weertman [15]), but these have not been embraced by the community, and the natural three power law creep equations have persisted [16].The author also showed that in both Cu and Al, long-range internal stresses are not evident and the only the applied stress is relevant [17].

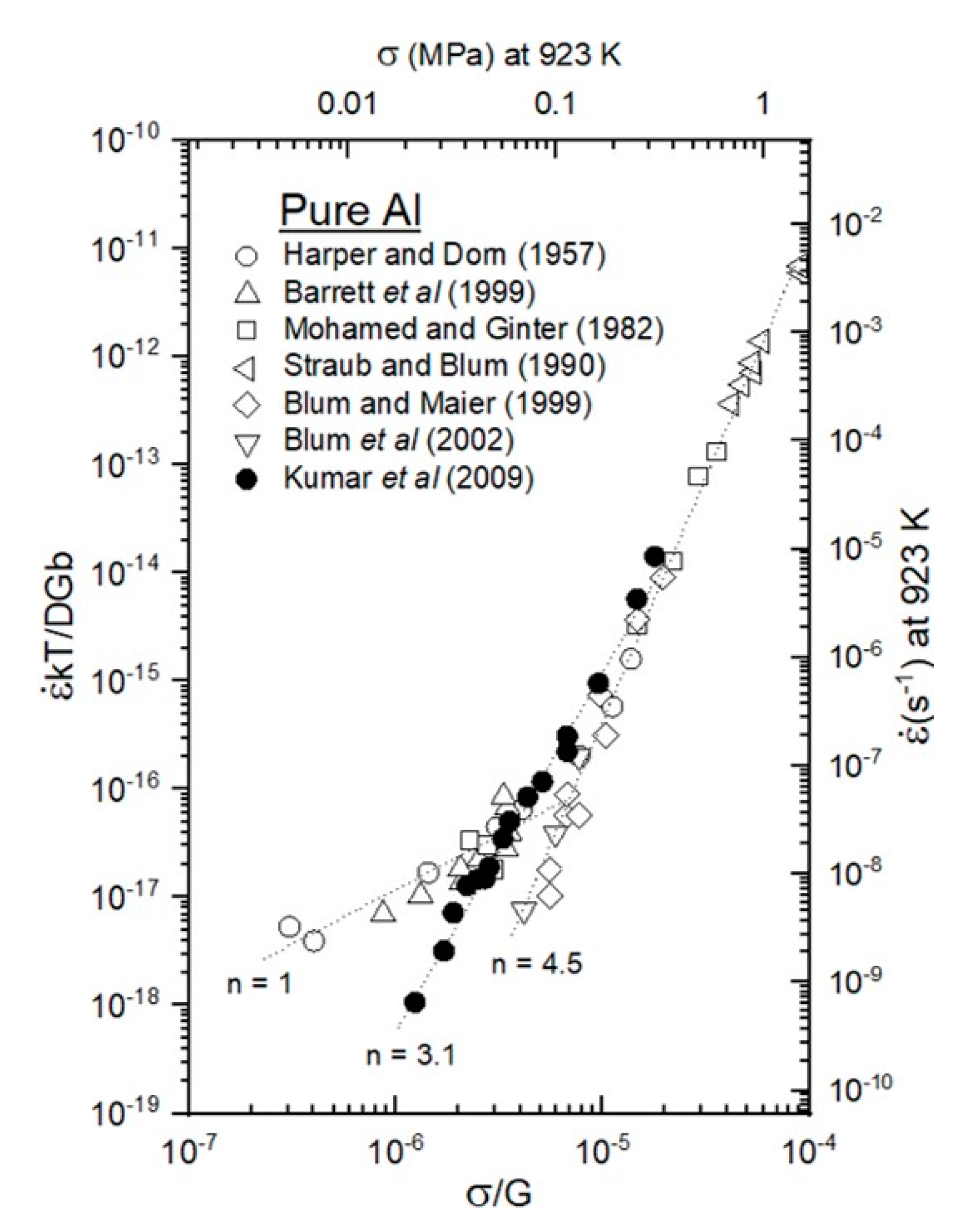
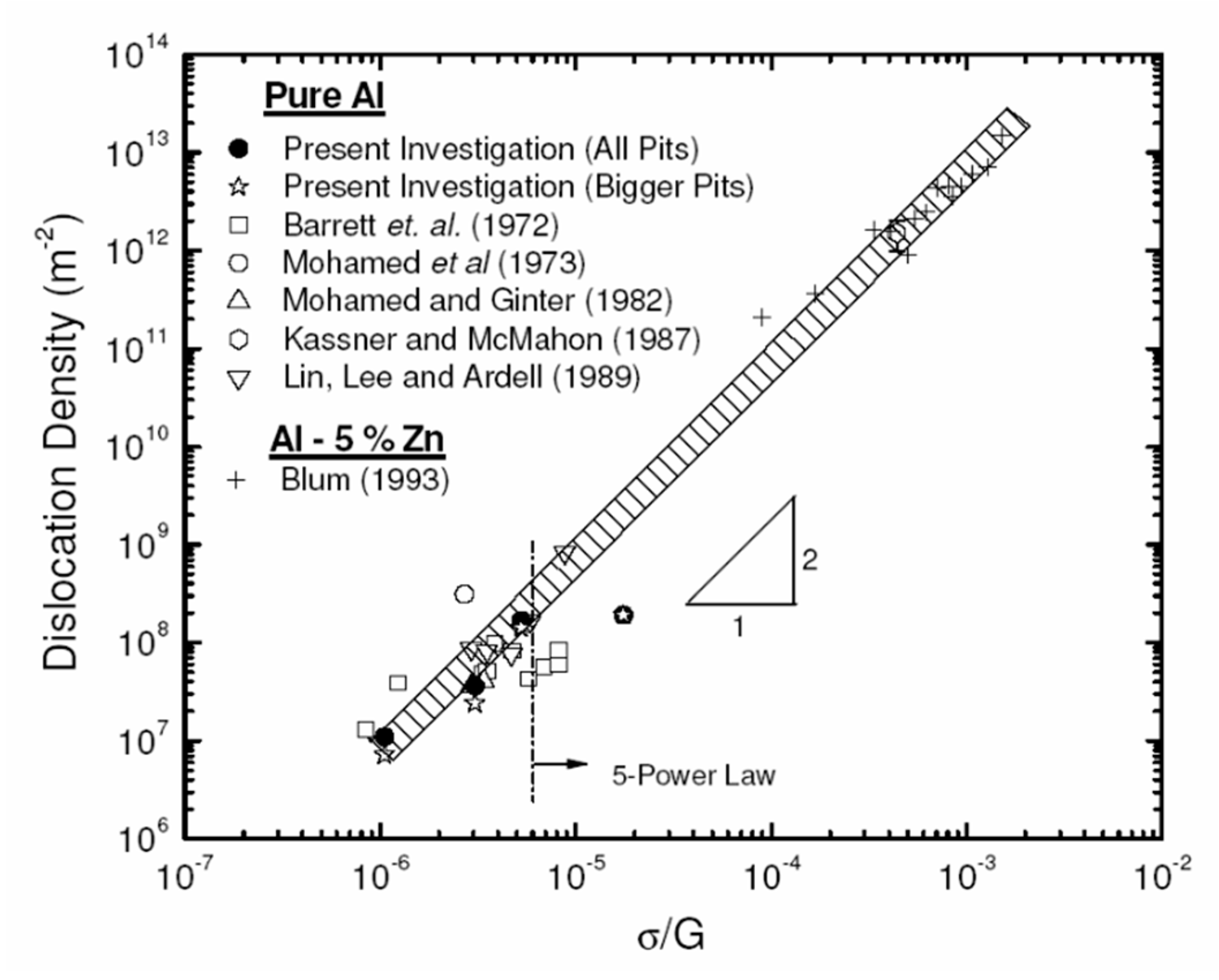
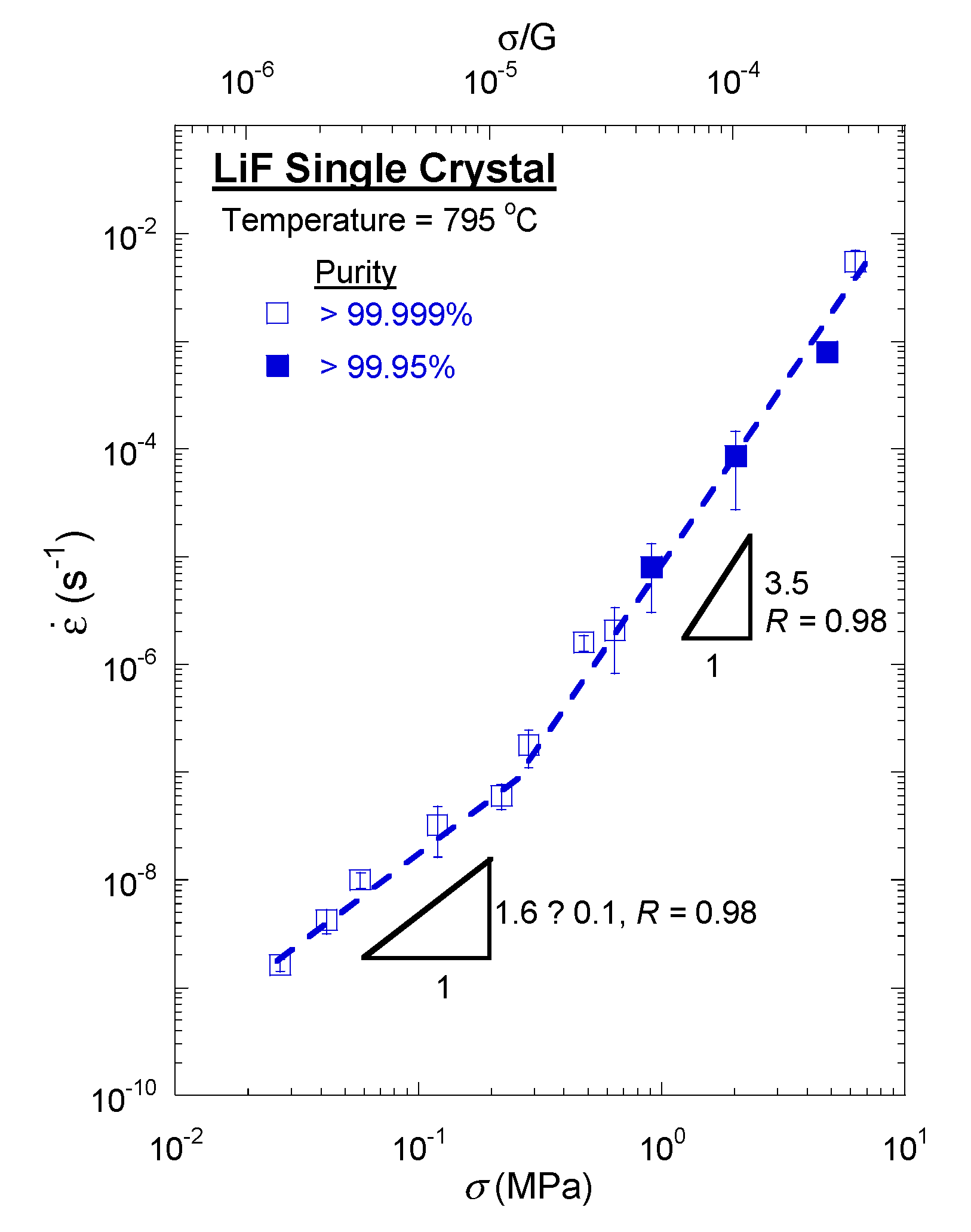
3. The So-Called Harper–Dorn Regime

Blum’s plot in Figure 1 also suggests that at very low stresses (often in association with high temperatures) a new creep regime has been reported (over 60 years ago) that was termed Harper–Dorn (HD) creep [18]:

$${\dot{\epsilon }}_{ss}=A_{HD}\left(\frac{D_{sd}Gb}{kT}\right){\left(\frac{\sigma }{G}\right)}^1$$

(7)

where A_HD is a constant and the other terms have their usual meanings. Many investigators observed that unlike the higher stress five-power law regime, the steady-state dislocation density is constant in the so-called Harper–Dorn regime. As pointed out in a review by the author [19], the power of one has been observed in a variety of materials including Al [5,6,7,20], Ti [21], Fe [22], Co [23], Zr [24], and Sn [20]. It has also been suggested to be observed in ceramics such as CaO [25], UO₂, [26] MgO [27,28], NaCl [29,30], and also ice [31]. In the case of Al and Pb [20], a failure to observe Harper–Dorn occurred, and five power-law was observed instead at stresses associated Harper–Dorn observations. The early low-stress experiments, primarily of metals, indicated that Harper–Dorn includes:

• Activation energy about equal to lattice self-diffusion,
• Grain-size independence with grain boundary shearing,
• Steady-state stress exponent of one,
• Dislocation density that appears independent of stress, and
• A primary creep stage.

Items 2 and 5 were considered important as these preclude Nabarro-Herring diffusional creep [3] (which may not exist). Nabarro-Herring does not have a primary creep stage and must have a grain size dependence. There have been several theories to justify Equation (7) [32,33,34,35,36] and all, basically, suggest that, since ρ is constant, only dislocation climb affects the stress exponent with a value of one (Equation (1)). Interestingly, more recent work by the author and others [37,38,39] indicates that at least under some conditions, the stress exponent is larger than one, as in Figure 2. The author’s work suggest that this is a consequence of a non-constant dislocation density. In fact, for Al single crystals deformed in the so-called Harper–Dorn regime, the exponent “p” is roughly two in Equation (4) giving rise to a stress exponent of about three. It is possible that, in some cases at high (Harper–Dorn) temperatures and/or low stresses, the network dislocation density is not constant as widely presumed.

The author speculated [39,40] that variation in the stress-exponent behavior in the Harper–Dorn regime might be explained by the value of the starting dislocation density in the material (just before the application of a stress) and the observation that even after annealing single crystals for a year, the dislocation density remains a constant; it reaches a “frustration” value (ρ_f). There is no quantitatively way to predict this value at this time. Further annealing cannot decrease below the value of ρ_f even after annealing at very high temperatures for over a year in the three materials examined, LiF, NaCl and Al, all single crystals.

The frustration dislocation density is fairly low and initial values of ρ just before applying the stress at elevated may be higher than the frustration value as illustrated in Figure 3. But also, as the author discovered [41] for Al, the initial dislocation density can be lower than ρ_f. Nes and Nost [42] discovered that very slow cooling of Al from the melt can produce dislocation densities that are especially low, significantly below the frustration dislocation density. In contrast, Figure 4 shows that the initial dislocation density is relatively high for LiF. Nes and Nost [42] also found a stress-dependency of the dislocation density in the Harper–Dorn regime. Dislocation density measurements using X-ray topography showed that ρ was dependent on stress by σ^1.3. Thus, in two cases [41,42], it appears that if the starting dislocation density is below ρ_f, then Harper–Dorn creep may not be observed and that (regular or normal) five-power law creep is observed instead. Blum et al. [38], also observed five-power law creep in Al, but did not determine the initial dislocation density. Additional experiments were performed by McNee and coworkers [40] on 99.999 pure Al. These investigators, consistent with Blum et al. and the author’s work [38,41], observed the extension of five-power-law creep into Harper–Dorn regime.

Most recently, Singh et al. [39] were the first to discover that low stress/high-temperature creep in LiF is consistent with Harper–Dorn creep (i.e., Equation (7)). Work on Pb by Mohammed et al. [20] also found that this metal does not evince Harper–Dorn creep. Dislocation densities were not measured, see Figure 3. Plots the dislocation density of Al deformed to steady state both within the five-power law and the Harper–Dorn regime.

Mohammed et al. [43] reported that the microstructure of the 99.9995 Al includes wavy grain boundaries, an inhomogeneous dislocation density distribution as determined by etch pits, small new grains forming at the specimen surface and large dislocation density gradients across grain boundaries. Well-defined subgrains were not observed. However, they found that the microstructure of the deformed 99.99 Al consists of a well-defined array of subgrains and exhibits five power law behavior. These observations led Mohammed et al. to conclude that the restoration mechanism taking place during so-called “Harper–Dorn creep” includes discontinuous dynamic recrystallization (DRX) in addition to dynamic recovery, at least in Al. This restoration mechanism could give rise to the periodic accelerations observed in the creep curve. It was suggested that it is difficult to accurately determine the stress exponent due to the appearance of periodic accelerations in the creep curves. However, Mohammed et al. [43] claimed that n = 1 exponents are only obtained if creep curves up to small strains (1–2%) are analyzed, as was typically the case in the past. Mohamed et al. estimated stress exponents of about 2.5 at larger strains for high purity polycrystalline DRX specimens of Al. Also, Singh et al. [25] in 99.999% pure LiF single crystals observed a power-1.5 behavior [14] in the HD regime. The exponent was independent of strain and the dislocation density is constant for a fixed stress for a period of over one year.

It is important to utilize single crystals in HD experiments as grain boundary migration can be an independent restoration mechanism [44]. Care must also be taken to ensure that discontinuous dynamic recrystallization is not occurring in Al as DRX may occur even at ambient temperature albeit at relatively large strains. [45,46]. It is possible that Mohammed et al. observed grain growth as an additional restoration mechanism rather than DRX [44]. Kumar et al. [41] never observed DRX nor subgrains in Al single crystals deformed in the HD regime and observed a stress exponent slightly over 3. Power-one is legitimate in some cases even in the absence of restoration mechanisms that are not significant in five power-law creep where steady-state is a balance between dynamic recovery and hardening. It should be pointed out that McNee et al. [47] also tested Al of both 99.999 and 99.99%purity and did not observe Harper–Dorn behavior with either purity. It should be mentioned that Blum et al. [38] did not confirm Harper–Dorn creep in 99.99% pure oligo-crystal aluminum. Rather they observed normal five-power law creep extend to very low stresses within the HD regime [38]. The starting dislocation density was not measured. According to Blum et al., one explanation for others not observing stress exponents of one (Harper–Dorn creep) is that there were insufficient strains to reach steady-state.

Figure 5 demonstrates that whenever the starting dislocation density is at or greater than ρ_f, then substantial hardening may not be observed as the dislocation density reduces to a frustration value (ρ_f) and a low (e.g., 1–2) stress exponent is observed. The as-grown material will recover some of the dislocation density before the application of the stress once the as-received material equilibrates to the test temperature. However, if the dislocation density is low then hardening can occur and (normal) five-power law behavior is observed. The basis for dislocation network frustration is unclear. Certainly, further decreases from ρ_f is thermodynamically favored. Ardell [9] proposed that coarsening of the network requires that Frank’s rule is satisfied at the nodes. The sum of the Burgers vectors must always sum to zero. Ardell suggested that frustration occurs when further coarsening is impossible when Frank’s rule can no longer be satisfied.

The LiF data is illustrated in Figure 6. It can be noted that the plot suggests a stress exponent of about 1.6, as mentioned earlier, in the HD regime (which is greater than the value of one in Equation (7)). Interestingly, other materials such as Cu and CaO [25] deformed in the HD regime also have values closer to 2 than 1. Recent work by Mompiou et al. and Kabir et al. [48,49] suggested that the climb velocity dependence on the stress has a stress exponent greater than 1 based on computational modeling of the climb process. Thus, there may be inaccuracy in Equation (1). The stress exponent of 1 in Equation (7) is a consequence of the effect of the applied stress on the dislocation climb-rate based on the analytic projection by Weertman. The deviation of the stress exponent may imply that the stress exponent for five power-law creep and Harper–Dorn creep (constant dislocation density with changes in stress) could be both higher than 3 (Figure 1) and 1, respectively. It should be mentioned that Harper and Dorn did not use the applied stress in obtaining a stress exponent of one for polycrystalline Al. Rather they subtracted a surface tension stress from the applied stress. The extrapolation of the stress to zero strain-rate results in a positive stress. Another investigation did not observe this. Had Harper and Dorn not subtracted this stress, a stress exponent of three would have been observed. Overall, it appears that Harper–Dorn (stress exponents of one) is not commonly observed in Al as is widely believed.

4. Power law Breakdown

There have been various explanations for PLB [50,51,52,53,54]: (1) It has been proposed that there is a change in the rate-controlling mechanism of steady-state plastic flow from dislocation climb-control in 5-PL to glide-control in PLB. However, this proposition is sometimes based on the presence of internal stress which does not appear to be reasonable as the long-range internal stresses (usually suggested to give rise to high local stresses at dislocation heterogeneities) appears to be low in both the PL and PLB regimes [17]. (2) Only recently has vacancy supersaturation from plasticity been experimentally verified in the PLB regime. The levels of supersaturation appear sufficient to create higher creep-rates, and thus PLB. The excess vacancies would decrease the activation energy for diffusion, as observed [13,14]. (3) The changes in the diffusion coefficient from D_sd to D_p (dislocation pipe diffusion coefficient) with large strain plasticity within the PLB regime may independently contribute to the observation of PLB [14]. Another point must be made in that references [55,56] experimentally verify vacancy supersaturation at ambient temperature. Other, somewhat higher temperatures, within PLB were not checked. Of course, higher temperature X-ray diffraction experiments are more difficult than those at ambient temperature. Perhaps future experiments could be performed at other temperatures within PLB to fully verify the coincidence between the onset of PLB and vacancy supersaturation.

5. Conclusions

Power-law, power-law-breakdown, and the so-called Harper–Dorn creep have been re-examined in terms of some of the latest developments within these creep regimes. It appears that within the Harper–Dorn regime, stress exponents that are higher than the expected value of one can be observed depending on the initial dislocation density. Very low starting dislocation densities may yield stress exponents closer to those observed in normal five power law creep. The five power-law creep exponent may be finally explained by incorporating more realistic dislocation climb-rate stress-exponents that may exceed a value of one according to recent computational work. Recent X-ray work assessing the vacancy concentration in plastically deformed materials suggests that power-law breakdown may be explained by vacancy supersaturation based on moving jogs leading to enhanced creep rates.