A New Computational Tool for the Development of Advanced Exergy Analysis and LCA on Single Effect LiBr–H2O Solar Absorption Refrigeration System
Abstract
:1. Introduction
2. Methodology
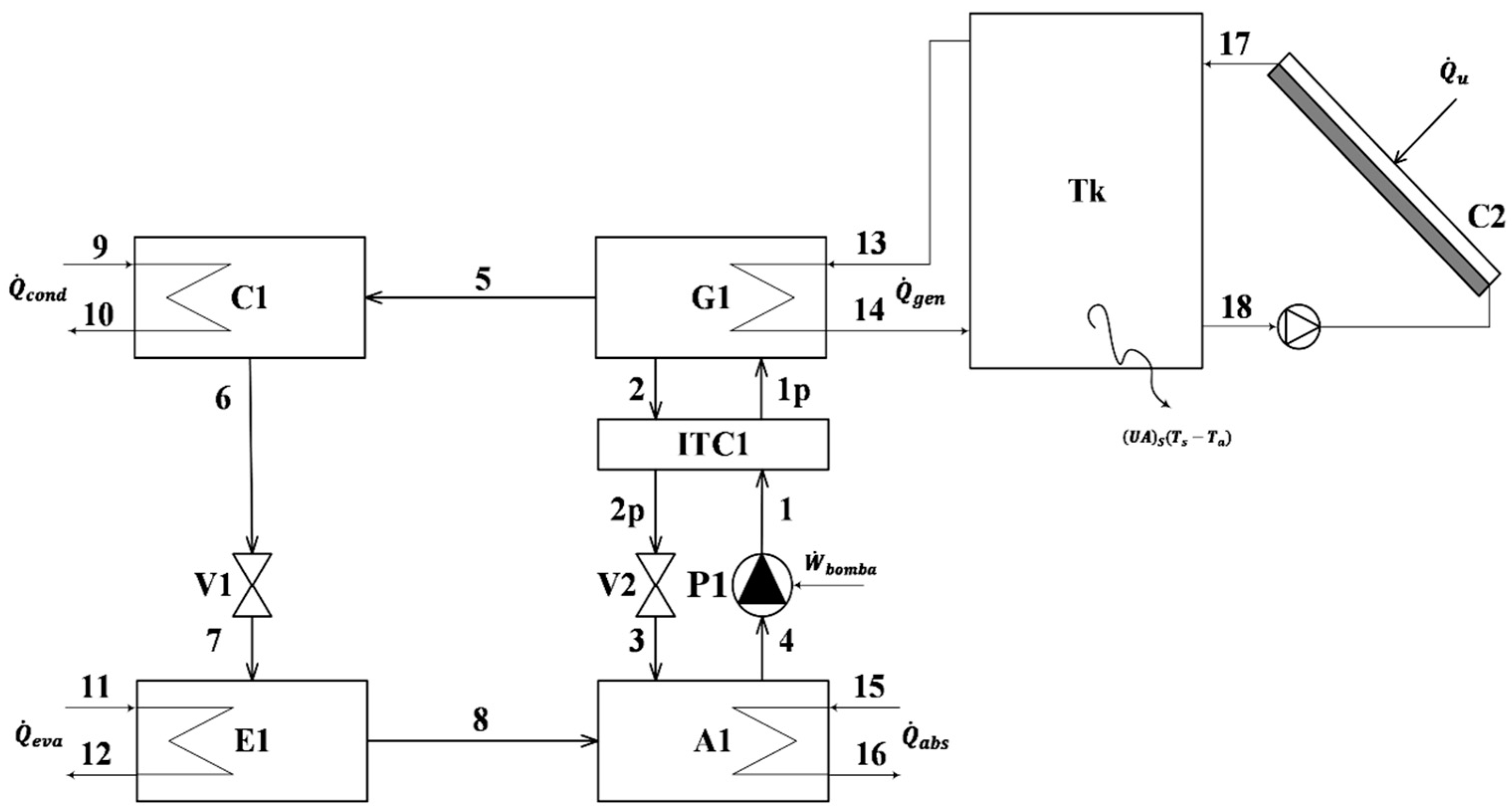
2.1. Description of the System
2.2. Thermodynamics Analysis: Vapor Absorption Refrigeration System
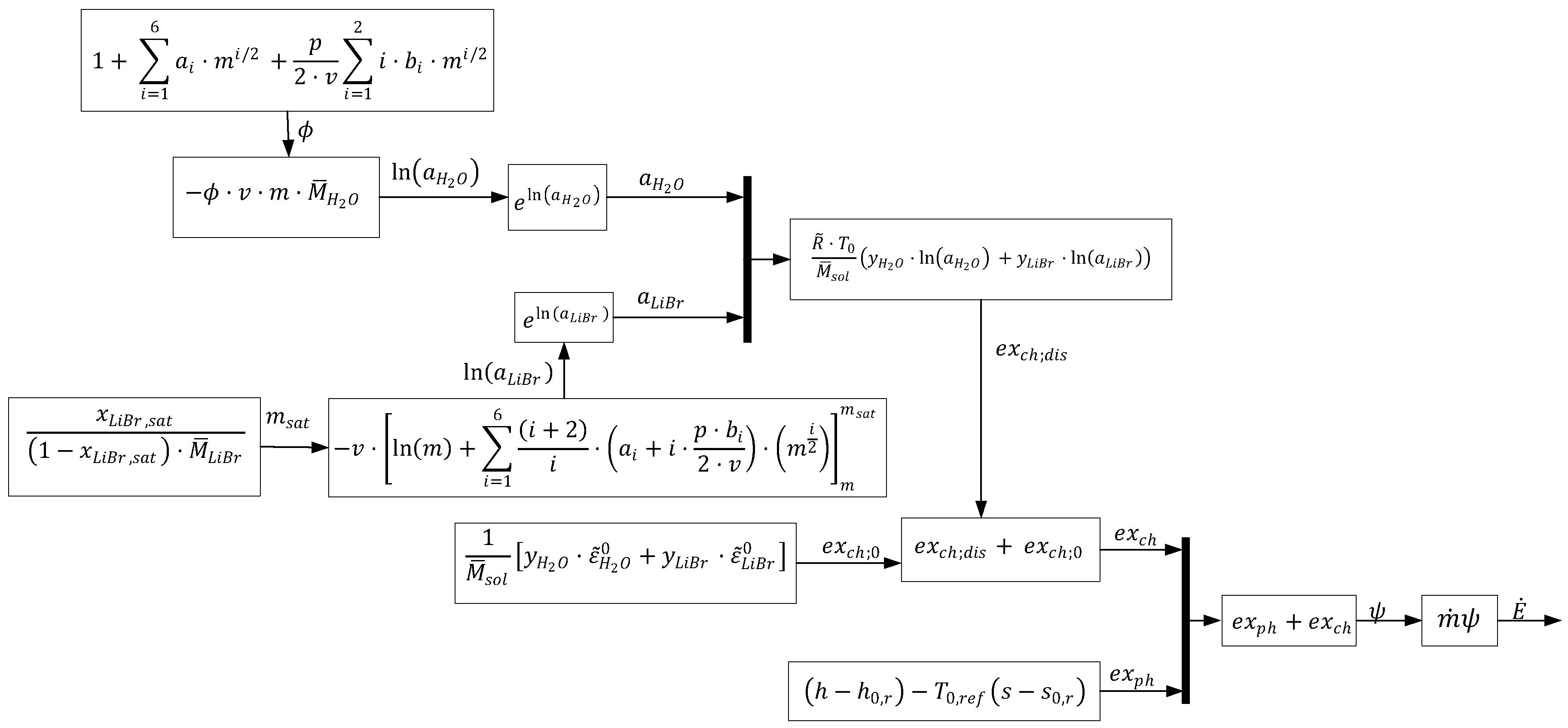
2.3. Exergy Disaggregation
2.4. Solar Collector and Storage Tank
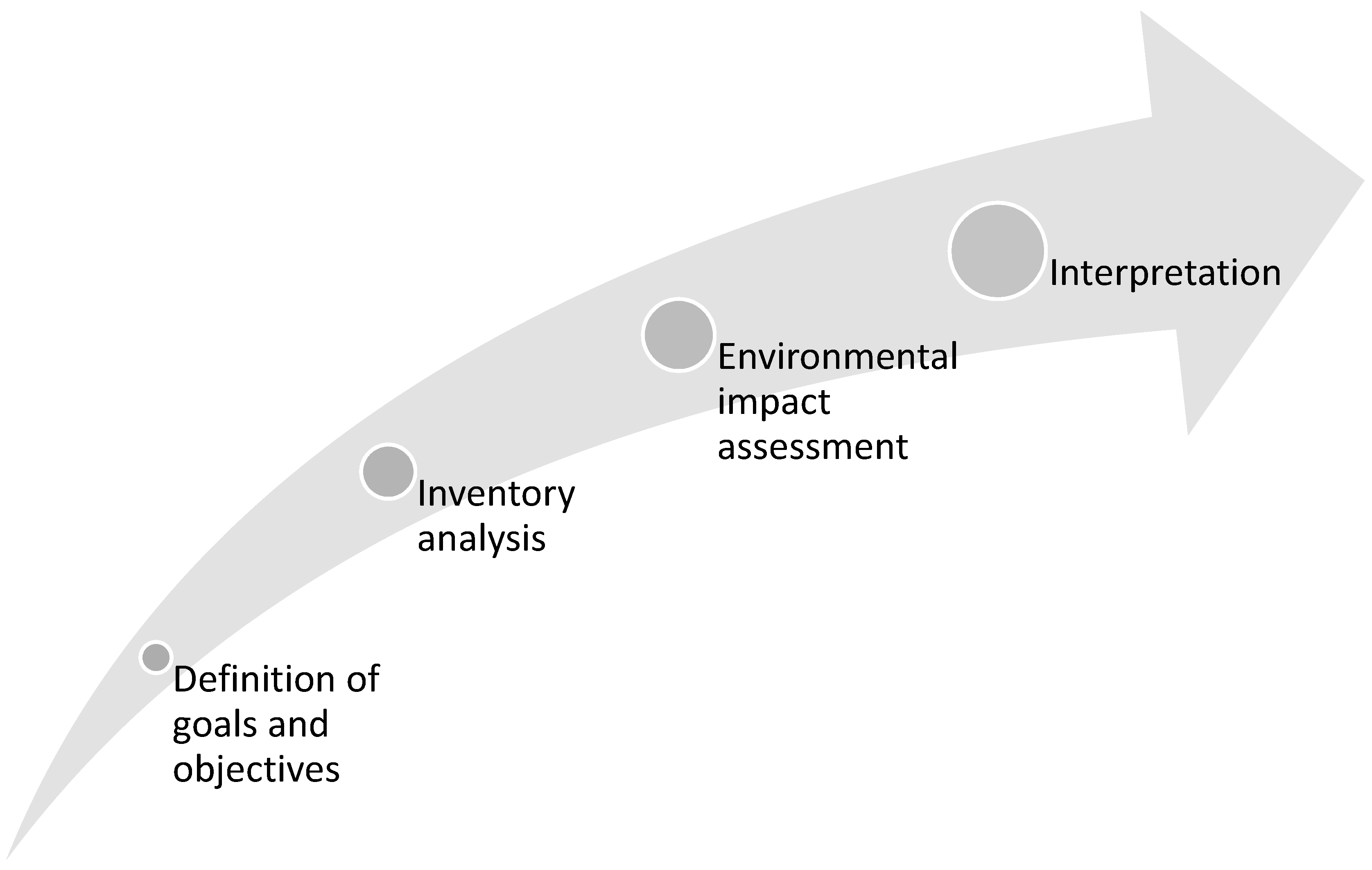
2.5. LCA Solar Absorption Refrigeration System
3. Results and Discussions
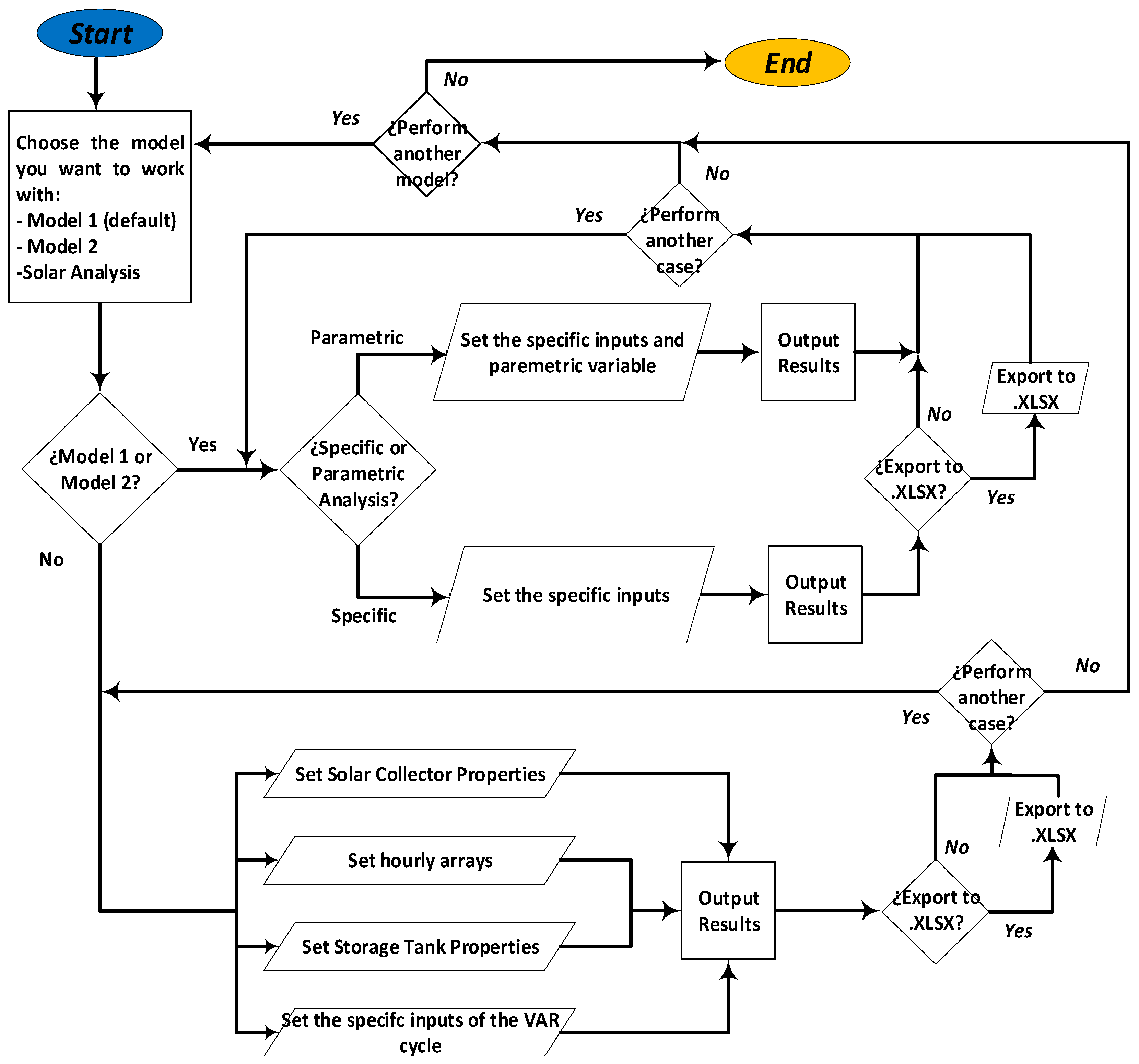
3.1. MATLAB App Designer Application
3.2. Properties Validation
3.3. Software Validation
3.4. Solar Analysis
3.5. LCA Solar Absorption Refrigeration System Results
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| IDEAM | Institute of Hydrology, Meteorology and Environmental Studies (in Spanish: Instituto de Hidrología, Meteorología y Estudios Ambientales) |
| VCR | Vapor compression refrigeration |
| VAR | Vapor absorption refrigeration |
| COP | Coefficient of performance |
| ECOP | Exergy efficiency |
| IR | Ratio of irreversibility |
| FDR | Exergy depletion ratio |
| HCFC | hydrochlorofluorocarbons |
| IAE | International Agency of Energy |
| ASHRAE | American Society of Heating, Refrigerating, and Air-Conditioning Engineers |
| LCA | Life cycle analysis |
| GUI | Graphical user interface |
| Nomenclature | |
| Exergy flow (kJ/kg) | |
| Total exergy (kW) | |
| Exergy efficiency (%) | |
| Mass flow rate (kg/s) | |
| Mass (kg) | |
| Heat transfer (kW) | |
| Temperature (K) | |
| Pressure (kPa) | |
| Pressure drop (kPa) | |
| LiBr–water concentration (%) | |
| Useful power produced by solar collector (kW) | |
| Solar collector area (m2) | |
| Total solar irradiance incident on the solar collector (Wh/m2) | |
| Instantaneous efficiency of the solar collector (%) | |
| Solar collector heat removal factor (dimensionless) | |
| Transmittance–absorbance product or optical efficiency (%) | |
| Thermal power required by VAR (kW) | |
| Product coefficient of losses per storage tank area (W/°C) | |
| interval (°C) | |
| Temperature of the storage tank during the next interval (°C) | |
| Room temperature (°C) | |
| Apparent temperature of the Sun (°C) | |
| Calorific capacity (J/K) | |
| in | Input |
| out | Output |
| Superscripts | |
| AV | Avoidable |
| UN | Unavoidable |
| EN | Endogenous |
| EX | Exogenous |
| EN, AV | Endogenous avoidable |
| EN, UN | Endogenous unavoidable |
| EX, AV | Exogenous avoidable |
| EX, UN | Exogenous unavoidable |
| Subscripts | |
| s | Systems/plural |
| rev | Reversible cycle |
| Physical | |
| Chemical | |
| Saturated | |
| Absorber | |
| Generator | |
| Condenser | |
| Evaporator | |
| Solution heat exchanger | |
| Solution | |
| Indicates the property is in reference conditions. | |
| K-th component | |
| Fuel of K-th component | |
| Product of K-th component | |
| Destruction of K-th component | |
| Standard chemical property of pure species | |
| Chemical property destroyed due to the dissolution process | |
References
- Solano–Olivares, K.; Romero, R.J.; Santoyo, E.; Herrera, I.; Galindo–Luna, Y.R.; Rodríguez–Martínez, A.; Santoyo-Castelazo, E.; Cerezo, J. Life cycle assessment of a solar absorption air-conditioning system. J. Clean. Prod. 2019, 240, 118206. [Google Scholar] [CrossRef]
- Khan, J.; Arsalan, M.H. Solar power technologies for sustainable electricity generation—A review. Renew. Sustain. Energy Rev. 2016, 55, 414–425. [Google Scholar] [CrossRef]
- Alobaid, M.; Hughes, B.; Calautit, J.K.; O’Connor, D.; Heyes, A. A review of solar driven absorption cooling with photovoltaic thermal systems. Renew. Sustain. Energy Rev. 2017, 76, 728–742. [Google Scholar] [CrossRef] [Green Version]
- Shirmohammadi, R.; Soltanieh, M.; Romeo, L.M. Thermoeconomic analysis and optimization of post-combustion CO2 recovery unit utilizing absorption refrigeration system for a natural-gas-fired power plant. Environ. Prog. Sustain. Energy 2018, 37, 1075–1084. [Google Scholar] [CrossRef]
- Salmi, W.; Vanttola, J.; Elg, M.; Kuosa, M.; Lahdelma, R. Using waste heat of ship as energy source for an absorption refrigeration system. Appl. Therm. Eng. 2017, 115, 501–516. [Google Scholar] [CrossRef]
- Herold, K.E.; Radermacher, R.; Klein, S.A. Absorption Chillers and Heat Pumps; CRC Press: Boca Raton, FL, USA, 2016; ISBN 9781498714358. [Google Scholar]
- Mendoza, E.; Velásquez, M.; Medina, D.; Nuñez, J.R.; Grimaldo, J.W. An analysis of electricity generation with renewable resources in Germany. Int. J. Energy Econ. Policy 2020, 10, 361–367. [Google Scholar] [CrossRef]
- Nuñez, J.R.; Benitez, I.; Llosas, Y. Communications in Flexible Supervisor for Laboratory Research in Renewable Energy. IOP Conf. Ser. Mater. Sci. Eng. 2020, 844, 012016. [Google Scholar] [CrossRef]
- Gao, J.T.; Xu, Z.Y.; Chiu, J.N.W.; Su, C.; Wang, R.Z. Feasibility and economic analysis of solution transportation absorption system for long-distance thermal transportation under low ambient temperature. Energy Convers. Manag. 2019, 196, 793–806. [Google Scholar] [CrossRef]
- Núñez Alvarez, J.R.; Benítez, I.F.; Proenza, R.; Luis, V.S.; David, D.M. Metodología de diagnóstico de fallos para sistemas fotovoltaicos de conexión a red. Rev. Iberoam. Autom. Inf. Ind. 2020, 17, 94–105. [Google Scholar] [CrossRef]
- Ansarinasab, H.; Hajabdollahi, H.; Fatimah, M. Life cycle assessment (LCA) of a novel geothermal-based multigeneration system using LNG cold energy- integration of Kalina cycle, stirling engine, desalination unit and magnetic refrigeration system. Energy 2021, 231, 120888. [Google Scholar] [CrossRef]
- Murphy, M.P.A. COVID-19 and emergency eLearning: Consequences of the securitization of higher education for post-pandemic pedagogy. Contemp. Secur. Policy 2020, 41, 492–505. [Google Scholar] [CrossRef]
- Baran, E.; Baran, E.; AlZoubi, D. Human-Centered Design as a Frame for Transition to Remote Teaching during the COVID-19 Pandemic. J. Technol. Teach. Educ. 2020, 28, 365–372. [Google Scholar]
- Piero Rojas, J.; Valencia Ochoa, G.; Duarte Forero, J. Comparative Performance of a Hybrid Renewable Energy Generation System with Dynamic Load Demand. Appl. Sci. 2020, 10, 3093. [Google Scholar] [CrossRef]
- Brunet, R.; Cortés, D.; Guillén-Gosálbez, G.; Jiménez, L.; Boer, D. Minimization of the LCA impact of thermodynamic cycles using a combined simulation-optimization approach. Appl. Therm. Eng. 2012, 48, 367–377. [Google Scholar] [CrossRef]
- Valencia Ochoa, G.; Duarte Forero, J.; Rojas, J.P. A comparative energy and exergy optimization of a supercritical-CO2 Brayton cycle and Organic Rankine Cycle combined system using swarm intelligence algorithms. Heliyon 2020, 6, e04136. [Google Scholar] [CrossRef]
- Denzinger, C.; Berkemeier, G.; Winter, O.; Worsham, M.; Labrador, C.; Willard, K.; Altaher, A.; Schuleter, J.; Ciric, A.; Choi, J.K. Toward sustainable refrigeration systems: Life cycle assessment of a bench-scale solar-thermal adsorption refrigerator. Int. J. Refrig. 2021, 121, 105–113. [Google Scholar] [CrossRef]
- Barrozo, F.; Valencia, G.; Obregón, L.; Arango, A.; Nuñez, J.R. Energy, Economic and Environmental Evaluation of a Solar-Wind Power on-grid System: Case study in Colombia. Energies 2020, 13, 1662. [Google Scholar] [CrossRef]
- Diaz, G.A.; Duarte, J.O.; Garcia, J.; Rincon, A.; Fontalvo, A.; Bula, A.; Padilla, R.V. Maximum power from fluid flow by applying the first and second laws of thermodynamics. J. Energy Resour. Technol. Trans. ASME 2017, 139, 035021. [Google Scholar] [CrossRef]
- Liu, X.; Yang, X.; Yu, M.; Zhang, W.; Wang, Y.; Cui, P.; Zhu, Z.; Ma, Y.; Gao, J. Energy, exergy, economic and environmental (4E) analysis of an integrated process combining CO2 capture and storage, an organic Rankine cycle and an absorption refrigeration cycle. Energy Convers. Manag. 2020, 210, 112738. [Google Scholar] [CrossRef]
- Abas, N.; Kalair, A.R.; Khan, N.; Haider, A.; Saleem, Z.; Saleem, M.S. Natural and synthetic refrigerants, global warming: A review. Renew. Sustain. Energy Rev. 2018, 90, 557–569. [Google Scholar] [CrossRef]
- Valencia Ochoa, G.; Cárdenas Gutierrez, J.; Duarte Forero, J. Exergy, Economic, and Life-Cycle Assessment of ORC System for Waste Heat Recovery in a Natural Gas Internal Combustion Engine. Resources 2020, 9, 2. [Google Scholar] [CrossRef] [Green Version]
- Nuñez, J.R.; Benitez, I.; Martínez, A.; Díaz, S.; de Oliveira, J. Tools for the Implementation of a SCADA System in a Desalination Process. IEEE Lat. Am. Trans. 2019, 17, 11, 1858–1864. [Google Scholar]
- OECD/IEA. The Future of Cooling Opportunities for Energy-Efficient Air Conditioning; IEA: Paris, France, 2018. [Google Scholar]
- Ramírez, R.; Gutiérrez, A.S.; Cabello Eras, J.J.; Valencia, K.; Hernández, B.; Duarte Forero, J. Evaluation of the energy recovery potential of thermoelectric generators in diesel engines. J. Clean. Prod. 2019, 241, 118412. [Google Scholar] [CrossRef]
- Ochoa, G.V.; Isaza-Roldan, C.; Forero, J.D. A phenomenological base semi-physical thermodynamic model for the cylinder and exhaust manifold of a natural gas 2-megawatt four-stroke internal combustion engine. Heliyon 2019, 5, e02700. [Google Scholar] [CrossRef] [Green Version]
- Palomino, K.; Reyes, F.; Nuñez, J.; Valencia, G.; Herrera, R. Wind Speed Prediction Based on Univariate ARIMA and MCO on the Colombian Caribbean Coast. J. Eng. Sci. Technol. Rev. 2020, 13, 200–205. [Google Scholar] [CrossRef]
- Wonchala, J.; Hazledine, M.; Goni Boulama, K. Solution procedure and performance evaluation for a water-LiBr absorption refrigeration machine. Energy 2014, 65, 272–284. [Google Scholar] [CrossRef]
- Morosuk, T.; Tsatsaronis, G. A new approach to the exergy analysis of absorption refrigeration machines. Energy 2008, 33, 890–907. [Google Scholar] [CrossRef]
- Bell, I.H.; Wronski, J.; Quoilin, S.; Lemort, V. Pure and Pseudo-pure Fluid Thermophysical Property Evaluation and the Open-Source Thermophysical Property Library CoolProp. Ind. Eng. Chem. Res. 2014, 53, 2498–2508. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Kim, D.S.; Ferreira, C.A.I. A Gibbs energy equation for LiBr aqueous solutions. Int. J. Refrig. 2006, 29, 36–46. [Google Scholar] [CrossRef]
- Kaita, Y. Thermophysical property data for lithium bromide/water solutions at elevated temperatures. Int. J. Refrig. 2001, 24, 374–390. [Google Scholar] [CrossRef]
- Yuan, Z.; Herold, K.E. Thermodynamic properties of aqueous lithium bromide using a multiproperty free energy correlation. HVAC R Res. 2005, 11, 377–393. [Google Scholar] [CrossRef]
- Qin, S.; Chang, S.; Yao, Q. Modeling, thermodynamic and techno-economic analysis of coal-to-liquids process with different entrained flow coal gasifiers. Appl. Energy 2018, 229, 413–432. [Google Scholar] [CrossRef]
- Palacios-Bereche, R.; Gonzales, R.; Nebra, S.A. Exergy calculation of lithium bromide-water solution and its application in the exergetic evaluation of absorption refrigeration systems LiBr-H2O. Int. J. Energy Res. 2012, 36, 166–181. [Google Scholar] [CrossRef]
- Valencia Ochoa, G.; Piero Rojas, J.; Duarte Forero, J. Advance Exergo-Economic Analysis of a Waste Heat Recovery System Using ORC for a Bottoming Natural Gas Engine. Energies 2020, 13, 267. [Google Scholar] [CrossRef] [Green Version]
- Valencia, G.; Fontalvo, A.; Cárdenas, Y.; Duarte, J.; Isaza, C. Energy and Exergy Analysis of Different Exhaust Waste Heat Recovery Systems for Natural Gas Engine Based on ORC. Energies 2019, 12, 2378. [Google Scholar] [CrossRef] [Green Version]
- Morosuk, T.; Tsatsaronis, G. Advanced exergetic evaluation of refrigeration machines using different working fluids. Energy 2009, 34, 2248–2258. [Google Scholar] [CrossRef]
- Valencia Ochoa, G.; Acevedo Peñaloza, C.; Duarte Forero, J. Thermoeconomic Optimization with PSO Algorithm of Waste Heat Recovery Systems Based on Organic Rankine Cycle System for a Natural Gas Engine. Energies 2019, 12, 4165. [Google Scholar] [CrossRef] [Green Version]
- Valencia Ochoa, G.; Acevedo Peñaloza, C.; Duarte Forero, J. Thermo-Economic Assessment of a Gas Microturbine-Absorption Chiller Trigeneration System under Different Compressor Inlet Air Temperatures. Energies 2019, 12, 4643. [Google Scholar] [CrossRef] [Green Version]
- Duffie, J.; Beckman, W. Solar Engineering of thermal Processes; Wiley: New York, NY, USA, 2013; ISBN 9780123972705. [Google Scholar]
- IDEAM. Promedio Horario de la Radiación; las Flores Barranquilla: Estac, France, 2015; p. 1.
- Kerme, E.D.; Chafidz, A.; Agboola, O.P.; Orfi, J.; Fakeeha, A.H.; Al-Fatesh, A.S. Energetic and exergetic analysis of solar-powered lithium bromide-water absorption cooling system. J. Clean. Prod. 2017, 151, 60–73. [Google Scholar] [CrossRef]
- Farahat, S.; Sarhaddi, F.; Ajam, H. Exergetic optimization of flat plate solar collectors. Renew. Energy 2009, 34, 1169–1174. [Google Scholar] [CrossRef]
- Sadaghiyani, O.K.; Boubakran, M.S.; Hassanzadeh, A. Energy and exergy analysis of parabolic trough collectors. Int. J. Heat Technol. 2018, 36, 1, 147–158. [Google Scholar] [CrossRef]
- ISO 14040:2006(en), Environmental Management—Life Cycle Assessment—Principles and Framework. Available online: https://www.iso.org/obp/ui#iso:std:iso:14040:ed-2:v1:en (accessed on 25 May 2021).
- Modi, B.; Mudgal, A.; Patel, B. Energy and Exergy Investigation of Small Capacity Single Effect Lithium Bromide Absorption Refrigeration System. Energy Procedia 2017, 109, 203–210. [Google Scholar] [CrossRef]
- Misra, R.D.; Sahoo, P.K.; Gupta, A. Thermoeconomic optimization of a LiBr/H 2O absorption chiller using structural method. J. Energy Resour. Technol. Trans. ASME 2005, 127, 119–124. [Google Scholar] [CrossRef]
- Gong, S.; Goni Boulama, K. Parametric study of an absorption refrigeration machine using advanced exergy analysis. Energy 2014, 76, 453–467. [Google Scholar] [CrossRef]
- Gong, S.; Goni Boulama, K. Advanced exergy analysis of an absorption cooling machine: Effects of the difference between the condensation and absorption temperatures. Int. J. Refrig. 2015, 59, 224–234. [Google Scholar] [CrossRef]



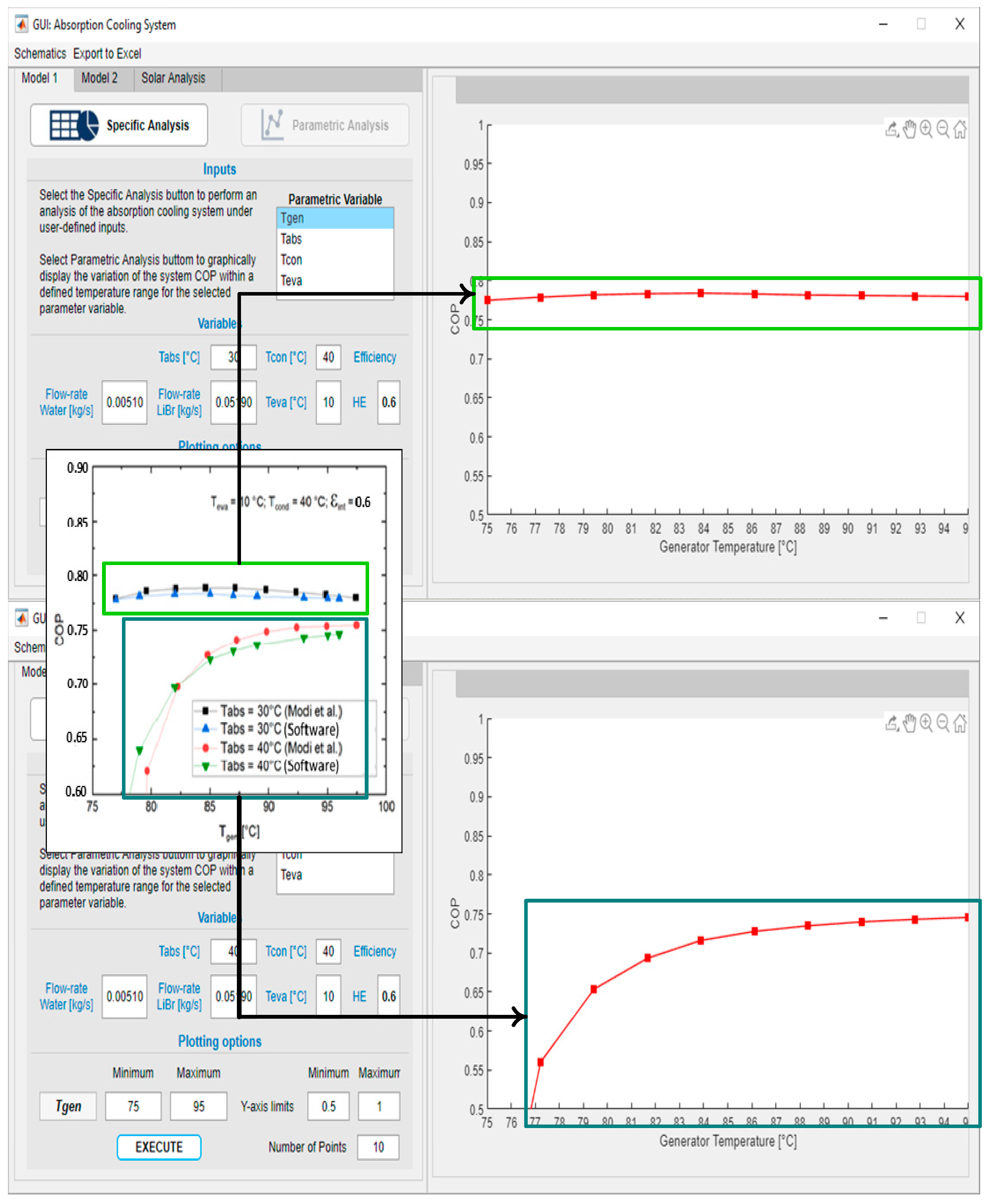

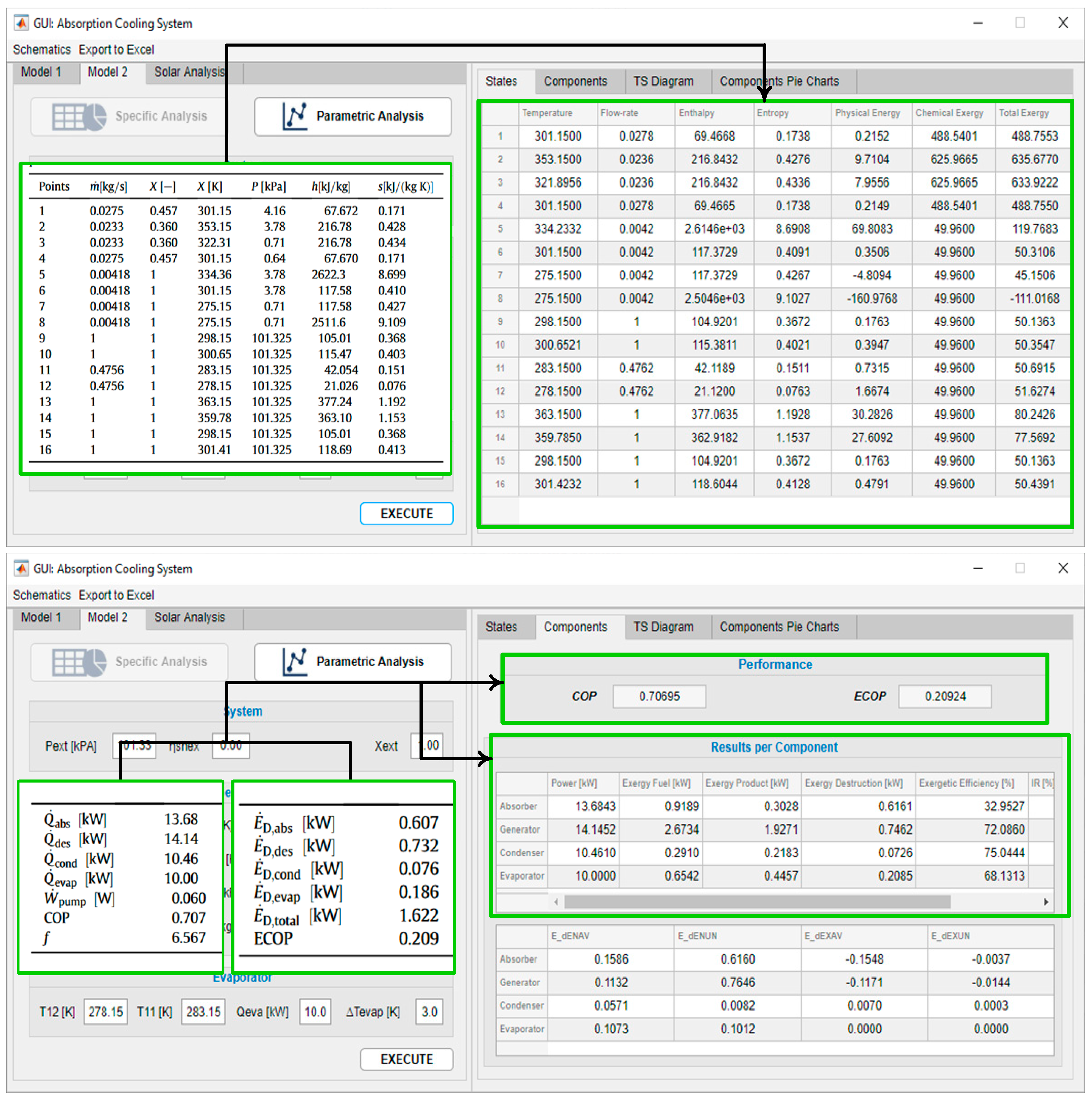
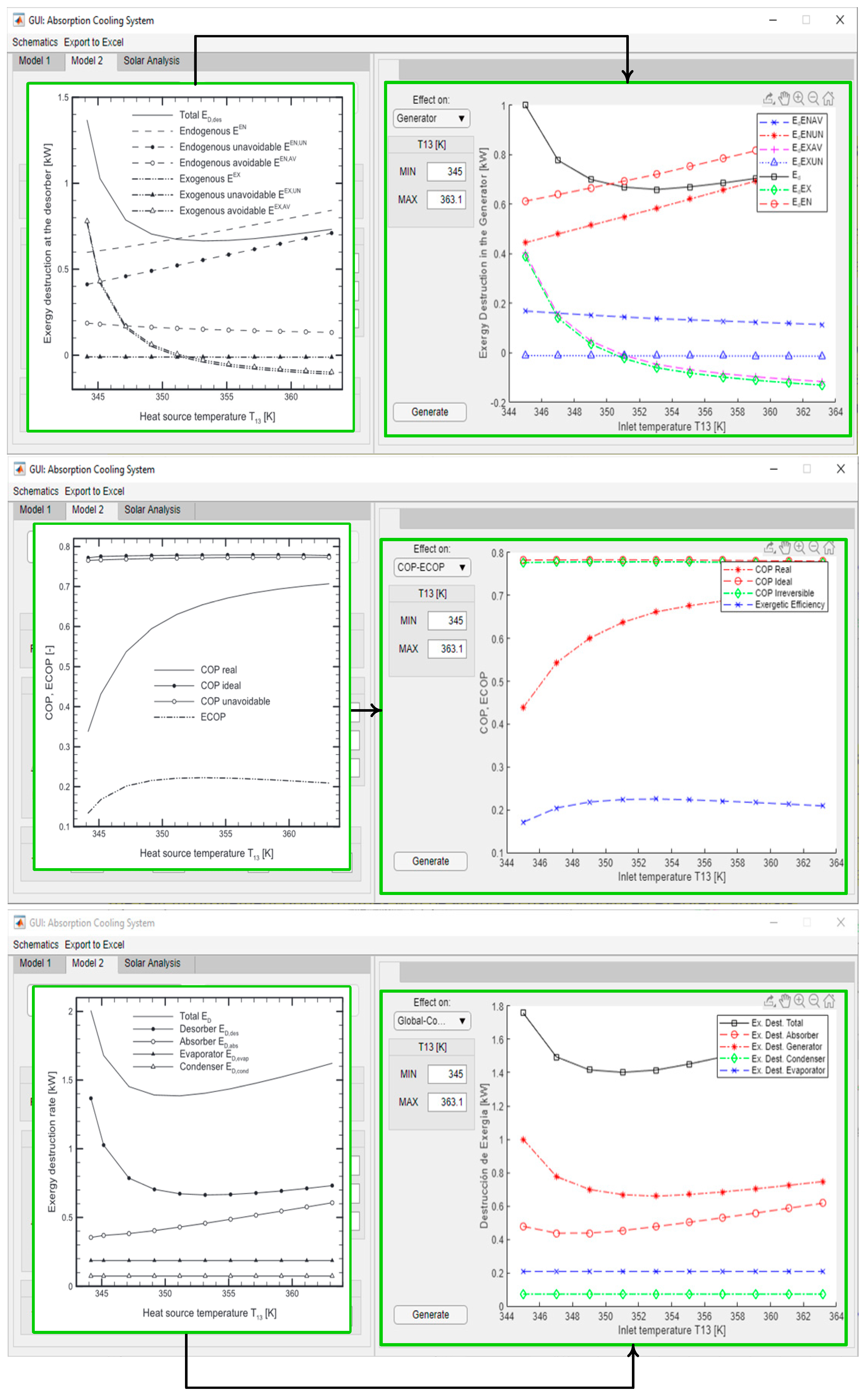
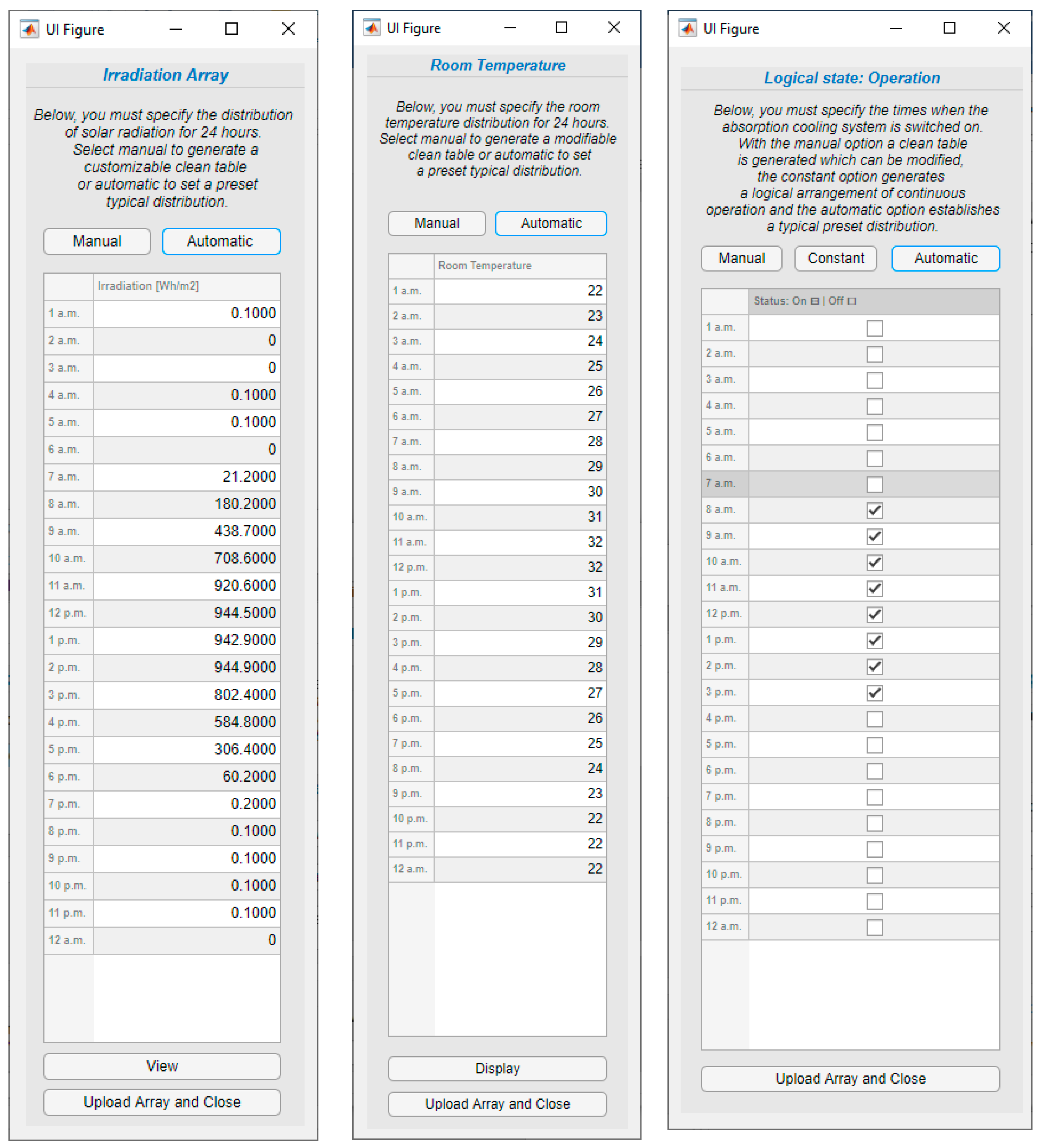
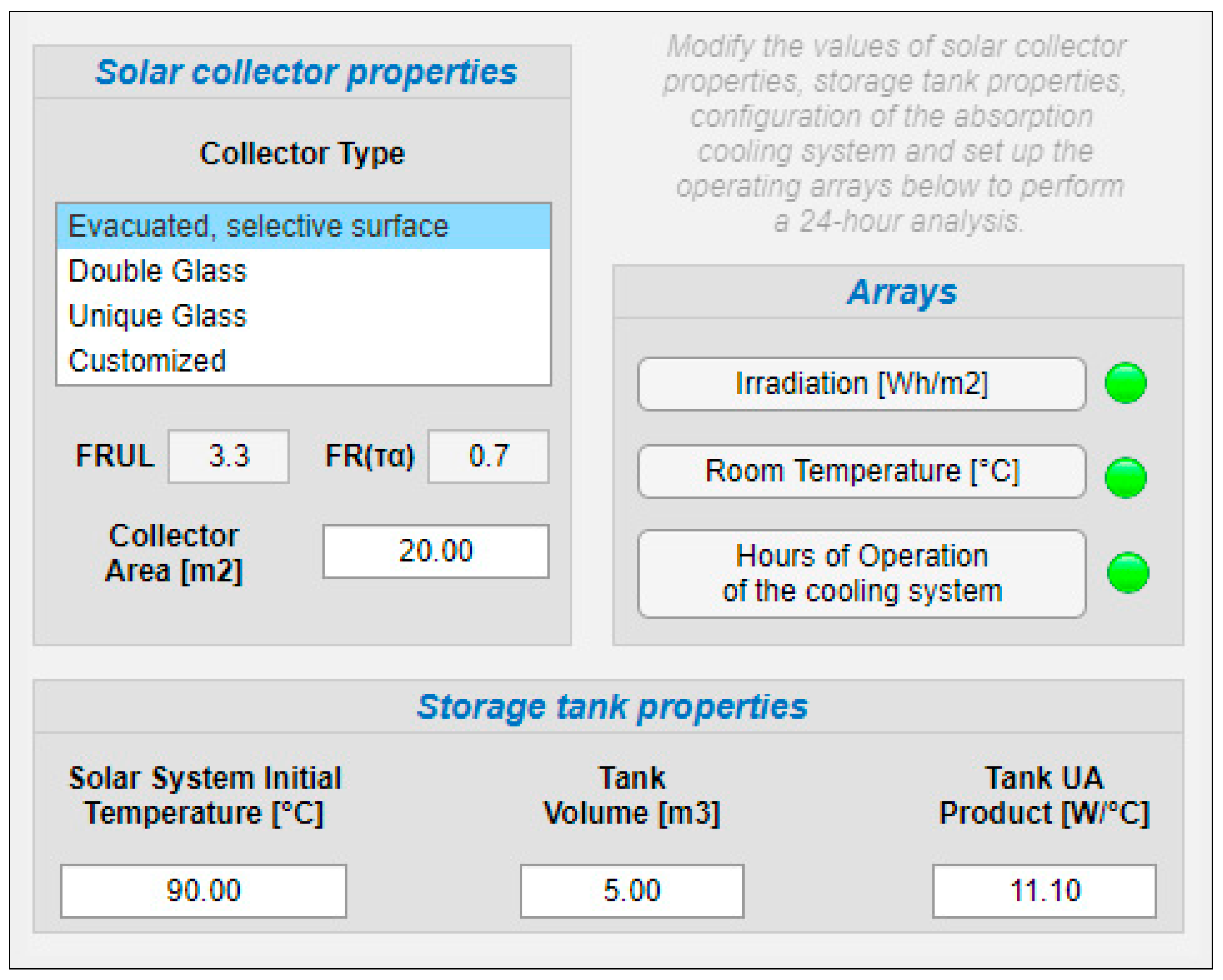
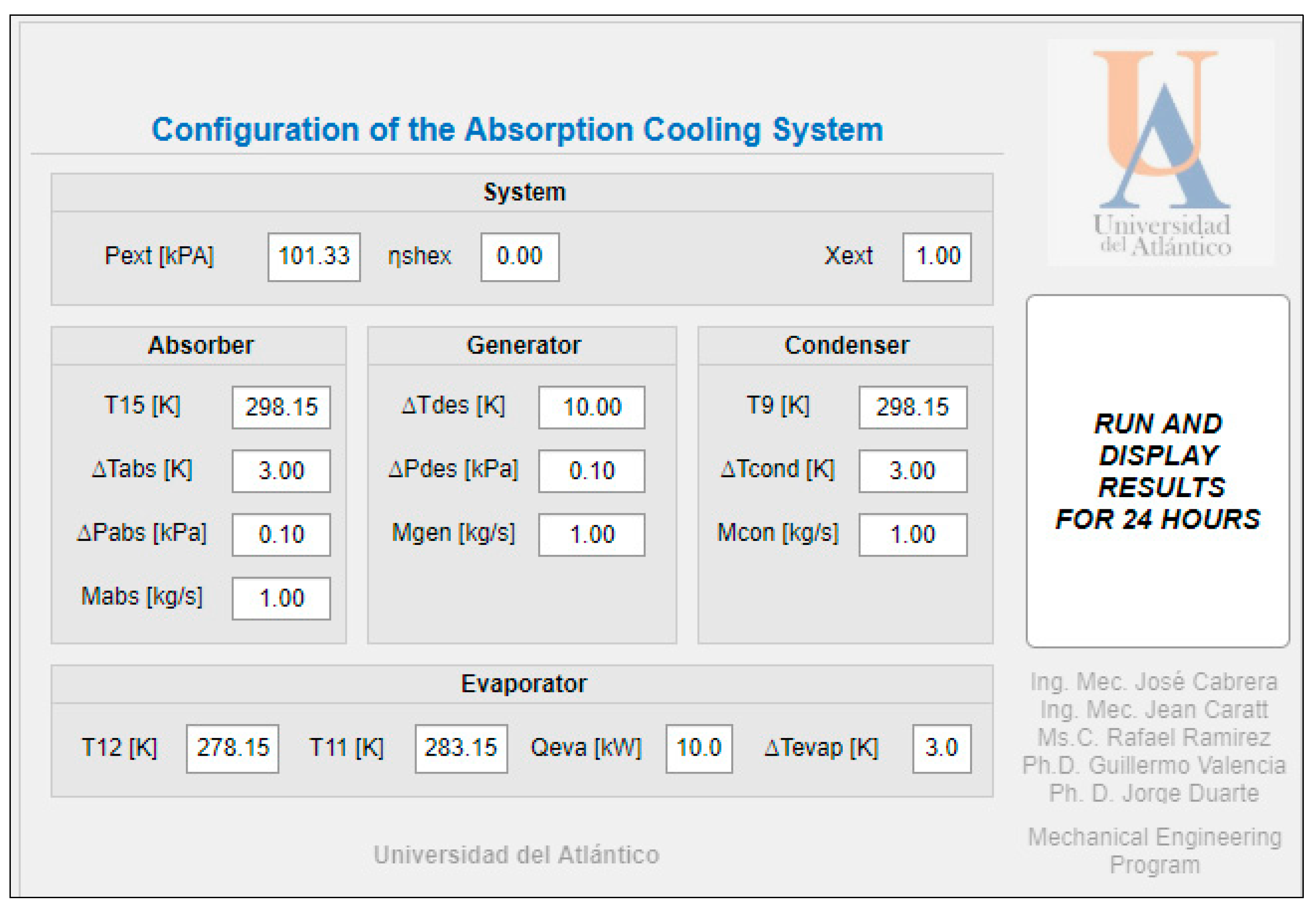

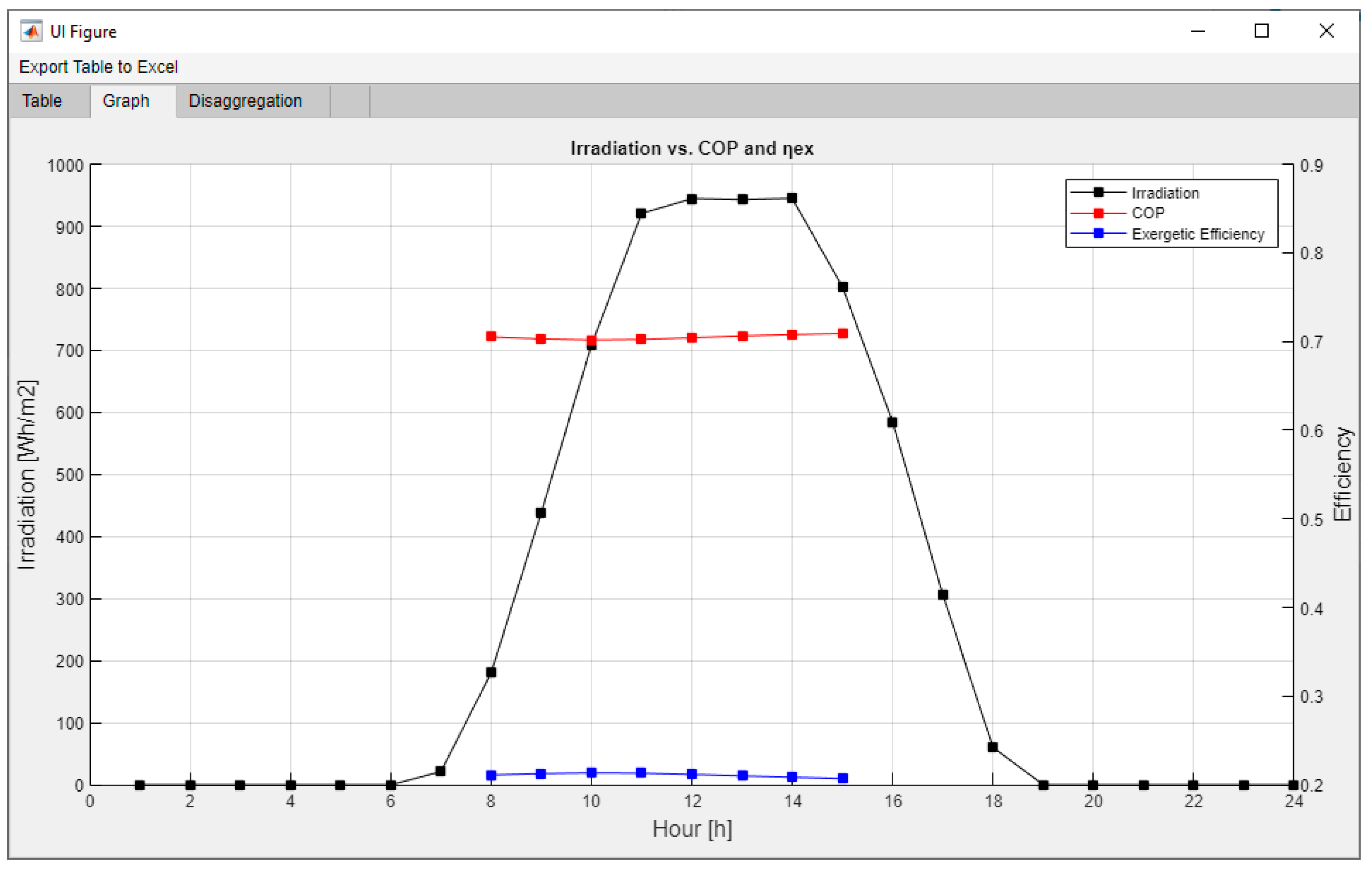

| Property | Input Variable Range | Reference | |
|---|---|---|---|
| Enthalpy, h (kJ/kg) | 45 < X < 65% | 40 < T < 190 °C | [31] |
| Entropy, s (kJ/kg·K) | 45 < X < 65% | 40 < T < 190 °C | [31] |
| Viscosity, μ (Pa·s) | 45 < X < 65% | 40 < T < 190 °C | [32] |
| Concentration, X (%) | 0.5 < P < 10 kPa | 40 < T < 190 °C | [33] |
| Density, ρ (kg/m3) | 45 < X < 65% | 40 < T < 190 °C | [32] |
| Specific heat, cp (kJ/kg·K) | 45 < X < 65% | 40 < T < 190 °C | [32] |
| Conductivity, k (W/m·K) | 30 < X < 65% | 40 < T < 190 °C | [32] |
| Component | |||
|---|---|---|---|
| Absorber | |||
| Generator | |||
| Condenser | |||
| Evaporator |
| States | (kg/s) | (K) | |||||||
|---|---|---|---|---|---|---|---|---|---|
| Gong 2014 | Adopted Property | %Error | Gong 2014 | Adopted Property | %Error | Gong 2014 | Adopted Property | %Error | |
| 1 | 0.02750 | 0.02783 | 1.19% | 0.457 | 0.455 | 0.45% | 301.15 | 301.15 | 0.00% |
| 2 | 0.02330 | 0.02364 | 1.45% | 0.360 | 0.358 | 0.45% | 353.15 | 353.15 | 0.00% |
| 3 | 0.02330 | 0.02364 | 1.45% | 0.360 | 0.358 | 0.45% | 322.31 | 321.90 | 0.13% |
| 4 | 0.02750 | 0.02783 | 1.19% | 0.457 | 0.455 | 0.45% | 301.15 | 301.15 | 0.00% |
| 5 | 0.00418 | 0.00419 | 0.22% | 1 | 1 | 0.00% | 334.36 | 334.23 | 0.04% |
| 6 | 0.00418 | 0.00419 | 0.22% | 1 | 1 | 0.00% | 301.15 | 301.15 | 0.00% |
| 7 | 0.00418 | 0.00419 | 0.22% | 1 | 1 | 0.00% | 275.15 | 275.15 | 0.00% |
| 8 | 0.00418 | 0.00419 | 0.22% | 1 | 1 | 0.00% | 275.15 | 275.15 | 0.00% |
| 9 | 1 | 1 | 0.00% | 1 | 1 | 0.00% | 298.15 | 298.15 | 0.00% |
| 10 | 1 | 1 | 0,00% | 1 | 1 | 0.00% | 300.65 | 300.65 | 0.00% |
| 11 | 0.47560 | 0.47621 | 0.13% | 1 | 1 | 0.00% | 283.15 | 283.15 | 0.00% |
| 12 | 0.47560 | 0.47621 | 0.13% | 1 | 1 | 0.00% | 278.15 | 278.15 | 0.00% |
| 13 | 1 | 1 | 0.00% | 1 | 1 | 0.00% | 363.15 | 363.15 | 0.00% |
| 14 | 1 | 1 | 0.00% | 1 | 1 | 0.00% | 359.78 | 359.79 | 0.00% |
| 15 | 1 | 1 | 0.00% | 1 | 1 | 0.00% | 298.15 | 298.15 | 0.00% |
| 16 | 1 | 1 | 0.00% | 1 | 1 | 0.00% | 301.41 | 301.42 | 0.00% |
| States | (kPa) | (kJ/kg) | (kJ/kg·K) | ||||||
|---|---|---|---|---|---|---|---|---|---|
| Gong 2014 | Adopted Property | %Error | Gong 2014 | Adopted Property | %Error | Gong 2014 | Adopted Property | %Error | |
| 1 | 4.16 | 4.16 | 0.03% | 67.672 | 69.47 | 2.65% | 0.171 | 0.174 | 1.61% |
| 2 | 3.78 | 3.78 | 0.08% | 216.78 | 216.84 | 0.03% | 0.428 | 0.428 | 0.10% |
| 3 | 0.71 | 0.71 | 0.56% | 216.78 | 216.84 | 0.03% | 0.434 | 0.434 | 0.10% |
| 4 | 0.64 | 0.64 | 0.72% | 67.67 | 69.47 | 2.65% | 0.171 | 0.174 | 1.61% |
| 5 | 3.78 | 3.78 | 0.08% | 2622.3 | 2614.61 | 0.29% | 8.699 | 8.691 | 0.09% |
| 6 | 3.78 | 3.78 | 0.08% | 117.58 | 117.37 | 0.18% | 0.41 | 0.409 | 0.22% |
| 7 | 0.71 | 0.71 | 0.56% | 117.58 | 117.37 | 0.18% | 0.427 | 0.427 | 0.07% |
| 8 | 0.71 | 0.71 | 0.56% | 2511.6 | 2504.56 | 0.28% | 9.109 | 9.103 | 0.07% |
| 9 | 101.325 | 101.325 | 0.00% | 105.01 | 104.92 | 0.09% | 0.368 | 0.367 | 0.22% |
| 10 | 101.325 | 101.325 | 0.00% | 115.47 | 115.38 | 0.08% | 0.403 | 0.402 | 0.21% |
| 11 | 101.325 | 101.325 | 0.00% | 42.054 | 42.12 | 0.15% | 0.151 | 0.151 | 0.05% |
| 12 | 101.325 | 101.325 | 0.00% | 21.026 | 21.12 | 0.45% | 0.076 | 0.076 | 0.33% |
| 13 | 101.325 | 101.325 | 0.00% | 377.24 | 377.06 | 0.05% | 1.192 | 1.193 | 0.07% |
| 14 | 101.325 | 101.325 | 0.00% | 363.1 | 362.92 | 0.05% | 1.153 | 1.154 | 0.06% |
| 15 | 101.325 | 101.325 | 0.00% | 105.01 | 104.92 | 0.09% | 0.368 | 0.367 | 0.22% |
| 16 | 101.325 | 101.325 | 0.00% | 118.69 | 118.60 | 0.07% | 0.413 | 0.413 | 0.04% |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Cabrera César, J.; Caratt Ortiz, J.; Ochoa, G.V.; Restrepo, R.R.; Alvarez, J.R.N. A New Computational Tool for the Development of Advanced Exergy Analysis and LCA on Single Effect LiBr–H2O Solar Absorption Refrigeration System. Lubricants 2021, 9, 76. https://doi.org/10.3390/lubricants9080076
Cabrera César J, Caratt Ortiz J, Ochoa GV, Restrepo RR, Alvarez JRN. A New Computational Tool for the Development of Advanced Exergy Analysis and LCA on Single Effect LiBr–H2O Solar Absorption Refrigeration System. Lubricants. 2021; 9(8):76. https://doi.org/10.3390/lubricants9080076
Chicago/Turabian StyleCabrera César, José, Jean Caratt Ortiz, Guillermo Valencia Ochoa, Rafael Ramírez Restrepo, and José R. Nuñez Alvarez. 2021. "A New Computational Tool for the Development of Advanced Exergy Analysis and LCA on Single Effect LiBr–H2O Solar Absorption Refrigeration System" Lubricants 9, no. 8: 76. https://doi.org/10.3390/lubricants9080076
APA StyleCabrera César, J., Caratt Ortiz, J., Ochoa, G. V., Restrepo, R. R., & Alvarez, J. R. N. (2021). A New Computational Tool for the Development of Advanced Exergy Analysis and LCA on Single Effect LiBr–H2O Solar Absorption Refrigeration System. Lubricants, 9(8), 76. https://doi.org/10.3390/lubricants9080076







