Nanotribological Performance Factors for Aqueous Suspensions of Oxide Nanoparticles and Their Relation to Macroscale Lubricity
Abstract
1. Introduction
2. Materials
2.1. Nanoparticles
2.2. Substrates
3. Experimental Method
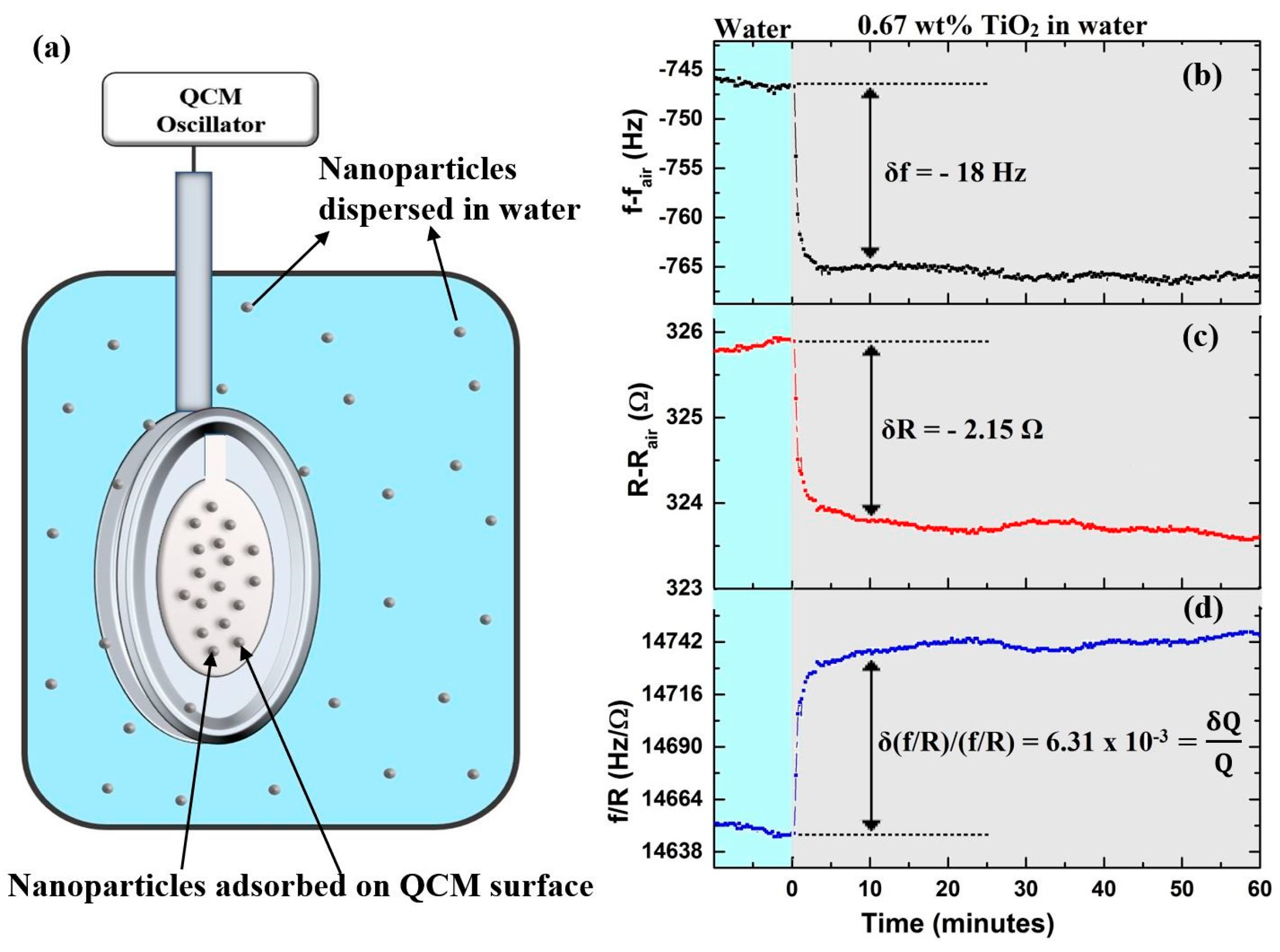
3.1. Quartz Crystal Microbalance Data Analysis
3.2. Macroscale Friction Measurements
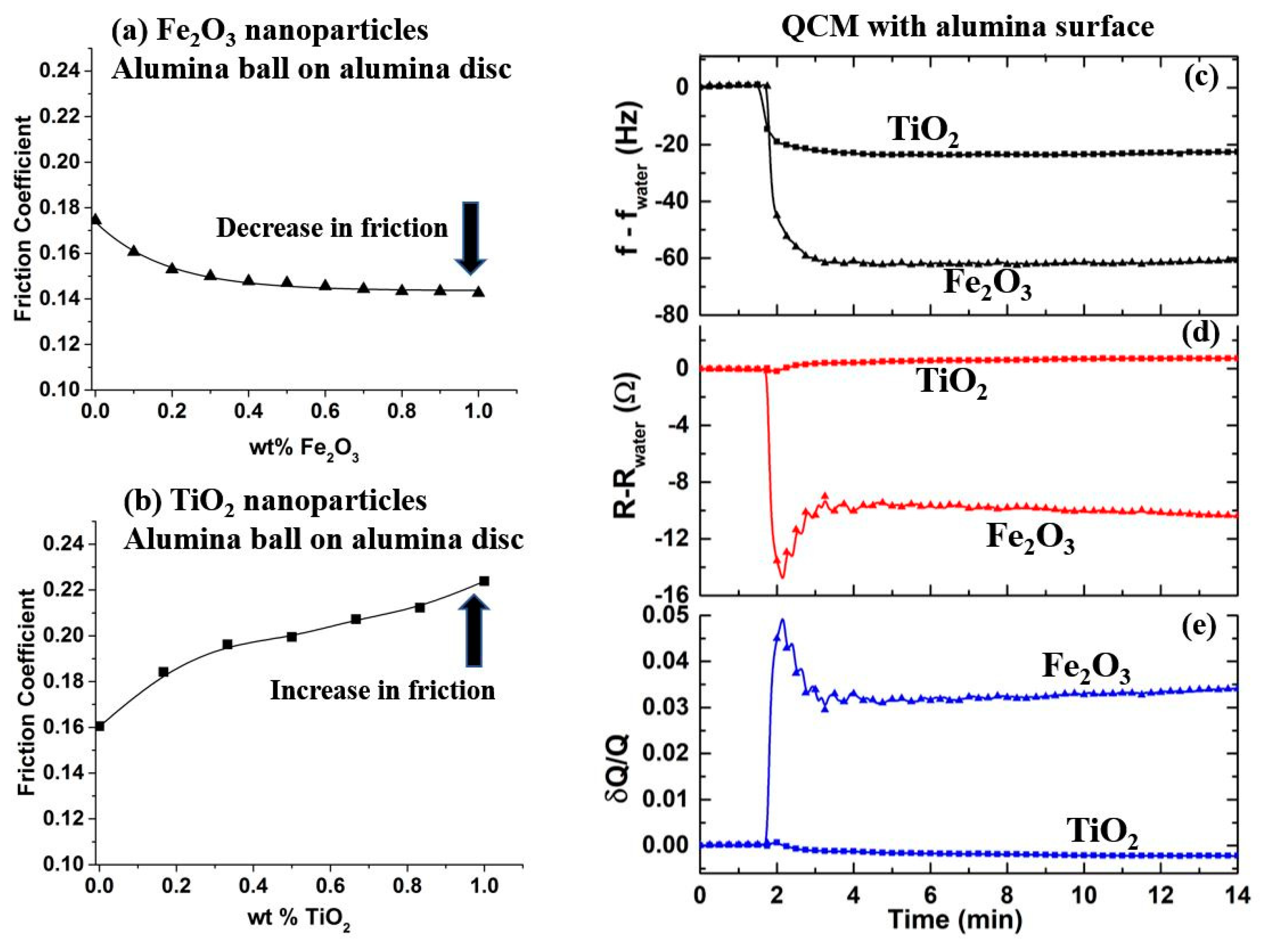
4. Results
4.1. Examples of QCM Response Types Upon Introduction of NP’s
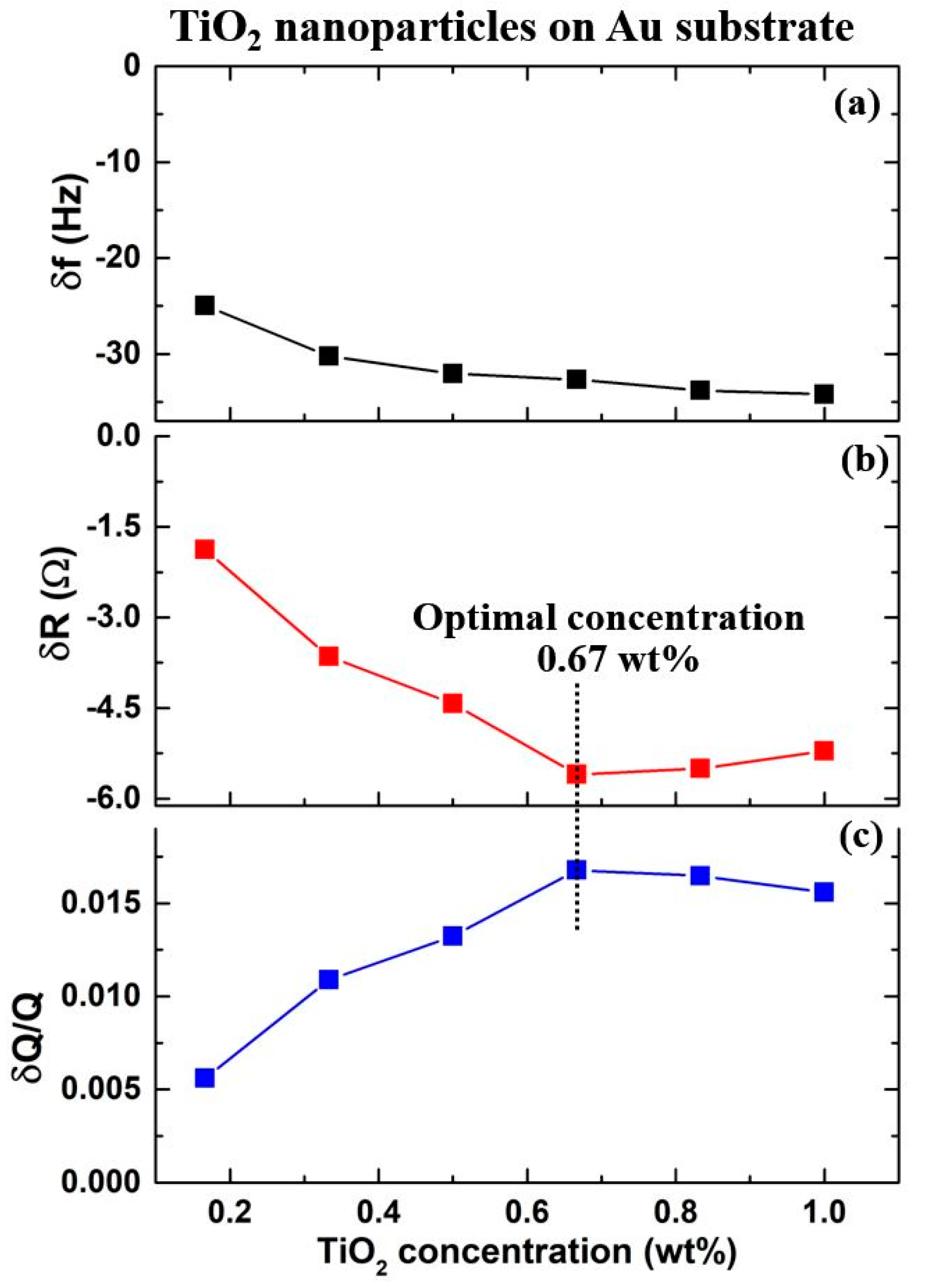
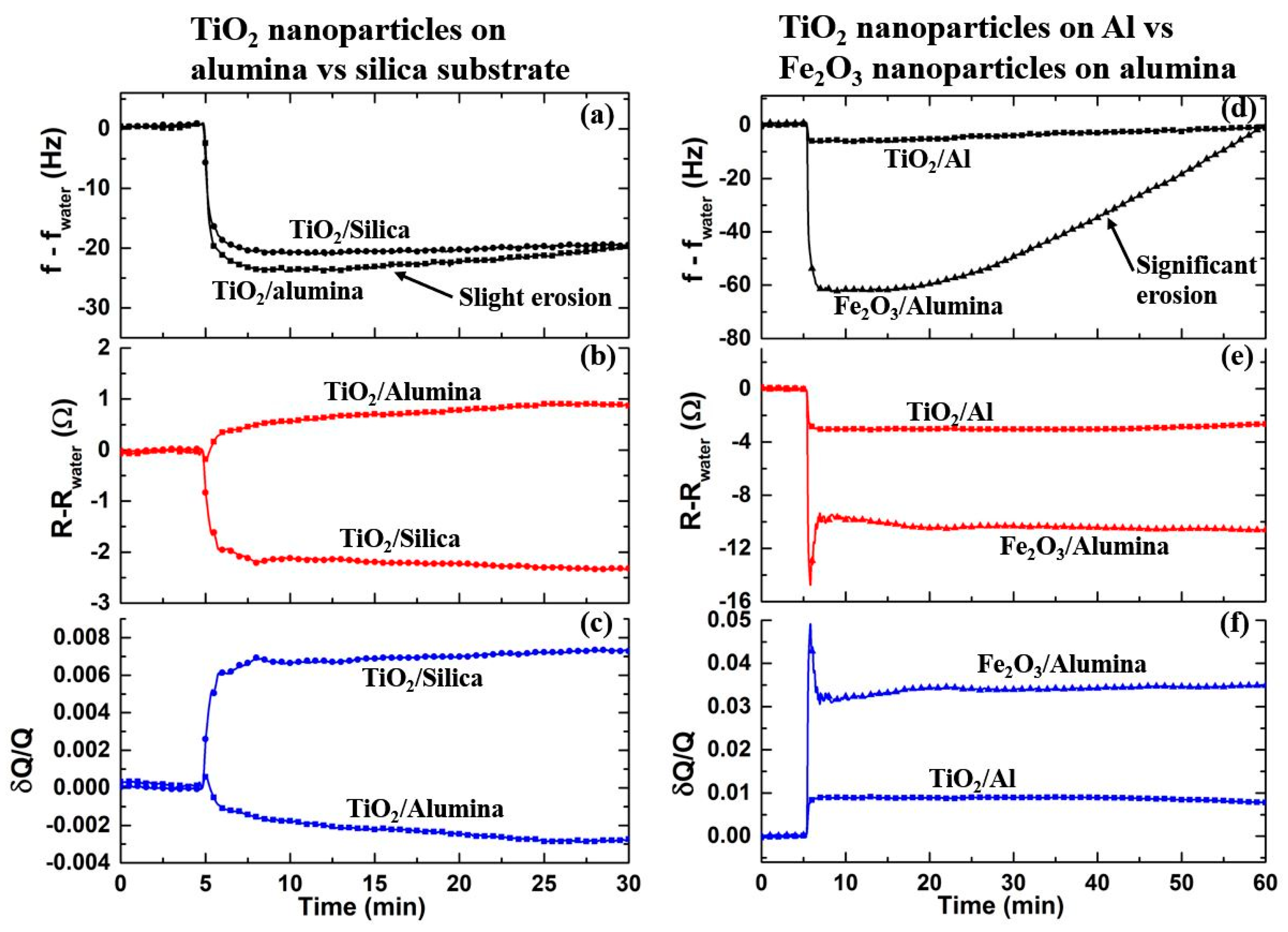
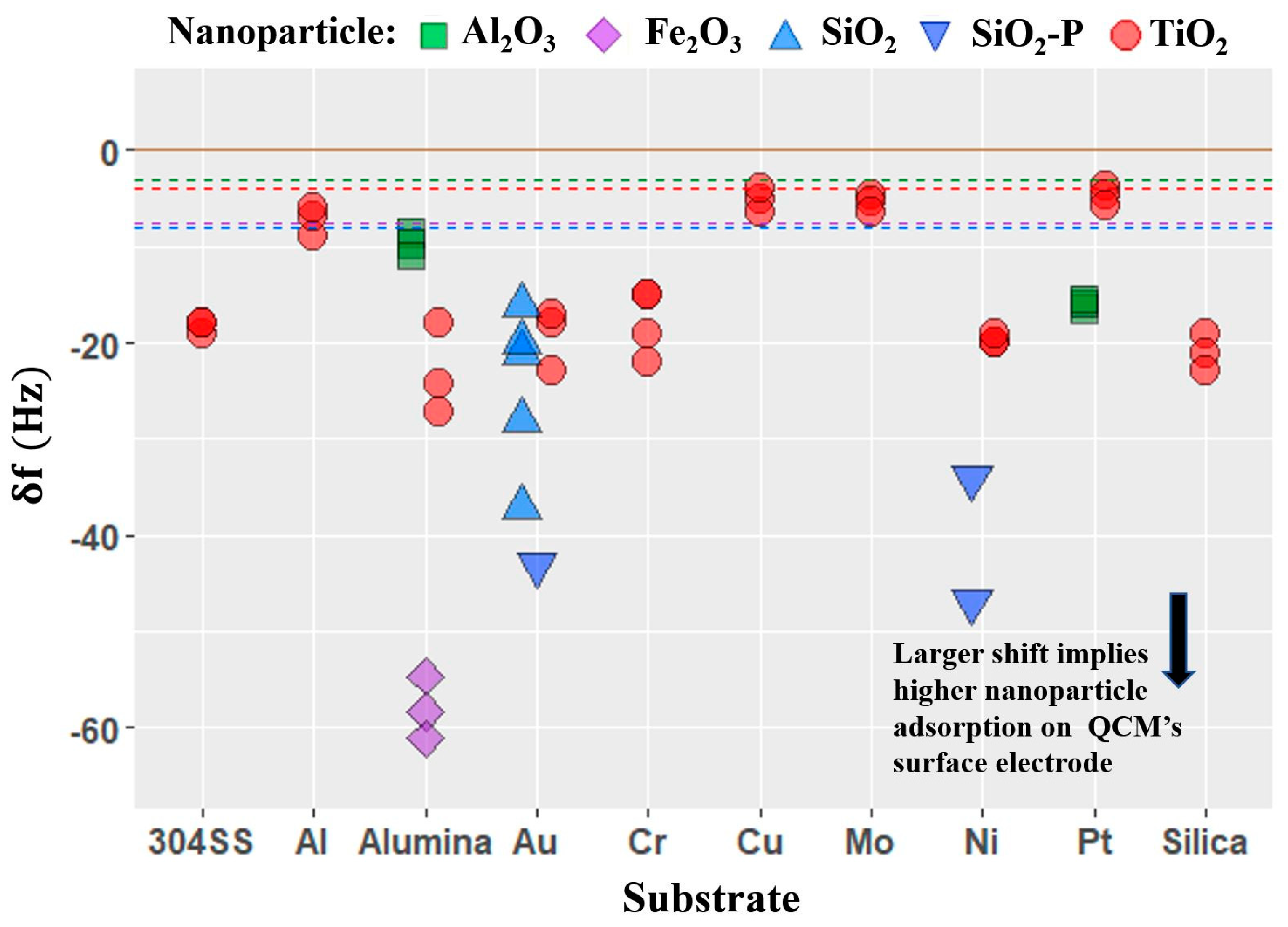
4.2. Frequency Response Upon Introduction of Nanoparticles
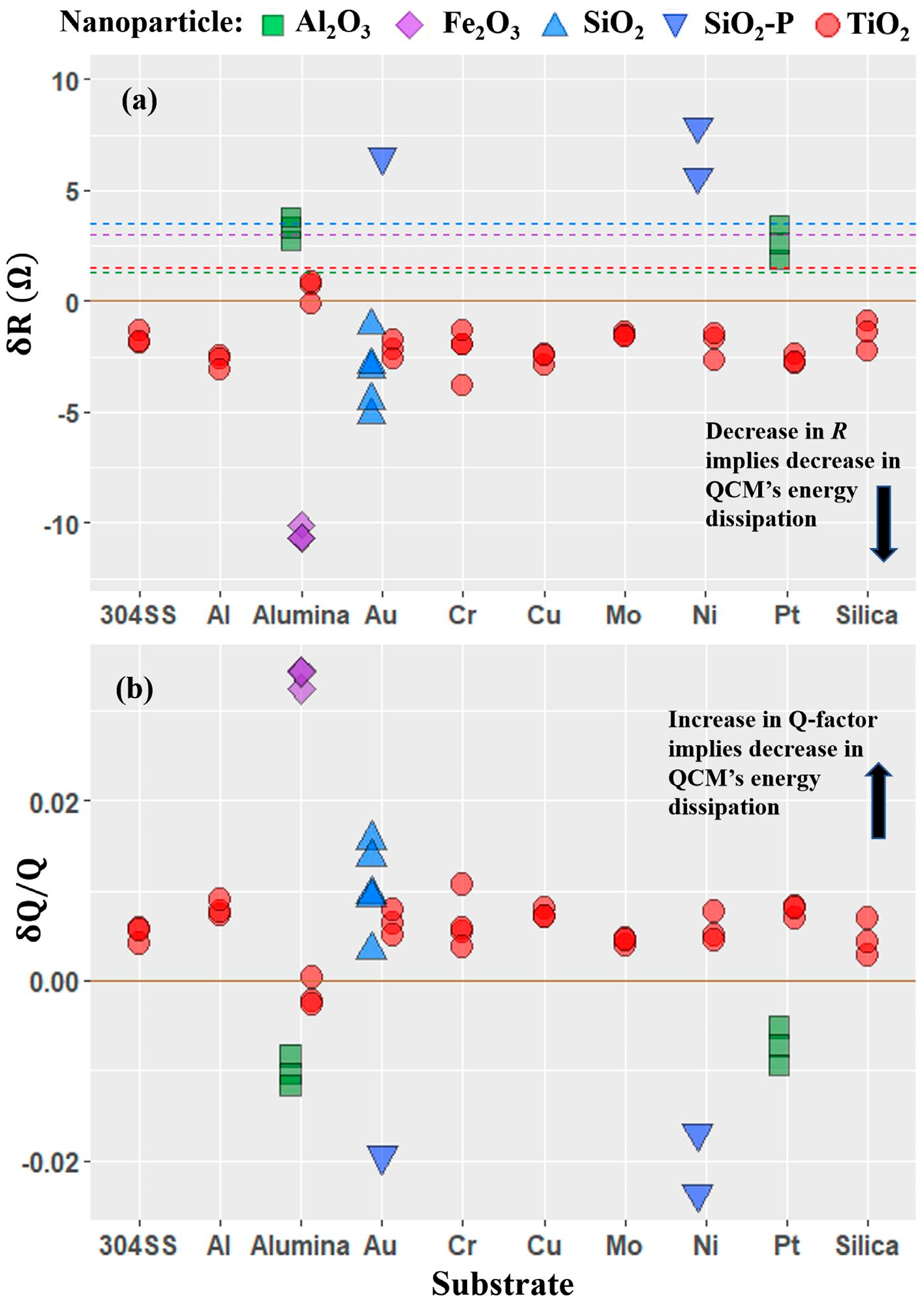
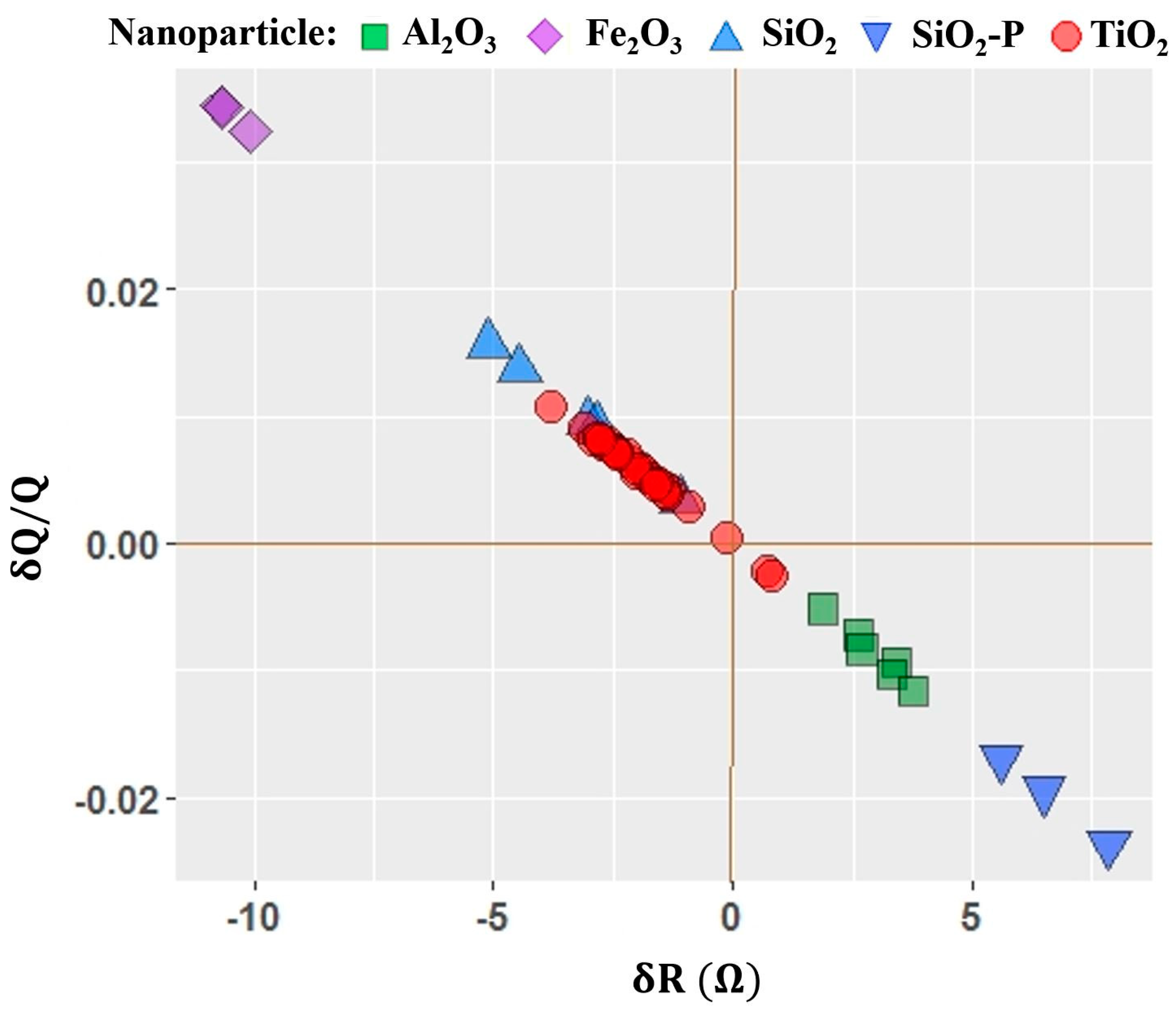
4.3. Motional Resistance and Oscillator Dissipation Response to Introduction of Nanoparticles
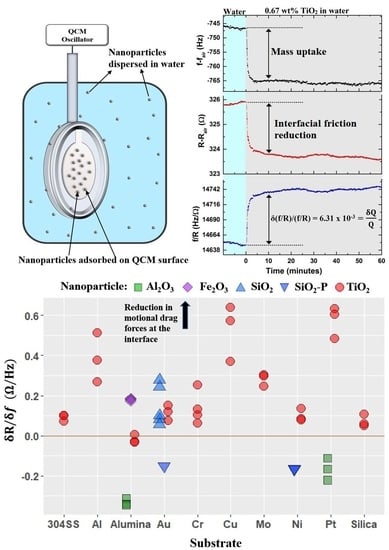
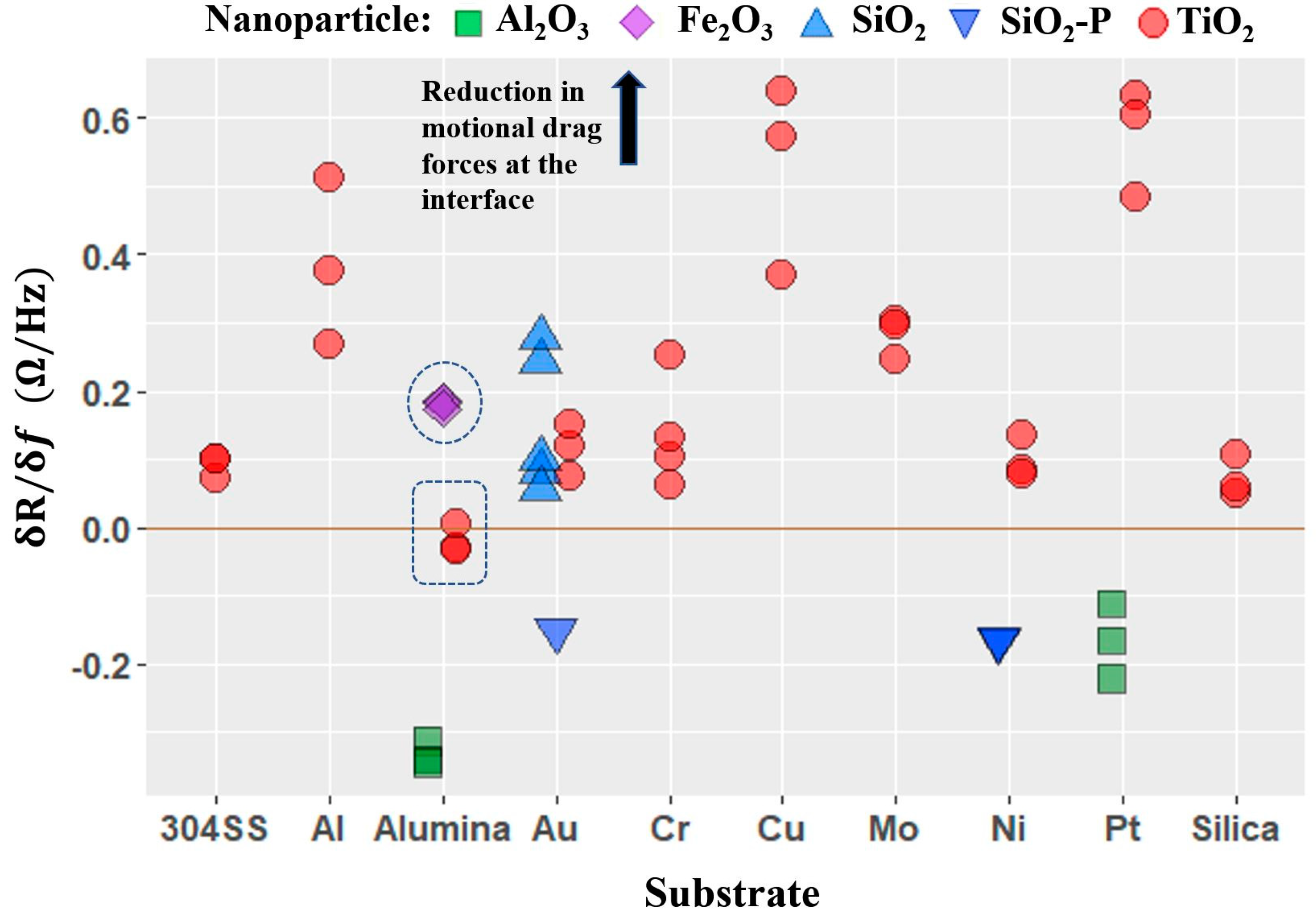
4.4. Nanotribological Performance Factor δR/δf
4.5. Macroscale Friction of Selected Systems and the Relation to QCM Measurements
5. Discussion
6. Conclusions
- Four QCM measurement parameters δf, δR, δQ/Q, and δR/δf were considered. Among these, the parameter δR/δf was identified to be the performance factor that characterized enhancement of lubricity upon introduction of nanoparticles the best.
- The performance factor δR/δf that describes the nanotribological effect measured with QCM was found to be applicable to a wider and more diverse set of nanoparticle–substrate combinations than had previously been reported. The extent of the regime of lubrication over which the performance factor correlates with macroscale lubricity is yet to be fully established.
- Variations in the performance factor from one nanoparticle to another are observed to be far greater than the variation from one substrate to another, consistent with nanoparticle properties playing a larger role than the substrate physical properties in determining the frictional drag force levels.
- The performance factor was not found to have an obvious correlation with any of the material properties considered. A comprehensive statistical analysis of the data would be required to possibly identify candidate material intrinsic properties that determine the performance of a system as lubricant.
Author Contributions
Funding
Conflicts of Interest
References
- Singh, R.; Kukrety, A.; Chouhan, A.; Atray, N.; Ray, S. Recent Progress in the Preparation of Eco-friendly Lubricant and Fuel Additives through Organic Transformations of Biomaterials. Mini-Rev. Org. Chem. 2017, 14, 44–55. [Google Scholar] [CrossRef]
- Kim, H.-J.; Kim, D.-E. Water Lubrication of Stainless Steel using Reduced Graphene Oxide Coating. Sci. Rep. 2015, 5, 17034. [Google Scholar] [CrossRef] [PubMed]
- Fan, M.; Du, X.; Ma, L.; Wen, P.; Zhang, S.; Dong, R.; Sun, W.; Yang, D.; Zhou, F.; Liu, W. In situ preparation of multifunctional additives in water. Tribol. Int. 2019, 130, 317–323. [Google Scholar] [CrossRef]
- Yu, X.; Zhou, J.; Jiang, Z. Developments and Possibilities for Nanoparticles in Water-Based Lubrication During Metal Processing. Rev. Nanosci. Nanotechnol. 2016, 5, 136–163. [Google Scholar] [CrossRef]
- Chen, Y.; Renner, P.; Liang, H. Dispersion of Nanoparticles in Lubricating Oil: A Critical Review. Lubricants 2019, 7, 7. [Google Scholar] [CrossRef]
- Dai, W.; Kheireddin, B.; Gao, H.; Liang, H. Roles of nanoparticles in oil lubrication. Tribol. Int. 2016, 102, 88–98. [Google Scholar] [CrossRef]
- Liu, Z.; Leininger, D.; Koolivand, A.; Smirnov, A.I.; Shenderova, O.; Brenner, D.W.; Krim, J. Tribological properties of nanodiamonds in aqueous suspensions: Effect of the surface charge. RSC Adv. 2015, 5, 78933–78940. [Google Scholar] [CrossRef]
- Hase, A.; Mishina, H. Wear elements generated in the elementary process of wear. Tribol. Int. 2009, 42, 1684–1690. [Google Scholar] [CrossRef]
- Curtis, C.K.; Marek, A.; Smirnov, A.I.; Krim, J. A comparative study of the nanoscale and macroscale tribological attributes of alumina and stainless steel surfaces immersed in aqueous suspensions of positively or negatively charged nanodiamonds. Beilstein J. Nanotechnol. 2017, 8, 2045–2059. [Google Scholar] [CrossRef]
- Vollath, D.; Szabó, D.V.; Hauβelt, J. Synthesis and properties of ceramic nanoparticles and nanocomposites. J. Eur. Ceram. Soc. 1997, 17, 1317–1324. [Google Scholar] [CrossRef]
- Thomas, S.; Harshita, B.S.P.; Mishra, P.; Talegaonkar, S. Ceramic Nanoparticles: Fabrication Methods and Applications in Drug Delivery. Curr. Pharm. Des. 2015, 21, 6165–6188. [Google Scholar] [CrossRef] [PubMed]
- Moreno-Vega, A.-I.; Gómez-Quintero, T.; Nuñez-Anita, R.-E.; Acosta-Torres, L.-S.; Castaño, V. Polymeric and Ceramic Nanoparticles in Biomedical Applications. J. Nanotechnol. 2012, 2012, 936041. [Google Scholar] [CrossRef]
- Ma, Y.; Bao, J.; Zhang, Y.; Li, Z.; Zhou, X.; Wan, C.; Huang, L.; Zhao, Y.; Han, G.; Xue, T. Mammalian Near-Infrared Image Vision through Injectable and Self-Powered Retinal Nanoantennae. Cell 2019, 177, 243–255. [Google Scholar] [CrossRef] [PubMed]
- Cohen, A.E. Control of Nanoparticles with Arbitrary Two-Dimensional Force Fields. Phys. Rev. Lett. 2005, 94, 118102. [Google Scholar] [CrossRef] [PubMed]
- Acharya, B.; Avva, K.; Thapa, B.; Pardue, T.; Krim, J. Synergistic Effect of Nanodiamond and Phosphate Ester Anti-Wear Additive Blends. Lubricants 2018, 6, 56. [Google Scholar] [CrossRef]
- Pardue, T.N.; Acharya, B.; Curtis, C.K.; Krim, J. A Tribological Study of γ-Fe2O3 Nanoparticles in Aqueous Suspension. Tribol. Lett. 2018, 66, 130. [Google Scholar] [CrossRef]
- Acharya, B.; Chestnut, M.; Marek, A.; Smirnov, A.I.; Krim, J. A Combined QCM and AFM Study Exploring the Nanoscale Lubrication Mechanism of Silica Nanoparticles in Aqueous Suspension. Tribol. Lett. 2017, 65, 115. [Google Scholar] [CrossRef]
- Acharya, B.; Seed, C.M.; Brenner, D.W.; Smirnov, A.I.; Krim, J. Tuning friction at material-nanoparticle-liquid interfaces with an external electric field. arXiv 2019, arXiv:1901.10512 v1. [Google Scholar]
- Acharya, B.; Sidheswaran, M.A.; Yungk, R.; Krim, J. Quartz crystal microbalance apparatus for study of viscous liquids at high temperatures. Rev. Sci. Instrum. 2017, 88, 025112. [Google Scholar] [CrossRef] [PubMed]
- Mizele, J.; Dandurand, J.L.; Schott, J. Determination of the surface energy of amorphous silica from solubility measurements in micropores. Surf. Sci. 1985, 162, 830–837. [Google Scholar] [CrossRef]
- Pagonis, V.; Ankjærgaard, C.; Murray, A.S.; Chen, R. Optically stimulated exoelectron emission processes in quartz: Comparison of experiment and theory. J. Lumin. 2009, 129, 1003–1009. [Google Scholar] [CrossRef]
- Vitos, L.; Ruban, A.V.; Skriver, H.L.; Kollár, J. The surface energy of metals. Surf. Sci. 1998, 411, 186–202. [Google Scholar] [CrossRef]
- Yaws, C.L. Yaws’ Handbook of Properties of the Chemical Elements; Knovel: New York, NY, USA, 2011. [Google Scholar]
- Lide, D.R. CRC Handbook of Chemistry and Physics, 2015–2016, 96th ed.; CRC Press: Boca Raton, FL, USA, 2014. [Google Scholar]
- Skriver, H.L.; Rosengaard, N.M. Surface-Energy and Work Function of Elemental Metals. Phys. Rev. B 1992, 46, 7157–7168. [Google Scholar] [CrossRef]
- Brinkmann, N.R.; Tschumper, G.S.; Schaefer, H.F. Electron affinities of the oxides of aluminum, silicon, phosphorus, sulfur, and chlorine. J. Chem. Phys. 1999, 110, 6240–6245. [Google Scholar] [CrossRef]
- Bratsch, S.G. Standard Electrode Potentials and Temperature Coefficients in Water at 298.15 K. J. Phys. Chem. Ref. Data 1989, 18, 1–21. [Google Scholar] [CrossRef]
- Samsonov, G. Handbook of the Physicochemical Properties of the Elements. In Handbook of the Physicochemical Properties of the Elements; Springer: New York, NY, USA, 1968; pp. 387–446. [Google Scholar]
- Chase, M.W., Jr. NIST-JANAF Themochemical Tables, 4th ed.; American Institute of Physics: College Park, MD, USA, 1998. [Google Scholar]
- Knovel Critical Tables (2nd Edition)—Knovel. Available online: http://app.knovel.com/web/toc.v/cid:kpKCTE000X/viewerType:toc/root_slug:knovel-critical-tables/url_slug:knovel-critical-tables (accessed on 1 November 2017).
- Stanford Research Systems. QCM 100 Quartz Crystal Microbalance Analog Controller—QCM 25 Crystal Oscillator; Stanford Research Systems, Inc.: Sunnyvale, CA, USA, 2002. [Google Scholar]
- Kanazawa, K.K.; Gordon, J.G. Frequency of a quartz microbalance in contact with liquid. Anal. Chem. 1985, 57, 1770–1771. [Google Scholar] [CrossRef]
- Alassi, A.; Benammar, M.; Brett, D. Quartz Crystal Microbalance Electronic Interfacing Systems: A Review. Sensors 2017, 17, 2799. [Google Scholar] [CrossRef]
- Mishra, P.C.; Mukherjee, S.; Nayak, S.K.; Panda, A. A brief review on viscosity of nanofluids. Int. Nano Lett. 2014, 4, 109–120. [Google Scholar] [CrossRef]
- Breki, A.; Nosonovsky, M. Einstein’s Viscosity Equation for Nanolubricated Friction. Langmuir 2018, 34, 12968–12973. [Google Scholar] [CrossRef]
- Manikandan, S.; Karthikeyan, N.; Rajan, K.S. Viscosity of Fe2O3 nanoparticles dispersion in water and ethylene glycol-water mixture (nanofluids). Int. J. Nanopart. 2013, 6, 10–27. [Google Scholar] [CrossRef]
- Sauerbrey, G. Verwendung von Schwingquarzen zur Wägung dünner Schichten und zur Mikrowägung. Zeitschrift für Physik 1959, 155, 206–222. [Google Scholar] [CrossRef]
- Ghaednia, H.; Babaei, H.; Jackson, R.L.; Bozack, M.J.; Khodadadi, J.M. The effect of nanoparticles on thin film elasto-hydrodynamic lubrication. Appl. Phys. Lett. 2013, 103, 263111. [Google Scholar] [CrossRef]
- Krim, J.; Widom, A. Damping of a crystal oscillator by an adsorbed monolayer and its relation to interfacial viscosity. Phys. Rev. B 1988, 38, 12184. [Google Scholar] [CrossRef]
- Kalil, T.; Wadia, C. Materials Genome Initiative for Global Competitiveness. Available online: https://www.mgi.gov/sites/default/files/documents/materials_genome_initiative-final.pdf (accessed on 25 April 2019).
- White, A. The Materials Genome Initiative: One year on. MRS Bull. 2012, 37, 715–716. [Google Scholar] [CrossRef]
| Nanoparticle | Average Diameter (nm) | pH | Zeta Potential (mV) | Concentrations Used in QCM Measurements (wt %) | Density (g/cm3) |
|---|---|---|---|---|---|
| Al2O3 | 30 | 4.5 | 60.9 | 0.17–1.00 | 3.95 |
| Fe2O3 | 5 | 7.0 * | 0.0 * | 0.2–1.00 | 5.24 |
| TiO2 | 40 | 7.7 | −32.7 | 0.33–1.00 | 4.23 |
| SiO2 | 75 | 9.6 | −21.1 | 0.50–1.75 | 1.87 |
| SiO2–P | 50 | 10.2 | −27.2 | 1.50 | 2.65 |
| Properties | Materials | |||||||||
|---|---|---|---|---|---|---|---|---|---|---|
| 304SS | Al | Al2O3 | Au | Cr | Cu | Mo | Ni | Pt | Silica | |
| Vickers Hardness (MPa) | 1700–2100 | 167 | 20594 | 188–216 | 1060 | 356 | 1530 | 638 | 549 | 6963 |
| Bulk Modulus (GPa) | 134–151 | 76 | 228 | 220 | 160 | 140 | 230 | 180 | 230 | 33.5–36.8 |
| Density (g/cm3) | 8.0 | 2.702 | 3.99 | 19.32 | 7.19 | 8.96 | 10.28 | 8.9 | 21.45 | 2.1977 |
| Electrical resistivity (Ω m) | 6.5–7.7 × 107 | 2.82 × 108 | 2.0 × 1011 | 2.44 × 108 | 1.25 × 107 | 1.68 × 108 | 5.0 × 108 | 6.9 × 108 | 1.05 × 108 | 1.0 × 1016 |
| Thermal Conductivity (W/m K) | 16.2 | 237 | 35 | 317 | 93.9 | 401 | 139 | 90.7 | 71.6 | 1.38 |
| Electron Affinity (eV) | 0.362 * | 0.43283 | 3.28 | 2.3086 | 0.67584 | 1.235 | 0.745 | 1.156 | 2.128 | 2.03 |
| Work Function (eV) | 4.4 | 4.06–4.26 | 1.95 | 5.31–5.47 | 4.5 | 4.79 | 4.655 | 5.04–5.35 | 5.53 | 1.20 |
| Shear Modulus (GPa) | 74-81 | 26 | 152 | 27 | 115 | 41 | 20 | 76 | 61 | 70 |
| Surface Energy (mJ/m2) | 700–1100 | 1270 | 900 | 1283 | 4123 | 1650 | 2953.5 | 2011 | 2299 | 396 |
| Standard Electrode Potential (V) | −0.490 * | −1.677 | −2.33 | 1.5 | −0.74 | 0.339 | −0.13 | −0.236 | 1.18 | −0.99 |
| Roughness (nm) | 2.08 | 9.94 | 5.43 | 1.08 | 2.24-3.17 | 2.22 | 4.71 | 3.90 | 1.71 | 3.26 |
| Substrate | Nanoparticle | ||||
|---|---|---|---|---|---|
| Al2O3 | Fe2O3 | SiO2 | SiO2-P | TiO2 | |
| 304SS | I | ||||
| Al | II, e | ||||
| Alumina | III | I, E | III, e | ||
| Au | I | III | I | ||
| Cr | I | ||||
| Cu | II, E | ||||
| Mo | II, E | ||||
| Ni | III | I | |||
| Pt | III | II | |||
| Silica | I | ||||
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Acharya, B.; Pardue, T.N.; Su, L.; Smirnov, A.I.; Brenner, D.W.; Krim, J. Nanotribological Performance Factors for Aqueous Suspensions of Oxide Nanoparticles and Their Relation to Macroscale Lubricity. Lubricants 2019, 7, 49. https://doi.org/10.3390/lubricants7060049
Acharya B, Pardue TN, Su L, Smirnov AI, Brenner DW, Krim J. Nanotribological Performance Factors for Aqueous Suspensions of Oxide Nanoparticles and Their Relation to Macroscale Lubricity. Lubricants. 2019; 7(6):49. https://doi.org/10.3390/lubricants7060049
Chicago/Turabian StyleAcharya, Biplav, Tyler N. Pardue, Liangliang Su, Alex I. Smirnov, Donald W. Brenner, and Jacqueline Krim. 2019. "Nanotribological Performance Factors for Aqueous Suspensions of Oxide Nanoparticles and Their Relation to Macroscale Lubricity" Lubricants 7, no. 6: 49. https://doi.org/10.3390/lubricants7060049
APA StyleAcharya, B., Pardue, T. N., Su, L., Smirnov, A. I., Brenner, D. W., & Krim, J. (2019). Nanotribological Performance Factors for Aqueous Suspensions of Oxide Nanoparticles and Their Relation to Macroscale Lubricity. Lubricants, 7(6), 49. https://doi.org/10.3390/lubricants7060049