Influence of Nitrile Butadiene Rubber (NBR) Shore Hardness and Polytetrafluoroethylene (PTFE) Elastic Modulus on the Sealing Characteristics of Step Rod Seals
Abstract
:1. Introduction
2. Theoretical Models
2.1. Geometrical Model
2.2. Mathematical Model
2.2.1. Mechanical Property Characterization of Seal Materials
2.2.2. Mixed EHL Model
2.3. Boundary Conditions
2.4. Numerical Method
- (1)
- Begin by specifying the initial material and structural properties of the seal and hydraulic oil, and the operating conditions such as rod speed, sealed pressure, and ambient temperature.
- (2)
- Solve the offline 2D finite element model to obtain the seal macro-deformation and stress distribution, static contact pressure distribution, and influence coefficient matrix.
- (3)
- Solve the Reynolds equation using the streamline-upwind/Petrov–Galerkin finite element method with a convergence criterion of 10−6 to obtain the fluid film pressure distribution, and calculate the fluid viscous shear stress distribution.
- (4)
- Calculate the asperity contact pressure distribution and the contact shear stress distribution.
- (5)
- Update the fluid film thickness distribution, and calculate the fluid film temperature distribution to update the fluid viscosity.
- (6)
- Compare the new fluid film thickness with the old values. If |hnew − hold|/hnew ≤ 10−6, then end the computation and output. If |hnew − hold|/hnew > 10−6, then return to step (3) to continue.
3. Results Analysis and Discussion
3.1. Validation
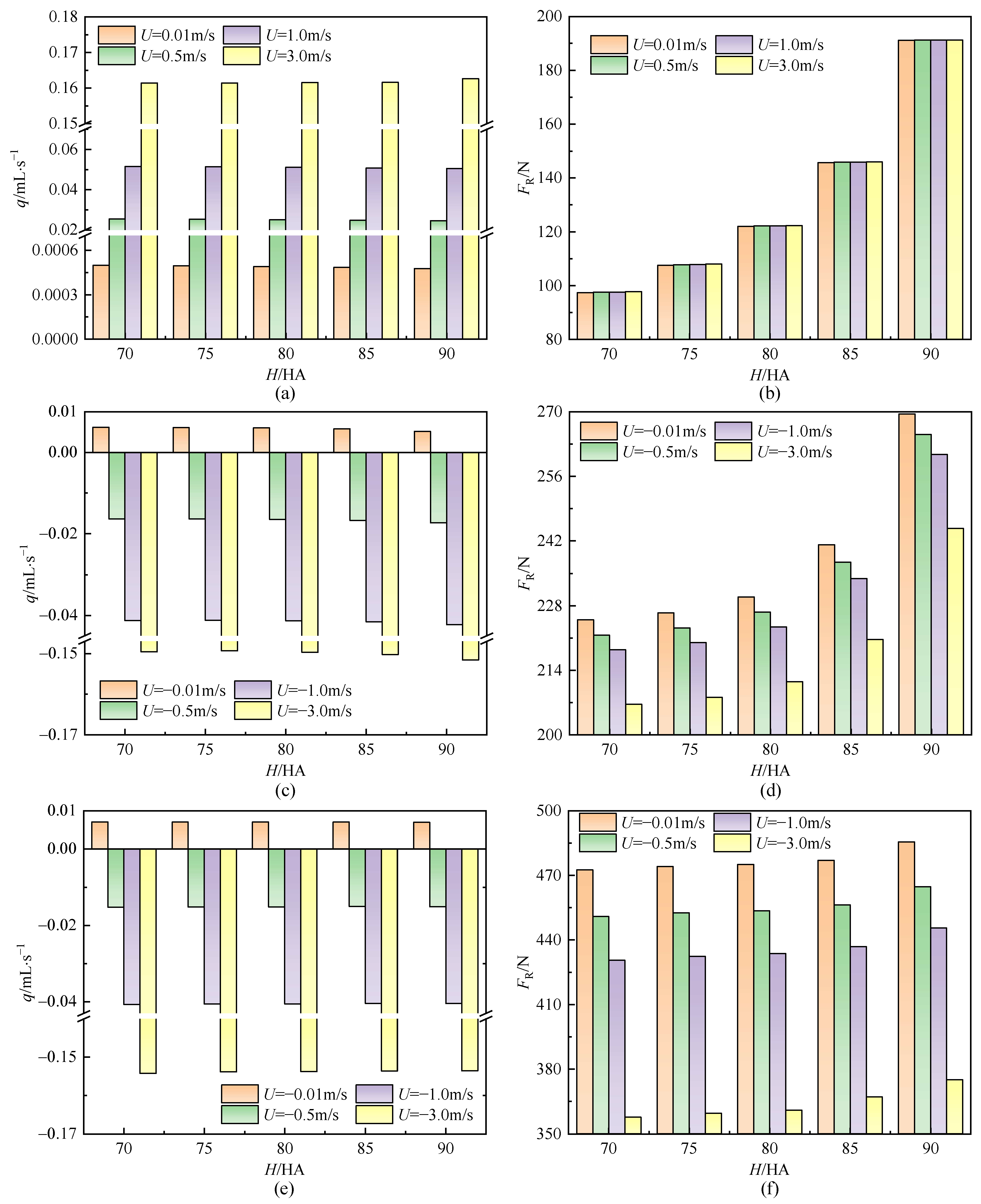
3.2. Influence of Rubber Hardness on the Sealing Characteristics
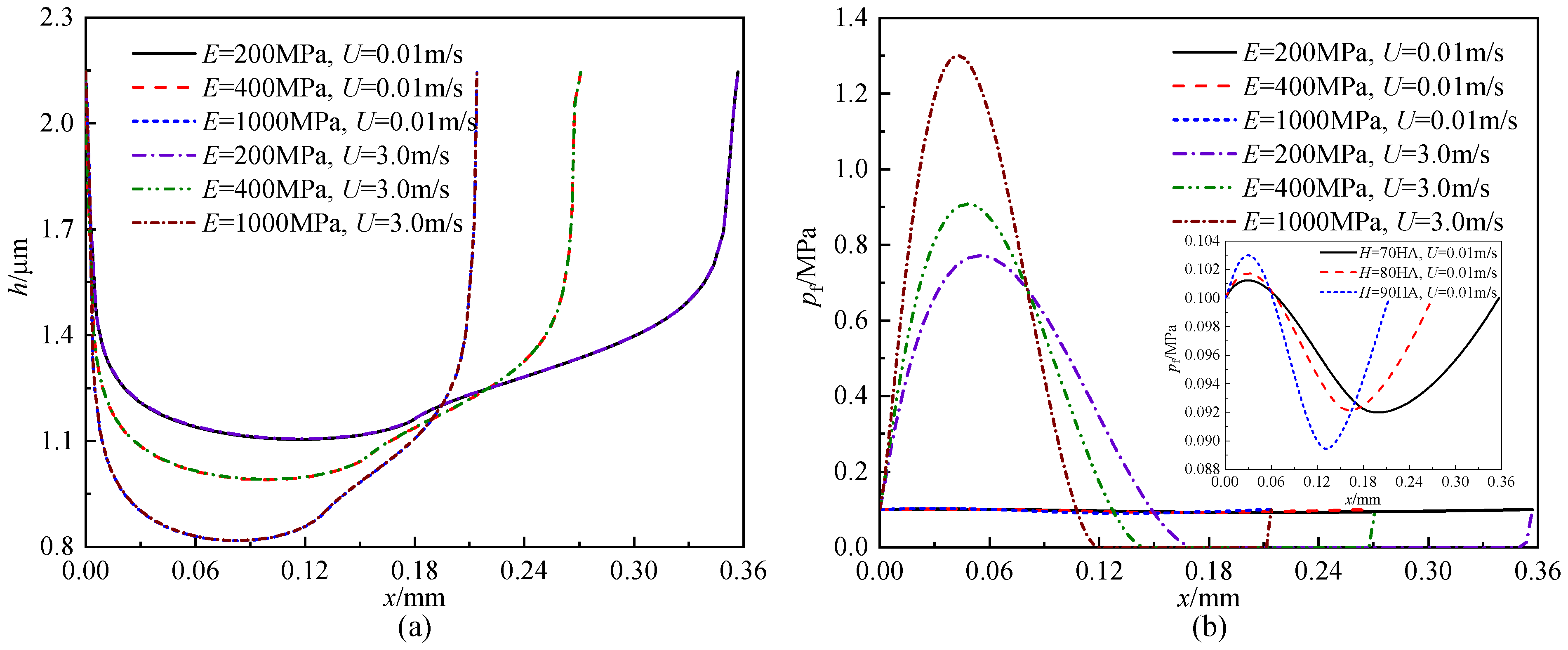
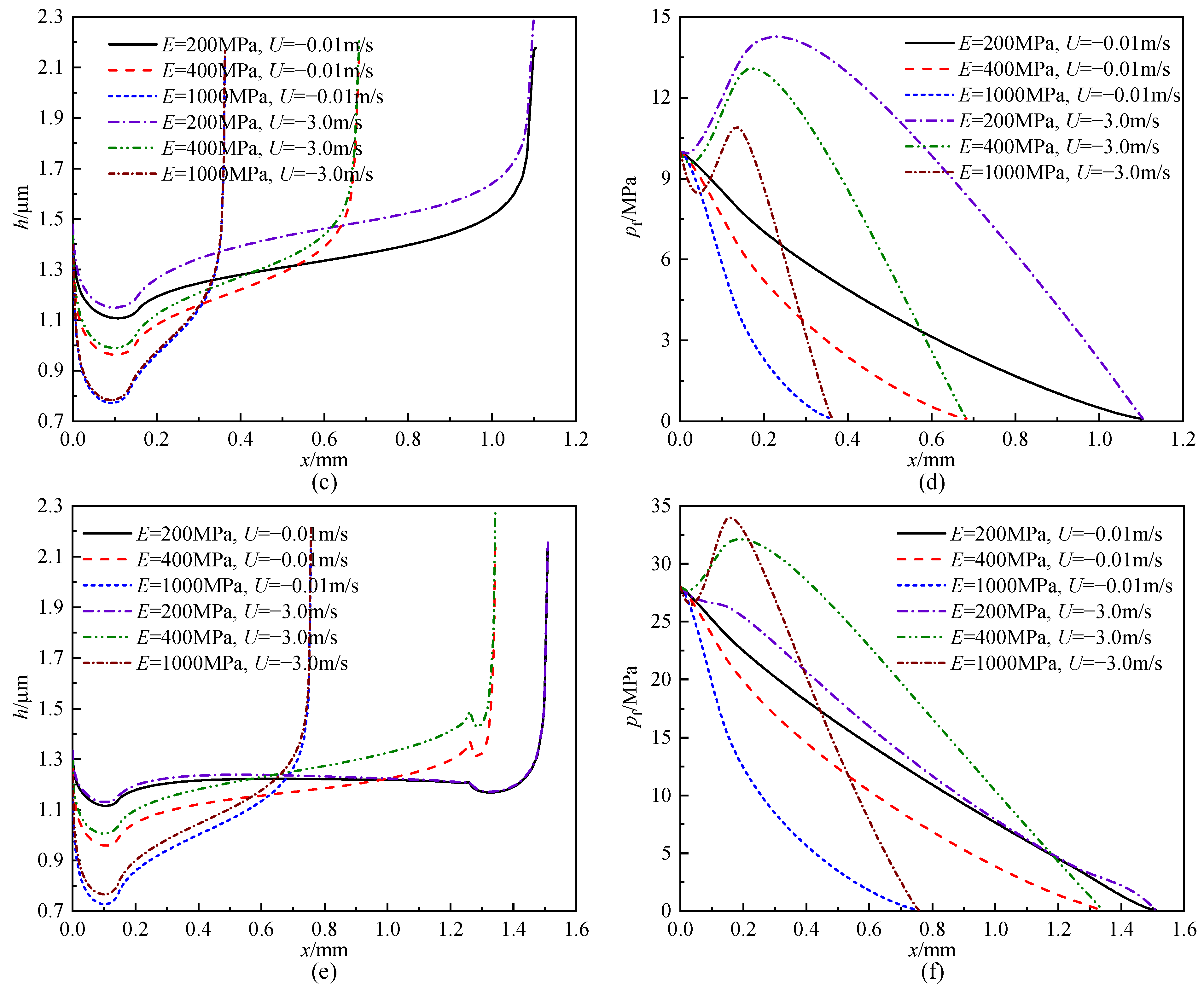
3.3. Influence of PTFE Elastic Modulus on the Sealing Characteristics
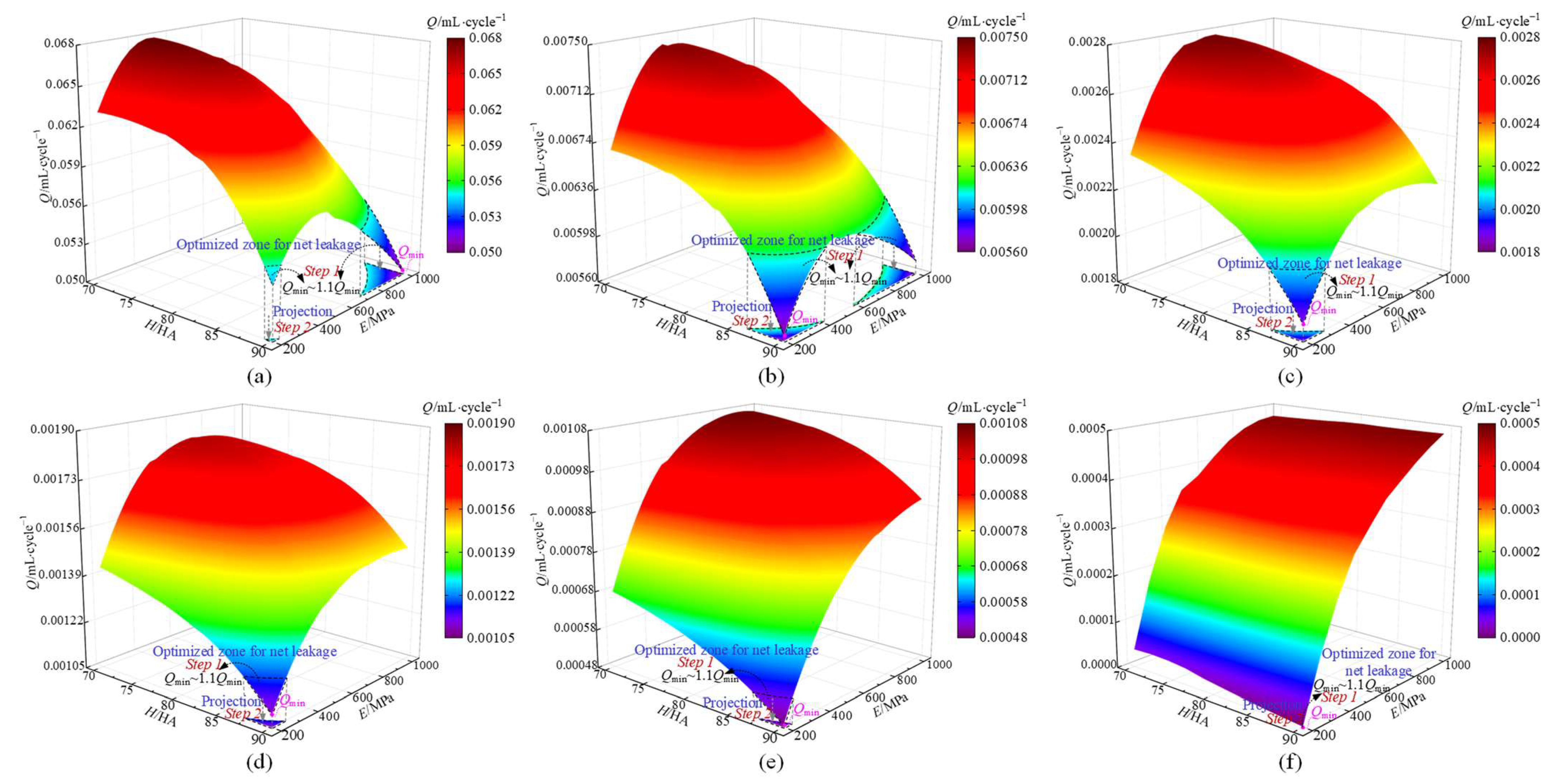
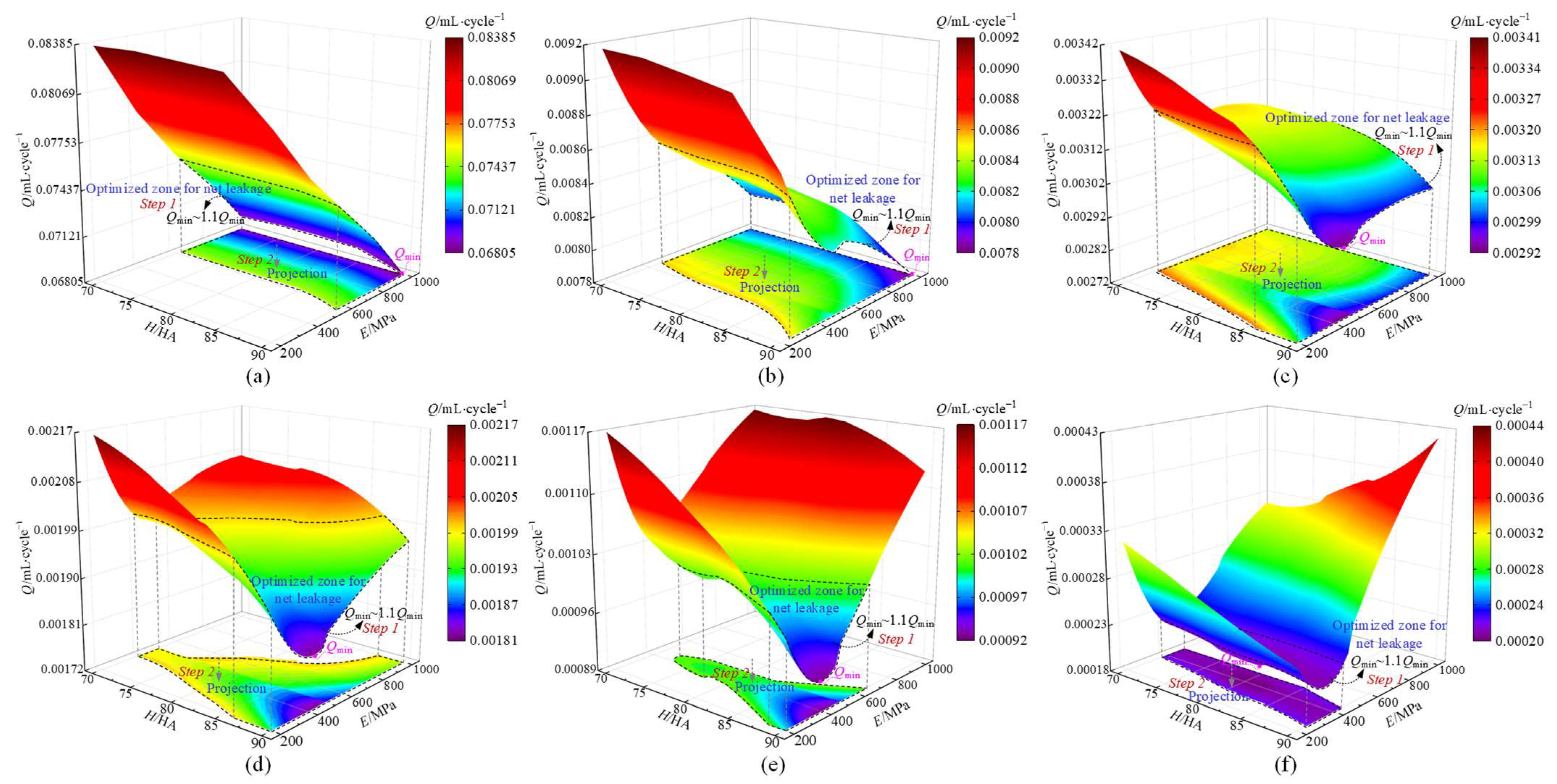
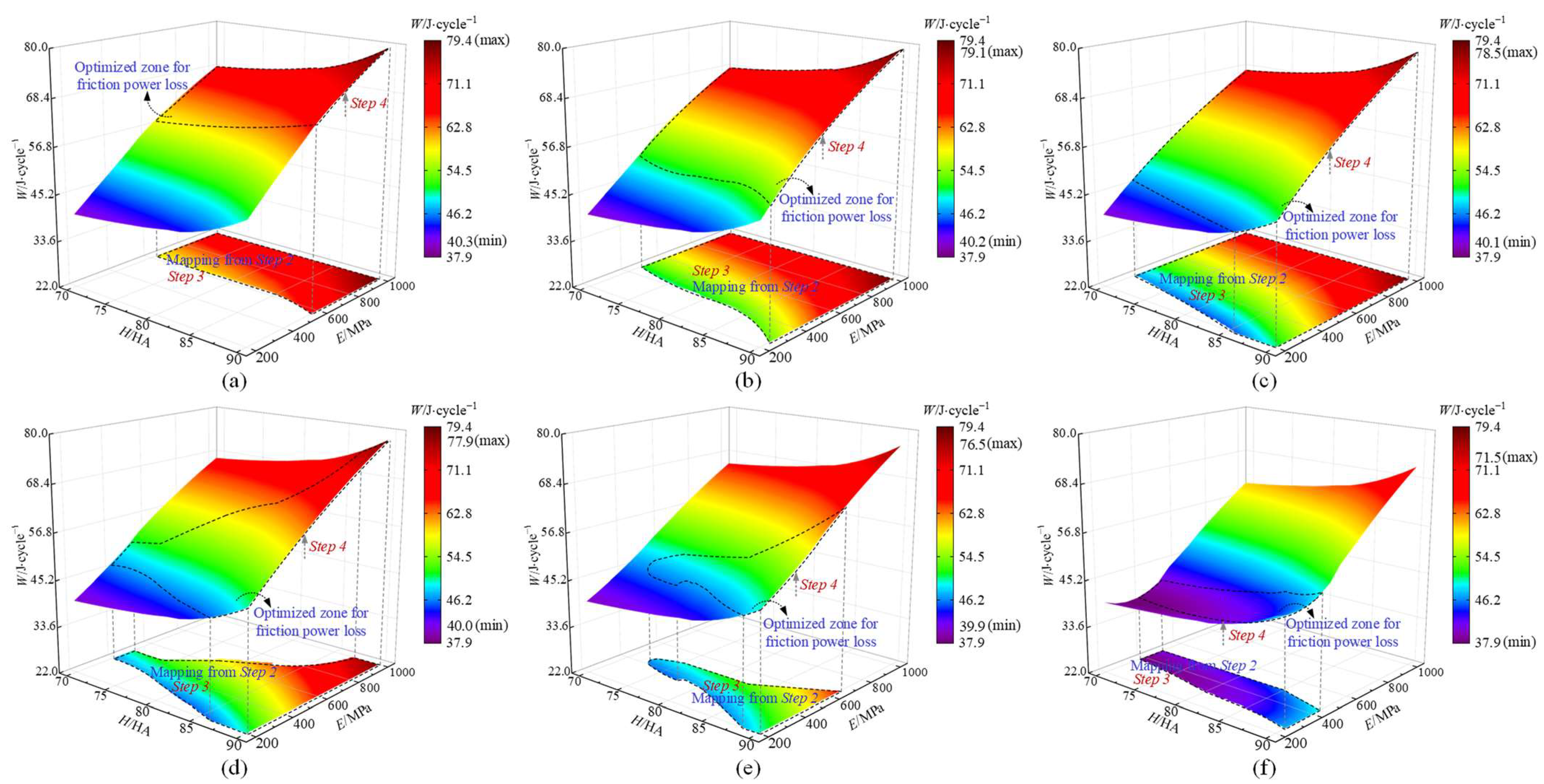
3.4. Optimized Selection of NBR Shore Hardness and PTFE Elastic Modulus
4. Conclusions
- NBR Shore hardness and PTFE elastic modulus have a significant influence on the deformation behavior and contact state of step rod seals, forming a sealing zone with different fluid film thickness and fluid film pressure, resulting in obvious changes in seal behavior and performance.
- As NBR Shore hardness and PTFE elastic modulus increase, the static contact pressure increases, the minimum film thickness decreases, the friction force increases, and the leakage rate decreases. There is a strong interaction between the influence of NBR Shore hardness and PTFE elastic modulus on leakage under different operating conditions.
- To reduce both leakage and friction in step rod seals, it is recommended that high NBR Shore hardness and low PTFE modulus be selected for moderate-low pressure or high speed conditions, and low NBR Shore hardness and high modulus be selected for high pressure or low speed conditions.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Nomenclature
| cR | Specific heat of the rod |
| C10, C01 | Mooney–Rivlin coefficients |
| D | Rod diameter |
| E, E0, E′ | PTFE elastic modulus, NBR elastic modulus, equivalent elastic modulus |
| fc | Dry friction coefficient |
| FR | Friction force |
| h, hS, hT | Nominal film thickness, static film thickness, truncated film thickness |
| H | NBR Shore hardness |
| In | Influence coefficient matrix |
| kR | Thermal conductivity of the rod |
| l | Half-length of the contact width |
| L | Length of the sealing zone |
| Lstroke | Stroke length |
| pa | Ambient pressure |
| pc | Asperity contact pressure |
| pcav | Cavitation pressure of fluid film |
| pf | Fluid film pressure |
| psc | Static contact pressure |
| psc.max | Maximum static contact pressure |
| psealed | Sealed pressure |
| q | Flow rate |
| Q | Net leakage |
| Qh | Heat generation rate |
| R | Radius of asperities |
| T, T0, Ta | Film temperature, reference temperature, ambient temperature |
| U | Rod speed |
| W | Friction power loss |
| α | Viscosity–pressure coefficient |
| β | Viscosity–temperature coefficient |
| η | Density of asperities |
| θ | Fluid density ratio |
| µ, µ0 | Lubricant dynamic viscosity, lubricant dynamic viscosity at T0 |
| υ | Poisson’s ratio of PTFE |
| ρ | The density of the fluid film |
| ρL | The density of the fluid film in liquid phase |
| ρR | The density of the rod |
| σ | Root mean square surface roughness |
| τc, τf | Contact shear stress, fluid viscous shear stress |
| ϕf, ϕfss, ϕfpp | Shear stress factors |
| ϕs, ϕx | Shear flow factor, pressure flow factor |
References
- Nikas, G.K. Eighty years of research on hydraulic reciprocating seals: Review of tribological studies and related topics since the 1930s. Proc. Inst. Mech. Eng. Part J J. Eng. Tribol. 2010, 224, 1–23. [Google Scholar] [CrossRef]
- Tan, B.; Stephens, L.S. Evaluation of viscoelastic characteristics of PTFE-Based materials. Tribol. Int. 2019, 140, 105870. [Google Scholar] [CrossRef]
- Yang, B.; Salant, R.F. Soft EHL simulations of U-Cup and step hydraulic rod seals. J. Tribol. 2009, 131, 021501. [Google Scholar] [CrossRef]
- Wang, B.; Peng, X.; Meng, X. Simulation of the effects of non-Newtonian fluid on the behavior of a step hydraulic rod seal based on a power law fluid model. J. Zhejiang Univ.-Sci. A 2018, 19, 824–842. [Google Scholar] [CrossRef]
- Heipl, O.; Murrenhoff, H. Friction of hydraulic rod seals at high velocities. Tribol. Int. 2015, 85, 66–73. [Google Scholar] [CrossRef]
- Li, Y.; Wang, J.; Lian, Z. High-speed friction and sealing characteristics of step seal under mixed lubrication conditions. China Mech. Eng. 2021, 32, 533–539. [Google Scholar] [CrossRef]
- Zhang, M.; Feng, Y. Numerical model of mixed lubrication and experimental study of reciprocating seal based on inverse lubrication theory. Lubricants 2022, 10, 153. [Google Scholar] [CrossRef]
- Ran, H.; Wang, S.; Liu, D. A multiscale wear model for reciprocating rod stepseal under mixed lubricating conditions based on linear elasticity. Proc. Inst. Mech. Eng. Part J J. Eng. Tribol. 2021, 235, 161–180. [Google Scholar] [CrossRef]
- Wang, J.; Li, J.; Ma, C. A performance degradation analysis method for a reciprocating rod seal in the wear process under mixed lubrication conditions. Proc. Inst. Mech. Eng. Part J J. Eng. Tribol. 2023, 237, 681–697. [Google Scholar] [CrossRef]
- Guo, F.; Jia, X.; Lv, M.; Wang, L.; Salant, R.F.; Wang, Y. The effect of aging in oil on the performance of a radial lip seal. Tribol. Int. 2014, 78, 187–194. [Google Scholar] [CrossRef]
- Peng, C.; Ouyang, X.; Schmitz, K.; Wang, W.; Guo, S.; Yang, H. Investigation of the tribological performance of reciprocating seals in a wide temperature range. Proc. Inst. Mech. Eng. Part J J. Eng. Tribol. 2021, 235, 2396–2414. [Google Scholar] [CrossRef]
- Nikas, G.K.; Sayles, R.S. Nonlinear elasticity of rectangular elastomeric seals and its effect on elastohydrodynamic numerical analysis. Tribol. Int. 2004, 37, 651–660. [Google Scholar] [CrossRef]
- Nikas, G.K. Parametric and optimization study of rectangular-rounded, hydraulic, elastomeric, reciprocating seals at temperatures between –54 and +135 °C. Lubricants 2018, 6, 77. [Google Scholar] [CrossRef]
- Bouaziz, R.; Truffault, L.; Borisov, R.; Ovalle, C.; Laiarinandrasana, L.; Miquelard-Garnier, G.; Fayolle, B. Elastic properties of polychloroprene rubbers in tension and compression during ageing. Polymers 2020, 12, 2354. [Google Scholar] [CrossRef]
- Gent, A.N. On the relation between indentation hardness and Young’s modulus. Rubber Chem. Technol. 1958, 31, 896–906. [Google Scholar] [CrossRef]
- Gent, A.N. Engineering with Rubber: How to Design Rubber Components, 3rd ed.; Carl Hanser Verlag: München, Germany, 2012; pp. 37–41. [Google Scholar]
- Ucar, H.; Basdogan, I. Dynamic characterization and modeling of rubber shock absorbers: A comprehensive case study. J. Low Freq. Noise Vib. Act. Control 2018, 37, 509–518. [Google Scholar] [CrossRef]
- Feyzullahoğlu, E. Abrasive wear, thermal and viscoelastic behaviors of rubber seal materials used in different working conditions. Proc. Inst. Mech. Eng. Part J J. Eng. Tribol. 2015, 229, 64–73. [Google Scholar] [CrossRef]
- Han, D.; Che, W. Comparison of the shear modulus of an offshore elastomeric bearing between numerical simulation and experiment. Appl. Sci. 2021, 11, 4384. [Google Scholar] [CrossRef]
- Liao, C.; Huang, W.; Wang, Y.; Suo, S.; Liu, Y. Fluid-solid interaction model for hydraulic reciprocating O-ring seals. Chin. J. Mech. Eng. 2013, 26, 85–94. [Google Scholar] [CrossRef]
- Zahabi, S.R.; Sheikhzadeh, M.; Shahramforouz, F.; Ko, F. The micro/macro mechanical approach of reinforced braid composite used in tribology. J. Compos. Mater. 2021, 55, 3813–3825. [Google Scholar] [CrossRef]
- Bao, Y.W.; Wang, W.; Zhou, Y.C. Investigation of the relationship between elastic modulus and hardness based on depth-sensing indentation measurements. Acta Mater. 2004, 52, 5397–5404. [Google Scholar] [CrossRef]
- Wang, B.; Peng, X.; Meng, X. Analysis of sealing performance of a hydraulic Glyd-ring seal based on soft EHL model. Tribology 2018, 38, 75–83. [Google Scholar] [CrossRef]
- Yang, B.; Salant, R.F. Elastohydrodynamic lubrication simulation of O-ring and U-cup hydraulic seals. Proc. Inst. Mech. Eng. Part J J. Eng. Tribol. 2011, 225, 603–610. [Google Scholar] [CrossRef]
- Bhushan, B. Principles and Applications of Tribology, 2nd ed.; Wiley: New York, NY, USA, 1999; pp. 403–444. [Google Scholar]
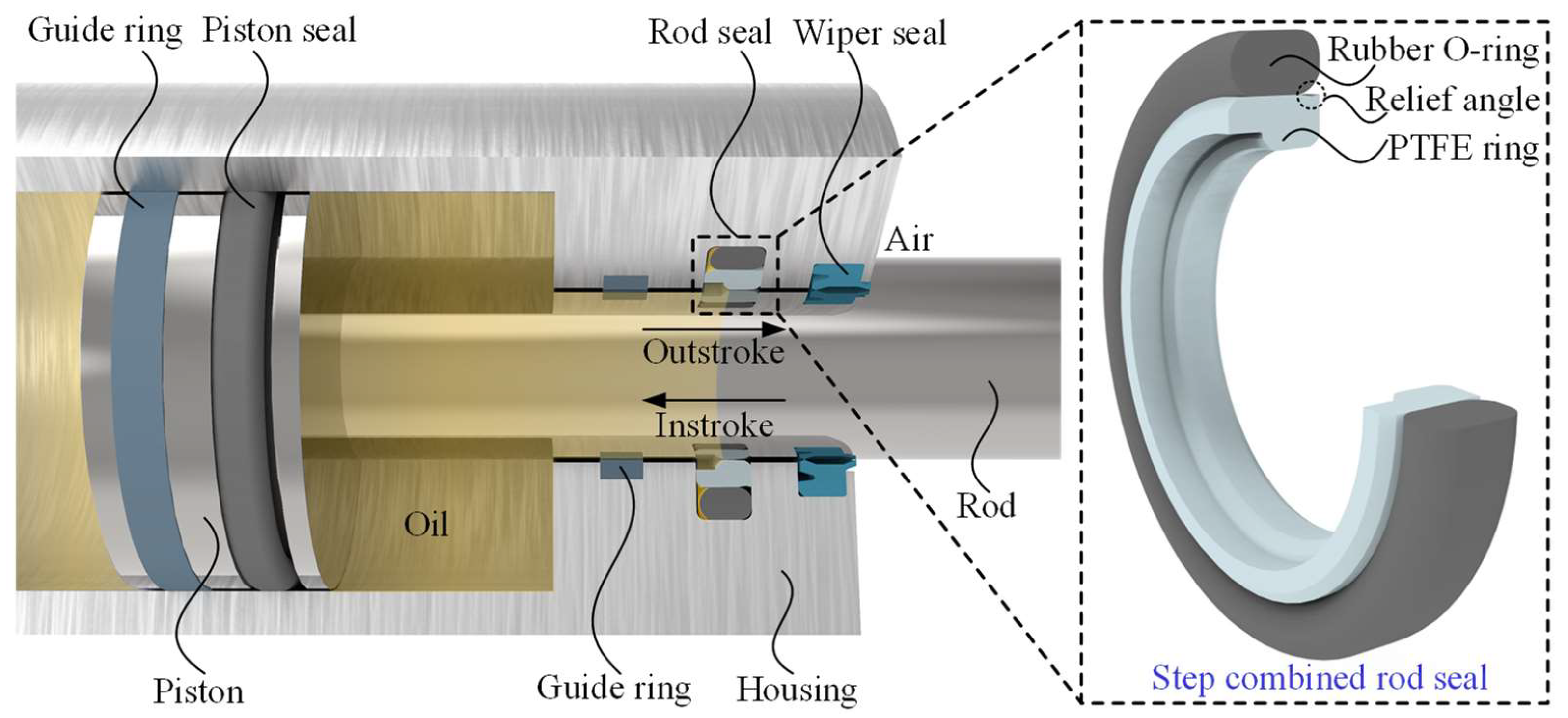
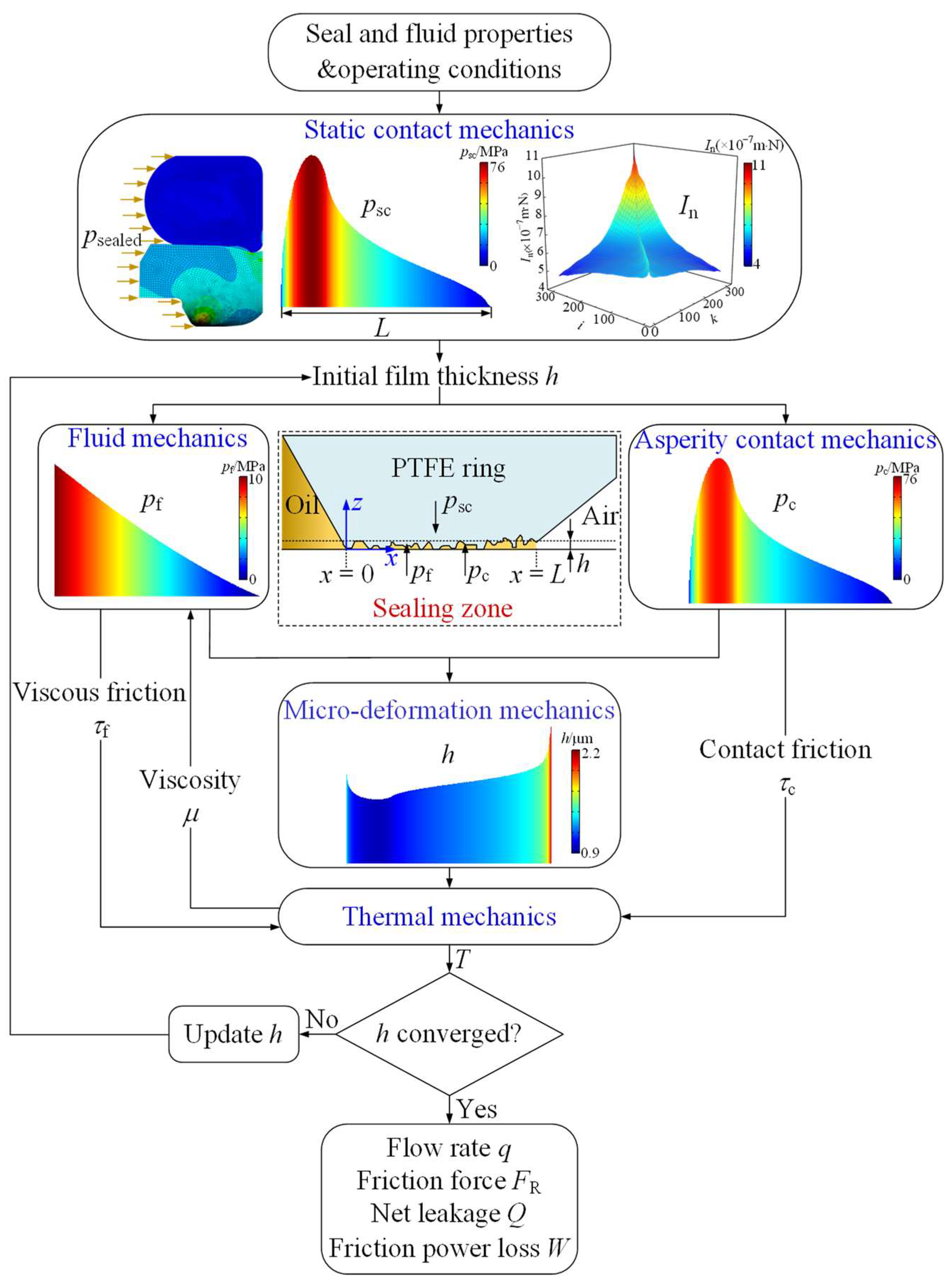

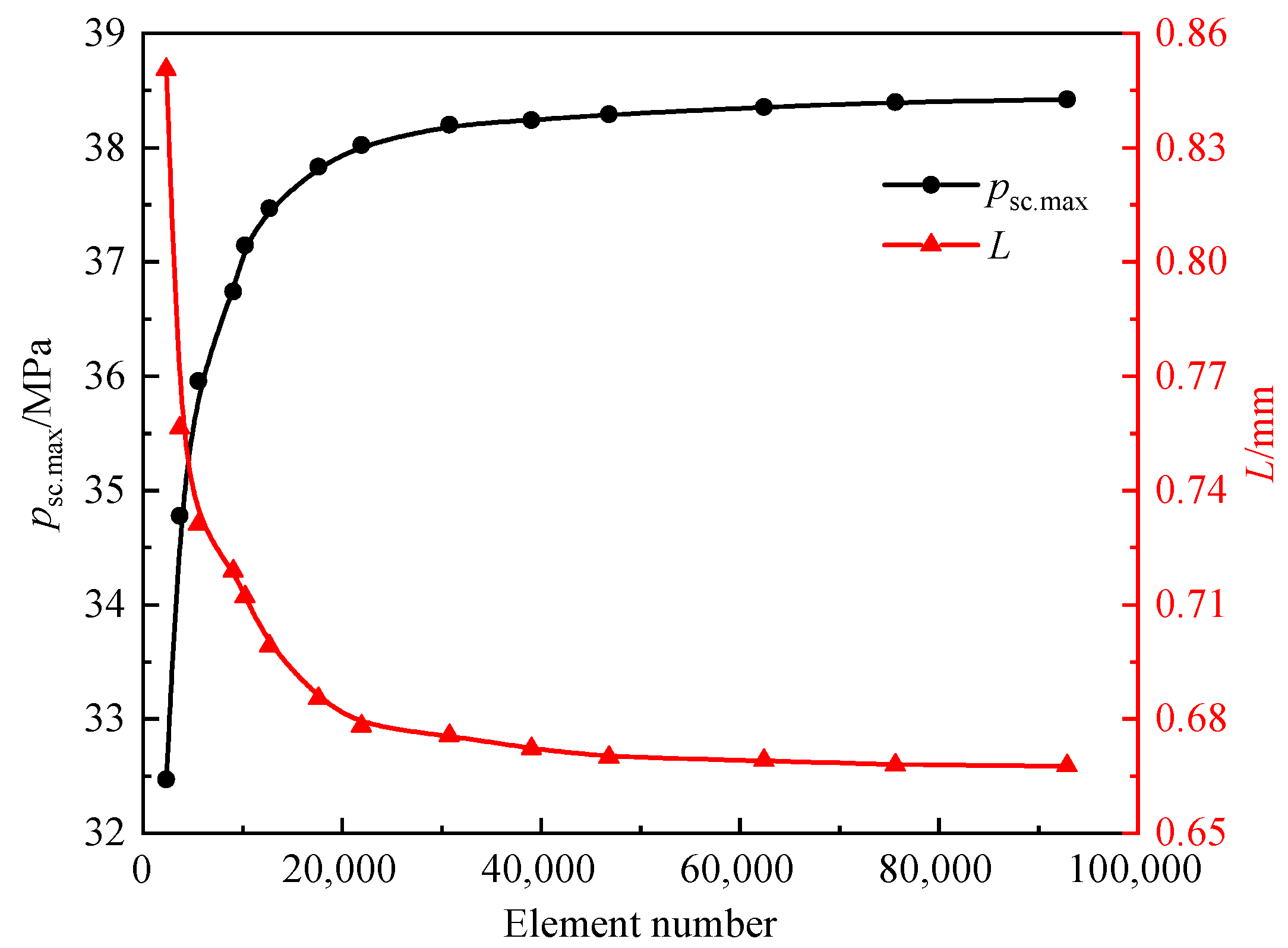

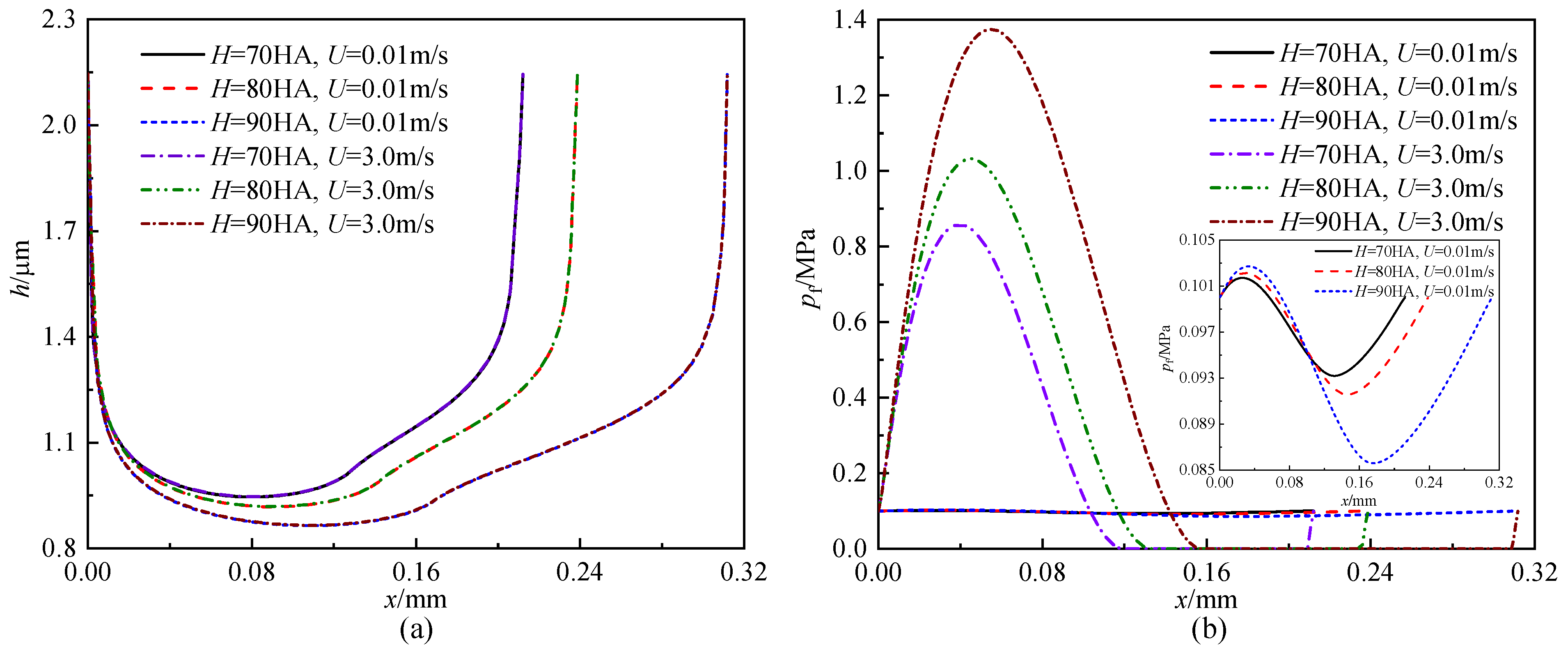




| Item and Symbol | Dimensions and Data |
|---|---|
| O-ring material | Nitrile butadiene rubber |
| Rubber Shore hardness, H | 70 HA–90 HA |
| PTFE ring material | Polytetrafluoroethylene (PTFE) |
| Poisson’s ratio of PTFE, υ | 0.45 |
| PTFE elastic modulus, E | 200 MPa–1000 MPa |
| O-ring cross-section diameter, d | 3.53 mm |
| Rod diameter, D | 32 mm |
| Stroke length, Lstroke | 100 mm |
| Specific heat of rod, cR | 460 J·kg–1·K–1 |
| Thermal conductivity of rod, kR | 46 W·m −1·K–1 |
| Density of rod, ρR | 7900 kg·m–3 |
| Reference viscosity, µ0 | 0.0117 Pa·s |
| Viscosity–pressure coefficient, α | 2.0 × 10–8 Pa–1 |
| Viscosity–temperature coefficient, β | 1.63335 × 10–2 K–1 |
| Rod speed, U | 0.01 m·s–1–3.0 m·s–1 |
| Sealed pressure, psealed | 0.1 MPa (Outstroke); 10 MPa/28 MPa (Instroke) |
| RMS roughness of seal, σ | 0.6 µm |
| Dry friction coefficient, fc | 0.1 |
| Radius of asperities, R | 1.0 µm |
| Density of asperities, η | 1.0 × 1014 m–2 |
| Ambient temperature, Ta | 300 K |
| Reference temperature, T0 | 313.5 K |
| Sealed Pressure psealed (MPa) | Rod Speed U (m/s) | NBR Shore Hardness H (HA) | PTFE Elastic Modulus E (MPa) |
|---|---|---|---|
| 10 (Moderate-low pressure) | 0.01–0.1 (Low speed) | 85–90 | 200–300 |
| 10 (Moderate-low pressure) | 0.1–0.5 (Moderate speed) | 85–90 | 200–260 |
| 10 (Moderate-low pressure) | 0.5–3.0 (High speed) | 85–90 | 200–220 |
| 28 (High pressure) | 0.01–0.1 (Low speed) | 70–80 | 600–800 |
| 28 (High pressure) | 0.1–0.5 (Moderate speed) | 70–85 | 400–500 |
| 28 (High pressure) | 0.5–3.0 (High speed) | 70–90 | 350–450 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, B.; Li, X.; Peng, X.; Li, Y.; Li, X.; Chen, Y.; Jin, J. Influence of Nitrile Butadiene Rubber (NBR) Shore Hardness and Polytetrafluoroethylene (PTFE) Elastic Modulus on the Sealing Characteristics of Step Rod Seals. Lubricants 2023, 11, 367. https://doi.org/10.3390/lubricants11090367
Wang B, Li X, Peng X, Li Y, Li X, Chen Y, Jin J. Influence of Nitrile Butadiene Rubber (NBR) Shore Hardness and Polytetrafluoroethylene (PTFE) Elastic Modulus on the Sealing Characteristics of Step Rod Seals. Lubricants. 2023; 11(9):367. https://doi.org/10.3390/lubricants11090367
Chicago/Turabian StyleWang, Bingqing, Xiaoxuan Li, Xudong Peng, Yuntang Li, Xiang Li, Yuan Chen, and Jie Jin. 2023. "Influence of Nitrile Butadiene Rubber (NBR) Shore Hardness and Polytetrafluoroethylene (PTFE) Elastic Modulus on the Sealing Characteristics of Step Rod Seals" Lubricants 11, no. 9: 367. https://doi.org/10.3390/lubricants11090367
APA StyleWang, B., Li, X., Peng, X., Li, Y., Li, X., Chen, Y., & Jin, J. (2023). Influence of Nitrile Butadiene Rubber (NBR) Shore Hardness and Polytetrafluoroethylene (PTFE) Elastic Modulus on the Sealing Characteristics of Step Rod Seals. Lubricants, 11(9), 367. https://doi.org/10.3390/lubricants11090367





