Pivot Stiffness Effect on Transient Dynamic Characteristic of Tilting Pad Journal Bearing-Rotor System Passing through Critical Speed
Abstract
1. Introduction
2. Modeling
2.1. Hydrodynamic Lubrication Model of TPJB
2.2. Transient Dynamic Model of TPJB-Rotor System
3. Solving Method
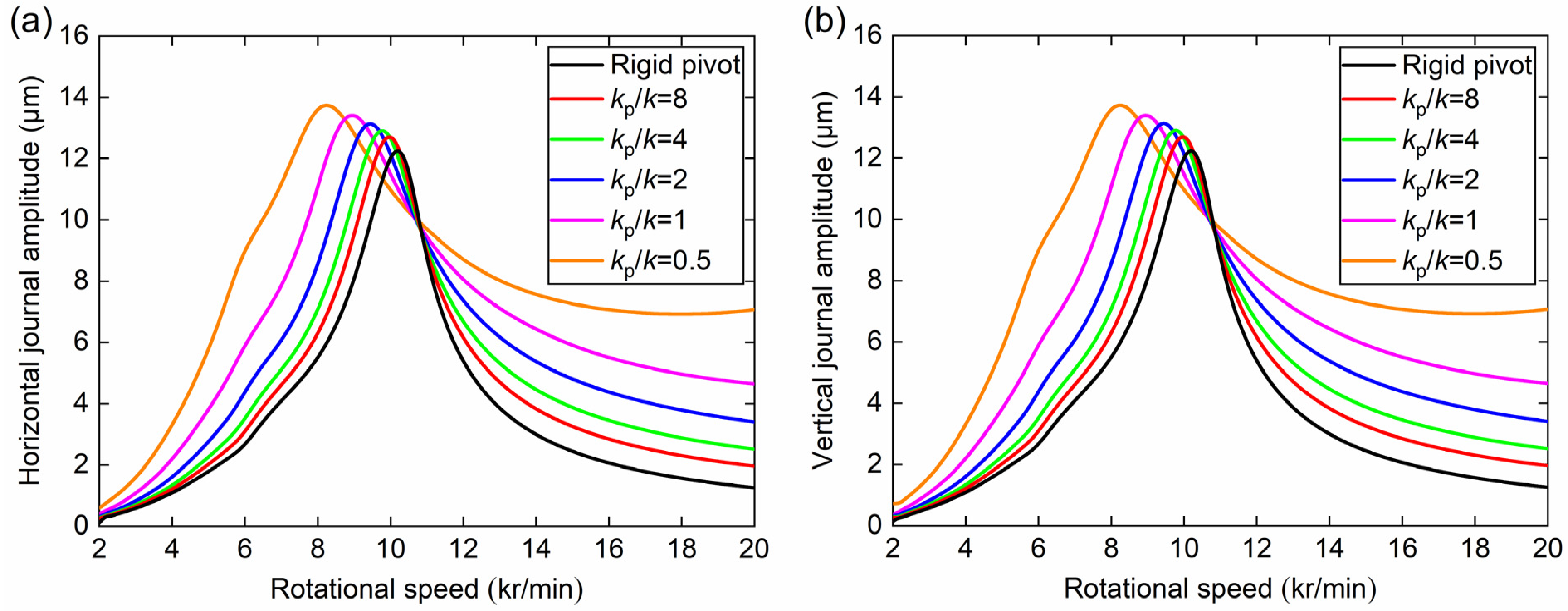
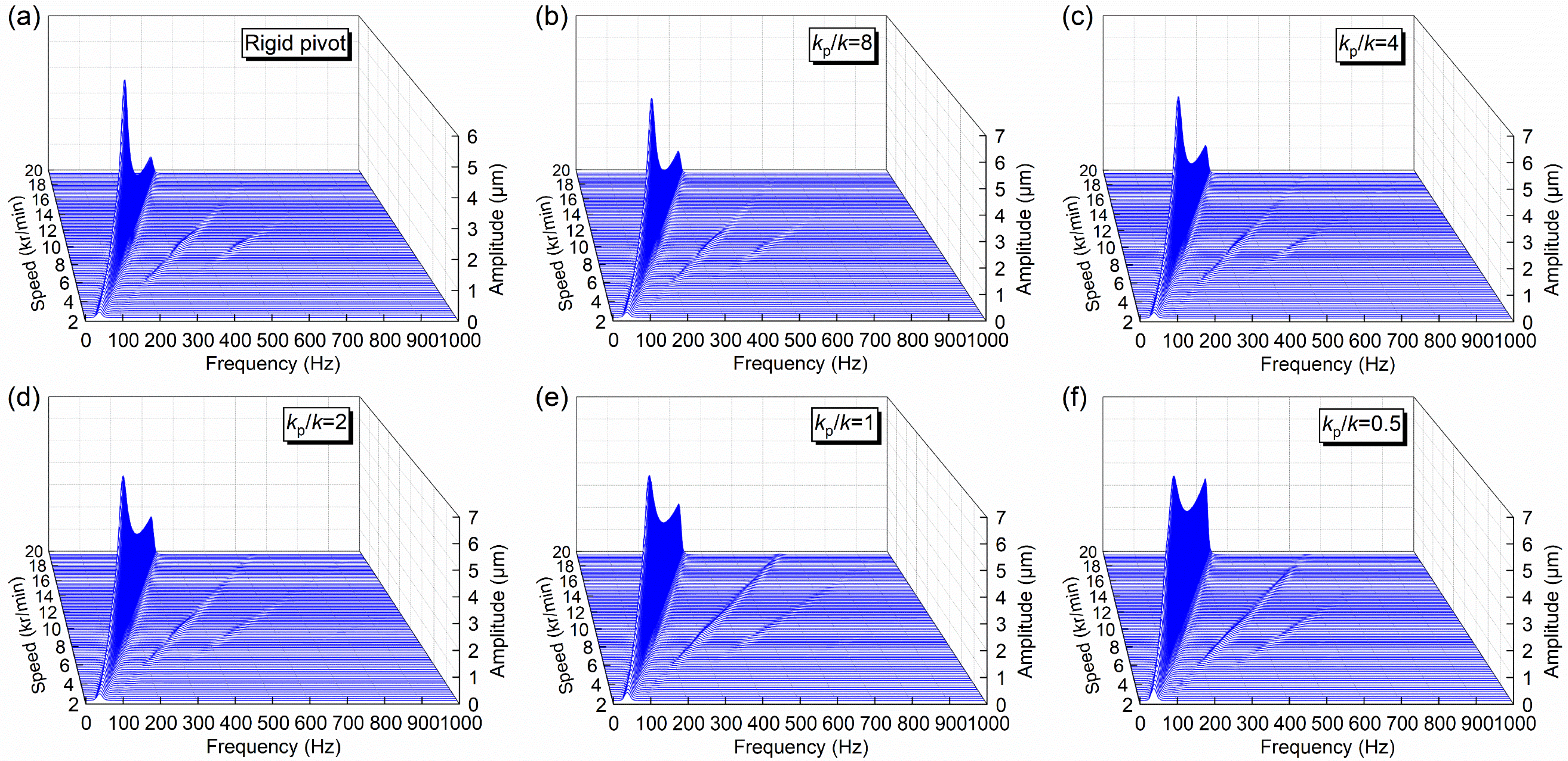
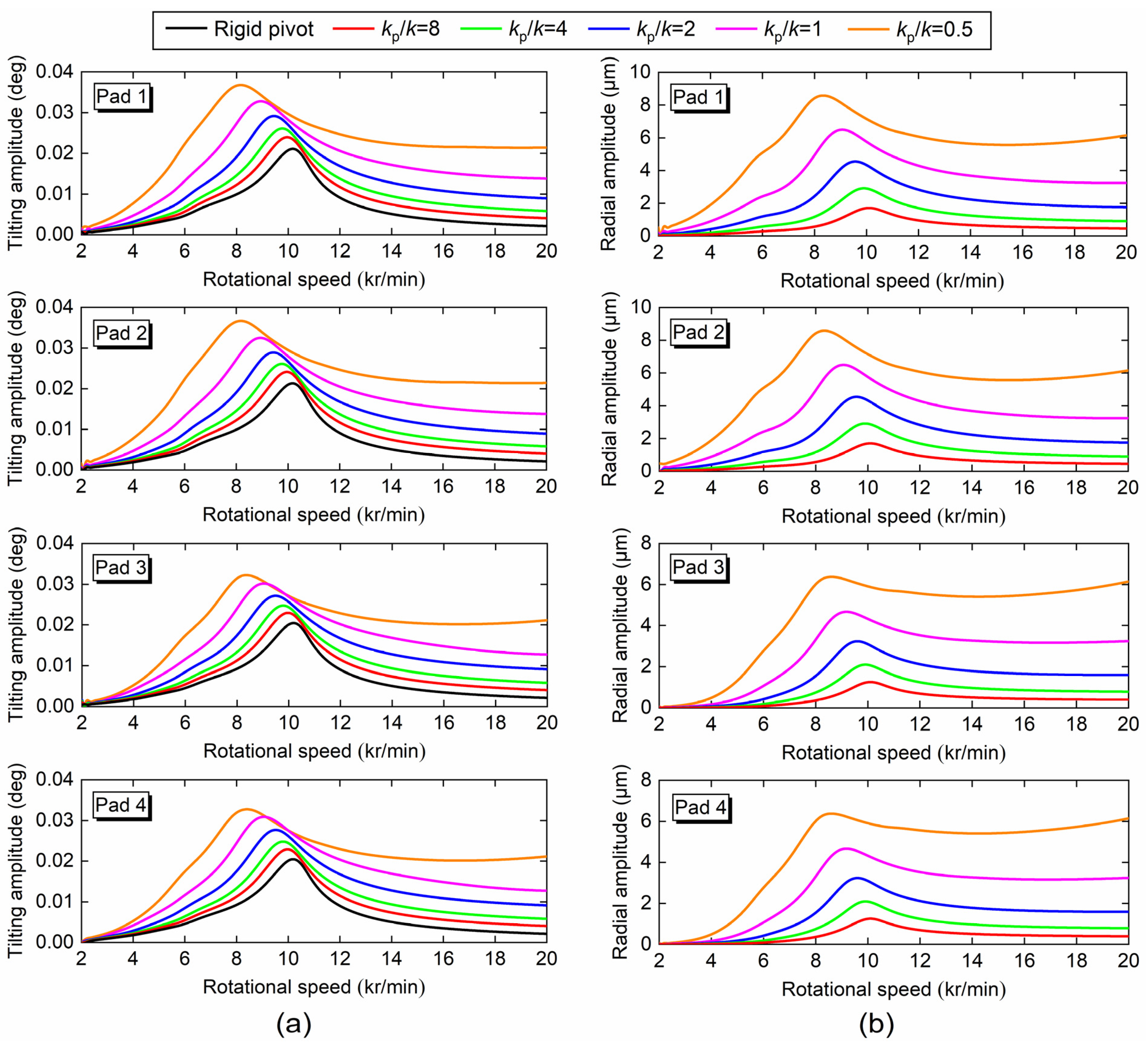
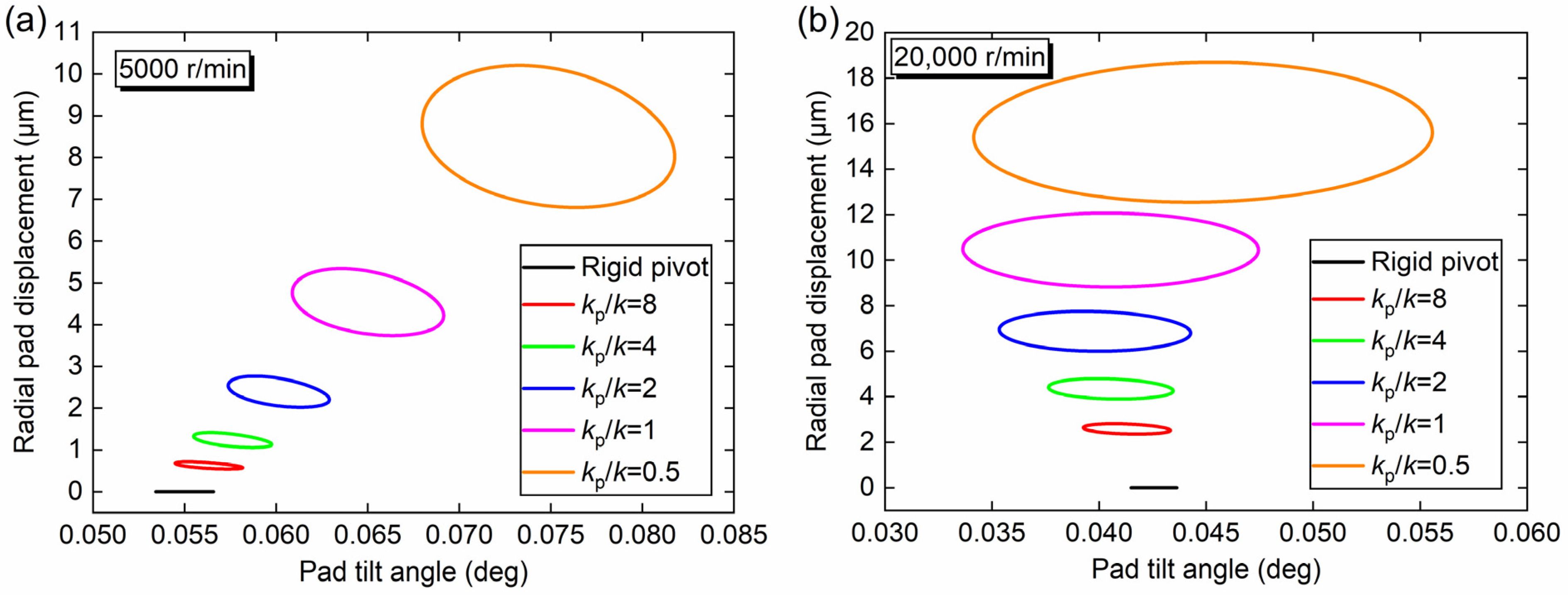
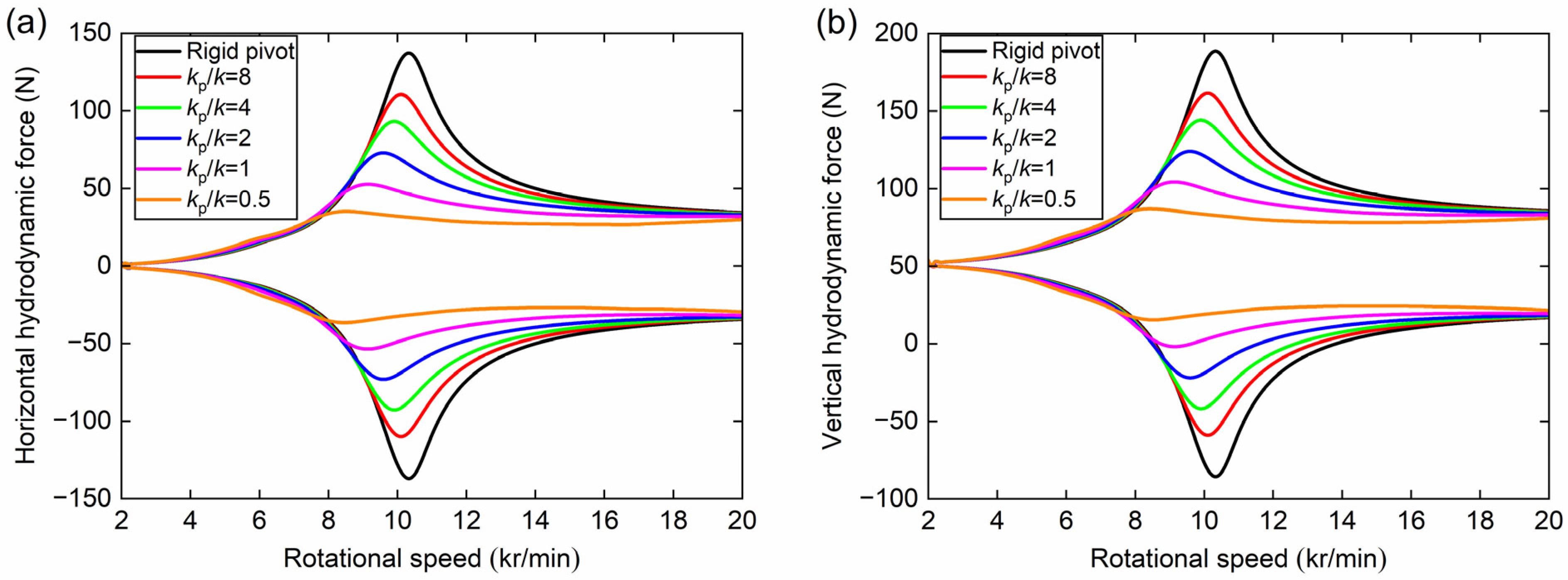
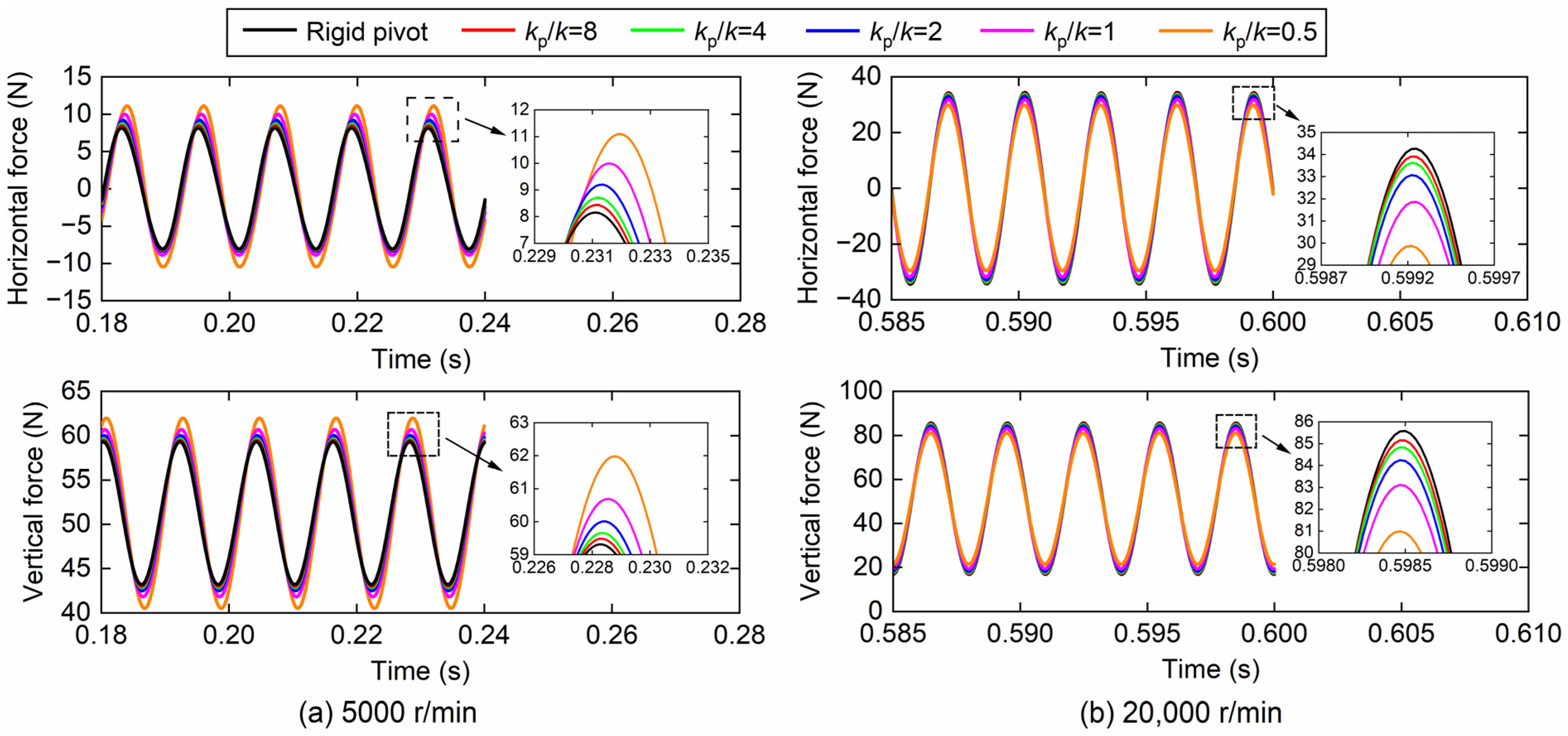
4. Results and Discussion
5. Conclusions
- For a rigid rotor with working speed below the critical speed, the working amplitude of the rotor disc, journal, and pads and the maximum hydrodynamic force in the TPJB could be reduced by increasing the pivot stiffness.
- For a flexible rotor with working speed above the critical speed, the resonance amplitude, working amplitude, and critical speed of the rotor disc and the maximum hydrodynamic force in the TPJB could be reduced by decreasing the pivot stiffness, which helps the rotor system to pass through the critical speed safely and avoid its working speed adjacent to the critical speed. At the same time, the amplitude of the journal and pads would increase accordingly.
- The pivot stiffness has little effect on the vibrational frequency characteristic of a stable TPJB-rotor system passing through the critical speed. Due to the nonlinear hydro-dynamic bearing force, there are super-synchronous harmonic components with minor amplitudes in the system: 2× rotational frequency is found in the disc vibration; 2× and 3× rotational frequencies are found in the journal and pad vibrations.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Kirk, R.G.; Reedy, S.W. Evaluation of pivot stiffness for typical tilting-pad journal bearing designs. J. Vib. Acoust. Stress Reliab. Des. 1988, 110, 165–171. [Google Scholar] [CrossRef]
- Yan, Z.Y.; Lu, Y.; Zheng, T.S. An analytical complete model of tilting-pad journal bearing considering pivot stiffness and damping. J. Tribol. 2011, 133, 011702. [Google Scholar] [CrossRef]
- San Andres, L.; Tao, Y.J. The role of pivot stiffness on the dynamic force coefficients of tilting pad journal bearings. J. Eng. Gas Turbines Power 2013, 135, 112505. [Google Scholar] [CrossRef]
- Dimond, T.; Younan, A.A.; Allaire, P.; Nicholas, J. Modal frequency response of a four-pad tilting pad bearing with spherical pivots, finite pivot stiffness, and different pad preloads. J. Vib. Acoust. 2013, 135, 041101. [Google Scholar] [CrossRef]
- Mehdi, S.M.; Jang, K.E.; Kim, T.H. Effects of pivot design on performance of tilting pad journal bearings. Tribol. Int. 2018, 119, 175–189. [Google Scholar] [CrossRef]
- Dang, P.V.; Chatterton, S.; Pennacchi, P. The effect of the pivot stiffness on the performances of five-pad tilting pad bearings. Lubricants 2019, 7, 61. [Google Scholar] [CrossRef]
- Jin, Y.Z.; Chen, F.; Zhang, F.; Yuan, X.Y. Nonlinear dynamic performance of tilting-pad journal bearing with adjustable elastic pivot design. Tribol. Int. 2019, 136, 533–547. [Google Scholar] [CrossRef]
- Shi, Z.Y.; Jin, Y.Z.; Yuan, X.Y. Influence of pivot design on nonlinear dynamic analysis of vertical and horizontal rotors in tilting pad journal bearings. Tribol. Int. 2019, 140, 105859. [Google Scholar] [CrossRef]
- Shi, Z.Y.; Jin, Y.Z.; Yuan, X.Y.; Chen, R.L. Effect of pivot stiffness on nonlinear dynamic characteristics of tilting pad journal bearings. Tribol. Int. 2020, 146, 106222. [Google Scholar] [CrossRef]
- Zhang, F.; Yin, P.; Liu, Y.Y.; Wang, J.M. Numerical and experimental study on dynamic characteristics of tilting-pad journal bearings considering pivot stiffness in a vertical rotor system. Ind. Lubr. Tribol. 2021, 73, 1275–1285. [Google Scholar] [CrossRef]
- Ciulli, E.; Forte, P.; Antonelli, F.; Minelli, R.; Panara, D. Tilting pad journal bearing ball and socket pivots: Experimental determination of stiffness. Machines 2022, 10, 81. [Google Scholar] [CrossRef]
- Hei, D.; Lu, Y.J.; Zhang, Y.F.; Liu, F.X.; Zhou, C.; Muller, N. Nonlinear dynamic behaviors of rod fastening rotor-hydrodynamic journal bearing system. Arch. Appl. Mech. 2015, 85, 855–875. [Google Scholar] [CrossRef]
- Maharshi, K.; Mukhopadhyay, T.; Roy, B.; Roy, L.; Dey, S. Stochastic dynamic behaviour of hydrodynamic journal bearings including the effect of surface roughness. Int. J. Mech. Sci. 2018, 142, 370–383. [Google Scholar] [CrossRef]
- Cui, S.H.; Gu, L.; Wang, L.Q.; Xu, B.; Zhang, C.W. Numerical analysis on the dynamic contact behavior of hydrodynamic journal bearings during start-up. Tribol. Int. 2018, 121, 260–268. [Google Scholar] [CrossRef]
- Ma, J.J.; Zhang, H.; Lou, S.; Chu, F.L.; Shi, Z.Q.; Gu, F.S.; Ball, A.D. Analytical and experimental investigation of vibration characteristics induced by tribofilm-asperity interactions in hydrodynamic journal bearings. Mech. Syst. Signal Proc. 2021, 150, 107227. [Google Scholar] [CrossRef]
- Chen, S.A.; Xiang, G.; Fillon, M.; Guo, J.; Wang, J.X.; Cai, J.L. On the tribo-dynamic behaviors during start-up of water lubricated bearing considering imperfect journal. Tribol. Int. 2022, 174, 107685. [Google Scholar] [CrossRef]
- Xiang, G.; Wang, J.X.; Han, Y.F.; Yang, T.Y.; Dai, H.M.; Yao, B.W.; Zhou, C.D.; Wang, L.W. Investigation on the nonlinear dynamic behaviors of water-lubricated bearings considering mixed thermoelastohydrodynamic performances. Mech. Syst. Signal Proc. 2022, 169, 108627. [Google Scholar] [CrossRef]
- Sayed, H.; El-Sayed, T.A. Nonlinear dynamics and bifurcation analysis of journal bearings based on second order stiffness and damping coefficients. Int. J. Non-Linear Mech. 2022, 142, 103972. [Google Scholar] [CrossRef]
- Xie, Z.L.; Jiao, J.; Yang, K.; He, T.; Chen, R.G.; Zhu, W.D. Experimental and numerical exploration on the nonlinear dynamic behaviors of a novel bearing lubricated by low viscosity lubricant. Mech. Syst. Signal Proc. 2023, 182, 109349. [Google Scholar] [CrossRef]
- Abu-Mahfouz, I.; Adams, M.L. Numerical study of some nonlinear dynamics of a rotor supported on a three-pad tilting pad journal bearing (TPJB). J. Vib. Acoust. 2005, 127, 262–272. [Google Scholar] [CrossRef]
- Okabe, E.P.; Cavalca, K.L. Rotordynamic analysis of systems with a non-linear model of tilting pad bearings including turbulence effects. Nonlinear Dyn. 2009, 57, 481–495. [Google Scholar] [CrossRef]
- Ying, J.Y.; Jiao, Y.H.; Chen, Z.B. Nonlinear dynamics analysis of tilting pad journal bearing-rotor system. Shock Vib. 2011, 18, 45–52. [Google Scholar] [CrossRef]
- Hei, D.; Lu, Y.J.; Zhang, Y.F.; Lu, Z.Y.; Gupta, P.; Muller, N. Nonlinear dynamic behaviors of a rod fastening rotor supported by fixed-tilting pad journal bearings. Chaos Solitons Fractals 2014, 69, 129–150. [Google Scholar] [CrossRef]
- Cha, M.; Glavatskih, S. Nonlinear dynamic behaviour of vertical and horizontal rotors in compliant liner tilting pad journal bearings: Some design considerations. Tribol. Int. 2015, 82, 142–152. [Google Scholar] [CrossRef]
- Tofighi-Niaki, E.; Asgharifard-Sharabiani, P.; Ahmadian, H. Nonlinear dynamics of a flexible rotor on tilting pad journal bearings experiencing rub-impact. Nonlinear Dyn. 2018, 94, 2937–2956. [Google Scholar] [CrossRef]
- Zhao, Z.M.; Ji, F.; Guan, Y.S.; Yuan, X.Y. Vibration and critical characteristics of the tilting pads journal bearing-rotor system. Ind. Lubr. Tribol. 2019, 71, 295–300. [Google Scholar] [CrossRef]
- Kim, S.; Palazzolo, A.B. Pad-pivot friction effect on nonlinear response of a rotor supported by tilting-pad journal bearings. J. Tribol. 2019, 141, 091701. [Google Scholar] [CrossRef]
- Jin, Y.Z.; Shi, Z.Y.; Zhang, X.J.; Yuan, X.Y. Rapid solution for analysis of nonlinear fluid film force and dynamic behavior of a tilting-pad journal bearing-rotor system with turbulent and thermal effects. Friction 2020, 8, 343–359. [Google Scholar] [CrossRef]
- Hojjati, M.; Navazi, H.M.; Haddadpour, H. Nonlinear vibrations of a rotor on nonlinear tilting-pad-journal-bearings. J. Braz. Soc. Mech. Sci. Eng. 2021, 43, 168. [Google Scholar] [CrossRef]
- Li, S.; Lu, Y.J.; Zhang, Y.F.; Zhao, X.W.; Luo, H.B.; Meng, J.C. Modeling and analysis of dynamic behaviors for a flexible rotor system supported in tilting pad aerodynamic bearings. Appl. Math. Model. 2022, 110, 262–284. [Google Scholar] [CrossRef]
- Taylor, C.M.; Dowson, D. Turbulent lubrication theory—Application to design. J. Lubr. Technol. 1974, 96, 36–46. [Google Scholar] [CrossRef]
- Jin, Y.Z.; Chen, F.; Xu, J.M.; Yuan, X.Y. Nonlinear dynamic analysis of low viscosity fluid-lubricated tilting-pad journal bearing for different design parameters. Friction 2020, 8, 930–944. [Google Scholar] [CrossRef]
- Jin, Y.Z.; Yuan, X.Y. Analytical method for hydrodynamic force in finite-length tilting-pad journal bearing including turbulence effect. J. Tribol. 2020, 142, 091802. [Google Scholar] [CrossRef]
- Taniguchi, S.; Makino, T.; Takeshita, K.; Ichimura, T. A thermohydrodynamic analysis of large tilting-pad journal bearing in laminar and turbulent-flow regimes with mixing. J. Tribol. 1990, 112, 542–548. [Google Scholar] [CrossRef]
- Zhang, S.L.; Xing, Y.; Xu, H.; Pei, S.Y.; Zhang, L. An experimental study on vibration suppression of adjustable elliptical journal bearing-rotor system in various vibration states. Mech. Syst. Signal Proc. 2020, 141, 106477. [Google Scholar] [CrossRef]

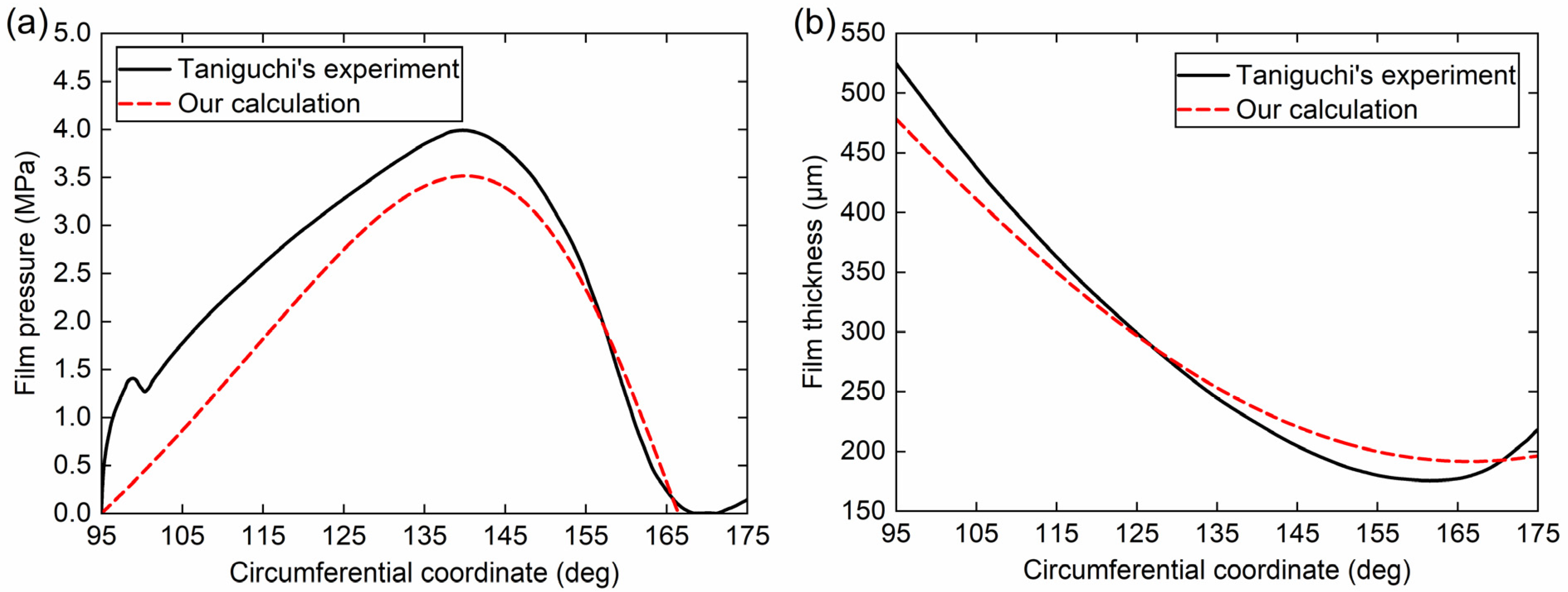
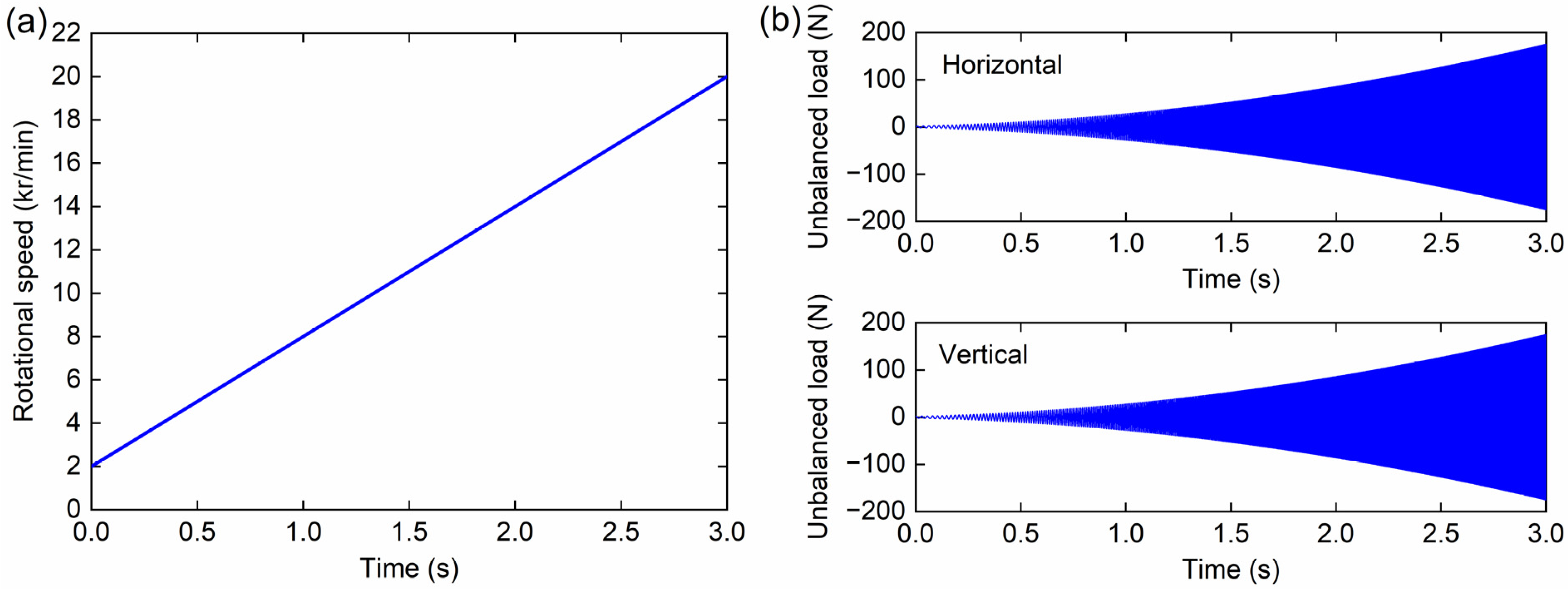
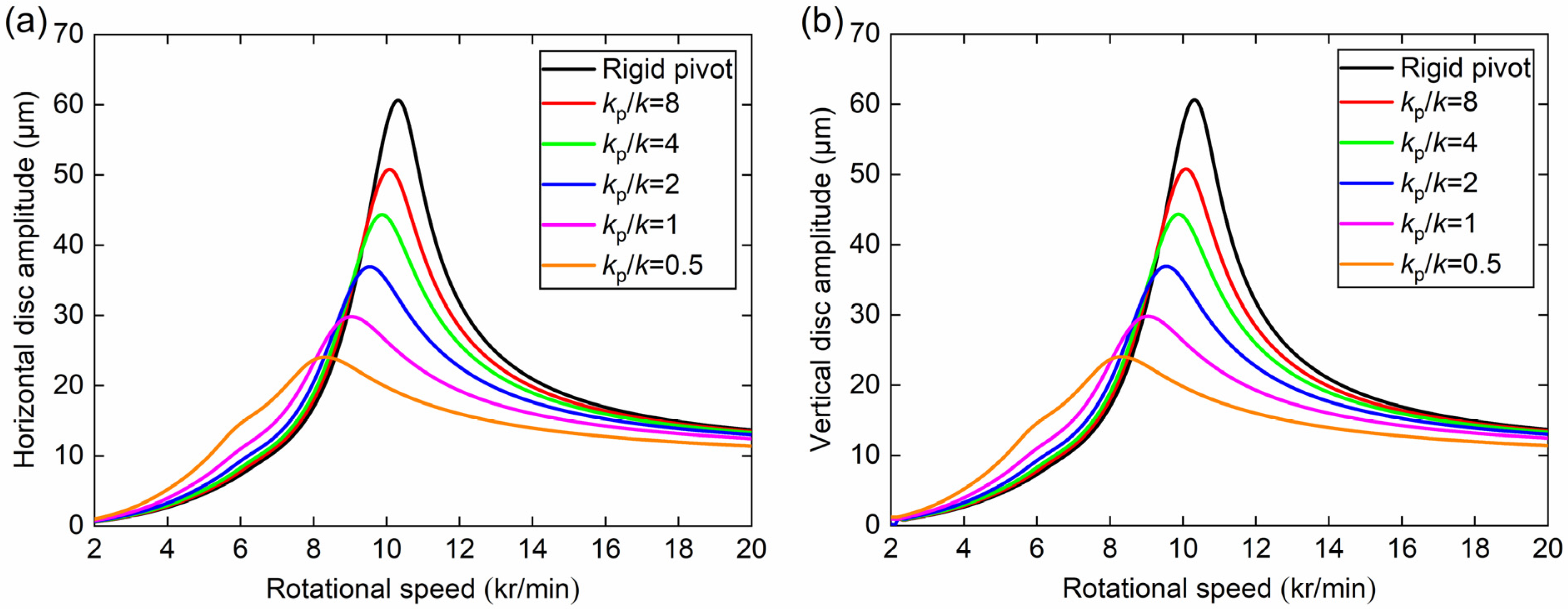

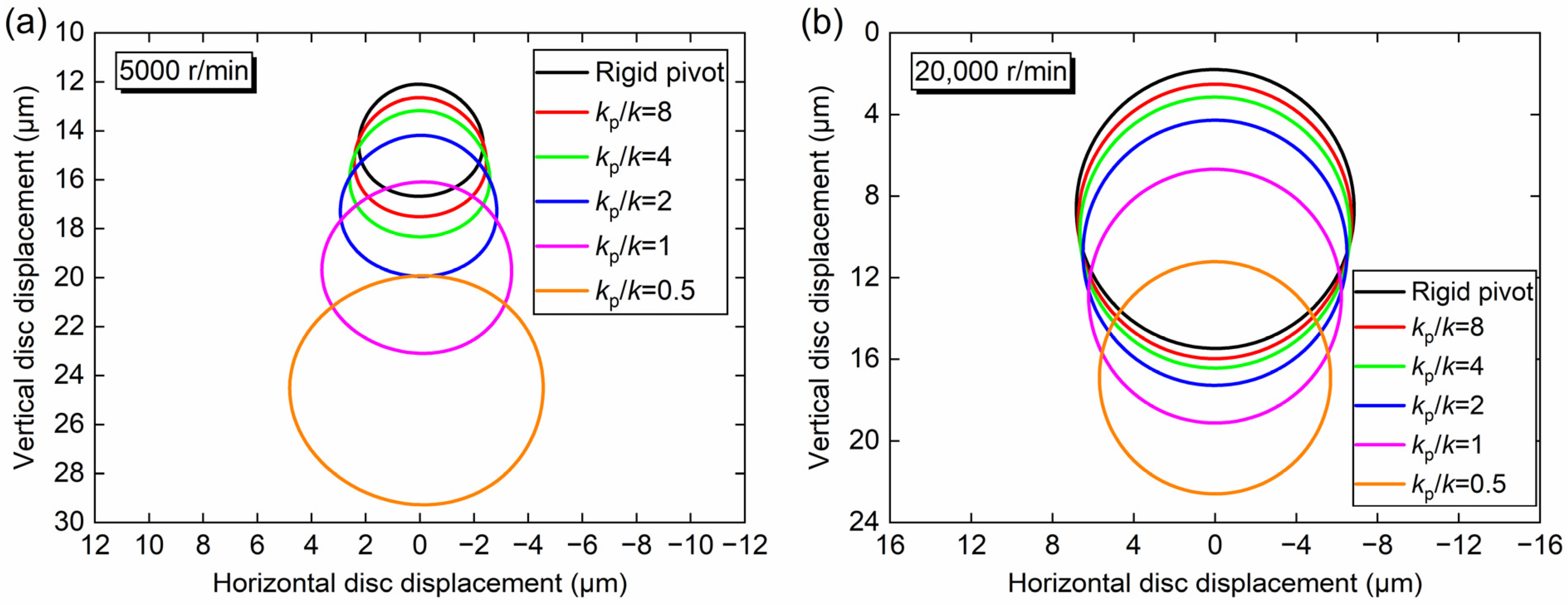


| Rotor System | Our Rotor Model | Zhang’s Results [35] |
|---|---|---|
| Rigid rotor | 23,672 r/min | 22,064 r/min |
| Flexible rotor | 1492 r/min | 1518 r/min |
| TPJB-Rotor System | Parameter | Value |
|---|---|---|
| TPJB | Journal radius (R) | 20 mm |
| Bearing length (L) | 30 mm | |
| Radial pad clearance (cp) | 0.04 mm | |
| Radial bearing clearance (cb) | 0.02 mm | |
| Pivot offset | 0.5 | |
| Pad thickness | 4 mm | |
| Pad density | 7850 kg·m−3 | |
| Pad arc angle | 80° | |
| Pivot position angle (βi) | 45°, 135°, 225°, 315° | |
| Fluid density (ρ) | 1221.9 kg·m−3 | |
| Fluid viscosity (μ) | 3.5324 × 10−4 Pa·s | |
| Pivot stiffness ratio (kp/k) | 0.5, 1, 2, 4, 8, rigid pivot | |
| Rotor | Shaft diameter (D) | 40 mm |
| Shaft length of span (l) | 499 mm | |
| Disc diameter (2Rd) | 178 mm | |
| Disc thickness (d) | 30 mm | |
| Unbalanced mass eccentricity (eu) | 5 μm | |
| Rotational speed (ω) | 2000~20,000 r/min | |
| Rotational acceleration (a) | 100 r/s2 | |
| Young’s modulus (E) | 206 GPa | |
| Rotor density (ρr) | 7850 kg·m−3 |
| Pivot Stiffness Ratio (kp/k) | System Damping Ratio |
|---|---|
| Rigid pivot | 0.085 |
| 8 | 0.103 |
| 4 | 0.119 |
| 2 | 0.146 |
| 1 | 0.195 |
| 0.5 | 0.281 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Jin, Y.; Niu, Q.; Qu, Y.; Yuan, X. Pivot Stiffness Effect on Transient Dynamic Characteristic of Tilting Pad Journal Bearing-Rotor System Passing through Critical Speed. Lubricants 2023, 11, 125. https://doi.org/10.3390/lubricants11030125
Jin Y, Niu Q, Qu Y, Yuan X. Pivot Stiffness Effect on Transient Dynamic Characteristic of Tilting Pad Journal Bearing-Rotor System Passing through Critical Speed. Lubricants. 2023; 11(3):125. https://doi.org/10.3390/lubricants11030125
Chicago/Turabian StyleJin, Yingze, Qiuli Niu, Yuanpeng Qu, and Xiaoyang Yuan. 2023. "Pivot Stiffness Effect on Transient Dynamic Characteristic of Tilting Pad Journal Bearing-Rotor System Passing through Critical Speed" Lubricants 11, no. 3: 125. https://doi.org/10.3390/lubricants11030125
APA StyleJin, Y., Niu, Q., Qu, Y., & Yuan, X. (2023). Pivot Stiffness Effect on Transient Dynamic Characteristic of Tilting Pad Journal Bearing-Rotor System Passing through Critical Speed. Lubricants, 11(3), 125. https://doi.org/10.3390/lubricants11030125





