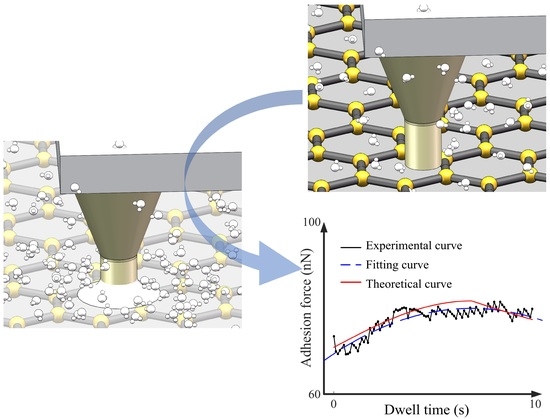
Study on Adhesion Force of Graphene under Cylinder–Plane Contact
Abstract
1. Introduction
2. Materials and Methods
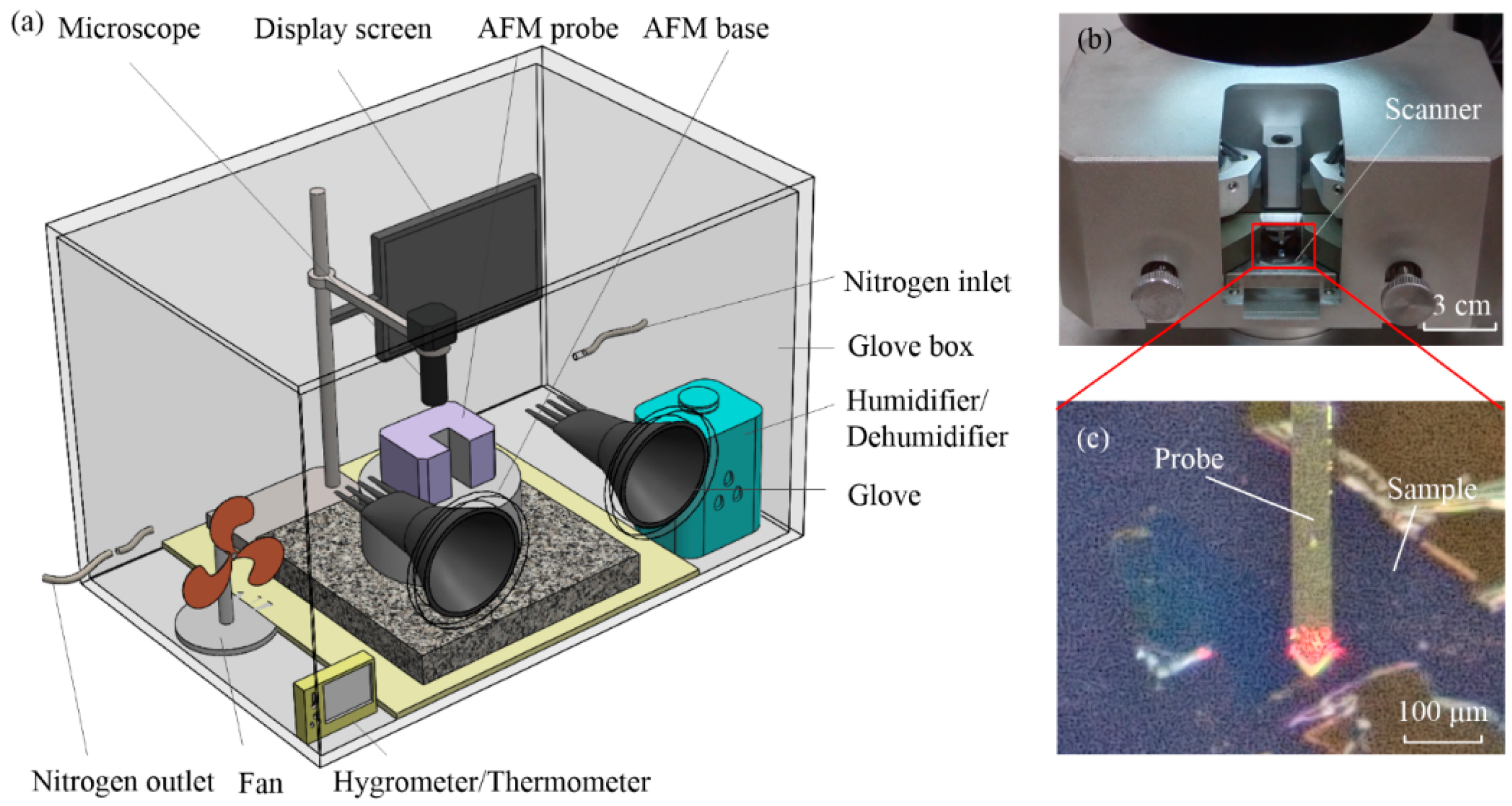
2.1. Preparation of Experiment
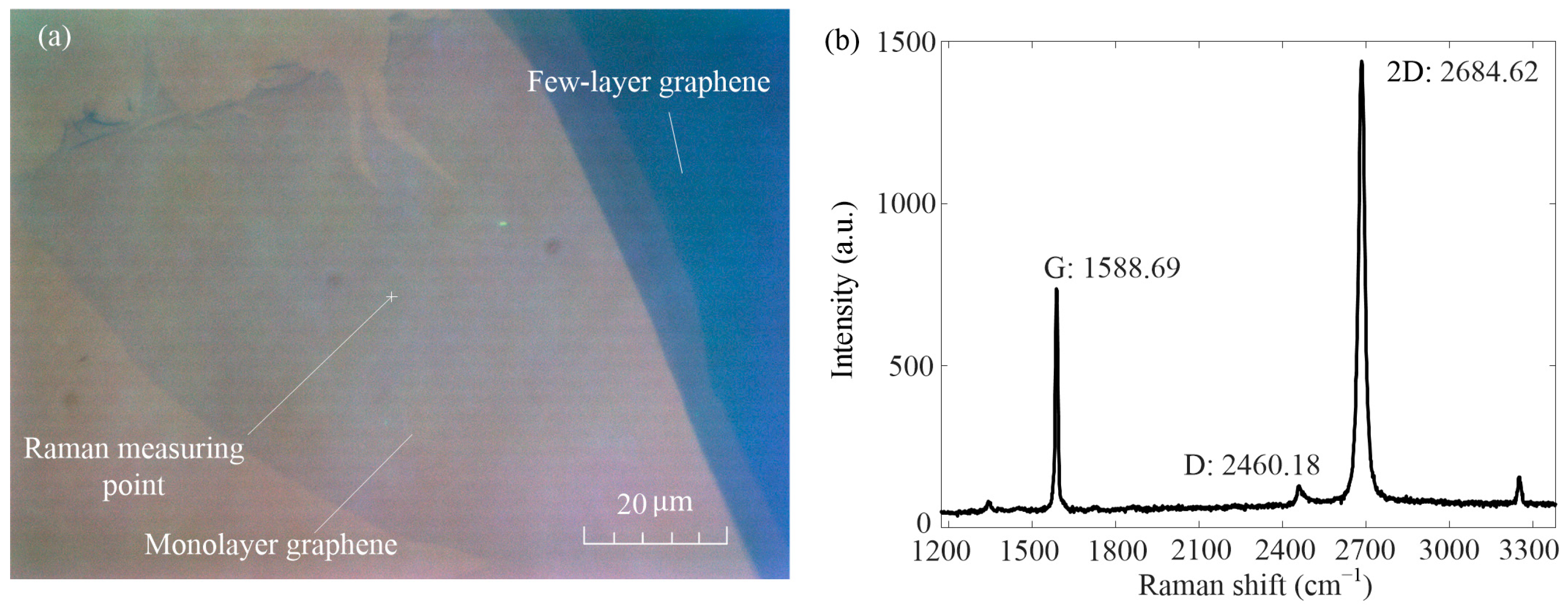
2.2. Characterization Methods
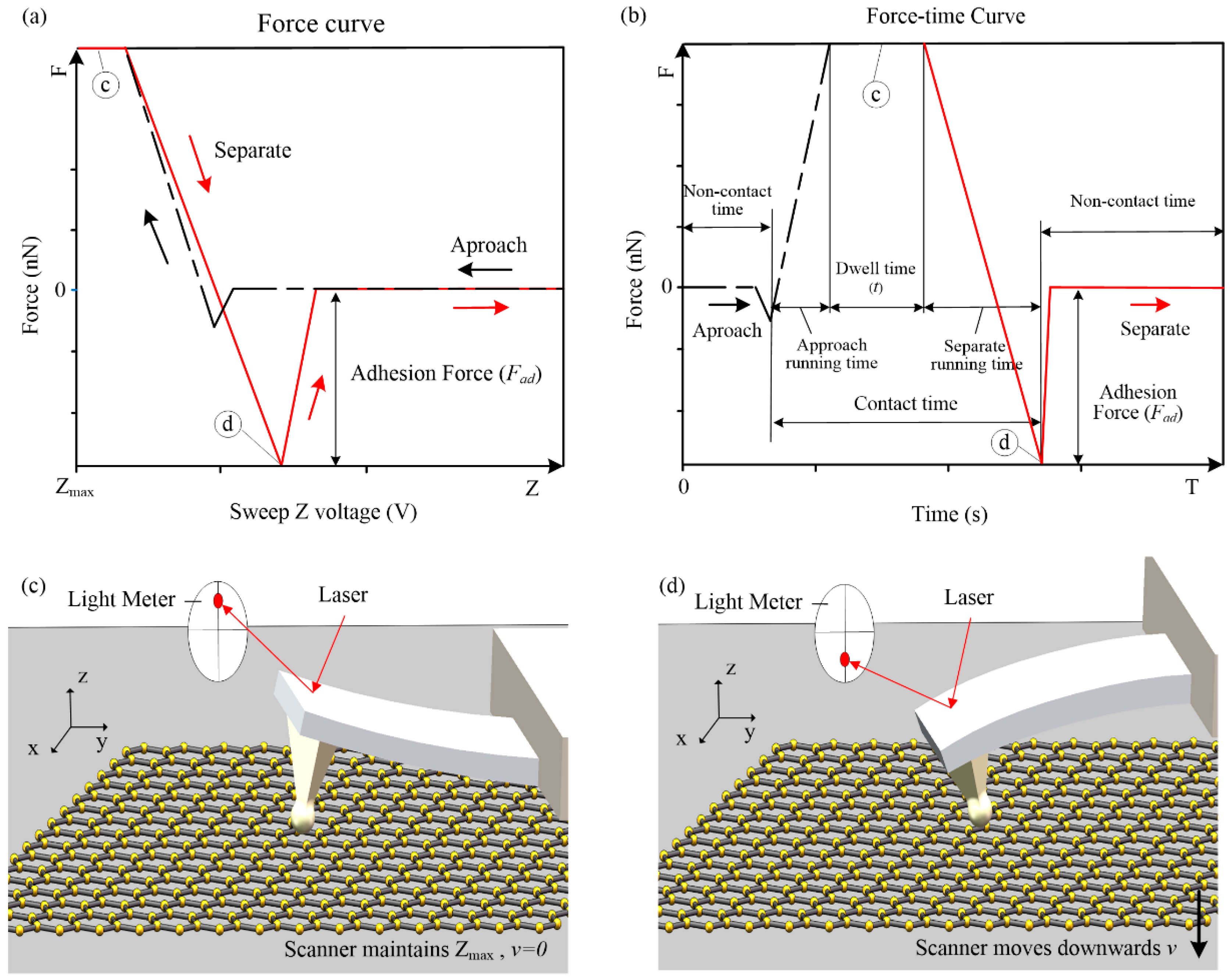
2.3. Measurement Method
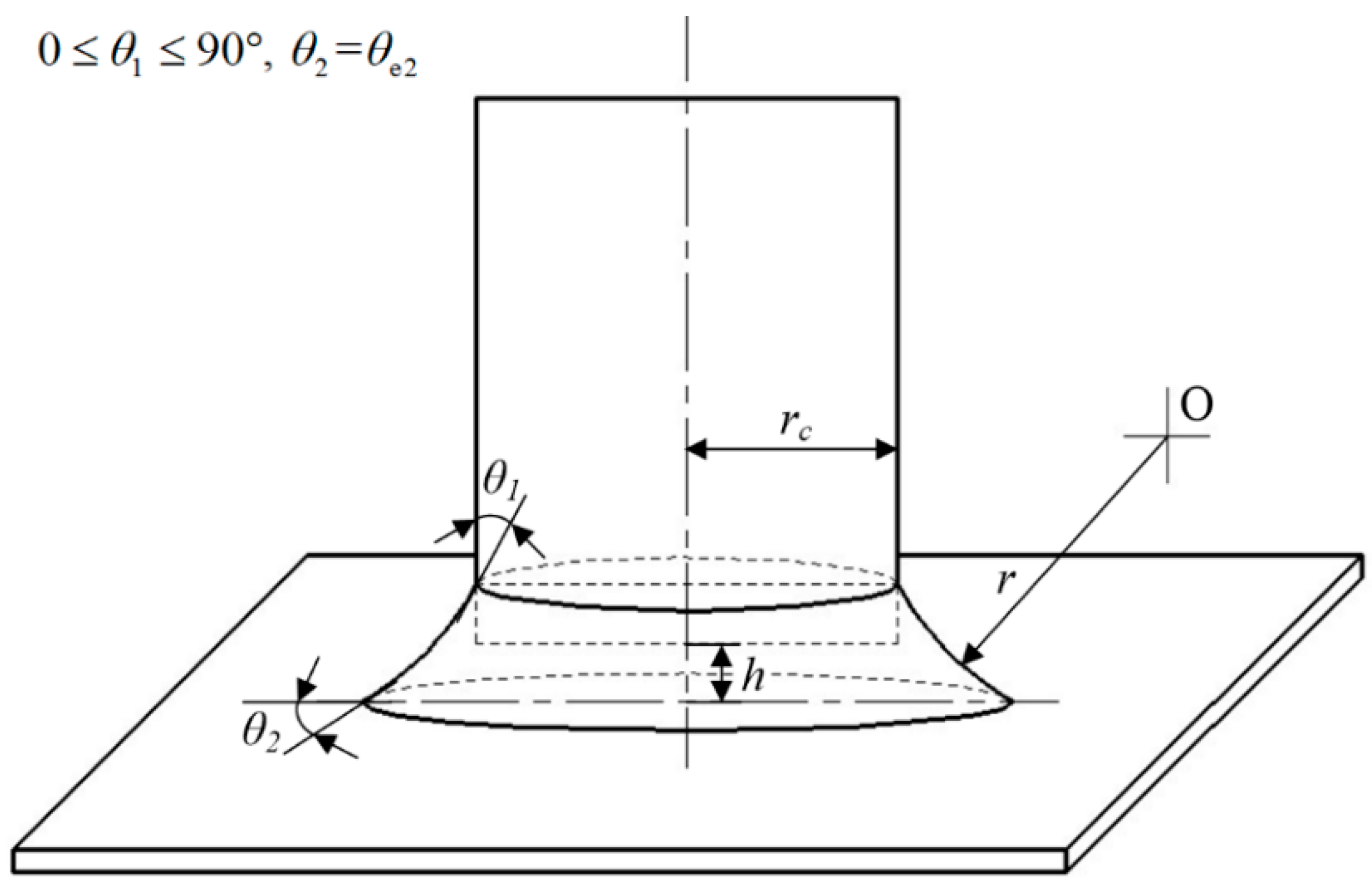
3. Theoretical Model
3.1. Change Process of the Meniscus
3.2. Van der Waals Force under Cylinder–Plane Contact
3.3. Theoretical Capillary Force under Cylinder–Plane Contact
4. Experimental and Theoretical Results
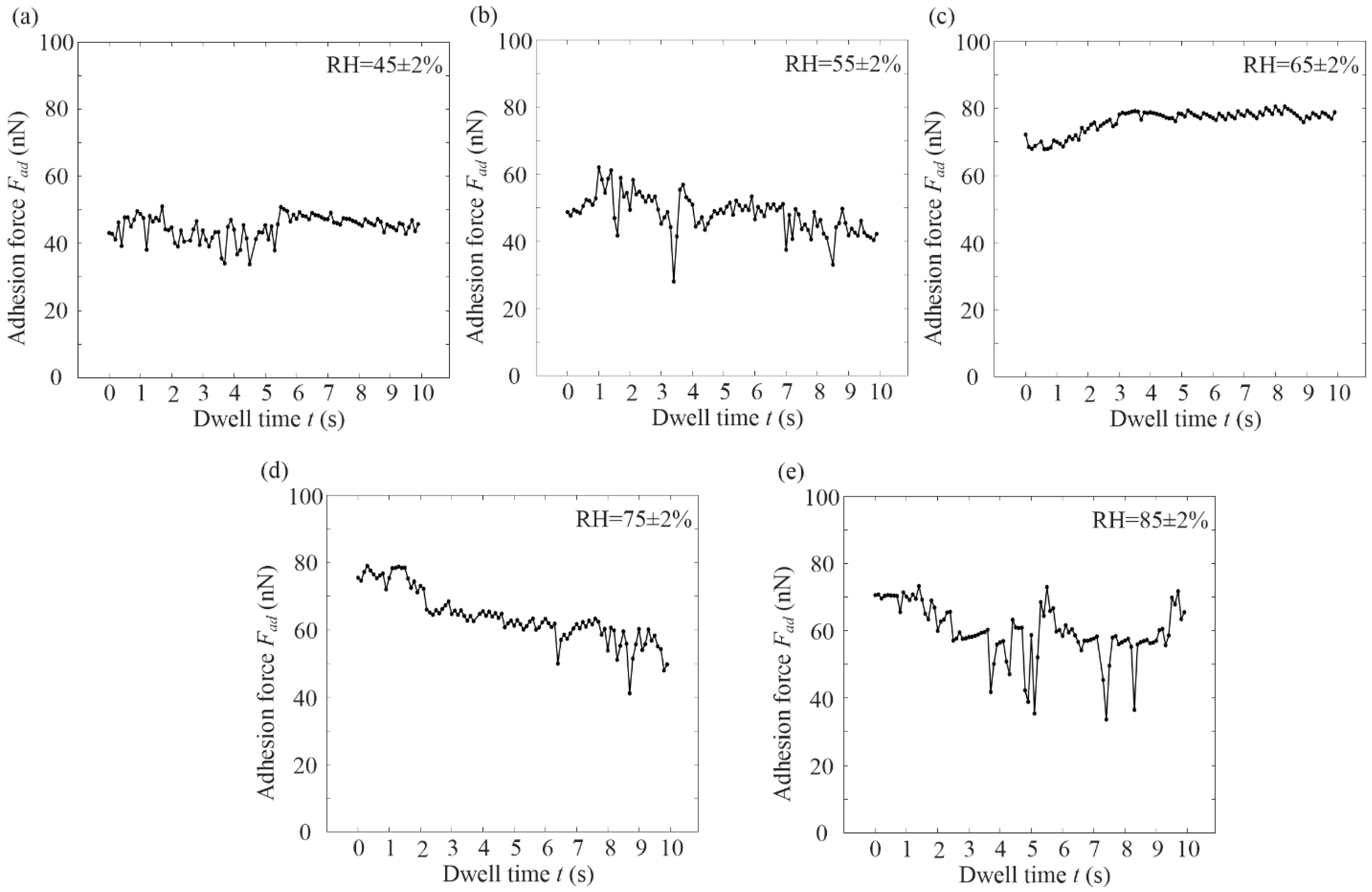
4.1. The Variation Rule of Graphene Adhesion Force with the Dwell Time under Cylinder–Plane Contact
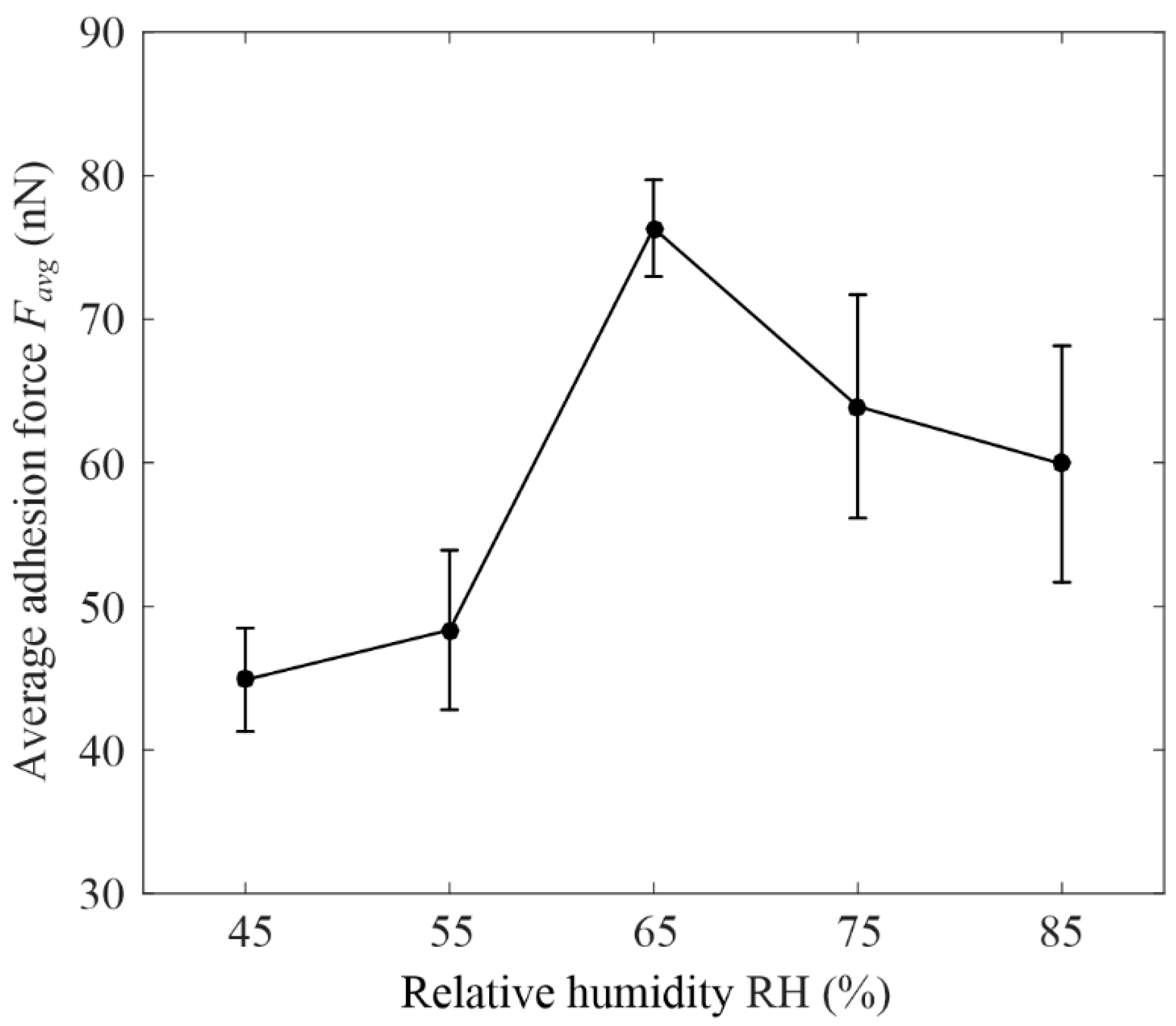
4.2. Variation Rule of Graphene Adhesion Force with the Relative Humidity under Cylinder–Plane Contact
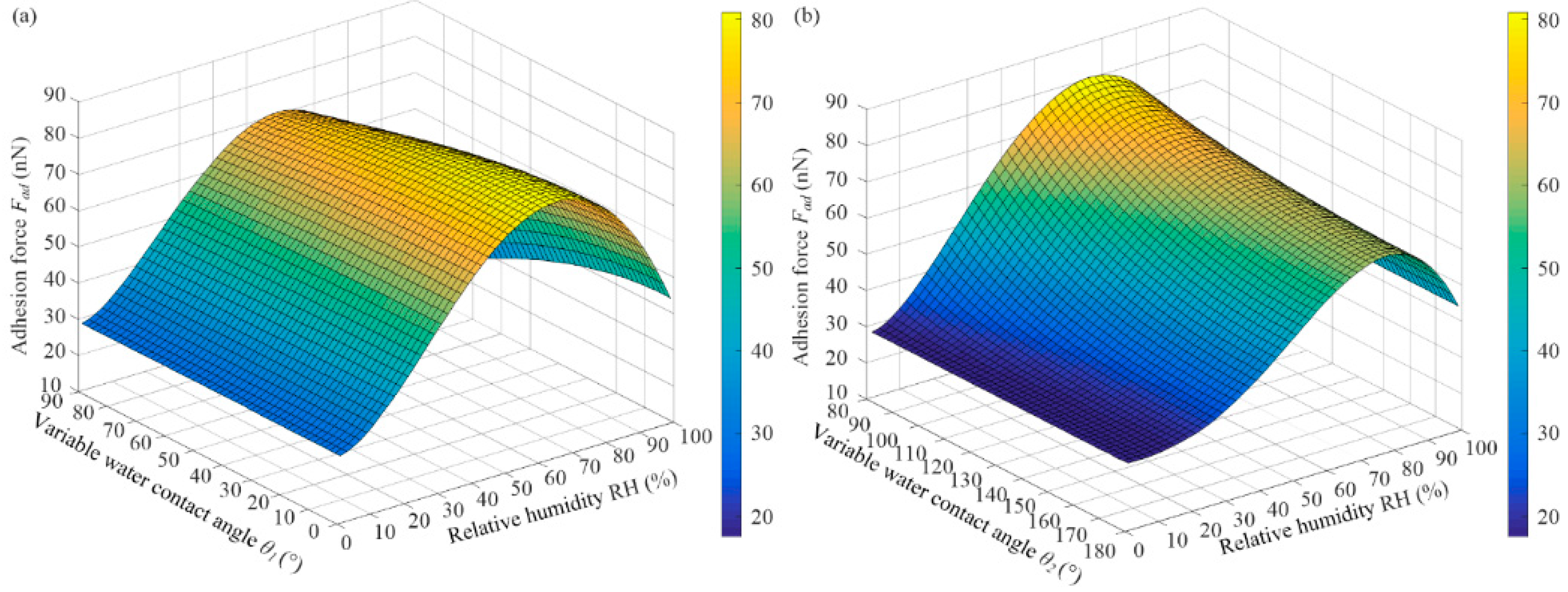
4.3. Theoretical Results
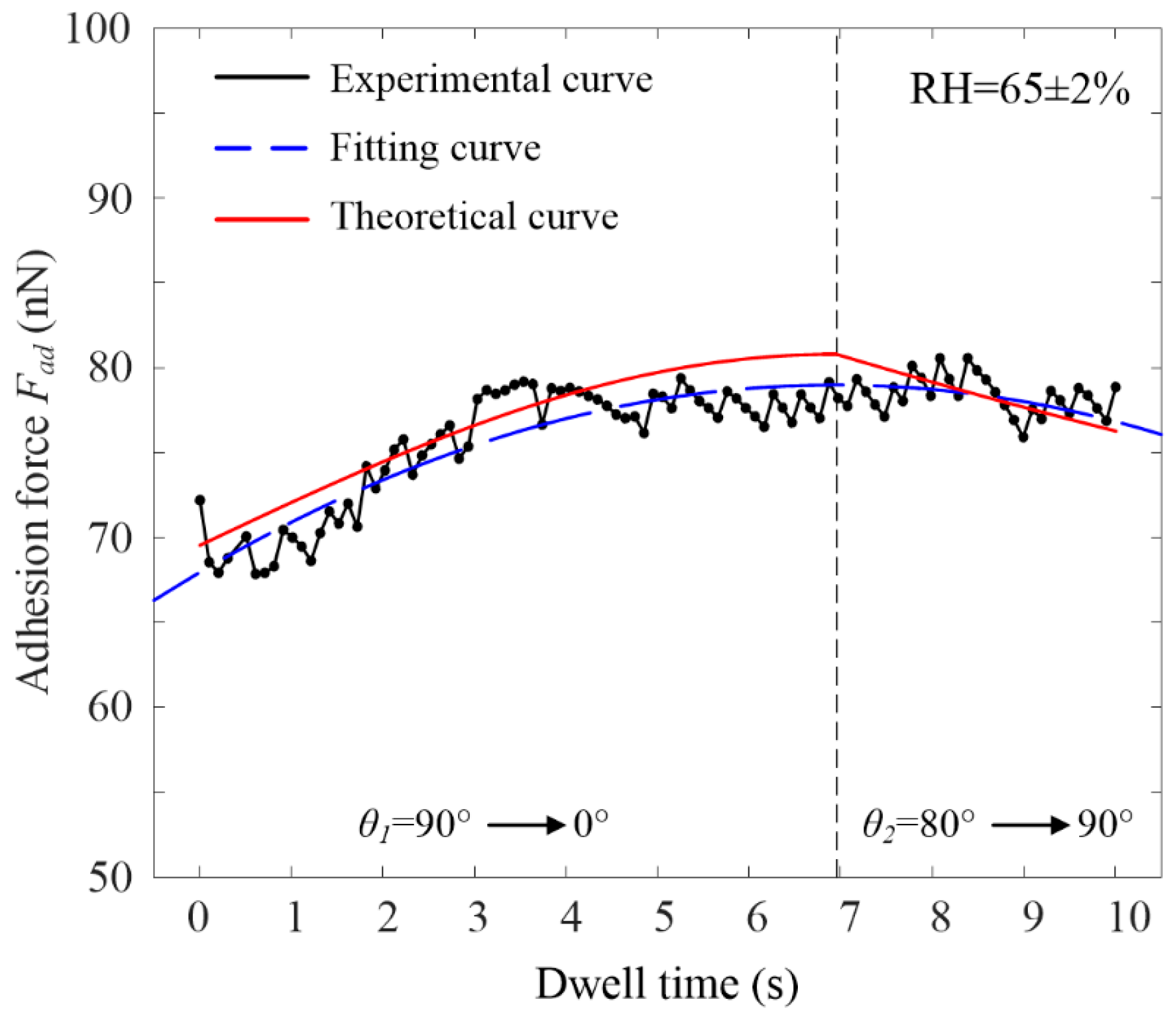
4.4. Comparison of Experimental and Theoretical Results
5. Conclusions
- At a low relative humidity (RH < 45%) under cylinder–plane contact, dwell time had little effect on graphene adhesion force. When RH was 55% and 65%, the adhesion force-versus-time curve had a maximum value and eventually tended to be stable. When RH was 75% and 85%, the adhesion force-versus-time curve decreased and tended to be stable.
- Meniscus cannot be formed at low RH. At RH > 55%, the meniscus condensed in the cylinder’s contact area. First, the VWCA of graphene was 80° and remained constant. The VWCA of the cylindrical probe decreased when the Kelvin circle moved horizontally outward until it was tangent to the cylinder. Finally, when the RH was high, a water film formed on the surface of the graphene, causing the VWCA of graphene to increase, resulting in a decrease in adhesion force.
- Theoretically, it is necessary to consider the influence of the roughness of the cylindrical probe and the graphene surface. Therefore, the contact area was considered to be a number of nanoscale cylinders in contact with the plane. Finally, combining the roughness calculation model of the vdW force with the modified Rumpf model, the adhesion force model of the cylinder–plane contact was obtained in terms of dwell time and VWCA. The maximum error between the theoretical curve and the experimental curve was only about 6%.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Novoselov, K.S.; Fal’ko, V.I.; Colombo, L.; Gellert, P.R.; Schwab, M.G.; Kim, K. A roadmap for graphene. Nature 2012, 490, 192–200. [Google Scholar] [CrossRef] [PubMed]
- Yu, W.; Sisi, L.; Haiyan, Y.; Jie, L. Progress in the functional modification of graphene/graphene oxide: A review. RSC Adv. 2020, 10, 15328–15345. [Google Scholar] [CrossRef]
- Xia, F.; Mueller, T.; Lin, Y.M.; Valdes-Garcia, A.; Avouris, P. Ultrafast graphene photodetector. Nat. Nanotechnol. 2009, 4, 839–843. [Google Scholar] [CrossRef]
- Markandan, K.; Zhang, Z.; Chin, J.; Cheah, K.H.; Tang, H.-B. Fabrication and preliminary testing of hydroxylammonium nitrate (HAN)-based ceramic microthruster for potential application of nanosatellites in constellation formation flying. Microsyst. Technol. 2019, 25, 4209–4217. [Google Scholar] [CrossRef]
- Lv, C.; Hu, C.; Luo, J.; Liu, S.; Qiao, Y.; Zhang, Z.; Song, J.; Shi, Y.; Cai, J.; Watanabe, A. Recent advances in graphene-based humidity sensors. Nanomaterials 2019, 9, 422. [Google Scholar] [CrossRef] [PubMed]
- Zhao, Y.-P.; Wang, L.S.; Yu, T.X. Mechanics of adhesion in MEMS—A review. J. Adhesion Sci. Technol. 2003, 17, 519–546. [Google Scholar] [CrossRef]
- Melios, C.; Giusca, C.E.; Panchal, V.; Kazakova, O. Water on graphene: Review of recent progress. 2D Mater. 2018, 5, 022001. [Google Scholar] [CrossRef]
- Pourzand, H.; Tabib-Azar, M. Graphene thickness dependent adhesion force and its correlation to surface roughness. Appl. Phys. Lett. 2014, 104, 171603. [Google Scholar] [CrossRef]
- Lai, T.M.; Zhu, T.; Chen, Y.G.; Guo, M.L. Different evolution behaviors of adhesion force with relative humidity at silica/silica and silica/graphene interfaces studied using atomic force microscopy. Langmuir 2021, 37, 13075–13084. [Google Scholar] [CrossRef]
- Zhu, J.; Deng, H.; Xue, W.; Wang, Q. Effect of low temperature oxygen plasma treatment on microstructure and adhesion force of graphene. Appl. Surf. Sci. 2018, 428, 941–947. [Google Scholar] [CrossRef]
- Wei, Z.; Sun, Y.; Ding, W.; Wang, Z. The formation of liquid bridge in different operating modes of AFM. Sci. China Phys. Mech. 2016, 59, 694611. [Google Scholar] [CrossRef]
- Butt, H.-J.; Cappella, B.; Kappl, M. Force measurements with the atomic force microscope: Technique, interpretation and applications. Surf. Sci. Rep. 2005, 59, 1–152. [Google Scholar] [CrossRef]
- Dos Santos Ferreira, O.; Gelinck, E.; de Graaf, D.; Fischer, H. Adhesion experiments using an AFM—Parameters of influence. Appl. Surf. Sci. 2010, 257, 48–55. [Google Scholar] [CrossRef]
- Xiao, X.D.; Qian, L.M. Investigation of humidity-dependent capillary force. Langmuir 2000, 16, 8153–8158. [Google Scholar] [CrossRef]
- Butt, H.J.; Farshchi-Tabrizi, M.; Kappl, M. Using capillary forces to determine the geometry of nanocontacts. J. Appl. Phys. 2006, 131, 184702. [Google Scholar] [CrossRef]
- Lai, T.; Li, P. Direct Evidence of a Radius of Collection Area for Thin Film Flow in Liquid Bridge Formation by Repeated Contacts Using AFM. Langmuir 2019, 35, 6585–6593. [Google Scholar] [CrossRef]
- Obata, K.J.; Motokado, T.; Saito, S.; Takahashi, K. A scheme for micro-manipulation based on capillary force. J. Fluid Mech. 2004, 498, 113–121. [Google Scholar] [CrossRef]
- Rabinovich, Y.I.; Singh, A.; Hahn, M.; Brown, S.; Moudgil, B. Kinetics of liquid annulus formation and capillary forces. Langmuir 2011, 27, 13514–13523. [Google Scholar] [CrossRef]
- Weeks, B.L.; Vaughn, M.W.; Deyoreo, J.J. Direct imaging of meniscus formation in atomic force microscopy using environmental scanning electron microscopy. Langmuir 2005, 21, 8096–8098. [Google Scholar] [CrossRef]
- Qian, J.; Gao, H. Scaling effects of wet adhesion in biological attachment systems. Acta Biomater. 2006, 2, 51–58. [Google Scholar] [CrossRef]
- Yang, Q.; Sun, P.Z.; Fumagalli, L.; Stebunov, Y.V.; Haigh, S.J.; Zhou, Z.W.; Grigorieva, I.V.; Wang, F.C.; Geim, A.K. Capillary condensation under atomic-scale confinement. Nature 2020, 588, 250–253. [Google Scholar] [CrossRef]
- Lai, T.; Huang, P. Study on microscale adhesion between solid surfaces with scanning probe. Sci. China Technol. Sci. 2013, 56, 2934–2952. [Google Scholar] [CrossRef]
- Çolak, A.; Wormeester, H.; Zandvliet, H.J.W.; Poelsema, B. Surface adhesion and its dependence on surface roughness and humidity measured with a flat tip. Appl. Surf. Sci. 2012, 258, 6938–6942. [Google Scholar] [CrossRef]
- Boucher, E.A.; Evans, M.J.B.; McGarry, S. Capillary phenomena. XX. Fluid bridges between horizontal solid plates in a gravitational field. J. Colloid Interf. Sci. 1982, 89, 154–165. [Google Scholar] [CrossRef]
- Tselishchev, Y.G.; Val’Tsifer, V.A. Influence of the type of contact between particles joined by a liquid bridge on the capillary cohesive forces. Colloid J. 2003, 65, 385–389. [Google Scholar] [CrossRef]
- De Souza, E.J.; Brinkmann, M.; Mohrdieck, C.; Crosby, A.; Arzt, E. Capillary Forces between Chemically Different Substrates. Langmuir 2008, 24, 10161–10168. [Google Scholar] [CrossRef]
- De Souza, E.J.; Brinkmann, M.; Mohrdieck, C.; Arzt, E. Enhancement of Capillary Forces by Multiple Liquid Bridges. Langmuir 2008, 24, 8813–8820. [Google Scholar] [CrossRef] [PubMed]
- Butt, H.J.; Kappl, M. Normal capillary forces. Adv. Colloid Interfac. 2009, 146, 48–60. [Google Scholar] [CrossRef] [PubMed]
- Farshchi-Tabrizia, M.; Kappl, M.; Butt, H.-J. Influence of humidity on adhesion: An atomic force microscope study. J. Adhes. Sci. Technol. 2008, 22, 181–203. [Google Scholar] [CrossRef]
- van Spengen, W.M. The Electrostatic Actuation of MEMS with High Voltage Amplifiers. Falco Systems Application Note: 2018. Available online: http://www.falco-systems.com/the_electrostatic_actuation_of_MEMS_with_high_voltage_amplifiers.html (accessed on 1 October 2022).
- Spengen, W.M.V.; Puers, R.; Wolf, I.D. A physical model to predict stiction in MEMS. J. Micromech. Microeng. 2002, 12, 702–713. [Google Scholar] [CrossRef]
- Wang, L.-F.; Huang, Q.-A.; Han, L. RF MEMS Switch. In Microbial Toxins; Springer: Singapore, 2017; pp. 1–38. [Google Scholar] [CrossRef]
- Gkouzou, A.; Kokorian, J.; Janssen, G.C.A.M.; van Spengen, W.M. Controlling adhesion between multi-asperity contacting surfaces in MEMS devices by local heating. J. Micromech. Microeng. 2016, 26, 095020. [Google Scholar] [CrossRef]
- Zang, X.; Zhou, Q.; Chang, J.; Liu, Y.; Lin, L. Graphene and carbon nanotube (CNT) in MEMS/NEMS applications. Microelectron. Eng. 2015, 132, 192–206. [Google Scholar] [CrossRef]
- Fan, X.; Forsberg, F.; Smith, A.D.; Schröder, S.; Wagner, S.; Rödjegård, H.; Fischer, A.C.; Östling, M.; Lemme, M.C.; Niklaus, F. Graphene ribbons with suspended masses as transducers in ultra-small nanoelectromechanical accelerometers. Nat. Electron. 2019, 2, 394–404. [Google Scholar] [CrossRef]
- Shi, K.; Hu, M.F.; Huang, P. Influences of relative humidity and dwell time on silica/graphene adhesion force of a cone-plane contact. Langmuir 2022, 38, 12432–12440. [Google Scholar] [CrossRef] [PubMed]
- Lai, T.M.; Chen, M.; Zhang, Y.T. Contact time dependence of adhesion force at silica/silica interface on AFM: Influence of relative humidity and contact history. Appl. Surf. Sci. 2022, 600, 154175. [Google Scholar] [CrossRef]
- Lai, T.; Guo, M.; Chen, Y.; Zhu, T. Shielding effect of hydrophobic graphene on contact time dependence of adhesion force and instability with repeated contacts at silica/silica interface. J. Adhesion 2022, 99, 449–472. [Google Scholar] [CrossRef]
- Sirghi, L. Transport mechanisms in capillary condensation of water at a single-asperity nanoscopic contact. Langmuir 2012, 28, 2558–2566. [Google Scholar] [CrossRef]
- Malard, L.M.; Pimenta, M.A.; Dresselhaus, G.; Dresselhaus, M.S. Raman spectroscopy in graphene. Phys. Rep. 2009, 473, 51–87. [Google Scholar] [CrossRef]
- Ferrari, A.C.; Meyer, J.C.; Scardaci, V.; Casiraghi, C.; Lazzeri, M.; Mauri, F.; Piscanec, S.; Jiang, D.; Novoselov, K.S.; Roth, S.; et al. Raman spectrum of graphene and graphene layers. Phys. Rev. Lett. 2006, 97, 187401. [Google Scholar] [CrossRef]
- Wei, Z.; Zhao, Y.P. Growth of liquid bridge in AFM. J. Phy. D Appl. Phys. 2007, 40, 4368–4375. [Google Scholar] [CrossRef]
- Tang, B.Y.; Tang, C.; Chen, L.; Xiao, C.; Rosenkranz, A.; Qian, L.M. Nanoscopic humidity-dependent adhesion behaviors of 2D materials. Appl. Surf. Sci. 2022, 572, 151394. [Google Scholar] [CrossRef]
- Bazrafshan, M.; de Rooij, M.B.; Schipper, D.J. Adhesive force model at a rough interface in the presence of thin water films: The role of relative humidity. Int. J. Mech. Sci. 2018, 140, 471–485. [Google Scholar] [CrossRef]
- Sun, Y.; Li, Y.; Dong, X.; Bu, X.; Drelich, J.W. Spreading and adhesion forces for water droplets on methylated glass surfaces. Colloids Surf. A Physicochem. Eng. Asp. 2020, 591, 124562. [Google Scholar] [CrossRef]
- Uhlig, M.R.; Garcia, R. In Situ Atomic-Scale Imaging of Interfacial Water under 3D Nanoscale Confinement. Nano Lett. 2021, 21, 5593–5598. [Google Scholar] [CrossRef] [PubMed]
- Chiou, Y.C.; Olukan, T.A.; Almahri, M.A.; Apostoleris, H.; Chiu, C.H.; Lai, C.Y.; Lu, J.Y.; Santos, S.; Almansouri, I.; Chiesa, M. Direct measurement of the magnitude of the van der waals interaction of single and multilayer graphene. Langmuir 2018, 34, 12335–12343. [Google Scholar] [CrossRef]
- Sedin, D.L.; Rowlen, K.L. Adhesion forces measured by atomic force microscopy in humid air. Anal. Chem. 2000, 72, 2183–2189. [Google Scholar] [CrossRef]
- Harrison, A.J.; Corti, D.S.; Beaudoin, S.P. Capillary forces in nanoparticle adhesion: A review of AFM methods. Part. Sci. Technol. 2015, 33, 526–538. [Google Scholar] [CrossRef]
- Rabinovich, Y.I.; Adler, J.J.; Ata, A.; Singh, R.K.; Moudgil, B.M. Adhesion between Nanoscale Rough Surfaces. J. Colloid Interf. Sci. 2000, 232, 10–16. [Google Scholar] [CrossRef]



Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Shi, K.; Hu, M.; Huang, P. Study on Adhesion Force of Graphene under Cylinder–Plane Contact. Lubricants 2023, 11, 126. https://doi.org/10.3390/lubricants11030126
Shi K, Hu M, Huang P. Study on Adhesion Force of Graphene under Cylinder–Plane Contact. Lubricants. 2023; 11(3):126. https://doi.org/10.3390/lubricants11030126
Chicago/Turabian StyleShi, Kai, Manfeng Hu, and Ping Huang. 2023. "Study on Adhesion Force of Graphene under Cylinder–Plane Contact" Lubricants 11, no. 3: 126. https://doi.org/10.3390/lubricants11030126
APA StyleShi, K., Hu, M., & Huang, P. (2023). Study on Adhesion Force of Graphene under Cylinder–Plane Contact. Lubricants, 11(3), 126. https://doi.org/10.3390/lubricants11030126