Elastohydrodynamic Lubrication of Compliant Circular Contacts near Glass-Transition Temperature
Abstract
1. Introduction
2. Materials and Methods
2.1. Material of Experimental Specimens
2.2. Lubricant Properties
2.3. Experimental Apparatus
2.4. Experimental Conditions and Methods
3. Results
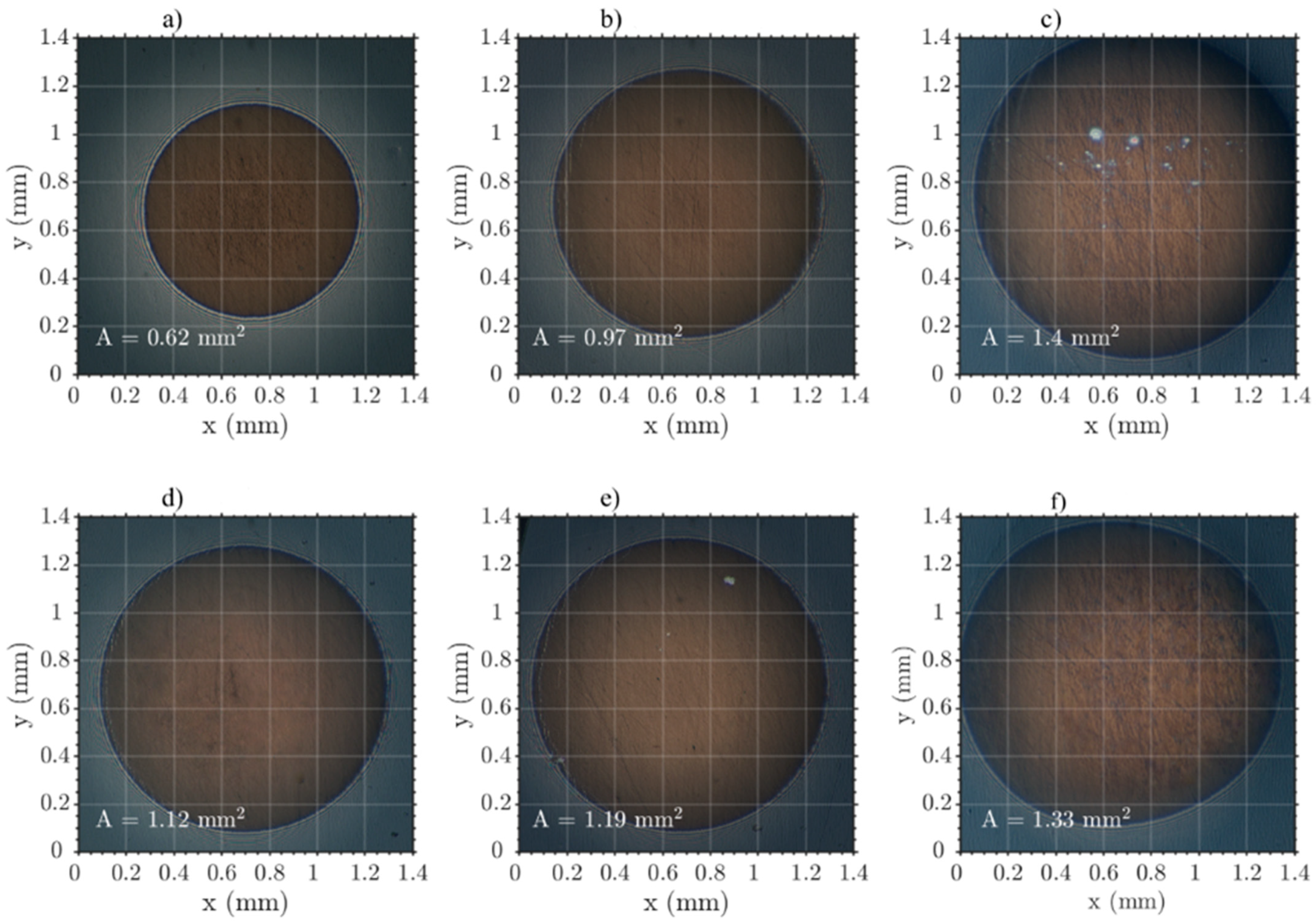
3.1. Static Contacts—Change of Contact Geometry
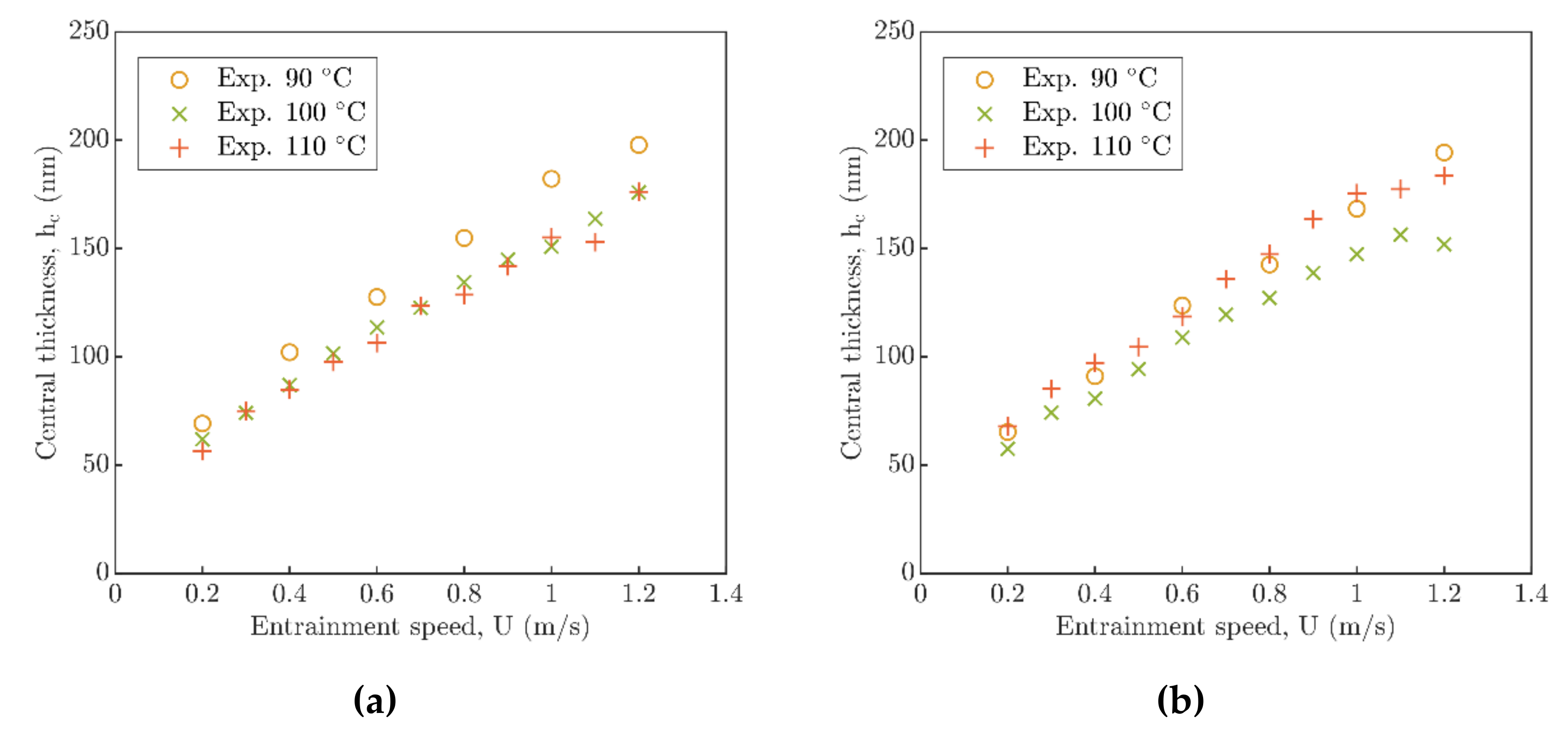
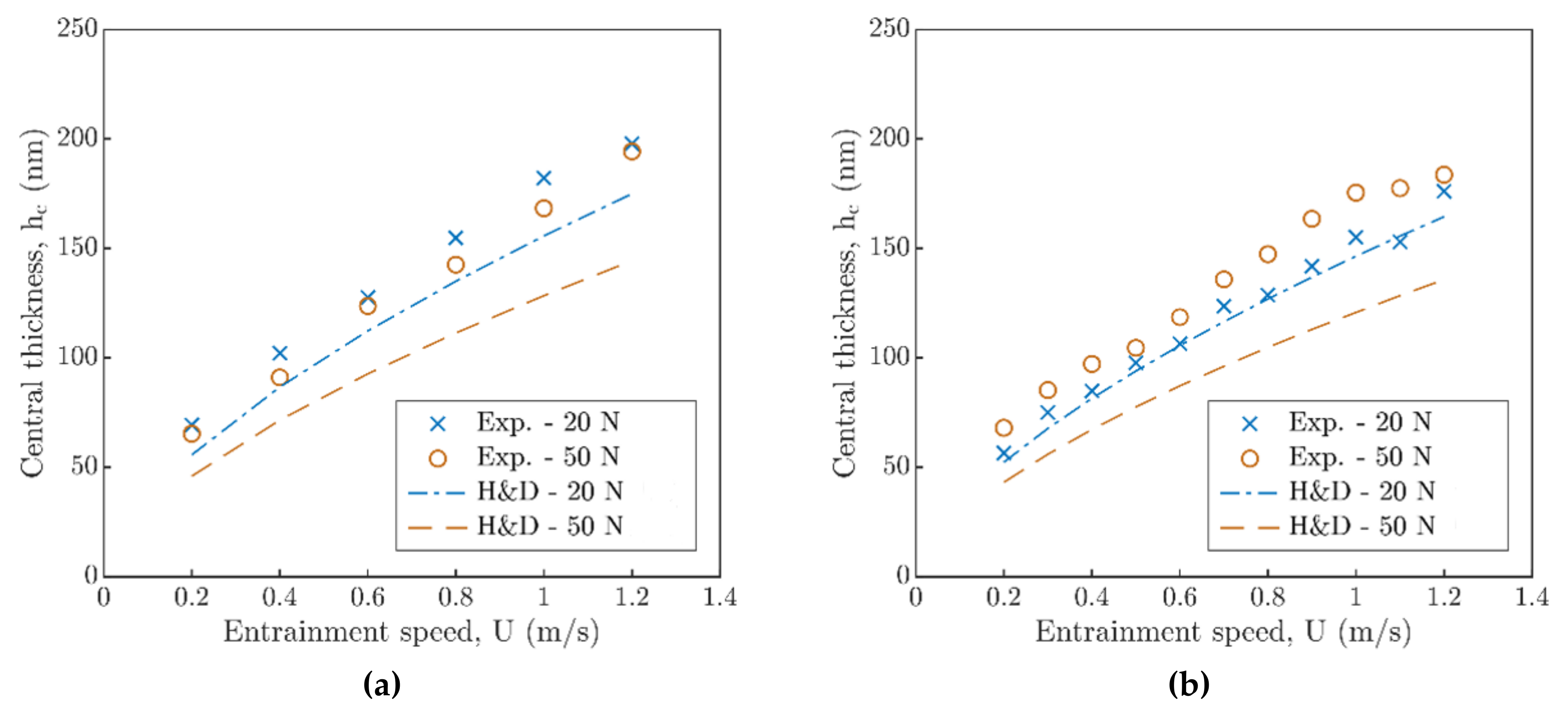
3.2. Film Thickness of Lubricant
3.3. Running Contact—Change of Contact Geometry
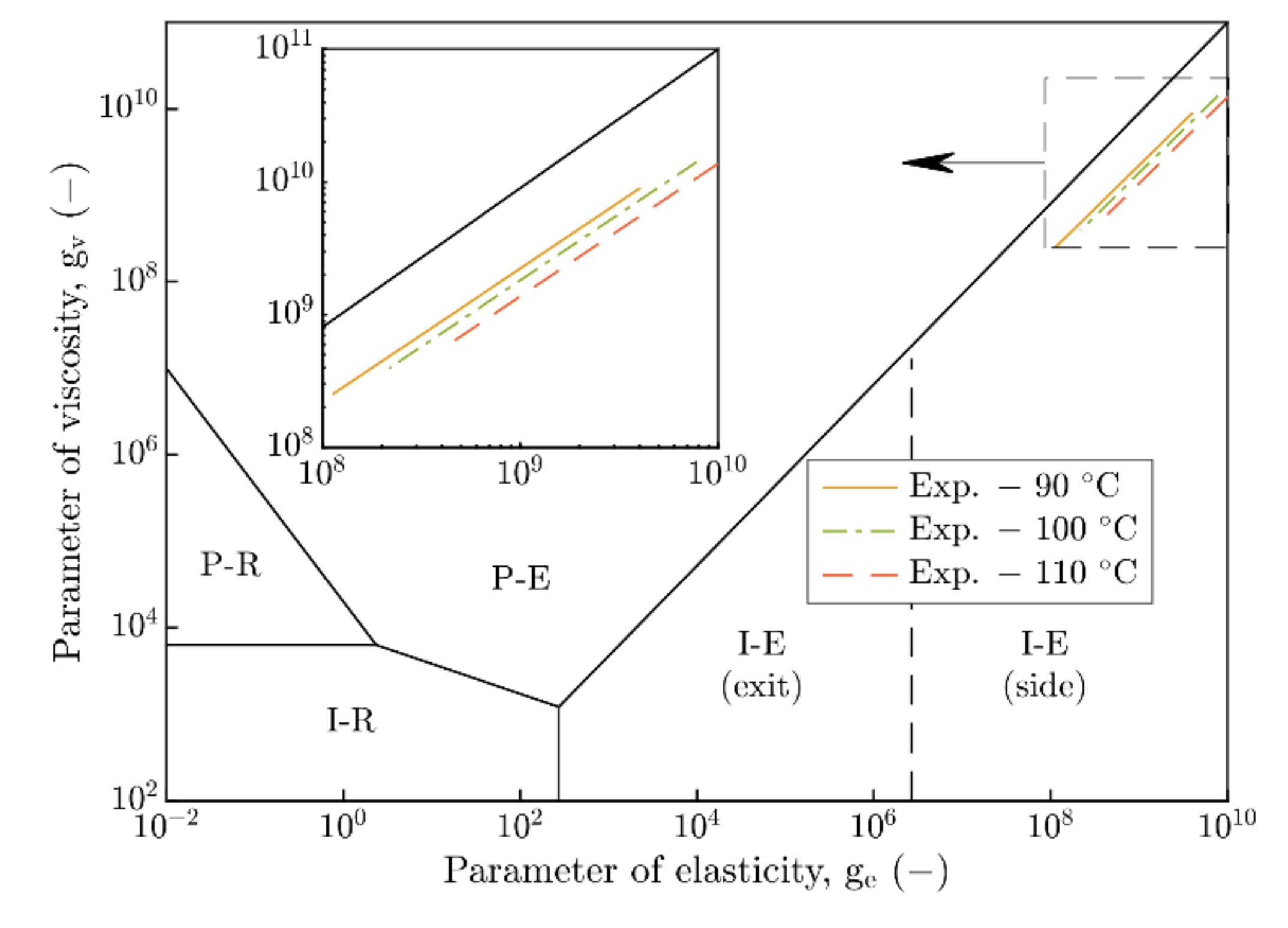
4. Discussion
5. Conclusions
- No difference was observed in the change of the position of the minimum thickness hm suggested by Hooke [20] as well as transition of hm from the exit to the inlet of the contact with an increase of entrainment speed as a result of phenomena of viscoelastic behavior of PMMA described by Putignano et al. [43];
- Above the glass-transition temperature Tg of PMMA, central film thickness increases with load W and, at the same time, it differs even more from prediction models [19,20] with increase of entrainment speed U. The possible explanation of this phenomenon is the transition from the linear to nonlinear region of viscoelasticity according to the observed step change in ellipticity k and contact area A above Tg;
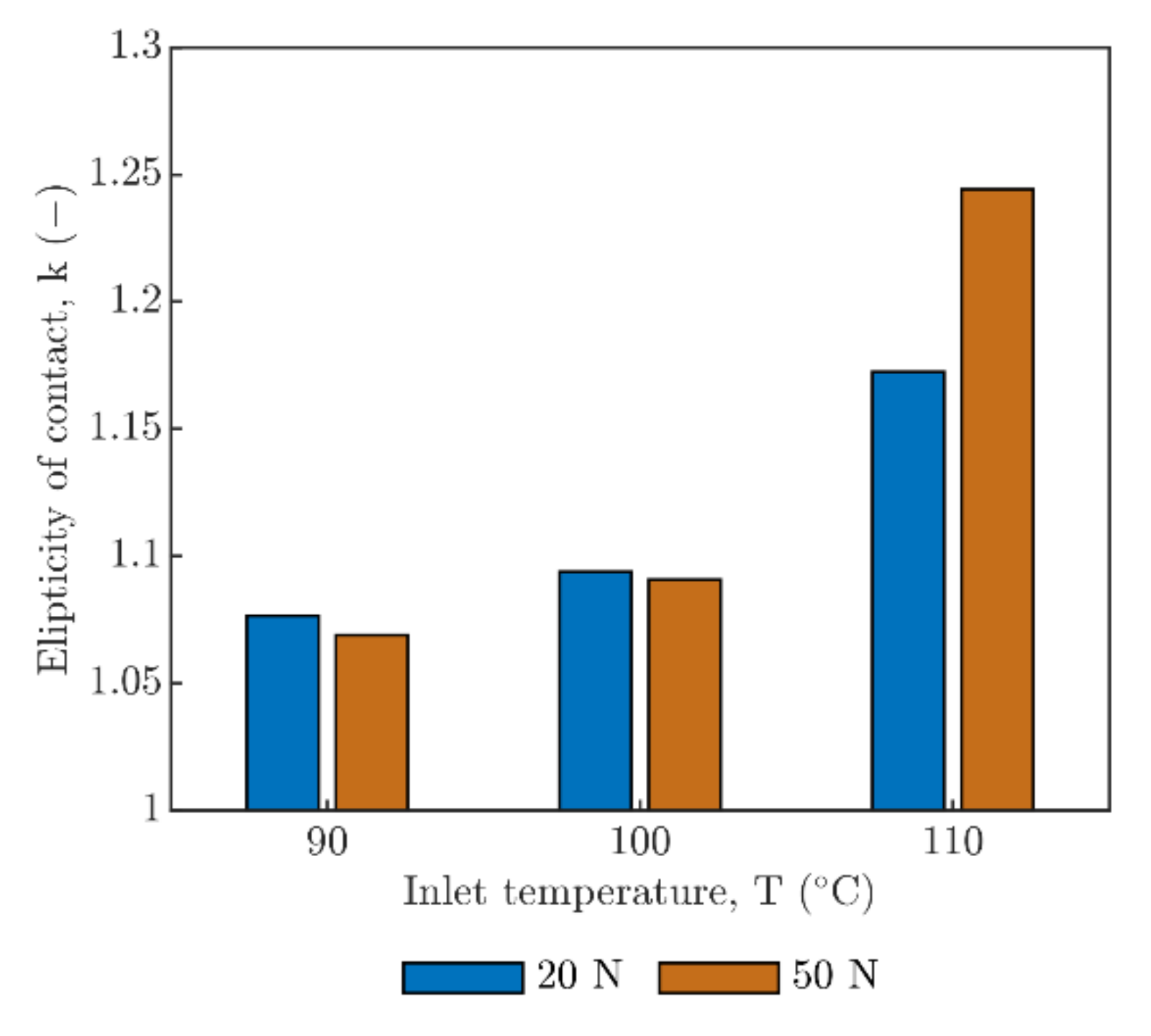
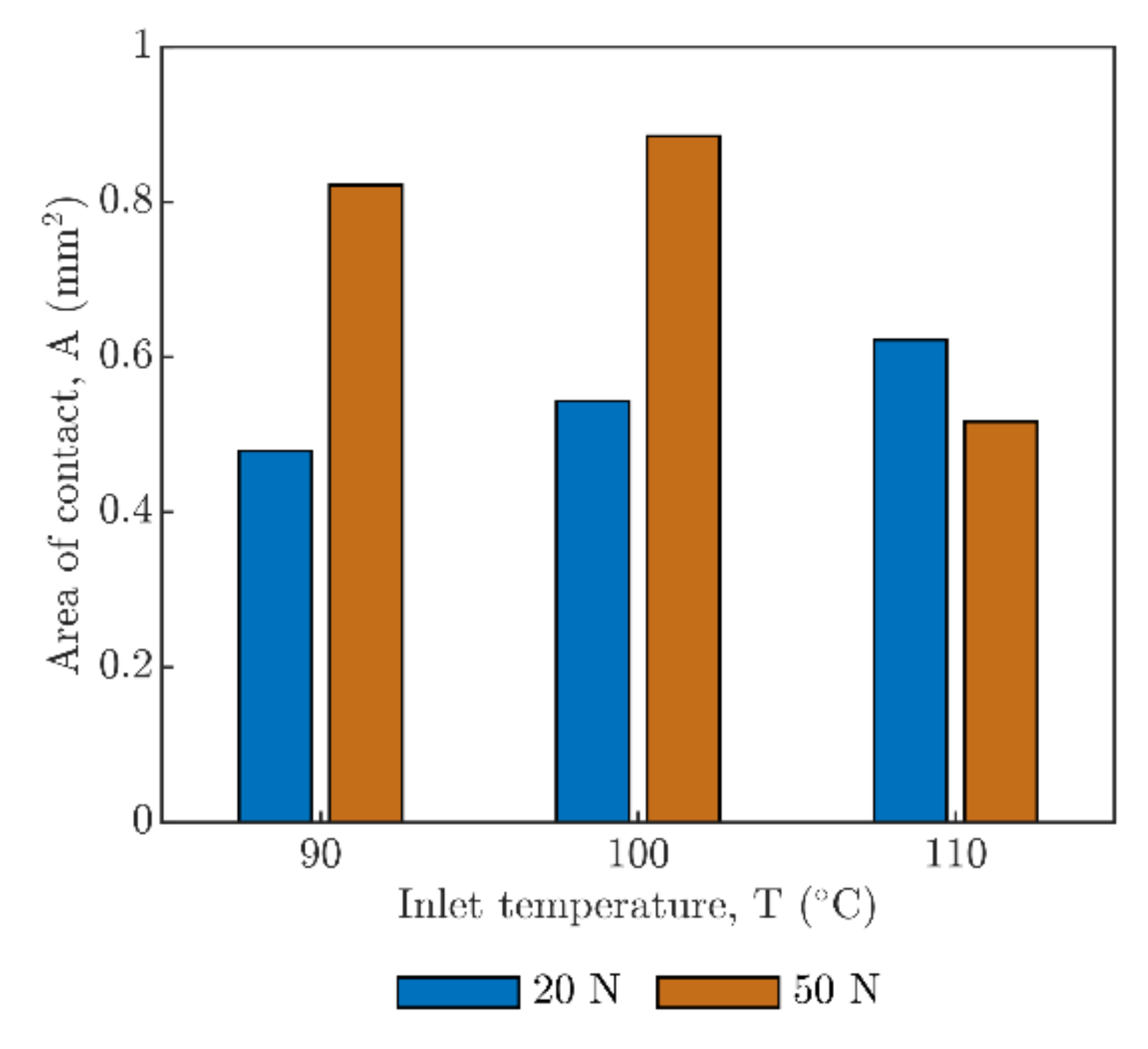
- Ellipticity k and contact area A manifested a gradual increase hand by hand with increase of inlet temperature T and load W up to Tg. Above Tg, viscoelastic deformation occurs, where the contact becomes wide elliptical with k > 1, and to the reduction of contact area A with increasing load. The former could be attributed to the inlet temperature involved in the thermal softening of polymer. The latter could be described by load dependence where, after exceeding the PMMA yield strength, the material exhibits strain softening/hardening depending on temperature, pressure (load), and strain rate [43,44,45,46,47]. However, deviations of contact shape (ellipticity k and contact area A) are probably a result of combination of both effects.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Nomenclature
| A | Area of contact, (mm2) | |
| a | Semi-contact radius, (µm) | a3 = 3WR′/2E′ |
| CI, CII | Light beam I and II, (-) | |
| E | Young’s modulus, (GPa) | |
| E1, E2 | Young’s modulus of solid 1 and 2, (GPa) | |
| EL | Elastic part of E (Storage modulus), (MPa) | |
| ES | Viscous part of E (Loss modulus), (MPa) | |
| E′ | Reduced Young’s modulus, (GPa) | 2/E′ = (1 − υ12)/E1 + (1 − υ22)/E2 |
| Dimensionless parameter of material, (-) | ||
| ge | Parameter of elasticity, (-) | |
| gv | Parameter of viscosity, (-) | |
| hm | Minimum film thickness, (nm) | |
| hm,exit | Minimum film thickness at contact exit, (nm) | |
| hm,side | Minimum film thickness on side lobes, (nm) | |
| hc | Central film thickness, (nm) | |
| hcp | Predicted central film thickness, (nm) | |
| k | Parameter of ellipticity, (-) | |
| P | Parameter of pressure, (-) | |
| p0 | Maximum contact pressure, (MPa) | p0 = 3W/2πa2 |
| R1, R2 | Curvature radius of solid 1 and 2, (mm) | |
| R′ | Reduced curvature radius, (mm) | 1/R′ = 1/R1 + 1/R2 |
| Rq | Root mean square roughness (RMS), (µm) | |
| Rt/Re | Parameter of ellipticity by Greenwood, (-) | |
| S | Speed parameter, (-) | |
| T | Inlet temperature, (°C) | |
| Tg | Glass-transition temperature, (°C) | |
| U | Entrainment speed, (m/s) | U = (U1 + U2)/2 |
| Dimensionless speed parameter, (-) | ||
| U1, U2 | Surface speed of solid 1 and 2, (m/s) | |
| W | Normal load, (N) | |
| Dimensionless load parameter, (-) | ||
| α | Pressure-viscosity coefficient, (GPa−1) | |
| η0 | Dynamic viscosity at atm., (Pa s) | |
| υ1, υ2 | Poisson’s ratio of solid 1 and 2, (-) | |
| υ40 | Kinematic viscosity at 40 °C, (mm2/s) | |
| υ100 | Kinematic viscosity at 100 °C, (mm2/s) | |
| ρ15 | Density of lubricant at 15 °C, (kg/m3) | |
| φ | Phase shift, (-) |
Appendix A
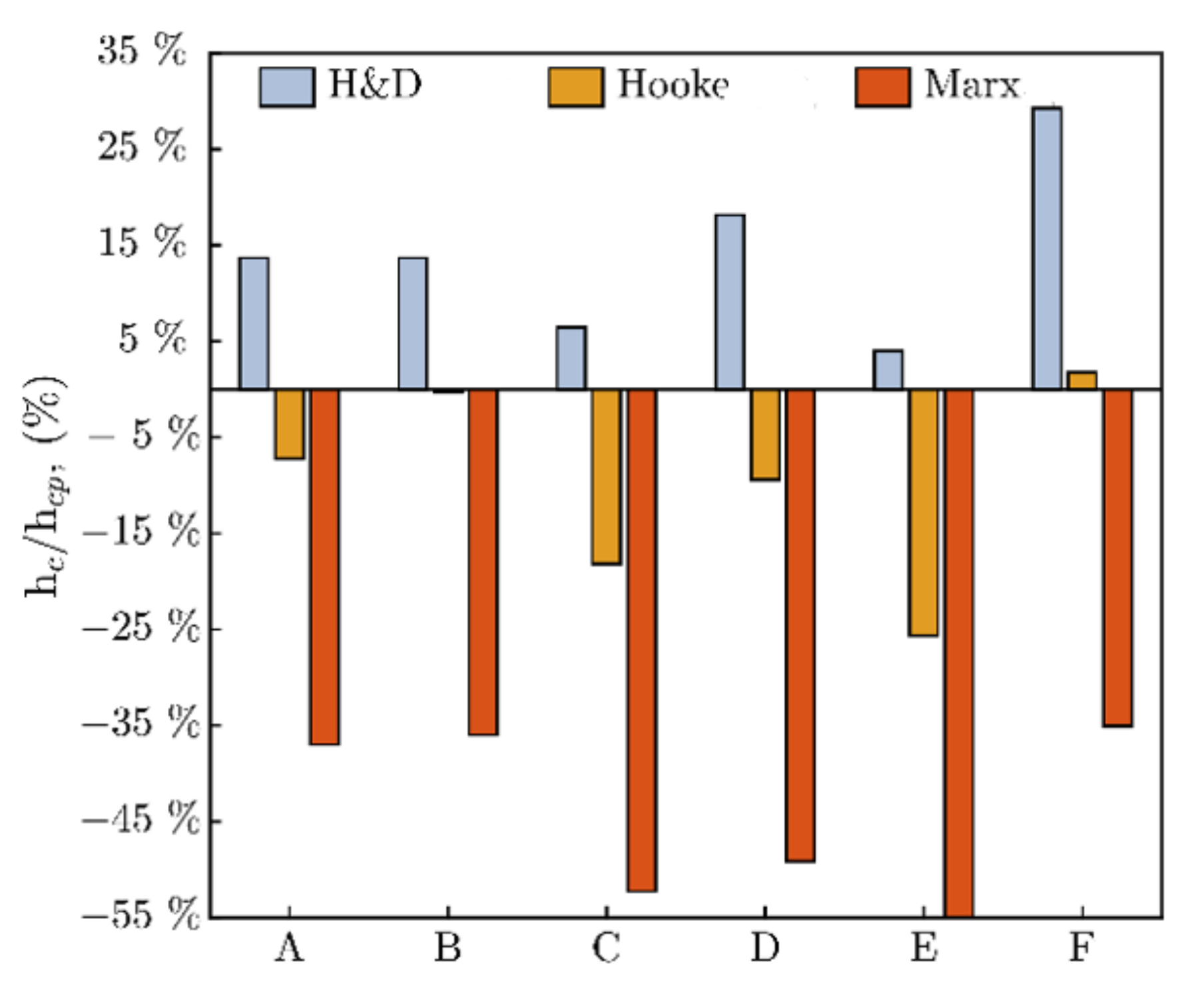
| Exp. | A | B | C | D | E | F |
|---|---|---|---|---|---|---|
| Exp. cond. | 90 °C, 20 N | 90 °C, 50 N | 100 °C, 20 N | 100 °C, 50 N | 110 °C, 20 N | 110 °C, 50 N |
| H&D, [19] | 13.7% | 13.7% | 6.4% | 18.1% | 4.0% | 4.9% |
| Hooke, [20] | −7.3% | −0.3% | −18.2% | −9.4% | −25.7% | −25.2% |
| Marx, [28] | −37.0% | −36.0% | −52.3% | −49.2% | −63.8% | −59.9% |
References
- Gilbert, M. Chapter 1—Plastics Materials: Introduction and Historical Development. In Brydson’s Plastics Materials, 8th ed.; Gilbert, M., Ed.; Butterworth-Heinemann: Amsterdam, The Netherlands, 2017; pp. 1–18. ISBN 978-0-323-35824-8. [Google Scholar]
- Dearn, K.D.; Hoskins, T.J.; Andrei, L.; Walton, D. Lubrication regimes in high-performance polymer spur gears. Adv. Tribol. 2013, 2013, 987251. [Google Scholar] [CrossRef]
- Kunishima, T.; Nagai, Y.; Kurokawa, T.; Bouvard, G.; Abry, J.C.; Fridrici, V.; Kapsa, P. Tribological behavior of glass fiber reinforced-PA66 in contact with carbon steel under high contact pressure, sliding and grease lubricated conditions. Wear 2020, 456–457, 203383. [Google Scholar] [CrossRef]
- Harrass, M.; Friedrich, K.; Almajid, A.A. Tribological behavior of selected engineering polymers under rolling contact. Tribol. Int. 2010, 43, 635–646. [Google Scholar] [CrossRef]
- Dearn, K.D.; Hoskins, T.J.; Petrov, D.G.; Reynolds, S.C.; Banks, R. Applications of dry film lubricants for polymer gears. Wear 2013, 298–299, 99–108. [Google Scholar] [CrossRef]
- Terashima, K.; Tsukamoto, N.; Nishida, N.; Shi, J. Development of Plastic Gear for Power Transmission: Abnormal Wear on the Tooth Root and Tooth Fracture near Pitch Point. Bull. JSME 1986, 29, 1598–1604. [Google Scholar] [CrossRef]
- Tsukamoto, N. Investigation about the Strength of Plastic Gear: 2nd Report; Abrasion of the Nylon Gear for Power Transmission, Meshing with the Steel Gear. JSME Int. J. Ser. B Fluids Therm. Eng. 1981, 24, 872–881. [Google Scholar] [CrossRef][Green Version]
- Cummins, R.A.; Doyle, E.D.; Rebecchi, B. Wear damage to spur gears. Wear 1974, 27, 115–120. [Google Scholar] [CrossRef]
- Fetters, L.J.; Lohse, D.J.; Richter, D.; Witten, T.A.; Zirkel, A. Connection between Polymer Molecular Weight, Density, Chain Dimensions, and Melt Viscoelastic Properties. Macromolecules 1994, 27, 4639–4647. [Google Scholar] [CrossRef]
- Mao, K. A new approach for polymer composite gear design. Wear 2007, 262, 432–441. [Google Scholar] [CrossRef]
- Mao, K.; Langlois, P.; Hu, Z.; Alharbi, K.; Xu, X.; Milson, M.; Li, W.; Hooke, C.J.; Chetwynd, D. The wear and thermal mechanical contact behaviour of machine cut polymer gears. Wear 2015, 332–333, 822–826. [Google Scholar] [CrossRef]
- Masjedi, M.; Khonsari, M.M. Mixed lubrication of soft contacts: An engineering look. Proc. Inst. Mech. Eng. Part J. Eng. Tribol. 2017, 231, 263–273. [Google Scholar] [CrossRef]
- Johnson, K.L. Regimes of elastohydrodynamic lubrication. J. Mech. Eng. Sci. 1970, 12, 9–16. [Google Scholar] [CrossRef]
- Dowson, D. Paper 10: Elastohydrodynamics. Proc. Inst. Mech. Eng. Conf. Proc. 1967, 182, 151–167. [Google Scholar] [CrossRef]
- Greenwood, J.A. An extension of the Grubin theory of elastohydrodynamic lubrication. J. Phys. D Appl. Phys. 1972, 5, 2195–2211. [Google Scholar] [CrossRef]
- Spikes, H. Basics of EHL for practical application. Lubr. Sci. 2015, 27, 45–67. [Google Scholar] [CrossRef]
- Hooke, C.J.; O’Donoghue, J.P. Elastohydrodynamic Lubrication of Soft, Highly Deformed Contacts. J. Mech. Eng. Sci. 1972, 14, 34–48. [Google Scholar] [CrossRef]
- Hooke, C. The Elastohydrodynamic Lubrication of Heavily Loaded Contacts. J. Mech. Eng. Sci. 1977, 19, 149–156. [Google Scholar] [CrossRef]
- Hamrock, B.J.; Dowson, D. Elastohydrodynamic lubrication of elliptical contacts for materials of low elastic modulus i—Fully flooded conjunction. J. Tribol. 1978, 100, 236–245. [Google Scholar] [CrossRef]
- Hooke, C.J. The Elastohydrodynamic Lubrication of Elliptical Point Contacts Operating in the Isoviscous Region. Proc. Inst. Mech. Eng. Part J. Eng. Tribol. 1995, 209, 225–234. [Google Scholar] [CrossRef]
- Myers, T.G.; Hall, R.W.; Savage, M.D.; Gaskell, P.H. The Transition Region of Elastohydrodynamic Lubrication. Proc. R. Soc. Lond. A Math. Phys. Eng. Sci. 1991, 432, 467–479. [Google Scholar]
- Hamrock, B.J.; Dowson, D. Isothermal Elastohydrodynamic Lubrication of Point Contacts—4. Starvation Results. Am. Soc. Mech. Eng. 1976, 99, 223–228. [Google Scholar]
- Dowson, D.; Dunn, J.F.; Taylor, C.M. The Piezo-Viscous Fluid, Rigid Solid Regime of Lubrication. Proc. Inst. Mech. Eng. Part C J. Mech. Eng. Sci. 1983, 197, 43–52. [Google Scholar] [CrossRef]
- Stupkiewicz, S.; Lengiewicz, J.; Sadowski, P.; Kucharski, S. Finite deformation effects in soft elastohydrodynamic lubrication problems. Tribol. Int. 2016, 93, 511–522. [Google Scholar] [CrossRef]
- Hooke, C.J. Calculation of clearances in soft point contacts. J. Tribol. 1988, 110, 167–173. [Google Scholar] [CrossRef]
- Myant, C.; Fowell, M.; Spikes, H.A.; Stokes, J.R. An investigation of lubricant film thickness in sliding compliant contacts. Tribol. Trans. 2010, 53, 684–694. [Google Scholar] [CrossRef]
- Esfahanian, M.; Hamrock, B.J. Fluid-film lubrication regimes revisited. Tribol. Trans. 1991, 34, 628–632. [Google Scholar] [CrossRef]
- Marx, N.; Guegan, J.; Spikes, H.A. Elastohydrodynamic film thickness of soft EHL contacts using optical interferometry. Tribol. Int. 2016, 99, 267–277. [Google Scholar] [CrossRef]
- Myant, C.; Reddyhoff, T.; Spikes, H.A. Laser-induced fluorescence for film thickness mapping in pure sliding lubricated, compliant, contacts. Tribol. Int. 2010, 43, 1960–1969. [Google Scholar] [CrossRef][Green Version]
- Hartl, M.; Krupka, I.; Poliscuk, R.; Liska, M.; Molimard, J.; Querry, M.; Vergne, P. Thin film colorimetric interferometry. Tribol. Trans. 2001, 44, 270–276. [Google Scholar] [CrossRef]
- Reddyhoff, T.; Choo, J.H.; Spikes, H.A.; Glovnea, R.P. Lubricant flow in an elastohydrodynamic contact using fluorescence. Tribol. Lett. 2010, 38, 207–215. [Google Scholar] [CrossRef]
- Kaneta, M.; Kanada, T.; Nishikawa, H. Optical interferometric observations of the effects of a moving dent on point contact EHL. Tribol. Ser. 1997, 32, 69–79. [Google Scholar]
- Ciulli, E.; Draexl, T.; Stadler, K. Film thickness analysis for EHL contacts under steady-state and transient conditions by automatic digital image processing. Adv. Tribol. 2015, 55, 678–705. [Google Scholar]
- Lin, Y.; Bilotti, E.; Bastiaansen, C.W.M.; Peijs, T. Transparent semi-crystalline polymeric materials and their nanocomposites: A review. Polym. Eng. Sci. 2020, 60, 2351–2376. [Google Scholar] [CrossRef]
- Djukic, S.; Bocahut, A.; Bikard, J.; Long, D.R. Mechanical properties of amorphous and semi-crystalline semi-aromatic polyamides. Heliyon 2020, 6, e03857. [Google Scholar] [CrossRef]
- Johnson, J.A.; Jones, D.W. The mechanical properties of PMMA and its copolymers with ethyl methacrylate and butyl methacrylate. J. Mater. Sci. 1994, 29, 870–876. [Google Scholar] [CrossRef]
- Jee, A.Y.; Lee, M. Mechanical properties of polycarbonate and poly (methyl methacrylate) films reinforced with surface-functionalized nanodiamonds. J. Nanosci. Nanotechnol. 2011, 11, 533–536. [Google Scholar] [CrossRef] [PubMed]
- Ali, U.; Karim, K.J.B.A.; Buang, N.A. A Review of the Properties and Applications of Poly (Methyl Methacrylate) (PMMA). Polym. Rev. 2015, 55, 678–705. [Google Scholar] [CrossRef]
- Mathiesen, D.; Vogtmann, D.; Dupaix, R.B. Characterization and constitutive modeling of stress-relaxation behavior of Poly (methyl methacrylate) (PMMA) across the glass transition temperature. Mech. Mater. 2014, 71, 74–84. [Google Scholar] [CrossRef]
- Gold, P.W.; Schmidt, A.; Dicke, H.; Loos, J.; Assmann, C. Viscosity-pressure-temperature behaviour of mineral and synthetic oils. J. Synth. Lubr. 2001, 18, 51–79. [Google Scholar] [CrossRef]
- Liu, H.; Link, F.; Lohner, T.; Stahl, K. Computational fluid dynamics simulation of geared transmissions with injection lubrication. Proc. Inst. Mech. Eng. Part C J. Mech. Eng. Sci. 2019, 233, 7412–7422. [Google Scholar] [CrossRef]
- Boumbimba, R.M.; Ahzi, S.; Bahlouli, N.; Ruch, D.; Gracio, J. Dynamic mechanical properties of PMMA/organoclay nanocomposite: Experiments and modeling. J. Eng. Mater. Technol. Trans. ASME 2011, 133, 1–6. [Google Scholar] [CrossRef]
- Putignano, C.; Dini, D. Soft Matter Lubrication: Does Solid Viscoelasticity Matter? ACS Appl. Mater. Interfaces 2017, 9, 42287–42295. [Google Scholar] [CrossRef]
- Boyce, M.C.; Parks, D.M.; Argon, A.S. Large inelastic deformation of glassy polymers. part I: Rate dependent constitutive model. Mech. Mater. 1988, 7, 15–33. [Google Scholar] [CrossRef]
- Richeton, J.; Ahzi, S.; Vecchio, K.S.; Jiang, F.C.; Adharapurapu, R.R. Influence of temperature and strain rate on the mechanical behavior of three amorphous polymers: Characterization and modeling of the compressive yield stress. Int. J. Solids Struct. 2006, 43, 2318–2335. [Google Scholar] [CrossRef]
- Richeton, J.; Ahzi, S.; Vecchio, K.S.; Jiang, F.C.; Makradi, A. Modeling and validation of the large deformation inelastic response of amorphous polymers over a wide range of temperatures and strain rates. Int. J. Solids Struct. 2007, 44, 7938–7954. [Google Scholar] [CrossRef]
- De Deus, J.F.; Souza, G.P.; Corradini, W.A.; Atvars, T.D.Z.; Akcelrud, L. Relaxations of poly (methyl methacrylate) probed by covalently attached anthryl groups. Macromolecules 2004, 37, 6938–6944. [Google Scholar] [CrossRef]
- Chaudhri, M.M. Impact breakage of semi-brittle spheres. Powder Technol. 2004, 143–144, 31–40. [Google Scholar] [CrossRef]
- Liu, Y.; Liaw, B. Drop-weight impact tests and finite element modeling of cast acrylic/aluminum plates. Polym. Test. 2009, 28, 808–823. [Google Scholar] [CrossRef]
- Putignano, C.; Reddyhoff, T.; Carbone, G.; Dini, D. Experimental investigation of viscoelastic rolling contacts: A comparison with theory. Tribol. Lett. 2013, 51, 105–113. [Google Scholar] [CrossRef]
- Carbone, G.; Putignano, C. A novel methodology to predict sliding and rolling friction of viscoelastic materials: Theory and experiments. J. Mech. Phys. Solids 2013, 61, 1822–1834. [Google Scholar] [CrossRef]
- Blumenthal, W.R. Influence of Temperature and Strain Rate on the Compressive Behavior of PMMA and Polycarbonate Polymers. AIP Conf. Proc. 2003, 665, 665–668. [Google Scholar]
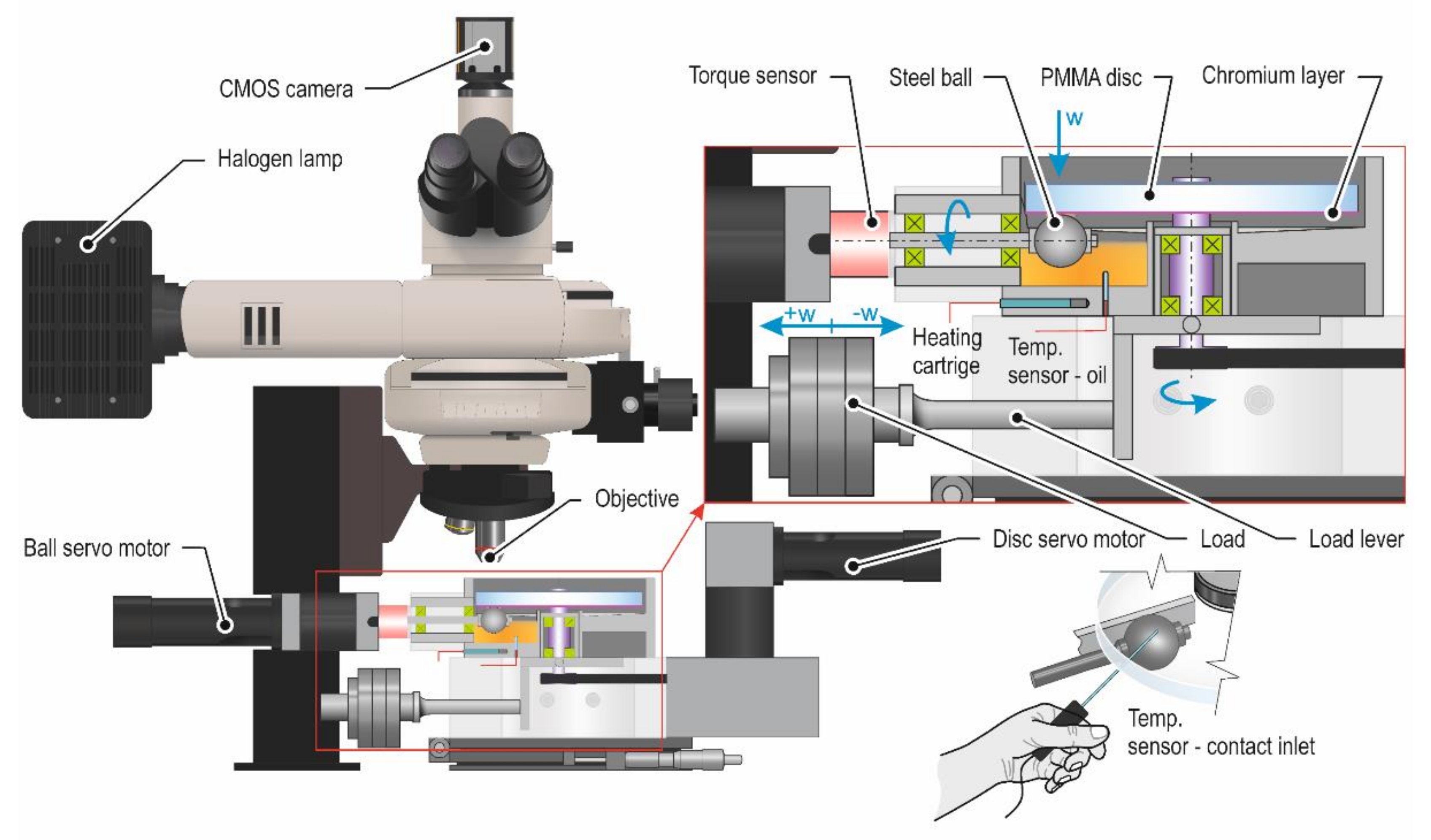
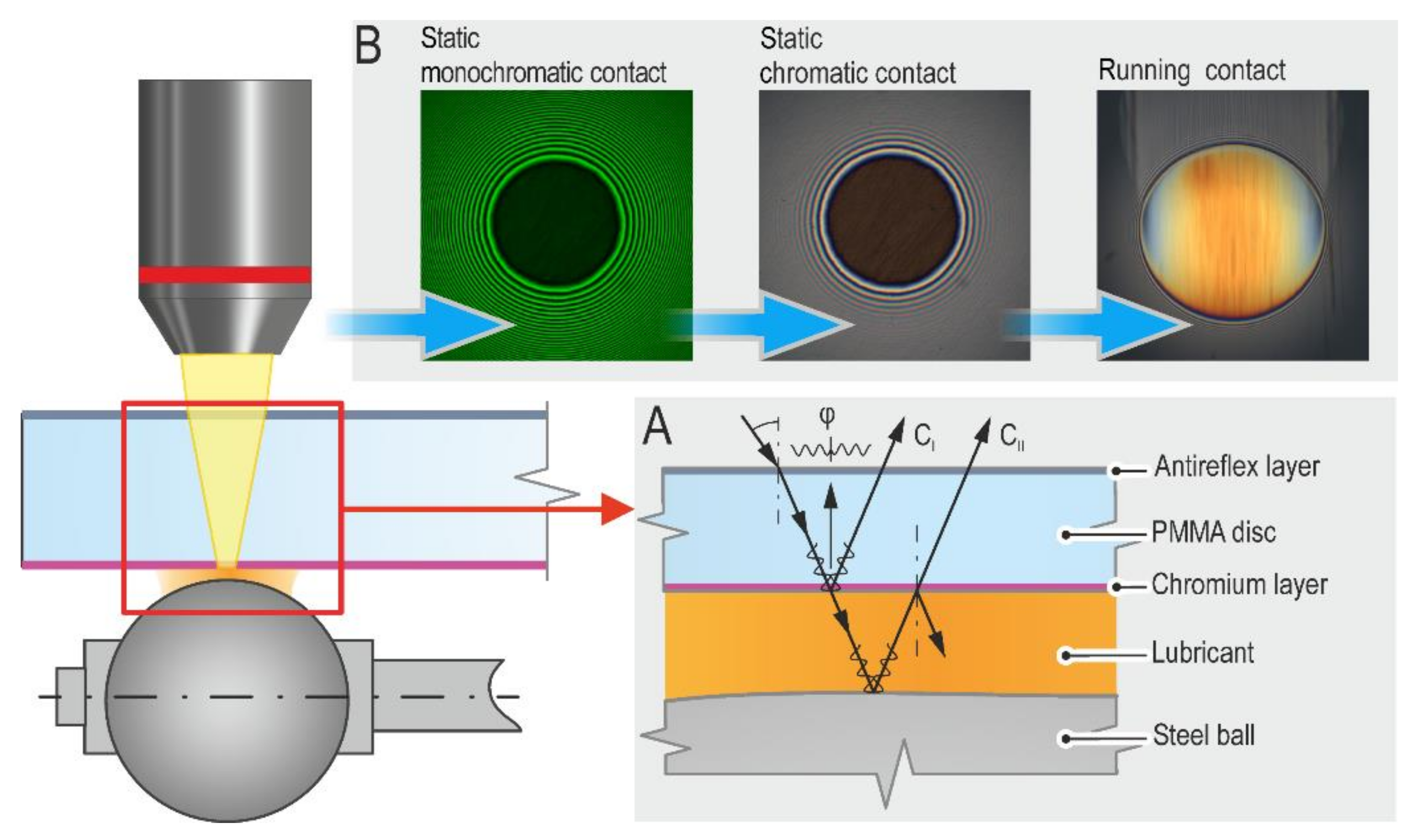
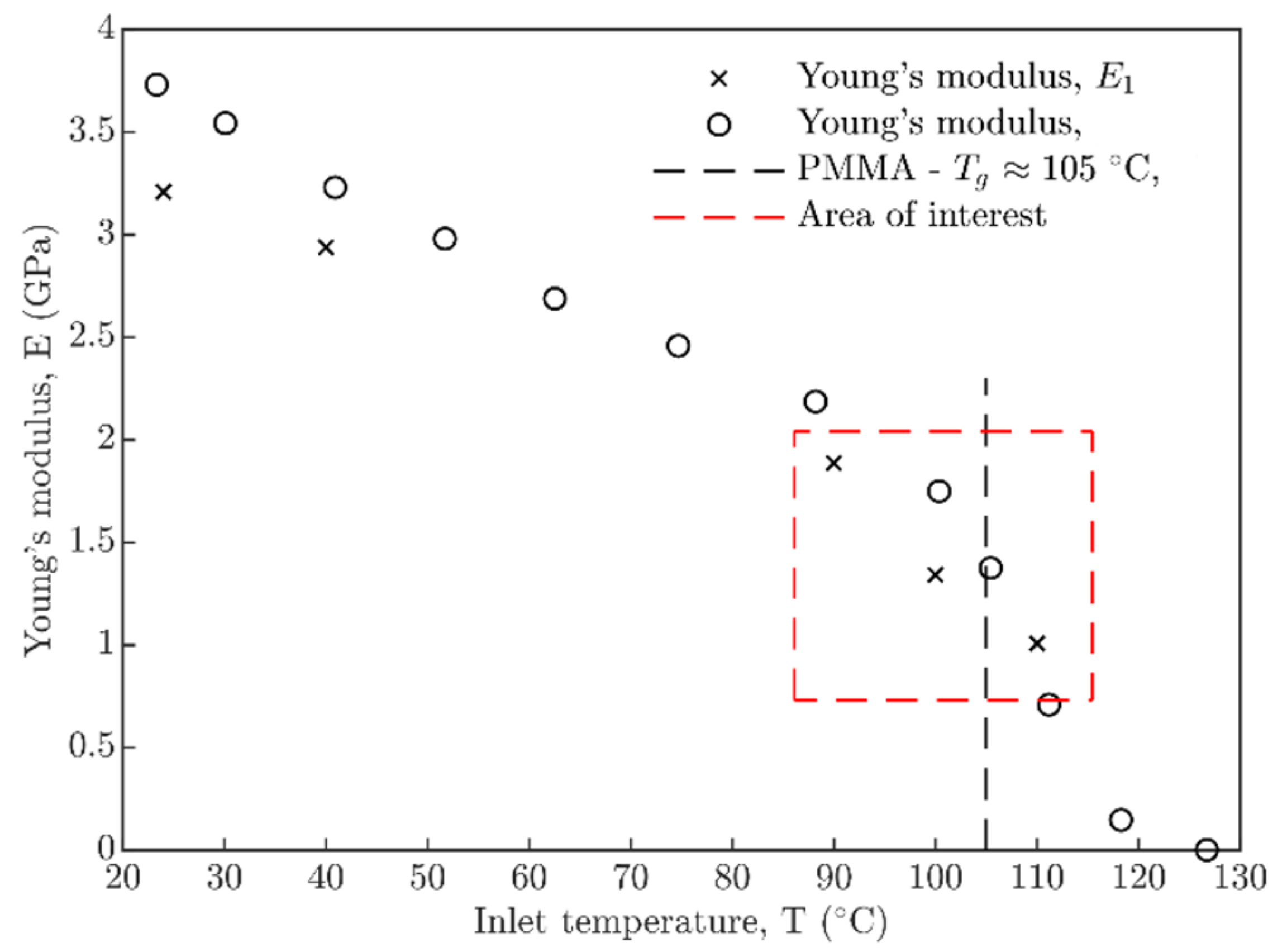



| Specimens | Specification | Young’s Modulus, E | Poisson’s Ratio, υ | Glass-Transition Temperature, Tg | RMS Roughness, Rq |
|---|---|---|---|---|---|
| PMMA disc | Polymethyl-methacrylate | 3.3 GPa | 0.39 | 105 °C | Rq < 0.005 µm |
| Steel ball | 100Cr6 | 206 GPa | 0.30 | - | Rq < 0.01 µm |
| Oil | Kinematic Viscosity at 40 °C, υ40 | Kinematic Viscosity at 100 °C, υ100 | Density at 15 °C, ρ15 |
|---|---|---|---|
| FVA3 | 95.0 mm2/s | 10.7 mm2/s | 864 kg/m3 |
| Parameter | Value |
|---|---|
| Entrainment speed, U | 0.2–1.2 m/s |
| Load, W | 20, 50 N |
| Maximal contact pressure (24 °C), p0 | 45, 62 MPa |
| Dynamic viscosity of FVA3 at atm., η0 | 0.013–0.008 Pa s |
| Inlet temperature, T | 90–110 °C |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Krupka, J.; Dockal, K.; Krupka, I.; Hartl, M. Elastohydrodynamic Lubrication of Compliant Circular Contacts near Glass-Transition Temperature. Lubricants 2022, 10, 155. https://doi.org/10.3390/lubricants10070155
Krupka J, Dockal K, Krupka I, Hartl M. Elastohydrodynamic Lubrication of Compliant Circular Contacts near Glass-Transition Temperature. Lubricants. 2022; 10(7):155. https://doi.org/10.3390/lubricants10070155
Chicago/Turabian StyleKrupka, Jiri, Krystof Dockal, Ivan Krupka, and Martin Hartl. 2022. "Elastohydrodynamic Lubrication of Compliant Circular Contacts near Glass-Transition Temperature" Lubricants 10, no. 7: 155. https://doi.org/10.3390/lubricants10070155
APA StyleKrupka, J., Dockal, K., Krupka, I., & Hartl, M. (2022). Elastohydrodynamic Lubrication of Compliant Circular Contacts near Glass-Transition Temperature. Lubricants, 10(7), 155. https://doi.org/10.3390/lubricants10070155