Determination of the Preload of Bolts for Structural Health Monitoring of a Multi-Bolted Joint: FEM Approach
Abstract
:1. Introduction
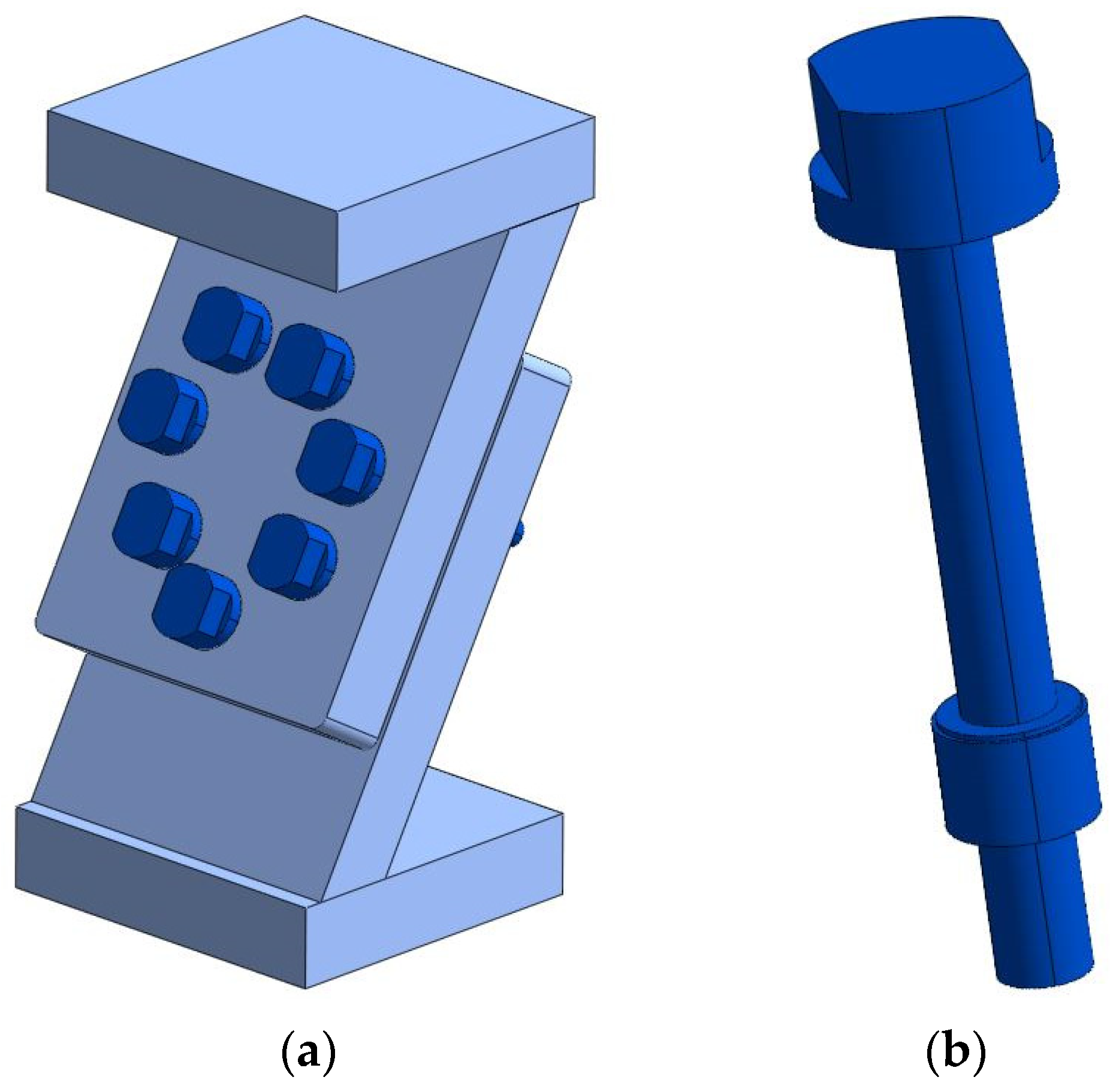
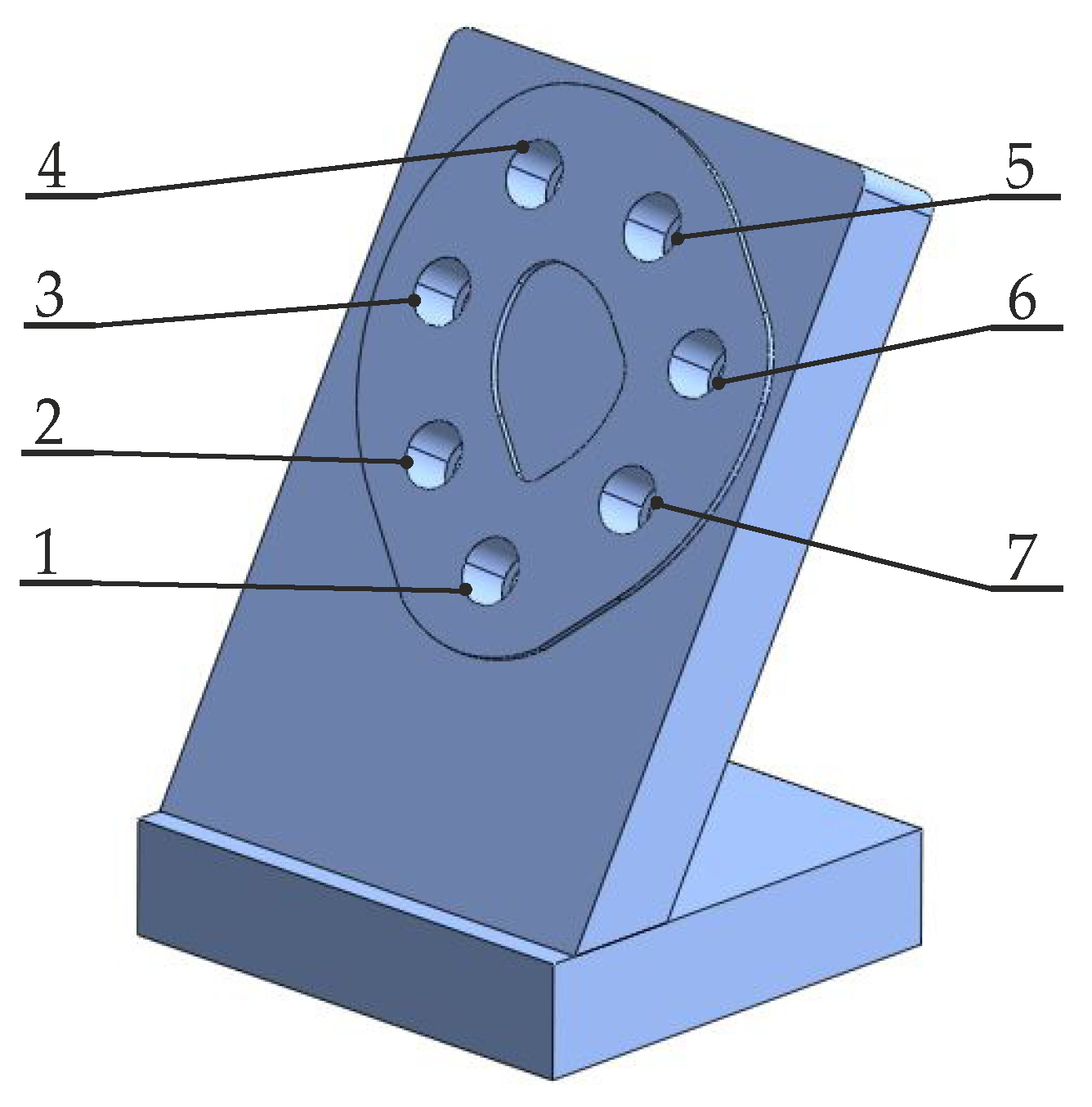
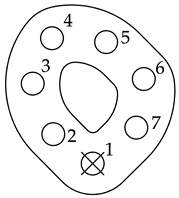
2. Physical Model of the Joint
3. Research Procedure
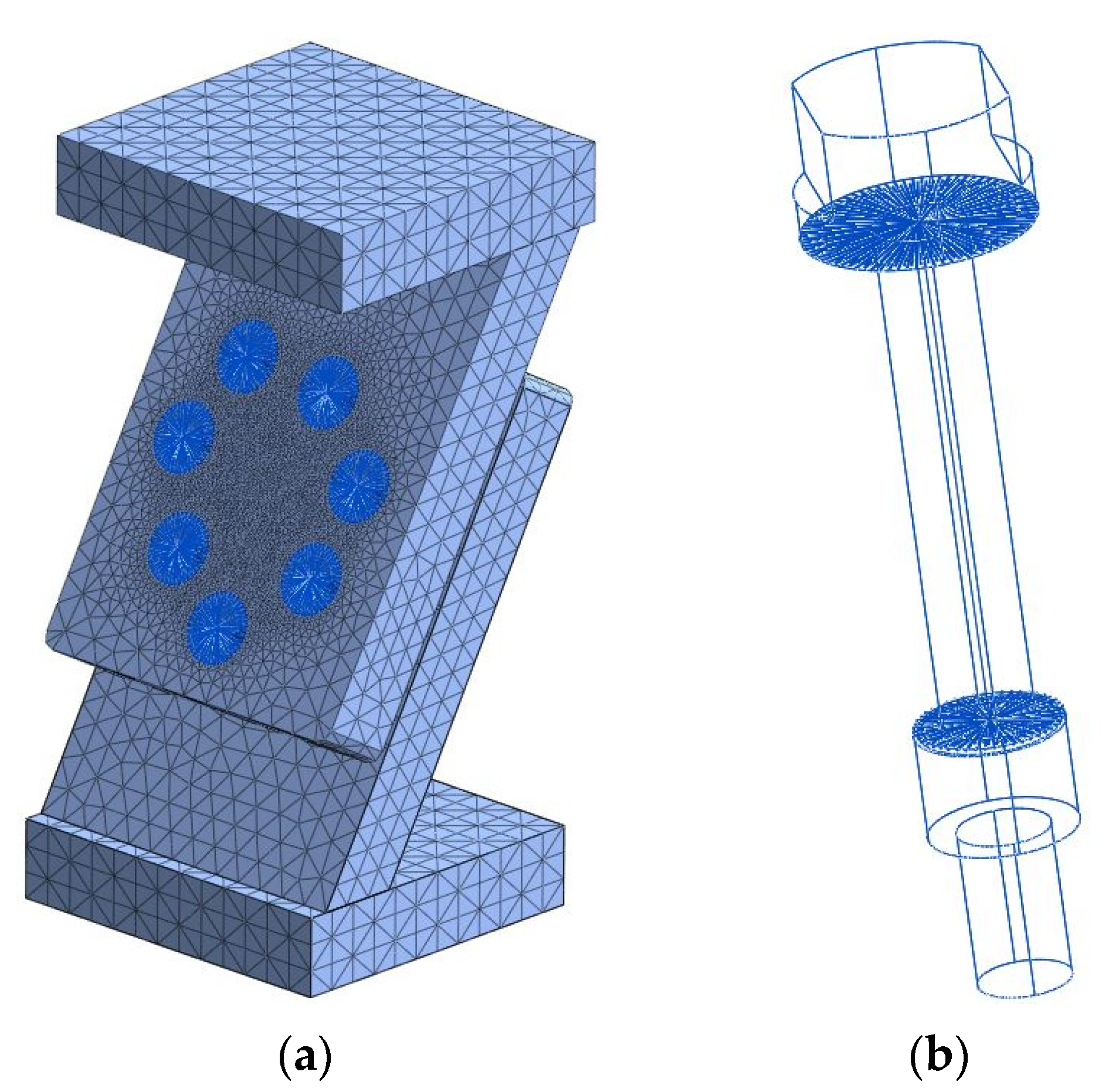
4. FEM-Based Model of the Joint
- Normal stiffness factor equal to 10;
- Tangential stiffness factor equal to 1;
- Coefficient of static friction for a pair of ground surfaces equal to 0.14 [50].
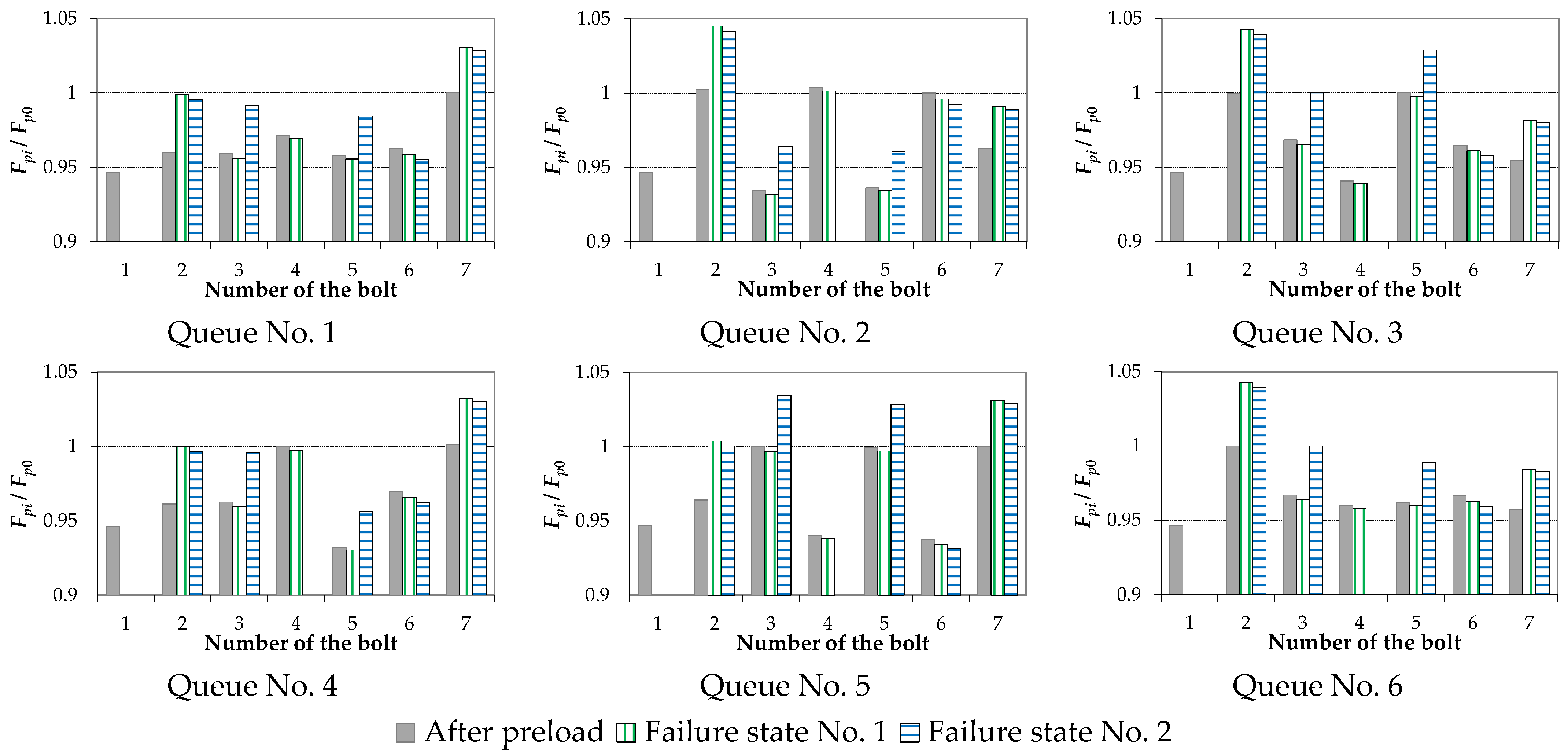
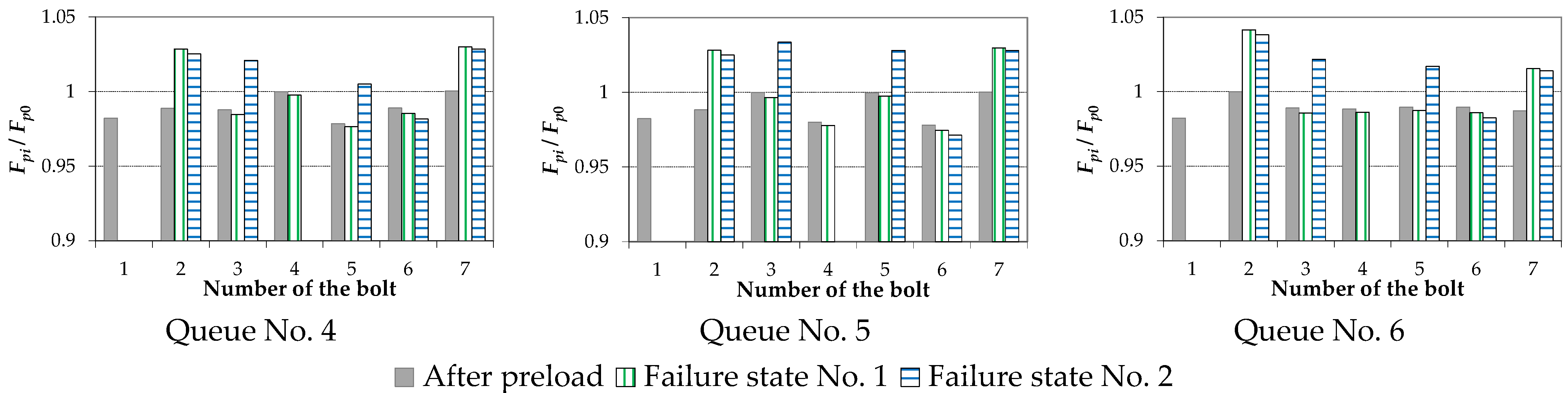
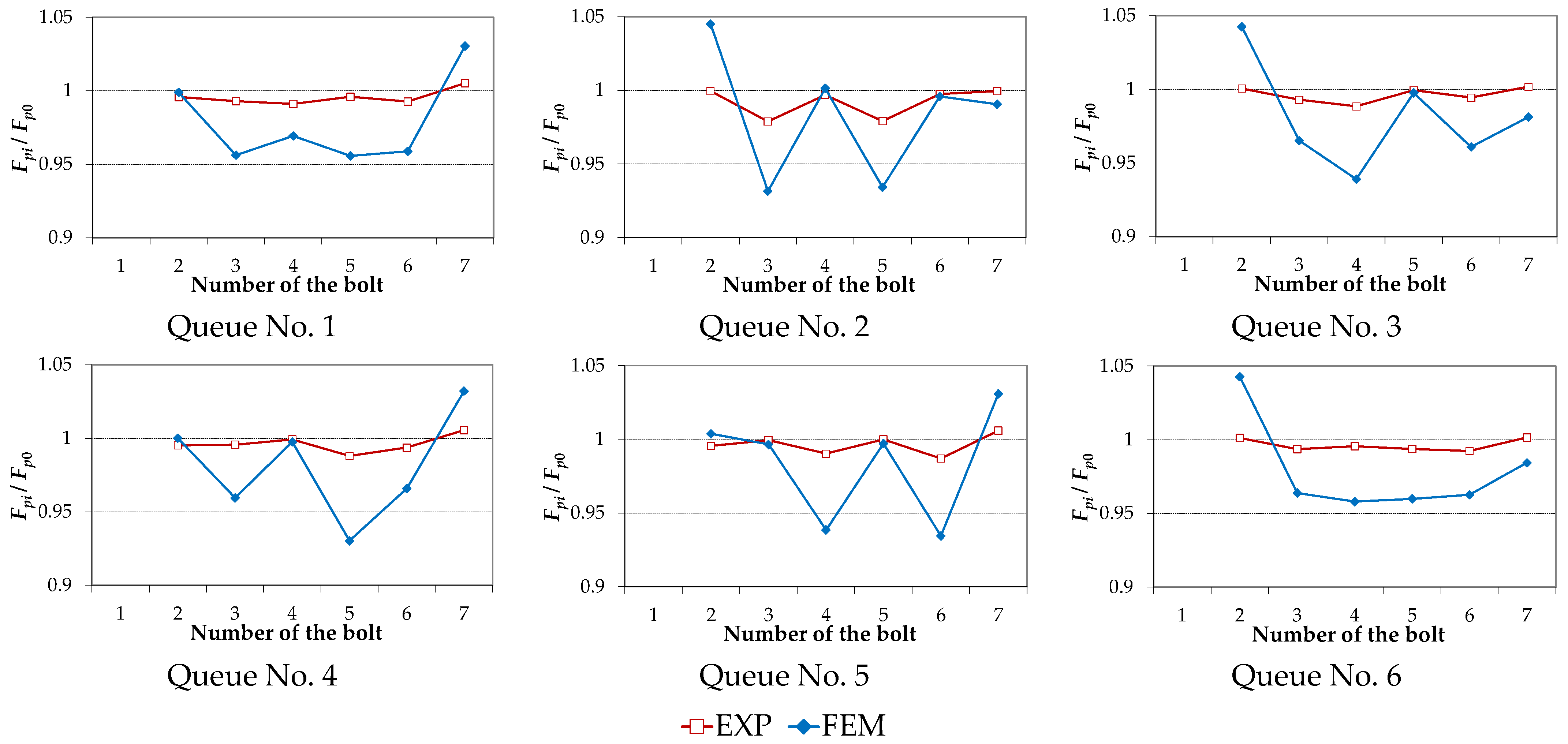
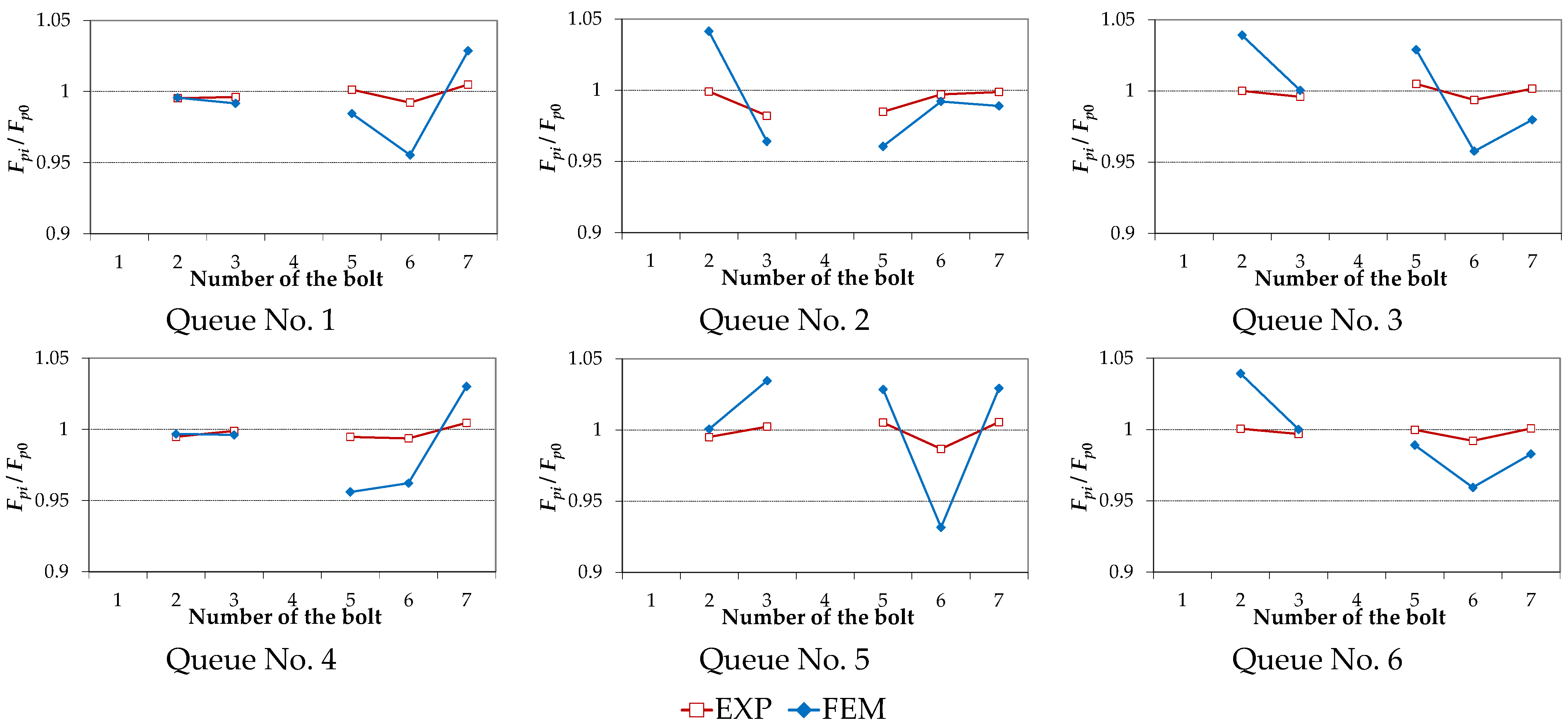
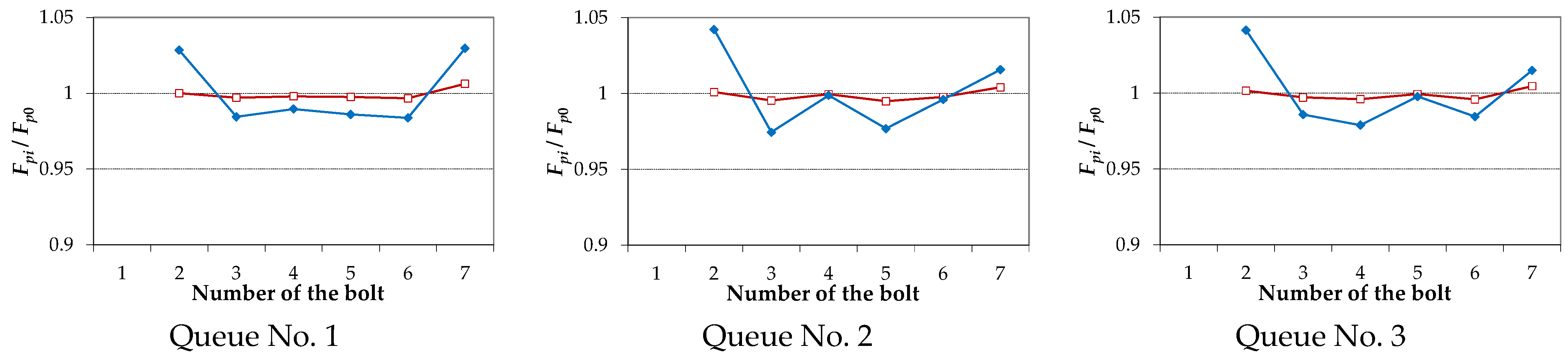
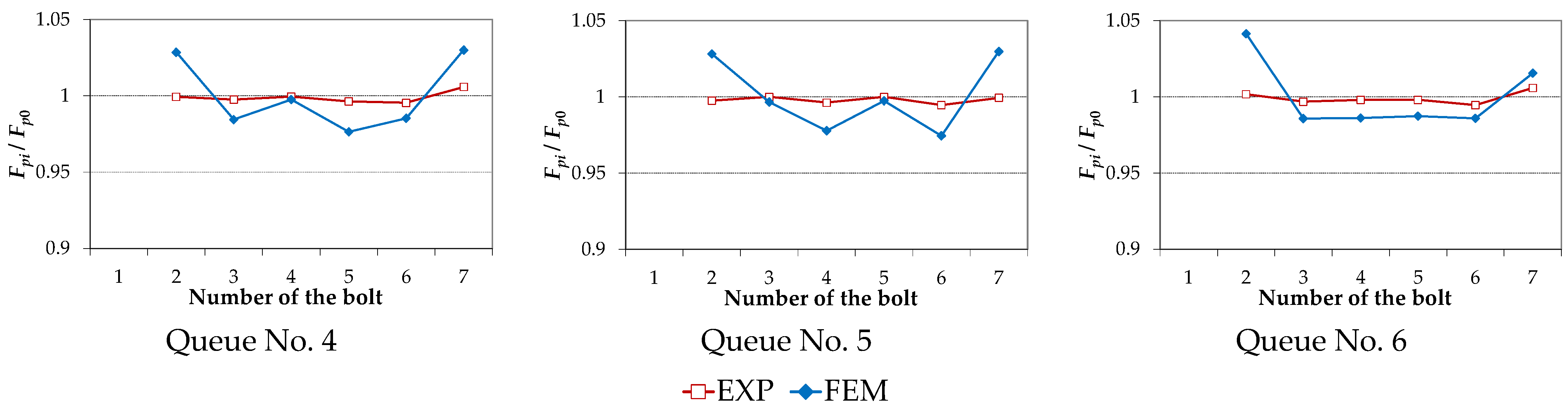
5. Calculation Results and Discussion
- It was confirmed, as in the case of experimental tests [23], that in the damaged state of the joint, the largest increment of the force occurs in two bolts closest to the damaged one.
- The variability of the force chart in the bolts after the joint damage is determined by the order in which the bolts were preloaded.
- The preload charts for the respective bolts in the state of the joint damage reveal a certain variability.
- By increasing the number of passes during the multi-bolted joint preloading, it was possible to reduce the variability of values of the bolt forces in the state of the joint damage.
6. Concluding Remarks
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Nazarko, P.; Ziemianski, L. Force identification in bolts of flange connections for structural health monitoring and failure prevention. Procedia Struct. Integr. 2017, 5, 460–467. [Google Scholar] [CrossRef]
- Qin, X.; Peng, C.; Zhao, G.; Ju, Z.; Lv, S.; Jiang, M.; Sui, Q.; Jia, L. Full life-cycle monitoring and earlier warning for bolt joint loosening using modified vibro-acoustic modulation. Mech. Syst. Signal Process. 2022, 162, 108054. [Google Scholar] [CrossRef]
- Demetgul, M.; Senyurek, V.Y.; Uyandik, R.; Tansel, I.N.; Yazicioglu, O. Evaluation of the health of riveted joints with active and passive structural health monitoring techniques. Measurement 2015, 69, 42–51. [Google Scholar] [CrossRef]
- Winkler, J.; Hendy, C. Improved structural health monitoring of London’s docklands light railway bridges using digital image correlation. Struct. Eng. Int. 2017, 27, 435–440. [Google Scholar] [CrossRef]
- Tochaei, E.N.; Fang, Z.; Taylor, T.; Babanajad, S.; Ansari, F. Structural monitoring and remaining fatigue life estimation of typical welded crack details in the Manhattan Bridge. Eng. Struct. 2021, 231, 111760. [Google Scholar] [CrossRef]
- Jamrozik, W.; Górka, J.; Wyględacz, B.; Kiel-Jamrozik, M. FEM-based thermogram correction for Inconel 625 joint hardness clustering. Materials 2022, 15, 1113. [Google Scholar] [CrossRef]
- Kudu, F.N.; Bayraktar, A.; Bakir, P.G.; Türker, T.; Altunişik, A.C. Ambient vibration testing of Berta Highway Bridge with post-tension tendons. Steel Compos. Struct. 2014, 16, 23–46. [Google Scholar] [CrossRef]
- Yan, G.; Dyke, S.J.; Irfanoglu, A. Experimental validation of a damage detection approach on a full-scale highway sign support truss. Mech. Syst. Signal Process. 2012, 28, 195–211. [Google Scholar] [CrossRef]
- Pérez, M.A.; Font-Moré, J.; Fernández-Esmerats, J. Structural damage assessment in lattice towers based on a novel frequency domain-based correlation approach. Eng. Struct. 2021, 226, 111329. [Google Scholar] [CrossRef]
- Pham, C.H.; Hancock, G.J. Tension field action for cold-formed sections in shear. J. Constr. Steel Res. 2012, 72, 168–178. [Google Scholar] [CrossRef]
- Pham, C.H.; Zelenkin, D.; Hancock, G.J. Effect of flange restraints on shear tension field action in cold-formed C-sections. J. Constr. Steel Res. 2017, 129, 42–53. [Google Scholar] [CrossRef]
- Martowicz, A.; Sendecki, A.; Salomon, M.; Rosiek, M.; Uhl, T. Application of electromechanical impedance-based SHM for damage detection in bolted pipeline connection. Nondestruct. Test. Eval. 2016, 31, 17–44. [Google Scholar] [CrossRef]
- Thien, A.B.; Chiamori, H.C.; Ching, J.T.; Wait, J.R.; Park, G. The use of macro-fibre composites for pipeline structural health assessment. Struct. Control Health Monit. 2008, 15, 43–63. [Google Scholar] [CrossRef]
- Blachowski, B.; Swiercz, A.; Gutkiewicz, P.; Szelążek, J.; Gutkowski, W. Structural damage detectability using modal and ultrasonic approaches. Measurement 2016, 85, 210–221. [Google Scholar] [CrossRef]
- Li, J.; Hao, H. Health monitoring of joint conditions in steel truss bridges with relative displacement sensors. Measurement 2016, 88, 360–371. [Google Scholar] [CrossRef] [Green Version]
- Beskhyroun, S.; Oshima, T.; Mikami, S. Wavelet-based technique for structural damage detection. Struct. Control Health Monit. 2010, 17, 473–494. [Google Scholar] [CrossRef]
- Beskhyroun, S.; Oshima, T.; Mikami, S.; Miyamori, Y. Assessment of vibration-based damage identification techniques using localized excitation source. J. Civ. Struct. Health Monit. 2013, 3, 207–223. [Google Scholar] [CrossRef]
- Kromanis, R.; Kripakaran, P. SHM of bridges: Characterising thermal response and detecting anomaly events using a temperature-based measurement interpretation approach. J. Civ. Struct. Health Monit. 2016, 6, 237–254. [Google Scholar] [CrossRef] [Green Version]
- Kromanis, R.; Kripakaran, P. Data-driven approaches for measurement interpretation: Analysing integrated thermal and vehicular response in bridge structural health monitoring. Adv. Eng. Inform. 2017, 34, 46–59. [Google Scholar] [CrossRef] [Green Version]
- Kunwar, A.; Jha, R.; Whelan, M.; Janoyan, K. Damage detection in an experimental bridge model using Hilbert-Huang transform of transient vibrations. Struct. Control Health Monit. 2013, 20, 1–15. [Google Scholar] [CrossRef]
- Whelan, M.J.; Gangone, M.V. Effect of measurement uncertainties on strain-based damage diagnostics for highway bridges. J. Civ. Struct. Health Monit. 2015, 5, 321–335. [Google Scholar] [CrossRef]
- Becht, P.; Deckers, E.; Claeys, C.; Pluymers, B.; Desmet, W. Loose bolt detection in a complex assembly using a vibro-acoustic sensor array. Mech. Syst. Signal Process. 2019, 130, 433–451. [Google Scholar] [CrossRef]
- Grzejda, R.; Parus, A. Health assessment of a multi-bolted connection due to removing selected bolts. FME Trans. 2021, 49, 634–642. [Google Scholar] [CrossRef]
- Rucka, M.; Zima, B.; Kędra, R. Application of guided wave propagation in diagnostics of steel bridge components. Arch. Civ. Eng. 2014, 60, 493–515. [Google Scholar] [CrossRef] [Green Version]
- Qin, Z.; Han, Q.; Chu, F. Bolt loosening at rotating joint interface and its influence on rotor dynamics. Eng. Fail. Anal. 2016, 59, 456–466. [Google Scholar] [CrossRef]
- Blachowski, B.; Gutkowski, W. Effect of damaged circular flange-bolted connections on behaviour of tall towers, modelled by multilevel substructuring. Eng. Struct. 2016, 111, 93–103. [Google Scholar] [CrossRef]
- Patil, C.S.; Roy, S.; Jagtap, K.R. Damage detection in frame structure using piezoelectric actuator. Mater. Today Proc. 2017, 4, 687–692. [Google Scholar] [CrossRef]
- Hasni, H.; Jiao, P.; Alavi, A.H.; Lajnef, N.; Masri, S.F. Structural health monitoring of steel frames using a network of self-powered strain and acceleration sensors: A numerical study. Autom. Constr. 2018, 85, 344–357. [Google Scholar] [CrossRef]
- Grzejda, R.; Parus, A. Experimental studies of the process of tightening an asymmetric multi-bolted connection. IEEE Access 2021, 9, 47372–47379. [Google Scholar] [CrossRef]
- Grzejda, R.; Parus, A.; Kwiatkowski, K. Experimental studies of an asymmetric multi-bolted connection under monotonic loads. Materials 2021, 14, 2353. [Google Scholar] [CrossRef]
- Baranowski, P.; Damaziak, K.; Małachowski, J. Brake system studies using numerical methods. Eksploat. Niezawodn. Maint. Reliab. 2013, 15, 337–342. [Google Scholar]
- Woch, M. Reliability analysis of the PZL-130 Orlik TC-II aircraft structural component under real operating conditions. Eksploat. Niezawodn. Maint. Reliab. 2017, 19, 287–295. [Google Scholar] [CrossRef]
- Wysmulski, P. The analysis of buckling and post buckling in the compressed composite columns. Arch. Mater. Sci. Eng. 2017, 85, 35–41. [Google Scholar] [CrossRef]
- Croccolo, D.; De Agostinis, M.; Vincenzi, N. A contribution to the selection and calculation of screws in high duty bolted joints. Int. J. Press. Vessels Pip. 2012, 96–97, 38–48. [Google Scholar] [CrossRef]
- Croccolo, D.; De Agostinis, M.; Vincenzi, N. Influence of tightening procedures and lubrication conditions on titanium screw joints for lightweight applications. Tribol. Int. 2012, 55, 68–76. [Google Scholar] [CrossRef]
- Croccolo, D.; De Agostinis, M.; Fini, S.; Olmi, G.; Robusto, F.; Vincenzi, N. Steel screws on aluminium nuts: Different engagement ratio tapped threads compared to threaded inserts with a proper tolerance choice. Tribol. Int. 2019, 138, 297–306. [Google Scholar] [CrossRef]
- Majda, P.; Jastrzębska, J. Measurement uncertainty of generalized stiffness of machine tools. Measurement 2021, 170, 108692. [Google Scholar] [CrossRef]
- Wang, G.; Ding, Y. The interface friction in the friction-type bolted joint of steel truss bridge: Case study. Balt. J. Road Bridge Eng. 2020, 15, 187–210. [Google Scholar] [CrossRef]
- Daniūnas, A.; Urbonas, K. Influence of the semi-rigid bolted steel joints on the frame behaviour. J. Civ. Eng. Manag. 2010, 16, 237–241. [Google Scholar] [CrossRef] [Green Version]
- PN-EN 1993-1-8, 2006; Eurocode 3: Design of Steel Structures, Part 1–8: Design of Joints. Polish Committee for Standardization: Warsaw, Poland, 2006.
- Palenica, P.; Powałka, B.; Grzejda, R. Assessment of modal parameters of a building structure model. Springer Proc. Math. Stat. 2016, 181, 319–325. [Google Scholar] [CrossRef]
- Grzejda, R. Modelling nonlinear multi-bolted systems on the assembly state. Procedia Eng. 2017, 206, 1808–1812. [Google Scholar] [CrossRef]
- Stosiak, M. The modelling of hydraulic distributor slide–sleeve interaction. Arch. Civ. Mech. Eng. 2012, 12, 192–197. [Google Scholar] [CrossRef]
- Zmarzły, P. Influence of bearing raceway surface topography on the level of generated vibration as an example of operational heredity. Indian J. Eng. Mater. Sci. 2020, 27, 356–364. [Google Scholar]
- Zmarzły, P. Technological heredity of the turning process. Tech. Gaz. 2020, 27, 1194–1203. [Google Scholar] [CrossRef]
- Jaszak, P. The elastic serrated gasket of the flange bolted joints. Int. J. Press. Vessels Pip. 2019, 176, 103954. [Google Scholar] [CrossRef]
- Jamia, N.; Jalali, H.; Taghipour, J.; Friswell, M.I.; Khodaparast, H.H. An equivalent model of a nonlinear bolted flange joint. Mech. Syst. Signal Process. 2021, 153, 107507. [Google Scholar] [CrossRef]
- Yang, B.; Sun, Q.; Lin, Q.; Wang, L.; Zhang, X.; Ma, Y. Influence mechanism of bolted joint with geometric irregularity bearing surface on anti-loosening performance. Int. J. Press. Vessels Pip. 2021, 191, 104364. [Google Scholar] [CrossRef]
- Grzejda, R. Impact of nonlinearity of the contact layer between elements joined in a multi-bolted system on its preload. Int. J. Appl. Mech. Eng. 2017, 22, 921–930. [Google Scholar] [CrossRef] [Green Version]
- Grzesik, W. Effect of the machine parts surface topography features on the machine service. Mechanik 2015, 88, 587–593. (In Polish) [Google Scholar] [CrossRef] [Green Version]
- Łukaszewicz, A. Temperature field in the contact zone in the course of rotary friction welding of metals. Mater. Sci. 2019, 55, 39–45. [Google Scholar] [CrossRef]
- Borawski, A.; Szpica, D.; Mieczkowski, G. Verification tests of frictional heat modelling results. Mechanika 2020, 26, 260–264. [Google Scholar] [CrossRef]
- Soleimanpour, R.; Soleimani, S.M.; Mohammad, N.K. Damage detection and localization in loose bolted joints. Procedia Struct. Integr. 2022, 37, 956–963. [Google Scholar] [CrossRef]


| Designation | Meaning | Level |
|---|---|---|
| Single-pass preloading | ||
| Fpi | Preloading of the i-th bolt | Fpi = 22 kN |
| Multi-pass preloading | ||
| Fpi1 | Preload of the i-th bolt in pass No. 1 | 0.2 Fpi = 4.4 kN |
| Fpi2 | Preload of the i-th bolt in pass No. 2 | 0.6 Fpi = 13.2 kN |
| Fpi3 | Preload of the i-th bolt in pass No. 3 | Fpi = 22 kN |
| Queue No. | Order of Bolts | Queue No. | Order of Bolts |
|---|---|---|---|
| 1 | 1-2-3-4-5-6-7 | 4 | 1-5-2-6-3-7-4 |
| 2 | 1-3-5-7-2-4-6 | 5 | 1-6-4-2-7-5-3 |
| 3 | 1-4-7-3-6-2-5 | 6 | 1-7-6-5-4-3-2 |
| 1 | 2 |
|---|---|
 |  |
| Queue No. | Single-Pass Preloading | Multi-Pass Preloading | ||
|---|---|---|---|---|
| State No. 1 | State No. 2 | State No. 1 | State No. 2 | |
| 1 | 4.04 | 3.37 | 4.04 | 3.32 |
| 2 | 4.28 | 3.17 | 4.16 | 3.23 |
| 3 | 4.28 | 3.31 | 4.16 | 3.28 |
| 4 | 4.03 | 3.47 | 4.03 | 3.35 |
| 5 | 4.10 | 3.47 | 4.05 | 3.37 |
| 6 | 4.28 | 3.41 | 4.16 | 3.31 |
| Queue No. | Single-Pass Preloading | Multi-Pass Preloading | ||
|---|---|---|---|---|
| State No. 1 | State No. 2 | State No. 1 | State No. 2 | |
| 1 | 4.05 | 3.71 | 2.84 | 2.54 |
| 2 | 4.85 | 4.23 | 4.13 | 3.82 |
| 3 | 5.01 | 3.91 | 3.99 | 3.69 |
| 4 | 5.84 | 3.88 | 2.92 | 2.63 |
| 5 | 5.32 | 5.60 | 3.07 | 3.11 |
| 6 | 4.15 | 3.86 | 3.97 | 3.67 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Grzejda, R.; Warzecha, M.; Urbanowicz, K. Determination of the Preload of Bolts for Structural Health Monitoring of a Multi-Bolted Joint: FEM Approach. Lubricants 2022, 10, 75. https://doi.org/10.3390/lubricants10050075
Grzejda R, Warzecha M, Urbanowicz K. Determination of the Preload of Bolts for Structural Health Monitoring of a Multi-Bolted Joint: FEM Approach. Lubricants. 2022; 10(5):75. https://doi.org/10.3390/lubricants10050075
Chicago/Turabian StyleGrzejda, Rafał, Mariusz Warzecha, and Kamil Urbanowicz. 2022. "Determination of the Preload of Bolts for Structural Health Monitoring of a Multi-Bolted Joint: FEM Approach" Lubricants 10, no. 5: 75. https://doi.org/10.3390/lubricants10050075
APA StyleGrzejda, R., Warzecha, M., & Urbanowicz, K. (2022). Determination of the Preload of Bolts for Structural Health Monitoring of a Multi-Bolted Joint: FEM Approach. Lubricants, 10(5), 75. https://doi.org/10.3390/lubricants10050075







