Dynamics of Test Particles and Twin Peaks QPOs around Regular Black Holes in Modified Gravity
Abstract
1. Introduction
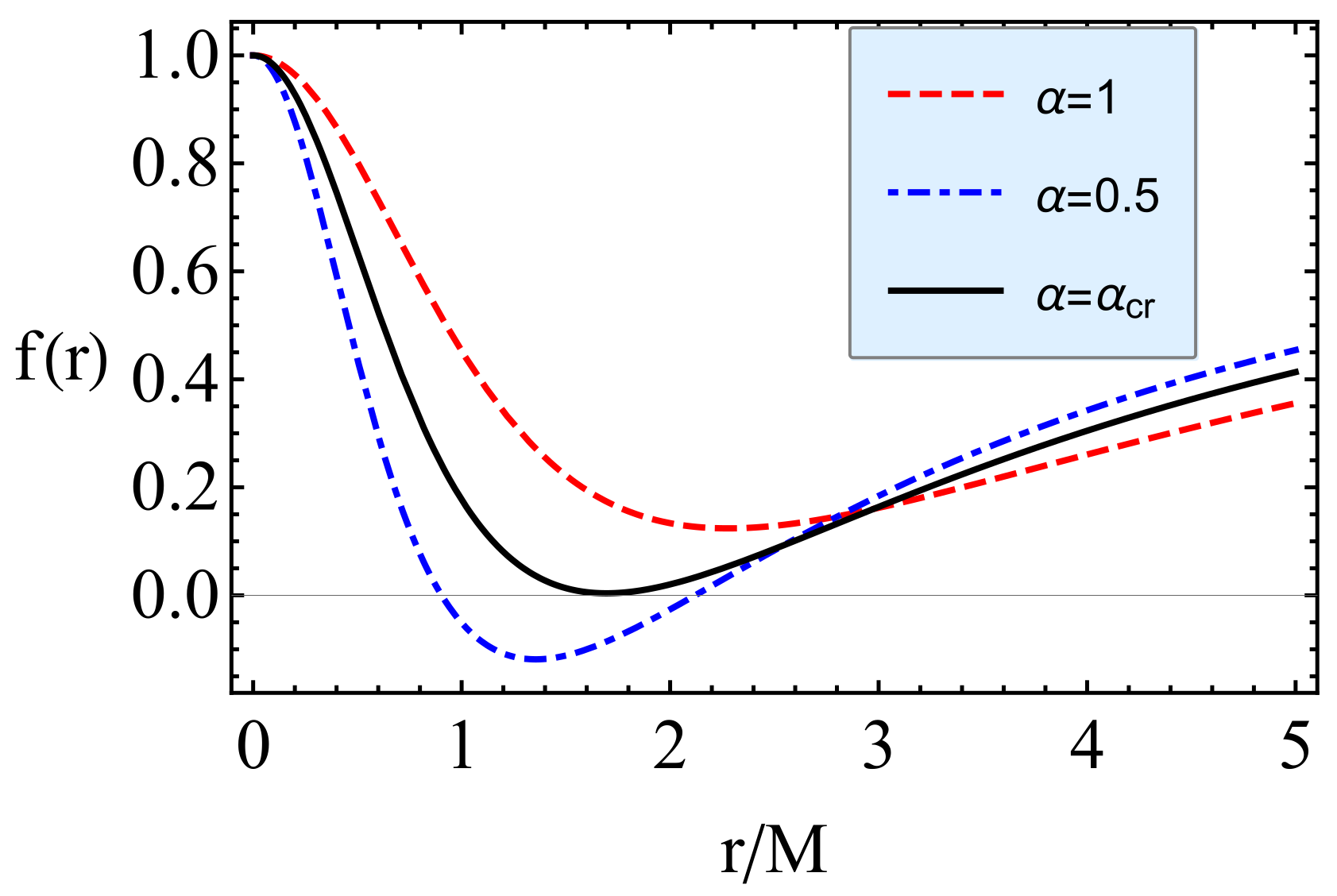
2. The Spacetime Properties
- In the case when two event horizons do exist: inner and outer ones;
- In the case when there are no event horizons;
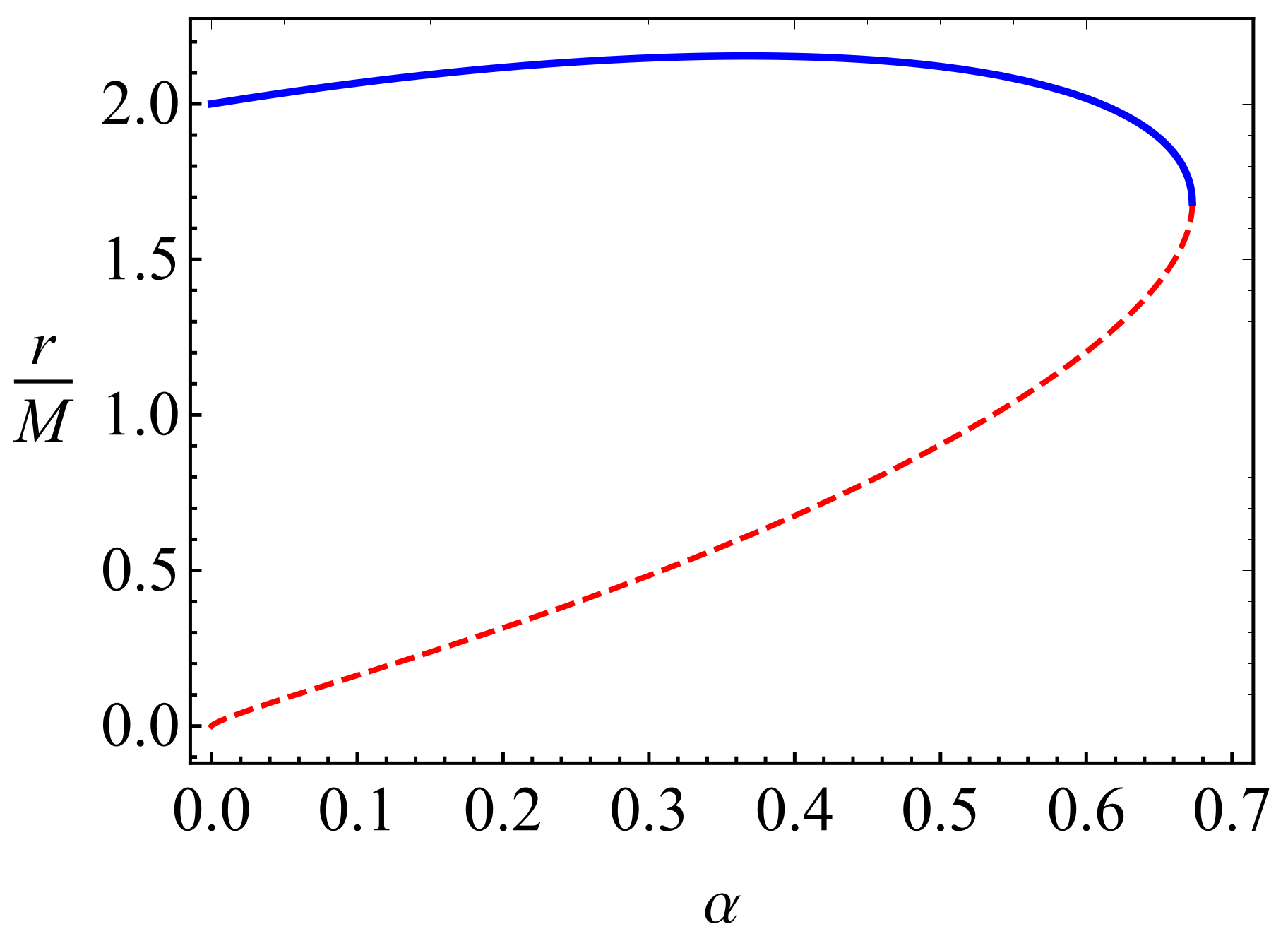
- If we have extreme regular MOG BH and the two horizons coincide (see Figure 2).
3. Test Particle Motion
3.1. Equation of Motion
3.2. Stable Circular Orbits
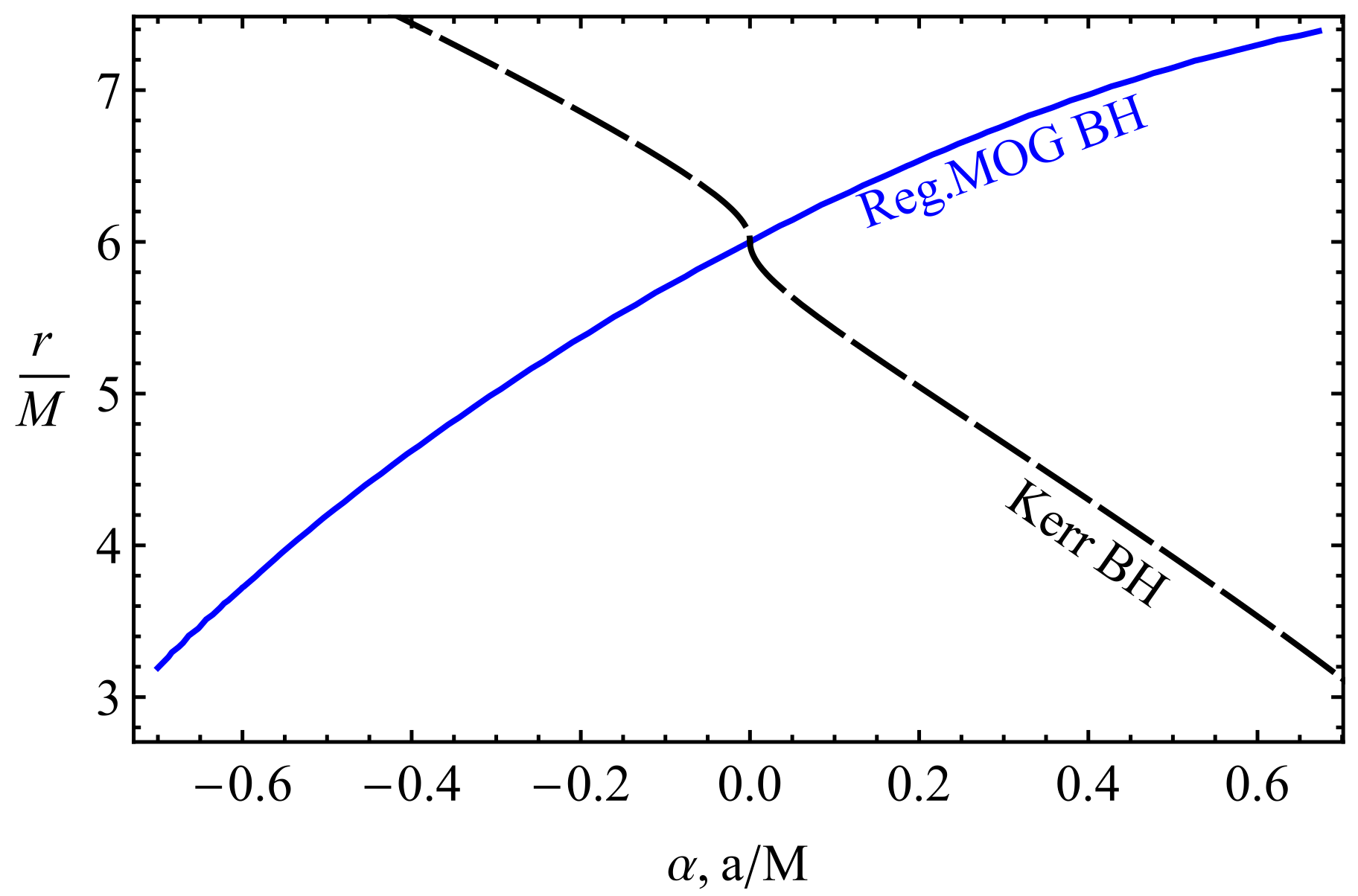
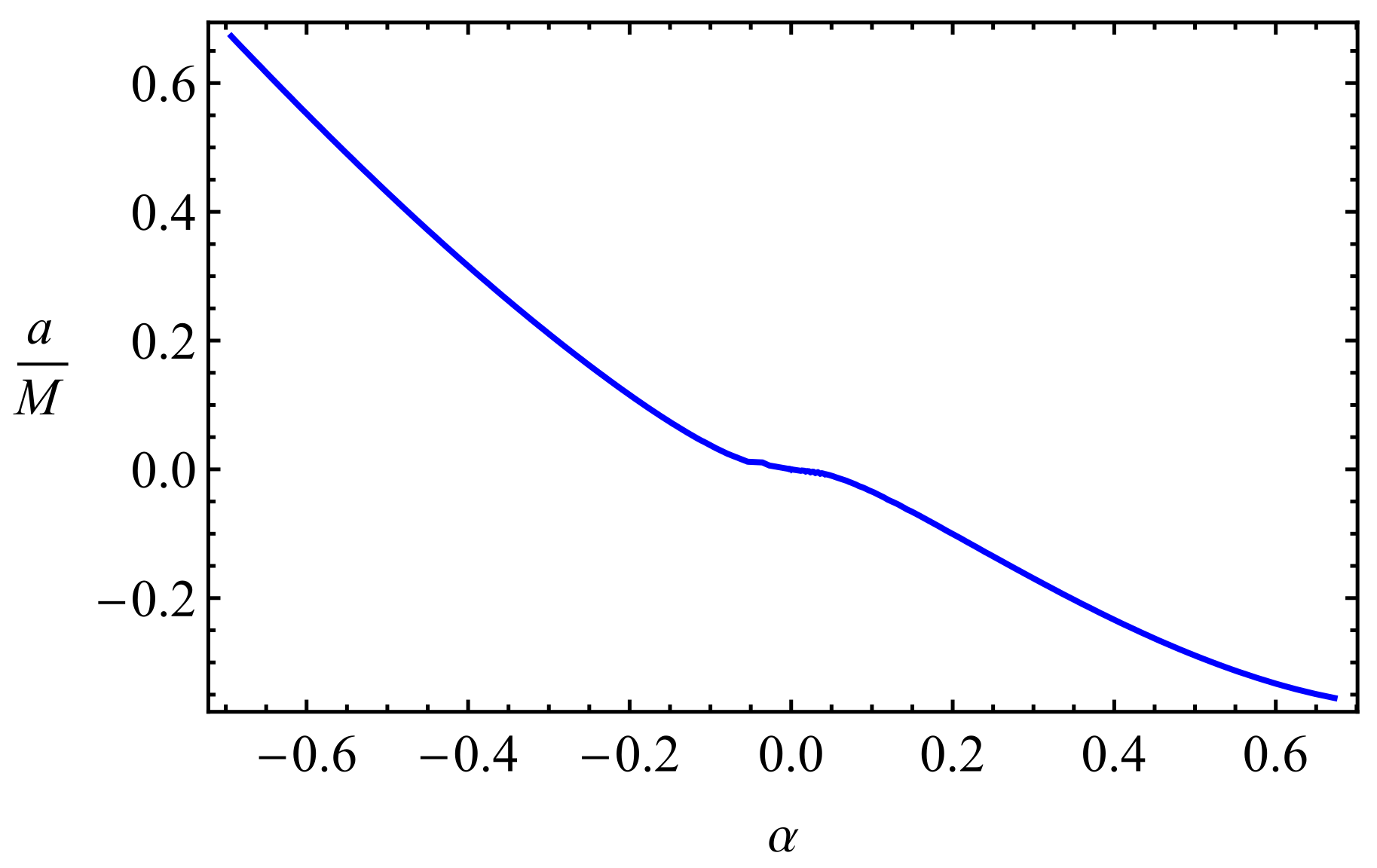
3.3. Regular MOG BH versus Kerr BH: The Same ISCO
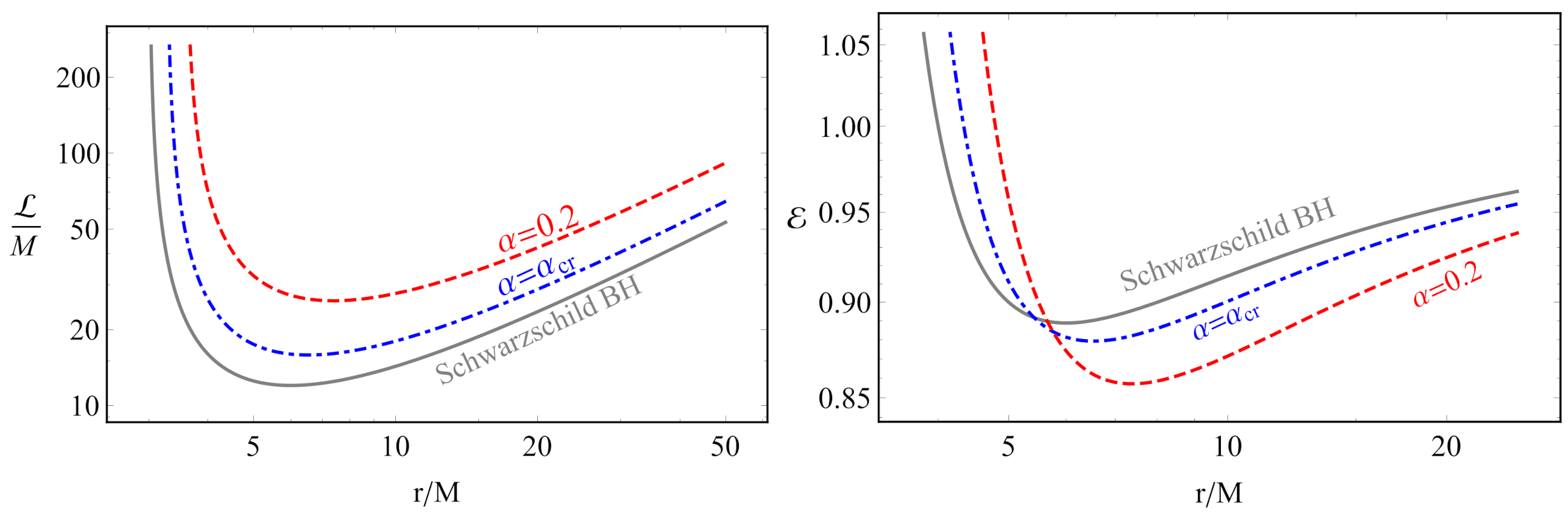
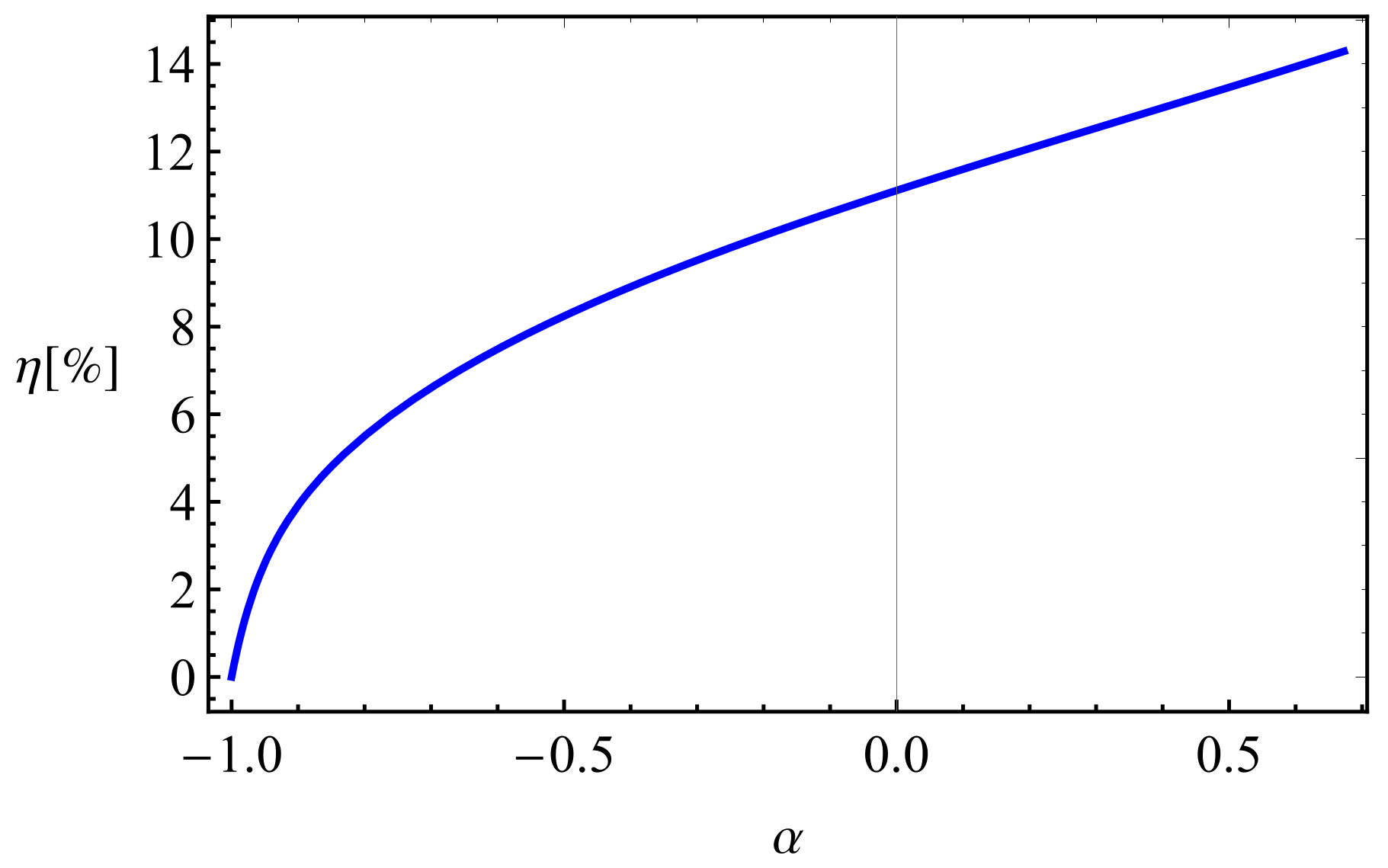
3.4. The Energy Extraction Efficiency
3.5. Regular MOG BH versus Kerr BH: The Same Energy Efficiency
4. Fundamental Frequencies
4.1. Keplerian Frequency
4.2. Harmonic Oscillations
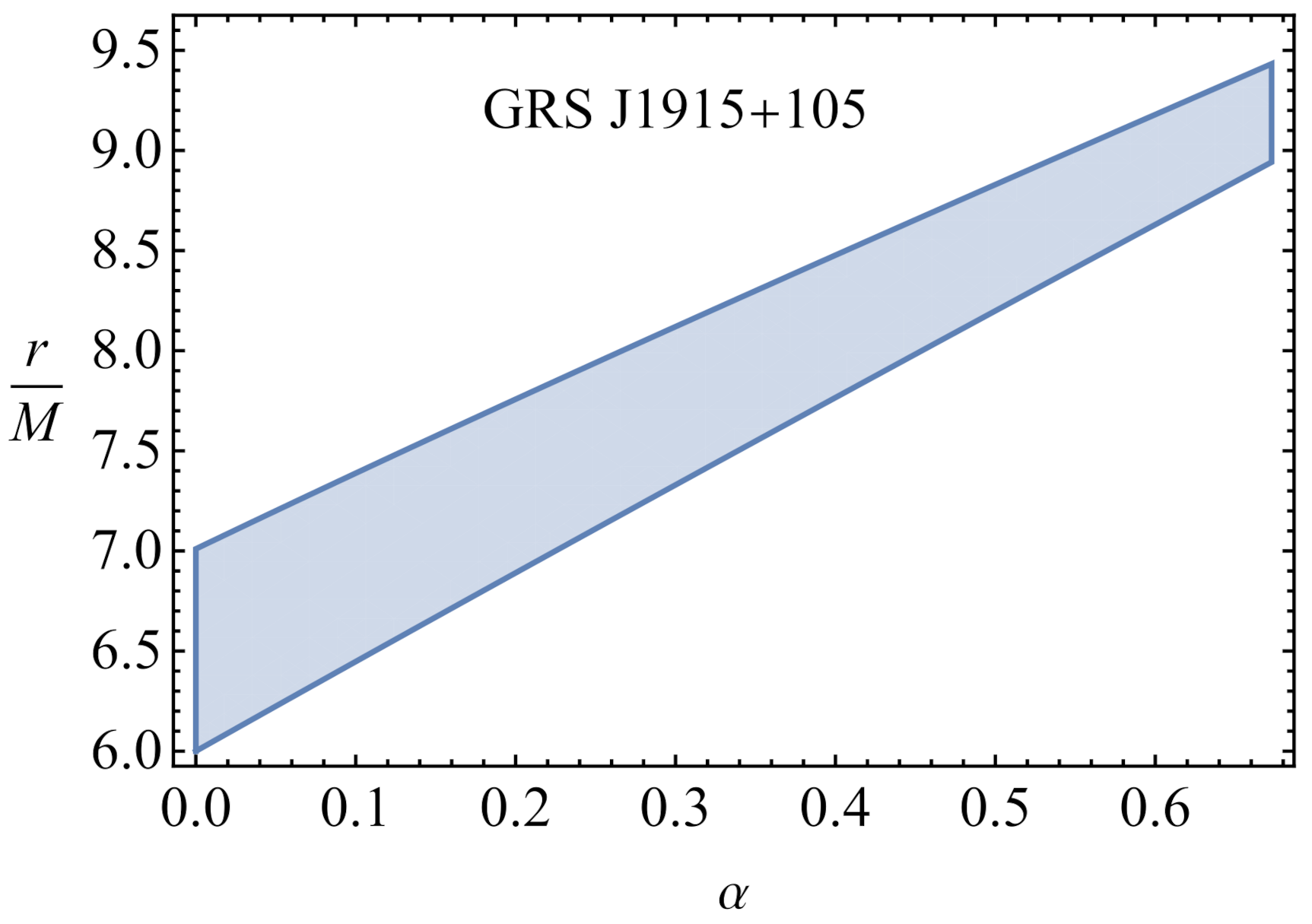
5. Astrophysical Applications
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Bambi, C. Testing black hole candidates with electromagnetic radiation. Rev. Mod. Phys. 2017, 89, 025001. [Google Scholar] [CrossRef]
- Vogt, R. The U.S. LIGO Project. In Proceedings of the Sixth Marcel Grossman Meeting on General Relativity, Kyoto, Japan, 23–29 June 1991; Sato, H., Nakamura, T., Eds.; World Scientific: Singapore, 1992; pp. 244–266. [Google Scholar]
- Akiyama, K. First M87 Event Horizon Telescope Results. I. The Shadow of the Supermassive Black Hole. Astrophys. J. 2019, 875, L1. [Google Scholar] [CrossRef]
- Akiyama, K. First M87 Event Horizon Telescope Results. VI. The Shadow and Mass of the Central Black Hole. Astrophys. J. 2019, 875, L6. [Google Scholar] [CrossRef]
- Schwarzschild, K. Über das Gravitationsfeld eines Massenpunktes nach der Einsteinchen Theorie. Sitzungsberichte Der KöNiglich PreußIschen Akad. Der Wiss. 1916, 92, 189–196. [Google Scholar]
- Reissner, H. Über die Eigengravitation des elektrischen Feldes nach der Einsteinschen Theorie. Ann. Der Phys. 1916, 355, 106–120. [Google Scholar] [CrossRef]
- Nordström, G. On the Energy of the Gravitation field in Einstein’s Theory. K. Ned. Akad. Van Wet. Proc. Ser. B Phys. Sci. 1918, 20, 1238–1245. [Google Scholar]
- Bardeen, J.M. Kerr Metric Black Holes. Nature 1970, 226, 64. [Google Scholar] [CrossRef]
- Bahrami Asl, B.; Hendi, S.H.; Sajadi, S.N. Complexity Conjecture of Regular Electric Black Holes. arXiv 2021, arXiv:2102.12515. [Google Scholar]
- Hendi, S.H.; Sajadi, S.N.; Khademi, M. Physical properties of a regular rotating black hole: Thermodynamics, stability, and quasinormal modes. Phys. Rev. D 2021, 10, 064016. [Google Scholar] [CrossRef]
- Hayward, S.A. Energy and entropy conservation for dynamical black holes. Phys. Rev. D 2004, 70, 104027. [Google Scholar] [CrossRef]
- Dymnikova, I.; Korpusik, M. Thermodynamics of Regular Cosmological Black Holes with the de Sitter Interior. Entropy 2011, 13, 1967–1991. [Google Scholar] [CrossRef]
- Dymnikova, I.; Galaktionov, E. Regular rotating electrically charged black holes and solitons in non-linear electrodynamics minimally coupled to gravity. Class. Quantum Gravity 2015, 32, 165015. [Google Scholar] [CrossRef]
- Toshmatov, B.; Bambi, C.; Ahmedov, B.; Stuchlík, Z.; Schee, J. Scalar perturbations of nonsingular nonrotating black holes in conformal gravity. Phys. Rev. D 2017, 96, 064028. [Google Scholar] [CrossRef]
- Toshmatov, B.; Stuchlík, Z.; Ahmedov, B. Rotating black hole solutions with quintessential energy. Eur. Phys. J. Plus 2017, 132, 98. [Google Scholar] [CrossRef]
- Bronnikov, K.A. Comment on “Regular Black Hole in General Relativity Coupled to Nonlinear Electrodynamics”. Phys. Rev. Lett. 2000, 85, 4641. [Google Scholar] [CrossRef]
- Toshmatov, B.; Stuchlík, Z.; Ahmedov, B. Comment on “Construction of regular black holes in general relativity”. Phys. Rev. D 2018, 98, 028501. [Google Scholar] [CrossRef]
- Toshmatov, B.; Ahmedov, B.; Abdujabbarov, A.; Stuchlík, Z. Rotating regular black hole solution. Phys. Rev. D. 2014, 89, 104017. [Google Scholar] [CrossRef]
- Toshmatov, B.; Abdujabbarov, A.; Stuchlík, Z.; Ahmedov, B. Quasinormal modes of test fields around regular black holes. Phys. Rev. D 2015, 91, 083008. [Google Scholar] [CrossRef]
- Stuchlík, Z.; Schee, J. Circular geodesic of Bardeen and Ayon-Beato-Garcia regular black-hole and no-horizon spacetimes. Int. J. Mod. Phys. D 2015, 24, 50020. [Google Scholar] [CrossRef]
- Schee, J.; Stuchlík, Z. Gravitational lensing and ghost images in the regular Bardeen no-horizon spacetimes. JCAP 2015, 6, 48. [Google Scholar] [CrossRef]
- Toshmatov, B.; Stuchlík, Z.; Schee, J.; Ahmedov, B. Electromagnetic perturbations of black holes in general relativity coupled to nonlinear electrodynamics. Phys. Rev. D 2018, 97, 084058. [Google Scholar] [CrossRef]
- Toshmatov, B.; Stuchlík, Z.; Ahmedov, B. Electromagnetic perturbations of black holes in general relativity coupled to nonlinear electrodynamics: Polar perturbations. Phys. Rev. D 2018, 98, 085021. [Google Scholar] [CrossRef]
- Moffat, J.W.; Zhoolideh Haghighi, M.H. Modified gravity (MOG) and the Abell 1689 cluster acceleration data. Eur. Phys. J. Plus 2017, 132, 417. [Google Scholar] [CrossRef]
- Jawad, A.; Ali, F.; Jamil, M.; Debnath, U. Dynamics of Particles Around a Regular Black Hole with Nonlinear Electrodynamics. Commun. Theor. Phys. 2016, 66, 509. [Google Scholar] [CrossRef]
- Hussain, S.; Jamil, M. Timelike geodesics of a modified gravity black hole immersed in an axially symmetric magnetic field. Phys. Rev. D 2015, 92, 043008. [Google Scholar] [CrossRef]
- Babar, G.Z.; Jamil, M.; Lim, Y.K. Dynamics of a charged particle around a weakly magnetized naked singularity. Int. J. Mod. Phys. D 2016, 25, 1650024. [Google Scholar] [CrossRef]
- Bañados, M.; Silk, J.; West, S.M. Kerr Black Holes as Particle Accelerators to Arbitrarily High Energy. Phys. Rev. Lett. 2009, 103, 111102. [Google Scholar] [CrossRef]
- Majeed, B.; Jamil, M. Dynamics and center of mass energy of colliding particles around black hole in f(R) gravity. Int. J. Mod. Phys. D 2017, 26, 1741017. [Google Scholar] [CrossRef]
- Zakria, A.; Jamil, M. Center of mass energy of the collision for two general geodesic particles around a Kerr–Newman-Taub-NUT black hole. J. High Energy Phys. 2015, 2015, 147. [Google Scholar] [CrossRef]
- Brevik, I.; Jamil, M. Black holes in the turbulence phase of viscous rip cosmology. Int. J. Geom. Methods Mod. Phys. 2019, 16, 1950030. [Google Scholar] [CrossRef]
- Chen, S.; Wang, M.; Jing, J. Chaotic motion of particles in the accelerating and rotating black holes spacetime. J. High Energy Phys. 2016, 2016, 82. [Google Scholar] [CrossRef]
- Hashimoto, K.; Tanahashi, N. Universality in chaos of particle motion near black hole horizon. Phys. Rev. D 2017, 95, 024007. [Google Scholar] [CrossRef]
- Dalui, S.; Majhi, B.R.; Mishra, P. Presence of horizon makes particle motion chaotic. Phys. Lett. B 2019, 788, 486–493. [Google Scholar] [CrossRef]
- Han, W. Chaos and dynamics of spinning particles in Kerr spacetime. Gen. Relativ. Gravit. 2008, 40, 1831–1847. [Google Scholar] [CrossRef][Green Version]
- De Moura, A.P.S.; Letelier, P.S. Chaos and fractals in geodesic motions around a nonrotating black hole with halos. Phys. Rev. E 2000, 61, 6506–6516. [Google Scholar] [CrossRef]
- Moffat, J.W. Scalar tensor vector gravity theory. JCAP 2006, 2006, 004. [Google Scholar] [CrossRef]
- Moffat, J.W.; Rahvar, S. The MOG weak field approximation and observational test of galaxy rotation curves. Mon. Not. R. Astron. Soc. 2013, 436, 1439–1451. [Google Scholar] [CrossRef]
- Moffat, J.W. Modified gravity black holes and their observable shadows. Eur. Phys. J. C 2015, 75, 130. [Google Scholar] [CrossRef]
- Moffat, J.W. Black holes in modified gravity (MOG). Eur. Phys. J. C 2015, 75, 175. [Google Scholar] [CrossRef]
- Moffat, J.W.; Toth, V.T. Rotational velocity curves in the Milky Way as a test of modified gravity. Phys. Rev. D 2015, 91, 043004. [Google Scholar] [CrossRef]
- Wondrak, M.F.; Nicolini, P.; Moffat, J.W. Superradiance in modified gravity (MOG). JCAP 2018, 2018, 021. [Google Scholar] [CrossRef]
- Moffat, J.W.; Toth, V.T. The bending of light and lensing in modified gravity. Mon. Not. R. Astron. Soc. 2009, 397, 1885–1892. [Google Scholar] [CrossRef]
- Atamurotov, F.; Abdujabbarov, A.; Rayimbaev, J. Weak gravitational lensing Schwarzschild-MOG black hole in plasma. Eur. Phys. J. C 2021, 81, 118. [Google Scholar] [CrossRef]
- Rayimbaev, J.; Tadjimuratov, P. Can modified gravity silence radio-loud pulsars? Phys. Rev. D 2020, 102, 024019. [Google Scholar] [CrossRef]
- Haydarov, K.; Rayimbaev, J.; Abdujabbarov, A.; Palvanov, S.; Begmatova, D. Magnetized particle motion around magnetized Schwarzschild-MOG black hole. Eur. Phys. J. C 2020, 80, 399. [Google Scholar] [CrossRef]
- Bambi, C.; Cárdenas-Avendaño, A.; Dauser, T.; García, J.A.; Nampalliwar, S. Testing the Kerr Black Hole Hypothesis Using X-Ray Reflection Spectroscopy. Astrophys J. 2017, 842, 76. [Google Scholar] [CrossRef]
- Rezzolla, L.; Yoshida, S.; Zanotti, O. Oscillations of vertically integrated relativistic tori-I. Axisymmetric modes in a Schwarzschild space-time. Mon. Not. R. Astron. Soc. 2003, 344, 978–992. [Google Scholar] [CrossRef]
- Török, G.; Kotrlová, A.; Srámková, E.; Stuchlík, Z. Confronting the models of 3:2 quasiperiodic oscillations with the rapid spin of the microquasar GRS 1915+105. Astron. Astrophys. 2011, 531, A59. [Google Scholar] [CrossRef]
- Stuchlík, Z.; Kološ, M. Models of quasi-periodic oscillations related to mass and spin of the GRO J1655-40 black hole. Astron. Astrophys. 2016, 586, A130. [Google Scholar] [CrossRef]
- Šrámková, E.; Török, G.; Kotrlová, A.; Bakala, P.; Abramowicz, M.A.; Stuchlík, Z.; Goluchová, K.; Kluźniak, W. Black hole spin inferred from 3:2 epicyclic resonance model of high-frequency quasi-periodic oscillations. Astron. Astrophys. 2015, 578, A90. [Google Scholar] [CrossRef][Green Version]
- Kotrlová, A.; Šrámková, E.; Török, G.; Stuchlík, Z.; Goluchová, K. Super-spinning compact objects and models of high-frequency quasi-periodic oscillations observed in Galactic microquasars. II. Forced resonances. Astron. Astrophys. 2017, 607, A69. [Google Scholar] [CrossRef][Green Version]
- Stuchlík, Z.; Kotrlová, A.; Török, G. Resonant radii of kHz quasi-periodic oscillations in Keplerian discs orbiting neutron stars. Astron. Astrophys. 2011, 525, A82. [Google Scholar] [CrossRef]
- Kološ, M.; Tursunov, A.; Stuchlík, Z. Possible signature of the magnetic fields related to quasi-periodic oscillations observed in microquasars. Eur. Phys. J. C 2017, 77, 860. [Google Scholar] [CrossRef]
- Stuchlík, Z.; Kotrlová, A.; Török, G. Multi-resonance orbital model of high-frequency quasi-periodic oscillations: Possible high-precision determination of black hole and neutron star spin. Astron. Astrophys. 2013, 552, A10. [Google Scholar] [CrossRef]
- Kološ, M.; Shahzadi, M.; Stuchlík, Z. Quasi-periodic oscillations around Kerr-MOG black holes. Eur. Phys. J. C 2020, 80, 133. [Google Scholar] [CrossRef]
- Stuchlík, Z.; Kološ, M.; Kovář, J.; Slaný, P.; Tursunov, A. Influence of Cosmic Repulsion and Magnetic Fields on Accretion Disks Rotating around Kerr Black Holes. Universe 2020, 6, 26. [Google Scholar] [CrossRef]
- Rezzolla, L.; Yoshida, S.; Maccarone, T.J.; Zanotti, O. A new simple model for high-frequency quasi-periodic oscillations in black hole candidates. Mon. Not. R. Astron. Soc. 2003, 344, L37–L41. [Google Scholar] [CrossRef]
- Stuchlík, Z.; Kološ, M. HF QPOs in the neutron star binary system XTE J1701-462 fitted by the model of oscillating string loops. In Proceedings of the RAGtime 14–16: Workshops on Black Holes and Neutron Stars, Opava, Czech Republic, 11–19 October 2014; pp. 243–255. [Google Scholar]
- Stuchlík, Z.; Kološ, M. Mass of intermediate black hole in the source M82 X-1 restricted by models of twin high-frequency quasi-periodic oscillations. Mon. Not. R. Astron. Soc. 2015, 451, 2575–2588. [Google Scholar] [CrossRef]
- Ayón-Beato, E.; García, A. Regular Black Hole in General Relativity Coupled to Nonlinear Electrodynamics. Phys. Rev. Lett. 1998, 80, 5056–5059. [Google Scholar] [CrossRef]
- Mureika, J.R.; Moffat, J.W.; Faizal, M. Black hole thermodynamics in MOdified Gravity (MOG). Phys. Lett. B 2016, 757, 528–536. [Google Scholar] [CrossRef]
- Moffat, J.W. LIGO GW150914 and GW151226 gravitational wave detection and generalized gravitation theory (MOG). Phys. Lett. B 2016, 763, 427–433. [Google Scholar] [CrossRef]
- Rahvar, S.; Moffat, J.W. Propagation of electromagnetic waves in MOG: Gravitational lensing. Mon. Not. R. Astron. Soc. 2019, 482, 4514–4518. [Google Scholar] [CrossRef]
- Moffat, J.W.; Toth, V.T. Masses and shadows of the black holes Sagittarius A* and M87* in modified gravity. Phys. Rev. D 2020, 101, 024014. [Google Scholar] [CrossRef]
- Rayimbaev, J.; Figueroa, M.; Stuchlík, Z.; Juraev, B. Test particle orbits around regular black holes in general relativity combined with nonlinear electrodynamics. Phys. Rev. D 2020, 101, 104045. [Google Scholar] [CrossRef]
- Abdujabbarov, A.; Rayimbaev, J.; Turimov, B.; Atamurotov, F. Dynamics of magnetized particles around 4-D Einstein Gauss–Bonnet black hole. Phys. Dark Universe 2020, 30, 100715. [Google Scholar] [CrossRef]
- Juraeva, N.; Rayimbaev, J.; Abdujabbarov, A.; Ahmedov, B.; Palvanov, S. Distinguishing magnetically and electrically charged Reissner-Nordström black holes by magnetized particle motion. Eur. Phys. J. C 2021, 81, 70. [Google Scholar] [CrossRef]
- Abdujabbarov, A.; Rayimbaev, J.; Atamurotov, F.; Ahmedov, B. Magnetized Particle Motion in γ-Spacetime in a Magnetic Field. Galaxies 2020, 8, 76. [Google Scholar] [CrossRef]
- Bardeen, J.M.; Press, W.H.; Teukolsky, S.A. Rotating Black Holes: Locally Nonrotating Frames, Energy Extraction, and Scalar Synchrotron Radiation. Astrophys. J. 1972, 178, 347–370. [Google Scholar] [CrossRef]
- Novikov, I.D.; Thorne, K.S. Astrophysics of black holes. In Black Holes (Les Astres Occlus); Dewitt, C., Dewitt, B.S., Eds.; Gordon & Breach: New York, NY, USA, 1973; pp. 343–450. [Google Scholar]
- Bian, W.H.; Zhao, Y.H. Accretion Rates and the Accretion Efficiency in AGNs. PASJ 2003, 55, 599–603. [Google Scholar] [CrossRef]
- Bokhari, A.H.; Rayimbaev, J.; Ahmedov, B. Test particles dynamics around deformed Reissner-Nordström black hole. Phys. Rev. D 2020, 102, 124078. [Google Scholar] [CrossRef]
- Stella, L.; Vietri, M.; Morsink, S.M. Correlations in the Quasi-periodic Oscillation Frequencies of Low-Mass X-Ray Binaries and the Relativistic Precession Model. Astrophys. J. 1999, 524, L63–L66. [Google Scholar] [CrossRef]
- Abramowicz, M.A.; Kluźniak, W. A precise determination of black hole spin in GRO J1655-40. Astron. Astrophys. 2001, 374, L19–L20. [Google Scholar] [CrossRef]
- Kato, S. Resonant Excitation of Disk Oscillations by Warps: A Model of kHz QPOs. Publ. Astron. Soc. Jpn. 2004, 56, 905–922. [Google Scholar] [CrossRef]
- Kato, S. Frequency Correlation of QPOs Based on a Resonantly Excited Disk-Oscillation Model. Publ. Astron. Soc. Jpn. 2008, 60, 889. [Google Scholar] [CrossRef]
- Stuchlík, Z.; Vrba, J. Epicyclic Oscillations around Simpson–Visser Regular Black Holes and Wormholes. Universe 2021, 7, 279. [Google Scholar] [CrossRef]



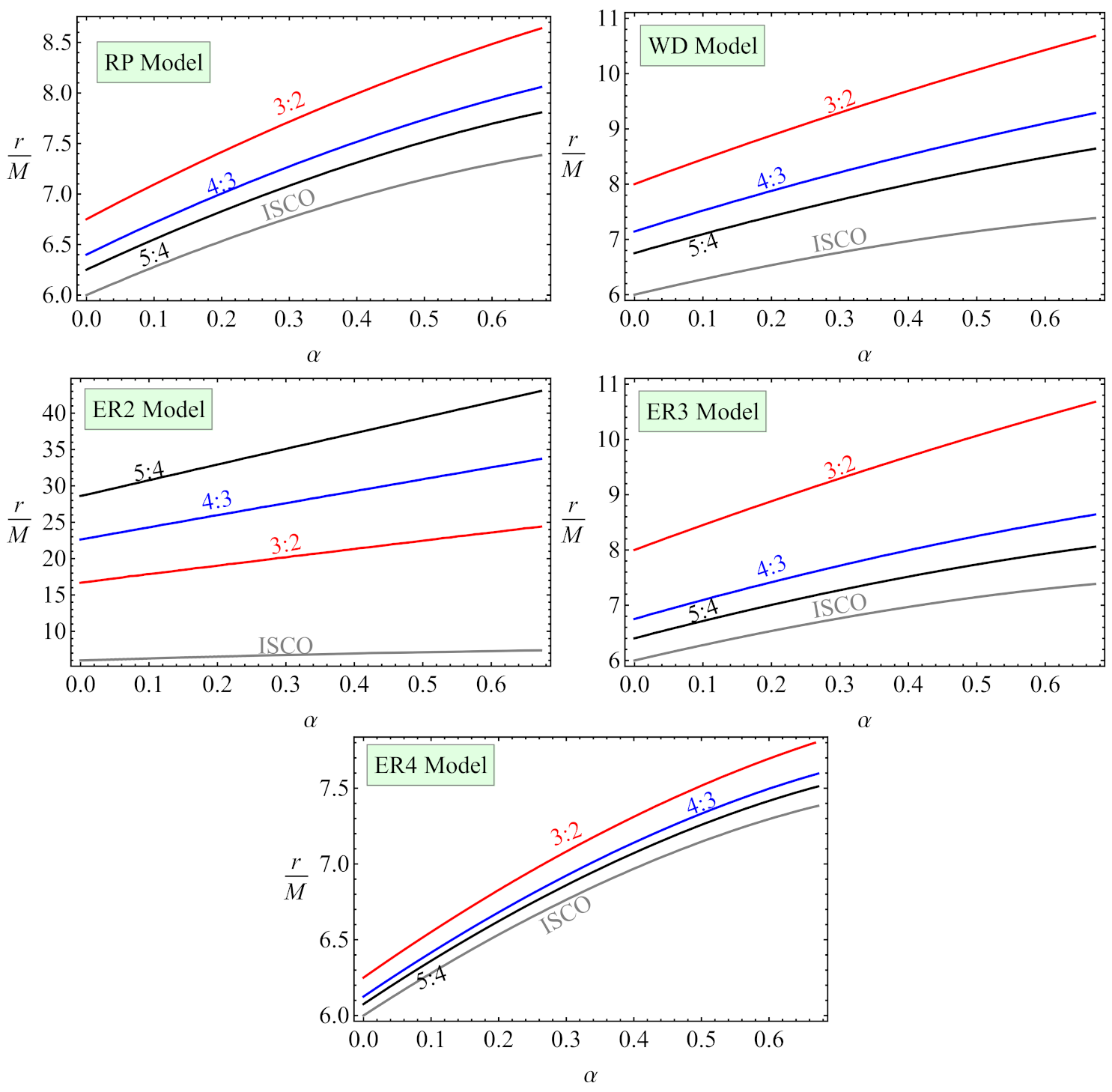
| Models | ||
|---|---|---|
| RP | 100–120 | 150–180 |
| WD | 112–141 | 168–211 |
| ER2 | 26–38 | 39–57 |
| ER3 | 112–143 | 168–215 |
| ER4 | 140–165 | 210–248 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Rayimbaev, J.; Tadjimuratov, P.; Abdujabbarov, A.; Ahmedov, B.; Khudoyberdieva, M. Dynamics of Test Particles and Twin Peaks QPOs around Regular Black Holes in Modified Gravity. Galaxies 2021, 9, 75. https://doi.org/10.3390/galaxies9040075
Rayimbaev J, Tadjimuratov P, Abdujabbarov A, Ahmedov B, Khudoyberdieva M. Dynamics of Test Particles and Twin Peaks QPOs around Regular Black Holes in Modified Gravity. Galaxies. 2021; 9(4):75. https://doi.org/10.3390/galaxies9040075
Chicago/Turabian StyleRayimbaev, Javlon, Pulat Tadjimuratov, Ahmadjon Abdujabbarov, Bobomurat Ahmedov, and Malika Khudoyberdieva. 2021. "Dynamics of Test Particles and Twin Peaks QPOs around Regular Black Holes in Modified Gravity" Galaxies 9, no. 4: 75. https://doi.org/10.3390/galaxies9040075
APA StyleRayimbaev, J., Tadjimuratov, P., Abdujabbarov, A., Ahmedov, B., & Khudoyberdieva, M. (2021). Dynamics of Test Particles and Twin Peaks QPOs around Regular Black Holes in Modified Gravity. Galaxies, 9(4), 75. https://doi.org/10.3390/galaxies9040075








