The overall framework used in this section is the so-called Friedmann model, i.e., we seek a solution of EFE (
9)
for some fictitious metric
representing a maximally symmetric space (we have restricted ourselves for the time being to the flat space case,
). On the r.h.s. of (13) is a similarly fictitious,
-independent e-m tensor
which, in turn, is assumed to represent the coarse-grained matter distribution
with
K a normalized kernel. The time
in (14), denoted also by
, is known as the
conformal time, and the reader is warned to keep that in mind, as the standard equations of cosmology will take a somewhat unusual form. The ‘tilde pseudo tensors’
and
are therefore involved in dynamical changes on cosmological time scales, while the fluctuations around them are only relevant on much smaller scales.
3.1. The Cosmological Redshift
Matter in the universe, even if contributing negligibly to the coarse-grained e-m
, is indispensable in two complementary senses. First, without matter, there are no astronomical objects and no equipment to observe them—the latter mandated by the slimness of our equivalence principle. Second, without matter, there is no ZPF; from the discussion in
Section 2.1, it follows that matter and the ZPF are just different facets of the same physical entity.
We shall represent (the centers of) particles in the universe by a collection of world-lines, , which is compatible with the time-independent homogeneity of . Such world-lines must be those of comoving particles, viz., have the form , so as to respect the above compatibility condition at any time. By virtue of derived from the metric (14) vanishing, those are indeed the world-lines of ‘freely falling’ particles. Mach’s vague principle is thereby given a concrete meaning, as the world-line of a fixed spatial coordinates triplet, belonging to a local frame which is rotating relative to the local comoving frame, will no longer solve the geodesic Equation (12).
Next, we wish to investigate the observational consequences of a gradual change in the intensity of the ZPF over cosmological time scales—a consequence of (21) and (22). The main challenge we face is in the need to give meaning to a comparison of properties of matter at distinct conformal times, without resorting to ‘universal gauges’ at every point in the universe, manifested in the (full) equivalence principle. Instrumental to our analysis is the following
postulate of scale equilibrium: For any given particle species, elementary or composite, there exists a fixed ratio between the (average) energy density of the particle at
x and
. By the symmetry of a Friedman model and the assumption of scale-clustering (
Section 2.1), within each constant-
slice, all such particles should have a common density. However, that the above ratio is
-independent, viz., that the ‘average vacuum energy density’ sets the standard for energy density
7 is more subtle, and although it can be motivated further, we only expect it to emerge as an approximation in a rigorous analysis, incorporating also the fluctuations in the density of matter and its phase transitions over cosmological time scales.
As a first example, we analyze a primary observable in cosmology, known as the
luminosity distance of an isotropically radiating astronomical object,
Above,
, referred to as the
proper flux, is the measured energy-flux in terms of some energy-flux standard, as determined by an astronomer with (fixed) coordinates
(the spatial part
) at conformal-time
, and
L is the object’s total power, or luminosity, as would have been determined by the astronomer, had the remote astronomical object been ‘teleported’ to earth (or the astronomer to the object) from its point in spacetime
(‘S’ for source/star/supernova...) with the retarded conformal-time,
, where
Defining the
proper distance,
, between two points at a given conformal-time as the minimal number of local length gauges exactly fitting between them, the homogeneity of space implies
with
depending on the choice of standard length gauge and on time. Since null geodesics satisfy
, a wave of sufficiently high-frequency,
, viz.,
, retains its wavelength in the
x coordinates. Given the definition of the
redshift,
z,
Equation (25) then implies that a standard length gauge, when teleported to an earlier conformal time, measures a larger coordinates interval by a factor
, i.e.,
or, equivalently,
The proper-flux of a distant object can be computed either classically, via Poynting’s theorem, or by resorting to the language of ‘photons’, with identical results (that the concept of a photon is compatible with a CE ontology is shown in [
16] and in greater detail in [
9]). The former, somewhat longer derivation, appears in
Appendix A. In the case of photons, (26) leads to
(This standard expression can be found in virtually any textbook on GR, but there, the proper-distance derived from the metric is being used). The first term in (29) is just the luminosity,
L (as determined, e.g., by an astronomer near
) divided by the surface area of a sphere with proper radius
, over which the emitted photons are distributed. The second term involves our slim equivalence principle, namely, the assumption that the proportionality constant relating the measured energy and frequency of a photon, both having scaling dimension
, is the same at
and
, hence one power of
, and that the rate at which photons penetrate the sphere of radius
r, on which earth resides, is diminished by another such factor
8. Plugging (29) in (23) gives
Expression (30), coinciding with that of standard cosmology, implies other commonalities: Using (27), the observed angular diameter of a sphere with proper diameter
D is the standard
with
the so-called angular diameter distance. Using (30), the sphere’s surface brightness,
, has the usual
dependence. Both relations apply also in the case of
.
To test our cosmological model against observations, we still need to express
r in (30) as a function of
z or, equivalently,
z as a function of
. To this end, we change coordinates to the local Minkowskian frame at both
and
, implying
there. In these new coordinates, the previously identical coordinate extent of a single wave cycle transforms as
, and the value of the ZPF density as
. We thus arrive at two (locally) Minkowskians frames, with length gauges whose representations are related by a scale transformation (8) for some
. Our postulate of scale equilibrium, combined with the scaling dimension of energy density,
, readily gives the ratio between the local coordinate extents,
ℓ, of the length gauges
Combined with the transformation of
, the definition of the redshift, (
26), gives
To make contact with standard cosmological terminology, we take the derivative of (
27) with respect to
at
. The derivative of
z (at
) is computed using (32),
The (locally) exponential expansion implied by (33) (even for a constant
) is misleading due to the non-physical nature of the conformal time
. To see its physical content, we proceed as follows. Denoting by
the coordinate interval spanned by a comoving standard length-gauge,
and (33) imply the shrinkage
By our slim equivalence principle, this must also be true for the conformal-time interval between two consecutive ticks of a comoving physical clock and, in particular, of a ‘light clock’—two parallel mirrors, separated by a single standard length gauge, with light ray bouncing in between (this choice respects our implicit
choice). It can then be easily shown that the growth rate of
with respect to the time,
, shown by a comoving light-clock (our counterpart of the cosmological time in standard cosmology), satisfies
Choosing
, (
25) mandates
. Setting
in (35) and dividing by
, (
27) then implies that, in our model, corresponding to the usual
z-dependent Hubble parameter, viz.,
(in conformal time), is
, where
The extra (
z + 1) above, reflecting the different time standards of
A and
S, could have been deduced also from (25) and (
28). Combining with (32) and (35) then gives an important relation
which, with (30), gives
with
the locally inferred, dimensionful Hubble’s constant. Equation (35) can be naively interpreted as either an expansion of the universe, a collective shrinkage of matter, or both—
neither will be truly adequate.To fully define a cosmological model, we still need to specify a third equation, complementing (21) and (22) in determining the three unknown functions of
:
. It should be clear that the ratio
is a quantity which, in principle, can be derived from the underlying physics. Specifically, insofar as our postulate of scale equilibrium holds true, each particle can be seen as a standard length gauge undergoing a shrinkage (34). From our postulate of scale equilibrium, the effect this has on
is deduced by taking a large coordinate volume and calculating the resulting differential change in the part of that volume taken by matter, with the obvious result
Cosmic scale phase transitions of matter would only have a marginal influence on the validity of (40), but in the early universe (40) is completely invalid; see
Section 4.
Using (
21) for the mixture
(
20),
(33) can also be written
Eliminating
between (40) and (41) gives
Next, we extend our model to curved spaces, using the metric
where
k is any non-vanishing real. By virtue of the spatial part also describing a homogeneous space, the previous flat space results can essentially be copy-pasted, with only three differences. First,
in (
25) applies only in the local Cartesian coordinates, where
effectively equals
r; the proper distance then becomes the number of standard length gauges exactly fitting along a geodesic curve connecting
and
at
, making this number an extremum with respect to small deformations of the curve. Second, the r.h.s. of (
22) receives a term
. Third, in the expression of (30), for the luminosity distance,
. To arrive at these results, one only needs to decompose the previous teleportation operation into two commuting stages: spatial, using the three metric, and the previous, temporal stage, implementing some
local scale transformation by a factor
.
As a concrete example for the above procedure, consider the observational signature of a standard ruler. A transverse comoving ruler of length ℓ is defined as two mutually close comoving points of equal r, measured by a local observer to have a proper separation equal to . To compute its observed angular size, , we decompose its teleportation to its location in spacetime, , into two commuting stages. In the first, the three-metric in the observer’s hyper-surface is used to move the ruler from the origin to . Making standard use of the spatial part of the metric (43), the angular separation of the ruler’s end-points is calculated to be . Then, temporally teleporting the ruler to the retarded conformal time increases by a factor which is dropped out by the definition of a comoving standard ruler. As null geodesics in our coordinates x satisfy , is clearly the measured angular size of the ruler. With (31) we then get , which is the expression for the angular size also in standard cosmology.
Standard rulers ‘pointing at us’ have their observational signature in the redshift difference between their end points. Repeating the above procedure, the spatial teleportation stage gives a coordinate separation . After temporally teleporting to the retarded time, (38) readily gives a redshift difference , again, formally coinciding with standard cosmology.
Finally, we compare the differential proper-time progression, viz., that of
, as a function of the redshift
z, with the corresponding progression of the cosmological time,
t, in standard cosmology. Using (38), and (
28) (equivalently (
27)), gives
as in standard cosmology.
3.2. Comparison with Observations
The slimness of our equivalence principle necessitates prudence in associating the dynamical variables of the model with observations. We have already seen that to convert a coordinate difference to proper distance, one must divide the former by the coordinate extent,
, of a standard length gauge. Similarly, to convert
to the corresponding measurement, one must divide it by the numerical value of the energy-density associated with a standard energy-density gauge, expressed in local
x-coordinates—call it
. Now, the postulate of scale equilibrium tells us that
. It then follows from (40) that the proper (viz., measured) density behaves as
, which is the standard, purely geometrical expression for (non-relativistic) matter density. Similarly, (32) gives the standard
. The fluctuations,
, representing objects in the universe, must also be divided by
before being compared with the fluctuations at a different
surface. Using the transformation rule of standard gauges (as defined at the end of
Section 2), it can then easily be shown that the measured (“Proper”) values of
and
(and of the speed-of-light
) as deduced by a local observer from linearized/Newtonian gravity, are teleportation invariant, viz., just some numbers which depend on the choice of standard gauges being teleported.
The above scheme for coping with scale covariance is sufficient if one is interested in the reports of future and past generations of astronomers relative to ours, but it cannot provide the proportionality constants, and , without explicitly representing the gauges as CE solutions. This fact restricts the reports of present-day astronomers using our model to dimensionless quantities only which, by their nature, do not depend on such proportionality constants. Whether knowingly or not, this is a restriction cosmologists tend to take upon themselves anyhow.
As the ultimate result must be dimensionless, rather than expressing it as a combination of independent dimensionful quantities, one may as well do the same with a maximal set of independent dimensionless quantities. Besides experiment-specific dimensionless quantities, such as angles, redshifts, or luminosity ratios, there are only five such independent quantities:
(where
, etc.); the baryonic and radiation ‘density parameters’, respectively,
where
in our dark-matter-free model. Note indeed the absence of
and
.
The remaining two dimensionless parameters could be
k and
, but a more conventional choice would be:
with
the current Hubble distance.
The baryonic density parameter, is directly determined from the ‘count’ of baryonic matter. The figure published by the Planck collaboration, , is consistent with the direct count. Nonetheless, given that just a couple of years ago, the direct count gave only half this value, the Planck figure should better be viewed as a lower bound, and with a much larger uncertainty. The radiation parameter is currently negligible, .
Our related dark-matter model ([
9] and
Section 4) must be further advanced in order to provide independent stringent bounds on
but those will most likely be on the order of 1. As for
, all model-independent bounds on its value are consistent with
. Finally, as the Hubble distance is so much larger than the size of galaxies, any value of
which is not absurdly large is compatible with observations involving local gravitation.
Moving to actual observations, there are two primary ways of determining the
relative values of the luminosity distance,
, at different redshifts (or equivalently, by (31), of
or
), using either standard candles, primarily type Ia supernovae, or the so-called transverse BAO standard ruler [
17]. Comparing the relative values of the Hubble distance,
, makes use of the line-of-sight BAO ruler. Note that the ‘ruler’s length’,
ℓ, and the ‘candle’s luminosity’,
L, do not appear in such relative distances.
To compute our model’s
and
, one propagates backwards in
(from
) the three dynamical variables,
, and
, via the system (21), (22) and (40), with
,
, and
as initial conditions, arriving via (32) and (42) at
and
, respectively, which is used to express
in terms of
z. For astronomers to currently see a redshift (rather than blueshift),
must be a monotonically increasing function in the current epoch of the universe, further taken to be positive. Like in standard cosmology, the map from our model’s seven
9 variables and constants to the five (current, dimensionless) observable parameters, implies a two-dimensional parameter degeneracy.
Normalizing both distances by
for convenience, in the regime
, our model reduces to standard (Einstein–de-Sitter) cosmology. This is so because, in such regime,
. Substituting in (32)
, using (40), viz.,
, and solving for
gives
as in standard, conformal time cosmology (when
). From (38) then follows that the luminosity distance (30) has the standard
z-dependence.
The above coincidence certainly does not imply that the principle of scale covariance is invalid in a negligible-ZPF universe. It does explain, nonetheless, why complete disregard for such a fundamental principle—assumed, of course, to be respected by nature—could have resulted in a cosmological model which is not in severe conflict with observations.
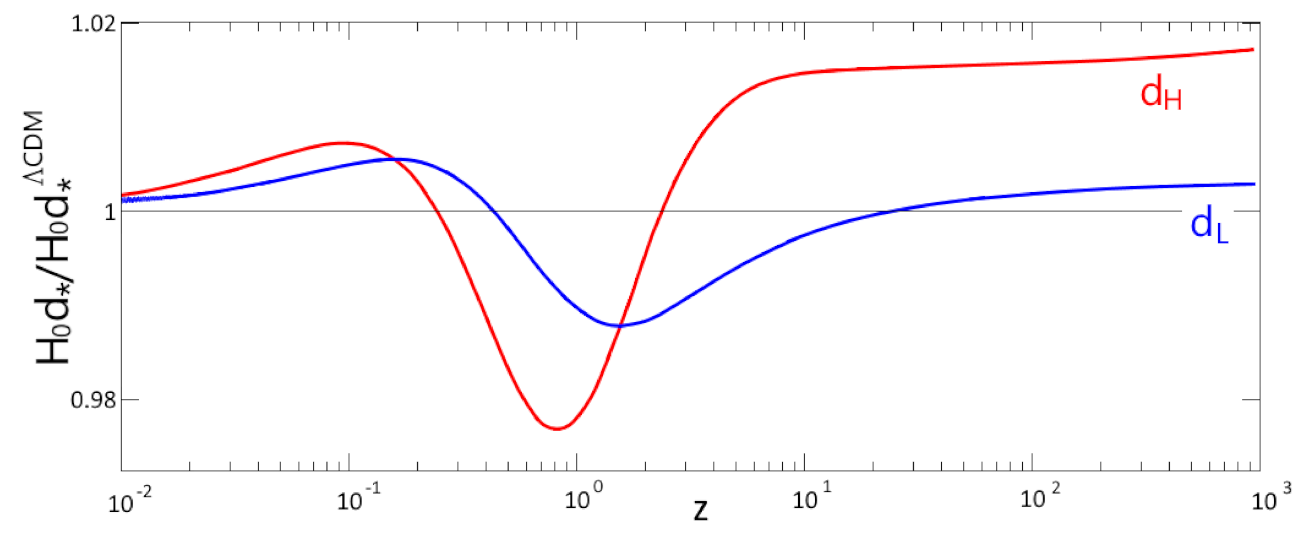
It appears, then, that consistency with observations requires
but, remarkably, our model comes close to reproducing the
CDM curves already at
(
Figure 1). This relatively small value of
is further mandated by our associated dark-matter model, which is briefly discussed in
Section 4 next.
In the regime, spacetime becomes effectively Minkowskian on large enough scales. To wit, since in this regime , we get in (32) and a vanishing (42), implying the vanishing of . This regime is relevant to the far future where indeed vanishes asymptotically, but the de-Sitter (future conformal-) singularity implies the divergence of in (42), and the vanishing of necessarily comes with the divergence of . A nearly balanced battle of infinities ends with an eternally increasing—in terms of proper-time (36)—unbounded , viz., a ‘big-rip’ scenario. Note, nonetheless, that once all standard gauges are ripped apart, teleportation becomes ill-defined; hence, the predictions of our model, as is, are meaningless (as in the case of the very early universe which is discussed below).