Neutrino Charge in a Magnetized Media
Abstract
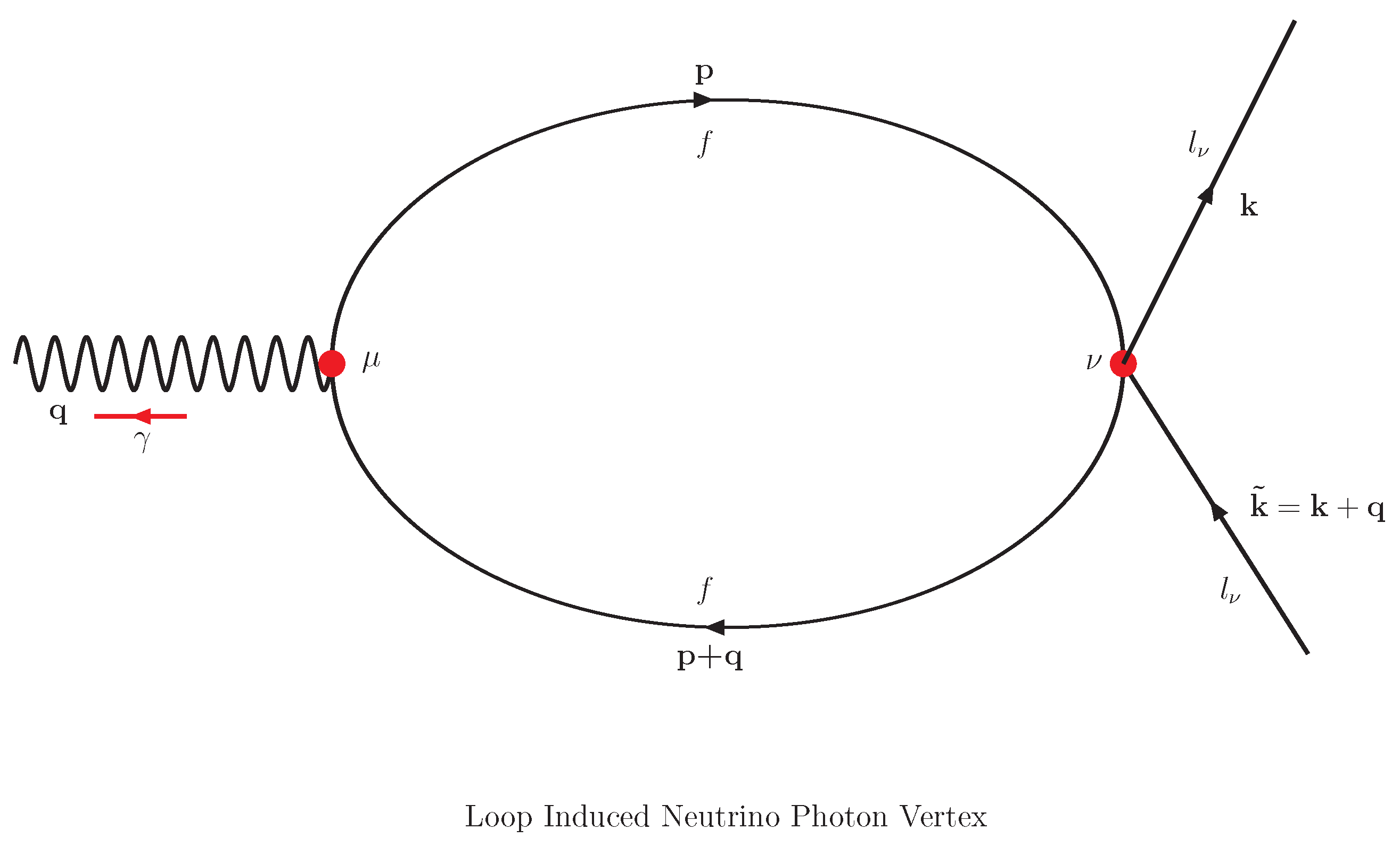
1. Introduction
1.1. Intrinsic Charge of Neutrino
1.2. Induced Charge of Neutrino
2. Formalism
3. General Analysis
4. One Loop Calculation of the Axial Polarisation Tensor
4.1. The Expression for in Thermal Medium and in the Presence of a Background Uniform Magnetic Field
4.2. to Even and Odd Orders in the Magnetic Field
5. Gauge Invariance
5.1. Gauge Invariance for to Even Orders in the External Field
5.2. Gauge Invariance for to Odd Orders in the External Field
6. Effective Charge
6.1. Effective Charge to Odd Orders in External Field
6.1.1. Effective Charge for
6.1.2. Effective Charge for
6.2. Effective Charge at Even Order in the External Field and Their Coupling with the Magnetic Fields
7. Conclusions
8. Outlook
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Appendix A. Structure of Axial Polarization Tensor
References
- Raffelt, G.G. Stars as Laboratories for Fundamental Physics; University of Chicago Press: Chicago, IL, USA, 1996. [Google Scholar]
- Weinberg, S. Gravitation and Cosmology: Principles and Applications of General Theory of Relativity; John Wiley & Sons: New York, NY, USA, 1972. [Google Scholar]
- Winberg, S. Cosmology; Oxford University Press: Oxford, UK, 2008. [Google Scholar]
- Dolgov, A.D. Cosmological Implications of Neutrinos. Surv. High Energy Phys. 2002, 17, 91–114. [Google Scholar] [CrossRef][Green Version]
- Llamas-Bugarin, A.; Gutierrez-Rodriguez, A.; Gonzalez-Sanchez, A.; Hernandez-Ruiz, M.A.; Espinoza-Garrido, A.; Chubikalo, A. Stellar energy loss rates beyond the standard model. Eur. Phys. J. Plus 2020, 135, 481. [Google Scholar] [CrossRef]
- Raffelt, G.G. Astrophysics probes of particle physics. Phys. Rept. 2000, 333, 593. [Google Scholar] [CrossRef]
- Raffelt, G.G. New bound on neutrino dipole moments from globular-cluster stars. Phys. Rev. Lett. 1990, 64, 2856. [Google Scholar] [CrossRef]
- Viaux, N.; Catelan, M.; Stetson, P.B.; Raffelt, G.G.; Redondo, J.; Valcarce, A.A.R. Particle-physics constraints from the globular cluster M5: Neutrino dipole moments. Astron. Astrophys. 2013, 558, A12. [Google Scholar] [CrossRef]
- Arceo-Díaz, S.; Schröder, K.P.; Zuber, K.; Jack, D. Constraint on the magnetic dipole moment of neutrinos by the tip-RGB luminosity in ω-Centauri. Astropart. Phys. 2015, 70, 1. [Google Scholar] [CrossRef]
- Giunti, C.; Kouzakov, K.; Li, Y.F.; Lokhov, A.; Studenikin, A.; Zhou, S. Electromagnetic neutrinos in laboratory experiments and astrophysics. Annalen Phys. 2016, 528, 198. [Google Scholar] [CrossRef]
- Grimus, W.; Maltoni, M.; Schwetz, T.; Tortola, M.A.; Valle, J.W.F. Constraining Majorana neutrino electromagnetic properties from the LMA-MSW solution of the solar neutrino problem. Nucl. Phys. B 2003, 648, 376–396. [Google Scholar] [CrossRef][Green Version]
- Cooperstein, J. Neutrinos in supernovae. Phys. Rep. 1988, 163, 95. [Google Scholar] [CrossRef]
- Bethe, H.A. Supernova mechanisms. Rev. Mod. Phys. 1990, 62, 801. [Google Scholar] [CrossRef]
- Bludman, S.; Feng, D.H.; Gaisser, T.; Pittel, S. The Physics of Supernovae. Phys. Rep. 1995, 256, 1. [Google Scholar]
- Bethe, H.A.; Wilson, J.R. Revival of a stalled supernova shock by neutrino heating. Astroph. J. 1985, 295, 14. [Google Scholar] [CrossRef]
- Janka, H.T. Neutrino emission from Supernovae. In Handbook of Supernovae; Alsabti, A., Murdin, P., Eds.; Springer: Cham, Switzerland, 2017; ISBN 978-3-319-21845-8. [Google Scholar]
- Yamazaki, R.; Toma, K.; Ioka, K.; Nakamura, T. Tail emission of prompt gamma-ray burst jets. MNRAS 2006, 369, 311. [Google Scholar] [CrossRef][Green Version]
- Bernardini, M.G. Gamma-ray bursts and magnetars: Observational signatures and predictions. JHEAP 2015, 7, 64. [Google Scholar] [CrossRef]
- Wijers, R. The burst, the burster and its lair. Nature 1998, 393, 13. [Google Scholar] [CrossRef]
- Kumar, P.; Zhang, B. The physics of gamma-ray bursts and relativistic jets. Phys. Rep. 2015, 561, 1. [Google Scholar] [CrossRef]
- Horowitz, C.J.; Li, G. Cumulative Parity Violation in Supernovae. Phys. Rev. Lett. 1998, 80, 3694. [Google Scholar] [CrossRef]
- Hines, D.; Peer, A. Physics of Gamma-Ray Bursts Prompt Emission. Adv. Astron. 2015, 2015, 907321. [Google Scholar]
- Arzoumanian, Z.; Chernoffs, D.F.; Corder, J.M. The velocity distribution of isolated radio pulsars. Astrophys. J. 2002, 568, 289. [Google Scholar] [CrossRef]
- Balaji, S.; Ramirez-Quezada, M.; Zhou, Y.-L. CP violation and circular polarisation in neutrino radiative decay. J. High Energy Phys. 2020, 4, 178. [Google Scholar] [CrossRef]
- Grigoriev, A.; Kupcheva, E.; Ternov, A. Neutrino spin oscillations in polarized matter. Phys. Lett. B 2019, 797, 135861. [Google Scholar] [CrossRef]
- Abdi, M.; Mohammadi, R.; Xue, S.-S.; Zarei, M. Distinguishing Dirac from Majorana neutrinos in a microwave cavity. arXiv 2004, arXiv:1909.01536. [Google Scholar]
- Fujikawa, K.; Shrock, R. The Magnetic Moment of a massive neutrino and neutrino spin rotation. Phys. Rev. Lett. 1980, 45, 963. [Google Scholar] [CrossRef]
- Nowakowski, M.; Paschos, E.A.; Rodriguez, J.M. All electromagnetic form-factors. Eur. J. Phys. 2005, 26, 545. [Google Scholar] [CrossRef]
- Wong, T.H.; Li, H.B. Neutrino magnetic moments. Mod. Phys. Lett. A 2005, 20, 1103. [Google Scholar] [CrossRef]
- Balantekin, A.B. Neutrino magnetic moment. AIP Conf. Proc. 2006, 847, 128. [Google Scholar]
- Giunti, C.; Studenikin, A. Neutrino electromagnetic properties. Phys. Atom.Nucl. 2009, 72, 2089. [Google Scholar] [CrossRef]
- Studenikin, A. Neutrino magnetic moment: A window to new physics. Nucl. Phys. Proc. Suppl. 2009, 188, 220. [Google Scholar] [CrossRef]
- Broggini, C.; Giunti, C.; Studenikin, A. Electromagnetic properties of neutrinos. Adv. High Energy Phys. 2012, 2012, 459526. [Google Scholar] [CrossRef]
- Akhmedov, E. Majorana neutrinos and other Majorana particles: Theory and experiment. arXiv 2014, arXiv:1412.3320. [Google Scholar]
- Giunti, C.; Studenikin, A. Neutrino electromagnetic interactions: A window to new physics. Rev. Mod. Phys. 2015, 87, 531. [Google Scholar] [CrossRef]
- Studenikin, A. Status and perspectives of neutrino magnetic moments. J. Phys. Conf. Ser. 2016, 718, 062076. [Google Scholar]
- Studenikin, A. Electromagnetic properties of neutrinos: Three new phenomena in neutrino spin oscillations. EPJ Web Conf. 2016, 125, 04018. [Google Scholar] [CrossRef]
- Beda, A.G.; Brudanin, V.B.; Egorov, V.G.; Medvedev, D.V.; Pogosov, V.S.; Shirchenko, M.V. The results of search for the neutrino magnetic moment in GEMMA experiment. Adv. High Energy Phys. 2012, 2012, 350150. [Google Scholar] [CrossRef]
- Agostini, M.; Borexino Collaboration. Limiting neutrino magnetic moments with Borexino Phase-II solar neutrino data. Phys. Rev. D 2017, 96, 091103. [Google Scholar] [CrossRef]
- Bell, N.F.; Cirigliano, V.; Ramsey-Musolf, M.J.; Vogel, P.; Wise, M.B. How magnetic is the Dirac neutrino? Phys. Rev. Lett. 2005, 95, 151802. [Google Scholar] [CrossRef] [PubMed]
- Bell, N.F.; Gorchtein, M.; Ramsey-Musolf, M.J.; Vogel, P.; Wang, P. Model independent bounds on magnetic moments of Majorana neutrinos. Phys. Lett. B 2006, 642, 377. [Google Scholar] [CrossRef]
- Studenikin, A. New bounds on neutrino electric millicharge from limits on neutrino magnetic moment. Europhys. Lett. 2014, 107, 21001, Erratum in Europhys. Lett. 2014, 107, 39901. [Google Scholar] [CrossRef]
- Gninenko, S.; Krasnikov, N.; Rubbia, A. New limit on milicharged particles from reactor neutrino experiments and the PVLAS anomaly. Phys. Rev. D 2007, 75, 075014. [Google Scholar] [CrossRef]
- Zyla, P.A. Partice Data Group. Prog. Theor. Exp. Phys. 2020, 2020, 083C01. [Google Scholar]
- Studenikin, A.I.; Tokarev, I. Millicharged neutrino with anomalous magnetic moment in rotating magnetized matter. Nucl. Phys. B 2014, 884, 396. [Google Scholar] [CrossRef]
- Bernabeu, J.; Papavassiliou, J.; Binosi, D. The neutrino charge radius in the presence of fermion masses. Nucl. Phys. B 2005, 716, 352. [Google Scholar]
- Kouzakov, K.A.; Studenikin, A.I. Electromagnetic properties of massive neutrinos in low-energy elastic neutrino-electron scattering. Phys. Rev. D 2017, 95, 055013. [Google Scholar] [CrossRef]
- Cisneros, A. Effect of neutrino magnetic moment on solar neutrino observations. Astrophys. Space Sci. 1971, 10, 87. [Google Scholar] [CrossRef]
- Studenikin, A. Neutrinos in electromagnetic fields and moving media. Phys. Atom. Nucl. 2004, 67, 993. [Google Scholar] [CrossRef]
- Studenikin, A. Neutrino in magnetic fields: From the first studies to the new effects in neutrino oscillations. arXiv 2004, arXiv:0407010. [Google Scholar]
- Kartavtsev, A.; Raffelt, G.G.; Vogel, H. Neutrino propagation in media: Flavor-, helicity-, and pair correlations. Phys. Rev. D 2015, 91, 125020. [Google Scholar] [CrossRef]
- Studenikin, A. Neutrino spin and spin-flavour oscillations in transversally moving or polarized matter. J. Phys. Conf. Ser. 2017, 888, 012221. [Google Scholar] [CrossRef]
- Gouvea, A.; Shalgar, S. Effect of transition magnetic moments on collective supernova neutrino oscillations. J. Cosmol. Astropart. Phys. 2012, 1210, 027. [Google Scholar] [CrossRef]
- Gouvea, A.; Shalgar, S. Transition magnetic moments and collective neutrino oscillations: Three-flavor effects and detectability. J. Cosmol. Astropart. Phys. 2013, 1304, 018. [Google Scholar] [CrossRef]
- Kosmas, T.S. Non-standard neutrino-nucleus interactions. In Proceedings of the Invited Talk in Neutrinos and Dark Matter in Nuclear Physics 2015 (NDM-15), Jyvaskyla, Finland, 1–5 June 2015. [Google Scholar]
- Schechter, J.; Valle, J.W.F. Majorana neutrinos and magnetic fields. Phys. Rev. D 1981, 24, 1883, Erratum in 1982, D2, 283. [Google Scholar] [CrossRef]
- Shrock, R.E. Electromagnetic properties and decays of Dirac and Majorana neutrinos in a general class of gauge theories. Nucl. Phys. B 1982, 206, 359. [Google Scholar] [CrossRef]
- Kayser, B. Majorana Neutrinos and their Electromagnetic Properties. Phys.Rev. D 1982, 26, 1662. [Google Scholar] [CrossRef]
- Nieves, J.F. Electromagnetic properties of majorana neutrinos. Phys. Rev. D 1982, 26, 3152. [Google Scholar] [CrossRef]
- Beacom, J.F.; Vogel, P. Neutrino magnetic moments, flavor mixing, and the superKamiokande solar data. Phys. Rev. Lett. 1999, 83, 5222–5225. [Google Scholar] [CrossRef]
- Papoulias, D.; Kosmas, T. Neutrino transition magnetic moments within the non-standard neutrino–nucleus interactions. Phys. Lett. B 2015, 747, 454–459. [Google Scholar] [CrossRef]
- Beringer, J. Review of Particle Physics (RPP). Phys. Rev. D 2012, 86, 010001. [Google Scholar] [CrossRef]
- Tortola, M. Constraining neutrino magnetic moment with solar and reactor neutrino data. PoS AHEP 2003, 2003, 022. [Google Scholar]
- Kosmas, T.S.; Miranda, O.G.; Papoulias, D.K.; Tortola, J.W.F. Probing neutrino magnetic moments at the Spallation Neutron Source facility. Phys. Rev. D 2015, 92, 013011. [Google Scholar] [CrossRef]
- Wong, H. A Search of Neutrino Magnetic Moments with a High-Purity Germanium Detector at the Kuo-Sheng Nuclear Power Station. Phys. Rev. D 2007, 75, 012001. [Google Scholar] [CrossRef]
- Wong, H.T.; Li, H.-B.; Lin, S.-T. Enhanced sensitivities for the searches of neutrino magnetic moments through atomic ionization. Phys. Rev. Lett. 2010, 105, 061801. [Google Scholar] [CrossRef]
- Deniz, M. Measurement of Neutrino-Electron Scattering Cross-Section with a CsI(Tl) Scintillating Crystal Array at the Kuo-Sheng Nuclear Power Reactor. Phys. Rev. D 2010, 81, 072001. [Google Scholar] [CrossRef]
- Vogel, P.; Engel, J. Neutrino electromagnetic form-factors. Phys. Rev. D 1989, 39, 3378. [Google Scholar] [CrossRef]
- Miranda, O.G. Constraining the neutrino magnetic moment with anti- neutrinos from the sun. Phys. Rev. Lett. 2004, 93, 051304. [Google Scholar] [CrossRef]
- Miranda, O.G.; Rashba, T.I.; Rez, A.I.; Valle, J.W.F. Enhanced solar anti-neutrino flux in random magnetic fields. Phys. Rev. D 2004, 70, 113002. [Google Scholar] [CrossRef]
- Barranco, J.; Miranda, O.; Rashba, T. Improved limit on electron neutrino charge radius through a new evaluation of the weak mixing angle. Phys. Lett. B 2008, 662, 431. [Google Scholar] [CrossRef][Green Version]
- Hirsch, M.; Nardi, E.; Restrepo, D. Bounds on the tau and muon neutrino vector and axial vector charge radius. Phys. Rev. D 2003, 67, 033005. [Google Scholar] [CrossRef]
- Bernabeu, J.; Cabral-Rosetti, L.; Papavassiliou, J.; Vidal, J. On the charge radius of the neutrino. Phys. Rev. D 2000, 62, 113012. [Google Scholar] [CrossRef]
- Simon, E. SICANE: A Detector array for the measurement of nuclear recoil quenching factors using a monoenergetic neutron beam. Nucl. Instrum. Meth. A 2003, 507, 643. [Google Scholar] [CrossRef]
- Barranco, J. Constraining non-standard interactions in nu/e e or anti- nu/e e scattering. Phys. Rev. D 2006, 73, 113001. [Google Scholar] [CrossRef]
- Barranco, J.; Miranda, O.; Rashba, T. Low energy neutrino experiments sensitivity to physics beyond the Standard Model. Phys. Rev. D 2007, 76, 073008. [Google Scholar] [CrossRef]
- Miranda, O.; Nunokawa, H. Non standard neutrino interactions. arXiv 2015, arXiv:1505.06254. [Google Scholar]
- Giunti, C.; Kouzakov, K.A.; Li, Y.F.; Lokhov, A.V.; Studenikin, A.I.; Zhou, S. Electromagnetic neutrinos in terrestrial experiments and astrophysics. arXiv 2015, arXiv:1506.05387. [Google Scholar]
- Kosmas, T.; Vergados, J.; Civitarese, O.; Faessler, A. Study of the muon number violating mu to e conversion in a nucleus by using quasiparticle RPA. Nucl. Phys. A 1994, 570, 637. [Google Scholar] [CrossRef]
- Kosmas, T.S. Exotic μ−→e− conversion in nuclei: Energy moments of the transition strength and average energy of the outgoing e−. Nucl. Phys. A 2001, 683, 443. [Google Scholar] [CrossRef]
- Beda, A.; Brudanin, V.; Egorov, V.; Medvedev, D.; Pogosov, V. Gemma experiment: The results of neutrino magnetic moment search. Phys. Part Nucl. Lett. 2013, 10, 139. [Google Scholar] [CrossRef]
- Nieves, J.F.; Pal, P.B. Induced charge of neutrinos in a medium. Phys. Rev. D 1994, 49, 1398. [Google Scholar] [CrossRef]
- Mohapatra, R.N.; Pal, P.B. Massive neutrinos in Physics and Astrophysics. In World Scientific Lecture Notes in Physics; World Scientific: Singapore, 1998; p. 60. [Google Scholar]
- Oraevsky, V.N.; Semikoz, V.B.; Smorodinsky, Y.A. Polarization Loss and Induced Electric Charge of Neutrinos in Plasmas. JETP Lett. 1986, 43, 709. [Google Scholar]
- Altherr, T.; Salati, P. The electric charge of neutrinos and plasmon decay. Nuc. Phys. B 1994, 421, 662. [Google Scholar] [CrossRef][Green Version]
- Babu, K.S.; Mohapatra, R.N. Quantization of electric charge from anomaly constraints and a Majorana neutrino. Phys. Rev. D 1990, 41, 271. [Google Scholar] [CrossRef]
- Foot, R.; Joshi, G.C.; Lew, H.; Volkas, R. Charge quantization in the standard model and some of its extentations. Mod. Phys. Lett. A 1990, 5, 2721. [Google Scholar] [CrossRef]
- Foot, R.; Lew, H.; Volkas, R. Electric-charge quantization. J. Phys. G Nucl. Part. Phys. 1993, 19, 361. [Google Scholar] [CrossRef]
- Davidson, S.; Hannestad, S.; Raffelt, G. Updated bounds on milli-charged particles. J. High Energy Phys. 2000, 5, 003. [Google Scholar] [CrossRef]
- Geng, C.Q.; Marshak, R.E. Uniqueness of quark and lepton representations in the standard model from the anomalies viewpoint. Phys. Rev. D 1989, 39, 693. [Google Scholar] [CrossRef] [PubMed]
- Minahan, J.A.; Ramond, P.; Warner, R.C. Comment on anomaly cancellation in the standard model. Phys. Rev. D 1990, 41, 715. [Google Scholar] [CrossRef]
- Bardeen, W.A.; Gastmans, R.; Lautrup, B. Static quantities in Weinberg’s model of weak and electromagnetic interactions. Nucl. Phys. B 2002, 46, 319. [Google Scholar] [CrossRef]
- Gross, D.; Jackiw, R. Effect of Anomalies on Quasi-Renormalizable Theories. Phys. Rev. D 1972, 6, 477. [Google Scholar] [CrossRef]
- Monyonko, N.M.; Reid, J.H. What is the charge radius of the neutrino? Prog. Theor. Phys. 1985, 73, 3. [Google Scholar] [CrossRef]
- Hill, R.J.; Tomalak, O. On the effective theory of neutrino-electron and neutrino-quark interactions. Phys. Lett. B 2020, 805, 135466. [Google Scholar] [CrossRef]
- Lucio Martinez, J.L.; Rosado, A.; Zepeda, A. Neutrino charge in the linear Rξ gauge. Phys. Rev. D 1983, 29, 7. [Google Scholar]
- Dvornikov, M.; Studenikin, A. Electric charge and magnetic moment of a massive neutrino. Phys. Rev. D 2004, 69, 073001. [Google Scholar] [CrossRef]
- DeRaad, L.L., Jr.; Milton, K.A.; Hari, N.D. Photon decay into neutrinos in a strong magnetic field. Phys. Rev. D 1976, 14, 3326. [Google Scholar] [CrossRef]
- Ioannisian, A.N.; Raffelt, G.G. Cherenkov radiation by massless neutrinos in a magnetic field. Phys. Rev. D 1997, 55, 7038. [Google Scholar] [CrossRef]
- Vainshtein, A. Perturbative and non perturbative renormalisation of anomalous quark triangles. Phys. Lett. B 2003, 569, 187–193. [Google Scholar] [CrossRef]
- Schwinger, J. On gauge Invariance and Vacuum Polarization. Phys. Rev. 1951, 82, 664. [Google Scholar] [CrossRef]
- Tsai, W.Y. Modified electron propagation function in strong magnetic fields. Phys. Rev. D 1974, 10, 1342. [Google Scholar] [CrossRef]
- Dittrich, W. Effective Lagrangians at finite temperatures. Phys. Rev. D 1979, 19, 2385. [Google Scholar] [CrossRef]
- Elmfors, P.; Grasso, D.; Raffelt, G. Neutrino dispersion in magnetized media and spin oscillations in the early universe. Nucl. Phys. B 1996, 479, 3. [Google Scholar] [CrossRef]
- Ganguly, A.K.; Konar, S.; Pal, P.B. Faraday effect: A field theoretical point of view. Phys. Rev. D 1999, 60, 105014. [Google Scholar] [CrossRef]
- Bhattacharya, K.; Ganguly, A.K. Axial-vector-vector amplitude and neutrino effective charge in a magnetized medium. Phys. Rev. D 2003, 68, 053011. [Google Scholar] [CrossRef]
- Bhattacharya, K.; Ganguly, A.K.; Konar, S. Neutrinos interactions in a magnetized medium. Phys. Rev. D 2001, 65, 013007. [Google Scholar] [CrossRef]
- Bellac, M.L. Thermal Field Theory; Cambridge University Press: Cambridge, UK, 1996. [Google Scholar]
- Ofengeim, D.D.; Zyuzin, D.A. Thermal spectrum and neutrino cooling rate of the vela pulsar. Particles 2018, 1, 194–202. [Google Scholar] [CrossRef]
- Barbiellini, G.; Coeeoni, G. Electric charge of the neutrinos from SN1987A. Nature 1987, 329, 21. [Google Scholar] [CrossRef]
- Davidson, S.; Campbell, B.; Bailey, D.C. Limits on particles of small electric charge. Phys. Rev. D 1991, 43, 2314. [Google Scholar] [CrossRef]
- Caprini, C.; Ferreira, P.G. Constraints on the electrical charge asymmetry of the universe. J. Cosmol. Astropart. Phys. 2005, 2, 006. [Google Scholar] [CrossRef]
- Chen, J.-W.; Chi, H.-C.; Li, H.-B.; Liu, C.-P.; Singh, L.; Wong, H.-T.; Wu, C.L.; Wu, C.-P. Constraints on millicharged neutrinos via analysis of data from atomic ionisations with germanium detectors at sub-keV sensitivities. Phys. Rev. D 2014, 90, 011301(R). [Google Scholar] [CrossRef]
- Chen, J.W.; Chi, H.C.; Huang, K.N.; Li, H.B.; Liu, C.P.; Singh, L.; Wong, H.T.; Wu, C.L.; Wu, C.P. Constraining neutrino electromagnetic properties by germanium detectors. Phys. Rev. D 2015, 91, 013005. [Google Scholar] [CrossRef]
- Kosmas, T.S.; Miranda, O.G.; Papoulias, D.K.; Tortola, M.; Valle, J.W.F. Sensitivities to neutrino electromagnetic properties at the TEXONO experiment. Phys. Lett. B 2015, 750, 459. [Google Scholar] [CrossRef]
- Raffelt, G.G. Limits on neutrino electromagnetic properties—An update. Phys. Rep. 1999, 320, 319. [Google Scholar] [CrossRef]
- Fukuda, Y.; Super-Kamiokande Collaboration. Evidence for oscillations for atmospheric neutrinos. Phys. Rev. Lett. 1998. [Google Scholar] [CrossRef]
- Ahmad, Q.R.; SNO Collaboration. Measurement of the Rate of νe + d → p + p + e− interactions produced by 8B solar neutrinos at the sudbury neutrino observatory. Phys. Rev. Lett. 2001, 87, 071301. [Google Scholar] [CrossRef] [PubMed]
- Chaubey, A.; Jaiswal, M.K.; Ganguly, A.K. Exploring scalar-photon interactions in energetic astrophysical events. Phys. Rev. D 2020, 102, 123029. [Google Scholar] [CrossRef]
- Ganguly, A.K.; Jain, P.; Mandal, S. Photon and axion oscillation in a magnetized medium: A general treatment. Phys. Rev. D 2009, 79, 115014. [Google Scholar] [CrossRef]
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ganguly, A.K.; Singh, V.; Singh, D.; Chaubey, A. Neutrino Charge in a Magnetized Media. Galaxies 2021, 9, 22. https://doi.org/10.3390/galaxies9020022
Ganguly AK, Singh V, Singh D, Chaubey A. Neutrino Charge in a Magnetized Media. Galaxies. 2021; 9(2):22. https://doi.org/10.3390/galaxies9020022
Chicago/Turabian StyleGanguly, Avijit K., Venktesh Singh, Damini Singh, and Ankur Chaubey. 2021. "Neutrino Charge in a Magnetized Media" Galaxies 9, no. 2: 22. https://doi.org/10.3390/galaxies9020022
APA StyleGanguly, A. K., Singh, V., Singh, D., & Chaubey, A. (2021). Neutrino Charge in a Magnetized Media. Galaxies, 9(2), 22. https://doi.org/10.3390/galaxies9020022





