Design, Analysis and Experiment of a Modular Deployable Continuum Robot
Abstract
1. Introduction
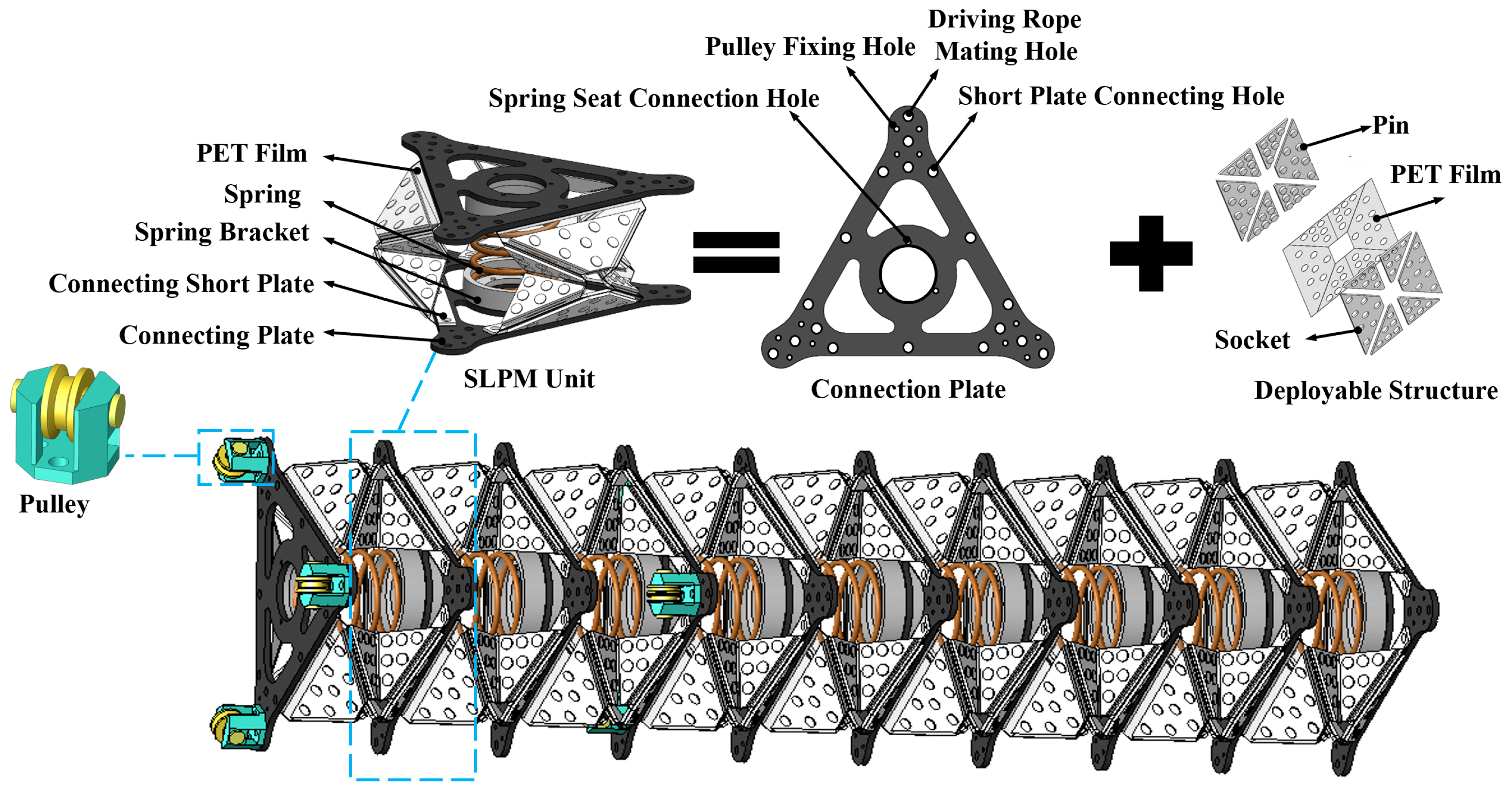
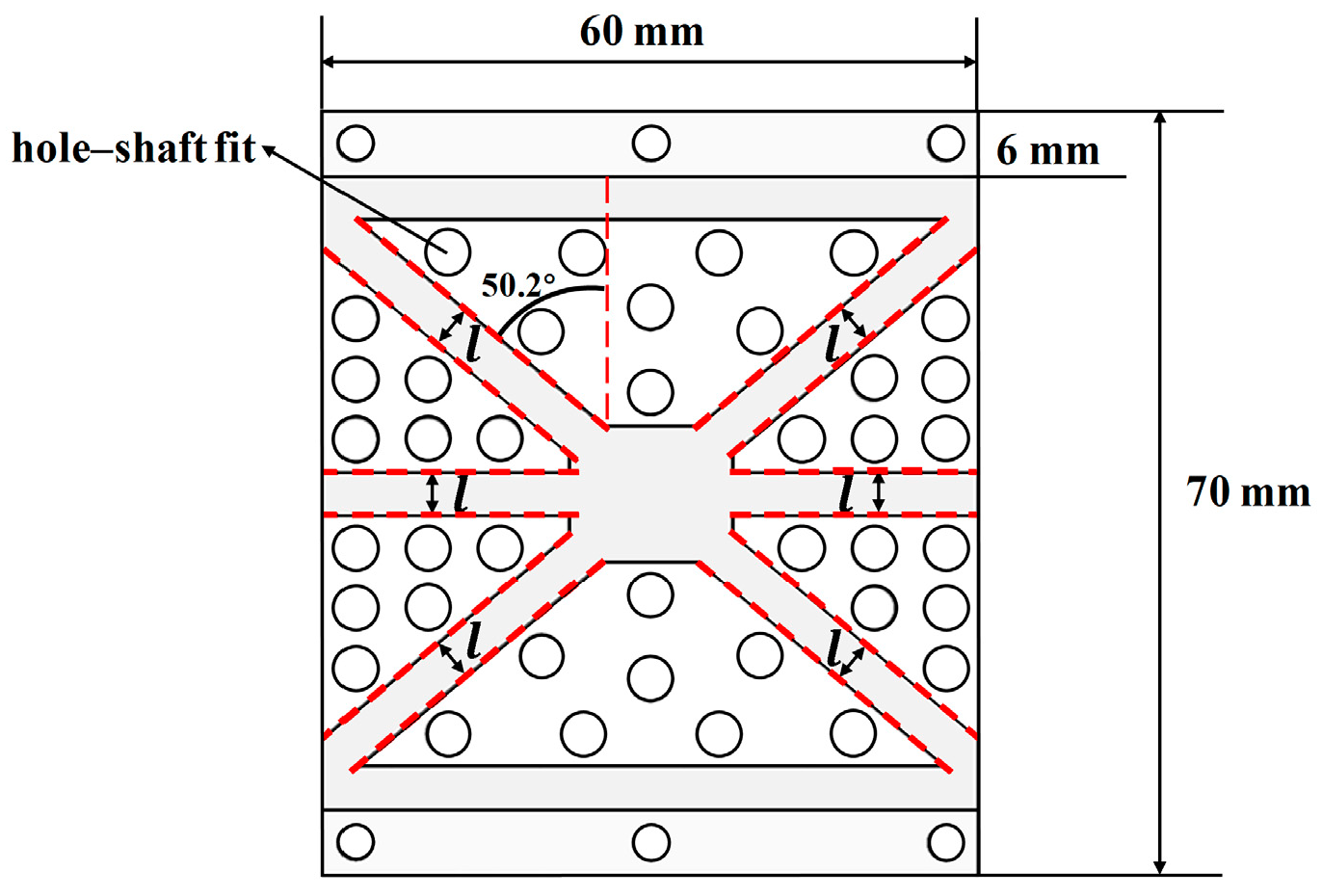
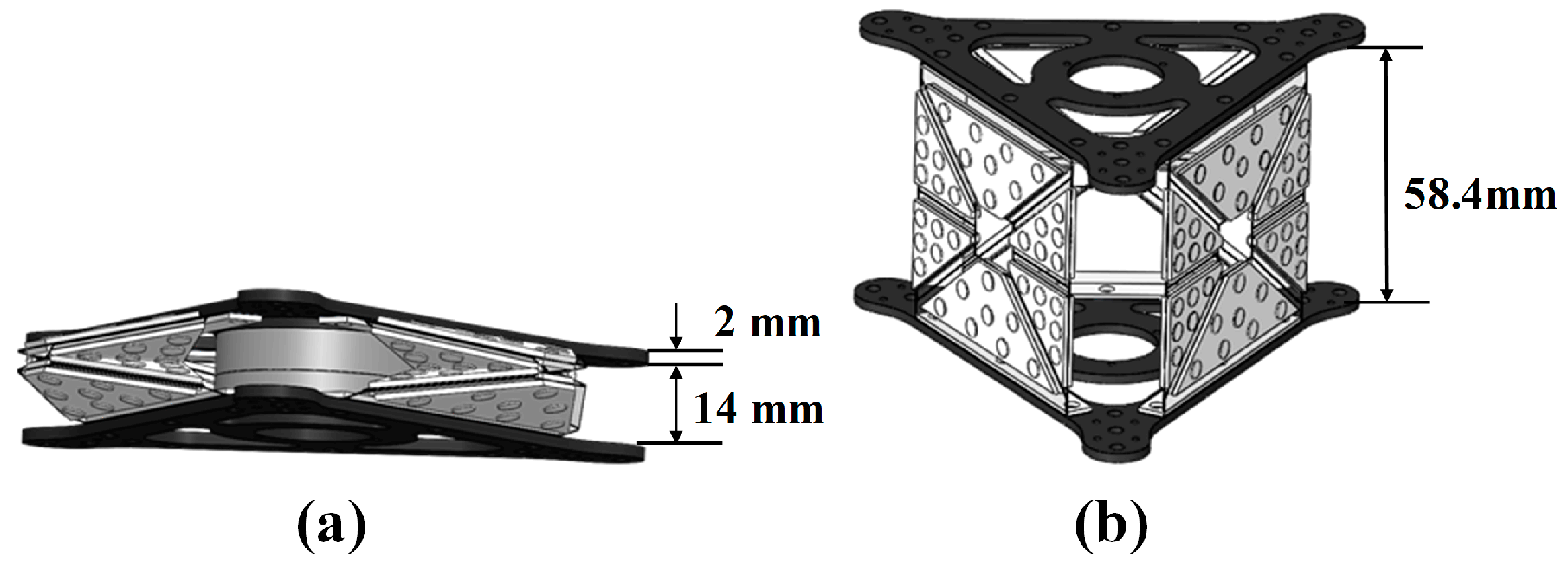
2. Design of SLPM Unit Based on Origami Structure
3. Kinematic Analysis
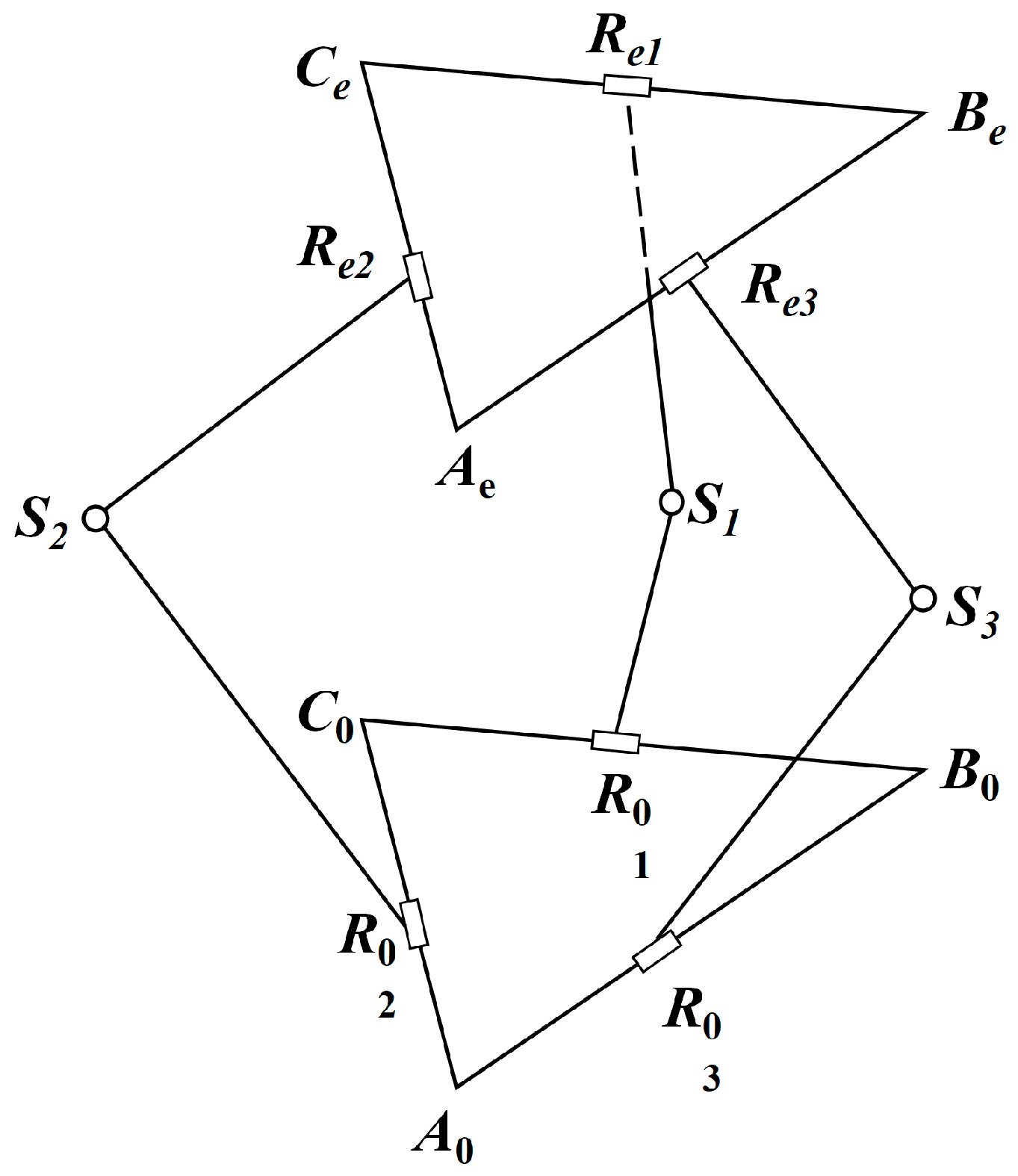
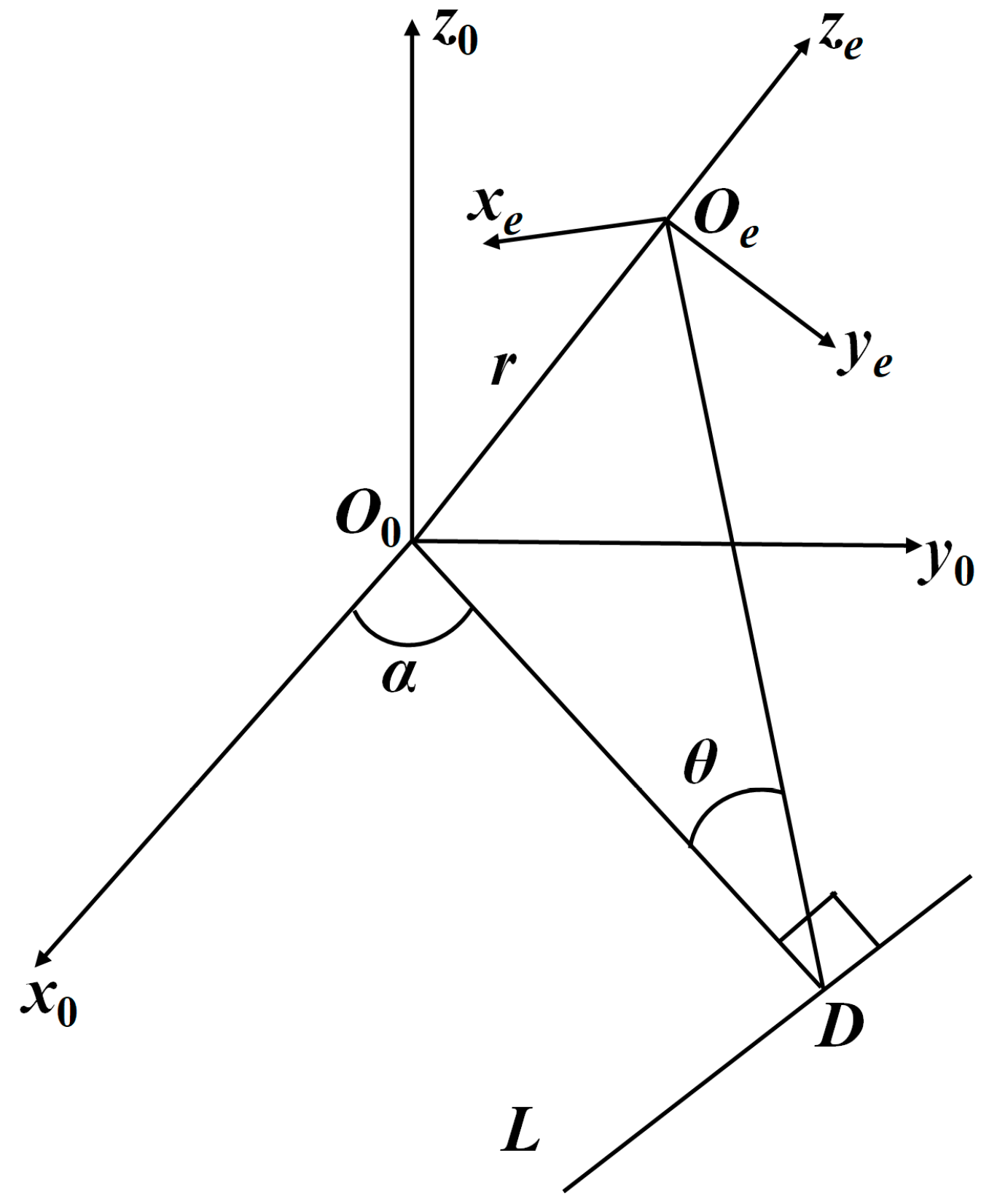
3.1. Forward Kinematics
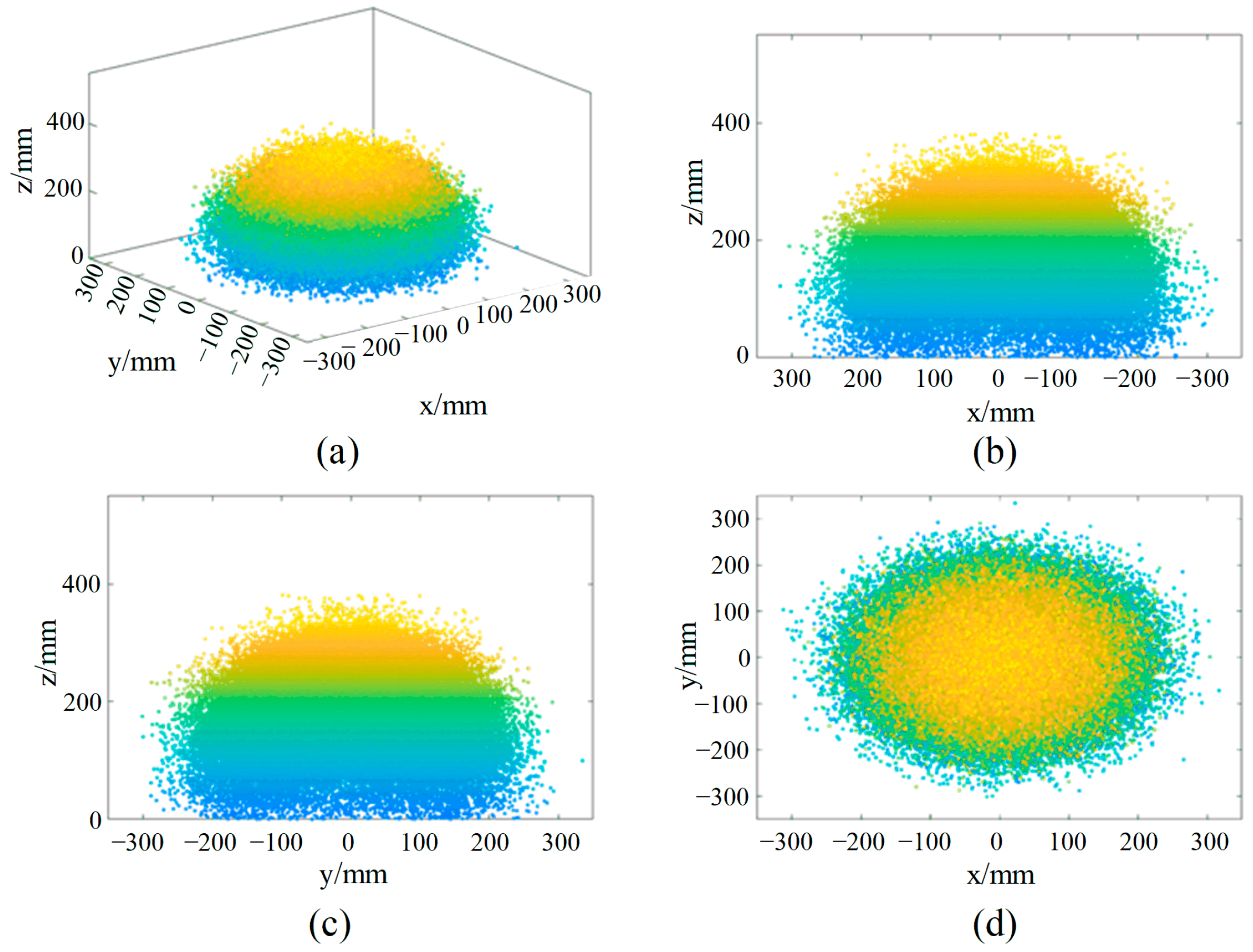
3.2. Workspace
3.3. Inverse Kinematics
3.3.1. Inverse Kinematics of Single Module
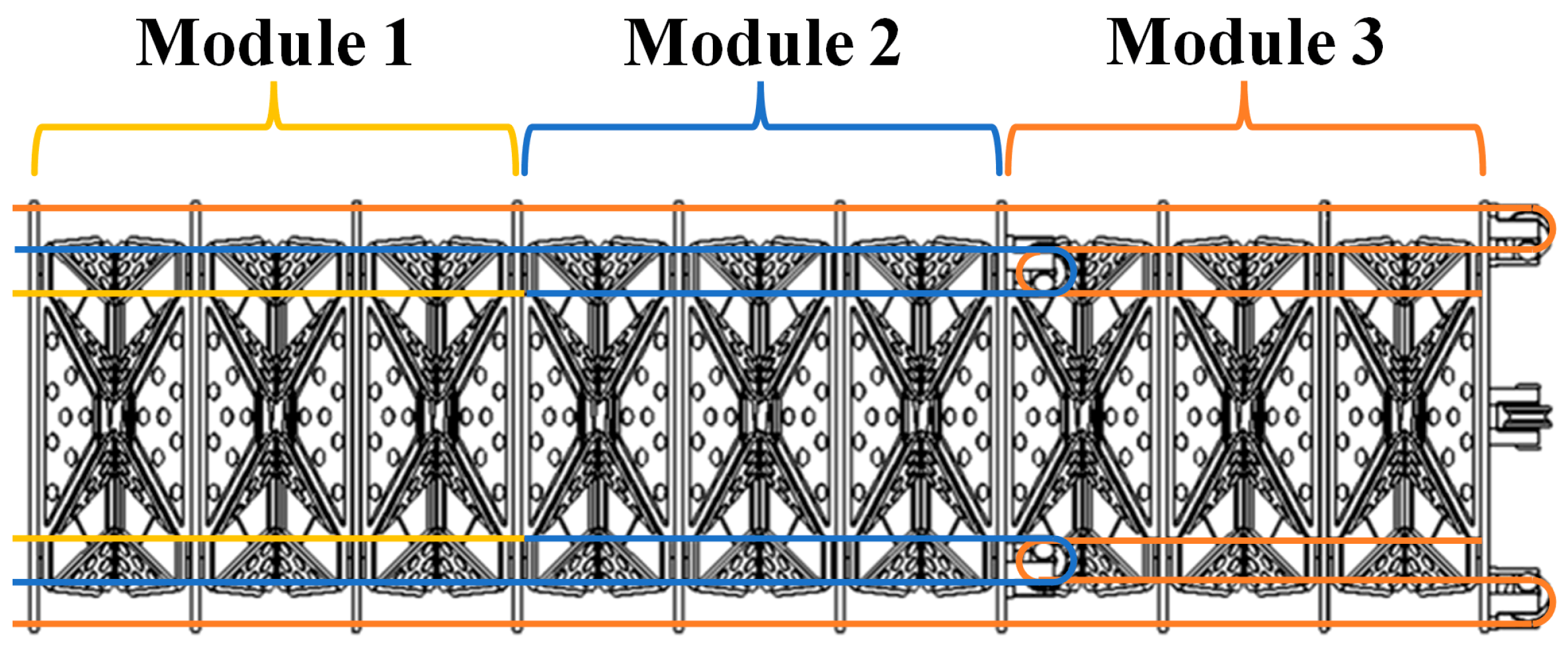
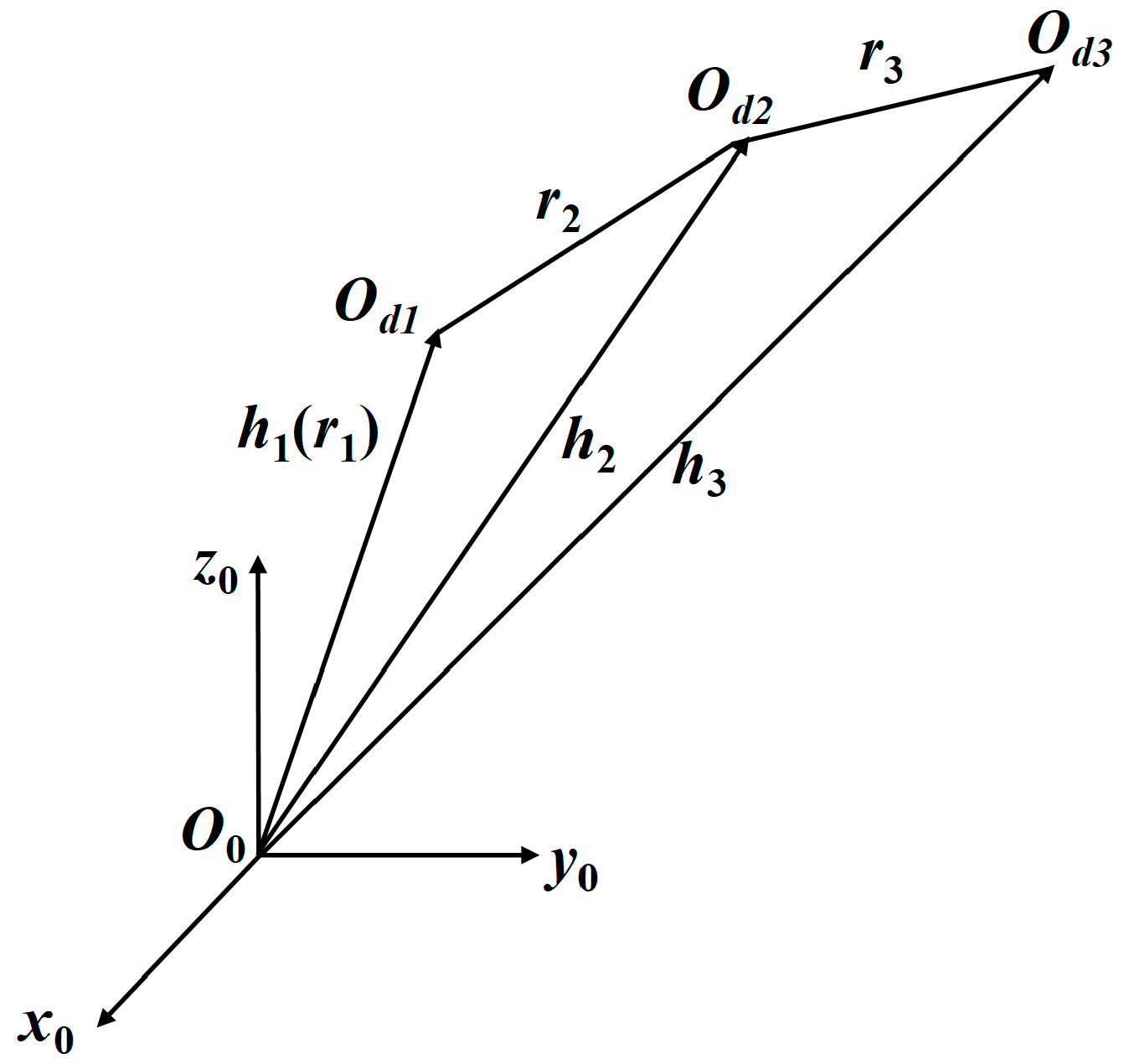
3.3.2. Inverse Kinematics of Robot
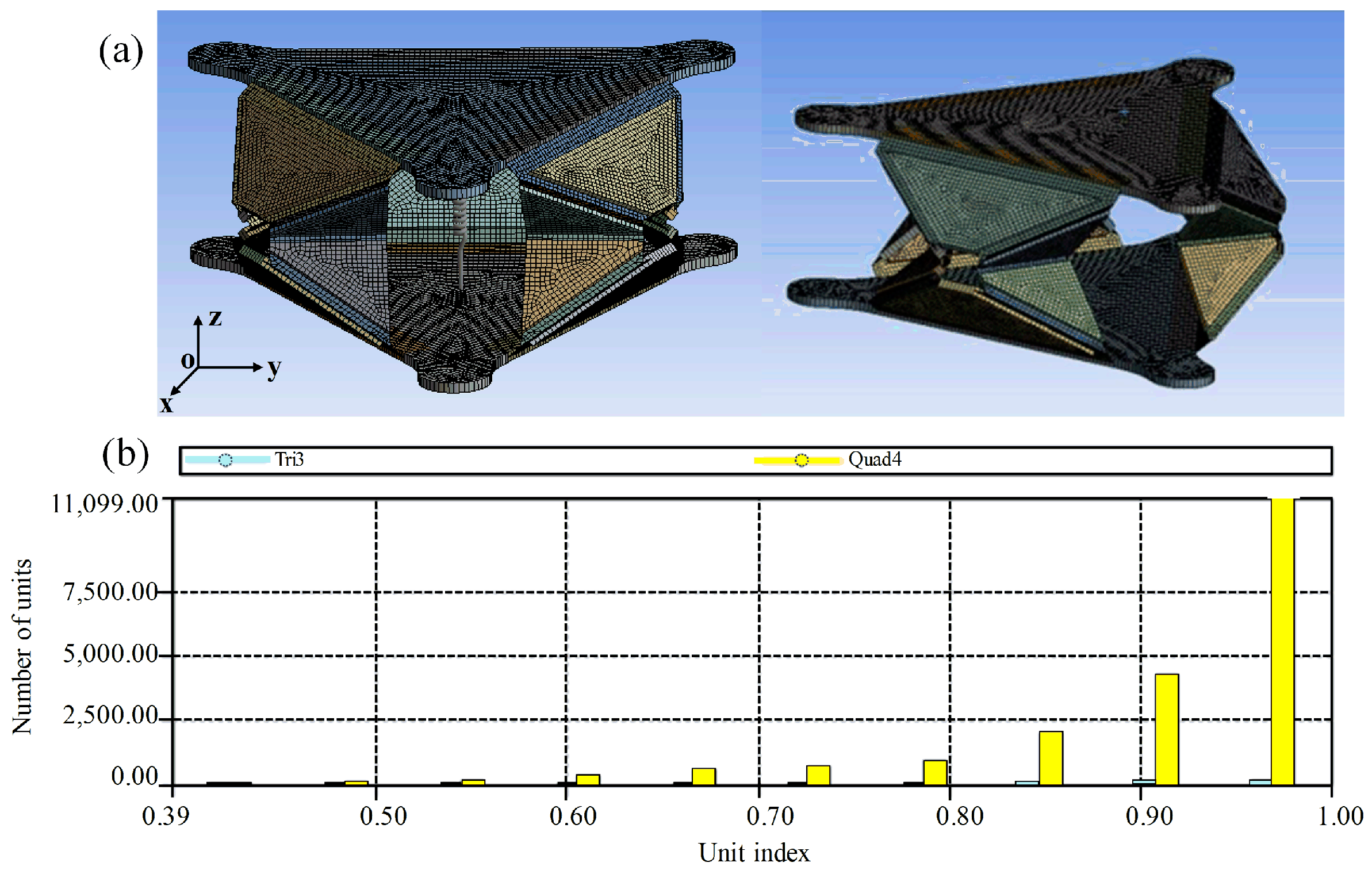
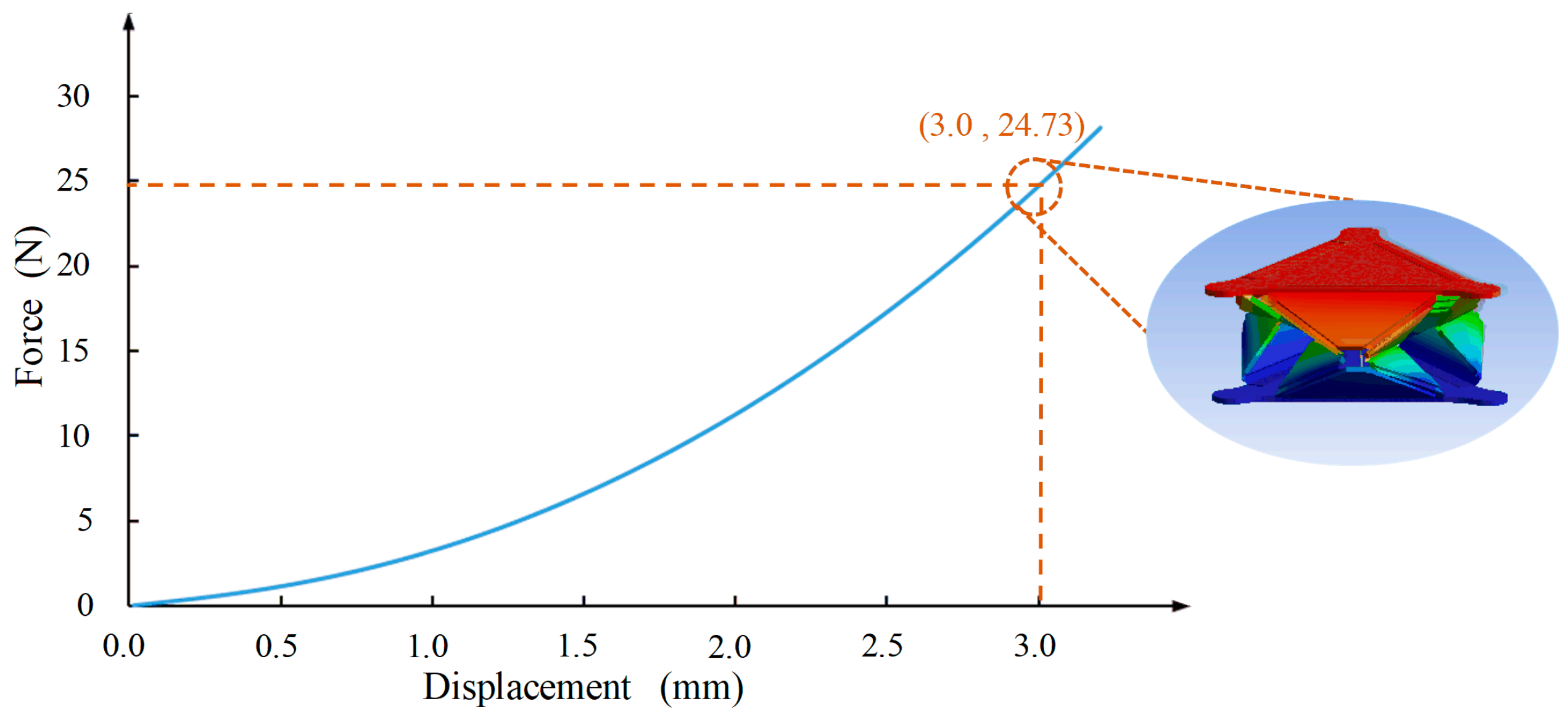
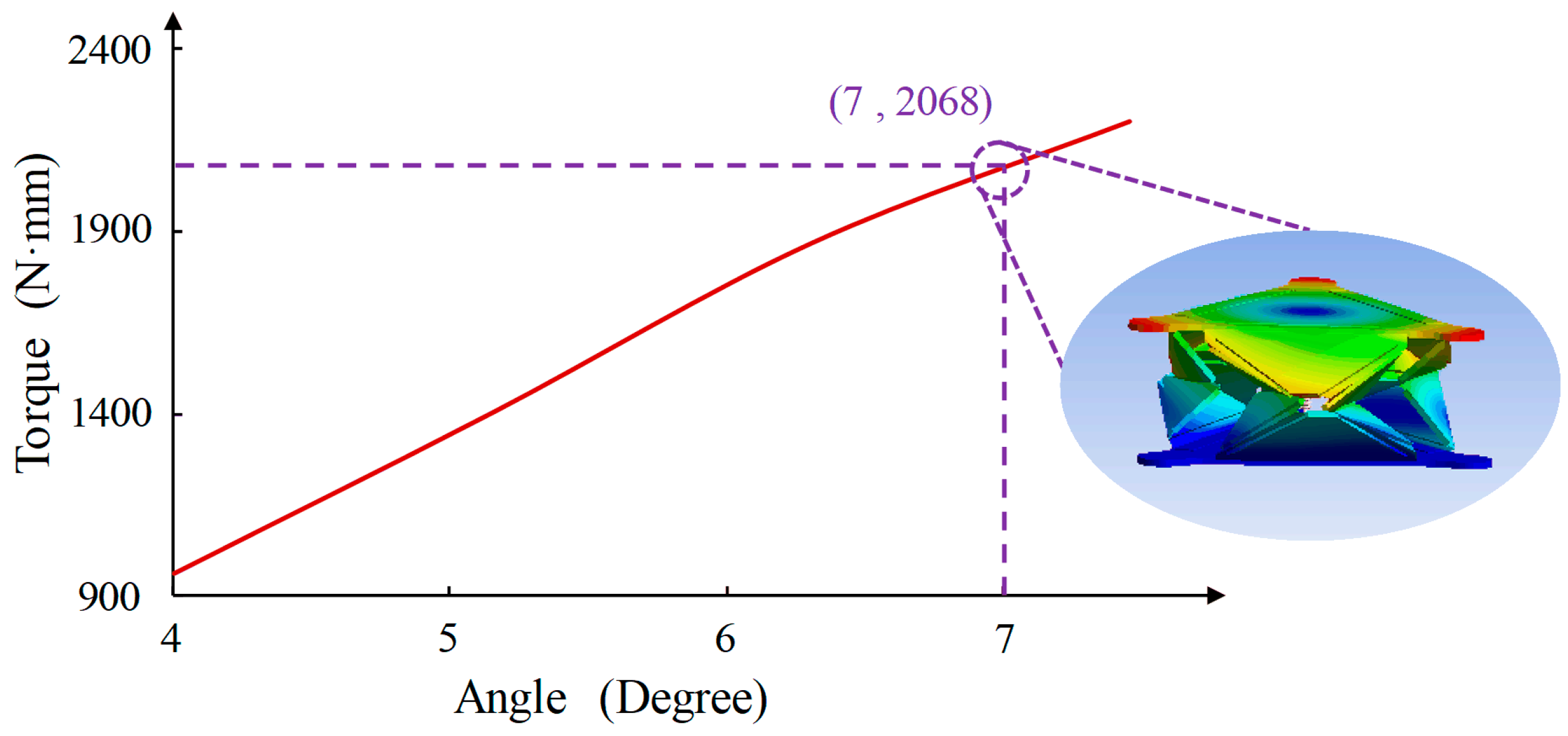
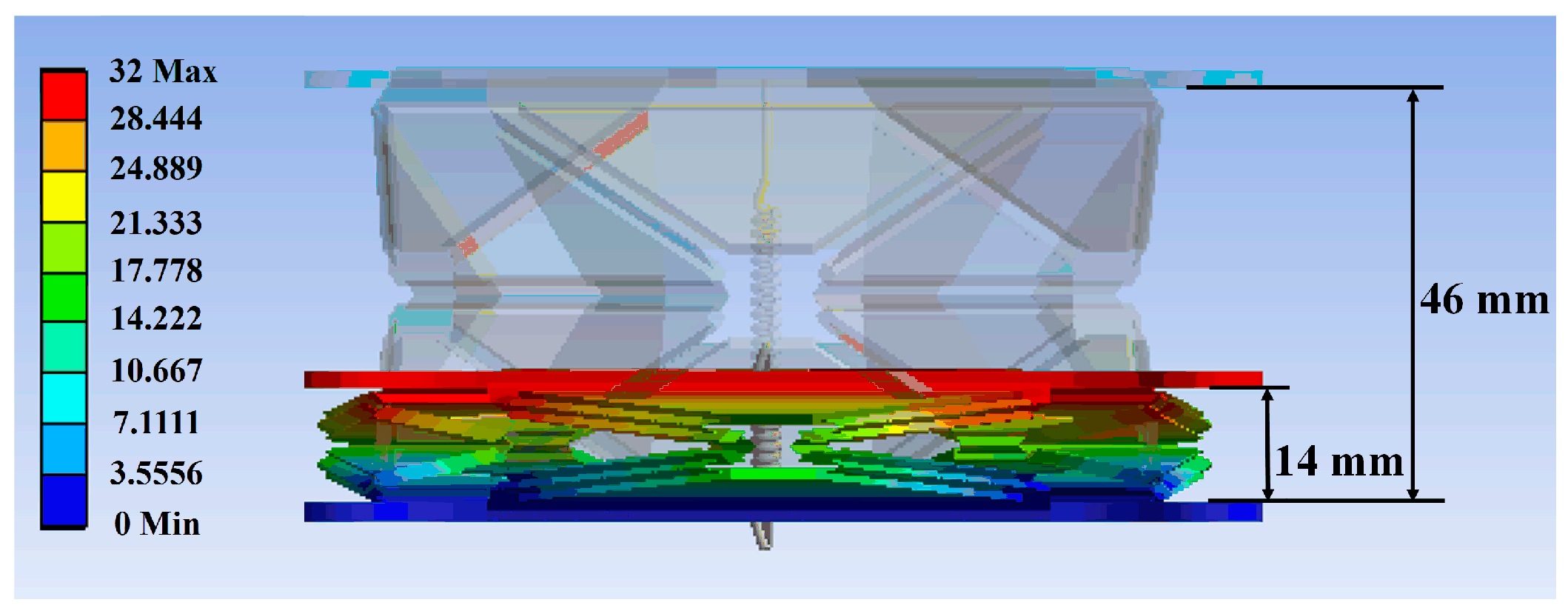
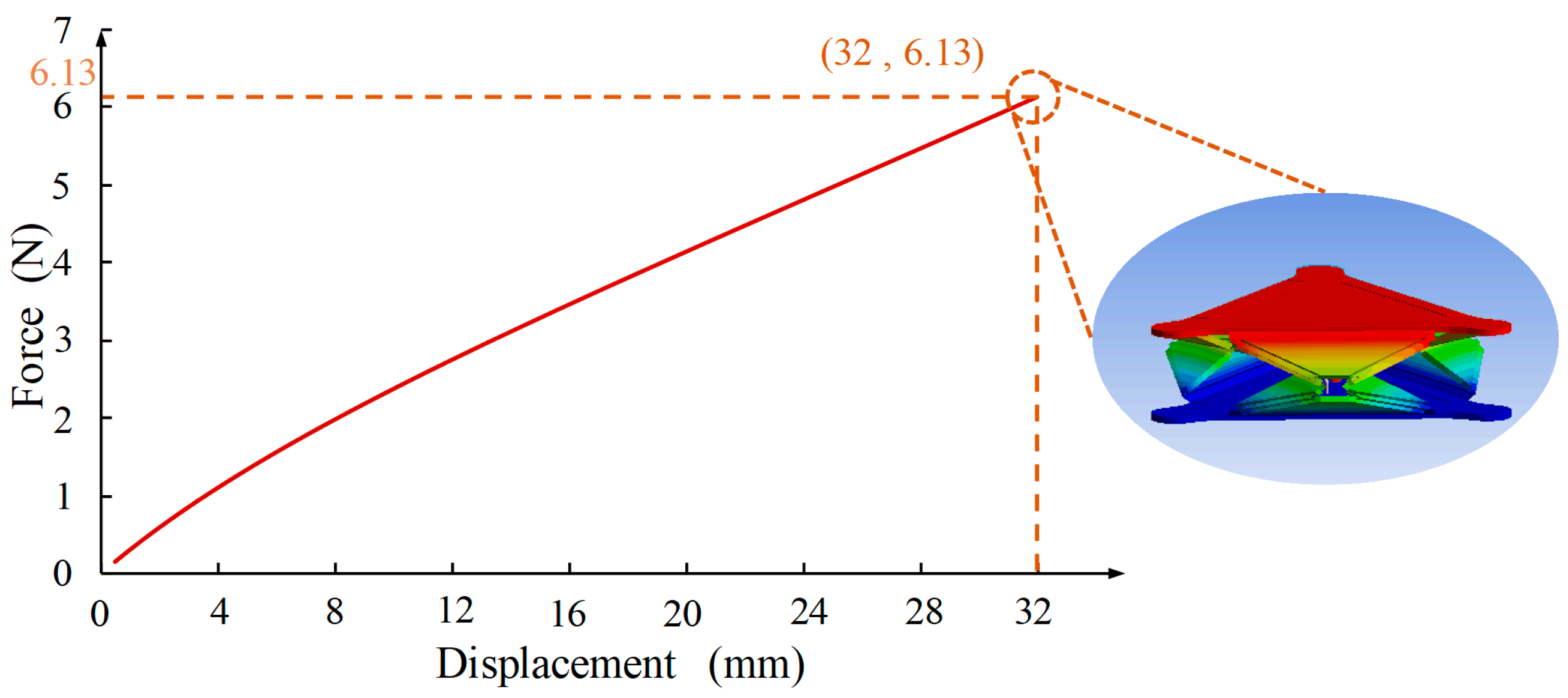
4. Finite Element Simulation
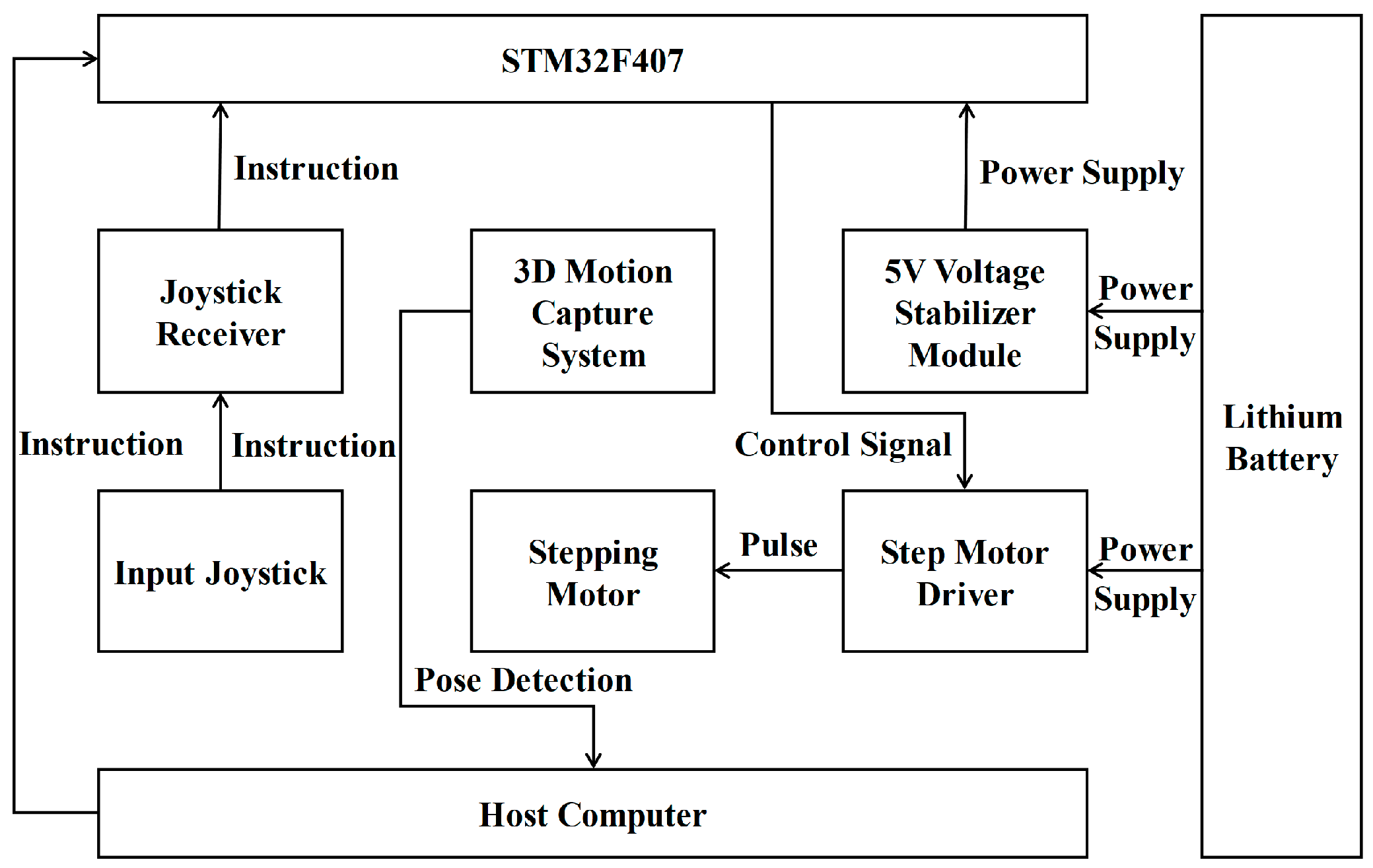
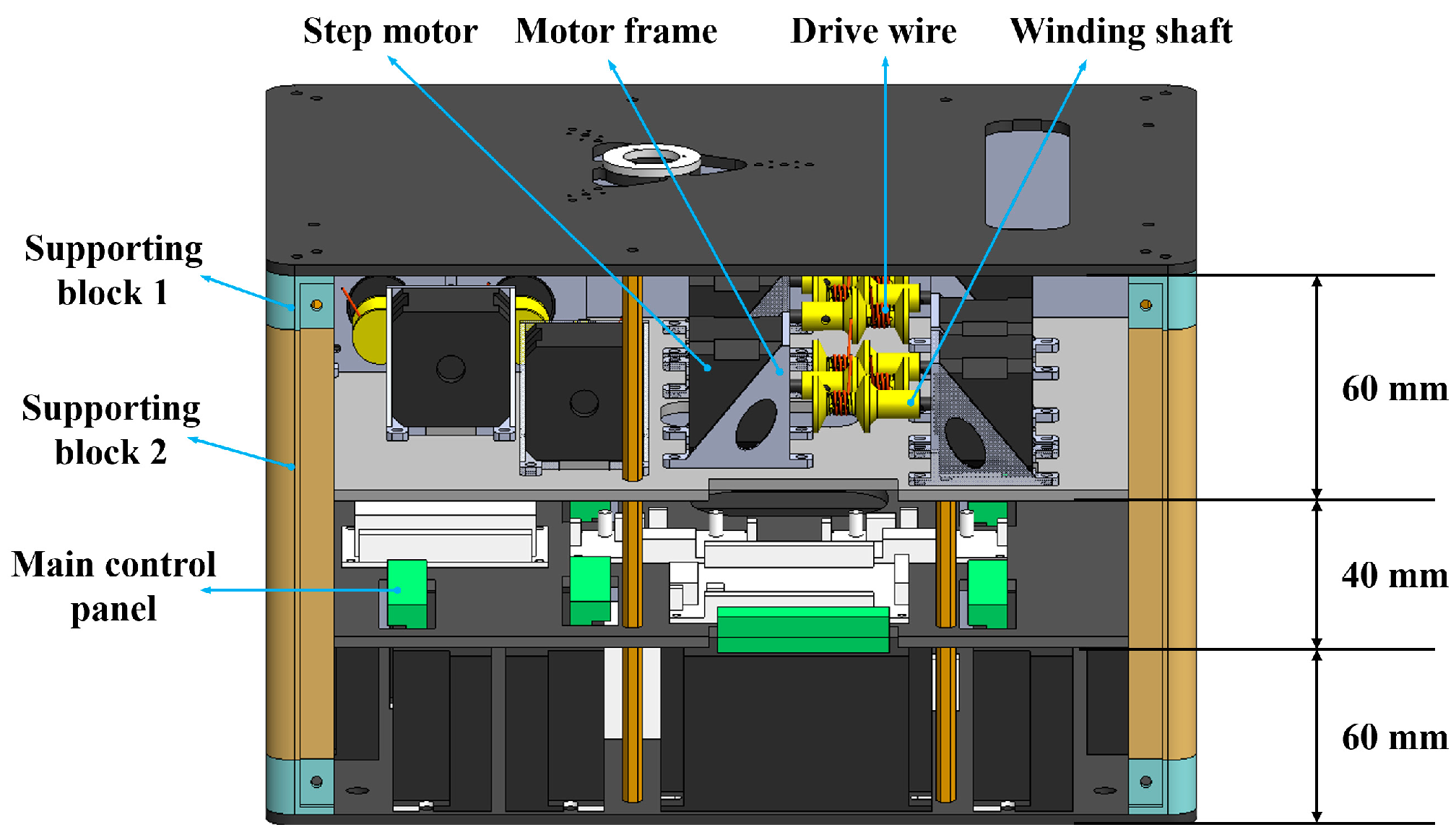
5. Control System and Experiment
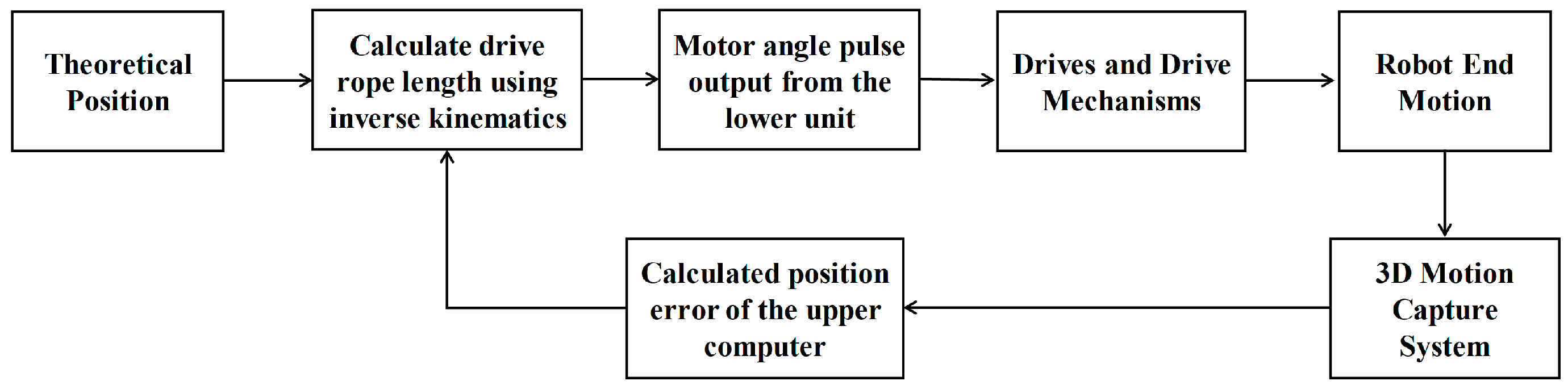
5.1. Control System
5.2. Experiment of Continuum Robot
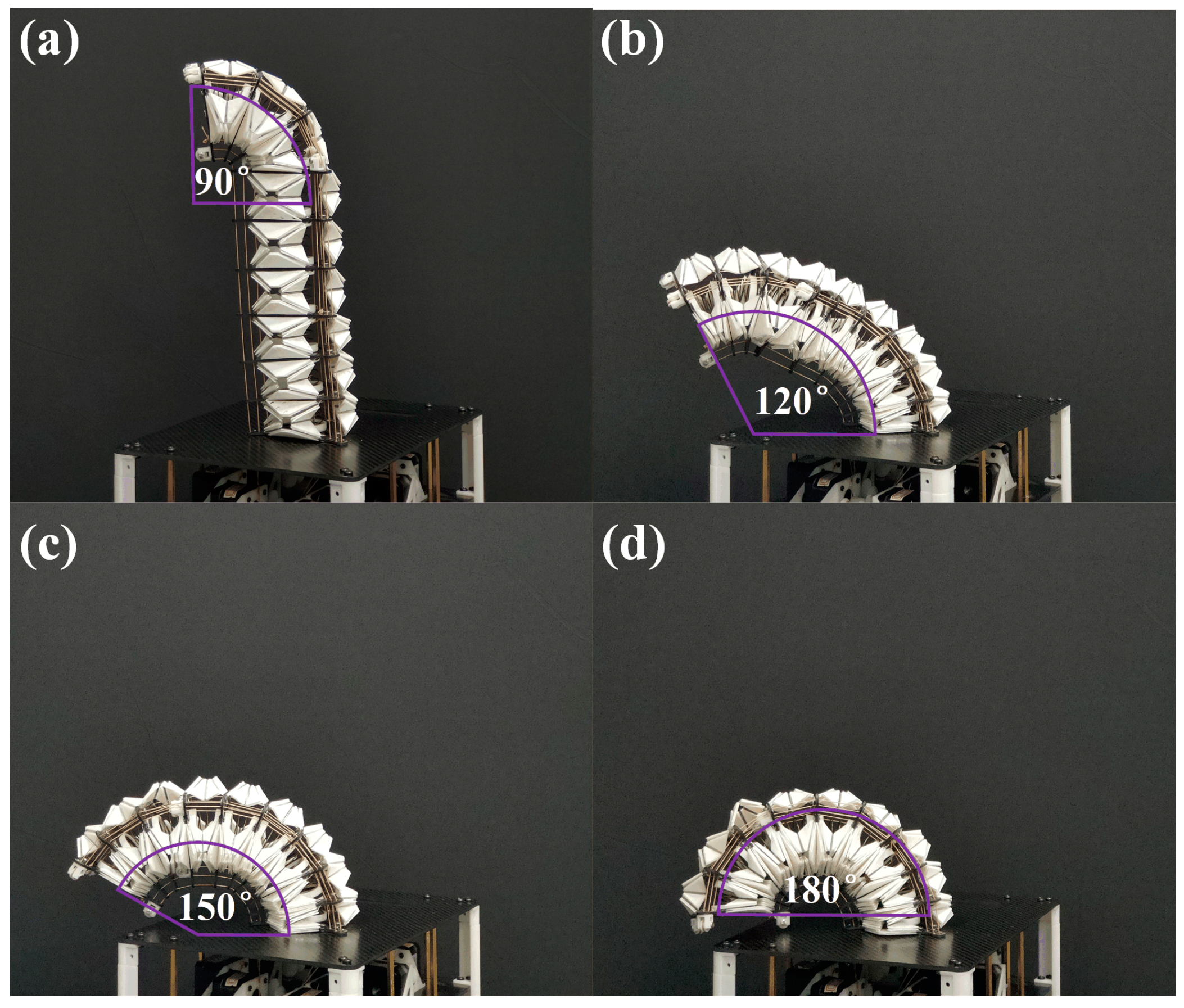
5.2.1. Folding Performance Evaluation
5.2.2. Bending Performance Evaluation
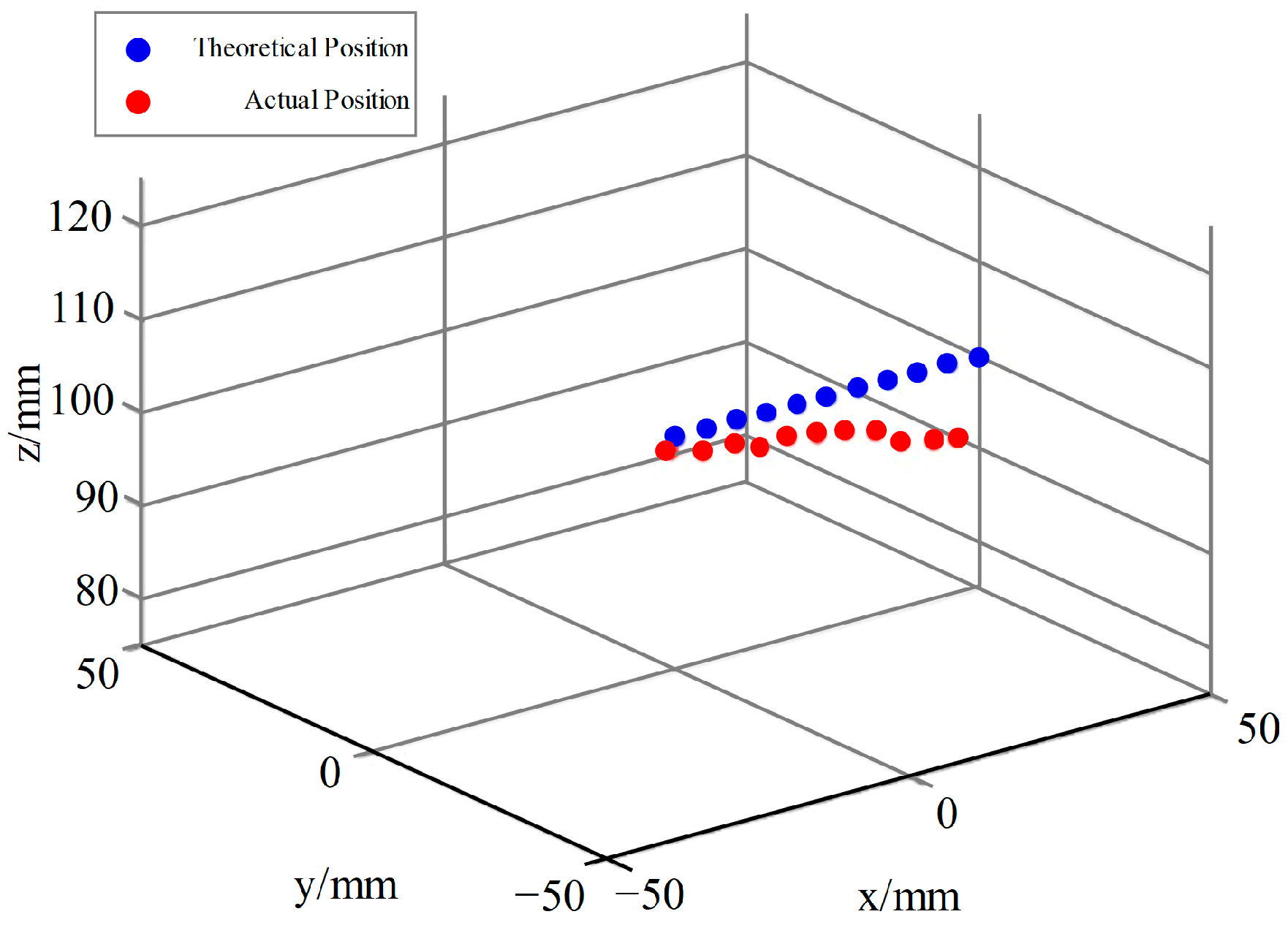
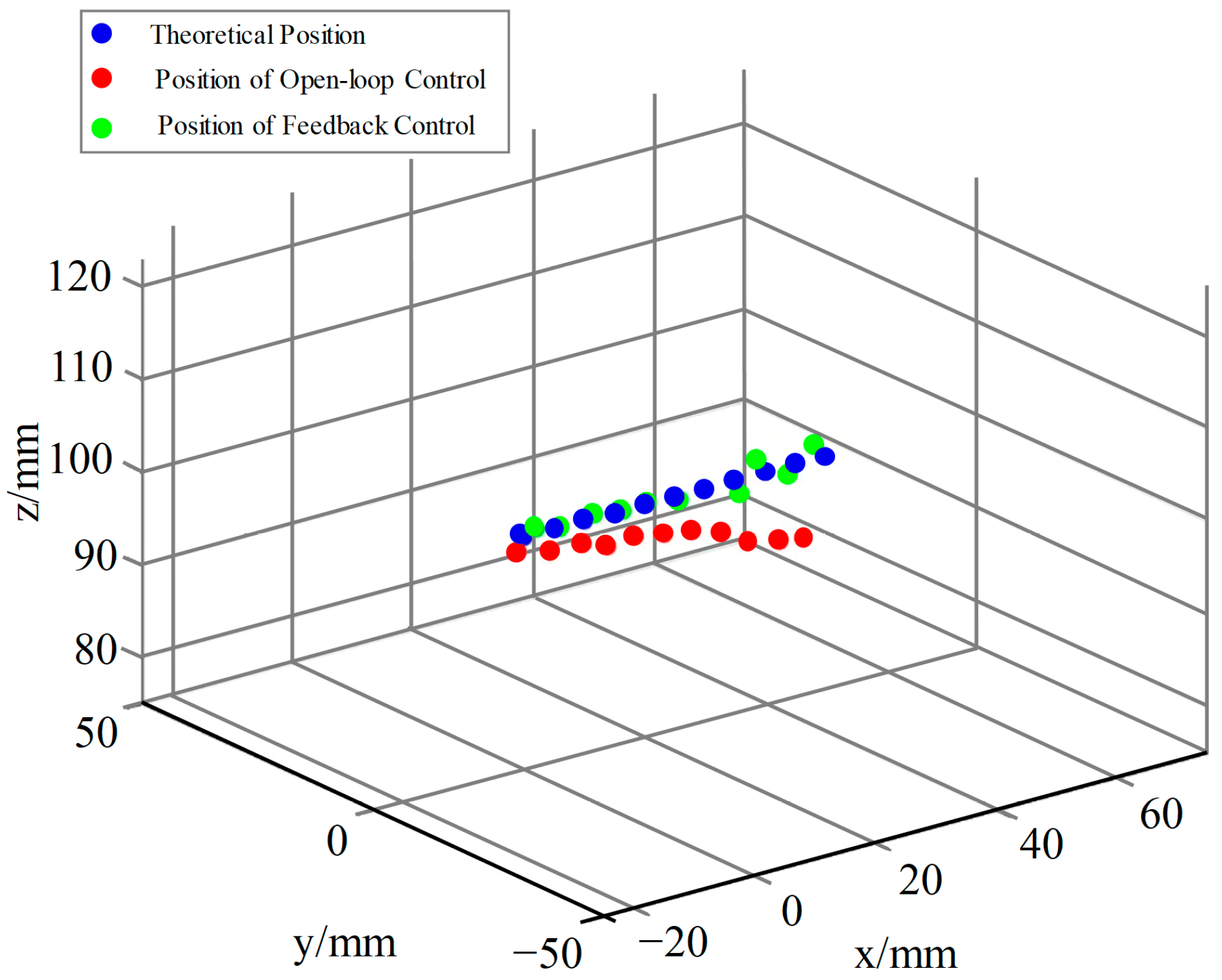
5.2.3. Accuracy Testing and Optimisation
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Russo, M.; Sadati, S.M.H.; Dong, X.; Mohammad, A.; Walker, I.D.; Bergeles, C.; Xu, K.; Axinte, D.A. Continuum Robots: An Overview. Adv. Intell. Syst. 2023, 5, 2200367. [Google Scholar] [CrossRef]
- Gravagne, I.A.; Rahn, C.D.; Walker, I.D. Large deflection dynamics, and control for planar continuum robots. IEEE/ASME Trans. Mechatron. 2003, 8, 299–307. [Google Scholar] [CrossRef]
- Hannan, M.W.; Walker, I.D. Kinematics and the implementation of an elephant’s trunk manipulator and other continuum style robots. J. Robot. Syst. 2003, 20, 45–63. [Google Scholar] [CrossRef] [PubMed]
- Jones, B.A.; Walker, I.D. Kinematics for multisection continuum robots. IEEE Trans. Robot. 2006, 22, 43–55. [Google Scholar] [CrossRef]
- Neppalli, S.; Csencsits, M.A.; Jones, B.A.; Walker, I.D. Closed-Form Inverse Kinematics for Continuum Manipulators. Adv. Robot. 2009, 23, 2077–2091. [Google Scholar] [CrossRef]
- Braganza, D.; Dawson, D.M.; Walker, I.D.; Nath, N. A neural network controller for continuum robots. IEEE Trans. Robot. 2007, 23, 1270–1277. [Google Scholar] [CrossRef]
- Xu, K.; Simaan, N. An investigation of the intrinsic force sensing capabilities of continuum robots. IEEE Trans. Robot. 2008, 24, 576–587. [Google Scholar] [CrossRef]
- Xu, K.; Simaan, N. Analytic Formulation for Kinematics, Statics, and Shape Restoration of Multibackbone Continuum Robots via Elliptic Integrals. J Mech Robot 2010, 2, 011006. [Google Scholar] [CrossRef]
- Sun, Y.; Lueth, T.C. Enhancing Torsional Stiffness of Continuum Robots Using 3-D Topology Optimized Flexure Joints. IEEE/ASME Trans. Mechatron. 2023, 28, 1844–1852. [Google Scholar] [CrossRef]
- Geng, S.N.; Wang, Y.Y.; Wang, C.; Kang, R.J. A Space Tendon-Driven Continuum Robot. In Proceedings of the Advances in Swarm Intelligence: 9th International Conference, Shanghai, China, 17–22 June 2018. [Google Scholar]
- Ranzani, T.; Gerboni, G.; Cianchetti, M.; Menciassi, A. A bioinspired soft manipulator for minimally invasive surgery. Bioinspiration Biomim. 2015, 10, 035008. [Google Scholar] [CrossRef]
- Greer, J.D.; Morimoto, T.K.; Okamura, A.M.; Hawkes, E.W. Series pneumatic artificial muscles (sPAMs) and application to a soft continuum robot. In Proceedings of the 2017 IEEE International Conference on Robotics and Automation (ICRA), Marina Bay Sands, Singapore, 29 May–3 June 2017; pp. 5503–5510. [Google Scholar]
- Caasenbrood, B.; Pogromsky, A.; Nijmeijer, H. A Computational Design Framework for Pressure-driven Soft Robots through Nonlinear Topology Optimization. In Proceedings of the 2020 3rd IEEE International Conference on Soft Robotics (RoboSoft), New Haven, CT, USA, 15 May–15 July 2020; pp. 633–638. [Google Scholar]
- Chautems, C.; Tonazzini, A.; Floreano, D.; Nelson, B.J. A variable stiffness catheter controlled with an external magnetic field. In Proceedings of the 2017 IEEE/RSJ International Conference on Intelligent Robots and Systems (IROS), Vancouver, Canada, 24–28 September 2017; pp. 181–186. [Google Scholar]
- Dupont, P.E.; Lock, J.; Itkowitz, B.; Butler, E. Design and Control of Concentric-Tube Robots. IEEE Trans. Robot. 2009, 26, 209–225. [Google Scholar] [CrossRef] [PubMed]
- Donat, H.; Gu, J.C.; Steil, J.J. Real-Time Shape Estimation for Concentric Tube Continuum Robots with a Single Force/Torque Sensor. Front. Robot. AI 2021, 8, 734033. [Google Scholar] [CrossRef] [PubMed]
- Chikhaoui, M.T.; Granna, J.; Starke, J.; Burgner-Kahrs, J. Toward motion coordination control and design optimization for dual-arm concentric tube continuum robots. IEEE Robot. Autom. Lett. 2018, 3, 1793–1800. [Google Scholar] [CrossRef]
- Liu, H.B.; Teng, X.Y.; Qiao, Z.Z.; Yu, H.B.; Cai, S.X.; Yang, W.G. A concentric tube magnetic continuum robot with multiple stiffness levels and high flexibility for potential endovascular intervention. J. Magn. Magn. Mater. 2024, 597, 172023. [Google Scholar] [CrossRef]
- Bryson, C.E.; Rucker, D.C. Toward Parallel Continuum Manipulators. In Proceedings of the 2014 IEEE International Conference on Robotics and Automation (ICRA), Hong Kong, China, 31 May–5 June 2014; pp. 778–785. [Google Scholar]
- Till, J.; Bryson, C.E.; Chung, S.; Orekhov, A.; Rucker, D.C. Efficient Computation of Multiple Coupled Cosserat Rod Models for Real-Time Simulation and Control of Parallel Continuum Manipulators. In Proceedings of the 2015 IEEE international conference on robotics and automation (ICRA), Washington, DC, USA, 25–30 May 2015; pp. 5067–5074. [Google Scholar]
- Black, C.B.; Till, J.; Rucker, C. Parallel Continuum Robots: Modeling, Analysis, and Actuation-Based Force Sensing. IEEE Trans. Robot. 2017, 34, 29–47. [Google Scholar] [CrossRef]
- Orekhov, A.L.; Black, C.B.; Till, J.; Chung, S.; Rucker, D.C. Analysis and validation of a teleoperated surgical parallel continuum manipulator. IEEE Robot. Autom. Lett. 2016, 1, 828–835. [Google Scholar] [CrossRef]
- Orekhov, A.L.; Bryson, C.E.; Till, J.; Chung, S.; Rucker, D.C. A Surgical Parallel Continuum Manipulator with a Cable-Driven Grasper. In Proceedings of the 2015 37th Annual International Conference of the IEEE Engineering in Medicine and Biology Society (EMBC), Milan, Italy, 25–29 August 2015; pp. 5264–5267. [Google Scholar]
- Wu, G.L.; Shi, G.L. Experimental statics calibration of a multi-constraint parallel continuum robot. Mech. Mach. Theory 2019, 136, 72–85. [Google Scholar] [CrossRef]
- Lilge, S.; Nuelle, K.; Boettcher, G.; Spindeldreier, S.; Burgner-Kahrs, J. Tendon Actuated Continuous Structures in Planar Parallel Robots: A Kinematic Analysis. J. Mech. Robot. 2021, 13, 011025. [Google Scholar] [CrossRef]
- Mauze, B.; Dahmouche, R.; Laurent, G.J.; André, A.N.; Rougeot, P.; Sandoz, P.; Clévy, C. Nanometer Precision with a Planar Parallel Continuum Robot. IEEE Robot. Autom. Lett. 2020, 5, 3806–3813. [Google Scholar] [CrossRef]
- Castledine, N.P.; Boyle, J.H.; Kim, J. Design of a Modular Continuum Robot Segment for use in a General Purpose Manipulator. In Proceedings of the 2019 International Conference on Robotics and Automation (ICRA), Montreal, Canada, 20–24 May 2019; pp. 4430–4435. [Google Scholar]
- Yang, H.D.; Asbeck, A.T. Design and Characterization of a Modular Hybrid Continuum Robotic Manipulator. IEEE/ASME Trans. Mechatron. 2020, 25, 2812–2823. [Google Scholar] [CrossRef]
- Gomez, V.; Hernando, M.; Aguado, E.; Bajo, D.; Rossi, C. Design and Kinematic Modeling of a Soft Continuum Telescopic Arm for the Self-Assembly Mechanism of a Modular Robot. Soft Robot. 2024, 11, 347–360. [Google Scholar] [CrossRef] [PubMed]
- Yang, J.Z.; Peng, H.J.; Zhou, W.Y.; Zhang, J.; Wu, Z.G. A modular approach for dynamic modeling of multisegment continuum robots. Mech. Mach. Theory 2021, 165, 104429. [Google Scholar] [CrossRef]
- Dai, J.S. Configuration transformation and mathematical description of manipulation of origami cartons. In Proceedings of the Origami6: Proceedings of the 6th International Meeting of Origami Science, Mathematics, and Education, Tokyo, Japan, 11–13 August 2015; pp. 163–173. [Google Scholar]
- Chen, Y.; Liang, J.B.; Shi, P.; Feng, J.; Sareh, P.; Dai, J.S. Inverse design of programmable Poisson’s ratio and in-plane stiffness for generalized four-fold origami. Compos. Struct. 2023, 311, 116789. [Google Scholar] [CrossRef]
- Wang, R.Q.; Song, Y.Q.; Dai, J.S. Reconfigurability of the origami-inspired integrated 8R kinematotropic metamorphic mechanism and its evolved 6R and 4R mechanisms. Mech. Mach. Theory 2021, 161, 104245. [Google Scholar] [CrossRef]
- Zhuang, Z.; Guan, Y.; Xu, S.; Dai, J.S. Reconfigurability in automobiles—structure, manufacturing and algorithm for automobiles. Int. J. Automot. Manuf. Mater. 2022, 1, 1–11. [Google Scholar] [CrossRef]
- Zhang, K.T.; Qiu, C.; Dai, J.S. An Extensible Continuum Robot With Integrated Origami Parallel Modules. J. Mech. Robot. 2016, 8, 031010. [Google Scholar] [CrossRef]
- Guan, Y.T.; Zhuang, Z.M.; Zhang, Z.; Dai, J.S. Design, Analysis, and Experiment of the Origami Robot Based on Spherical-Linkage Parallel Mechanism. J. Mech. Des. 2023, 145, 081701. [Google Scholar] [CrossRef]
- Hanna, B.H.; Lund, J.M.; Lang, R.J.; Magleby, S.P.; Howell, L.L. Waterbomb base: A symmetric single-vertex bistable origami mechanism. Smart Mater. Struct. 2014, 23, 094009. [Google Scholar] [CrossRef]
- Lee, D.Y.; Kim, J.K.; Sohn, C.Y.; Heo, J.M.; Cho, K.J. High-load capacity origami transformable wheel. Sci. Robot. 2021, 6, eabe0201. [Google Scholar] [CrossRef]
- Zhang, K.T.; Fang, Y.F.; Fang, H.R.; Dai, J.S. Geometry and Constraint Analysis of the Three-Spherical Kinematic Chain Based Parallel Mechanism. J. Mech. Robot. 2010, 2, eabe0201. [Google Scholar] [CrossRef]
- Zhuang, Z.M.; Zhang, Z.; Guan, Y.T.; Wei, W.; Li, M.; Tang, Z.; Kang, R.J.; Song, Z.B.; Dai, J.S. Design and Control of SLPM-Based Extensible Continuum Arm. J. Mech. Robot. 2022, 14, 061003. [Google Scholar] [CrossRef]
- Li, Y.N.; Huang, H.L.; Li, B. Design of a Deployable Continuum Robot Using Elastic Kirigami-Origami. IEEE Robot. Autom. Lett. 2023, 8, 8382–8389. [Google Scholar] [CrossRef]
- Dai, J. Screw algebra and kinematic approaches for mechanisms and robotics. In Springer Tracks in Advanced Robotics; Springer: London, UK, 2014. [Google Scholar]
- Rastegar, J.; Fardanesh, B. Manipulation workspace analysis using the Monte Carlo method. Mech. Mach. Theory 1990, 25, 233–239. [Google Scholar] [CrossRef]
- Zhai, Z.R.; Wang, Y.; Jiang, H.Q. Origami-inspired, on-demand deployable and collapsible mechanical metamaterials with tunable stiffness. Proc. Natl. Acad. Sci. 2018, 115, 2032–2037. [Google Scholar] [CrossRef] [PubMed]
- Zhang, Z.; Tang, S.J.; Fan, W.C.; Xun, Y.H.; Wang, H.; Chen, G.L. Design and analysis of hybrid-driven origami continuum robots with extensible and stiffness-tunable sections. Mech. Mach. Theory 2022, 169, 104607. [Google Scholar] [CrossRef]









| Material | Density (g/cm3) | Poisson’s Ratio | Thickness/mm | |
|---|---|---|---|---|
| Connection Plate | carbon fiber | 1.80 | 0.1 | 2.0 |
| PET Film | PET | 1.38 | 0.384 | 0.1 |
| Rigid Plate | PLA | 0.42 | 1.0 |
| Trajectory | Mean Error before Compensation/mm | Mean Error after Compensation/mm | Average Error Reduction Rate |
|---|---|---|---|
| Linear | 5.0630 | 1.7926 | 64.6% |
| Circular | 4.5760 | 1.5634 | 65.8% |
| Square | 4.1516 | 1.4532 | 65.0% |
| Trajectory | Standard Deviation before Compensation/mm | Standard Deviation after Compensation/mm | Average Error Reduction Rate |
|---|---|---|---|
| Linear | 2.4591 | 0.7580 | 69.2% |
| Circular | 2.3087 | 0.3608 | 84.4% |
| Square | 1.6345 | 0.5207 | 68.1% |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Jia, A.; Liu, X.; Guan, Y.; Liu, Y.; Helian, Q.; Liu, C.; Zhuang, Z.; Kang, R. Design, Analysis and Experiment of a Modular Deployable Continuum Robot. Machines 2024, 12, 544. https://doi.org/10.3390/machines12080544
Jia A, Liu X, Guan Y, Liu Y, Helian Q, Liu C, Zhuang Z, Kang R. Design, Analysis and Experiment of a Modular Deployable Continuum Robot. Machines. 2024; 12(8):544. https://doi.org/10.3390/machines12080544
Chicago/Turabian StyleJia, Aihu, Xinyu Liu, Yuntao Guan, Yongxi Liu, Qianze Helian, Chenshuo Liu, Zheming Zhuang, and Rongjie Kang. 2024. "Design, Analysis and Experiment of a Modular Deployable Continuum Robot" Machines 12, no. 8: 544. https://doi.org/10.3390/machines12080544
APA StyleJia, A., Liu, X., Guan, Y., Liu, Y., Helian, Q., Liu, C., Zhuang, Z., & Kang, R. (2024). Design, Analysis and Experiment of a Modular Deployable Continuum Robot. Machines, 12(8), 544. https://doi.org/10.3390/machines12080544





