A Survey of Planar Underactuated Mechanical System
Abstract
1. Introduction
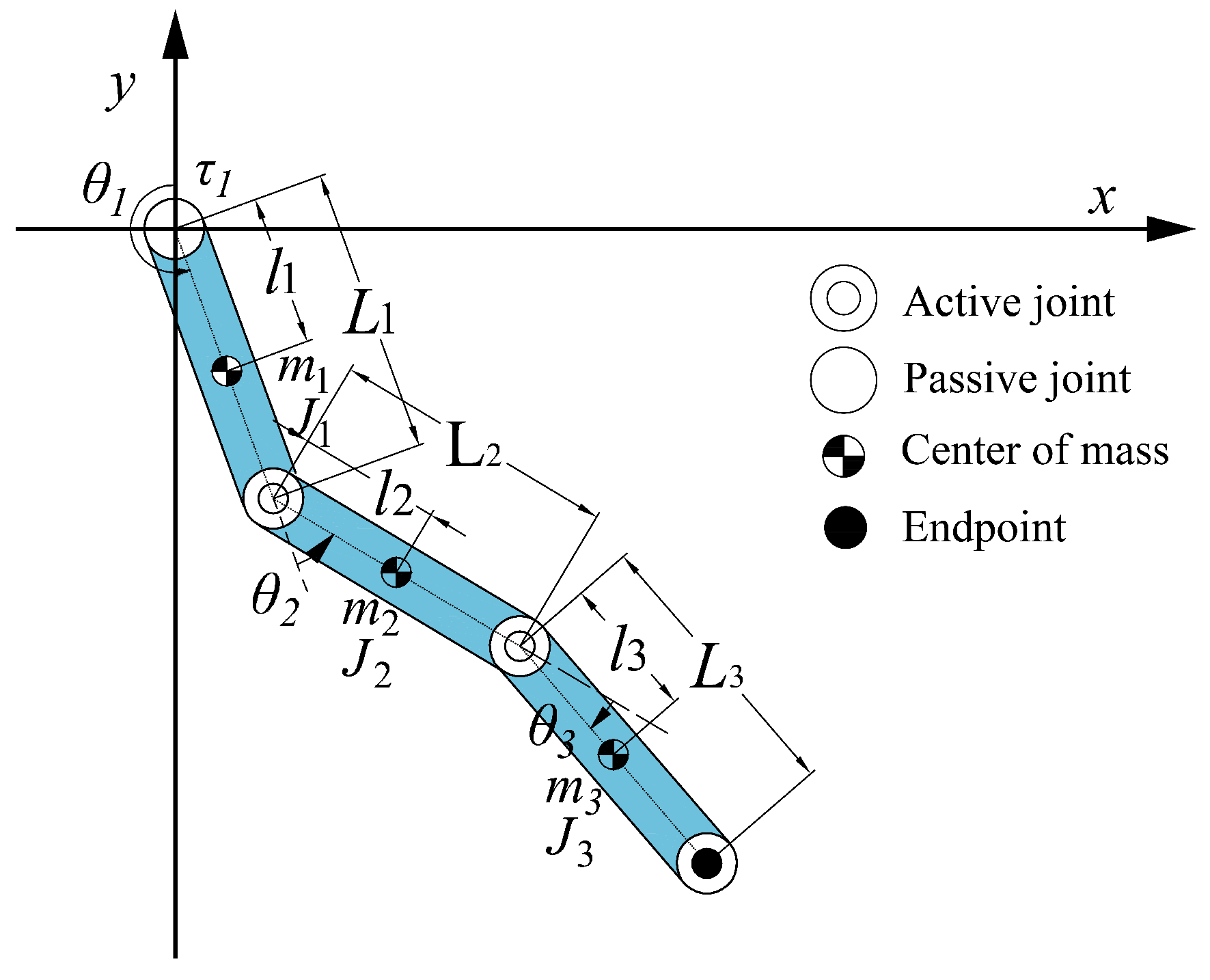
2. Modeling of Planar Underactuated Manipulator
2.1. Modeling and Analysis
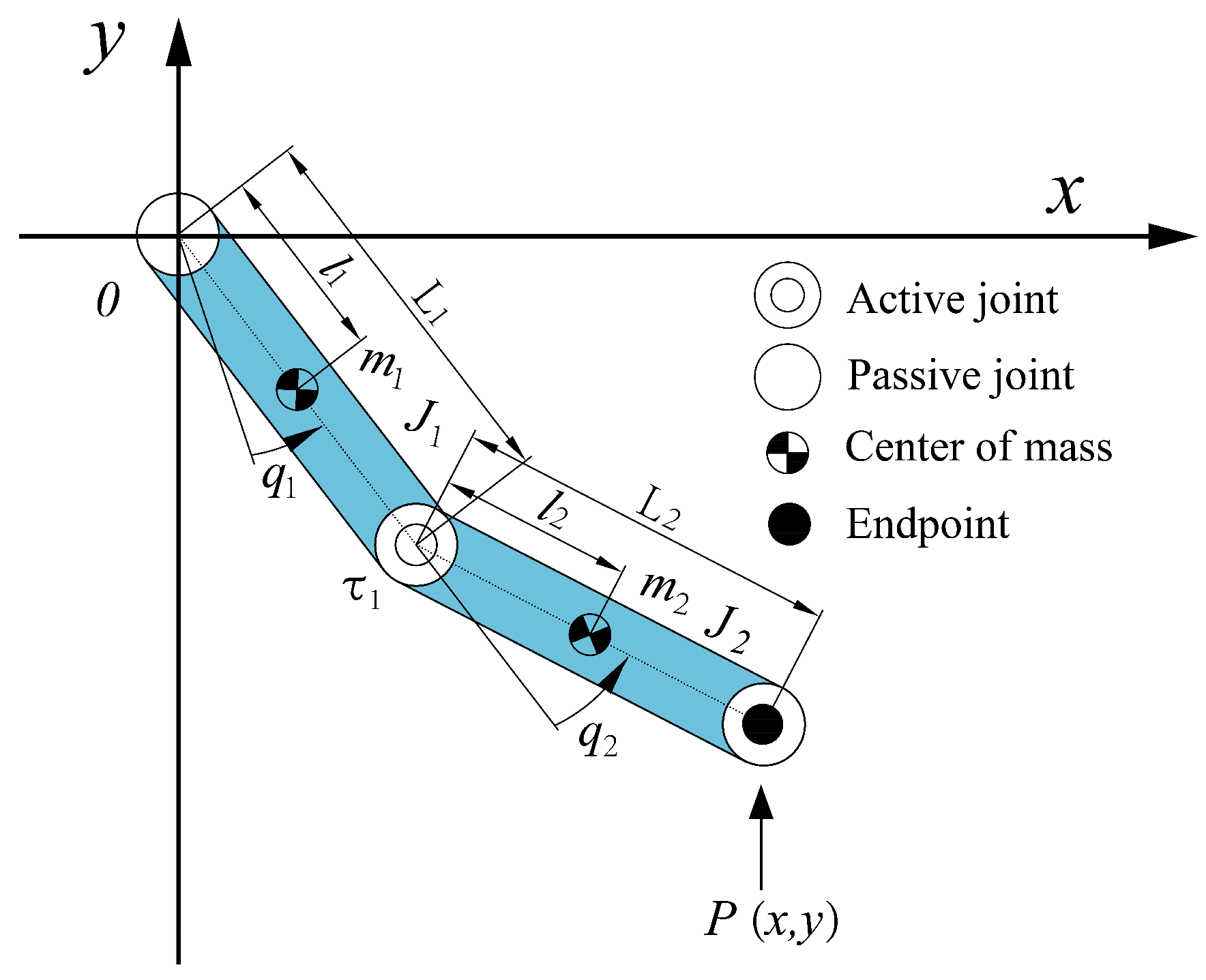
2.1.1. Dynamic Model
2.1.2. Kinematic Model
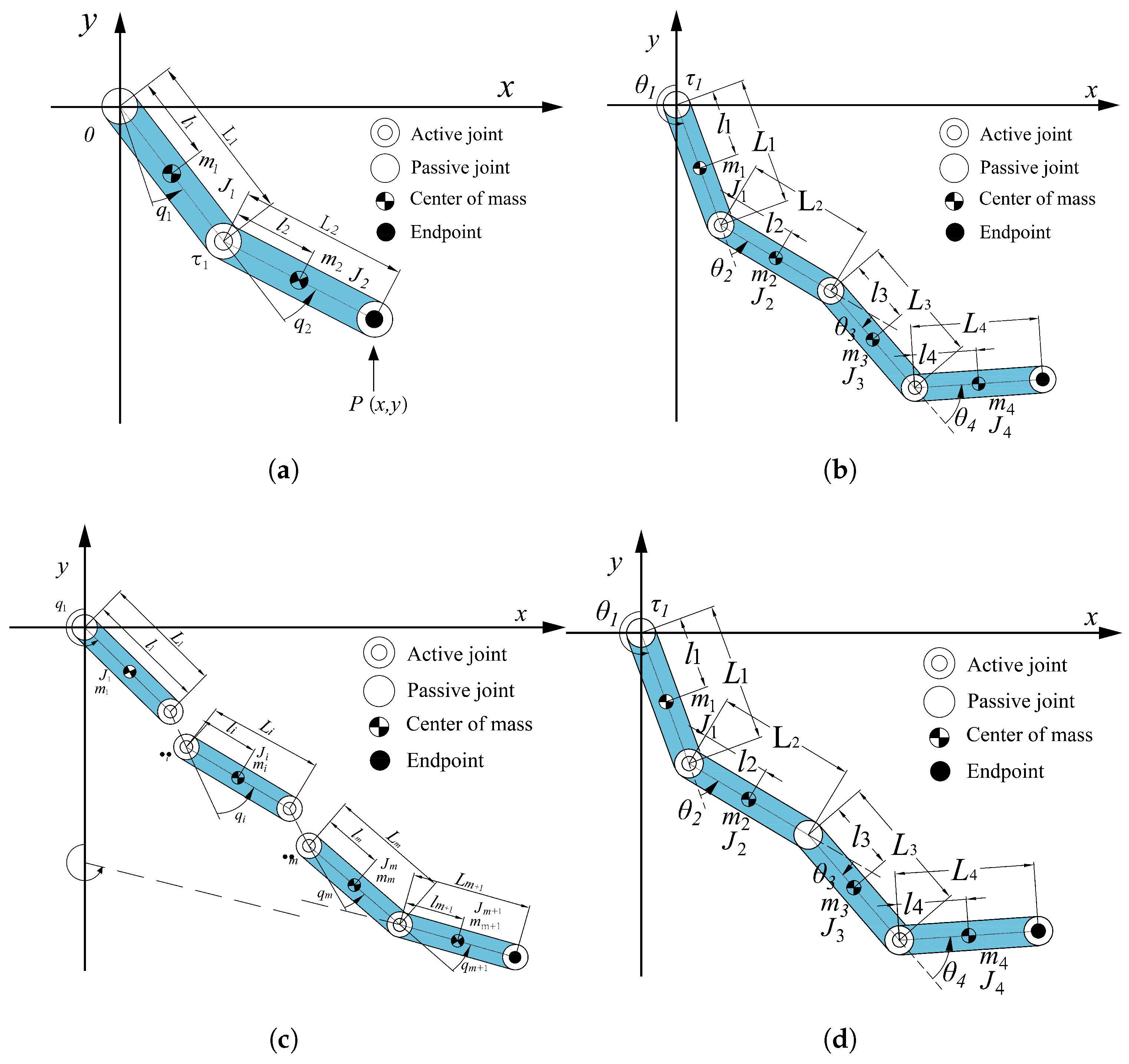
2.2. Position of the Passive Joint
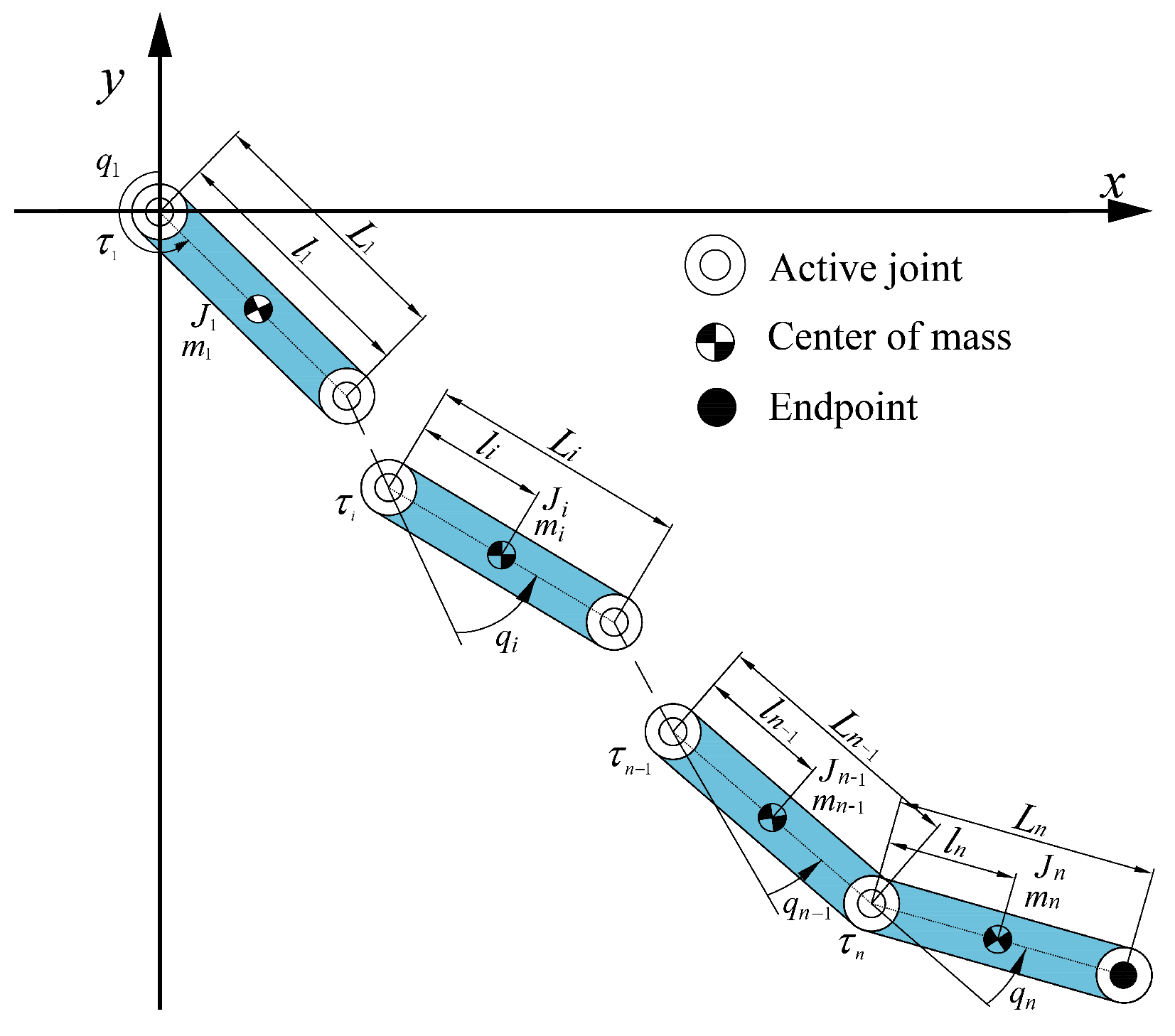
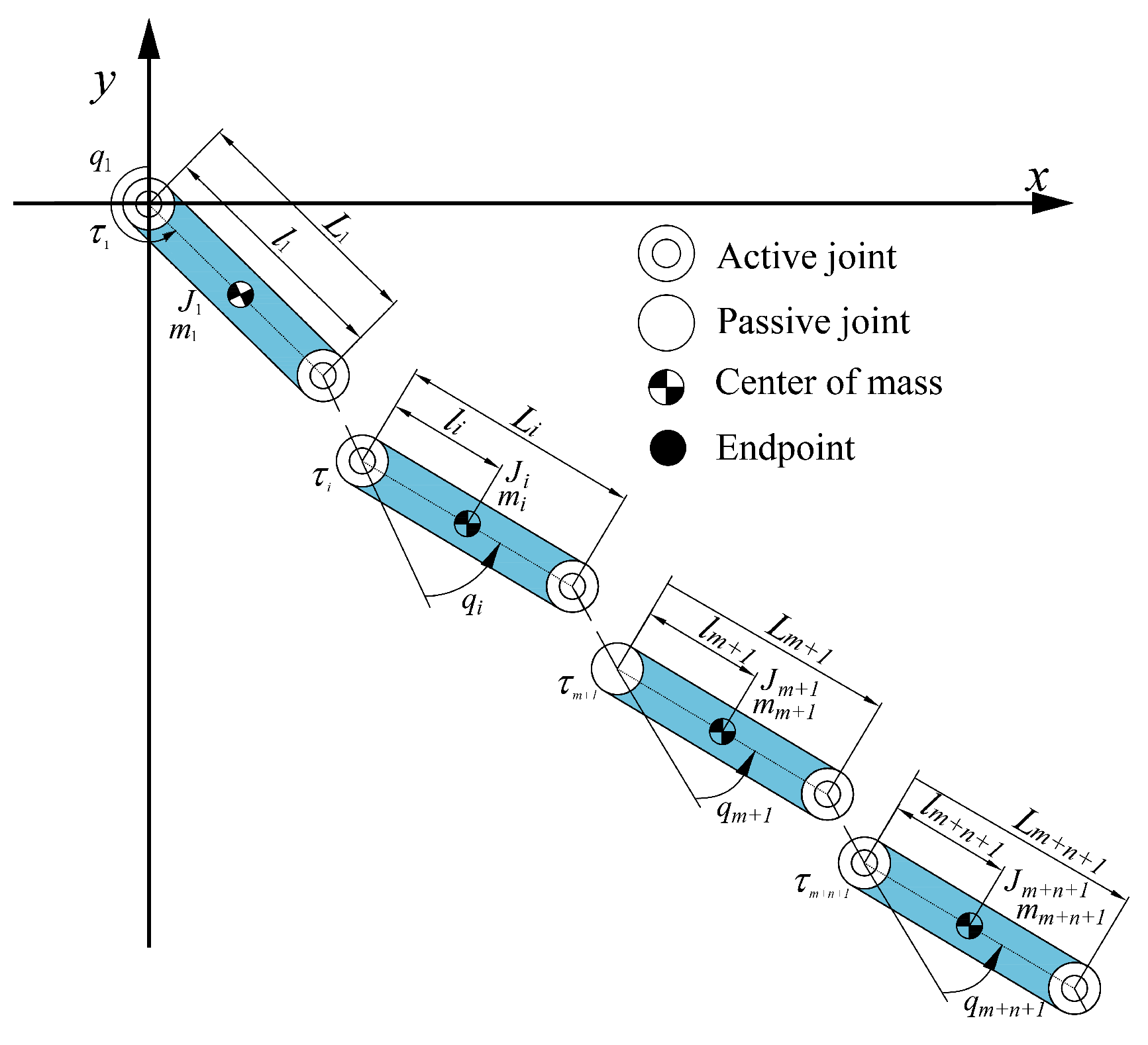
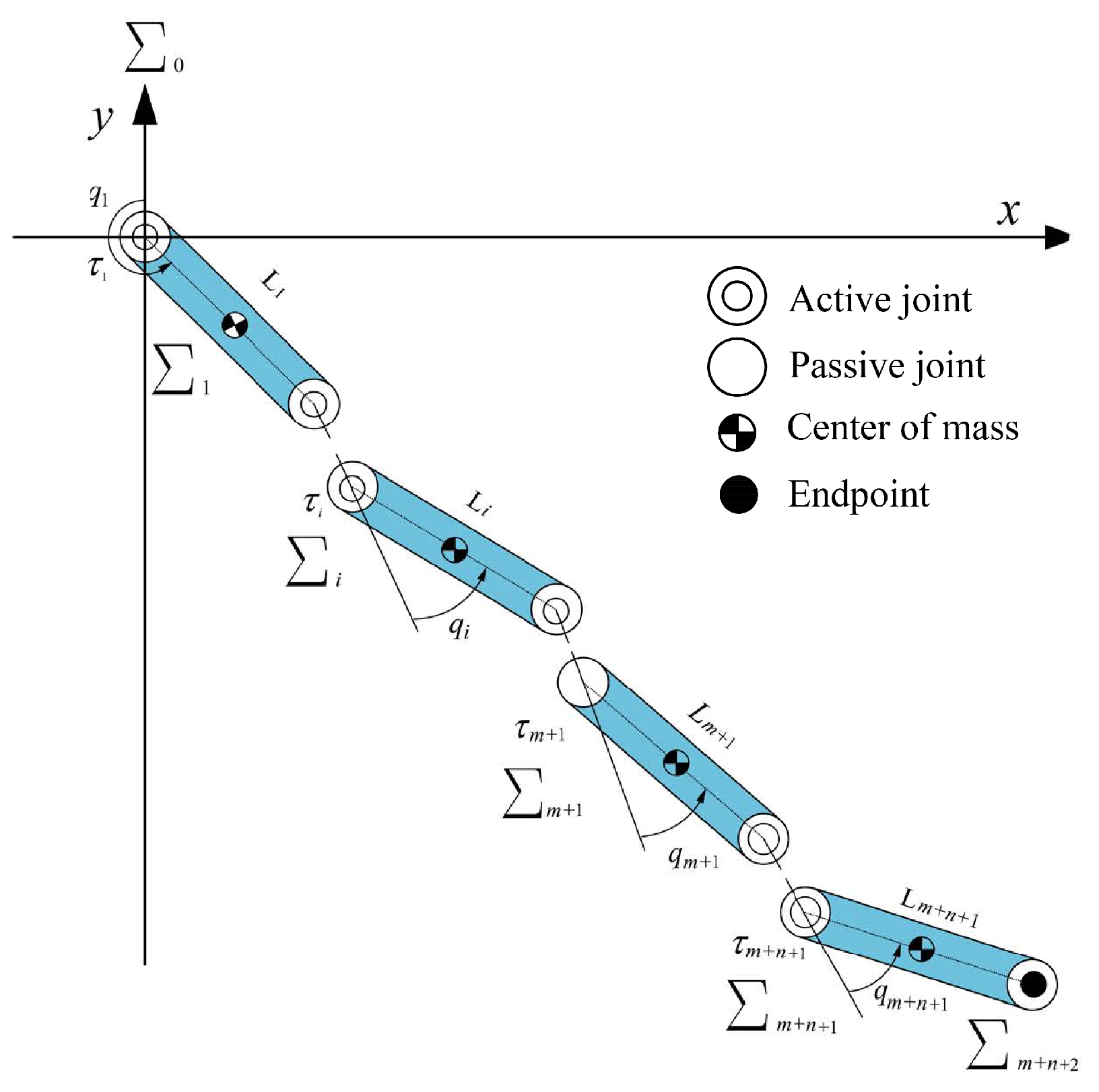
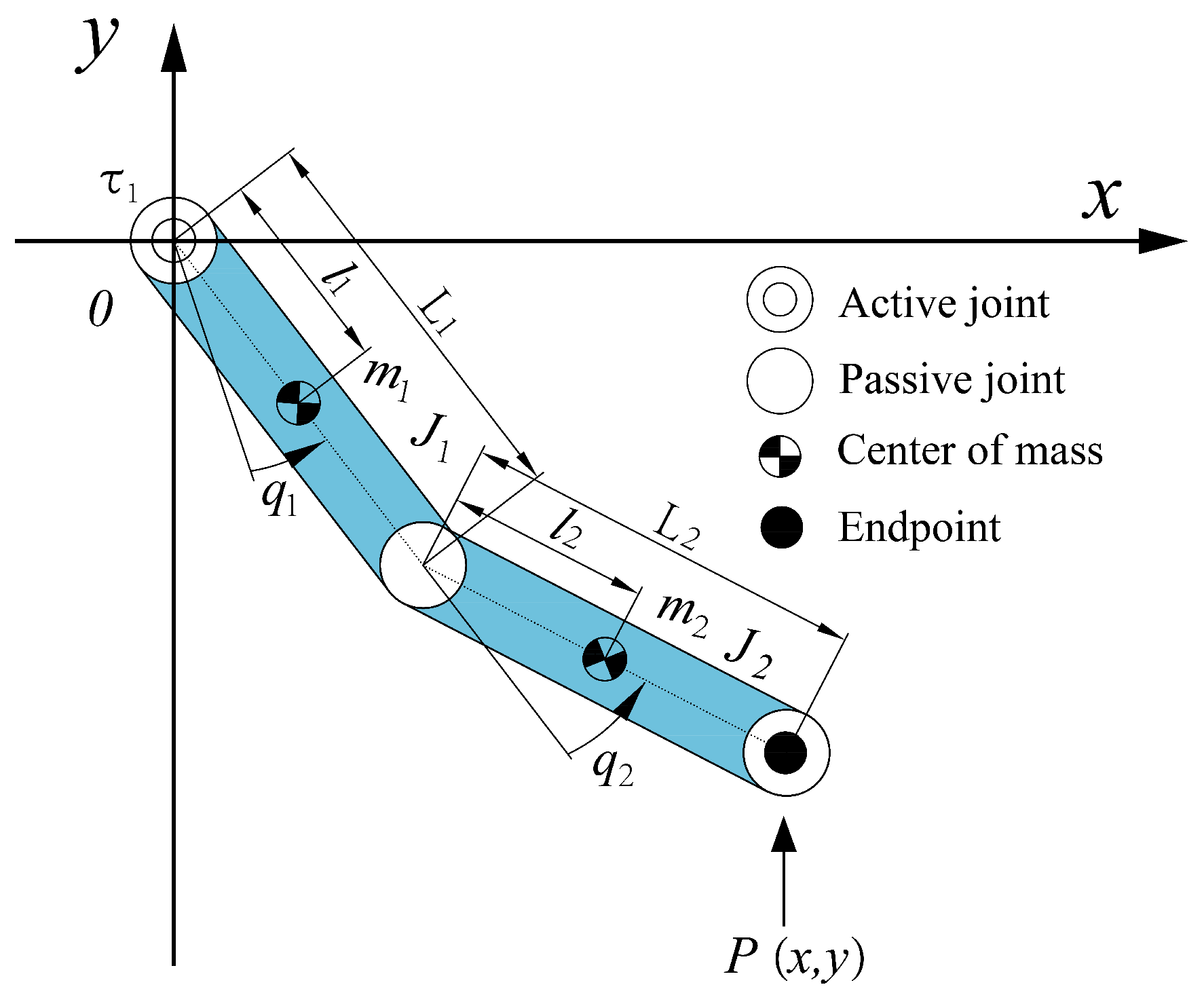
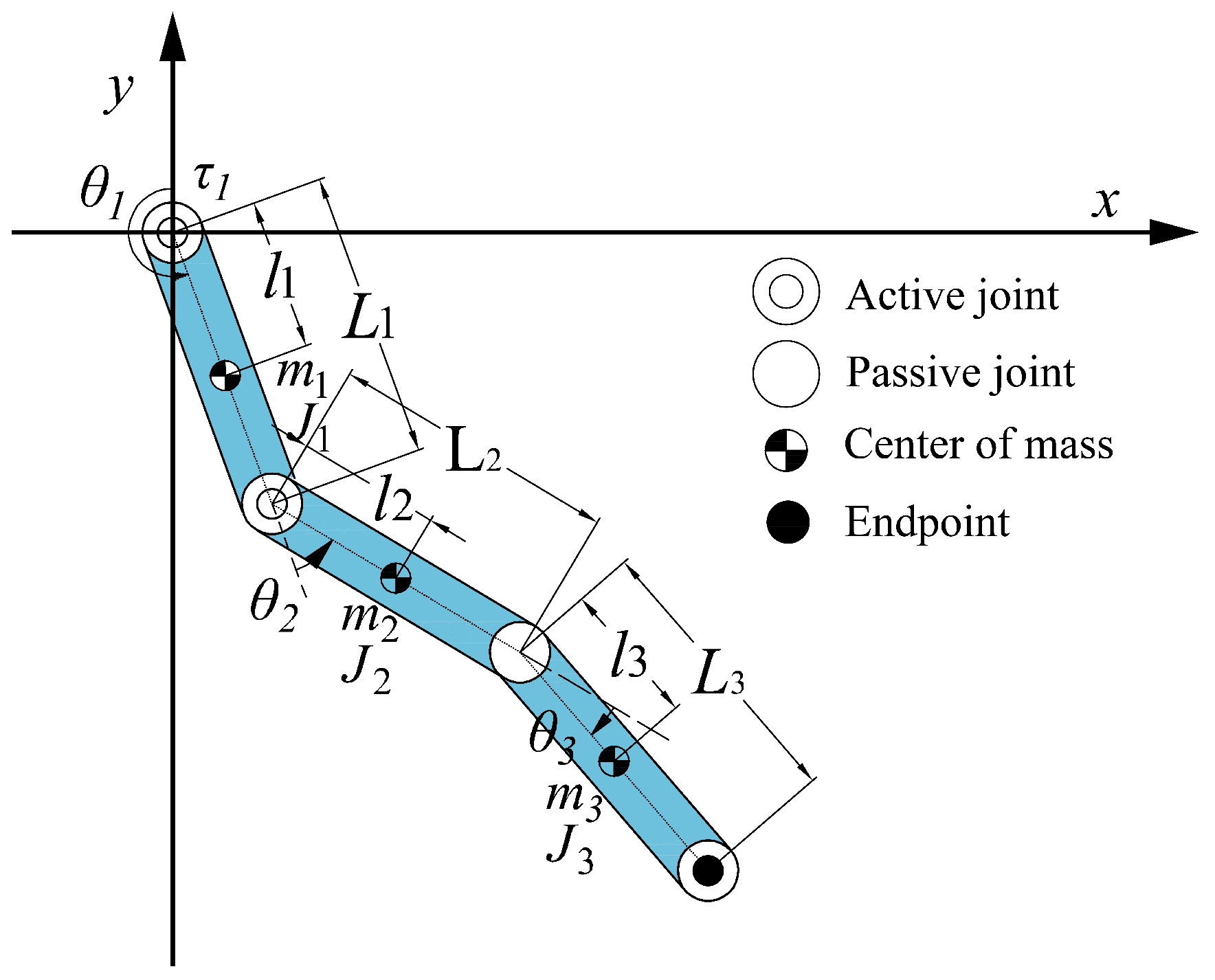
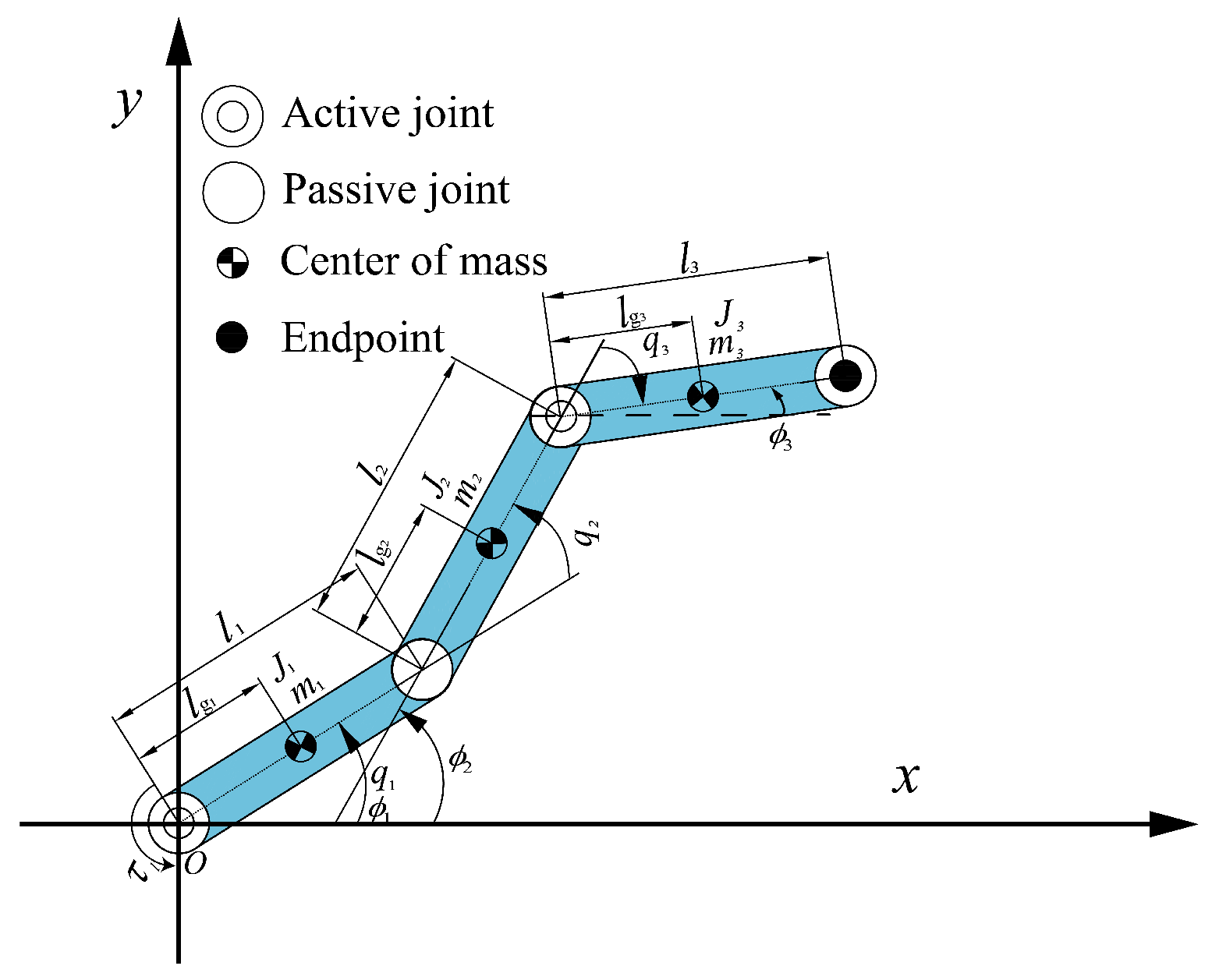
- Configuration 1: The planar manipulator, .
- Configuration 2: The planar manipulator, .
- Configuration 3: The planar P manipulator, .denotes the applied torque at the i-th joint and is the applied torque vector.
2.3. Control Properties
3. Control Techniques
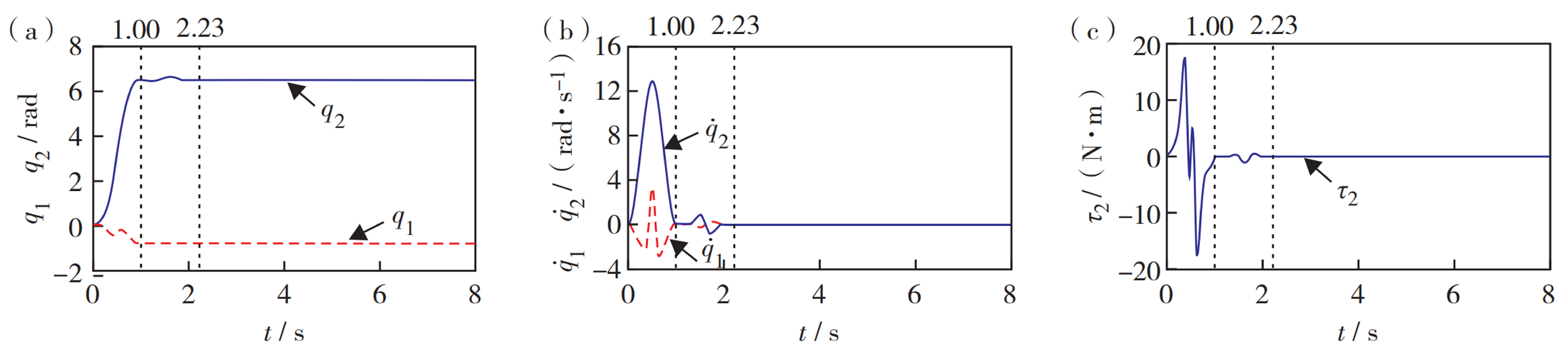
3.1. The Passive First Joint
3.1.1. Acrobot
3.1.2. PAA Manipulator System
3.1.3. Manipulator System
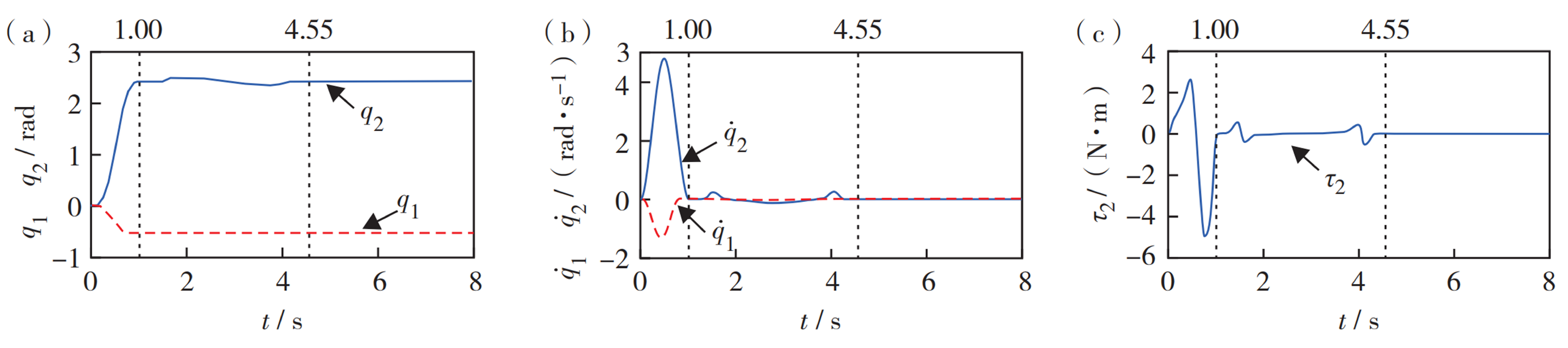
3.2. The Passive Last Joint
3.2.1. Pendubot
3.2.2. AAP Manipulator
3.2.3. P Manipulator Systems
3.3. The Passive Middle Joint
3.3.1. APA Manipulator Systems
3.3.2. Manipulator Systems
3.3.3. Manipulator Systems
4. Current Challenges of PUM
4.1. Theoretical Analysis
4.1.1. Research on Uncertainty and Robustness of System Model
4.1.2. Research on Unified Control Method
4.1.3. Research on Multi Passive Joints Underactuated System
4.2. Practical Applications
4.2.1. Anti-Interference
4.2.2. Multi-Underactuated Manipulator Coordination
4.2.3. Space Underactuated Manipulator
5. Concluding Remarks and Future Research
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Taghieh, A.; Zhang, C.; Alattas, K.A.; Bouteraa, Y.; Rathinasamy, S.; Mohammadzadeh, A. A predictive type-3 fuzzy control for underactuated surface vehicles. Ocean Eng. 2022, 266, 113014. [Google Scholar] [CrossRef]
- Fan, Y.; Qiu, B.; Liu, L.; Yang, Y. Global fixed-time trajectory tracking control of underactuated USV based on fixed-time extended state observer. ISA Trans. 2023, 132, 267–277. [Google Scholar] [CrossRef]
- Yu, Y.; Yang, Z.; Han, C.; Liu, H. Fuzzy Adaptive Back-Stepping Sliding Mode Controller for High-Precision Deflection Control of the Magnetically Suspended Momentum Wheel. IEEE Trans. Ind. Electron. 2018, 65, 3530–3538. [Google Scholar] [CrossRef]
- Hwang, C.L.; Wu, H.M.; Shih, C.L. Fuzzy Sliding-Mode Underactuated Control for Autonomous Dynamic Balance of an Electrical Bicycle. IEEE Trans. Control Syst. Technol. 2009, 17, 658–670. [Google Scholar] [CrossRef]
- Nourmohammadi, A.; Jafari, M.; Zander, T.O. A Survey on Unmanned Aerial Vehicle Remote Control Using Brain-Computer Interface. IEEE Trans. Hum.-Mach. Syst. 2018, 48, 337–348. [Google Scholar] [CrossRef]
- Abdessameud, A.; Tayebi, A. Formation control of VTOL Unmanned Aerial Vehicles with communication delays. Automatica 2011, 47, 2383–2394. [Google Scholar] [CrossRef]
- Donaire, A.; Romero, J.G.; Ortega, R. A Robust IDA-PBC Approach for Handling Uncertainties in Underactuated Mechanical Systems (vol 63, pg 3495, 2018). IEEE Trans. Autom. Control 2020, 65, 3223–3226. [Google Scholar] [CrossRef]
- Yang, T.; Sun, N.; Fang, Y. Adaptive Fuzzy Control for a Class of MIMO Underactuated Systems with Plant Uncertainties and Actuator Deadzones: Design and Experiments. IEEE Trans. Cybern. 2022, 52, 8213–8226. [Google Scholar] [CrossRef]
- Elhaki, O.; Shojaei, K.; Mehrmohammadi, P. Reinforcement learning-based saturated adaptive robust neural-network control of underactuated autonomous underwater vehicles. Expert Syst. Appl. 2022, 197, 116714. [Google Scholar] [CrossRef]
- Aurora, H.; Martin Syre, W.; Kristin Ytterstad, P. A Theoretical Analysis of the Velocity Obstacle Method for Nonholonomic Vehicles and Underactuated Surface Vessels. IEEE Trans. Control Syst. Technol. 2024, 32, 1801–1816. [Google Scholar]
- Zhang, A.; She, J.; Qiu, J.; Yang, C.; Alsaadi, F. A new control method for global stabilisation of translational oscillator with rotational actuator. Int. J. Syst. Sci. 2019, 50, 954–960. [Google Scholar] [CrossRef]
- Lu, J.; Meng, Z. Underactuated Attitude–Orbit Coupling Control for Microsatellite Based on a Single Orbital Thruster. IEEE Trans. Aerosp. Electron. Syst. 2024, 60, 2082–2092. [Google Scholar] [CrossRef]
- Shao, J.; Zhou, Q.; Xiao, Y.; Bernelli-Zazzera, F.; Sun, Z. Feasibility analysis and saturation control for underactuated spacecraft formation reconfiguration in elliptic orbits. Proc. Inst. Mech. Eng. Part G-J. Aerosp. Eng. 2024, 238, 211–227. [Google Scholar] [CrossRef]
- Kusznir, T.; Smoczek, J. Nonlinear Model Predictive Control with Evolutionary Data-Driven Prediction Model and Particle Swarm Optimization Optimizer for an Overhead Crane. Appl. Sci. 2024, 14, 5112. [Google Scholar] [CrossRef]
- Zhang, G.; Bian, W.; Li, J.; Zhang, W. Robust adaptive synchronized event-triggered formation control of USVs with the fault amendment strategy. Ocean Eng. 2023, 281, 114832. [Google Scholar] [CrossRef]
- Fan, L.; Zhang, A.; Liang, X.; Zhang, X.; Qiu, J. Fixed-time stabilization of underactuated cart-pendulum system based on hierarchical sliding mode control method. Nonlinear Dyn. 2024, 112, 11187–11194. [Google Scholar] [CrossRef]
- Zhai, M.; Diao, S.; Yang, T.; Wu, Q.; Fang, Y.; Sun, N. Adaptive Fuzzy Control for Underactuated Robot Systems With Inaccurate Actuated States and Unavailable Unactuated States. IEEE Trans. Autom. Sci. Eng. 2024, 1–13. [Google Scholar] [CrossRef]
- Gao, S.; Li, X.; Liu, J. Adaptive direct RBFNN consensus control for a class of unknown nonlinear underactuated systems. Int. J. Syst. Sci. 2024, 1–13. [Google Scholar] [CrossRef]
- Wang, Y.; Qu, Y.; Zhao, S.; Fu, H. Adaptive neural containment maneuvering of underactuated surface vehicles with prescribed performance and collision avoidance. Ocean Eng. 2024, 297, 116779. [Google Scholar] [CrossRef]
- Zhu, Z.; Zhao, H.; Xian, Y.; Chen, Y.H.; Sun, H.; Ma, J. Stackelberg Game-Based Control Design for Fuzzy Underactuated Mechanical Systems With Inequality Constraints. IEEE Trans. Syst. Man Cybern. Syst. 2024, 54, 6345–6357. [Google Scholar] [CrossRef]
- Xia, J.; Ouyang, H. Chattering Free Sliding-Mode Controller Design for Underactuated Tower Cranes with Uncertain Disturbance. IEEE Trans. Ind. Electron. 2024, 71, 4963–4975. [Google Scholar] [CrossRef]
- Gutierrez-Oribio, D.; Stefanou, I.; Plestan, F. Passivity-based control of underactuated mechanical systems with Coulomb friction: Application to earthquake prevention. Automatic 2024, 165, 111661. [Google Scholar] [CrossRef]
- Feng, Y.; Zhang, H.; Gu, C. The Prescribed-Time Sliding Mode Control for Underactuated Bridge Crane. Electronics 2024, 13, 219. [Google Scholar] [CrossRef]
- Lai, X.; Wang, Y.; Wu, M.; Cao, W. Stable Control Strategy for Planar Three-Link Underactuated Mechanical System. IEEE-ASME Trans. Mechatron. 2016, 21, 1345–1356. [Google Scholar] [CrossRef]
- Shu-chen, D.; Li, P.; Shang-ling, Q.; Rong-qiang, L.; Josephat, B. Dynamic modelling and PFL-based trajectory tracking control for underactuated cable-driven truss-like manipulator. J. Cent. South Univ. 2021, 28, 3127–3146. [Google Scholar] [CrossRef]
- Zhang, K.K.; Zhou, B.; Jiang, H.; Duan, G.R. Finite-Time Control of a Class of Nonlinear Underactuated Systems with Application to Underactuated Axisymmetric Spacecraft. IEEE Trans. Aerosp. Electron. Syst. 2023, 59, 7061–7071. [Google Scholar] [CrossRef]
- Fu, M.; Wang, L. Event-triggered-based finite-time cooperative path following control for underactuated multiple marine surface vehicles. Asian J. Control 2023, 25, 1005–1018. [Google Scholar] [CrossRef]
- Kazemi, H.; Majd, V.J.; Moghaddam, M.M. Modeling and robust backstepping control of an underactuated quadruped robot in bounding motion. Robotica 2013, 31, 423–439. [Google Scholar] [CrossRef]
- Manduca, G.; Santaera, G.; Miraglia, M.; Van Vuuren, G.J.; Dario, P.; Stefanini, C.; Romano, D. A Bioinspired Control Strategy Ensures Maneuverability and Adaptability for Dynamic Environments in an Underactuated Robotic Fish. J. Intell. Robot. Syst. 2024, 110, 69. [Google Scholar] [CrossRef]
- Abdurahman, B.; Savvaris, A.; Tsourdos, A. Switching LOS guidance with speed allocation and vertical course control for path-following of unmanned underwater vehicles under ocean current disturbances. Ocean Eng. 2019, 182, 412–426. [Google Scholar] [CrossRef]
- Bessa, W.M.; Otto, S.; Kreuzer, E.; Seifried, R. An adaptive fuzzy sliding mode controller for uncertain underactuated mechanical systems. J. Vib. Control 2019, 25, 1521–1535. [Google Scholar] [CrossRef]
- Backus, S.B.; Onishi, R.; Bocklund, A.; Berg, A.; Contreras, E.D.; Parness, A. Design and testing of the JPL-Nautilus Gripper for deep-ocean geological sampling. J. Field Robot. 2020, 37, 972–986. [Google Scholar] [CrossRef]
- Liljeback, P.; Pettersen, K.Y.; Stavdahl, A.; Gravdahl, J.T. Controllability and Stability Analysis of Planar Snake Robot Locomotion. IEEE Trans. Autom. Control 2011, 56, 1365–1380. [Google Scholar] [CrossRef]
- Zhang, A.; She, J.; Qiu, J.; Yang, C.; Alsaadi, F. Design of motion trajectory and tracking control for underactuated cart-pendulum system. Int. J. Robust Nonlinear Control 2019, 29, 2458–2470. [Google Scholar] [CrossRef]
- Celikovsky, S.; Anderle, M. Feedback equivalence of the chained mechanical system to the almost linear form and its use for the sustainable multi-step walking design. J. Frankl. Inst.-Eng. Appl. Math. 2024, 361, 107086. [Google Scholar] [CrossRef]
- Tang, L.; Wang, C.; Ma, S.; Li, Y.; Li, B. Multidirectional Planar Motion Transmission on a Single-Motor Actuated Robot via Microscopic Galumphing. Adv. Sci. 2024, 11, 2307738. [Google Scholar] [CrossRef]
- Quigley, M.; Salisbury, C.; Ng, A.Y.; Salisbury, J.K. Mechatronic design of an integrated robotic hand. Int. J. Robot. Res. 2014, 33, 706–720. [Google Scholar] [CrossRef]
- Higashimori, M.; Sakashita, R.; Shibata, A. Single-Actuator-Based Three-DoF Planar Manipulation via a Viscoelastic and Nonparallel Hybrid Joint Mechanism. IEEE Trans. Robot. 2019, 35, 602–617. [Google Scholar] [CrossRef]
- Krupinski, S.; Allibert, G.; Hua, M.D.; Hamel, T. An Inertial-Aided Homography-Based Visual Servo Control Approach for (Almost) Fully Actuated Autonomous Underwater Vehicles. IEEE Trans. Robot. 2017, 33, 1041–1060. [Google Scholar] [CrossRef]
- Meza-Sanchez, M.; Rodriguez-Linan, M.C.; Clemente, E.; Herrera, L. Evolutionary design of swing-up controllers for stabilization task of underactuated inverted pendulums. Genet. Program. Evolvable Mach. 2023, 24, 9. [Google Scholar] [CrossRef]
- Romano, D.; Wahi, A.; Miraglia, M.; Stefanini, C. Development of a Novel Underactuated Robotic Fish with Magnetic Transmission System. Machines 2022, 10, 755. [Google Scholar] [CrossRef]
- Guan, H.; Wong, K.C. Spring-Damped Underactuated Swashplateless Rotor on a Bicopter Unmanned Aerial Vehicle. Machines 2024, 12, 296. [Google Scholar] [CrossRef]
- Salamat, B.; Elsbacher, G. Centralized Control in Networks of Underactuated Nonidentical Euler-Lagrange Systems Using a Generalised Multicoordinates Transformation. IEEE Access 2022, 10, 58311–58319. [Google Scholar] [CrossRef]
- Pappalardo, C.M.; Guida, D. Forward and Inverse Dynamics of a Unicycle-Like Mobile Robot. Machines 2019, 7, 5. [Google Scholar] [CrossRef]
- Esposito, D.; Centracchio, J.; Andreozzi, E.; Savino, S.; Gargiulo, G.D.; Naik, G.R.; Bifulco, P. Design of a 3D-Printed Hand Exoskeleton Based on Force-Myography Control for Assistance and Rehabilitation. Machines 2022, 10, 57. [Google Scholar] [CrossRef]
- Kim, Y.; Kwon, S. Balancing-Prioritized Anti-Slip Control of a Two-Wheeled Inverted Pendulum Robot Vehicle on Low-Frictional Surfaces with an Acceleration Slip Indicator. Machines 2023, 11, 553. [Google Scholar] [CrossRef]
- Maruskin, J.M.; Bloch, A.M. The Boltzmann-Hamel equations for the optimal control of mechanical systems with nonholonomic constraints. Int. J. Robust Nonlinear Control 2011, 21, 373–386. [Google Scholar] [CrossRef]
- Ma, B.L.; Tso, S.K. Unified controller for both trajectory tracking and point regulation of second-order nonholonomic chained systems. Robot. Auton. Syst. 2008, 56, 317–323. [Google Scholar] [CrossRef]
- Zhang, A.; Lai, X.; Wu, M.; She, J. Stabilization of underactuated two-link gymnast robot by using trajectory tracking strategy. Appl. Math. Comput. 2015, 253, 193–204. [Google Scholar] [CrossRef]
- Lai, X.Z.; She, J.H.; Cao, W.H.; Yang, S.X. Stabilization of underactuated planar acrobot based on motion-state constraints. Int. J. Non-Linear Mech. 2015, 77, 342–347. [Google Scholar] [CrossRef]
- Wen-An, J.; Zhuang-Jun, L.; Shao-Kai, L. Mei symmetries and Mei conserved quantities for higher-order nonholonomic constraint systems. Chin. Phys. 2011, 20, 3. [Google Scholar] [CrossRef]
- Zhang, P.; Lai, X.; Wang, Y.; Su, C.; Ye, W.; Wu, M. A novel position-posture control method using intelligent optimization for planar underactuated mechanical systems. Mech. Mach. Theory 2019, 140, 258–273. [Google Scholar] [CrossRef]
- Zhang, P.; Lai, X.; Wang, Y.; Wu, M. Effective position-posture control strategy based on switching control for planar three-link underactuated mechanical system. Int. J. Syst. Sci. 2017, 48, 2202–2211. [Google Scholar] [CrossRef]
- Gao, X.; Ren, Z.; Zhai, L.; Jia, Q.; Liu, H. Two-Stage Switching Hybrid Control Method Based on Improved PSO for Planar Three-Link Under-Actuated Manipulator. IEEE Access 2019, 7, 76263–76273. [Google Scholar] [CrossRef]
- Lai, X.; Zhang, P.; Wang, Y.; Chen, L.; Wu, M. Continuous State Feedback Control Based on Intelligent Optimization for First-Order Nonholonomic Systems. IEEE Trans. Syst. Man Cybern.-Syst. 2020, 50, 2534–2540. [Google Scholar] [CrossRef]
- Zhang, P.; Lai, X.; Wang, Y.; Su, C.Y.; Wu, M. A quick position control strategy based on optimization algorithm for a class of first-order nonholonomic system. Inf. Sci. 2018, 460, 264–278. [Google Scholar] [CrossRef]
- Zhang, P.; Lai, X.; Wang, Y.; Wu, M. Motion planning and adaptive neural sliding mode tracking control for positioning of uncertain planar underactuated manipulator. Neurocomputing 2019, 334, 197–205. [Google Scholar] [CrossRef]
- Meng, Q.; Lai, X.; Yan, Z.; Wu, M. Tip Position Control and Vibration Suppression of a Planar Two-Link Rigid-Flexible Underactuated Manipulator. IEEE Trans. Cybern. 2022, 52, 6771–6783. [Google Scholar] [CrossRef]
- Lai, X.; Zhang, P.; Wang, Y.; Wu, M. Position-Posture Control of a Planar Four-Link Underactuated Manipulator Based on Genetic Algorithm. IEEE Trans. Ind. Electron. 2017, 64, 4781–4791. [Google Scholar] [CrossRef]
- Lai, X.Z.; Wang, Y.W.; Cao, J.Q.; Wu, M. A simple and quick control strategy for a class of first-order nonholonomic manipulator. Nonlinear Dyn. 2016, 85, 2261–2276. [Google Scholar] [CrossRef]
- Wang, Y.; Lai, X.; Chen, L.; Ding, H.; Wu, M. A quick control strategy based on hybrid intelligent optimization algorithm for planar n-link underactuated manipulators. Inf. Sci. 2017, 420, 148–158. [Google Scholar] [CrossRef]
- Wang, Y.W.; Lai, X.Z.; Zhang, P.; Su, C.Y.; Wu, M. A new control method for planar four-link underactuated manipulator based on intelligence optimization. Nonlinear Dyn. 2019, 96, 573–583. [Google Scholar] [CrossRef]
- Wang, Y.; Lai, X.; Zhang, P.; Wu, M. Adaptive robust control for planar n-link underactuated manipulator based on radial basis function neural network and online iterative correction method. J. Frankl. Inst.-Eng. Appl. Math. 2018, 355, 8373–8391. [Google Scholar] [CrossRef]
- Wang, Y.; Lai, X.; Zhang, P.; Wu, M. Control Strategy Based on Model Reduction and Online Intelligent Calculation for Planar <i>n</i>-Link Underactuated Manipulators. IEEE Trans. Syst. Man Cybern.-Syst. 2020, 50, 1046–1054. [Google Scholar] [CrossRef]
- Tafrishi, S.A.; Svinin, M.; Yamamoto, M. Inverse dynamics of underactuated planar manipulators without inertial coupling singularities. Multibody Syst. Dyn. 2021, 52, 407–429. [Google Scholar] [CrossRef]
- De Luca, A.; Mattone, R.; Oriolo, G. Stabilization of underactuated robots: Theory and experiments for a planar 2R manipulator. In Proceedings of the International Conference on Robotics and Automation, Albuquerque, NM, USA, 20–25 April 1997; Volume 4, pp. 3274–3280. [Google Scholar] [CrossRef]
- De Luca, A.; Mattone, R.; Oriolo, G. Control of underactuated mechanical systems: Application to the planar 2R robot. In Proceedings of the 35th IEEE Conference on Decision and Control, Kobe, Japan, 11–13 December 1996; Volume 2, pp. 1455–1460. [Google Scholar] [CrossRef]
- Luca, A.D.; Mattone, R.; Oriolo, G. Stabilization of an Underactuated Planar 2R Manipulator. Int. J. Robust Nonlinear Control 2000, 10, 181–198. [Google Scholar] [CrossRef]
- He, G.P.; Wang, Z.L.; Zhang, J.; Geng, Z.Y. Characteristics analysis and stabilization of a planar 2R underactuated manipulator. Robotica 2016, 34, 584–600. [Google Scholar] [CrossRef]
- Suzuki, T.; Koinuma, M.; Nakamura, Y. Chaos and nonlinear control of a nonholonomic free-joint manipulator. In Proceedings of the IEEE International Conference on Robotics and Automation, Minneapolis, MN, USA, 22–28 April 1996; Volume 3, pp. 2668–2675. [Google Scholar] [CrossRef]
- Suzuki, T.; Nakamura, Y. Control of manipulators with free-joints via the averaging method. In Proceedings of the International Conference on Robotics and Automation, Albuquerque, NM, USA, 20–25 April 1997; Volume 4, pp. 2998–3005. [Google Scholar] [CrossRef]
- Rosas-Flors, J.; Alvarez-Gallegos, J.; Castro-Linares, R. Trajectory planning and control of an underactuated planar 2R manipulator. In Proceedings of the 2001 IEEE International Conference on Control Applications (CCA’01) (Cat. No.01CH37204), Mexico City, Mexico, 7 September 2001; pp. 548–552. [Google Scholar] [CrossRef]
- Wu, J.; Wang, Y.; Ye, W.; Su, C.Y. Control strategy based on Fourier transformation and intelligent optimization for planar Pendubot. Inf. Sci. 2019, 491, 279–288. [Google Scholar] [CrossRef]
- Zhang, A.; Lai, X.; Wu, M.; She, J. Global stabilization of underactuated spring-coupled three-link horizontal manipulator using position measurements only. Appl. Math. Model. 2015, 39, 1917–1928. [Google Scholar] [CrossRef]
- Ramirez-Neria, M.; Sira-Ramirez, H.; Garrido-Moctezuma, R.; Luviano-Juarez, A.; Gao, Z. Active Disturbance Rejection Control for Reference Trajectory Tracking Tasks in the Pendubot System. IEEE Access 2021, 9, 102663–102670. [Google Scholar] [CrossRef]
- Xin, X.; Makino, K.; Izumi, S.; Yamasaki, T.; Liu, Y. Anti-Swing control of the Pendubot using damper and spring with positive or negative stiffness. Int. J. Robust Nonlinear Control 2021, 31, 4227–4246. [Google Scholar] [CrossRef]
- Harandi, M.R.J.; Taghirad, H.D. On the matching equations of kinetic energy shaping in IDA-PBC. J. Frankl.-Inst.-Eng. Appl. Math. 2021, 358, 8639–8655. [Google Scholar] [CrossRef]
- Parulski, P.; Bartkowiak, P.; Pazderski, D. Evaluation of Linearization Methods for Control of the Pendubot. Appl. Sci. 2021, 11, 7615. [Google Scholar] [CrossRef]
- Turrisi, G.; Capotondi, M.; Gaz, C.; Modugno, V.; Oriolo, G.; De Luca, A. On-Line Learning for Planning and Control of Underactuated Robots with Uncertain Dynamics. IEEE Robot. Autom. Lett. 2022, 7, 358–365. [Google Scholar] [CrossRef]
- Wei, C.; Chen, Y.H.; Chai, T.; Fu, J. Adaptive robust control for Pendubot with matched-mismatched uncertainty via constraint-following. Robotica 2023, 41, 1550–1567. [Google Scholar] [CrossRef]
- Wei, C.; Vardulakis, A.; Chai, T. Stabilization of the Pendubot: A polynomial matrix approach. IMA J. Math. Control Inf. 2023, 40, 332–352. [Google Scholar] [CrossRef]
- Xin, X.; Wang, Z.; Liu, Y. Parameterization of minimal order strongly stabilizing controllers for two-link underactuated planar robot with single sensor. Automatica 2023, 158, 111280. [Google Scholar] [CrossRef]
- Yan, Z.; Lai, X.; Meng, Q.; Wu, M.; She, J.; Iwasaki, M. Modeling, analysis, and adaptive neural modified-backstepping control of an uncertain horizontal pendubot with double flexible joints. Control Eng. Pract. 2023, 139, 105647. [Google Scholar] [CrossRef]
- Gulan, M.; Salaj, M.; RohaI-Ilkiv, B. Tracking Control of Unforced and Forced Equilibrium Positions of the Pendubot System: A Nonlinear MHE and MPC Approach. Actuators 2023, 12, 343. [Google Scholar] [CrossRef]
- Mahindrakar, A.D.; Banavar, R.N.; Reyhanoglu, M. Controllability and point-to-point control of 3-DOF planar horizontal underactuated manipulators. Int. J. Control 2005, 78, 1–13. [Google Scholar] [CrossRef]
- Arai, H.; Tanie, K. Nonhoronomic control of a three-DOF planar underactuated manipulator. IEEE Trans. Robot. Autom. 1998, 14, 681–695. [Google Scholar] [CrossRef]
- de Luca, A.; Oriolo, G. Motion planning and trajectory control of an underactuated three-link robot via dynamic feedback linearization. In Proceedings of the 2000 ICRA. Millennium Conference. IEEE International Conference on Robotics and Automation. Symposia Proceedings (Cat. No.00CH37065), San Francisco, CA, USA, 24–28 April 2000; Volume 3, pp. 2789–2795. [Google Scholar] [CrossRef]
- Shiroma, N.; Arai, H.; Tanie, K. Nonlinear control of a planar free link under a nonholonomic constraint. In Proceedings of the 1997 8th International Conference on Advanced Robotics. Proceedings. ICAR’97, Monterey, CA, USA, 7–9 July 1997; pp. 103–109. [Google Scholar] [CrossRef]
- Lynch, K.M.; Shiroma, N.; Arai, H.; Tanie, K. Collision-free trajectory planning for a 3-DoF robot with a passive joint. Int. J. Robot. Res. 2000, 19, 1171–1184. [Google Scholar] [CrossRef]
- Arai, H. Controllability of a 3-DOF manipulator with a passive joint under a nonholonomic constraint. In Proceedings of the IEEE International Conference on Robotics and Automation, Minneapolis, MN, USA, 22–28 April 1996; Volume 4, pp. 3707–3713. [Google Scholar] [CrossRef]
- Arai, H. Position control of a 3-DOF manipulator with a passive joint under a nonholonomic constraint. In Proceedings of the IEEE/RSJ International Conference on Intelligent Robots and Systems. IROS ’96, Osaka, Japan, 8 November 1996; Volume 1, p. 74. [Google Scholar] [CrossRef]
- Lynch, K.; Shiroma, N.; Arai, H.; Tanie, K. Motion planning for a 3-DOF robot with a passive joint. In Proceedings of the 1998 IEEE International Conference on Robotics and Automation (Cat. No.98CH36146), Leuven, Belgium, 20 May 1998; Volume 2, pp. 927–932. [Google Scholar] [CrossRef]
- Arai, H.; Tanie, K.; Shiroma, N. Feedback control of a 3-DOF planar underactuated manipulator. In Proceedings of the International Conference on Robotics and Automation, Albuquerque, NM, USA, 25 April 1997; Volume 1, pp. 703–709. [Google Scholar] [CrossRef]
- Arai, H.; Tachi, S. Dynamic control of a manipulator with passive joints in an operational coordinate space. In Proceedings of the 1991 IEEE International Conference on Robotics and Automation, Sacramento, CA, USA, 9–11 April 1991; Volume 2, pp. 1188–1194. [Google Scholar] [CrossRef]
- Liu, Q.; Yu, Y.; Su, L. A new method for position control of planar 3-Dof underactuated robots. In Proceedings of the 2008 7th World Congress on Intelligent Control and Automation, Chongqing, China, 25–27 June 2008; pp. 9060–9065. [Google Scholar] [CrossRef]
- Urrea, C.; Kern, J.; Alvarez, E. Design and implementation of fault-tolerant control strategies for a real underactuated manipulator robot. Complex Intell. Syst. 2022, 8, 5101–5123. [Google Scholar] [CrossRef]
- Wu, H.; Kong, L.; Li, Q.; Wang, H.; Chen, G. A Comparative Study on Kinematic Calibration for a 3-DOF Parallel Manipulator Using the Complete-Minimal, Inverse-Kinematic and Geometric-Constraint Error Models. Chin. J. Mech. Eng. (Engl. Ed.) 2023, 36, 121. [Google Scholar] [CrossRef]
- Huang, Z.; Lai, X.; Zhang, P.; Meng, Q.; Wu, M. A general control strategy for planar 3-DoF underactuated manipulators with one passive joint. Inf. Sci. 2020, 534, 139–153. [Google Scholar] [CrossRef]
- Yan, Z.; Lai, X.; Meng, Q.; She, J.; Wu, M. Double-loop frame-based adaptive neural sliding-mode control of single-input 3-DOF flexible-joint manipulator. Int. J. Syst. Sci. 2024, 55, 1177–1190. [Google Scholar] [CrossRef]
- De Luca, A.; Iannitti, S. A simple STLC test for mechanical systems underactuated by one control. In Proceedings of the 2002 IEEE International Conference on Robotics and Automation (Cat. No.02CH37292), Washington, DC, USA, 11–15 May 2002; Volume 2, pp. 1735–1740. [Google Scholar] [CrossRef]
- Kobayashi, K.; Yoshikawa, T. Controllability of under-actuated planar manipulators with one unactuated joint. In Proceedings of the 2000 IEEE/RSJ International Conference on Intelligent Robots and Systems (IROS 2000) (Cat. No.00CH37113), Takamatsu, Japan, 31 October–5 November 2000; Volume 1, pp. 133–138. [Google Scholar] [CrossRef]
- De Luca, A.; Oriolo, G. Trajectory Planning and Control for Planar Robots with Passive Last Joint. Int. J. Robot. Res. 2002, 21, 575–590. [Google Scholar] [CrossRef]
- De Luca, A.; Iannitti, S.; Mattone, R.; Oriolo, G. Control problems in underactuated manipulators. In Proceedings of the 2001 IEEE/ASME International Conference on Advanced Intelligent Mechatronics. Proceedings (Cat. No.01TH8556), Como, Italy, 8–12 July 2001; Volume 2, pp. 855–861. [Google Scholar] [CrossRef]
- Huang, Z.; Wei, S.; Hou, M.; Wang, L. Finite-time control strategy for swarm planar underactuated robots via motion planning and intelligent algorithm. Meas. Control 2023, 56, 813–819. [Google Scholar] [CrossRef]
- Lai, X.; Chen, H.; Wang, Y.; Yuan, Y.; Wu, M. Trajectory tracking control with specified posture for planar four-link real underactuated manipulator. Int. J. Robot. Autom. 2019, 34, 194–202. [Google Scholar] [CrossRef]
- Lai, X.; Xiong, P.; Wu, M. Stable control strategy for a second-order nonholonomic planar underactuated mechanical system. Int. J. Syst. Sci. 2019, 50, 2126–2141. [Google Scholar] [CrossRef]
- Chen, H.; Lai, X. Piecewise control strategy for a planar four-link underactuated manipulator with friction. In Proceedings of the 2018 37th Chinese Control Conference (CCC), Wuhan, China, 25–27 July 2018; pp. 3716–3719. [Google Scholar]
- Huang, Z.; Lai, X.; Wang, Y.; Wu, M. Position control for planar 3R underactuated manipulator with second-order non-holonomic constraints. J. Southeast Univ. (Nat. Sci. Ed.) 2019, 49, 245–250. [Google Scholar]
- De Luca, A.; Iannitti, S.; Mattone, R.; Oriolo, G. Underactuated manipulators: Control properties and techniques. Mach. Intell. Robot. Control 2002, 4, 113–125. [Google Scholar]
- Bhave, M.; Janardhanan, S.; Dewan, L. Configuration control of planar underactuated robotic manipulator using terminal sliding mode. IFAC-PapersOnLine 2016, 49, 148–153. [Google Scholar] [CrossRef]
- Xiong, P.; Lai, X.; Wu, M. Position control strategy based on energy attenuation for planar three-link underactuated manipulator. In Proceedings of the 2016 35th Chinese Control Conference (CCC), Chengdu, China, 27–29 July 2016; pp. 704–708. [Google Scholar]
- Li, J.; Wang, L.; Chen, Z.; Huang, Z. Drift suppression control based on online intelligent optimization for planar underactuated manipulator with passive middle joint. IEEE Access 2021, 9, 38611–38619. [Google Scholar] [CrossRef]
- Huang, Z.; Lai, X.; Zhang, P.; Wang, Y.; Wu, M. Virtual model reduction-based control strategy of planar three-link underactuated manipulator with middle passive joint. Int. J. Control Autom. Syst. 2021, 19, 29–39. [Google Scholar] [CrossRef]
- Xiong, P.; Lai, X.; Wu, M. Position control for planar four-link underactuated mechanical system based on model degeneration. Control Decis. 2015, 30, 1277–1283. [Google Scholar]
- Xiong, P.; Lai, X.; Wu, M. A stable control for second-order nonholonomic planar underactuated mechanical system: Energy attenuation approach. Int. J. Control 2018, 91, 1630–1639. [Google Scholar] [CrossRef]
- Huang, Z.; Li, X.; Wei, Z.; Wan, X.; Wang, L. A stable control method for planar robot with underactuated constraints via motion planning and intelligent optimization. Meas. Control 2023, 56, 1826–1834. [Google Scholar] [CrossRef]
- Xiong, P.; Lai, X.; Wu, M. Position and attitude control for a class of planar underactuated mechanical system with second order non-holonomic constraints. J. Southeast Univ. 2015, 45, 690–695. [Google Scholar]
- Xiong, P.; Lai, X.; Wu, M. Position and posture control for a class of second-order nonholonomic underactuated mechanical system. IMA J. Math. Control Inf. 2018, 35, 523–533. [Google Scholar] [CrossRef]
- Lai, X.Z.; Pan, C.Z.; Wu, M.; Yang, S.X. Unified control of n-link underactuated manipulator with single passive joint: A reduced order approach. Mech. Mach. Theory 2012, 56, 170–185. [Google Scholar] [CrossRef]
- Liu, D.; Lai, X.; Wang, Y.; Wan, X.; Wu, M. Position control for planar four-link underactuated manipulator with a passive third joint. ISA Trans. 2019, 87, 46–54. [Google Scholar] [CrossRef] [PubMed]
- Wang, Y.; Chen, S.; Zhang, P. Position-posture Control Strategy for Planar Underactuated Manipulators with Second-order Nonholonomic Constraint. Int. J. Control Autom. Syst. 2022, 20, 4015–4025. [Google Scholar] [CrossRef]
- Wu, J.; Ye, W.; Wang, Y.; Su, C.Y. A general position control method for planar underactuated manipulators with second-order nonholonomic constraints. IEEE Trans. Cybern. 2019, 51, 4733–4742. [Google Scholar] [CrossRef] [PubMed]
- Sun, N.; Fang, Y. A review for the control of a class of underactuated systems. CAAI Trans. Intell. Syst. 2011, 6, 200–207. [Google Scholar]
- Huang, Z.; Hou, M.; Hua, Y.; Yu, C.; Wang, L. A General Stable Control Method for R-Type Underactuated Robot with Three Different Initial Situations. Appl. Sci. 2023, 13, 5565. [Google Scholar] [CrossRef]
- Suzuki, T.; Kinoshita, T.; Gunji, S. Analysis and control of 3R underactuated manipulator. In Proceedings of the 2008 SICE Annual Conference, Tokyo, Japan, 20–22 August 2008; pp. 3086–3091. [Google Scholar]
- Huang, Z.; Wei, Z.; Wang, W.; Gong, X.; Zeng, B.; Wang, L. Controllability Analysis and Stable Control for Planar Non-One Passive Joint Underactuated Robot with Bi-Directional Approach. In Proceedings of the 2023 42nd Chinese Control Conference (CCC), Tianjin, China, 24–26 July 2023; pp. 507–510. [Google Scholar]
- Dong, Z.; Li, J.; Liu, W.; Zhang, H.; Qi, S.; Zhang, Z. Adptive heading control of underactuated unmanned surface vehicle based on improved backpropagation neural network. Pol. Marit. Res. 2023, 30, 54–64. [Google Scholar] [CrossRef]
- Peng, J.; Wu, H.; Zhang, C.; Chen, Q.; Meng, D.; Wang, X. Modeling, Cooperative Planning and Compliant Control of Multi-arm Space Continuous Robot for Target Manipulation. Appl. Math. Model. 2023, 121, 690–713. [Google Scholar] [CrossRef]
- Hattori, M.; Yabuno, H. Reachable area of an underactuated space manipulator subjected to simple spinning. Nonlinear Dyn. 2008, 51, 345–353. [Google Scholar] [CrossRef]




| Advantages | Disadvantages |
|---|---|
| Lower cost | Increased control complexity |
| Simple structure | Performance constraint |
| Small size | Precision and stability challenges |
| Low energy consumption | Poor adaptability to environmental changes |
| Easy to maintain and repair | Increased difficulty in design and optimization |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Huang, Z.; Yu, C.; Zeng, B.; Gong, X.; Zhou, H. A Survey of Planar Underactuated Mechanical System. Machines 2024, 12, 829. https://doi.org/10.3390/machines12120829
Huang Z, Yu C, Zeng B, Gong X, Zhou H. A Survey of Planar Underactuated Mechanical System. Machines. 2024; 12(12):829. https://doi.org/10.3390/machines12120829
Chicago/Turabian StyleHuang, Zixin, Chengsong Yu, Ba Zeng, Xiangyu Gong, and Hongjian Zhou. 2024. "A Survey of Planar Underactuated Mechanical System" Machines 12, no. 12: 829. https://doi.org/10.3390/machines12120829
APA StyleHuang, Z., Yu, C., Zeng, B., Gong, X., & Zhou, H. (2024). A Survey of Planar Underactuated Mechanical System. Machines, 12(12), 829. https://doi.org/10.3390/machines12120829







