An Experimental and Numerical Study of the Laser Ablation of Bronze
Abstract
1. Introduction
2. Materials and Methods
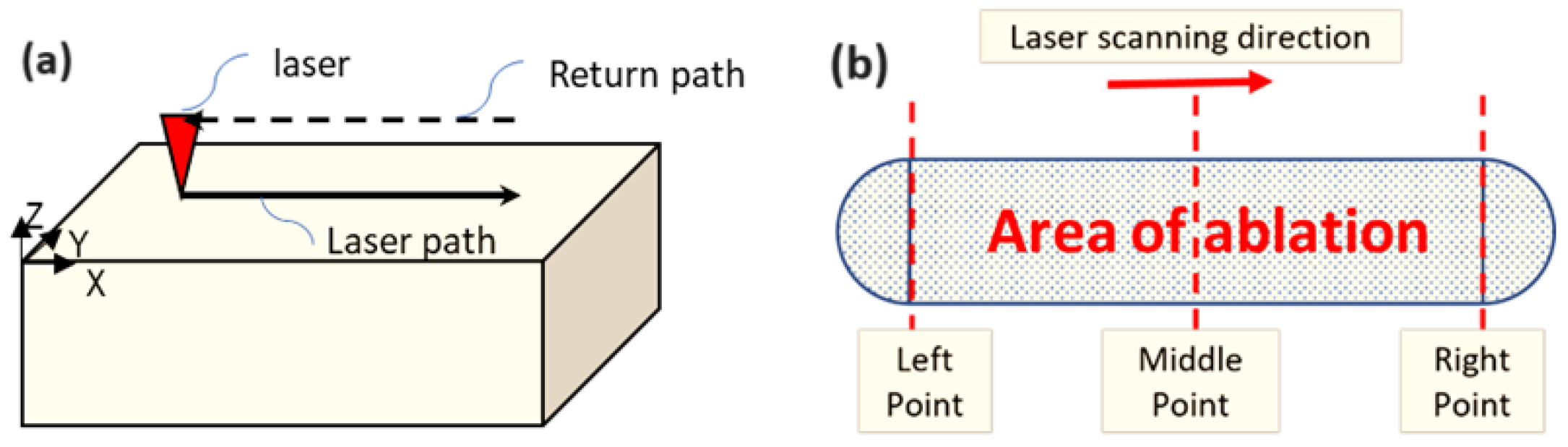
2.1. Experimental Process
2.2. Modeling and Simulation Methodology
3. Results and Discussion
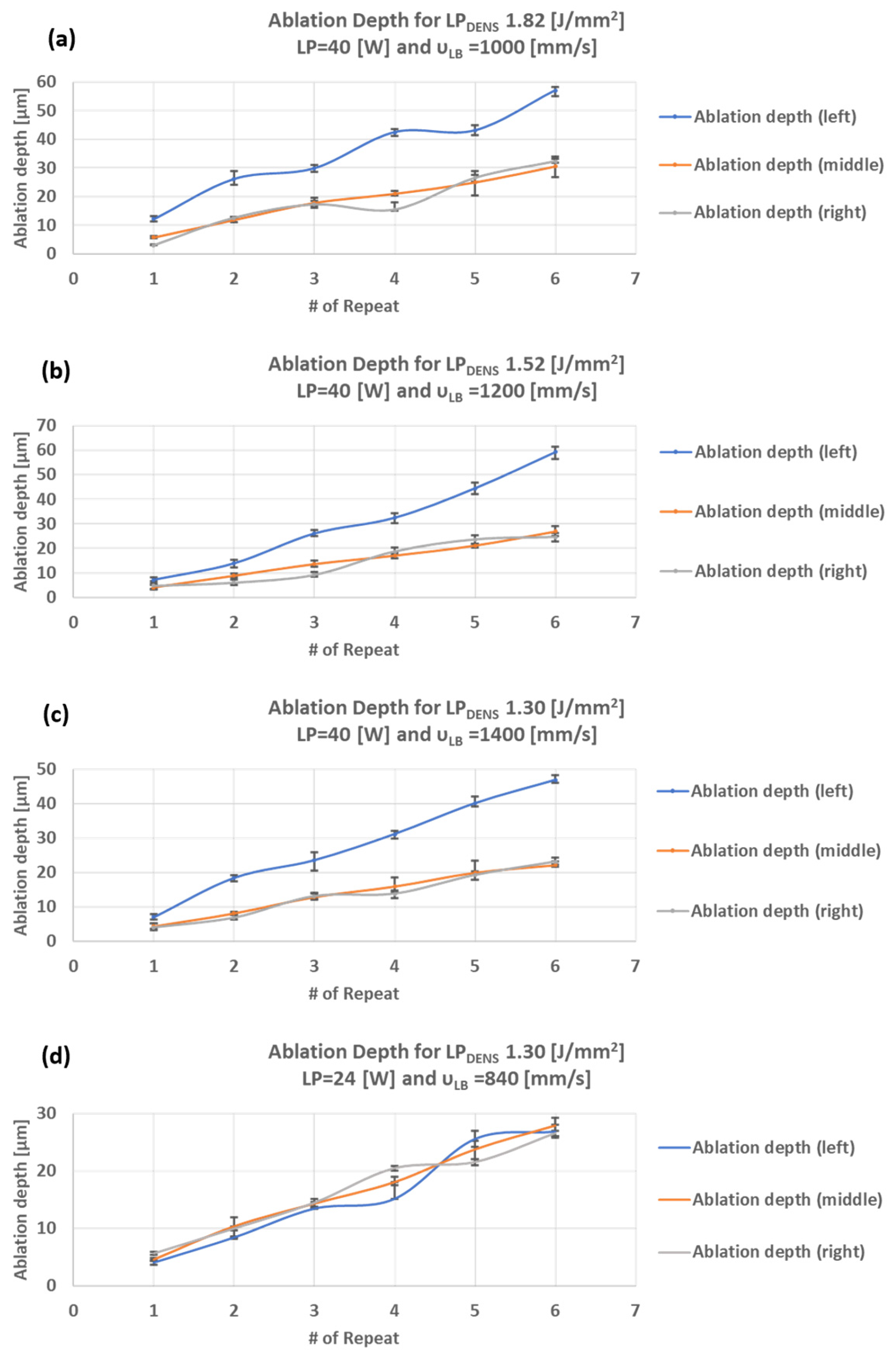
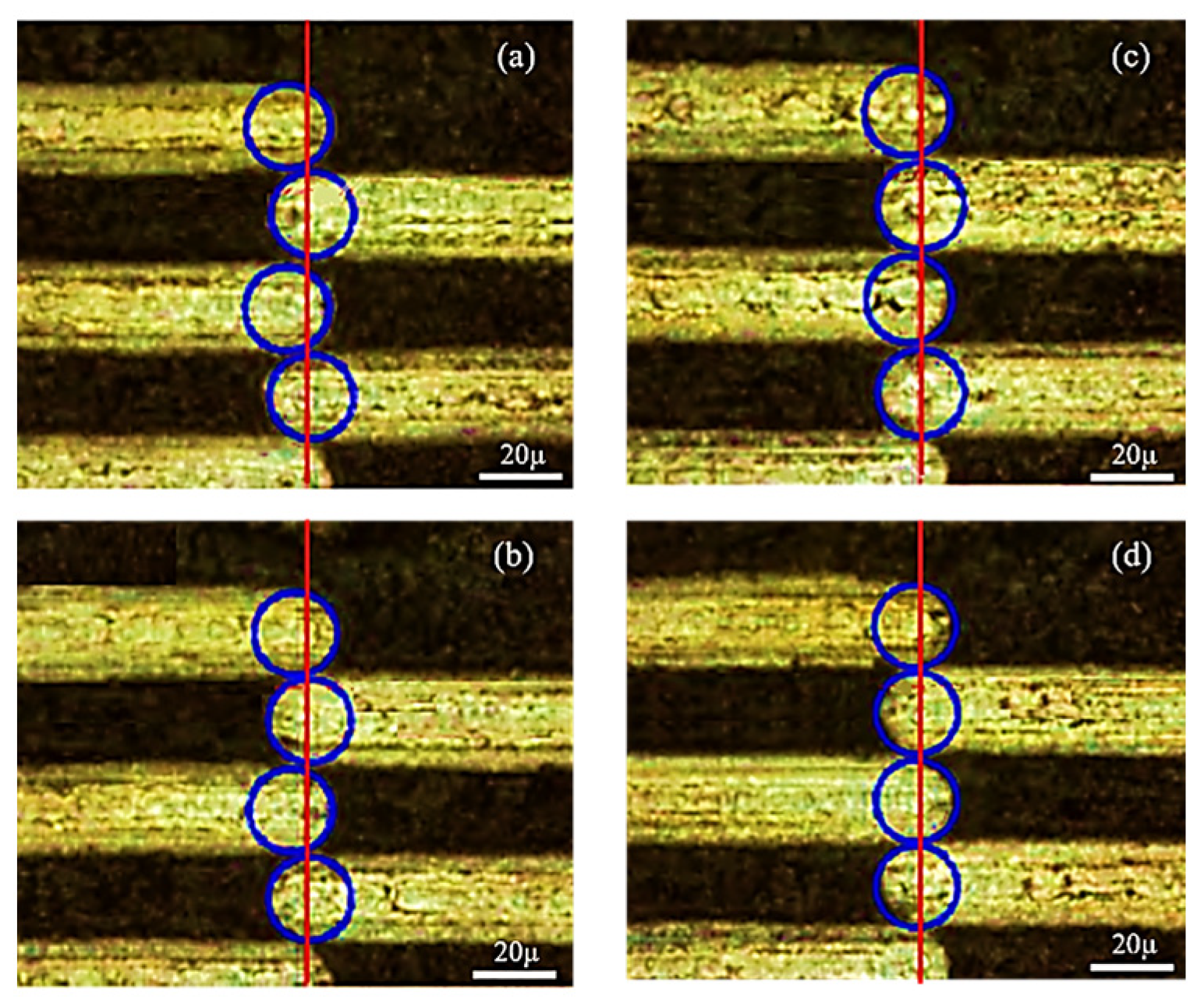
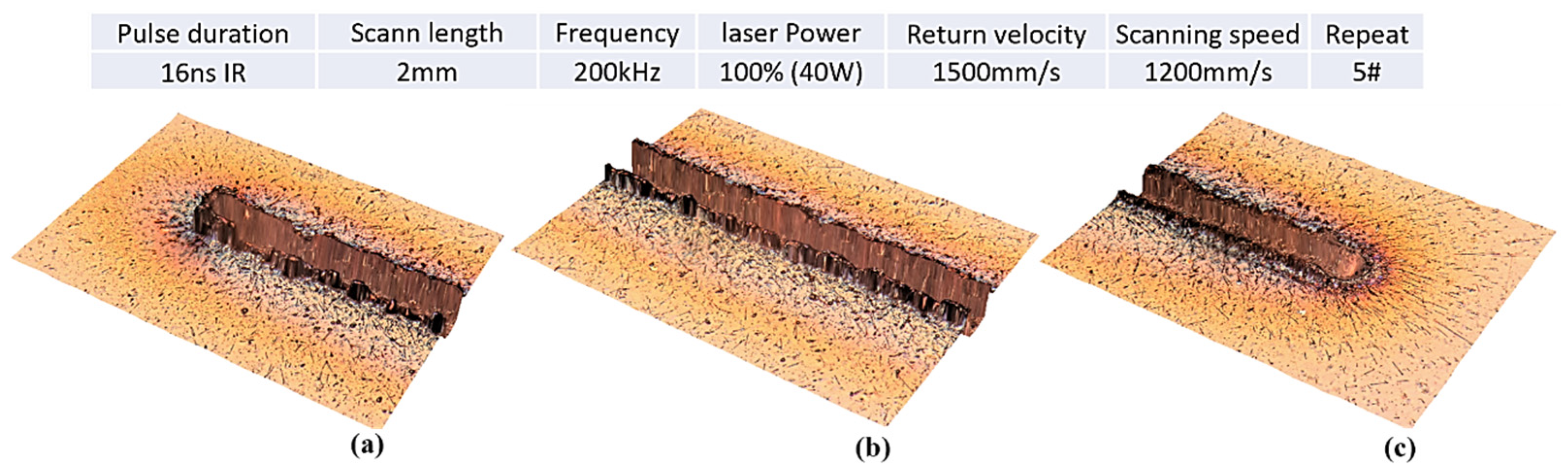
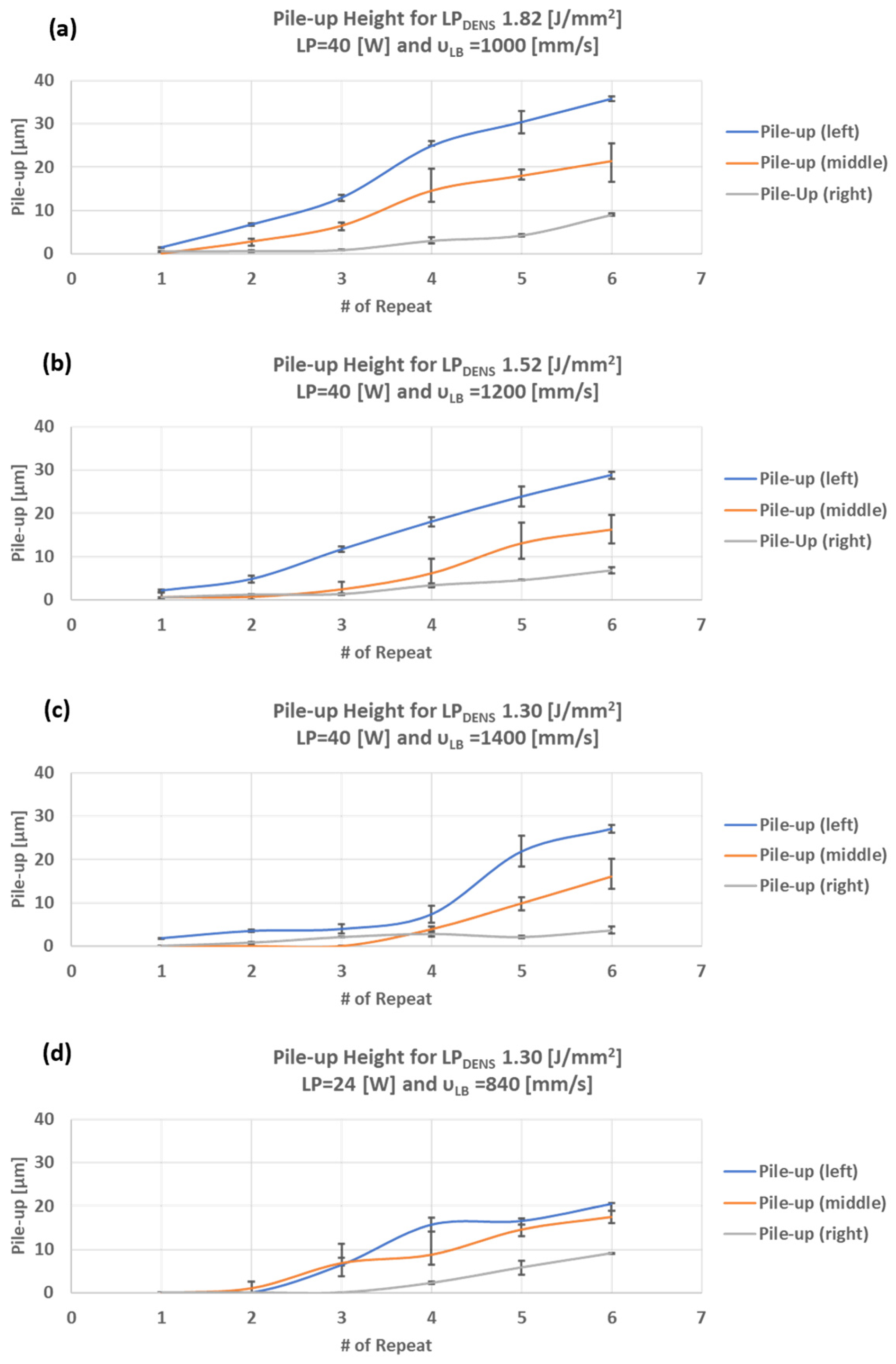
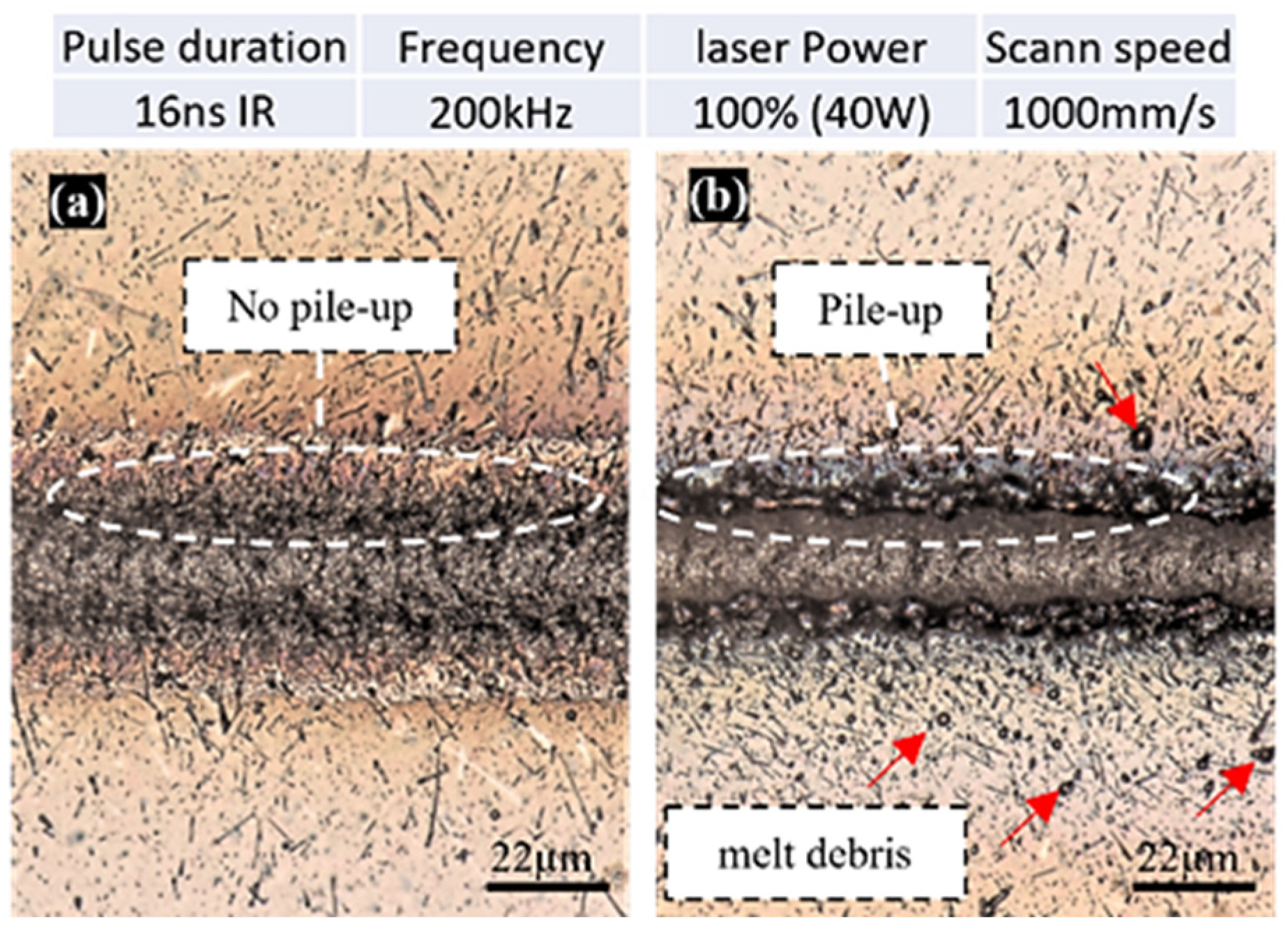
3.1. Experimental Results—Ablation Depth and Pile-Up Formation
3.2. Simulation Results
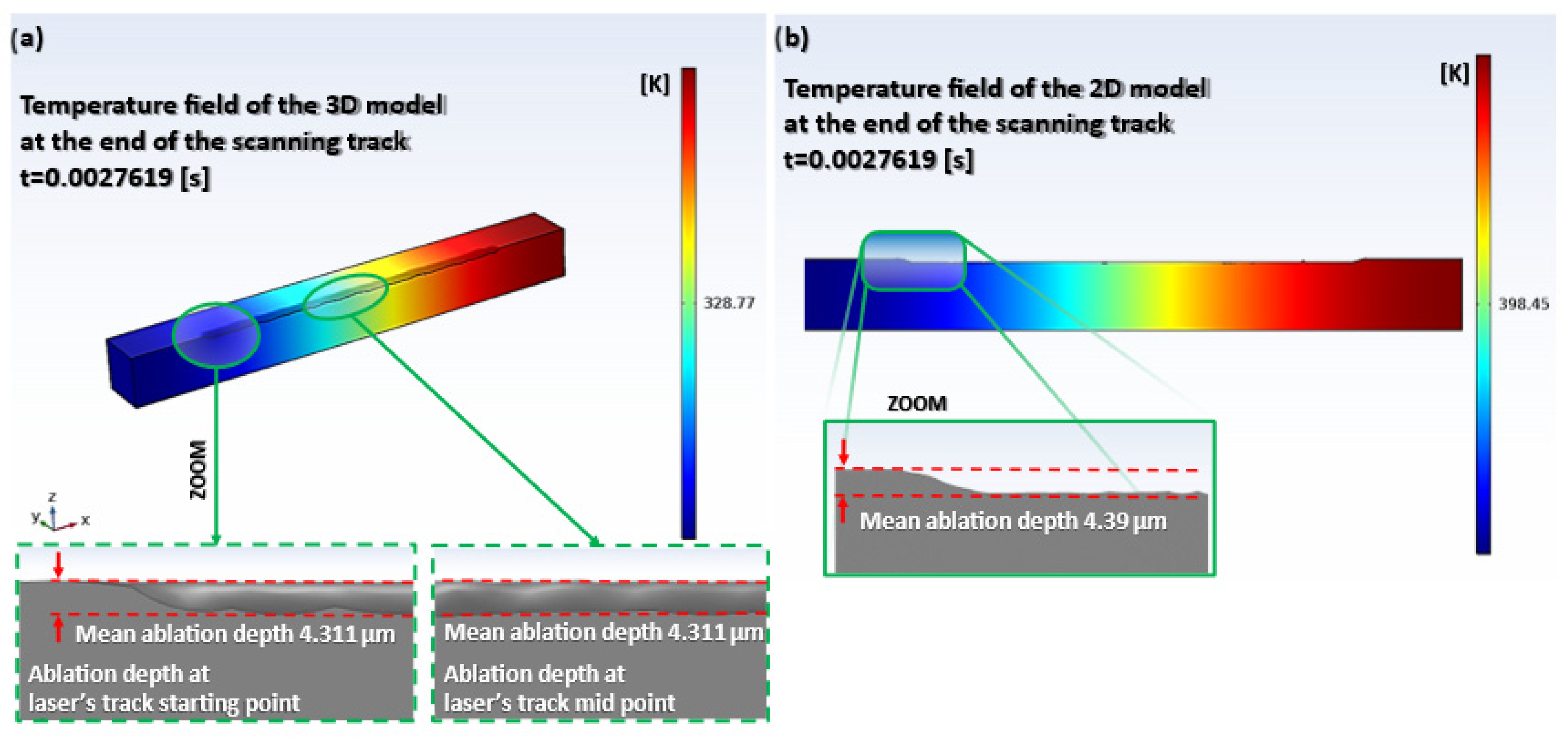
3.2.1. Mean Effective Absorptivity Estimation
3.2.2. Cavity Wall Generation
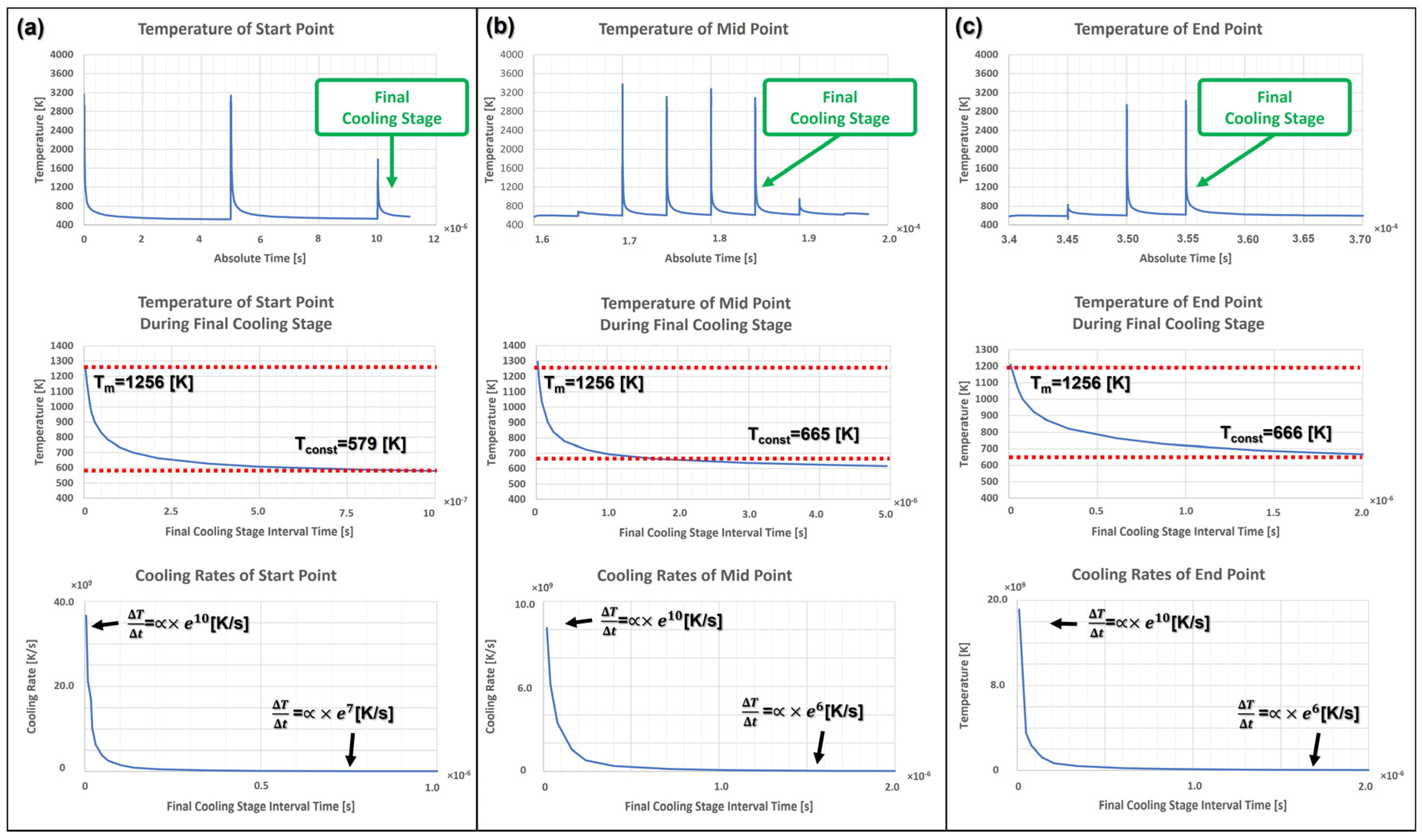
3.2.3. Cooling Rates
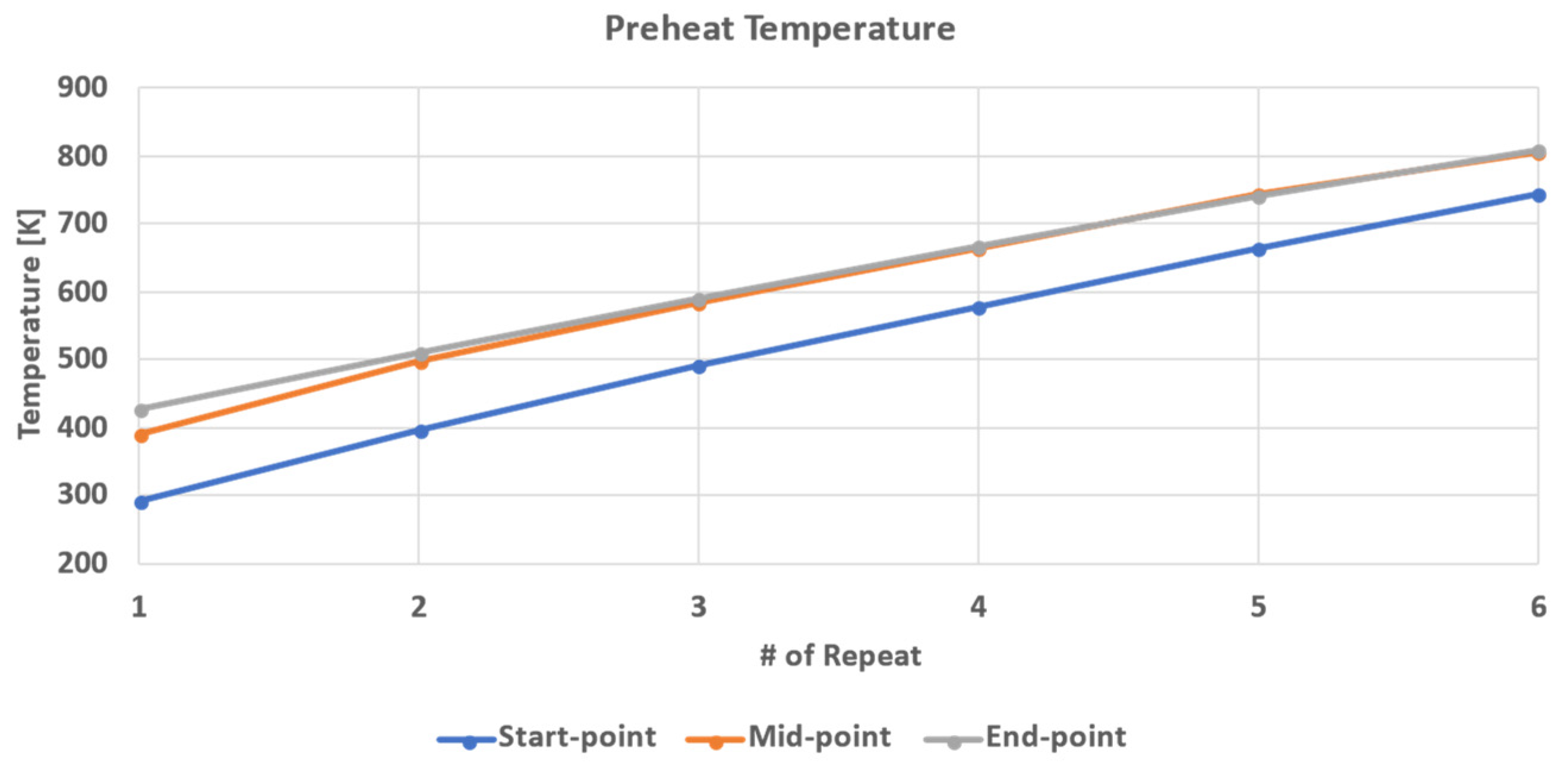
- Start-point, which is the first point irradiated by the laser in each pattern repeat.
- Mid-point, which is the point exactly in the middle of the scanning line.
- End-point, which is the last point irradiated by the laser in each pattern repeat.
- In order to calculate the cooling rates, the following formulation is used [31]:
3.2.4. Preheat Temperature
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Davim, J.P. Nontraditional Machining Processes; Springer: London, UK, 2013. [Google Scholar]
- Lange, D.F.; de Schoonderbeek, A.; Meijer, J. Melt ejection during laser drilling. In International Congress on Applications of Lasers & Electro-Optics; Laser Institute of America: Orlando, FL, USA, 2004; p. 542. [Google Scholar]
- Czotscher, T.; Vollertsen, F. Analysis of Melting and Melt Expulsion during Nanosecond Pulsed Laser Ablation. Phys. Procedia 2016, 83, 53. [Google Scholar] [CrossRef]
- Sportelli, M.C.; Izzi, M.; Volpe, A.; Clemente, M.; Picca, R.A.; Ancona, A.; Lugarà, P.M.; Palazzo, G.; Cioffi, N. The Pros and Cons of the Use of Laser Ablation Synthesis for the Production of Silver Nano-Antimicrobials. Antibiotics 2018, 7, 67. [Google Scholar] [CrossRef]
- Finger, J.; Kalupka, C.; Reininghaus, M. High power ultra-short pulse laser ablation of IN718 using high repetition rates. J. Mater. Process. Technol. 2015, 226, 221. [Google Scholar] [CrossRef]
- Semerok, A.; Chaléard, C.; Detalle, V.; Lacour, J.-L.; Mauchien, P.; Meynadier, P.; Nouvellon, C.; Sallé, B.; Palianov, P.; Perdrix, M. Experimental investigations of laser ablation efficiency of pure metals with femto, pico and nanosecond pulses. Appl. Surf. Sci. 1999, 138–139, 311. [Google Scholar] [CrossRef]
- Schulz, W.; Eppelt, U.; Poprawe, R. Review on laser drilling I. Fundamentals, modeling, and simulation. J. Laser Appl. 2013, 25, 012006. [Google Scholar] [CrossRef]
- Hribar, L.; Gregorčič, P.; Senegačnik, M.; Jezeršek, M. The Influence of the Processing Parameters on the Laser-Ablation of Stainless Steel and Brass during the Engraving by Nanosecond Fiber Laser. Nanomaterials 2022, 12, 232. [Google Scholar] [CrossRef] [PubMed]
- Carr, C.W.; Radousky, H.B.; Demos, S.G. Wavelength dependence of laser-induced damage: Determining the damage initiation mechanisms. Phys. Rev. Lett. 2003, 91, 127402. [Google Scholar] [CrossRef]
- Jee, Y.; Becker, M.F.; Walser, R.M. Laser-induced damage on single-crystal metal surfaces. J. Opt. Soc. Am. B 1988, 5, 648–659. [Google Scholar] [CrossRef]
- Natoli, J.-Y.; Gallais, L.; Akhouayri, H.; Amra, C. Laser-induced damage of materials in bulk, thin-film, and liquid forms. Appl. Opt. 2002, 41, 3156–3166. [Google Scholar] [CrossRef]
- Demos, S.G.; Staggs, M.; Minoshima, K.; Fujimoto, J. Characterization of laser induced damage sites in optical components. Opt. Express 2002, 10, 1444–1450. [Google Scholar] [CrossRef]
- Hopp, B.; Smausz, T.; Lentner, M.; Kopniczky, J.; Tápai, C.; Gera, T.; Csizmadia, T.; Ehrhardt, M.; Lorenz, P.; Zimmer, K. Stability investigation of laser darkened metal surfaces. Appl. Phys. A 2017, 123, 598. [Google Scholar] [CrossRef]
- Bashir, S.; Vaheed, H.; Mahmood, K. Nanosecond pulsed laser ablation of brass in a dry and liquid-confined environment. Appl. Phys. A 2013, 110, 389–395. [Google Scholar] [CrossRef]
- Vorobyev, A.Y.; Guo, C. Thermal response and optical absorptance of metals under femtosecond laser irradiation. Nat. Sci. 2011, 3, 488–495. [Google Scholar] [CrossRef][Green Version]
- Bashir, S.; Rafique, M.S.; Husinsky, W. Identification of non-thermal and thermal processes in femtosecond laser-ablated aluminum. Radiat. Eff. Defects Solids 2013, 168, 902–911. [Google Scholar] [CrossRef]
- Maisterrena-Epstein, R.; Camacho-López, S. Nanosecond laser ablation of bulk Al, Bronze, and Cu: Ablation rate saturation and laserinduced oxidation. Superf. Y Vacío 2007, 20, 1–5. [Google Scholar]
- Bordag, M. Drude model and Lifshitz formula. Eur. Phys. J. C 2011, 71, 1788. [Google Scholar] [CrossRef]
- Lucarini, V.; Saarinen, J.J.; Peiponen, K.E.; Vartiainen, E.M. Kramers-Kronig Relations in Optical Materials Research; Springer: Berlin/Heidelberg, Germany, 2005. [Google Scholar]
- Johnson, P.B.; Christy, R.W. Optical Constants of the Noble Metals. Phys. Rev. B 1972, 6, 4370. [Google Scholar] [CrossRef]
- Bunaziv, I.; Akselsen, O.M.; Ren, X.; Nyhus, B.; Eriksson, M. Laser Beam and Laser-Arc Hybrid Welding of Aluminium Alloys. Metals 2021, 11, 1150. [Google Scholar] [CrossRef]
- Holman, J.P. Heat Transfer, 10th ed.; McGraw-Hill Higher Education: Boston, MA, USA, 2010. [Google Scholar]
- Alexopoulou, V.E.; Papazoglou, E.L.; Karmiris-Obratański, P.; Markopoulos, A.P. 3D finite element modeling of selective laser melting for conduction, transition and keyhole modes. J. Manuf. Process. 2022, 75, 877–894. [Google Scholar] [CrossRef]
- Mills, K.C. Recommended Values of Thermophysical Properties for Selected Commercial Alloys; Woodhead Publishing Limited: Cambridge, UK, 2002. [Google Scholar]
- Sharafat, S.; Ghoniem, N. Summary of Thermo-Physical Properties of Sn, and Compounds of Sn–H. Sn–O, Sn–C, Sn–Li, and Sn–Si and Comparison of Properties of Sn, Sn–Li, and Pb–Li. PhD. Thesis, University of California Los Angeles, Los Angeles, CA, USA, 2000. [Google Scholar]
- Shaikh, S.M.; Hariharan, V.S.; Yadav, S.K.; Murty, B.S. CALPHAD and rule-of-mixtures: A comparative study for refractory high entropy alloys. Intermetallics 2020, 127, 106926. [Google Scholar] [CrossRef]
- Lalancette, F.; Désilets, M.; Pansiot, B.; LeBreux, M.; Bilodeau, J.-F. Dimensional reduction of a 3D thermoelectric model to create a reliable and time-efficient 2D model representing an aluminum electrolysis cell. Int. J. Heat Mass Transf. 2023, 202, 123777. [Google Scholar] [CrossRef]
- Tang, M.; Shi, Y.; Zhu, W.; Zhang, N.; Zhang, L. A Convenient and High-Efficient Laser Micro-Engraving Treatment for Controllable Preparation of Microstructure on Al Alloy. Materials 2018, 11, 2297. [Google Scholar] [CrossRef] [PubMed]
- Wang, L.; Zhang, Y.; Chia, H.Y.; Yan, W. Mechanism of keyhole pore formation in metal additive manufacturing. Npj Comput. Mater. 2022, 8, 22. [Google Scholar] [CrossRef]
- Meng, C.; Lu, F.-G.; Cui, H.-C.; Tang, X.-H. Research on formation and stability of keyhole in stationary laser welding on aluminum MMCs reinforced with particles. Int. J. Adv. Manuf. Technol. 2013, 67, 2917–2925. [Google Scholar] [CrossRef]
- O’Sullivan, C.T. Newton’s law of cooling—A critical assessment Am. J. Phys. 1990, 58, 956. [Google Scholar]
- Diez, M.; Ametowobla, M.; Graf, T. Time-Resolved Reflectivity and Temperature Measurements During Laser Irradiation of Crystalline Silicon. J. Laser Micro/Nanoeng. 2017, 12, 230–234. [Google Scholar]


| Parameters | Values |
|---|---|
| Average laser power | 40 [W] |
| Laser pulse duration | 16 [ns] |
| Laser frequency | 200 [kHz] |
| Laser beam diameter | 22 [μm] |
| Laser scanning speed | 1000–1200–1400 [mm/s] |
| Laser wavelength | 1064 [nm] |
| Laser beam distribution | Gaussian |
| Sequence | Polishing Pad | Fluid | Rotational Speed [U/min] | Rotation Direction | Axial Force [N] | Polishing Time [min] |
|---|---|---|---|---|---|---|
| Pre-Grinding | SiC-Paper P320 | H2O | 250–300 | >>Up | 30 | 0.5 |
| Polishing | Beta | Diamond suspension, 9 [µm] | 120–150 | >>Up | 30 | 3–4 |
| Polishing | Sigma | Diamond suspension, 3 [µm] | 120–150 | >>Up | 30 | 1–2 |
| Final polishing | Omega | Eposil F 0.1 [µm] * | 120–150 | > <Down | 15 | 1–2 |
| Property | Temperature Range [K] | Expression/Value |
|---|---|---|
| Density [kg/m3] | 273–1313 1313–1350 1350–2857 | 7262 − 0.486 (T − 298) 6768.71 − 9.3 (T − 1313) 6425 − 0.65 (T − 1350) |
| Heat Capacity [J/kgK] | 273–1313 1313–1323 | 353 + 0.3T − 10−4T2 0.75 (T − 1313) + 574.5 |
| Thermal Conductivity [W/mK] | 273–773 773–1313 1313–1373 | 7.925 + 0.1375T 78.36 − 0.067 (T − 773) 42 − 0.25 (T − 1313) |
| Latent Heat of Sublimation [KJ/kg] | --- | 5797 |
| Ablation Temperature [K] | --- | 2857 |
| Emissivity Coefficient | --- | 0.023 |
| Ablation Depth in [μm] | ||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| # | LPDENS = 1.82 [J/mm2] | LPDENS = 1.52 [J/mm2] | LPDENS = 1.3 [J/mm2] | LPDENS = 1.3 [J/mm2] (24 W) | ||||||||
| Left | Middle | Right | Left | Middle | Right | Left | Middle | Right | Left | Middle | Right | |
| 1 | 12.11 | 5.77 | 2.99 | 7.13 | 4.05 | 4.78 | 7.00 | 4.31 | 3.96 | 4.00 | 4.59 | 5.63 |
| 2 | 26.13 | 11.84 | 12.55 | 13.86 | 8.82 | 6.04 | 18.41 | 8.10 | 6.77 | 8.40 | 10.37 | 9.96 |
| 3 | 29.87 | 17.80 | 17.18 | 26.06 | 13.59 | 9.22 | 23.55 | 12.85 | 13.03 | 13.43 | 14.33 | 14.44 |
| 4 | 42.42 | 20.96 | 15.43 | 32.48 | 17.07 | 18.75 | 31.15 | 15.94 | 13.76 | 15.15 | 18.08 | 20.44 |
| 5 | 43.05 | 24.93 | 26.47 | 44.55 | 21.16 | 23.66 | 40.08 | 19.95 | 19.19 | 25.59 | 23.76 | 21.50 |
| 6 | 56.99 | 30.47 | 32.24 | 59.32 | 26.95 | 24.62 | 46.84 | 22.14 | 23.07 | 26.88 | 27.91 | 26.50 |
| Pile-Up in [μm] | ||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| # | LPDENS = 1.82 [J/mm2] | LPDENS = 1.52 [J/mm2] | LPDENS = 1.3 [J/mm2] | LPDENS = 1.3 [J/mm2] (24 W) | ||||||||
| Left | Middle | Right | Left | Middle | Right | Left | Middle | Right | Left | Middle | Right | |
| 1 | 1.40 | 0.00 | 0.58 | 2.15 | 0.75 | 0.53 | 1.83 | 0.00 | 0.00 | 0.00 | 0.00 | 0.00 |
| 2 | 6.70 | 2.78 | 0.60 | 4.80 | 0.79 | 1.18 | 3.51 | 0.00 | 0.77 | 0.00 | 1.07 | 0.00 |
| 3 | 12.90 | 6.43 | 0.85 | 11.65 | 2.50 | 1.30 | 3.96 | 0.00 | 2.10 | 6.41 | 6.86 | 0.00 |
| 4 | 24.87 | 14.54 | 3.00 | 18.05 | 6.16 | 3.35 | 7.36 | 3.91 | 2.83 | 15.75 | 8.79 | 2.21 |
| 5 | 30.40 | 17.99 | 4.25 | 23.84 | 13.02 | 4.55 | 21.88 | 9.90 | 2.11 | 16.60 | 14.58 | 5.78 |
| 6 | 35.80 | 21.39 | 9.00 | 28.80 | 16.14 | 6.85 | 27.11 | 16.10 | 3.67 | 20.56 | 17.46 | 9.03 |
| # | Mean Effective Absorption Coefficient | Experimental Ablation Depth [μm] | Simulation Ablation Depth [μm] | Error |
|---|---|---|---|---|
| 1 | 0.090 | 4.358 | 4.390 | 0.7% |
| 2 | 0.090 | 8.438 | 8.553 | 1.4% |
| 3 | 0.090 | 12.750 | 12.475 | −2.2% |
| 4 | 0.090 | 15.800 | 15.938 | 0.9% |
| 5 | 0.090 | 20.125 | 20.079 | −0.2% |
| 6 | 0.045 | 21.820 | 21.723 | −0.4% |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ghadiri Zahrani, E.; Alexopoulou, V.E.; Papazoglou, E.L.; Azarhoushang, B.; Markopoulos, A. An Experimental and Numerical Study of the Laser Ablation of Bronze. Machines 2024, 12, 63. https://doi.org/10.3390/machines12010063
Ghadiri Zahrani E, Alexopoulou VE, Papazoglou EL, Azarhoushang B, Markopoulos A. An Experimental and Numerical Study of the Laser Ablation of Bronze. Machines. 2024; 12(1):63. https://doi.org/10.3390/machines12010063
Chicago/Turabian StyleGhadiri Zahrani, Esmaeil, Vasiliki E. Alexopoulou, Emmanouil L. Papazoglou, Bahman Azarhoushang, and Angelos Markopoulos. 2024. "An Experimental and Numerical Study of the Laser Ablation of Bronze" Machines 12, no. 1: 63. https://doi.org/10.3390/machines12010063
APA StyleGhadiri Zahrani, E., Alexopoulou, V. E., Papazoglou, E. L., Azarhoushang, B., & Markopoulos, A. (2024). An Experimental and Numerical Study of the Laser Ablation of Bronze. Machines, 12(1), 63. https://doi.org/10.3390/machines12010063








