Topology and Size Optimized Design and Laser Welding of the U-Frame for Free-Space Laser Communication Telescopes
Abstract
:1. Introduction
2. Finite Element Analysis of the Original Structure
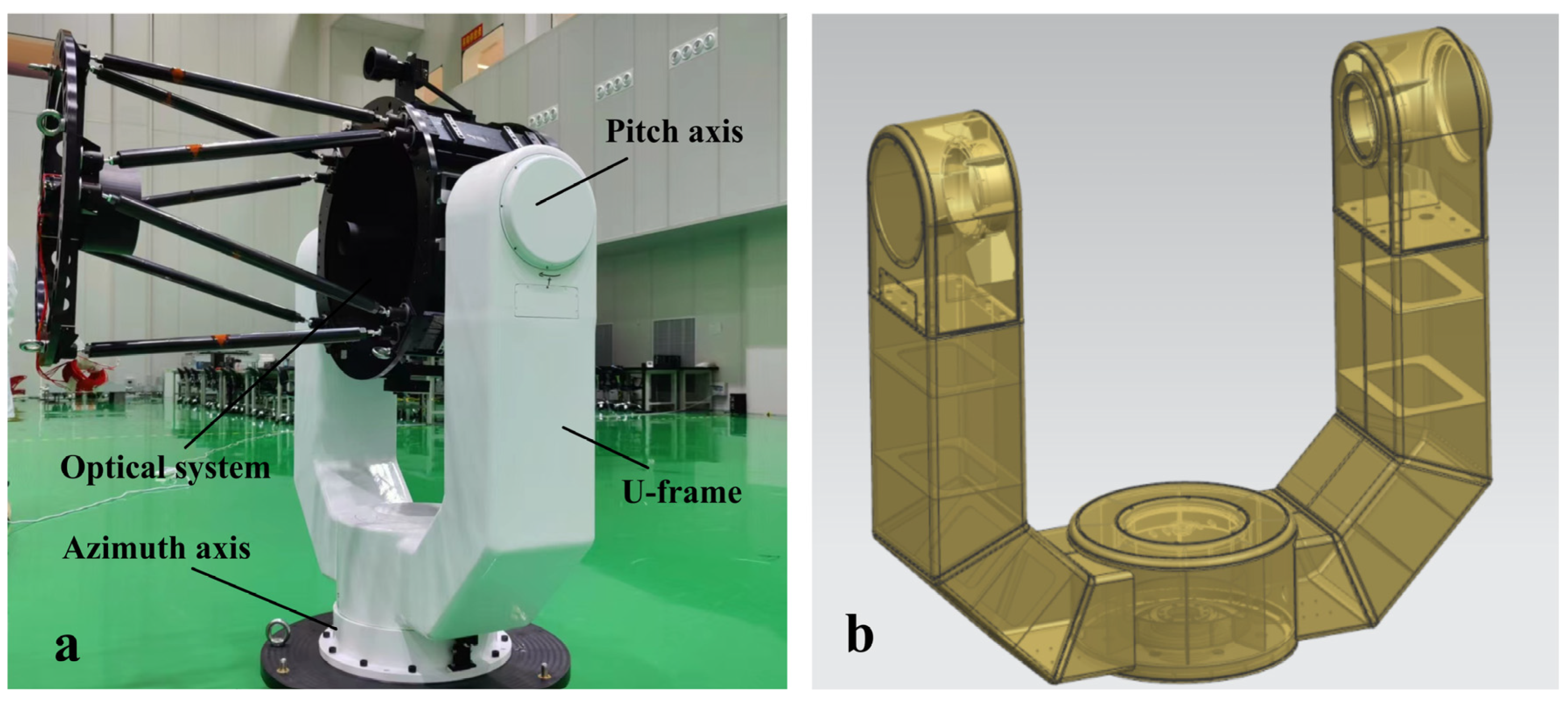
2.1. Introduction of U-Frame
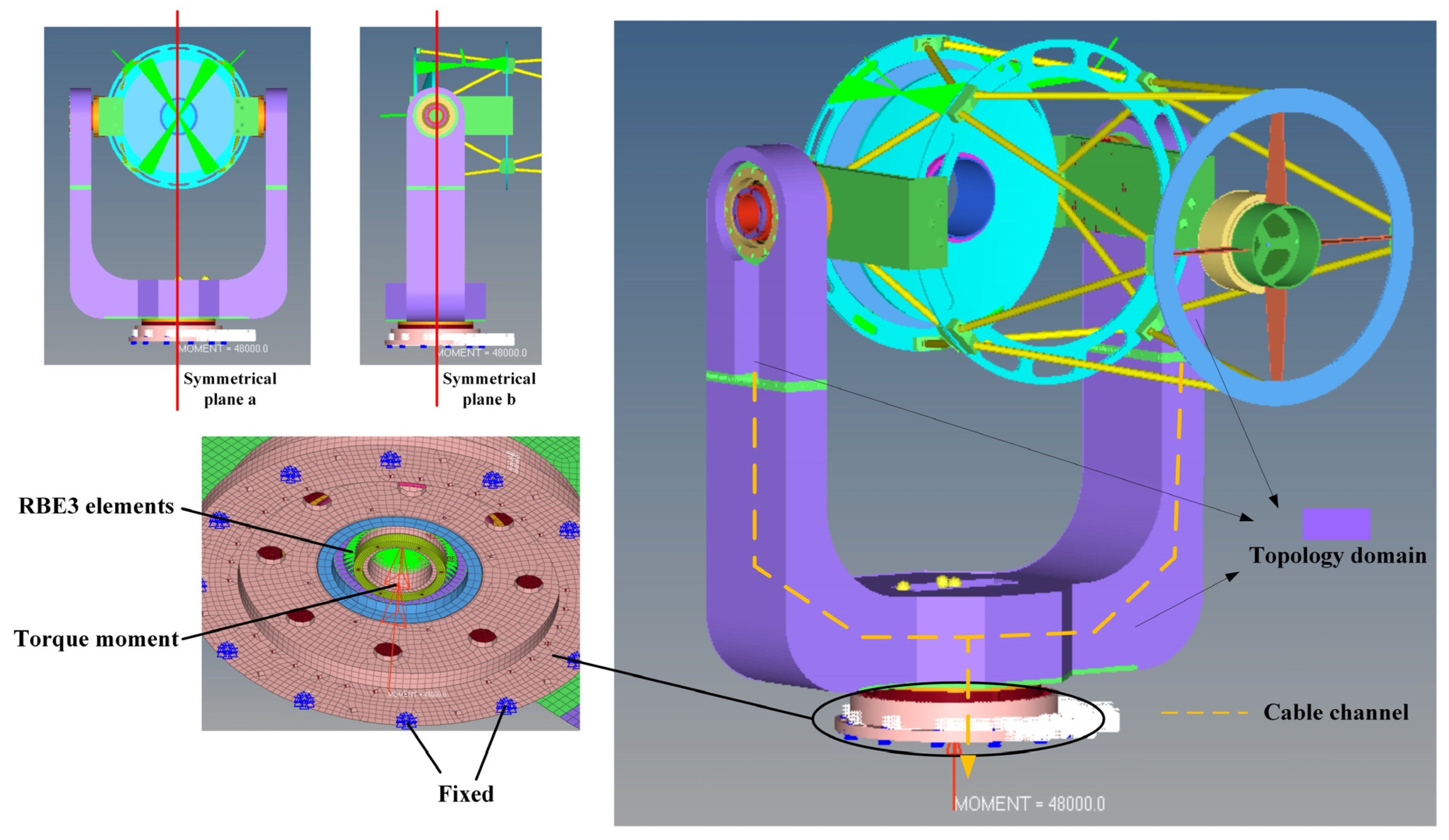
2.2. Working Condition Analysis
- (1)
- When stationary, the entire system is primarily influenced by gravity in the vertical direction.
- (2)
- When the telescope is subject to other disturbances or fixed in transit on a vehicle, the other directions are affected by 0.2 times the force of gravity due to the slope of the road.
- (3)
- When the telescope is tracking, the motor provides a maximum rotation torque of approximately 50 Nm to the entire system.
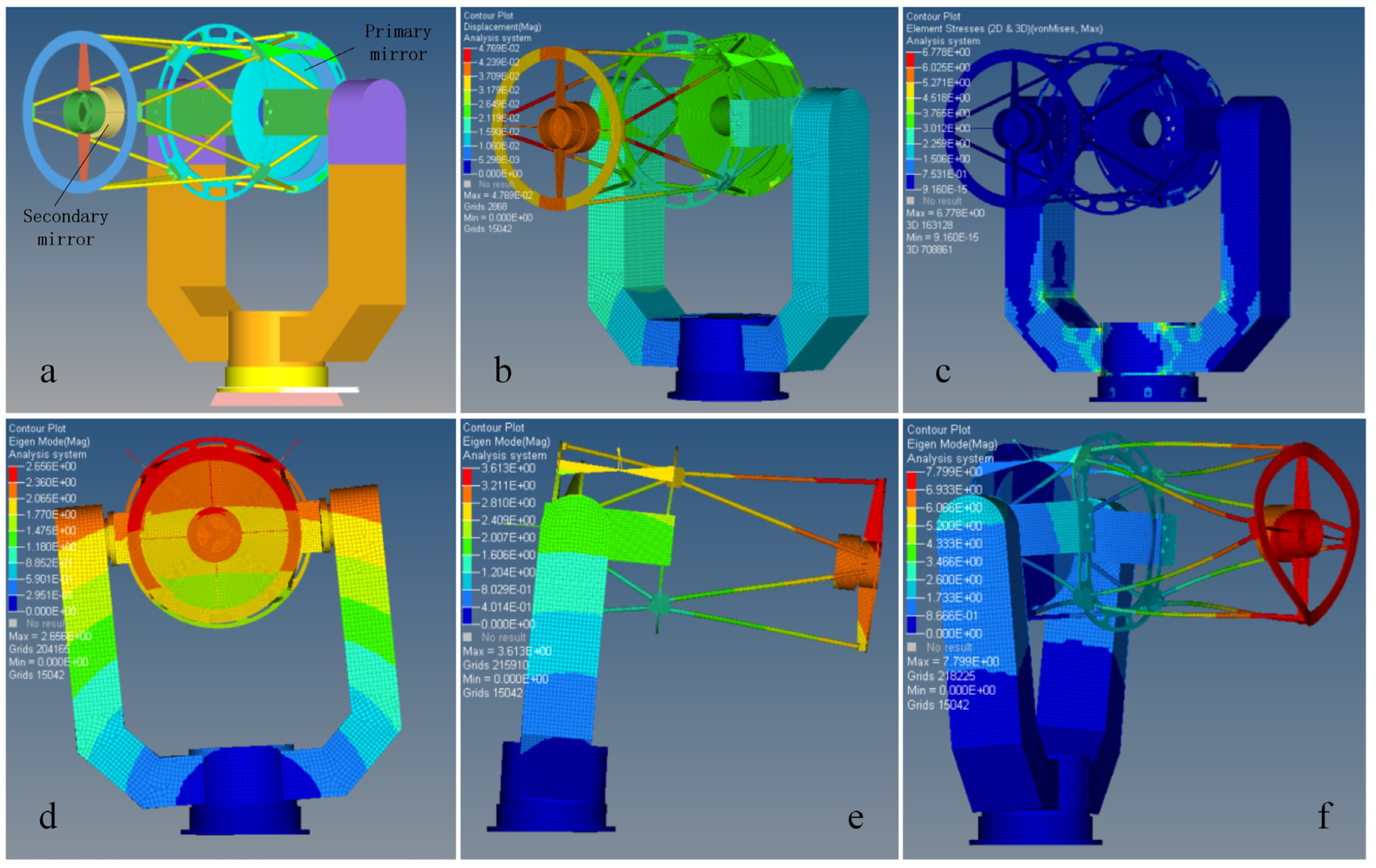
2.3. Finite Element Analysis of the Original Structure
3. Topology and Size Optimization
3.1. Topology Optimization
3.1.1. Mathematical Model
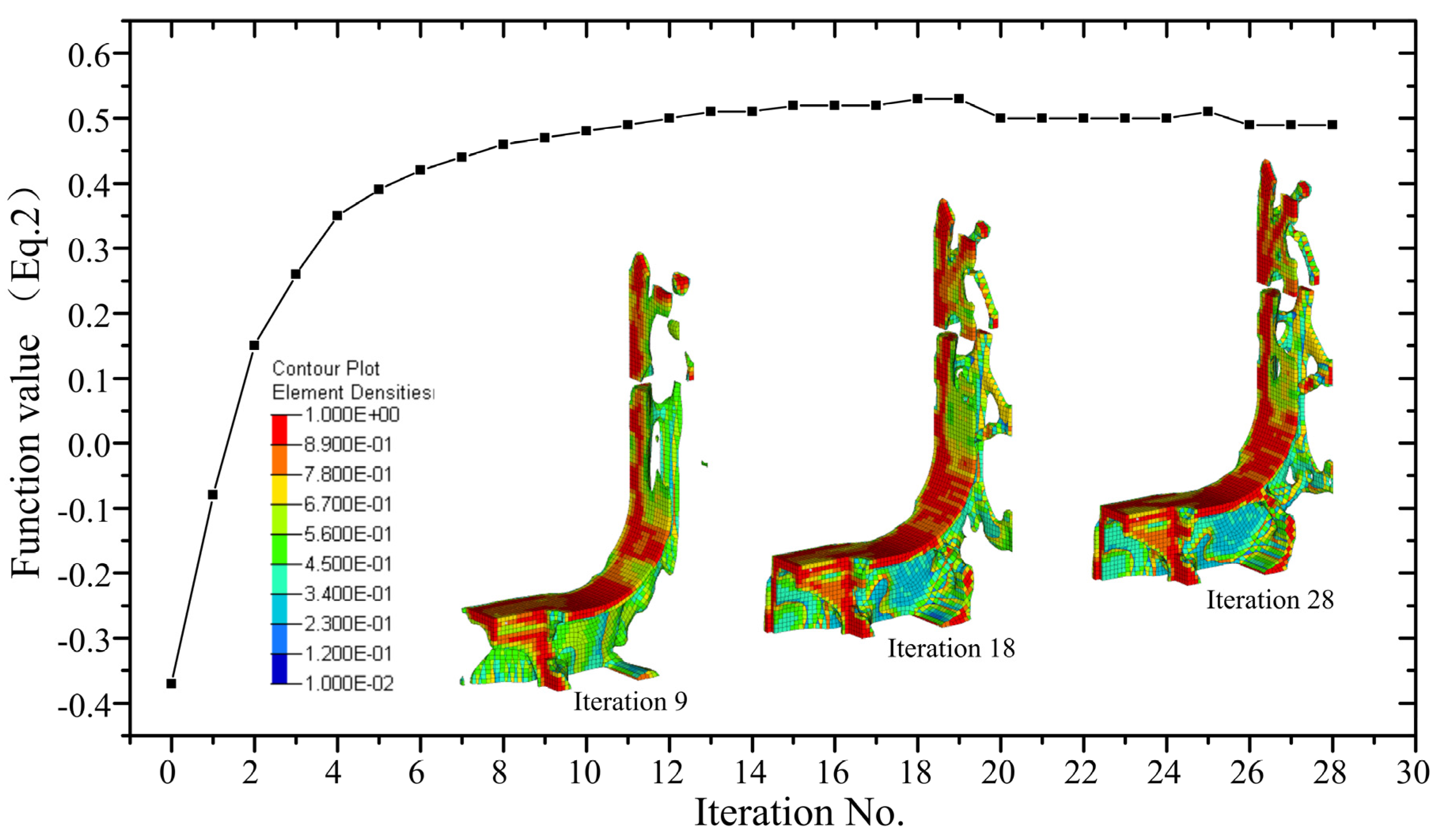
3.1.2. Topology Optimization Process
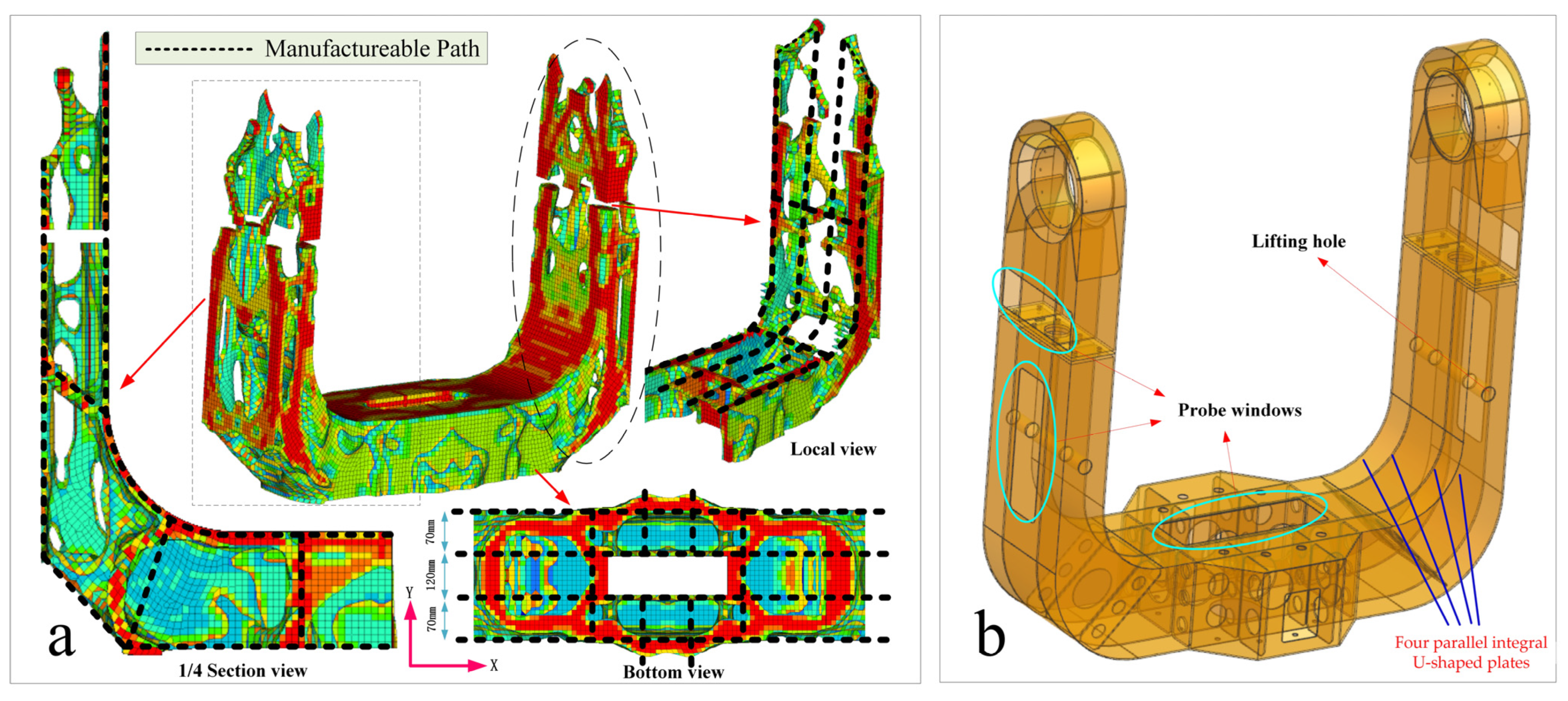
3.1.3. Reconstruction of the Optimized Structure
3.2. Laser Welding Forming Process
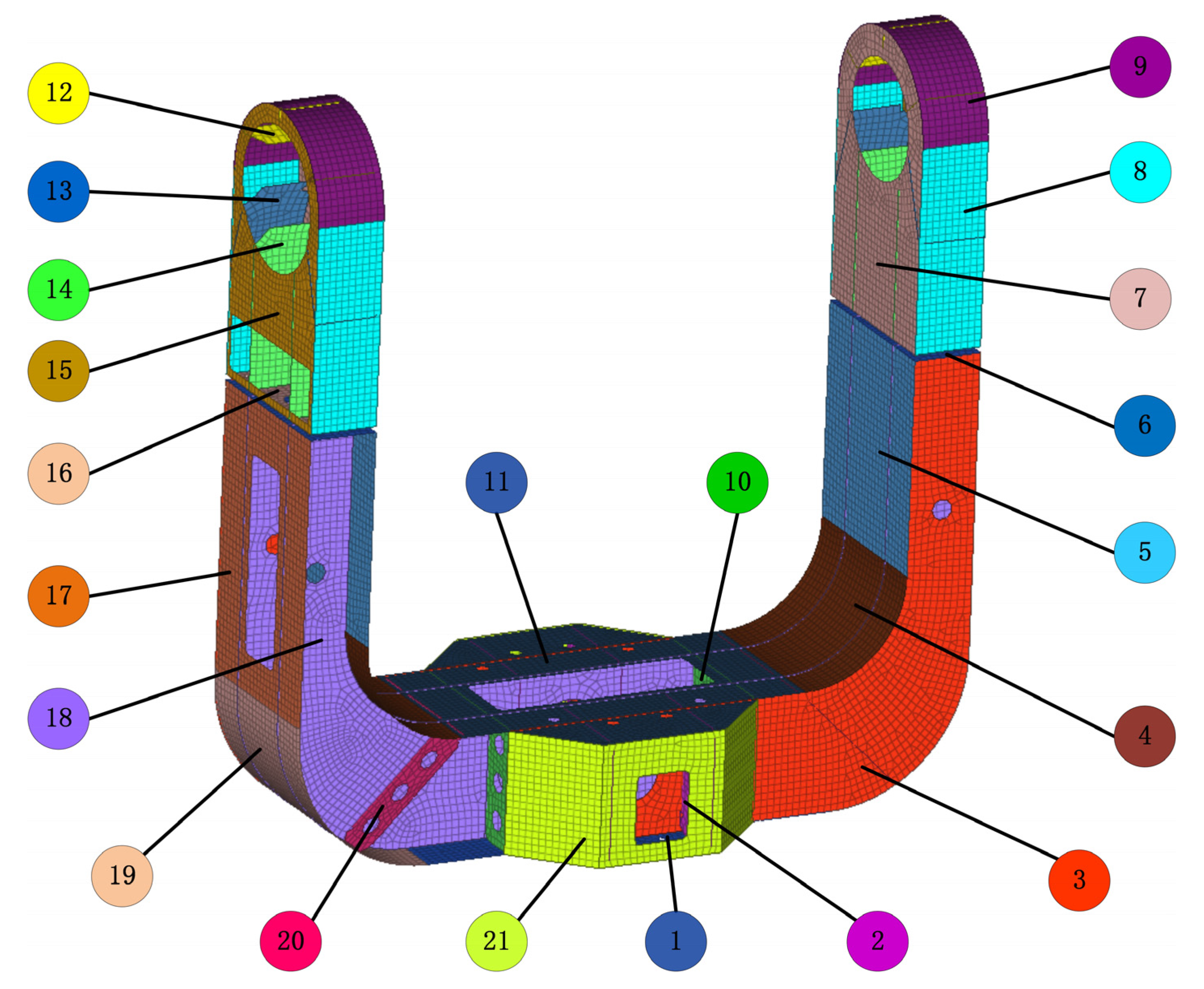
3.3. Size Optimization
3.3.1. Mathematical Model
3.3.2. Size Optimization Results
4. Experimental Validation
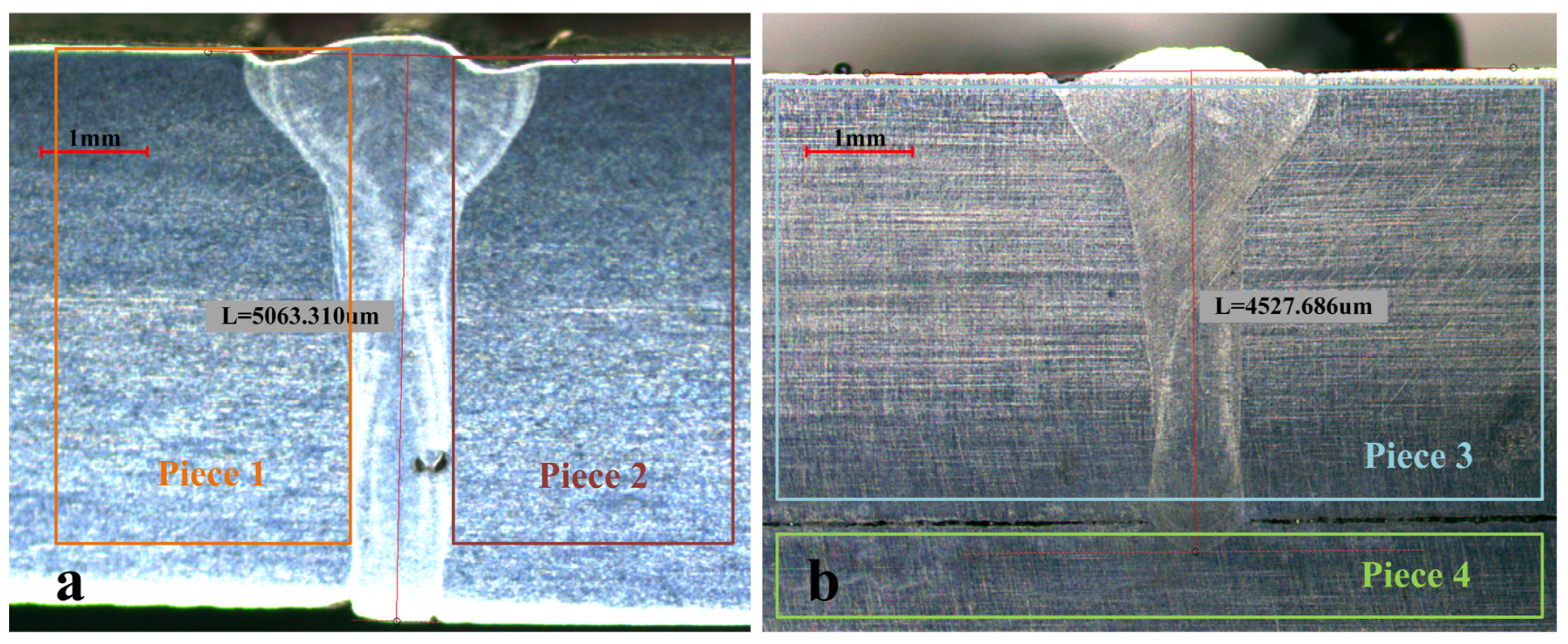
4.1. Tensile Experiment of Laser Welding
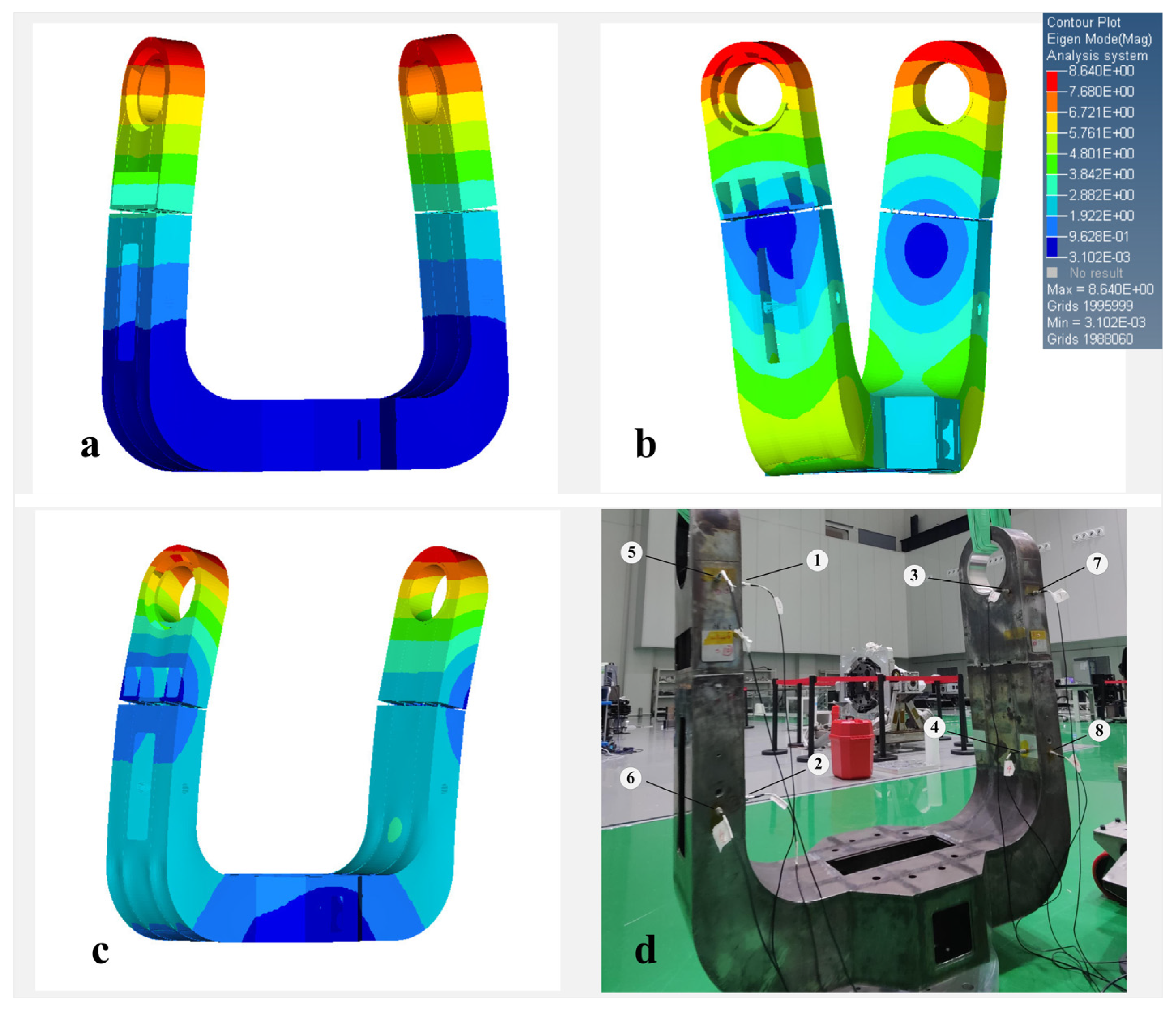
4.2. Free Modal Experiments of the Prototype
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Medina, I.; Hernández-Gómez, J.J.; Torres-San Miguel, C.R.; Santiago, L.; Couder-Castañeda, C. Prototype of a Computer Vision-Based CubeSat Detection System for Laser Communications. Int. J. Aeronaut. Space Sci. 2021, 22, 717–725. [Google Scholar] [CrossRef]
- Fischer, E.; Kudielka, K.; Berkefeld, T.; Soltau, D.; Perdigués-Armengol, J.; Sodnik, Z. Adaptive optics upgrades for laser communications to the ESA optical ground station. In Proceedings of the International Conference on Space Optics-ICSO 2020, Online, 30 March–2 April 2021; SPIE: Bellingham, WA, USA, 2021; Volume 118522, pp. 971–980. [Google Scholar] [CrossRef]
- McManamon, P.; Vedadi, A.; Willner, A.E.; Choudhary, D.; Montifiore, N.; Harlev, O. High capacity and access rate, data storage using laser communications. Opt. Eng. 2021, 60, 015105. [Google Scholar] [CrossRef]
- Israel, D.J.; Edwards, B.L.; Butler, R.L.; Moores, J.D.; Piazzolla, S.; du Toit, N.; Braatz, L. Early results from NASA’s laser communications relay demonstration (LCRD) experiment program. Free.-Space Laser Commun. 2023, 12413, 10–24. [Google Scholar] [CrossRef]
- Hu, Z.; Li, S.; Shao, M.; An, Z. Thermal integration analysis of optical machines for space-based laser communications. In Proceedings of the International Conference on Precision Instruments and Optical Engineering, Shanghai, China, 25–27 August 2023; SPIE: Bellingham, WA, USA, 2023; Volume 12585, pp. 7–16. [Google Scholar] [CrossRef]
- Wang, J.P.; Bilyeu, B.; Boroson, D.; Caplan, D.; Robinson, B.; Schieler, C.; Haworth, K. High-rate 256+ Gbit/s laser communications for enhanced high-resolution imaging using space-based very long baseline interferometry (VLBI). Free.-Space Laser Commun. 2023, 12413, 54–65. [Google Scholar] [CrossRef]
- Schieler, C.M.; Riesing, K.M.; Bilyeu, B.C.; Chang, J.S.; Garg, A.S.; Gilbert, N.J.; Keer, B. On-orbit demonstration of 200-Gbps laser communication downlink from the TBIRD CubeSat. Free.-Space Laser Commun. 2023, 12413, 1241302. [Google Scholar] [CrossRef]
- Kim, W.H. BER Analysis of IM and BPPM for Satellite-to-Ground Laser Communications. In Proceedings of the 2020 International Conference on Electronics, Information, and Communication (ICEIC), Barcelona, Spain, 19–22 January 2020; IEEE: New York, NY, USA, 2020. [Google Scholar] [CrossRef]
- Toyoshima, M. Recent Trends in Space Laser Communications for Small Satellites and Constellations. J. Light. Technol. 2020, 39, 693–699. [Google Scholar] [CrossRef]
- Huang, B.; Li, Z.; Tian, X.; Yang, L.; Han, C.; Chen, B. Concurrent topology and fiber orientation optimization of CFRP structures in space-borne optical remote sensor. Optik 2022, 267, 169652. [Google Scholar] [CrossRef]
- Jung, Y.; Lim, S.; Kim, J.; Min, S. Lightweight design of electric bus roof structure using multi-material topology optimisation. Struct. Multidiscip. Optim. 2020, 61, 1273–1285. [Google Scholar] [CrossRef]
- Lieu, Q.X. A novel topology framework for simultaneous topology, size and shape optimization of trusses under static, free vibration and transient behavior. Eng. Comput. 2022, 38, 1–25. [Google Scholar] [CrossRef]
- Zargham, S.; Ward, T.A.; Ramli, R.; Badruddin, I.A. Topology optimization: A review for structural designs under vibration problems. Struct. Multidiscip. Optim. 2016, 53, 1157–1177. [Google Scholar] [CrossRef]
- Lim, J.; You, C.; Dayyani, I. Multi-objective topology optimization and structural analysis of periodic spaceframe structures. Mater. Des. 2020, 190, 108552. [Google Scholar] [CrossRef]
- Song, J.; Zhang, Y.; Guo, X.; Gao, H.; Wen, W.; Cui, H. Topology and shape optimization of twin-web turbine disk. Struct. Multidiscip. Optim. 2022, 65, 44. [Google Scholar] [CrossRef]
- Qu, Y.; Jiang, Y.; Feng, L.; Li, X.; Liu, B.; Wang, W. Lightweight design of multi-objective topology for a large-aperture space mirror. Appl. Sci. 2018, 8, 2259. [Google Scholar] [CrossRef]
- Zhang, Y.; Shan, Y.; Liu, X.; He, T. An integrated multi-objective topology optimization method for automobile wheels made of lightweight materials. Struct. Multidiscip. Optim. 2021, 64, 1585–1605. [Google Scholar] [CrossRef]
- Kim, J.; Kim, J.J.; Jang, I.G. Integrated topology and shape optimization of the five-spoke steel wheel to improve the natural frequency. Struct. Multidiscip. Optim. 2022, 65, 78. [Google Scholar] [CrossRef]
- Zhu, J.; Cai, X.; Ma, D.; Zhang, J.; Ni, X. Improved structural design of wind turbine blade based on topology and size optimization. Int. J. Low-Carbon Technol. 2022, 17, 69–79. [Google Scholar] [CrossRef]
- Zeng, A.; Li, F. Optimal design of rectangular mirror based on topology and size optimization. In Proceedings of the AOPC 2022: Infrared Devices and Infrared Technology; and Terahertz Technology and Applications, Beijing, China, 18–20 December 2022; SPIE: Bellingham, WA, USA, 2023; Volume 12555, pp. 113–118. [Google Scholar] [CrossRef]
- Tian, X.; Sun, X.; Liu, G.; Deng, W.; Wang, H.; Li, Z.; Li, D. Optimization design of the jacket support structure for offshore wind turbine using topology optimization method. Ocean Eng. 2022, 243, 110084. [Google Scholar] [CrossRef]
- Cui, Y.; Yu, Y.; Yu, J.; Li, Z. Topology-size-material Joint Optimization Design of Long-pan Unsupported Decks. China Mech. Eng. 2022, 33, 2879. [Google Scholar] [CrossRef]
- Song, J.; Chen, J.; Wu, Y.; Li, L. Topology Optimization-Driven Design for Offshore Composite Wind Turbine Blades. J. Mar. Sci. Eng. 2022, 10, 1487. [Google Scholar] [CrossRef]
- Park, C.H.; Son, Y.S.; Kim, B.I.; Ham, S.Y.; Lee, S.W.; Lim, H.C. Design of tracking mount and controller for mobile satellite laser ranging system. Adv. Space Res. 2012, 49, 177–184. [Google Scholar] [CrossRef]
- Hedrick, R.L.; Keller, A.; Haberman, J.; Iott, K.; Hull, T.B.; Clarkson, A.R.; Genet, R. New paradigms for producing high-performing meter class ground-based telescopes. In Modern Technologies in Space-and Ground-based Telescopes and Instrumentation; SPIE: Bellingham, WA, USA, 2010; Volume 7739, pp. 627–641. [Google Scholar] [CrossRef]
- Chen, Y.X.; Gao, L. Quality study on laser welding 304 stainless sheet. Metalurgija 2023, 62, 65–67. [Google Scholar]
- Rozvany, G.I.N. A critical review of established methods of structural topology optimization. Struct. Multidiscip. Optim. 2009, 37, 217–237. [Google Scholar] [CrossRef]
- Sadeghian, A.; Iqbal, N. A review on dissimilar laser welding of steel-copper, steel-aluminum, aluminum-copper, and steel-nickel for electric vehicle battery manufacturing. Opt. Laser Technol. 2022, 146, 107595. [Google Scholar] [CrossRef]
- Li, Y.; Xiong, M.; He, Y.; Xiong, J.; Tian, X.; Mativenga, P. Multi-objective optimization of laser welding process parameters: The trade-offs between energy consumption and welding quality. Opt. Laser Technol. 2022, 149, 107861. [Google Scholar] [CrossRef]



| Young Modulus E (GPa) | Poisson Ratio | Density (kg/m3) | Yield Strength (MPa) | |
|---|---|---|---|---|
| 304 | 199 | 0.3 | 7850 | ≥205 |
| Q235 | 210 | 0.3 | 7850 | ≥235 |
| R1 | R2 | R3 | R4 | R5 | R7 | R10 | R11 | R12 | R13 | R14 | R16 | R17 | R18 | R19 | |
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| T1 | 7 | 7 | 7 | 7 | 7 | 7 | 7 | 7 | 7 | 7 | 7 | 7 | 7 | 7 | 7 |
| T2 | 4 | 4 | 4 | 4 | 4 | 4 | 4 | 4 | 4 | 4 | 4 | 4 | 4 | 4 | 4 |
| T3 | 4 | 4 | 4 | 4 | 4 | 4 | 4 | 4 | 4 | 4 | 4 | 4 | 4 | 4 | 4 |
| T4 | 4 | 4 | 4 | 4 | 4 | 4 | 4 | 4 | 4 | 4 | 4 | 4 | 4 | 4 | 4 |
| T5 | 4 | 4 | 4 | 4 | 3 | 4 | 4 | 4 | 4 | 4 | 4 | 4 | 4 | 4 | 3 |
| T6 | 10 | 10 | 10 | 10 | 10 | 10 | 10 | 10 | 10 | 10 | 10 | 10 | 10 | 10 | 10 |
| T7 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 |
| T8 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 |
| T9 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 |
| T10 | 4 | 5 | 5 | 4 | 4 | 4 | 4 | 4 | 4 | 4 | 4 | 4 | 4 | 4 | 4 |
| T11 | 4 | 4 | 4 | 4 | 4 | 4 | 4 | 4 | 4 | 4 | 4 | 4 | 4 | 4 | 4 |
| T12 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 |
| T13 | 5 | 2 | 2 | 3 | 3 | 6 | 6 | 6 | 3 | 6 | 2 | 3 | 3 | 5 | 3 |
| T14 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 |
| T15 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 |
| T16 | 10 | 10 | 10 | 10 | 10 | 10 | 10 | 10 | 10 | 10 | 10 | 10 | 10 | 10 | 10 |
| T17 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 |
| T18 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 3 |
| T19 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 |
| T20 | 3 | 3 | 3 | 3 | 3 | 3 | 3 | 3 | 3 | 3 | 3 | 3 | 3 | 3 | 3 |
| T21 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 | 2 |
| Weight (kg) | 92.68 | 92.63 | 93.00 | 92.33 | 92.68 | 90.72 | 92.53 | 93.66 | 92.07 | 92.53 | 92.63 | 92.81 | 91.14 | 91.11 | 91.10 |
| Without Welding | By Laser Welding | By TIG Welding | |
|---|---|---|---|
| Tensile strength (MPa) | 795 | 773 | 474 |
| Yield strength (MPa) | 359 | 293 | 256 |
| Tensile elongation at fracture (%) | 68.5 | 69 | 15 |
| Modal No. | Simulation Results (Hz) | Experiment Results (Hz) | Error |
|---|---|---|---|
| 1 | 77.58 | 76.58 | −1.29% |
| 2 | 126.70 | 117.39 | −7.35% |
| 3 | 134.63 | 136.43 | +1.34% |
| Mean | 113.00 | 110.13 | −2.53% |
| Original Design | Optimized Design | Percent Change | |
|---|---|---|---|
| Dynamic performance (the sum of the first three order frequencies) | 89.12 Hz | 95.47 Hz | +7.13% |
| Static performance (flexibility) | 31.10 | 21.89 | −29.61% |
| Optomechanical performance (relative deformation angle) | 3.66″ | 3.67″ | +0.27% |
| Weight | 129.4 kg | 90.72 kg | −29.89% |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, G.; Huo, Z.; Yuan, J.; Tan, L.; Zhang, L.; Li, J. Topology and Size Optimized Design and Laser Welding of the U-Frame for Free-Space Laser Communication Telescopes. Machines 2023, 11, 868. https://doi.org/10.3390/machines11090868
Li G, Huo Z, Yuan J, Tan L, Zhang L, Li J. Topology and Size Optimized Design and Laser Welding of the U-Frame for Free-Space Laser Communication Telescopes. Machines. 2023; 11(9):868. https://doi.org/10.3390/machines11090868
Chicago/Turabian StyleLi, Guangzhen, Zhanwei Huo, Jian Yuan, Luyang Tan, Lei Zhang, and Ji Li. 2023. "Topology and Size Optimized Design and Laser Welding of the U-Frame for Free-Space Laser Communication Telescopes" Machines 11, no. 9: 868. https://doi.org/10.3390/machines11090868
APA StyleLi, G., Huo, Z., Yuan, J., Tan, L., Zhang, L., & Li, J. (2023). Topology and Size Optimized Design and Laser Welding of the U-Frame for Free-Space Laser Communication Telescopes. Machines, 11(9), 868. https://doi.org/10.3390/machines11090868





