Study of Surface Integrity of SiCp/Al Composites Using High-Speed Milling under Cryogenic Liquid Nitrogen Conditions
Abstract
1. Introduction
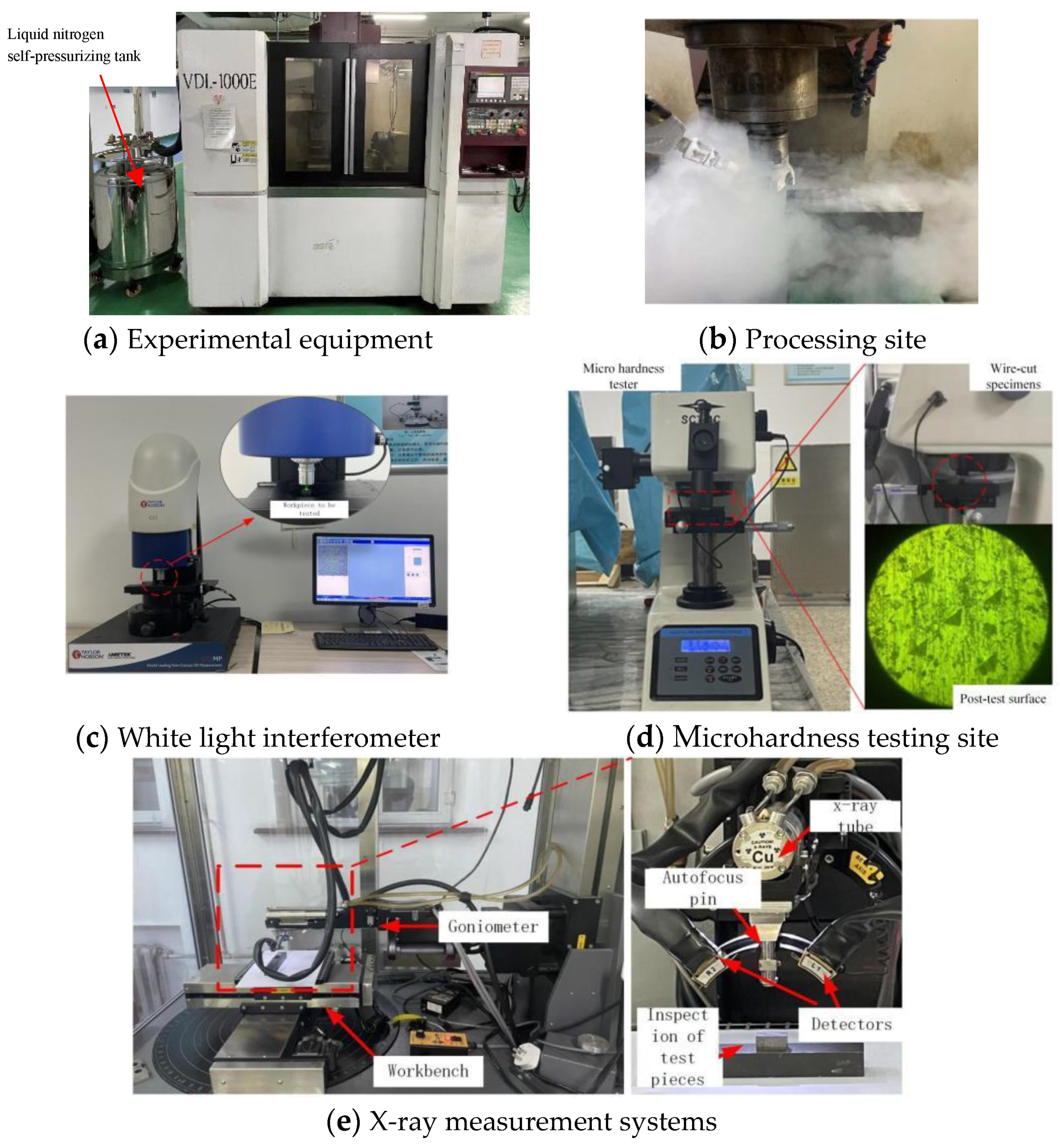
2. Test Conditions and Scheme
2.1. Test Conditions
2.2. Test Scheme
3. Analysis of Surface Roughness and Surface Morphology
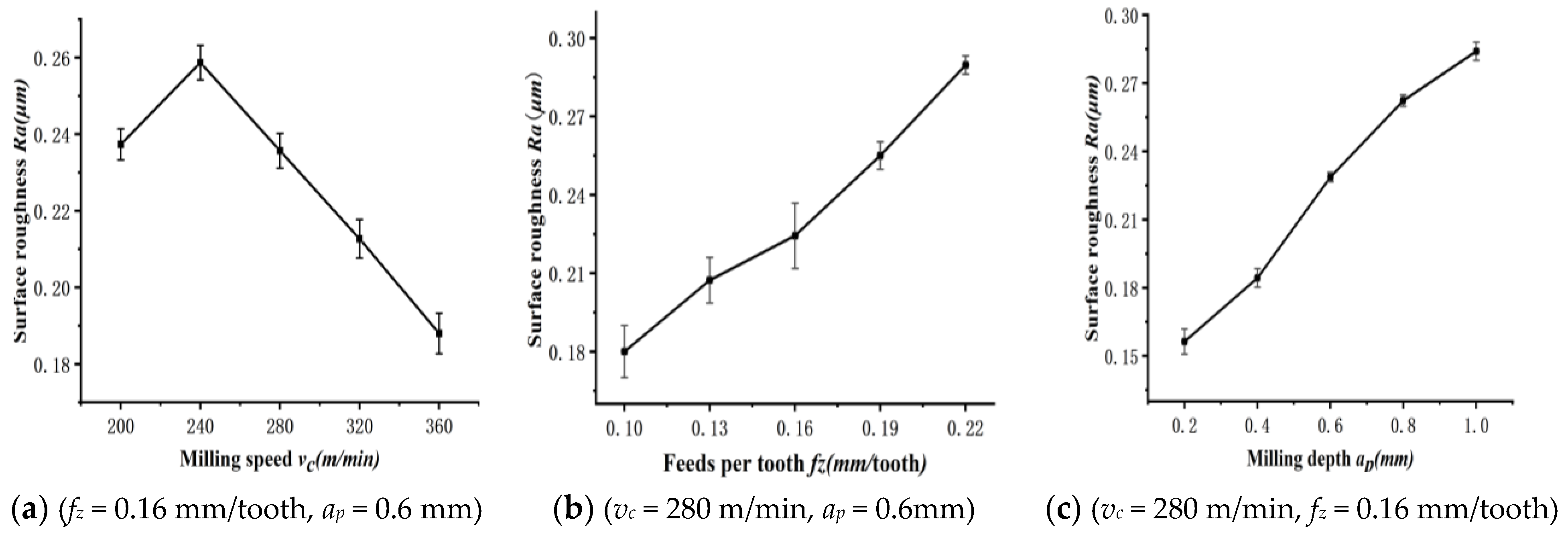
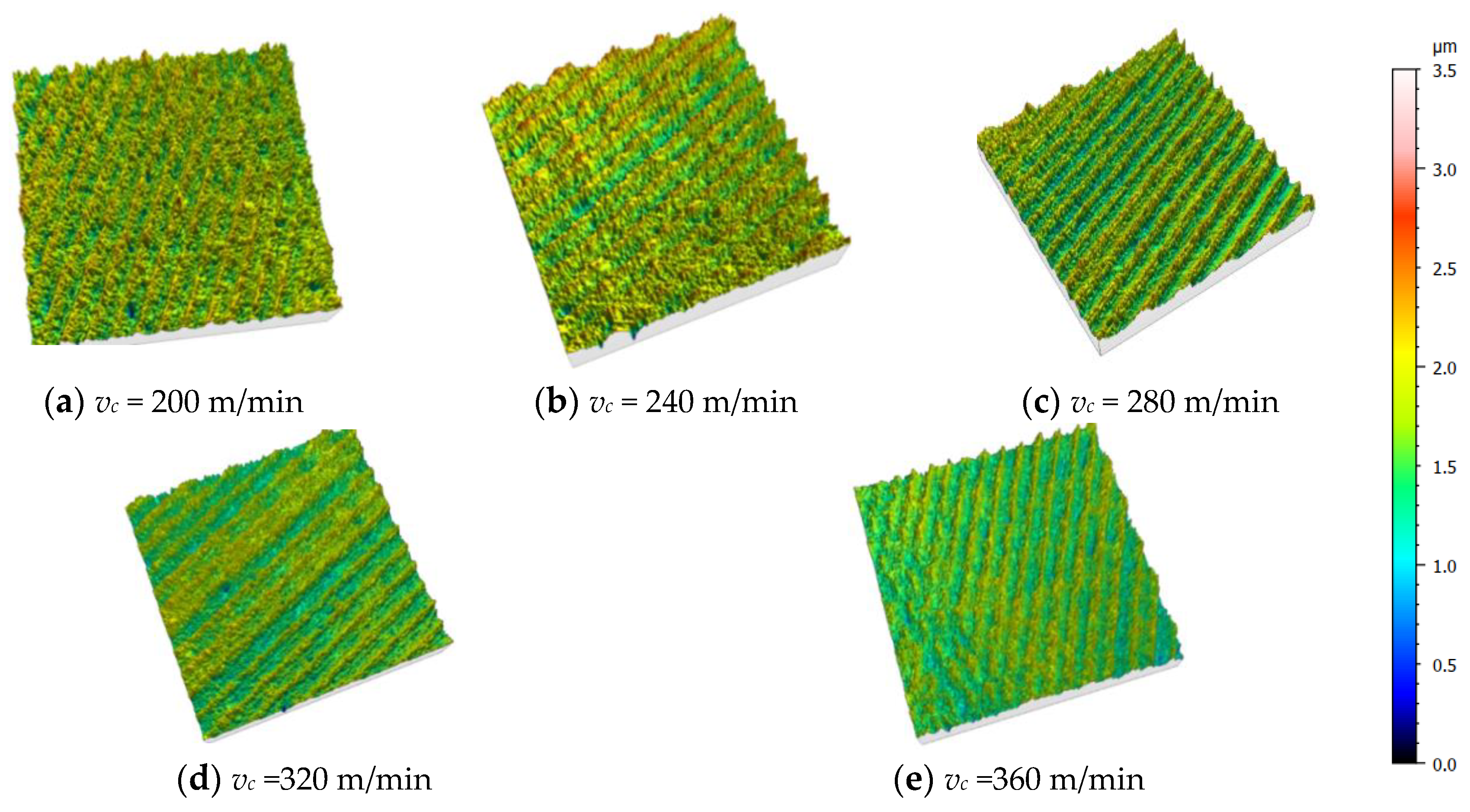
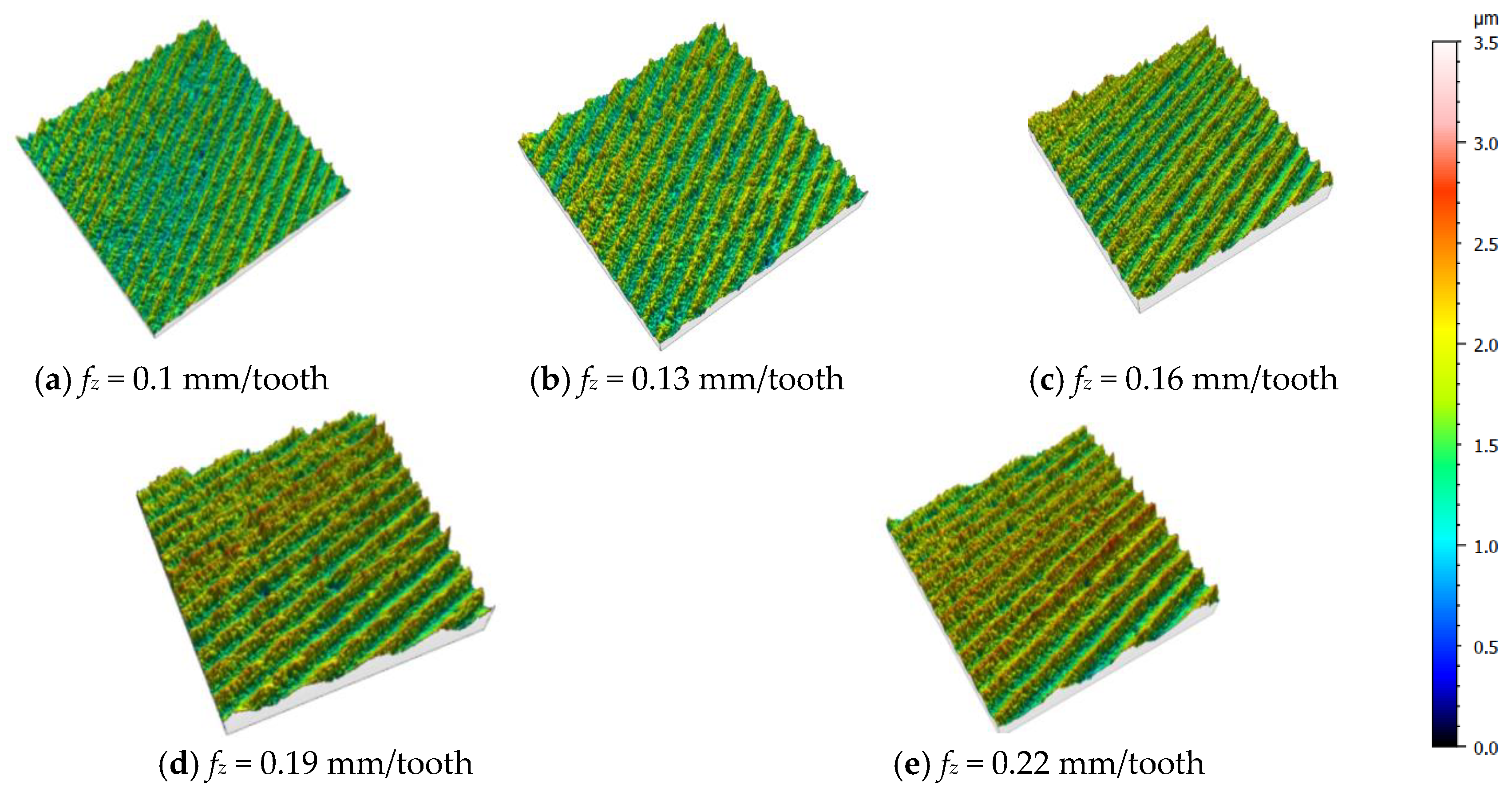
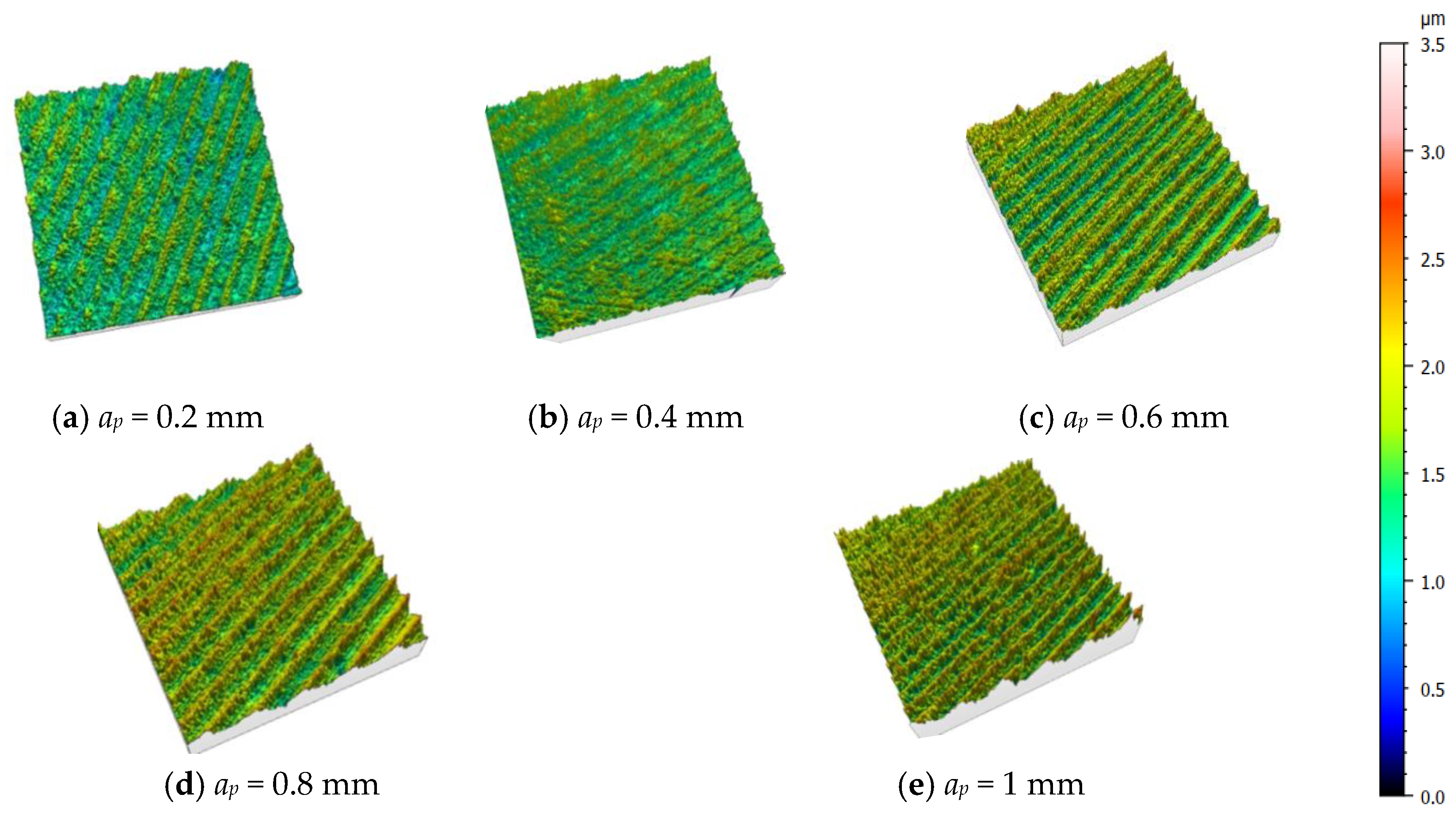
3.1. Effects of Milling Speed, Feed Rate, and Milling Depth on Surface Roughness and Surface Morphology
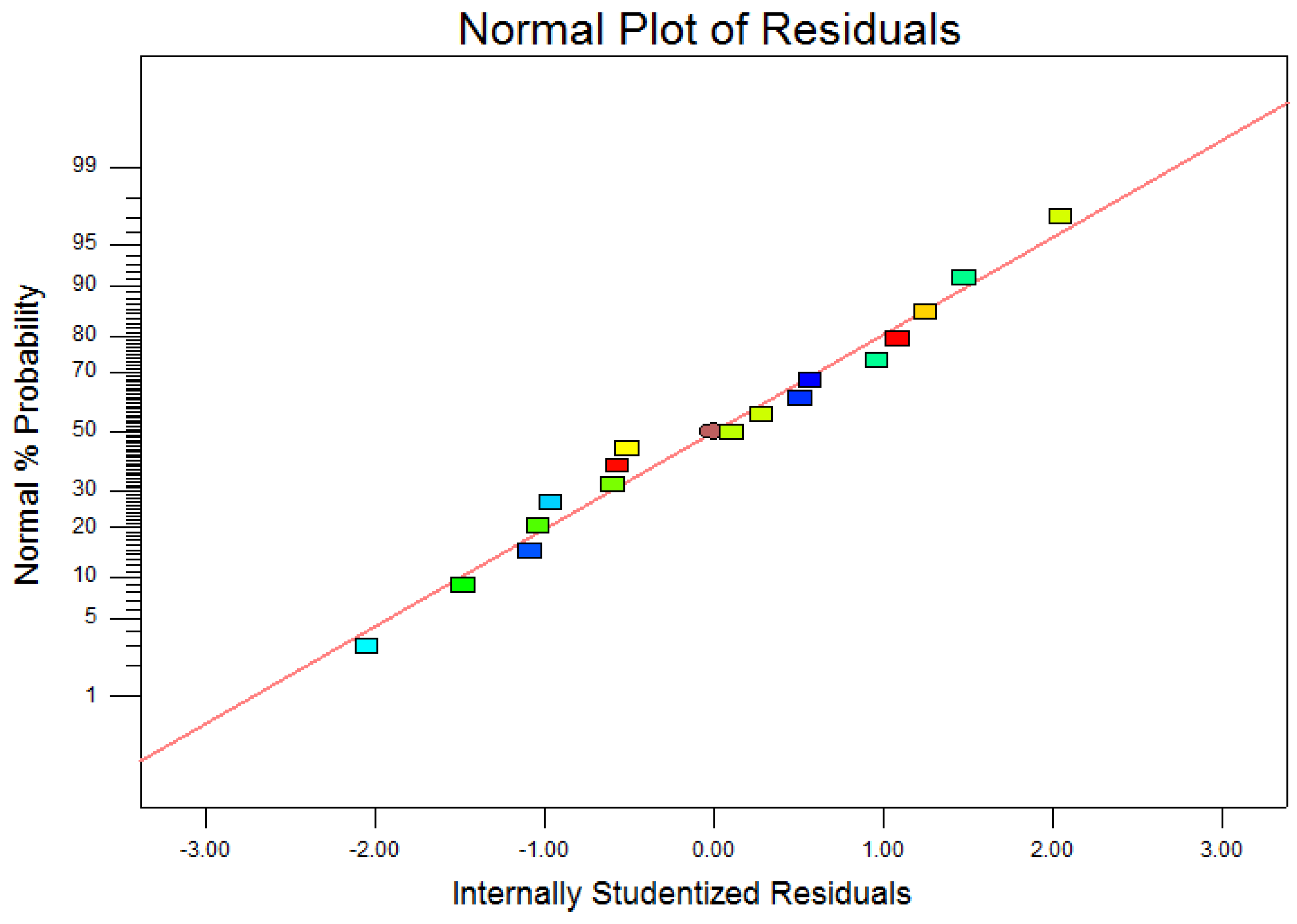
3.2. Construction of Response Surface Method-Based Surface Roughness Prediction Model
- (1)
- Single-factor effect: fz, ap, vc;
- (2)
- Interaction: fz…ap interaction, vc…fz interaction, vc…ap interaction;
- (3)
- Secondary effects: ap2, fz2, vc2.
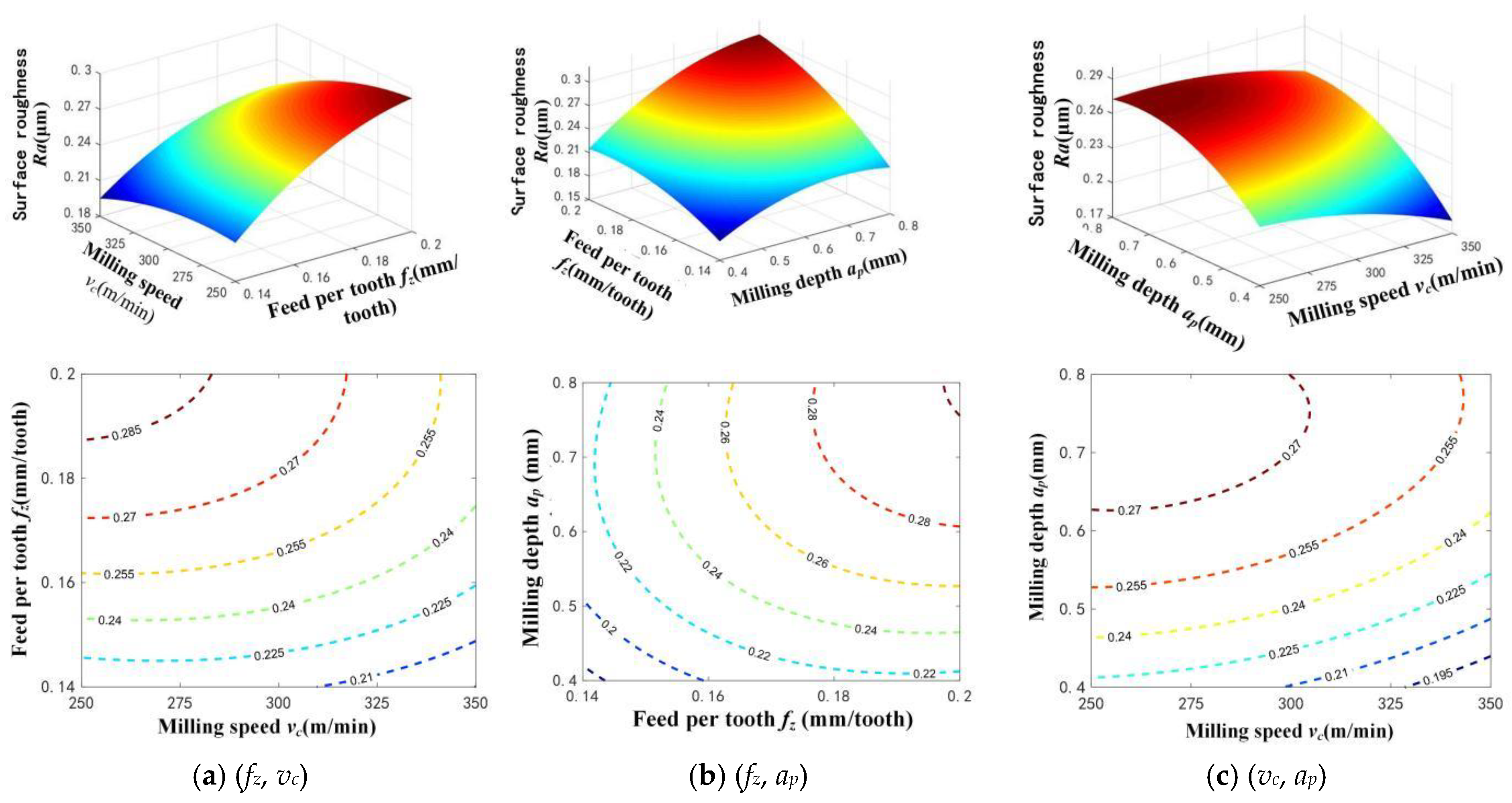
3.3. Effect of the Interaction of Feed Rate, Milling Speed, and Milling Depth on Surface Roughness
3.3.1. Effect of Feed Rate fz and Milling Speed vc on Surface Roughness
3.3.2. Effect of Feed Rate fz and Milling Depth ap on Surface Roughness
3.3.3. Effect of Milling Speed vc and Milling Depth ap on Surface Roughness
4. Analysis of Surface Microhardness
4.1. Effects of Milling Speed, Feed Rate, and Milling Depth on Surface Microhardness
4.1.1. Effect of Milling Speed vc on Surface Microhardness
4.1.2. Effect of Feed Rate fz on Surface Microhardness
4.1.3. Effect of Milling Depth ap on Surface Microhardness
5. Analysis of Surface Residual Stresses
5.1. Building of a Residual Stress model Based on Finite Element Simulation
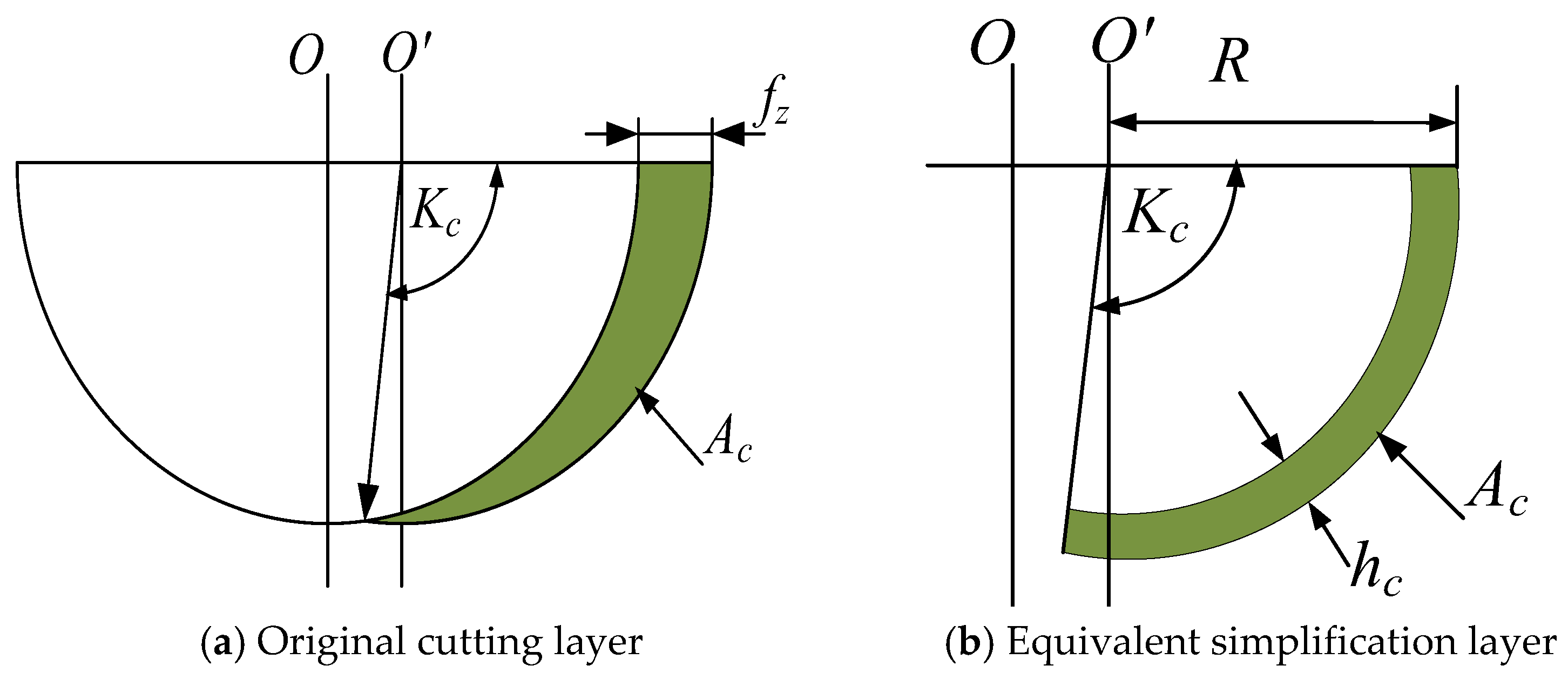
5.1.1. Equal Cutting Thickness Model
5.1.2. Equal Cutting Thickness Model
5.1.3. Boundary Conditions and Friction Models
5.2. Analysis of Residual Stress Simulation Results
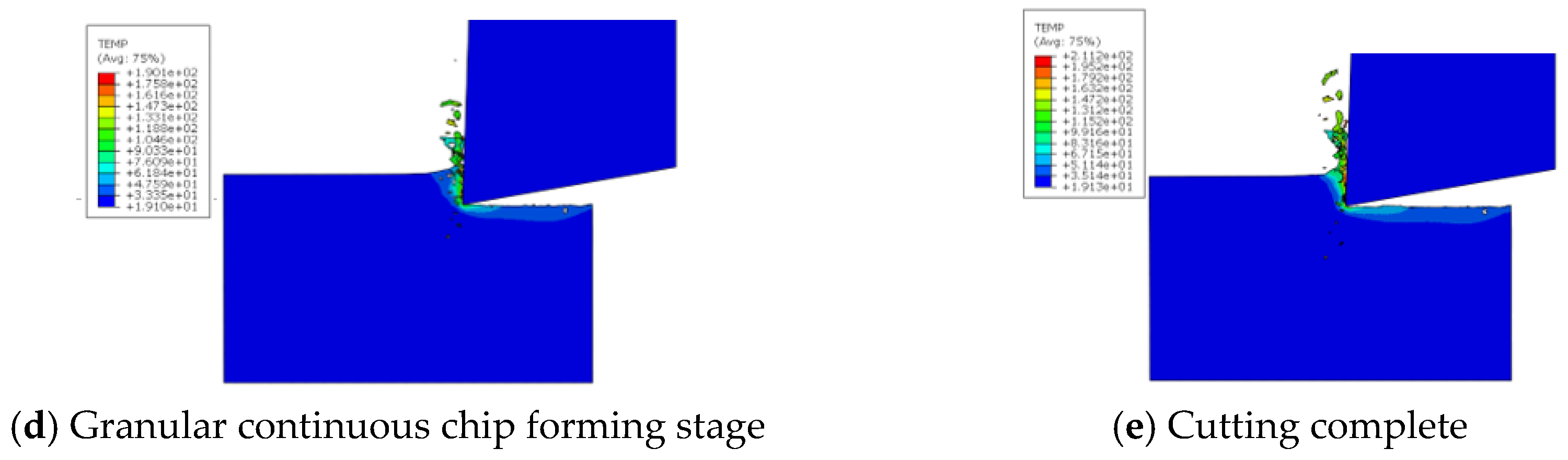
5.2.1. Chip Forming Process and Analysis
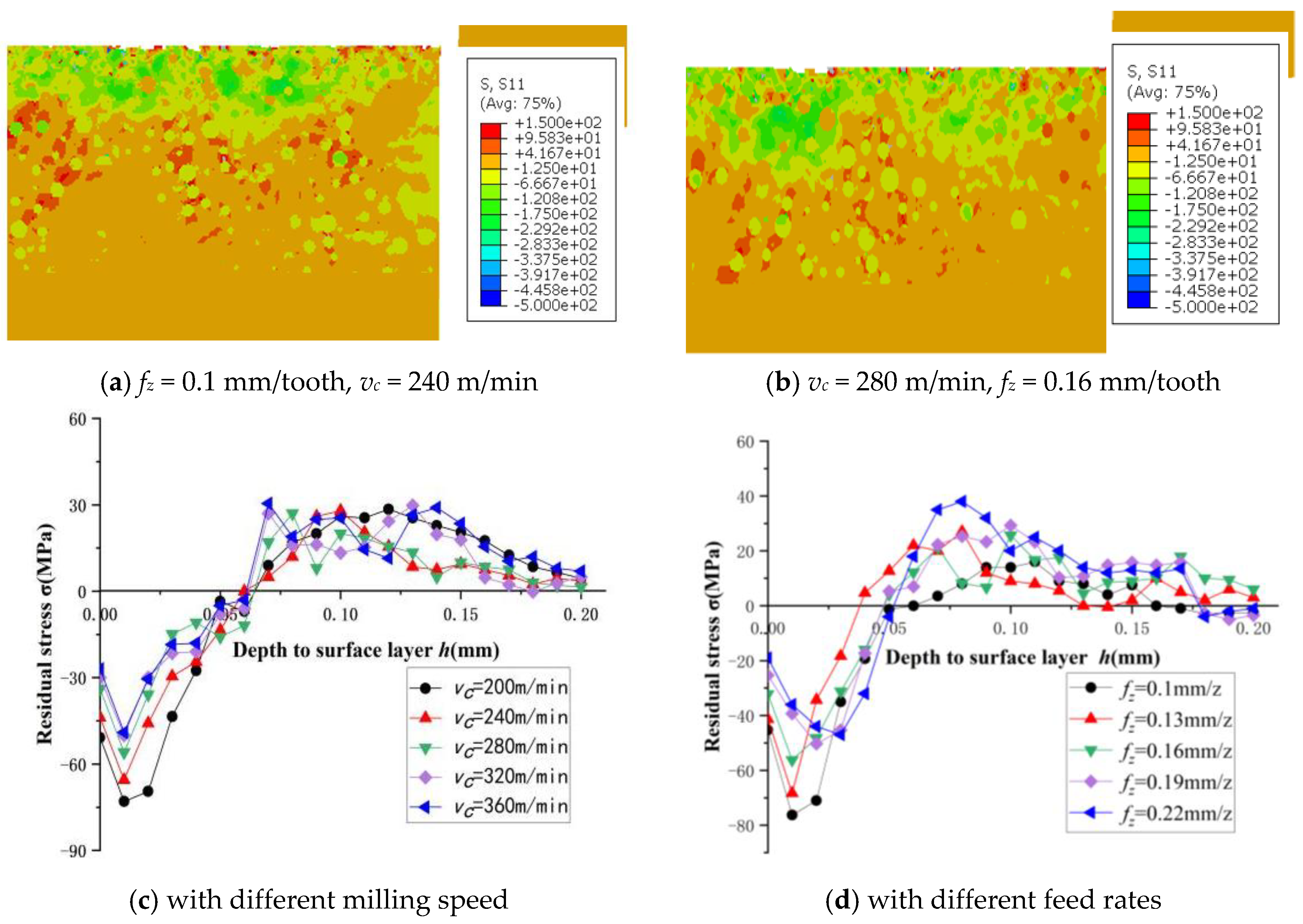
5.2.2. Effects of Milling Speed and Feed per Tooth on Residual Stress
5.3. Effects of Milling Speed, Feed Rate, and Milling Depth on Surface Residual Stress
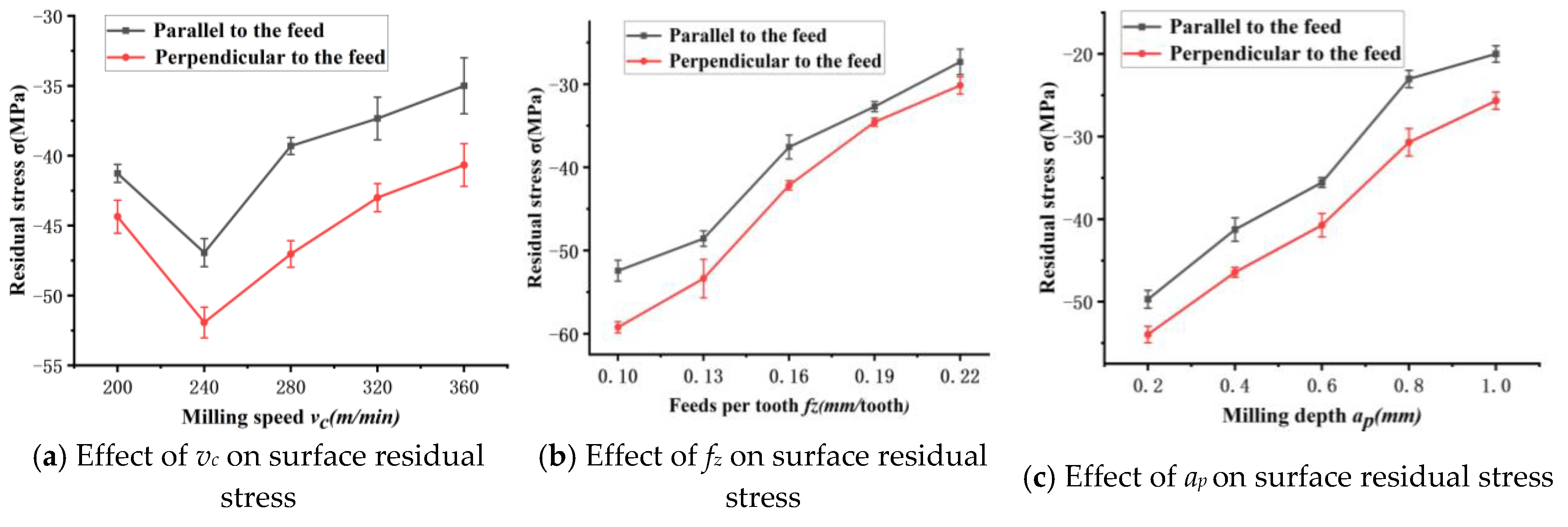
5.3.1. Effect of Milling Speed vc on Surface Residual Stress
5.3.2. Effect of Feed Rate fz on Surface Residual Stress
5.3.3. Effect of Depth ap on Surface Residual Stress
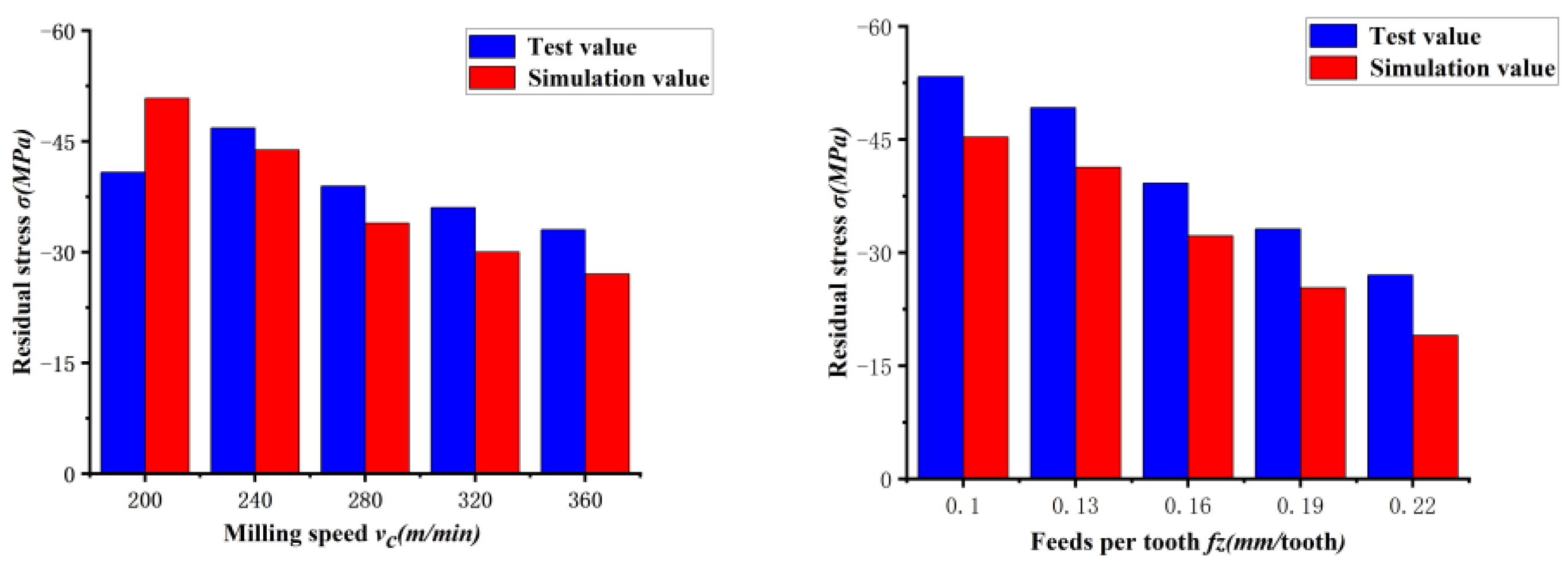
5.4. Simulation vs. Experiment
6. Conclusions
- The surface roughness end milling test was carried out under the low-temperature auxiliary cooling of liquid nitrogen, and the three-dimensional topography of the machined surface was observed. It was found that the number of surface pits could be significantly reduced with an increase in milling speed. Moreover, higher milling depths can cause defects with large areas of jagged tips on the surface.
- By conducting a central combination test, a second-order surface roughness prediction model was established and tested based on the test data, in which the model and coefficients were significant, the maximum error was 7.91%, the average error was 3.25%, and the model was more reliable. The results of the interaction analysis show that a high level of both feed rate and milling depth is not recommended for cryogenic liquid nitrogen-assisted cooling conditions.
- Surface microhardness measurements were performed on the specimens, and the results show that as the milling speed increased, the surface microhardness value first increased, and then, became smaller, and its maximum value was obtained at 240m/min. Therefore, under low-temperature liquid nitrogen conditions, it is recommended to use a speed of more than 240 m/min for milling. When the milling depth increases in the range of 0.2~1 mm, the degree of work hardening will increase, and the feed rate will be the same as the trend.
- By simulating the surface residual stresses, it was found that when a larger feed rate or higher milling speed is used, higher surface residual tensile stresses appear in the surface layer. The surface of the specimen after end milling undergoes surface residual compressive stresses, and when the milling speed increases in the range of 240 mm/tooth~360 mm/tooth, the surface residual compressive stresses show a trend of first increasing, and then, decreasing. There is a tendency for surface residual compressive stresses to convert to tensile stresses as a result of increased milling depth or feed rates. In addition, as the milling depth and the feed per tooth increase, the interference effect of the SiC particles on internal residual stress transfer also increases. Therefore, it is not recommended to use both a high depth of cutting and high feed per tooth. Upon using test data to verify the simulation values, we found that the trends of the two are basically the same, and that they have high reliability.
Author Contributions
Funding
Conflicts of Interest
References
- Wang, C.; Zhou, L.; Wang, Y.; Ding, H. The effect of microstructure on mechanical properties of SiCp/Al composites was simulated numerically. J. Mater. Sci. Eng. 2015, 33, 122–126. [Google Scholar] [CrossRef]
- Zhou, Y.; Zhang, D.; Wang, W.; Li, L. Research status and aerospace application of sic particle reinforced aluminum matrix composites formed by selective laser melting. Aeronaut. Manuf. Technol. 2018, 61, 68–73. [Google Scholar] [CrossRef]
- Bao, Y.; Zhang, X.; Lu, S.; Zhang, H. Investigation on the removal characteristics of single-point cutting high-volume fraction SiCp/Al composites. Int. J. Adv. Manuf. Technol. 2021, 118, 881–894. [Google Scholar] [CrossRef]
- Zhu, W. Microstructure and Interface Characterization of Aluminum-Based Silicon Carbide Composites with Medium and High Volume Fraction. Master’s Thesis, Shenyang University, Shenyang, China, 2021. [Google Scholar]
- Ouyang, L.; Luo, C. Application of composite materials and its progress. Automot. Technol. Mater. 2000, 28–31. [Google Scholar]
- Liu, J.; Cheng, K.; Ding, H.; Chen, S.; Zhao, L. An Investigation of Surface Defect Formation in Micro Milling the 45% SiCp/al Composite. Procedia CIRP 2016, 45, 211–214. [Google Scholar] [CrossRef]
- Li, Z.; Hou, S. Research progress in cutting of sic reinforced aluminum matrix composites. Tool Eng. 2017, 51, 9–13. [Google Scholar] [CrossRef]
- Zhou, Y. Silicon carbide particle reinforced aluminum matrix composites. Tool Eng. 2017, 51, 7–10. [Google Scholar] [CrossRef]
- Wang, T.; Xie, L.; Wang, X.; Jiao, L.; Shen, J.; Xu, H.; Nie, F. Surface Integrity of High Speed Milling of Al/SiC/65p Aluminum Matrix Composites. Procedia CIRP 2013, 15, 475–480. [Google Scholar] [CrossRef]
- Bian, R.; He, N.; Li, L.; Zhan, Z.B.; Wu, Q.; Shi, Z.Y. Precision Milling of High Volume Fraction SiCp/Al Composites with Monocrystalline Diamond end Mill. Int. J. Adv. Manuf. Technol. 2013, 71, 411–419. [Google Scholar] [CrossRef]
- Zhou, L.; Zhang, D.; Wang, C.; Liu, D. Microstate SiCp/Al composite milling residual stress simulation analysis. Chin. J. Mater. Sci. Eng. 2016, 16, 5–10. [Google Scholar] [CrossRef]
- Xiang, J.; Pang, S.; Xie, L.; Hu, X.; Peng, S.; Wang, T. Investigation of Cutting Forces Surface Integrity, and Tool Wear when High-Speed Milling of High-Volume Fraction SiCp/Al6063 Composites in PCD Tooling. Int. J. Adv. Manuf. Technol. 2018, 98, 1237–1251. [Google Scholar] [CrossRef]
- Muthukrishnan, N.; Murugan, M.; Rao, K.P. Machinability Issues in Turning of Al-SiC (10p) Metal Matrix Composites. Int. J. Adv. Manuf. Technol. 2008, 39, 211–218. [Google Scholar] [CrossRef]
- Huang, S.; Guo, L.; Yang, H.; Su, Y.; Xu, L. Study on characteristics in high-speed milling SiCp/Al composites with small particles and high volume fraction by adopting PCD cutters with different grain sizes. Int. J. Adv. Manuf. Technol. 2019, 102, 9–12. [Google Scholar] [CrossRef]
- Huang, S.; Guo, L.; He, H.; Yang, H.; Su, Y.; Xu, L. Experimental study on SiCp/Al composites with different volume fractions in high-speed milling with PCD tools. Int. J. Adv. Manuf. Technol. 2018, 97, 5–8. [Google Scholar] [CrossRef]
- Li, M. Surface Quality Study of SiCp/Al Composites by High-Speed Milling. Master’s Thesis, Harbin Institute of Technology, Harbin, China, 2020; pp. 39–46. [Google Scholar] [CrossRef]
- Kaynak, Y.; Lu, T.; Jawahir, I.S. Cryogenic Machining-Induced Surface Integrity: A Review and Comparison with Dry, MQL, and Flood-Cooled Machining. Mach. Sci. Technol. 2014, 18, 149–198. [Google Scholar] [CrossRef]
- Zhou, L.; Huang, S.; Yu, X. Machining Characteristicsin Cryogenic Grinding of SiCp/Al Composites. Acta Metall. Sin. 2014, 27, 869–874. [Google Scholar] [CrossRef]
- Shi, R. Study on the Surface Characteristics of SiCp/Al Composites Machined by High-Speed Milling with Low-Temperature Trace Lubrication. Master’s Thesis, Harbin University of Science and Technology, Harbin, China, 2020; pp. 35–39. [Google Scholar] [CrossRef]
- Jiang, X.; Li, B.; Yang, J.; Zuo, X.; Li, K. An approach for analyzing and controlling residual stress generation during high-speed circular milling. Int. J. Adv. Manuf. Technol. 2013, 66, 1439–1448. [Google Scholar] [CrossRef]
- Masmiati, N.; Sarhan, A.A. Optimizing cutting parameters in inclined end milling for minimum surface residual stress-Taguchi approach. Measurement 2015, 60, 267–275. [Google Scholar] [CrossRef]
- Li, S.; Niu, Q.; Li, C.; Yu, Z.; TaeJo, K. Study on surface quality of SiCp/Al composites for low-temperature milling of coated tools. Aerosp. Mater. Technol. 2021, 51, 68–72. [Google Scholar]
- Tang, L. Study on the Surface Quality and Tool Wear of SiCp/Al Composites for Milling. Master’s Thesis, Hunan University of Science and Technology, Xiangtan, China, 2017; pp. 35–41. [Google Scholar]
- Zhang, D. Study on Milling Residual Stress of SiCp/Al Composites. Master’s Thesis, Shenyang Ligong University, Shenyang, China, 2016; pp. 35–40. [Google Scholar]
- Chen, H. Study on the Preparation of Ultrafine Crystalline Strip from Low-Temperature Cutting Aluminum Alloy 7075 and Its Properties. Master’s Thesis, South China University of Technology, Guangzhou, China, 2020; pp. 19–23. [Google Scholar] [CrossRef]
- Zhang, P.; Cui, C.; Zhang, D.; Zhou, L. Finite element analysis of milling residual stress of SiCp/Al composites. Tool Technol. 2017, 51, 52–56. [Google Scholar] [CrossRef]
- Jin, J. Optimization of SiCp/Al Composite Milling Tool Parameters and Low Temperature Minimum Lubrication Test Study. Harbin University of Science and Technology, Harbin, China, 2021. [Google Scholar] [CrossRef]




| The Element Type | C | Mg | Al | Si | Cu |
|---|---|---|---|---|---|
| Weight ratio (%) | 9.43 | 0.51 | 43.13 | 45.13 | 1.80 |
| Proportion of atomic number (%) | 19.59 | 0.47 | 34.7 | 44.34 | 0.9 |
| Factors | Horizontal Parameters | Processing Environment |
|---|---|---|
| Milling speed vc (m/min) | 200, 240, 280, 320, 360 | Cryogenic liquid nitrogen |
| Feed per tooth fz (mm/tooth) | 0.1, 0.13, 0.16, 0.19, 0.22 | |
| Milling depth ap (mm) | 0.2, 0.4, 0.6, 0.8, 1 | |
| Milling width ae (mm) | 8 | |
| Liquid nitrogen flow rate (g/s) | 6 |
| Factor | Unit | Symbol | Level | ||
|---|---|---|---|---|---|
| −1 | 0 | 1 | |||
| Milling speed | m/min | vc | 250 | 300 | 350 |
| Feed per tooth | mm/tooth | fz | 0.14 | 0.17 | 0.2 |
| Milling depth | mm | ap | 0.4 | 0.6 | 0.8 |
| Milling width | mm | ae | 8 | ||
| Trial Serial Number | Canonical Variables | Actual Parameters | Ra (μm) | ||||
|---|---|---|---|---|---|---|---|
| ×1 | ×2 | ×3 | Milling SPEED | Feed Per Tooth | Milling Depth | ||
| 1 | −1 | −1 | 0 | 250.00 | 0.14 | 0.60 | 0.222 |
| 2 | 0 | 0 | 0 | 300.00 | 0.17 | 0.60 | 0.252 |
| 3 | 1 | −1 | 0 | 350.00 | 0.14 | 0.60 | 0.188 |
| 4 | −1 | 1 | 0 | 250.00 | 0.2 | 0.60 | 0.299 |
| 5 | 1 | 1 | 0 | 350.00 | 0.2 | 0.60 | 0.239 |
| 6 | 0 | 0 | 0 | 300.00 | 0.17 | 0.60 | 0.253 |
| 7 | −1 | 0 | −1 | 250.00 | 0.17 | 0.40 | 0.218 |
| 8 | 1 | 0 | −1 | 350.00 | 0.17 | 0.40 | 0.184 |
| 9 | −1 | 0 | 1 | 250.00 | 0.17 | 0.80 | 0.269 |
| 10 | 0 | 0 | 0 | 300.00 | 0.17 | 0.60 | 0.263 |
| 11 | 1 | 0 | 1 | 350.00 | 0.17 | 0.80 | 0.248 |
| 12 | 0 | −1 | −1 | 300.00 | 0.14 | 0.40 | 0.178 |
| 13 | 0 | 1 | −1 | 300.00 | 0.2 | 0.40 | 0.221 |
| 14 | 0 | −1 | 1 | 300.00 | 0.14 | 0.80 | 0.203 |
| 15 | 0 | 1 | 1 | 300.00 | 0.2 | 0.80 | 0.298 |
| 16 | 0 | 0 | 0 | 300.00 | 0.17 | 0.60 | 0.261 |
| 17 | 0 | 0 | 0 | 300.00 | 0.14 | 0.60 | 0.250 |
| Aluminum | A (Mpa) | B (Mpa) | C | m | n |
|---|---|---|---|---|---|
| Parameters | 176.5 | 63.99 | 0.0036 | 0.859 | 0.183 |
| Material Properties | Aluminum Alloy | SiC Particles | PCD Cutter |
|---|---|---|---|
| Density (g/cm3) | 2.7 | 3.13 | 4.25 |
| Young’s modulus (Gpa) | 71.9 | 420 | 1147 |
| Poisson’s ratio | 0.34 | 0.14 | 0.07 |
| Thermal conductivity (W/m/°C) | 180 | 81 | 2100 |
| Specific heat capacity (J/kg/°C) | 880 | 427 | 525 |
| Coefficient of thermal expansion (10−6°C−1) | 23.5 | 21.8 | 4 |
| d1 | d2 | d3 | d4 | d5 |
|---|---|---|---|---|
| 0.074 | 0.089 | −2.441 | −4.76 | 0 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, H.; Qu, L.; Ding, C. Study of Surface Integrity of SiCp/Al Composites Using High-Speed Milling under Cryogenic Liquid Nitrogen Conditions. Machines 2023, 11, 608. https://doi.org/10.3390/machines11060608
Zhang H, Qu L, Ding C. Study of Surface Integrity of SiCp/Al Composites Using High-Speed Milling under Cryogenic Liquid Nitrogen Conditions. Machines. 2023; 11(6):608. https://doi.org/10.3390/machines11060608
Chicago/Turabian StyleZhang, Huiping, Liqiang Qu, and Chenglong Ding. 2023. "Study of Surface Integrity of SiCp/Al Composites Using High-Speed Milling under Cryogenic Liquid Nitrogen Conditions" Machines 11, no. 6: 608. https://doi.org/10.3390/machines11060608
APA StyleZhang, H., Qu, L., & Ding, C. (2023). Study of Surface Integrity of SiCp/Al Composites Using High-Speed Milling under Cryogenic Liquid Nitrogen Conditions. Machines, 11(6), 608. https://doi.org/10.3390/machines11060608





