Bearing Current and Shaft Voltage in Electrical Machines: A Comprehensive Research Review
Abstract
1. Introduction
2. Failure Modes of the Electric Motors
3. Bearing Current and Shaft Voltage
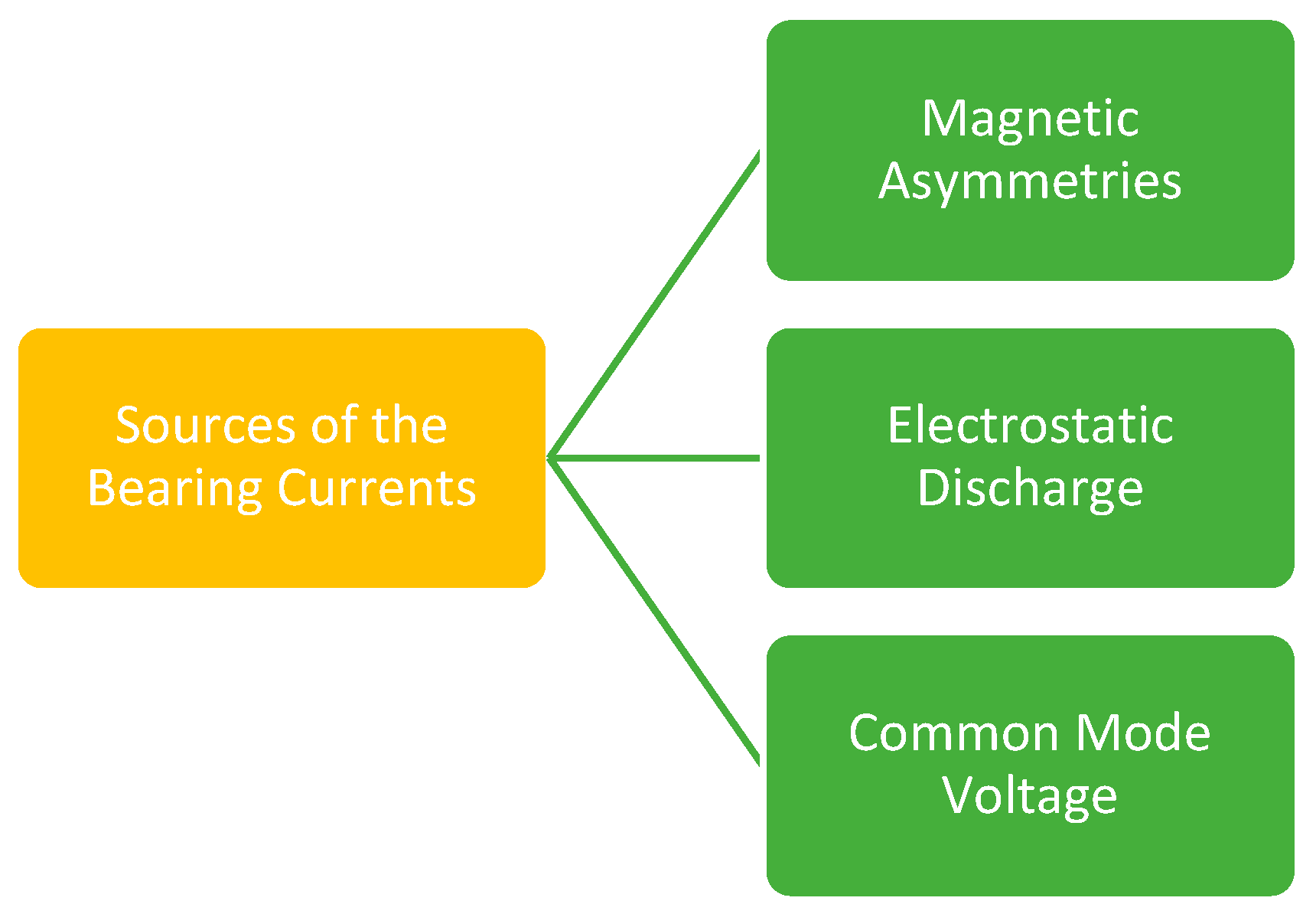
3.1. Sources of the Bearing Current and Shaft Voltage
3.1.1. Magnetic Asymmetries
3.1.2. Electrostatic Discharge
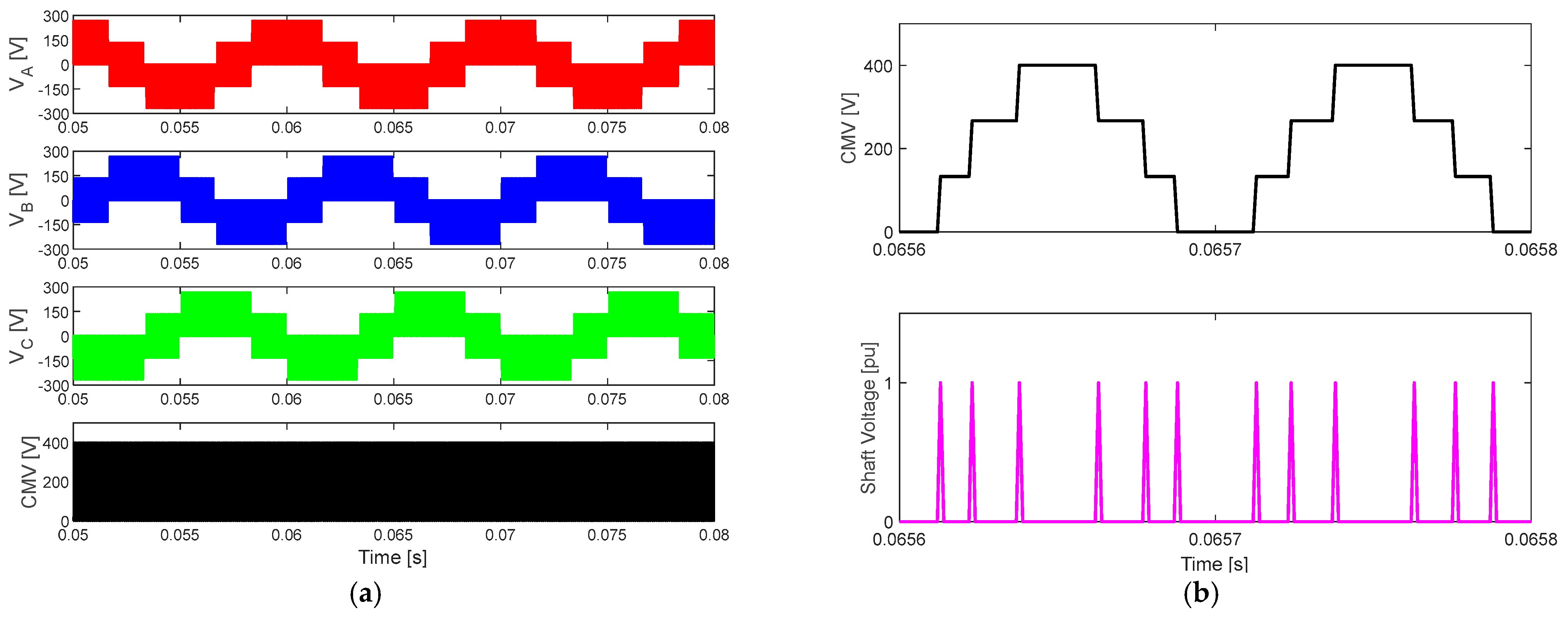
3.1.3. Common Mode Voltage
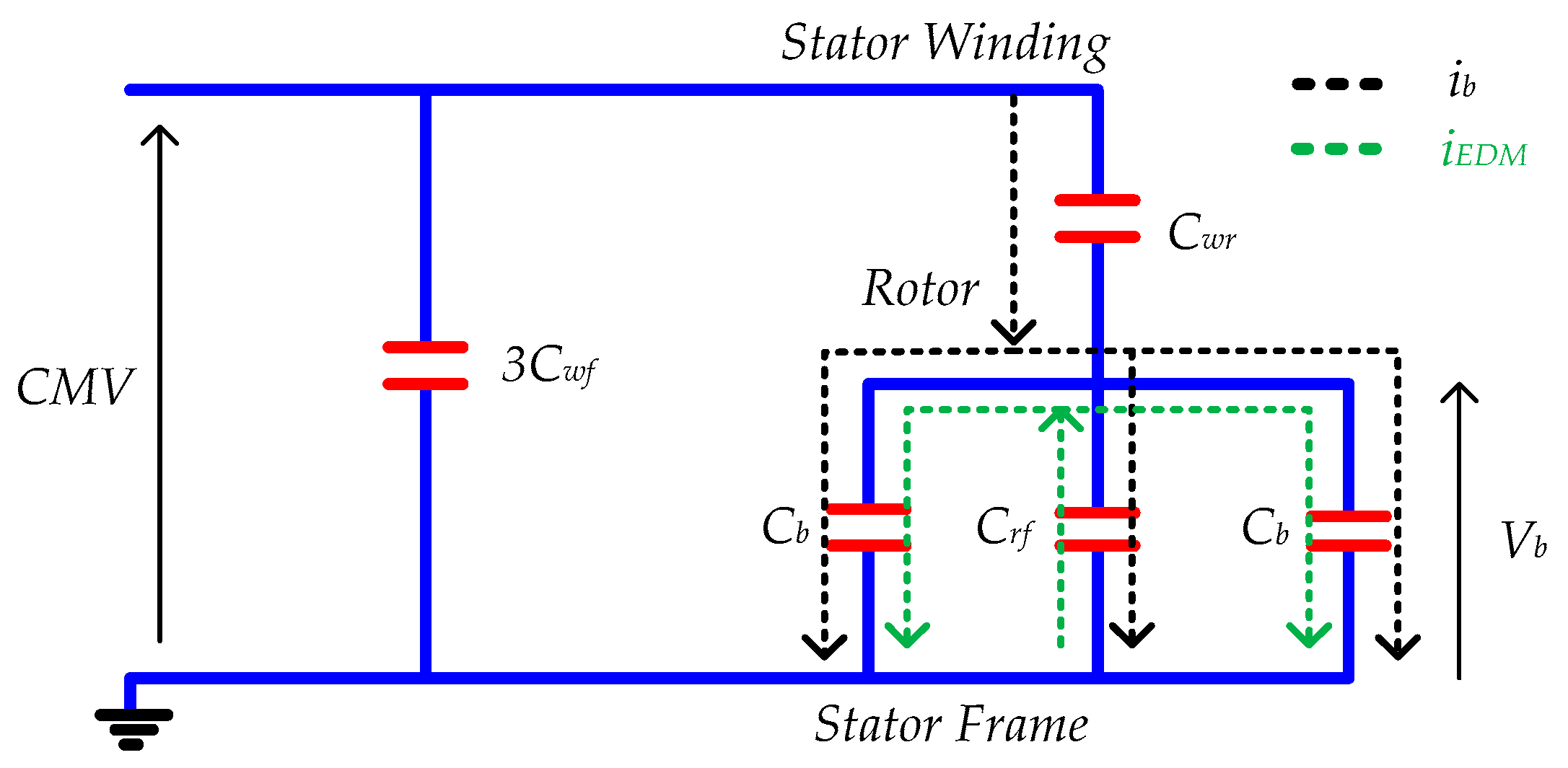
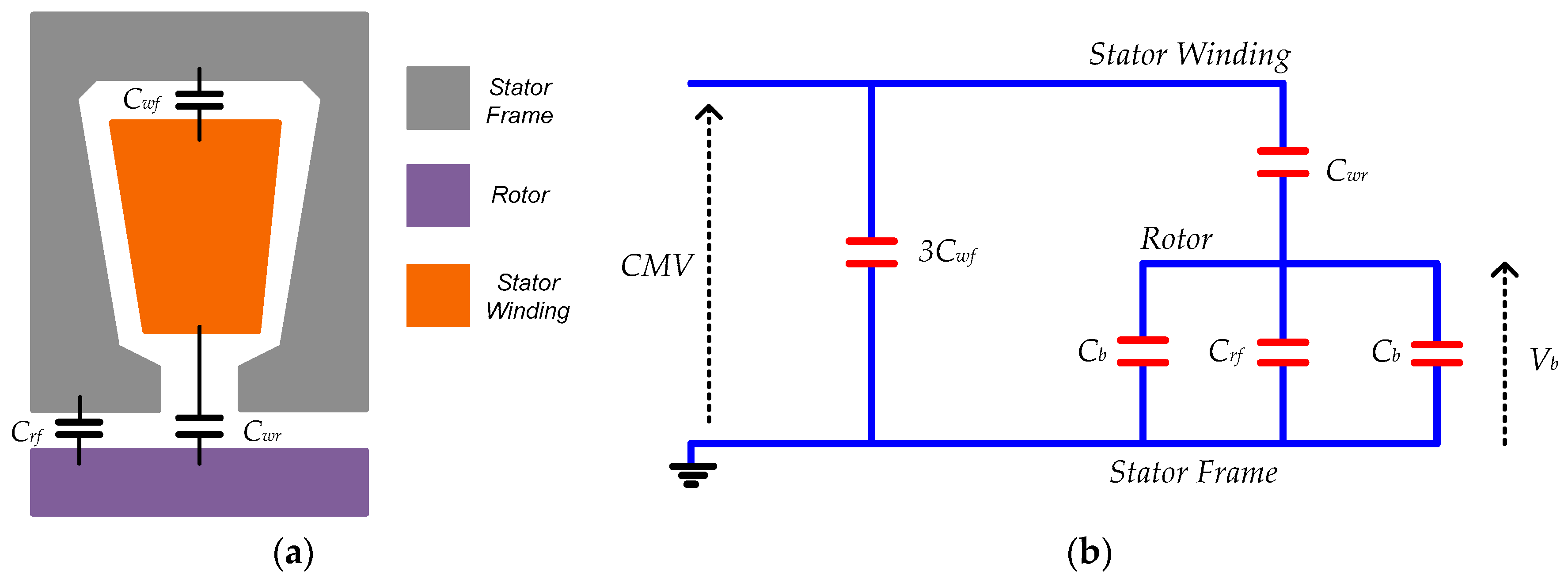
3.2. Bearing Voltage Ratio and Motor Capacitances
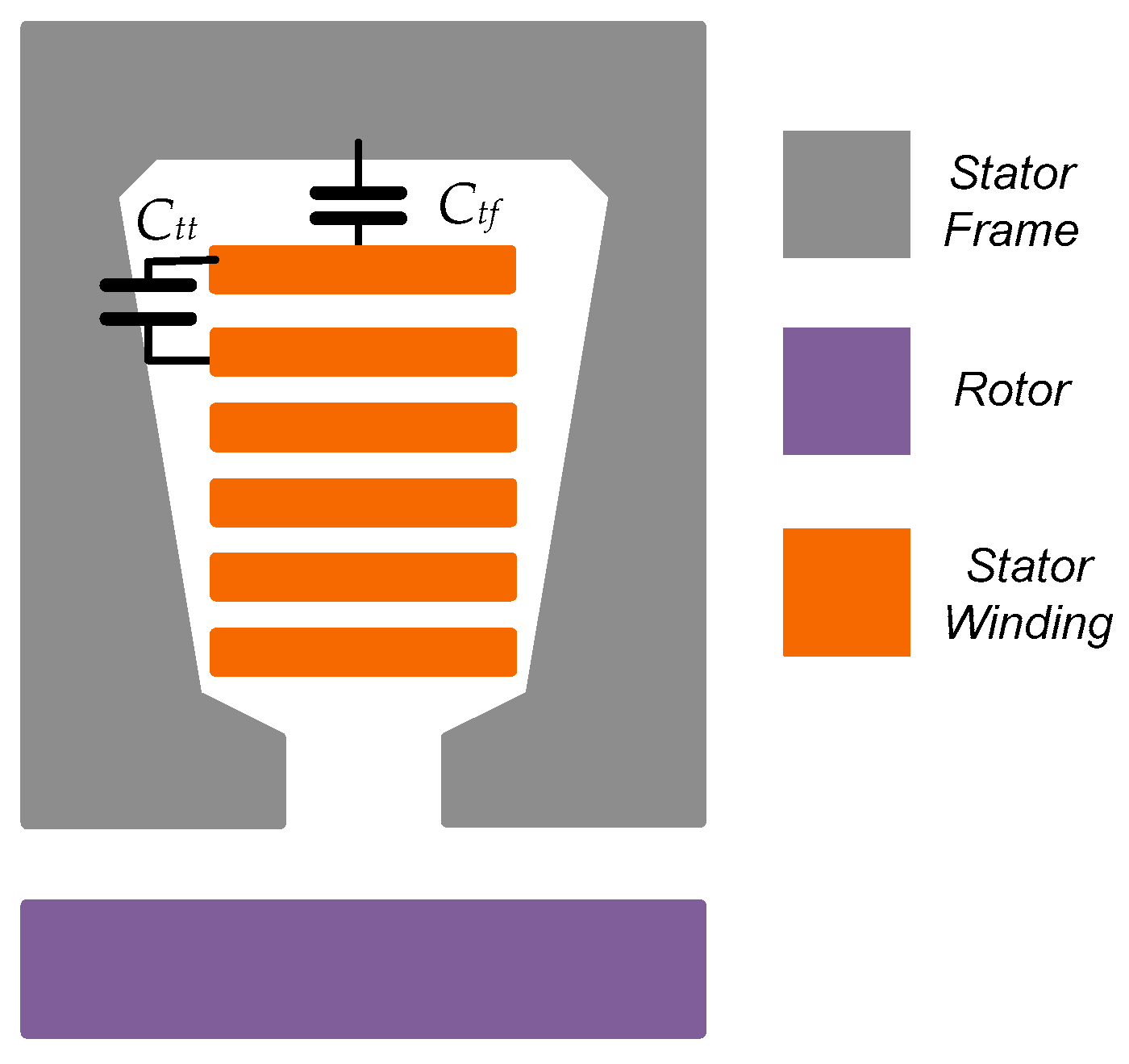
3.2.1. Stator Winding to Stator Frame Capacitance
3.2.2. Stator Winding to Rotor Capacitance
3.2.3. Rotor to Frame Capacitance
3.2.4. Phase to Phase Capacitances
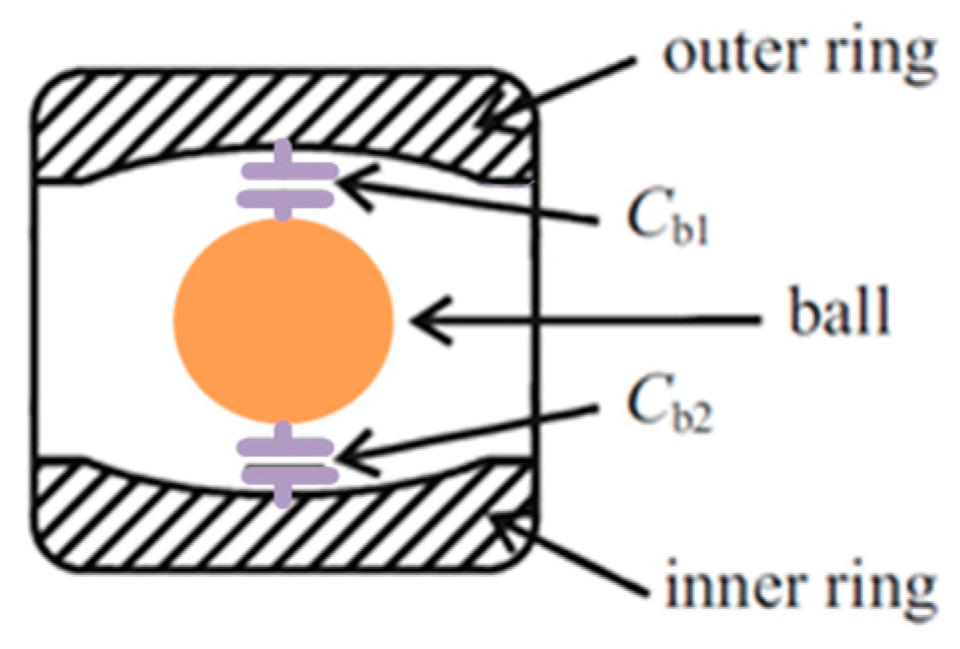
3.2.5. Bearing Capacitance
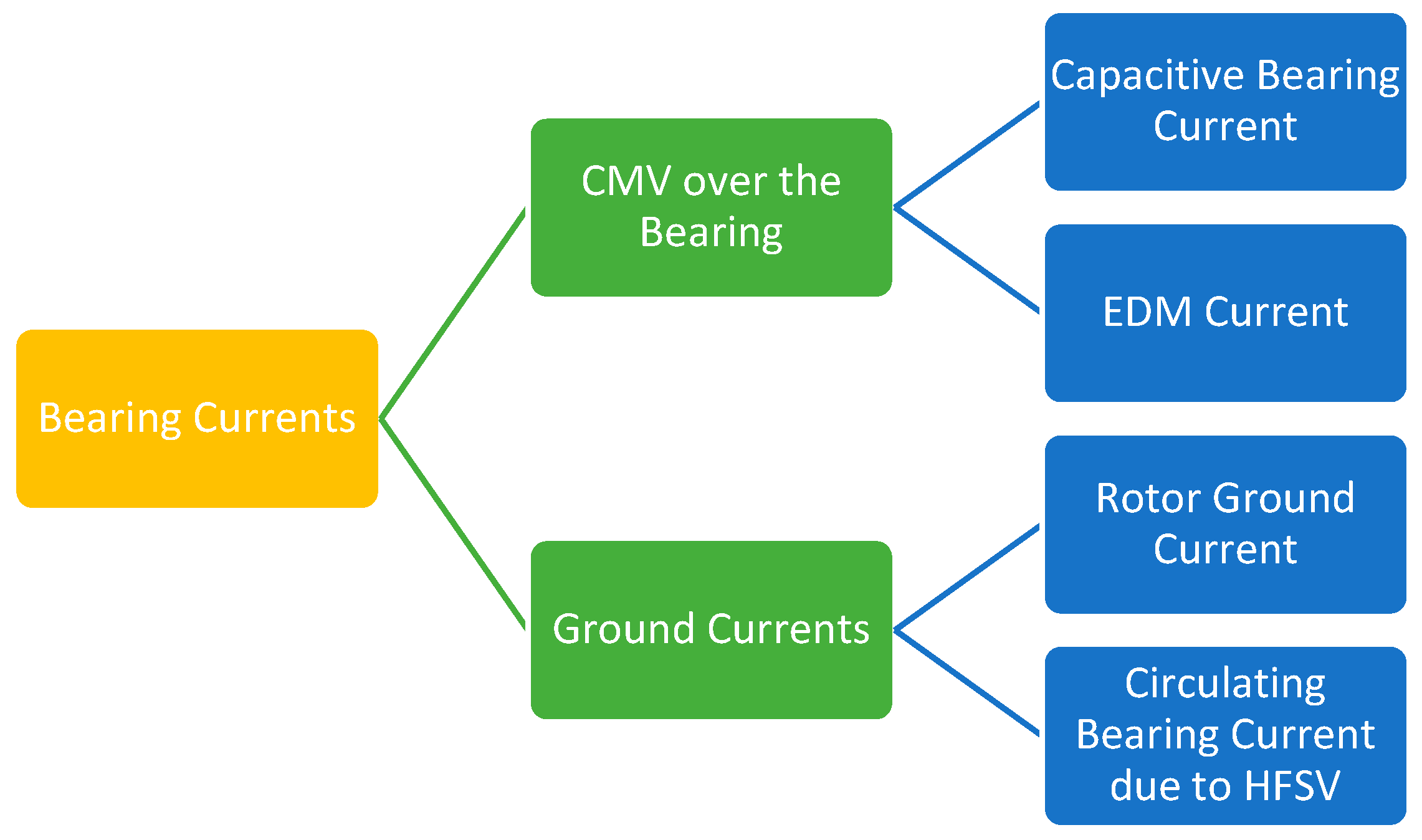
3.3. The Types of Bearing Current
3.3.1. Capacitive Bearing Currents
3.3.2. Electrostatic Discharge Currents
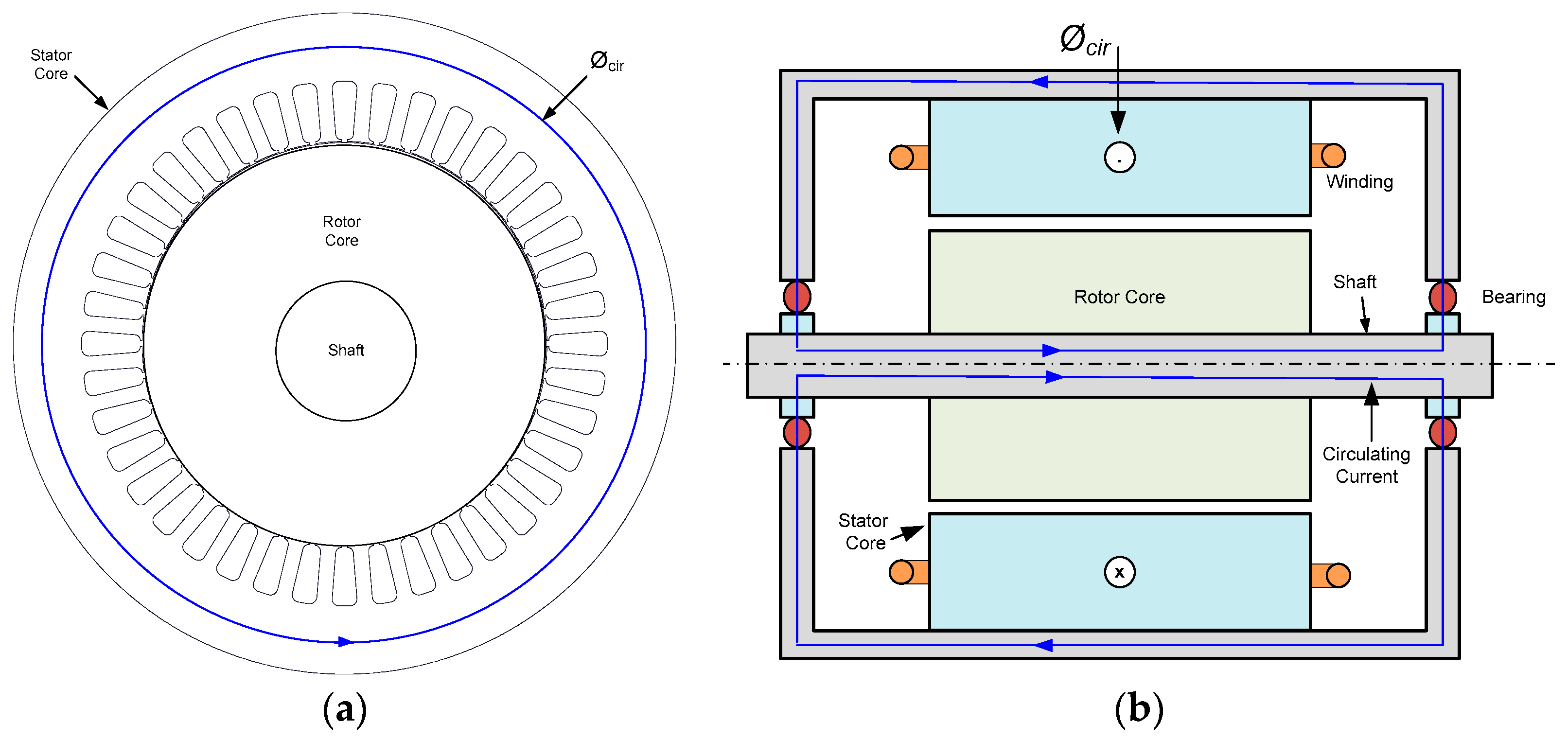
3.3.3. Circulating Bearing Currents
3.3.4. Rotor Ground Currents
4. Measurements of Bearing Current and Shaft Voltage
5. Modelling of Bearing Current
5.1. Analytical Calculation of Machine Capacitances
5.2. Finite Element Modeling and Calculation of EDM Current
5.3. Analytical Calculation of Circulating Current
5.4. Finite Element Modeling of the Circulating Current
5.5. Combined Model of EDM and Circulating Current
6. Mitigation of Bearing Current
6.1. Mitigation through the Motor
6.2. Mitigation through the Connection between the Motor and the Inverter
6.3. Mitigation through the Inverter
6.4. Mitigation Using Multiphase Systems
7. Conclusions
Author Contributions
Funding
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Alger, P.L.; Samson, H.W. Shaft currents in electric machines. J. Am. Inst. Electr. Eng. 1923, 42, 1325–1334. [Google Scholar] [CrossRef]
- Jaritz, M.; Jaeger, C.; Bucher, M.; Smajic, J.; Vukovic, D.; Blume, S. An Improved Model for Circulating Bearing Currents in Inverter-Fed AC Machines. In Proceedings of the 2019 IEEE International Conference on Industrial Technology (ICIT), Melbourne, VIC, Australia, 13–15 February 2019; pp. 225–230. [Google Scholar] [CrossRef]
- Alger, P.L.; Samson, H.W. Shaft Currents in Electric Machines. Trans. Am. Inst. Electr. Eng. 1924, 43, 235–245. [Google Scholar] [CrossRef]
- Kalaiselvi, J.; Srinivas, S. Bearing Currents and Shaft Voltage Reduction in Dual-Inverter-Fed Open-End Winding Induction Motor with Reduced CMV PWM Methods. IEEE Trans. Ind. Electron. 2015, 62, 144–152. [Google Scholar] [CrossRef]
- Qiu, J.; He, Y.; Xu, L.; Jiao, Q. A Novel SVPWM Technique for Leakage Current Reduction and Neutral-Point Voltage Balance in Transformer less Three-Level Inverters. In Proceedings of the 2020 4th International Conference on HVDC (HVDC), Xi’an, China, 6–9 November 2020; pp. 560–565. [Google Scholar] [CrossRef]
- Knebusch, B.; Junemann, L.; Holtje, P.; Mertens, A.; Ponick, B. Measurement Principle for Measuring High Frequency Bearing Currents in Electric Machines and Drive Systems. In Proceedings of the 2022 24th European Conference on Power Electronics and Applications (EPE’22 ECCE Europe), Hanover, Germany, 5–9 September 2022; pp. 1–9. [Google Scholar]
- Han, D.; Li, S.; Lee, W.; Choi, W.; Sarlioglu, B. Trade-off between switching loss and common mode EMI generation of GaN devices analysis and solution. In Proceedings of the 2017 IEEE Applied Power Electronics Conference and Exposition (APEC), Tampa, FL, USA, 26–30 March 2017; pp. 843–847. [Google Scholar]
- Tang, H.; Dai, H.-L.; Du, Y. Bearing Fault Detection for Doubly fed Induction Generator Based on Stator Current. IEEE Trans. Ind. Electron. 2022, 69, 5267–5276. [Google Scholar] [CrossRef]
- Êvo, M.T.; Alzamora, A.M.; Zaparoli, I.O.; de Paula, H. Inverter-Induced Bearing Currents: A Thorough Study of the Cause-and-Effect Chains. In IEEE Industry Applications Magazine; IEEE: New York, NY, USA, 2023; Volume 29, pp. 57–66. [Google Scholar] [CrossRef]
- Gaetano, D.D.; Zhu, W.; Sun, X.; Chen, X.; Griffo, A.; Jewell, G.W. Experimental Ball Bearing Impedance Analysis under Different Speed and Electrical Conditions. In IEEE Transactions on Dielectrics and Electrical Insulation; IEEE: Piscataway, NJ, USA, 2023. [Google Scholar] [CrossRef]
- Im, J.-H.; Kang, J.-K.; Lee, Y.-K.; Hur, J. Shaft Voltage Elimination Method to Reduce Bearing Faults in Dual Three-Phase Motor. IEEE Access 2022, 10, 81042–81053. [Google Scholar] [CrossRef]
- Wang, F. Motor shaft voltages and bearing currents and their reduction in multilevel medium-voltage PWM voltage-source-inverter drive applications. IEEE Trans. Ind. Appl. 2000, 36, 1336–1341. [Google Scholar] [CrossRef]
- Plazenet, T.; Boileau, T.; Caironi, C.; Nahid-Mobarakeh, B. An overview of shaft voltages and bearing currents in rotating machines. In Proceedings of the 2016 IEEE Industry Applications Society Annual Meeting, Portland, OR, USA, 2–6 October 2016; pp. 1–8. [Google Scholar] [CrossRef]
- Gurpinar, E.; Iannuzzo, F.; Yang, Y.; Castellazzi, A.; Blaabjerg, F. Design of low-inductance switching power cell for GaN HEMT based inverter. IEEE Trans. Ind. Appl. 2018, 54, 1592–1601. [Google Scholar] [CrossRef]
- Akagi, H.; Tamura, S. A passive EMI filter for eliminating both bearing current and ground leakage current from an inverter-driven motor. IEEE Trans. Power Electron. 2006, 21, 1459–1469. [Google Scholar] [CrossRef]
- IEC TS 60034-25:2014; Rotating Electrical Machines—Part 25: AC Electrical Machines Used in Power Drive Systems—Application Guide. IEC: Geneva, Switzerland, 2014.
- Mechlinski, M.; Schröder, S.; Shen, J.; Doncker, R.W.D. Grounding concept and common-mode filter design methodology for transformerless MV drives to prevent bearing current issues. IEEE Trans. Ind. Appl. 2017, 53, 5393–5404. [Google Scholar] [CrossRef]
- Jung, J.; Park, Y.; Lee, S.B.; Cho, C.H.; Kim, K.; Wiedenbrug, E.J.; Teska, M. Monitoring Journal-Bearing Faults: Making Use of Motor Current Signature Analysis for Induction Motors. IEEE Ind. Appl. Mag. 2017, 23, 12–21. [Google Scholar] [CrossRef]
- Liu, Z.; Wang, P.; Sun, W.; Shen, Z.; Jiang, D. Sawtooth Carrier-Based PWM Methods with Common-Mode Voltage Reduction for Symmetrical Multiphase Two-Level Inverters with Odd Phase Number. IEEE Trans. Power Electron. 2021, 36, 1171–1183. [Google Scholar] [CrossRef]
- Ding, L.; Li, Y.W. Simultaneous DC Current Balance and CMV Reduction for Parallel CSC System with Interleaved Carrier-Based SPWM. IEEE Trans. Ind. Electron. 2020, 67, 8495–8505. [Google Scholar] [CrossRef]
- Xu, Y.; Wang, Z.; Li, C.; He, J. Common-Mode Voltage Reduction and Fault-Tolerant Operation of Four-Leg CSI-Fed Motor Drives. IEEE Trans. Power Electron. 2021, 36, 8570–8574. [Google Scholar] [CrossRef]
- Costello, M.J. Shaft voltages and rotating machinery. IEEE Trans. Ind. Appl. 1993, 29, 419–426. [Google Scholar] [CrossRef]
- Macdonald, D.; Gray, W. A practical guide to understanding bearing damage related to PWM drives. In Proceedings of the Conference Record of 1998 Annual Pulp and Paper Industry Technical Conference (Cat. No.98CH36219), Portland, ME, USA, 21–26 June 1998; pp. 159–165. [Google Scholar] [CrossRef]
- Bjekic, M.; Stojanovic, D.; Rosic, M.; Bozic, M. Analysis of mitigation techniques for bearing currents in PWM inverter drives. Metal. Int. 2013, 18, 91–97. [Google Scholar]
- von Jouanne, A.; Zhang, H.; Wallace, A.K. An evaluation of mitigation techniques for bearing currents, EMI and overvoltages in ASD applications. IEEE Trans. Ind. Appl. 1998, 34, 1113–1122. [Google Scholar] [CrossRef]
- Das, A.; Ray, S. A Review on Diagnostic Techniques of Bearing Fault and its modeling in Induction Motor. In Proceedings of the 2020 IEEE Calcutta Conference (CALCON), Kolkata, India, 28–29 February 2020; pp. 502–505. [Google Scholar] [CrossRef]
- Yang, L.; Wang, S.; Feng, J. Electromagnetic interference modeling and suppression techniques in variable-frequency drive systems. Front. Mech. Eng. 2018, 13, 329–353. [Google Scholar] [CrossRef]
- Hadden, T.; Jiang, J.W.; Bilgin, B.; Yang, Y.; Sathyan, A.; Dadkhah, H.; Emadi, A. A Review of Shaft Voltages and Bearing Currents in EV and HEV Motors. In Proceedings of the IECON 2016—42nd Annual Conference of the IEEE Industrial Electronics Society, Florence, Italy, 23–26 October 2016; pp. 1578–1583. [Google Scholar] [CrossRef]
- Busse, D.; Erdman, J.; Kerkman, R.; Schlegel, D.; Skibinski, G. Characteristics of shaft voltage and bearing currents. IEEE Ind. Appl. Mag. 1997, 3, 21–32. [Google Scholar] [CrossRef]
- Zhu, W.; De Gaetano, D.; Chen, X.; Jewell, G.W.; Hu, Y. A Review of Modeling and Mitigation Techniques for Bearing Currents in Electrical Machines with Variable-Frequency Drives. IEEE Access 2022, 10, 125279–125297. [Google Scholar] [CrossRef]
- Tong, W. Mechanical Design of Electric Motors, 1st ed.; CRC Press: Boca Raton, FL, USA, 2014. [Google Scholar]
- Venkataraman, B.; Godsey, B.; Premerlani, W.; Shulman, E.; Thakur, M.; Midence, R. Fundamentals of a motor thermal model and its applications in motor protection. In Proceedings of the 58th Annual Conference for Protective Relay Engineers, College Station, TX, USA, 30 March–1 April 2005; pp. 127–144. [Google Scholar]
- Motor Reliability Working Group. Report of large motor reliability survey of industrial and commercial installations, Part I. IEEE Trans. Ind. Appl. 1985, 21, 853–864. [Google Scholar]
- Motor Reliability Working Group. Report of large motor reliability survey of industrial and commercial installations, Part II. IEEE Trans. Ind. Appl. 1985, 21, 865–872. [Google Scholar]
- Motor Reliability Working Group. Report of large motor reliability survey of industrial and commercial installations, Part III. IEEE Trans. Ind. Appl. 1987, 23, 153–158. [Google Scholar]
- Cornell, E.P.; Owen, E.L.; Appiarius, J.C.; McCoy, R.M.; Albrecht, P.F.; Houghtaling, D.W. Improved motors for utility applications. In Final Report; General Electric Co.: Palo Alto, CA, USA, 1982. [Google Scholar]
- Thorsen, O.V.; Dalva, M. A Survey of Faults on Induction Motors in Offshore Oil Industry, Petrochemical Industry, Gas Terminals, and Oil Refineries. IEEE Trans. Ind. Appl. 1994, 31, 1186–1196. [Google Scholar] [CrossRef]
- Thorsen, O.V.; Dalva, M. Failure identification and analysis for high voltage induction motors in petrochemical industry. In Proceedings of the Conference Record of 1998 IEEE Industry Applications Conference. Thirty-Third IAS Annual Meeting (Cat. No.98CH36242), St. Louis, MO, USA, 12–15 October 1998; Volume 1, pp. 291–298. [Google Scholar] [CrossRef]
- Schiferl, R.F.; Melfi, M.J. Bearing current remediation options. IEEE Ind. Appl. Mag. 2004, 10, 40–50. [Google Scholar] [CrossRef]
- Hussain, A.; Agarwal, P. Comparative analysis of Multi-level Inverter topologies for Bearing current in Induction motor. In Proceedings of the IEEE IAS Global Conference on Renewable Energy and Hydrogen Technologies (GlobConHT), Male, Maldives, 11–12 March 2023; pp. 1–6. [Google Scholar] [CrossRef]
- Shami, U.T.; Akagi, H. Mechanism of shaft end-to-end voltage appearing in an inverter-driven motor. In Proceedings of the 2009 International Conference on Electrical Machines and Systems, Tokyo, Japan, 15 November 2009; pp. 1–6. [Google Scholar] [CrossRef]
- Park, J.-K.; Jeong, C.-L.; Bianchi, N.; Hur, J. Frame-to-Shaft Voltage and End-to-End Shaft Voltage Analysis According to Eccentricity in IPMSMs. In Proceedings of the 2018 IEEE Energy Conversion Congress and Exposition (ECCE), Portland, OR, USA, 23–27 September 2018; pp. 3255–3262. [Google Scholar] [CrossRef]
- Anagha, E.R.; Nisha, P.V.; Sindhu, T.K. Design of an active EMI filter for bearing current elimination in VFD. In Proceedings of the 2018 IEEE International Symposium on Electromagnetic Compatibility and 2018 IEEE Asia-Pacific Symposium on Electromagnetic Compatibility (EMC/APEMC), Suntec City, Singapore, 14–18 May 2018; pp. 131–134. [Google Scholar] [CrossRef]
- Turzynski, M.; Chrzan, P.J. Reducing Common-Mode Voltage and Bearing Currents in Quasi-Resonant DC-Link Inverter. IEEE Trans. Power Electron. 2020, 35, 9553–9562. [Google Scholar] [CrossRef]
- Quabeck, S.; Grau, V.; De Doncker, R.W. Modeling and Mitigation of Bearing Currents in Electrical Traction Drives. In Proceedings of the 2020 23rd International Conference on Electrical Machines and Systems (ICEMS), Hamamatsu, Japan, 24–27 November 2020; pp. 1101–1106. [Google Scholar] [CrossRef]
- Shami, U.T.; Akagi, H. Identification and Discussion of the Origin of a Shaft End-to-End Voltage in an Inverter-Driven Motor. IEEE Trans. Power Electron. 2010, 25, 1615–1625. [Google Scholar] [CrossRef]
- Eull, M.; Preindl, M. A Soft Switching Inverter Minimizing Bearing Currents in 800V Electric Vehicle Drives. In Proceedings of the 2021 IEEE 13th International Symposium on Diagnostics for Electrical Machines, Power Electronics and Drives (SDEMPED), Dallas, TX, USA, 22–25 August 2021; pp. 457–463. [Google Scholar] [CrossRef]
- Collin, R.; Yokochi, A.; von Jouanne, A. EDM Damage Assessment and Lifetime Prediction of Motor Bearings Driven by PWM Inverters. In Proceedings of the 2022 IEEE Energy Conversion Congress and Exposition (ECCE), Detroit, MI, USA, 9–13 October 2022; pp. 1–6. [Google Scholar] [CrossRef]
- Raymond Ong, K.J. An Investigation of Shaft Current in a Large SLEEVE bearing Induction Machine. Ph.D. Thesis, McMaster University, Hamilton, Canada, 1999. [Google Scholar]
- Muetze, A.; Binder, A. Practical rules for assessment of inverter induced bearing currents in inverter-fed AC motors up to 500 kW. IEEE Trans. Ind. Electron. 2007, 54, 1614–1622. [Google Scholar] [CrossRef]
- Sakaidani, Y.; Kondo, M. Bearing Fault Detection for Railway Traction Motors through Leakage Current. In Proceedings of the 2018 XIII International Conference on Electrical Machines (ICEM), Alexandroupoli, Greece, 3–6 September 2018; pp. 1768–1774. [Google Scholar] [CrossRef]
- Krein, P.T. Electrostatic discharge issues in electric vehicles. IEEE Trans. Ind. Appl. 1996, 32, 1278–1284. [Google Scholar] [CrossRef]
- He, F.; Xie, G.; Luo, J. Electrical bearing failures in electric vehicles. Friction 2020, 8, 4–28. [Google Scholar] [CrossRef]
- Abu-Rub, H.; Bayhan, S.; Moinoddin, S.; Malinowski, M.; Guzinski, J. Medium-voltage drives: Challenges and existing technology. IEEE Power Electron. Mag. 2016, 3, 29–41. [Google Scholar] [CrossRef]
- Weicker, M.; Binder, A. Characteristic Parameters for Electrical Bearing Damage. In Proceedings of the 2022 International Symposium on Power Electronics, Electrical Drives, Automation and Motion (SPEEDAM), Sorrento, Italy, 22–24 June 2022; pp. 785–790. [Google Scholar] [CrossRef]
- Alcaide, A.M.; Wang, X.; Yan, H.; Leon, J.I.; Monopoli, V.G.; Buticchi, G.; Vazquez, S.; Liserre, M.; Franquelo, L.G. Common-Mode Voltage Mitigation of Dual Three-Phase Voltage Source Inverters in a Motor Drive Application. IEEE Access 2021, 9, 67477–67487. [Google Scholar] [CrossRef]
- Kumar, M.; Jayaraman, K. Design of a Modified Single-Stage and Multistage EMI Filter to Attenuate Common-Mode and Differential-Mode Noises in SiC Inverter. IEEE J. Emerg. Sel. Top. Power Electron. 2022, 10, 4290–4302. [Google Scholar] [CrossRef]
- Arora, T.G.; Renge, M.M.; Aware, M.V. Effects of switching frequency and motor speed on common mode voltage, common mode current and shaft voltage in PWM inverter-fed induction motors. In Proceedings of the 2017 12th IEEE Conference on Industrial Electronics and Applications (ICIEA), Siem Reap, Cambodia, 18–20 June 2017; pp. 583–588. [Google Scholar] [CrossRef]
- Heino, T. Bearing Currents and Their Mitigation in Frequency Converter-Driven Induction Motors. Bachelor’s Thesis, Novia University of Applied Sciences, Vasa, Finland, 2014. [Google Scholar]
- Chen, S.; Lipo, T.A.; Fitzgerald, D. Source of induction motor bearing currents caused by PWM inverters. IEEE Trans. Energy Convers. 1996, 11, 25–32. [Google Scholar] [CrossRef]
- Lai, Y.S.; Chen, P.S.; Lee, H.K.; Chou, J. Optimal common-mode voltage reduction PWM technique for inverter control with consideration of the dead-time effects-part II: Applications to IM drives with diode front end. IEEE Trans. Ind. Appl. 2004, 40, 1613–1620. [Google Scholar] [CrossRef]
- Muetze, A. Bearing Currents in Inverter-Fed AC-Motors. Ph.D. Thesis, Technische Universität Darmstadt: Darmstadt, Germany, 2004. [Google Scholar]
- Muetze, A.; Tamminen, J.; Ahola, J. Influence of motor operating parameters on discharge bearing current activity. IEEE Trans. Ind. Appl. 2011, 47, 1767–1777. [Google Scholar] [CrossRef]
- Erdman, J.; Kerkman, R.J.; Schlegel, D.; Skibinski, G. Effect of PWM inverters on AC motor bearing currents and shaft voltages. In Proceedings of the 1995 IEEE Applied Power Electronics Conference and Exposition—APEC’95, Dallas, TX, USA, 5–9 March 1995; Volume 1, pp. 24–33. [Google Scholar] [CrossRef]
- Dahl, D.; Sosnowski, D.; Schlegel, D.; Kerkman, R.J.; Pennings, M. Field Experience Identifying Electrically Induced Bearing Failures. In Proceedings of the Conference Record of 2007 Annual Pulp and Paper Industry Technical Conference, Williamsburg, VA, USA, 24–28 June 2007; pp. 155–163. [Google Scholar] [CrossRef]
- Rendusara, D.; Enjeti, P. A method to reduce common mode and differential mode dv/dt at the motor terminals in PWM rectifier/PWM inverter type adjustable speed drive systems. In Proceedings of the APEC ‘98 Thirteenth Annual Applied Power Electronics Conference and Exposition, Anaheim, CA, USA, 15–19 February 1998; Volume 2, pp. 1010–1016. [Google Scholar] [CrossRef]
- Tawfiq, K.B.; Mansour, A.S.; Sergeant, P. Mathematical Design and Analysis of Three-Phase Inverters: Different Wide Bandgap Semiconductor Technologies and DC-Link Capacitor Selection. Mathematics 2023, 11, 2137. [Google Scholar] [CrossRef]
- Gao, W.; Zhang, X. The output shaft voltage analysis of an inverter under wide-range input voltages in electric vehicles (EV). In Proceedings of the 2009 IEEE 6th International Power Electronics and Motion Control Conference, Wuhan, China, 17–20 May 2009; pp. 1587–1592. [Google Scholar] [CrossRef]
- Esmaeli, A.; Sun, Y.; Sun, L. Mitigation of the adverse effects of PWM inverter through active filter technique. In Proceedings of the 2006 1st International Symposium on Systems and Control in Aerospace and Astronautics, Harbin, China, 19–21 January 2006; pp. 5–774. [Google Scholar] [CrossRef]
- Sun, Y.; Esmaeli, A.; Sun, L. A New Method to Mitigate the Adverse Effects of PWM Inverter. In Proceedings of the 2006 1st IEEE Conference on Industrial Electronics and Applications, Singapore, 24–26 May 2006; pp. 1–4. [Google Scholar] [CrossRef]
- Wang, F. Motor shaft voltages and bearing currents and their reduction in multi-level medium voltage PWM voltage source inverter drive applications. In Proceedings of the Conference Record of the 1999 IEEE Industry Applications Conference. Thirty-Forth IAS Annual Meeting (Cat. No.99CH36370), Phoenix, AZ, USA, 3–7 October 1999; pp. 1602–1607. [Google Scholar] [CrossRef]
- Chen, S.; Lipo, T.A.; Fitzgerald, D. Modeling of motor bearing currents in PWM inverter drives. IEEE Trans. Ind. Appl. 1996, 32, 1365–1370. [Google Scholar] [CrossRef]
- Magdun, O.; Gemeinder, Y.; Binder, A. Investigation of influence of bearing load and bearing temperature on EDM bearing currents. In Proceedings of the 2010 IEEE Energy Conversion Congress and Exposition, Atlanta, GA, USA, 12–16 September 2010; pp. 2733–2738. [Google Scholar] [CrossRef]
- Muetze, A.; Binder, A. Calculation of motor capacitances for prediction of the voltage across the bearings in machines of inverter-based drive systems. IEEE Trans. Ind. Appl. 2007, 43, 665–672. [Google Scholar] [CrossRef]
- Busse, D.; Erdman, J.; Kerkman, R.J.; Schlegel, D.; Skibinski, G. Bearing currents and their relationship to PWM drives. IEEE Trans. Power Electron. 1997, 12, 243–252. [Google Scholar] [CrossRef]
- Abu-Rub, H.; Malinowski, M.; Al-Haddad, K. Common-Mode Voltage and Bearing Currents in PWM Inverters: Causes, Effects and Prevention. In Power Electronics for Renewable Energy Systems, Transportation and Industrial Applications; IEEE: Piscataway, NJ, USA, 2014; pp. 664–694. [Google Scholar] [CrossRef]
- Oh, H.W.; Willwerth, A.H. New motor design with conductive micro fiber shaft grounding ring prevents bearing failure in PWE inverter driven motors. In Proceedings of the 2007 Electrical Insulation Conference and Electrical Manufacturing Expo, Nashville, TN, USA, 22–24 October 2007; pp. 240–246. [Google Scholar] [CrossRef]
- Chambers, D.; von Jouanne, A.; Wallace, A.; Dai, S.; Baker, R.H. Eliminate the adverse effects of PWM operation [in AC motor drives]. In Proceedings of the Conference Record of the 2001 IEEE Industry Applications Conference, 36th IAS Annual Meeting (Cat. No.01CH37248), Chicago, IL, USA, 30 September–4 October 2001; Volume 3, pp. 2033–2040. [Google Scholar] [CrossRef]
- Adabi, J.; Zare, F.; Ledwich, G.; Ghosh, A.; Lorenz, R.D. Bearing damage analysis by calculation of capacitive coupling between inner and outer races of a ball bearing. In Proceedings of the 2008 13th International Power Electronics and Motion Control Conference, Poznan, Poland, 1–3 September 2008; pp. 903–907. [Google Scholar] [CrossRef]
- Mäki-Ontto, P. Modeling and Reduction of Shaft Voltages in AC Motors Fed by Frequency Converters. Ph.D. Thesis, Helsinki University of Technology, Helsinki, Finland, 2006. [Google Scholar]
- Plazenet, T.; Boileau, T.; Caironi, C.; Nahid-Mobarakeh, B. A Comprehensive Study on Shaft Voltages and Bearing Currents in Rotating Machines. IEEE Trans. Ind. Appl. 2018, 54, 3749–3759. [Google Scholar] [CrossRef]
- IEEE Standard 112-1996; IEEE Standard Test procedure for Polyphase Induction Motors and Generators. IEEE: Piscataway, NJ, USA, 2004.
- Chen, S.; Lipo, T.A. Circulating type motor bearing current in inverter drives. IEEE Ind. Appl. Mag. 1998, 4, 32–38. [Google Scholar] [CrossRef]
- Fan, F.; See, K.Y.; Banda, J.K.; Liu, X.; Gupta, A.K. Investigation and mitigation of premature bearing degradation in motor drive system. IEEE Electromagn. Compat. Mag. 2019, 8, 75–81. [Google Scholar] [CrossRef]
- Zheng, J.; Xiang, D.; Li, H.; Quach, D.-C. An Investigation into the Effect of Bearing Grease Degradation on the High-frequency $dv/dt$ Bearing Current in an Inverter-fed Motor System. In Proceedings of the 2021 6th International Conference on Power and Renewable Energy (ICPRE), Shanghai, China, 17–20 September 2021; pp. 543–547. [Google Scholar] [CrossRef]
- Devaney, M.J.; Eren, L. Detecting motor bearing faults. IEEE Instrum. Meas. Mag. 2004, 7, 30–50. [Google Scholar] [CrossRef]
- Jaeger, C.; Grinbaum, I.; Smajic, J. Numerical simulation and measurement of common-mode and circulating bearing currents. In Proceedings of the 2016 XXII International Conference on Electrical Machines (ICEM), Lausanne, Switzerland, 4–7 September 2016; pp. 486–491. [Google Scholar] [CrossRef]
- Muetze, A.; Binder, A.; Vogel, H.; Hering, J. Experimental evaluation of the endangerment of ball bearings due to inverter-induced bearing currents. In Proceedings of the 39th IAS Annual Meeting Conference Record of the 2004 IEEE Industry Applications Conference, Seattle, WA, USA, 3–7 October 2004; pp. 1989–1995. [Google Scholar]
- Shaft Grounding Rings, Electro-Static-Technology; Datasheet: Mechanic Falls, ME, USA, 2007.
- Sarkimaki, V. Radio Frequency Measurement Method for Detecting Bearing Currents in Induction Motors. Ph.D. Thesis, Lappeenranta-Lahti University of Technology, Lappeenranta, Finland, 2009. [Google Scholar]
- Ahola, J.; Niskanen, V.; Muetze, A. On the role of the shaft end in the radio-frequency emission of discharge bearing currents in induction motors. In Proceedings of the 2011 14th European Conference on Power Electronics and Applications, Birmingham, UK, 30 August–1 September 2011; pp. 1–10. [Google Scholar]
- Ahola, J.; Sarkimaki, V.; Muetze, A.; Tamminen, J. Radio-frequency based detection of electrical discharge machining bearing currents. IET Elect. Power Appl. 2011, 5, 386–392. [Google Scholar] [CrossRef]
- EDD Pen TKED1, MP5355E-1, SKF; Datasheet: Gothenburg, Sweden, 2008.
- Naik, R.; Nondahl, T.A.; Mel, M.J.; Schiferl, R.; Wang, J.-S. Distributed parameter circuit model for shaft voltage prediction in induction motors fed by PWM based AC drives. In Proceedings of the Conference Record of the 2001 IEEE Industry Applications Conference, 36th IAS Annual Meeting, Chicago, IL, USA, 30 September–4 October 2001; pp. 1118–1122. [Google Scholar]
- Naik, R.; Nondahl, T.A.; Mel, M.J.; Schiferl, R.; Wang, J.-S. Circuit model for shaft voltage prediction in induction motors fed by PWM based AC drives. IEEE Trans. Ind. Appl. 2003, 39, 1294–1299. [Google Scholar] [CrossRef]
- Vidmar, G.; Miljavec, D. A universal high-frequency three-phase electric-motor model suitable for the delta- and star-winding connections. IEEE Trans. Power Electron. 2015, 30, 4365–4376. [Google Scholar] [CrossRef]
- Ito, K.; Sato, Y.; Zanma, T.; Doki, S.; Ishida, M. Identification of the parameters of the high-frequency equivalent circuit of PM synchronous motor based on genetic algorithm. Electr. Eng. Jpn. 2009, 167, 57–66. [Google Scholar] [CrossRef]
- Jia, K.; Bohlin, G.; Enohnyaket, M.; Thottappillil, R. Modelling an AC motor with high accuracy in a wide frequency range. Electr. Power Appl. IET 2013, 7, 116–122. [Google Scholar] [CrossRef]
- Boglietti, A.; Cavagnino, A.; Lazzari, M. Experimental High-Frequency Parameter Identification of AC Electrical Motors. IEEE Trans. Ind. Appl. 2007, 43, 23–29. [Google Scholar] [CrossRef]
- Magdun, O.; Binder, A. High-Frequency Induction Machine Modeling for Common Mode Current and Bearing Voltage Calculation. IEEE Trans. Ind. Appl. 2014, 50, 1780–1790. [Google Scholar] [CrossRef]
- Muetze, A.; Binder, A. Calculation of circulating bearing currents in machines of inverter-based drive systems. IEEE Trans. Ind. Electron. 2007, 54, 932–938. [Google Scholar] [CrossRef]
- Erdman, J.M.; Kerkman, R.J.; Schlegel, D.W.; Skibinski, G.L. Effect of PWM inverters on AC motor bearing currents and shaft voltages. IEEE Trans. Ind. Appl. 1996, 32, 250–259. [Google Scholar] [CrossRef]
- Schuster, M.; Springer, J.; Binder, A. Comparison of a 1.1 kW-induction machine and a 1.5 kW-PMSM regarding common-mode bearing currents. In Proceedings of the 2018 International Symposium on Power Electronics, Electrical Drives, Automation and Motion (SPEEDAM), Amalfi, Italy, 20–22 June 2018; pp. 1–6. [Google Scholar] [CrossRef]
- Êvo, M.T.A.; Paula, H. Electrostatic shielding for bearings discharge currents attenuation: Analysis of its effectiveness, losses and impact on the motor performance-A study for design guidelines. IET Electr. Power Appl. 2020, 14, 1050–1059. [Google Scholar]
- Liu, Y.; Cao, J.; Song, Y.; Xu, G.; Li, L. Research on bearing current detection method of high-speed motor driven by PWM inverter. In Proceedings of the 2019 22nd International Conference on Electrical Machines and Systems (ICEMS), Harbin, China, 11–14 August 2019; pp. 1–6. [Google Scholar]
- Kindl, V.; Skala, B.; Pechanek, R.; Byrtus, M.; Hruska, K. Calculation of induction machine parasitic capacitances using finite element method. In Proceedings of the ELEKTRO, Strbske Pleso, Slovakia, 16–18 May 2016; pp. 176–179. [Google Scholar]
- Li, J.; Liu, R.; Zheng, B.; Zhang, Y.; Soc, I.I.A. The effects of end part of winding on parasitic capacitances of induction motor fed by PWM inverter. In Proceedings of the 2012 15th International Conference on Electrical Machines and Systems (ICEMS), Sapporo, Japan, 21–24 October 2012; pp. 1–5. [Google Scholar]
- Maki-Ontto, P.; Kinnunen, H.; Luomi, J. Three-phase model for the circuit simulation of common-mode phenomena and shaft voltages in AC motor drive systems. In Proceedings of the IEEE International Conference on Electric Machines and Drives, San Antonio, TX, USA, 15 May 2005; pp. 437–443. [Google Scholar]
- Mäki-Ontto, P.; Luomi, J. Circumferential flux as a source of bearing current of converter-fed AC machines. In Proceedings of the NORPIE, Stockholm, Sweden, 12–14 August 2002; pp. 12–14. [Google Scholar]
- Mohammed, O.A.; Ganu, S.; Abed, N.; Liu, S.; Liu, Z. High frequency PM synchronous motor model determined by FE analysis. IEEE Trans. Magn. 2006, 42, 1291–1294. [Google Scholar] [CrossRef]
- Magdun, O.; Gemeinder, Y.; Binder, A. Rotor impedance of the high frequency circulating bearing current path in inverter-fed AC machines. In Proceedings of the 2013 IEEE Energy Conversion Congress and Exposition, Denver, CO, USA, 15–19 September 2013; pp. 3512–3519. [Google Scholar]
- Bobon, A.; Zientek, P.; Niestroj, R.; Pasko, M.; Kwak, J. The computational and measurement methods of research on factors affecting the flow of bearing currents in high power induction motors. In Proceedings of the 2016 13th Selected Issues of Electrical Engineering and Electronics (WZEE), Rzeszow, Poland, 4–8 May 2016; pp. 1–6. [Google Scholar]
- Bubert, A.; Zhang, J.; De Doncker, R.W. Modeling and measurement of capacitive and inductive bearing current in electrical machines. In Proceedings of the 2017 Brazilian Power Electronics Conference (COBEP), Juiz de Fora, Brazil, 19–22 November 2017; pp. 1–6. [Google Scholar] [CrossRef]
- Xing, S.; Wu, Z. Characteristic Research of Bearing Currents in Inverter-Motor Drive Systems. In Proceedings of the 2006 CES/IEEE 5th International Power Electronics and Motion Control Conference, Shanghai, China, 14–16 August 2006; pp. 1–4. [Google Scholar] [CrossRef]
- Muetze, A.; Binder, A. Experimental evaluation of mitigation techniques for bearing currents in inverter-supplied drive-systems—Investigations on induction motors up to 500 kW. In Proceedings of the IEEE International Electric Machines and Drives Conference, 2003. IEMDC’03., Madison, WI, USA, 1–4 June 2003; Volume 3, pp. 1859–1865. [Google Scholar] [CrossRef]
- Ke, Z.; Pan, J.; Na, R.; Zhang, F.; Li, H.; Potty, K.; Zhang, J.; Wang, J.; Xu, L. Common Mode Votage Compensation for Capacitor Voltage Ripple Reduction of 7-kV SiC-based Modular Multilevel Converter. In Proceedings of the 2018 1st Workshop on Wide Bandgap Power Devices and Applications in Asia (WiPDA Asia), Xi’an, China, 17–19 May 2018; pp. 1–6. [Google Scholar] [CrossRef]
- Busse, D.F.; Erdman, J.M.; Kerkman, R.J.; Schlegel, D.W.; Skibinski, G.L. An evaluation of the electrostatic shielded induction motor: A solution for rotor shaft voltage buildup and bearing current. IEEE Trans. Ind. Appl. 1997, 33, 1563–1570. [Google Scholar] [CrossRef]
- Bai, B.; Wang, Y.; Wang, X. Suppression for Discharging Bearing Current in Variable-Frequency Motors Based on Electromagnetic Shielding Slot Wedge. IEEE Trans. Magn. 2015, 51, 8109404. [Google Scholar] [CrossRef]
- Maki-Ontto, P.; Luomi, J. Bearing current prevention of converter-fed AC machines with a conductive shielding in stator slots. In Proceedings of the IEEE International Electric Machines and Drives Conference, 2003. IEMDC’03, Madison, WI, USA, 1–4 June 2003; pp. 274–278. [Google Scholar]
- Yea, M.; Han, K.J. Modified Slot Opening for Reducing Shaft-to-Frame Voltage of AC Motors. Energies 2020, 13, 760. [Google Scholar] [CrossRef]
- Park, J.-K.; Wellawatta, T.R.; Choi, S.-J.; Hur, J. Mitigation Method of the Shaft Voltage According to Parasitic Capacitance of the PMSM. IEEE Trans. Ind. Appl. 2017, 53, 4441–4449. [Google Scholar] [CrossRef]
- Maetani, T.; Isomura, Y.; Watanabe, A.; Iimori, K.; Morimoto, S. Suppressing Bearing Voltage in an Inverter-Fed Ungrounded Brushless DC Motor. IEEE Trans. Ind. Electron. 2013, 60, 4861–4868. [Google Scholar] [CrossRef]
- Maetani, T.; Morimoto, S.; Iimori, K.; Isomura, Y.; Watanabe, A. Approaches to suppressing shaft voltage in brushless DC motor driven by PWM inverter. In Proceedings of the 2011 International Conference on Electrical Machines and Systems, Beijing, China, 20–23 August 2011; pp. 1–6. [Google Scholar] [CrossRef]
- Isomura, Y.; Yamamoto, K.; Morimoto, S.; Maetani, T.; Watanabe, A.; Nakano, K. Approaches to suppressing shaft voltage in non-insulated rotor brushless DC motor driven by PWM inverter. In Proceedings of the 2013 International Conference on Electrical Machines and Systems (ICEMS), Busan, Republic of Korea, 26–29 October 2013; pp. 1242–1247. [Google Scholar] [CrossRef]
- Isomura, Y.; Yamamoto, K.; Morimoto, S.; Maetani, T.; Watanabe, A.; Nakano, K. Study of the Further Reduction of Shaft Voltage of Brushless DC Motor with Insulated Rotor Driven by PWM Inverter. IEEE Trans. Ind. Appl. 2014, 50, 3738–3743. [Google Scholar] [CrossRef]
- Kim, K.-T.; Hur, J. Optimization of magnetic flux-path design for reduction of shaft voltage in IPM-type BLDC motor. J. Electr. Eng. Technol. 2014, 9, 2187–2193. [Google Scholar] [CrossRef]
- Muetze, A.; Oh, H.W. Application of static charge dissipation to mitigate electric discharge bearing currents. IEEE Trans. Ind. Appl. 2008, 44, 135–143. [Google Scholar] [CrossRef]
- Muetze, A.; Oh, H.W. Current-Carrying Characteristics of Conductive Microfiber Electrical Contact for High Frequencies and Current Amplitudes: Theory and Applications. IEEE Trans. Power Electron. 2010, 25, 2082–2092. [Google Scholar] [CrossRef]
- Vedde, A.; Neuburger, M.; Cheshire, C.; Gliese, F. Optimization of a Passive Common Mode EMI Filter by Adding an Active Feedback Loop. In Proceedings of the 2021 IEEE Southern Power Electronics Conference (SPEC), Kigali, Rwanda, 6–9 December 2021; pp. 1–6. [Google Scholar] [CrossRef]
- Chen, W.; Yang, X.; Wang, Z. An active EMI filtering technique for improving passive filter low-frequency performance. IEEE Trans. Electromagn. Compat. 2006, 48, 172–177. [Google Scholar] [CrossRef]
- Müller, D.; Schweitzer, D.N.; Beltle, M.; Tenbohlen, S. An Active Common Mode EMI Filter Approach introducing Predictive Pulsed Compensation. In Proceedings of the 2019 International Symposium on Electromagnetic Compatibility—EMC EUROPE, Barcelona, Spain, 2–6 September 2019; pp. 1003–1008. [Google Scholar] [CrossRef]
- Mei, C.; Balda, J.C.; Waite, W.P. Cancellation of common-mode Voltages for induction motor drives using active method. IEEE Trans. Energy Convers. 2006, 21, 380–386. [Google Scholar] [CrossRef]
- Mei, C.; Balda, J.C.; Waite, W.P.; Carr, K. Active cancellation of common-mode voltages on drives rated 460-V and higher. In Proceedings of the IEEE International Electric Machines and Drives Conference, 2003, IEMDC’03, Madison, WI, USA, 1–4 June 2003; Volume 3, pp. 1845–1851. [Google Scholar] [CrossRef]
- Hava, A.M.; Ün, E. Performance Analysis of Reduced Common-Mode Voltage PWM Methods and Comparison with Standard PWM Methods for Three-Phase Voltage-Source Inverters. IEEE Trans. Power Electron. 2009, 24, 241–252. [Google Scholar] [CrossRef]
- Un, E.; Hava, A.M. A Near State PWM Method with Reduced Switching Frequency and Reduced Common Mode Voltage for Three-Phase Voltage Source Inverters. In Proceedings of the 2007 IEEE International Electric Machines & Drives Conference, Antalya, Turkey, 3–5 May 2007; pp. 235–240. [Google Scholar] [CrossRef]
- Cacciato, M.; Consoli, A.; Scarcella, G.; Testa, A. Reduction of common mode currents in PWM inverter motor drives. IAS ‘97. In Proceedings of the Conference Record of the 1997 IEEE Industry Applications Conference Thirty-Second IAS Annual Meeting, New Orleans, LA, USA, 5–9 October 1997; Volume 1, pp. 707–714. [Google Scholar] [CrossRef]
- Ming, W.-L.; Zhong, Q.-C. Synchronverter-based transformerless PV inverters. In Proceedings of the IECON 2014—40th Annual Conference of the IEEE Industrial Electronics Society, Dallas, TX, USA, 29 October–1 November 2014; pp. 4396–4401. [Google Scholar] [CrossRef]
- Guo, X.; Xu, D.; Wu, B. Four-Leg Current-Source Inverter with a New Space Vector Modulation for Common-Mode Voltage Suppression. IEEE Trans. Ind. Electron. 2015, 62, 6003–6007. [Google Scholar] [CrossRef]
- Syed, A.; Kalyani, S.T. Three-phase eight switch inverters with reduced common mode voltage for transformerless photovoltaic systems. In Proceedings of the 2016 International Conference on Electrical, Electronics, and Optimization Techniques (ICEEOT), Chennai, India, 3–5 March 2016; pp. 2840–2844. [Google Scholar] [CrossRef]
- Guo, X.; Xu, D.; Wu, B. Three-phase DC-bypass topologies with reduced leakage current for transformerless PV systems. In Proceedings of the 2015 IEEE Energy Conversion Congress and Exposition (ECCE), Montreal, QC, Canada, 20–24 September 2015; pp. 43–46. [Google Scholar] [CrossRef]
- Guo, X.; Xu, D.; Wu, B. Three-phase seven-switch inverter with common mode voltage reduction for transformerless photovoltaic system. In Proceedings of the IECON 2014—40th Annual Conference of the IEEE Industrial Electronics Society, Dallas, TX, USA, 29 October–1 November 2014; pp. 2279–2284. [Google Scholar] [CrossRef]
- Jeong, W.-S.; Choo, K.-M.; Lee, J.-H.; Won, C.-Y. Space Vector-Based Common-Mode Currents Reduction Method for H8 Inverter Topology in Low-Voltage DC Microgrid. In Proceedings of the 2019 IEEE 4th International Future Energy Electronics Conference (IFEEC), Singapore, 25–28 November 2019; pp. 1–7. [Google Scholar] [CrossRef]
- Jeong, W.-S.; Choo, K.-M.; Lee, J.-H.; Won, C.-Y. A Common-Mode Voltage Reduction Method of FCS-MPC in H8 Inverter for SPMSM Drive System Considering Dead-Time Effect. In Proceedings of the 2019 10th International Conference on Power Electronics and ECCE Asia (ICPE 2019-ECCE Asia), Busan, Republic of Korea, 27–30 May 2019; pp. 1–8. [Google Scholar] [CrossRef]
- Debatal, S.; Roy, T.; Dasgupta, A.; Sadhu, P.K. A Novel Structure of Switched Capacitor Multilevel Inverter with Reduced Device Count. In Proceedings of the 2018 National Power Engineering Conference (NPEC), Madurai, India, 9–10 March 2018; pp. 1–6. [Google Scholar] [CrossRef]
- Agrawal, H.; Gupta, A.K.; Agarwal, V. A novel 3-phase, transformerless H-8 topology with low variation in CMV to reduce leakage current. In Proceedings of the 2016 IEEE International Conference on Power Electronics, Drives and Energy Systems (PEDES), Trivandrum, India, 14–17 December 2016; pp. 1–5. [Google Scholar] [CrossRef]
- Li, J.; Huang, A.; Bhattacharya, S.; Lukic, S. ETO light multilevel converters for large electric vehicle and hybrid electric vehicle drives. In Proceedings of the 2009 IEEE Vehicle Power and Propulsion Conference, Dearborn, MI, USA, 7–10 September 2009; pp. 1455–1460. [Google Scholar] [CrossRef]
- BenAbdelghani, A.; Martins, C.A.; Roboam, X.; Meynard, T.A. Use of extra degrees of freedom in multilevel drives. IEEE Trans. Ind. Electron. 2002, 49, 965–977. [Google Scholar] [CrossRef]
- Choudhury, S.; Nayak, S.; Dash, T.P.; Rout, P.K. A comparative analysis of five level diode clamped and cascaded H-bridge multilevel inverter for harmonics reduction. In Proceedings of the 2018 Technologies for Smart-City Energy Security and Power (ICSESP), Bhubaneswar, India, 28–30 March 2018; pp. 1–6. [Google Scholar] [CrossRef]
- Golwala, H.; Chudamani, R. New Three-Dimensional Space Vector-Based Switching Signal Generation Technique Without Null Vectors and With Reduced Switching Losses for a Grid-Connected Four-Leg Inverter. IEEE Trans. Power Electron. 2016, 31, 1026–1035. [Google Scholar] [CrossRef]
- Karugaba, S.; Ojo, O.; Abreham, M. Carrier based PWM scheme for a three-level diode-clamped five-phase voltage source inverter ensuring capacitor voltage balancing. In Proceedings of the 2011 Twenty-Sixth Annual IEEE Applied Power Electronics Conference and Exposition (APEC), Fort Worth, TX, USA, 6–11 March 2011; pp. 1194–1201. [Google Scholar] [CrossRef]
- Hota, A.; Agarwal, V. A Modified 2-level Three-Phase Inverter Topology with Common Mode Voltage Performance of a 3-level Inverter. In Proceedings of the 2021 International Conference on Sustainable Energy and Future Electric Transportation (SEFET), Hyderabad, India, 21–23 January 2021; pp. 1–6. [Google Scholar] [CrossRef]
- Hota, A.; Agarwal, V. A New Three-Phase Inverter Topology for Reducing the dv/dt and Peak-to-Peak Value of Common Mode Voltage. IEEE Trans. Ind. Electron. 2022, 69, 11979–11986. [Google Scholar] [CrossRef]
- Hota, A.; Agarwal, V. A New H8 Inverter Topology with Low Common Mode Voltage and Phase Current THD for 3-φ Induction Motor Drive Applications. IEEE Trans. Ind. Appl. 2022, 58, 6245–6252. [Google Scholar] [CrossRef]
- Tran, T.-T.; Nguyen, M.-K.; Duong, T.-D.; Lim, Y.-C.; Zare, F. A switched-capacitor-voltage-doubler based boost inverter for common mode voltage reduction. IEEE Access 2019, 7, 98618–98629. [Google Scholar] [CrossRef]
- Duong, T.-D.; Nguyen, M.-K.; Tran, T.-T.; Lim, Y.-C.; Choi, J.-H.; Wang, C. Modulation techniques for a modified three-phase quasi switched boost inverter with common-mode voltage reduction. IEEE Access 2020, 8, 160670–160683. [Google Scholar] [CrossRef]
- Tawfiq, K.B.; Ibrahim, M.N.; EL-Kholy, E.E.; Sergeant, P. Construction of Synchronous Reluctance Machines with Combined Star-Pentagon Configuration Using Standard Three-Phase Stator Frames. IEEE Trans. Ind. Electron. 2022, 69, 7582–7595. [Google Scholar] [CrossRef]
- Tawfiq, K.B.; Ibrahim, M.N.; Sergeant, P. Analysis of Different Rewinding Configurations of Five-phase Synchronous Reluctance Machines. In Proceedings of the 2022 International Conference on Electrical Machines (ICEM), Valencia, Spain, 5–8 September 2022; pp. 455–461. [Google Scholar] [CrossRef]
- Tawfiq, K.B.; Abdou, A.F.; El-Kholy, E.E.; Shokrall, S.S. Application of matrix converter connected to wind energy system. In Proceedings of the IEEE 18th International Middle East Power Systems Conference (MEPCON), Cairo, Egypt, 27–29 December 2016; pp. 604–609. [Google Scholar]
- Tawfiq, K.B.; Ibrahim, M.N.; Rezk, H.; El-Kholy, E.E.; Sergeant, P. Mathematical Modelling, Analysis and Control of a Three to Five-Phase Matrix Converter for Minimal Switching Losses. Mathematics 2021, 9, 96. [Google Scholar] [CrossRef]
- Toliyat, H.; Shi, R.; Xu, H. A DSP-based vector control of five-phase synchronous reluctance motor. In Proceedings of the Conference Record of the 2000 IEEE Industry Applications Conference. Thirty-Fifth IAS Annual Meeting and World Conference on Industrial Applications of Electrical Energy, Rome, Italy, 8–12 October 2000. [Google Scholar]
- Iqbal, A.; Levi, E. Space vector modulation schemes for a five-phase voltage source inverter. In Proceedings of the 2005 European Conference on Power Electronics and Applications, Dresden, Germany, 11–14 September 2005. [Google Scholar]
- Duran, M.; Levi, E. Multi-dimensional approach to multi-phase space vector pulse width modulation. In Proceedings of the IECON 2006—32nd Annual Conference on IEEE Industrial Electronics, Paris, France, 6–10 November 2006. [Google Scholar]
- Ryu, H.-M.; Kim, J.-H.; Sul, S.-K. Analysis of multiphase space vector pulse width modulation based on multiple d-q spaces concept. In Proceedings of the IPEMC Conference, Xi’an, China, 14–16 August 2004. [Google Scholar]
- Xue, S.; Wen, X. Simulation analysis of two novel multiphase SVPWM strategies. In Proceedings of the 2005 IEEE International Conference on Industrial Technology, Hong Kong, 14–17 December 2005. [Google Scholar]
- Hussain, H.A.; Toliyat, H.A. Reduction of Shaft Voltages and Bearing Currents in Five-Phase Induction Motors. Master’s Thesis, Texas A&M University, College Station, TX, USA, 2012. [Google Scholar]






| Failure Mode | IEEE Study | EPRI Study | Average (%) | ||
|---|---|---|---|---|---|
| Failure Contribution | (%) | Failure Component | (%) | ||
| Mechanical failure | High vibration | 15.5 | Sleeve bearings | 16 | |
| Poor lubrication | 15.2 | Antifriction bearings | 8 | ||
| - | - | Trust bearings | 5 | ||
| - | - | Rotor shaft | 2 | ||
| - | - | Rotor core | 1 | ||
| Total | 30.7 | Total | 32 | 31.35 | |
| Electrical failure | Normal deterioration | 26.4 | Stator ground insulation | 23 | |
| Persistent overload | 4.2 | Turn insulation | 4 | ||
| - | - | Bracing | 3 | ||
| - | - | Cage | 5 | ||
| - | - | Core | 1 | ||
| Total | 30.6 | Total | 36 | 33.3 | |
| Environmental and other reasons | High ambient temperature | 3 | Bearing seals | 6 | |
| Abnormal moisture | 5.8 | Oil leakage | 3 | ||
| Abnormal voltage | 1.5 | Frame | 1 | ||
| Abnormal frequency | 0.6 | Wedge | 1 | ||
| Abrasive chemicals | 4.2 | - | - | ||
| Poor ventilation cooling | 3.9 | - | - | ||
| Other reasons | 19.7 | Other components | 21 | ||
| Total | 38.70 | Total | 32.00 | 35.35 | |
| Rogowski Coil | High-Frequency Voltage Probe | Radio Frequency Antenna | |
|---|---|---|---|
| EDM current | There is no detection. | Detection of events with a suitable threshold. | Detection of events with a suitable threshold. |
| Circulating current | This current can be detected if the coil is placed inside the machine. | There was no detection at the industrial stage. Lately, detection began at the research stage. | There was no detection at the industrial stage. Lately, detection began at the research stage. |
| Rotor ground current | This current can be detected if the coil is placed outside the machine. | There was no detection at the industrial stage. Lately, detection began at the research stage. | There was no detection at the industrial stage. Lately, detection began at the research stage. |
| Comment | Extremely intrusive. | Relatively intrusive Sensitive to electromagnetic interference. | Non-intrusive. Sensitive to electromagnetic interference. |
| Topology | Number of Switches | %THD (Ia, Ib, Ic) | Peak to Peak of CMV | dv of the CMV |
|---|---|---|---|---|
| Two-level inverter | 6 | 1.34, 1.34, 1.34 | /3 | |
| Three-level NPC | 12 | 0.62, 0.62, 0.62 | /4 | /6 |
| [138] | 7 | 1.38, 1.38, 1.38 | /3 | /3 |
| [141] | 7 | 1.38, 1.38, 1.38 | /3 | /3 |
| [143] | 8 | 1.34, 1.34, 1.34 | /3 | /3 |
| [140] | 8 | 1.34, 1.34, 1.34 | /3 | /3 |
| [142] | 8 | 1.34, 1.34, 1.34 | /3 | /3 |
| [145] | 8 | 1.38, 1.38, 1.38 | /3 | /3 |
| [144] | 8 | 1.34, 1.34, 1.34 | /3 | /3 |
| [154] | 8 | 1.34, 1.34, 1.34 | /3 | /3 |
| [155] | 8 | 1.87, 1.87, 1.87 | /3 | /3 |
| [152] | 8 | 0.98, 1.33, 1.33 | /3 | /6 |
| 3-Phase System | 5-Phase System with Constant Voltage | 5-Phase System with Constant Voltage | |
|---|---|---|---|
| DC voltage | 1.0 | 1.0 | 0.6 |
| Motor rated voltage | 1.0 | 1.0 | 0.6 |
| Motor rated current | 1.0 | 0.6 | 1.0 |
| Rated speed | 1.0 | 1.0 | 1.0 |
| Peak to peak CMV | 1.0 | 1.0 | 0.6 |
| dv/dt of CMV | 1.0 | 0.6 | 0.36 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Tawfiq, K.B.; Güleç, M.; Sergeant, P. Bearing Current and Shaft Voltage in Electrical Machines: A Comprehensive Research Review. Machines 2023, 11, 550. https://doi.org/10.3390/machines11050550
Tawfiq KB, Güleç M, Sergeant P. Bearing Current and Shaft Voltage in Electrical Machines: A Comprehensive Research Review. Machines. 2023; 11(5):550. https://doi.org/10.3390/machines11050550
Chicago/Turabian StyleTawfiq, Kotb B., Mehmet Güleç, and Peter Sergeant. 2023. "Bearing Current and Shaft Voltage in Electrical Machines: A Comprehensive Research Review" Machines 11, no. 5: 550. https://doi.org/10.3390/machines11050550
APA StyleTawfiq, K. B., Güleç, M., & Sergeant, P. (2023). Bearing Current and Shaft Voltage in Electrical Machines: A Comprehensive Research Review. Machines, 11(5), 550. https://doi.org/10.3390/machines11050550