Detection for Disc Cutter Wear of TBM Using Magnetic Force
Abstract
1. Introduction
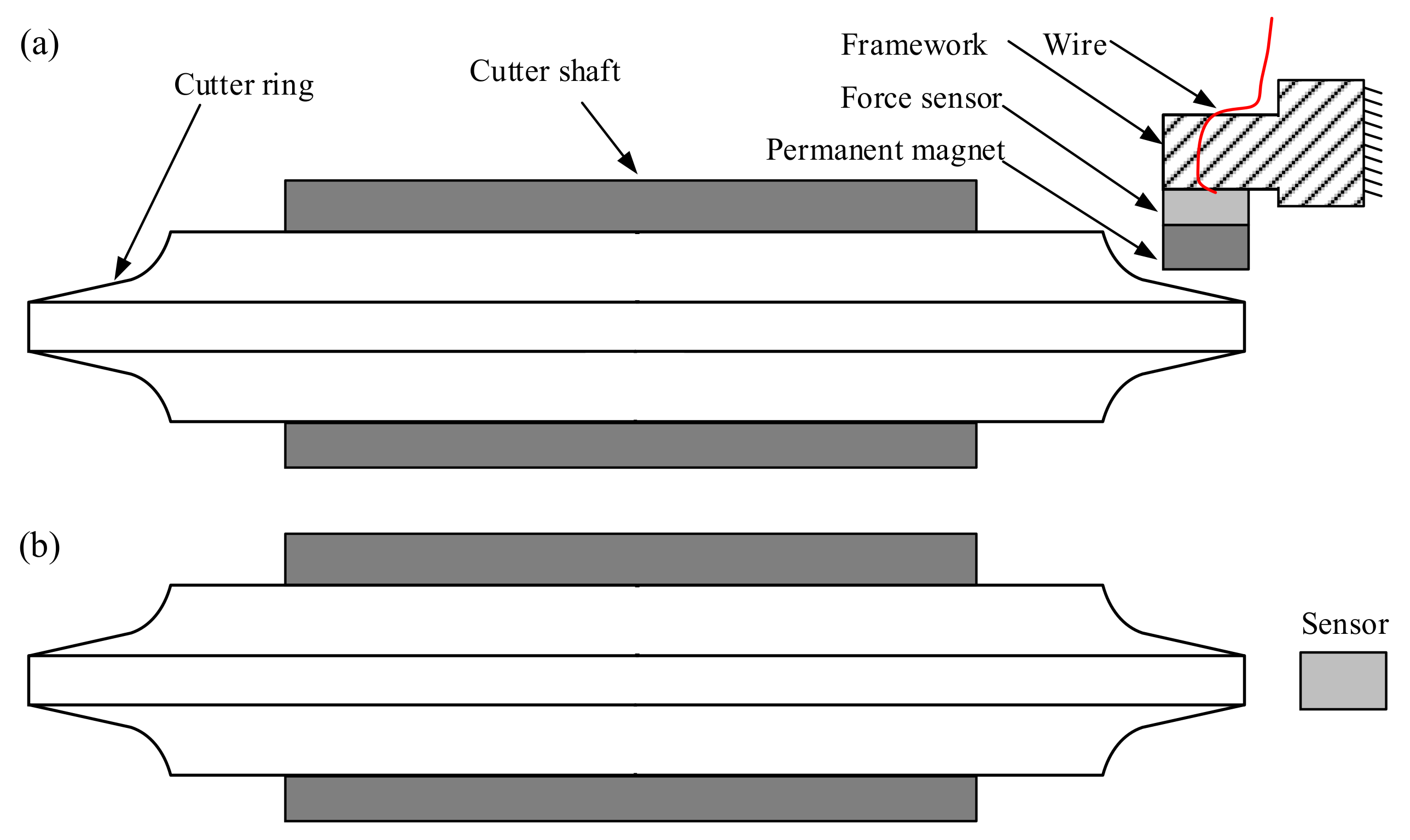
2. Principle and Architecture of the Detecting System
3. Effect of the Placement of the Permanent Magnet on Magnetic Force
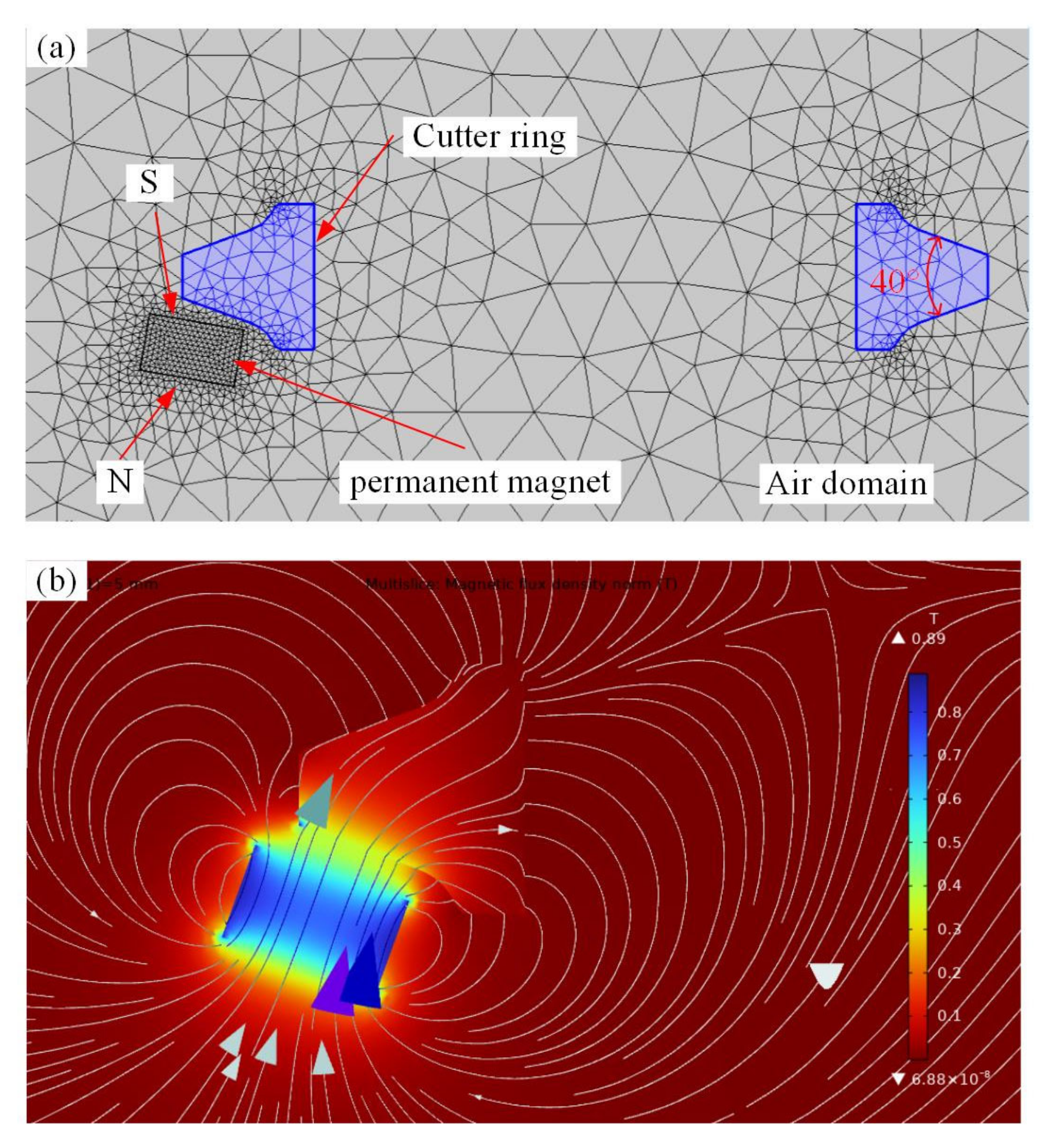
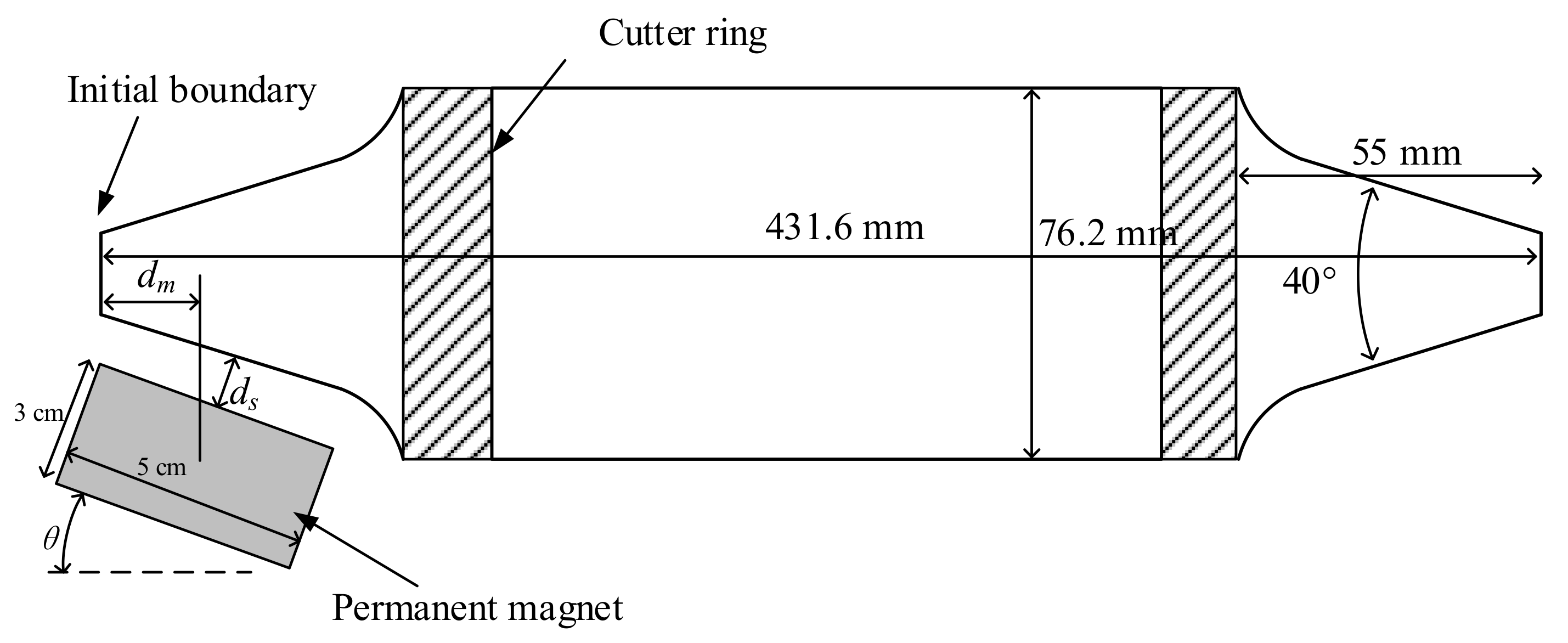
3.1. Finite Element Model
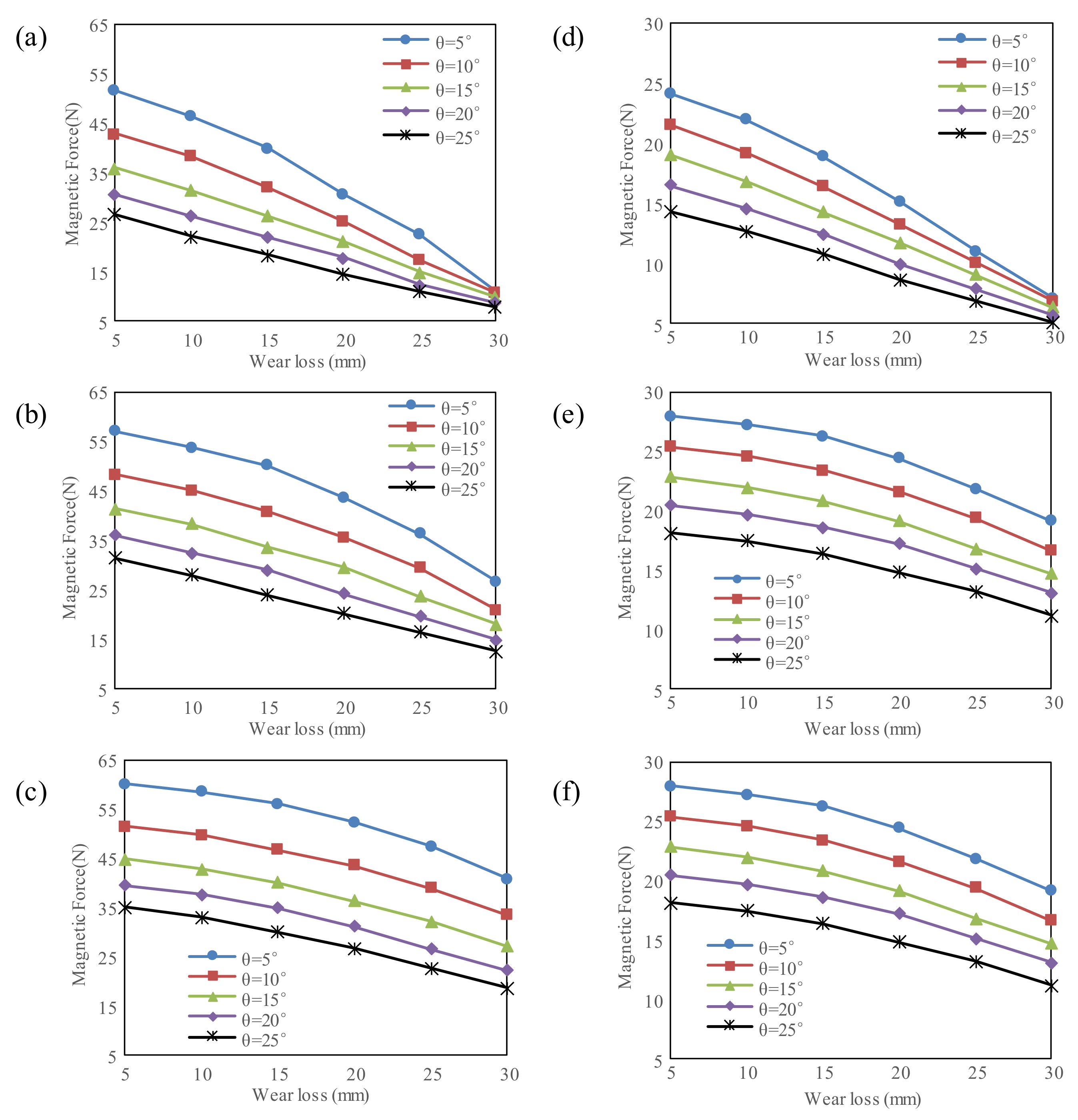
3.2. Simulating Results
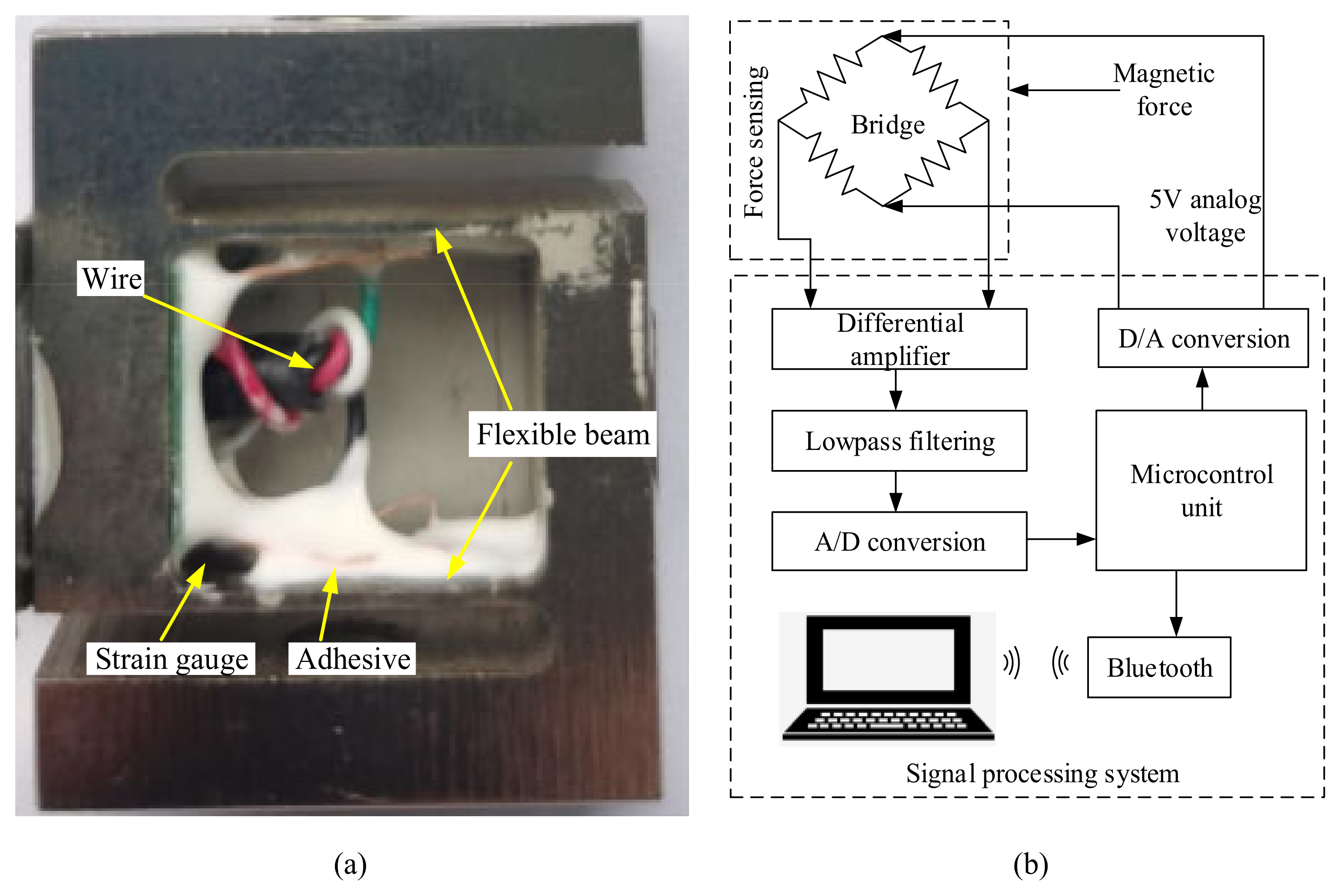
4. Force Sensor
5. Experimental Testing
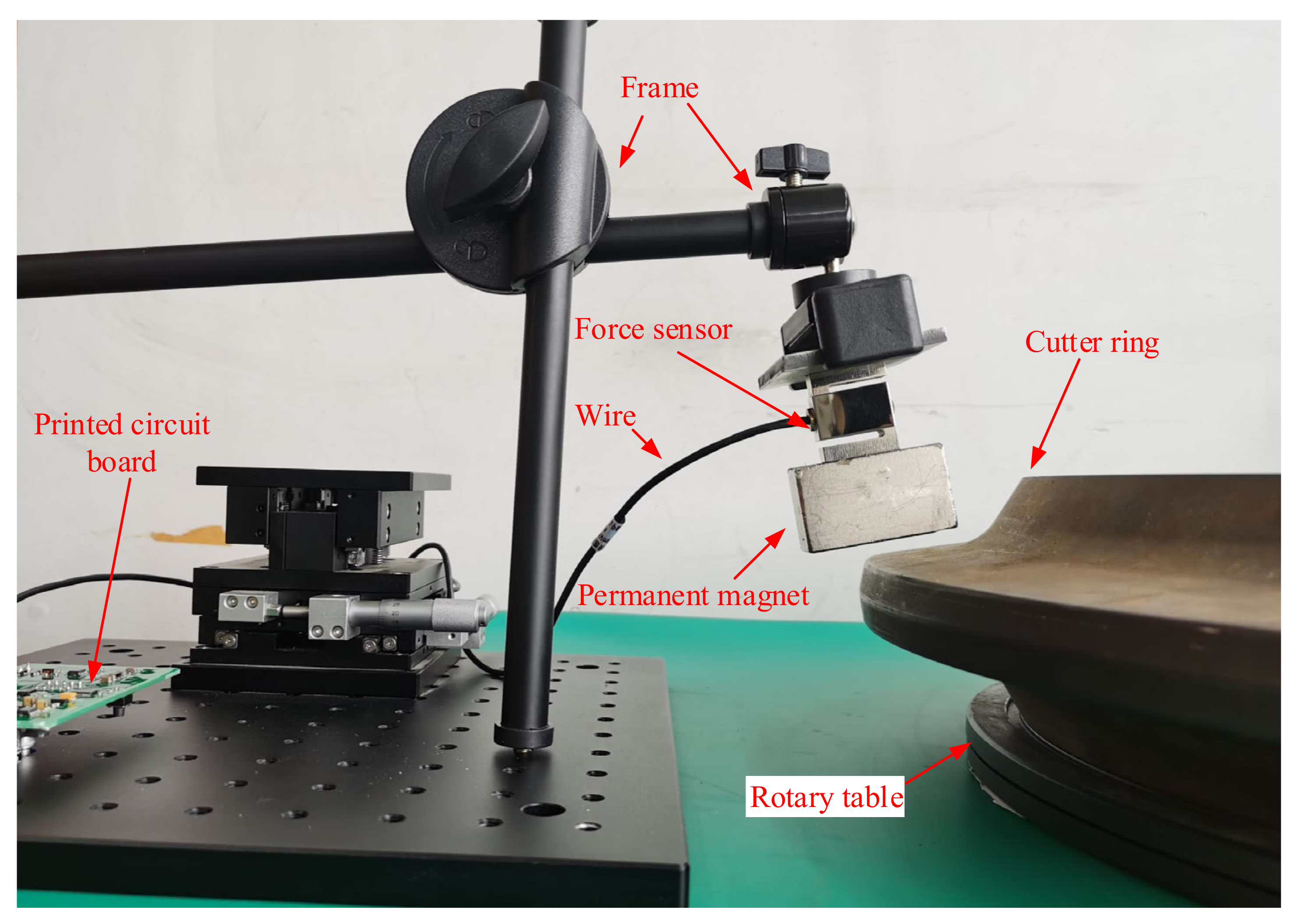
5.1. Experimental Method
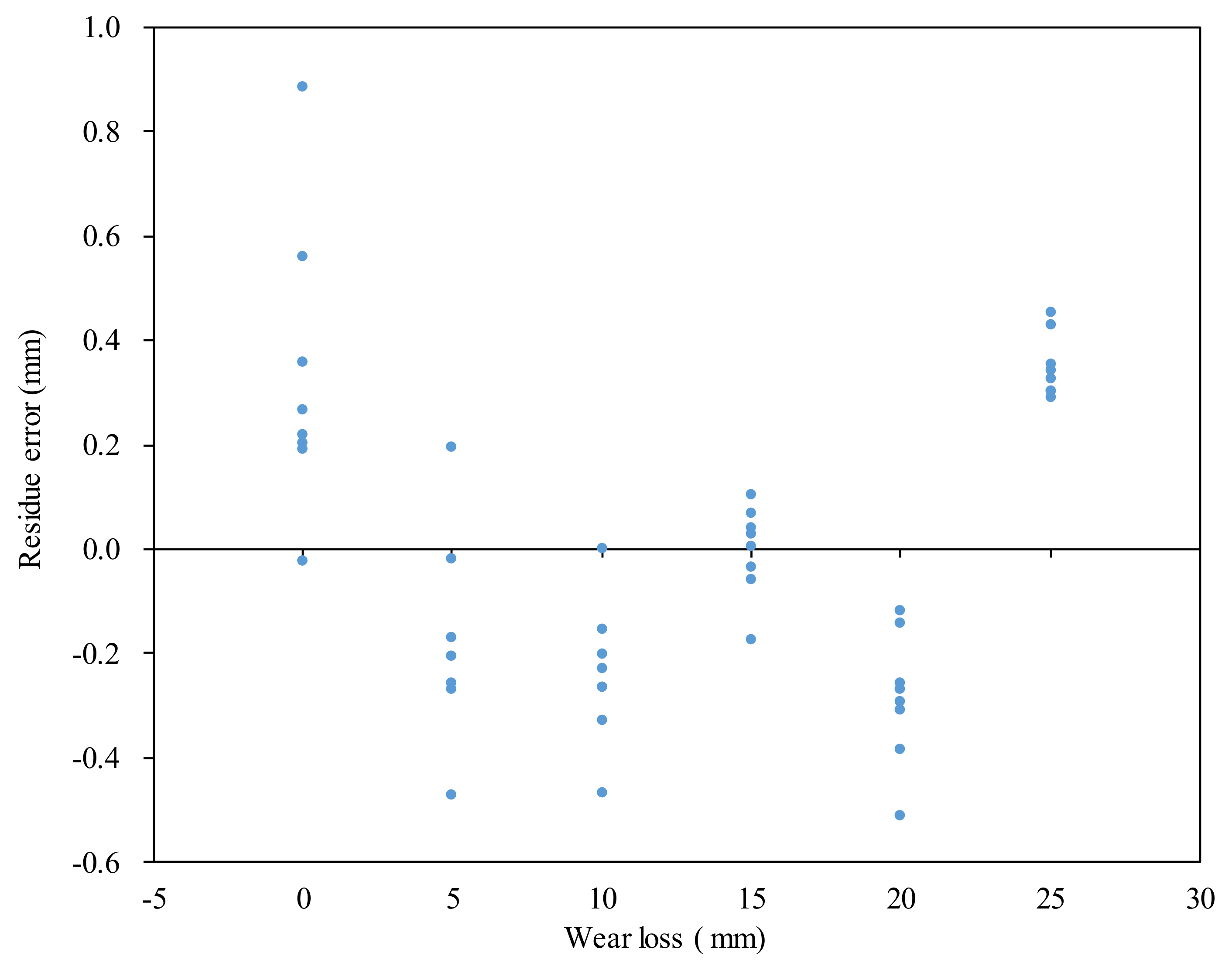
5.2. Experimental Results
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Yang, B.; Chen, S.; Sun, S.; Deng, L.; Li, Z.; Li, W.; Li, H. Vibration suppression of tunnel boring machines using non-resonance approach. Mech. Syst. Signal Process 2020, 145, 106969. [Google Scholar] [CrossRef]
- Chen, G.; Yang, Y.; Huang, T. Vibration analysis of open TBM gripping-thrusting-regripping mechanism. Mech. Mach. Theory 2019, 134, 95–116. [Google Scholar] [CrossRef]
- She, L.; Zhang, S.-r.; Wang, C.; Li, Y.-l.; Du, M. A new method for wear estimation of TBM disc cutter based on energy analysis. Tunn. Undergr. Space Technol. 2023, 131, 104840. [Google Scholar] [CrossRef]
- Alber, M. Stress dependency of the Cerchar abrasivity index (CAI) and its effects on wear of selected rock cutting tools. Tunn. Undergr. Space Technol. 2008, 23, 351–359. [Google Scholar] [CrossRef]
- Ren, D.J.; Shen, S.L.; Arulrajah, A.; Cheng, W.-C. Prediction Model of TBM Disc Cutter Wear During Tunnelling in Heterogeneous Ground. Rock Mech. Rock Eng. 2018, 51, 3599–3611. [Google Scholar] [CrossRef]
- Wang, L.; Kang, Y.; Zhao, X.; Zhang, Q. Disc cutter wear prediction for a hard rock TBM cutterhead based on energy analysis. Tunn. Undergr. Space Technol. 2015, 50, 324–333. [Google Scholar] [CrossRef]
- Yang, J.; Yan, C. Wear Predication of Tunnel Boring Machine Cutters Based on In-situ Measured Data. J. Southwest Jiaotong Univ. 2019, 54, 1283–1292. [Google Scholar]
- Sahinoglu, K.U.; Ozer, U. The prediction of cutter wear from temperature measurements on TBM discs and cutting face. Arab. J. Geosci. 2020, 13, 207. [Google Scholar] [CrossRef]
- Su, W.; Li, X.; Jin, D.; Yang, Y.; Qin, R.; Wang, X. Analysis and prediction of TBM disc cutter wear when tunneling in hard rock strata: A case study of a metro tunnel excavation in Shenzhen, China. Wear 2020, 446–447, 203190. [Google Scholar] [CrossRef]
- Wang, L.; Li, H.; Zhao, X.; Zhang, Q. Development of a prediction model for the wear evolution of disc cutters on rock TBM cutterhead. Tunn. Undergr. Space Technol. 2017, 67, 147–157. [Google Scholar] [CrossRef]
- Pu, X.; Jia, L.; Shang, K.; Chen, L.; Yang, T.; Chen, L.; Gao, L.; Qian, L. A New Strategy for Disc Cutter Wear Status Perception Using Vibration Detection and Machine Learning. Sensors 2022, 22, 6686. [Google Scholar] [CrossRef] [PubMed]
- Burd, H.J.; Houlsby, G.T.; Augarde, C.E.; Liu, G. Modelling tunnelling-induced settlement of masonry buildings. Geotech. Eng. 2000, 143, 17–29. [Google Scholar] [CrossRef]
- Lan, H.; Xia, Y.; Ji, Z.; Fu, J.; Miao, B. Online monitoring device of disc cutter wear—Design and field test. Tunn. Undergr. Space Technol. 2019, 89, 284–294. [Google Scholar] [CrossRef]
- Wang, J.; Zhu, W.; Jiang, L.; Jiang, J.; Liu, T. A multi-channel real-time detection method for tunnel boring machine cutter wear based on Chirped Fiber Bragg Gratings. AIP Adv. 2019, 9, 015312. [Google Scholar] [CrossRef]
- Ji, Z.Y.; Xia, Y.M.; Lan, H.; Yang, M.; Lin, L.K. Cutterhead mud-caking detection method and application based on cutter wear and temperature measurement. J. Adv. Mech. Des. Syst. Manuf. 2019, 13, 13. [Google Scholar] [CrossRef]
- Zhang, B.; Liu, J.Q.; Guo, W. TBM Cutter Wear Detection Device. Patent CN103512527A, 15 January 2014. [Google Scholar]
- Zhang, B.; Liu, J.Q.; Guo, W. Monitoring Device of Disc Cutter Wear on TBM. Patent CN103234903B, 19 August 2015. [Google Scholar]
- Zhang, M.; Yuan, D.; Huang, Q.; Huang, C. Analysis of dynamic abrasion of shield cutters in sandy cobble stratum. Chin. J. Rock Mech. Eng. 2008, 27, 397–402. [Google Scholar]
- Guo, J.B.; Wang, Y.J.; Liu, J.Z.; Niu, J.C. The Research and Design of a New Shield Cutter Wear Detection System. Adv. Mat. Res. 2013, 711, 381–384. [Google Scholar] [CrossRef]
- Lan, H.; Xia, Y.; Zhu, Z.; Ji, Z.; Mao, J. Development of on-line rotational speed monitor system of TBM disc cutter. Tunn. Undergr. Space Technol. 2016, 57, 66–75. [Google Scholar] [CrossRef]
- Zhang, X.B.; Liu, Q.S.; Zhang, J.M. Real-time monitoring technology for wear of cutters and monitoring and analysis of cutterhead vibration of TBM. Tunnel. Constr. 2017, 37, 380–385. [Google Scholar]
- Zheng, W.; Zhao, H.M.; Lan, H.; Tan, Q.; Shu, B.; Xia, Y.M. Design of on-line monitoring system for tunnel boring machine’s disc cutter wear. Instrum. Tech. Sen. 2015, 4, 46–50. [Google Scholar]
- Ren, D.Z.; Sun, X.P. Design on wear detection system for TBM cutter based on CC1101. Min. Process. Equip. 2015, 43, 120–124. [Google Scholar]
- Sun, Z.H.; Zhang, D.L.; Zhang, J.N. Wireless real-time disc cutter wear monitoring system for composite shield machine. Tunnel Constr. 2016, 36, 485–489. [Google Scholar]
- Li, D.; Sun, Z.; Ren, D.; Sun, W. Study of Application of Eddy-current Sensor to Disc-cutter Wear Monitoring System of Shield Machines. Tunnel Constr. 2016, 5, 766–770. [Google Scholar]
- Zhang, M.; Cao, M.; Wei, H. Design of 3D Visual Monitoring System for Shield Disc Cutter Wear. Instr. Tech. Sens. 2021, 9, 58–62. [Google Scholar]
- Wang, F.; Men, C.; Kong, X.; Meng, L. Optimum Design and Application Research of Eddy Current Sensor for Measurement of TBM Disc Cutter Wear. Sensors 2019, 19, 4230. [Google Scholar] [CrossRef]
- Bai, X. TBM cutting tool wear detection system based on displacement sensor. Master’s Thesis, Wuhan University, Wuhan, China, 2018. [Google Scholar]
- Gong, Q.; Wu, F.; Wang, D.; Qiu, H.; Yin, L. Development and Application of Cutterhead Working Status Monitoring System for Shield TBM Tunnelling. Rock. Mech. Rock. Eng. 2021, 54, 1731–1753. [Google Scholar] [CrossRef]
- Schomburg, W.K.; Reinertz, O.; Sackmann, J.; Schmitz, K. Equations for the approximate calculation of forces between cuboid magnets. J. Mag. Mag. Mat. 2020, 506, 166694. [Google Scholar] [CrossRef]
- COMSOL—Software for Multiphysics Simulation. Available online: http://www.comsol.com (accessed on 5 March 2023).
- Liu, M.; Zhang, Q.; Shao, Y.; Liu, C.; Zhao, Y. Research of a Novel 3D Printed Strain Gauge Type Force Sensor. Micromachines 2019, 10, 20. [Google Scholar] [CrossRef]
- Wang, C.; Reddy, J.; Lee, K. Shear Deformable Beams and Plates. Relationships with Classical Solutions; Elsevier: Amsterdam, The Netherlands, 2000. [Google Scholar]




Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Han, J.; Xiang, H.; Feng, Q.; He, J.; Li, R.; Zhao, W. Detection for Disc Cutter Wear of TBM Using Magnetic Force. Machines 2023, 11, 388. https://doi.org/10.3390/machines11030388
Han J, Xiang H, Feng Q, He J, Li R, Zhao W. Detection for Disc Cutter Wear of TBM Using Magnetic Force. Machines. 2023; 11(3):388. https://doi.org/10.3390/machines11030388
Chicago/Turabian StyleHan, Jialin, Hongjiang Xiang, Qiuyue Feng, Jiangbo He, Rong Li, and Wensheng Zhao. 2023. "Detection for Disc Cutter Wear of TBM Using Magnetic Force" Machines 11, no. 3: 388. https://doi.org/10.3390/machines11030388
APA StyleHan, J., Xiang, H., Feng, Q., He, J., Li, R., & Zhao, W. (2023). Detection for Disc Cutter Wear of TBM Using Magnetic Force. Machines, 11(3), 388. https://doi.org/10.3390/machines11030388




