Effect of the Stiffness of the Turntable Bearing Joint on the Dynamic Characteristics of the Five-Axis Machine Tool Rotary System
Abstract
:1. Introduction
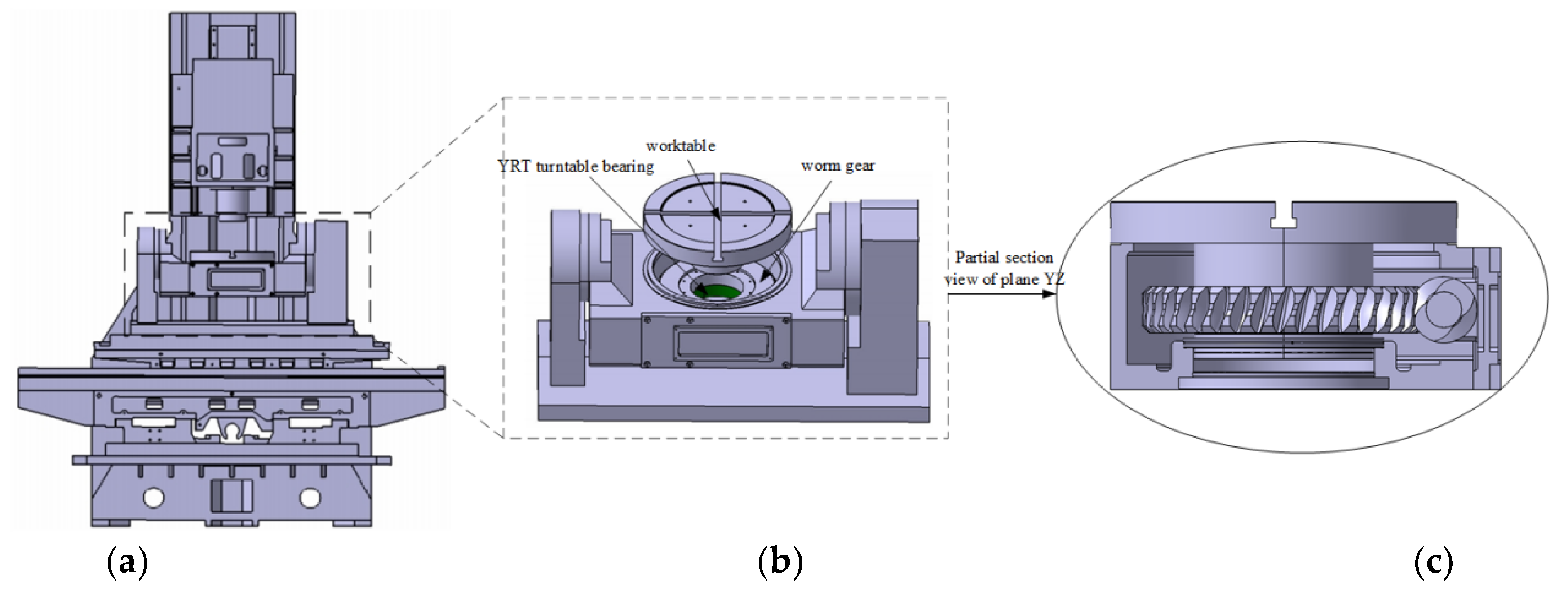
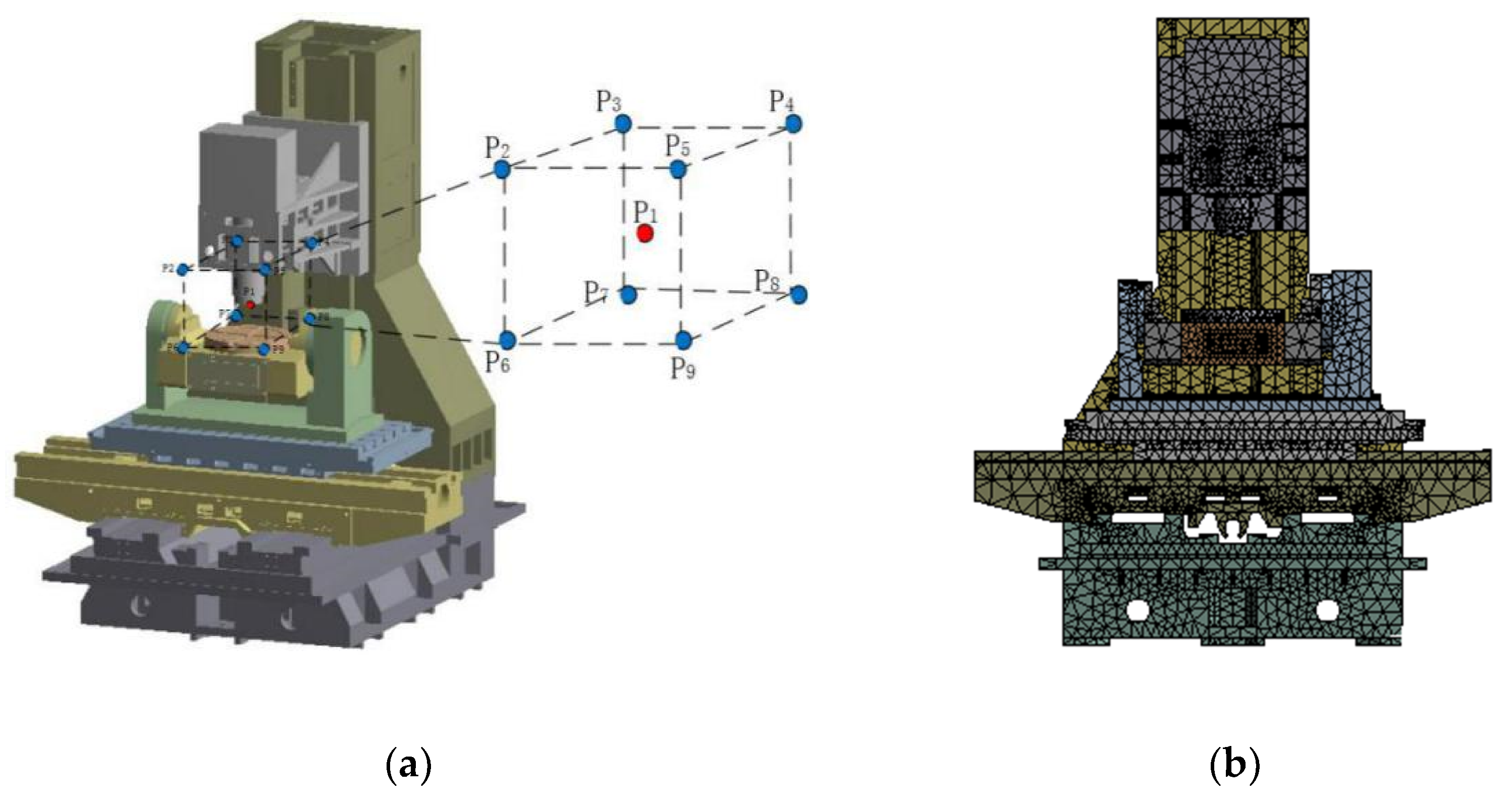
2. Basic Structure of Turntable Rotary System
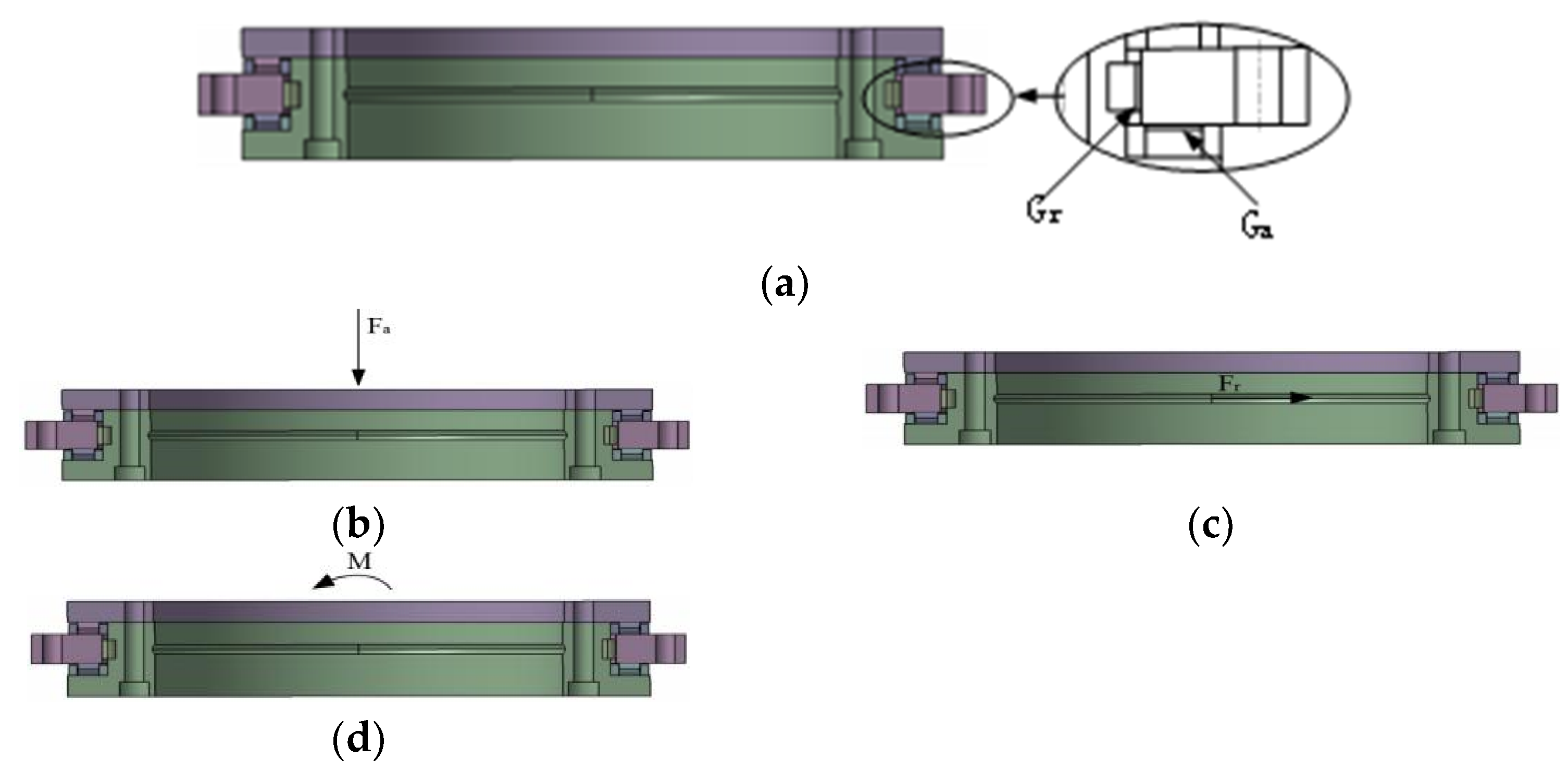
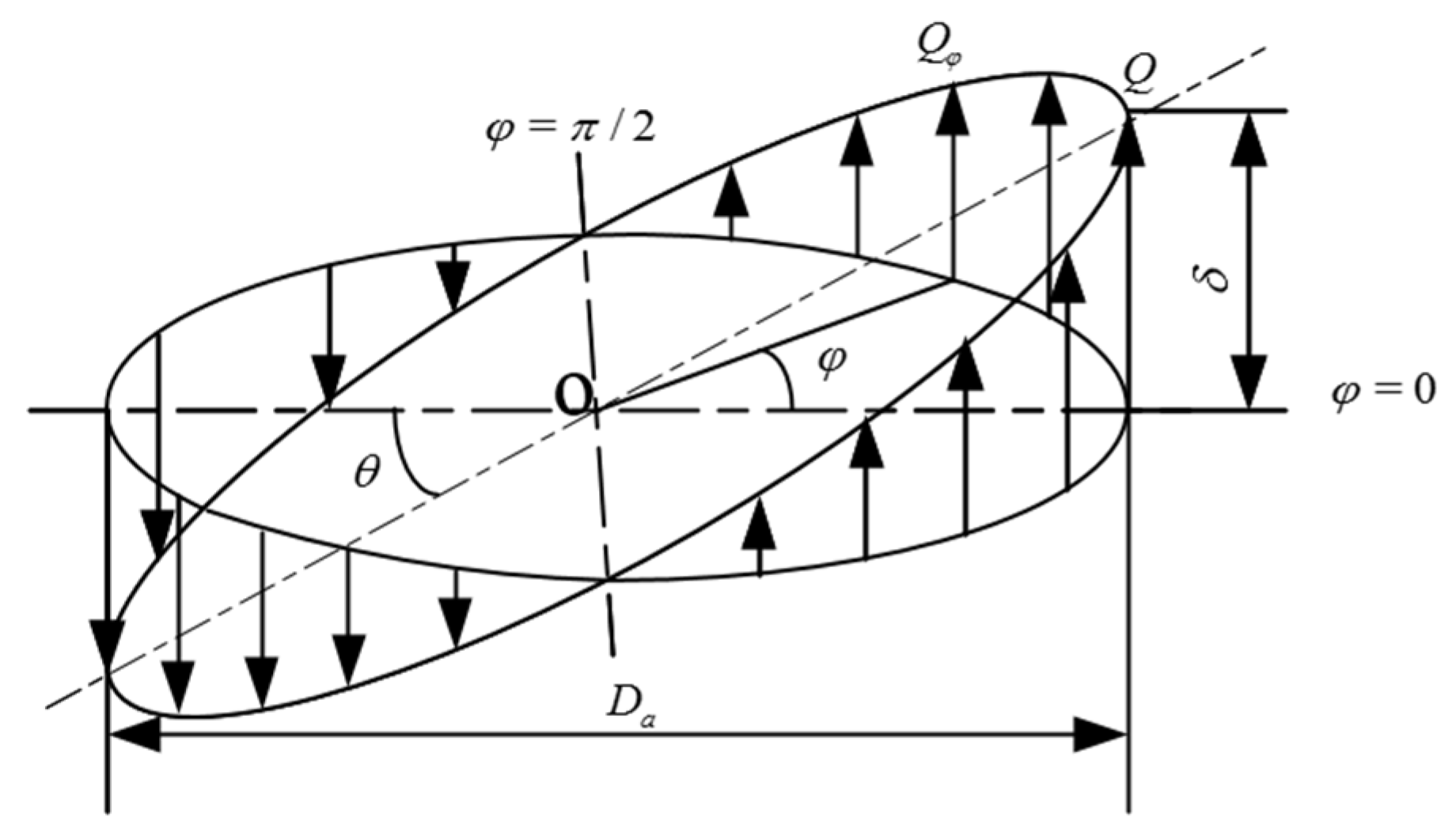
2.1. Axial Stiffness Modeling of Turntable Bearing
2.2. Radial Stiffness Modeling of Turntable Bearing
2.3. Angular Stiffness Modeling of Turntable Bearing
3. Analysis of Influencing Factors of Bearing Stiffness of Turntable
3.1. Effect of Clearance on Bearing Stiffness of Turntable
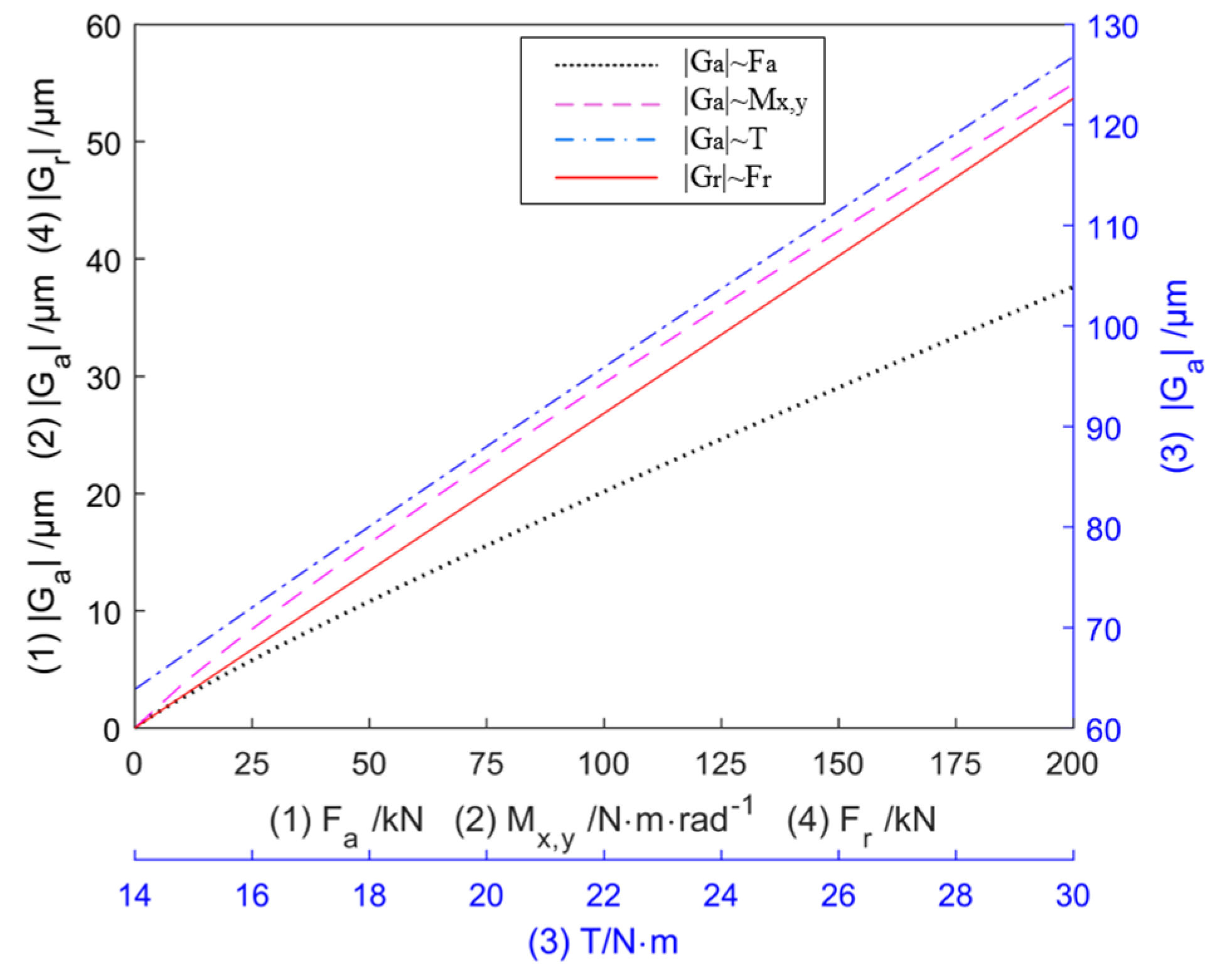
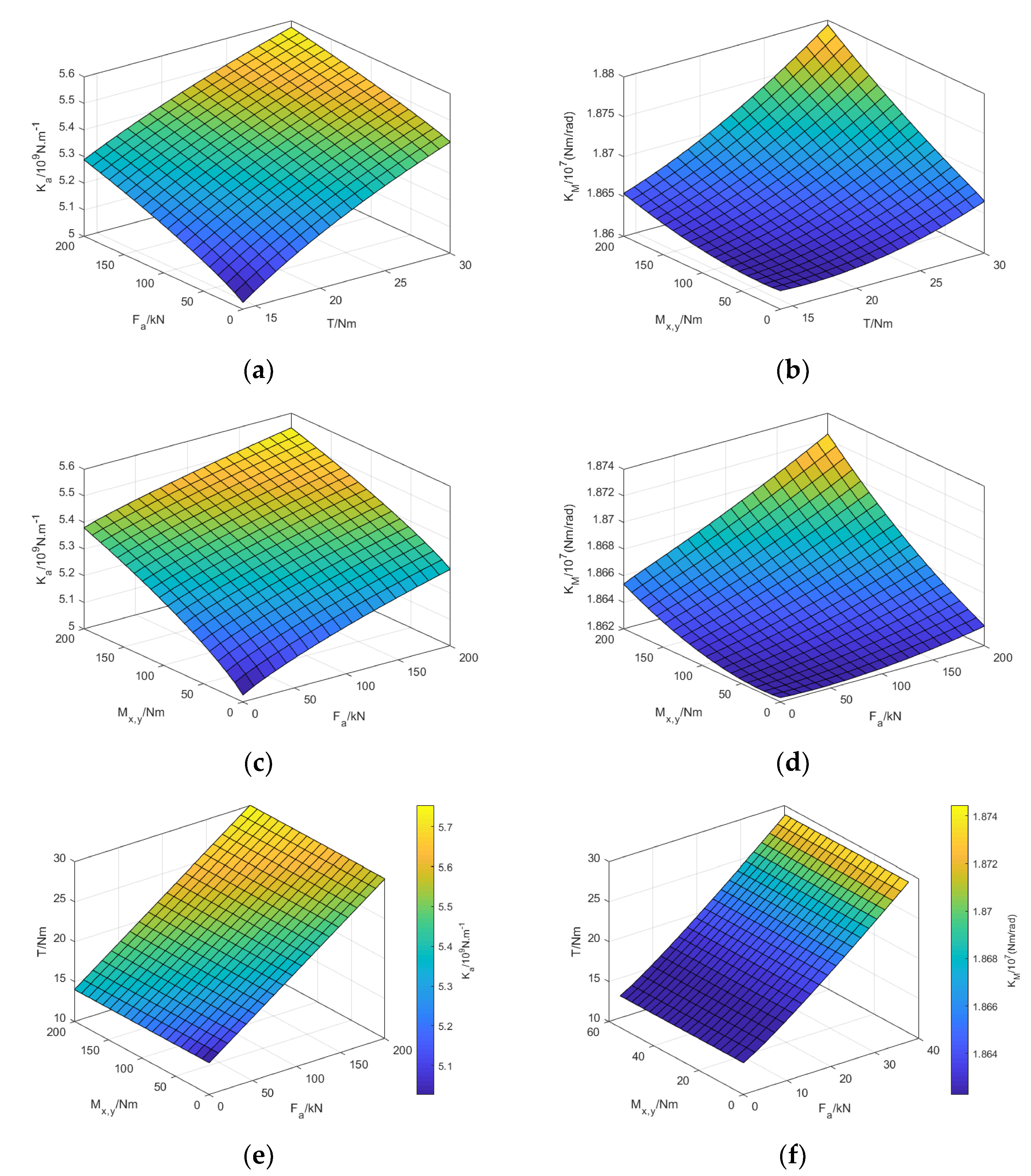
3.2. Effect of External Load on Bearing Stiffness of Turntable
4. Analysis of Dynamic Characteristics of the Rotary System of the Turntable
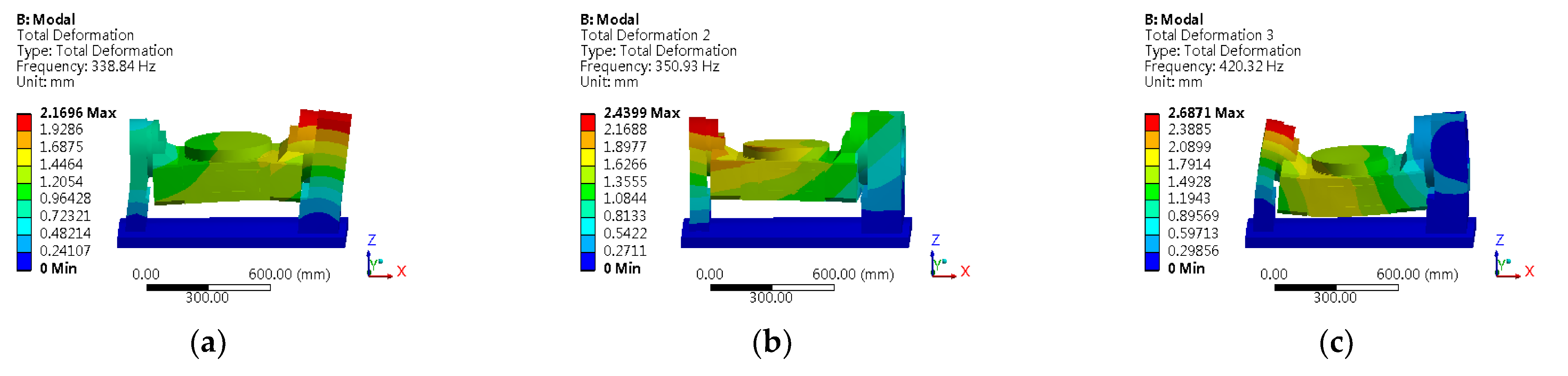
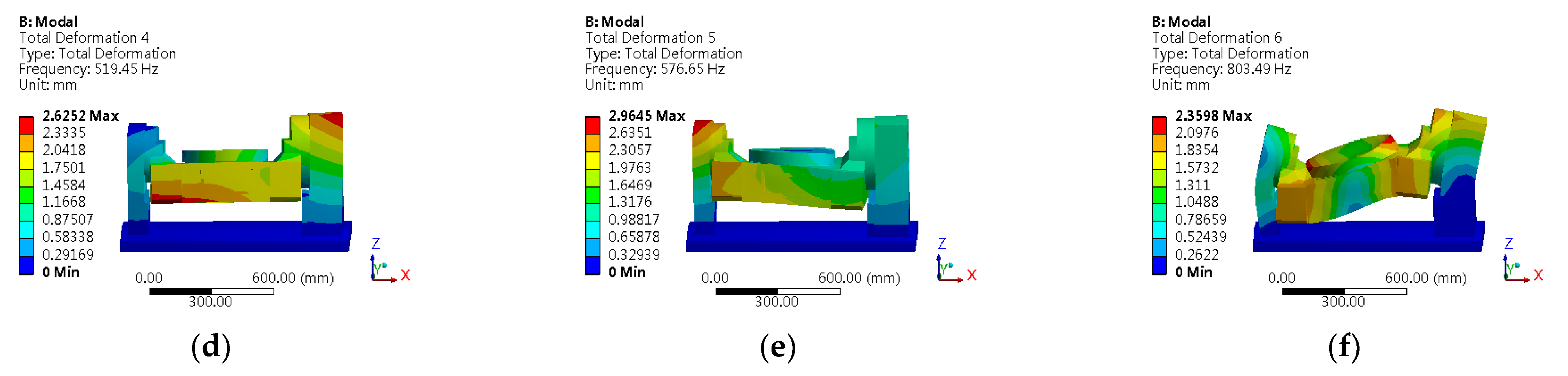
4.1. Dynamic Characteristics Analysis of Rotary System at Different Swing Angles of the Turntable
4.2. Dynamic Characteristics Analysis of Rotary Table System under Different Bearing Stiffness of the Turntable
5. Analysis of Dynamic Characteristics of Two Axis Rotary Table Five-Axis Machine Tool
6. Conclusions
- (1)
- The stiffness of the joint bearing of the rotary table system is calculated, and the effect of bearing clearance and external load on the stiffness is analyzed. The increase in the screw tightening torque T and external load Fa and Mx,y increases , which in turn increases Ka and KM accordingly. The increase in KM is significant, while Ka increases gradually when increases to 20 μm. As the radial clearance Kr increases, the radial stiffness first increases significantly and then tends to be stable.
- (2)
- When the swing angle of the turntable changes from −90° to 90°, the first-order natural frequency of the turntable decreases with the increase in the swing angle. The natural frequency of the positive angle is larger than that of the corresponding negative angle. When the swing angle is 0°, the natural frequency reaches the maximum (338.84 Hz), and when the swing angle is −90°, the natural frequency reaches the minimum (261.41 Hz). With the change in swing angle, the change in the first order natural frequency of the turntable is 77.43 Hz.
- (3)
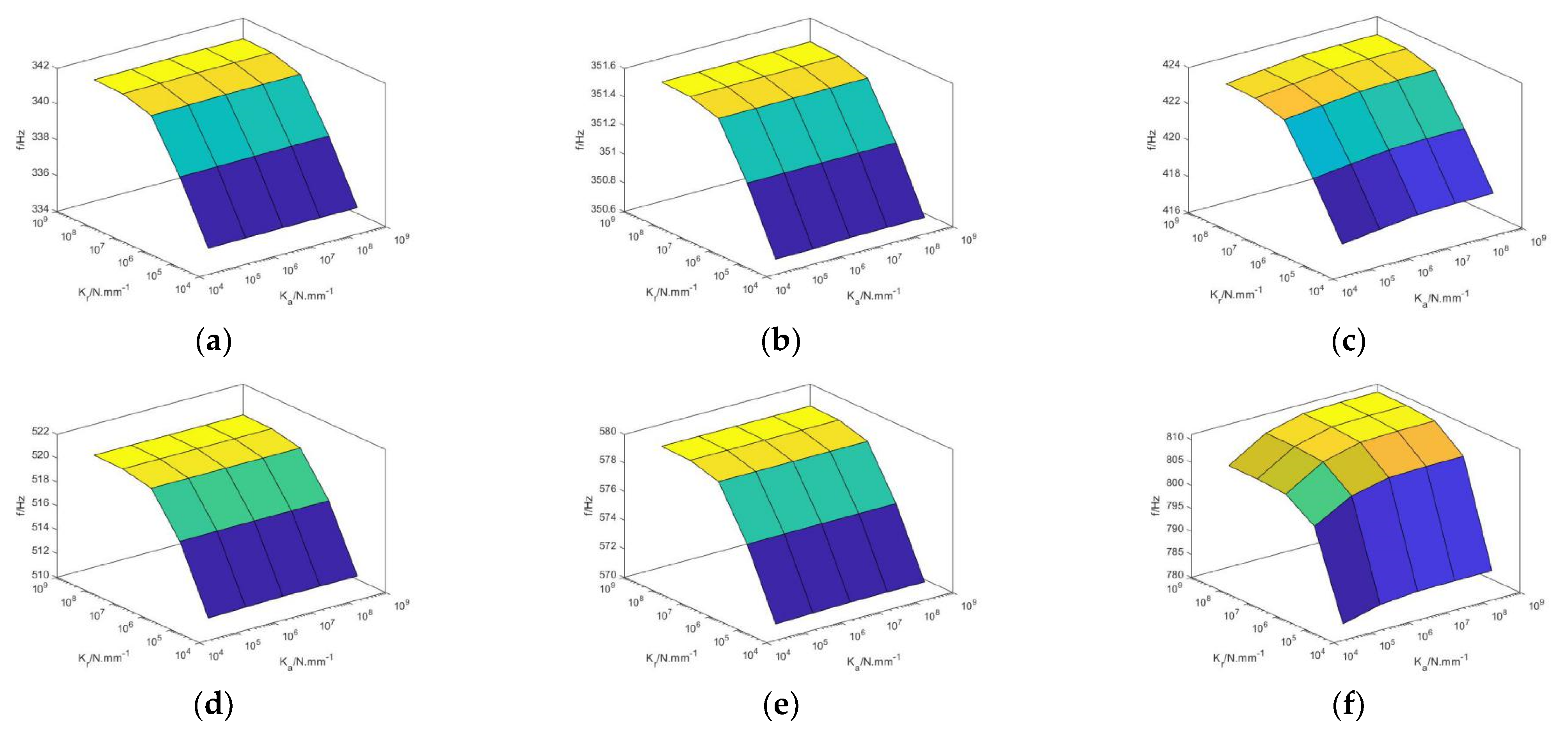
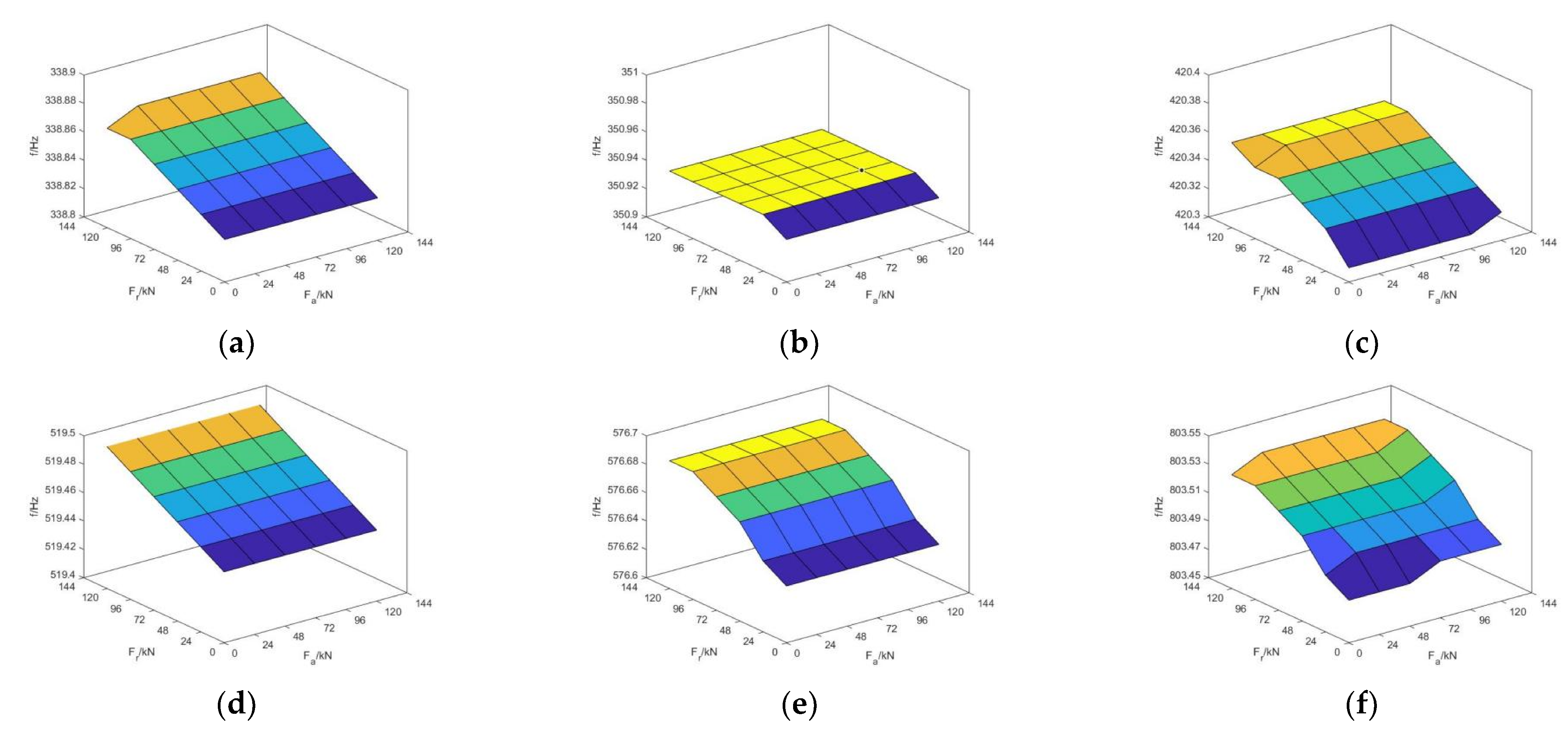
- In the process of changing the turntable bearing stiffness, the change in the radial stiffness of the turntable bearing joint has a great influence on the natural frequency of the turntable. When the axial stiffness of the turntable bearing changes from 4.95 × 105 N/mm to 4.95 × 109 N/mm, and the radial stiffness changes from 3.95 × 105 N/mm to 3.95 × 109 N/mm, the first order natural frequency of the turntable does not change significantly with the axial stiffness of the turntable bearing joint, but increase with the increase in the radial stiffness of the turntable bearing joint, and the change in the first order natural frequency is 30.3 Hz. However, in the process of external axial force 0 to 120 kN and radial force 0 to 120 kN of the turntable bearing, the change in external load of turntable bearing has little influence on the natural frequency of the turntable, and the natural frequency of the turntable changes less than by 1 Hz.
- (4)
- The dynamic characteristic of the two-axis turntable five-axis machine tools mainly decided by the change in the Z-axis. The higher the Z-axis guide stays near the workbench, the higher is the first-order natural frequency of the machine tool.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Lim, T.C.; Singh, R. Vibration transmission through rolling element bearings, part I: Bearing stiffness formulation. J. Sound Vibr. 1990, 139, 179–199. [Google Scholar] [CrossRef]
- Guo, Y.; Parker, R.G. Stiffness matrix calculation of rolling element bearings using a finite element/contact mechanics model. Mech. Mach. Theory 2012, 51, 32–45. [Google Scholar] [CrossRef]
- Gupta, T.C. Parametric studies on dynamic stiffness of ball bearings supporting a flexible rotor. J. Vib. Control 2019, 25, 2175–2188. [Google Scholar] [CrossRef]
- Hao, X.; Gu, X.; Zhou, X.; Liao, X.; Han, Q. Distribution characteristics of stress and displacement of rings of cylindrical roller bearing. Proc. Inst. Mech. Eng. Part C-J. Eng. Mech. Eng. Sci. 2019, 233, 4348–4358. [Google Scholar] [CrossRef]
- Göncz, P.; Drobne, M.; Glodež, S. Computational model for determination of dynamic load capacity of large three-row roller slewing bearings. Eng. Fail. Anal. 2013, 32, 44–53. [Google Scholar] [CrossRef]
- Li, Y.; Jiang, D. Strength check of a three-row roller slewing bearing based on a mixed finite element model. Proc. Inst. Mech. Eng. Part C-J. Eng. Mech. Eng. Sci. 2017, 231, 3393–3400. [Google Scholar] [CrossRef]
- Li, Y.F.; Lei, J. Determination of permissible contact stress of case hardened raceway of roller slewing bearing. Sci. Prog. 2020, 103, 0036850420980609. [Google Scholar]
- Wang, H.; He, P.; Pang, B.; Gao, X. A new computational model of large three-row roller slewing bearings using nonlinear springs. Proc. Inst. Mech. Eng. Part C-J. Eng. Mech. Eng. Sci. 2017, 231, 3831–3839. [Google Scholar] [CrossRef]
- He, P.; Liu, R.; Hong, R.; Wang, H.; Yang, G.; Lu, C. Hardened raceway calculation analysis of a three-row roller slewing bearing. Int. J. Mech. Sci. 2018, 137, 133–144. [Google Scholar] [CrossRef]
- He, P.; Wang, Y. Effect of high-strength bolts and supporting structures on the carrying capacity of three-row roller slewing bearings. Proc. Inst. Mech. Eng. Part C-J. Eng. Mech. Eng. Sci. 2021, 235, 2053–2064. [Google Scholar] [CrossRef]
- Wei, W.; Lv, D.; Zhang, J. Static stiffness modeling of turntable bearing and analysis of influencing factors. Acad. J. XJTU. 2014, 48, 8–14. [Google Scholar]
- Li, T.J.; Ding, X.H.; Cheng, K.; Wu, T. Dynamic optimization method with applications for machine tools based on approximation model. Proc. Inst. Mech. Eng. Part C-J. Eng. Mech. Eng. Sci. 2018, 232, 2009–2022. [Google Scholar] [CrossRef]
- Chen, Y.; Zhao, C.; Li, Z.; Lu, Z. Analysis on dynamic contact characteristics and dynamic stiffness estimating method of single nut ball screw pair based on the whole rolling elements model. Appl. Sci. 2020, 10, 5795. [Google Scholar] [CrossRef]
- Chen, H.; Tan, Z.; Tan, F.; Yin, G. Dynamic performance analysis and optimization method of the horizontal machining center based on contact theory. Int. J. Adv. Manuf. Technol. 2020, 108, 3055–3073. [Google Scholar] [CrossRef]
- Yu, H.; Ran, Y.; Zhang, G.; Ying, G. A dynamic time-varying reliability model for linear guides considering wear degradation. Nonlinear Dyn. 2021, 103, 699–714. [Google Scholar] [CrossRef]
- Liu, J.; Ma, C.; Wang, S.; Wang, S.; Yang, B. Contact stiffness of spindle-tool holder based on fractal theory and multi-scale contact mechanics model. Mech. Syst. Signal Proc. 2019, 119, 363–379. [Google Scholar] [CrossRef]
- Majumdar, A.; Bhushan, B. Fractal model of elastic-plastic contact between rough surfaces. J. Tribol-T. ASME. 1991, 113, 1–11. [Google Scholar] [CrossRef]
- Miao, X.; Huang, X. A complete contact model of a fractal rough surface. Wear 2014, 309, 146–151. [Google Scholar] [CrossRef]
- Li, D.; Botto, D.; Li, R.; Xu, C.; Zhang, W. Experimental and theoretical studies on friction contact of bolted joint interfaces. Int. J. Mech. Sci. 2022, 236, 107773. [Google Scholar] [CrossRef]
- Yuan, Y.; Xu, K.; Zhao, K. A fractal model of contact between rough surfaces for a complete loading–unloading process. Proc. Inst. Mech. Eng. Part C-J. Eng. Mech. Eng. Sci. 2020, 234, 2923–2935. [Google Scholar] [CrossRef]
- Harris, T.A.; Kotzalas, M.N. Rolling Bearing Analysis; Advanced Concepts of Bearing Technology; CRC Press: Boca Raton, FL, USA, 2006; pp. 209–256. [Google Scholar]


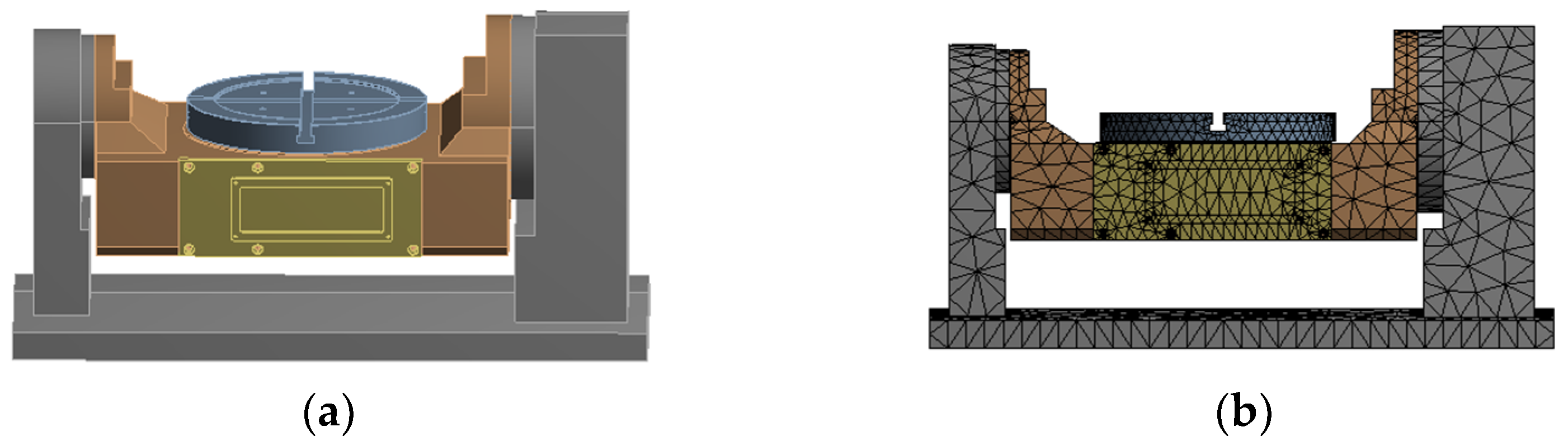


| Parameters | Value |
|---|---|
| Number of axial radial rollers Za, Zr | 70, 115 |
| Axial radial roller diameter Daw, Drw/mm | 5, 5 |
| Axial radial roller length La, Lr/mm | 8, 8 |
| Diameter of axial radial roller raceway Da, Dr/mm | 199, 183.5 |
| Number of screws Zb | 36 |
| Screw diameter db/mm | 7 |
| Type of Junction | Axial Stiffness (N/m) | Radial Stiffness (N/m) | Angular Stiffness (N/m) |
|---|---|---|---|
| Turntable supports Bearing joint | - | ||
| - | |||
| Turntable bearing joint | |||
| Worm gear and worm Pair joint | - |
| Mode Order | 1th | 2th | 3th | 4th | 5th | 6th |
|---|---|---|---|---|---|---|
| Theoretical value/Hz | 338.84 | 350.93 | 420.32 | 519.45 | 576.65 | 803.49 |
| Theoretical vibration mode | X-direction bending vibration of worktable | Y-direction bending vibration of worktable | Z-direction bending vibration of worktable | X-direction torsional vibration of table | Z-direction torsional vibration of table | Y-direction torsional vibration of table |
| Mode Order Theoretical Value/Hz | 1th | 2th | 3th | 4th | 5th | 6th |
|---|---|---|---|---|---|---|
| 0° (the swing angles of the turntable)/Hz | 338.84 | 350.93 | 420.32 | 519.45 | 576.65 | 803.49 |
| 30° | 320.24 | 352.23 | 462.45 | 517.91 | 569.32 | 805.48 |
| −30° | 313.13 | 348.52 | 463.74 | 522.77 | 554.97 | 823.51 |
| 60° | 291.93 | 350.75 | 501.87 | 545.23 | 562.45 | 833.19 |
| −60° | 280.96 | 349.02 | 498.07 | 530.73 | 568.04 | 870.91 |
| 90° | 271.83 | 329.28 | 495.88 | 533.42 | 677 | 855.99 |
| −90° | 261.41 | 328.54 | 488.72 | 540.1 | 679.81 | 907.91 |
| Type of Junction | Interface Structure | Axial Stiffness (N/µm) | Normal Stiffness (N/µm) |
|---|---|---|---|
| Linear guide pair | Cradle and bed | - | 348 |
| Slide rails and beams | - | 326 | |
| Spindle and slide rails | - | 304 | |
| Slide rails and columns | - | 305 | |
| Ball screw pair | Cradle and bed | 267 | - |
| Slide rails and spindle | 262 | - | |
| Slide rails and beams | 253 | - | |
| Slide rails and columns | 257 | - |
| Mode Order | 1th | 2th | 3th | 4th | 5th | 6th |
|---|---|---|---|---|---|---|
| Theoretical value/Hz | 35.55 | 45.24 | 88.97 | 96.90 | 99.47 | 112.73 |
| Theoretical vibration mode | Y-direction bending vibration of column | X-direction bending vibration of spindle box | Y-direction bending vibration of worktable | Y-direction bending vibration of spindle box | Z-direction torsional vibration of column | X-direction bending vibration of worktable |
| Spindle End Position Parameters (mm) | f1 (Hz) | f2 (Hz) | f3 (Hz) | f4 (Hz) | f5 (Hz) | f6 (Hz) | |||
|---|---|---|---|---|---|---|---|---|---|
| 0° | 0 | 0 | 0 | 35.55 | 45.24 | 88.97 | 96.90 | 99.47 | 112.73 |
| 0° | −150 | −150 | 150 | 34.05 | 43.35 | 86.82 | 94.19 | 98.89 | 106.78 |
| 0° | −150 | 150 | 150 | 34.04 | 43.33 | 83.54 | 94.16 | 99.26 | 105.38 |
| 0° | 150 | 150 | 150 | 34.04 | 43.33 | 86.72 | 94.34 | 99.30 | 110.78 |
| 0° | 150 | −150 | 150 | 34.05 | 43.35 | 89.79 | 94.47 | 99.05 | 112.24 |
| 0° | −150 | −150 | −150 | 37.06 | 46.95 | 86.88 | 98.32 | 99.13 | 106.95 |
| 0° | −150 | 150 | −150 | 37.05 | 46.93 | 83.58 | 98.32 | 99.56 | 105.45 |
| 0° | 150 | 150 | −150 | 37.05 | 46.93 | 86.82 | 98.47 | 99.60 | 110.83 |
| 0° | 150 | −150 | −150 | 37.06 | 46.95 | 89.98 | 98.49 | 99.32 | 112.39 |
| 30° | 0 | 0 | 0 | 35.54 | 45.25 | 88.61 | 96.89 | 99.45 | 112.88 |
| 30° | −150 | −150 | 150 | 34.04 | 43.37 | 86.38 | 94.19 | 98.86 | 106.81 |
| 30° | −150 | 150 | 150 | 34.03 | 43.35 | 83.27 | 94.17 | 99.25 | 105.46 |
| 30° | 150 | 150 | 150 | 34.03 | 43.35 | 86.36 | 94.32 | 99.29 | 110.86 |
| 30° | 150 | −150 | 150 | 34.04 | 43.37 | 89.37 | 94.42 | 99.04 | 112.34 |
| 30° | −150 | −150 | −150 | 37.04 | 46.95 | 86.43 | 98.29 | 99.09 | 106.99 |
| 30° | −150 | 150 | −150 | 37.03 | 46.93 | 83.30 | 98.30 | 99.54 | 105.55 |
| 30° | 150 | 150 | −150 | 37.03 | 46.93 | 86.45 | 98.43 | 99.58 | 110.91 |
| 30° | 150 | −150 | −150 | 37.04 | 46.95 | 89.52 | 98.44 | 99.29 | 112.48 |
| 60° | 0 | 0 | 0 | 35.54 | 45.25 | 86.83 | 96.80 | 99.43 | 112.24 |
| 60° | −150 | −150 | 150 | 34.04 | 43.37 | 84.67 | 94.13 | 98.78 | 106.10 |
| 60° | −150 | 150 | 150 | 34.03 | 43.35 | 81.71 | 94.12 | 99.21 | 104.74 |
| 60° | 150 | 150 | 150 | 34.03 | 43.35 | 84.67 | 94.23 | 99.28 | 110.19 |
| 60° | 150 | −150 | 150 | 34.04 | 43.37 | 87.54 | 94.25 | 99.01 | 111.69 |
| 60° | −150 | −150 | −150 | 37.04 | 46.95 | 84.70 | 98.25 | 98.99 | 106.30 |
| 60° | −150 | 150 | −150 | 37.03 | 46.93 | 81.73 | 98.26 | 99.49 | 104.83 |
| 60° | 150 | 150 | −150 | 37.03 | 46.93 | 84.73 | 98.36 | 99.57 | 110.25 |
| 60° | 150 | −150 | −150 | 37.04 | 46.95 | 87.61 | 98.35 | 99.25 | 111.82 |
| −30° | 0 | 0 | 0 | 35.54 | 45.25 | 88.01 | 96.85 | 99.47 | 112.27 |
| −30° | −150 | −150 | 150 | 34.04 | 43.37 | 85.78 | 94.16 | 98.87 | 106.20 |
| −30° | −150 | 150 | 150 | 34.03 | 43.35 | 82.73 | 94.15 | 99.26 | 104.85 |
| −30° | 150 | 150 | 150 | 34.03 | 43.35 | 85.74 | 94.29 | 99.31 | 110.36 |
| −30° | 150 | −150 | 150 | 34.04 | 43.37 | 88.73 | 94.35 | 99.07 | 111.73 |
| −30° | −150 | −150 | −150 | 37.04 | 46.95 | 85.81 | 98.28 | 99.09 | 106.38 |
| −30° | −150 | 150 | −150 | 37.03 | 46.93 | 82.76 | 98.29 | 99.55 | 104.93 |
| −30° | 150 | 150 | −150 | 37.03 | 46.93 | 85.81 | 98.41 | 99.61 | 110.41 |
| −30° | 150 | −150 | −150 | 37.04 | 46.95 | 88.84 | 98.41 | 99.32 | 111.86 |
| −60° | 0 | 0 | 0 | 35.54 | 45.25 | 85.80 | 96.76 | 99.47 | 111.27 |
| −60° | −150 | −150 | 150 | 34.04 | 43.37 | 83.62 | 94.10 | 98.80 | 105.10 |
| −60° | −150 | 150 | 150 | 34.03 | 43.35 | 80.78 | 94.10 | 99.23 | 103.75 |
| −60° | 150 | 150 | 150 | 34.03 | 43.35 | 83.62 | 94.19 | 99.31 | 109.37 |
| −60° | 150 | −150 | 150 | 34.04 | 43.37 | 86.44 | 94.19 | 99.06 | 110.71 |
| −60° | −150 | −150 | −150 | 37.04 | 46.95 | 83.64 | 98.24 | 99.00 | 105.29 |
| −60° | −150 | 150 | −150 | 37.03 | 46.93 | 80.79 | 98.25 | 99.50 | 103.85 |
| −60° | 150 | 150 | −150 | 37.03 | 46.93 | 83.66 | 98.33 | 99.60 | 109.42 |
| −60° | 150 | −150 | −150 | 37.04 | 46.95 | 86.48 | 98.32 | 99.31 | 110.84 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wu, S.; Lei, W.; Yu, T.; Dong, Z.; Liu, T. Effect of the Stiffness of the Turntable Bearing Joint on the Dynamic Characteristics of the Five-Axis Machine Tool Rotary System. Machines 2023, 11, 389. https://doi.org/10.3390/machines11030389
Wu S, Lei W, Yu T, Dong Z, Liu T. Effect of the Stiffness of the Turntable Bearing Joint on the Dynamic Characteristics of the Five-Axis Machine Tool Rotary System. Machines. 2023; 11(3):389. https://doi.org/10.3390/machines11030389
Chicago/Turabian StyleWu, Shi, Weijie Lei, Tai Yu, Zeyu Dong, and Taorui Liu. 2023. "Effect of the Stiffness of the Turntable Bearing Joint on the Dynamic Characteristics of the Five-Axis Machine Tool Rotary System" Machines 11, no. 3: 389. https://doi.org/10.3390/machines11030389
APA StyleWu, S., Lei, W., Yu, T., Dong, Z., & Liu, T. (2023). Effect of the Stiffness of the Turntable Bearing Joint on the Dynamic Characteristics of the Five-Axis Machine Tool Rotary System. Machines, 11(3), 389. https://doi.org/10.3390/machines11030389






