Rapid Calculation and Optimization of Vibration and Noise of Permanent-Magnet Synchronous Motors for EVs Based on Equivalent Structural Network
Abstract
:1. Introduction
2. Equivalent Structural Network
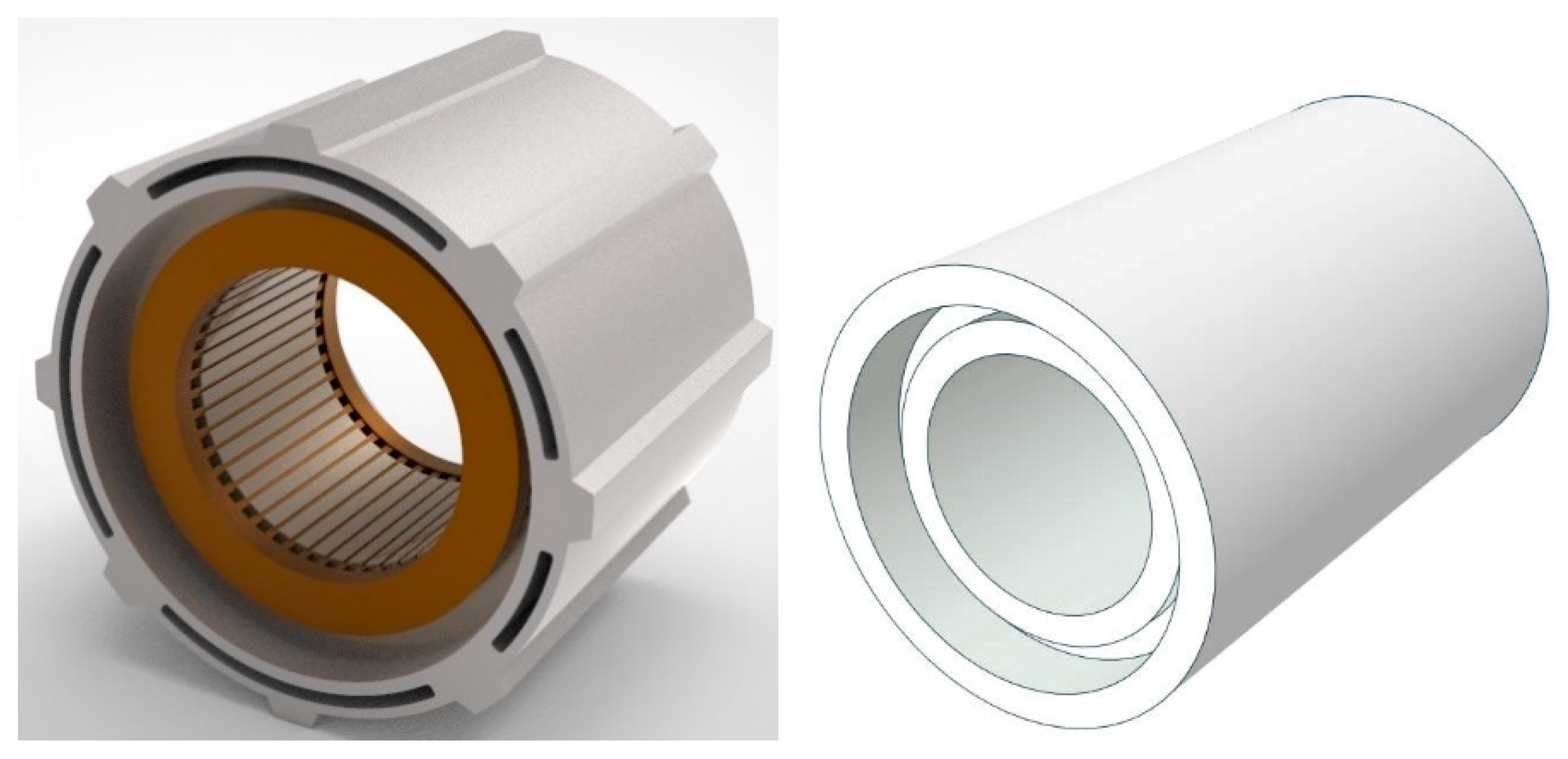
2.1. Analytical Model of the Cylindrical Shell and ESN
2.2. Structural Transfer Function of a 30 kW IPMSM and FEM Verification
2.3. Modal Hammering Test
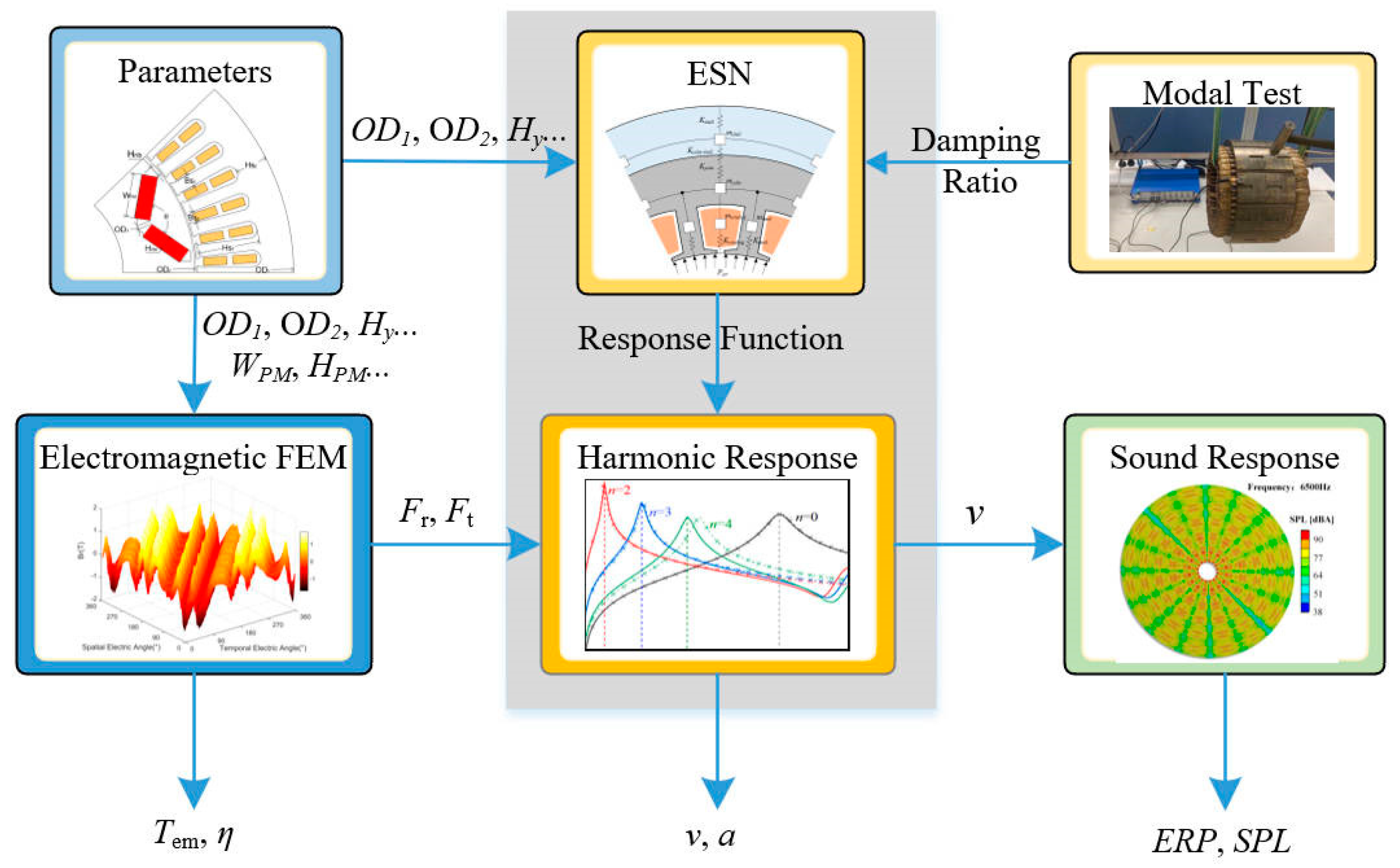
3. Electromagnetic Vibration and Noise Rapid Calculation Based on ESN
3.1. Rapid Calculation Process
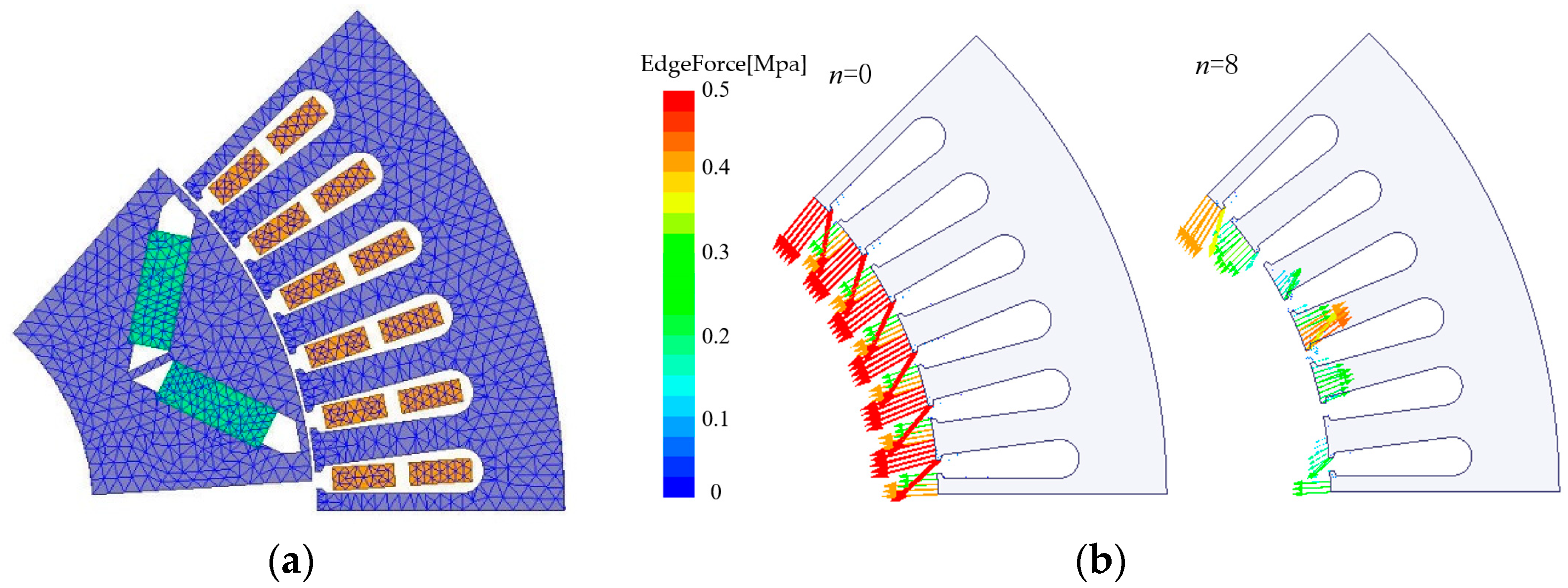
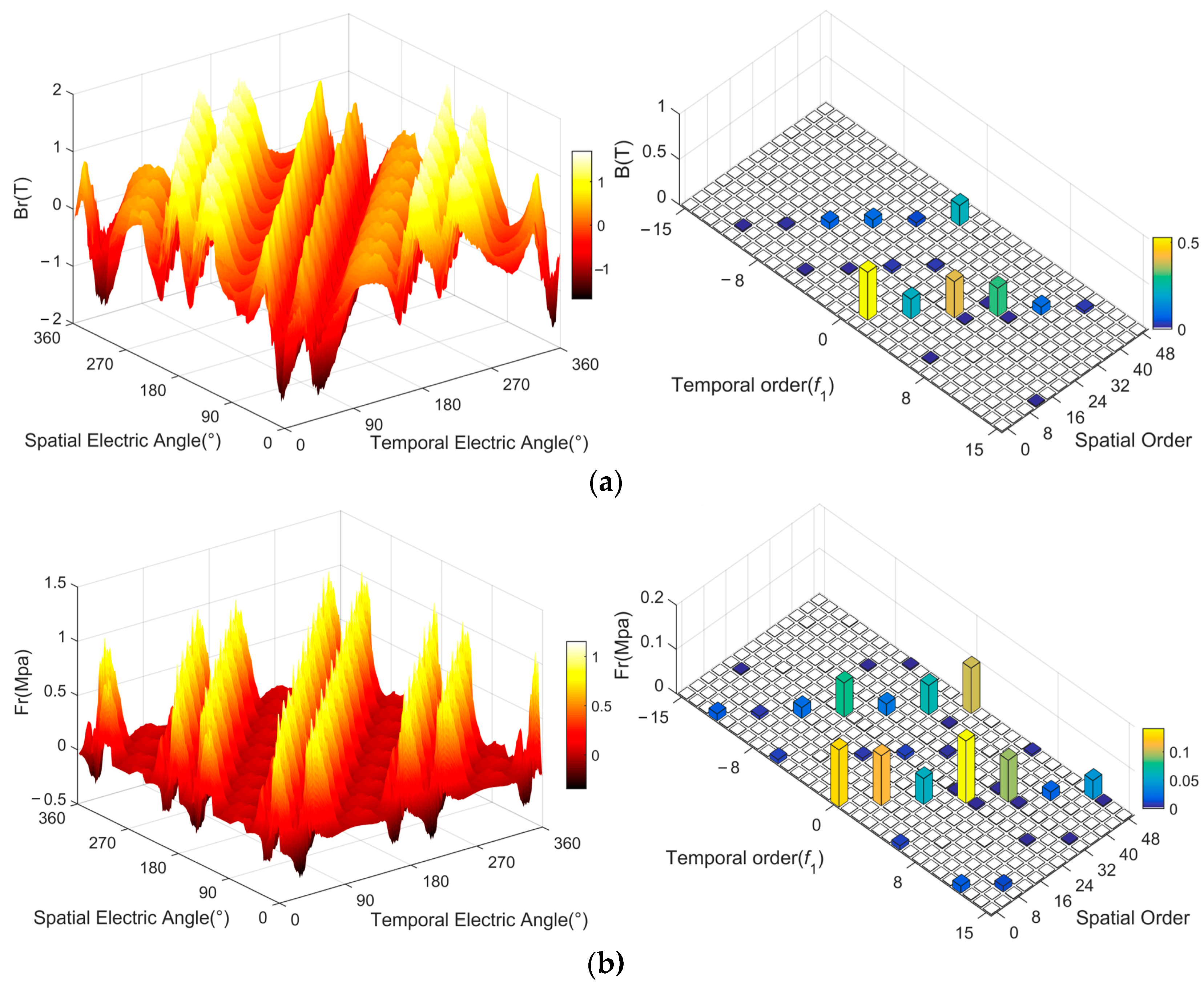
3.2. Air-Gap Electromagnetic-Force Calculation by Electromagnetic-Field FEM
3.3. Acceleration and ERPL Calculation by ESN and Modal Superposition Method
4. Optimization of Electromagnetic Vibration Noise
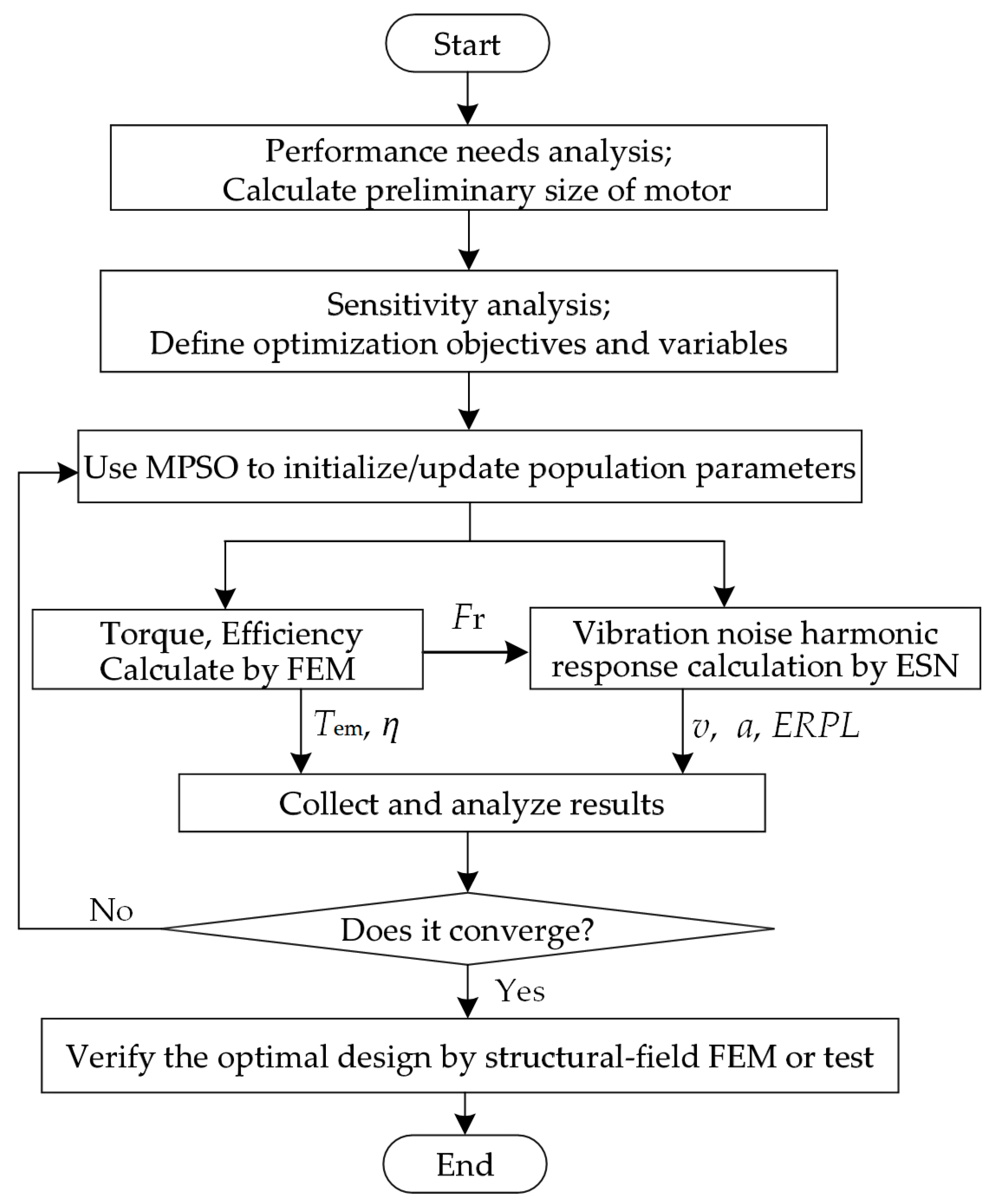
4.1. Optimization Flow
4.2. Sensitivity Analysis
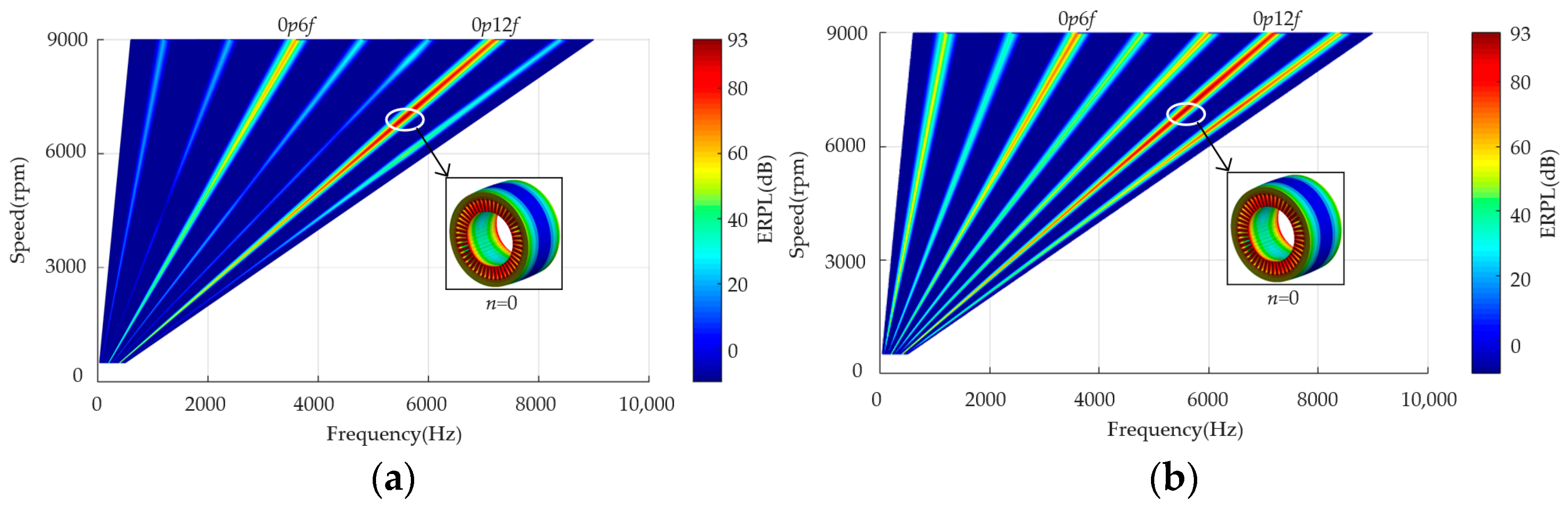
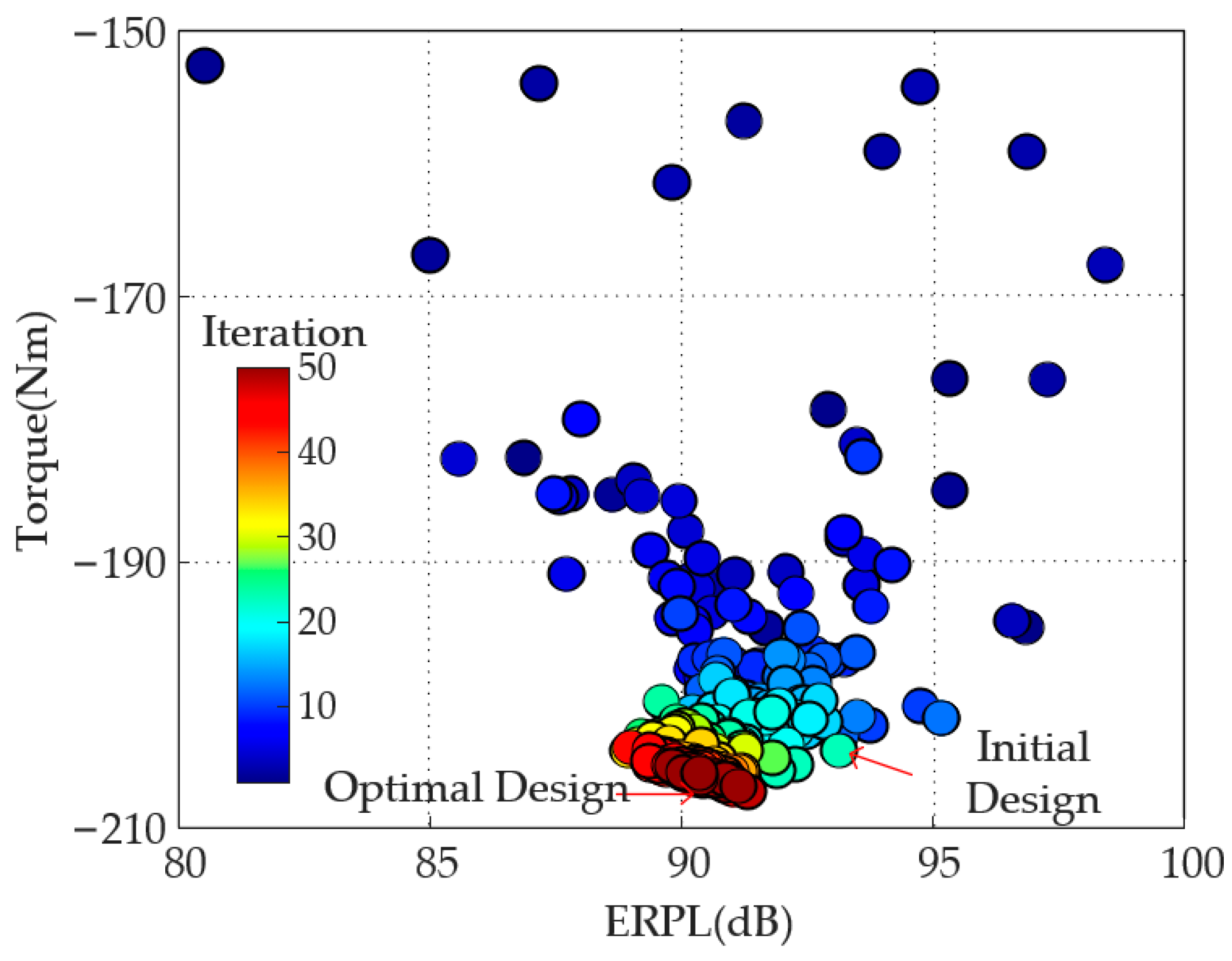
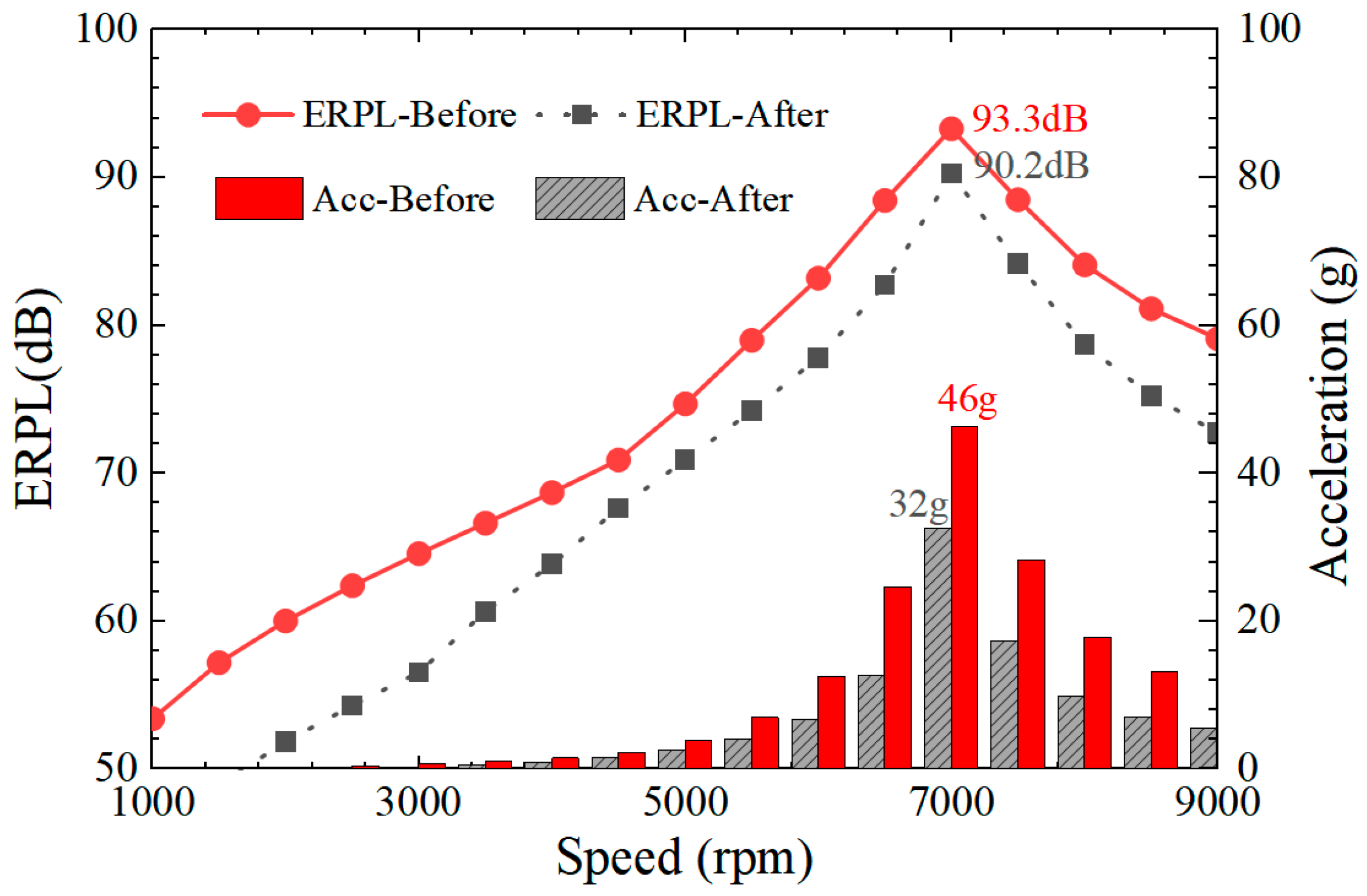
4.3. Optimization Process and Results
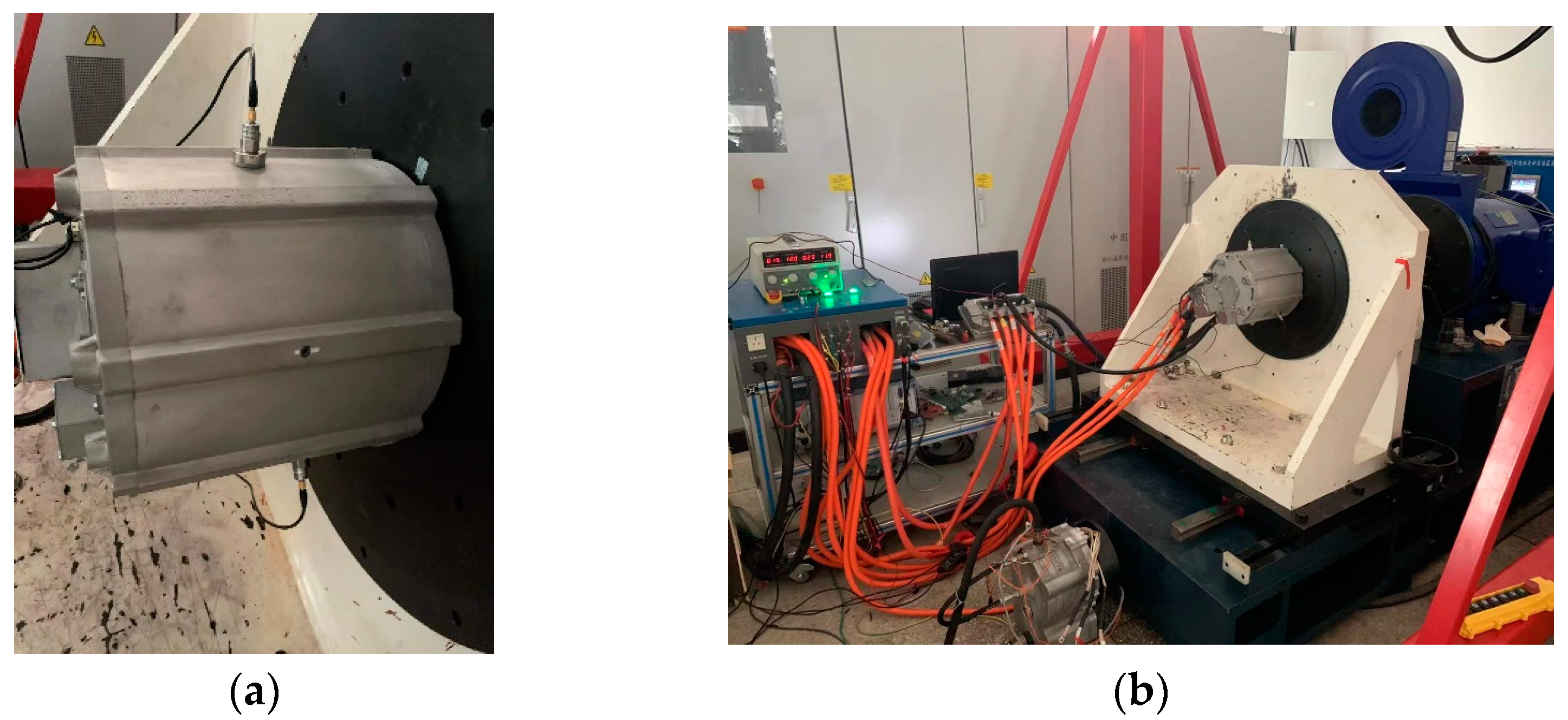
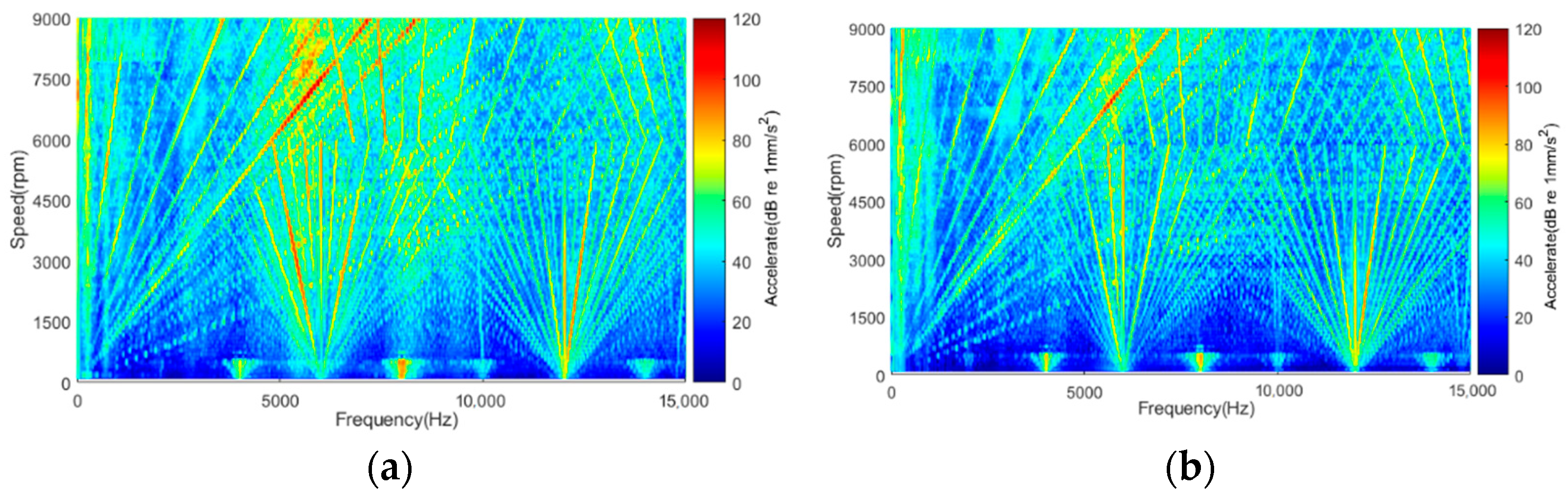
5. Experimental Section
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Husain, I.; Ozpineci, B.; Islam, M. Electric Drive Technology Trends, Challenges and Opportunities for Future Electric Vehicles. Proc. IEEE 2021, 109, 1039–1059. [Google Scholar] [CrossRef]
- Yang, W.; Fang, Z.; He, K. Analysis of Development and Application of In-Wheel Motor System for Electric Vehicle. Appl. Mech. Mater. 2015, 703, 409–412. [Google Scholar] [CrossRef]
- Ifedi, C.; Mecrow, B.; Brockway, S. Fault Tolerant In-Wheel Motor Topologies for High Performance Electric Vehicles. IEEE Trans. Ind. Appl. 2013, 49, 1249–1257. [Google Scholar] [CrossRef]
- Fang, Y.; Zhang, T. Sound Quality Investigation and Improvement of an Electric Powertrain for Electric Vehicles. IEEE Trans. Ind. Electron. 2018, 65, 1149–1157. [Google Scholar] [CrossRef]
- Yang, Z.; Shang, F.; Brown, I.; Krishnamurthy, M. Comparative Study of Interior Permanent Magnet, Induction, and Switched Reluctance Motor Drives for EV and HEV Applications. IEEE Trans. Transport. Electrif. 2015, 1, 245–254. [Google Scholar] [CrossRef]
- McCloskey, A.; Xabier, A.; Xabier, B. Analytical Calculation of Vibrations of Electromagnetic Origin in Electrical Machines. Mech. Syst. Signal. Process. 2018, 98, 557–569. [Google Scholar] [CrossRef]
- Torregrossa, D.; Fahimi, B.; Member, S. Fast Computation of Electromagnetic Vibrations in Electrical Machines via Field Reconstruction Method and Knowledge of Mechanical Impulse Response. IEEE Trans. Ind. Electron. 2012, 59, 839–847. [Google Scholar] [CrossRef]
- Fang, H.; Li, D.; Qu, R.; Yan, P. Modulation Effect of Slotted Structure on Vibration Response in Electrical Machines. IEEE Trans. Ind. Electron. 2019, 66, 2998–3007. [Google Scholar] [CrossRef]
- Ma, C.; Liu, Q.; Wang, D.; Li, Q.; Wang, L. A Novel Black and White Box Method for Diagnosis and Reduction of Abnormal Noise of Hub Permanent-Magnet Synchronous Motors for Electric Vehicles. IEEE Trans. Ind. Electron. 2016, 63, 1153–1167. [Google Scholar] [CrossRef]
- Wang, S.; Hong, J.; Sun, Y.; Cao, H. Analysis of Zeroth-Mode Slot Frequency Vibration of Integer Slot Permanent-Magnet Synchronous Motors. IEEE Trans. Ind. Electron. 2019, 67, 2954–2964. [Google Scholar] [CrossRef]
- Lin, F.; Zuo, S.; Deng, W.; Wu, S. Modeling and Analysis of Electromagnetic Force, Vibration, and Noise in Permanent-Magnet Synchronous Motor Considering Current Harmonics. IEEE Trans. Ind. Electron. 2016, 63, 7455–7466. [Google Scholar] [CrossRef]
- He, S.; Huang, Z.; Qin, R.; Chen, D. Numerical Prediction of Electromagnetic Vibration and Noise of Permanent-Magnet Direct Current Commutator Motors with Rotor Eccentricities and Glue Effects. IEEE Trans. Magn. 2012, 48, 1924–1931. [Google Scholar] [CrossRef]
- Lin, F.; Zuo, S.; Deng, W.; Wu, S. Noise Prediction and Sound Quality Analysis of Variable-Speed Permanent Magnet Synchronous Motor. IEEE Trans. Energy Convers. 2017, 32, 698–706. [Google Scholar] [CrossRef]
- Saito, A.; Kuroishi, M.; Nakai, H. Empirical Vibration Synthesis Method for Electric Machines by Transfer Functions and Electromagnetic Analysis. IEEE Trans. Energy Convers. 2016, 31, 1601–1609. [Google Scholar] [CrossRef]
- Fang, H.; Li, D.; Guo, J.; Xu, Y.; Qu, R. Hybrid Model for Electromagnetic Vibration Synthesis of Electrical Machines Considering Tooth Modulation and Tangential Effects. IEEE Trans. Ind. Electron. 2021, 68, 7284–7293. [Google Scholar] [CrossRef]
- Kawa, M.; Kiyota, K.; Furqani, J.; Chiba, A. Acoustic Noise Reduction of a High-Efficiency Switched Reluctance Motor for Hybrid Electric Vehicles with Novel Current Waveform. IEEE Trans. Ind. Appl. 2019, 55, 2519–2528. [Google Scholar] [CrossRef]
- Cho, S.; Hwang, J.; Kim, C. A Study on Vibration Characteristics of Brushless DC Motor by Electromagnetic-Structural Coupled Analysis Using Entire Finite Element Model. IEEE Trans. Energy Convers. 2019, 33, 1712–1718. [Google Scholar] [CrossRef]
- Gieras, J.; Wang, C.; Lai, J. Noise of Polyphase Electric Motors. Boca Raton; CRC Press: Boca Raton, FL, USA, 2018. [Google Scholar]
- Hu, S.; Zuo, S.; Wu, H.; Liu, M. An Analytical Method for Calculating the Natural Frequencies of a Motor Considering Orthotropic Material Parameters. IEEE Trans. Ind. Electron. 2019, 66, 7520–7528. [Google Scholar] [CrossRef]
- Chai, F.; Li, Y.; Pei, Y. Accurate Modelling and Modal Analysis of Stator System in Permanent Magnet Synchronous Motor with Concentrated Winding for Vibration Prediction. IET Electr. Power. Appl. 2018, 12, 1225–1232. [Google Scholar] [CrossRef]
- McDevitt, T.; Campbell, R.; Jenkins, D. An Investigation of Induction Motor Zeroth-Order Magnetic Stresses, Vibration, And Sound Radiation. IEEE Trans. Magn. 2004, 40, 774–7774. [Google Scholar] [CrossRef]
- Liu, H.; Song, T.; Du, J.; Liu, B. Optimization and Performance Comparison of Hairpin-Winding PMSM for Electric Vehicles Under Drive Cycle. Trans. Nanjing Univ. Aeronaut. Astronaut. 2021, 5, 713–724. [Google Scholar]







| Variables | Value | Variables | Value |
|---|---|---|---|
| OD1/mm | 240 | Hy/mm | 15 |
| OD2/mm | 210 | Ht/mm | 22 |
| OD3/mm | 141 | Bs1/mm | 5.2 |
| Bs2/mm | 4.2 | Slots\poles | 48\8 |
| Parameters | Shell | Stator | Winding |
|---|---|---|---|
| Material | Aluminum alloy | Silicon steel | Copper |
| Density/kg·m−3 | 2700 | 7850 | 8900 |
| Young’s modulus/MPa | 71 | 206 | 95 |
| Poisson’s ratio | 0.33 | 0.35 | 0.33 |
| Mode Number | Test | ESN | FEM | ||
|---|---|---|---|---|---|
| Frequency | Error | Frequency | Error | ||
| n = 0 | 5740 | 5770 | 0.5% | 5695 | −0.8% |
| n = 2 | 647 | 691 | 6.8% | 642 | −0.8% |
| n = 3 | 1750 | 1650 | −5.7% | 1719 | −1.8% |
| n = 4 | 3046 | 2999 | −1.5% | 3038 | 0.2% |
| Variables | Value | Variables | Value |
|---|---|---|---|
| Phase | 3 | Poles | 4 |
| DC voltage | 336 Vdc | AC voltage | 220 Vac |
| Max current | 260 A | Max speed | 9000 rpm |
| Rated torque | 95 Nm | Rated power | 30 kW |
| Max torque | 200 Nm | Peak power | 60 kW |
| Stator OD | 210 mm | Active length | 100 mm |
| Air gap | 0.65 mm |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Song, T.; Liu, H.; Bu, B.; Zhang, Z. Rapid Calculation and Optimization of Vibration and Noise of Permanent-Magnet Synchronous Motors for EVs Based on Equivalent Structural Network. Machines 2022, 10, 281. https://doi.org/10.3390/machines10040281
Song T, Liu H, Bu B, Zhang Z. Rapid Calculation and Optimization of Vibration and Noise of Permanent-Magnet Synchronous Motors for EVs Based on Equivalent Structural Network. Machines. 2022; 10(4):281. https://doi.org/10.3390/machines10040281
Chicago/Turabian StyleSong, Tengfei, Huijuan Liu, Binbin Bu, and Zhenyang Zhang. 2022. "Rapid Calculation and Optimization of Vibration and Noise of Permanent-Magnet Synchronous Motors for EVs Based on Equivalent Structural Network" Machines 10, no. 4: 281. https://doi.org/10.3390/machines10040281
APA StyleSong, T., Liu, H., Bu, B., & Zhang, Z. (2022). Rapid Calculation and Optimization of Vibration and Noise of Permanent-Magnet Synchronous Motors for EVs Based on Equivalent Structural Network. Machines, 10(4), 281. https://doi.org/10.3390/machines10040281





