Forecasting Model Based on Neutrosophic Logical Relationship and Jaccard Similarity
Abstract
:1. Introduction
2. Preliminaries
2.1. Definition of Fuzzy-Fluctuation Time Series (FFTS)
2.2. Basic Concept of Neutrosophic Logical Relationship (NLR)
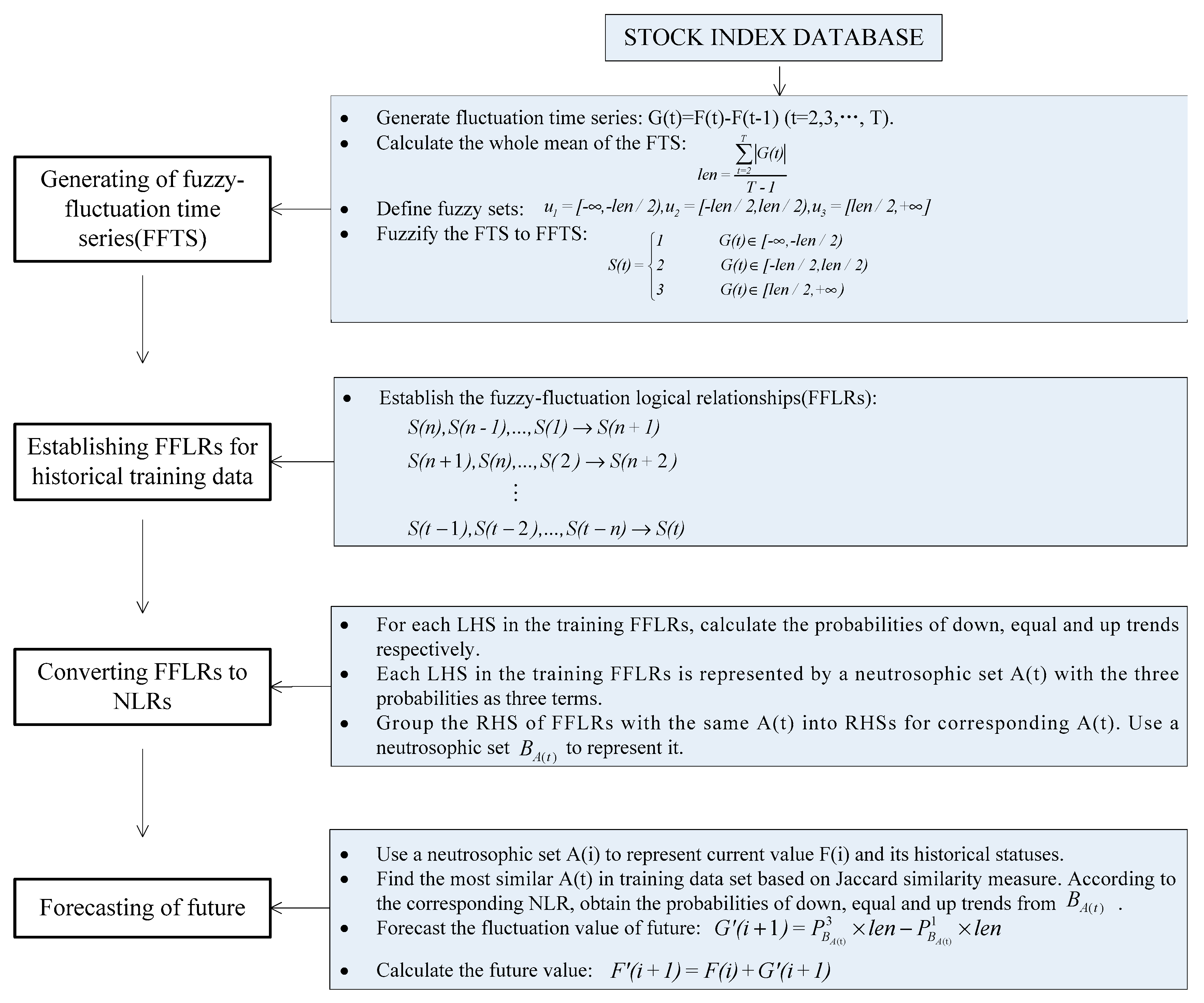
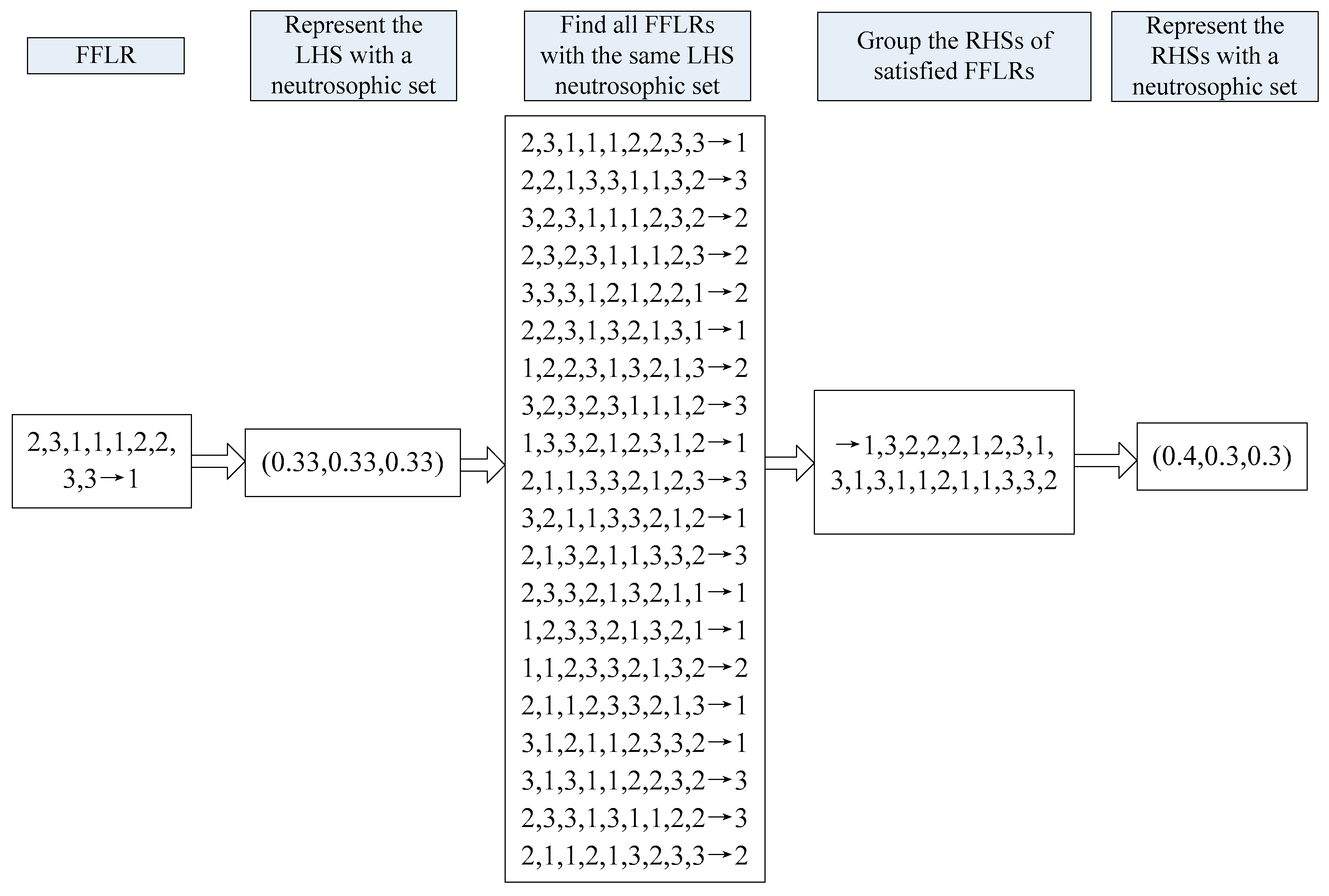
3. A Novel Forecasting Model Based on Neutrosophic Logical Relationships
4. Empirical Analysis
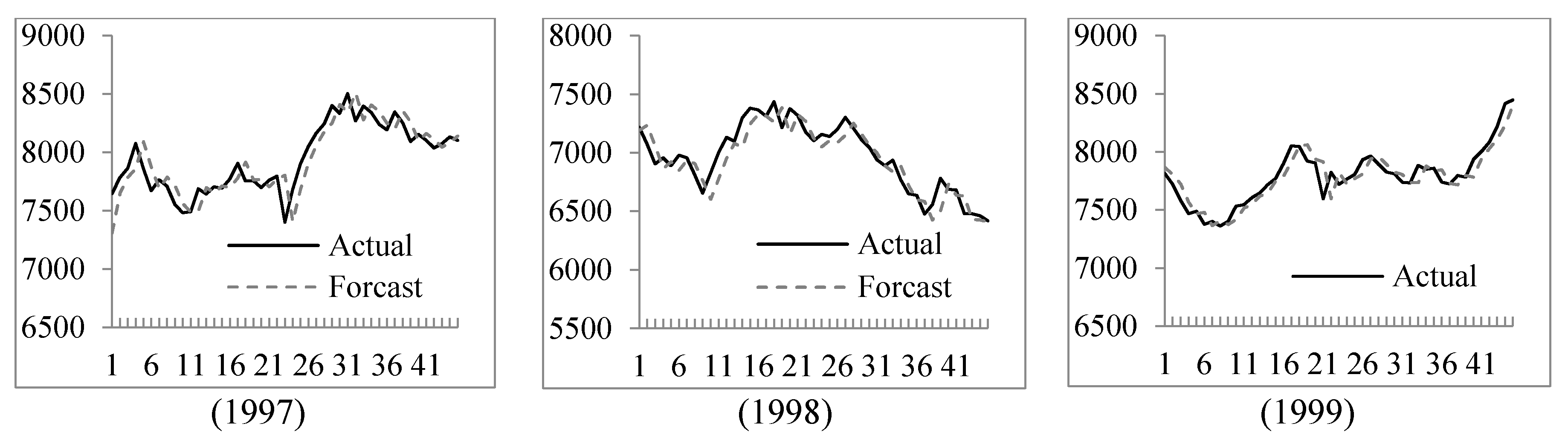
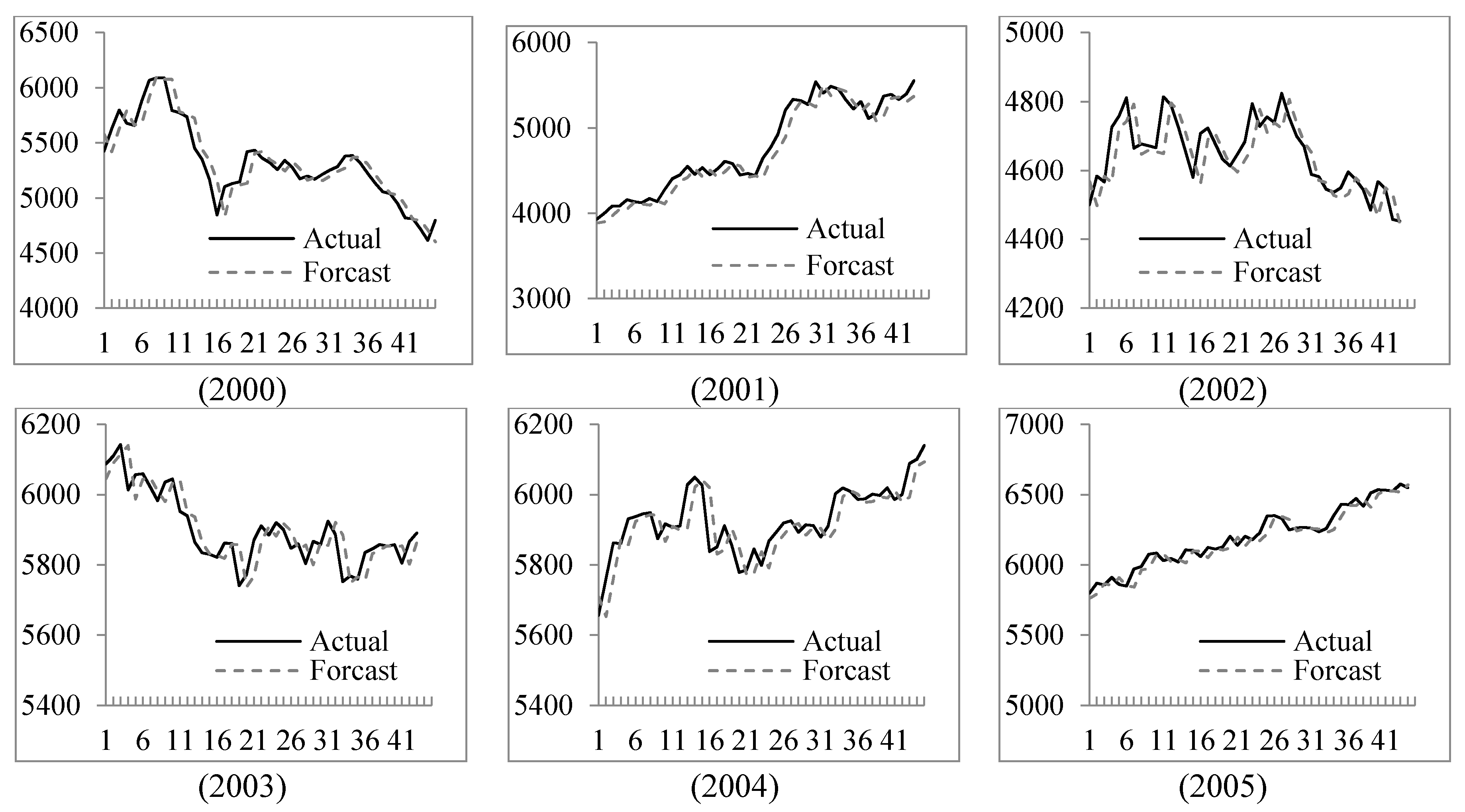
4.1. Forecasting Taiwan Stock Exchange Capitalization Weighted Stock Index
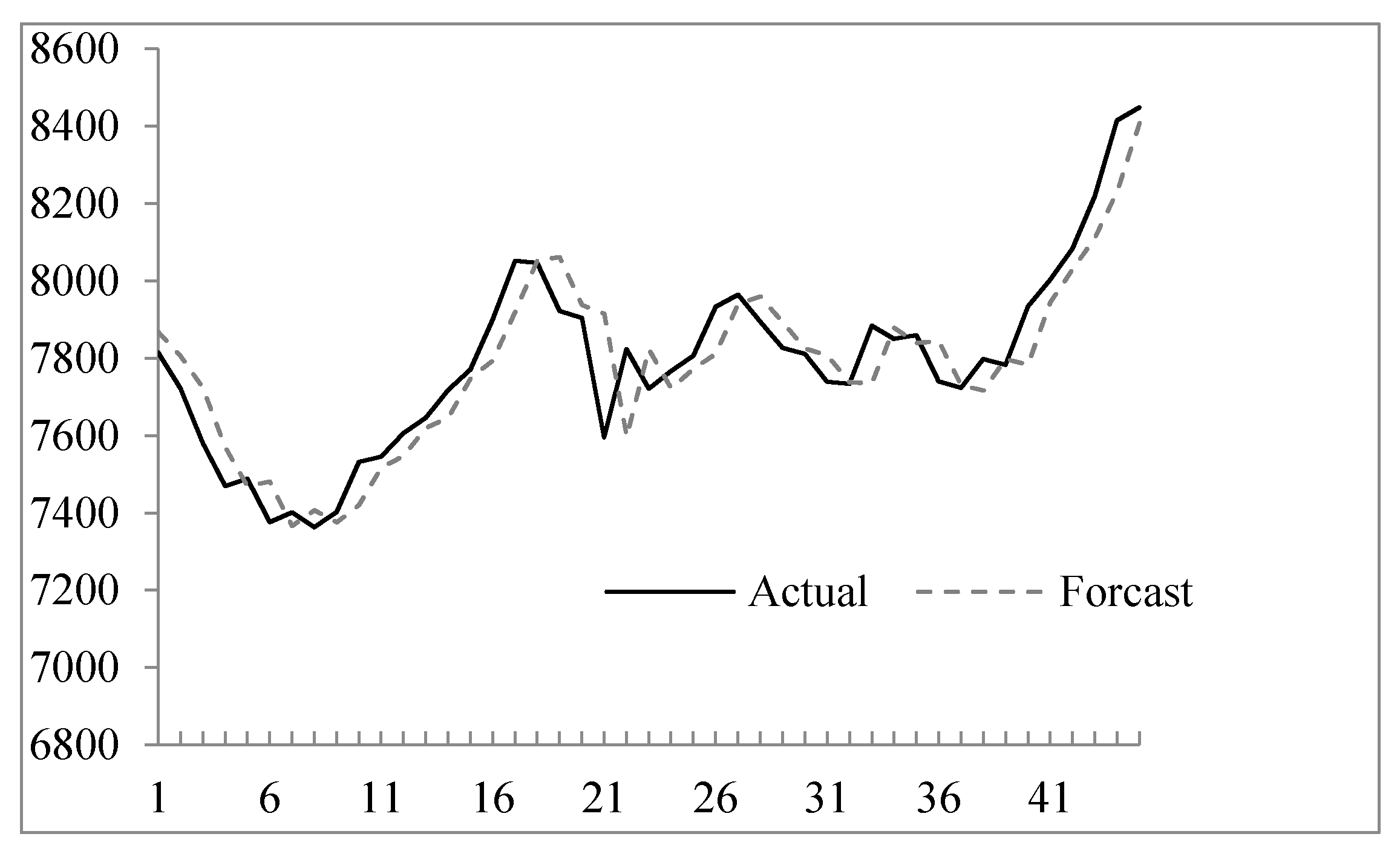
4.2. Forecasting Shanghai Stock Exchange Composite Index
5. Conclusions
Acknowledgments
Author Contributions
Conflicts of Interest
Appendix A
| Date (MM/DD/YYYY) | TAIEX | Fluctuation | Fuzzified | Date (MM/DD/YYYY) | TAIEX | Fluctuation | Fuzzified | Date (MM/DD/YYYY) | TAIEX | Fluctuation | Fuzzified |
|---|---|---|---|---|---|---|---|---|---|---|---|
| 1/5/1999 | 6152.43 | - | - | 4/17/1999 | 7581.5 | 114.68 | 3 | 7/26/1999 | 7595.71 | −128.81 | 1 |
| 1/6/1999 | 6199.91 | 47.48 | 3 | 4/19/1999 | 7623.18 | 41.68 | 2 | 7/27/1999 | 7367.97 | −227.74 | 1 |
| 1/7/1999 | 6404.31 | 204.4 | 3 | 4/20/1999 | 7627.74 | 4.56 | 2 | 7/28/1999 | 7484.5 | 116.53 | 3 |
| 1/8/1999 | 6421.75 | 17.44 | 2 | 4/21/1999 | 7474.16 | −153.58 | 1 | 7/29/1999 | 7359.37 | −125.13 | 1 |
| 1/11/1999 | 6406.99 | −14.76 | 2 | 4/22/1999 | 7494.6 | 20.44 | 2 | 7/30/1999 | 7413.11 | 53.74 | 3 |
| 1/12/1999 | 6363.89 | −43.1 | 1 | 4/23/1999 | 7612.8 | 118.2 | 3 | 7/31/1999 | 7326.75 | −86.36 | 1 |
| 1/13/1999 | 6319.34 | −44.55 | 1 | 4/26/1999 | 7629.09 | 16.29 | 2 | 8/2/1999 | 7195.94 | −130.81 | 1 |
| 1/14/1999 | 6241.32 | −78.02 | 1 | 4/27/1999 | 7550.13 | −78.96 | 1 | 8/3/1999 | 7175.19 | −20.75 | 2 |
| 1/15/1999 | 6454.6 | 213.28 | 3 | 4/28/1999 | 7496.61 | −53.52 | 1 | 8/4/1999 | 7110.8 | −64.39 | 1 |
| 1/16/1999 | 6483.3 | 28.7 | 2 | 4/29/1999 | 7289.62 | −206.99 | 1 | 8/5/1999 | 6959.73 | −151.07 | 1 |
| 1/18/1999 | 6377.25 | −106.05 | 1 | 4/30/1999 | 7371.17 | 81.55 | 3 | 8/6/1999 | 6823.52 | −136.21 | 1 |
| 1/19/1999 | 6343.36 | −33.89 | 2 | 5/3/1999 | 7383.26 | 12.09 | 2 | 8/7/1999 | 7049.74 | 226.22 | 3 |
| 1/20/1999 | 6310.71 | −32.65 | 2 | 5/4/1999 | 7588.04 | 204.78 | 3 | 8/9/1999 | 7028.01 | −21.73 | 2 |
| 1/21/1999 | 6332.2 | 21.49 | 2 | 5/5/1999 | 7572.16 | −15.88 | 2 | 8/10/1999 | 7269.6 | 241.59 | 3 |
| 1/22/1999 | 6228.95 | −103.25 | 1 | 5/6/1999 | 7560.05 | −12.11 | 2 | 8/11/1999 | 7228.68 | −40.92 | 2 |
| 1/25/1999 | 6033.21 | −195.74 | 1 | 5/7/1999 | 7469.33 | −90.72 | 1 | 8/12/1999 | 7330.24 | 101.56 | 3 |
| 1/26/1999 | 6115.64 | 82.43 | 3 | 5/10/1999 | 7484.37 | 15.04 | 2 | 8/13/1999 | 7626.05 | 295.81 | 3 |
| 1/27/1999 | 6138.87 | 23.23 | 2 | 5/11/1999 | 7474.45 | −9.92 | 2 | 8/16/1999 | 8018.47 | 392.42 | 3 |
| 1/28/1999 | 6063.41 | −75.46 | 1 | 5/12/1999 | 7448.41 | −26.04 | 2 | 8/17/1999 | 8083.43 | 64.96 | 3 |
| 1/29/1999 | 5984 | −79.41 | 1 | 5/13/1999 | 7416.2 | −32.21 | 2 | 8/18/1999 | 7993.71 | −89.72 | 1 |
| 1/30/1999 | 5998.32 | 14.32 | 2 | 5/14/1999 | 7592.53 | 176.33 | 3 | 8/19/1999 | 7964.67 | −29.04 | 2 |
| 2/1/1999 | 5862.79 | −135.53 | 1 | 5/15/1999 | 7576.64 | −15.89 | 2 | 8/20/1999 | 8117.42 | 152.75 | 3 |
| 2/2/1999 | 5749.64 | −113.15 | 1 | 5/17/1999 | 7599.76 | 23.12 | 2 | 8/21/1999 | 8153.57 | 36.15 | 2 |
| 2/3/1999 | 5743.86 | −5.78 | 2 | 5/18/1999 | 7585.51 | −14.25 | 2 | 8/23/1999 | 8119.98 | −33.59 | 2 |
| 2/4/1999 | 5514.89 | −228.97 | 1 | 5/19/1999 | 7614.6 | 29.09 | 2 | 8/24/1999 | 7984.39 | −135.59 | 1 |
| 2/5/1999 | 5474.79 | −40.1 | 2 | 5/20/1999 | 7608.88 | −5.72 | 2 | 8/25/1999 | 8127.09 | 142.7 | 3 |
| 2/6/1999 | 5710.18 | 235.39 | 3 | 5/21/1999 | 7606.69 | −2.19 | 2 | 8/26/1999 | 8097.57 | −29.52 | 2 |
| 2/8/1999 | 5822.98 | 112.8 | 3 | 5/24/1999 | 7588.23 | −18.46 | 2 | 8/27/1999 | 8053.97 | −43.6 | 1 |
| 2/9/1999 | 5723.73 | −99.25 | 1 | 5/25/1999 | 7417.03 | −171.2 | 1 | 8/30/1999 | 8071.36 | 17.39 | 2 |
| 2/10/1999 | 5798 | 74.27 | 3 | 5/26/1999 | 7426.63 | 9.6 | 2 | 8/31/1999 | 8157.73 | 86.37 | 3 |
| 2/20/1999 | 6072.33 | 274.33 | 3 | 5/27/1999 | 7469.01 | 42.38 | 2 | 9/1/1999 | 8273.33 | 115.6 | 3 |
| 2/22/1999 | 6313.63 | 241.3 | 3 | 5/28/1999 | 7387.37 | −81.64 | 1 | 9/2/1999 | 8226.15 | −47.18 | 1 |
| 2/23/1999 | 6180.94 | −132.69 | 1 | 5/29/1999 | 7419.7 | 32.33 | 2 | 9/3/1999 | 8073.97 | −152.18 | 1 |
| 2/24/1999 | 6238.87 | 57.93 | 3 | 5/31/1999 | 7316.57 | −103.13 | 1 | 9/4/1999 | 8065.11 | −8.86 | 2 |
| 2/25/1999 | 6275.53 | 36.66 | 2 | 6/1/1999 | 7397.62 | 81.05 | 3 | 9/6/1999 | 8130.28 | 65.17 | 3 |
| 2/26/1999 | 6318.52 | 42.99 | 3 | 6/2/1999 | 7488.03 | 90.41 | 3 | 9/7/1999 | 7945.76 | −184.52 | 1 |
| 3/1/1999 | 6312.25 | −6.27 | 2 | 6/3/1999 | 7572.91 | 84.88 | 3 | 9/8/1999 | 7973.3 | 27.54 | 2 |
| 3/2/1999 | 6263.54 | −48.71 | 1 | 6/4/1999 | 7590.44 | 17.53 | 2 | 9/9/1999 | 8025.02 | 51.72 | 3 |
| 3/3/1999 | 6403.14 | 139.6 | 3 | 6/5/1999 | 7639.3 | 48.86 | 3 | 9/10/1999 | 8161.46 | 136.44 | 3 |
| 3/4/1999 | 6393.74 | −9.4 | 2 | 6/7/1999 | 7802.69 | 163.39 | 3 | 9/13/1999 | 8178.69 | 17.23 | 2 |
| 3/5/1999 | 6383.09 | −10.65 | 2 | 6/8/1999 | 7892.13 | 89.44 | 3 | 9/14/1999 | 8092.02 | −86.67 | 1 |
| 3/6/1999 | 6421.73 | 38.64 | 2 | 6/9/1999 | 7957.71 | 65.58 | 3 | 9/15/1999 | 7971.04 | −120.98 | 1 |
| 3/8/1999 | 6431.96 | 10.23 | 2 | 6/10/1999 | 7996.76 | 39.05 | 2 | 9/16/1999 | 7968.9 | −2.14 | 2 |
| 3/9/1999 | 6493.43 | 61.47 | 3 | 6/11/1999 | 7979.4 | −17.36 | 2 | 9/17/1999 | 7916.92 | −51.98 | 1 |
| 3/10/1999 | 6486.61 | −6.82 | 2 | 6/14/1999 | 7973.58 | −5.82 | 2 | 9/18/1999 | 8016.93 | 100.01 | 3 |
| 3/11/1999 | 6436.8 | −49.81 | 1 | 6/15/1999 | 7960 | −13.58 | 2 | 9/20/1999 | 7972.14 | −44.79 | 1 |
| 3/12/1999 | 6462.73 | 25.93 | 2 | 6/16/1999 | 8059.02 | 99.02 | 3 | 9/27/1999 | 7759.93 | −212.21 | 1 |
| 3/15/1999 | 6598.32 | 135.59 | 3 | 6/17/1999 | 8274.36 | 215.34 | 3 | 9/28/1999 | 7577.85 | −182.08 | 1 |
| 3/16/1999 | 6672.23 | 73.91 | 3 | 6/21/1999 | 8413.48 | 139.12 | 3 | 9/29/1999 | 7615.45 | 37.6 | 2 |
| 3/17/1999 | 6757.07 | 84.84 | 3 | 6/22/1999 | 8608.91 | 195.43 | 3 | 9/30/1999 | 7598.79 | −16.66 | 2 |
| 3/18/1999 | 6895.01 | 137.94 | 3 | 6/23/1999 | 8492.32 | −116.59 | 1 | 10/1/1999 | 7694.99 | 96.2 | 3 |
| 3/19/1999 | 6997.29 | 102.28 | 3 | 6/24/1999 | 8589.31 | 96.99 | 3 | 10/2/1999 | 7659.55 | −35.44 | 2 |
| 3/20/1999 | 6993.38 | −3.91 | 2 | 6/25/1999 | 8265.96 | −323.35 | 1 | 10/4/1999 | 7685.48 | 25.93 | 2 |
| 3/22/1999 | 7043.23 | 49.85 | 3 | 6/28/1999 | 8281.45 | 15.49 | 2 | 10/5/1999 | 7557.01 | −128.47 | 1 |
| 3/23/1999 | 6945.48 | −97.75 | 1 | 6/29/1999 | 8514.27 | 232.82 | 3 | 10/6/1999 | 7501.63 | −55.38 | 1 |
| 3/24/1999 | 6889.42 | −56.06 | 1 | 6/30/1999 | 8467.37 | −46.9 | 1 | 10/7/1999 | 7612 | 110.37 | 3 |
| 3/25/1999 | 6941.38 | 51.96 | 3 | 7/2/1999 | 8572.09 | 104.72 | 3 | 10/8/1999 | 7552.98 | −59.02 | 1 |
| 3/26/1999 | 7033.25 | 91.87 | 3 | 7/3/1999 | 8563.55 | −8.54 | 2 | 10/11/1999 | 7607.11 | 54.13 | 3 |
| 3/29/1999 | 6901.68 | −131.57 | 1 | 7/5/1999 | 8593.35 | 29.8 | 2 | 10/12/1999 | 7835.37 | 228.26 | 3 |
| 3/30/1999 | 6898.66 | −3.02 | 2 | 7/6/1999 | 8454.49 | −138.86 | 1 | 10/13/1999 | 7836.94 | 1.57 | 2 |
| 3/31/1999 | 6881.72 | −16.94 | 2 | 7/7/1999 | 8470.07 | 15.58 | 2 | 10/14/1999 | 7879.91 | 42.97 | 3 |
| 4/1/1999 | 7018.68 | 136.96 | 3 | 7/8/1999 | 8592.43 | 122.36 | 3 | 10/15/1999 | 7819.09 | −60.82 | 1 |
| 4/2/1999 | 7232.51 | 213.83 | 3 | 7/9/1999 | 8550.27 | −42.16 | 2 | 10/16/1999 | 7829.39 | 10.3 | 2 |
| 4/3/1999 | 7182.2 | −50.31 | 1 | 7/12/1999 | 8463.9 | −86.37 | 1 | 10/18/1999 | 7745.26 | −84.13 | 1 |
| 4/6/1999 | 7163.99 | −18.21 | 2 | 7/13/1999 | 8204.5 | −259.4 | 1 | 10/19/1999 | 7692.96 | −52.3 | 1 |
| 4/7/1999 | 7135.89 | −28.1 | 2 | 7/14/1999 | 7888.66 | −315.84 | 1 | 10/20/1999 | 7666.64 | −26.32 | 2 |
| 4/8/1999 | 7273.41 | 137.52 | 3 | 7/15/1999 | 7918.04 | 29.38 | 2 | 10/21/1999 | 7654.9 | −11.74 | 2 |
| 4/9/1999 | 7265.7 | −7.71 | 2 | 7/16/1999 | 7411.58 | −506.46 | 1 | 10/22/1999 | 7559.63 | −95.27 | 1 |
| 4/12/1999 | 7242.4 | −23.3 | 2 | 7/17/1999 | 7366.23 | −45.35 | 1 | 10/25/1999 | 7680.87 | 121.24 | 3 |
| 4/13/1999 | 7337.85 | 95.45 | 3 | 7/19/1999 | 7386.89 | 20.66 | 2 | 10/26/1999 | 7700.29 | 19.42 | 2 |
| 4/14/1999 | 7398.65 | 60.8 | 3 | 7/20/1999 | 7806.85 | 419.96 | 3 | 10/27/1999 | 7701.22 | 0.93 | 2 |
| 4/15/1999 | 7498.17 | 99.52 | 3 | 7/21/1999 | 7786.65 | −20.2 | 2 | 10/28/1999 | 7681.85 | −19.37 | 2 |
| 4/16/1999 | 7466.82 | −31.35 | 2 | 7/22/1999 | 7678.67 | −107.98 | 1 | 10/29/1999 | 7706.67 | 24.82 | 2 |
| 4/17/1999 | 7581.5 | 114.68 | 3 | 7/23/1999 | 7724.52 | 45.85 | 3 | 10/30/1999 | 7854.85 | 148.18 | 3 |
| Date (MM/DD/YYYY) | FFLR | LHS of NLR | Date (MM/DD/YYYY) | FFLR | LHS of NLR | Date (MM/DD/YYYY) | FFLR | LHS of NLR | Date (MM/DD/YYYY) | FFLR | LHS of NLR |
|---|---|---|---|---|---|---|---|---|---|---|---|
| 1/18/1999 | 2,3,1,1,1,2,2,3,3→2 | (0.33,0.33,0.33) | 4/3/1999 | 3,3,2,2,1,3,3,1,1→3 | (0.33,0.22,0.44) | 6/11/1999 | 2,3,3,3,3,2,3,3,3→2 | (0,0.22,0.78) | 8/21/1999 | 3,2,1,3,3,3,3,2,3→3 | (0.11,0.22,0.67) |
| 1/19/1999 | 1,2,3,1,1,1,2,2,3→1 | (0.44,0.33,0.22) | 4/6/1999 | 1,3,3,2,2,1,3,3,1→1 | (0.33,0.22,0.44) | 6/14/1999 | 2,2,3,3,3,3,2,3,3→2 | (0,0.33,0.67) | 8/23/1999 | 2,3,2,1,3,3,3,3,2→2 | (0.11,0.33,0.56) |
| 1/20/1999 | 2,1,2,3,1,1,1,2,2→2 | (0.44,0.44,0.11) | 4/7/1999 | 2,1,3,3,2,2,1,3,3→2 | (0.22,0.33,0.44) | 6/15/1999 | 2,2,2,3,3,3,3,2,3→2 | (0,0.44,0.56) | 8/24/1999 | 2,2,3,2,1,3,3,3,3→2 | (0.11,0.33,0.56) |
| 1/21/1999 | 2,2,1,2,3,1,1,1,2→2 | (0.44,0.44,0.11) | 4/8/1999 | 2,2,1,3,3,2,2,1,3→2 | (0.22,0.44,0.33) | 6/16/1999 | 2,2,2,2,3,3,3,3,2→2 | (0,0.56,0.44) | 8/25/1999 | 1,2,2,3,2,1,3,3,3→1 | (0.22,0.33,0.44) |
| 1/22/1999 | 2,2,2,1,2,3,1,1,1→2 | (0.44,0.44,0.11) | 4/9/1999 | 3,2,2,1,3,3,2,2,1→3 | (0.22,0.44,0.33) | 6/17/1999 | 3,2,2,2,2,3,3,3,3→3 | (0,0.44,0.56) | 8/26/1999 | 3,1,2,2,3,2,1,3,3→3 | (0.22,0.33,0.44) |
| 1/25/1999 | 1,2,2,2,1,2,3,1,1→1 | (0.44,0.44,0.11) | 4/12/1999 | 2,3,2,2,1,3,3,2,2→2 | (0.11,0.56,0.33) | 6/21/1999 | 3,3,2,2,2,2,3,3,3→3 | (0,0.44,0.56) | 8/27/1999 | 2,3,1,2,2,3,2,1,3→2 | (0.22,0.44,0.33) |
| 1/26/1999 | 1,1,2,2,2,1,2,3,1→1 | (0.44,0.44,0.11) | 4/13/1999 | 2,2,3,2,2,1,3,3,2→2 | (0.11,0.56,0.33) | 6/22/1999 | 3,3,3,2,2,2,2,3,3→3 | (0,0.44,0.56) | 8/30/1999 | 1,2,3,1,2,2,3,2,1→1 | (0.33,0.44,0.22) |
| 1/27/1999 | 3,1,1,2,2,2,1,2,3→3 | (0.33,0.44,0.22) | 4/14/1999 | 3,2,2,3,2,2,1,3,3→3 | (0.11,0.44,0.44) | 6/23/1999 | 3,3,3,3,2,2,2,2,3→3 | (0,0.44,0.56) | 8/31/1999 | 2,1,2,3,1,2,2,3,2→2 | (0.22,0.56,0.22) |
| 1/28/1999 | 2,3,1,1,2,2,2,1,2→2 | (0.33,0.56,0.11) | 4/15/1999 | 3,3,2,2,3,2,2,1,3→3 | (0.11,0.44,0.44) | 6/24/1999 | 1,3,3,3,3,2,2,2,2→1 | (0.11,0.44,0.44) | 9/1/1999 | 3,2,1,2,3,1,2,2,3→3 | (0.22,0.44,0.33) |
| 1/29/1999 | 1,2,3,1,1,2,2,2,1→1 | (0.44,0.44,0.11) | 4/16/1999 | 3,3,3,2,2,3,2,2,1→3 | (0.11,0.44,0.44) | 6/25/1999 | 3,1,3,3,3,3,2,2,2→3 | (0.11,0.33,0.56) | 9/2/1999 | 3,3,2,1,2,3,1,2,2→3 | (0.22,0.44,0.33) |
| 1/30/1999 | 1,1,2,3,1,1,2,2,2→1 | (0.44,0.44,0.11) | 4/17/1999 | 2,3,3,3,2,2,3,2,2→2 | (0,0.56,0.44) | 6/28/1999 | 1,3,1,3,3,3,3,2,2→1 | (0.22,0.22,0.56) | 9/3/1999 | 1,3,3,2,1,2,3,1,2→1 | (0.33,0.33,0.33) |
| 2/1/1999 | 2,1,1,2,3,1,1,2,2→2 | (0.44,0.44,0.11) | 4/19/1999 | 3,2,3,3,3,2,2,3,2→3 | (0,0.44,0.56) | 6/29/1999 | 2,1,3,1,3,3,3,3,2→2 | (0.22,0.22,0.56) | 9/4/1999 | 1,1,3,3,2,1,2,3,1→1 | (0.44,0.22,0.33) |
| 2/2/1999 | 1,2,1,1,2,3,1,1,2→1 | (0.56,0.33,0.11) | 4/20/1999 | 2,3,2,3,3,3,2,2,3→2 | (0,0.44,0.56) | 6/30/1999 | 3,2,1,3,1,3,3,3,3→3 | (0.22,0.11,0.67) | 9/6/1999 | 2,1,1,3,3,2,1,2,3→2 | (0.33,0.33,0.33) |
| 2/3/1999 | 1,1,2,1,1,2,3,1,1→1 | (0.67,0.22,0.11) | 4/21/1999 | 2,2,3,2,3,3,3,2,2→2 | (0,0.56,0.44) | 7/2/1999 | 1,3,2,1,3,1,3,3,3→1 | (0.33,0.11,0.56) | 9/7/1999 | 3,2,1,1,3,3,2,1,2→3 | (0.33,0.33,0.33) |
| 2/4/1999 | 2,1,1,2,1,1,2,3,1→2 | (0.56,0.33,0.11) | 4/22/1999 | 1,2,2,3,2,3,3,3,2→1 | (0.11,0.44,0.44) | 7/3/1999 | 3,1,3,2,1,3,1,3,3→3 | (0.33,0.11,0.56) | 9/8/1999 | 1,3,2,1,1,3,3,2,1→1 | (0.44,0.22,0.33) |
| 2/5/1999 | 1,2,1,1,2,1,1,2,3→1 | (0.56,0.33,0.11) | 4/23/1999 | 2,1,2,2,3,2,3,3,3→2 | (0.11,0.44,0.44) | 7/5/1999 | 2,3,1,3,2,1,3,1,3→2 | (0.33,0.22,0.44) | 9/9/1999 | 2,1,3,2,1,1,3,3,2→2 | (0.33,0.33,0.33) |
| 2/6/1999 | 2,1,2,1,1,2,1,1,2→2 | (0.56,0.44,0) | 4/26/1999 | 3,2,1,2,2,3,2,3,3→3 | (0.11,0.44,0.44) | 7/6/1999 | 2,2,3,1,3,2,1,3,1→2 | (0.33,0.33,0.33) | 9/10/1999 | 3,2,1,3,2,1,1,3,3→3 | (0.33,0.22,0.44) |
| 2/8/1999 | 3,2,1,2,1,1,2,1,1→3 | (0.56,0.33,0.11) | 4/27/1999 | 2,3,2,1,2,2,3,2,3→2 | (0.11,0.56,0.33) | 7/7/1999 | 1,2,2,3,1,3,2,1,3→1 | (0.33,0.33,0.33) | 9/13/1999 | 3,3,2,1,3,2,1,1,3→3 | (0.33,0.22,0.44) |
| 2/9/1999 | 3,3,2,1,2,1,1,2,1→3 | (0.44,0.33,0.22) | 4/28/1999 | 1,2,3,2,1,2,2,3,2→1 | (0.22,0.56,0.22) | 7/8/1999 | 2,1,2,2,3,1,3,2,1→2 | (0.33,0.44,0.22) | 9/14/1999 | 2,3,3,2,1,3,2,1,1→2 | (0.33,0.33,0.33) |
| 2/10/1999 | 1,3,3,2,1,2,1,1,2→1 | (0.44,0.33,0.22) | 4/29/1999 | 1,1,2,3,2,1,2,2,3→1 | (0.33,0.44,0.22) | 7/9/1999 | 3,2,1,2,2,3,1,3,2→3 | (0.22,0.44,0.33) | 9/15/1999 | 1,2,3,3,2,1,3,2,1→1 | (0.33,0.33,0.33) |
| 2/20/1999 | 3,1,3,3,2,1,2,1,1→3 | (0.44,0.22,0.33) | 4/30/1999 | 1,1,1,2,3,2,1,2,2→1 | (0.44,0.44,0.11) | 7/12/1999 | 2,3,2,1,2,2,3,1,3→2 | (0.22,0.44,0.33) | 9/16/1999 | 1,1,2,3,3,2,1,3,2→1 | (0.33,0.33,0.33) |
| 2/22/1999 | 3,3,1,3,3,2,1,2,1→3 | (0.33,0.22,0.44) | 5/3/1999 | 3,1,1,1,2,3,2,1,2→3 | (0.44,0.33,0.22) | 7/13/1999 | 1,2,3,2,1,2,2,3,1→1 | (0.33,0.44,0.22) | 9/17/1999 | 2,1,1,2,3,3,2,1,3→2 | (0.33,0.33,0.33) |
| 2/23/1999 | 3,3,3,1,3,3,2,1,2→3 | (0.22,0.22,0.56) | 5/4/1999 | 2,3,1,1,1,2,3,2,1→2 | (0.44,0.33,0.22) | 7/14/1999 | 1,1,2,3,2,1,2,2,3→1 | (0.33,0.44,0.22) | 9/18/1999 | 1,2,1,1,2,3,3,2,1→1 | (0.44,0.33,0.22) |
| 2/24/1999 | 1,3,3,3,1,3,3,2,1→1 | (0.33,0.11,0.56) | 5/5/1999 | 3,2,3,1,1,1,2,3,2→3 | (0.33,0.33,0.33) | 7/15/1999 | 1,1,1,2,3,2,1,2,2→1 | (0.44,0.44,0.11) | 9/20/1999 | 3,1,2,1,1,2,3,3,2→3 | (0.33,0.33,0.33) |
| 2/25/1999 | 3,1,3,3,3,1,3,3,2→3 | (0.22,0.11,0.67) | 5/6/1999 | 2,3,2,3,1,1,1,2,3→2 | (0.33,0.33,0.33) | 7/16/1999 | 2,1,1,1,2,3,2,1,2→2 | (0.44,0.44,0.11) | 9/27/1999 | 1,3,1,2,1,1,2,3,3→1 | (0.44,0.22,0.33) |
| 2/26/1999 | 2,3,1,3,3,3,1,3,3→2 | (0.22,0.11,0.67) | 5/7/1999 | 2,2,3,2,3,1,1,1,2→2 | (0.33,0.44,0.22) | 7/17/1999 | 1,2,1,1,1,2,3,2,1→1 | (0.56,0.33,0.11) | 9/28/1999 | 1,1,3,1,2,1,1,2,3→1 | (0.56,0.22,0.22) |
| 3/1/1999 | 3,2,3,1,3,3,3,1,3→3 | (0.22,0.11,0.67) | 5/10/1999 | 1,2,2,3,2,3,1,1,1→1 | (0.44,0.33,0.22) | 7/19/1999 | 1,1,2,1,1,1,2,3,2→1 | (0.56,0.33,0.11) | 9/29/1999 | 1,1,1,3,1,2,1,1,2→1 | (0.67,0.22,0.11) |
| 3/2/1999 | 2,3,2,3,1,3,3,3,1→2 | (0.22,0.22,0.56) | 5/11/1999 | 2,1,2,2,3,2,3,1,1→2 | (0.33,0.44,0.22) | 7/20/1999 | 2,1,1,2,1,1,1,2,3→2 | (0.56,0.33,0.11) | 9/30/1999 | 2,1,1,1,3,1,2,1,1→2 | (0.67,0.22,0.11) |
| 3/3/1999 | 1,2,3,2,3,1,3,3,3→1 | (0.22,0.22,0.56) | 5/12/1999 | 2,2,1,2,2,3,2,3,1→2 | (0.22,0.56,0.22) | 7/21/1999 | 3,2,1,1,2,1,1,1,2→3 | (0.56,0.33,0.11) | 10/1/1999 | 2,2,1,1,1,3,1,2,1→2 | (0.56,0.33,0.11) |
| 3/4/1999 | 3,1,2,3,2,3,1,3,3→3 | (0.22,0.22,0.56) | 5/13/1999 | 2,2,2,1,2,2,3,2,3→2 | (0.11,0.67,0.22) | 7/22/1999 | 2,3,2,1,1,2,1,1,1→2 | (0.56,0.33,0.11) | 10/2/1999 | 3,2,2,1,1,1,3,1,2→3 | (0.44,0.33,0.22) |
| 3/5/1999 | 2,3,1,2,3,2,3,1,3→2 | (0.22,0.33,0.44) | 5/14/1999 | 2,2,2,2,1,2,2,3,2→2 | (0.11,0.78,0.11) | 7/23/1999 | 1,2,3,2,1,1,2,1,1→1 | (0.56,0.33,0.11) | 10/4/1999 | 2,3,2,2,1,1,1,3,1→2 | (0.44,0.33,0.22) |
| 3/6/1999 | 2,2,3,1,2,3,2,3,1→2 | (0.22,0.44,0.33) | 5/15/1999 | 3,2,2,2,2,1,2,2,3→3 | (0.11,0.67,0.22) | 7/26/1999 | 3,1,2,3,2,1,1,2,1→3 | (0.44,0.33,0.22) | 10/5/1999 | 2,2,3,2,2,1,1,1,3→2 | (0.33,0.44,0.22) |
| 3/8/1999 | 2,2,2,3,1,2,3,2,3→2 | (0.11,0.56,0.33) | 5/17/1999 | 2,3,2,2,2,2,1,2,2→2 | (0.11,0.78,0.11) | 7/27/1999 | 1,3,1,2,3,2,1,1,2→1 | (0.44,0.33,0.22) | 10/6/1999 | 1,2,2,3,2,2,1,1,1→1 | (0.44,0.44,0.11) |
| 3/9/1999 | 2,2,2,2,3,1,2,3,2→2 | (0.11,0.67,0.22) | 5/18/1999 | 2,2,3,2,2,2,2,1,2→2 | (0.11,0.78,0.11) | 7/28/1999 | 1,1,3,1,2,3,2,1,1→1 | (0.56,0.22,0.22) | 10/7/1999 | 1,1,2,2,3,2,2,1,1→1 | (0.44,0.44,0.11) |
| 3/10/1999 | 3,2,2,2,2,3,1,2,3→3 | (0.11,0.56,0.33) | 5/19/1999 | 2,2,2,3,2,2,2,2,1→2 | (0.11,0.78,0.11) | 7/29/1999 | 3,1,1,3,1,2,3,2,1→3 | (0.44,0.22,0.33) | 10/8/1999 | 3,1,1,2,2,3,2,2,1→3 | (0.33,0.44,0.22) |
| 3/11/1999 | 2,3,2,2,2,2,3,1,2→2 | (0.11,0.67,0.22) | 5/20/1999 | 2,2,2,2,3,2,2,2,2→2 | (0,0.89,0.11) | 7/30/1999 | 1,3,1,1,3,1,2,3,2→1 | (0.44,0.22,0.33) | 10/11/1999 | 1,3,1,1,2,2,3,2,2→1 | (0.33,0.44,0.22) |
| 3/12/1999 | 1,2,3,2,2,2,2,3,1→1 | (0.22,0.56,0.22) | 5/21/1999 | 2,2,2,2,2,3,2,2,2→2 | (0,0.89,0.11) | 7/31/1999 | 3,1,3,1,1,3,1,2,3→3 | (0.44,0.11,0.44) | 10/12/1999 | 3,1,3,1,1,2,2,3,2→3 | (0.33,0.33,0.33) |
| 3/15/1999 | 2,1,2,3,2,2,2,2,3→2 | (0.11,0.67,0.22) | 5/24/1999 | 2,2,2,2,2,2,3,2,2→2 | (0,0.89,0.11) | 8/2/1999 | 1,3,1,3,1,1,3,1,2→1 | (0.56,0.11,0.33) | 10/13/1999 | 3,3,1,3,1,1,2,2,3→3 | (0.33,0.22,0.44) |
| 3/16/1999 | 3,2,1,2,3,2,2,2,2→3 | (0.11,0.67,0.22) | 5/25/1999 | 2,2,2,2,2,2,2,3,2→2 | (0,0.89,0.11) | 8/3/1999 | 1,1,3,1,3,1,1,3,1→1 | (0.67,0,0.33) | 10/14/1999 | 2,3,3,1,3,1,1,2,2→2 | (0.33,0.33,0.33) |
| 3/17/1999 | 3,3,2,1,2,3,2,2,2→3 | (0.11,0.56,0.33) | 5/26/1999 | 1,2,2,2,2,2,2,2,3→1 | (0.11,0.78,0.11) | 8/4/1999 | 2,1,1,3,1,3,1,1,3→2 | (0.56,0.11,0.33) | 10/15/1999 | 3,2,3,3,1,3,1,1,2→3 | (0.33,0.22,0.44) |
| 3/18/1999 | 3,3,3,2,1,2,3,2,2→3 | (0.11,0.44,0.44) | 5/27/1999 | 2,1,2,2,2,2,2,2,2→2 | (0.11,0.89,0) | 8/5/1999 | 1,2,1,1,3,1,3,1,1→1 | (0.67,0.11,0.22) | 10/16/1999 | 1,3,2,3,3,1,3,1,1→1 | (0.44,0.11,0.44) |
| 3/19/1999 | 3,3,3,3,2,1,2,3,2→3 | (0.11,0.33,0.56) | 5/28/1999 | 2,2,1,2,2,2,2,2,2→2 | (0.11,0.89,0) | 8/6/1999 | 1,1,2,1,1,3,1,3,1→1 | (0.67,0.11,0.22) | 10/18/1999 | 2,1,3,2,3,3,1,3,1→2 | (0.33,0.22,0.44) |
| 3/20/1999 | 3,3,3,3,3,2,1,2,3→3 | (0.11,0.22,0.67) | 5/29/1999 | 1,2,2,1,2,2,2,2,2→1 | (0.22,0.78,0) | 8/7/1999 | 1,1,1,2,1,1,3,1,3→1 | (0.67,0.11,0.22) | 10/19/1999 | 1,2,1,3,2,3,3,1,3→1 | (0.33,0.22,0.44) |
| 3/22/1999 | 2,3,3,3,3,3,2,1,2→2 | (0.11,0.33,0.56) | 5/31/1999 | 2,1,2,2,1,2,2,2,2→2 | (0.22,0.78,0) | 8/9/1999 | 3,1,1,1,2,1,1,3,1→3 | (0.67,0.11,0.22) | 10/20/1999 | 1,1,2,1,3,2,3,3,1→1 | (0.44,0.22,0.33) |
| 3/23/1999 | 3,2,3,3,3,3,3,2,1→3 | (0.11,0.22,0.67) | 6/1/1999 | 1,2,1,2,2,1,2,2,2→1 | (0.33,0.67,0) | 8/10/1999 | 2,3,1,1,1,2,1,1,3→2 | (0.56,0.22,0.22) | 10/21/1999 | 2,1,1,2,1,3,2,3,3→2 | (0.33,0.33,0.33) |
| 3/24/1999 | 1,3,2,3,3,3,3,3,2→1 | (0.11,0.22,0.67) | 6/2/1999 | 3,1,2,1,2,2,1,2,2→3 | (0.33,0.56,0.11) | 8/11/1999 | 3,2,3,1,1,1,2,1,1→3 | (0.56,0.22,0.22) | 10/22/1999 | 2,2,1,1,2,1,3,2,3→2 | (0.33,0.44,0.22) |
| 3/25/1999 | 1,1,3,2,3,3,3,3,3→1 | (0.22,0.11,0.67) | 6/3/1999 | 3,3,1,2,1,2,2,1,2→3 | (0.33,0.44,0.22) | 8/12/1999 | 2,3,2,3,1,1,1,2,1→2 | (0.44,0.33,0.22) | 10/25/1999 | 1,2,2,1,1,2,1,3,2→1 | (0.44,0.44,0.11) |
| 3/26/1999 | 3,1,1,3,2,3,3,3,3→3 | (0.22,0.11,0.67) | 6/4/1999 | 3,3,3,1,2,1,2,2,1→3 | (0.33,0.33,0.33) | 8/13/1999 | 3,2,3,2,3,1,1,1,2→3 | (0.33,0.33,0.33) | 10/26/1999 | 3,1,2,2,1,1,2,1,3→3 | (0.44,0.33,0.22) |
| 3/29/1999 | 3,3,1,1,3,2,3,3,3→3 | (0.22,0.11,0.67) | 6/5/1999 | 2,3,3,3,1,2,1,2,2→2 | (0.22,0.44,0.33) | 8/16/1999 | 3,3,2,3,2,3,1,1,1→3 | (0.33,0.22,0.44) | 10/27/1999 | 2,3,1,2,2,1,1,2,1→2 | (0.44,0.44,0.11) |
| 3/30/1999 | 1,3,3,1,1,3,2,3,3→1 | (0.33,0.11,0.56) | 6/7/1999 | 3,2,3,3,3,1,2,1,2→3 | (0.22,0.33,0.44) | 8/17/1999 | 3,3,3,2,3,2,3,1,1→3 | (0.22,0.22,0.56) | 10/28/1999 | 2,2,3,1,2,2,1,1,2→2 | (0.33,0.56,0.11) |
| 3/31/1999 | 2,1,3,3,1,1,3,2,3→2 | (0.33,0.22,0.44) | 6/8/1999 | 3,3,2,3,3,3,1,2,1→3 | (0.22,0.22,0.56) | 8/18/1999 | 3,3,3,3,2,3,2,3,1→3 | (0.11,0.22,0.67) | 10/29/1999 | 2,2,2,3,1,2,2,1,1→2 | (0.33,0.56,0.11) |
| 4/1/1999 | 2,2,1,3,3,1,1,3,2→2 | (0.33,0.33,0.33) | 6/9/1999 | 3,3,3,2,3,3,3,1,2→3 | (0.11,0.22,0.67) | 8/19/1999 | 1,3,3,3,3,2,3,2,3→1 | (0.11,0.22,0.67) | 10/30/1999 | 2,2,2,2,3,1,2,2,1→2 | (0.22,0.67,0.11) |
| 4/2/1999 | 3,2,2,1,3,3,1,1,3→3 | (0.33,0.22,0.44) | 6/10/1999 | 3,3,3,3,2,3,3,3,1→3 | (0.11,0.11,0.78) | 8/20/1999 | 2,1,3,3,3,3,2,3,2→2 | (0.11,0.33,0.56) |
References
- Robinson, P.M. Time Series with Long Memory; Oxford University Press: New York, NY, USA, 2003. [Google Scholar]
- Stepnicka, M.; Cortez, P.; Donate, J.P.; Stepnickova, L. Forecasting seasonal time series with computational intelligence: On recent methods and the potential of their combinations. Expert Syst. Appl. 2013, 40, 1981–1992. [Google Scholar] [CrossRef] [Green Version]
- Conejo, A.J.; Plazas, M.A.; Espinola, R.; Molina, A.B. Day-ahead electricity price forecasting using the wavelet transform and ARIMA models. IEEE Trans. Power Syst. 2005, 20, 1035–1042. [Google Scholar] [CrossRef]
- Engle, R.F. Autoregressive conditional heteroscedasticity with estimates of the variance of United Kingdom inflation. Econometrica 1982, 50, 987–1007. [Google Scholar] [CrossRef]
- Bollerslev, T. Generalized autoregressive conditional heteroscedasticity. J. Econom. 1986, 31, 307–327. [Google Scholar] [CrossRef]
- Song, Q.; Chissom, B.S. Forecasting enrollments with fuzzy time series—Part I. Fuzzy Sets Syst. 1993, 54, 1–9. [Google Scholar] [CrossRef]
- Song, Q.; Chissom, B.S. Fuzzy time series and its models. Fuzzy Sets Syst. 1993, 54, 269–277. [Google Scholar] [CrossRef]
- Song, Q.; Chissom, B.S. Forecasting enrollments with fuzzy time series—Part II. Fuzzy Sets Syst. 1994, 62, 1–8. [Google Scholar] [CrossRef]
- Chen, S.M.; Chen, S.W. Fuzzy forecasting based on two-factors second-order fuzzy-trend logical relationship groups and the probabilities of trends of fuzzy logical relationships. IEEE Trans. Cybern. 2015, 45, 405–417. [Google Scholar] [PubMed]
- Chen, S.M.; Jian, W.S. Fuzzy forecasting based on two-factors second-order fuzzy-trend logical relationship groups, similarity measures and PSO techniques. Inf. Sci. 2017, 391–392, 65–79. [Google Scholar] [CrossRef]
- Rubio, A.; Bermudez, J.D.; Vercher, E. Improving stock index forecasts by using a new weighted fuzzy-trend time series method. Expert Syst. Appl. 2017, 76, 12–20. [Google Scholar] [CrossRef]
- Efendi, R.; Ismail, Z.; Deris, M.M. A new linguistic out-sample approach of fuzzy time series for daily forecasting of Malaysian electricity load demand. Appl. Soft Comput. 2015, 28, 422–430. [Google Scholar] [CrossRef]
- Sadaei, H.J.; Guimaraes, F.G.; Silva, C.J.; Lee, M.H.; Eslami, T. Short-term load forecasting method based on fuzzy time series, seasonality and long memory process. Int. J. Approx. Reason. 2017, 83, 196–217. [Google Scholar] [CrossRef]
- Askari, S.; Montazerin, N. A high-order multi-variate fuzzy time series forecasting algorithm based on fuzzy clustering. Expert Syst. Appl. 2015, 42, 2121–2135. [Google Scholar] [CrossRef]
- Lahrimi, S. Intraday stock prime forecasting based on variational mode decomposition. J. Comput. Sci. 2016, 12, 23–27. [Google Scholar]
- Lahrimi, S. A variational mode decomposition approach for analysis and forecasting of economic and financial time series. Expert Syst. Appl. 2016, 55, 268–276. [Google Scholar]
- Jia, J.Y.; Zhao, A.W.; Guan, S. Forecasting based on high-order fuzzy-fluctuation trends and particle swarm optimization machine learning. Symmetry 2017, 9, 124. [Google Scholar] [CrossRef]
- Huarng, K.H. Effective lengths of intervals to improve forecasting in fuzzy time series. Fuzzy Sets Syst. 2001, 123, 387–394. [Google Scholar] [CrossRef]
- Egrioglu, E.; Aladag, C.H.; Basaran, M.A.; Uslu, V.R.; Yolcu, U. A new approach based on the optimization of the length of intervals in fuzzy time series. J. Intell. Fuzzy Syst. 2011, 22, 15–19. [Google Scholar]
- Egrioglu, E.; Aladag, C.H.; Yolcu, U.; Uslu, V.R.; Basaran, M.A. Finding an optimal interval length in high order fuzzy time series. Expert Syst. Appl. 2010, 37, 5052–5055. [Google Scholar] [CrossRef]
- Wang, L.; Liu, X.; Pedrycz, W. Effective intervals determined by information granules to improve forecasting in fuzzy time series. Expert Syst. Appl. 2013, 40, 5673–5679. [Google Scholar] [CrossRef]
- Yolcu, U.; Egrioglu, E.; Uslu, V.R.; Basaran, M.A.; Aladag, C.H. A new approach for determining the length of intervals for fuzzy time series. Appl. Soft Comput. 2009, 9, 647–651. [Google Scholar] [CrossRef]
- Zhao, A.W.; Guan, S.; Guan, H.J. A computational fuzzy time series forecasting model based on GEM-based discretization and hierarchical fuzzy logical rules. J. Intell. Fuzzy Syst. 2016, 31, 2795–2806. [Google Scholar] [CrossRef]
- Huarng, K.; Yu, T.H.K. Ratio-based lengths of intervals to improve fuzyy time series forecasting. IEEE Trans. Syst. Man Cybern. Part B Cybern. 2006, 36, 328–340. [Google Scholar] [CrossRef]
- Yu, H.K. Weighted fuzzy time series models for TAIEX forecasting. Phys. A Stat. Mech. Appl. 2005, 349, 609–624. [Google Scholar] [CrossRef]
- Aladag, C.H.; Basaran, M.A.; Egrioglu, E.; Yolcu, U.; Uslu, V.R. Forecasting in high order fuzzy time series by using neural networks to define fuzzy relations. Expert Syst. Appl. 2009, 36, 4228–4231. [Google Scholar] [CrossRef]
- Cai, Q.; Zhang, D.; Zheng, W.; Leung, S.C.H. A new fuzzy time series forecasting model combined with ant colony optimization and auto-regression. Knowl. Based Syst. 2015, 74, 61–68. [Google Scholar] [CrossRef]
- Chen, S.; Chang, Y. Multi-variable fuzzy forecasting based on fuzzy clustering and fuzzy rule interpolation techniques. Inf. Sci. 2010, 180, 4772–4783. [Google Scholar] [CrossRef]
- Chen, S.; Chen, C. TAIEX forecasting based on fuzzy time series and fuzzy variation groups. IEEE Trans. Fuzzy Syst. 2011, 19, 1–12. [Google Scholar] [CrossRef]
- Chen, S.; Chu, H.; Sheu, T. TAIEX forecasting using fuzzy time series and automatically generated weights of multiple factors. IEEE Trans. Syst. Man Cybern. Part A Syst. Hum. 2012, 42, 1485–1495. [Google Scholar] [CrossRef]
- Ye, F.; Zhang, L.; Zhang, D.; Fujita, H.; Gong, Z. A novel forecasting method based on multi-order fuzzy time series and technical analysis. Inf. Sci. 2016, 367–368, 41–57. [Google Scholar] [CrossRef]
- Cheng, C.H.; Chen, T.L.; Teoh, H.J.; Chiang, C.H. Fuzzy time-series based on adaptive expectation model for TAIEX forecasting. Expert Syst. Appl. 2008, 34, 1126–1132. [Google Scholar] [CrossRef]
- Egrioglu, E.; Yolcu, U.; Aladag, C.H.; Kocak, C. An ARMA type fuzzy time series forecasting method based on particle swarm optimization. Math. Probl. Eng. 2013, 2013, 935815. [Google Scholar] [CrossRef]
- Kocak, C. A new high order fuzzy ARMA time series forecasting method by using neural networks to define fuzzy relations. Math. Probl. Eng. 2015, 2015, 128097. [Google Scholar] [CrossRef]
- Kocak, C. ARMA(p,q) type high order fuzzy time series forecast method based on fuzzy logic relations. Appl. Soft Comput. 2017, 59, 92–103. [Google Scholar] [CrossRef]
- Smarandache, F. A Unifying Field in Logics: Neutrosophic Logic. Neutrosophy, Neutrosophic Set, Neutrosophic Probability, 2nd ed.; American Research Press: Rehoboth, MA, USA, 1999. [Google Scholar]
- Fu, J.; Ye, J. Simplified neutrosophic exponential similarity measures for the initial evaluation/diagnosis of benign prostatic hyperplasia symptoms. Symmetry 2017, 9, 154. [Google Scholar] [CrossRef]
- Chen, J.; Ye, J. Vector similarity measures between refined simplified neutrosophic sets and their multiple attribute decision-making method. Symmetry 2017, 9, 153. [Google Scholar] [CrossRef]
- Majumdar, P.; Samanta, S.K. On similarity and entropy of neutrosophic sets. J. Intell. Fuzzy Syst. 2014, 26, 1245–1252. [Google Scholar]
- Peng, J.J.; Wang, J.; Zhang, H.Y.; Chen, X.H. An outranking approach for multi-criteria decision-making problems with simplified neutrosophic sets. Appl. Soft Comput. 2014, 25, 336–346. [Google Scholar] [CrossRef]
- Liu, P.D.; Wang, Y.M. Multiple attribute decision making method based on single-valued neutrosophic normalized weighted Bonferroni mean. Neural Comput. Appl. 2014, 25, 2001–2010. [Google Scholar] [CrossRef]
- Ye, J.; Fu, J. Multi-period medical diagnosis method using a single valued neutrosophic similarity measure based on tangent function. Comput. Methods Programs Biomed. 2015, 123, 142–149. [Google Scholar] [CrossRef] [PubMed]
- Herrera, F.; Herrera-Viedma, E.; Verdegay, J.L. A model of consensus in group decision making under linguistic assessments. Fuzzy Sets Syst. 1996, 79, 73–87. [Google Scholar] [CrossRef]
- Chen, M.Y.; Chen, B.T. A hybrid fuzzy time series model based on granular computing for stock price forecasting. Inf. Sci. 2015, 294, 227–241. [Google Scholar] [CrossRef]
- Chang, J.R.; Wei, L.Y.; Cheng, C.H. A hybrid ANFIS model based on AR and volatility for TAIEX Forecasting. Appl. Soft Comput. 2011, 11, 1388–1395. [Google Scholar] [CrossRef]
- Chen, S.M.; Manalu, G.M.T.; Pan, J.S.; Liu, H.C. Fuzzy forecasting based on two-factors second-order fuzzy-trend logical relationship groups and particle swarm optimization techniques. IEEE Trans. Cybern. 2013, 43, 1102–1117. [Google Scholar] [CrossRef] [PubMed]
- Cheng, C.H.; Wei, L.Y.; Liu, J.W.; Chen, T.L. OWA-based ANFIS model for TAIEX forecasting. Econ. Model. 2013, 30, 442–448. [Google Scholar] [CrossRef]
- Hsieh, T.J.; Hsiao, H.F.; Yeh, W.C. Forecasting stock markets using wavelet trans-forms and recurrent neural networks: An integrated system based on artificial bee colony algorithm. Appl. Soft Comput. 2011, 11, 2510–2525. [Google Scholar] [CrossRef]
- Diebold, F.X.; Mariano, R.S. Comparing predictive accuracy. J. Bus. Econ. Stat. 1995, 13, 134–144. [Google Scholar]
| NLRs | NLRs | NLRs |
|---|---|---|
| (0.33,0.33,0.33)→(0.4,0.3,0.3) | (0.22,0.33,0.44)→(0,0.6,0.4) | (0.22,0.78,0)→(0.5,0.5,0) |
| (0.44,0.33,0.22)→(0.23,0.46,0.31) | (0.22,0.44,0.33)→(0.33,0.33,0.33) | (0.33,0.67,0)→(0,0,1) |
| (0.44,0.44,0.11)→(0.4,0.33,0.27) | (0.11,0.56,0.33)→(0.17,0.5,0.33) | (0.11,0.11,0.78)→(0,1,0) |
| (0.33,0.44,0.22)→(0.54,0.23,0.23) | (0.11,0.67,0.22)→(0.17,0.33,0.5) | (0,0.22,0.78)→(0,1,0) |
| (0.33,0.56,0.11)→(0.25,0.5,0.25) | (0.22,0.56,0.22)→(0.25,0.5,0.25) | (0,0.33,0.67)→(0,1,0) |
| (0.56,0.33,0.11)→(0.36,0.27,0.36) | (0.11,0.44,0.44)→(0,0.38,0.63) | (0.56,0.22,0.22)→(0.25,0.25,0.5) |
| (0.67,0.22,0.11)→(0,1,0) | (0.11,0.33,0.56)→(0.33,0.17,0.5) | (0.44,0.11,0.44)→(0.5,0.5,0) |
| (0.56,0.44,0)→(0,0,1) | (0.11,0.22,0.67)→(0.43,0.43,0.14) | (0.56,0.11,0.33)→(1,0,0) |
| (0.44,0.22,0.33)→(0.29,0.43,0.29) | (0,0.56,0.44)→(0.33,0,0.67) | (0.67,0,0.33)→(0,1,0) |
| (0.33,0.22,0.44)→(0.31,0.38,0.31) | (0,0.44,0.56)→(0.14,0.43,0.43) | (0.67,0.11,0.22)→(0.5,0.25,0.25) |
| (0.22,0.22,0.56)→(0.25,0.25,0.5) | (0.11,0.78,0.11)→(0,0.8,0.2) | (0.22,0.67,0.11)→(0,0,1) |
| (0.33,0.11,0.56)→(0,0.5,0.5) | (0,0.89,0.11)→(0.25,0.75,0) | |
| (0.22,0.11,0.67)→(0.29,0.29,0.43) | (0.11,0.89,0)→(0.5,0.5,0) |
| Date (MM/DD/YYYY) | Actual | Forecast | (Forecast − Actual)2 | Date (MM/DD/YYYY) | Actual | Forecast | (Forecast − Actual)2 |
|---|---|---|---|---|---|---|---|
| 11/1/1999 | 7814.89 | 7882.90 | 4625.36 | 12/1/1999 | 7766.20 | 7720.87 | 2054.81 |
| 11/2/1999 | 7721.59 | 7842.94 | 14,725.82 | 12/2/1999 | 7806.26 | 7766.20 | 1604.80 |
| 11/3/1999 | 7580.09 | 7721.59 | 20,022.25 | 12/3/1999 | 7933.17 | 7797.76 | 18,335.87 |
| 11/4/1999 | 7469.23 | 7580.09 | 12,289.94 | 12/4/1999 | 7964.49 | 7924.67 | 1585.63 |
| 11/5/1999 | 7488.26 | 7469.23 | 362.14 | 12/6/1999 | 7894.46 | 7955.99 | 3785.94 |
| 11/6/1999 | 7376.56 | 7488.26 | 12,476.89 | 12/7/1999 | 7827.05 | 7885.96 | 3470.39 |
| 11/8/1999 | 7401.49 | 7365.51 | 1294.56 | 12/8/1999 | 7811.02 | 7827.05 | 256.96 |
| 11/9/1999 | 7362.69 | 7390.44 | 770.06 | 12/9/1999 | 7738.84 | 7802.52 | 4055.14 |
| 11/10/1999 | 7401.81 | 7351.64 | 2517.03 | 12/10/1999 | 7733.77 | 7745.64 | 140.90 |
| 11/11/1999 | 7532.22 | 7486.82 | 2061.16 | 12/13/1999 | 7883.61 | 7707.42 | 31,042.92 |
| 11/15/1999 | 7545.03 | 7521.17 | 569.30 | 12/14/1999 | 7850.14 | 7857.26 | 50.69 |
| 11/16/1999 | 7606.20 | 7545.03 | 3741.77 | 12/15/1999 | 7859.89 | 7823.79 | 1303.21 |
| 11/17/1999 | 7645.78 | 7606.20 | 1566.58 | 12/16/1999 | 7739.76 | 7859.89 | 14,431.22 |
| 11/18/1999 | 7718.06 | 7673.83 | 1956.29 | 12/17/1999 | 7723.22 | 7728.71 | 30.14 |
| 11/19/1999 | 7770.81 | 7731.66 | 1532.72 | 12/18/1999 | 7797.87 | 7723.22 | 5572.62 |
| 11/20/1999 | 7900.34 | 7799.71 | 10,126.40 | 12/20/1999 | 7782.94 | 7797.87 | 222.90 |
| 11/22/1999 | 8052.31 | 7924.99 | 16,210.38 | 12/21/1999 | 7934.26 | 7782.94 | 22,897.74 |
| 11/23/1999 | 8046.19 | 8052.31 | 37.45 | 12/22/1999 | 8002.76 | 7947.86 | 3014.01 |
| 11/24/1999 | 7921.85 | 8046.19 | 15,460.44 | 12/23/1999 | 8083.49 | 8056.32 | 738.21 |
| 11/25/1999 | 7904.53 | 7936.30 | 1009.33 | 12/24/1999 | 8219.45 | 8137.05 | 6789.76 |
| 11/26/1999 | 7595.44 | 7918.98 | 104,678.13 | 12/27/1999 | 8415.07 | 8233.90 | 32,822.57 |
| 11/29/1999 | 7823.90 | 7629.44 | 37,814.69 | 12/28/1999 | 8448.84 | 8390.42 | 3412.90 |
| 11/30/1999 | 7720.87 | 7845.15 | 15,445.52 | Root Mean Square Error(RMSE) | 98.76 | ||
| n | Average | |||||||||
|---|---|---|---|---|---|---|---|---|---|---|
| 2 | 3 | 4 | 5 | 6 | 7 | 8 | 9 | 10 | ||
| RMSE | 100.22 | 100.9 | 100.66 | 99.81 | 102.83 | 103.48 | 100.36 | 98.76 | 108.99 | 99.03 |
| Year | |||||||||
|---|---|---|---|---|---|---|---|---|---|
| 1997 | 1998 | 1999 | 2000 | 2001 | 2002 | 2003 | 2004 | 2005 | |
| RMSE | 141.89 | 119.85 | 99.03 | 128.62 | 125.64 | 66.29 | 53.2 | 56.11 | 55.83 |
| Methods | RMSE | S |
|---|---|---|
| Yu’s Method (2005) [25] | 145 | 1.82 ** |
| Hsieh et al.’s Method (2011) [48] | 94 | −0.42 |
| Chang et al.’s Method (2011) [45] | 100 | 0.21 |
| Cheng et al.’s Method (2013) [47] | 103 | 0.42 |
| Chen et al.’s Method (2013) [46] | 102.11 | 0.39 |
| Chen and Chen’s Method (2015) [9] | 103.9 | 0.29 |
| Chen and Chen’s Method (2015) [44] | 92 | −0.51 |
| Zhao et al.’s Method (2016) [23] | 110.85 | 1.16 |
| Jia et al.’s Method (2017) [17] | 99.31 | 0.11 |
| The Proposed Method | 99.03 | - |
| Year | |||||||||
|---|---|---|---|---|---|---|---|---|---|
| 2007 | 2008 | 2009 | 2010 | 2011 | 2012 | 2013 | 2014 | 2015 | |
| RMSE | 113.47 | 71.6 | 49.14 | 45.35 | 27.74 | 25.83 | 19.95 | 41.42 | 64.6 |
© 2017 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Guan, H.; Guan, S.; Zhao, A. Forecasting Model Based on Neutrosophic Logical Relationship and Jaccard Similarity. Symmetry 2017, 9, 191. https://doi.org/10.3390/sym9090191
Guan H, Guan S, Zhao A. Forecasting Model Based on Neutrosophic Logical Relationship and Jaccard Similarity. Symmetry. 2017; 9(9):191. https://doi.org/10.3390/sym9090191
Chicago/Turabian StyleGuan, Hongjun, Shuang Guan, and Aiwu Zhao. 2017. "Forecasting Model Based on Neutrosophic Logical Relationship and Jaccard Similarity" Symmetry 9, no. 9: 191. https://doi.org/10.3390/sym9090191
APA StyleGuan, H., Guan, S., & Zhao, A. (2017). Forecasting Model Based on Neutrosophic Logical Relationship and Jaccard Similarity. Symmetry, 9(9), 191. https://doi.org/10.3390/sym9090191





