1. Introduction
The so-called isoperimetric problem in mathematics is concerned with the determination of the shape of spatial (or planar) objects which have the largest possible volume (or area) enclosed with given surface area (or circumference). The isoperimetric problem for polyhedra can be reflected in a question as follows: What polyhedron maximizes the volume if the surface area and the number of faces
n are given? The problem can be quantified by the so-called Steinitz number [
1]
, a dimensionless quantity in terms of the surface area
A and volume
V of the polyhedron, such that solutions of the isoperimetric problem minimize
S. Lindelöf proved that in order to reach the maximum volume, the polyhedron must have an insphere such that all faces are tangent to this sphere at their centroid [
2,
3]. If the radius of such an inscribed sphere is taken to be unity, one can easily find that
. This way, the problem is reformulated so as to find polyhedra with
n faces circumscribed about the unit sphere which have the minimum surface area (or volume).
An alternative measure of roundness of solids applied in various fields of science is the so-called isoperimetric quotient (
) introduced by Pólya [
4], which is a normed inverse of the Steinitz number:
The isoperimetric quotient is a positive dimensionless number that takes unity for the sphere and less for other solids. The closer the
is to unity, the more spherical the polyhedron is. Goldberg proposed a lower bound [
5] for the Steinitz number, from which a lower bound
for the surface area can be easily obtained if Lindelöf’s necessary condition is satisfied:
where,
Furthermore, on the one hand, as the isoperimetric quotient is inversely proportional to the Steinitz number, for a given n an upper bound for the maximum value of can be simply derived as . On the other hand, any polyhedron constructed with n faces constitutes a lower bound for the maximum value of since it has a surface area larger than or equal to that of the optimal polyhedron. A polyhedron here is referred to as optimal if it has the largest possible among all possible polyhedra of any topology for the given n. Any candidate polyhedron construction is called hereafter conjectural unless rigorous mathematical proof is provided for its optimality.
For this isoperimetric problem where no symmetry constraint is enforced, mathematical proof of optimality exists only in certain cases of a small number of faces. Fejes Tóth [
6] proved that Goldberg’s formula provides a lower bound of the minimum value of the Steinitz number for any
n, and it is exact for
. In this way he proved that the regular tetrahedron, hexahedron, and dodecahedron are the optimal polyhedra in the cases of 4, 6, and 12 faces, respectively. The case of 5 faces is also proven [
2,
3].
Conjectured solutions with numerically optimized geometry were shown by Goldberg [
5] for
to
to
, preceding Fejes Tóth’s evidence, and later by Schoen [
7,
8] for cases up to
. Mutoh [
9] also dealt with polyhedra with minimum volume circumscribed about the unit sphere, and by using a computer-aided search, provided a series of conjectured optimal polyhedra with the number of faces ranging between 4 and 30. In an earlier paper [
10] the authors pointed out that in some cases the isoperimetric problem for
n faces and the problem of the minimum covering of a sphere by
n equal circles have the same proven or conjectured solution (the points of tangency of the faces and the centres of the circles are identical). In some cases, the obtained polyhedra are only topologically identical. Sometimes, the topology of the optimal polyhedron matches that of a suboptimal (nearly optimal) circle covering, and not the optimal one. We developed an iterative numerical method for determining a locally optimal solution of the isoperimetric problem, where the starting point for the topology of the polyhedron is a conjectured optimal circle covering on a sphere. Using this method and starting with the conjectured best coverings with 50 [
11] and 72 [
12] equal circles, conjectural solutions to the isoperimetric problem for
and 72 were obtained [
10].
Some of the above-mentioned proven or conjectured solutions have tetrahedral, octahedral or icosahedral symmetry even though such symmetry constraints were not enforced. The solutions for
, 6, and 12 investigated by Fejes Tóth [
6] have tetrahedral, octahedral, and icosahedral symmetry, respectively. The conjectured solution suggested by Goldberg [
5] for
has tetrahedral symmetry, and for
and 42 has icosahedral symmetry. The conjectured solution for
provided in [
10] has icosahedral symmetry.
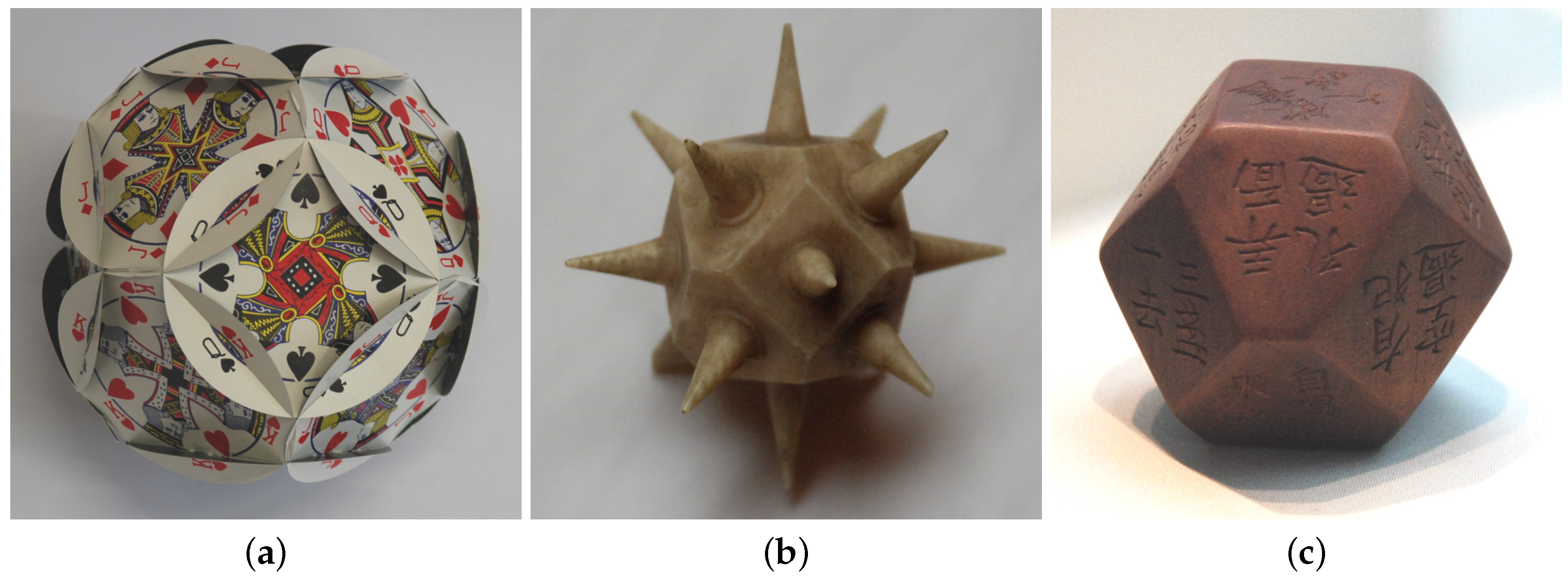
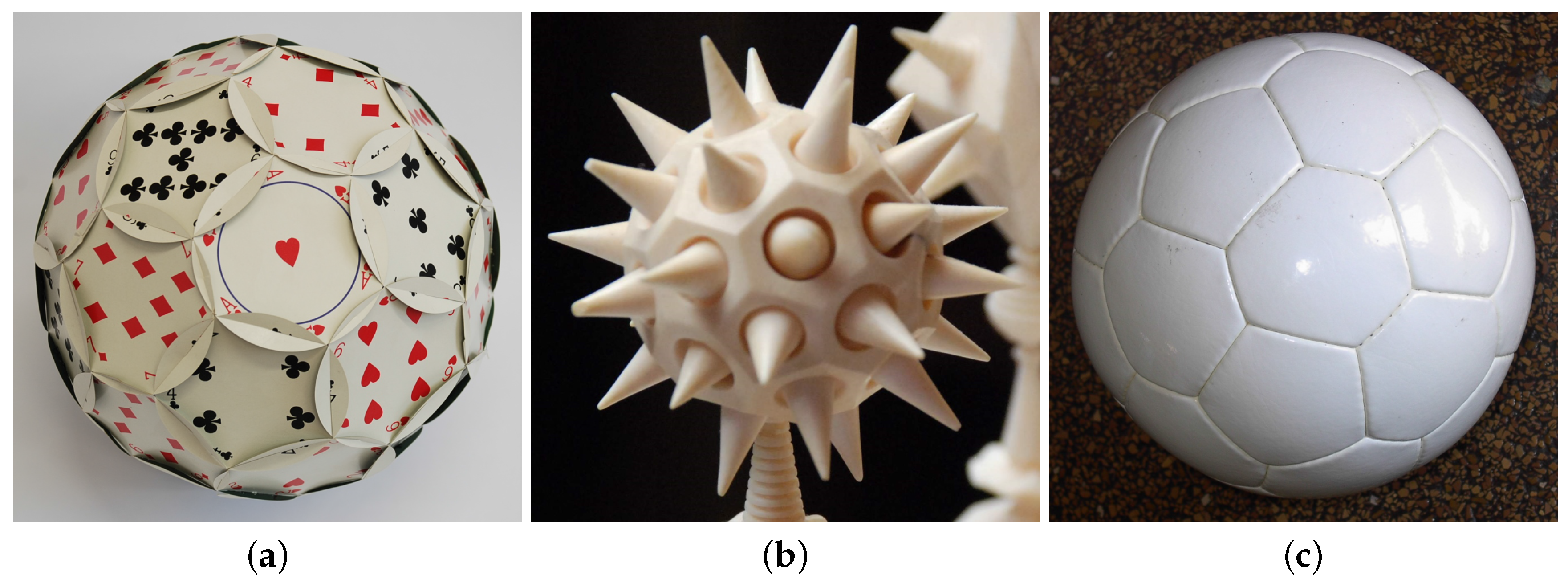
It turns out that in some artistic or practical applications it is not the solutions to the unconstrained isoperimetric problem which play an important role, but rather those with tetrahedral, octahedral or icosahedral symmetry constraints. For instance, a turned ivory piece from Germany, and a wooden die from Korea are exact representations of the conjectured roundest polyhedron with octahedral symmetry constraints for
(
Figure 1). Similarly, another turned ivory object in Germany and a modern soccer ball show the conjectured roundest polyhedron with icosahedral symmetry constraint for
(
Figure 2). More details on these artifacts can be found in [
13]. Multi-symmetric roundest polyhedra for
can be particularly important in practical applications, e.g., soccer ball design [
14,
15]. The conjectured solution for the case of 14 (32) faces is an
isodistant truncation of the octahedron (icosahedron) where the centroids of the faces lie at the vertices and the face centres of a spherical octahedron (icosahedron). A truncation of a Platonic polyhedron is said to be
isodistant if the distances from the centre of the Platonic polyhedron to the truncating planes and to the faces of the Platonic polyhedron are equal, that is, if the truncated polyhedron has an insphere. Polyhedra with 26 and 62 faces are obtained by double isodistant truncations of the octahedron and the icosahedron, respectively [
14,
15].
The artistic and practical interest in finding the roundest object as shown above is an important motivation to study the isoperimetric problem for polyhedra with symmetry constraints. The primary aim of this paper is to prove the solutions for small values of
n (
) and to present conjectured solutions for
. Recalling that some solutions for small even numbers of polygonal faces have been proven by Fejes Tóth [
6], our new results complete the sequence of proven and conjectured solutions for even
n up to 22. Goldberg [
16] has shown how to construct multi-symmetric polyhedra by using a triangular lattice on the tetrahedron, octahedron, and icosahedron (the details will be discussed later in the paper). After his name, these polyhedra now are called Goldberg polyhedra. The secondary aim of this paper is to present octahedrally symmetric Goldberg polyhedra for
and 38 as conjectural solutions, and icosahedrally symmetric Goldberg polyhedra for
. In this way it is possible to extend the list of icosahedral Goldberg polyhedra up to
.
2. Multi-Symmetric Point Arrangements on the Sphere
If a plane is tangent to a sphere, then the position of the plane is uniquely determined by the position of the point of tangency. In this way we can investigate the faces of polyhedra having an insphere via investigating the points of tangency on spherical tetrahedra, octahedra, and icosahedra. Identifying and exploring the degrees of freedom of tangent placement on these spherical polyhedra allows the generation of polyhedra with varying face numbers and set symmetries, among which our numerical method can identify the locally optimal (roundest) solution.
Consider the Platonic polyhedra
with triangular faces and
q-valent vertices where
(that is the regular tetrahedron, octahedron, icosahedron). Let
V,
E, and
F denote their number of vertices, edges, and faces, respectively. According to the relationships derived in §10.3 of Coxeter’s book [
17]:
The symmetry of these polyhedra is characterized by the fact that
q-fold, 2-fold and 3-fold axes of rotation go through their vertices, edge midpoints and face centres, respectively. If we take one face of the spherical version of these polyhedra, then we can put one point of tangency or none to a vertex, any number of points of tangency on an edge, and a number of points of tangency on a face, divisible by three (points arranged in threefold rotational symmetry) or the remainder after division by three is one (points arranged in threefold rotational symmetry and a point in the face centre). Let
v,
e, and
f denote the number of points of tangency at a vertex, on an edge, and on a face, respectively. Then these non-negative integers can be expressed as follows:
such that
. In this way the number of all points of tangency on the sphere is:
Since V, E, and F are even, it is obvious that n is even, too. Additionally, .
Proposition 1. Any even number of points, not less than 4, can be arranged on the sphere in tetrahedral symmetry.
Proof. For tetrahedral symmetry, Equation (
6) yields
. We prove the proposition if we see that
is an arbitrary natural number
. First select
,
,
In this case
,
Then select
,
,
In this case
,
Finally, select
,
,
In this case
,
The union of these three sets of numbers contains all natural numbers
, consequently, the set of numbers
n contains all even numbers
. ☐
Remark 1. From the proposition it follows that for any even number n ≥ 4 there exists a polyhedron with n faces and tetrahedral symmetry. In the proof, for f we used only two values out of the infinitely many. Therefore, for many values of n, there exist not only one but more polyhedra with different topology in tetrahedral symmetry.
In the case of octahedral symmetry, the number of points of tangency takes the form . It can be verified quickly that there exist polyhedra with an even number of faces and octahedral symmetry if except if ,
In the case of icosahedral symmetry, the number of points of tangency takes the form . Here, it can be verified quickly that there exist polyhedra with n faces and icosahedral symmetry only for the following values of n: , , , , where
The vertices, edge midpoints, and face centres are the points of a face triangle of the tetrahedron, octahedron, and icosahedron, through which rotation axes pass. If a point of tangency is put to such a point, then the position of the point of tangency is fixed. It cannot be slightly moved without destroying the symmetry. We say that the degree of freedom of the point of tangency is zero in this position. If we put one point of tangency on an edge and another one symmetrically to the edge midpoint, then the point and its symmetrical counterpart can be moved along the edge without breaking the symmetry. We say that the degree of freedom of the point of tangency is one. If we put a point of tangency inside the triangle and two others according to threefold symmetry with respect to the face centre, then we can move the point and its symmetrical counterparts simultaneously in the triangle so that the symmetry is maintained. We say that the degree of freedom of the point of tangency is two.
Consider the simplest cases where
v,
e, and
f in Equation (
5) take the values 1 or 0. Since here the points of tangency have zero degree of freedom, they uniquely determine the respective polyhedra. Since the number of 3-tuples of a 2-set is
, we have eight possibilities, but the case
, as meaningless, is left out. Thus in each of the tetrahedral, octahedral, icosahedral symmetries, we have seven polyhedra. The face numbers
n of these polyhedra (the numbers of points of tangency) are collected in
Table 1.
Polyhedra determined by the data of
Table 1 have also planes of symmetry. Thus, their symmetry groups are the full tetrahedral, octahedral, and icosahedral groups, respectively. Among these 21 polyhedra some are identical. It is trivial to see, for instance, that in the case
, each of the two polyhedra is the same regular tetrahedron. This kind of coincidence in some other cases will be shown later.
For the tetrahedral arrangements of points of tangency surely will also contain points with one and/or two degrees of freedom. That means that the configuration with the highest can be found only by an optimization process.
For larger values of
n, it is expected that trivalent polyhedra with mostly hexagonal faces provide the best results, especially in icosahedral symmetry. Trivalent vertices are found to be visually less “pointed”, consider for example the classic 32-panel soccer ball. Such polyhedra are the Goldberg polyhedra [
16] explained below.
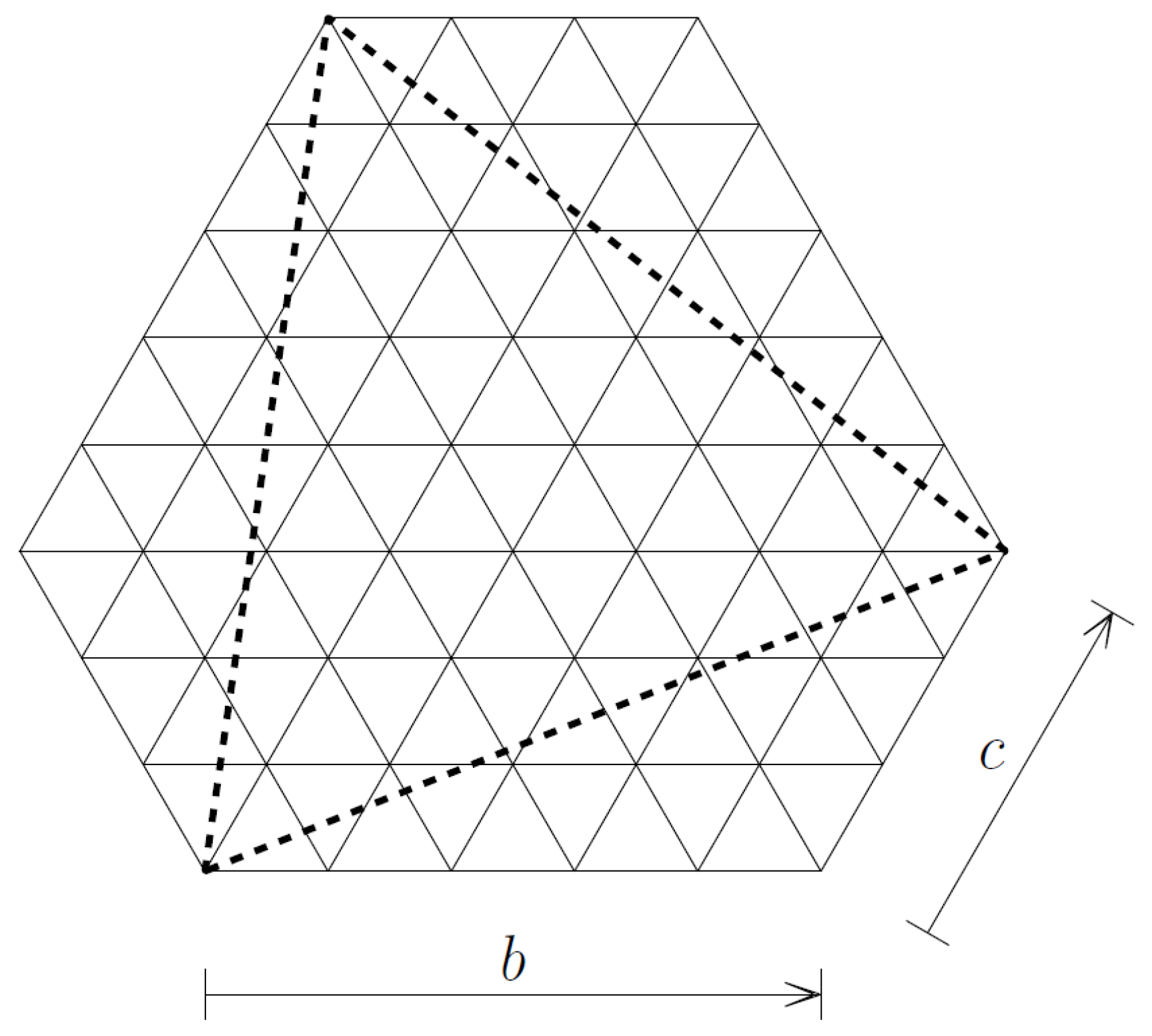
In virus research, Caspar and Klug [
18] discovered a regular tessellation on the regular triangle-faced polyhedra
,
, which consist of small equal equilateral triangles. Coxeter [
19] denoted this tessellation by
, where
indicates that
q and more than
q (i.e., six) small triangles meet at each vertex, some of which are folded, such that they tessellate the surface of the regular polyhedron
. The subscripts
b and
c show that a vertex of the regular polyhedron
can be arrived at from an adjacent one along the edges of the tessellation by
b steps on the vertices in one direction, then proceeding by
c steps after a change in direction by 60°.
Figure 3 shows a part of this tessellation.
The pair of non-negative integers
b,
c, called Goldberg–Coxeter parameters, generate the tessellation and determine the so-called triangulation number
T.
By “blowing up” the tessellation, we obtain a triangular lattice on the sphere. This lattice determines a polyhedron whose all vertices lie on the sphere and all faces are triangles. Its numbers of vertices
, edges
, and faces
can be expressed with
T, by using the expressions for
V,
E, and
F, respectively in (
4), in the form:
The reciprocal (dual) of this triangle-faced polyhedron is called Goldberg polyhedron, which is a trivalent polyhedron which has
q-gonal faces, and
hexagonal faces. For
, the Goldberg polyhedra are good candidates for the roundest polyhedra with
n faces, especially with octahedral and icosahedral symmetry where,
and,
respectively.
When the above-mentioned spherical triangular lattice is applied in the case of small values of
n, sometimes not all vertices are considered as points of tangency. Sometimes extra points of tangency, which are not vertices of the lattice, are added to the point system. In
Figure 4, in a schematic view, we present a part of the triangular lattice—lying on a face of the spherical tetrahedron, octahedron or icosahedron—together with the points of tangency, for the polyhedra shown in
Figure 5. The degree of freedom of the points of tangency is also indicated. The subfigures of
Figure 4 are in correspondence with those of
Figure 5.
5. Discussion
A numerical iterative method developed recently by the authors has now been applied to produce conjectured solutions for the isoperimetric problem of polyhedra if tetrahedral, octahedral, or icosahedral symmetry constraints are prescribed. The algorithm ensures that the polyhedra are local optima, and they are either identical or close to global optima if initial face arrangements are chosen appropriately. In some of the cases such initial arrangements for the conjectured solutions proposed by the authors originated from optimal circle coverings on a sphere. The small differences between the actual values and the corresponding upper bounds (e.g., for ) render the polyhedra likely to be global optima.
Using our numerical investigations, we have found (proven or conjectured) the roundest Goldberg polyhedra with icosahedral symmetry for the Goldberg–Coxeter parameters
,
,
,
,
,
,
; that is for face numbers
. This list is complete up to
. We also have octahedrally symmetric Goldberg polyhedra for the Goldberg–Coxeter parameters
,
,
,
and
, that is, for face numbers
and 38, as well as tetrahedrally symmetric Goldberg polyhedra for the Goldberg–Coxeter parameters
,
,
and
, that is for face numbers
and 20. Here the tetrahedral case
is special because the two trivalent vertices on each of the edges of the underlying tetrahedron coincide forming four-valent vertices, and the hexagons become triangles. In this way, the truncated tetrahedron becomes a regular octahedron. Recently, intense research was conducted into constructing “equilateral” Goldberg polyhedra [
21]. This raised the question as to whether these polyhedra could be used to extend the range of the conjectured roundest Goldberg polyhedra. Unfortunately, the answer is in the negative. The “equilateral” Goldberg polyhedra are only “nearly” spherical, and so they have no insphere.
We note that Schoen [
22] mentioned that recently Deeter numerically determined the solution for
without enforcing any symmetry. The optimal polyhedron he obtained has icosahedral symmetry
. Unfortunately, the numerical data of this polyhedron are not provided, so we could not decide whether his polyhedron is identical to ours.
Four out of the five Platonic polyhedra are the roundest multi-symmetric polyhedra for
. A natural question is whether among the semi-regular polyhedra there are some which are the roundest for
. The semi-regular polyhedra have tetrahedral, octahedral or icosahedral symmetry, look quite spherical, and additionally their
values are known [
23]. The Archimedean polyhedra are out of question because they have no insphere. However, it is possible to modify them according to isodistant truncation, as happened, for instance, to the truncated octahedron and truncated icosahedron (
and 32). The Archimedean duals, that is, the Catalan polyhedra are worth investigating because they do have an insphere. Among the Archimedean duals there are two polyhedra for
, four polyhedra for
, one polyhedron for
, one polyhedron for
, four polyhedra for
, and one polyhedron for
. Polyhedra for
are not interesting because the roundest is the regular dodecahedron. Among the four polyhedra for
, the dual of the snub cube is the best, for which
However, the points of tangency have two degrees of freedom here, therefore by optimization this
value can be increased. In the case of
, the rhombic triacontahedron has
, which is smaller than that we found for the polyhedron in
Figure 5l. In the case of
, the dual of the great rhombicuboctahedron has
, which is smaller than that for
given by Goldberg, therefore it is probably not optimal. Among the four polyhedra for
, the dual of the snub dodecahedron is the best, for which
However, because of an argument similar to that in the case of
, the polyhedron is not optimal. In the case of
, the dual of the great rhombicosidodecahedron has
, which is much smaller than that we have for
, therefore, probably it cannot be optimal.
Figure 1 and
Figure 2 show some applications for
and 32. After having some new results we discovered that the conjectured roundest polyhedron obtained for
(
Figure 5h) decorates the memorial to Thomas Bodley in the chapel of Merton College, Oxford, which was erected in 1615 [
24,
25].
 denote points of tangency with zero, one, and two degrees of freedom, respectively.
denote points of tangency with zero, one, and two degrees of freedom, respectively.
 denote points of tangency with zero, one, and two degrees of freedom, respectively.
denote points of tangency with zero, one, and two degrees of freedom, respectively.





