Status of X(1835) and
Abstract
:1. Introduction
2. and Interaction
2.1. Historical Development: Working with Effective Range Expansion
2.2. Updates: More Refined Treatment of Interaction and FSI
2.3. Potential Experimental Searches
3. Mesonic Decay of
4. Conclusions and Outlook
Acknowledgments
Author Contributions
Conflicts of Interest
References
- Bai, J.Z.; Ban, Y.; Bian, J.G.; Cai, X.; Chang, J.F.; Chen, H.F.; Chen, H.S.; Chen, J.; Chen, J.; Chen, J.C.; et al. Observation of a near threshold enhancement in the mass spectrum from radiative J/ψ→γ decays. Phys. Rev. Lett. 2003, 91. [Google Scholar] [CrossRef] [PubMed]
- Olive, K.A.; Agashe, K.; Amsler, C.; Antonelli, M.; Arguin, J.-F.; Asner, D. M.; Baer, H.; Band, H.R.; Barnett, R.M.; Basaglia, T.; et al. Review of Particle Physics. Particle Data Group Collaboration. Chin. Phys. C 2014, 38. [Google Scholar] [CrossRef]
- Fermi, E.; Yang, C.N. Are mesons elementary particles? Phys. Rev. 1949, 76. [Google Scholar] [CrossRef]
- Li, B.A. A possible 0-+ glueball candidate X(1835). Phys. Rev. D 2006, 74. [Google Scholar] [CrossRef]
- Kochelev, N.; Min, D.P. X(1835) as the lowest mass pseudoscalar glueball and proton spin problem. Phys. Lett. B 2006, 633, 283–288. [Google Scholar] [CrossRef]
- Kochelev, N.; Min, D.P. Eta(c): Glueball mixing and resonance X(1835). Phys. Rev. D 2005, 72. [Google Scholar] [CrossRef]
- He, X.G.; Li, X.Q.; Liu, X.; Ma, J.P. Some properties of the newly observed X(1835) state at BES. Eur. Phys. J. C 2007, 49, 731–736. [Google Scholar] [CrossRef]
- Huang, T.; Zhu, S.L. X(1835): A Natural candidate of eta-prime’s second radial excitation. Phys. Rev. D 2006, 73. [Google Scholar] [CrossRef]
- Albaladejo, M.; Oller, J.A.; Roca, L. Dynamical generation of pseudoscalar resonances. Phys. Rev. D 2010, 82. [Google Scholar] [CrossRef]
- Kang, X.W.; Kubis, B.; Hanhart, C.; Meißner, U.G. Bl4 decays and the extraction of |Vub|. Phys. Rev. D 2014, 89. [Google Scholar] [CrossRef]
- Kang, X.W.; Kubis, B.; Hanhart, C.; Meißner, U.G. Bl4 decays and the extraction of |Vub|. In Proceedings of the 8th International Workshop on Chiral Dynamics, Pisa, Italy, 29 June–3 July 2015.
- Kang, X.W. Chiral Dynamics and Final State Interactions in Semileptonic B Meson Decay and Antinucleon-Nucleon Scattering, Ph.D. Thesis, Bonn University, Bonn, Germany, 2014. [Google Scholar]
- Kang, X.-W.; Haidenbauer, J.; Meißner, U.-G. Near-threshold invariant mass spectrum measured in J/ψ and ψ′ decays. Phys. Rev. D 2015, 91. [Google Scholar] [CrossRef]
- Haidenbauer, J.; Kang, X.-W.; Meißner, U.-G. The electromagnetic form factors of the proton in the timelike region. Nucl. Phys. A 2014, 929, 102–104. [Google Scholar] [CrossRef]
- Kang, X.W. Antiproton-proton interaction and related hadron physics. In Proceedings of the 8th International Workshop on Chiral Dynamics, Pisa, Italy, 29 June–3 July 2015.
- Röder, M.; Borodina, E.; Clement, H.; Doroshkevich, E.; Dzhygadlo, R.; Ehrhardt, K.; Erhardt, A.; Eyrich, W.; Gast, W.; Gillitzer, A.; et al. Final-State Interactions in the Process →pK+Λ. Eur. Phys. J. A 2013, 49. [Google Scholar] [CrossRef]
- Datta, A.; O’Donnell, P.J. A New state of baryonium. Phys. Lett. B 2003, 567, 273–276. [Google Scholar] [CrossRef]
- De Rujula, A.; Georgi, H.; Glashow, S.L. Hadron masses in a gauge theory. Phys. Rev. D 1975, 12. [Google Scholar] [CrossRef]
- Zou, B.S.; Chiang, H.C. One pion exchange final state interaction and the near threshold enhancement in J/ψ→γ decays. Phys. Rev. D 2004, 69. [Google Scholar] [CrossRef]
- Zeng, Z.Q.; Guo, X.H.; Shen, P.N.; Ding, Y.B. final state interaction in J/ψ→γ. Chin. Phys. C 2012, 36. [Google Scholar] [CrossRef]
- Taylor, J.R. Scattering Theory: The Quantum Theory on Nonrelativistic Collisions; Johan Wiley and Sons, Inc.: New York, NY, USA, 1972; Chapter 11 and 12. [Google Scholar]
- Goldberger, M.L.; Watson, K.M. Collision Theory; Wiley: New York, NY, USA, 1964; Chapater 9.3. [Google Scholar]
- Ablikim, M.; Bai, J.Z.; Ban, Y.; Cai, X.; Chen, H.F.; Chen, H.S.; Chen, H.X.; Chen, J.C.; Chen, J.; Chen, Y.B.; et al. Study of J/ψ decaying into ω. Eur. Phys. J. C 2008, 53, 15–20. [Google Scholar] [CrossRef]
- Ablikim, M.; Achasov, M.N.; Albayrak, O.; Ambrose, D.J.; An, F.F.; Bai, J.Z.; Ban, Y.; Becker, J.; Bian, J.M.; Boger, E.; et al. Study of J/ψ→ω at BESIII. Phys. Rev. D 2013, 87. [Google Scholar] [CrossRef]
- Ericson, T.E.O.; Weise, W. Pions and Nuclei; Clarendon Press: Oxford, UK, 1988; pp. 44–46. [Google Scholar]
- Kang, X.-W.; Haidenbauer, J.; Meißner, U.-G. Antinucleon-nucleon interaction in chiral effective field theory. JHEP 2014, 1402. [Google Scholar] [CrossRef]
- Klempt, E.; Batty, C.; Richard, J.M. The Antinucleon-nucleon interaction at low energy : Annihilation dynamics. Phys. Rept. 2005, 413, 197–317. [Google Scholar] [CrossRef]
- Loiseau, B.; Wycech, S. Antiproton-proton channels in J/ψ decays. Phys. Rev. C 2005, 72. [Google Scholar] [CrossRef]
- Dedonder, J.-P.; Loiseau, B.; El-Bennich, B.; Wycech, S. On the structure of the X(1835) baryonium. Phys. Rev. C 2009, 80. [Google Scholar] [CrossRef]
- Sibirtsev, A.; Haidenbauer, J.; Krewald, S.; Meißner, U.G.; Thomas, A.W. Near threshold enhancement of the mass spectrum in J/ψ decay. Phys. Rev. D 2005, 71. [Google Scholar] [CrossRef]
- Entem, D.R.; Fernandez, F. Final state interaction effects in near threshold enhancement of the mass spectrum in B and J/ψ decays. Phys. Rev. D 2007, 75. [Google Scholar] [CrossRef]
- Watson, K.M. The Effect of final state interactions on reaction cross-sections. Phys. Rev. 1952, 88, 1163–1171. [Google Scholar] [CrossRef]
- Migdal, A.B. The Theory of Nuclear Reactions with Production of Slow Particles. JETP 1955, 1, 2–6. [Google Scholar]
- Kerbikov, B.; Stavinsky, A.; Fedotov, V. Model independent view on the low mass proton anti-proton enhancement. Phys. Rev. C 2004, 69. [Google Scholar] [CrossRef]
- Bugg, D.V. Reinterpreting several narrow "resonances" as threshold cusps. Phys. Lett. B 2004, 598, 8–14. [Google Scholar] [CrossRef] [Green Version]
- Gotta, D. Precision spectroscopy of light exotic atoms. Prog. Part. Nucl. Phys. 2004, 52, 133–195. [Google Scholar] [CrossRef]
- Hippchen, T.; Holinde, K.; Plessas, W. The bonn meson exchange potential in the system. Phys. Rev. C 1989, 39, 761–765. [Google Scholar] [CrossRef]
- Hippchen, T.; Haidenbauer, J.; Holinde, K.; Mull, V. Meson - baryon dynamics in the nucleon - anti-nucleon system. 1. The Nucleon - anti-nucleon interaction. Phys. Rev. C 1991, 44, 1323–1336. [Google Scholar] [CrossRef]
- Alexander, J.P.; Cassel, D.G.; Das, S.; Ehrlich, R.; Fields, L.; Gibbons, L.; Gray, S.W.; Hartill, D.L.; Heltsley, B.K.; Kreinick, D.L.; et al. Study of ψ(2S) Decays to γ, π0 and η and search for threshold enhancements. Phys. Rev. D 2010, 82. [Google Scholar] [CrossRef]
- Ablikim, M.; Achasov, M.N.; Alberto, D.; Ambrose, D.J.; An, F.F.; An, Q.; An, Z.H.; Bai, J.Z.; Baldini Ferroli, R.B.F.; Ban, Y.; et al. Spin-parity analysis of mass threshold structure in J/ψ and ψ′ radiative decays. Phys. Rev. Lett. 2012, 108. [Google Scholar] [CrossRef] [PubMed]
- Chen, G.Y.; Ma, J.P. Scattering at NLO order in an effective theory. Phys. Rev. D 2011, 83. [Google Scholar] [CrossRef]
- Chen, G.Y.; Dong, H.R.; Ma, J.P. Rescattering effect and near threshold enhancement of system. Phys. Rev. D 2008, 78. [Google Scholar] [CrossRef]
- Chen, G.Y.; Dong, H.R.; Ma, J.P. Near threshold enhancement of system and elastic scattering. Phys. Lett. B 2010, 692, 136–142. [Google Scholar] [CrossRef]
- Zhou, D.; Timmermans, R.G.E. Energy-dependent partial-wave analysis of all antiproton-proton scattering data below 925 MeV/c. Phys. Rev. C 2012, 86. [Google Scholar] [CrossRef]
- Grach, I.L.; Kerbikov, B.O.; Simonov, Y.A. Effective range analysis of low-energy nucleon - anti-nucleon Interaction. Phys. Lett. B 1988, 208. [Google Scholar] [CrossRef]
- Pirner, H.J.; Kerbikov, B.; Mahalanabis, J. Updating the effective range expansion of low-energy scattering. Z. Phys. A 1991, 338, 111–112. [Google Scholar] [CrossRef]
- Carbonell, J.; Richard, J.M.; Wycech, S. On the relation between protonium level shifts and nucleon - anti-nucleon scattering amplitudes. Z. Phys. A 1992, 343, 325–329. [Google Scholar] [CrossRef]
- Mull, V.; Holinde, K. Combined description of scattering and annihilation with a hadronic model. Phys. Rev. C 1995, 51, 2360–2371. [Google Scholar] [CrossRef]
- El-Bennich, B.; Lacombe, M.; Loiseau, B.; Wycech, S. Paris potential constrained by recent antiprotonic-atom data and antineutron-proton total cross sections. Phys. Rev. C 2009, 79. [Google Scholar] [CrossRef]
- Ziegler, M.; Ahmad, S.; Amsler, C.; Armenteros, R.; Auld, E.G.; Axen, D.A.; Bailey, D.; Barlag, S.; Beer, G.A.; Bizot, J.C.; et al. Measurement of the Strong Interaction Shift and Broadening of the Ground State of the Atom. Phys. Lett. B 1988, 206. [Google Scholar] [CrossRef]
- Augsburger, M.; Anagnostopoulos, D.F.; Borchert, G.; Chatellard, D.; Egger, J.-P.; El-Khoury, P.; Gorke, H.; Gotta, D.; Hauser, P.; Indelicato, P.; et al. Measurement of the strong interaction parameters in anti-protonic hydrogen and probable evidence for an interference with inner bremsstrahlung. Nucl. Phys. A 1999, 658, 149–162. [Google Scholar] [CrossRef]
- Haidenbauer, J.; Hanhart, C.; Kang, X.W.; Meißner, U.G. Origin of the structures observed in e+e- annihilation into multipion states around the threshold. Phys. Rev. D 2015, 92. [Google Scholar] [CrossRef]
- Kang, X.-W. Bound state nature of X(1835). Unpublished work. 2016. [Google Scholar]
- Haidenbauer, J.; Meißner, U.G.; Sibirtsev, A. Near threshold enhancement in B and J/ψ decay. Phys. Rev. D 2006, 74. [Google Scholar] [CrossRef]
- Mecking, B.A.; Adams, G.; Ahmad, S.; Anciant, E.; Anghinolfi, M.; Asavapibhop, B.; Asryan, G.; Audit, G.; Auger, T.; Avakian, H.; et al. The CEBAF large acceptance spectrometer (CLAS). Nucl. Instrum. Meth. A 2003, 503, 513–553. [Google Scholar] [CrossRef]
- Haidenbauer, J. Near threshold enhancement in J/ψ decays. Available online: http://www.kitpc.ac.cn/files/activities/PF20120611/report/Johann%20Haidenbauer.pdf (accessed on 12 January 2016).
- Lutz, M.F.M.; Pire, B.; Scholten, O.; Timmermans, R.; Erni, W.; Keshelashvili, I.; Krusche, B.; Steinacher, M.; Heng, Y.; Liu, Z.; et al. Physics performance report for PANDA: Strong interaction studies with antiprotons. Available online: https://inspirehep.net/record/816045 (accessed on 12 January 2016).
- Ablikim, M.; Bai, J.Z.; Bian, J.G.; Cai, X.; Chen, H.F.; Chen, H.S.; Chen, H.X.; Chen, J.C.; Chen, J.; Chen, Y. B.; et al. Observation of a resonance X(1835) in J/ψ→π+π-η′. Phys. Rev. Lett. 2005, 95. [Google Scholar] [CrossRef] [PubMed]
- Ablikim, M.; Achasov, M.N.; An, L.; An, Q.; An, Z.H.; Bai, J.Z.; Baldini, R.; Ban, Y.; Becker, J.; Berger, N.; et al. Confirmation of the X(1835) and observation of the resonances X(2120) and X(2370) in J/ψ→γπ+π-η′. Phys. Rev. Lett. 2011, 106. [Google Scholar] [CrossRef] [PubMed]
- Gao, C.S.; Zhu, S.L. Understanding the possible proton anti-proton bound state observed by BES collaboration. Available online: http://arxiv.org/pdf/hep-ph/0308205.pdf (accessed on 2 September 2015).
- Zhu, S.L.; Gao, C.S. X(1835): A possible baryonium? Commun. Theor. Phys. 2006, 46. [Google Scholar] [CrossRef]
- Ma, Y.L. Strong and electromagnetic decays of X(1835) as a baryonium state. J. Phys. G 2009, 36. [Google Scholar] [CrossRef]
- Bugg, D.V. Data on J/ψ→γ(K± π∓) and γ(ηπ+π-). Unpublished work. arXiv:0907.3015, 2009. [Google Scholar]
- Shan, J.; (Institute of High Energy Physics, Beijing, China). Personal communication, 2015.
- Ding, G.J.; Yan, M.L. Proton-antiproton annihilation in baryonium. Phys. Rev. C 2005, 72. [Google Scholar] [CrossRef]
- Yan, M.L. X(1835) as proton-antiproton bound state in Skyrme model. Unpublished work. 2006. [Google Scholar]
- Ding, G.J.; Yan, M.L. Productions of X(1835) as baryonium with sizable gluon content. Eur. Phys. J. A 2006, 28, 351–360. [Google Scholar] [CrossRef]
- Yu, J.S.; Sun, Z.F.; Liu, X.; Zhao, Q. Categorizing resonances X(1835), X(2120) and X(2370) in the pseudoscalar meson family. Phys. Rev. D 2011, 83. [Google Scholar] [CrossRef]
- Klempt, E.; Zaitsev, A. Glueballs, hybrids, multiquarks. Experimental facts versus QCD inspired concepts. Phys. Rept. 2007, 454, 1–202. [Google Scholar] [CrossRef]
- Li, D.M.; Ma, B. X(1835) and η(1760) observed by BES Collaboration. Phys. Rev. D 2008, 77. [Google Scholar] [CrossRef]
- Wang, Z.G. Analysis of the X(1835) and related baryonium states with Bethe-Salpeter equation. Eur. Phys. J. A 2011, 47. [Google Scholar] [CrossRef]
- Zhang, C.C.; Aihara, H.; Asner, D.M.; Aushev, T.; Bakich, A.M.; Ban, Y.; Belous, K.; Bischofberger, M.; Browder, T.E.; Chen, A.; et al. First study of ηc, η(1760) and X(1835) production via η′π+π- final states in two-photon collisions. Phys. Rev. D 2012, 86. [Google Scholar] [CrossRef]
- Ablikim, M.; Achasov, M.N.; Ai, X.C.; Albayrak, O.; Albrecht, M.; Ambrose, D.J.; Amoroso, A.; An, F.F.; An, Q.; Bai, J.Z.; et al. Observation and spin-parity determination of the X(1835) in J/ψ→γ η. Phys. Rev. Lett. 2015, 115. [Google Scholar] [CrossRef] [PubMed]
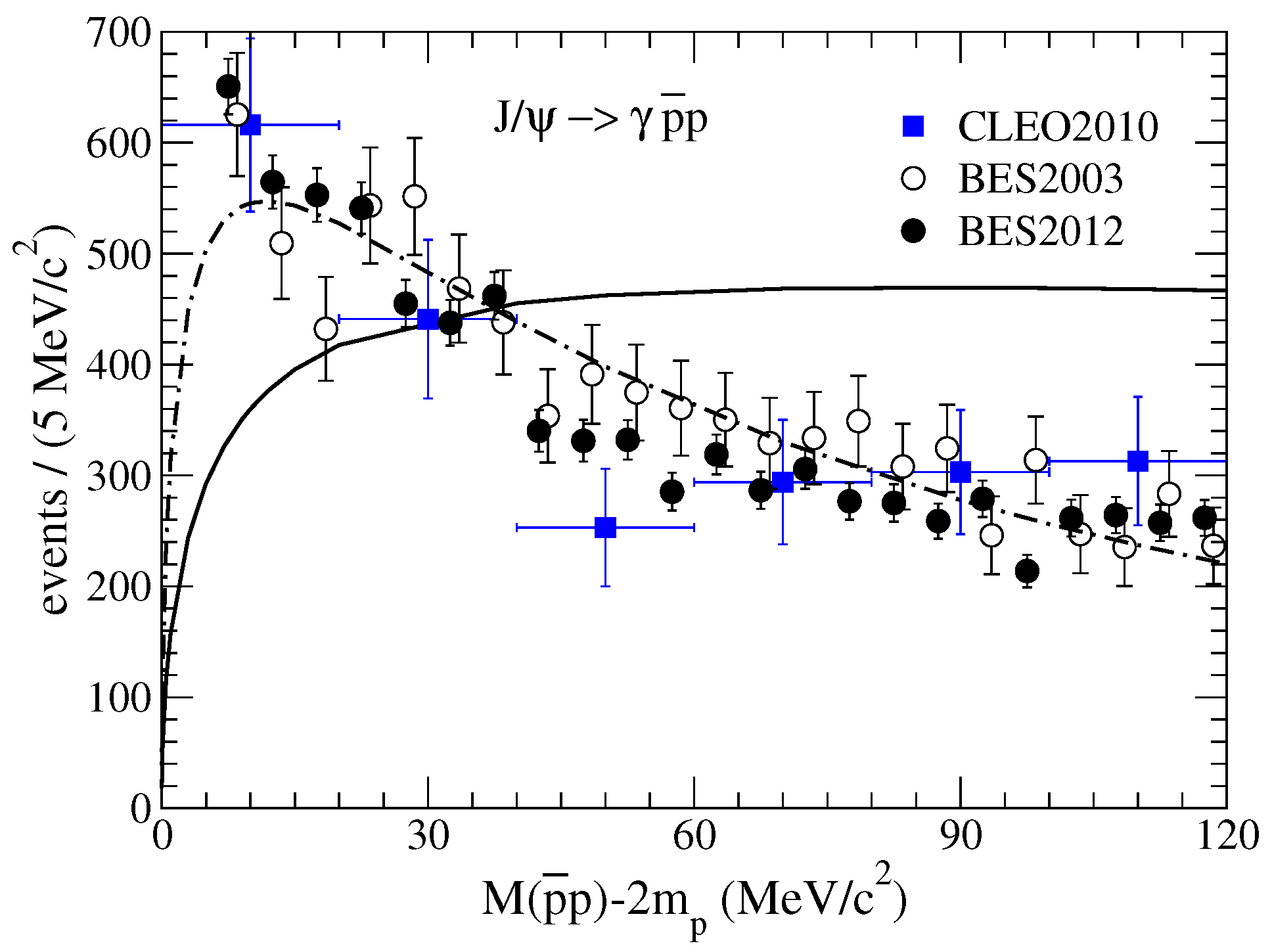
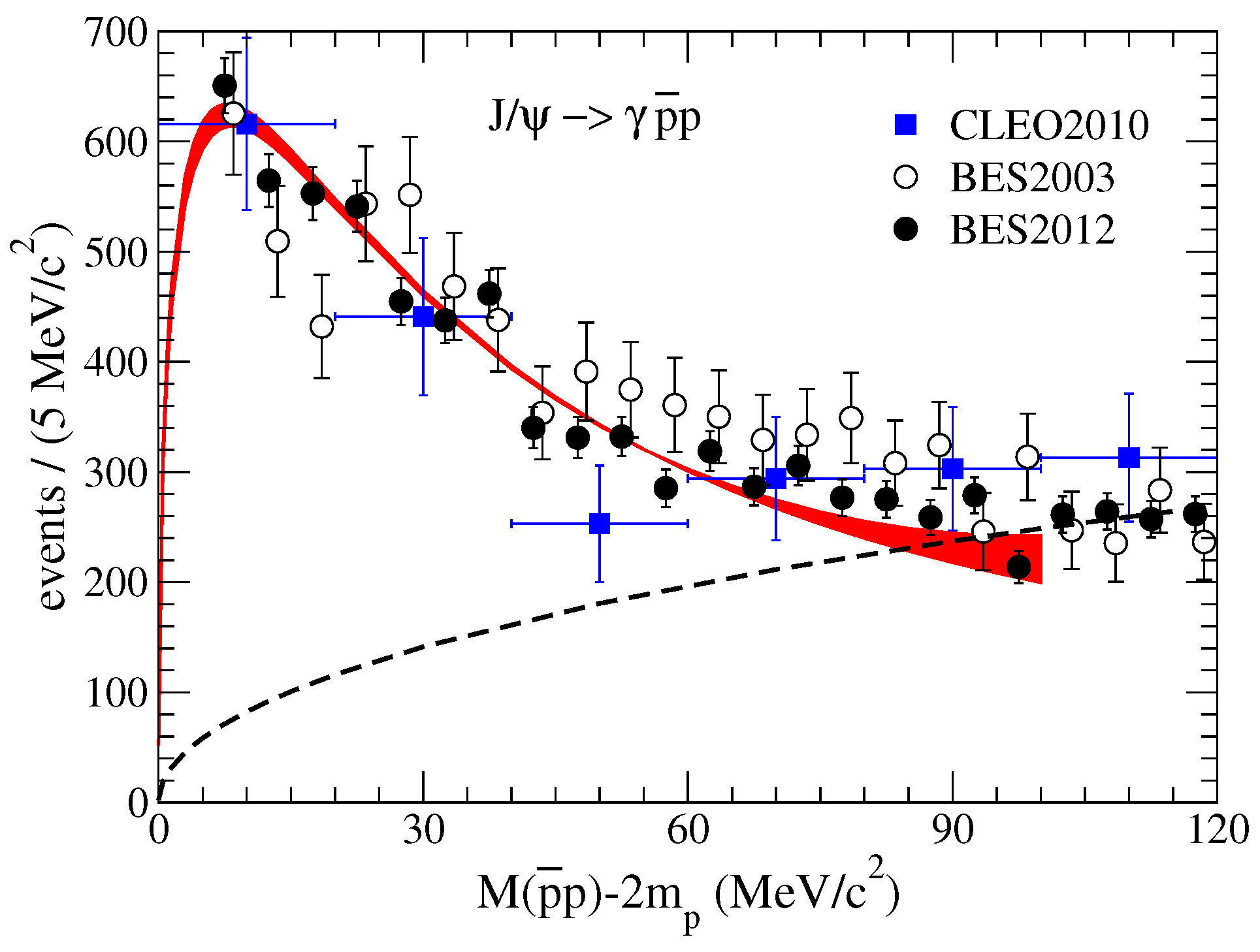
| I = 0 | I = 1 | |
|---|---|---|
| Chiral NLO [26] | −0.21 − i (1.20 1.21) | (1.03 1.04) − i (0.56 0.58) |
| Chiral NNLO [26] | −0.21 − i (1.21 1.22) | (1.02 1.04) − i (0.57 0.61) |
| Jülich model D [48] | −0.23 − i 1.01 | 0.99 − i 0.58 |
| Paris2009 [49] | 1.115 − i 0.856 | 0.684 − i 0.473 |
| Grach et al. [45] | 1.2 − i 0.9 | 0.1 − i 0.4 |
| Pirner et al. [46] | 1.1 − i 0.4 | −0.3 − i 0.8 |
| (eV) | Γ (eV) | |
|---|---|---|
| Chiral NLO [26] | −(306 361) | (1528 1553) |
| Chiral NNLO [26] | −(302 361) | (1545 1589) |
| Jülich model D [48] | −330 | 1380 |
| Paris2009 [49] | −778 | 1038 |
| Grach et al. | −564 | 1128 |
| Pirner et al. | −608 | 1042 |
| Experiment [50] | −740 ± 150 | 1600 ± 400 |
| [51] | −440 ± 75 | 1200 ± 250 |
© 2016 by the authors; licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons by Attribution (CC-BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liu, Y.-F.; Kang, X.-W.
Status of X(1835) and
Liu Y-F, Kang X-W.
Status of X(1835) and
Liu, Yong-Feng, and Xian-Wei Kang.
2016. "Status of X(1835) and
Liu, Y.-F., & Kang, X.-W.
(2016). Status of X(1835) and





