On the Self-Mobility of Point-Symmetric Hexapods

Abstract

In this article, we study necessary and sufficient conditions for the self-mobility of point symmetric hexapods (PSHs). Specifically, we investigate orthogonal PSHs and equiform PSHs. For the latter ones, we can show that they can have non-translational self-motions only if they are architecturally singular or congruent. In the case of congruency, we are even able to classify all types of existing self-motions. Finally, we determine a new set of PSHs, which have so-called generalized Dietmaier self-motions. We close the paper with some comments on the self-mobility of hexapods with global/local symmetries.

1. Introduction

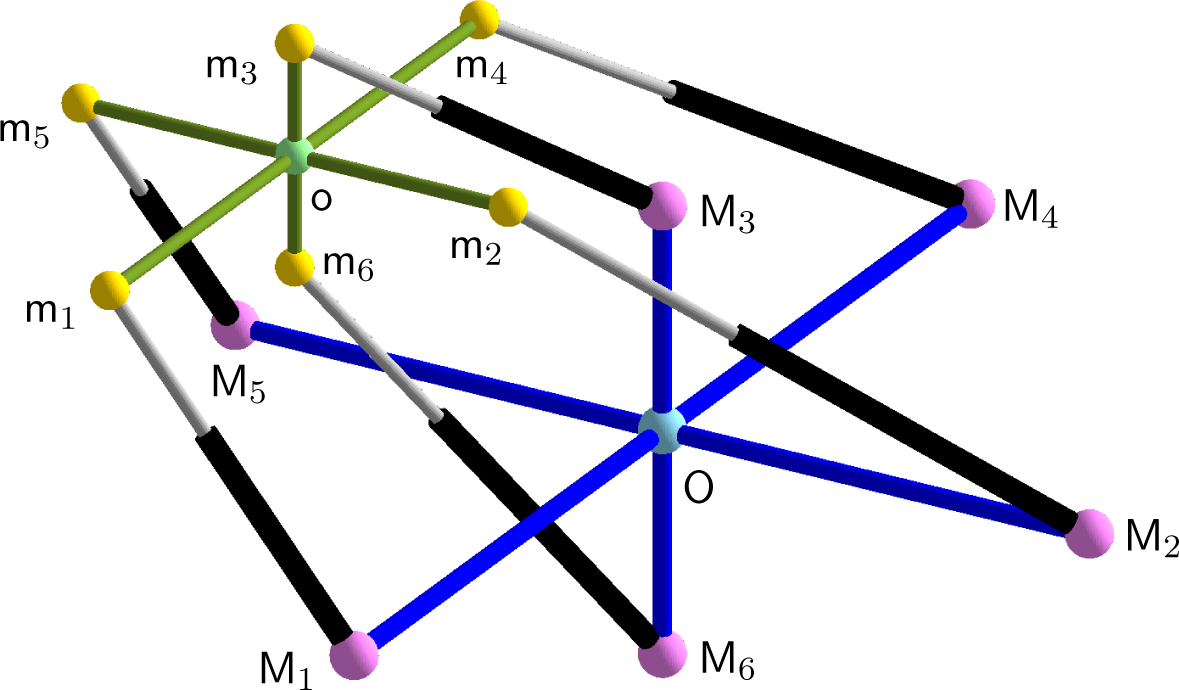
The geometry of a hexapod is given by the six base anchor points M_i with coordinates M_i := (A_i, B_i, C_i)^T with respect to the fixed frame and by the six platform anchor points m_i with coordinates m_i := (a_i, b_i, c_i)^T with respect to the moving frame (for i = 1, …, 6). Each pair (M_i, m_i) of corresponding anchor points of the fixed body Σ₀ (base) and the moving body Σ (platform) is connected by an SPS-leg, where only the prismatic joint (P) is active and the spherical joints (S) are passive. Note that for a hexapod, (M_i, m_i) ≠ (M_j, m_j) holds for pairwise distinct i, j ∈ {1, …, 6}. Moreover, a hexapod is called planar, if M₁, …, M₆, as well as m₁, …, m₆ are coplanar; otherwise, it is called non-planar.

If the geometry of this so-called parallel manipulator of the Stewart–Gough type is given, as well as the lengths R_i ≥ 0 of the six legs, the hexapod is generically rigid. However, under particular conditions, this body-bar framework can perform an n-dimensional motion (n > 0), which is called self-motion (=continuous flexion).

Note that these overconstrained motions are also solutions to the still unsolved problem posed by the French Academy of Science for the “Prix Vaillant” of the year 1904, which is also known as the Borel–Bricard problem (cf. [1–3]) and reads as follows: “Determine and study all displacements of a rigid body in which distinct points of the body move on spherical paths.”

The necessary and sufficient condition for the infinitesimal flexibility of the hexapodal manipulator is that the carrier lines of the six legs belong to a linear line complex [4,5]. The corresponding configurations of the hexapod are called singular (or shaky). It is well known that hexapods, which are singular in every possible configuration, possess self-motions in each pose (over the field C of complex numbers). These so-called architecturally singular manipulators are well studied and classified (for the planar case, we refer to [6–9], and for the non-planar case, see [10,11]). In contrast, only a few self-motions of non-architecturally singular hexapods are known [12], as their computation is a very complicated task.

In this article, we study a special class of hexapods, which is defined as follows:

Definition 1. A hexapod is called point-symmetric if it has the following properties (after a possible necessary relabeling of anchor points):

• M_i and M_i+3 are symmetric with respect to a point O of the fixed frame for i = 1, 2, 3;
• m_i and m_i+3 are symmetric with respect to a point o of the moving frame for i = 1, 2, 3;
• None of the following three distance conditions are satisfied for all distinct i, j ∈ {1, 2, 3} : (a)${\overline{\mathrm{OM}}}_i$; (b)${\overline{\mathrm{om}}}_i$; (c)${\overline{\mathrm{OM}}}_i$.

In the remainder of this article, we abbreviate “point-symmetric hexapod” as PSH. Item 3 of Definition 1 excludes trivial cases of architectural singularity; thus, only the following cases of architecturally singular PSHs remain:

Corollary 2. A PSH is architecturally singular if and only if one of the following cases hold (after a possible necessary renumbering of anchor points and the exchange of the platform and the base):

• m₁, …, m₆ are collinear;
• m₁, m₂, m₄, m₅ are collinear, M₁, M₂, M₄, M₅ are collinear and CR(m₁, m₂, m₄, m₅) = CR(M₁, M₂, M₄, M₅) holds, where CR denotes the cross-ratio;
• The platform and base are planar, and there exists a regular affinity with M_i ↦ m_i for i = 1, …, 6.

Proof. The corollary can directly be concluded from the literature on architecturally singularity (see, e.g., Theorem 1 of [6] and Theorem 3 of [10]). □

Definition 3. A PSH is called orthogonal if it fulfills the following conditions:

• OM₁, OM₂, OM₃ are pairwise orthogonal;
• om₁, om₂, om₃ are pairwise orthogonal.

In the remainder of this article, we abbreviate “orthogonal PSH” as OPSH. An OPSH is illustrated in Figure 1. Moreover, note that an OPSH cannot be architecturally singular due to Corollary 2.

Remark 1. For better imagining the OPSH’s geometry (with${\overline{\mathrm{OM}}}_i$ for i = 1, 2, 3), the anchor points can be interpreted as the vertices of an ellipsoid in the fixed/moving space.

1.1. Results on OPSHs

Without loss of generality (w.l.o.g.), we can assume for any OPSH that M₁ (resp. m₁) is located on the x-axis, M₂ (resp. m₂) on the y-axis and M₃ (resp. m₃) on the z-axis of the fixed frame (resp. moving frame). Therefore, we get:

$$\begin{array}{lll}A:=A_4=-A_1,& B:=B_5=-B_2,& C:=C_6=-C_3\end{array}$$

(1)

$$\begin{array}{lll}a:=a_4=-a_1,& b:=b_5=-b_2,& c:=c_6=-c_3\end{array}$$

(2)

and

$$A_2$$

(3)

$$a_2$$

(4)

We can assume w.l.o.g. that A, a, B, b, C ≥ 0 holds, as the indices of the anchor points can be permuted and the role of the platform and the base can be exchanged.

Under the assumption that the local symmetry of the platform and the base is extended to the legs in the following way:

$$R_i$$

(5)

Dietmaier determined in Equation (13) of [13] the two necessary and sufficient conditions for the finite mobility of the OPSH. The first condition reads as follows:

$$a$$

(6)

and therefore, it only depends on the geometry of the OPSH. In the second equation, which is given by:

$$($$

(7)

also the remaining free leg lengths R₁, R₂, R₃ are involved. If these two conditions are fulfilled, the OPSH has a one-dimensional flexion, which we call the Dietmaier self-motion.

It can easily be checked that the two conditions given in Equations (6) and (7) are fulfilled independently of R₁, R₂, R₃ if and only if A = a, B = b and C = c hold. As a consequence, there exists an orientation preserving congruence transformation τ with:

$$\tau$$

(8)

Therefore, hexapods, which possess this property, are called congruent.

All self-motions of congruent OPSHs were determined by Husty et al. in Example 3.3.8 of [14]. The following three types can be distinguished: two-dimensional translatoric self-motion, one-dimensional Schönflies self-motion and Dietmaier self-motion.

Remark 2. If τ of Equation (8) is an orientation reversing congruence transformation, we get a so-called reflection-congruent hexapod. In the more general case of τ being an equiform transformation, we name the hexapod an equiform one.

1.2. Outline of the Article

In Section 2, we give the basics of bond theory for hexapods and list some known results obtained by this method, including two corollaries for PSHs. After this preparatory work, we give in Section 3 necessary conditions for OPSHs in order to have a self-motion, which are also sufficient over ℂ.

In Section 4.1, we study the self-mobility of equiform PSHs in detail. Specifically, we classify all self-motions of congruent PSHs, which generalizes the above cited result of Husty et al. In Section 4.2, we determine a new set of PSHs with generalized Dietmaier self-motions.

We close the paper with some comments on the self-mobility of hexapods with global/local symmetries and list some open problems in this context, which are dedicated to future research.

2. Bond Theory

In this section, we give a short introduction to the theory of bonds presented in [15], which was motivated by the bond theory of overconstrained closed linkages with revolute joints given in [16]. We start with the direct kinematic problem of hexapods and proceed with the definition of bonds.

Due to the result of Husty [17], it is advantageous to work with Study parameters (e₀ : e₁ : e₂ : e₃ : f₀ : f₁ : f₂ : f₃) for solving the forward kinematics of hexapods. Note that the first four homogeneous coordinates (e₀ : e₁ : e₂ : e₃) are the so-called Euler parameters. Now, all real points of the Study parameter space P⁷ (seven-dimensional projective space), which are located on the so-called Study quadric $\mathrm{\Psi }$, correspond to an Euclidean displacement, with the exception of the three-dimensional subspace E of Ψ given by e₀ = e₁ = e₂ = e₃ = 0, as its points cannot fulfill the condition N ≠ 0 with $N$. The translation vector t := 2(t₁, t₂, t₃)^T and the rotation matrix R of the corresponding Euclidean displacement x ↦ Rx + t are given by:

$$t_1$$

(9)

and

$$\mathbf{R}$$

(10)

if the normalizing condition N = 1 is fulfilled. All points of the complex extension of P⁷, which cannot fulfill this normalizing condition, are located on the so-called exceptional cone N = 0 with vertex E.

By using the Study parametrization of Euclidean displacements, the condition that the point m_i is located on a sphere centered in M_i with radius R_i is a quadratic homogeneous equation according to Husty [17]. This so-called sphere condition Λ_i has the following form:

$$\begin{array}{l}{\mathrm{\Lambda }}_i:(a_i^2+b_i^2+c_i^2+A_i^2+B_i^2+C_i^2-R_i^2)N+4(f_0^2+f_1^2+f_2^2+f_3^2)\\ -2(a_i{A}_i+b_i{B}_i+c_i{C}_i)e_0^2-2(a_i{A}_i-b_i{B}_i-c_i{C}_i)e_1^2\\ +2(a_i{A}_i-b_i{B}_i+c_i{C}_i)e_2^2-2(a_i{A}_i+b_i{B}_i-c_i{C}_i)e_3^2\\ +4(c_i{B}_i-b_i{C}_i)e_0{e}_1-4(c_i{A}_i-a_i{C}_i)e_0{e}_2+4(b_i{A}_i-a_i{B}_i)e_0{e}_3\\ -4(b_i{A}_i+a_i{B}_i)e_1{e}_2-4(c_i{A}_i+a_i{C}_i)e_1{e}_3-4(c_i{B}_i+b_i{C}_i)e_2{e}_3\\ +4(a_i-A_i)(e_0{f}_1-e_1{f}_0)+4(b_i-B_i)(e_0{f}_2-e_2{f}_0)+4(c_i-C_i)(e_0{f}_3-e_3{f}_0)\\ +4(a_i+A_i)(e_3{f}_2-e_2{f}_3)+4(b_i+B_i)(e_1{f}_3-e_3{f}_1)+4(c_i+C_i)(e_2{f}_1-e_1{f}_2)=0\end{array}$$

(11)

Now, the solution for the direct kinematics over C of a hexapod can be written as the algebraic variety V of the ideal spanned by Ψ, Λ₁, …, Λ₆, N = 1. In general, V consists of a discrete set of points, which correspond with the (at most) 40 solutions of the forward kinematic problem.

We consider the algebraic motion of the mechanism, which are the points on the Study quadric that the constraints define; i.e., the common points of the seven quadrics Ψ, Λ₁, …, Λ₆. If the manipulator has an n-dimensional self-motion, then the algebraic motion also has to be of this dimension. Now, the points of the algebraic motion with N ≠ 0 equal the kinematic image of V. However, we can also consider the points of the algebraic motion, which belong to the exceptional cone N = 0. An exact mathematical definition of these so-called bonds can be given as follows (cf. Remark 5 of [15]):

Definition 4. For a hexapod, the set$\mathcal{B}$ of bonds is defined as:

$$\mathcal{B}$$

(12)

where V* denotes the variety V after the removal of all components, which correspond to pure translational motions. Moreover ZarClo(V*) is the Zariski closure of V*, i.e., the zero locus of all algebraic equations that also vanish on V*.

The restriction to motions, which are not pure translations (=non-translational motions) is caused by the following approach used for the computation of bonds: in the first step, we project the algebraic motion of the hexapod into the Euler parameter space P³ by the elimination of f₀,…,f₃. This projection is denoted by π_f. In the second step, we determine those points of the projected point set π_f (V), which are located on the quadric N = 0; i.e.,

$${\mathcal{B}}_f$$

(13)

Note that this set of projected bonds, which is denoted by ${\mathcal{B}}_f$, cannot be empty for a non-translational self-motion. Moreover, it is important to note that the set of bonds only depends on the geometry of the hexapod, and not on the leg lengths (cf. Theorem 1 of [15]).

Remark 3. A more sophisticated bond theory for pentapods and hexapods is based on a special compactification of SE(3), where the sphere condition Λ_i is only linear in 17 motion parameters. This approach, which was presented in [18], has many theoretical advantages compared to the method described above, but it is not suited for direct computations due to the large number of motion parameters. In contrast, the approach of the paper at hand was already successfully used for direct computations in [15,19–21].

Clearly, the kernel of this projection π_f equals the group of translational motions. As a consequence, a component of V, which corresponds to a pure translational motion, is projected to a single point O (with N ≠ 0) of the Euler parameter space P³ by the elimination of f₀, …, f₃. Therefore, the intersection of O and N = 0 equals Ø, which warrants the exclusion of pure translational motions within this approach. However, this does not cause any trouble, as all hexapods with pure translational self-motion were already characterized by the author in [15] as follows:

A hexapod possesses a pure translational self-motion, if and only if the platform can be rotated about the center m₁ = M₁ into a pose, where the vectors $\overrightarrow{{\mathrm{M}}_i{\mathrm{m}}_i}$ for i = 2, …, 6 fulfill the condition $r$. Moreover, all one-dimensional self-motions are circular translations, which can easily be seen by considering a normal projection of the manipulator in the direction of the parallel vectors $\overrightarrow{{\mathrm{M}}_i{\mathrm{m}}_i}$ for i = 2, …, 6. If all of these six vectors are zero-vectors, which corresponds with the case that the platform and the base are congruent, we get the already mentioned two-dimensional translational self-motion of a hexapod (cf. [19]).

The above given characterization of hexapods with translational self-motions can be reformulated/tightened for PSH as follows:

Corollary 5. A PSH possesses a pure translational self-motion, if and only if the platform can be rotated about the center o = O into a pose, where the vectors${\overrightarrow{{\mathrm{M}}_i\mathrm{m}}}_i$ for i = 1, 2, 3 fulfill the condition$r$.

Proof. The proof follows directly from the fact that the midpoint property is invariant under parallel projections. □

Moreover, we have the following result on multidimensional self-motions of PSHs:

Corollary 6. A non-architecturally singular PSH can only have an n-dimensional self-motion with n > 1 if it is congruent. The self-motion is the two-dimensional translation.

Proof. All non-architecturally singular hexapods with a two-dimensional (or higher-dimensional) self-motion were determined in [22] and [21], respectively. Theorem 5 of [22] implies the result immediately. □

3. OPSH With Self-Motions

Due to the above outlined computational approach of bonds, we divide the discussion of self-motions into two sections; namely translational and non-translational ones.

3.1. Necessary Condition for Translational Self-Motions

Based on Corollary 5, the following statement can be proven for OPSH:

Theorem 7. A pure translational self-motion of an OPSH can only exist in one of the following five cases:

$$\begin{array}{ll}\begin{array}{l}(\alpha )a-A=e_2=e_3=0,\\ (\gamma )c-C=e_1=e_2=0,\\ (\epsilon )a-A=b-B=c+C=e_3=0\end{array}& \begin{array}{l}(\beta )b-B=e_1=e_3=0\\ (\delta )c+C=e_0=e_3=0\end{array}\end{array}$$

Therefore, a necessary condition on the geometry is that$\overline{{\mathrm{OM}}_i}$ holds for at least one i ∈ {1, 2, 3}.

Proof. Due to Corollary 5, we compute the vectors $\overrightarrow{{\mathrm{M}}_i{\mathrm{m}}_i}$ by Rm_i − NM_i and express the condition for their linear dependency by K_k = o, where o denotes the zero-vector and K_k is given by:

$${\mathbf{K}}_k$$

(14)

for pairwise distinct i, j, k ∈ {1, 2, 3}. We denote the condition implied by the first, second and third line of K_k = o by $K_k^1$ and $K_k^3$, respectively. Therefore, we have to show that this resulting system of nine equations $K_1^1$ can only be fulfilled in one of the five cases α, …, ε. This can be done as follows:

Due to item 3(c) of Definition 1, we can assume that abc ≠ 0 (after a maybe necessary exchange of the platform and the base). Therefore, we can cancel the factors 2aN from $K_2^1$ and $K_3^1$, 2bN from $K_1^2$ and $K_3^2$, 2cN from $K_1^3$ and $K_3^3$ and only N from $K_1^1$, $K_2^2$ and $K_3^3$, respectively. We denote the remaining expressions by $G_i^j$. Then, we consider the following linear combinations:

$$\begin{array}{ll}{w}_1:G_3^2-G_2^3=2e_2{e}_3(A+a)=0,& w_2:G_3^2+G_2^3=2e_0{e}_1(A-a)=0\end{array}$$

(15)

$$\begin{array}{ll}{w}_3:G_3^1-G_1^3=2e_0{e}_2(b-B)=0,& w_4:G_3^1+G_1^3=2e_1{e}_3(b+B)=0\end{array}$$

(16)

$$\begin{array}{ll}{w}_5:G_1^2-G_2^1=2e_1{e}_2(c+C)=0,& w_6:G_1^2+G_2^1=2e_0{e}_3(c-C)=0\end{array}$$

(17)

As a, b > 0 and A, B ≥ 0 holds w₁ and w₄ can only be fulfilled in the following two cases:

• e₁ = e₂ = 0: Then, w₆ is either fulfilled for c = C (⇒ case γ) or for:

  • e₀ = 0: We get $G_3^3$, which cannot vanish without contradiction (w.c.).
  • e₃ = 0: In this case, we get:

    $$G_1^1$$

    (18)

    Therefore, a = A (⇒ case α), b = B (⇒ case β) or c = C (⇒ case γ) has to hold.
• e₃ = 0: For the discussion of this case, we can assume that e₁ = e₂ = 0 does not hold. We distinguish the following cases:

  • e₁e₂ ≠ 0: Under this assumption, w₅ yields c = −C. Then, we get:

    $$G_1^1$$

    (19)

    which implies either e₀ = 0 (⇒ case δ) or the reflection-congruent OPSH (⇒ case ε).
  • e₁ = 0, e₂ ≠ 0: Now, w₃ can only vanish either for b = B (⇒ case β) or e₀ = 0. In the latter case, we get $G_3^3$, which also implies b = B (⇒ case β).
  • e₂ = 0, e₁ ≠ 0: This case can be discussed analogously to the last one, with the sole exception that we always end up in the case α.

End of all cases. □

We close this subsection by giving some comments on the cases α, …, ε:

Note that the conditions given in the cases α, …, δ are not sufficient for the existence of a real translational self-motion, as in each case, one homogeneous quadratic equation in two Euler parameters remains, which may have complex solutions. In the case of a real solution, the loop M_i, m_i, m_i+3, M_i+3 forms a parallelogram during the translatoric self-motion of case α (i = 1), β (i = 2), γ (i = 3) and δ (i = 3).

Contrary, in case ε, we get for each orientation of the two-dimensional set determined by e₃ = 0, a real translatoric self-motion. For more details on this reflection-congruent case, we refer to [20].

Finally, it should again be noted that all translational self-motions are one-parametric circular translations with the exception of the congruent OPSH, which has a two-dimensional translatoric mobility. Note that the congruent OPSH belongs to all three cases α, β, γ simultaneously.

3.2. Necessary Condition for Non-Translational Self-Motions

Within this section, we prove the following main theorem:

Theorem 8. An OPSH can have a non-translational self-motion only if either Equation (6) is fulfilled or abcABC = 0 holds.

Proof. The proof of this theorem is done by the bond theory presented in Section 2; i.e., we determine the conditions for the existence of a projected bond. For the computational proof, we need the two linear combinations:

$$G_1$$

(20)

which read in detail as follows:

$$G_1$$

(21)

$$G_2$$

(22)

Note that G₁ and G₂ do not depend on f₀, f₁, f₂, f₃. Besides these two expressions, also the following three equations:

$${\mathrm{\Delta }}_{1,4}$$

(23)

$${\mathrm{\Delta }}_{2,5}$$

(24)

$${\mathrm{\Delta }}_{3,6}$$

(25)

are of interest, as they are linear in f₀, f₁, f₂, f₃. Now, we split the proof up into a general case and a special one.

General case: For this case, we assume that ν ≠ 0 holds, where ν is given by:

$$\nu$$

(26)

Under this assumption, we can solve the system Ψ, Δ_1,4, Δ_2,5, Δ_3,6, which is linear in f₀, f₁, f₂, f₃ for these variables. Plugging the obtained expressions in Λ₁, we obtain in the numerator the expression NG₀[5064], where G₀ is homogeneous of degree six in the Euler parameters. Note that the number in the brackets gives the number of terms.

Therefore, a real self-motion can only exist if the sextic surface G₀ = 0 and the two quadrics G₁ = G₂ = 0 have a curve in common. If this is the case, there also has to exist at least one intersection point (projected bond) of this curve with the exceptional quadric N = 0.

In the next step, we eliminate e₀ by computing the resultant H_i of G_i and N for i = 0, 1, 2. We get:

$$H_0$$

(27)

$$H_1$$

(28)

Now, we proceed with the elimination procedure by calculating the resultant L_k of H_i and H_j for pairwise distinct i, j, k ∈ {0, 1, 2}. Then, we eliminate e₁ by computing the resultant Q_k of L_i and L_j for pairwise distinct i, j, k ∈ {0, 1, 2}. Now, the necessary condition for the existence of a projected bond is that the greatest common divisor of Q₀, Q₁, Q₂ vanishes, which reads as follows (up to powers of the given factors):

$$e_2$$

(29)

As the back-substitution of e₂ = 0 implies e₁ = e₃ = e₀ = 0 (a contradiction), the theorem is proven for the general case.

Special case: Now, we consider the special case ν = 0. Therefore, we have to determine the condition for the existence of a common point of the four quadrics G₁ = G₂ = ν = N = 0.

If we replace G₀ by ν, the same elimination procedure as in the general case yields the following greatest common divisor (up to powers of the given factors) of the resulting final expressions:

$$e_2$$

(30)

As the back-substitution of e₂ = 0 implies again e₁= e₃ = e₀ = 0 (a contradiction), the theorem is proven. □

Remark 4. A consequence of the Theorems 7 and 8 is that an OPSH with$0$ for i = 1, 2, 3, which does not fulfill Equation (6), is free of self-motions. This is of importance for practical applications, as self-motions are dangerous, because they are uncontrollable and, thus, a hazard to man and machine. Therefore, being able to avoid OPSH designs that engender self-motion is of interest to engineers. Note that also the later given Theorem 4.1 should be read in this context.

Note that the condition abcABC = 0 is even sufficient for the existence of a real self-motion due to the following two examples. For the description of these trivial examples, we can assume (after a maybe necessary relabeling of anchor points and an exchange of platform and base) that a = 0 holds; i.e., m₁ = m₄ = o:

• Butterfly self-motions: If the platform is located in a way that the y-axis (z-axis) of the moving frame coincides with the z-axis (y-axis) of the fixed frame, then the platform can rotate freely around this line.
• Spherical four-bar self-motion: If the platform is in a configuration where the centers of the moving frame and fixed frame coincide (⇔ o = O), then the manipulator can perform a spherical four-bar motion with center o = O.

If the condition given in Equation (6) is fulfilled, we will see later on (cf. Theorem 12 under consideration of Remark 7) that this is already sufficient for the existence of a self-motion over ℂ, namely a Dietmaier self-motion. In the following, we want to take a closer look at the Dietmaier self-motion, in order to clarify the algebraic reasoning for this mobility.

3.2.1. Dietmaier Self-Motions

Under the assumption that the leg lengths fulfill Equation (5), the equations Δ_1,4, Δ_2,5, Δ_3,6 are homogeneous linear equations in f₀, …, f₃. Therefore, we distinguish the following two cases:

ν ≠ 0: In this case, we can solve Ψ, Δ_1,4, Δ_2,5, Δ_3,6 for f₀, …, f₃, which yields f₀ = f₁ = f₂ = f₃ = 0. As a consequence, we can only have a pure spherical self-motion, as o = O holds.

By projecting the OPSH onto a sphere centered in o = O and identifying antipodal points, we get a three-legged spherical three-dof RPR manipulator if abcABC ≠ 0 holds. Due to Theorem 6 of [15], it is known that such a spherical mechanism can only have a self-motion if two platform or base anchor points coincide, which cannot be the case, due to the underlying OPSH structure. Therefore, abcABC = 0 has to hold, and we get the above mentioned trivial solution of spherical four-bar self-motions (including special butterfly self-motions). Note that these are not Dietmaier self-motions, as for abcABC = 0, the condition given in Equation (6) cannot be fulfilled without contradiction over ℝ.

ν = 0: The above study shows that ν = 0 has to hold for a Dietmaier self-motion. Note that ν = G₁ = G₂ = 0 are three homogeneous quadratic equations in the Euler parameters without mixed terms e_ie_j for i 6= j ∈ {0, …, 3}. Under the Dietmaier conditions given in Equations (6) and (7), the three quadrics in the Euler parameter space are linearly dependent, which is already sufficient for the existence of a self-motion over ℂ. Based on this observation, we will determine a new set of PSH with non-translatoric self-motions in Section 4.2.

Example 1. For a detailed example of a Dietmaier self-motion, please see [13]. We only want to note in this context an example with rational coordinates fulfilling Equation (6):

$$a$$

(31)

Remark 5. It is still an open problem to determine all non-translational self-motions of an OPSH besides the known Dietmaier self-motion, spherical four-bar self-motion and the butterfly self-motion. It is only known (cf. last paragraph of Section 1.1) that congruent OPSHs also have Schönflies self-motions.

In this context, it should finally be noted that equiform OPSHs, which are not congruent, cannot possess non-translational self-motions, as Equation (6) has no solution over R. We will generalize this result in the next section.

4. PSHs with Self-Motions

The geometric characterization of PSHs with translational self-motions was already done in Corollary 5. Therefore, we focus on conditions for non-translational self-motions, where trivial examples are again the butterfly self-motion (which exists if four anchor points are collinear) and the spherical four-bar self-motion (which exists if $\overline{{\mathrm{OM}}_i}$ or $\overline{{\mathrm{om}}_i}$ holds). The procedure for the computation of such necessary conditions is the same as the one given in the proof of Theorem 8, but due to the number of involved variables and the resulting lengths and degrees of the obtained equations, the author failed to compute the final expressions Q₀, Q₁, Q₂ running Maple 17 on a computer with 12 GB RAM (not even for planar PSHs). Therefore, we restrict ourselves to equiform/congruent PSHs, which are studied next.

4.1. Equiform/Congruent PSHs

Theorem 9. If an equiform PSH has a non-translational self-motion, then it is either congruent or architecturally singular.

Proof. W.l.o.g., we can choose coordinate systems in the platform and the base in a way that we have:

$$a_{i+3}$$

(32)

for i = 1, 2, 3 with b₁ = c₁ = c₂ = B₁ = C₁ = C₂ = 0 and the remaining coordinates are coupled by:

$$a_i$$

(33)

where μ ≠ 0 is the similarity factor.

We proceed with analogous computations as in the proof of Theorem 8, but now, ν is given by:

$$\nu$$

(34)

For the general case (ν ≠ 0), we end up with the following necessary condition:

$$\mu$$

(35)

μA₁ = 0 contradicts the definition of a PSH, and B₂ = 0 results in an architecturally singular design (cf. Item 2 of Corollary 2). Moreover, $B_3^2$ implies B₃ = C₃ = 0, and we get again Item 2 of Corollary 2. The last factor can only be fulfilled for C₃ = 0 and A₂B₃ − A₃B₂ = 0, which also implies Item 2 of Corollary 2.

For the special case (ν = 0), we end up with the following necessary condition:

$$\mu$$

(36)

with

$$F_1$$

(37)

$$F_2$$

(38)

$$F_3$$

(39)

$$F_4$$

(40)

μA₁ = 0 contradicts the definition of a PSH, μ = 1 yields a congruent PSH and B₂ = 0 results in Item 2 of Corollary 2. Therefore, we can assume B₂ ≠ 0, which implies that F_i can only be fulfilled over ℝ for C₃ = 0 for i = 1, 2, 3, 4. Therefore, C₃ = 0 has to hold, which implies a planar equiform hexapod. These manipulators were already studied in detail in [23,24] with the result that they cannot have self-motions if they are not architecturally singular. This closes the proof of the theorem. □

In the context with Theorem 9, it should be noted that equiform hexapods have a translational self-motion if and only if they are reflection congruent or congruent (cf. [20]). In the latter case, we get the already mentioned two-dimensional translation. The non-translational self-motions of congruent PSHs, which were excluded from Theorem 9, are the content of the next theorem, which generalizes the result of Husty et al., mentioned at the end of Section 1.1. For the formulation of this theorem, the following definition is needed:

Definition 10. A self-motion of a PSH is called generalized Dietmaier self-motion, if Equation (5) holds and the equations Ψ, Δ_1,4, Δ_2,5, Δ_3,6 are linearly dependent with respect to f₀, …, f₃.

Theorem 11. A congruent PSH, which is not architecturally singular, can have the following two types of non-translation self-motions:

• Line-symmetric self-motions (including Schönflies self-motions as a special case),
• Generalized Dietmaier self-motions.

Proof. We use the same coordinatization as in the proof of Theorem 9 under consideration of μ = 1. Moreover, we can assume that the congruent PSH is non-planar, because it is well-known (cf. [24]) that planar ones can only have translational self-motions, if they are not architecturally singular.

Within the proof, we distinguish the following two cases:

• e₃ ≠ 0: Under this assumption, we can solve Ψ, Δ_1,4, Δ_2,5 for f₁, f₂, f₃. Plugging the obtained expressions into Δ_3,6 yields in the numerator $N$ with:

  $$E$$

  (41)

  Therefore, E = 0 has to hold. Beside this condition, we have again the linear combinations G1 and G₂ of Equation (20), which now read as follows:

  $$G_1$$

  (42)

  $$G_2$$

  (43)

  The last remaining condition results from Λ₁ after plugging the expressions for f₁, f₂, f₃ into it. However, this condition is not of interest, as one can always solve it for f₀ in case of a non-translational self-motion. Therefore, the two quadrics G₁ = G₂ = 0 and the plane E = 0 in the Euler parameter space have to have a curve s in common. We distinguish the following cases:

  • E = 0 is not fulfilled identically: In this case, we consider the expression of E after replacing e_i by f_i for i = 1, 2, 3. This yields:

    $$F$$

    (44)

    If we plug the obtained solutions for f₁, f₂, f₃ into F, the numerator factors into two factors, where one of it is E. Therefore, E = 0 implies F = 0; thus, we have two linear relations between the Study parameters. According to [25], this is one possible characterization of line-symmetric motions. In general, the curve s is a regular conic section, but it can also happen that it consists of one or two straight lines, implying Schönflies self-motions.
    Remark 6. Note that the geometric characterization of parallel manipulators with a Schönflies self-motion was already done in [26], and therefore, this case can only be a special case of it.
  • E = 0 is fulfilled identically: As A₁B₂C₃ ≠ 0 holds, this can only be the case if Equation (5) holds. Therefore, we get a generalized Dietmaier self-motion, which corresponds in the generic case with a curve s of degree four in the Euler parameter space.
• e₃ = 0: Now, Δ_1,4 and Δ_2,5 do not depend on f₀, f₁, f₂. Elimination of f₃ implies that E = 0 has to hold with:

  $$E$$

  (45)

  If E = 0 is not fulfilled identically, it already determines a straight line in the Euler parameter space, and therefore, we can only end up with a Schönflies self-motion.
  For R₁ = R₄ and R₂ = R₅, the equation E = 0 is fulfilled identically. Now, Δ_1,4 and Δ_2,5 can only vanish without contradiction for f₃ = 0. Due to e₃ = f₃ = 0, we can only end up with a line-symmetric self-motion.

This finishes the proof of Theorem 11. □

Example 2. We give an example of a line-symmetric self-motion, which is not a Schönflies motion, as this is the essential new possibility compared to the classification of congruent OPSHs. The geometry is given by:

$$A_1$$

(46)

We can compute f₁, f₂, f₃ from Ψ, Δ_1,4, Δ_2,5. Moreover, for the choice:

$$R_4^2$$

(47)

we get$e_1$ from the condition E = 0. Now, G₂ is a real-valued multiple of G₁, which determines the conic s (and therefore, the self-motion) by the equation:

$$($$

(48)

This equation can be solved for e₀ and plugged into Λ₁. As this final equation is quadratic in f₀, the self-motion is in general not rational.

A real line-symmetric self-motion (cf. Figure 2) is, for example, obtained for the following values: For$R_2^2$, $R_5^2$, $R_1^2$ and e₃ = 1, the self-motion is real if e₂ is within the interval [−t, t] with:

$$t$$

(49)

4.2. Generalized Dietmaier Self-Motions

In this section, we want to determine further PSHs (beside congruent PSHs), which have generalized Dietmaier self-motions. Under the assumption of Equation (5), we see that the equations Δ_1,4, Δ_2,5, Δ_3,6 are homogeneous linear in f₀, …, f₃. Therefore, a necessary and sufficient condition for a generalized Dietmaier self-motion is that the three quadrics G₁ = G₂ = ν = 0 in the Euler parameter space have a curve in common, where G₁ and G₂ are defined as in Equation (20) and ν is the remaining factor of the determinant of the coefficient matrix of Ψ, Δ_1,4, Δ_2,5, Δ_3,6 with respect to f₀, …, f₃ after splitting away N.

W.l.o.g., we can assume coordinate systems in the platform and the base in a way that o and O, respectively, are their origins, m₁ and M₁ are on their x-axes and m₂ and M₂ are located in their xy-planes, which implies:

$$\begin{array}{l}{b}_1=c_1=c_2=B_1=C_1=C_2=0,a_{i+3}=-a_i,A_{i+3}=-A_i\\ b_{i+3}=-b_i,B_{i+3}=-B_i,c_{i+3}=-c_i,C_{i+3}=-C_i\end{array}$$

(50)

for i = 1, 2, 3. With respect to these coordinate systems, the three equations have the following numbers of terms: G₁[48], G₂[68], ν[64]. The resultant of each of these two equations with respect to any Euler parameter is a quartic in the remaining ones with more than 20,000 terms. Therefore, the author was not able to compute the general conditions for the existence of a common curve s of G₁ = G₂ = ν = 0 (as the calculation of the resultant of two such quartics failed by Maple 17 on a computer with 12 GB RAM).

Therefore, we restrict to the following case: The quadrics G₁ = G₂ = ν = 0 are linearly dependent as for the original Dietmaier self-motion (cf. Section 3.2.1); i.e., there exists a linear combination:

$$L$$

(51)

As a consequence, the curve s in the Euler parameter space is of degree four.

Theorem 12. The following set D of non-architecturally singular PSHs with non-planar platform and non-planar base has a generalized Dietmaier self-motion:

$$\begin{array}{l}{b}_3=\frac{A_1{B}_3{c}_3}{a_1{C}_3},a_2=\frac{a_1{A}_2{b}_2{C}_3^3+(B_3{a}_1{b}_2{C}_3-A_1{c}_3{B}_3{B}_2)(A_2{B}_3-A_3{B}_2)}{c_3{C}_3^2{B}_2{a}_1}\\ a_3=\frac{A_1{B}_3{A}_2{a}_1{b}_2{C}_3^3-(c_3{B}_2{a}_1^2{C}_3^2-A_1{B}_3^2{a}_1{b}_2{C}_3+A_1^2{B}_3^2{B}_2{c}_3)(A_2{B}_3-A_3{B}_2)}{C_3^3{B}_2{a}_1^2{b}_2}\end{array}$$

(52)

and the following condition$k_6$ remains with:

$$\begin{array}{l}{k}_6=A_1{b}_2^2{a}_1^2{A}_2^2,k_5=B_2{a}_1^3{b}_2{c}_3(A_2^2+A_1^2+B_2^2)\\ k_4=A_1{a}_1^2[a_1^2{B}_2^2(c_3^2+b_2^2)+b_2^2(2B_3^2{A}_2^2-2A_2{B}_3{B}_2{A}_3+B_2^2{c}_3^2)]\\ k_3=B_2{a}_1{b}_2{c}_3[A_1^2(2B_3^2{A}_2^2+B_2^2{a}_1^2+B_3^2{a}_1^2-2A_2{B}_3{B}_2{A}_3)+a_1^2{(A_2{B}_3-A_3{B}_2)}^2]\\ k_2=A_1{a}_1^2[A_1^2{B}_2^2{B}_3^2{c}_3^2+(B_3^2{b}_2^2+B_2^2{c}_3^2){(A_2{B}_3-A_3{B}_2)}^2]\\ k_1=2b_2{A}_1^2{a}_1{B}_3^2{B}_2{c}_3{(A_2{B}_3-A_3{B}_2)}^2,k_0=B_2^2{A}_1^3{c}_3^2{B}_3^2{(A_2{B}_3-A_3{B}_2)}^2\end{array}$$

(53)

with respect to the coordinate systems of Equation (50). Therefore, $\mathcal{D}$ is a seven-dimensional set of PSHs (excl. similarities), where each design possesses at least a two-parametric set of generalized Dietmaier self-motions over$\mathbb{C}$. Only in the case of congruence we get a three-parametric set of generalized Dietmaier self-motions.

Proof. Due to the non-planarity assumption of the platform and the base, we can assume a₁A₁b₂B₂c₃C₃ 6= 0. Moreover, we can even assume w.l.o.g. that a₁, A₁, b₂, B₂ > 0 holds.

For the computational proof, we denote the coefficient of $e_0^i$ of L by L^ijkl. Then, we consider the following set of 10 necessary and sufficient equations:

$$\begin{array}{l}{w}_{10}:L^{2000}+L^{0200}+L^{0020}+L^{0002}=0,w_2:L^{2000}-L^{0200}-L^{0020}+L^{0002}=0\\ w_3:L^{2000}-L^{0200}+L^{0020}-L^{0002}=0,w_4:L^{2000}+L^{0200}-L^{0020}-L^{0002}=0\\ w_5:L^{1100}+L^{0011}=0,w_6:L^{1100}-L^{0011}=0,w_7:L^{1100}+L^{0011}=0\\ w_8:L^{1010}-L^{0101}=0,w_9:L^{1001}+L^{0110}=0,w_{10}:L^{1100}-L^{0011}=0\end{array}$$

(54)

In detail, these equations read as follows:

$$\begin{array}{l}{w}_1:\lambda (A_1{B}_2{C}_3-a_1{b}_2{c}_3)+{\lambda }_1(a_2^2+A_2^2+b_2^2+B_2^2-a_1^2-A_1^2+R_1^2-R_2^2)+\\ {\lambda }_2(a_2^2+A_2^2+b_2^2+B_2^2+c_3^2+C_3^2-a_1^2-A_1^2+R_1^2-R_3^2)=0\\ w_2:\lambda (a_1{b}_2{C}_3-A_1{B}_2{c}_3)-2{\lambda }_2{c}_3{C}_3=0\\ w_3:\lambda (a_1{c}_3{B}_2-A_1{C}_3{b}_2)-2{\lambda }_1{b}_2{B}_2-2{\lambda }_2{b}_3{B}_3=0\\ w_4:\lambda (b_2{c}_3{A}_1-B_2{C}_3{a}_1)+2{\lambda }_1(a_1{A}_1-a_2{A}_2)+2{\lambda }_2(a_1{A}_1-a_3{A}_3)=0\\ w_5:\lambda A_1(B_3{b}_2-B_2{b}_3)-2{\lambda }_2{b}_3{C}_3=0\\ w_6:\lambda a_1(B_2{b}_3-B_3{b}_2)+2{\lambda }_2{B}_3{c}_3=0\\ w_7:\lambda [A_1(a_2{b}_3-a_3{b}_2)+a_1(A_2{B}_3-A_3{B}_2)]+2{\lambda }_2{a}_3{C}_3=0\\ w_8:\lambda [A_1(B_2{a}_3-B_3{a}_2)+a_1(A_2{B}_3-A_3{B}_2)]+2{\lambda }_2{a}_3{C}_3=0\\ w_9:\lambda C_3(a_1{A}_2-A_1{a}_2)-2{\lambda }_1{a}_2{B}_2-2{\lambda }_2{a}_3{B}_3=0\\ w_{10}:\lambda c_3(A_1{a}_2-a_1{A}_2)+2{\lambda }_1{A}_2{B}_2+2{\lambda }_2{A}_3{b}_3=0\end{array}$$

(55)

Note that only the equation w₁ depends on the leg lengths R₁, R₂, R₃. W.l.o.g., we can solve w₂, w₃ for λ₁ and λ₂. As λ = 0 implies λ₁ = λ₂ = 0 a contradiction, we can set λ = 1. Then, w₅ implies b₃ of Equation (52), and w₈ can only vanish w.c. for:

$$a_3$$

(56)

Then, w₉ implies a₂ of Equation (52). Substituting this expression into Equation (56) yields the condition for a₃ given in Equation (52). Now, one condition on the geometry of the PSH remains, namely w₃, which is the equation given in Equation (53).

Remark 7. Note that w₃ implies the condition of Equation (6) under the additional assumption A₂ = A₃ = B₃ = 0 of an OPSH. Note that therefore the original Dietmaier self-motion is included as a special case.

Besides this condition, only w₁ is not fulfilled, which has the following structure: $q_0$. Therefore, each design of D has at least a two-parametric set of generalized Dietmaier self-motions. We only get a three-parametric set if this linear relation in $R_1^2$, $R_2^2$ and $R_3^2$ is fulfilled identically, which is studied next.

The condition q₃ = 0 implies $c_3$. Then, q₂ can only vanish w.c. for a₁ = A₁. Now, q₁ = 0 is already fulfilled identically, and we remain with the conditions w₃ and q₀ = 0. It can easily be seen that w₃ implies b₂ = B₂; i.e., the congruent PSH. Now, q₀ = 0 is also fulfilled identically. □

Remark 8. Finally, it should be noted that a geometric interpretation of the algebraic conditions determining the set D is missing. This also includes the geometric meaning of Equation (6) (cf. Remark 7).

Based on the set of equations w₁, …, w₁₀ used in the proof of Theorem 12, it can be shown by a straightforward discussion of cases that a linear combination of Equation (51) does not exist for non-architecturally singular PSHs, if the platform or the base or both are planar. Therefore, the only missing cases yielding a generalized Dietmaier self-motion are those where s is a cubic curve, a conic section or a straight line in the Euler parameter space. The latter case is of less interest, as it can only be a special case of the manipulators given in [26] (cf. Remark 6).

Example 3. In the following, we give an example for a generalized Dietmaier self-motion. The PSH of the set D is given by:

$$\begin{array}{l}{A}_1=5,A_2=c_3=a_1=3,B_2=b_3=2,B_3=1\\ A_3=\frac{3}{2},b_2=\frac{4}{3},a_2=\frac{5}{3},C_3=a_3=\frac{5}{2}\end{array}$$

(57)

Under consideration of Equation (5), the leg lengths are determined by:

$$R_1^2$$

(58)

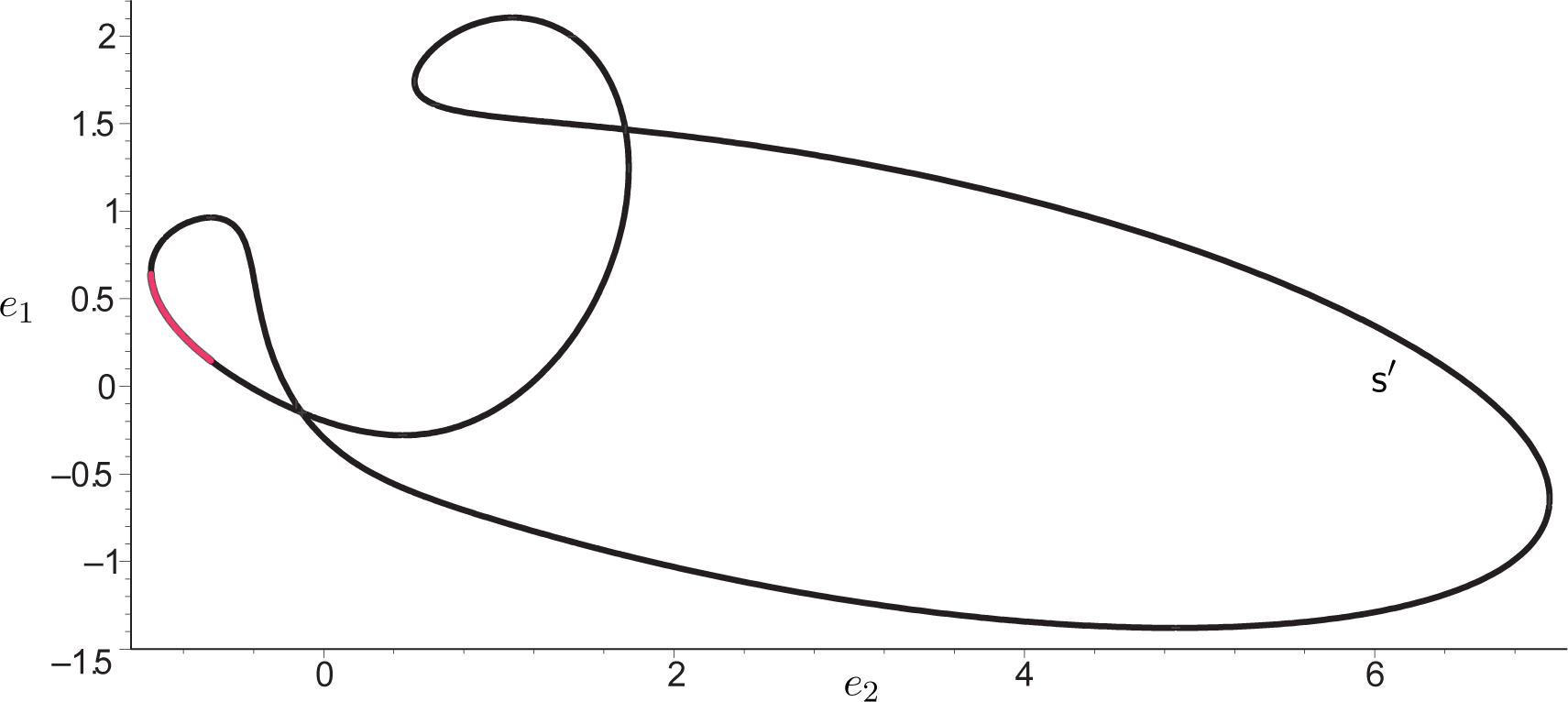
The projection of the quartic curve s in the Euler parameter space onto the e₁e₂e₃-plane by the elimination of e₀ yields the planar quartic curve s^′ given by:

$$\begin{array}{l}1,131,008e_1^4-2,583,808e_1^2{e}_2{e}_3+588,819e_2^2{e}_3^2-2,187,728e_2^2{e}_1{e}_3-1,503,016e_2^3{e}_3+\\ 3,478,718e_3^2{e}_1{e}_2+890,008e_3^3{e}_2-363,296e_1^3{e}_2-2,745,696e_1^3{e}_3+1,077,168e_3^3{e}_1+\\ 499,406e_2^3{e}_1+212,389e_2^4+149,462e_3^4+1,653,813e_1^2{e}_2^2+1,028,597e_1^2{e}_3^2=0\end{array}$$

(59)

which is illustrated in Figure 3. The red plotted part of s′ corresponds with the real self-motion illustrated in Figure 4.

5. Conclusions and Future Work

We formulated necessary conditions for the geometry of OPSHs in order to possess a self-motion (cf. Theorems 7 and 8). Moreover, these conditions are also sufficient for the existence of such motions over ℂ. We also gave a full discussion of self-motions of equiform/congruent PSHs, which are not architecturally singular (cf. Section 4.1). Due to the large number of unknowns, a classification of general PSHs with self-motions remains open. Even though not all PSHs with a generalized Dietmaier self-motion (cf. Definition 10) could be determined, we were able to solve the most general case of generalized Dietmaier self-motions (cf. Theorem 12), which implies new solutions to the more than 100-year-old Borel–Bricard problem.

This study also shows that even for PSHs, which have a simplified geometry due to the symmetry assumptions, the computation of self-motions remains a very challenging/complicated task. This gives us just an idea of the complexity of the still open Borel–Bricard problem.

5.1. Hexapodal Self-Motions Viewed under the Aspect of Symmetry

One can think of other local symmetries in the platform and the base, respectively, besides the point-symmetry studied within this article; e.g., line-symmetry, plane-symmetry, 120°-rotational symmetry, etc. Results on self-motions of equiform/congruent plane-symmetric hexapods were already given in [19,20], respectively. Moreover, a full discussion of self-motions of the original Stewart–Gough hexapod (the platform and base both have a 120°-rotational symmetry) was given by Karger and Husty in [27]. Clearly, one can also study hexapods with mixed local symmetries; e.g., the platform is point-symmetric and the base is plane-symmetric. The self-mobility of hexapods with local symmetries is dedicated to future research.

Obviously, the local symmetries in the platform and base are preserved under a self-motion. However, there also exist hexapods with self-motions, which preserve the global symmetry of the hexapod. Examples for this phenomenon are the Bricard octahedra of Type 1 (=line-symmetric octahedron) and Type 2 (=plane-symmetric octahedron; see, e.g., [28]), respectively, as they can also be seen as hexapods.

A generalization of the self-motions of Type 1 Bricard octahedra was obtained by the author in [29]. These planar hexapods with so-called Type 2 DM (=Darboux–Mannheim) self-motions do not possess the global symmetry property any longer, but their self-motions are still line-symmetric ones (cf. [25]). In this context, it should finally be noted that most of the known hexapodal self-motions (cf. [12]) are line-symmetric motions.