Three-Dimensional Seismic Analysis of Symmetrical Double-O-Tube Shield Tunnel

Abstract

The symmetrical Double-O-Tube (DOT) shield tunneling method, first developed in Japan in the 1980s, offers advantages in optimizing cross-sectional area and reducing construction space. While past studies have primarily focused on construction-induced settlement or empirical modeling, this study presents the first comprehensive three-dimensional seismic analysis of Taiwan’s first DOT shield tunnel, part of the CA450A contract of the Taoyuan International Airport MRT. A detailed numerical simulation is conducted using PLAXIS 3D 2024 with the Hardening Soil model, capturing both static and dynamic responses under earthquake loading. Notably, the analysis incorporates full-direction seismic input (3D) using Arias intensity-based filtering and scaling to assess the tunnel’s mechanical behavior under varying seismic intensities. Key structural responses such as displacement, axial force, shear force, and bending moment are evaluated. The findings reveal critical deformation patterns and stress concentrations in the central support structure, offering novel insights for the seismic design of complex multi-cell shield tunnels in high-risk seismic zones.

1. Introduction

1.1. Background

Taiwan, situated in the seismically active Pacific Ring of Fire, experiences around 40,000 earthquakes annually, with over 1000 being perceptible. Its complex topography, dominated by mountains and hills, leads to high population densities in basins and plains. To address urban congestion, underground transportation systems have been actively developed. However, underground construction faces challenges due to utilities, railway projects, and metro expansions. Traditional single-bore shield tunnels are complex, risky, time-consuming, and costly. To overcome these issues, Taiwan introduced the Double-O-Tube (DOT) shield tunneling method, which reduces tunnel size, minimizes structural impact, and enhances efficiency. The DOT method was ultimately chosen over twin single-bore tunnels, and the Taipei-Sanchong section of the Taoyuan Airport MRT, utilizing this approach, has been in operation for several years.

This study focuses on the Taipei-Sanchong section of the Taoyuan Airport MRT, utilizing the finite element software PLAXIS 3D 2024 for numerical analysis. The research simulates the stress distribution and displacement changes of the completed tunnel under seismic conditions to examine its overall mechanical behavior. Additionally, this study evaluates the tunnel’s response to different earthquake intensity levels, providing valuable insights for future engineering applications.

1.2. The Literature Review

Ke [1] investigated ground settlement induced by DOT shield tunnel construction using the superposition and equal-area methods. The study found that both empirical approaches produced similar settlement trough predictions, aligning well with observed data but showing slight differences in maximum settlement values. These methods, based on a normal distribution curve, identify the inflection point (i-value) as a key parameter. Additionally, PLAXIS analysis was used to obtain full-section settlement curves, which closely matched empirical predictions in inflection point locations. This research provides valuable insights into evaluating and predicting ground settlement in shield tunnel construction.

Wei [2] investigated ground settlement effects induced by DOT shield tunneling using different soil models, including the Hardening Soil (HS) with Small Strain Model (SSM), Modified Cam-Clay Model, and Soft Soil Creep Model. The study revealed significant variations in settlement predictions among these models. The HS with SSM demonstrated better accuracy in capturing the convergent settlement pattern above the tunnel face, while the Modified Cam-Clay Model predicted a wider settlement trough, indicating lower predictive precision. Monitoring data indicated that most settlement in this case was due to immediate settlement, with compression and secondary compression accounting for only 0.7–2% in most sections. These findings highlight the critical influence of ground conditions on settlement behavior and provide comparative insights into model selection for DOT shield tunnel analysis.

A seismic time history typically includes all variations in acceleration from the onset of the earthquake to the period of strong ground motion and eventually back to background noise levels. However, for engineering design purposes, attention is usually focused only on the strong motion portion of the time history. Several methods have been developed to evaluate the duration of strong ground motion during an earthquake.

One common approach is to use bracketed duration (Bolt [3]), which is defined as the time interval between the first and last exceedance of a specified acceleration threshold—commonly 0.05 g. Another method defines the duration based on the time interval between when 5% and 95% of the total energy has been accumulated (Trifunac and Brady [4]). Additionally, Boore [5] used the concept of corner period, and McCann and Shah (1979) evaluated strong motion duration based on the rate of change in cumulative root mean square (RMS) acceleration. Power spectral density has also been used to define strong motion duration (Vanmarcke and Lai [6]). Other definitions of earthquake duration have also been proposed by researchers such as Perez [7] and Trifunac and Westermo [8]).

The application of duration time is not limited to the assessment of ground motion; it also plays a role in representing the equivalent number of cycles in earthquake engineering. This concept was initially introduced during the development of procedures for evaluating liquefaction potential (Seed et al. [9]). The primary objective was to convert irregular shear stress time histories into a series of uniform harmonic stress cycles, thereby introducing the concept of equivalent significant stress cycles.

Another parameter closely related to root mean square acceleration is Arias intensity (Arias [10]). Arias intensity is a measure used to quantify the energy of ground motion during an earthquake, typically defined as half the integral of the squared acceleration time history. This parameter takes into account the temporal variation of seismic acceleration and is widely used in earthquake engineering to assess the physical damage to structures caused by seismic events. Arias intensity has units of velocity, usually expressed in meters per second (m/s).

The calculation of Arias intensity is based on the integration of the entire acceleration time history of an earthquake and is therefore independent of the specific method used to define the duration of strong ground motion. It quantifies the cumulative energy of ground motion during an earthquake and serves as a significant parameter independent of the definition of strong motion duration.

According to research by Ang [11], a linear relationship was found between maximum deformation and absorbed hysteretic energy. This implies that, as the maximum deformation increases, the structural damage index also increases correspondingly. This highlights the importance of maximum deformation in the process of structural damage, with absorbed hysteretic energy being one of the key factors in this relationship.

The Applied Technology Council [12] defined the standard response spectrum by normalizing it using two factors. The Effective Peak Acceleration (EPA) is defined as the average spectral acceleration over the period range of 0.1 to 0.5 s divided by 2.5, which is the standard amplification factor for a 5% damping spectrum. The Effective Peak Velocity (EPV) is defined as the average spectral velocity over a 1 s period, also divided by 2.5.

Figure 1 illustrates the relationship between EPA and EPV, as well as the variation in average spectral acceleration and velocity over the period range. The purpose is to smooth the design response spectrum and reduce the influence of localized peaks on EPA and EPV. These parameters are now widely adopted in building design codes.

Shen et al. [13] investigated tunnel failure mechanisms and seismic damage factors after the Wenchuan Earthquake. They analyzed data from 52 damaged tunnels, classifying structural damage based on a new severity standard. The study examined damage characteristics in shallow- and deep-buried tunnels, as well as pavement damage. Using real cases, numerical simulations with FLAC and artificial ground motions were conducted to explore seismic damage at tunnel portals. The relative displacement (RD) method was used to analyze portal deformation, helping explain their behavior under strong earthquakes.

Zhou et al. [14] studied the impact of contact interface properties on the seismic response of tunnels with composite lining, considering both cases with and without a buffer layer. A finite element model was developed using the convergence–confinement and tracking element methods. A modified response acceleration method (MRAM) was proposed and validated for its accuracy and efficiency. Using MRAM, the effects of interface conditions, peak ground acceleration (PGA), and buffer layer thickness were analyzed. Results show that interface properties significantly influence tunnel seismic response, including internal forces and tensile damage. Idealized interface conditions (no-slip or full-slip) greatly amplify or reduce seismic effects. The findings offer valuable guidance for tunnel seismic design.

Ren et al. [15] addressed the challenge of accurately estimating the longitudinal seismic response of shield tunnels, which is more complex than the transverse response. A theoretical model was developed using a Timoshenko beam on Winkler foundations, incorporating residual and seismic-induced axial forces. The model was solved using the finite difference method and validated through case studies of 6.2 m diameter shield tunnels. Results showed that including axial force increased tunnel stiffness and reduced internal forces and deformation. The study also analyzed the effects of residual axial force, seismic wavelength, incidence angle, and foundation stiffness. Notably, wavelengths between 20 and 100 m and higher foundation stiffness increased joint opening and deformation. These findings offer theoretical guidance for longitudinal seismic design of shield tunnels.

Yang et al. [16] highlight that the seismic response of shield tunnels differs significantly from that of aboveground structures. While aboveground structures are mainly affected by peak acceleration and frequency, shield tunnels are more influenced by ground displacement due to surrounding soil. This study emphasizes that applying aboveground seismic concepts to tunnels is inappropriate. Through dynamic time history analysis, it was found that tunnel leveling has minimal impact, and seismic response is closely tied to relative ground displacement. A strong linear relationship was observed between tunnel inclination and internal forces, suggesting that inclination can serve as a simple and effective indicator for seismic response, reducing the need for complex analyses and improving design efficiency.

Wang et al. [17] reviewed studies on tunnel seismic performance following the 1999 Chi-Chi Earthquake (Mw 7.6), which damaged 49 tunnels within 60 km of the epicenter, including three collapses, challenging the belief that tunnels, especially mountain tunnels, are inherently earthquake-resistant. Research over the past two decades has included damage investigations, physical modeling, numerical analyses, and field monitoring. Findings show tunnels generally suffer less damage than surface structures, with most damage linked to adjacent ground failure or fault crossings. Seismic damage often occurs in shallow tunnels, near slopes or portals, and under certain geological conditions. Shaking table and centrifuge tests reveal tunnel responses such as rocking, ovaling, and racking, with residual earth pressures and lining forces remaining post-quake. Nonuniform seismic excitation causes greater tunnel response than uniform loading. Physical modeling also helps validate and calibrate numerical simulations. Modern seismic tunnel analysis benefits from detailed 3D geological models and region-specific ground motion assessments. Because residual stresses persist after seismic events, damage may accumulate, highlighting the need for improved evaluation methods and increased field monitoring of tunnel seismic responses.

While existing research has explored ground settlement behavior, contact interface effects, and general seismic responses in shield tunnels, no prior study has conducted a full three-dimensional dynamic analysis specifically on the symmetrical Double-O-Tube (DOT) shield tunnel structure under real earthquake loading in Taiwan. Furthermore, unlike previous work that uses simplified models or 2D assumptions, this study adopts a high-fidelity PLAXIS 3D model with detailed soil–structure interaction, 3D seismic inputs, and intensity-scaling analysis. By integrating Arias intensity, free-field boundary conditions, and directional displacement evaluation, this research provides a novel contribution to the understanding of DOT tunnel performance under seismic excitation, which is crucial for infrastructure resilience in seismic-prone urban environments.

2. Methodology

2.1. Research Methods and Content

PLAXIS 3D is a finite element software originally developed in 1987 by Delft University of Technology to address dike design challenges in the Netherlands’ soft soils, later joined by the University of Stuttgart. In 1993, PLAXIS B.V. was founded, and PLAXIS 3D was officially released in 2010 for 3D geotechnical analysis. The software is widely used to simulate soil and rock behavior, supporting the design and safety assessment of underground structures. It is capable of handling various geotechnical applications, including deep excavations, slope stability, tunneling, pipelines, and groundwater flow.

The software provides a wide array of functions and options, allowing users to define material properties, loading conditions, and boundary conditions to realistically simulate the behavior of soil and rock. PLAXIS 3D has been widely applied in infrastructure, civil engineering, underground tunneling, shield tunneling, and geotechnical engineering. It is widely recognized as a reliable tool for soil and rock mechanics analysis and plays a vital role in engineering practice.

(1)

Finite Element Method

PLAXIS is based on the finite element method, which discretizes complex soil and rock structures into small mesh elements and then uses differential equations and mechanical principles to simulate the behavior of soil and rock. Users can create geometric models, define material parameters, apply loads and boundary conditions, and then perform both static and dynamic analyses to obtain the results of their studies.

(2)

Application of Soil Models

PLAXIS 3D offers a wide range of soil behavior models, which have evolved alongside advances in soil mechanics research and ongoing software updates. These models account for various characteristics of soil materials, such as strain hardening (or softening), critical state behavior, shrinkage and swelling, creep, and more. Currently, the software includes a total of 16 models available for user analysis, including the Mohr–Coulomb model, Linear Elastic model, Hoek–Brown model, Hardening Soil model, and Hardening Soil Small-Strain model, among others. For detailed information, users can refer to the PLAXIS 3D 2024 User Manual.

Compared to the elastic–perfectly plastic model, the hardening plasticity model features an evolving yield surface that expands with plastic deformation, making it more suitable for capturing the nonlinear behavior of soils and rocks. There are two main types of hardening: shear hardening, which simulates irreversible deformation under shear stress, and compression hardening, which describes plastic deformation due to consolidation or isotropic stress.

The Hardening Soil model is an advanced model that combines the Mohr–Coulomb failure criterion with plasticity theory. It accounts for stress-dependent stiffness and shows a hyperbolic stress–strain relationship in triaxial tests, offering a more realistic soil response than traditional models. Unlike idealized linear models, this approach reflects real soil behavior, including dilatancy and yield cap effects.

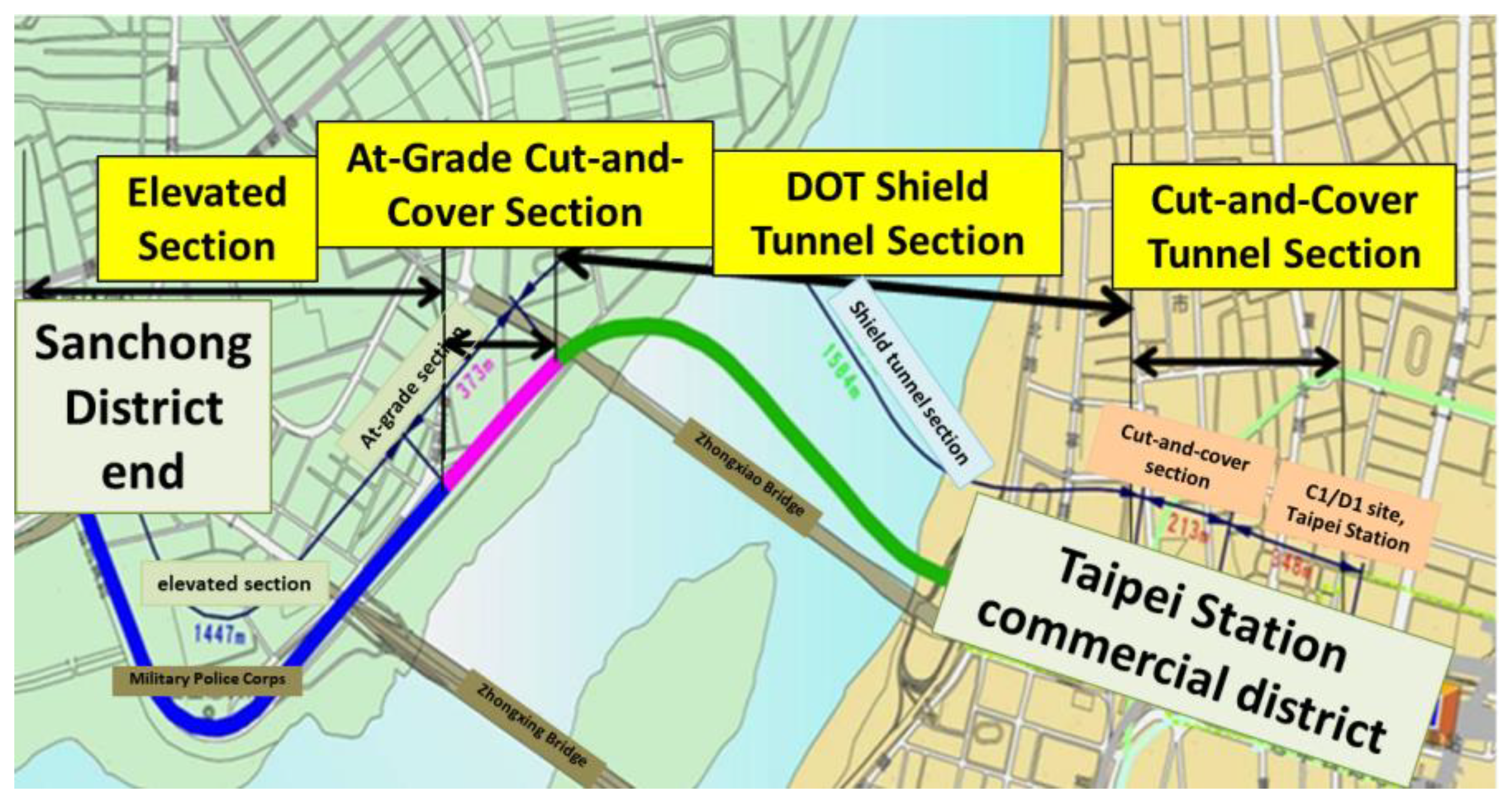
2.2. Case Introduction

The CA450A section of the Taoyuan Airport MRT line spans between Taipei and Sanchong Stations, covering key segments such as elevated tracks, at-grade and cut-and-cover sections, and shield tunnels. This project involves various construction methods, with the shield tunnel between Sanchong and Taipei being the main focus of this study. The CA450A project was managed by the Northern Region Engineering Office of the Taipei City Government’s Department of Rapid Transit Systems. Design was handled by CECI Engineering Consultants, while construction was jointly executed by Dahsin Engineering Co., Ltd. and Shimizu Corporation (Taiwan Branch) (Taipei, Taiwan). Shield tunneling began on 8 December 2009 and was completed on 5 December 2010. The project scope is shown in Figure 2.

In this case study, the CA450A contract section is located within the SC and T2 zones of the Taipei Basin. The DOT shield tunnel section extends from the Sanchong side in New Taipei City, crossing under the Tamsui River, and continues to the west side of Beimen Station (G14) on the Songshan Line in Taipei City. The terrain along the route is flat, with ground elevations of approximately EL. 3 m at the Sanchong end and EL. 4 m at the Taipei end. The area is situated within the alluvial soil deposits of the Tamsui River basin in the Taipei Basin. The geological conditions are characteristic of Quaternary sediments, primarily composed of clay or sandy gravel.

The soil layers in this area can be divided into three main formations: the Xinzhuang Formation, the Jingmei Formation, and the Songshan Formation. The soil profile is illustrated in Figure 3. The shield tunnel section passes through the Songshan Formation, and the simplified geotechnical parameters for this section are presented in

Table 1. Simplified soil layer parameters for the DOT shield tunnel section.

Layer Depth (m)	Soil Classification	Average γ ($\mathbf{T}/{\mathbf{m}}^2$)	Average N	Su ($\mathbf{T}/{\mathbf{m}}^2$)	${\mathit{c}}^{\mathbf{\prime }}$	${\mathit{\phi }}^{\mathbf{\prime }}$ (°)	E ($\mathbf{k}\mathbf{N}/{\mathbf{m}}^2$)
6.6	CL	1.94	4	1.3	0	28	6500
14.9	SM	1.98	8	-	0	29	20,000
24.5	CL	1.9	7	4.4	0	29	22,000
37.2	SM	1.88	18	-	0	32	45,000
45.9	CL	1.93	24	10	0	32	50,000
52.3	SM	1.97	26	-	0	33	65,000
56	GM	2.32	100	-	0	37	250,000

.

3. Numerical Simulation

This study utilizes the three-dimensional finite element software PLAXIS 3D 2024 for simulation analysis. This section focuses on introducing the steps of the numerical simulation. The detailed procedure is as follows.

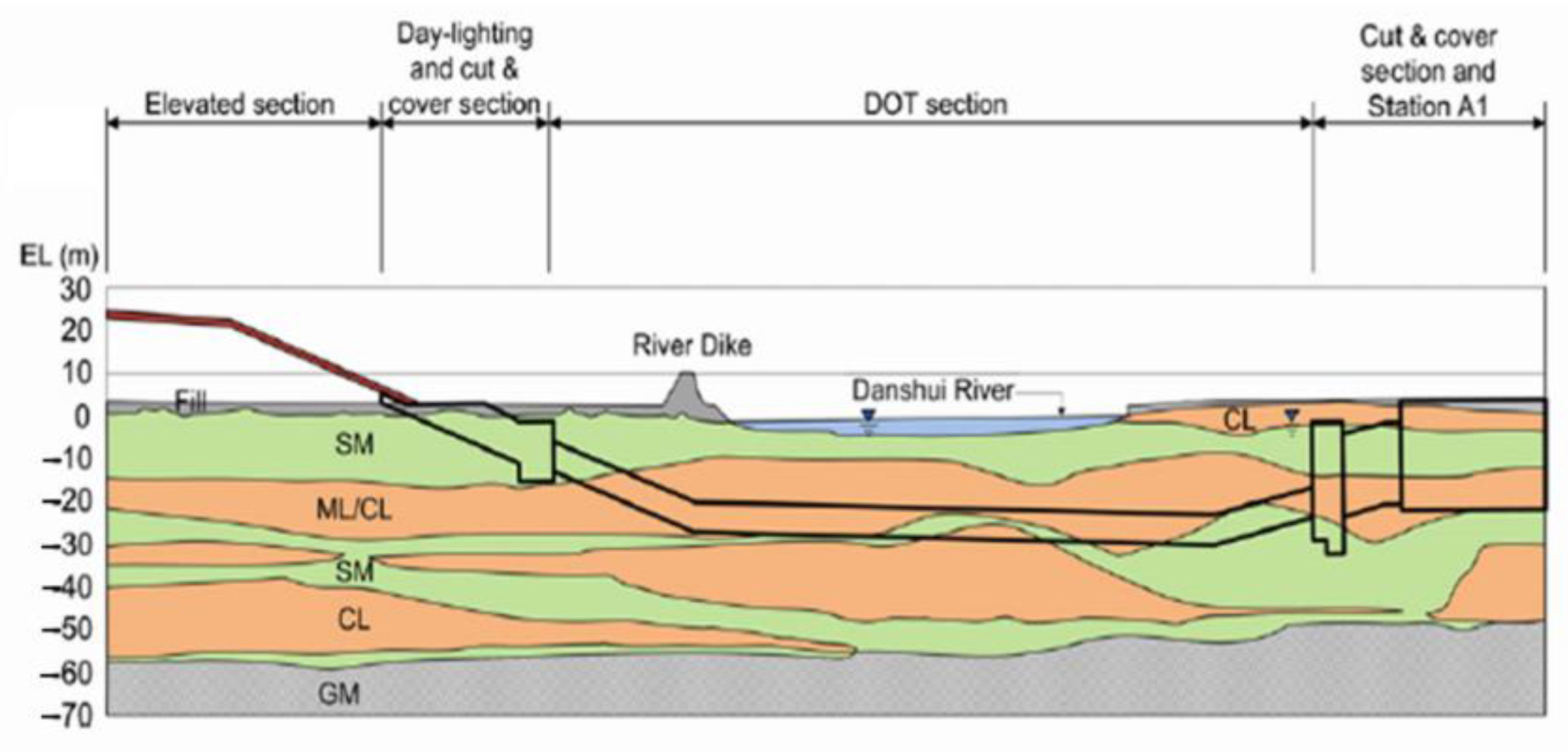
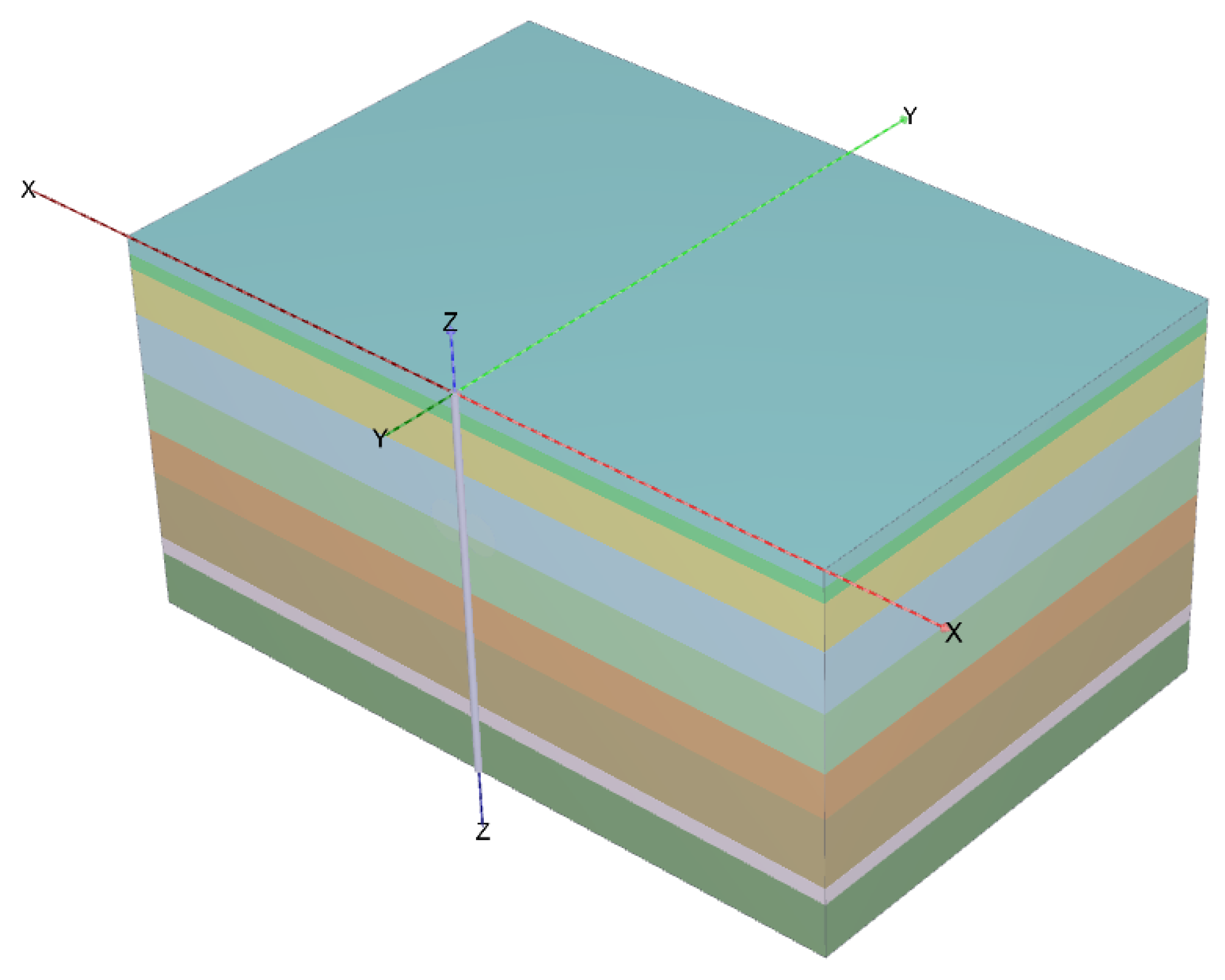
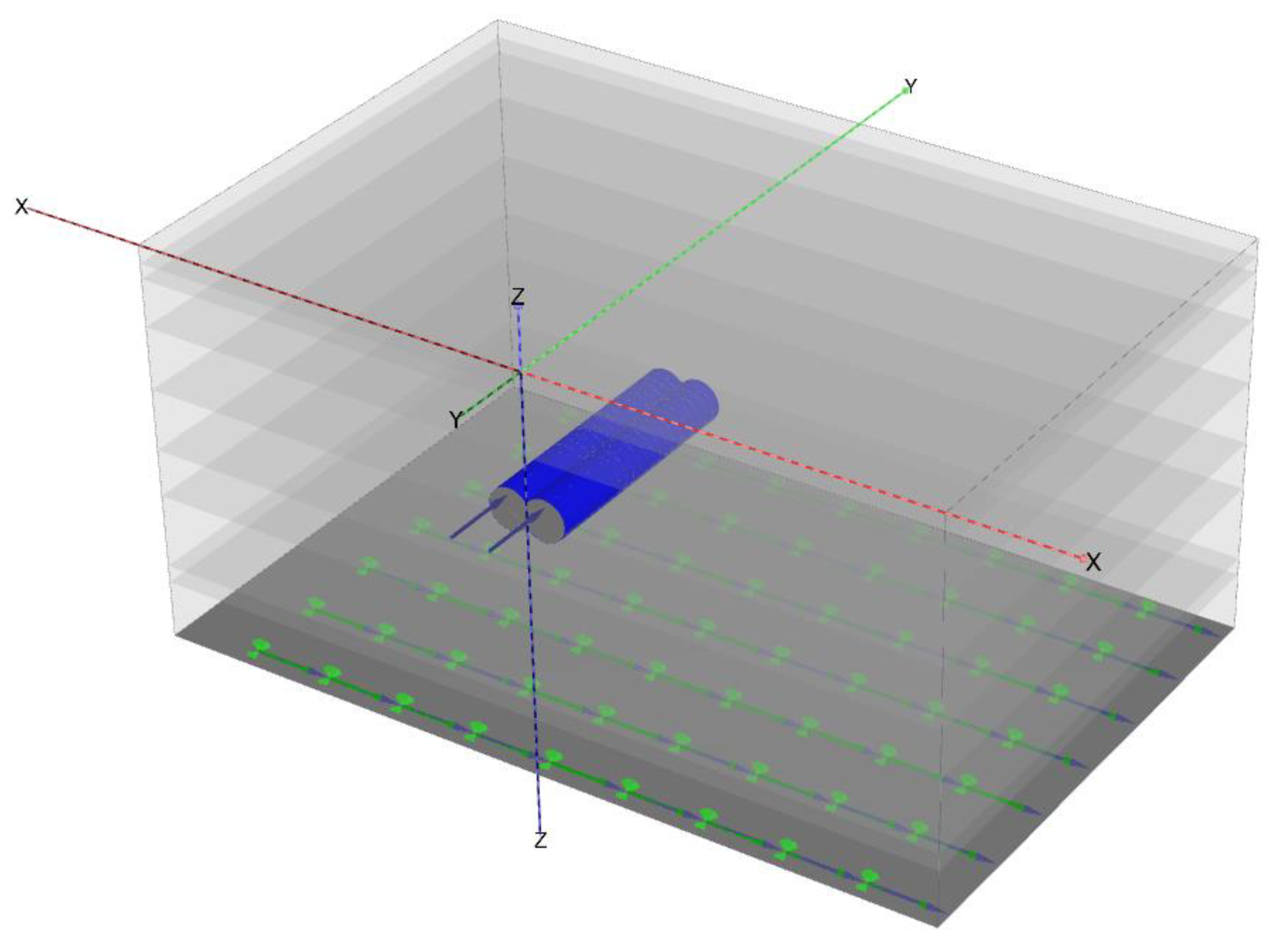
3.1. Model Setup

The model uses 10-node tetrahedral elements. Units are set as follows: meters (m) for length, kilonewtons (kN) for force, and days for time. The unit weight of water is set to 10 kN/m³. Model boundaries are defined as X-direction from −60 m to 60 m, Y-direction from 0 m to 78 m, and Z-direction (depth) from −60 m to 0 m, with the groundwater table at −3 m. The overall model size is 120 m × 78 m × 60 m, as shown in Figure 4.

The soil profile in the model is divided into nine layers as follows:

-

Layer 1: Backfill (SF), depth GL 0 to −2.7 m;

-

Layer 2: Silty Clay (CL), depth GL −2.7 to −5 m;

-

Layer 3: Silty Sand (SM), depth GL −5 to −12.1 m;

-

Layer 4: Silty Clay (CL), depth GL −12.1 to −21.5 m;

-

Layer 5: Silty Clay (CL), depth GL −21.5 to −30.7 m;

-

Layer 6: Silty Sand (SM), depth GL −30.7 to −37.8 m;

-

Layer 7: Silty Clay (CL), depth GL −37.8 to −48.8 m;

-

Layer 8: Silty Sand (SM), depth GL −48.8 to −51.4 m;

-

Layer 9: Silty Gravel (GM), depth GL −51.4 to −60 m.

The soil parameters required for the Hardening Soil model include ${\gamma }_{unsat}$, ${\gamma }_{sat}$, $c^{\prime }$, ${\phi }^{\prime }$, $E_{50}^{ref}$, $E_{oed}^{ref}$, $E_{ur}^{ref}$, ${\nu }_{ur}$, $\psi$, and m. According to the PLAXIS manual, the unloading/reloading Poisson’s ratio is set to ${\nu }_{ur}=0.2$ (the default value in the software), and the reference pressure is $P_{ref}=100\mathrm{k}\mathrm{P}\mathrm{a}$. Based on a comprehensive review of borehole reports, laboratory test data, and empirical formulas discussed in this section, the Hardening Soil model is adopted for both static and dynamic analyses in this study. Due to space limitations, these are summarized in

Table 2. Soil material parameters.

USCS	Depth (m)	${\mathit{\gamma }}_{\mathit{u}\mathit{n}\mathit{s}\mathit{a}\mathit{t}}$ ($\mathbf{k}\mathbf{N}/{\mathbf{m}}^3$)	${\mathit{\gamma }}_{\mathit{s}\mathit{a}\mathit{t}}$ ($\mathbf{k}\mathbf{N}/{\mathbf{m}}^3$)	N	Su ($\mathbf{k}\mathbf{N}/{\mathbf{m}}^2$)	${\mathit{E}}_{50}^{\mathit{r}\mathit{e}\mathit{f}}$ ($\mathbf{k}\mathbf{N}/{\mathbf{m}}^2$)	${\mathit{E}}_{\mathit{o}\mathit{e}\mathit{d}}^{\mathit{r}\mathit{e}\mathit{f}}$ ($\mathbf{k}\mathbf{N}/{\mathbf{m}}^2$)	${\mathit{E}}_{\mathit{u}\mathit{r}}^{\mathit{r}\mathit{e}\mathit{f}}$ ($\mathbf{k}\mathbf{N}/{\mathbf{m}}^2$)	${\mathit{\nu }}^{\mathbf{\prime }}$	${\mathit{v}}_{\mathit{u}}$	${\mathit{c}}^{\mathbf{\prime }}$ ($\mathbf{k}\mathbf{N}/{\mathbf{m}}^2$)	${\mathit{\phi }}^{\mathbf{\prime }}$ (°)	$\mathit{\psi }$ (°)
SF	0~−2.7	16.1	19.1	5	-	12,500	10,000	37,500	0.3	-	0.2	28	0
CL	−2.7~−5	14.8	18.5	9	14	11,200	8960	33,600	0.3	0.495	0.3	25	0
SM	−5~−12.1	15.4	18.8	10	-	25,000	20,000	75,000	0.3	-	0.3	30	0
CL	−12.1~−21.5	14.7	19	6	38	30,400	24,320	91,200	0.3	0.495	0.5	27	0
CL	−21.5~−30.7	15.4	19.3	13	63	50,400	40,320	151,200	0.3	0.495	0.3	29	0
SM	−30.7~−37.8	15	17.5	21	-	52,500	42,000	157,500	0.3	-	0.5	31	1
CL	−37.8~−48.8	14.7	19.2	22	100	80,000	64,000	240,000	0.3	0.495	0.3	31	1
SM	−48.8~−51.4	16.2	19.3	39	-	97,500	78,000	292,500	0.3	-	0.5	33	3
GM	−51.4~−60	16.9	21	50	-	125,000	100,000	375,000	0.3	-	0.1	35	5

.

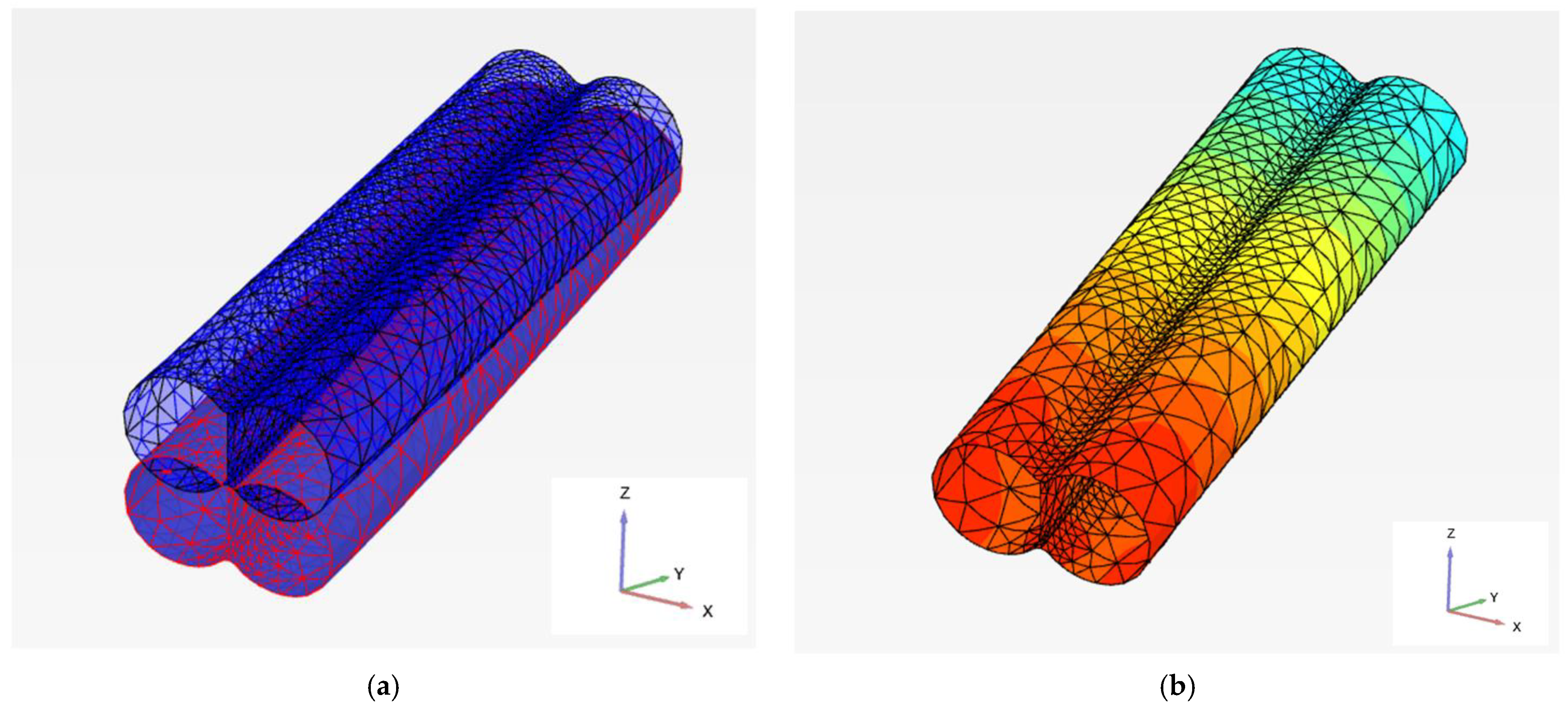
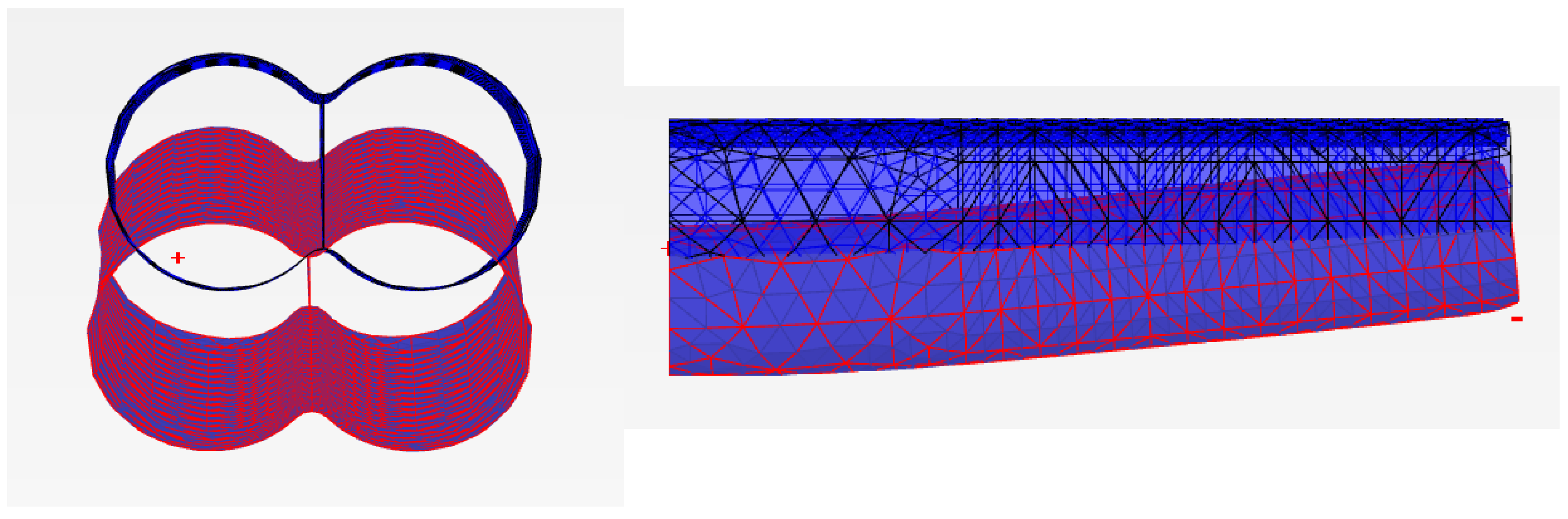
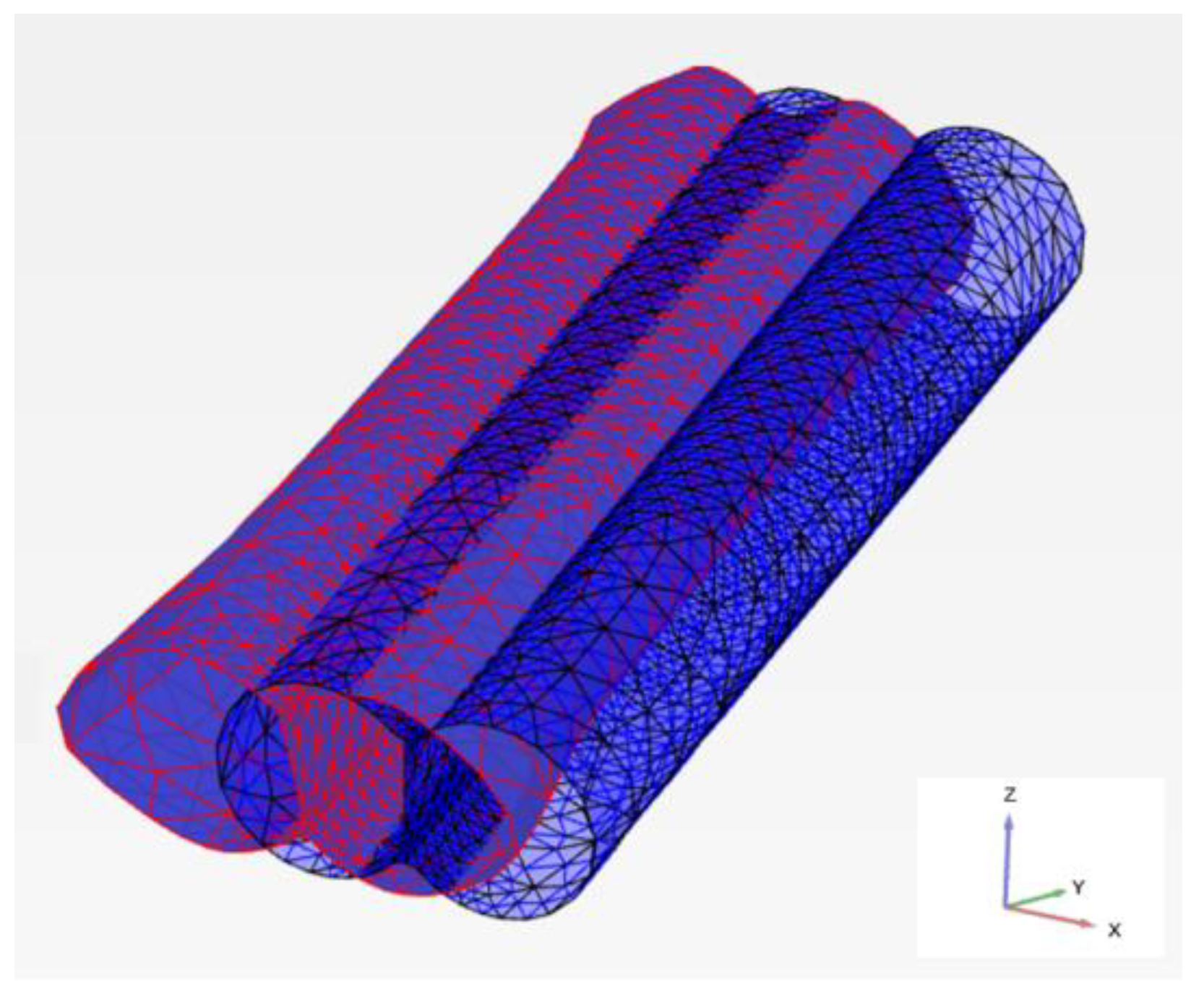
3.2. Tunnel Installation

In this study, the simulation process begins with determining the tunnel cross-sectional geometry, followed by configuring the material properties and tunnel alignment and, finally, proceeding with the tunnel excavation sequence. The tunnel lining structure is modeled using the Tunnel Designer in PLAXIS combined with plate elements. The material properties are set to concrete, as shown in Figure 5.

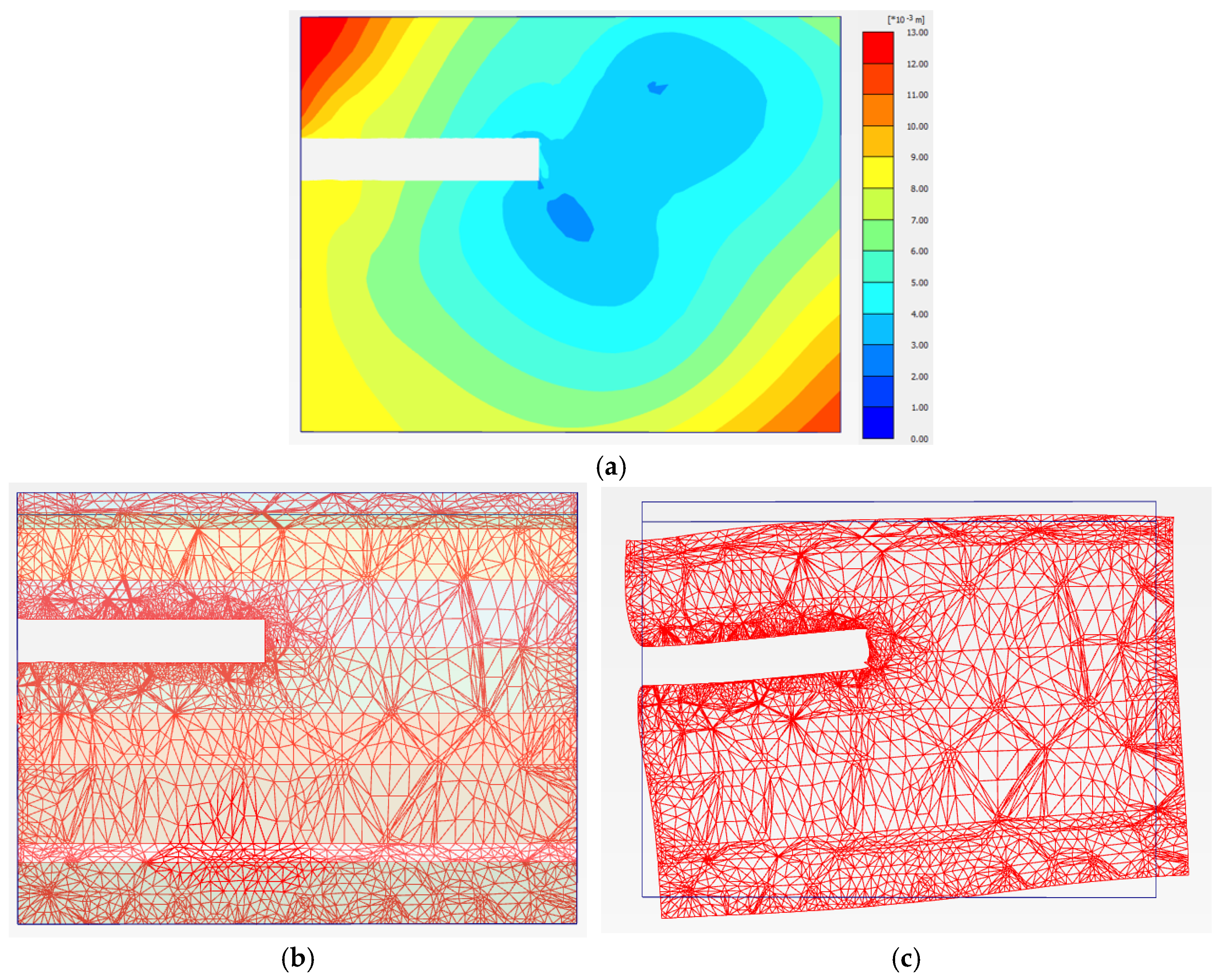
3.3. Mesh Generation

After defining the model geometry and assigning material properties to each soil layer and structural element, PLAXIS offers five built-in mesh options—Very Coarse, Coarse, Medium, Fine, and Very Fine—along with an option to apply mesh refinement for improved accuracy.

To balance computational efficiency and accuracy, this study adopts the “Medium + Refined Mesh” setting for both static and dynamic analyses. As shown in Figure 6, the generated mesh consists of 41,250 elements and 73,981 nodes.

3.4. Seepage Conditions

In PLAXIS 3D 2024 effective stress analysis, total stress consists of soil’s effective stress and pore water pressure. The pore water pressure includes static water pressure, seepage pressure, and excess pore pressure. Static and seepage pressures are typically generated based on water level differences and significantly influence soil’s mechanical behavior and stability. Excess pore pressure is induced by structural loading and occurs under undrained conditions over a short period.

If the project is not affected by groundwater, the water table condition can be omitted by setting the phreatic level at the bottom of the model. This approach reasonably simulates the static stress environment of the soil in underground engineering. In this study, based on the average groundwater level in the Taipei area, the phreatic surface is set at a depth of 3 m below ground level to reflect actual site conditions.

3.5. Dynamic Boundaries

In static analysis, boundary displacements are applied to the finite element model as needed. These boundary conditions can be fully free or fixed in one or two directions. For vertical mesh boundaries, nonphysical (synthetic) boundaries are commonly used to prevent boundary conditions from affecting structural deformations in the model.

In dynamic analysis, boundaries are placed farther from the structure than in static analysis to avoid stress wave reflections that could distort the results. However, this increases computational effort and memory usage due to the need for additional elements.

To eliminate wave reflections and suppress scattered waves, the following methods can be used to treat boundaries:

• Use of semi-infinite elements (boundary elements);
• Adjustment of boundary material properties (low stiffness and high viscosity);
• Application of viscous boundaries (dashpots);
• Use of free-field and compliant boundaries (boundary elements).

When using viscous boundaries, the model employs dashpots instead of fixed constraints in specific directions. This means that, when earthquake-induced motion occurs at the boundary, the dashpots can effectively absorb the additional stresses, preventing rebound effects. In PLAXIS, the implementation of viscous boundaries is based on the theory proposed by Lysmer and Kuhlmeyer.

When free-field boundaries are selected, wave reflections can occur at the far-end boundaries during wave propagation simulation, potentially affecting the model. To address this, free-field elements can be added on both sides of the model, with properties similar to those of the adjacent soil layers. These elements can receive equivalent normal and shear forces from the main domain, effectively simulating wave propagation in real ground conditions.

To efficiently absorb stress waves from internal structures, two dashpots are typically added at each node along the lateral boundaries. These dashpots help dissipate the energy of the propagating waves, preventing reflections from interfering with the model. In the model setup, if one vertical boundary is set as a free-field boundary, the opposite vertical boundary must also be set as a free-field boundary to ensure consistency and effectiveness of the boundary conditions.

This type of boundary condition is commonly used in seismic analysis, especially when dynamic loading is applied along the bottom boundary of the model, as it effectively simulates the propagation and reflection of seismic waves in the soil.

In the dynamic analysis of this study, the fourth method is adopted. Since the dynamic analysis is performed in three directions simultaneously, the boundaries at $X_{max}$, $X_{min},Y_{max},\mathrm{a}\mathrm{n}\mathrm{d}{Y}_{min}$ are set as free-field, while$Z_{min}$ is defined as a compliant base. $Z_{max}$ is left undefined. For surface displacements, the X and Y directions are set as prescribed and the Z direction is fixed.

3.6. Damping

Under dynamic loading, the fundamental equation governing time-dependent volumetric motion is:

$$M\ddot{u}+C\dot{u}+Ku=F$$

(1)

where:

−

M is the mass matrix;

−

C is the damping matrix;

−

K is the stiffness matrix;

−

F is the load vector;

−

$\ddot{u}$ is acceleration;

−

$\dot{u}$ is velocity;

−

u is displacement.

In PLAXIS 3D 2024, the matrix M represents the total mass of materials (e.g., soil, water, or structures), reflecting their inertial behavior during vibration. The matrix C represents damping, which is influenced by irreversible processes such as friction, plasticity, or viscous deformation. Higher damping typically leads to greater energy dissipation during shaking, significantly affecting the dynamic response of structures.

The damping matrix C is usually defined as a function of the mass and stiffness matrices, commonly referred to as Rayleigh damping, with the formula:

$$C={\alpha }_{R}M+{\beta }_{R}K$$

(2)

This formulation constrains damping based on Rayleigh damping coefficients. When ${\alpha }_R>{\beta }_R$, low-frequency vibrations are more effectively attenuated; conversely, when ${\alpha }_R<{\beta }_R$, high-frequency vibrations are more suppressed.

This setting is based on the findings of Benjamin [19], who reported a strong correlation between Cumulative Absolute Velocity (CAV) and structural damage. They noted that a CAV of 0.30 g-sec (filtered above 10 Hz) corresponds to the lower bound of Modified Mercalli Intensity (MMI) VII shaking. This reference has been incorporated into the main text. Additionally, according to the seismic tunnel analysis experience shared by Dr. Lee, a retired expert from CECI Engineering Consultants, damping coefficients α and β are mainly applied to the soil. A damping ratio of at least 12% is recommended to reduce earthquake-induced dynamic responses, with a typical design frequency of 10 Hz. In practice, α is set to zero (external damping not considered) and β is calculated as β = damping ratio/(π × frequency). Thus, β is set to 0.00382 in the model. No damping matrix is applied to the structural components.

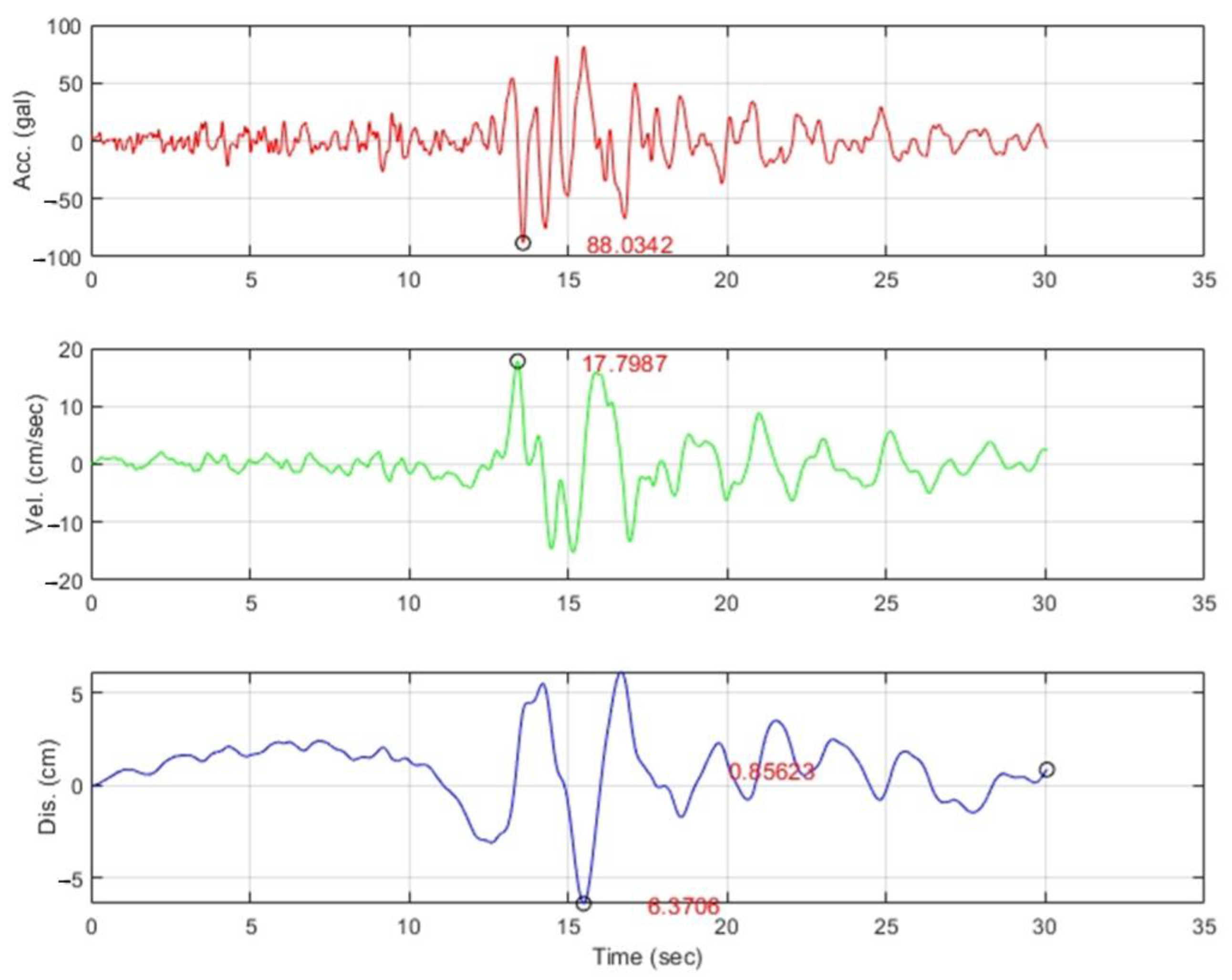
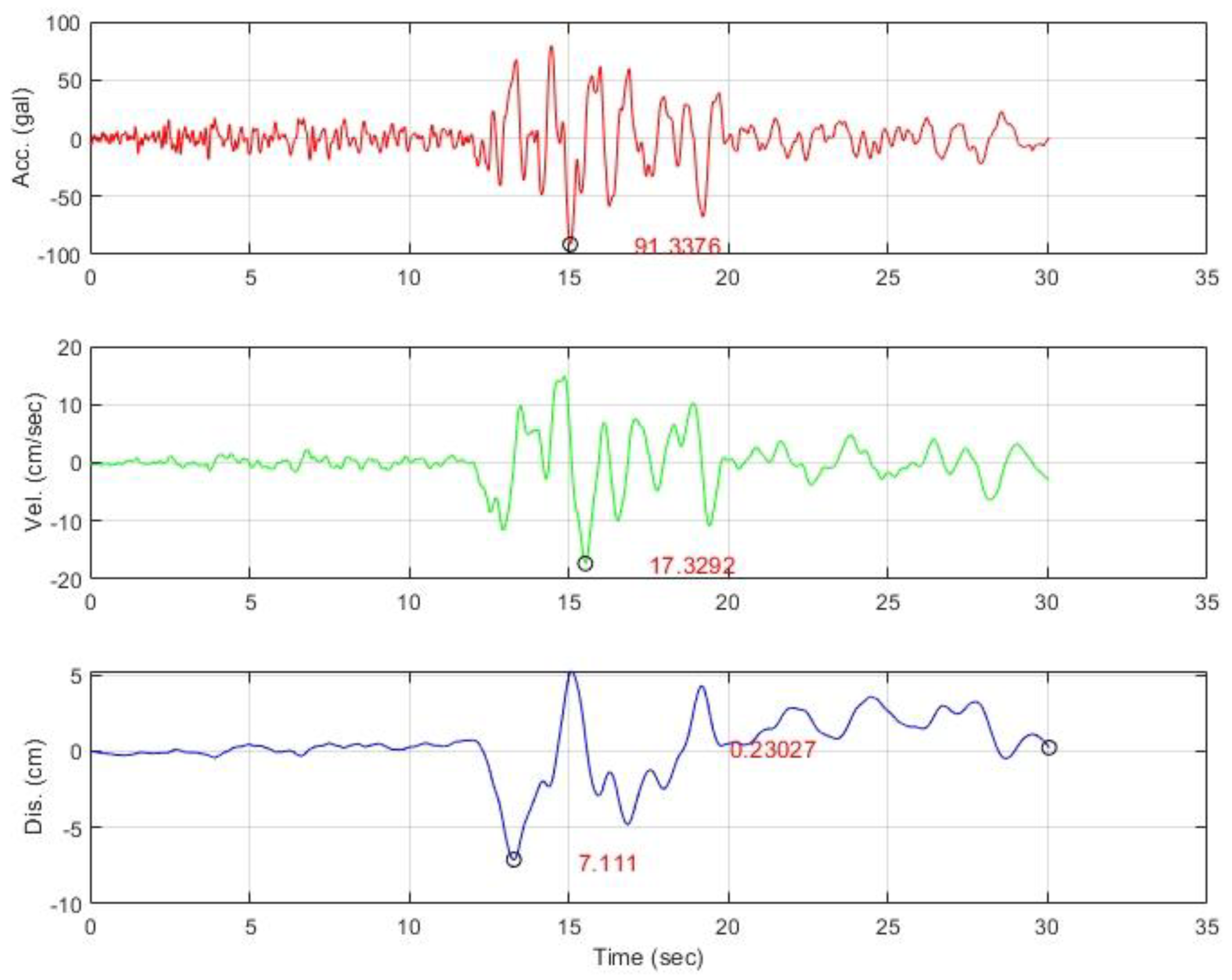
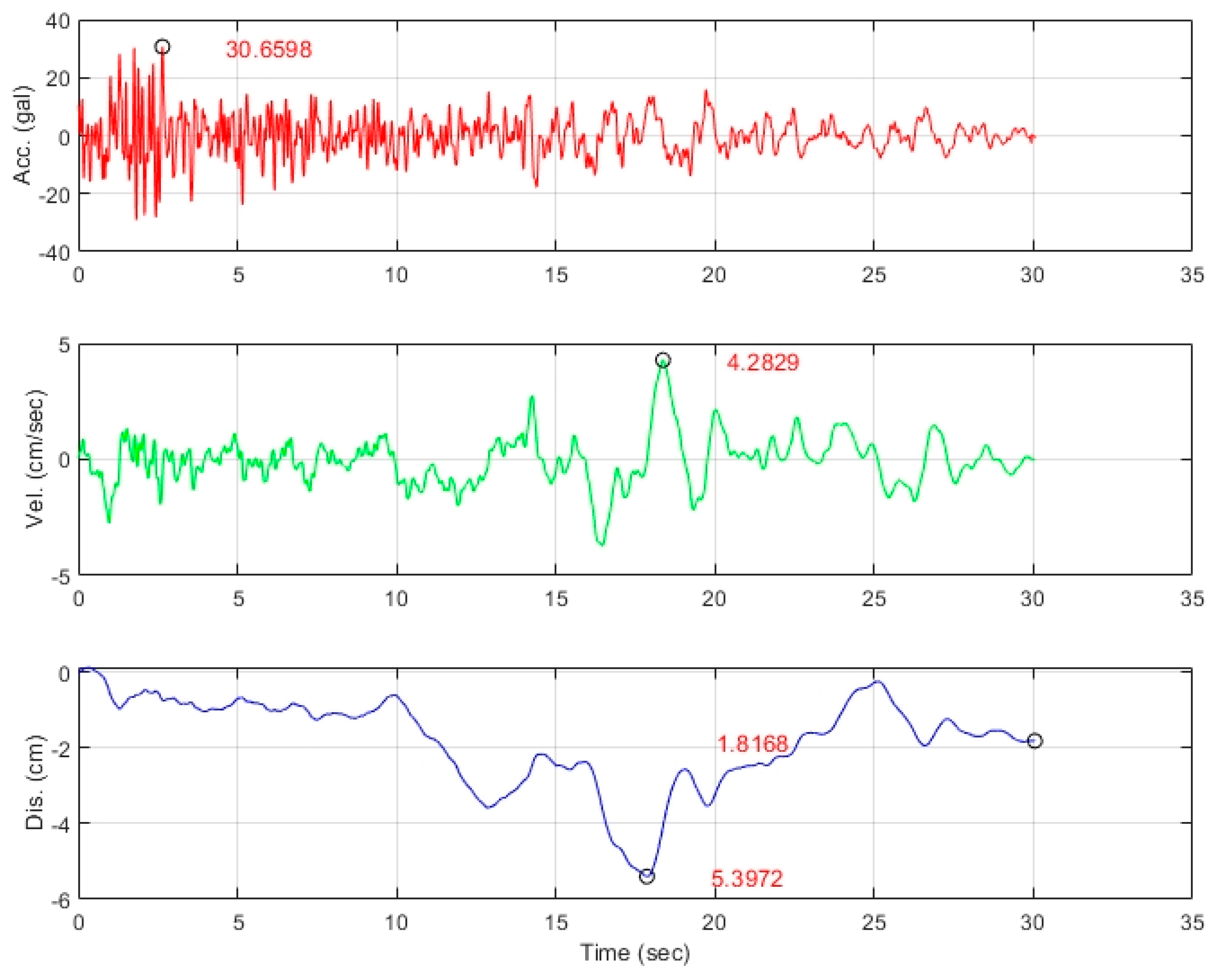
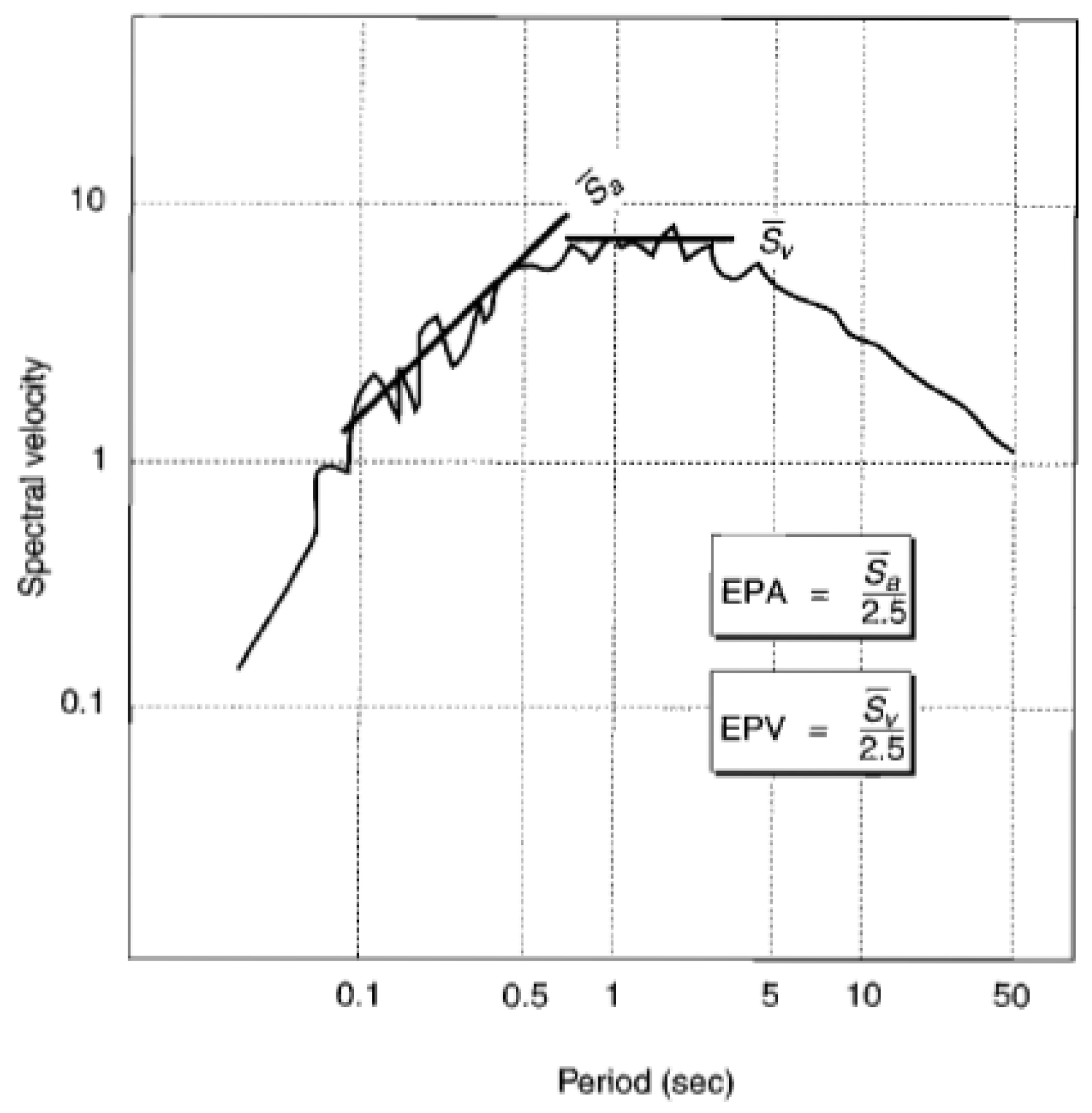
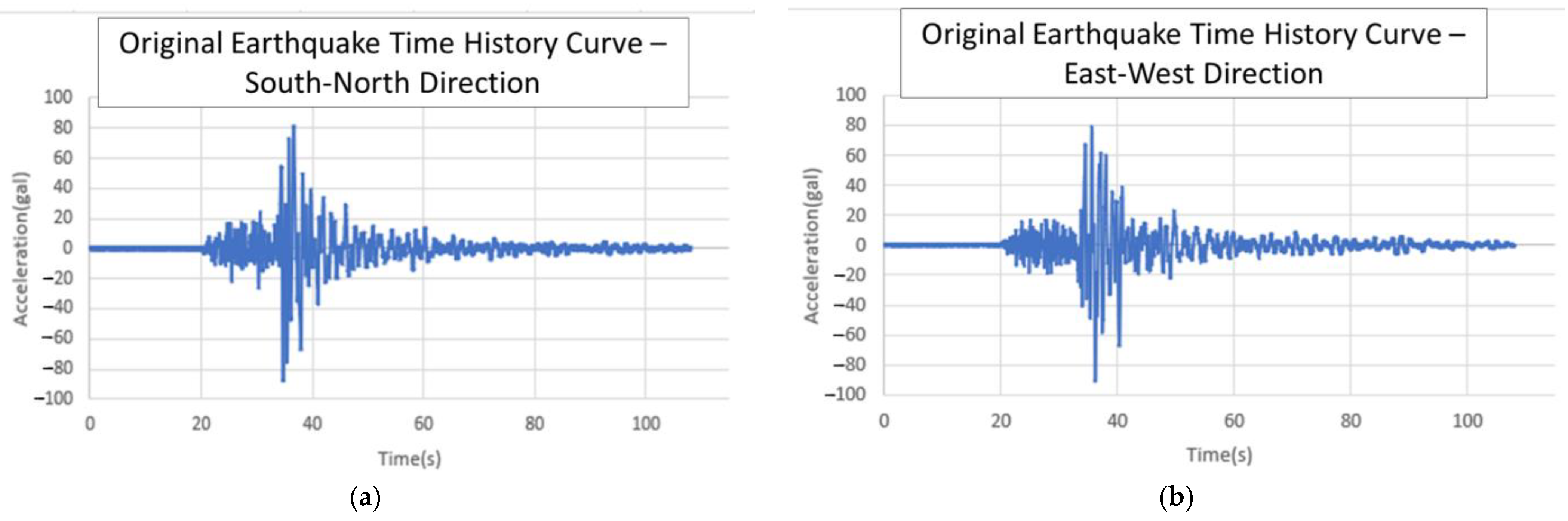
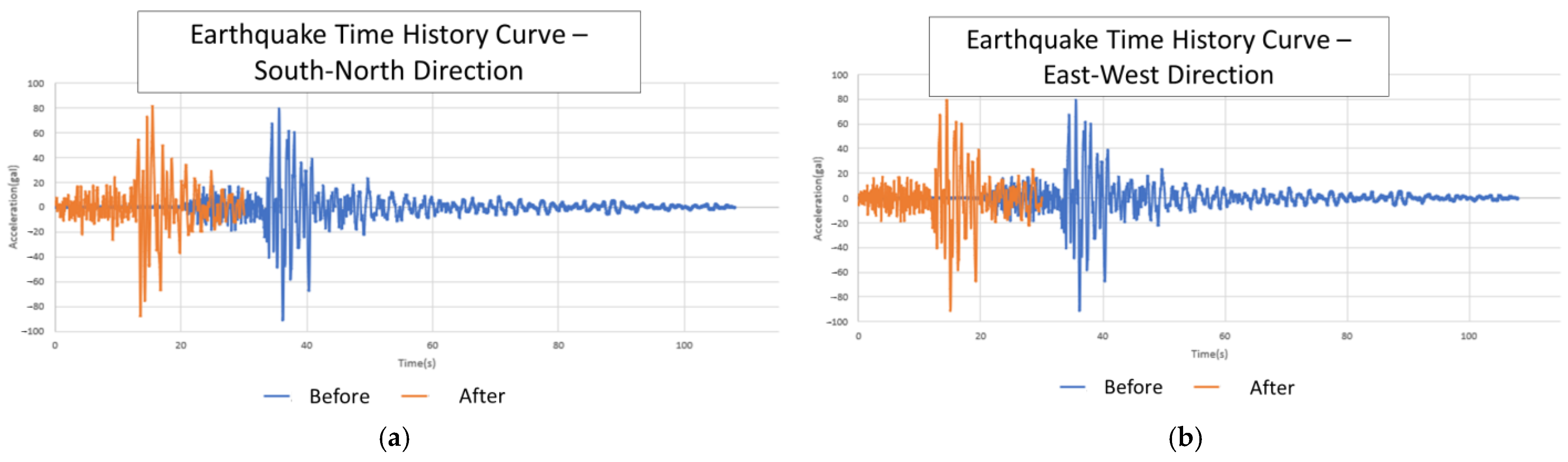
3.7. Earthquake Data

The earthquake used in this study is one of the most significant seismic events to have affected northern Taiwan in recent years. It occurred with a moment magnitude of 6.8 and is commonly referred to as the “331 Earthquake”. The seismic data were obtained from the TAP012 Rixin Elementary School station, which is the closest station to the project site. The data source is the Seismological Center of the Central Weather Administration (CWA).

According to the latest intensity classification system by the CWA, the northern region experienced an intensity level of 5− (weak). The original earthquake record lasted 107.995 s, with the peak ground acceleration (PGA) of 0.083 g occurring at 36.63 s. The acceleration time history is divided into north–south and east–west components. Before conducting dynamic analysis, the original ground motion records must be adjusted based on the study requirements, as shown in Figure 7a,b.

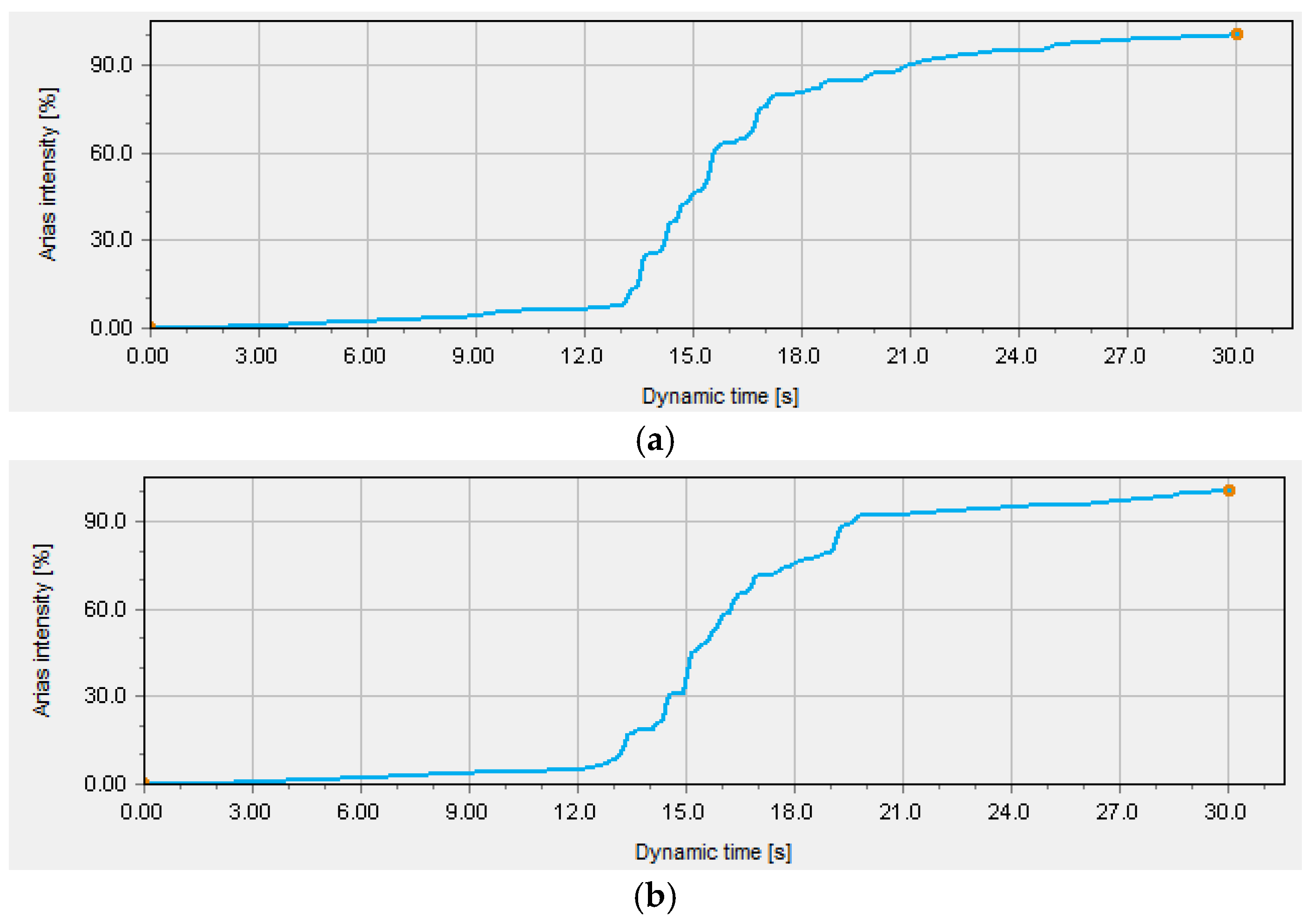
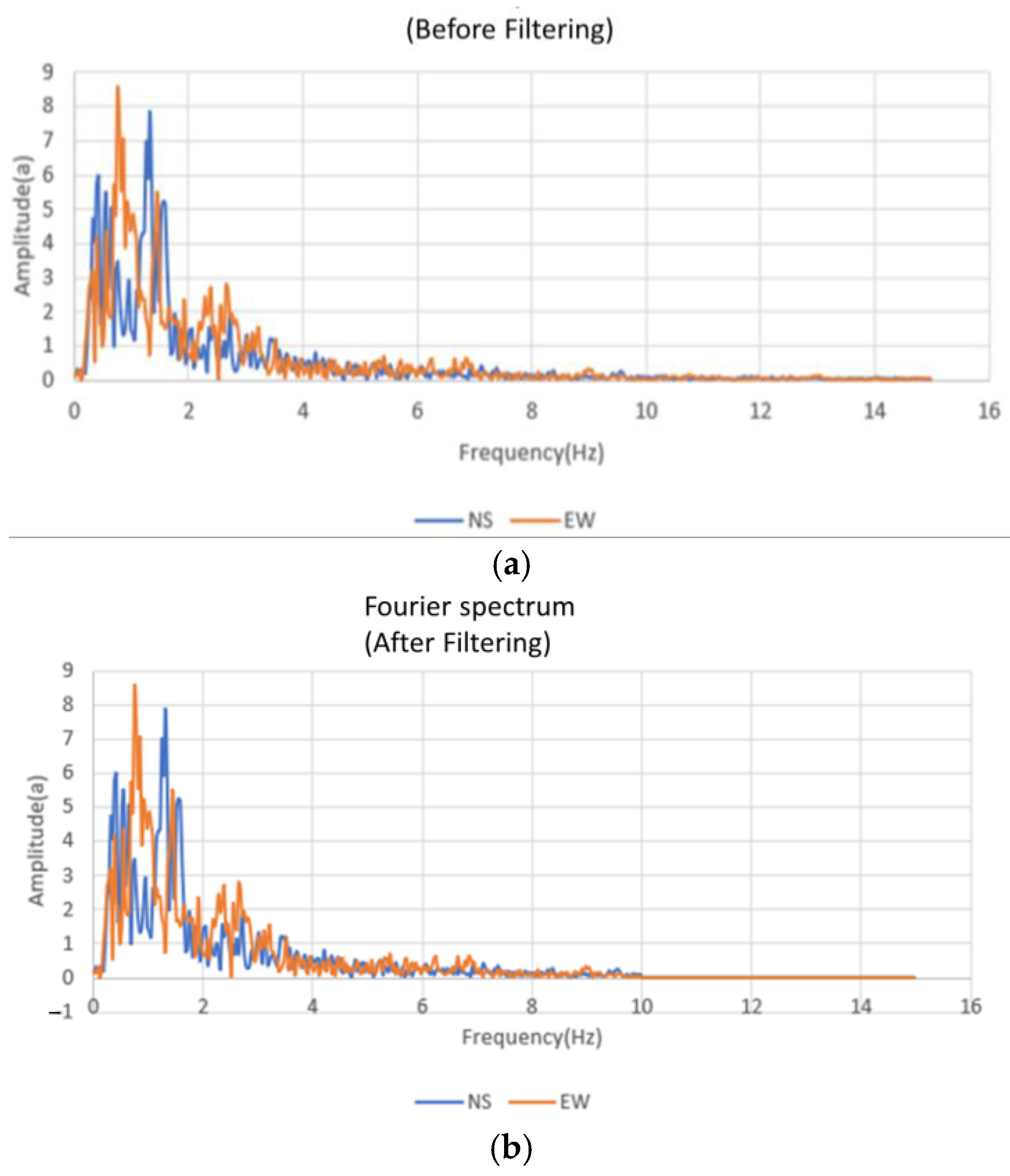
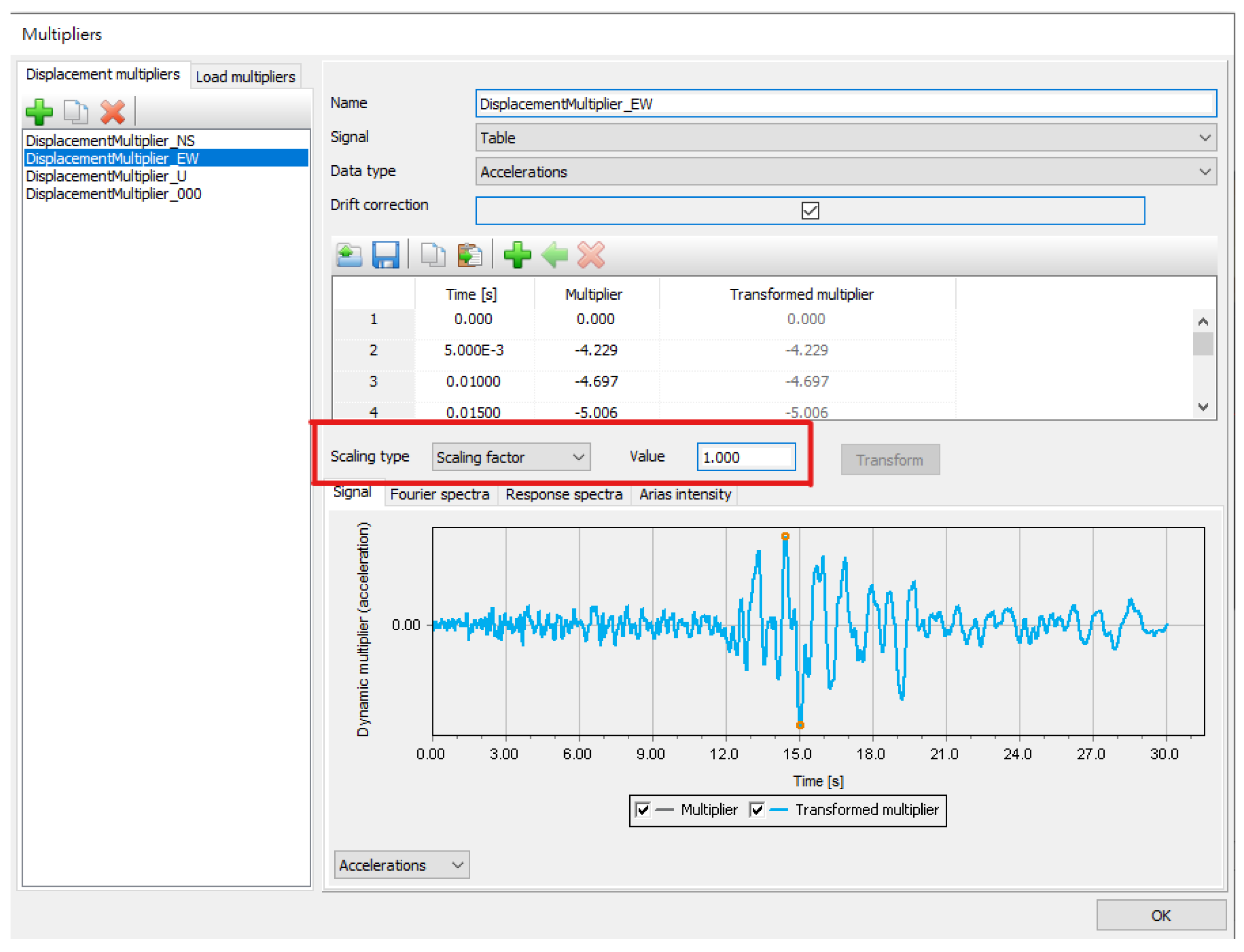
3.8. Ground Motion Scaling

The original earthquake time history is 107.995 s. Considering that dynamic analysis is highly time-consuming and that the vibration amplitudes at the beginning and end of the record are nearly zero, with most of the seismic energy concentrated in the middle portion, the record was processed accordingly. The time history was imported into PLAXIS, and the built-in Arias intensity conversion function was used, as shown in Figure 8a,b.

Based on the study by Trifunac and Brady (1975b) [4], the first and last 5% of the seismic energy were excluded, retaining the portion containing 90% of the total energy. As a result, the corrected earthquake duration is approximately 30.04 s. This adjustment significantly reduces the computational time required for dynamic analysis. The time histories before and after adjustment are shown in Figure 9a,b.

The Fourier spectrum reveals that the complete earthquake time history consists of amplitudes across a wide range of frequencies. If the unfiltered time history is directly input into the model, high-frequency signal components will also be introduced, which may lead to numerical instability and significant noise during analysis.

From the Fourier spectrum, it can be observed that the majority of seismic energy is concentrated in the low-frequency range. Therefore, a cutoff frequency of 10 Hz was selected, and MATLAB 2024 was used to filter out frequency components above 10 Hz. The results are shown in Figure 10a,b.

3.9. Seismic Load Amplification

The earthquake used in this study was recorded at the TAP012 Rixin Elementary School station, with a peak ground acceleration of 87.972 gal (approximately 0.08 g), corresponding to Intensity 5− based on Taiwan’s CWA classification.

To examine the tunnel’s displacement and mechanical response under varying seismic intensities, the original ground motion was scaled using PLAXIS’s built-in scaling factor, as shown in Figure 11. Only the amplitude was adjusted, without modifying the time duration.

Scaling factors were based on the minimum values defined by the CWA: 1.75× (Intensity 5+), 3.125× (Intensity 6−), 5.5× (Intensity 6+), and 10× (Intensity 7). Each scaled input was used for analysis, followed by data comparison and interpretation.

3.10. Baseline Correction

Baseline correction refers to the process of adjusting parts of the earthquake time history that do not return to the original baseline. The purpose is to eliminate residual responses in the model and ensure the accuracy and reliability of the data. This correction typically involves adding low-frequency components or adjusting the baseline to remove drift, which becomes especially significant when acceleration data are integrated to obtain velocity and displacement. Without correction, even small offsets can be amplified during integration. By applying baseline correction, the seismic data can more accurately reflect real ground motion, as illustrated in Figure A1, Figure A2 and Figure A3.

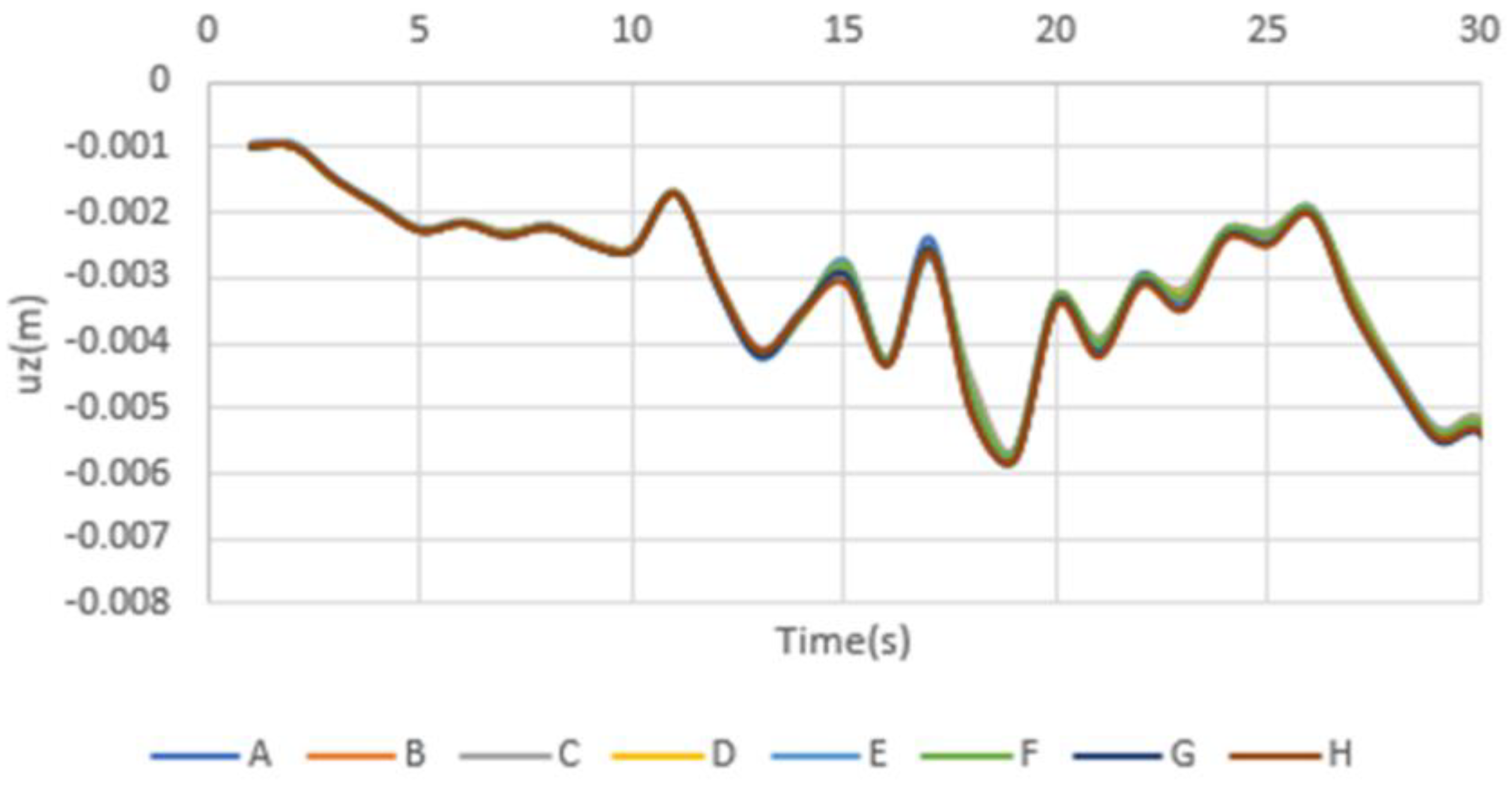
3.11. Calculation and Output Results

The process begins by calculating the initial stress and strain states. Before starting Phase 1, the program automatically sets “Reset displacements to zero”, which means any permanent deformation before excavation is set to zero. The analysis then proceeds according to the predefined construction stages.

Once the calculation is completed, results can be viewed using the Table function in PLAXIS 3D OUTPUT, including displacement, stress distribution, and more. The output is displayed in the form of contour plots or vector diagrams. Numerical results are presented in tables and exported to Excel for further organization and tabulation. The Curves Manager in PLAXIS 3D OUTPUT can also be used to plot displacement, axial force changes, and other data at structural elements and observation points for comparison.

Finally, the analysis results are compiled into tables, from which related data are extracted and reorganized for further interpretation and analysis.

4. Discussion

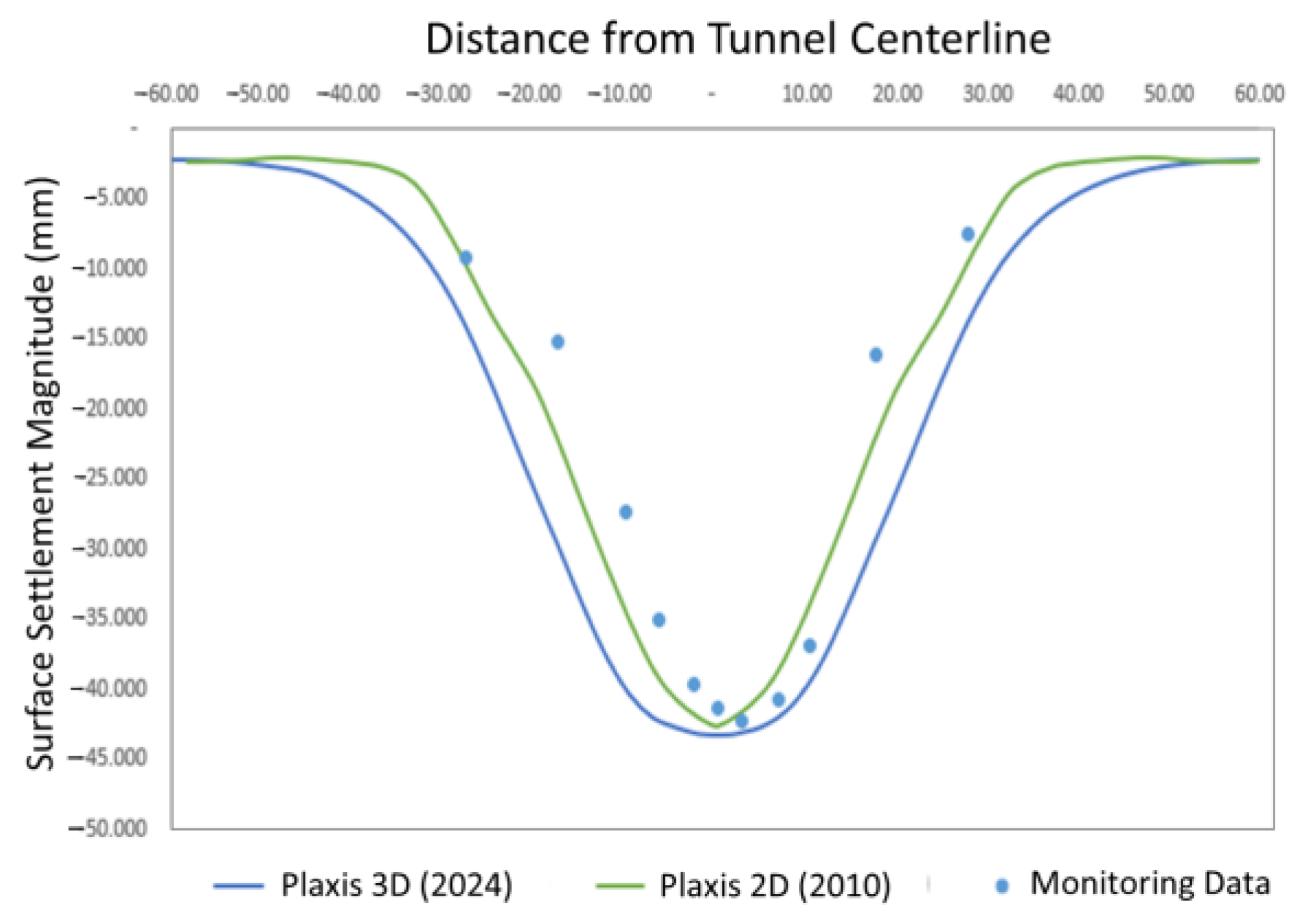
4.1. Influence of Tunnel Excavation on Surface Settlement

Tunnel excavation can cause deformation in surrounding structures, influenced by factors such as geological conditions, tunnel geometry, in situ and geostress, excavation method, support type, and construction sequence.

A previous study (Ke [1]) analyzed surface settlement due to tunnel excavation using two empirical methods (superposition and equal area methods) and compared PLAXIS 2D results with field monitoring data. Building on this, the present study investigates the impact of tunnel excavation on surface settlement under static conditions using PLAXIS 3D 2024. The tunnel depth, soil, and tunnel parameters follow those used in previous research. Results are compared with field measurements and past studies.

(1)

Causes of Surface Settlement Induced by Tunnel Excavation

As tunnel excavation progresses, the shield machine disturbs the surrounding soil, causing a release of stress from its original equilibrium state and altering the stress distribution. This change leads to ground displacement and surface settlement.

In this study, the volume loss around the shield tunnel during excavation is simulated by applying a cross-sectional contraction rate. This volume loss is commonly referred to as the “soil loss rate” in empirical methods and the literature. According to Peck’s (1969) settlement trough concept, the soil loss rate represents the volume of the surface settlement trough. It is defined as the percentage of the settlement trough area per unit length relative to the tunnel cross-sectional area. In this study, a section contraction ratio of 1.5% was adopted to simulate the settlement induced by tunnel excavation, based on the prior analysis and field experience reported by Ke [1].

(2)

Comparison of Surface Settlement Results Induced by Tunnel Excavation

This study compares its surface settlement results with those analyzed by Ke [1]. As shown in Figure 12, which illustrates the comparison of settlement troughs, the predicted settlement trough is wider than that observed in monitoring data. This may be due to an overestimation of the cross-sectional reduction rate, leading to greater simulated soil volume loss. However, the maximum surface settlement is closely aligned with both the monitoring data and Ke’s analysis, with the maximum settlement directly above the tunnel being approximately 40 mm.

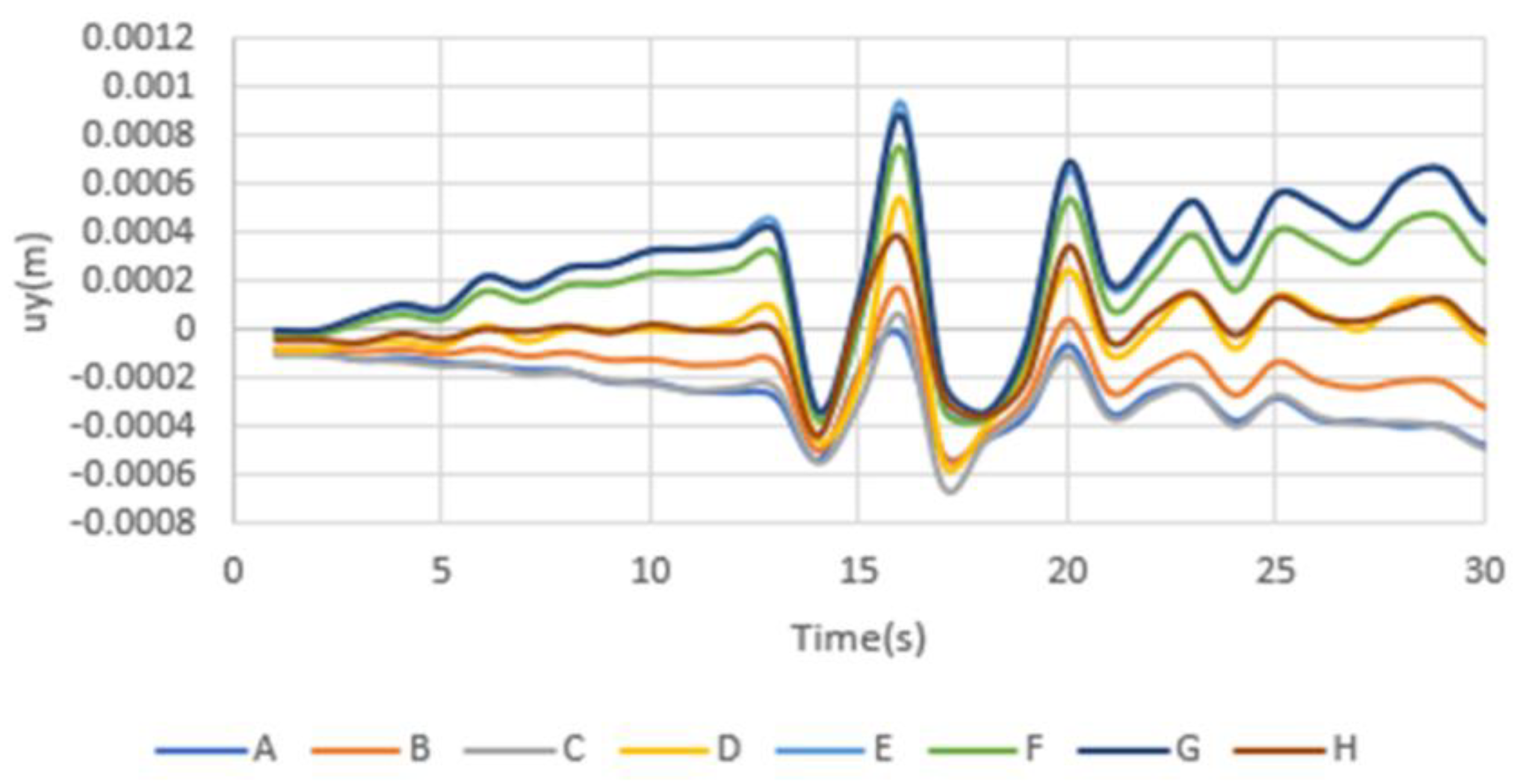
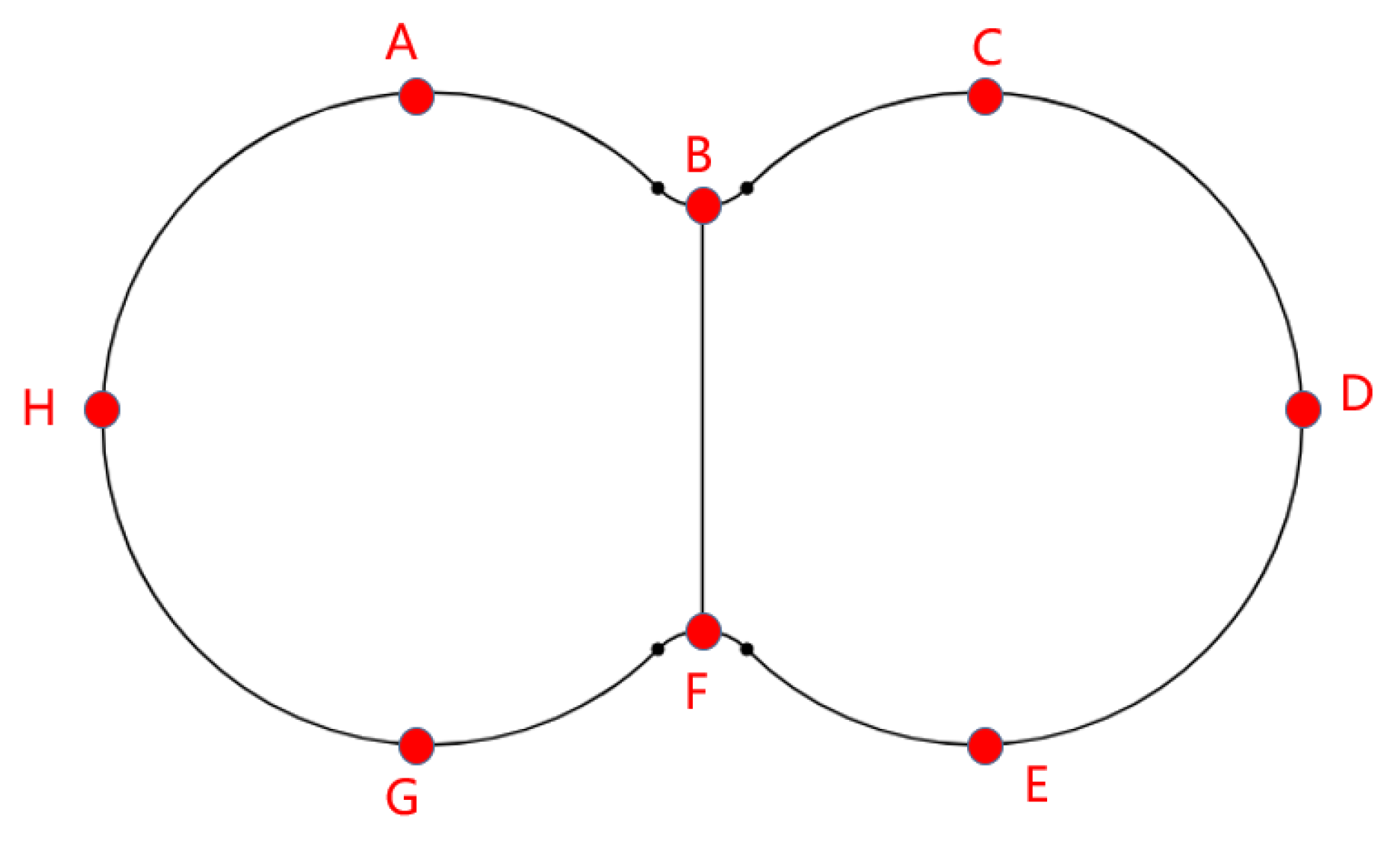
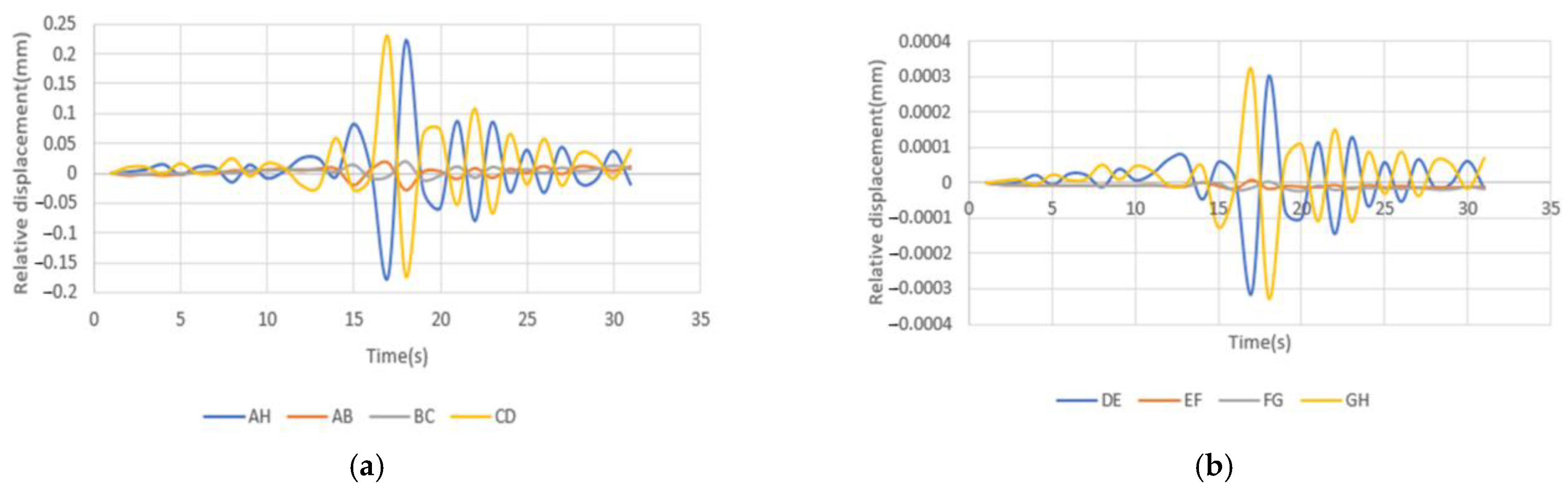
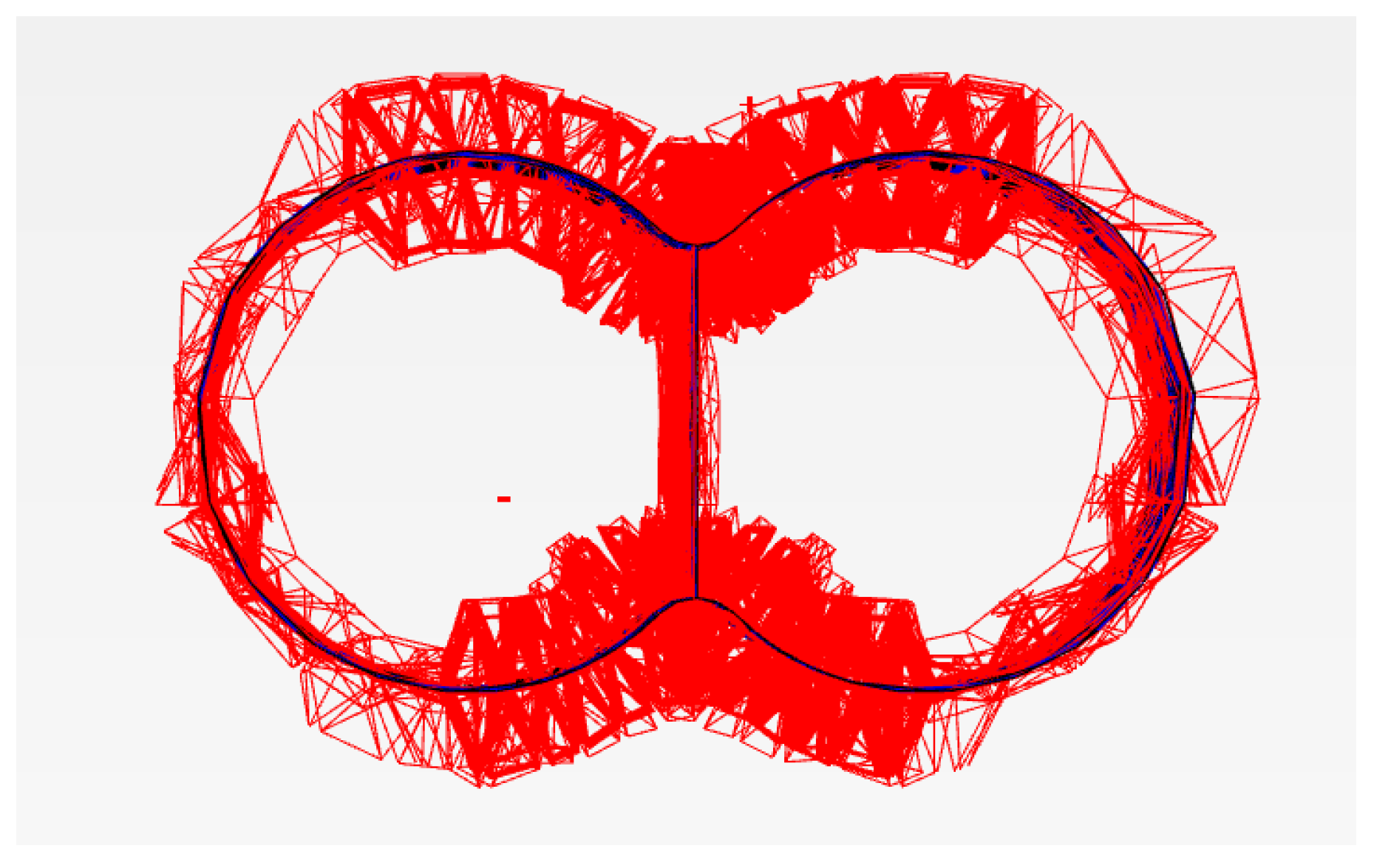
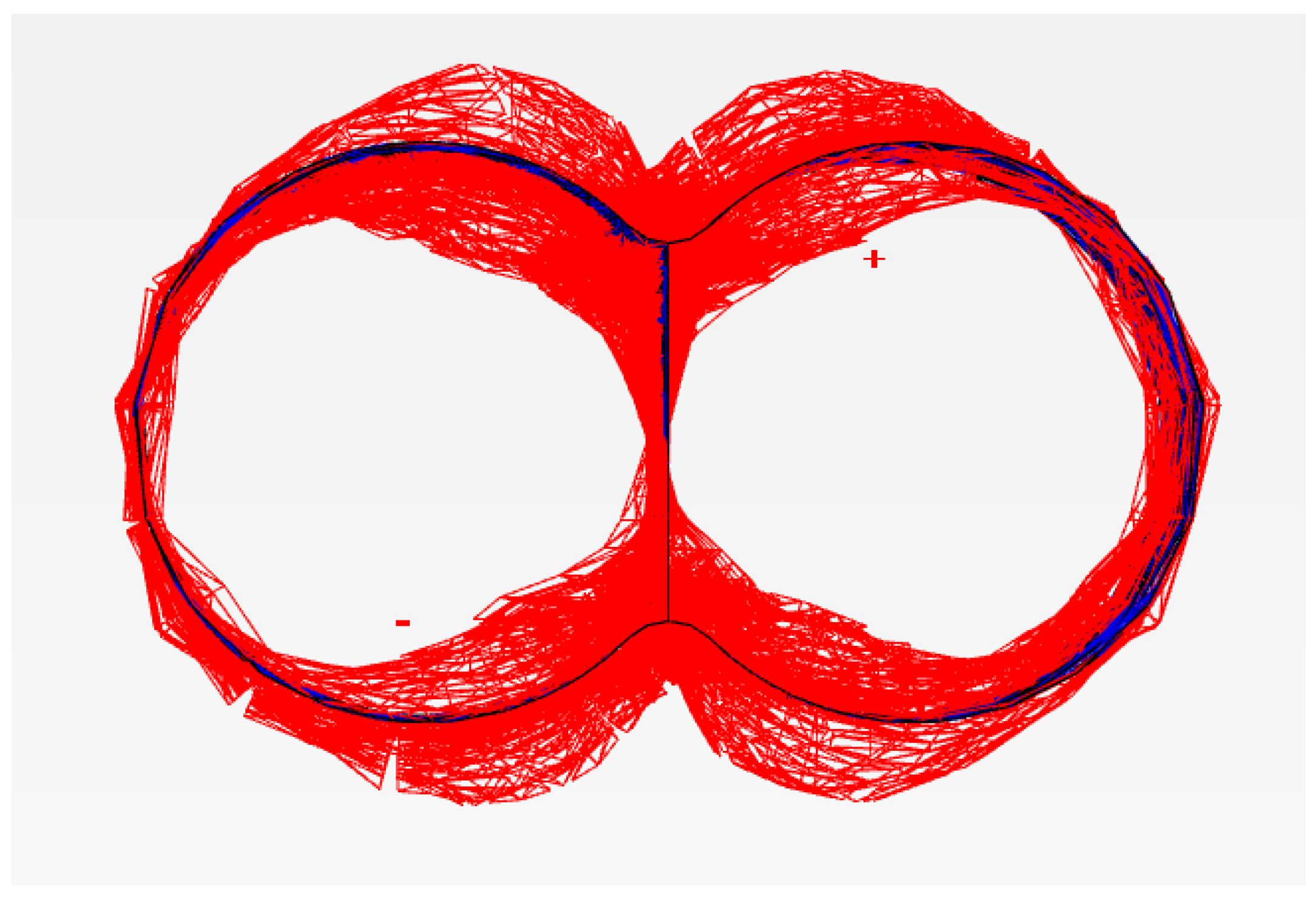
4.2. Displacement Analysis of Tunnel Under Seismic Loading

The earthquake records used in this study include north–south, east–west, and vertical components. In this section, seismic forces from all three directions are applied to analyze the tunnel cross-section. Eight monitoring points are placed around the tunnel perimeter to assess the displacement caused by seismic loading on various parts of the tunnel.

(1)

Soil Deformation

Following the same directional approach as the previous section, the model is analyzed through both longitudinal and cross-sectional views to examine soil deformation.

As shown in Figure 13a–c, the cross-sectional views of the tunnel indicate greater deformation in the soil above and on both sides of the tunnel, with a maximum displacement of approximately 2.2 cm. In the longitudinal views, shown in Figure 14a–c, significant deformation is observed in the soil above the front end of the tunnel, with a maximum value of 1.28 cm.

(2)

Tunnel Cross-Section Analysis

Eight monitoring points were placed around the tunnel cross-section, as shown in Figure 15. The co-ordinates of each point within the model are listed in

Table A1. Co-ordinates of monitoring points (unit: m).

	X	Y	Z
A	−2.7	17.25	−17.49
B	0	17	−18.57
C	2.7	17.25	−17.49
D	5.7	17.25	−20.26
E	2.47	16.84	−23.68
F	0	17	−22.6
G	−2.47	16.84	−23.68
H	−5.7	17.25	−20.26

. Points A and C are located at the tunnel crown, E and G at the invert, D and H at the sidewalls, and B and F at the top and bottom of the central column. To minimize boundary effects and prevent distortion of results, the analyzed section corresponds to the midpoint of the tunnel length. The analysis was conducted along each principal axis. From Figure 16, it can be observed that the maximum deformation in the X-direction occurs at around 17 s during the earthquake, reaching approximately 1 cm, with the tunnel cross-section shifting southward. In the later stages of the earthquake, displacement at each monitoring point shows convergence, approaching zero. The X-direction displacement after the earthquake is shown in Figure 17.

The Y-axis displacement due to the earthquake is shown in Figure A4. The tunnel can be divided into two parts: the upper portion (A, B, and C) experiences compression and shifts westward (shortening), reaching a maximum of ~0.075 cm at 15 s; the lower portion (E, F, and G) is under tension and shifts eastward (elongation), with a maximum of ~0.1 cm. Post-earthquake Y-axis displacement is shown in Figure A5.

Figure A6 shows uniform Z-axis tilting across the tunnel cross-section, with a maximum displacement of ~0.7 cm occurring after the earthquake. Post-seismic Z-axis displacement and contour/mesh diagrams are shown in Figure A7 and Figure A8.

This section uses the relative displacement (RD) method to analyze tunnel cross-section deformation during the earthquake. Four measurement lines were set on both the upper (AH, AB, BC, and CD) and lower (DE, EF, FG, and GH) parts of the tunnel. Figure 18a,b show that RD values in the upper structure are significantly larger, indicating greater relative movement compared to the lower part. The upper structure reached maximum RD values of +0.23 mm (CD) and −0.17 mm (AH) at 17 s, while the lower part peaked at ±0.00032 mm (GH). Ideally, RD values should return to zero post-earthquake but residual values remain, suggesting permanent deformation or residual strain in the tunnel.

This section also analyzes the RD values along the diagonal lines AE and CG to better understand the overall displacement direction of the tunnel cross-section during the earthquake. As shown in Figure 19a,b, the maximum diagonal RD values occurred at 13 s with +0.000141 mm (AE) and −0.00011 mm (CG) and at 17 s with +0.0008 mm (AE) and −0.0007 mm (CG), both recorded at 16 s. The results indicate that both AE and CG directions experienced repeated tensile-compressive cyclic stresses during the seismic event.

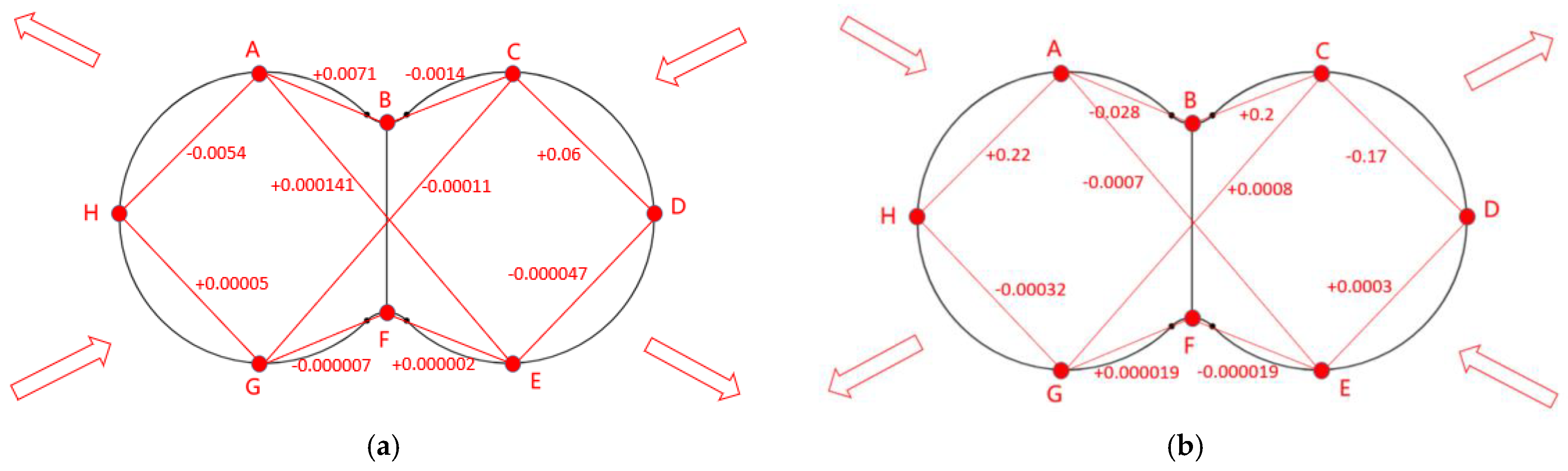
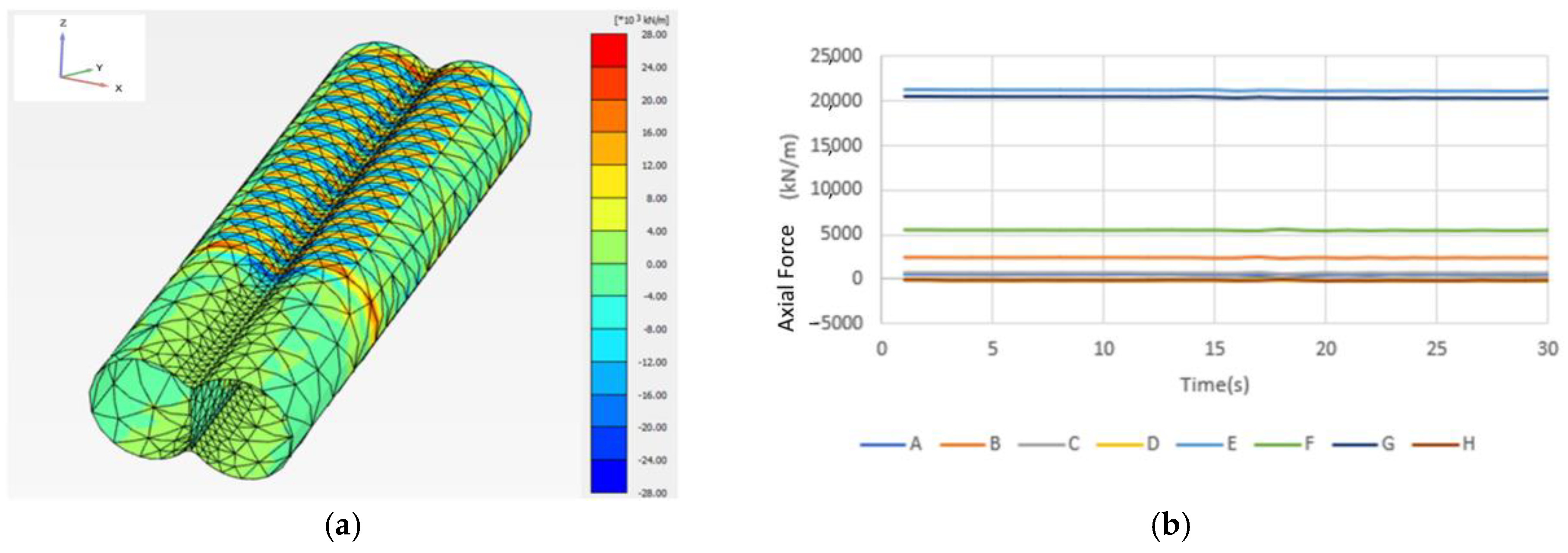
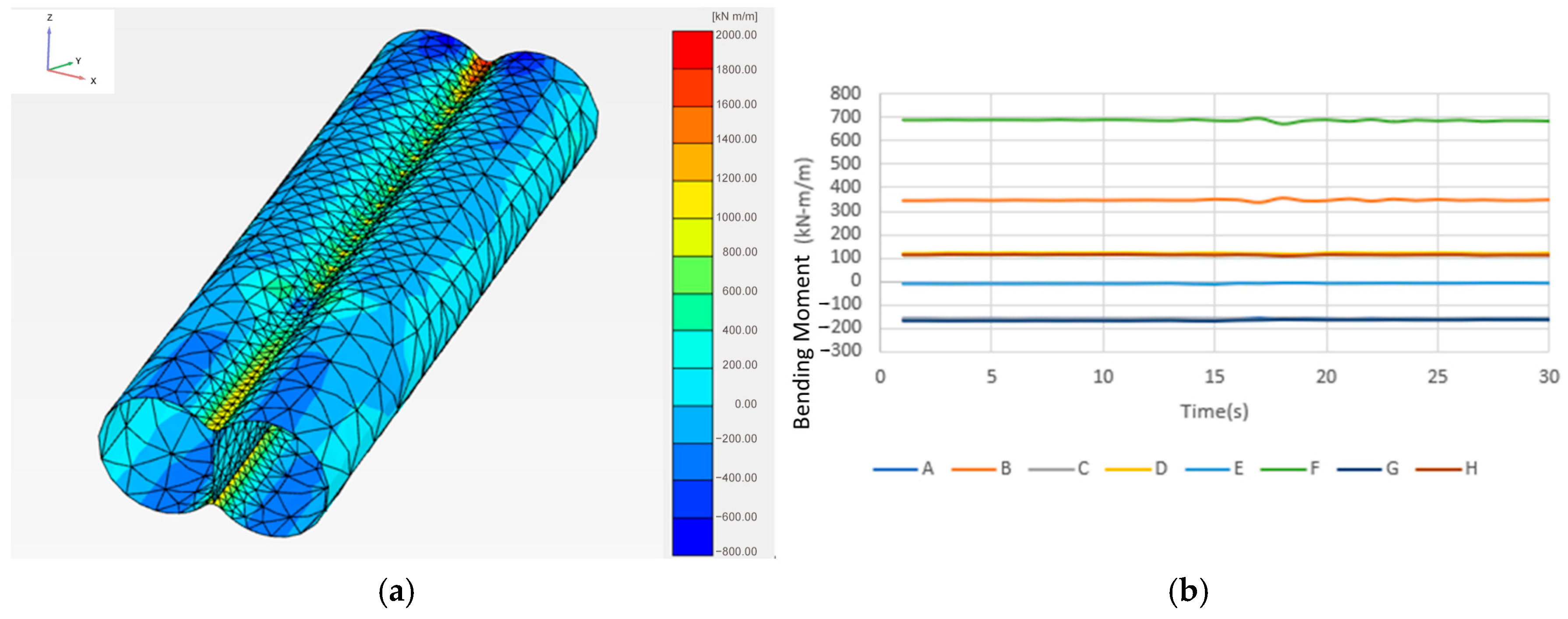
4.3. Mechanical Behavior Analysis of Tunnel Under Seismic Loading

(1)

Axial Force Analysis

This section analyzes the axial force variation in the Double-O-Tube shield tunnel under seismic loading. Figure 20 presents the front view of the tunnel’s axial force distribution. As shown in Figure 20 and Figure 21b, the lower sections of the tunnel (E and G) consistently experience the highest axial forces during the earthquake, reaching approximately 21,000 kN/m.

(2)

Shear Force Analysis

This section analyzes the variation in shear force in the Double-O-Tube shield tunnel during seismic loading. Figure 22 presents the front view of the tunnel’s shear force distribution. As shown in Figure 23a,b, the upper and lower ends of the central column (points B and F) experience the maximum shear force during the earthquake, with values reaching approximately 1500 kN/m.

(3)

Bending Moment Analysis

This section analyzes the variation in bending moments in the Double-O-Tube shield tunnel during seismic loading. Figure 24 presents the front view of the tunnel’s bending moment distribution. As shown in Figure 25a,b, the lower end of the central column (point F) experiences the highest bending moment during the earthquake, reaching approximately 700 kN-m/m. In contrast, the sidewalls (D and H) and the lower edges of the tunnel (E and G) are subjected to minimal bending moments throughout the seismic event.

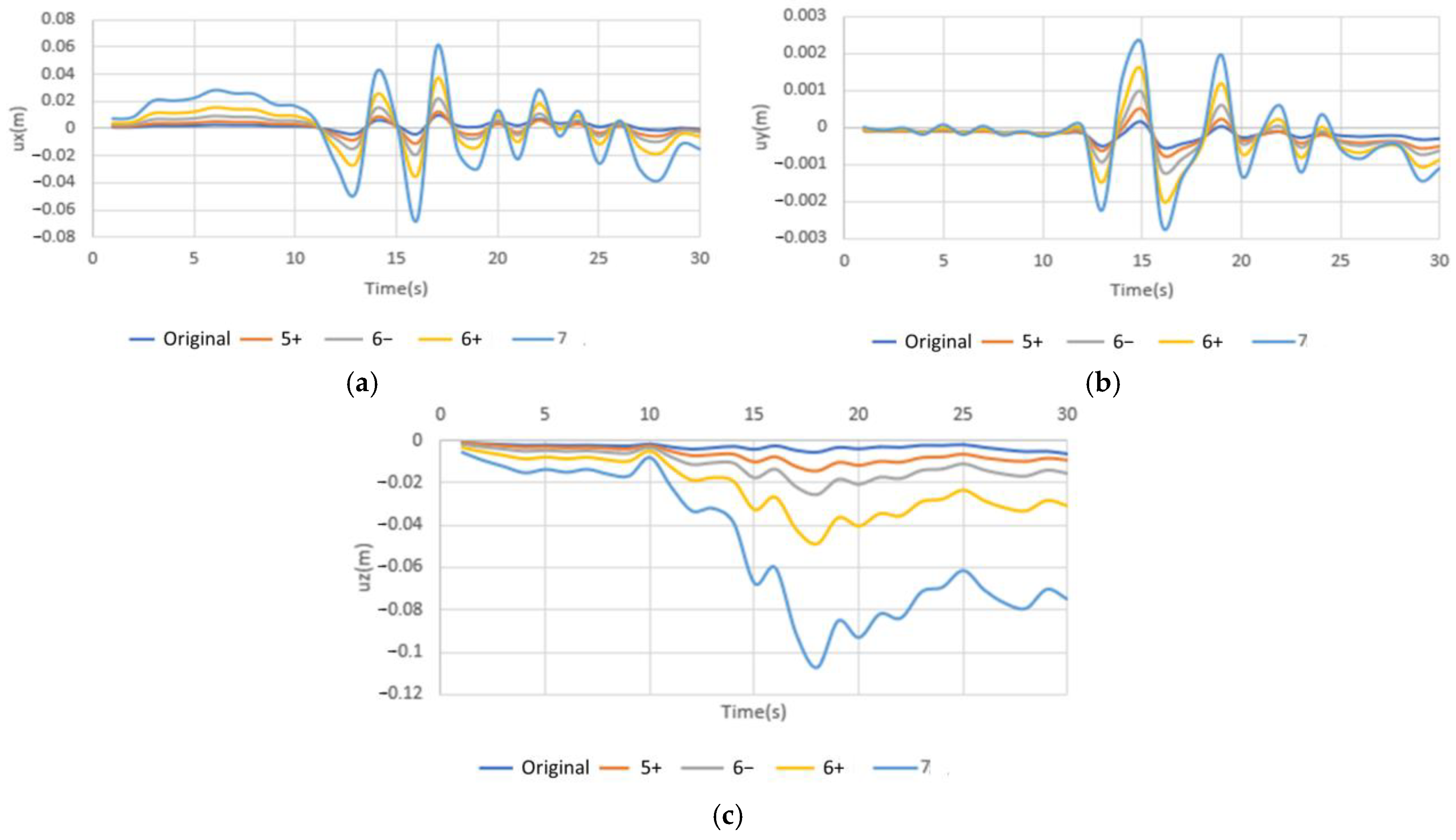
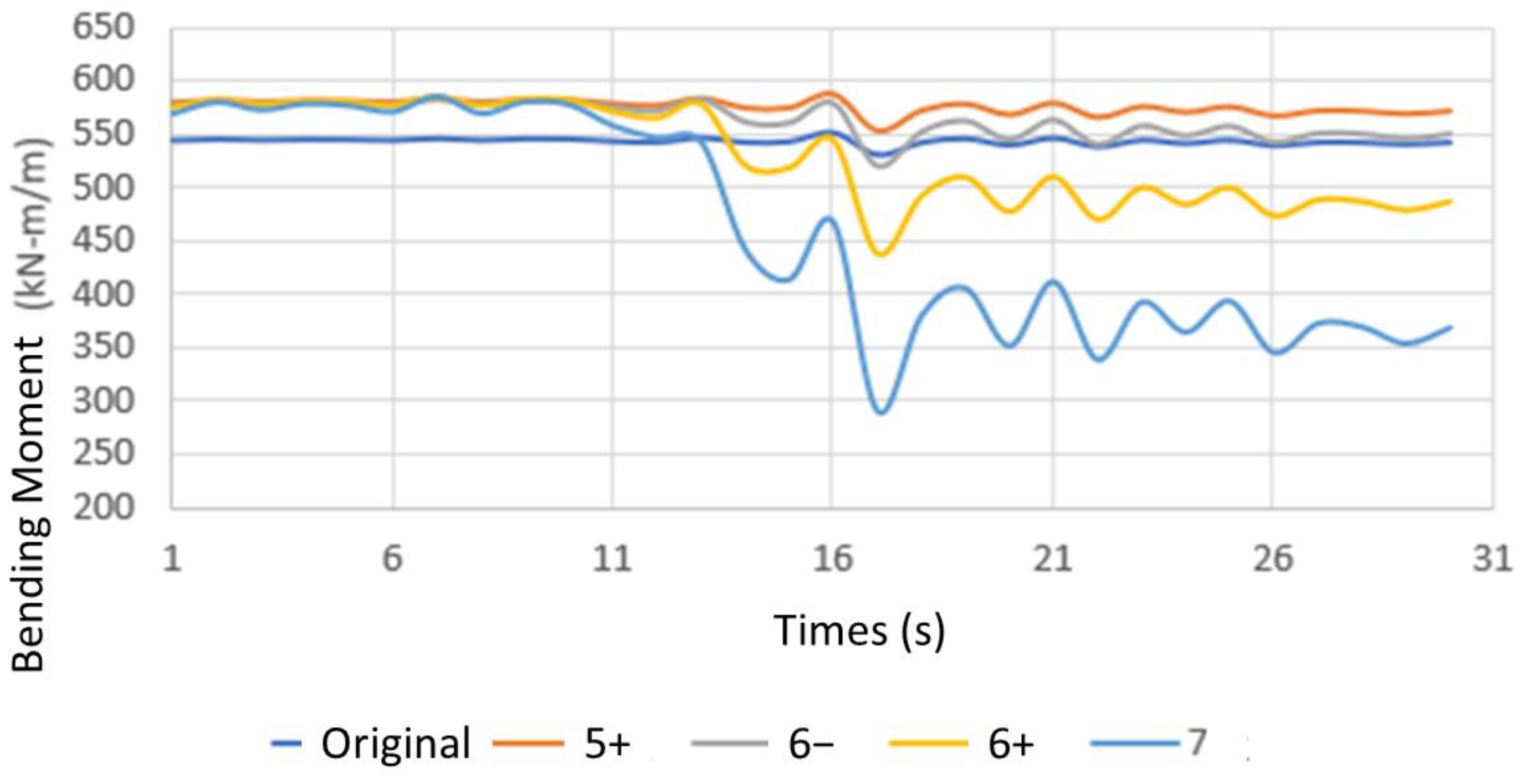
4.4. Mechanical Behavior Analysis of Tunnel Under Earthquakes with Varying Intensities

This section investigates how the mechanical behavior of the tunnel changes under earthquakes of varying intensities by proportionally scaling the acceleration of the original seismic model.

(1)

Displacement Behavior of Tunnel

This section analyzes the three-axis displacement at the top of the tunnel’s central column (Point B) under varying earthquake intensities, as shown in Figure 26a,c and

Table 3. Displacement comparison table at Point B of tunnel under different seismic intensities.

	Original	5+	6−	6+	7
X-Axis Amplification Ratio (%)	−	75	205	390	573
Y-Axis Amplification Ratio (%)	−	155	408	754	1227
Z-Axis Amplification Ratio (%)	−	50	167	452	1220

. As seismic intensity increases, displacement in all directions rises significantly:

-

X-axis: Displacement increases to ~1.75× (Intensity 5+), 3.05× (6−), 4.9× (6+), and 6.73× (7) of the original.

-

Y-axis: Increases to ~2.55×, 5.08×, 8.54×, and 13.27×, respectively.

-

Z-axis: Increases to ~1.5×, 2.67×, 5.52×, and 13.2×, respectively.

This indicates that higher seismic intensity leads to significantly greater tunnel deformation.

(2)

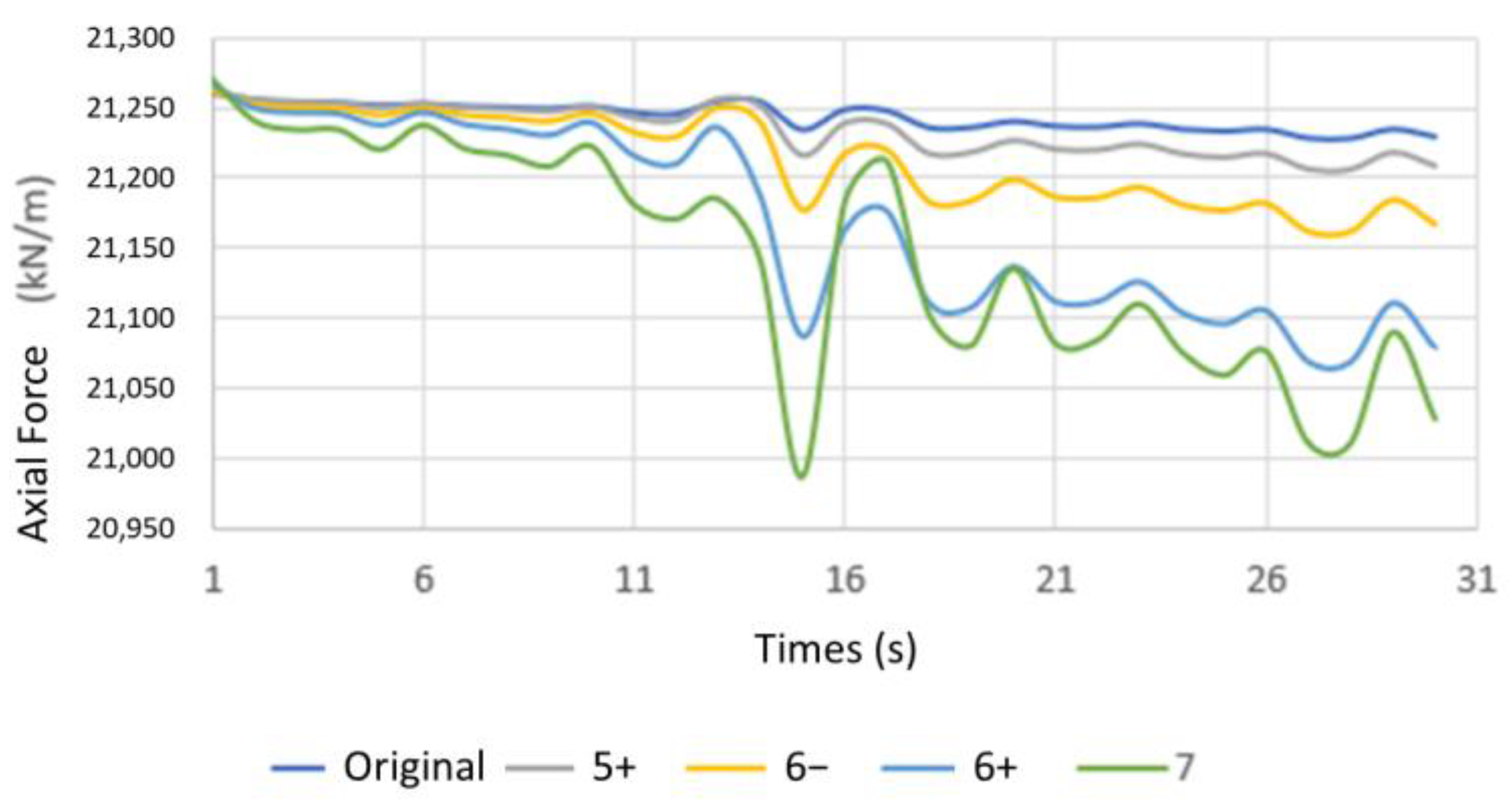
Changes in Tunnel Mechanical Behavior Under Earthquakes of Varying Intensities

This section investigates how tunnel mechanical behavior varies under different seismic intensities, focusing on the axial force N2 at the tunnel bottom edge (Point E), the shear force Q12 at the top of the central column (Point B), and the bending moment M22 at the bottom of the central column (Point F).

▪

Axial Force Variation at Point E of Tunnel

This section analyzes axial force changes at the tunnel’s bottom edge (Point E) under varying seismic intensities, with the results overlaid as shown in Figure 27. Point E was chosen due to its highest axial force in the original case. As seismic intensity increases, axial force fluctuations grow but the overall values decrease. This is due to increased bending stress from positive bending moments, reducing compressive force at the bottom.

▪

Shear Force Variation at Point B of Tunnel

This section analyzes shear force variation at the top of the tunnel’s central column (Point B) under different seismic intensities, overlaid in Figure 28. Point B was chosen due to its highest shear in the original case. At 14–15 s, a magnitude 7 earthquake produces a shear difference about 9.05 times greater than the original.

The results reveal complex seismic behavior, where peak shear and bending moment locations may shift, deviating from the typical assumption that maximum moment occurs where shear is zero.

▪

Bending Moment Variation at Point F of Tunnel

This section examines the bending moment variation at the bottom of the tunnel’s central column (Point F) under different seismic intensities, overlaid as shown in Figure 29. Point F was selected for analysis because it exhibited the highest bending moment under the original earthquake. Between 13 and 17 s into the seismic event, the bending moment variation under a magnitude 7 earthquake is about 15 times that of the original.

This suggests that the tunnel structure experiences bending behavior similar to fatigue failure during strong seismic loading. Therefore, when designing for high-intensity earthquakes, enhanced bending resistance and seismic measures are necessary to ensure the tunnel’s stability and safety.

4.5. Research Limitation

(1)

Soil Stiffness Considerations

A key limitation of this study lies in the assumption of identical soil stiffness for both static and dynamic conditions. It is well recognized that soils exhibit strain-rate-dependent behavior, with higher stiffness typically observed under dynamic loading due to lower strain levels. As a result, applying the same modulus for both scenarios may lead to overestimated displacements and less accurate dynamic responses.

(2)

Modeling Simplification in Plaxis 3D

To improve computational efficiency, structural components such as tunnel linings were modeled using plate elements in Plaxis 3D. While this approach is practical and commonly used for geotechnical analysis, it may not capture out-of-plane shear effects, local 3D stress concentrations, or detailed joint behaviors. As a result, stress and deformation predictions near structural discontinuities or complex loading areas may be less accurate. Future studies may consider using solid elements or sub-modeling techniques to improve local accuracy in critical zones.

5. Conclusions

This study investigates the mechanical behavior of a Double-O-Tube (DOT) shield tunnel under seismic loading through both static and dynamic numerical analyses using PLAXIS 3D 2024. Prior to the dynamic simulation, the model was preprocessed by defining appropriate boundary conditions and applying seismic input data with Arias intensity adjustment, frequency filtering, and baseline correction to ensure the reliability of the results. The tunnel’s displacement and stress responses were then evaluated under varying seismic intensities. The key findings and practical recommendations are summarized below.

5.1. Summary of Findings

(1)

Static analysis: the simulated settlement trough exhibited some deviation from field monitoring data, likely due to model simplifications, such as the use of plate elements for the tunnel lining and central column, and the omission of long-term consolidation effects. However, the predicted maximum settlement was consistent with observed values, indicating reasonable accuracy in capturing localized ground deformation.

(2)

Dynamic response characteristics: the dynamic analysis revealed that the highest axial forces occurred at the bottom edge of the tunnel (Points E and G), the greatest shear force at the top of the central column (Point B), and maximum bending moments at both the top and bottom of the column (Points B and F). These results highlight the critical role of the central support structure during seismic loading.

(3)

Displacement patterns: the tunnel experienced significant displacement in both the vertical and north–south directions, with peak values reaching approximately 0.7 cm and 1.0 cm, respectively. The upper portion of the tunnel moved in the negative direction, while the lower portion moved in the positive direction, consistent with the development of a positive bending moment.

(4)

Soil stiffness considerations: the same Young’s modulus was used in both static and dynamic analyses. Since dynamic loading conditions typically result in higher soil stiffness, this assumption may have led to overestimated displacements in the dynamic simulations.

These results underscore the importance of accurately modeling soil–structure interaction and accounting for dynamic soil behavior in seismic tunnel analysis.

5.2. Recommendations

Based on the numerical analysis results, the following recommendations are proposed for the design and seismic assessment of shield tunnels in seismic-prone regions:

(1)

Reinforcement of central support structures: as seismic forces were concentrated around the top and bottom of the central column, enhanced reinforcement and structural detailing in this area are recommended to improve seismic performance.

(2)

Consideration of displacement direction and bending behavior: the simulation revealed opposite directional displacements in the upper and lower tunnel sections, indicating significant bending effects. Segmental lining and joints should be designed to accommodate rotational and differential deformations.

(3)

Use of dynamic soil stiffness parameters: since soil exhibits higher stiffness under dynamic loading, it is recommended to increase the Young’s modulus by a factor of 2–3 or apply strain-dependent modulus adjustments to avoid overestimation of seismic displacements.

(4)

Improved soil–structure interaction modeling: to enhance the accuracy of settlement prediction, future models should consider using solid elements for tunnel linings and central columns, as well as include long-term consolidation effects.

(5)

Standardization of seismic input processing: frequency filtering, Arias intensity matching, and baseline correction have a significant impact on simulation outcomes. Establishing standardized preprocessing procedures is essential for improving model reliability and consistency.

Overall, accurate representation of dynamic soil behavior and structural response is essential to ensure safe and reliable design of shield tunnels in seismic environments.