Shape Transition and Coexistence in ⁶⁶Se Studied with Phenomenological and Microscopic Models

Abstract

A comprehensive theoretical investigation of shape coexistence and transition phenomena in the neutron-deficient nucleus ${}^{66}\mathrm{Se}$, using complementary microscopic and phenomenological approaches, is presented. The analysis employs the Covariant Density Functional Theory with the Density-Dependent Meson Exchange Model interaction to map the potential energy surface. This microscopic foundation is complemented by calculations using the Bohr–Mottelson Hamiltonian with a sextic oscillator potential, specifically adapted to explore shape coexistence between spherical and $\gamma$-unstable configurations. The latter model successfully reproduces the experimental energy spectrum, including the critical low-lying $0_2^{+}$ state at 1226 keV—a key signature of shape coexistence. An analysis of probability density distributions indicates a distinctive manifestation of shape coexistence wherein different shapes exist without significant mixing in the states. These findings provide crucial insights into the structural dynamics of ${}^{66}\mathrm{Se}$ and establish it as an important case study for understanding shape evolution in neutron-deficient nuclei beyond the $N=Z$ line.

1. Introduction

Shape coexistence in atomic nuclei represents one of the most intriguing phenomena in nuclear structure physics, characterized by the simultaneous presence, in the same nucleus, of distinct shapes such as spherical, triaxial, oblate, and prolate, within a narrow energy range at low excitation energies. This behavior, first proposed by Morinaga in light nuclei [1], has since been observed across the nuclear chart and continues to challenge our theoretical understanding of nuclear structure, particularly in regions where the shell structure undergoes rapid changes near the proton dripline [2,3,4,5,6].

The mass region $A=60-80$ offers an exceptionally rich landscape for investigating shape coexistence phenomena. Within this region, selenium isotopes have attracted significant experimental and theoretical interest, due to their proximity to the proton dripline and their potential to exhibit rapid shape transitions. The nucleus ${}^{66}\mathrm{Se}$, positioned beyond the $N=Z$ line on the proton-rich side of stability, presents a particularly compelling case study due to its exotic location and complex structural properties. The pioneering work on the first $\gamma$ spectroscopy of ${}^{66}\mathrm{Se}$ and ${}^{65}\mathrm{As}$, using two-neutron removal reactions at intermediate beam energies, revealed that the ground state of ${}^{66}\mathrm{Se}$ likely possesses an oblate ground-state deformation [7]. This conclusion was supported by an analysis of Coulomb-energy differences and comparisons with shell-model calculations restricted to the $p{f}_{5/2}{g}_{9/2}$ valence space [8]. Additional experimental evidence strengthening the case for shape coexistence in ${}^{66}\mathrm{Se}$ was reported in a subsequent investigation using neutron knock-out reactions [9]. This study identified five $\gamma$-ray transitions, two previously detected (927 keV, 1460 keV) and three new (744 keV, 1210 keV, 1661 keV) along with coincidence relationships between several transitions. Based on comparison with theoretical calculations, four new excited states were proposed, collectively suggesting that ${}^{66}\mathrm{Se}$ exhibits shape coexistence.

To comprehensively understand the shape coexistence and shape transition mechanisms in ${}^{66}\mathrm{Se}$, we employ two complementary theoretical frameworks that capture both microscopic and collective aspects of nuclear structure. The first approach uses Covariant Density Functional Theory (CDFT) with the Density-Dependent Meson Exchange Model (DD-ME2) interaction [10,11,12,13], which provides a detailed mapping of the potential energy surface (PES) and ground-state deformation properties. CDFT has demonstrated remarkable predictive power across the nuclear chart and offers particular advantages in analyzing shape coexistence through multidimensional deformation energy landscapes. The second approach applies the Bohr–Mottelson Hamiltonian [14] numerically solved for a sextic oscillator potential [15,16]. A phenomenological model is especially well suited for describing collective excitations and shape dynamics. The sextic potential’s flexibility allows us to characterize regions of shape mixing between competing configurations and to study the evolution of shape coexistence with increasing excitation energy. By integrating these complementary approaches, our investigation aims to characterize coexisting shapes in ${}^{66}\mathrm{Se}$ through an analysis of potential energy surfaces and energy spectra; establish connections between microscopic shell structure and collective modes that give rise to shape coexistence; compare theoretical predictions for spectroscopic observables, including energy levels with available experimental data; calculate electromagnetic transition rates; and explore the role of ${}^{66}\mathrm{Se}$ in the broader context of shape evolution across selenium isotopes and neighboring nuclei in this mass region.

The present paper is organized as follows: Section 2 outlines the theoretical frameworks employed in our investigation, detailing the CDFT approach with the DD-ME2 functional and the Bohr–Mottelson Hamiltonian with sextic potential. Section 3 presents our results on energy surfaces, spectroscopic properties, and electromagnetic transitions in ${}^{66}\mathrm{Se}$, comparing theoretical predictions with experimental observations. Finally, Section 4 summarizes the main achievements of the present work.

2. Theoretical Models

The following subsections provide a concise overview of the theoretical frameworks, followed by numerical results and their comparison with the available experimental data.

2.1. Covariant Density Functional Theory with Meson-Exchange Model

The Covariant Density Functional Theory (CDFT) [10,11,12,13,17], founded on Energy-Density Functionals (EDFs), stands as one of the most powerful approaches in nuclear Density Functional Theory. CDFT has demonstrated remarkable effectiveness in nuclear structure analysis [18,19,20] and excels in accurately reproducing the properties of both ground and excited states across the nuclear chart [21,22]. In the meson-exchange model formulation, nuclei are represented as systems of Dirac nucleons that interact through the exchange of finite-mass mesons, resulting in finite-range interactions [23]. The standard Lagrangian density incorporating medium-dependent vertices that defines the meson-exchange model [24] is given by:

$$\mathcal{L}={\mathcal{L}}_N+{\mathcal{L}}_m+{\mathcal{L}}_{int}.$$

(1)

In this formulation, ${\mathcal{L}}_N$ represents the Lagrangian of the free nucleon:

$${\mathcal{L}}_N=\overline{\psi }\left(i{\gamma }_{\mu }{\partial }^{\mu }-m\right)\psi ,$$

(2)

where m is the bare nucleon mass and the symbol $\psi$ denotes the Dirac spinors. Further, ${\mathcal{L}}_m$ is the Lagrangian for the free meson fields and electromagnetic field:

$$\begin{array}{c}\hfill \begin{array}{c}{\mathcal{L}}_m=+{\displaystyle \frac{1}{2}}{\partial }_{\mu }\sigma {\partial }^{\mu }\sigma -{\displaystyle \frac{1}{2}}{m}_{\sigma }^2{\sigma }^2-{\displaystyle \frac{1}{4}}{\Omega }_{\mu \nu }{\Omega }^{\mu \nu }+{\displaystyle \frac{1}{2}}{m}_{\omega }^2{\omega }^2-{\displaystyle \frac{1}{4}}{\overrightarrow{R}}_{\mu \nu }{\overrightarrow{R}}^{\mu \nu }\\ +{\displaystyle \frac{1}{2}}{m}_{\rho }^2{\overrightarrow{\rho }}_{\mu }{\overrightarrow{\rho }}^{\mu }-{\displaystyle \frac{1}{4}}{F}_{\mu \nu }{F}^{\mu \nu },\end{array}\end{array}$$

(3)

where the parameters $m_{\sigma }$, $m_{\omega }$, and $m_{\rho }$ represent the masses of the $\sigma$, $\omega$, and $\rho$ mesons, respectively, while ${\Omega }_{\mu \nu }$, ${\overrightarrow{R}}^{\mu \nu }$, and $F_{\mu \nu }$ are field tensors. Arrows denote isovectors and boldface symbols are used for vectors in ordinary space. The minimal set of interaction terms is contained in ${\mathcal{L}}_{int}$:

$${\mathcal{L}}_{int}=-g_{\sigma }\overline{\psi }\psi \sigma -g_{\omega }\overline{\psi }{\gamma }^{\mu }\psi {\omega }_{\mu }-g_{\rho }\overline{\psi }\overrightarrow{\tau }{\gamma }^{\mu }\psi {\overrightarrow{\rho }}_{\mu }-e\overline{\psi }{\gamma }^{\mu }\psi A_{\mu },$$

(4)

whereby $g_{\sigma }$, $g_{\omega }$, and $g_{\rho }$ are denoted the coupling constants for mesons with the nucleons, and e represents the electric charge of the proton.

In this present work, we implement a finite-range pairing interaction, that is separable in coordinate space, as introduced by Tian et al. [25]. This interaction is characterized in the particle–particle ($pp$) channel by:

$$V^{pp}(r_1,r_2,r_1^{\prime },r_2^{\prime })=-G\delta (R-R^{\prime })P(r)P(r^{\prime }),$$

(5)

where $R={\displaystyle \frac{1}{\sqrt{2}}}(r_1+r_2)$ is the centre of mass, and $r={\displaystyle \frac{1}{\sqrt{2}}}(r_1-r_2)$ are the relative coordinates. $P(r)$ represents the form factor, which is defined as:

$$P(r)={\displaystyle \frac{1}{{(4\pi a^2)}^{3/2}}}{e}^{-{\displaystyle \frac{r^2}{4a^2}}}.$$

(6)

The pairing strength (G) and pairing width (a) parameters have been fitted to reproduce the density dependence of the gap at the Fermi surface in nuclear matter derived from the Gogny force. Specifically, for the D1S parametrization [25] of the Gogny force, these parameters were determined to be $G=728$ $\mathrm{MeV}·{\mathrm{fm}}^3$ and $a=0.6442$ fm.

The investigation of the energy-surface map as a function of the quadrupole deformation parameter is generated by solving the Relativistic Hartree–Bogoliubov (RHB) equation with the quadratic constrained method [26]. This approach employs an unrestricted variation of the following function:

$$⟨\widehat{H}⟩+\sum _{\mu =0,2}{C}_{2\mu }(⟨{\widehat{Q}}_{2\mu }⟩-q_{2\mu }),$$

(7)

where $⟨\widehat{H}⟩$ denotes the total energy, and $⟨{\widehat{Q}}_{2\mu }⟩$ are the expectation values of mass quadrupole operators,

$$⟨{\widehat{Q}}_{20}⟩=2z^2-x^2-y^2\mathrm{and}⟨{\widehat{Q}}_{22}⟩=x^2-y^2,$$

(8)

$q_{2\mu }$ is the constrained value of the multipole moment, while $C_{2\mu }$ denotes the corresponding stiffness constant [26]. For a self-consistent solution, the quadratic constraint adds an extra force term ${\sum }_{\mu =0,2}{\lambda }_{\mu }{\widehat{Q}}_{2\mu }$ to the system, where ${\lambda }_{\mu }=2C_{2\mu }{(⟨{\widehat{Q}}_{2\mu }⟩-q_{2\mu })}^2$. Such a term is necessary to force the system to a point in deformation space different from the stationary point.

2.2. Bohr Hamiltonian with Sextic Oscillator Potential

The Bohr Hamiltonian for a quadrupole deformation, written in the intrinsic reference frame, has the following form in the five dimensional space [14]:

$$\begin{array}{c}\hfill \begin{array}{ccc}H& =& -{\displaystyle \frac{{\hslash }^2}{2B}}\left({\displaystyle \frac{1}{{\beta }^4}}{\displaystyle \frac{\partial }{\partial \beta }}{\beta }^4{\displaystyle \frac{\partial }{\partial \beta }}+{\displaystyle \frac{1}{{\beta }^{2}sin3\gamma }}{\displaystyle \frac{\partial }{\partial \gamma }}sin3\gamma {\displaystyle \frac{\partial }{\partial \gamma }}\right)\\ & & +{\displaystyle \frac{{\hslash }^2}{8B{\beta }^2}}{\displaystyle \sum _{k=1}^3}{\displaystyle \frac{{\widehat{L}}_k^2}{{sin}^2\left(\gamma -\frac{2}{3}\pi k\right)}}+V(\beta ,\gamma ).\end{array}\end{array}$$

(9)

Here, B represents the mass parameter, $\beta$ and $\gamma$ are the intrinsic polar coordinates describing the deviation from sphericity and axiallity, while the operators ${\widehat{L}}_k$ denote projections of the total angular momentum $\widehat{L}$ in the intrinsic reference frame, and V represents the energy potential.

In the present study, we consider a coexistence between an approximately spherical vibrator [27] and a $\gamma$-unstable system [28,29]. This approach yields a $\gamma$-independent potential, meaning the potential in the Bohr Hamiltonian (9) depends solely on the $\beta$ variable, i.e., $V(\beta ,\gamma )=V(\beta )$ [28]. Therefore, to achieve exact separation of variables, we assume the total wave functions takes the form $\mathbf{\Psi }(\beta ,\gamma ,{\theta }_i)=\psi (\beta )\mathbf{\Phi }(\gamma ,{\theta }_i)$, where $({\theta }_i,i=1,2,3.)$ are the Euler angles describing rotations. Consequently, we can derive the following two differential equations for the collective variables [28,29]:

$$\left[-{\displaystyle \frac{1}{{\beta }^4}}{\displaystyle \frac{\partial }{\partial \beta }}{\beta }^4{\displaystyle \frac{\partial }{\partial \beta }}+{\displaystyle \frac{\mathrm{\Lambda }}{{\beta }^2}}+v(\beta )\right]\psi (\beta )=\epsilon \psi (\beta ),$$

(10)

$$\left[-{\displaystyle \frac{1}{sin3\gamma }}{\displaystyle \frac{\partial }{\partial \gamma }}sin3\gamma {\displaystyle \frac{\partial }{\partial \gamma }}+{\displaystyle \frac{1}{4}}\sum _{k=1}^3{\displaystyle \frac{{\widehat{L}}_k^2}{{sin}^2\left(\gamma -\frac{2\pi }{3}k\right)}}\right]\mathbf{\Phi }(\gamma ,{\theta }_i)=\mathrm{\Lambda }\mathbf{\Phi }(\gamma ,{\theta }_i),$$

(11)

where the reduced potential and total energy are defined as $v(\beta )=(2B/{\hslash }^2)V(\beta )$ and $\epsilon =(2B/{\hslash }^2)E$, respectively. The solution of $\gamma$ and the Euler angles in Equation (11) was derived by Bes [29]. The eigenvalues of the second order Casimir operator of SO(5) are expressed as $\mathrm{\Lambda }=\tau (\tau +3)$, with $\tau$ is the seniority quantum number characterizing the irreducible representations of SO(5) and taking values $\tau =0,1,2,\dots ,$ [30].

Our focus now shifts to the solvability of Equation (10), which takes the following form:

$$\left[-{\displaystyle \frac{d^2}{d{\beta }^2}}-{\displaystyle \frac{4}{\beta }}{\displaystyle \frac{d}{d\beta }}+{\displaystyle \frac{\tau (\tau +3)}{{\beta }^2}}+v(\beta )\right]\psi (\beta )=\epsilon \psi (\beta ),$$

(12)

following the expansion of the kinetic term operator with respect to the $\beta$ variable. Further, we employ the sextic oscillator potential as the appropriate potential energy. This particular potential is well suited for describing the coexistence and mixing phenomena between spherical and deformed nuclear shapes, being expressed as [15,16,31,32,33,34]:

$$v(\beta )=a{\beta }^2+b{\beta }^4+c{\beta }^6.$$

(13)

Exploiting the scaling properties inherent to polynomial potentials [35], one can remove one of these parameters through the variable transformation $\beta =a^{-1/4}y$, yielding:

$$\left[-{\displaystyle \frac{d^2}{d{y}^2}}-{\displaystyle \frac{4}{y}}{\displaystyle \frac{d}{dy}}+{\displaystyle \frac{\tau (\tau +3)}{y^2}}+v_1{y}^2+v_2{y}^4+v_3{y}^6\right]F(y)=\tilde{\epsilon }F(y).$$

(14)

In this expression, we have introduced the following parameter transformations $v_1=1$, $v_2=b{a}^{-{\displaystyle \frac{3}{2}}}$, $v_3=c{a}^{-2}$, $\tilde{\epsilon }(1,v_2,v_3)=a^{-{\displaystyle \frac{1}{2}}}\epsilon (a,b,c)$. In what follows, $v_1$ will be dropped, keeping only the free parameters explicitly retained. In terms of the remained parameters, the potential can simultaneously present two minima, a spherical and a deformed one, if and only if $v_2<0$ and $v_3>0$. The two minima can be also degenerated in relation to their corresponding energy for $v_2=-2q$ and $v_3=q^2$, where q is a positive real parameter directly related to the height of the barrier (maximum) separating the two degenerated minim [15].

The resulting Equation (14) is solved numerically using a basis of Bessel functions of the first kind, an approach initially proposed by Budaca et al. [16] for a $\gamma$-unstable case. These basis functions are themselves solutions to the same equation when considering an Infinite Square Well Potential (ISWP) of the well-known E(5) model [36], which describes the critical point of the phase transition between spherical vibrator and $\gamma$-unstable systems:

$$f_{n,\nu }(y)={\displaystyle \frac{\sqrt{2}}{y_w}}{\displaystyle \frac{y^{-\frac{3}{2}}{J}_{n,\nu }\left(\frac{z_{n,\nu }}{y_w}y\right)}{J_{\nu +1}(z_{n,\nu })}},\nu =\tau +{\displaystyle \frac{3}{2}}.$$

(15)

Here, $y_w$ represents the position of the infinite wall of the ISWP [36], while $J_{n,\nu }$ denotes the Bessel function of the first kind of index $\nu$, with $z_{n,\nu }$ being its n^th zero. The wave-function solution to Equation (14) with sextic oscillator potential can therefore be expressed as an expansion in this orthogonal basis:

$$F_{\xi ,\tau }(y)=\sum _{n=1}^{n_{Max}}{A}_n^{\xi }{f}_{n,\nu }(y),$$

(16)

where $n_{Max}$ determines the dimension of the truncation basis, $\xi =1,2,\dots ,n_{Max}$ corresponds to the $\beta$ vibrational excitation quantum number, and $A_n^{\xi }$ are the eigenvector components that will be determined through diagonalization of the corresponding Hamiltonian matrix. The matrix representation of Equation (14), using the wave-function expansion from Equation (16), takes the form:

$$\sum _{n=1}^{n_{Max}}{A}_n^{\xi }{H}_{mn}=\tilde{\epsilon }\sum _{n=1}^{n_{Max}}{A}_n^{\xi }{\delta }_{mn},$$

(17)

with a general matrix element having the expression:

$$H_{mn}={\left({\displaystyle \frac{z_{n,\nu }}{y_w}}\right)}^2{\delta }_{mn}+y_w^2{I}_{mn}^{(\nu ,1)}+v_2{y}_w^4{I}_{mn}^{(\nu ,2)}+v_3{y}_w^6{I}_{mn}^{(\nu ,3)},$$

(18)

where

$$I_{mn}^{(\nu ,i)}={\displaystyle \frac{2}{J_{\nu +1}(z_{m,\nu })J_{\nu +1}(z_{n,\nu })}}{\int }_0^1{J}_{\nu }\left(z_{m,\nu }x\right)J_{\nu }\left(z_{n,\nu }x\right)x^{2i+1}dx.$$

(19)

The indices $i=1,2,3,$ represent the matrix elements corresponding to the potential terms $y^{2i}$, which are independent of $y_w$ due to the change of variable $x=y/y_w$.

The total energy is given by the eigenvalue of Equation (17):

$$E_{\xi ,\tau ,L}={\displaystyle \frac{\sqrt{a}{\hslash }^2}{2B}}\left[{\tilde{\epsilon }}_{\xi ,\tau }(v_2,v_3)+dL(L+1)\right],$$

(20)

while an additional term, $dL(L+1)$, coming from the $SO(5)$ symmetry operator ${\widehat{L}}^2$, is incorporated into the Hamiltonian to split the degeneracy of the $\tau$ energy multiplet without changing the wave functions [37]. When the absolute energies are normalized to the energy of the first exited state ($2_1^{+}$) of the ground band,

$$R_{\xi ,\tau ,L}={\displaystyle \frac{E_{\xi ,\tau ,L}-E_{1,0,0}}{E_{1,1,2}-E_{1,0,0}}},$$

(21)

the scaling factor $\sqrt{a}{\hslash }^2/2B$ is eliminated, leaving only three free parameters, $v_2$, $v_3$, and d, to be involved in the fitting procedure. The $y_w$ is not treated as a free parameter. Instead, it is fixed during the numerical diagonalization process to intersect the tail of the sextic oscillator potential at a very high-spin state, typically the highest-energy state included in the fitting procedure.

The model presented above is suitable for describing shape coexistence between spherical and $\gamma$-unstable shapes and it was introduced in its complete form in [16]. To assess the presence of shape coexistence and mixing phenomena within a nucleus, several key observables are analyzed: the energy spectrum, the shape of the sextic potential, the probability density distributions for the ground and excited states, the quadrupole transition probabilities between states belonging to different bands. The definitions of these quantities are provided below.

The density distribution probability for deformation is defined as the square of the wave function (16) multiplied by $y^4$

$${\rho }_{\xi ,\tau }(y)={\left[F_{\xi ,\tau }(y)\right]}^2{y}^4:$$

(22)

For the calculation of $E2$ electromagnetic transitions, the quadrupole transition operator is used [14,27]:

$$\begin{array}{c}\hfill \begin{array}{ccc}{T}_{2,\mu }^{(E2)}& =& {\displaystyle \frac{3R^{2}Ze}{4\pi }}{\beta }_{M}y\times \hfill \\ & & \left[D_{\mu ,0}^2({\theta }_i)cos\gamma +{\displaystyle \frac{1}{\sqrt{2}}}\left(D_{\mu ,2}^2({\theta }_i)+D_{\mu ,-2}^2({\theta }_i)\right)sin\gamma \right],\hfill \end{array}\end{array}$$

(23)

derived from the quadrupole coordinates ${\alpha }_{2,\mu }$ of the nuclear surface. This operator’s expression incorporates: the nuclear radius $R=R_0{A}^{1/3}$, where $R_0=1.2fm$ and A is the atomic mass; the charge number Z and elementary charge e; the scaling parameter ${\beta }_M$, which is determined from the experimental $E2$ electromagnetic transitions between the $2_1^{+}$ and $0_1^{+}$ states; and the Wigner matrices [38] associated with rotations over the Euler angles ${\theta }_1$, ${\theta }_2$ and ${\theta }_3$.

Given that the wave functions are expressed in terms of the scaled variable y, the following relation ${\beta }_2=y{\beta }_M$ restores the correspondence with the quadrupole deformation variable ${\beta }_2$. The quadrupole electromagnetic transitions, denoted by $B(E2)$, are calculated using the the operator transition form Equation (23), accounting for contributions from all five variables $(y,\gamma ,{\theta }_1,{\theta }_2,{\theta }_3)$ [16]:

$$\begin{array}{c}\hfill \begin{array}{c}B(E2;\xi \tau L\to {\xi }^{\prime }{\tau }^{\prime }{L}^{\prime })={\left({\displaystyle \frac{3R^{2}Ze}{4\pi }}\right)}^2{\beta }_M^2\times \\ ({\tau }^{\prime }{\alpha }^{\prime }{L}^{\prime }{;112\left|\right|\tau \alpha L)}^2⟨\tau \left|\right|\left|Q\right|\left|\right|{\tau }^{\prime }{⟩}^2{(B_{\xi \tau ;{\xi }^{\prime }{\tau }^{\prime }})}^2,\end{array}\end{array}$$

(24)

where $({\tau }_1{\alpha }_1{L}_1;{\tau }_2{\alpha }_2{L}_2||{\tau }_3{\alpha }_3{L}_3)$ is the $SO(5)$ Clebsch–Gordon coefficient [39],

$$⟨\tau \left|\right|\left|Q\right|\left|\right|{\tau }^{\prime }⟩=\sqrt{{\displaystyle \frac{\tau }{2\tau +3}}}{\delta }_{\tau ,{\tau }^{\prime }+1}+\sqrt{{\displaystyle \frac{\tau +3}{2\tau +3}}}{\delta }_{\tau ,{\tau }^{\prime }-1},$$

(25)

is the $SO(5)$ reduced matrix element of the quadrupole moment [40,41], while the integral over y is denoted by

$$B_{\xi \tau ;{\xi }^{\prime }{\tau }^{\prime }}=⟨F_{\xi ,\tau }(y)\left|y\right|F_{{\xi }^{\prime },{\tau }^{\prime }}(y)⟩.$$

(26)

3. Numerical Applications

The phenomenological and microscopic models presented in Section 2 are employed here to investigate the nuclear structure and shape characteristics of ${}^{66}\mathrm{Se}$ isotope. The selection of this nucleus as a candidate for exhibiting shape coexistence and mixing phenomena is supported by a preliminary analysis of the available experimental data [7,9]. Our Covariant Density Functional Theory (CDFT) calculations [10,11,12,13] with the Density-Dependent Meson-Exchange DD-ME2 interaction [13] reveal the potential energy surface for this nucleus, illustrated in Figure 1, with the corresponding parameters listed in Table 1. These results strongly support our description within the $\gamma$-unstable Bohr Hamiltonian with sextic potential. The analysis reveals a notably $\gamma$-soft energy surface with a shallow oblate global minimum at $\beta =0.26$. A comparable behavior has been observed using the Gogny D1S force as a function of quadrupole degrees, as reported in Ref. [7]. However, this observation does not definitively rule out shape coexistence and mixing phenomena, as a comprehensive assessment requires correlation with excited state information. To address this, we will employ the Bohr–Mottelson model [14,27] with the sextic oscillator potential [15].

Figure 1. The potential energy surface for ${}^{66}\mathrm{Se}$ in the $({\beta }_2,\gamma )$ plane, obtained from CDFT calculations [10,11,12,13] with DD-ME2 parameter sets [13]. The color scale shown at the right has the units of MeV, and is scaled such that the ground state has a zero MeV energy.

Table 1. The parameters of the DD-ME2 functional, with masses expressed in MeV and all other parameters being dimensionless.

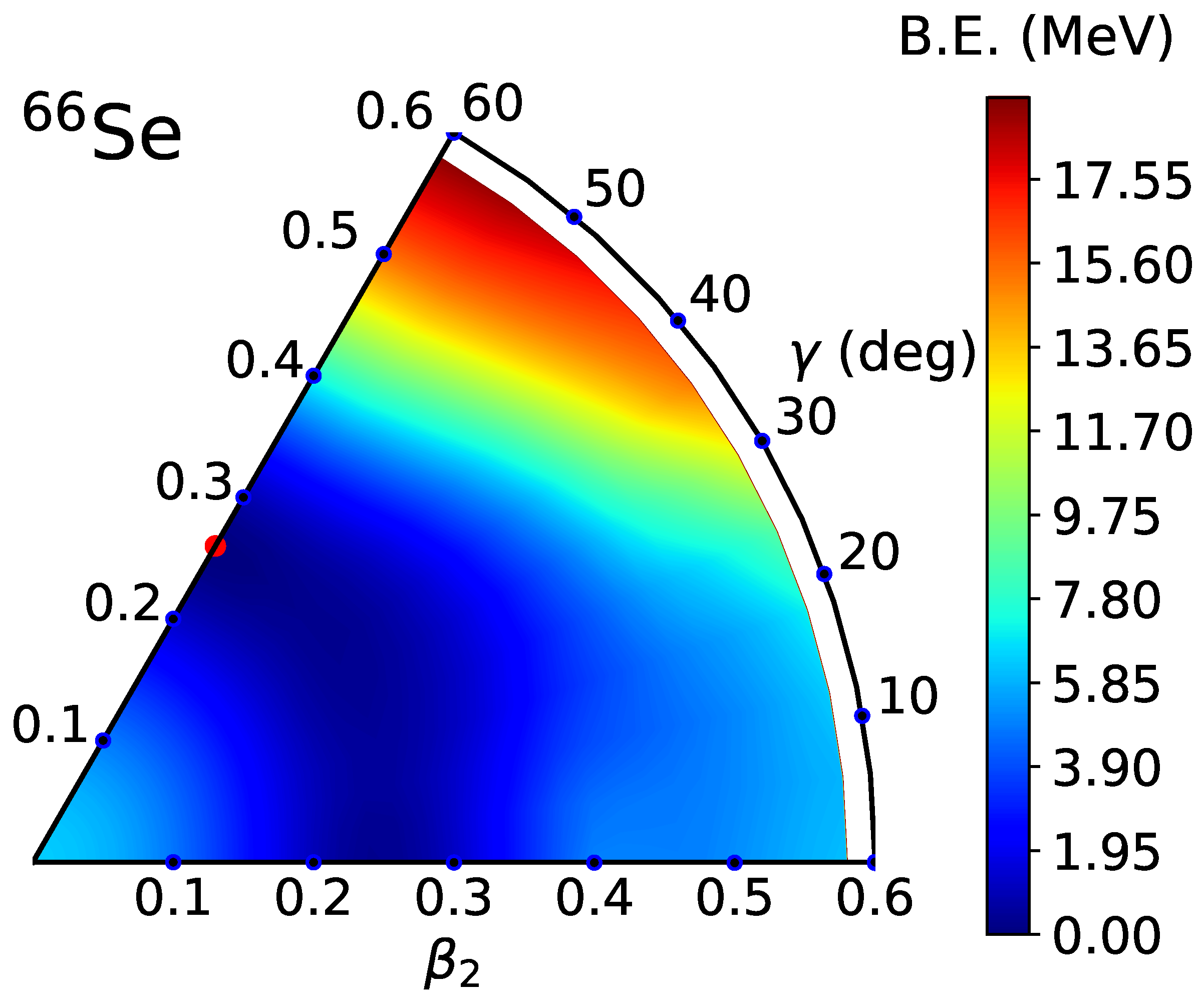
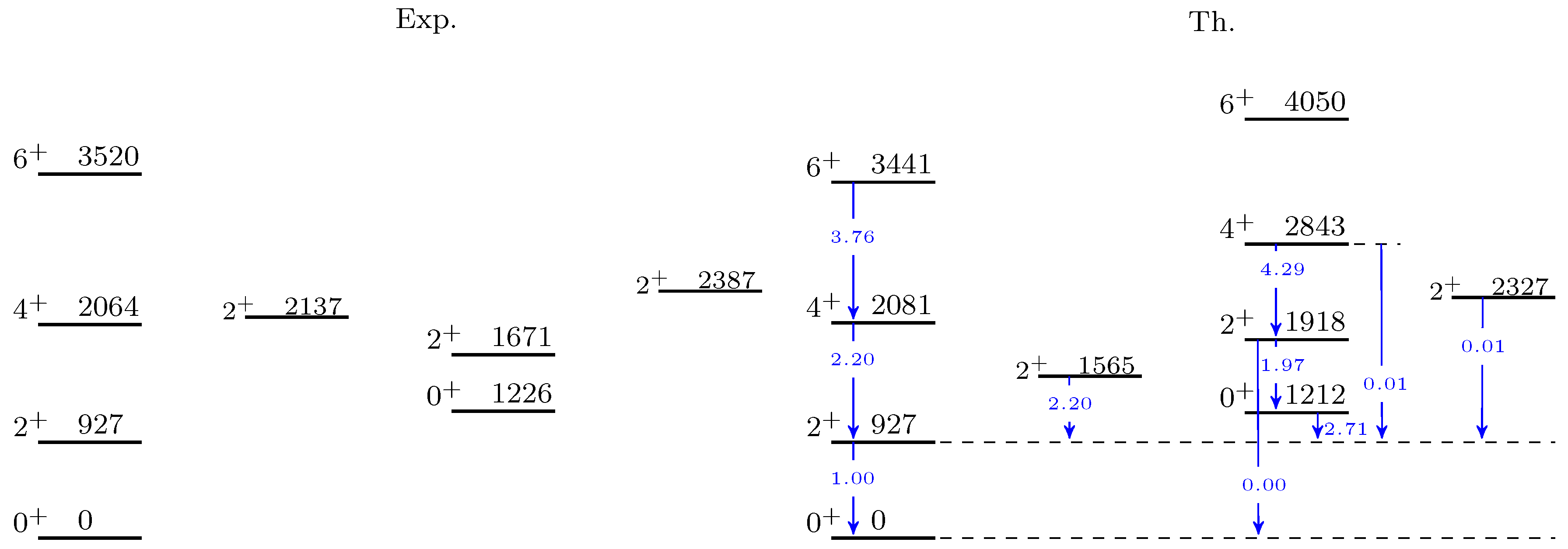
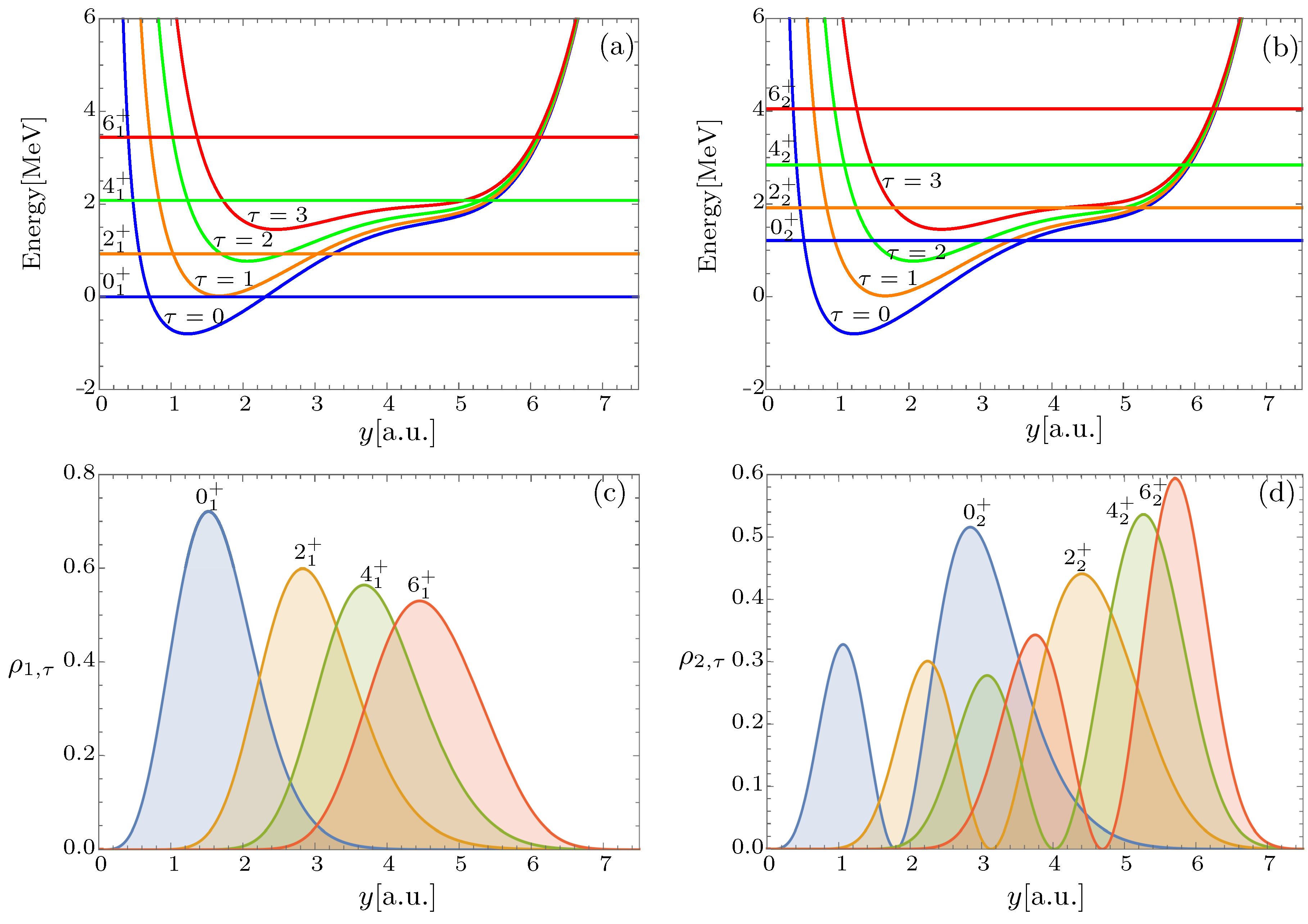
Parameter	DD-ME2
m	939
$m_{\sigma }$	550.1238
$m_{\omega }$	783.000
$m_{\rho }$	763.000
$g_{\sigma }$	10.5396
$g_{\omega }$	13.0189
$g_{\rho }$	3.6836
$a_{\sigma }$	1.3881
$b_{\sigma }$	1.0943
$c_{\sigma }$	1.7057
$d_{\sigma }$	0.4421
$a_{\omega }$	1.3892
$b_{\omega }$	0.9240
$c_{\omega }$	1.4620
$d_{\omega }$	0.4775
$a_{\rho }$	0.5647

Drawing from these experimental and theoretical insights, we found it most appropriate to describe the lowest collective states of ${}^{66}\mathrm{Se}$ using the collective model proposed by Budaca et al. [15] and subsequently adapted [32,33] to explore shape coexistence and mixing between an approximately spherical shape and a $\gamma$-unstable triaxial configuration. Our model implementation employs a systematic root mean square procedure. We adjust the free parameters $v_2$, $v_3$ and d to accurately reproduce three key experimental observations: the experimental energies of the ground-state and $\beta$ bands, and an additional $2_3^{+}$ state, which we interpret it within our model as the head of the first $\gamma$ band. The parameter values, determined through this procedure, are presented in Table 2. The scaling parameter ${\displaystyle \frac{\sqrt{a}{\hslash }^2}{2B}}$ is fixed such that to reproduce the experimental energy of the first excited state $2_1^{+}$ of the ground band.

Table 2. The free parameters of the model, $v_2$, $v_3$, d, and $y_w$ fitted for the experimental data of ${}^{66}\mathrm{Se}$ [8,9]. The root mean square ($rms$) corresponds to the fit quality on the dimensionless energy ratios normalized to the first excited energy of the $2_1^{+}$ state. The scaling parameter $\sqrt{a}{\hslash }^2/2B$ is fixed such that to exactly reproduce the energy of the $2_1^{+}$ state.

Nucleus	${\mathit{v}}_2$	${\mathit{v}}_3$	d	${\mathit{y}}_{\mathit{w}}$	$\frac{\sqrt{\mathit{a}}{\mathit{\hslash }}^2}{2\mathit{B}}$ [keV]	$\mathit{rms}$
${}^{66}\mathrm{Se}$	−0.0434384787	0.0006814582	0.084722	7.500	434.94	0.278

Figure 2 compares our theoretical energy spectra with experimental measurements and presents the calculated electromagnetic transitions. From this figure, one can see that the ground-state band energies are reproduced with high precision. Concerning the low-energy $0_2^{+}$ state, positioned at 1226 keV in experimental measurements, serves as a critical signature for shape coexistence and is remarkably well reproduced by our model, which predicts a value of 1212 keV. For comparative context, the Shell Model, as presented in Figure 3 of [9], predicts 1180 keV for this state. Beyond this specific state, our model also demonstrates good agreement with the other experimental data of this nucleus when compared to the Shell Model. For example, in the ground band, excepting the energy of the $2_1^{+}$ state which in the present model is exactly reproduced by fixing the scaling parameter, but also the energy of the $6_1^{+}$ which is not calculated in [9], one has a very good reproduction of the energy for the $4_1^{+}$ state. The experimental value for this state is 2064 keV, while the values for the present model and the Shell Model are 2081 keV and 2221 keV, respectively. Concerning the $2_2^{+}$ state, apparently its energy better fits the Shell Model picture, but if one calculates the corresponding absolute deviation in energy for both models, one obtains 247 keV and 279 keV for the present model and for the Shell Model, respectively. Thus, the energy for this state calculated with the present model is slightly closer to the experiment than that given by the Shell Model. Instead, the energy of the $2_3^{+}$ at 2137 keV is clearly overestimated by the present model (1565 keV) and well predicted by the Shell Model (1962 keV). Finally, for the last state $2_4^{+}$ (2387 keV), considered in both studies, one has again a very good result for our model at 2327 keV, compared to the 2271 keV obtained with the Shell Model. This concordance with both experimental measurements and the independent Shell Model calculation strongly validates the reliability and accuracy of our theoretical approach.

Figure 2. The calculated energy spectrum, in units of keV, for the ground-state band (g.s.b.), $\beta$-band (up to $6^{+}$) and an associated head of the $\gamma$-band, as well as electromagnetic $E2$ transitions, are compared with the experimental data for ${}^{66}\mathrm{Se}$ [8,9].

Figure 3. The probability density distribution ${\rho }_{\xi ,\tau }$, given by Equation (22), is plotted as a function of y for the states of the ground band (c) and $\beta$ band (d) together with the corresponding effective potentials and energy levels in panel (a), respectively panel (b).

The calculated $B(E2)$ transition rates exhibit patterns characteristic of collective behavior, with stronger intra-band transitions and some weaker inter-band transitions, further supporting the interpretation of shape coexistence without significant mixing between different configurations in ${}^{66}\mathrm{Se}$. Nevertheless, there is at least an inter-band transition, $B(E2;0_2^{+}\to 2_1^{+})=2.7$, which is relatively strong. This has happened because the two peaks for the probability density distribution of deformation corresponding to $0_2^{+}$ are above the same minimum of the potential, like the peak of the $2_1^{+}$ state. Moreover, the dominant peak of the $0_2^{+}$ state is largely overlapping with the one of the $2_1^{+}$ state. These matches favor an increased value for the transition matrix element between these states and, if it will be confirmed by the experiment, it could be interpreted as the presence of the shape coexistence with mixing. It should be noted here that experimental data for electromagnetic $E2$ transitions, as well as the energy levels of the $4_2^{+}$ and $6_2^{+}$ states are not currently available for this nucleus, making these theoretical predictions particularly valuable for guiding future experimental investigations of the nucleus. In the absence of an experimental reference point to definitively calibrate the transition operator’s scaling parameter, we have normalized our $B(E2)$ values relative to the $B(E2;2_1^{+}\to 0_1^{+})$ transition.

All these data are analyzed in relation to the probability density distribution of deformation ${\rho }_{\xi ,\tau }(y)$, given by Equation (22), which is graphically represented in Figure 3 as a function of y for all considered states of the ground and $\beta$ bands and associated effective potentials with included state-dependent centrifugal contribution:

$$V_{eff}(y)={\displaystyle \frac{\sqrt{a}{\hslash }^2}{2B}}\left[{\displaystyle \frac{\tau (\tau +3)+2}{y^2}}+y^2+v_2{y}^4+v_3{y}^6\right].$$

(27)

Here, the additional term $2/y^2$ comes from a change of the function as $\psi (y)=y^{-2}g(y)$. Usually, such a transformation is involved to get a one dimensional Schrödinger equation form for the $\beta$ variable [42]. Therefore, in Figure 3, effective potentials (27) for $\tau =0,1,2$ and 3 are plotted, respectively, as well as absolute energies (20) for ($\xi =1,2$; $\tau =0,1,2,3$) (full horizontal lines) for ground and $\beta$ bands.

From this figure, one can remark that our probability density distribution plots for the ground and $\beta$ bands reveal a fascinating progression of nuclear deformation with increasing total angular momentum. As the angular momentum evolves from $0^{+}$ to $6^{+}$, we observe a systematic shift in the y deformation across the potential’s energy landscape. The $0^{+}$ state is positioned above the less deformed minimum, while the $2^{+}$ and $4^{+}$ states are situated between the two potential minima. Notably, the $6^{+}$ state emerges above the more deformed minimum. This progression suggests a distinct shape-phase transition within the nuclear band, characterized by a progressive change in nuclear deformation as the angular momentum increases. Such a behavior provides critical insights into the complex nuclear structural dynamics and the interplay between nuclear shape and angular momentum. Our analysis of the probability density distributions reveals crucial information about the structural characteristics of the nuclear states. Notably, the absence of dual peaks for states in the ground band or triple peaks for states in the $\beta$ band suggests a lack of shape mixing within our theoretical framework. However, this does not preclude the presence of shape coexistence. Rather, the distinct y deformation values observed across different states indicate the existence of shape coexistence without mixing, a more subtle manifestation of the phenomenon. This finding represents an important distinction in nuclear structure physics, where shape coexistence can manifest without the characteristic wave-function mixing that typically produces multiple peaks in probability density distributions. The clear progression of deformation across states therefore supports a picture of shape coexistence where different shapes exist within the nucleus but maintain their separate identities in the eigenstates of the system.

Another interesting phenomenon, which can be described by the present model, is related to the so-called $B(E2)$ anomaly phenomenon characterized by sub-unitary values of $B_{4/2}=B(E2;4_1^{+}\to 2_1^{+})/B(E2;2_1^{+}\to 0_1^{+})$ or other unusually small $B(E2)$ transition ratios within the yrast band [43]. The phenomenon, observed already in many nuclei, was firstly described involving algebraic models [43,44,45,46,47,48] based on the Interacting Boson Model [49]. A first proof for the ability of the present model to reproduce such behavior was given in [50], where the sub-unitary $B(E2;8_1^{+}\to 6_1^{+})$ transition in ${}^{80}\mathrm{Ge}$ was very well reproduced. Another example is given in [34] for ${}^{44}\mathrm{Ca}$, where a significant back-bending in both energy and $B(E2;6_1^{+}\to 4_1^{+})$ transition is well described through the same mechanism, namely, assuming different ${\beta }_2$ deformations for the two states separated by a high barrier. Thus, even if the states belong to the same band, they manifest different deformations or collective rotation-vibration motion.

4. Conclusions

In this work, we have investigated the nuclear structure of ${}^{66}\mathrm{Se}$ with a particular focus on shape coexistence and transition phenomena using complementary theoretical approaches. Our findings lead to several important conclusions regarding the nature of deformation and collective behavior in this neutron-deficient nucleus. The potential energy surfaces in the $({\beta }_2,\gamma )$ plane are determined for the ground state involving Covariant Density Functional Theory calculations with the Density-Dependent Meson Exchange functional. The obtained results strongly support our description within the $\gamma$-unstable Bohr Hamiltonian with sextic potential.

Our phenomenological approach, involving the Bohr–Mottelson Hamiltonian with a sextic oscillator potential, successfully reproduced the experimental energy spectrum with remarkable accuracy. Particularly noteworthy is the excellent agreement for the low-lying $0_2^{+}$ state, a critical signature of shape coexistence, which our model places at 1212 keV compared to the experimental value of 1226 keV. This agreement, together with the overall reproduction of the ground-state band energies, strongly validates our theoretical framework. The absence of dual or triple peaks in the probability density distributions indicates a lack of significant shape mixing, yet the distinct deformation values observed across different states clearly support the existence of shape coexistence. This represents a subtle but important manifestation of the phenomenon, where different shapes coexist within the nucleus while maintaining their separate identities in the eigenstates of the system.