Composite Anti-Disturbance Static Output Control of Networked Nonlinear Markov Jump Systems with General Transition Probabilities Under Deception Attacks
Abstract
1. Problem Statement and Preliminaries
- Notation: The symmetric elements in a matrix are represented by ∗. means the operation of adding the matrix X and its transpose together. is the mathematical expectation of x. denotes the null space matrix of .
- The closed-loop system (9) with is stochastically stable;
- The augmented closed-loop system meets
- (a)
- , for all , ;
- (b)
- ;
- (c)
- such that
2. Main Results
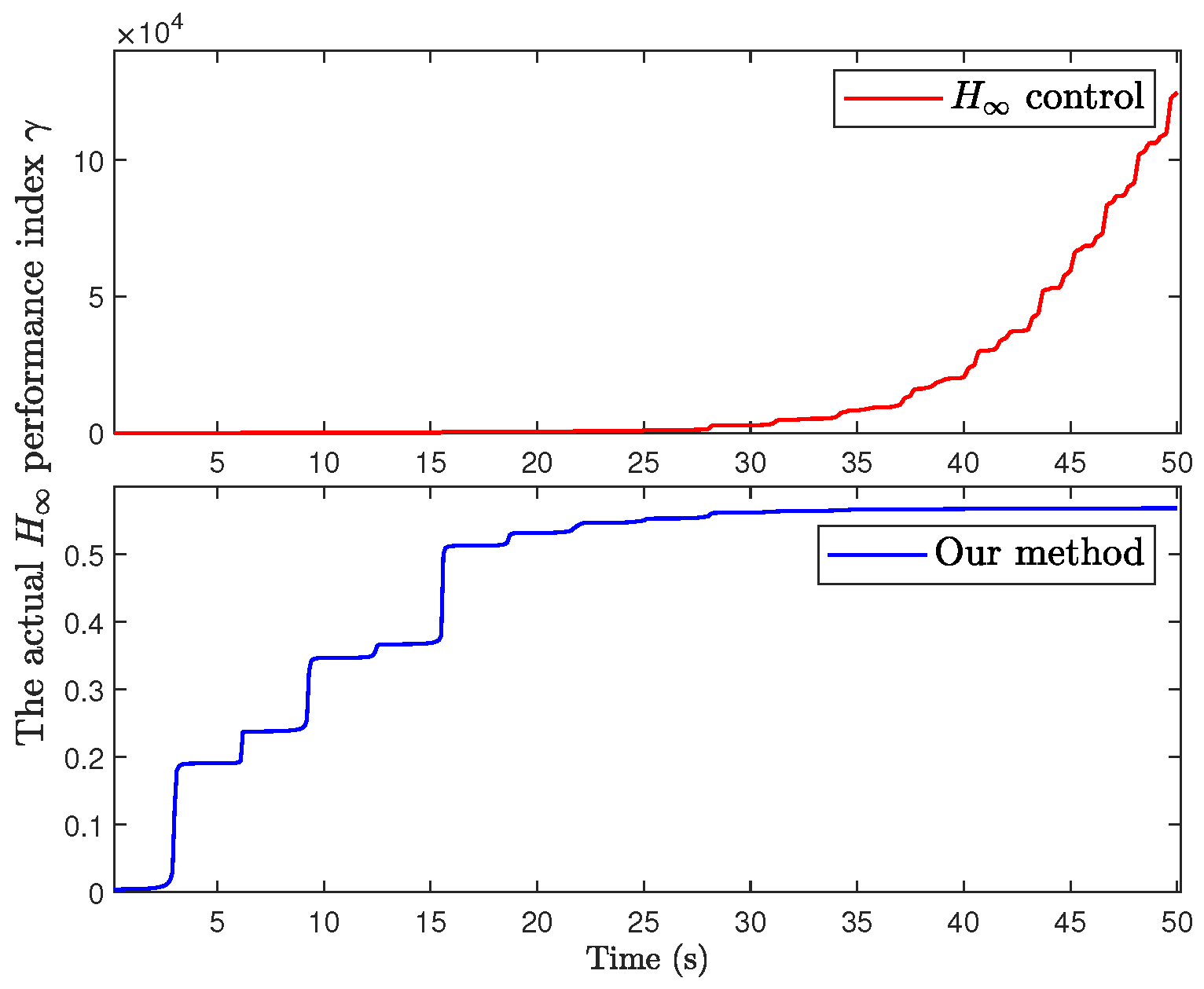
3. Simulation Results
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Shi, P.; Liu, M.; Zhang, L. Fault-tolerant sliding-mode-observer synthesis of Markovian jump systems using quantized measurements. IEEE Trans. Ind. Electron. 2015, 62, 5910–5918. [Google Scholar] [CrossRef]
- Wang, B.; Zhu, Q. The stabilization problem for a class of discrete-time semi-Markov jump singular systems. Automatica 2025, 171, 111960. [Google Scholar] [CrossRef]
- Tartaglione, G.; Ariola, M.; Amato, F. An observer-based output feedback controller for the finite-time stabilization of Markov jump linear systems. IEEE Control Syst. Lett. 2019, 3, 763–768. [Google Scholar] [CrossRef]
- Tan, Y.; Liu, J.; Xie, X.; Tian, E.; Liu, J. Dynamic-memory event-triggered sliding-mode secure control for nonlinear semi-Markov jump systems with stochastic cyber attacks. IEEE Trans. Autom. Sci. Eng. 2024, 21, 202–214. [Google Scholar] [CrossRef]
- Cao, Z.; Niu, Y.; Zou, Y. Adaptive neural sliding mode control for singular semi-Markovian jump systems against actuator attacks. IEEE Trans. Syst. Man Cybern. Syst. 2019, 51, 1523–1533. [Google Scholar] [CrossRef]
- Zong, G.; Ren, H. Guaranteed cost finite-time control for semi-Markov jump systems with event-triggered scheme and quantization input. Int. J. Robust Nonlinear Control 2019, 29, 5251–5273. [Google Scholar] [CrossRef]
- Shen, M.; Gu, Y.; Zhu, S.; Zong, G.; Zhao, X. Mismatched quantized H∞ output-feedback control of fuzzy Markov jump systems with a dynamic guaranteed cost triggering scheme. IEEE Trans. Fuzzy Syst. 2023, 32, 1681–1692. [Google Scholar] [CrossRef]
- Li, X.; Zhang, W.; Lu, D. Stability and stabilization analysis of Markovian jump systems with generally bounded transition probabilities. J. Frankl. Inst. 2020, 357, 8416–8434. [Google Scholar] [CrossRef]
- Zhang, L.; Boukas, E.K. Stability and stabilization of Markovian jump linear systems with partly unknown transition probabilities. Automatica 2009, 45, 463–468. [Google Scholar] [CrossRef]
- Shen, M.; Ye, D.; Wang, Q.G. Event-triggered H∞ filtering of Markov jump systems with general transition probabilities. Inf. Sci. 2017, 418, 635–651. [Google Scholar] [CrossRef]
- Liu, X.; Ma, G.; Pagilla, P.R.; Ge, S.S. Dynamic output feedback asynchronous control of networked Markovian jump systems. IEEE Trans. Syst. Man Cybern. Syst. 2018, 50, 2705–2715. [Google Scholar] [CrossRef]
- Antunes, D.J.; Qu, H. Frequency-domain analysis of networked control systems modeled by Markov jump linear systems. IEEE Trans. Control Netw. Syst. 2021, 8, 906–916. [Google Scholar] [CrossRef]
- Yan, S.; Gu, Z.; Park, J.H.; Shen, M. Fusion-based event-triggered H∞ state estimation of networked autonomous surface vehicles with measurement outliers and cyber-attacks. IEEE Trans. Intell. Transp. Syst. 2024, 25, 7541–7551. [Google Scholar] [CrossRef]
- Yan, S.; Gu, Z.; Park, J.H.; Xie, X.; Dou, C. Probability-density-dependent load frequency control of power systems with random delays and cyber-attacks via circuital implementation. IEEE Trans. Smart Grid 2022, 13, 4837–4847. [Google Scholar] [CrossRef]
- Guo, L.; Chen, W. Disturbance attenuation and rejection for systems with nonlinearity via DOBC approach. Int. J. Robust Nonlinear Control 2005, 15, 109–125. [Google Scholar] [CrossRef]
- Wang, B.; Zhu, Q.; Li, S. Stabilization of discrete-time hidden semi-Markov jump linear systems with partly unknown emission probability matrix. IEEE Trans. Autom. Control 2024, 69, 1952–1959. [Google Scholar] [CrossRef]
- Chen, M.; Shi, P.; Lim, C.C. Robust constrained control for MIMO nonlinear systems based on disturbance observer. IEEE Trans. Autom. Control 2015, 60, 3281–3286. [Google Scholar] [CrossRef]
- Ding, K.; Zhu, Q. Extended dissipative anti-disturbance control for delayed switched singular semi-Markovian jump systems with multi-disturbance via disturbance observer. Automatica 2021, 128, 109556. [Google Scholar] [CrossRef]
- Wang, F.; Gao, H.; Wang, K.; Zhou, C.; Zong, Q.; Hua, C. Disturbance observer-based finite-time control design for a quadrotor UAV with external disturbance. IEEE Trans. Aerosp. Electron. Syst. 2020, 57, 834–847. [Google Scholar] [CrossRef]
- Alhelou, H.H.; Parthasarathy, H.; Nagpal, N.; Agarwal, V.; Nagpal, H.; Siano, P. Decentralized stochastic disturbance observer-based optimal frequency control method for interconnected power systems with high renewable shares. IEEE Trans. Ind. Inform. 2021, 18, 3180–3192. [Google Scholar]
- Ding, S.; Hou, Q.; Wang, H. Disturbance-observer-based second-order sliding mode controller for speed control of PMSM drives. IEEE Trans. Energy Convers. 2022, 38, 100–110. [Google Scholar] [CrossRef]
- Liu, Y.; Wang, H.; Guo, L. Composite robust H∞ control for uncertain stochastic nonlinear systems with state delay via a disturbance observer. IEEE Trans. Autom. Control 2018, 63, 4345–4352. [Google Scholar] [CrossRef]
- Yao, X.; Guo, L. Composite anti-disturbance control for Markovian jump nonlinear systems via disturbance observer. Automatica 2013, 49, 2538–2545. [Google Scholar] [CrossRef]
- Zhao, Y.; Li, X.; Song, S. Observer-based sliding mode control for stabilization of mismatched disturbance systems with or without time delays. IEEE Trans. Syst. Man Cybern. Syst. 2021, 51, 7337–7345. [Google Scholar] [CrossRef]
- Hwang, S.; Kim, H.S. Extended disturbance observer-based integral sliding mode control for nonlinear system via T–S fuzzy model. IEEE Access 2020, 8, 116090–116105. [Google Scholar] [CrossRef]
- Yao, X.; Guo, L. Composite disturbance-observer-based output feedback control and passive control for Markovian jump systems with multiple disturbances. IET Control Theory Appl. 2014, 8, 873–881. [Google Scholar] [CrossRef]
- Boyd, S.P.; Ghaoui, L.E.; Feron, E.; Balakrishnan, V. Linear Matrix Inequalities in Systems and Control Theory; SIAM: Philadelphia, PA, USA, 1994. [Google Scholar]
- Wang, Y.; Xie, L.; de Souza, C.E. Robust control of a class of uncertain nonlinear systems. Syst. Control Lett. 1992, 19, 139–149. [Google Scholar] [CrossRef]
- Yan, S.; Gu, Z.; Park, J.H.; Xie, X. Sampled memory-event-triggered fuzzy load frequency control for wind power systems subject to outliers and transmission delays. IEEE Trans. Cybern. 2023, 53, 4043–4053. [Google Scholar] [CrossRef]
- Yao, X.; Zhang, L.; Zheng, W.X. Uncertain disturbance rejection and attenuation for semi-Markov jump systems with application to 2-degree-freedom robot arm. IEEE Trans. Circuits Syst. Regul. Pap. 2021, 68, 3836–3845. [Google Scholar] [CrossRef]
- Yan, S.; Gu, Z.; Ahn, C.K. Memory-event-triggered H∞ filtering of unmanned surface vehicles with communication delays. IEEE Trans. Circuits Syst. II Express Briefs 2021, 68, 2463–2467. [Google Scholar] [CrossRef]
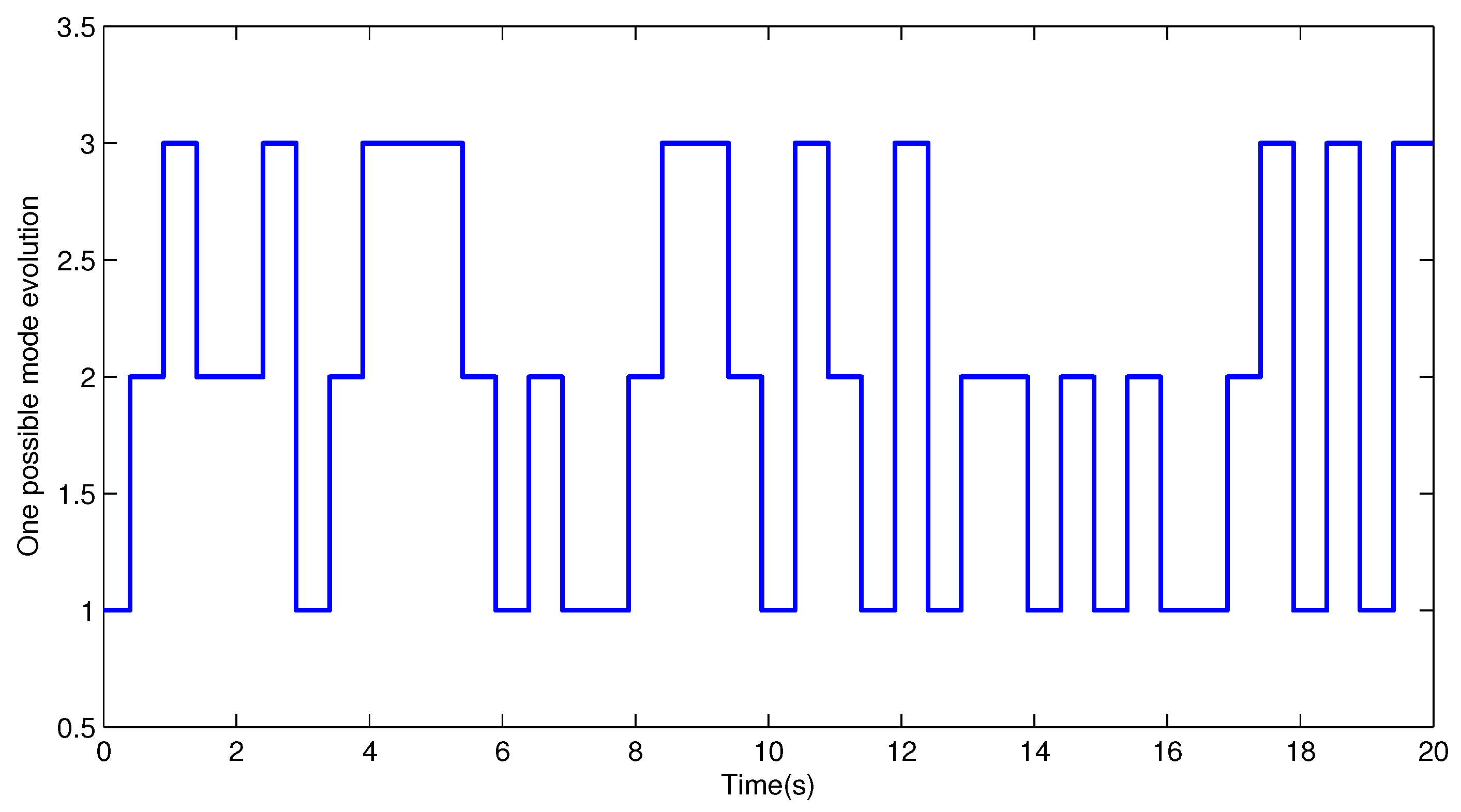
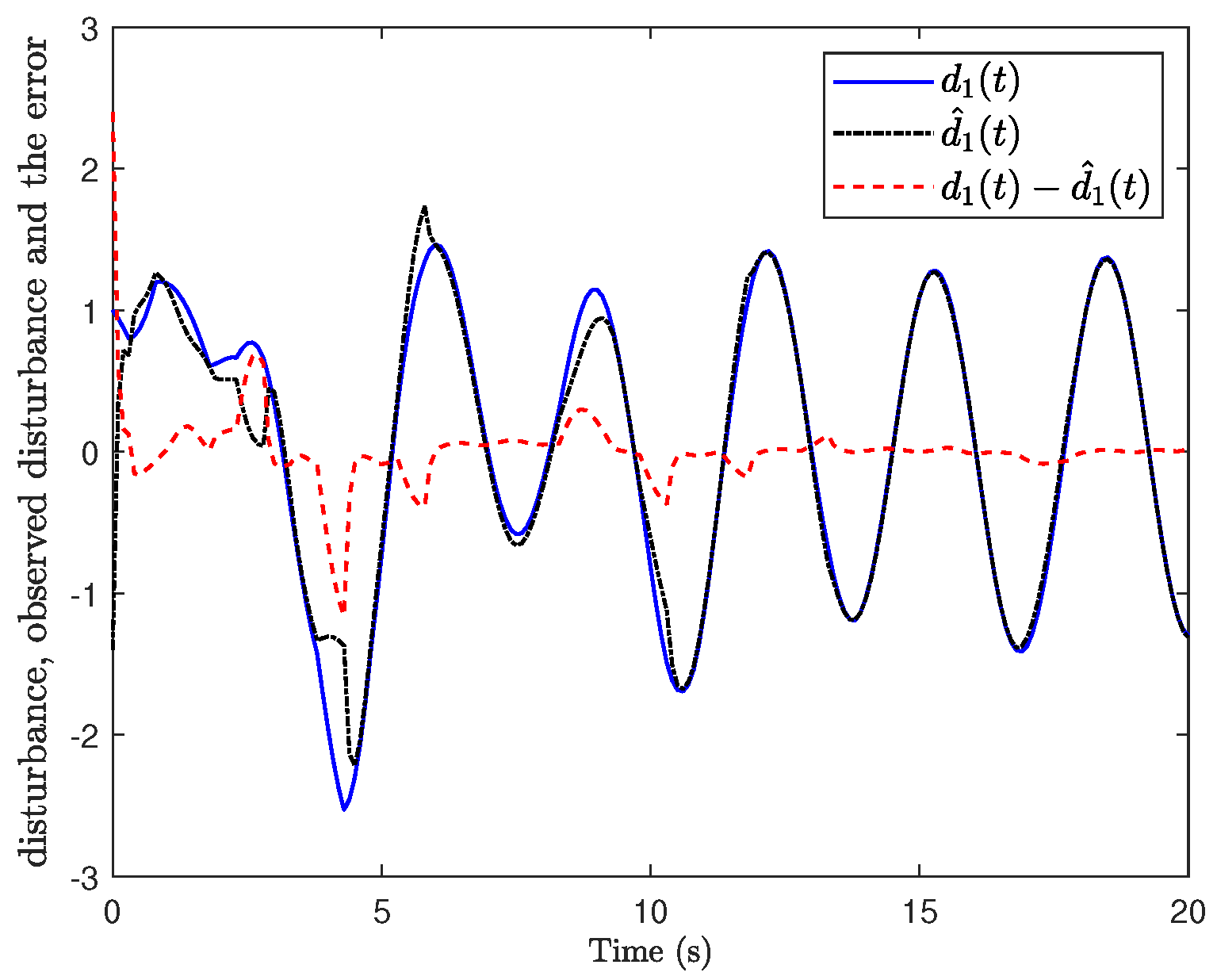
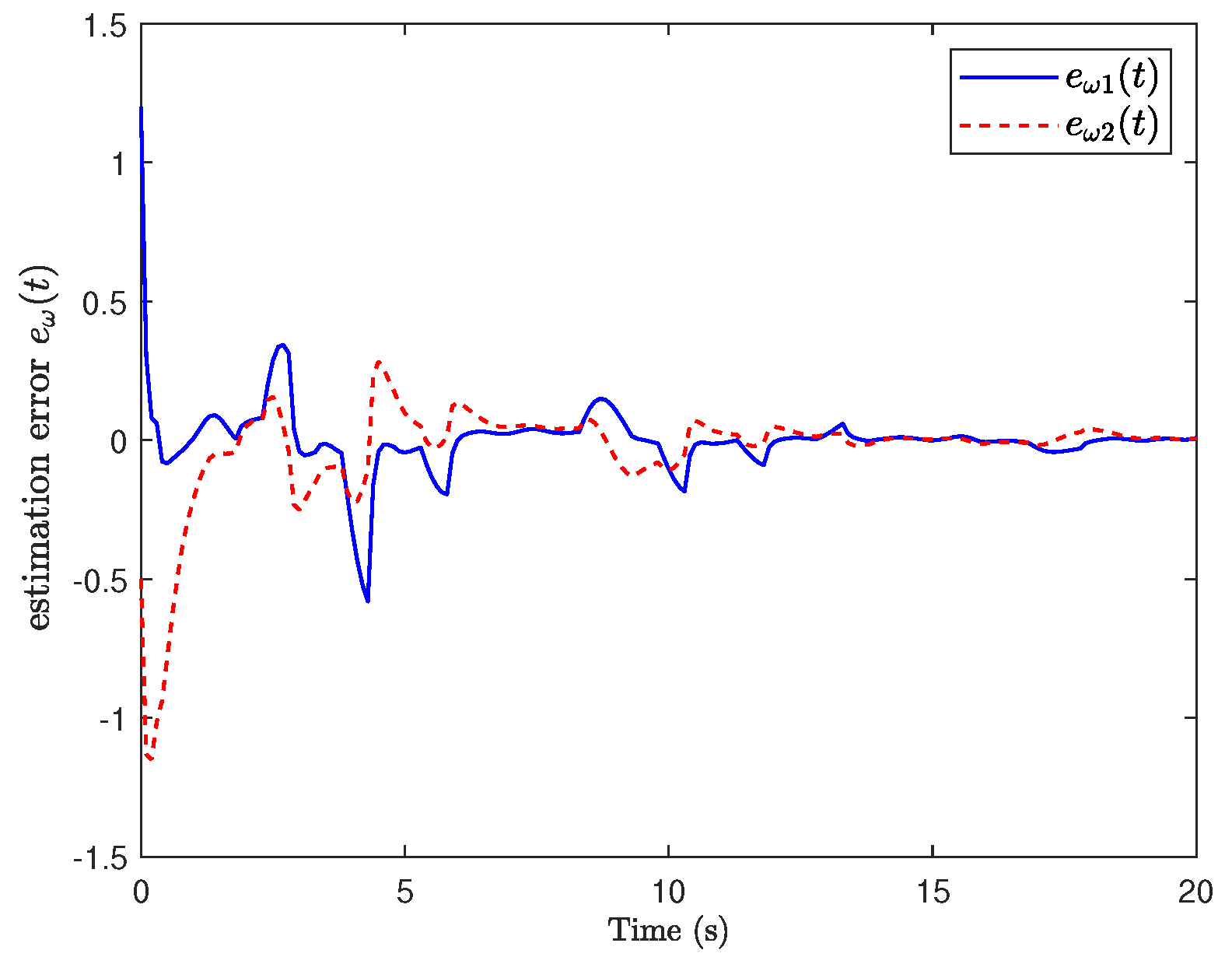

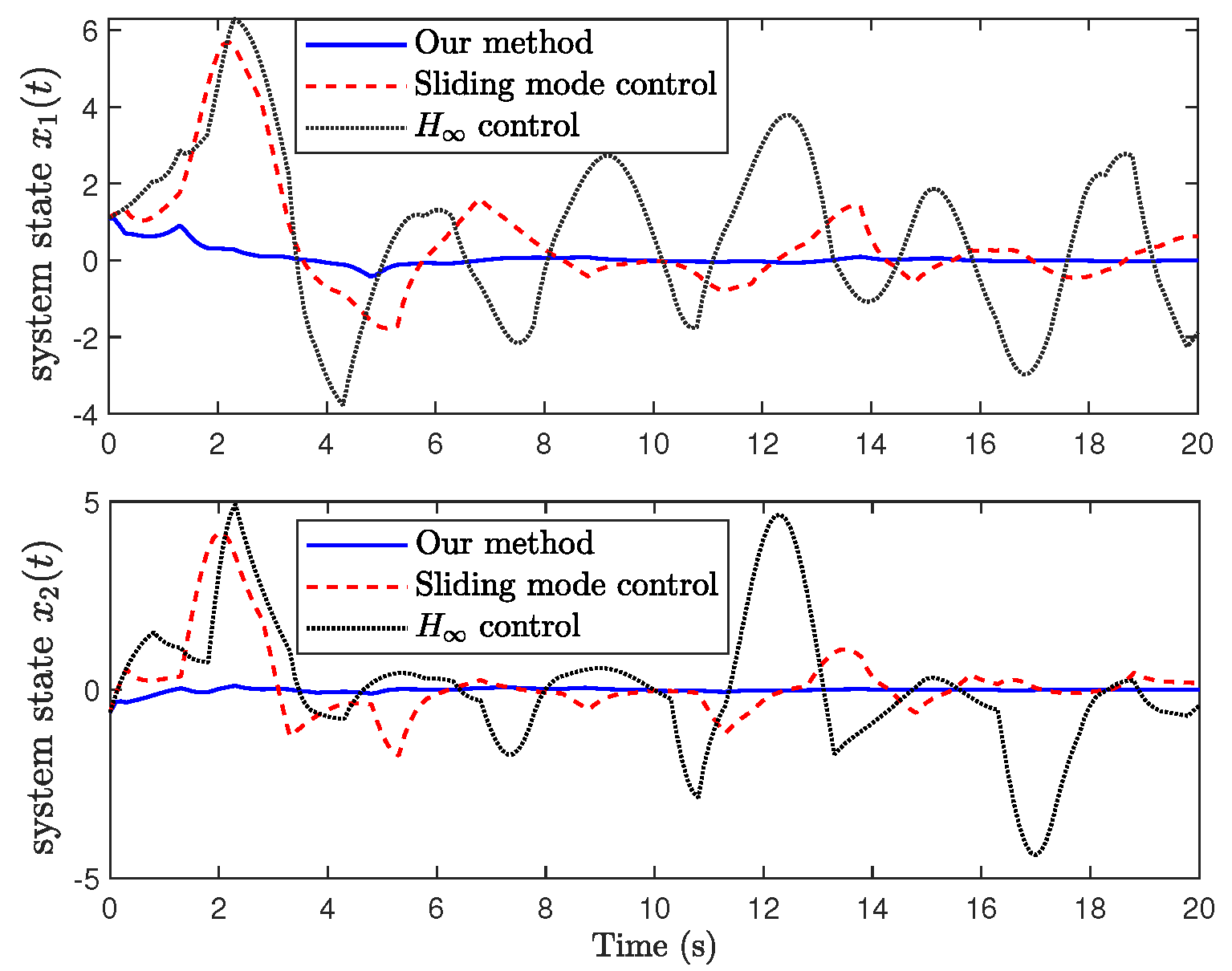

| 0.3 | 0.5 | 0.8 | |
|---|---|---|---|
| 0.6134 | 0.7852 | 0.9725 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lin, J.; Ding, L.; Yan, S. Composite Anti-Disturbance Static Output Control of Networked Nonlinear Markov Jump Systems with General Transition Probabilities Under Deception Attacks. Symmetry 2025, 17, 658. https://doi.org/10.3390/sym17050658
Lin J, Ding L, Yan S. Composite Anti-Disturbance Static Output Control of Networked Nonlinear Markov Jump Systems with General Transition Probabilities Under Deception Attacks. Symmetry. 2025; 17(5):658. https://doi.org/10.3390/sym17050658
Chicago/Turabian StyleLin, Jing, Liming Ding, and Shen Yan. 2025. "Composite Anti-Disturbance Static Output Control of Networked Nonlinear Markov Jump Systems with General Transition Probabilities Under Deception Attacks" Symmetry 17, no. 5: 658. https://doi.org/10.3390/sym17050658
APA StyleLin, J., Ding, L., & Yan, S. (2025). Composite Anti-Disturbance Static Output Control of Networked Nonlinear Markov Jump Systems with General Transition Probabilities Under Deception Attacks. Symmetry, 17(5), 658. https://doi.org/10.3390/sym17050658







