Force-Finding of Large-Scale Cable Net Glass Curtain Structures Using Inherent Symmetry and Nonlinear Structural Stiffness
Abstract
1. Introduction
2. Method
2.1. Geometric and Symmetry Representations of Cable Nets Described by Graph Products
2.2. Nonlinear Force-Finding Analysis Based on Overall Structural Stiffness
2.3. Flexible Boundary Conditions
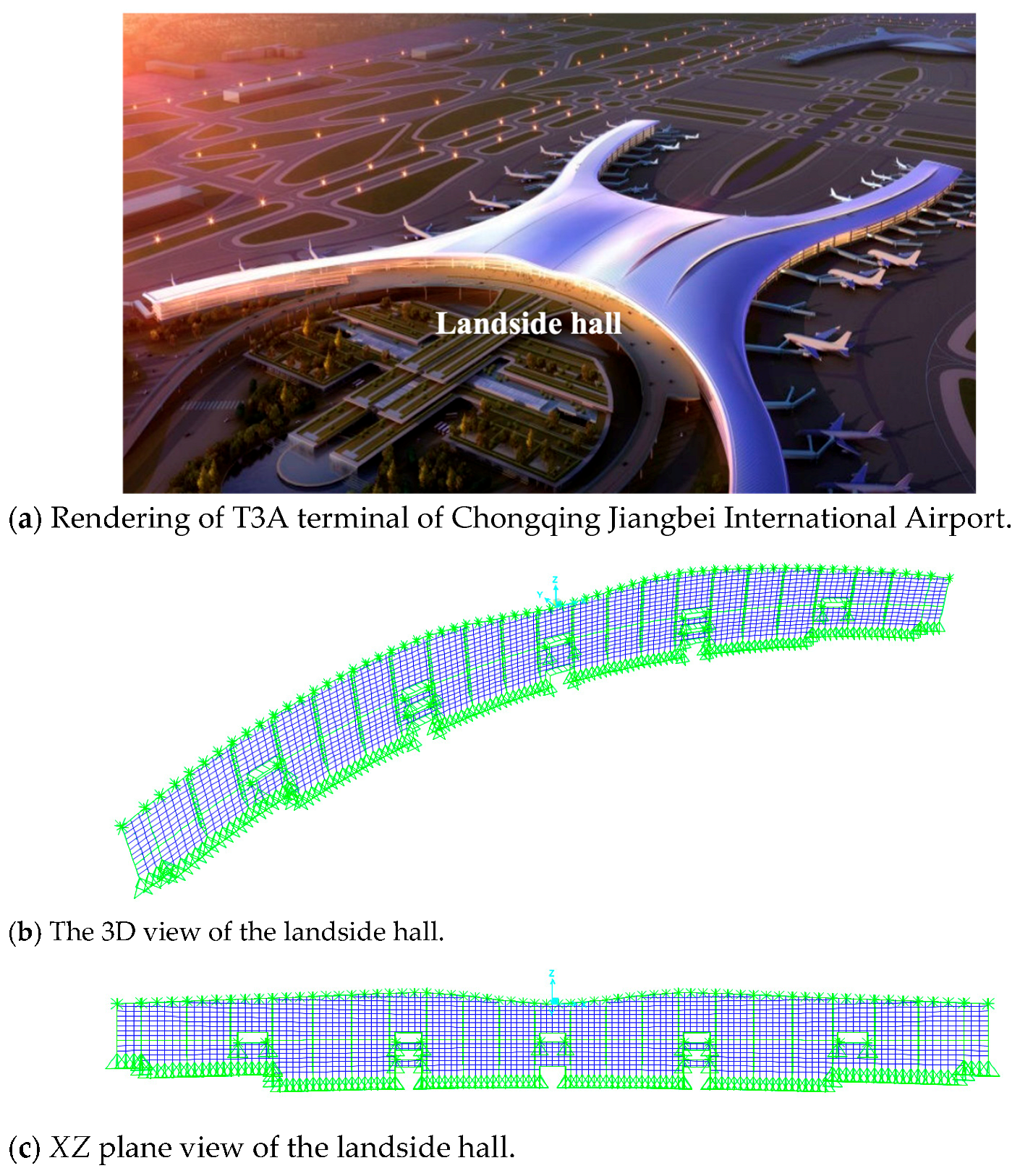
3. Engineering Example
3.1. Engineering Background
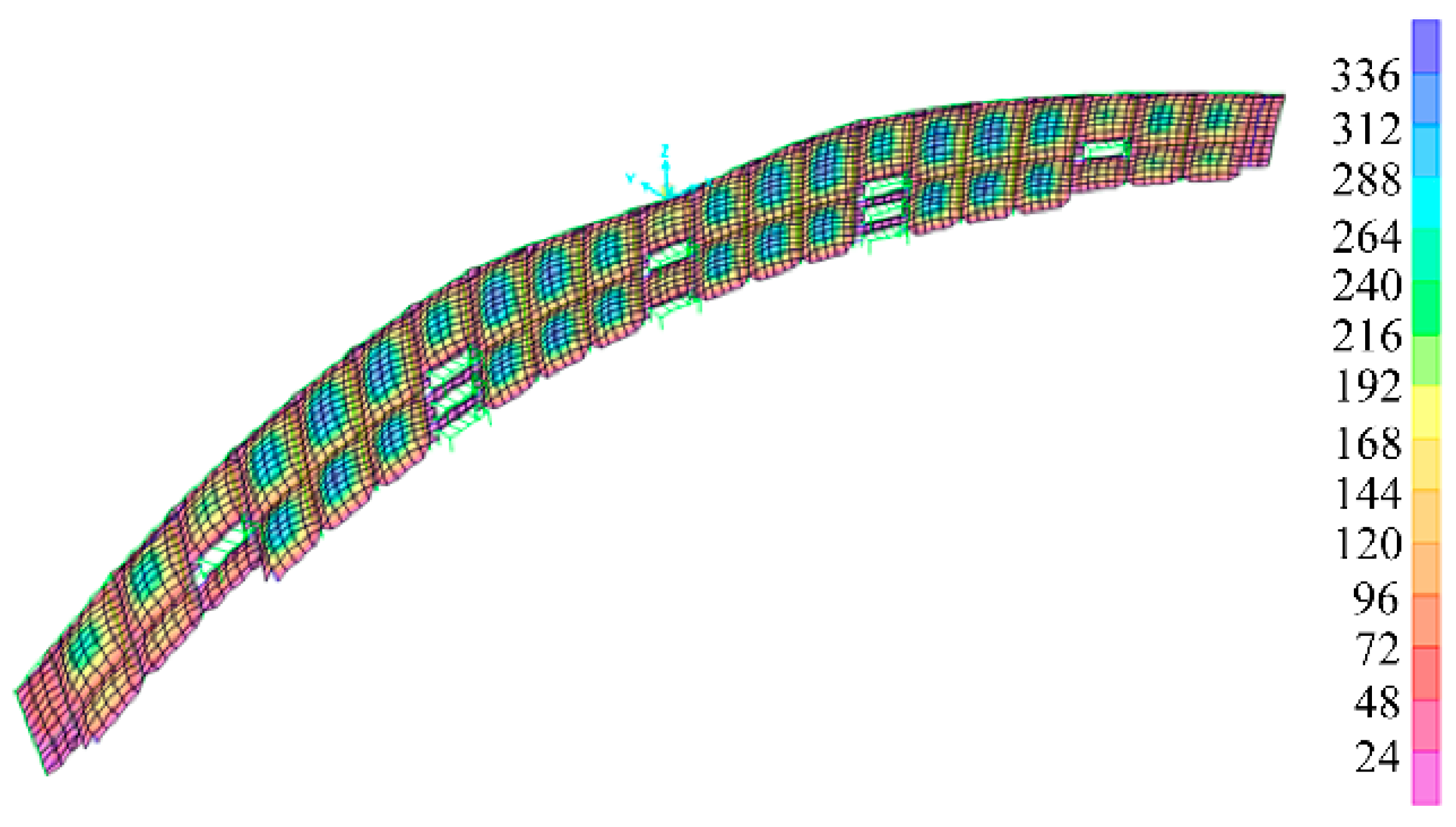
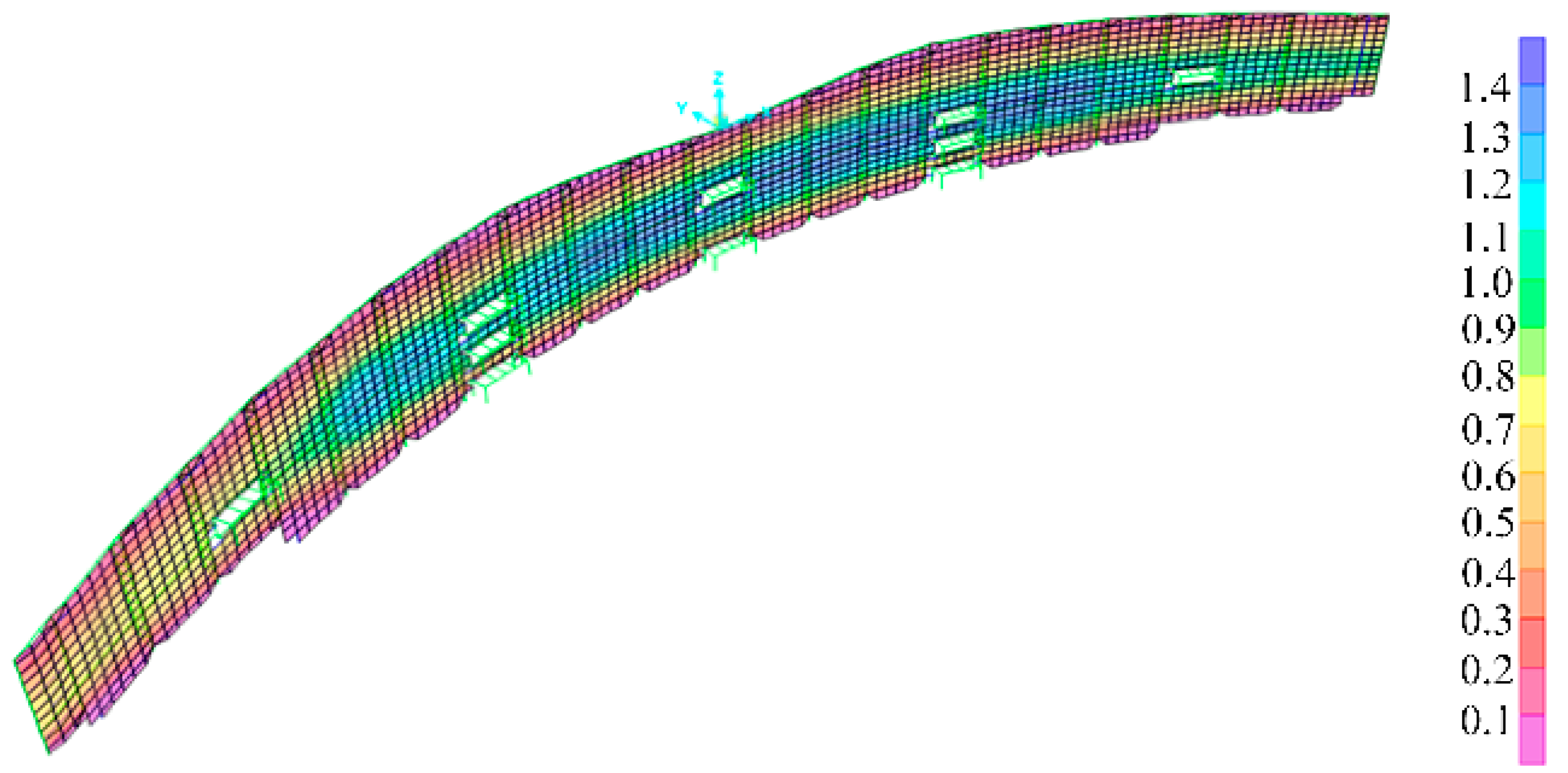
3.2. Structural Analysis Results
3.2.1. Modal Analysis
3.2.2. Lower-Order Natural Frequencies and Vibration Modes
3.2.3. Deformation Behavior
3.3. Flexible Boundaries and Supports
3.4. Initial Geometric Defects
4. Discussion
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Xiang, Y.; Luo, Y.; Guo, X.; Xiong, Z.; Shen, Z. A linearized approach for the seismic response analysis of flexible cable net structures. Soil. Dyn. Earthq. Eng. 2016, 88, 92–108. [Google Scholar] [CrossRef]
- Nie, R.; He, B.; Hodges, D.H.; Ma, X. Form finding and design optimization of cable network structures with flexible frames. Comput. Struct. 2019, 220, 81–91. [Google Scholar] [CrossRef]
- Tang, Y.; Li, T.; Hao, L.; Ma, X. Extended nonlinear force density method for form-finding of cable-membrane structures. J. Aerosp. Eng. 2017, 30, 04016101. [Google Scholar] [CrossRef]
- Dutta, S.; Ghosh, S. Form-finding of frame-supported tensile membrane structures using stochastic optimisation. Structures 2021, 32, 2211–2221. [Google Scholar] [CrossRef]
- Xian, X.; Luo, Y. Force finding of tensegrity systems using simulated annealing algorithm. J. Struct. Eng. 2010, 136, 1027–1031. [Google Scholar]
- Yamamoto, M.; Gan, B.S.; Fujita, K.; Kurokawa, J. A genetic algorithm based form-finding for tensegrity structure. Procedia Eng. 2011, 14, 2949–2956. [Google Scholar] [CrossRef]
- Koohestani, K. Form-finding of tensegrity structures via genetic algorithm. Int. J. Solids Struct. 2012, 49, 739–747. [Google Scholar] [CrossRef]
- Linkwitz, K.; Schek, H.J. Einige bemerkungen zur berechnung von vorgespannten seilnetzkonstruktionen. Ing.-Arch. 1971, 40, 145–158. [Google Scholar] [CrossRef]
- Schek, H.J. The force density method for form finding and computation of general networks. Comput. Methods Appl. Mech. Eng. 1974, 3, 115–134. [Google Scholar] [CrossRef]
- Liew, A. Constrained force density method optimisation for compression-only shell structures. Structures 2020, 28, 1845–1856. [Google Scholar] [CrossRef]
- Zhao, Z.; Yu, D.; Zhang, T.; Cai, Q. Intelligent design algorithm for branching structures based on updated force density method. J. Build. Eng. 2022, 57, 104858. [Google Scholar] [CrossRef]
- Aboul-Nasr, G.; Mourad, S.A. An extended force density method for form finding of constrained cable nets. Case Stud. Struct. Eng. 2015, 3, 19–32. [Google Scholar] [CrossRef]
- Maurin, B.; Motro, R. The surface stress density method as a form-finding tool for tensile membranes. Eng. Struct. 1998, 20, 712–719. [Google Scholar] [CrossRef]
- Hayashi, K.; Ohsaki, M. FDMopt: Force density method for optimal geometry and topology of trusses. Adv. Eng. Softw. 2019, 133, 12–19. [Google Scholar] [CrossRef]
- Luo, X.Q.; Zhang, Q.L.; Chen, L. Form-finding of a mixed structure with cable nets and tubular trusses. J. Constr. Steel Res. 2012, 72, 192–202. [Google Scholar] [CrossRef]
- Chen, Y.; Sun, Q.; Feng, J. Improved form-finding of tensegrity structures using blocks of symmetry-adapted force density matrix. J. Struct. Eng. 2018, 144, 04018174. [Google Scholar] [CrossRef]
- Zhang, S.; Zhang, S.; Zhang, Y.; Ye, J. Force density sensitivity form-finding design method for cable-mesh reflector antennas considering interactive effects between cable network and supporting truss. Eng. Struct. 2021, 244, 112722. [Google Scholar] [CrossRef]
- Ye, J.; Feng, R.; Zhou, S.; Tian, J. The modified force-density method for form-finding of membrane structures. Int. J. Steel Struct. 2012, 12, 299–310. [Google Scholar] [CrossRef]
- Zhao, Z.; Yu, D.; Zhang, T.; Gao, H. Form-finding and optimization for free form grid structures supported by branching columns based on updated force density method. Structures 2022, 44, 1190–1203. [Google Scholar] [CrossRef]
- Otter, J.R.H. Computations for prestressed concrete reactor pressure vessels using dynamic relaxation. Nucl. Struct. Eng. 1965, 1, 61–75. [Google Scholar] [CrossRef]
- Boulaud, R.; Douthe, C.; Sab, K. Modelling of curtain effect in rockfall barrier with the dynamic relaxation. Int. J. Solids Struct. 2020, 200–201, 297–312. [Google Scholar] [CrossRef]
- Collins, M.; Cosgrove, T. Dynamic relaxation modelling of braced bending active gridshells with rectangular sections. Eng. Struct. 2019, 187, 16–24. [Google Scholar] [CrossRef]
- Wang, X.; Cai, J.; Yang, R.; Feng, J. Form-finding of deployable mesh reflectors using dynamic relaxation method. Acta Astronaut. 2018, 151, 380–388. [Google Scholar] [CrossRef]
- Barnes, M.R. Form-finding and analysis of prestressed nets and membranes. Comput. Struct. 1988, 30, 685–695. [Google Scholar] [CrossRef]
- Rezaiee-Pajand, M.; Mohammadi-Khatami, M. Nonlinear analysis of cable structures using the dynamic relaxation method. Front. Struct. Civ. Eng. 2021, 15, 253–274. [Google Scholar] [CrossRef]
- Li, N.; Zong, W.; Fan, Y.; Lu, J. Form-finding optimization methods for free-form reticulated shells: Reverse realization and numerical simulation. Adv. Mech. Eng. 2017, 9, 1687814017737256. [Google Scholar] [CrossRef]
- Tu, G.; Chen, C.; Gong, Z.; Wang, Y. A form-finding method for branching structures based on dynamic relaxation. Appl. Sci. 2021, 11, 7165. [Google Scholar] [CrossRef]
- Bagrianski, S.; Halpern, A.B. Form-finding of compressive structures using Prescriptive Dynamic Relaxation. Comput. Struct. 2014, 132, 65–74. [Google Scholar] [CrossRef]
- Bessini, J.; Lázaro, C.; Monleón, S. A form-finding method based on the geometrically exact rod model for bending-active structures. Eng. Struct. 2017, 152, 549–558. [Google Scholar] [CrossRef]
- Haug, E.; De Kermel, P.; Gawenat, B.; Michalski, A. Industrial design and analysis of structural membranes. Int. J. Space Struct. 2009, 24, 191–204. [Google Scholar] [CrossRef]
- Wan, Z.; Ohlbrock, P.O.; D’Acunto, P.; Cao, Z.; Fan, F.; Schwartz, J. A form-finding approach for the conceptual design of air-supported structures using 3D graphic statics. Comput. Struct. 2021, 243, 106401. [Google Scholar] [CrossRef]
- Chen, D.; Zhang, Y.; Qian, H.; Wang, H.; Jin, X. Numerical approach for simulating the tensioning process of complex prestressed cable-net structures. J. Civ. Eng. Manag. 2021, 27, 571–578. [Google Scholar] [CrossRef]
- Zhu, W.; Ge, Y.; Fang, G.; Cao, J. A novel shape finding method for the main cable of suspension bridge using nonlinear finite element approach. Appl. Sci. 2021, 11, 4644. [Google Scholar] [CrossRef]
- Arcaro, V.; Adeli, H. Form-finding and analysis of hyperelastic tensegrity structures using unconstrained nonlinear programming. Eng. Struct. 2019, 191, 439–446. [Google Scholar] [CrossRef]
- Argyris, J.H.; Angelopoulos, T.; Bichat, B. A general method for the shape finding of lightweight tension structures. Comput. Methods Appl. Mech. Eng. 1974, 3, 135–149. [Google Scholar] [CrossRef]
- Lu, J.; Dong, X.; Zhao, X.; Wu, X.; Shu, G. Form-finding analysis for a new type of cable-strut tensile structures generated by semi-regular tensegrity. Adv. Struct. Eng. 2017, 20, 772–783. [Google Scholar] [CrossRef]
- Chen, Y.; Feng, J.; Wu, Y. Novel form-finding of tensegrity structures using ant colony systems. J. Mech. Robot. 2012, 4, 031001. [Google Scholar] [CrossRef]
- Chen, Y.; Feng, J.; Wu, Y. Prestress stability of pin-jointed assemblies using ant colony systems. Mech. Res. Commun. 2012, 41, 30–36. [Google Scholar] [CrossRef]
- Kabasi, S.; Marbaniang, A.L.; Ghosh, S. Physics-informed neural networks for the form-finding of tensile membranes by solving the Euler–Lagrange equation of minimal surfaces. Thin Walled Struct. 2023, 182, 110309. [Google Scholar] [CrossRef]
- Sareh, P. The least symmetric crystallographic derivative of the developable double corrugation surface: Computational design using underlying conic and cubic curves. Mater. Des. 2019, 183, 108128. [Google Scholar] [CrossRef]
- Chen, Y.; Feng, J.; Ma, R.; Zhang, Y. Efficient symmetry method for calculating integral prestress modes of statically indeterminate cable-strut structures. J. Struct. Eng. 2015, 141, 04014240. [Google Scholar] [CrossRef]
- Chen, Y.; Yan, J.; Sareh, P.; Feng, J. Feasible prestress modes for cable-strut structures with multiple self-stress states using particle swarm optimization. J. Comput. Civ. Eng. 2020, 34, 04020003. [Google Scholar] [CrossRef]
- Zhang, L.Y.; Li, Y.; Cao, Y.P.; Feng, X.Q. Stiffness matrix based form-finding method of tensegrity structures. Eng. Struct. 2014, 58, 36–48. [Google Scholar] [CrossRef]
- Fan, L.; Xu, R.; Shi, P.; Feng, X.; Chen, Y. Simplified form-finding for tensegrity structures through reference joints of symmetry orbits. Structures 2023, 49, 1157–1167. [Google Scholar] [CrossRef]
- Zhang, J.Y.; Guest, S.D.; Ohsaki, M. Symmetric prismatic tensegrity structures. Part I: Configuration and stability. Int. J. Solids Struct. 2009, 46, 1–14. [Google Scholar] [CrossRef]
- Liu, H.; Zhang, W.; Yuan, H.; Zhu, J.; Zheng, J. Modified double-control form-finding analysis for suspendomes considering the construction process and the friction of cable-strut joints. Eng. Struct. 2016, 120, 75–81. [Google Scholar] [CrossRef]
- Wallin, M.; Ristinmaa, M. Topology optimization utilizing inverse motion based form finding. Comput. Methods Appl. Mech. Eng. 2015, 289, 316–331. [Google Scholar] [CrossRef]
- Chen, Y.; Zhou, H.; Gao, J.; Shen, Z.; Xie, T.; Sareh, P. Accurate force evaluation in prestressed cable-strut structures: A robust sparse bayesian learning method with feedback-driven error optimization. Eng. Struct. 2025, 330, 119878. [Google Scholar] [CrossRef]
- Zhang, J.Y.; Ohsaki, M. Force identification of prestressed pin-jointed structures. Comput. Struct. 2011, 89, 2361–2368. [Google Scholar] [CrossRef]
- Quagliaroli, M.; Malerba, P.G.; Albertin, A.; Pollini, N. The role of prestress and its optimization in cable domes design. Comput. Struct. 2015, 161, 17–30. [Google Scholar] [CrossRef]
- Serra, M.; Shahbazian, A.; Da Silva, L.S.; Marques, L.; Rebelo, C.; Vellasco, P.C.G.d.S. A full scale experimental study of prestressed stayed columns. Eng. Struct. 2015, 100, 490–510. [Google Scholar] [CrossRef]




| C2v | E | σx | σy | C2 |
|---|---|---|---|---|
| ΓT | 3 | 1 | 1 | -1 |
| Γ(j) | 195 | 15 | 13 | 1 |
| Γ(b) | 362 | 14 | 12 | 0 |
| Γ(k) | 96 | 2 | 2 | 0 |
| Γ(m) − Γ(s) | 127 | −1 | −1 | −1 |
| Material | Elastic Modulus (N/mm2) | Poisson’s Ratio | Linear Expansion Coefficient | Weight (N/mm3) | Yield Strength (N/mm2) |
|---|---|---|---|---|---|
| Stainless steel cable | 1.3 × 105 | 0.3 | 1.8 × 10−5 | 7.85 × 10−5 | 1200 (tension only) |
| Q345 steel | 2.0 × 105 | 1.17 × 10−5 | 345 |
| Location | Fx/kN | Fy/kN | Fz/kN |
|---|---|---|---|
| Bottom of columns on both sides | 1297 | 201 | 1647 |
| Bottom of wind-resistant truss columns | 73 | 428 | 2822 |
| Vertical cables connected to the bottom | 4 | 24 | 310 |
| Cross-cables | 401 | 29 | 7 |
| Type of Defect | Dmax/mm | Ftop/kN | Fi/kN | Fu/kN |
|---|---|---|---|---|
| No initial defect | 336 | 411 | 157–224 | 54–401 |
| The initial defect is L/1000 | 338 | 416 | 159–230 | 45–420 |
| The initial defect is L/300 | 385 | 427 | 153–236 | 40–429 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Fan, L.; Li, Y.; Chen, Y.; Feng, X.; Gao, J.; Cao, X.; Feng, J. Force-Finding of Large-Scale Cable Net Glass Curtain Structures Using Inherent Symmetry and Nonlinear Structural Stiffness. Symmetry 2025, 17, 643. https://doi.org/10.3390/sym17050643
Fan L, Li Y, Chen Y, Feng X, Gao J, Cao X, Feng J. Force-Finding of Large-Scale Cable Net Glass Curtain Structures Using Inherent Symmetry and Nonlinear Structural Stiffness. Symmetry. 2025; 17(5):643. https://doi.org/10.3390/sym17050643
Chicago/Turabian StyleFan, Linzi, Yixin Li, Yao Chen, Xiaodong Feng, Jiangjun Gao, Xingwang Cao, and Jian Feng. 2025. "Force-Finding of Large-Scale Cable Net Glass Curtain Structures Using Inherent Symmetry and Nonlinear Structural Stiffness" Symmetry 17, no. 5: 643. https://doi.org/10.3390/sym17050643
APA StyleFan, L., Li, Y., Chen, Y., Feng, X., Gao, J., Cao, X., & Feng, J. (2025). Force-Finding of Large-Scale Cable Net Glass Curtain Structures Using Inherent Symmetry and Nonlinear Structural Stiffness. Symmetry, 17(5), 643. https://doi.org/10.3390/sym17050643





