On Symmetry Properties of Tensors for Electromagnetic Deformable Solids
Abstract
1. Introduction
Notation
2. Balance Equations and Symmetry in Electromagnetic Solids
2.1. Electromagnetic Fields and Forces in Matter
2.2. Balance of Linear and Angular Momentum
2.3. Remarks on the Symmetry Condition
3. Balance of Energy and Second Law of Thermodynamics
- The postulate of the second law. The admissible thermodynamic processes are those satisfying the balance equations and the inequality
4. Lagrangian and Eulerian Fields Versus the Symmetry Condition
4.1. Stress Tensor and Symmetry Conditions
4.2. Lagrangian Fields Versus the Symmetry Condition
4.3. Electromagnetic Interactions in Micropolar Media
5. Constitutive Models with the Field
5.1. Solutions to the Thermodynamic Restriction (43)
5.2. Solutions to the Thermodynamic Restriction (44)
6. Models for Positive and Negative Magnetostriction
7. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A. Some Notes on Vector and Tensor Algebra
Appendix B. Lagrangian Fields and Euclidean Invariance
References
- Fano, R.M.; Chu, L.J.; Adler, R.B. Electromagnetic Fields, Energy, and Forces; MIT Press: Cambridge MA, USA, 1968. [Google Scholar]
- Maugin, G.A. Continuum Mechanics of Electromagnetic Solids; North-Holland: Amsterdam, The Netherlands, 1988. [Google Scholar]
- Pao, Y.-H.; Hutter, K. Electrodynamics for moving elastic solids and viscous fluids. Proc. IEEE 1975, 63, 1011–1021. [Google Scholar]
- Dorfmann, L.; Ogden, R.W. The nonlinear theory of magnetoelasticity and the role of the Maxwell stress: A review. Proc. R. Soc. A 2023, 479, 592. [Google Scholar] [CrossRef]
- Spaldin, N.A. A beginner’s guide to the modern theory of polarization. J. Solid State Chem. 2012, 195, 2–10. [Google Scholar] [CrossRef]
- Griffiths, D.J. Introduction to Electrodynamics; Cambridge University Press: Cambridge, UK, 2024. [Google Scholar]
- Gurtin, M.E.; Fried, E.; Anand, L. The Mechanics and Thermodynamics of Continua; Cambridge University Press: Cambridge, UK, 2010. [Google Scholar]
- Kovetz, A. Electromagnetic Theory; Oxford University Press: Oxford, UK, 2000. [Google Scholar]
- Morro, A.; Giorgi, C. Mathematical Methods of Continuum Physics; Birkhäuser: Cham, Switzerland, 2023. [Google Scholar]
- Bustamante, R.; Dorfmann, A.; Ogden, R.W. On electric body forces and Maxwell stresses in nonlinearly electroelastic solids. Int. J. Eng. Sci. 2009, 47, 1131–1141. [Google Scholar] [CrossRef]
- Bustamante, R.; Rajagopal, K.R. On a new class of electroelastic bodies. I. Proc. R. Soc. A 2013, 469, 521–536. [Google Scholar] [CrossRef]
- Dorfmann, A.; Ogden, R.W. Nonlinear magnetoelastic deformations. Q. J. Mech. Appl. Math. 2004, 57, 599–622. [Google Scholar] [CrossRef]
- Saxena, P.; Hossain, M.; Steinmann, P. Nonlinear magneto-viscoelasticity of transversally isotropic magnetoactive polymers. Proc. R. Soc. A 2014, 470, 82–104. [Google Scholar] [CrossRef]
- Müller, I. On the entropy inequality. Arch. Ration. Mech. Anal. 1967, 26, 118–141. [Google Scholar] [CrossRef]
- Casas-Vázquez, J.; Jou, D. Temperature in non-equilibrium states: A review of open problems and current proposals. Rep. Prog. Phys. 2003, 66, 1937–2023. [Google Scholar] [CrossRef]
- Gujrati, P.D. Irreversibility, dissipation, and its measure. Symmetry 2025, 17, 232. [Google Scholar] [CrossRef]
- Dorfmann, L.; Ogden, R.W. Hard-magnetic soft magnetoelastic materials: Energy considerations. Int. J. Solids Struct. 2024, 294, 112789. [Google Scholar] [CrossRef]
- Straughan, B. Heat Waves; Springer: Berlin/Heidelberg, Germany, 2011. [Google Scholar]
- Gazda, P.; Nowicki, M.; Szewczyk, R. Comparison of stress-impedance effect in amorphous ribbons with positive and negative magnetostriction. Materials 2019, 12, 275. [Google Scholar] [CrossRef]
- Bienkowski, A. Magnetoelastic Villari effect in Mn-Zn ferrites. J. Magn. Magn. Mater. 2000, 215, 231–233. [Google Scholar] [CrossRef]
- Narita, F.; Fox, M.A. A review on piezoelectric, magnetostrictive, and magnetoelectric materials and device technologies for energy harvesting applications. Adv. Eng. Mater. 2018, 20, 1700743. [Google Scholar] [CrossRef]
- Gazda, P.; Nowicki, M. Giant stress-impedance effect in CoFeNiNoBSi alloy in variation of applied magnetic field. Materials 2021, 14, 1919. [Google Scholar] [CrossRef]
- Bieńkowski, A.; Kulikowski, J. Effect of stresses on the magnetostriction of Ni-Zn(Co) ferrites. J. Magn. Magn. Mater. 1991, 101, 122–124. [Google Scholar] [CrossRef]
- Daniel, L.; Rekik, M.; Hubert, O. A multiscale model for magneto-elastic behaviour including hysteresis effects. Arch. Appl. Mech. 2014, 84, 1307–1323. [Google Scholar] [CrossRef]
- Hubert, O. Multiscale magneto-elastic modeling of magnetic materials including isotropic second order stress effect. J. Magn. Magn. Mater. 2019, 491, 165564. [Google Scholar] [CrossRef]
- Szewczyk, R. Stress-induced anisotropy and stress dependence of saturation magnetostriction in the Jiles-Atherton-Sablik model of the magnetoelastic Villari effect. Arch. Metall. Mater. 2016, 61, 607–612. [Google Scholar] [CrossRef]
- Giorgi, C.; Morro, A. On the Modelling of Magneto-Mechanical Effects in Solids. Available online: https://papers.ssrn.com/sol3/papers.cfm?abstract_id=4750363 (accessed on 6 May 2024).
- Riesgo, G.; Elbaile, L.; Carrizo, J.; Crespo, R.D.; García, M.Á.; Torres, Y.; García, J.Á. Villari effect at low strain in magnetoactive materials. Materials 2020, 13, 2472. [Google Scholar] [CrossRef] [PubMed]
- Dorfmann, L.; Ogden, R.W. Nonlinear electroelasticity: Material properties, continuum theory and applications. Proc. R. Soc. A 2017, 473, 311. [Google Scholar] [CrossRef] [PubMed]
- Wang, B.; Kari, L. Constitutive model of isotropic magneto-sensitive rubber with amplitude, frequency, magnetic and temperature dependence under a continuum mechanics basis. Polymers 2021, 13, 472. [Google Scholar] [CrossRef] [PubMed]
- Mehnert, M.; Hossain, M.; Steinmann, P. Numerical modeling of thermo-electro-viscoelasticity with field-dependent material parameters. Int. J. Non-Linear Mech. 2018, 106, 13–24. [Google Scholar] [CrossRef]
- Giorgi, C.; Morro, A. Electrostriction and modelling of finitely deformable dielectrics. Acta Mech. 2024, 236, 229–240. [Google Scholar] [CrossRef]
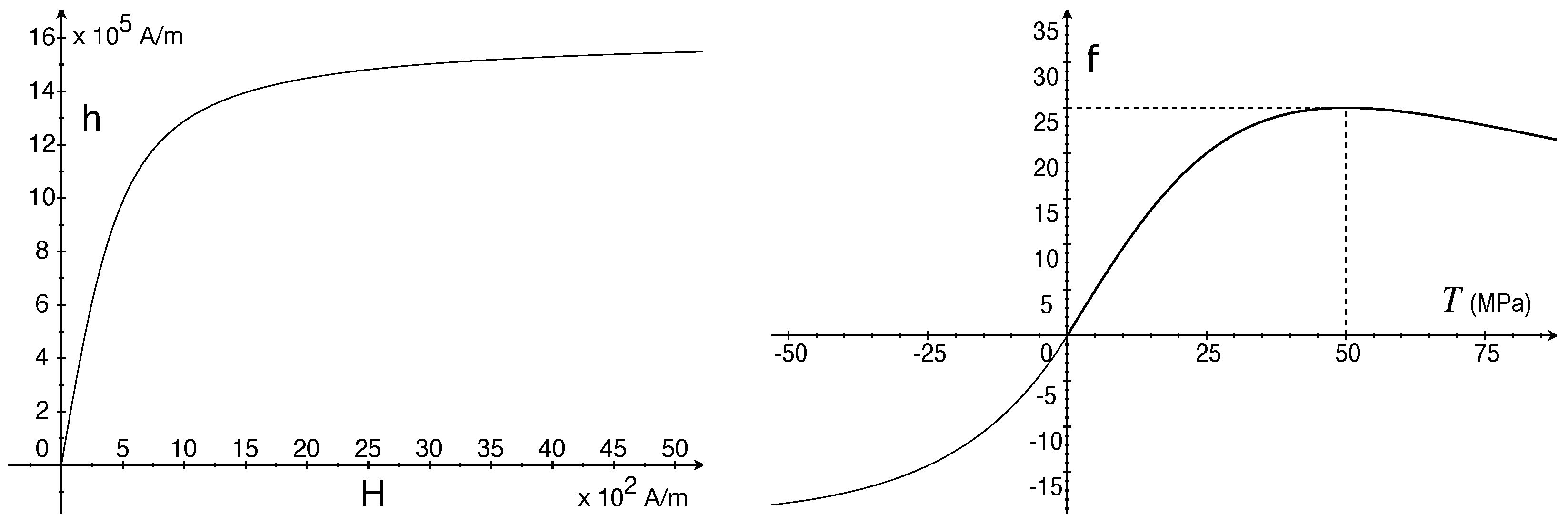

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Morro, A.; Giorgi, C. On Symmetry Properties of Tensors for Electromagnetic Deformable Solids. Symmetry 2025, 17, 557. https://doi.org/10.3390/sym17040557
Morro A, Giorgi C. On Symmetry Properties of Tensors for Electromagnetic Deformable Solids. Symmetry. 2025; 17(4):557. https://doi.org/10.3390/sym17040557
Chicago/Turabian StyleMorro, Angelo, and Claudio Giorgi. 2025. "On Symmetry Properties of Tensors for Electromagnetic Deformable Solids" Symmetry 17, no. 4: 557. https://doi.org/10.3390/sym17040557
APA StyleMorro, A., & Giorgi, C. (2025). On Symmetry Properties of Tensors for Electromagnetic Deformable Solids. Symmetry, 17(4), 557. https://doi.org/10.3390/sym17040557






