Characteristic Analysis of Local Wave Solutions for the (21)-Dimensional Asymmetric Nizhnik–Novikov–Veselov Equation
Abstract
1. Introduction
2. KP Hierarchy Reduction Method
3. Local Wave Solutions
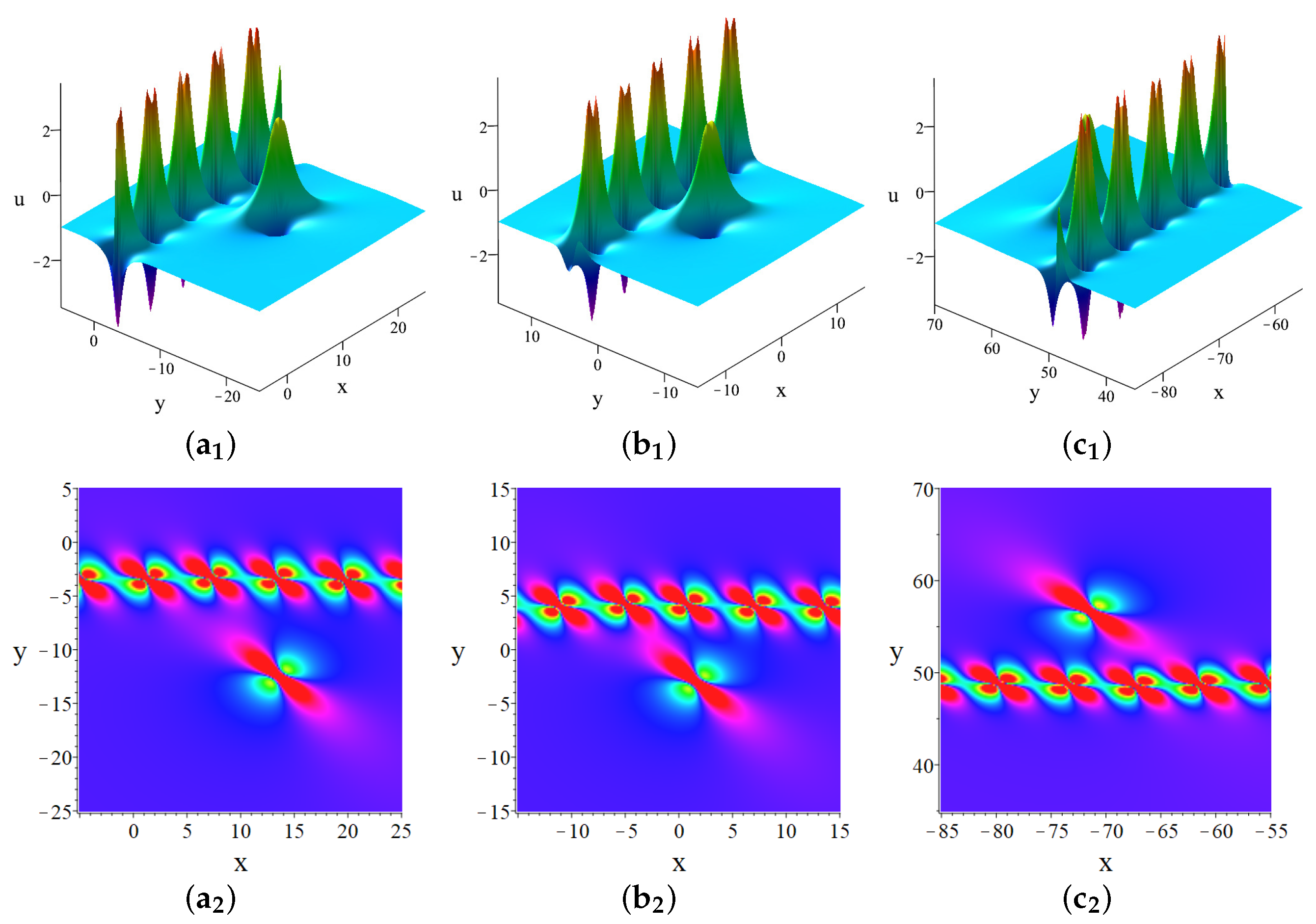
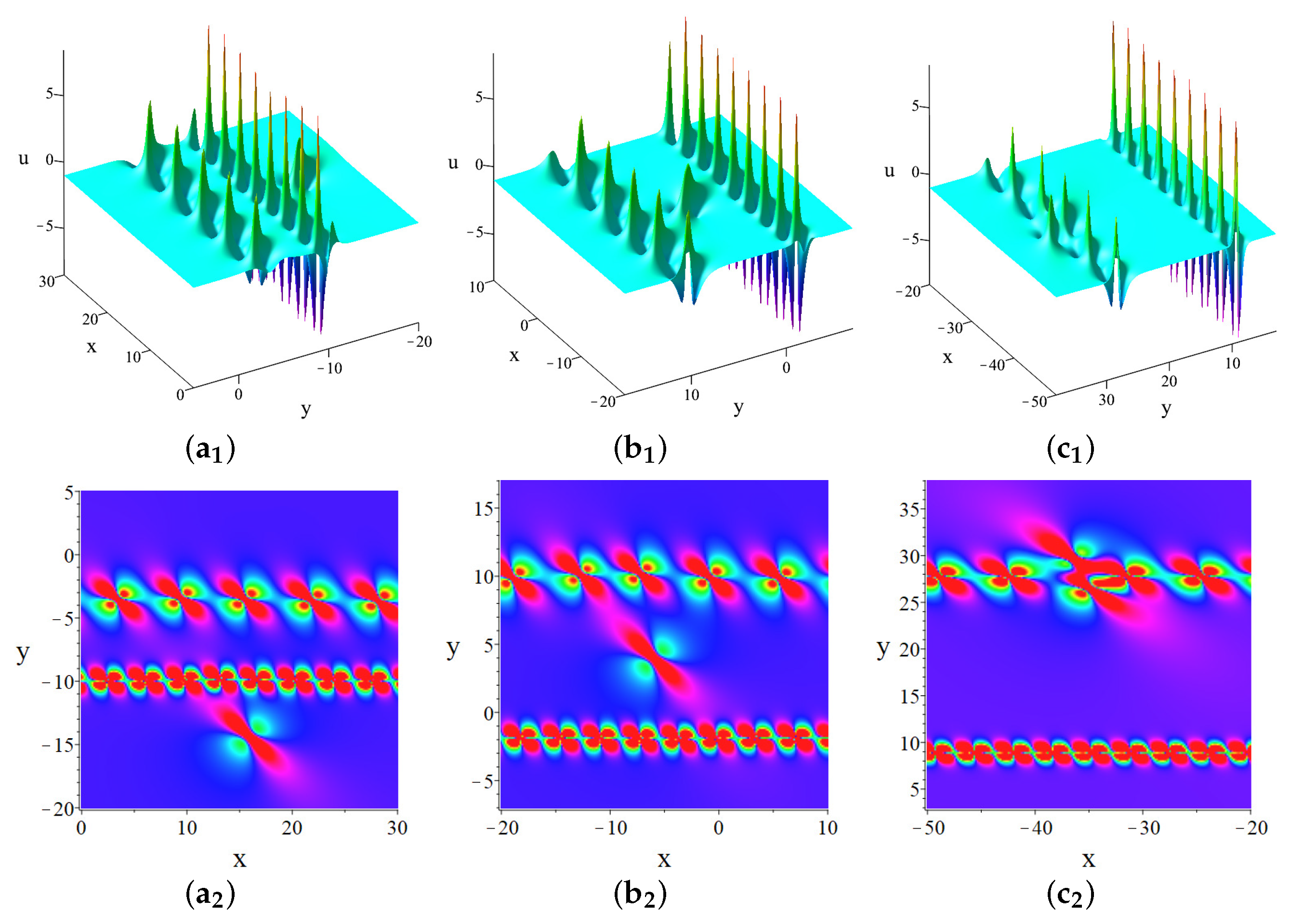
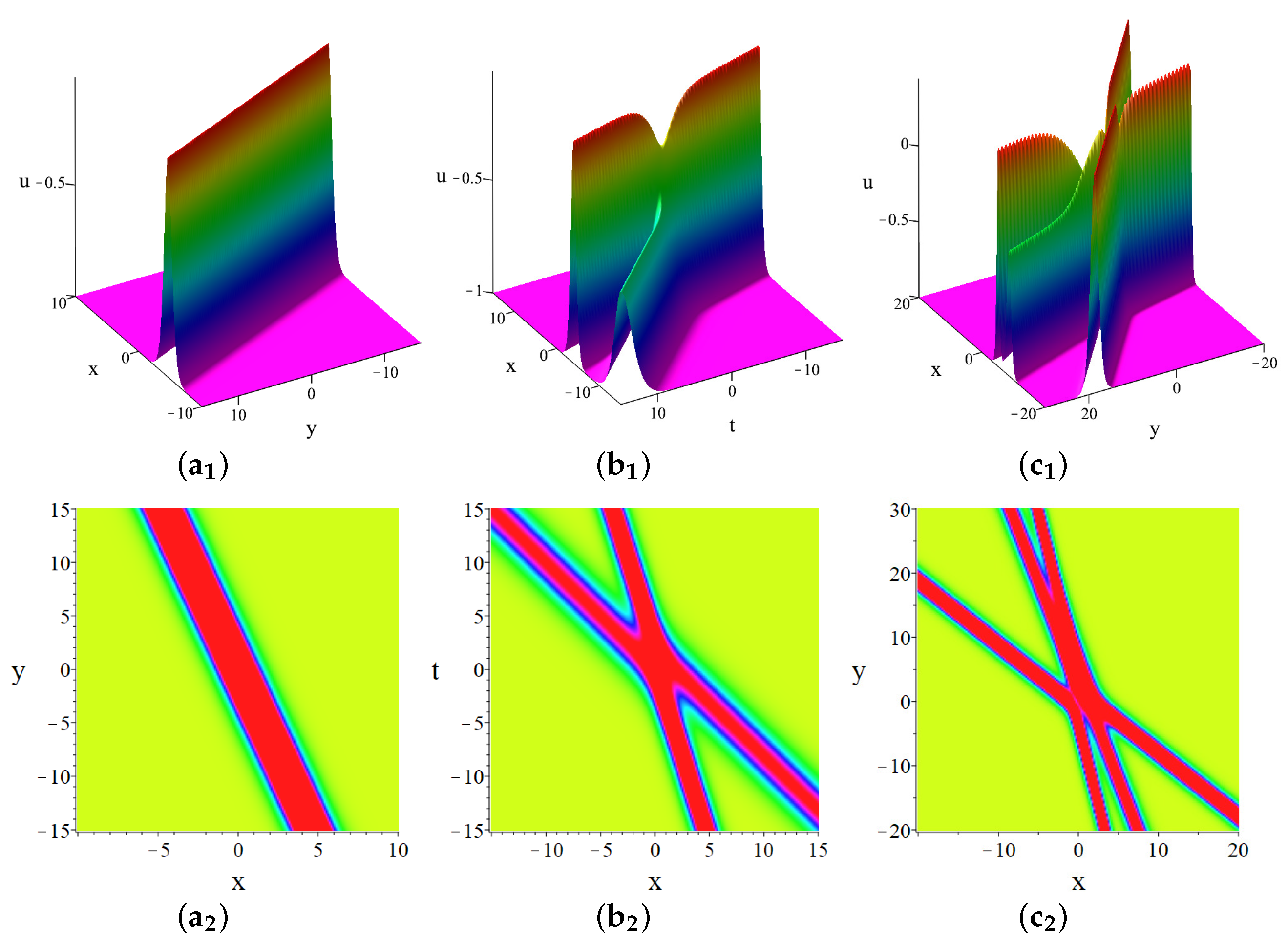
3.1. Soliton Solutions
3.2. Breather Solutions
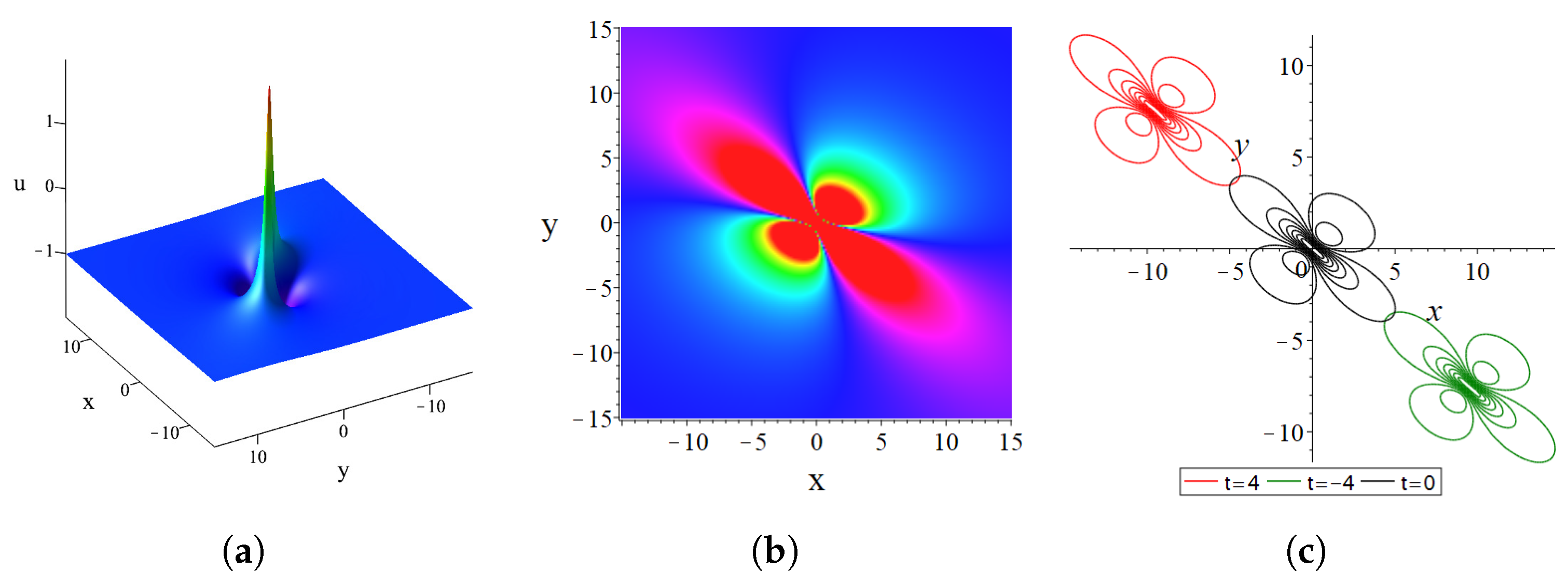
3.3. Lump Solutions
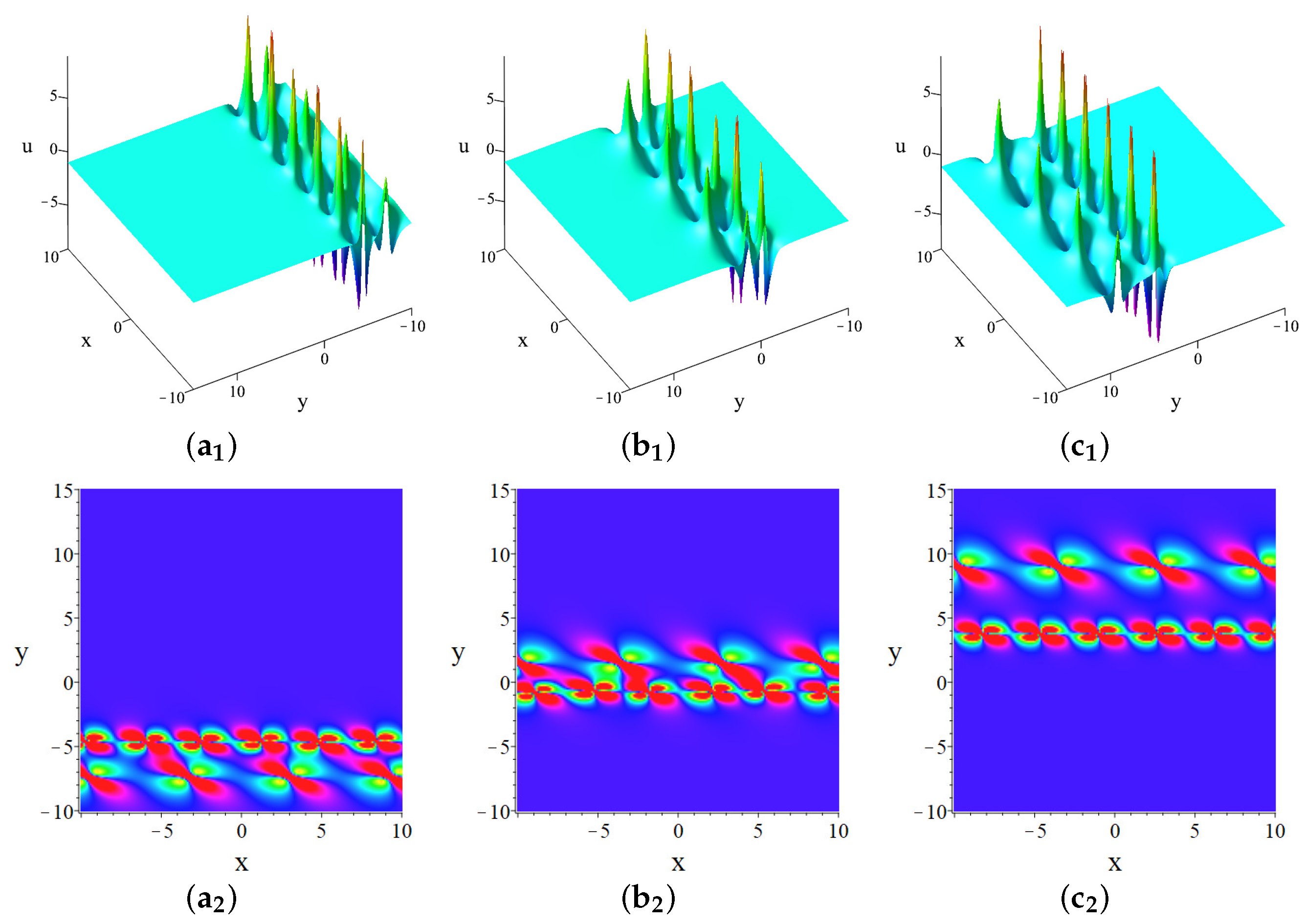
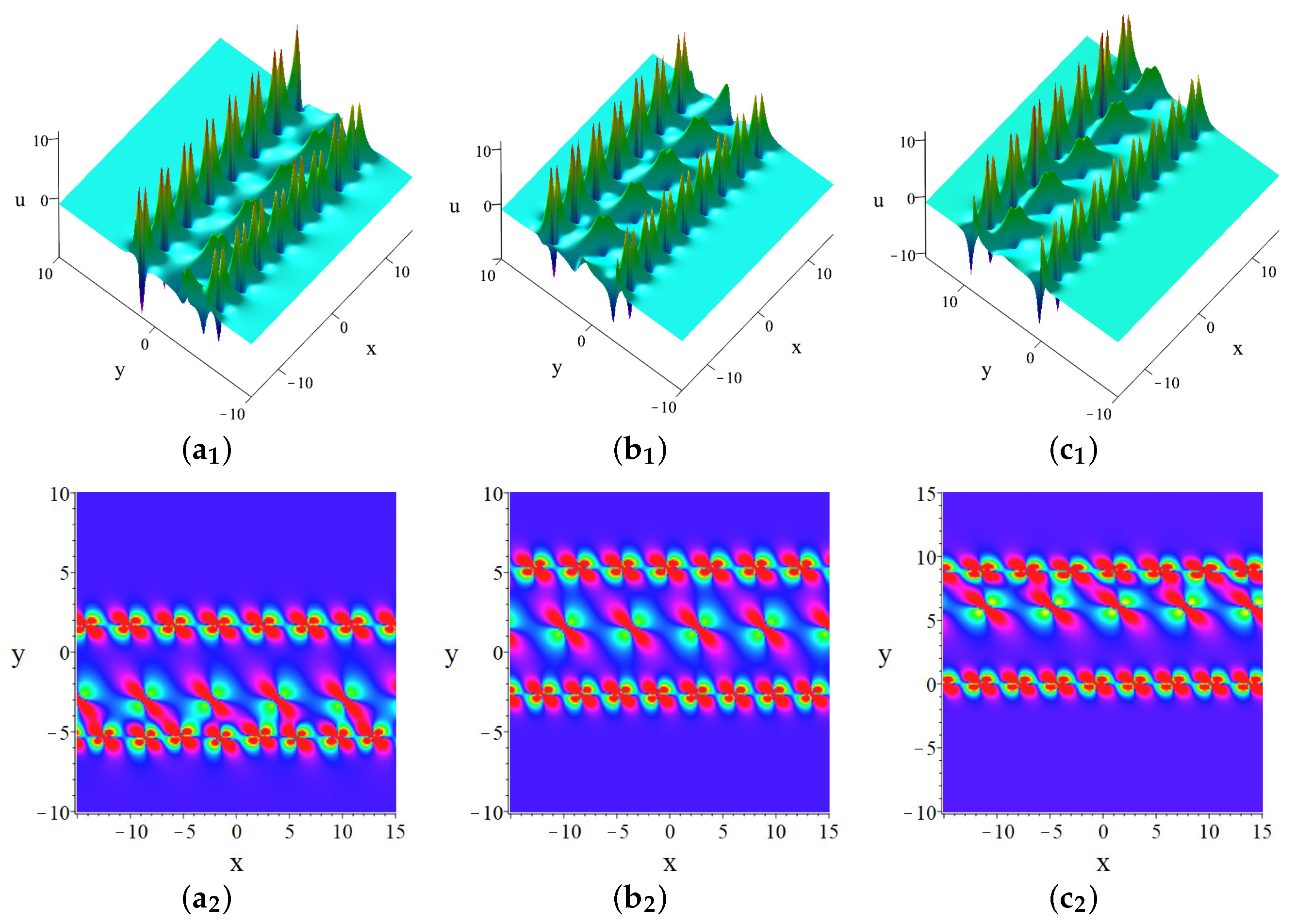
3.4. Hybrid Solutions
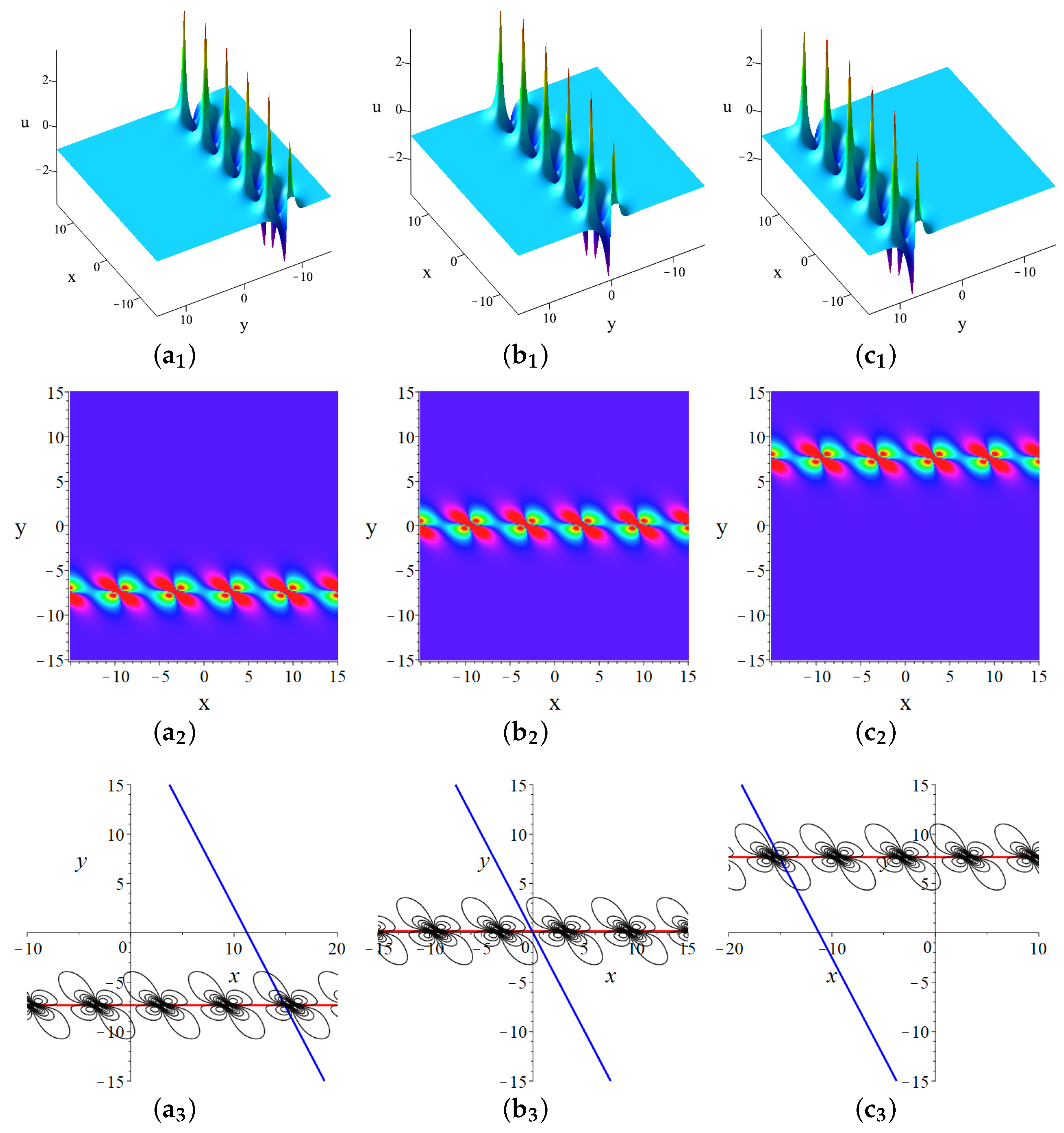
- When and , the first-order lump and one-breather hybrid solution is given by the determinantas depicted in Figure 6.
- When and , the first-order lump and two-breather hybrid solution is given by the determinantas depicted in Figure 7.
4. Modulation Stability Analysis
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Ablowitz, M.J.; Clarkson, P.A. Solitons, Nonlinear Evolution Equations and Inverse Scattering; Cambridge University Press: Cambridge, UK, 1991. [Google Scholar]
- Zhang, X.; Chen, Y.; Zhang, Y. Breather, lump and X soliton solutions to nonlocal KP equation. Comput. Math. Appl. 2017, 74, 2341–2347. [Google Scholar] [CrossRef]
- Johnson, R.S. On solutions of the Camassa-Holm equation. Proc. R. Soc. Lond. A 2003, 459, 1687–1708. [Google Scholar] [CrossRef]
- Kenig, C.E.; Ponce, G.; Vega, L. On the generalized Benjamin-Ono equation. Trans. Am. Math. Soc. 1994, 342, 155–172. [Google Scholar] [CrossRef]
- Zhou, Z.; Ma, W.X.; Zhou, R. Finite-dimensional integrable systems associated with the Davey-Stewartson I equation. Nonlinearity 2001, 14, 701. [Google Scholar]
- Ablowitz, M.J.; Musslimani, Z.H. Inverse scattering transform for the integrable nonlocal nonlinear Schrödinger equation. Nonlinearity 2016, 29, 915. [Google Scholar] [CrossRef]
- Ablowitz, M.J. Nonlinear waves and the inverse scattering transform. Optik 2023, 278, 170710. [Google Scholar] [CrossRef]
- Wahlquist, H.D.; Estabrook, F.B. Bäcklund transformation for solutions of the Korteweg-de Vries equation. Phys. Rev. Lett. 1973, 31, 1386. [Google Scholar]
- Hirota, R.; Satsuma, J. A variety of nonlinear network equations generated from the Bäcklund transformation for the Toda lattice. Prog. Theor. Phys. Suppl. 1976, 59, 64–100. [Google Scholar] [CrossRef]
- Lou, S.Y.; Hu, X.; Chen, Y. Nonlocal symmetries related to Bäcklund transformation and their applications. J. Phys. A-Math Theor. 2012, 45, 155209. [Google Scholar] [CrossRef]
- Matveev, V.B.; Salle, M.A. Darboux Transformations and Solitons; Springer: Berlin/Heidelberg, Germany, 1991. [Google Scholar]
- Guo, B.; Ling, L.; Liu, Q.P. Nonlinear Schrödinger equation: Generalized Darboux transformation and rogue wave solutions. Phys. Rev. E 2012, 85, 026607. [Google Scholar] [CrossRef]
- Deift, P.; Zhou, X. A steepest descent method for oscillatory Riemann-Hilbert problems. Bull. Am. Math. Soc. 1992, 26, 119–123. [Google Scholar] [CrossRef]
- Deift, P.; Zhou, X. A steepest descent method for oscillatory Riemann-Hilbert problems. Am. Math. Soc. 1993, 137, 295–368. [Google Scholar] [CrossRef]
- Hirota, R. The Direct Method in Soliton Theory; Cambridge University Press: Cambridge, UK, 2004. [Google Scholar]
- Wazwaz, A.M. Multiple-soliton solutions for the KP equation by Hirota’s bilinear method and by the tanh-coth method. Appl. Math. Comput. 2007, 190, 633–640. [Google Scholar]
- Ma, W.X. N-soliton solutions and the Hirota conditions in (2+1)-dimensions. Opt. Quantum Electron. 2020, 52, 511. [Google Scholar]
- Yang, B.; Yang, J. Overview of the Kadomtsev-Petviashvili hierarchy reduction method for solitons. Partial Differ. Equ. Appl. Math. 2022, 5, 100346. [Google Scholar]
- Zhang, L.H.; Liu, X.Q.; Bai, C.L. Symmetry, Reductions and new exact solutions of ANNV equation through Lax pair. Commun. Theor. Phys. 2008, 50, 1–6. [Google Scholar]
- Boiti, M.; Leon, J.J.P.; Manna, M.; Pempinelli, F. On the spectral transform of a Korteweg-de Vries equation in two spatial dimensions. Inverse Probl. 1986, 2, 271. [Google Scholar]
- Lou, S.Y.; Hu, X.B. Infinitely many Lax pairs and symmetry constraints of the KP equation. J. Math. Phys. 1997, 38, 6401–6427. [Google Scholar]
- Zhao, Z.; He, L. Resonance Y-type soliton and hybrid solutions of a (2+1)-dimensional asymmetrical Nizhnik-Novikov-Veselov equation. Appl. Math. Lett. 2021, 122, 107497. [Google Scholar]
- Liu, Y.; Wen, X.Y. Soliton, breather, lump and their interaction solutions of the (2+1)-dimensional asymmetrical Nizhnik-Novikov-Veselov equation. Adv. Differ. Equ. 2019, 2019, 332. [Google Scholar]
- Guo, L.; He, J.; Mihalache, D. Rational and semi-rational solutions to the asymmetric Nizhnik-Novikov-Veselov system. J. Phys. A—Math. Theor. 2021, 54, 095703. [Google Scholar]
- Wu, G.; Guo, Y. New complex wave solutions and diverse wave structures of the (2+1)-dimensional asymmetric Nizhnik-Novikov-Veselov equation. Fractal Fract. 2023, 7, 170. [Google Scholar] [CrossRef]
- Guo, L.; Wang, L.; Chen, L.; He, J. Dynamics of the rogue lump in the asymmetric Nizhnik-Novikov-Veselov system. Stud. Appl. Math. 2023, 151, 35–59. [Google Scholar]
- Zhang, J.F.; Liu, Y.L. Bäcklund transformation and localized coherent structure for the (2+1)-dimensional asymmetric Nizhnik-Novikov-Veselov equation. J. Shanghai Univ. (Engl. Ed.) 2022, 6, 191–195. [Google Scholar]
- Sato, M. Soliton equations as dynamical systems on a infinite dimensional Grassmann manifolds. N.-Holl. Math. Stud. 1983, 81, 259–271. [Google Scholar]
- Jimbo, M.; Miwa, T. Solitons and infinite dimensional Lie algebras. Publ. RIMS Kyoto Univ. 1983, 19, 943–1001. [Google Scholar]
- Ohta, Y.; Satsuma, J.; Takahashi, D.; Tokihiro, T. An elementary introduction to Sato theory. Prog. Theor. Phys. Suppl. 1988, 94, 210–241. [Google Scholar]
- Ohta, Y.; Wang, D.S.; Yang, J. General N-dark-dark solitons in the coupled nonlinear Schrödinger equations. Stud. Appl. Math. 2011, 127, 345–371. [Google Scholar]
- Ohta, Y.; Yang, J. General high-order rogue waves and their dynamics in the nonlinear Schrödinger equation. Proc. R. Soc. A 2012, 468, 1716–1740. [Google Scholar]
- Ohta, Y.; Yang, J. Rogue waves in the Davey-Stewartson I equation. Phys. Rev. E 2012, 86, 036604. [Google Scholar]
- Ohta, Y.; Yang, J. Dynamics of rogue waves in the Davey-Stewartson II equation. J. Phys. A—Math. Theor. 2013, 46, 105202. [Google Scholar] [CrossRef]
- Han, Y.; Tian, B.; Yuan, Y.Q.; Zhang, C.R.; Chen, S.S. Bilinear forms and bright-dark solitons for a coupled nonlinear Schrödinger system with variable coefficients in an inhomogeneous optical fiber. Chin. J. Phys. 2019, 62, 202–212. [Google Scholar] [CrossRef]
- Zhao, X.; Tian, B.; Qu, Q.X.; Li, H.; Zhao, X.H.; Zhang, C.R.; Chen, S.S. Kadomtsev-Petviashvili hierarchy reduction, soliton and semi-rational solutions for the (3+1)-dimensional generalized variable-coefficient shallow water wave equation in a fluid. Int. J. Comput. Math. 2022, 99, 407–425. [Google Scholar] [CrossRef]
- Zhao, Y.; Tian, B. Hybrid-wave solutions for a (2+1)-dimensional variable-coefficient Kadomtsev-Petviashvili equation in fluid mechanics and plasma physics. Phys. Fluids 2023, 35, 097106. [Google Scholar] [CrossRef]
- Liu, W.; Zheng, X.; Wang, C.; Li, S. Fission and fusion collision of high-order lumps and solitons in a (3+1)-dimensional nonlinear evolution equation. Nonlinear Dyn. 2019, 96, 2463–2473. [Google Scholar] [CrossRef]
- Liu, Y.; Li, B.; Wazwaz, A.M. Novel high-order breathers and rogue waves in the Boussinesq equation via determinants. Math. Methods Appl. Sci. 2020, 43, 3701–3715. [Google Scholar] [CrossRef]
- Sun, Y.; Tian, B.; Liu, L.; Chai, H.P.; Yuan, Y.Q. Rogue waves and lump solitons of the (3+1)-dimensional generalized B-type Kadomtsev-Petviashvili equation for water waves. Commun. Theor. Phys. 2017, 68, 693. [Google Scholar] [CrossRef]
- Alizadeh, F.; Hosseini, K.; Sirisubtawee, S.; Hincal, E. Classical and nonclassical Lie symmetries, bifurcation analysis, and Jacobi elliptic function solutions to a 3D-modified nonlinear wave equation in liquid involving gas bubbles. Bound. Value Probl. 2024, 2024, 111. [Google Scholar] [CrossRef]
- Hosseini, K.; Alizadeh, F.; Hinçal, E.; Ilie, M.; Osman, M.S. Bilinear Bäcklund transformation, Lax pair, Painlevé integrability, and different wave structures of a 3D generalized KdV equation. Nonlinear Dyn. 2024, 112, 18397–18411. [Google Scholar] [CrossRef]
- Hosseini, K.; Alizadeh, F.; Hinçal, E.; Baleanu, D.; Osman, M.S.; Wazwaz, A.M. Resonant multi-wave, positive multi-complexiton, nonclassical Lie symmetries, and conservation laws to a generalized Hirota bilinear equation. Mod. Phys. Lett. B 2024, 2550032. [Google Scholar] [CrossRef]
- Biondini, G.; Mantzavinos, D. Long-time asymptotics for the focusing nonlinear Schrödinger equation with nonzero boundary conditions at infinity and asymptotic stage of modulational instability. Commun. Pure Appl. Math. 2017, 70, 2300–2365. [Google Scholar]

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Chu, J.; Liu, Y.; Wu, H.; Yuen, M. Characteristic Analysis of Local Wave Solutions for the (21)-Dimensional Asymmetric Nizhnik–Novikov–Veselov Equation. Symmetry 2025, 17, 514. https://doi.org/10.3390/sym17040514
Chu J, Liu Y, Wu H, Yuen M. Characteristic Analysis of Local Wave Solutions for the (21)-Dimensional Asymmetric Nizhnik–Novikov–Veselov Equation. Symmetry. 2025; 17(4):514. https://doi.org/10.3390/sym17040514
Chicago/Turabian StyleChu, Jingyi, Yaqing Liu, Huining Wu, and Manwai Yuen. 2025. "Characteristic Analysis of Local Wave Solutions for the (21)-Dimensional Asymmetric Nizhnik–Novikov–Veselov Equation" Symmetry 17, no. 4: 514. https://doi.org/10.3390/sym17040514
APA StyleChu, J., Liu, Y., Wu, H., & Yuen, M. (2025). Characteristic Analysis of Local Wave Solutions for the (21)-Dimensional Asymmetric Nizhnik–Novikov–Veselov Equation. Symmetry, 17(4), 514. https://doi.org/10.3390/sym17040514







