Geometric Properties of the m-Leaf Function and Connected Subclasses †
Abstract
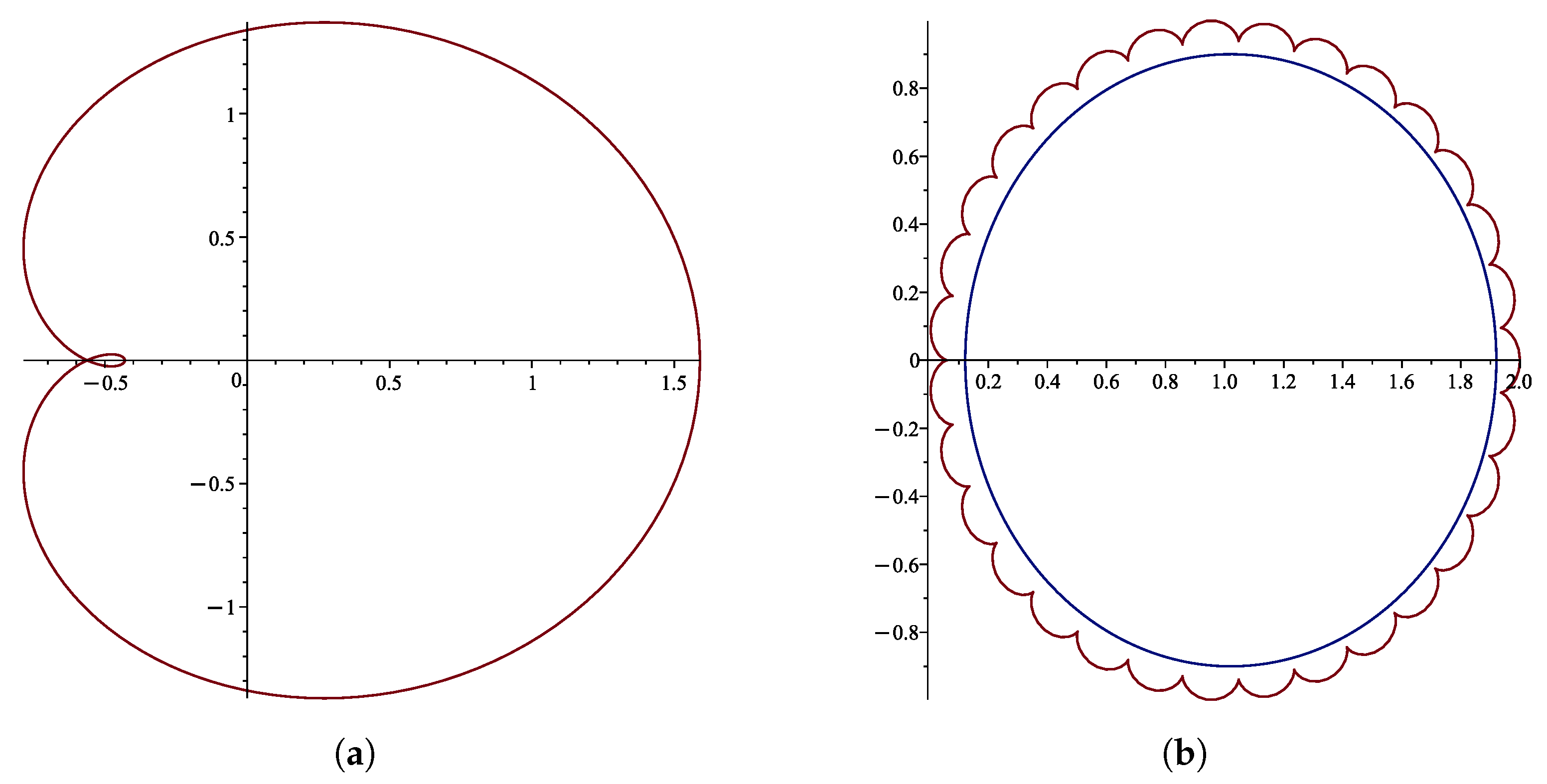
1. Introduction and Preliminary Results for the m-Leaf Function
2. Main Results
3. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Gandhi, S. Radius estimates for three leaf function and convex combination of starlike functions. In Mathematical Analysis I: Approximation Theory. ICRAPAM 2018; Deo, N., Gupta, V., Acu, A., Agrawal, P., Eds.; Springer Proceedings in Mathematics & Statistics, vol. 306; Springer: Singapore, 2020; pp. 173–184. [Google Scholar]
- Sunthrayuth, P.; Jawarneh, Y.; Naeem, M.; Iqbal, N.; Kafle, J. Some sharp results on coefficient estimate problems for four-feaf-type bounded turning functions. J. Funct. Spaces 2022, 2022, 8356125. [Google Scholar] [CrossRef]
- Alshehry, A.S.; Shah, R.; Bariq, A. The second Hankel determinant of logarithmic coefficients for starlike and convex functions involving four-leaf-shaped domain. J. Funct. Spaces 2022, 2022, 2621811. [Google Scholar] [CrossRef]
- Nevanlinna, R. Über die konforme Abbildund Sterngebieten. Oversikt Fin. Soc. Forh. 1921, 63, 48–403. [Google Scholar]
- Goluzin, G.M. Geometric Theory of Functions of a Complex Variable; American Mathematical Society: Providence, RI, USA, 1969; Volume 26. (In Russian) [Google Scholar]
- Pommerenke, C. Univalent Functions; Vandenhoeck and Ruprecht: Göttingen, Germany, 1975. [Google Scholar]
- Lindelöf, E. Mémoire sur certaines inégalités dans la théorie des fonctions monogènes et sur quelques propriétés nouvelles de ces fonctions dans le voisinage d’un point singulier essentiel. Acta Soc. Sci. Fenn. 1909, 35, 1–35. [Google Scholar]
- Littlewood, J.E. On inequalities in the theory of functions. Proc. Lond. Math. Soc. 1925, 23, 481–519. [Google Scholar] [CrossRef]
- Littlewood, J.E. Lectures on the Theory of Functions; Oxford University Press: London, UK, 1944. [Google Scholar]
- Rogosinski, W. On subordinate functions. Proc. Camb. Philos. Soc. 1939, 35, 1–26. [Google Scholar] [CrossRef]
- Rogosinski, W. On the coefficients of subordinate functions. Proc. Lond. Math. Soc. 1943, 48, 48–82. [Google Scholar] [CrossRef]
- Gunasekar, S.; Sudharsanan, B.; Ibrahim, M.; Bulboacă, T. Subclasses of analytic functions subordinated to the four-leaf function. Axioms 2024, 13, 155. [Google Scholar] [CrossRef]
- Duren, P.L. Univalent Functions. In Grundlehren der Mathemalischen Wissenstfhaften; Springer: New York, NY, USA, 1983; Volume 259. [Google Scholar]
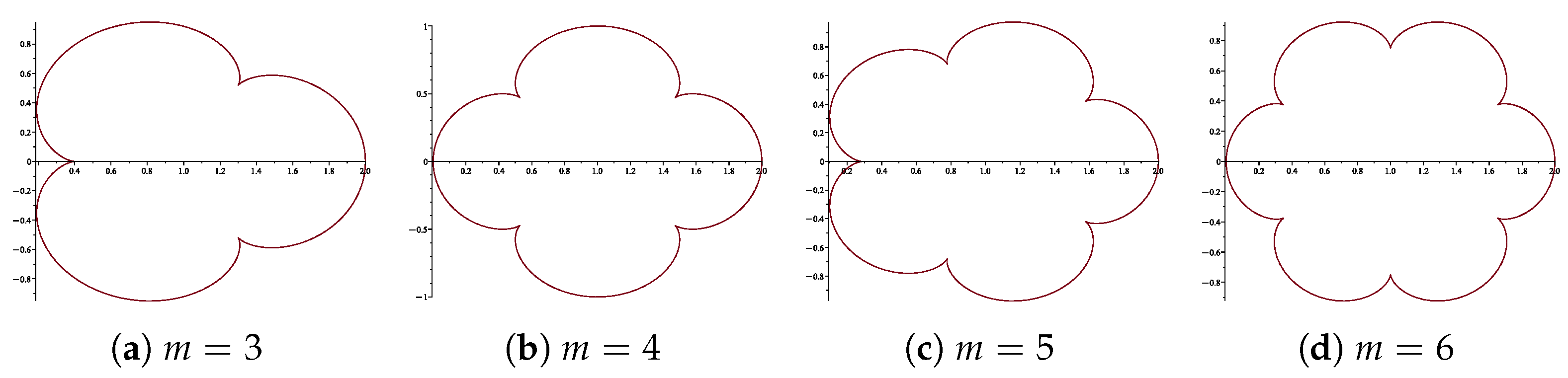
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Sudharsanan, B.; Gunasekar, S.; Bulboacă, T. Geometric Properties of the m-Leaf Function and Connected Subclasses. Symmetry 2025, 17, 438. https://doi.org/10.3390/sym17030438
Sudharsanan B, Gunasekar S, Bulboacă T. Geometric Properties of the m-Leaf Function and Connected Subclasses. Symmetry. 2025; 17(3):438. https://doi.org/10.3390/sym17030438
Chicago/Turabian StyleSudharsanan, Baskaran, Saravanan Gunasekar, and Teodor Bulboacă. 2025. "Geometric Properties of the m-Leaf Function and Connected Subclasses" Symmetry 17, no. 3: 438. https://doi.org/10.3390/sym17030438
APA StyleSudharsanan, B., Gunasekar, S., & Bulboacă, T. (2025). Geometric Properties of the m-Leaf Function and Connected Subclasses. Symmetry, 17(3), 438. https://doi.org/10.3390/sym17030438






