Nuclear-Spin-Dependent Chirogenesis: Hidden Symmetry Breaking of Poly(di-n-butylsilane) in n-Alkanes

Abstract

Since the 1960s, theorists have claimed that the electroweak force, which unifies parity-conserving electromagnetic and parity-violating weak nuclear forces, induces tiny parity-violating energy differences (10⁻¹⁰–10⁻²¹ eV) between mirror-image molecules. This study reports the dual mirror-symmetry-breaking and second-order phase transition characteristics of mirror-symmetric 7₃-helical poly(di-n-butylsilane) in n-alkanes under static (non-stirring) conditions. In particular, n-dodecane-h₂₆ significantly enhances the circular dichroism (CD) and circularly polarized luminescence (CPL) spectra. A new (−)-CD band emerges at 299 nm below T_C1 ~ 105 °C, with a helix–helix transition at T_C2 ~ 28 °C, and exhibits g_abs = +1.3 × 10⁻² at −10 °C. Synchronously, the CPL band at 340 nm exhibiting g_lum = −0.7 × 10⁻² at 60 °C inverts to g_lum = +2.0 × 10⁻² at 0 °C. Interestingly, clockwise and counterclockwise stirring of the mixture induced non-mirror-image CD spectra. n-Dodecane-d₂₆ weakens the g_abs values by an order of magnitude, and oppositely signed CD and a lower T_C1 of ~45 °C are observed. The notable H/D isotope effect suggests that the CH₃ termini of the polysilane and n-dodecane-h₂₆, which comprise a three identical nuclear spin-1/2 system in a triple-well potential, effectively work as unidirectional hindered rotors due to the handedness of nuclear-spin-dependent parity-violating universal forces. This is supported by the (−)-sign vibrational CD bands in the symmetric and asymmetric bending modes of the CH₃ group in n-dodecane-h₂₆.

1. Introduction

1.1. Hierarchy in Broken Symmetries

All hierarchical structures of nature are governed by four fundamental physical forces: the infinite range gravitational force (GF), the infinite range electromagnetic force (EMF), the ultrashort range (~10⁻⁹ nm) weak nuclear force (WF), and the ultrashort range (~10⁻⁶ nm) strong nuclear force (SF) [1,2,3,4,5,6,7,8,9,10,11,12,13,14,15,16,17,18,19,20,21,22,23,24,25,26,27,28,29,30,31,32,33]. Relative to the SF, the magnitudes of the GF, EMF, and WF are 10⁻⁴³, 10⁻², and 10⁻⁶, respectively [9,31]. The four forces are mediated by force-carrying vectoral bosons with integer spins: GF: the hypothetical massless graviton G (spin-2); WF: the massive charged W^± (spin-1, 80.4 GeV) and neutral Z⁰ bosons (spin-1, 91.2 GeV); EMF: the massless photon γ (spin-1); and SF: the massless eight gluons (spin-1) [31,32,33]. Aiming at a deeper understanding of hierarchical structures, it has been theoretically and experimentally investigated whether the seven symmetries—parity (P), charge conjugation (C), time reversal (T), and their combinations, CP, PT, CT, and CPT—are rigorously conserved in the four forces [1,2,3,4,5,6,7,8,9,10,11,12,13,14,15,16,17,18,19,20,21,22,23,24,25,26,27,31,32,33]. P- and CP-symmetry breaking has been investigated in particle (neutrinos) and subatomic (K- and B-mesons) physics. P-symmetry breaking has been established in nuclear and atomic physics but not in chemistry, bioscience, or materials science.

Recently, P-symmetry breaking became a hot topic in cosmology [24,25,26,27,28,29,30]. In 2020, astrophysicists reported hidden handedness imprinted in the cosmic microwave background (CMB) at 2.73 K. The sign and magnitude of the birefringent rotation from an observer were evaluated to be β_CMB = +0.35 ± 0.14° [28,29], corresponding to a Kuhn dissymmetry ratio, g_em of +2.0 (±0.8) × 10⁻³. Our life, biomolecules, biopolymers, and chemical reactions may thus experience a cosmological dextrorotatory force ranging from 0.3 to 630 GHz (0.01–21 cm⁻¹) with a peak of 160 GHz (5.35 cm⁻¹). The W^± bosons carry the handedness of the weak charged current (WCC) and permit exchange between quarks (fermions with spin-1/2), i.e., left-hand (LH) up quark (u, 2.2 MeV, charge +2/3) and LH down quark (d, 4.7 MeV, charge −1/3). However, interaction with right-hand (RH) u and RH d is not permitted [9,31,32,33]. Likewise, W⁺ and W⁻, respectively, transform between leptons (spin-1/2) from LH electron (e⁻, 0.51 MeV, charge −1) to LH electron-neutrino (ν_e < 2.2 × 10⁻⁶ MeV, charge 0) and from LH ν_e to LH e⁻ [9,31,32]. The W⁻-WCC in ⁶⁰Co → ⁶⁰Ni and W⁺-WCC in ⁵⁸Co → ⁵⁸Fe nuclear fissions [4,5,33], as well as other β^∓ decays of radiomarkers, i.e., ¹¹C, ¹³N, ¹⁵O, ¹⁸F, ³²P, are detectable as the opposite handedness of e⁻ and its anti-matter positron, e⁺ [33,34].

1.2. Atomic Parity Violation—Theories and Experiments

Inspired by parity-violation (PV) in the β^∓-decays [33], Weinberg, Glashow, and Salam in the 1960s proposed electroweak force (EWF) theory involving the Z⁰ boson to unify PV-WF and P-symmetry conserving (PC)-EMF. The EWF theory makes all of Z⁰, W^±, and γ possible in a massless family above ~10² GeV (~10¹⁵ K) [31,32]. In 1973, the detection of weak neutral current (WNC) and WCC by an elastic ν_e–anti-ν_e scattering using a liquid bubble chamber detector (called Gargamelle) filled with CF₃Br (possibly, C¹⁹F₃^79/81Br) at Conseil Européen pour la Recherche Nucléaire (CERN) was reported, followed by, in 1983, the detection of the Z⁰ as a production of an e⁻e⁺ pair using the proton (p)-anti-p collider (Super Proton Synchrotron, SPS) at CERN [35,36,37,38,39]. By combining the SF theory and the Higgs scalar boson (spin-0, 125.1 GeV), the EWF theory became the standard model of particle physics [31,32]. Also, around that time, theorists further hypothesized atomic parity violation (APV) and molecular parity violation (MPV) induced by the handedness of the WNC mediated by the Z⁰-boson. Probing the APV hypothesis of stable atoms met difficulties due to the absence of chemical chirality [40,41,42,43,44,45,46,47,48,49,50,51,52,53,54,55,56,57,58,59,60,61]. Although the APV of ¹H was computationally visualized as a handed spiral torus of e⁻ trajectory around p by admixing P-even 2s and P-odd 2p_1/2 states, its APV magnitude was far from a detectable level [50]. Theoretical predictions to amplify APV ∝ Z³ (Z: atomic number) for non-relativistic atoms and APV ∝ Z⁵ for relativistic atoms [46,47,48,49] led to the successful detection of weak APV effects on the order of g ~ 10⁻⁷ at forbidden electronic transitions for several high-Z atoms, including ¹³³Cs, ²⁰⁹Bi, ²⁰⁸Pb, ²⁰⁵Tl, and ¹⁷⁴Yb, in a collision-free gas state using high-resolution optical rotatory dispersion spectroscopy (ORD), fluorescence-detective circular dichroism spectroscopy, and dc-Stark interferometer [9,49,51,52,53,54,55].

In 1964 Michel [43], followed by Stodolsky in 1974 and 1982 [44,45], predicted the use of neutron optical activity to detect APV effects. Actually, in recent years, spin-polarized neutron resonance scattering (SPNRS) has been used experimentally and detected clear APV effects. Almost a dozen half-integer nuclear spin (HINS) high-Z isotopes (spin number, I) as solids, i.e., ⁸¹Br (I = 3/2), ⁹³Nb (I = 9/2), ¹⁰³Rh (I = 7/2), ¹⁰⁵Pd (I = 5/2), ¹⁰⁷Ag (I = 1/2), ¹¹¹Cd (I = 1/2), ¹¹⁵In (I = 9/2), ¹¹⁷Sn (I = 1/2), ¹²⁷I (I = 5/2), ¹³⁹La (I = 7/2), and integer nuclear spin (INS) radioisotope, ²³⁸U (I = 0), revealed huge APV effects on the order of g ~ 10^–3–10^–2 at resonance transitions by SPNRS experiments in the energy range of 0.5 to 1100 eV [56,57,58,59,60,61]. Among these HINS isotopes, ¹³⁹La shows a particularly large g value of ~ 2 × 10^–1 at 0.734 eV [58]. On the other hand, the INS isotopes, i.e., ⁸²Br (I = 5), ¹¹²Cd (I = 0), ¹¹⁸Sn (I = 0), ¹⁴⁰La (I = 3), showed greatly reduced APV effects even by the SPNRS [56,57]. These experiments strongly indicate the importance of HINS isotopes at the resonant transitions, enabling great amplification of APV effects, which is presumably also applicable to the nuclear-spin-dependent and/or the nuclear-spin-induced MPV effects.

1.3. Molecular Parity Violation—Theories and Experiments

In the 1960s and 1970s, MPV theorists estimated the order of magnitude of the parity-violating energy difference (PVED) between mirror-image molecules due to handed electron–nuclei interactions by admixing Z⁰-WNC to PC-EMF [62,63,64,65,66,67,68]. Comprehensive reviews covering those experiments, including the self-disproportionation of enantiomers (known as SDE), were published [69]. From the 1980s onward [4,5,6,7,8,10,16,17,18,19,20,68,70,71,72,73,74,75,76,77,78,79,80,81,82,83,84,85,86,87,88,89,90], sophisticated theories of electroweak quantum chemistry facilitated the rigorous prediction of the sign and magnitude of PVED for mirror-image molecules in mostly double-well potential (DWP) with rotational and/or inversion barriers (E_B). Because of the P-odd characteristics in the DWP, the potential energy for one mirror-image molecule is raised by E_PV; conversely, the other is pushed down by E_PV, similar to a seesaw. Therefore, the PVED is equal to 2E_PV = +E_PV − (−E_PV). The magnitude of PVED for isolated molecules, however, is too small to detect, being on the order of 10⁻¹⁰–10⁻²¹ eV (cf. 0.026 eV at 300 K), corresponding to 10⁻⁶–10⁻¹⁷ cm⁻¹ and 10⁴–10⁻⁷ Hz [18,19,84,85,86,87,88].

The MPV hypothesis is in sharp contrast to the accepted concepts of enantiomerism, diastereomerism, and racemism in modern stereochemistry based on PC-EMF theory [91,92,93,94]. Conventional stereochemical theory is predicated upon the rigorous preservation of energetic equality between mirror-image molecules, supramolecules, macromolecules, micelles, colloids, aggregates, gels, liquid crystals, and crystals. Chiroptical spectroscopic techniques [91,92,93,94], including electronic circular dichroism (CD), vibrational circular dichroism (VCD), Raman optical activity (ROA), and ORD, which detect the ground state chirality, and circularly polarized luminescence (CPL) to measure photoexcited chirality should therefore afford absolutely ideal mirror-image chiroptical spectra between the mirror-image substances, exhibiting identical magnitudes with opposite chiroptical signs at the same wavelengths and wavenumbers to at least three significant figures. A few researchers appear to be aware that the experimental results do not rigorously obey conventional stereochemical theory, as evidenced by differences in the chiroptical spectra, physicochemical properties, chemical reactions, and other aspects between supposed mirror-image molecules, oligomers, polymers, colloids, and crystals, as discussed in Section 3.9 [12,21,22,23,24,66,69,78,89,90,95,96,97,98,99,100,101,102,103,104,105,106,107,108,109,110,111,112,113,114,115].

Detecting MPV effects is therefore important in elucidating the missing link between the handedness of matter at all hierarchical levels of the matter world and the anti-matter world, ranging from LH-ν_e, LH-quarks, APV, L-amino acids and proteins, D-ribose and DNA/RNA, artificial molecules, and macromolecules; the homochiral living world, including helical bacteria, plants, and seashells; and the cosmological dextrorotatory rotation [1,3,8,12,13,18,19,20,21,22,23,24,25,26,27,28,29,30,31,32,33,49,55,60,62,70,71,72,74,82,86,95,96,97,98,99,100,101,102,103,104,105,106,107,108,109,110,111,112,113,114,115]. Aiming at amplifying the tiny PVED to detectable levels, six theoretical scenarios have so far been proposed, as described below.

• Scenario A. An accumulation model, often called a linear amplification model, was proposed in 1966 by Yamagata [62]. The overall PVED is proportional to repeat unit number (N), yielding N × E_PV in the systems. The idea is applicable to N-mer systems, i.e., macromolecules, supramolecules, micelles, colloids, micelles, colloids, aggregates, molecular liquids, liquid crystals, and crystals [12,66,71,78,95,96,97,98,99,100,101,102,103,104,105,106].
• Scenario B. E_PV ∝ Z⁵ is possible for high-Z atoms, while Z² and Z³, respectively, arise from spin-orbit-coupling (SOC, ξ) of constituents and the PV-WNC [5,6,67,70,73,75], which is similar to the Z³- and Z⁵-dependence of APV [42,46,47,48,49]. The idea is applicable to experiments using molecules involving high-Z atoms such as I, W, Re, Pb, Ir, Ru, and Os in periods 5 and 6 [17,18,69,73,75,76,77,78,79,95].
• Scenario C. In the early 1990s, a nonlinearly amplified MPV theory in a condensed phase was hypothesized by Salam based on Cooper e⁻(↑)-e⁻(↓) pairs (I = 0) in the Bardeen–Cooper–Schrieffer (BCS) theory of superconductivity and the ³He(↑)-³He(↑) pair (I = 1) and Bose–Einstein condensate (BEC) theory of superfluidity [107,108]. The hypothesis predicts that an L–D mixture of amino acids transforms into a single enantiomer at a critical temperature (T_c) by cooperative e⁻–n interactions. This cooperativity results in an entropy-driven, second-order phase transition detectable by emerging chiroptical signals and a jump of specific heat capacity [107,110,111,112]. Although T_c ~ 2.7 K was numerically obtained, a higher T_c ~ 250 K is optimistically conceivable [107]. However, because the rigid configuration of D-α-amino acids is unable to invert to L-configurations [96,97], non-rigid molecular rotamers in the absence of point chirality are suitable to validate the MPV hypothesis [18,19,67,109,110,111,112,113,114,115]. Similar phase transition models including mirror symmetric and dissymmetric bifurcations were proposed by Goldanskii et al. [2,3]. The selection between LH and RH at the bifurcation point is hypersensitive to external and internal fluctuations relative to the advantageous factors (rate constants in asymmetric chemical reactions) of chemical and physical chirality.
• Scenario D. The interplay between a smaller quantum tunneling splitting with an opposite parity, ΔE_±, in a DWP with a larger E_B and tiny P-odd E_PV leads to the resonant amplification of the MPV effect when satisfying ΔE_± ~ E_PV. In the cases where E_PV ≪ ΔE_± or E_PV ≫ ΔE_±, there is no detectable amplification [16,17,18,19,67,80,81,84,85,86,87,88]. Modulating the E_B vs. ΔE_±, kT, and E_PV causes a significant transition from coherence (oscillation) to decoherence (non-oscillation) due to environmental effects, leading to detectable MPV effects using steady-state and time-resolved chiroptical spectroscopy in a condensed phase [21,22,23,24,67,80,81,84,85,86,87,88,106,113,114,115].
• Scenario E. E_PV ∝ (E₀ − E_T)⁻¹ = ΔE_ST, where E₀ and E_T are the ground singlet (S₀) and photoexcited triplet (T₁) states [5,6], respectively, as confirmed by the direct S₀ → T₁ and T₁ → S₀ transition [116,117]. ΔE_ST is the difference in energy between the first excited (S₁) and T₁ states. Although the ¹H atom has the weakest ξ of 0.45 cm⁻¹ (5.5 × 10⁻⁵ eV, 1.3 × 10⁻³ kcal mol⁻¹), several light- and medium-Z number atoms have significant ξ in cm⁻¹: e.g., C (29), N (42), O (57), F (75), Si (211), P (247), S (288), Ge (1450), and Sn (4090) [118]. Molecules and polymers containing larger ξ atoms were found suitable for testing the MPV hypothesis. In particular, chain-like polysilanes consisting of Si–Si main chains with organic pendant side chains are one of the best candidates because summing up the SOCs amplifies the ξ of Si (211 cm⁻¹) × N-mer (Scenario A). This ξ can be further enhanced by HINS ²⁹Si (I = 1/2, natural abundance 4.7%, negative value of nuclear magnetic moment, μ_N = −0.55) [119]. For comparison, ¹³C (I = 1/2, natural abundance 1.1%, μ_N = +0.70), ¹H (I = 1/2, natural abundance 99.99%, μ_N = +2.79), and ²H (I = 1, μ_N = +0.86), respectively, have positive values of μ_N. The sign and magnitude of μ_N and I should contribute differently to the observable MPV effects.
• Scenario F. Most APV and MPV theories neglect the contribution of the nuclear spins, although advanced APV and MPV theories involving the nuclear spin effect have also been proposed. The anapole moment (AM) of the HINS ¹H atom was hypothesized in the late 1950s by Zel’dovich to account for PV β^±-decays [40,41,42]. The first PV molecular anapole moment (MAM) of diatomic molecules was theoretically discussed in the 1980s by Flambaun and Khriplovich, who suggested that the PV-MAM may be detectable by optical activity [120,121,122,123,124]. In 1997, the first PV atomic anapole moment (AAM) of ¹³³Cs vapor was detected using the dc-Stark technique [125,126]. So far, several hypothetical and realistic molecules and nanostructures [120,121,122,123,124,125,126,127,128,129,130,131,132,133,134,135,136,137,138,139,140,141,142,143,144,145,146], e.g., organometallic molecules involving ¹⁹⁵Pt and ²⁰⁷Pb, [127] M¹⁹F (M = ^203/205Tl, ³⁸Ba, ²²³Ra) [121,122,123,128,129,130], ⁹Be(NC)₂ and ²⁵Mg(NC)₂ [131], H₂X₂ (X = ¹⁷O, ³³S, ⁷⁷Se, ¹²⁵Te, ²⁰⁹Po) [132,133,134], chiral fluorooxirane and CHFClBr [135,136,137], and others [89,90,138,139,140,141,142,143,144,145,146] have been theoretically and experimentally investigated. The simplest experiment to validate nuclear-spin-dependent MPV and MAM is to compare HINS and INS isotopomers, that is, ordinary organic substances and their fully or partly deuterated analogs.

For almost 130 years, starting with Kipping and Pope in 1897 and 1898 [147,148], the chiroptical and physicochemical properties and solid-state structures of LH and RH molecules in crystals, biological oligomers, micelles, aggregates, synthetic polymers, and systems have been investigated and statistical analyses carried out [21,22,23,24,69,78,95,96,97,98,99,100,101,102,103,104,105,106,109,110,111,112,113,114,115,148]. However, most of these experimental results, including our previous polysilanes bearing (S)- and (R)-3,7-dimethyloctyl pendants with 96% ee [21,22,23,24] and (S)- and (R)-2-methylbutyl pendants with 99% ee (Scenarios A, B, C, and E) [24,106], remain a matter of debate because of non-100% ee sources, unresolved impurities and chiral dust, differences in the crystal sizes and molecular weights of polymers, the action of directionally opposite Coriolis forces in the northern and southern hemispheres, and non-natural materials.

Recently, the present authors reported clear MPV-related (−)-sign CPL spectra (g_lum ~ –2 × 10⁻³) at 25 °C from nearly 70 kinds of mirror-symmetric π-conjugated molecular rotamers without point chirality in achiral solvents under static conditions (Scenarios A, C, and D) [113,114,115]. The rotamers possess small hindered barriers of E_B ~ 1 kcal mol⁻¹ along the C–C, C–N, C–O, and C–S single bonds (for example, refs. [113,114,115] Aiming at approaching the condition ΔE_± ~ E_PV, it was effectively possible to increase E_B [113] by increasing the viscosity of the solvents (an environmental effect). The (−)-sign CPL spectra result from the S₁-state of metastable chiral rotamers with handedness. Among these rotamers, two pyrromethenes, 546 and 597, carrying multiple methyl groups as hindered rotors (Chart S1, Supplementary Materials (SM)), coumarin 6, coumarin 545, and rhodamine B revealed clear (−)-CD spectra (g_abs ~ −10⁻⁵) [114]. Additionally, Fujiki, Imai, and coworkers reported that a HINS molecular system, ^151/153Eu(β-diketonate)₃, involving six C¹⁹F₃ groups coordinated with ³¹P-containing enantiomeric ligands, reveals P-odd (−)-sign CPL spectra at ⁵D₀ → ⁴F_J (J = 3 at 650 nm and J = 4 at 700 nm) transitions [149]. This suggests nuclear-spin-induced MPV and/or PV-MAM effects (Scenario F) [150,151].

1.4. Our Experimental Approaches Toward Validation of Molecular Parity Violation Hypothesis

With the above six MPV scenarios in mind, our findings stimulated us to experimentally investigate the variable temperature (VT) CD and CPL spectral characteristics of rigid rod-like poly(di-n-butylsilane) (PDBS, Chart S1, Supplementary Materials) having a large characteristic ratio, C_∞ = 42.3, in n-hexane (Θ solvent) at 19.1 °C, to validate the MPV hypothesis [152,153]. PDBS is well-known to adopt mirror-symmetric 7₃-helices with λ_max = 319 nm in n-hexane and at λ_max 313–320 nm in the solid state, although it possesses no chiral centers [152,153,154,155,156]. The family of poly(dialkylsilane)s including PDBS are acknowledged to be excellent examples of helical polymers because they exhibit narrow phonon-side-band-free absorption and photoluminescence (PL) in the near-UV region and show quasi-one-dimensional Wannier-like exciton behavior, characteristic of a Si quantum wire [157,158,159,160,161,162,163]. The nature of the exciton is extremely sensitive to the helicity of the wire [152,153,154,155,156,157,158,159,160,161,162,163,164,165,166,167,168].

The present study focuses on PDBS in medium-length n-alkane solvents using VT CD/UV and CPL/PL spectroscopy, ranging from −10 °C to 110 °C. Herein, we report the dual mirror-symmetry breaking second-order phase transitions (chiroptical generation and chiroptical inversion) of PDBS in a range of longer chain solvents: n-C₁₁H₂₄, n-C₁₂H₂₆, n-C₁₃H₂₈, n-C₁₄H₃₀, and n-C₁₆H₃₄ under static conditions. Similar, though less obvious, effects were found in shorter chain solvents: n-C₈H₁₈, n-C₁₀H₂₂, and isooctane. In particular, very large amplitude effects in the CD and CPL spectra of PDBS were induced in n-C₁₂H₂₆. A new (−)-sign CD band emerges at λ_max 300 nm below T_C1 ~ 105 °C, followed by a switch from (−)- to (+)-sign CD bands due to a helix–helix transition at T_C2 ~ 28 °C, reaching g_abs = +1.3 × 10⁻² at −10 °C. Synchronously, the (−)-sign CPL band at 60 °C exhibited g_lum = –0.7 × 10⁻² at λ_em 340 nm and inverted to (+)-sign CPL with g_lum = +2.0 × 10⁻² at 0 °C. The signs and magnitudes of g_lum at T_C2 show a large fluctuation between +2.0 × 10⁻² and −1.0 × 10⁻². Notably, in n-C₁₂D₂₆, these g_abs values were an order of magnitude weaker, with opposite CD signs and a lower T_C1 of ~45 °C. Unexpectedly, clockwise (CW) and counterclockwise (CCW) stirring of PDBS in n-alkanes at 0, 20, and 40 °C did not result in mirror-image CD spectra. The notable H/D isotope effect allows us to propose that the three-fold symmetric CH₃ group, which is representative of three identical HINS systems (I = 3/2 (A) and 1/2 (E_a and E_b)) in a triple-well potential (TWP), works as a unidirectional hindered rotor, supporting several (−)-sign vibrational CD bands: symmetric bending (umbrella) at 1382 cm⁻¹, asymmetric bending at 1455 cm⁻¹, and other vibrational modes.

2. Results

2.1. Protocols for Reproducible Chiroptical Measurements of MPV Effects

To arrive at several protocols for testing for MPV effects, PDBS-H and PDBS-L were examined as homogeneous solutions in several n-alkanes and isooctane. The synthesis and characterization of PDBS–H and –L were reported in Section S2 and Figures S1 and S2 in the Supplementary Materials. PDBS-H has a higher number-average molecular weight (M_n) and weight-average molecular weight (M_w), and PDBS-L has lower M_n and M_w, while both have a similar polydispersity index (PDI): PDBS-H; M_n = 6.15 × 10⁴, M_w = 11.6 × 10⁴, PDI = 1.89 and PDBS-L; M_n = 4.55 × 10⁴, M_w = 8.28 × 10⁴, PDI = 1.82.

Furthermore, to validate a generality of MPV effects, poly{bis-(p-t-butylphenyl)silane), poly(n-hexyl-2-ethylbutylsilane), poly(methyl-n-propylsilane), poly(n-pentyl-n-propylsilane), poly(di-n-pentylsilane), poly(di-n-hexylsilane), and permethyldecasilane were examined (Chart S1, Supplementary Materials). The synthesis and characterization of the oligo- and polysilanes were mentioned in Section S2.4 and Figures S3 and S4 (Supplementary Materials). The MPV testing results are discussed in Section 3.8.

A prolonged search was carried out for a suitable system providing reproducible chiroptical measurements, the details of which are described in the experimental Section S2.2 in the Supplementary Materials. The protocol is briefly described below.

To exclude undesirable chiroptical effects induced by hydrodynamic lamellar/vortex/turbulent flow [169,170,171,172,173,174,175], the authors verified whether CW/CCW stirring, which mimics the P-symmetric Coriolis force in the northern and southern hemispheres resulting from the GF, and for comparison, static conditions, affects the resulting CD and UV spectra at 0, 20, and 40 °C. Although stirring direction-dependent CD spectra were obtained, it is still not yet clear why CW and CCW motions at the same stirring speeds did not induce mirror-symmetric CD spectra of PDBS-L and PDBS-H in n-C₁₂H₂₆, n-C₁₃H₂₈, n-C₁₄H₂₈, and n-C₁₆H₃₄. However, no obvious CD spectra of PDBS-L and PDBS-H in isooctane, n-C₆H₁₄, n-C₈H₁₈, and n-C₁₀H₂₂ under static and CW and CCW stirring conditions were induced. These unexpected results led to our adoption of static conditions to measure all the CD/UV spectra of the sample solutions and solvents at given temperatures. The anomaly in the effects of hydrodynamic flow on the CD spectral shapes and their chiroptical signs are described and discussed in Section 2.7 and Section 3.10 in the main text and Sections S3.7 and S3.8 in the Supplementary Materials.

More importantly, prior to the heating and cooling runs of the chiroptical experiments at a given temperature under static conditions, preliminary annealing at 60 °C for at least 15 min under static conditions was carried out to reorganize the n-butyl side chains and Si–Si main chains from their disordered conformations. The details are described in Section S3.1 of the Supplementary Materials.

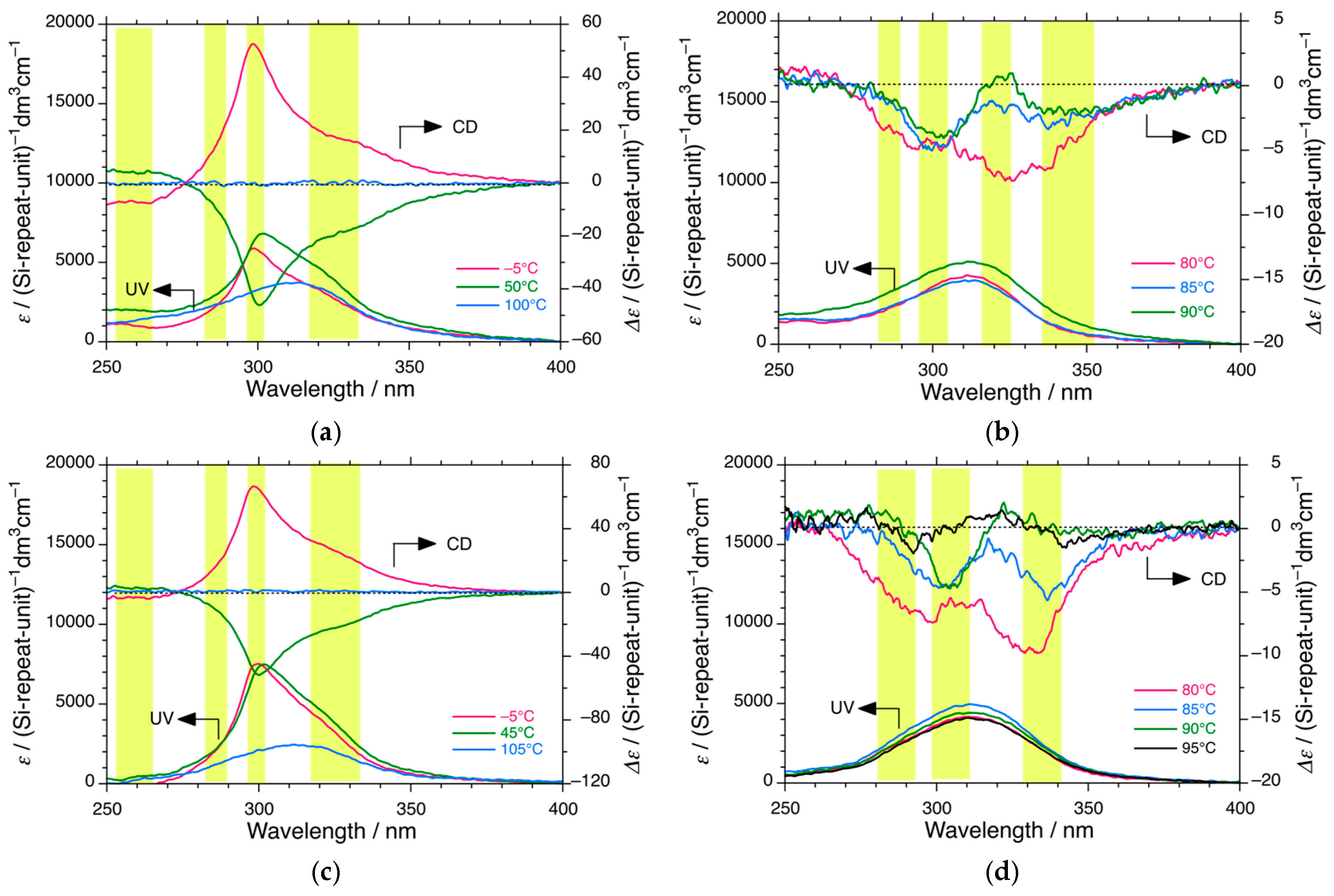
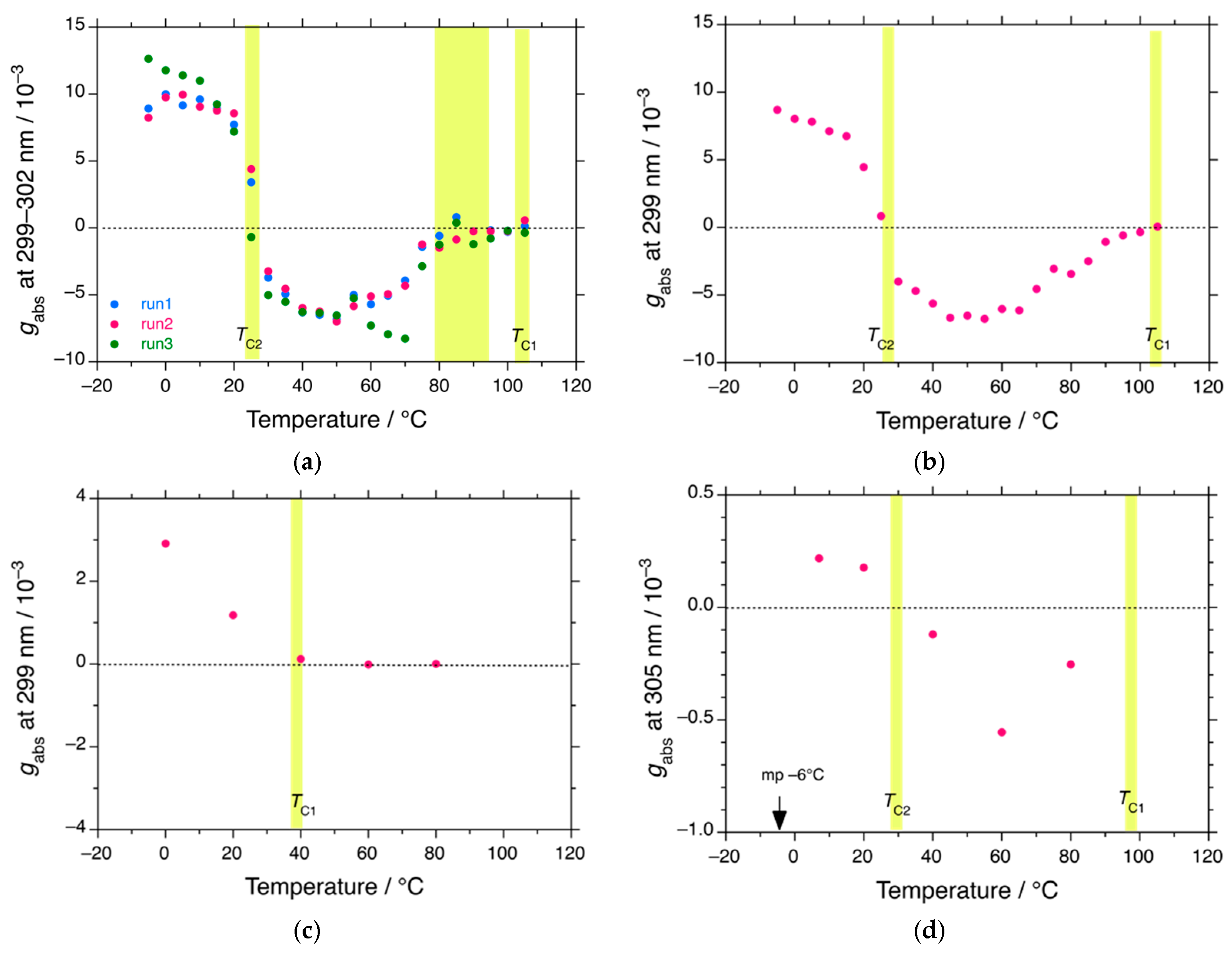
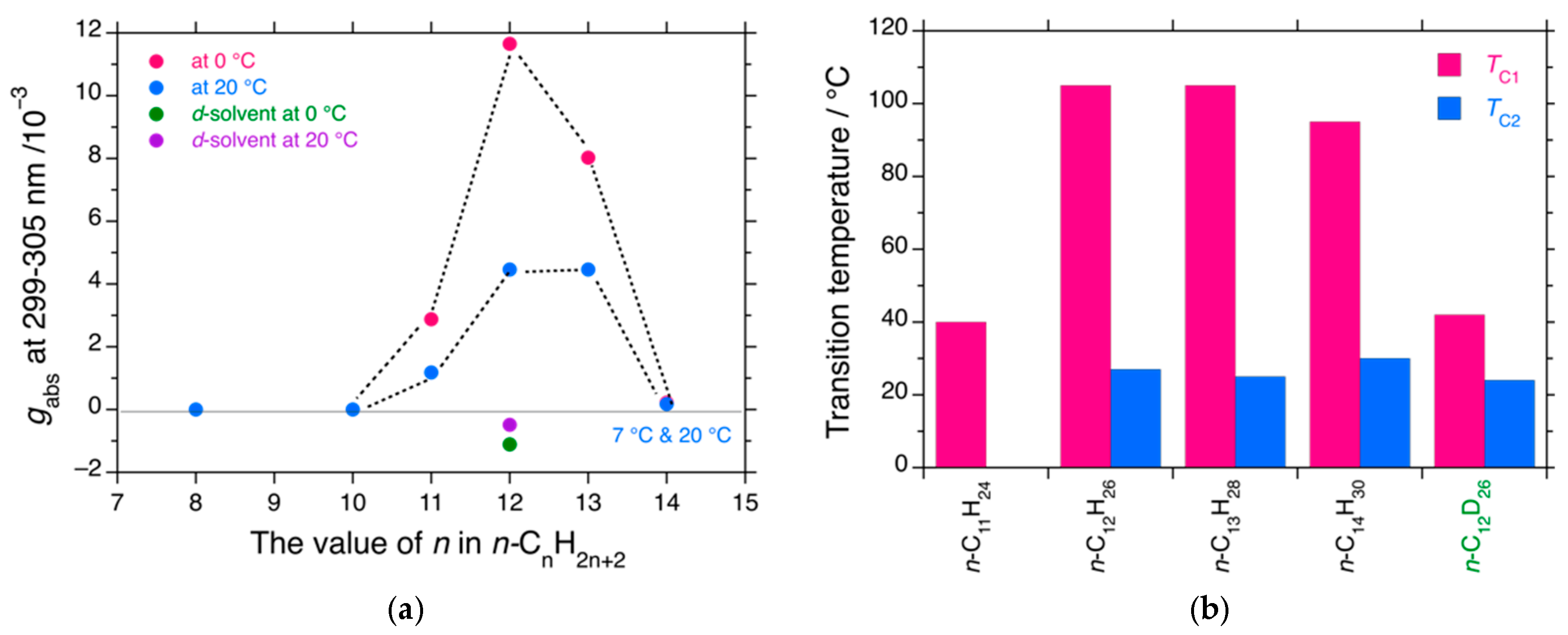
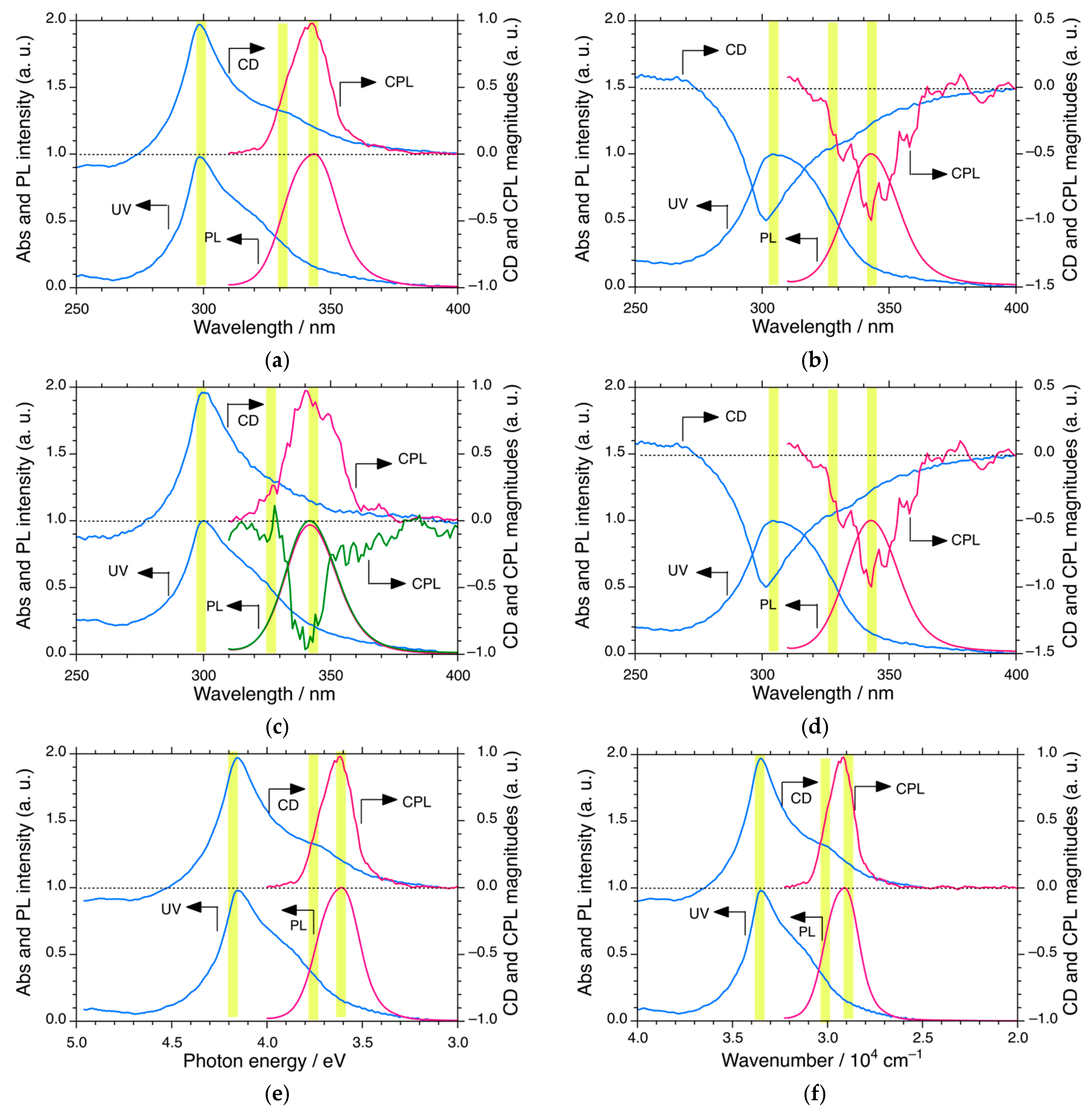
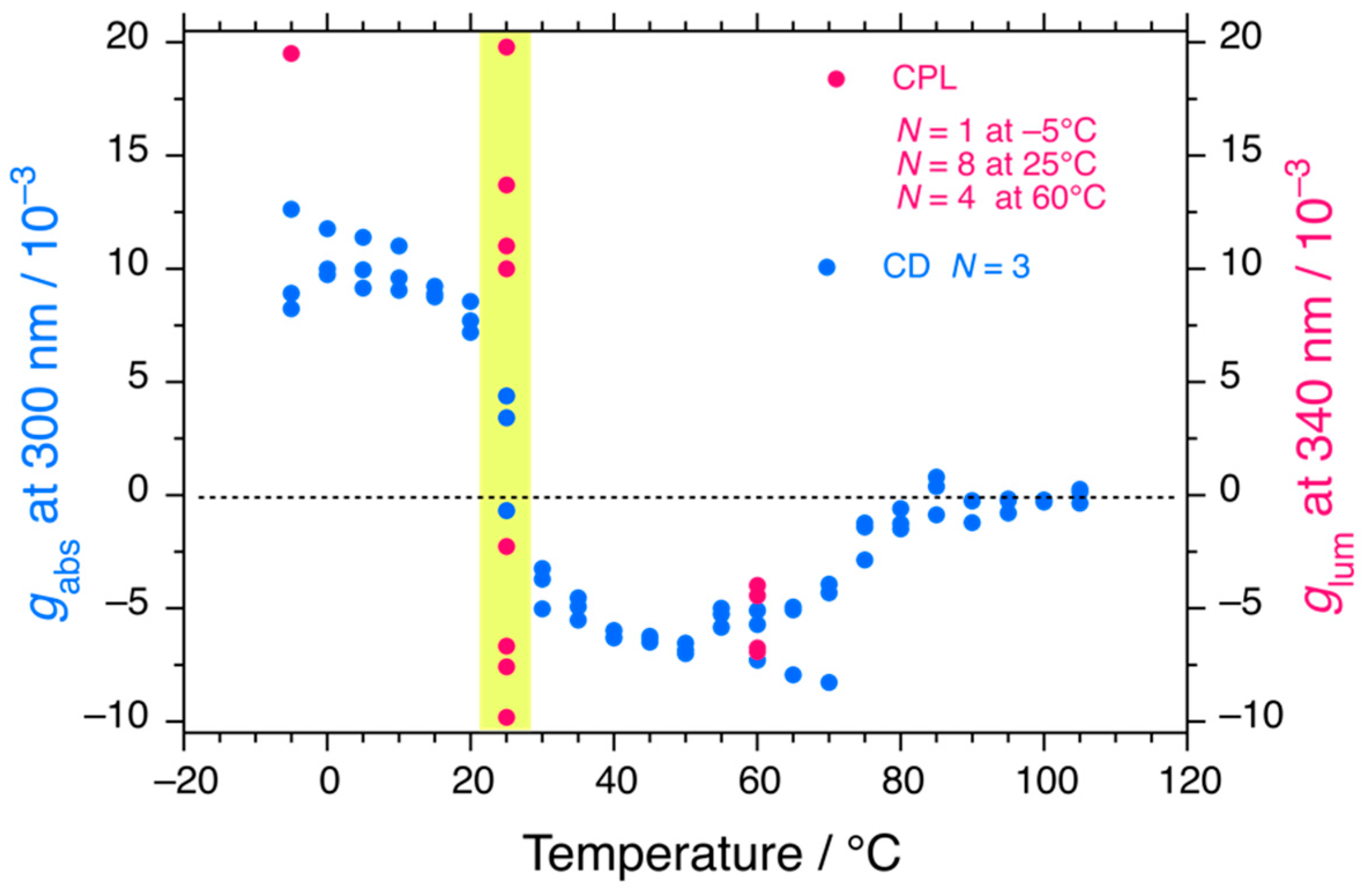
2.2. Effects of n-Alkane Chain Length and Temperature on CD-UV Characteristics of PDBS-L

To clarify the carbon number dependence of the n-alkanes, the VT-CD/UV spectra of PDBS-L in isotropic dilute solutions of n-C₈H₁₈, n-C₁₀H₂₂, n-C₁₂H₂₆, n-C₁₃H₂₈, n-C₁₄H₃₀, n-C₁₆H₃₄, and isooctane were measured under static conditions at 40, 20, and 0 °C for both heating and cooling runs, starting from 60 °C.

The CD and UV spectra of PDBS-L in isooctane and n-C₈H₁₈, n-C₁₀H₂₂, n-C₁₂H₂₆, and n-C₁₃H₂₈ at 0, 20, and 40 °C are shown in Figure S5a–c (Supplementary Materials). Cotton CD spectra emerging at ~300 nm in n-C₁₂H₂₆ and n-C₁₃H₂₈ were generated at 0, 20, and 40 °C, and n-C₁₆H₃₄ at 20 °C and 40 °C: (+)-sign at 0 °C, but (−)-sign at 20 °C and 40 °C. Less obvious CD spectra were recorded for the lower viscosity solvents isooctane, n-C₈H₁₈, and n-C₁₀H₂₂ at these temperatures.

When solutions of PDBS-L in n-C₁₂H₂₆, n-C₁₃H₂₈, n-C₁₄H₃₀, and n-C₁₆H₃₄ were cooled from 40 °C to 0 °C, the broader UV band at approximately 315 nm abruptly blueshifted to a narrower UV band at 299–302 nm. Such a blueshift has not yet been reported for this polymer. According to previous studies upon cooling of PDBS in n-hexane [152,153,154,155,156], the UV band around λ_max 320 nm at 22 °C undergoes an abrupt redshift around −35 °C, revealing a UV band at 355–360 nm at temperatures below −40 °C due to a change in conformation from the 7₃-helix of the Si main chain (dihedral angle (DH) ~ 150°) to other helices, e.g., 15₇-helix (~165°) and transoid 9₄-helix (~170°) [152,153,154,155,156,157,158,159,160].

The g_abs values of the bands around 300 nm at 0, 20, and 40 °C as a function of the n-alkane carbon number are plotted in Figure S5d (Supplementary Materials): g_abs = +6 × 10⁻⁴ in n-C₁₂H₂₆ at 0 °C was rather weak but fully recognizable. Importantly, the CD band at 299–302 nm emerges only when the corresponding UV band at 299–302 nm is the shorter tail of the 315 nm UV band, largely depending on the carbon number of n-alkanes and temperature. The sign of the CD band inverted between 0 °C and 20/40 °C, indicating the occurrence of a helix–helix transition. From the potential energy surfaces (PESs) of di-n-butyl 14-mer [152], diethyl-silane 4-mer [166], and diethylsilane 13-mer (discussed in Section 3.1), the 300 nm UV/CD band presumably derives from the DH 140–145° (and 220–225°) of the Si main chain as one of the local minima. These preliminary results encouraged a detailed investigation of the MPV effects of PDBS-H.

2.3. n-Alkane Carbon Number Dependence of CD-UV Characteristics of PDBS-H

The CD and UV spectra of PDBS-H in n-C₁₂H₂₆ and n-C₁₃H₂₈ over several temperatures are shown in Figure 1. For clarity, the corresponding raw CD and UV spectra of n-C₁₂H₂₆ are shown in Figure S6a–d, Supplementary Materials. The representative weaker CD and UV spectra of n-C₁₁H₂₄ and n-C₁₄H₃₀ are shown in Figure S7 (Supplementary Materials).

Clearly, PDBS-H in n-C₁₂H₂₆ reveals a (+)-sign 300 nm CD spectrum at the corresponding 300 nm UV band at −5 °C, and conversely, a (−)-sign 300 nm CD spectrum at the corresponding 300 nm UV band at 50 °C. The (+)/(−) 300 nm CD effects completely disappeared at 105 °C, coincident with a marked broadening of the UV band and bathochromic shift to 315 nm. Similarly, PDBS-H in n-C₁₃H₂₈ shows a (+)-300 nm CD band with coincident 300 nm UV absorption at −5 °C but a (−)-300 nm CD band and 300 nm UV absorption at 45 °C (Figure 1a,c).

The g_abs value of PDBS-H reaches +1.3 × 10⁻² in n-C₁₂H₂₆ at 0 °C and +0.9 × 10⁻² in n-C₁₃H₂₈ at −5 °C but inverts to −0.8 × 10⁻² in n-C₁₂H₂₆ at 50 °C and –0.7 × 10⁻² in n-C₁₃H₂₈ at 45 °C. The 300 nm CD band with (+) and (−)-signs disappeared at 105 °C, coincident with a marked broadening of the UV band and bathochromic shift to 315 nm. PDBS adopts equal fractions of RH and LH 7₃-helices at 105 °C, resulting in a CD-silent racemic mixture of helices. PDBS-H in n-C₁₂H₂₆ and n-C₁₃H₂₈ at −5 °C additionally has three broad CD bands: (+)-CD at ~325 nm, (−)-CD at ~260 nm, and less obvious (+)-CD in the tail of the 300 nm CD at ~285 nm. However, the CD signals of the three additional bands were inverted at 50 °C.

When PDBS-H is in n-C₁₂H₂₆ and n-C₁₃H₂₈ at elevated temperatures in the range of 80–95 °C, three or four additional (−)-CD bands at ~285, ~305, ~320, and ~335 nm are commonly observed (Figure 1b,d), indicating the generation of three or four intermediate helices in hot solutions. The magnitudes of the 300 nm CD spectra in n-C₁₁H₂₄ and n-C₁₄H₃₀ were much weaker (Figure S7, Supplementary Materials). The 300 nm CD signals in n-C₁₁H₂₄ emerge below 40 °C as a shoulder of the broader main UV band at 315 nm due to the 7₃-helix.

The g_abs values at the 300 nm CD bands of PDBS-H in n-C₁₁H₂₄, n-C₁₂H₂₆, n-C₁₃H₂₈, and n-C₁₄H₃₀ as a function of temperature are plotted in Figure 2. The temperature dependence of the g_abs curves reveals marked cooperativity which does not obey the Arrhenius law of thermal activation. As shown in Figure 2a,b, the (−)-CD 300 nm-CD bands in n-C₁₂H₂₆ and n-C₁₃H₂₈ were evident below 100–105 °C, diminished at ~28 °C, and inverted to (+)-sign g_abs below ~28 °C. The first critical temperature, T_C1 (100–105 °C), relates to the second-order phase transition from mirror-symmetric 7₃-helices to the 300 nm CD helix with handedness. The second critical temperature, T_C2 (~28 °C) relates to the second-order helix–helix transition of the 300 nm CD helix. From the temperature-independent g_abs values between −10 °C and T_C2 and between T_C2 and ~50 °C, the transition characteristics result from quantum tunneling between the RH and LH helices responsible for the 300 nm CD/UV band. The 300 nm CD/UV band at T_C2 indicates a superposition of the RH/LH helices with 50/50 populations. Similar quantum tunneling helix–helix transitions were reported for rigid rodlike polysilanes bearing (S)-3-methylpentyl and (R)-3,7-dimethyl-octyl co-pendants [21,22,23,24,167,168].

As shown in Figure 2c, the T_C1 value of PDBS-H in n-C₁₁H₂₄ decreased to 40 °C, and the T_C2 value could not be observed. As shown in Figure 2d, although the T_C1 and T_C2 values in n-C₁₄H₃₀ are nearly identical to those in n-C₁₂H₂₆ and n-C₁₃H₂₈, the absolute magnitude of g_abs over the entire temperature range is weaker by an order of magnitude. Thus, for PDBS-H, the values of g_abs, T_C1, and T_C2, associated with the emergence and disappearance of the 300 nm CD and UV bands, are very susceptible to the temperature and carbon number of the n-alkane solvent.

Interestingly, less obvious alterations in the physicochemical properties of n-C₁₂H₂₆ are evident as a jump in specific volume (cm³·g⁻¹, inverse of density) around 25 °C from an expected straight line of specific volume–temperature relationship (Figure S8 in Supplementary Materials) [176] and as a step-like increase in polarizability (dipole moment) of the CH₃ group at 1 THz (33 cm⁻¹, 4 × 10⁻³ eV) above 30 °C by VT-terahertz (THz) spectroscopy [177]. The occurrence of phase transitions due to change in the free-volume of n-C₁₂H₂₆ is weakly seen at ~30 °C, ~35 °C, and above 60 °C by the VT ortho-positronium (o-Ps) experiment [178]. These subtle transitions are reflected in T_C2 (24–30 °C) of PDBS-H in n-C₁₂H₂₆, n-C₁₃H₂₈, n-C₁₄H₃₀, and n-C₁₂D₂₆, as discussed in Section 2.5.

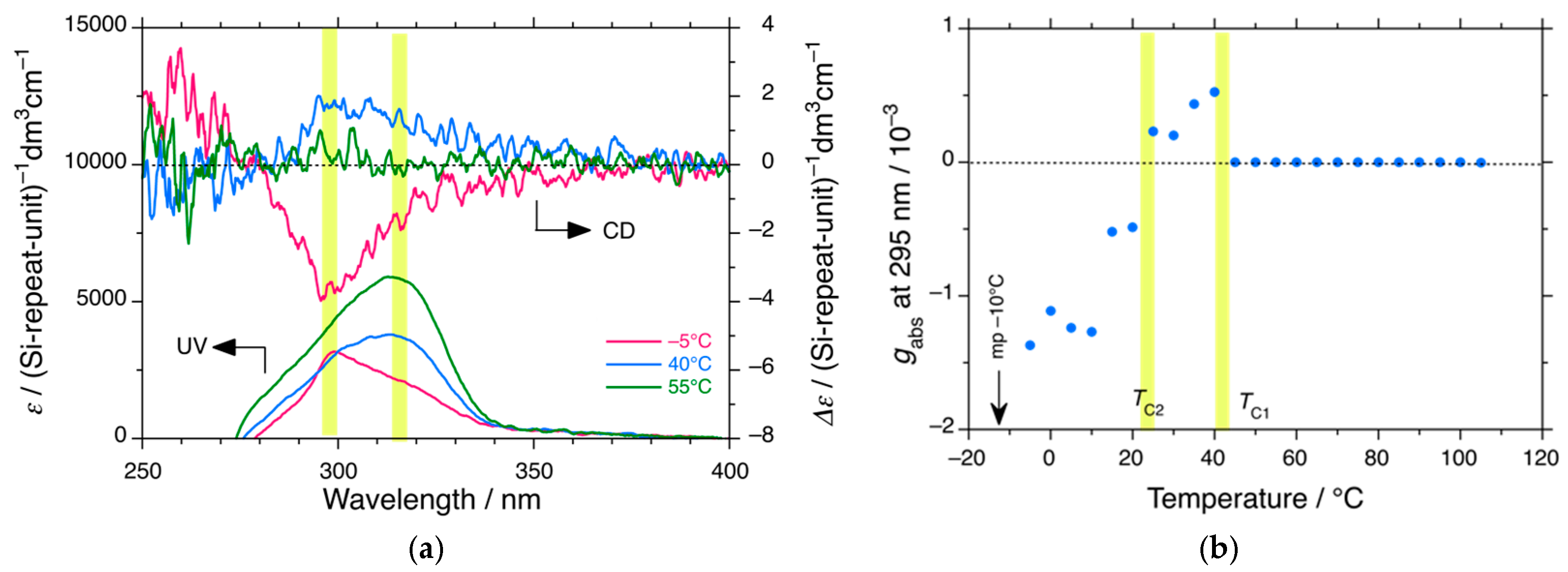
2.4. Noticeable H/D Isotope Effects in n-Dodecane

The MPV theory (Scenario C) relies on the BCS theory of superconductivity. To solve such fundamental problems, it is important to test the substances in condensed phases rather than just as isolated collision-free species, as Philip Anderson discussed in a 1972 essay titled “More is different: Broken symmetry and the nature of the hierarchical structure of science” [179].

According to the BCS theory, a Cooper pair (a singlet boson, ↑↓) is formed by an attractive force between two repulsive fermions, e⁻(↑) and e⁻(↓), by indirect interactions between electrons and phonons (lattice vibrations) [180,181]. In an analogy of why a hydrogen molecule is spontaneously produced by two hydrogen atoms, roughly speaking, the Cooper pair constitutes a very weak chemical bond to bind two unpaired e⁻(↑) and e⁻(↓), due to the Pauli exclusion principle, and dissociates at T_c. The Debye frequency (θ_D) connecting θ_D ∝ T_c is chosen from the highest frequency of the phonon vibrations. Historically, from clear experimental evidence, the product m^1/2·T_c is a constant for isotope effects of ¹⁹⁸Hg and ²⁰²Hg and other metals (e.g., Sn, Pb, and Tl). θ_D is proportional to m^−1/2, while m is the mass of metal isotopes [182,183,184]. In chemistry, θ_D is chosen from the reduced mass (μ_m) between ¹²C, ¹H, and ²H, in the general form μ_m = (m₁·m₂)/(m₁ + m₂). In the present study, the highest θ_D values were obtained from the specific optically active vibrations of liquid n-C₁₂H₂₆ and n-C₁₂D₂₆, which involve stretching (symmetric and asymmetric), scissoring and rocking, wagging, twisting, and torsion modes of the methyl and methylene groups. In particular, chiral asymmetric stretching, rocking, and twisting phonons of n-alkanes are crucial for inducing handed helicity in PDBS. It is thus anticipated that the smaller θ_D values of n-C₁₂D₂₆ make T_C1 and T_C2 significantly lower than those in n-C₁₂H₂₆.

The CD and UV spectra of PDBS-H in n-C₁₂D₂₆ were measured as a function of temperature. Representative CD and UV spectra are shown in Figure 3a, although UV signals shorter than 280 nm were not clearly subtracted. A (−)-CD band emerged at −5 °C, whereas a (+)-CD band emerged at 40 °C; these CD bands completely disappeared at 55 °C. The g_abs value of the 300 nm CD band in n-C₁₂D₂₆ as a function of temperature is plotted in Figure 3b. The absolute g_abs values of PDBS-H in n-C₁₂D₂₆ were clearly an order of magnitude weaker compared with those in n-C₁₂H₂₆. T_C1 decreased to ~42 °C, whereas T_C2 at ~25 °C remained nearly unchanged. Interestingly, the signs of the CD signals in n-C₁₂D₂₆ below T_C1 and T_C2 are opposite to those in n-C₁₂H₂₆. This notable H/D isotope effect of n-dodecane suggests that the helix–helix transition characteristics at T_C2 originate from PDBS-H itself since the difference in PES between n-C₁₂H₂₆ and n-C₁₂D₂₆ is almost negligible due to the bond length of C–D being only ~0.005 Å shorter than that of C–H [185].

2.5. Effects of Carbon Number and H/D Isotopes in n-Alkanes

To summarize the VT-CD/UV spectral characteristics described above, the g_abs values at the 300 nm CD band of PDBS-H at 0 and 20 °C as a function of the carbon number in n-alkanes are plotted in Figure 4a. The values of T_C1 and T_C2 as functions of the carbon number in n-alkanes are plotted in Figure 4b. A small difference in M_n and M_w between PDBS-H and PDBS-L resulted in a large difference in the MPV effects (Figure 4a and Figure S5, Supplementary Materials). According to Scenario A, there exist certain thresholds in DP_n and DP_w to cooperatively and nonlinearly elucidate the hidden MPV effects: PDBS-H (DP_n = 194, DP_w = 367) and PDBS-L (DP_n = 143, DP_w = 260).

Among the five medium-length n-alkane solvents, n-C₁₂H₂₆ exhibited the greatest MPV effects, accompanied by dual second-order phase transitions at T_C1 and T_C2 (Figure 4a). Similarly, n-C₁₃H₂₈ exhibited greater MPV effects, but the absolute g_abs values were somewhat weaker than those of n-C₁₂H₂₆. However, no odd–even effect of the carbon number in n-alkanes is observed. The absolute g_abs value of n-C₁₂D₂₆ was an order of magnitude lower than those of n-C₁₂H₂₆ and n-C₁₃H₂₈. As summarized in Figure 4b, n-C₁₂H₂₆, n-C₁₃H_28, and n-C₁₄H₂₈ had nearly identical T_C1 values of 100–105 °C, whereas n-C₁₁H₂₄ had a markedly lower T_C1 of 40 °C. All four of n-C₁₂H₂₆, n-C₁₃H₂₈, n-C₁₄H₂₈, and n-C₁₂D₂₆ showed nearly identical T_C2 values of 25–28 °C, although T_C2 in the shorter n-C₁₁H₂₄ should be observed below −10 °C.

2.6. Temperature Dependent CPL and CD Characteristics of PDBS-H in n-C₁₂H₂₆

The normalized CPL/PL and CD/UV spectra of PDBS-H in n-C₁₂H₂₆ at −5, 25, and 60 °C are compared in Figure 5a–d. For clarity, the normalized CD/UV and CPL/PL spectra at −5 °C as functions of photon energy (in eV) and wavenumbers (in cm⁻¹) are shown in Figure 5e,f.

At −5 °C below T_C2, the (+)-sign CPL spectrum affords g_lum (= ΔI/I) = +1.9 × 10⁻² at ~340 nm, while the corresponding CD spectrum shows g_abs = +1.3 × 10⁻² at 300 nm with an apparent Stokes’ shift of 4033 cm⁻¹ (0.50 eV). Similarly, at 60 °C, between T_C2 and T_C1, the (−)-sign CPL spectrum affords g_lum = −0.7 × 10⁻² at ~340 nm, whereas the corresponding CD spectrum has g_abs = –0.5 × 10⁻² at 300 nm with a similar Stokes shift at −5 °C. Interestingly, the CPL spectra at 25 °C near T_C2 greatly fluctuate between g_lum = +2.0 × 10⁻² and −1.0 × 10⁻², as shown in Figure 5c,d.

The g_abs values as a function of temperature and the g_lum value at the three temperatures are plotted in Figure 6. The spectra of PDBS at T_C2 show no bands due to the superposition of LH and RH helicities. The histograms of eight and four independent CPL measurements at 25 and 60 °C, respectively, are shown in Figure S9 (Supplementary Materials). The eight g_lum values at 25 °C are likely to obey a stochastic resonance rather than a sinusoidal function with time, implying that CPL in the S₁ state is hypersensitive to selection between RH and LH helicity due to the PVED-driven force to overcome fluctuations at 25 °C, whereas the four g_lum values at 60 °C are insensitive to fluctuations over time. In contrast, the results for the temperature region 25 °C ~ T_C2 are indicative of bifurcation for selection between the LH and RH helicities in the S₁ state of PDBS. The LH- or RH-helicity in the S₁ state was not determined prior to the CPL measurement because of quantum entanglement between the LH- and RH-helicities.

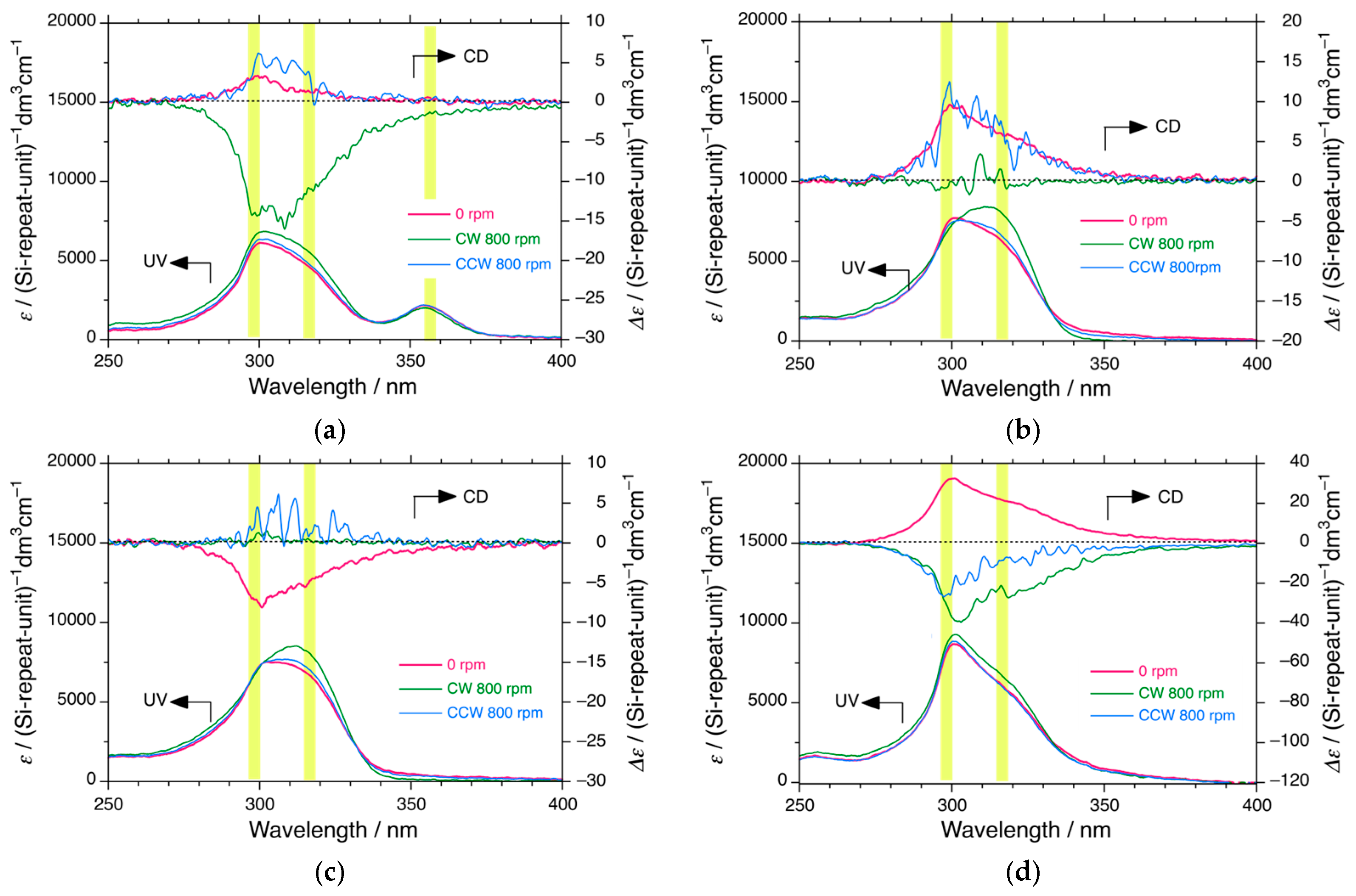
2.7. Non-Mirror-Symmetric CD Spectra of PDBS Under CW and CCW Stirring Directions

CD/UV spectra were recorded at 0 °C, 20 °C, and 40 °C for PDBS-L in n-C₁₂H₂₆, n-C₁₃H₂₈, n-C₁₄H₃₀, and n-C₁₆H₃₄ under static conditions and CW and CCW stirring at 100, 400, 600, and 800 rpm using a PTFE-coated cylindrical magnetic stir bar (2 mm in diameter × 7 mm in length) in a rectangular cuvette (10 mm path length and 35 mm in height) and the changes and differences are shown in Figure 7a–c and Figure S10a–h, Supplementary Materials, respectively. The changes in the CD/UV spectra of PDBS-H in n-C₁₂H₂₆ at 0 °C, 20 °C, and 40 °C under static and CW and CCW conditions at 1200 rpm are displayed in Figure S11 (Supplementary Materials).

Hydrodynamic flow in CW and CCW directions is recognized to induce mirror-image CD and CPL spectra from achiral chromophores/luminophores during aggregation by the removal of solvent and by gelation upon cooling achiral chromophores [169,170,171,172,173,174,175]. The chiroptical signs and product chirality are determined by the CW and CCW directions. Actually, in 1989, Goldanskii et al. pointed out that rotational hydrodynamic flow causes local P-symmetry breaking as a PC-EMF event [3].

Evidently, the non-mirror-image CD spectra under the CW and CCW flows of PDBS-L and PDBS-H in n-C₁₂H₂₆, n-C₁₃H₂₈, n-C₁₄H₃₀, and n-C₁₆H₃₄ below and above T_C2 and at ~T_C2 indicate the occurrence of a mechanism other than local P-symmetry breaking. Under gentle stirring at 100, 400, and 600 rpm at 20 °C, PDBS-L in n-alkanes experienced an un-identified PV-related force (Figure S10a,b, Supplementary Materials). The hydrodynamic CW and CCW flow under the GF is no longer the local P-symmetry breaking force and becomes a global PV force, as discussed in Section 3.9 and Section 3.10.

3. Discussion

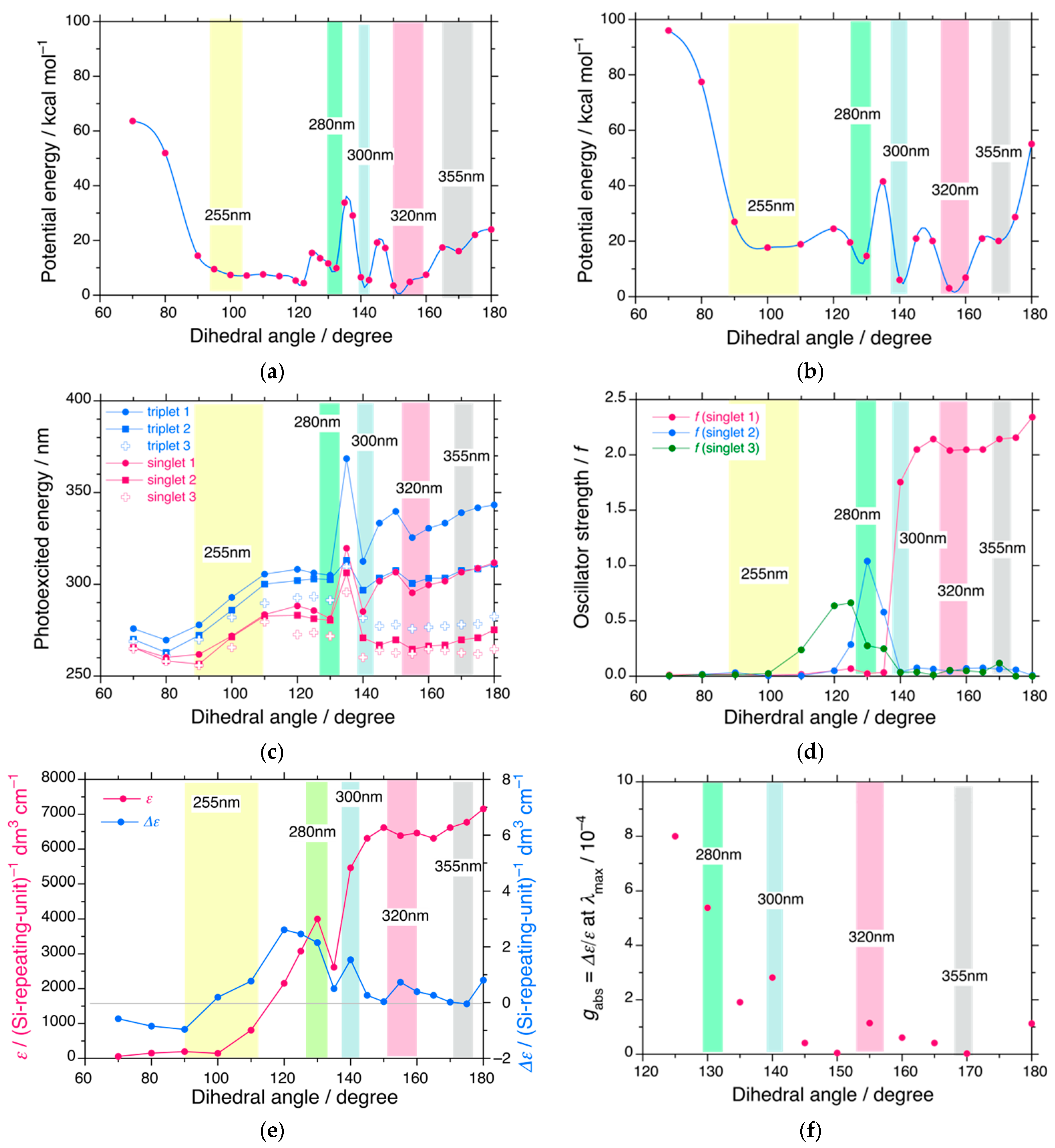
3.1. Potential Energy Surfaces at the Ground and Photoexcited States of PDBS

A primary question is the helical structure of PDBS in n-alkane solvents. The new helix responsible for the 300 nm CD and UV bands in a family of poly(di-n-alkylsilane)s and other poly(diorganosilane)s is yet not reported experimentally or theoretically [152,153,154,155,156,157,158,159,160,161,162,163]. Potential energy surfaces (PESs) at the ground and photoexcited states of Et-(Et₂Si)₁₃-Et (DES13) as a model of PDBS (Chart S2, Supplementary Materials) were calculated by semi-empirical PM3MM, DFT (B3LYP/6-31Gd), and TD-DFT, including three singlet and three triplet states (B3LYP/6-31Gd) using Gaussian 09 [186]. It is noted that the di-n-butylsilane 13-mer and its shorter 6-mer did not converge to optimized structures at the PM3MM level. The two PESs (PM3MM and DFT) normalized by 10 DH (dihedral angle) sets of the Si main chain as a function of DH are shown in Figure 8a,b. The photoexcitation energy (in nm) and oscillator strength (f) at the S₁ state as a function of DH are shown in Figure 8c and Figure 8d, respectively. The magnitudes of UV (ε) and CD (Δε) obtained with a full-width-at-half-maximum (fwhm) of 0.333 eV, and their g_abs values as a function of DH are depicted in Figure 8e and Figure 8f, respectively.

As shown in Figure 8a,b, DES13 possesses five minima at 170° (gray), 155° (red), 140° (blue), 130° (green), and 100° (yellow) in the Si main chain. The helix at 155° is the global minimum. The first metastable helix at 140° was connected to the deviant (D_±) helix of Et-(Et₂Si)₄-Et [166]. The 140°-helix is ascribed to the 300 nm CD/UV bands discussed in this work, presumably the 8₃-helix, while other local minima at 130° are likely to be 11₄-helices with λ_max 280 nm. The 170°- and 155°-helices correspond to the 15₇-helix (λ_max 355 nm) and 7₃-helix λ_max 315–320 nm), respectively [152,153,154,155,156]. The CD/UV bands due to the 130°-helix can be weakly observed as the tail of the 300 nm CD/UV bands (Figure 1). The 100°-helix is experimentally observable as weak (−)-CD and broad UV bands around λ_max 255–265 nm compared to the 300 nm (+)-CD band (Figure 1) because the chiroptical signs in the CD bands between 70° < DH < 100° and 100° < DH < 170° are theoretically opposite (Figure 8e).

The helix–helix transition capability between the four steep wells at ±140° and ±155°, associated with larger E_B values, plays a key role in observable MPV effects. The E_B between 140° and 150° was ~20 kcal mol⁻¹ by PM3MM and ~25 kcal mol⁻¹ by DFT. Similarly, the E_B between 150° and 210° was ~25 kcal mol⁻¹ by PM3MM and ~55 kcal mol⁻¹ by DFT. Chiroptical generation and inversion between RH and LH helices from the most stable +155° helix to the other +140°, −140°, and −155° helices are unlikely to occur easily because of the large E_B values.

The photoexcited energies (expressed in nm) at the S₁, S₂, and S₃ states (marked in red) and T₁, T₂, and T₃ states (marked in blue) significantly change as a function of DH due to the decrease in σ-conjugation of the Si wire (Figure 8c). The photoexcited energies at S₁, S₂, and S₃ tended to progressively blueshift when the DH changed from 180° to 80°, except for 145–150° and 135° located at barriers between 140° and 155° and between 130° and 140°. The UV/CD bands in the S₂ and S₃ states can be observed experimentally at wavelengths shorter than 280 nm and partly overlap with the UV/CD bands of the 100°-helix, whereas the UV/CD bands in the S₁ state appeared at wavelengths longer than 280 nm. The DH dependence of the photoexcited energies in the T₁, T₂, and T₃ states was nearly identical to those in the S₁, S₂, and S₃ states (Figure 8c). Their UV absorptions were experimentally less obvious because their f values (oscillator strengths) were theoretically zero.

It is noteworthy that when DH changes from 180° to 155°, the transition energy curve at the S₁ state approaches the curve at the T₂ state, and the curve at 140° is close to that of the T₃ state (Figure 8c). These situations led to mixing between the S₁, T₂, and T₃ states, resulting in a marked decrease in the e value, accompanied by an increase in De at 140°. Additionally, from E_PV ∝ ΔE_ST (Scenario E), a smaller S₀–T₁ transition energy at three energy minima, 130°, 140°, and 155°, in the range of 3.81 eV (325 nm) and 4.13 eV (300 nm) effectively contributes to amplify E_PV in the S₀ state because the T₁ is partly mixed with the S₁ and T₂ states.

The f values in the S₁, S₂, and S₃ states were altered by the DH (Figure 8d). When the DH decreases from 170° to 70°, f in the S₁ state progressively decreases from ~2.2 at 170° to ~0.0 at 130°. Conversely, f in the S₂ state increases from ~0.1 at 170° to 0.7–1.0 at 130–135°. Likewise, f in the S₃ state increases from ~0.1 at 170° to 0.7 at 120–125°. Consequently, when the DH changed from 180° to 125°, Δε increased; conversely, ε decreased (Figure 8e), leading to an increase in g_abs (= Δε/ε) (Figure 8e,f). Focusing on the three helices with DHs of 155°, 140°, and 130°, the UV/CD bands at 280 nm and 320 nm mainly arose from the S₁ state, while the UV/CD bands at 300 nm were mainly from the S₂ state (Figure 8d). The g_abs at 300 nm were approximately three times larger than those at 320 nm. This idea partly accounts for the reason why |g_abs| at 300 nm reaches ~10⁻² compared to |g_abs| at 320 nm of ~10⁻⁴ [22,23,159,160].

A considerably large E_B ranging from 15 to 50 kcal mol⁻¹ is crucial to largely suppress ΔE_± in multiple-well systems [113,187,188,189], leading to the emergence of observable MPV effects. These E_B values are intermediate in racemization processes between temperature-independent quantum tunneling and classical temperature-dependent Arrhenius law mechanisms [189]. From ΔE_± = 1/(2√π)·(hν·E_B)^1/2·exp(–E_B/hν), where h is the Planck constant, and ν is the frequency of vibration in a single well, tunnel-driven dynamic racemization is expressed as T_rac = h/ΔE_± [10,113,187,189]. For comparison, in the case of the quantum tunneling inversion of ammonia in a DWP, H₃N: ⇄ :NH₃, an E_B of 5 kcal mol⁻¹ at ν = 950 cm⁻¹ leads to a very fast T_rac ~ 10⁻¹¹ s, while the tunneling inversion of phosphine in a DWP, H₃P: ⇄ :PH₃, an E_B of 37 kcal mol⁻¹ at ν = 991 cm⁻¹ results in a very slow T_rac ~ 10⁷ s [10,113,189]. By placing two adjustable parameters in the above ΔE_± and T_rac equations, the T_rac values (in sec) as a function of E_B (kcal mol⁻¹) in the three cases of tunneling, Arrhenius law mechanisms, and their intermediate, are illustrated in Figure S12 (Supplementary Materials) [113,189]. The estimated E_B (PM3MM) of DES13 with two E_B ranges of ~20 kcal mol⁻¹ and ~25 kcal mol⁻¹ indicate an intermediate region, except for ~55 kcal mol⁻¹ (DFT) around DH 180° (Figure 8b) [189].

Qualitatively, a larger E_B in a DWP makes ΔE_± exponentially smaller, thereby satisfying the ΔE_± ~ E_PV condition, whereas a tiny E_PV is nearly unchanged around 10⁻¹⁰ eV. Experimentally, by increasing E_B, it is, in principle, possible to achieve ΔE_± ~ 10⁻¹⁰ eV by (i) increasing the viscosity of the solvents, (ii) increasing the steric hindrance between the side chains of chromophores, and (iii) cooling to a lower temperature. The cooperativity in the second-order phase transition originates from a continuous decrease from disordered to ordered states at low temperatures, i.e., superconductivity and BEC of HINS isotopes such as ⁸⁷Rb, ²³Na, ⁷Li, and ¹H [190].

3.2. Potential Energy Surfaces of H-(H₂Si)₁₃-H, Me-(Me₂Si)₁₃-Me, and Et-(Et₂Ge)₁₃-Et

The other PESs of H-(SiH₂)₁₃-H, Me-(SiMe₂)₁₃-Me, and Et-(GeEt₂)₁₃-Et (Chart S2 (Supplementary Materials)) obtained using PM3MM and DFT (B3LYP-631Gd) are compared in Figure S13 (Supplementary Materials). The global and local minima of H-(SiH₂)₁₃-H exist at 180° (trans-planar (TP)) and 60–70° (gauche helical (GH)), respectively, and the E_B values between TP and GH are as small as 0.6 kcal mol⁻¹ (PM3MM) and 0.8 kcal mol⁻¹ (DFT), respectively (Figure S13a,b, Supplementary Materials). However, the PES of Me-(SiMe₂)₁₃-Me suggests that the global and local minima are located at 180° (TP) and 90° (ortho helical (OH)), while the E_B values between the TP and OH conformers are 12 kcal mol⁻¹ (PM3MM) and 25 kcal mol⁻¹ (DFT), respectively (Figure S13c,d, Supplementary Materials). Similarly, the shallow energy levels at the two minima with lower E_B (indicated by yellow and gray) suggest a high susceptibility to fluctuations.

Similarly, the PES in hypothetical Et-(GeEt₂)₁₃-Et has two minima at ~155° and ~90°. Because of the similar shallow energy levels, the preference in the LH and RH helicity is rapidly lost owing to thermal fluctuations, as indicated by the blue, pink, and gray bars (Figure S13e,f, Supplementary Materials). Significant differences in local and global minima and their E_B values between Et-(SiEt₂)₁₃-Et and Et-(GeEt₂)₁₃-Et are largely ascribed to a subtle difference in bond lengths between Si–Si (2.10 Å) and Ge–Ge (2.22 Å) [185]: a shorter bond length results in a stronger steric repulsive force (Pauli exclusion principle) between bulkier pendant groups.

3.3. Potential Energy Surface with PVED of n-Alkanes

In principle, GH in n-alkanes can result in a metastable rotamer. Kikuchi et al. calculated the E_PV in a series of hypothetical GH n-alkanes [191,192]. The RH-GH of n-C₁₁H₂₄ is more stable by −5 × 10⁻¹⁸ kcal mol⁻¹. The E_PV of RH-GH linearly increases in proportion to the carbon number of n-alkanes (Scenario A) [191,192]. From studies of Raman, FT-IR, and theoretical simulations, the difference in energy between trans (t)- and gauche (g)-bonds per C–C bond, ΔE_tg, is 0.65–0.90 kcal mol⁻¹ in a series of n-alkanes from n-C₄H₁₀ to n-C₁₆H₃₄ [193,194,195,196,197,198]. DFT (B3LYP/6-311g**) calculations indicated that the E_B from t- to g-bonds is commonly ~3 kcal mol⁻¹ in a series of n-C₄H₁₀ to n-C₁₀H₂₂ [199,200]. In a series of carbon numbers from n-C₅H₁₂ to n-C₁₄H₃₀, liquid n-alkanes contain in total 34–41% as polar g-bonds, including one g-, two g-, and three g-sets, resulting in a weak dipole moment of 0.09 Debye [177,199,200,201,202,203,204,205,206,207,208,209]. Although n-C₄H₁₀ adopts ~16% g-bond, n-C₅H₁₂ and n-C₁₁H₂₄ contain ~25% g-bonds, and n-C₁₆H₃₄ is ~35% g-bonds [195,201,202,203,204,205,206]. According to a recent MM3 calculation of n-C₁₂H₂₆, the most dominant TP-conformer among the possible 7838 conformers is only ~15%, while five non-TP conformers, including one and two g-bonds, are ~15%, and other non-TP conformers are 70% [198]. On the other hand, Monte Carlo simulation indicates that TP-conformer content ranges from ~75% to ~85% for carbon numbers 6 to 12 in n-alkanes [197].

To confirm these results, we calculated two PESs of n-C₁₂H₂₆ as a function of DH 40–180° obtained using PM3MM and DFT (B3LYP/6-31Gd) (Figure S13g,h, Supplementary Materials). In both calculations, the PESs suggested a global minimum at 180° (TP) and a local minimum at 80° (OH), with an E_B of ~5 kcal mol⁻¹. The UV/CD spectra (fwhm 0.20 eV, TD-DFT) for several rotamers of n-C₁₂H₂₆ are summarized in Figure S14 (Supplementary Materials). Comparisons of the relative energy, dipole moments (μ, in Debye), and CD sign at λ_ext for 10 rotamers, including g⁺ ~ 60–80° (9 DH sets of C–C bonds), are listed in Table S1, Supplementary Materials. These data were sorted by the relative energy against TP [(t)₉], as shown in Figure S14 (Supplementary Materials).

The first metastable rotamer is the g⁺ rotamer [(g⁺)₁-(t)₈], which is unstable by 3.24 kcal mol⁻¹ relative to [(t)₉]. The value semi-quantitatively agrees well with the previous calculations [193,194,195,196,197,198,199,200]. The second metastable rotamers are a two g⁺ rotamer, [(g⁺)₂-(t)₇], and a one g⁺ rotamer, [(t)₁-(g⁺)-(t)₇], which are unstable by 4.23–4.45 kcal mol⁻¹. The third metastable rotamer is a one g⁺ rotamer, [(t)₂-(g⁺)-(t)₆], which is unstable by 5.74 kcal mol⁻¹. Interestingly, [(g⁺)-(t)₉] showed (+)-sign CD; conversely, [(g⁺)₂-(t)₇] and [(t)-(g⁺)-(t)₇] showed (−)-sign CD. Although the μ-value of TP is nearly zero, the other five rotamers, [(g⁺)₉], [(g⁺)₁-(t)₈], [(g⁺)₂-(t)₇], [(t)₂-(g⁺)-(t)₆], and [(t)₄-(g⁺)-(t)₄], show fairly large μ values ranging from 0.049 to 0.087 Debye. The other four g⁺-containing rotamers, [(t)₁-(g⁺)-(t)₇], [(g⁺)₃-(t)₆], [(g⁺)₄-(t)₅ ], and [(t)₃-(g⁺)-(t)₅], had smaller μ values (0.015 and 0.029 Debye). Liquid n-C₁₂H₂₆ and other n-alkanes are thus likely to contain a considerable amount of polar one- and two-g⁺ and/or g⁻ bonds and kinks (g⁺-t-g⁻ and g⁻-(t)_n-g⁺) [201,202,203,204,205,206,207,208,209]. The [(g⁺)₁-(t)₈], well-known as end-g⁺, is easily formed compared to interior g⁺-bonds, such as [(t)₁-(g⁺)-(t)₇], [(t)₂-(g⁺)-(t)₆], and [(t)₄-(g⁺)-(t)₄]. The fractions of the g-bonds increase as the temperature increases [201,202,203,204,205,206].

3.4. Electroweak Charges at Isotopes of PDBS and n-Alkanes

The electroweak charge, Q_W, is an important parameter in the Z⁰-force origin MPV theories [5,6,18,19,70,73,74,75,76,77,83]. Q_W is regarded as a naturally occurring additional charge on each spherical atom to afford the north and south poles induced by the handed WNC.

H_PV = − Γ/2 ∑ ∑ Q_W{p_i·σ_i, Δ³(r_i − r_a)}₊,

(1)

Γ = G_F/(2√2·m_e·c) = 5.731 × 10⁻¹⁷ a.u. equivalent to 1.559 × 10⁻¹⁵ eV.

(2)

Q_W = N_a − (1 − 4·sin²θ_w)·Z_a

(3)

Herein, the H_PV (PV-potential) is reduced by adding H_PV(e⁻-e⁻), H_PV(e⁻-p), and H_PV(e⁻-n) terms when e⁻–e⁻, e⁻–p, and e⁻–n interactions between e⁻, n, and p are considered. Additionally, { }₊ denotes an anticommutator. Γ combines the Fermi weak coupling constant G_F with the electron mass m_e and speed of light c. Each electron i has a charge density at each atomic nucleus, which is represented by a three-dimensional Dirac delta function, Δ³(r_i − r_a). In addition, p_i and σ_i are the electron momentum and Pauli spin-matrix operators, respectively. N_a and Z_a (=Z, mentioned above), respectively, are numbers of n and p of each atomic nucleus a in a molecule, and θ_w is the Weinberg mixing angle between W and Z⁰ bosons.

Using the recent value of sin²θ_w = 0.223 [210], it can be shown that for ¹H, Q_W = −0.108; for ²H (D), Q_W = +0.892; for ¹³C (NA 1.1%), +6.352; for ¹²C (NA 98.9%), +5.352; for ²⁹Si (NA 4.7%), +13.488; for ²⁸Si (NA 92.2%), +12.488; and for ³⁰Si (NA 3.1%), +15.849, where NA is the natural abundance of stable isotopes. Only the ¹H atom has a small (−)-sign Q_W. In contrast, ²H and other HINS and INS isotopes have a large (+)-Q_W. A large quantity of solvent organic molecules with multiple (−)-Q_W ¹H atoms surround the PDBS. The opposite sign in the Q_W between ¹H and ²H is related to the opposite CD sign characteristics between n-C₁₂H₂₆ and n-C₁₂D₂₆ and PDBS below T_C1 and T_C2 (Figure 2a and Figure 3b). In addition, (+)-Q_W HINS ²⁹Si can contribute to an MPV-causing “sergeant” to cooperatively align in a handed helical geometry of ²⁸Si and ³⁰Si as “soldiers” in the Si main chain, leading to the weaker MPV effect of PDBS observed in n-C₁₂D₂₆ [107].

3.5. CH₃ Hindered Rotor—Three Identical Nuclear Spin-1/2 System in a Triple-Well Potential

3.5.1. Ortho- and Para-Water to Account for the MPV-Experiment of L- and D-Oligopeptides

In 2006, Shinitzky et al. investigated the first-order α-helix-coil transition characteristics of an enantiomeric pair of L- and D-glutamic acid 24-mers [100]. They noticed subtle differences in the CD spectra between their random coils in H₂O at pH 10.5; however, no such difference was observed in the D₂O: H₂O (4:1) cosolvent. Isothermal titration calorimetry detected subtle differences in ΔH ~ −0.14 kcal per residue between the L- and D-oligopeptides in H₂O but did not show such ΔH in the D₂O: H₂O 4:1 mix. Based on the fact that H₂O consists of two nuclear spin isomers, para (↑↓, I = 0) and ortho (↑↑, I = 1) in 1:3 ratio, ortho-H₂O is assumed to solvate the L-oligopeptides preferentially over the D-oligopeptides [100]. The ortho- and para-spins in CH₂ moieties are applicable to n-alkanes and the n-butyl pendants of PDBS, although their total I values are perpetually 0 and 1, respectively.

3.5.2. Bi-Directional CH₃ Rotor by Tunneling and Non-Arrhenius Law Hopping

Based on the ortho-para spin states of H₂O, the nuclear spin states of CH₃ termini in n-alkanes and n-butyl pendants were first considered. A naive question is how three identical HINS atomic systems conserve P-symmetry, because even-number nuclear spin states (I = 0,1,2) are impossible. When considering a plausible explanation for the mechanism(s) of the observed MPV effects, the authors were aware of the uniqueness of the CH₃ group. Historically, in 1940, Koehler and Dennison were the first to argue theoretically regarding hindered and free rotations of the OH group in methanol using the energy potential V = 1/2·H·(1 − cos3θ) and energy splitting [211]. In the 1960s, Hilt and Hubbard [212] and, independently, Runnels [213] theoretically treated how a three identical nuclear spin-1/2 system relaxes along the three-fold hindered rotation axis. These old questions can nowadays be reduced to a geometrical frustration, i.e., spin frustration and degeneracy of HINS in odd-number rings including triangular lattices in condensed matter physics and topological material science [214,215,216,217,218,219].

From the notable HINS isotope effects to the APV and PV-MAM hypotheses (Scenario F), the present study highlights three-fold symmetric CH₃ and CD₃ groups as a fundamental issue in soft condensed matter. Thus far, it has been more-or-less established over the last four decades that CH₃ and CD₃ groups act as hindered rotors in a TWP owing to temperature-dependent quantum tunneling and non-Arrhenius law hopping mechanisms in the framework of PC-EMF [220,221,222,223,224,225,226,227,228,229,230,231,232,233,234,235,236,237,238,239,240,241,242,243,244]. Rotating the symmetry-top CH₄, NH₄⁺, and CH₃-group in a broad range of molecular crystals [220,221,222,223,224,225,226,227,228,229,230,231,232,233,234,235,236,237,238,239] and non-crystalline polymers [240,241,242,243,244] between 1 K and 390 K are elucidated by many theories and various measurements; i.e., inelastic neutron scattering (IENS), quasielastic neutron scattering (QENS), several ¹H-NMR techniques (i.e., spin lattice relaxation (T₁), low-magnetic-field, high-field to zero-field cycling, level crossing), ²H-NMR, electron nuclear double resonance (ENDOR), IR and Raman, microwave, viscoelastic relaxation, and photochemical hole burning [220]. The CH₃ group possesses a perpetual I = 3/2 (↑↑↑, ↓↓↓, A-state) and I = 1/2 (↑↓↑ and ↑↓↓, E-state). Contrarily, the CD₃ group of three identical ²H isotopes generates I = 0,1,3 (A-state) and I = 1,2 (E-state) [235,236,237,238,239]. However, theoretical and experimental investigations involving the nuclear spin-dependent MPV hypothesis have not yet been reported.

In 1991, Clough pointed out that the origins of CH₃ and CD₃ rotating motions in a TWP remain unanswered questions regarding the four symmetry breakings: geometrical, T-reversal, rotational, and permutational symmetries [227]. These issues can be reduced to the superposition of three identical 1/2-nuclear spin states among three indistinguishable ¹H atoms that split into four A-sublevels and two sets of E_a-/E_b-sublevels under a non-zero external magnetic field in NMR. The A- and E-states contradict the Pauli exclusion principle. Zero-field NMR spectroscopy and sophisticated chiroptical spectroscopy can be used to answer these questions [90]. In addition, the CH₃ rotating trajectory can be regarded as a set of helices in which the rotation is linked with environmental forces because the rotor acts as a clock and pendulum in the curvature of the space-time world [227]. However, no study has experimentally validated the four-symmetry breakings of CH₃ and CD₃ rotors.

The CH₃ and CD₃ hindered rotors can generate several g-bonds in liquid n-alkanes and n-butyl pendants due to the similar E_B values in non-deuterated and deuterated polymers [240,241,242,243,244]. Their quantum tunneling and non-Arrhenius law hopping characteristics are significantly different due to the inherent moment of inertia (I_rot) and rotational constant (B), B = ℏ²/(2I_R) when considered as rigid rotors: for CH₃, B = 0.655 meV (I_rot = 0.535 × 10⁻⁴⁶ kg m⁻²) and for CD₃, B = 0.328 meV (I_rot = 1.069 × 10⁻⁴⁶ kg m⁻²) [220,222,238]. In fact, the differences in the B and I_R of CD₃ result in the marked suppression of tunneling splitting (E_A–E) and tunneling frequency by one or two orders of magnitude [220,222,238]. The reduced tunneling frequency of the CD₃ rotor causes inefficient intra- and intermolecular chirality transfers.

By considering several graphical explanations for the permutational quantum tunneling of the CH₃ rotor in TWP [220,221,222,223,224,225,226,227,240,241,242,243,244], Figure 9a reproduces the hypothetical potential energy E(θ) as a function of CH₃ rotation angle (θ); E(θ) = V₃/2·{1 − cos(3θ)}, V₃ ≃ E_B = 25.2 meV (1.1 kcal mol⁻¹) while the V₃ is taken from CH₃NO₂ [221,224,225]. E(θ) exhibited three global minima at θ = ±120° and 0°. The E(θ) at each well (1, 2, 3) splits into A- and E_a-/E_b-states owing to quantum tunneling, whereas E_a and E_b have equal energies owing to degeneracy. The relative stability between A- and E_a/E_b-states alternatively changes with ν = 0, 1, 2 [222]. Representative IENS spectra at cryogenic temperatures revealed two well-resolved A → E_a/E_b and E_a → E_b/E_b → E_a transitions at zero frequency [221,227]. With an increase of temperature, tunneling splitting energy, E_A–E (in eV, cm⁻¹, Hz), exponentially decreases, approaching the zero-frequency. When E_a = E_b, a pair of CW and CCW rotations between three potential wells—well 1, well 2, and well 3—occurs equally, leading to a random hopping of bi-directional rotations resulting in the lack of macroscopic level optical activity.

3.5.3. Unidirectional CH₃ Rotor Induced by E_PV

To address the four symmetry questions raised by Clough [227], the authors would like to mention a toy model of unidirectional CW (or CCW) rotation at a macroscopic level as a low-entropy state. The E_a–E_b degeneracy is “lifted” by an external magnetic field (Zeeman effect), an external electric field (Stark effect), a built-in internal electric field (dipole moment), and possibly Z⁰-WNC. When the E_A–E ~ E_PV condition is satisfied (Scenario D), a handed CH₃ rotating action becomes possible. First, A, E_a, and E_b at well 2, biased by −E_PV, were postulated. At well 3, A*(3) = A − E_PV, E_a*(3) = E_a − E_PV, and E_b*(3) = E_b − E_PV; conversely, at well 1 biased by +E_PV, A*(1) = A + E_PV, E_a*(1)= E_a + E_PV, and E_b*(1) = E_b + E_PV.

Figure 9b illustrates the modified potential energy E(θ) biased by ±E_PV as a function of θ. Because A(2) ≠ A*(1) ≠ A*(3), E_a(2) ≠ E_a*(1) ≠ E_a*(3), and E_b(2) ≠ E_b*(1) ≠ E_b*(3) at ν = 0,1,2.., there is unequal permutational tunneling between three potential wells—well 1, well 2, and well 3—with CW rotation, [well(2) → well (3) → well(1) → ]_n, leading to handed CH₃ rotation at low temperatures. When E_a in well 2 (ν = 0) is coincident with E_b + E_PV in well 3 (ν = 0), resonant tunneling endowed with several chiral phonon modes occurs at ν = 0. Similarly, E_b in well 2 (ν = 1) is coincident with E_a + E_PV in well 3 (ν = 1), and resonant tunneling with the help of several chiral phonons (from ν = 0 to ν = 1) is possible. Even if non-Arrhenius law hopping is dominant at higher temperatures, handed permutational rotation is likely to occur with a small probability because of the high cooperativity in the condensed phase to minimize the entropy at the macroscopic level. This assumption can be confirmed by the emergence of several (−)-sign vibrational circular dichroism (VCD) signals at the corresponding IR bands, characteristic of CH₃ rotors and CH₂ skeletal g-bonds in several liquid n-alkanes, as discussed in Section 3.6.

To determine the relationship between the E_B dependence of the tunneling splitting/frequency (E_A–E) with a small E_PV, two universal correlation curves of E_A–E in CH₃ rotation at vibrational quantum number ν = 0 as functions of barrier heights, V₃ ≃ E_B (in kcal·mol⁻¹), E_B (from ν = 0 to ν = 1), and re-orientational temperature, θ_min (in K) are compared [222,224,225,226,227,228]. Here, θ_min determined by the T₁ measurement of ¹H-NMR is a crossover temperature between temperature-dependent quantum tunneling and non-Arrhenius law hopping processes, although the transition appears to be continuous.

Figure 9c shows the E_A–E in molecular crystals as functions of θ_min, V₃ ≃ E_B (red circles and red line), and E_B(0–1) (blue circles and blue line) [222,224,225,226,227], while Figure 9d plots the E_A–E obtained in non-crystalline polymers as a function of E_B (red line) [240,241,242,243,244], with red circles taken from Figure 9c [224,225,226]. The two E_A–E vs. E_B correlation curves are very similar, regardless of whether considering crystalline molecular or non-crystalline polymer solids. When the E_B of the CH₃ rotor, E_B(CH₃), varies slightly from 2 to 7 kcal mol⁻¹, the E_A–E decreases exponentially from 10⁻⁷ to 10⁻¹⁴ eV, corresponding to 10⁻³ to 10⁻¹⁰ cm⁻¹ (3 × 10⁷ to 3 × 10¹ Hz). Consequently, E_A–E becomes comparable to the theoretical E_PV, and as a result, the E_A–E (= ΔE_±) ~ E_PV condition (Scenario D) is experimentally feasible.

The E_B(CH₃) in the crystals ranges from 0.1 to 5 kcal mol⁻¹ (50–2500 K, 0.004–0.21 eV, 35–1750 cm⁻¹) [220]. At 4.2 K, the tunneling frequency of CH₃ rotors is ~ 300 KHz for even-carbon number n-alkanes including n-C₁₂H₂₆, and 340 KHz for odd-carbon number n-alkanes, including n-C₁₃H₂₈ [220,232,233,234]. The two frequencies correspond to E_A–E ~ (1.3–1.4) × 10⁻⁹ eV with E_B(CH₃) ~ 2.8 kcal mol⁻¹. On the other hand, the rotational frequency of E_B(CH₃) directly attached to the main chain of PMMA, which is known as γ–relaxation, is only ~1 KHz (4 × 10⁻¹² eV) below 120 K. The corresponding E_B(CH₃) values in isotactic- and syndiotactic-PMMA are evaluated to be 3.3 kcal mol⁻¹ and 6.7 kcal mol⁻¹, respectively [220,240,242].

The liquid n-alkanes containing PDBS in the range of −10 °C and 110 °C are likely to obey the E_A–E–E_B correlation curves in Figure 9c,d. When E_B(CH₃) ≳ 3.8 kcal mol⁻¹, the E_A–E approaches the predicted E_PV value of 10⁻¹⁰ eV, and when E_B(CH₃) ≳ 6.4–7.3 kcal mol⁻¹, the E_A–E approaches the E_PV value of 10⁻¹³ eV, as shown by the blue zone in Figure 9d and the green zone in Figure 9c. The rotational frequency of CH₃ rotors in n-butyl pendants becomes very small and E_B(CH₃) is likely to be ≳ 6 kcal mol⁻¹, which is considerably higher than E_B(CH₃) ~ 2.8 kcal mol⁻¹ of n-alkanes. Even with a higher E_B(CH₃), the CH₃ rotors of n-alkanes and n-butyl groups might feel the handed E_PV force embedded in the non-Arrhenius law hopping process.

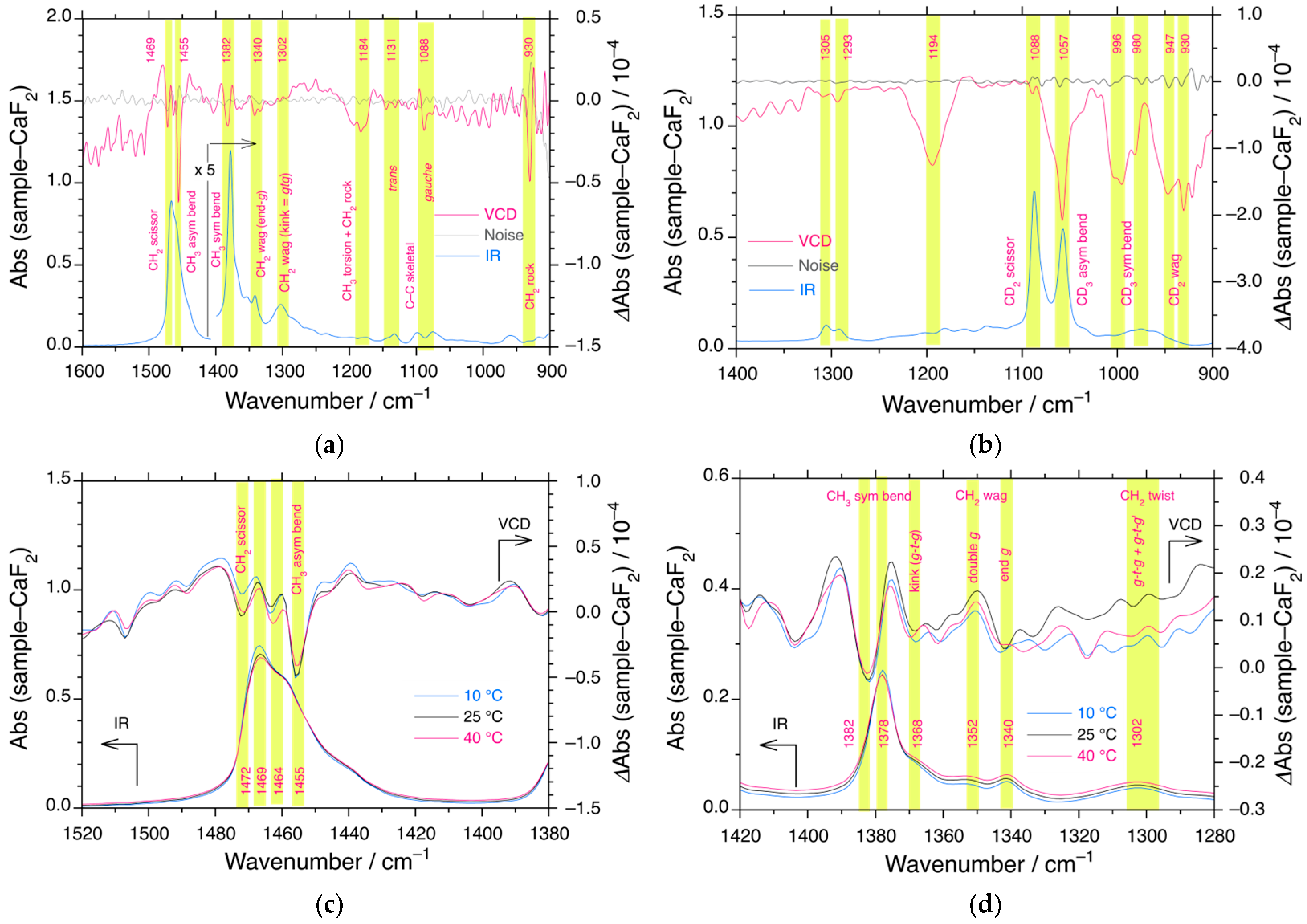
3.6. Are n-Alkanes Optically Active? VCD and IR Spectra of n-C₁₂H₂₆, n-C₁₂D₂₆, and Other n-Alkanes

3.6.1. Detecting the Gauche Bonds in n-Alkanes

The quantum tunneling of CH₃ hinders the rotor in TP n-alkanes in the solid state, which is a dominant process at cryogenic temperatures. The CH₃ rotation in odd-number carbon n-alkanes (carbon number from 9 to 29) results in non-TP conformers involving one and multiple g⁺ and g⁻ bonds above their melting points and liquid states [220,232,233,234]. As discussed in Section 3.3, our calculated E_B values from t- to one/two g-bonds of n-C₁₂H₂₆ are on the order of 3.24 to 5.74 kcal mol⁻¹ (Table S1, Supplementary Materials). The production of single, double, and kink g⁺ and/or g⁻-bonds are experimentally detectable by IR and Raman spectroscopy [178,193,194,195,196,197,198,200,201,202,203,204,205,206,207,208,209]. For example, using IR spectroscopy, several characteristic bands, i.e., 873/975/1078/1164/1341 cm⁻¹ for end-g bonds, 1353 cm⁻¹ for gg-bonds, and 1306/1366 cm⁻¹ for g-t-g* (kinks), respectively, can be recognized when compared to 1378 cm⁻¹ for the CH₃ group [178,201,202,203,204,205,206,207,208,209].

3.6.2. CD/UV Background Spectra of Liquid n-C₁₂H₂₆

In recent years, Ozaki et al. investigated the electronic transition states of n-C₅H₁₀ to n-C₁₄H₃₀ using attenuated total reflection far-ultraviolet (ATR-FUV) spectroscopy and TD-DFT/SAC-CI calculations [245,246,247]. They assigned several fundamental FUV bands of TP n-alkanes: the strongest FUV band around 145 nm was a mixture of HOMO → Rydberg 3p and HOMO-1 → Rydberg 3s transitions, and the weak shoulder FUV band around 165 nm was assigned to HOMO → Rydberg 3s. The major 145 nm and shoulder 165 nm bands should tail to the near-UV region in the range of 250–400 nm, which is seen as a structureless background (Figure S6a, Supplementary Materials). When n-alkanes are involved in a non-mirror-symmetric g⁺ or g⁻ geometry, the 165 nm band tails more strongly in the CD/UV region. In fact, n-C₁₂H₂₆ showed weak temperature-dependent CD and UV spectra even after subtracting the instrumental background at −5 °C, 50 °C, and 100 °C (Figure S6b, Supplementary Materials).

3.6.3. VCD/IR Spectra of n-Alkanes

Assuming g⁺ > g⁻ due to the E_PV of RH-GH in n-C₁₁H₂₄ [191,192], the CH₃ rotor then has a unique handedness, which is responsible for the transfer of solvent chirality to n-butyl pendants and hence the Si main chain. When n-C₁₂H₂₆ is between −10 °C and T_C2, the [(g⁺)-(t)₉] conformer showing (+)-sign CD as the dominant species strongly induces 300 nm RH-helicity and (+)-sign CD in PDBS. Conversely, when n-C₁₂H₂₆ is between T_C2 and T_C1, [(g⁺)₂-(t)₇] and [(g⁺)-(t)₉] as the dominant species induce (−)-sign CD helicity. When n-C₁₂H₂₆ is above T_C1, mutual cancellation between the (+)-and (−)-sign CD in g⁺-rotamers results in a lack of any induced MPV effects.

Inspired by the symmetry breakings proposed by Clough [227], if n-alkanes without point chirality adopt chiral conformers, VCD and ROA spectroscopy should detect several optically active vibration bands characteristic of the handedness of stretching, bending, rocking, and torsion modes of the CH₃ group, and stretching, bending, and twisting modes for CH₂. The VCD and IR spectra datasets of liquid n-C₁₂H₂₆ and n-C₁₂D₂₆ at 10 °C (below T_C2), 25 °C (~T_C2), and 40 °C (above T_C2) are shown in Figure 10a–d and Figures S15 and S16, Supplementary Materials.

Several (−)-sign VCD signals of n-C₁₂H₂₆ and n-C₁₂D₂₆ are observed. The VCD spectral profiles are independent of temperature, as shown in Figures S15 and S16 (Supplementary Materials). In line with the handbooks of IR/Raman spectroscopies [248,249], a previous IR study of liquid and solid n-C₁₂H₂₆ [178,201,250] assigned three major characteristic IR bands at 1466 cm⁻¹, 1456 cm⁻¹, and 1382 cm⁻¹, respectively, to CH₂ (scissoring), CH₃ (asym, bending), and CH₃ (sym, bending or “umbrella”). The t- and g-bonds in the C–C skeletal IR bands are observed at 1133 cm⁻¹ and 1076 cm⁻¹, respectively (Figure 10a). The (−)-sign VCD band at 1088 cm⁻¹ (a handed g-bond) is rather weak, and the VCD band of the t-bond is even less obvious. Although a sharper (−)-sign VCD band characteristic of CH₃ (rocking) is observed at 930 cm⁻¹, the corresponding IR bands around 929 cm⁻¹ are very weak. The combination band of CH₃ torsion and CH₂ rocking modes at 1184 cm⁻¹ is weak, and the corresponding IR bands are also not obvious.

The (−)-sign VCD band of n-C₁₂H₂₆ at 1456 cm⁻¹, which is coincident with the 1456-cm⁻¹ CH₂ bending IR band, is observed as a shoulder of the 1466-cm⁻¹ CH₂ scissoring IR band (Figure 10c). The dissymmetry ratio of the vibration at 1456 cm⁻¹, g = ΔAbs/Abs = −1.6 × 10⁻⁴, could be evidence of the unidirectional CH₃ rotation in n-C₁₂H₂₆, for example, from the A*-state at ν = 0 to the E_b* (or E_a*)-state at ν = 1. The multiple (−)-sign VCD bands around 1470 cm⁻¹ at the 1456-cm⁻¹ CH₂ scissoring IR band and the (−)-sign VCD and IR bands of the g-bond at 1088-cm⁻¹ are related to the preference of g⁺ over g⁻ bonds.

The VCD and IR spectra of liquid n-C₁₂D₂₆ can be observed more clearly, as shown in Figure 10b and Figure S16 (Supplementary Materials). Three major IR bands of n-C₁₂D₂₆ at 1088 cm⁻¹, 1057 cm⁻¹, and 975 cm⁻¹ were assigned to the CD₂ (scissoring), CD₃ (asym, bending), and CD₃ (sym, bending, ‘umbrella’) modes. Notably, the clear (−)-sign VCD band at 1057 cm⁻¹ is coincident with the 1057-cm⁻¹ CD₃ IR band. The value of g = –4.0 × 10⁻⁴ at 1057 cm⁻¹ is ~ two times larger than the corresponding 1456-cm⁻¹ VCD/IR bands of CH₃ in n-C₁₂H₂₆. This is evidence of the handed CD₃ rotor in n-C₁₂D₂₆ being in the same direction as the CH₃ rotor. At the shoulders of several broad IR bands centered at 980 cm⁻¹, three (−)-sign VCD bands centered at 996 cm⁻¹ accompanied by several (−)-sign VCD bands centered at 930 cm⁻¹ are observed, suggesting the inequality of the CD₃ bending modes. However, the VCD band at the CD₂ (scissoring) IR band at 1088 cm⁻¹ is less obvious, indicating no preference between g⁺ and g⁻ bonds.

Similarly, the other five liquids, n-C₈H₁₈, n-C₁₀H₂₂, n-C₁₃H₂₈, n-C₁₄H₃₀, and n-C₁₆H₃₄, at 25 °C revealed several (−)-sign VCD bands in the range 900–1600 cm⁻¹, as shown in Figure S17a–e (Supplementary Materials). Several characteristic VCD/IR bands of CH₂ at 1464–1472 cm⁻¹ (scissoring), CH₃ at 1455–1466 cm⁻¹ (asym, bending), CH₃ 1377–1380 cm⁻¹ and 1360–1361 cm⁻¹ (sym, bending), and the handed g-bonds at 1086–1088 cm⁻¹, respectively, can be seen. The VCD bands due to CH₂ around 1300 cm⁻¹ (twisting) and the t-bonds in the C–C skeletal IR bands at 1131–1135 cm⁻¹ were less obvious. Likewise, the combination band of CH₃ torsion and CH₂ rocking modes at 1172–1184 cm⁻¹ was weak, and the corresponding IR bands were less obvious.

3.6.4. VCD, ROA, and Near-IR CD Spectroscopy

The detection of several VCD/ROA bands and high-resolution IENS signals as torsional modes (from ν = 0 to ν = 1) in Figure 9b in the range of 1–350 cm⁻¹ might constitute direct evidence of MPV. The asymmetric (~2960 cm⁻¹) and symmetric (~2870 cm⁻¹) stretching modes of the CH₃ group and the asymmetric (~2960 cm⁻¹) and symmetric (~2850 cm⁻¹) modes of the CH₂ group provide additional evidence. Further, several overtones and combination bands, such as the first overtone CH₃ (torsion) at ~620 cm⁻¹, CH₃ (torsion) + CH₃ (asym, bending) at ~1800 cm⁻¹, and CH₃ (torsion) + CH₂/CH₃ (stretching) at ~3300 cm⁻¹, are useful [244].

A near-IR CD spectropolarimeter with an InGaAs detector covering ~1600 nm (~1.29 eV, ~6250 cm⁻¹) allowed the detection of optical activity in the second, third, and fourth overtones of C–H stretching, bending, and their combination bands as liquids as a function of temperature by choosing several path lengths in the range of 10–200 mm and subtracting the background spectra of the cuvette and window glass. The near-IR CD spectra of the CD₃ and CD₂ groups can be compared to those of the third and fourth overtone frequencies, which are reduced by ~1/√2.

3.7. Possible Scenarios for the MPV Effects of PDBS with n-Alkanes

Based on the above results and discussion, a possible scenario for the dual mirror-symmetry breaking of PDBS in n-dodecane is proposed. As mentioned in the Introduction, pyrromethenes 597 and 546, carrying three CH₃ groups (Chart S1, Supplementary Materials), showed clear (−)-CD spectra in solution at 25 °C [114]. From the temperature-independent (−)-sign VCD spectra of liquid n-alkanes, the CH₃ rotor consisting of three ¹H atoms (Q_W = –0.108 × 3 = –0.324) is likely to function as a unidirectional hindered rotor mediated by E_PV. The rotor characteristics obey the non-Arrhenius law of hopping at higher temperatures and quantum tunneling at lower temperatures, although there is no critical border and the transition between the two mechanisms is continuous as the temperature changes (Figure 9c,d, and Figure S12, Supplementary Materials). Similarly, the CD₃ rotor consisting of three ²H atoms prefers CW rotation because of the same (−)-sign VCD bands and a similar magnitude of g ~ −10⁻⁴ for the symmetric bending modes of CH₃ at 1456 cm⁻¹ and CD₃ at 1057 cm⁻¹.

According to the MPV theory for GH n-alkanes, the RH helix consisting of all-g⁺ bonds is more stable than the LH helix with all-g⁻ bonds, suggesting that the CH₃ rotor prefers CW rotation. The enantiomeric pairs between the g⁺ and g⁻ bonds and between the g⁺g⁺ and g⁻g⁻ bonds are energetically unequal. The g⁺ and g⁺g⁺ bonds are in excess of the corresponding g⁻ and g⁻g bonds. The CW-CH₃ rotor generates end-g⁺, other g⁺, and g⁺g⁺ bonds in TP n-alkanes and TP n-butyl pendants. Non-TP n-alkanes involving one and multiple g⁺ bonds and g⁺g⁺ bonds thus become optically active chiral solvents, enabling the induction of handed helicity in mirror-symmetrical 7₃-helical PDBS via intermolecular chirality transfer interactions.

To explain the notable H/D isotope effect of T_C1 evident in the g_abs–T curves in Figure 2a and Figure 3b, the relative ratio of θ_D and θ_D^rel between n-C₁₂D₂₆ and n-C₁₂H₂₆ was obtained from the asymmetric bending modes of CD₃ (1057 cm⁻¹) and CH₃ (1456 cm⁻¹), yielding θ_D^rel = 0.726. θ_D^rel predicted a T_C1 of 274 K (1 °C) for n-C₁₂D₂₆ when a T_C1 of 378 K for n-C₁₂H₂₆ was chosen. Similarly, the ratios of the highest asymmetric/symmetric stretching frequencies between CD₃ (2216/2073 cm⁻¹) and CH₃ (2959/2875 cm⁻¹) [251,252] led to θ_D^rel = 0.748/0.721, corresponding to T_C1 of 282 K (9 °C) and 273 K (0 °C), respectively. The fundamental vibrational modes of these solvent molecules account for T_C1 as an observable MPV effect. These simple evaluations agree with T_c ∝ θ_D predicted by Salam’s theory. In contrast, the (−)-sign Q_w of ¹H and (+)-sign Q_w of ²H as solvent molecules induced opposite helicity in PDBS below T_C1 and T_C2. The difference in |g_abs| is ascribed to the inherent difference in I_rot and B between CH₃ and CD₃ rotors, as indicated by the marked reductions in E_A–E by two to three orders of magnitude [220,222,235,236,237,238,239].

From Table S1, Supplementary Materials, it is interesting to note that the end g⁺ bond, (g⁺)₁-(t)₈, and middle g⁺ bond, (t)₂-(g⁺)₁-(t)6, show (+)-sign CD spectra at λ_ext of n-C₁₂H_26, whereas the g⁺g⁺ bond, (g⁺)₂-(t)₇, and other g⁺ bonds, like (t)₁-(g⁺)₁-(t)₇, show (−)-sign CD effects.

First, intramolecular chirality transfer was considered. (a) When T < T_C2, the ²⁹Si-driven torque overcomes the CH₃ torque, leading to a (+)-CD Si–Si bond helix in the presence of n-C₁₂D₂₆; conversely, when T_C2 < T < T_C1, the CH₃-rotor driven torque overcomes that of ²⁹Si, leading to a (−)-CD Si–Si bond helix (Figure 11a). On the other hand, below T_C2, the CW-CH₃ hindered rotor generates the (g⁺)₁-(t)₈ bond in n-C₁₂H₂₆ with (−)-sign CD (Figure 11b). When T_C2 < T < T_C1, the CW-CH₃ hindered rotor induced (t)₁-(g⁺)₁-(t)₇ and (g⁺)₂-(t)₇ rotamers with (−)-sign CD (Figure 11b). Next, intermolecular chirality transfer between n-C₁₂H₂₆ and PDBS was considered. When T < T_C2, the (g⁺)₁-(t)₈ rotamer induces a (−)-CD Si–Si helix. In contrast, when T_C2 < T < T_C1, the (t)₁-(g⁺)₁-(t)₇ and (g⁺)₂-(t)₇ rotamers induced the formation of a (+)-CD Si–Si helix. Another explanation for the observed optical activity of PDBS in n-C₁₂H₂₆ and n-C₁₂D₂₆ arises from the opposite Q_w values between the three ¹H isotopes in the CH₃ rotor (Q_w = −0.108 × 3 = −0.324) and the three ²H isotopes in the CD₃ rotor (Q_w = +0.892 × 3 = +2.772).

Two different CH₃ rotors (I = 3/2, μ_N = +2.79 × 3 = +8.37) of n-C₁₂H₂₆ and n-butyl of PDBS are schematically illustrated in Figure 11. Because the tunneling frequency and splitting of the CD₃ rotor markedly diminish relative to those of the CH₃ rotor [220,222,235,236,237,238,239], the helix preference of the PDBS in n-C₁₂D₂₆ is dominantly determined by the relative magnitude and direction of the rotational torques between the two CH₃ rotors and the 4.7% ²⁹Si (I = 1/2, μ_N = –0.55). From NMR experiments, it is known that ¹H and ¹³C isotopes prefer α-spin (↑), and conversely, ²⁹Si prefers β-spin (↓) by Zeeman splitting under an external magnetic field (H₀). It is possible that ¹H and ²⁹Si induce opposite rotational torque in the absence of H₀.

3.8. Added in Proof—Other Oligo- and Polysilanes for the MPV Validation

To validate the calculated results of the PESs (Figure 8 and Figure S13c–f, Supplementary Materials) experimentally, the CD/UV spectra of permethyldecasilane (PMDS) and poly(diethylsilane) (PDES) (Chart S1, Supplementary Materials) were measured under static conditions. PMDS in n-propanol did not exhibit CD effects because of the lower E_B in the tetra-wells (Figure S18a, Supplementary Materials). In contrast, PDES in n-propanol, n-butanol, and n-hexanol showed weak (+)-sign CD effects at longer λ_ext values relative to the corresponding UV spectra at shorter λ_max values (Figure S18b–d, Supplementary Materials); it is evident that the CD spectral profiles did not match the corresponding UV profiles. The (+)-sign g_abs of PDES in n-propanol as a function of temperature indicated T_C1 ~ 30 °C (Figure S18e, Supplementary Materials).

Prior to the VT-CD/UV spectral measurements of PDBS, PDES, and PMDS in n-alkanes, M.F. and J.R.K. at NTT Basic Research Labs in the 1980s and the 1990s were aware of the (−)-sign CD spectra of poly{bis-(p-t-butylphenyl)silane) (PBtBPS) (Chart S1, Supplementary Materials) in tetrahydrofuran (THF) at −10 °C, 25 °C, and 50 °C (Figure S19a, Supplementary Materials), in addition to the weak (−)-sign CD effects of poly(n-hexyl-2-methylpropylsilane) (PH2MPS) (Chart S1, Supplementary Materials) in isooctane at 0 °C (Figure S19b, Supplementary Materials). Importantly, the CD spectral profiles at shorter λ_ext values do not match the corresponding UV spectra.

In 2018, T.M., M.F., and Dr. Ayako Nakao working at NAIST recognized that PDBS-H in a mixture of n-C₁₂H₂₆ 98.3%/THF 1.7% (v/v) under static conditions reveals opposite signed temperature dependent CD spectra in n-C₁₂H₂₆ at 0 °C, 20 °C, and 45 °C when a small amount (50 μL) of 10⁻³ M THF solution of PDBS-H was added to 2.95 mL of n-C₁₂H₂₆ (Figure S19c, Supplementary Materials). However, the PDBS-H in pure THF did not exhibit any detectable CD effects at −10 °C. The oxygen atoms of a trace amount of THF coordinate to the Si 3d or Si σ* orbitals, resulting in intermolecular C–H/O interactions with the n-butyl pendants.

Interestingly, poly(n-hexyl-2-ethylbutylsilane) (PH2EBS) (Chart S1, Supplementary Materials) exhibited noticeable solvent and temperature dependence of the CD/UV spectra. The CD spectra of PH2EBS in n-C₁₂H₂₆ under static conditions are completely opposite to those in n-octanol at the three temperatures (Figure S19d,e, Supplementary Materials). The g_abs values at 310 nm as a function of temperature are shown in Figure S19f, Supplementary Materials. Clearly, PH2EBS undergoes a second-order helix–helix transition at T_C2 ~ 30 °C, which is associated with the oppositely signed CD signals in n-C₁₂H₂₆ and n-octanol. The CD spectrum of PH2EBS in n-octanol almost disappears at T_C1 ~ 110 °C and recovers mirror-symmetric 7₃-helices absorbing at 320 nm. The oxygen atom of n-octanol coordinates to the 3d orbital or Si σ* of the Si atom and/or stimulates the intermolecular C–H/O interactions with alkyl pendants.

Five other poly(dialkylsilane)s, including poly(methyl-n-propylsilane), poly(n-pentyl-n-propylsilane), poly(di-n-pentylsilane), and poly(di-n-hexylsilane) (Chart S1, Supplementary Materials), did not induce clear CD spectra in n-alkanes, n-alkanols, THF, and CHCl₃ at 0 °C. However, the authors found very clear CD and CPL spectra for several new polysilanes carrying designed achiral pendants in homogeneous solutions of n-alkanols, n-alkanes, and p-dioxane under static conditions. These results will be submitted elsewhere.

3.9. Other MPV-Related Experimental Studies

Most MPV scientists have sought possible answers to the issue of the origin of homochirality on Earth for the past 150 years: why and how have D-sugars and L-amino acids become the building blocks of our lives? And which deterministic or by-chance mechanism is the origin [1,2,3,4,5,6,7,8,9,10,11,12,13,14,15,16,17,18,19,20,21,22,23,24,25,26,27,28,29,30]? From the viewpoint of P-symmetry violation and CP-symmetry invariance in physics, true antipodes of D-sugars and L-amino acids are (L-sugars)* and (D-amino acids)* made up of anti-atoms [11,13,21,22,23,24]. The anti-atoms are comprised of anti-p, anti-n, and e⁺, whereas anti-p and anti-n, respectively, are made of anti-u and anti-d quarks [11,13,24]. Only e⁺ are manifested daily in regulated laboratories and hospitals utilizing short-half-life radioisotopes.

In recent years, synthetic biology, in designing mirror-image enzymes and pharmaceutics by producing non-natural DNA/RNA made up of L-sugars and non-natural proteins building-up D-amino acids, has received considerable attention in biotechnology and life science [253,254,255,256,257,258]. However, it remains unclear whether natural D- and non-natural L-sugars and natural L- and non-natural D-amino acids and their corresponding oligomers and macromolecules rigorously preserve mirror-image relationships in their structures and functionalities.

A few structural biologists are aware of noticeable differences between non-natural L- and natural D-RNA oligomers [259,260,261,262]. Single-strand RNA is classified as a floppy macromolecular rotamer with rotational and flip-flop freedom along C–C/C–O/C–N single bonds. When it produces a double-strand, there is a concomitant loss of freedom. In 2004, Betzel et al. reported clear dissimilarity between L- and D-RNA duplexes by crystallographic analysis: L-RNA adopts two types of helical duplexes with head-to-tail packing; conversely, D-RNA adopts a Watson–Crick type with an end-to-end duplex with a wobble-like pair. The discrepancy is ascribed to inherent differences in nature between D-ribose and L-ribose based on MPV calculations of D-ribose [259,260,261,262]. Subsequently, they reported other evidence, namely the different intensities of two Raman bands, at 1000 cm⁻¹ and 1700 cm⁻¹, between D- and L-RNA. The differences in intensity are ascribed to differences in the electronic levels between the D- and L-RNAs. Rypniewski et al. and Bolik et al. stated that the notable dissimilarity between D- and L-RNA crystals originates from the PVED-WNC of DL-ribose [259,260,261,262]. As an alternative explanation, phosphate O=PO₃⁻ with one HINS ³¹P atom (natural abundance: 100%) has a very large ξ = 475 cm⁻¹ [= P (247 cm⁻¹) + 4 × O (57 cm⁻¹)] and works as a unidirectional rotor along P–O–C bonds, leading to dissimilarity between the D-/L-RNA.

Compared to D-/L-RNA, the MPV effect of L-/D-amino acids has a long history. In 1973, Garay et al. were the first to report the differences in positronium (Ps) annihilation characteristics between five kinds of L- and D-amino acids using e⁺ as a probe [263]. Note that Ps generates two e⁺-e⁻ species of singlet (I = 0, para-Ps) with a shorter half-life (τ_s ~ 2·10⁻¹⁰ s) and triplet (I = 1, ortho-Ps) with a longer half-life (τ_t ~ (5–40)·10⁻⁹ s) in a 1-to-3 statistical ratio. The population of ortho-Ps relative to para-Ps is directly related to the annihilation intensity of ortho-Ps. Among the five DL pairs of amino acids, the annihilation intensity of ortho-Ps in L-amino acids was 6–33% weaker than that of the corresponding D-amino acids. D-Amino acids have greater free volumes and lower specific densities than L-amino acids. Although further clarification is needed, this astonishing result implies violations of CP- and P-symmetries between natural L- and non-natural D-α-amino acids. This means a violation of T-symmetry invariance between L- and D-α-amino acids.

Inspired by Salam’s hypothesis [107,108], in the early 2000s, Wang et al. reported differences between D-L-α-alanine crystals using VT solid-state ¹³C-/¹H-NMR, Raman spectroscopy, magnetic susceptibility, and DSC from room temperature to cryogenic temperatures [96,97]. An independent research team, however, did not observe such significant differences and ascribed the differences to unresolved impurities imbedded in non-natural D-alanine [264]. If Wang’s results are correct, IENS tunneling spectroscopy will detect differences in the CH₃ rotor between D- and L-α-alanine crystals at 4 K because the D-L chirality is unchanged. Recently, Bordallo et al. reported dissimilarity between crystalline L- and D-α-alanine by VT-polarized Raman spectroscopy and neutron diffraction [265] but had many debates regarding their results [266,267].

In 2021, Koralewski et al. reported that H₂O commonly shows (+)-sign-specific Verde constants at 546.1 nm in magneto-optical rotatory dispersion (MORD) spectroscopy at 291 K [268]. However, they ascribed the unexpected results to metal ion contamination, although L-α-threonine (CH₃) and L-α-leucine (isobutyl) had particularly large (−)-sign specific Verde constants. The anomaly can be connected to the handed CH₃ rotor action, although the handedness will depend on the solution temperature, pH, ortho-/para-H₂O ratio, and H₂O/D₂O ratio.

In 2006 and 2013, Romalis et al. reported nuclear-spin-induced optical rotation (NSOR) with (+)-sign rotation of 1–13 μrad·cm⁻¹·M⁻¹ (0.06–0.7 mdeg·cm⁻¹·M⁻¹) at 405 nm under B₀ = 5 G of achiral molecular liquids carrying CH₃ and C¹⁹F₃ rotors, i.e., methanol, ethanol, n-propanol, isopropanol, n-hexane, n-hexene, and perfluoro-n-hexane when the liquids were placed in a 0.85 T permanent magnet based on the early work with ¹²⁹Xe [144,145]. Interestingly, even H₂O exhibits a weak NSOR. The results suggest a nuclear-spin-dependent PV-MAM phenomenon because the organic molecules adopt chiral and/or helical geometries owing to the rotors. An enantiomeric pair of liquid molecules carrying CH₃ and C¹⁹F₃ revealed clear differences in absolute magnitude with the same or opposite signs of NSOR signals.

In 2015, Pandey et al. reported non-mirror-symmetric Cu²⁺ metal organic framework (MOF) crystals coordinated to D- and L-leucine derivatives [269]. The D- and L-homochiral Cu²⁺-MOFs revealed structurally distinct crystal packing, leading to notable difference in proton conductivity: the L-MOF showed significantly higher conductivity than the D-MOF. The D- and L-leucine residues induce mirror-symmetry-breaking MOFs differently, presumably leading to observable MPV functionality. The handedness of multiple CH₃ rotors due to the isopropyl groups of the D-L-leucine residues is responsible for the non-mirror MOF crystals.

Recently, to validate Salam’s hypothesis [107,108], Kozlova et al. designed a new MOF crystal incorporating diamagnetic Zn²⁺, diazabizyclooctane (DABCO), and terephthalate. DABCO, a two-fold symmetric hindered rotor, can exist as a mixture of LH and RH geometries [109,110,111,112]. The DABCO-Zn²⁺ MOF revealed three second-order phase transitions at 130 K (terephthalate), 60 K (DABCO), and 14 K (DABCO) from specific heats using adiabatic calorimetry and the solid-state ¹H-NMR T₁ value. The two transitions at 60 K and 14 K are ascribed to the structural transition of DABCO due to the dynamic Jahn–Teller effect of DABCO, whereas the transition at 130 K arises from the structural transition of BDC²⁻. A LH–RH preference at 60 K and 14 K of the MOF is not characterized because neither the calorimeter nor ¹H-NMR are chiroptical approaches [109,110,111,112]. It is interesting to note that the existence of two transitions of the MOF is very similar to that of T_C1 and T_C2 of PDBS in n-alkanes.

3.10. Perspectives

3.10.1. Is Parity Conserved Under the Gravitational Force?

From the non-mirror CD spectra of PDBS in n-alkanes in the CW and CCW flow, it is unclear whether the P-symmetry of GF is rigorously preserved. In materials science, CW and CCW flow under the GF has recently been recognized as a local PC-EMF event enforced by mirror-symmetrical hydrodynamics [3,169,170,171,172,173,174,175]. The hypersensitive hydrodynamic force under the GF is likely to break P-symmetry in response to a tiny local PV advantage factor and nuclear spin frustration beyond the P-symmetric Coriolis force [3,169,170,171,172,173,174,175]. To account for the non-mirror characteristics of the CW and CCW flow experiments, the authors came across a research community of PV-GF.

The general relativity theory of GF formulated by Albert Einstein in the early 20th century relies on a uniformly accelerated reference frame that conserves P-symmetry [270,271,272,273,274,275,276,277,278,279,280,281,282,283,284,285,286,287,288,289,290,291]. Since 1964, many researchers have theoretically discussed PV-GF: do LH- and RH-chiral objects placed in curved spacetime follow equally geodesic trajectories [274,275,276]? The possibility of theoretical and experimental breakthroughs in gravitational wave theory owing to differences between LH and RH polarization modes has prompted several experiments to validate the PV-GF hypothesis.

For PV-GF testing, researchers often use the Eötvös ratio, η = 2·(a_A − a_B)/(a_A + a_B) ≃ (m_g/m_i)_A − (m_g/m_i)_B = Δ(A, B), which is similar to Kuhn’s dissymmetry ratios, g_abs and g_lum, where a_A and a_B are the accelerations of two free-falling bodies A and B, and m_g and m_i are their gravitational and inertial masses, respectively [272]. Similar to the subtle E_PV of MPV theories, a tiny Eötvös ratio, η, in the PV-GF is estimated to be η = 10⁻¹⁵–10⁻²¹ eV and the spin-gravity coupling constant is |A_sg| = 10⁻¹⁸–10⁻²² eV [272]. To validate the PV-GF hypothesis on Earth, a precision rotational torsion pendulum under magnetic shielding at cryogenic temperature has been designed [270,271,272,273]. Carefully designed experiments to search for PV-GF have been proposed utilizing spin-particles, such as polarized e⁻ [277,278,279], a pair of HINS isotopes and a pair of HINS-INS isotopes (i.e., ⁸⁵Rb-⁸⁷Rb, ³⁹K-⁸⁷Rb, ⁸⁷Sr-⁸⁸Sr, ¹²⁹X-¹³¹Xe) [280,281,282,283,284], different masses and densities in Ti-Pt alloys [285,286], l- and d-quartz [287], nuclear-spin-induced chiral molecules [88,288,289], and nuclear-spin-independent chiral CHBrClF and helical H₂X₂ (X = O, S, Se, Te, Po) [290,291]. From his essay, it seems that Shinitzky might have been aware that subtle but detectable differences in density (specific gravity) and optical rotation between L- and D-molecular liquids and between L- and D-NaClO₃ crystals, stem from the handedness of the spatiotemporal galaxy and universe [103].

In 2008, 2015, and 2024, Bargueño et al. discussed the importance of nuclear spin dependence when enantiomers experienced PV-GF [88,288,289]. Molecules possessing CH₃ rotors are candidates to investigate the magnitude of the PV-GF on Earth. The role of the CH₃ rotor in the CW and CCW flow of n-alkanes on a macroscopic scale is to dynamically drive the CH₃ rotor and generate g⁺ > g⁻ (or g⁺ < g⁻) bonds by chirally sheared intra- and intermolecular interactions between n-alkane molecules. PDBS is a chiroptical probe that responds to the dynamic behavior of n-alkanes. The external hydrodynamic CW and CCW flow at 400–800 rpm (7–15 Hz) results in non-mirror-image CD spectra of PDBS in n-alkanes. Organic solvents with CH₃ rotors are likely to feel sensitively the coupling between the EMF and GF. Enantiomeric pairs of terpenes with multiple CH₃ rotors, such as limonene, pinene, carvone, and camphor, are thus candidates as rotating torsional pendulum chemical probes. Actually, noticeable non-mirror-image CD spectra under CW and CCW stirring were found when two optically active aggregates of π-conjugated polymers were stirred in the presence of (S)- and (R)-limonene carrying two CH₃ rotors [292,293].

3.10.2. Olfactory Receptors Discriminate Between CH₃ and CD₃ Groups of Molecules

One might be aware of the fact that the CH₃ group is ubiquitous in homochiral biomolecules such as lipids, L-amino acids (alanine, threonine, cysteine, valine, leucine, isoleucine, methionine), and thymine. In contrast, no CD₃-containing natural compounds have been detected. Actually, it was demonstrated that olfactory receptor proteins (ORPs) of fruit flies (Drosophila melanogaster) at 25 °C and honeybees (Apis mellifera) favor acetophenone-h₈ and n-octanol-h₁₇ but disfavor acetophenone-d₃ (Me), -d₅ (Ph), -d₈ (Me and Ph), and n-octanol-d₁₈ [294,295]. Interestingly, the fruit flies could not discriminate between benzaldehyde-h₈ and -d₈, presumably because of the lack of a CH₃ group. These odorants might therefore exist as racemic mixtures of rotamers due to the lack of rigid chirality.

To explain the discrimination capability of H-D isotopomers, a molecular vibration theory of olfaction has been proposed in addition to the traditional molecular shape theory of fitting odorant molecules in pocket-shaped ORPs, which belongs to classical biology [296]. However, the vibration theory neglects the CH₃ rotor dynamics of achiral odorants. Recently, quantum smell theories for ORPs have been proposed in quantum biology in terms of the asymmetric DWP and MPV of enantiomeric odorants [297,298]. Sensing odorant chirality is influenced by quantum tunneling between enantiomers in double-well potential. This theory led to the conjecture that the handed CH₃ rotor dynamics of odorants are crucial to ORPs. The hidden handedness of the CH₃ and CD₃ rotors and the handed intermolecular chirality transfer affect the discrimination capability of ORPs. The MPV hypothesis thus shines a light on a new horizon in quantum biology [299].

3.10.3. Candidates of the Handed Rotors

Non-radioactive non-rare molecules containing HINS isotopes, e.g., ¹H (I = 1/2), ¹¹B (I = 3/2), ¹³C (I = 1/2), ¹⁵N (I = 1/2), ¹⁷O (I = 5/2), ¹⁹F (I = 1/2), ³¹P (I = 1/2), ²⁹Si (I = 1/2), ³³S (I = 3/2), ^35/37Cl (I = 3/2), ^79/81Br (I = 3/2) and ¹²⁷I (I = 5/2), can be utilized to investigate nuclear-spin-dependent MPV and PV-GF hypotheses in odd-number wells. Several three-fold rotors, that is, CH₃, CF₃, NH₃⁺, and SO₃⁻ as pendants and BF₄⁻, NH₄⁺, and O=PO₃³⁻ as counter ions, can be introduced into various chromophores/luminophores and solvents. VCD/IR [300], ROA/Raman [300], THz (microwave) CD [301,302,303], and cavity-ring-down ORD/CD spectroscopy [304,305] allows the characterization of the simplest molecules with C_3v symmetry, i.e., CH₃X (X = CN, NC, ²H, ¹⁹F, ^35/37Cl, ^79/81Br, ¹²⁷I) and CHX₃ (X = ¹⁹F, ^35/37Cl, ^79/81Br, ¹²⁷I), by probing the C–H stretching and bending bands in CHX₃ and the asymmetric and symmetric bending bands of CH₃ in CH₃X [306,307,308,309,310,311,312,313,314]. When the NH₃⁺ group in zwitterionic species of L-amino acids acts as a handed rotor in ortho- and/or para-H₂O, L- and D-amino acids are no longer enantiomeric, which could provide a possible answer to biomolecular handedness on Earth. To our knowledge, time-dependent MPV theories that treat HINS isotopomers in odd-number wells, including three-, five-, seven-, and nine-wells, are rare or have not yet been reported because most MPV theories deal with even-number wells, typically DWP, in the ground state.

Simpler small molecules containing three-fold symmetric rotors in the absence of rigid chemical chirality, that is, methanol, ethanol, n-propanol, isopropanol, di-n-propyl ether, n-butane (a minimal g-bond), methylcyclohexane, zwitterions (glycine, β-alanine, and choline), acetaldehyde, acetone, diacetyl, t-butyl methyl ether, acetic acid, toluene, p-cymene, o-/m-/p-xylenes, several terpenes (i.e., geraniol, nerol, α-terpinene, γ-terpinene), and, for comparison, their ²H, ¹³C, and ¹⁵N isotopomers are commercially available candidates.

Particularly, it is vital to validate the generality of whether other three-fold symmetric rotors, such as C¹⁹F₃ in n-C₄F₁₀, n-C₆F₁₄, n-C₈F₁₈, 2,3,3,3-tetrafluoropropene, and fluorinated myristic acid generate non-mirror molecules and vibrational spectra [315,316,317,318,319,320]. The VCD/IR spectra of n-C₆F₁₄ and n-C₈F₁₈, which are molecular models of 13₆- and 15₇-helical poly(tetrafluoroethylene) [321,322,323], are shown in Figure S20a,b (Supplementary Materials), respectively. Hence, (−)-sign VCD signals due to the CF₃ symmetric stretching modes at 1335 cm⁻¹ (n-C₆F₁₄) and 1347 cm⁻¹ (n-C₈F₁₈) can be observed. Additionally, several (−)-sign VCD signals due to the CF₂ asymmetric and symmetric stretching modes are observed in the range of 1265–1150 cm⁻¹.

The nuclear-spin-dependent VCD/IR spectra of the methyl groups in C_3v symmetric CH₃CN and CD₃CN are compared in Figure S20c,d (Supplementary Materials), respectively. The VCD/IR spectra of CH₃CN clearly show several broader VCD bands due to the CH₃ group around ~1400 cm⁻¹ including sharper asymmetric bending (ν₆) at 1442 cm⁻¹ and symmetric bending (ν₃) at 1378 cm⁻¹ [307,324]. The broad VCD/IR bands are ascribed to anti-parallel dimers, tetramers, hexamers, and octamers [307,324]. However, CD₃CN showed less obvious VCD signals in the CD₃ group. Further, the VCD/IR spectra of monomeric CH₃CN/CD₃CN species in the gas phase and/or in a dilute solution in C^35/37Cl₄ will provide direct evidence of intermolecular hindered rotation transfer with handedness.

Another example is the comparison between toluene-h₈ and toluene-d₈, which are the simplest methyl-substituted aromatics. The nuclear-spin-dependent VCD/IR spectra are shown in Figure S20e,f (Supplementary Materials), respectively. Previously, polycrystalline samples of partially and fully deuterated toluene (C₆D₅CH₃ and C₆D₅CD₃) at cryogenic temperatures clearly showed INS spectra and well-resolved Raman scattering (<140 cm⁻¹) due to the hindered rotation of methyl groups in the TWP [220,325,326]. The (−)-sign VCD band of the C=C stretching mode at 1494 cm⁻¹ of toluene-h₈ is obvious, although the VCD bands of CH₃ bending modes (1456 and 1378 cm⁻¹) are weak, as is evident in Figure S20e (Supplementary Materials). Two intense (−)-sign VCD signals (1941 and 1858 cm⁻¹) at four weaker IR bands (1941, 1858, 1800, and 1731 cm⁻¹), which are unique overtone characteristics of monosubstituted benzene derivatives, are clearly observed [249,325,326]. However, the corresponding VCD signals of toluene-d₈ in the range of 1300–1400 cm⁻¹ range are very weak (Figure S20f, Supplementary Materials). The VCD-active IR modes are likely to be enhanced as overtones due to effective coupling of the two fundamental vibrational modes of toluene-h₈ [326].

Notably, p-cymene (4-isopropyltoluene), which is an aromatic monoterpene, exhibited clear VCD signals in the corresponding IR spectra (Figure S20g–i, Supplementary Materials). The two intense (−)-sign VCD signals (1941 and 1800 cm⁻¹) at two weaker IR bands (1941 and 1800 cm⁻¹), are overtones characteristic of 1,4-substituted benzene derivatives [249]. Conversely, a number of intense (+)-sign VCD signals at the corresponding IR bands in the range of 1000–1550 cm⁻¹ are clearly detected, i.e., C–H(CH₃) wagging mode at 1514 cm⁻¹, isopropyl symmetric and asymmetric bending modes (1382 and 1363 cm⁻¹) and p-substituted skeletal mode (1057 cm⁻¹) [327]. Because p-cymene is often used as a ligand in various ruthenium complexes, its optically active vibrational modes may be crucial for catalytic activity.

The calculated results can be readily validated using commercial and homemade VCD, ROA, and near-IR CD spectrometers. A theoretical and experimental understanding of the generality of the handedness of perpetual molecular rotors will allow the synthesis of both RH- and LH-chiral molecules and/or helical polymers without chiral catalysts with minimal energy, time, and cost in the near future.

4. Conclusions

Since the 1960s, many theorists have argued that the electroweak force (EWF) induces a parity-violating energy difference (E_PV) on the order of 10⁻¹⁰–10⁻²¹ eV between mirror-image molecules induced by the handedness of the weak neutral electron current (WNC). The six major theoretical scenarios are as follows: (i) linear amplification model (E_PV ∝ N), (ii) E_PV ∝ Z⁵ (Z: atomic number), (iii) nonlinear amplification model, (iv) tuning ΔE_± to adjust E_PV, (v) E_PV ∝ ΔE_ST, and (vi) nuclear-spin-induced anapole moment. In 1991/1992, Salam predicted that non-natural D-amino acids should undergo an EWF-origin P-symmetry violating second-order phase transition at 250 K (–23 °C) but that natural L-amino acids would not.

Inspired by Salam’s hypothesis and other theoretical MPV approaches, the present work investigates the detailed CD and CPL spectroscopic characteristics of rod-like poly(di-n-butylsilane) (PDBS) in homogeneous solutions of n-alkanes and isooctane ranging from −10 °C to 110 °C under static conditions, and, for comparison, under clockwise (CW) and counterclockwise (CCW) stirring conditions. PDBS adopts mirror-symmetric 7₃-helices in n-hexane and in the solid state.

This paper reports the serendipitous finding of dual mirror-symmetry breaking in PDBS: second-order phase transitions as a result of chiroptical generation at T_C1 ~ 105 °C, and chiroptical inversion due to a helix–helix transition at T_C2 ~ 28 °C. In particular, the solvent n-dodecane-h₂₆ induced a gigantic enhancement in the CD and CPL spectra of PDBS. A (−)-sign CD band newly emerged at a shorter λ_max (300 nm) below T_C1, followed by a helix–helix transition at T_C2, and eventually exhibited g_abs = +1.3 × 10⁻² at −10 °C. In a similar manner, (−)-sign CPL at 60 °C exhibited g_lum = –0.7 × 10⁻² at λ_em 340 nm but was inverted to (+)-CPL with g_lum = +2.0 × 10⁻² at 0 °C. Notably, in n-dodecane-d₂₆, these g_abs values are weaker by an order of magnitude at the lower T_C1 and associated with opposite CD signs.

The notable H/D effects between n-dodecane-h₂₆ and -d₂₆ led to the proposal that the three-fold symmetrical CH₃ groups of n-dodecane and the n-butyl pendants of PDBS work cooperatively as a unidirectional perpetual hindered rotor with handedness owing to three non-vanishing nuclear spin-1/2 systems in a triple-well potential. The CD₃ group is an inefficient hindered rotor compared to the CH₃ group because of the inherent difference in the moments of inertia and rotational constants between the CD₃ and CH₃ rotors. Several (−)-sign VCD signals characteristic of the asymmetric and symmetric bending modes of CH₃/CD₃, scissoring and twisting modes of CH₂/CD₂, and gauche-bonds of n-dodecane-h₂₆ were detected.

More interestingly, CW and CCW stirring in several n-alkanes at 0–40 °C induced completely non-mirror-image CD spectra of PDBS, which is consistent with the coupling of EWF with gravitational force, and which causes apparent P-symmetry breaking on Earth. The handedness of the CH₃ rotor may provide a possible answer to the issue of the missing link of handedness at all hierarchical levels, ranging from LH–ν_e, LH quarks, optically active atoms, biological proteins, DNA/RNA, homochiral living biospheres, and cosmological dextrorotatory rotation. Several handed molecular rotors of three identical nuclear spin-1/2 atoms in a triple-well potential could allow the future synthesis of RH- and LH-chiral molecules and/or helical polymers by optimizing achiral solvents at specific temperatures in the absence of conventional chemical chirality.

Several experimental studies that may provide evidence for the MPV hypothesis have been introduced and briefly discussed. A dozen of the simplest molecules carrying handed rotors without point chirality were proposed to verify the MPV hypothesis.

The term “chirogenesis” in the title was coined by Victor Borovkov and Yoshihisa Inoue in supramolecular chemistry and materials chemistry, based on the nuclear-spin-independent PC-EMF [328,329,330,331]. Nuclear-spin-dependent chirogenesis may be widely applicable to PV-EWF in general chemistry, a range of spectroscopy, molecular dynamics and molecular mechanics, materials science, quantum dynamics and quantum mechanics, particle physics, atomic physics, molecular physics, biology, astronomy, gravity theory, and cosmology.

5. Experimental Section

The synthesis and characterization of the monomers and polymers are summarized in the Supplementary Materials. All solvents were used as received. Instrumentation and analysis of NMR (¹H, ¹³C, ²⁹Si), VT-CD/UV, VT-CPL/PL, VT-VCD/IR spectroscopic data, and Gaussian 09 calculations obtained with PM3MM, DFT (B3LYP with 6-31G(d) basis set), and TD-DFT(B3LYP with 6-31G(d) basis set) are described in the Supplementary Materials. PBtBPS was synthesized by Wurtz-coupling of the corresponding diaryldichlorosilane [332]. PH2MPS and PH2EBS were synthesized by Wurtz-coupling of n-hexyl-2-methylpropyldichlorosilane and n-hexyl-2-ethylbutyldichlorosilane, respectively [106,333].