Edge-Irregular Reflexive Strength of Non-Planar Graphs
Abstract
1. Introduction
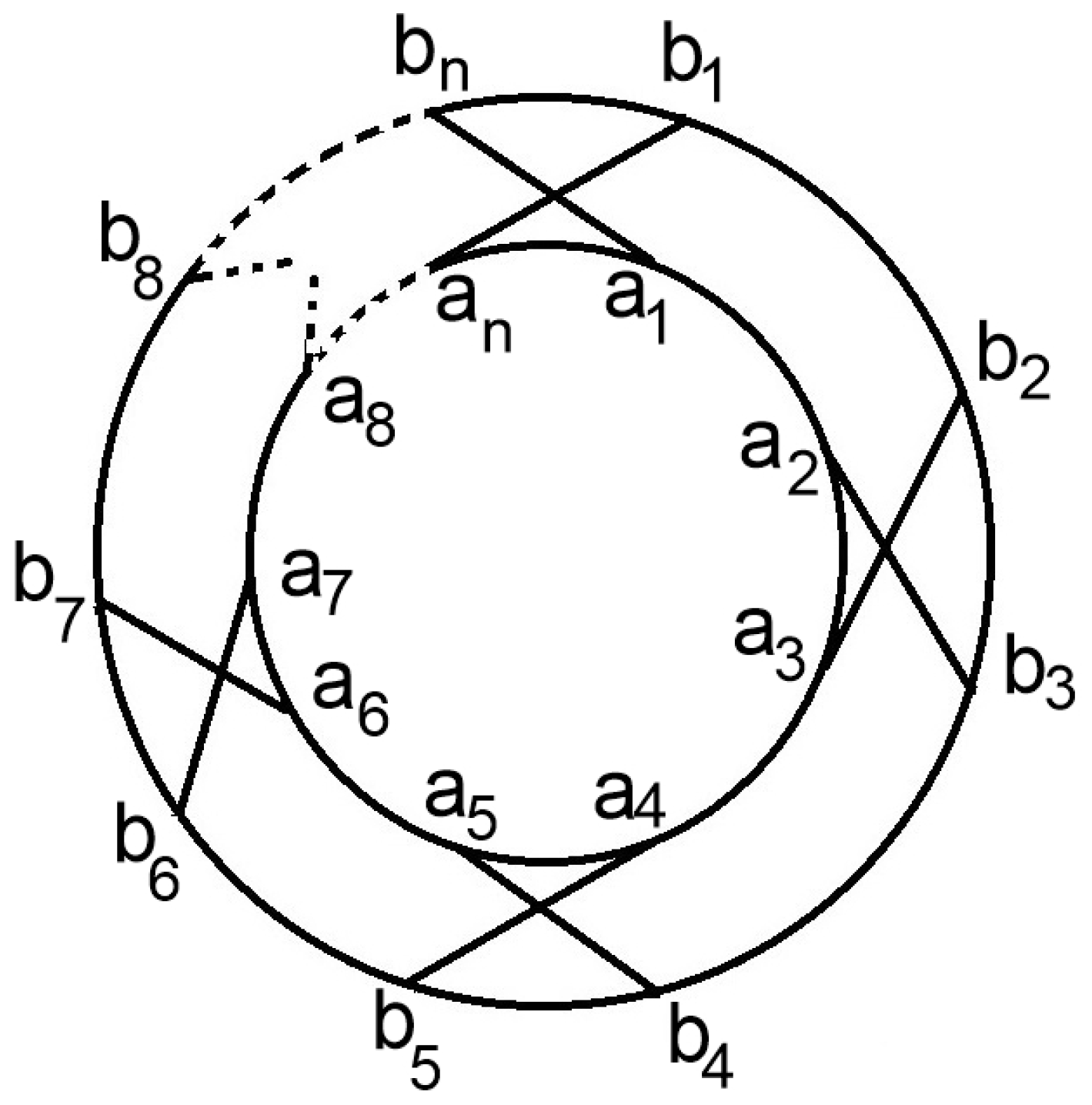
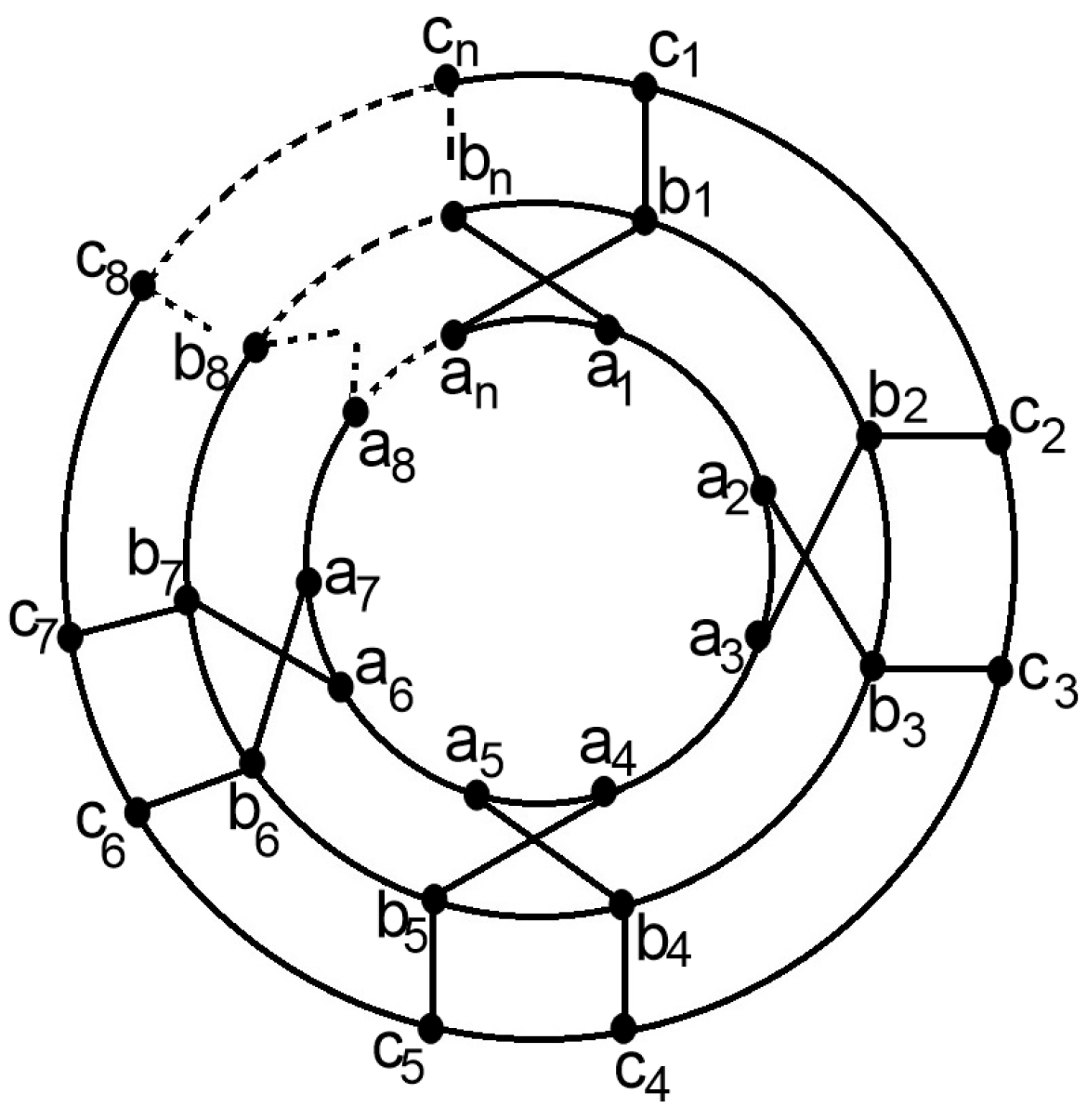
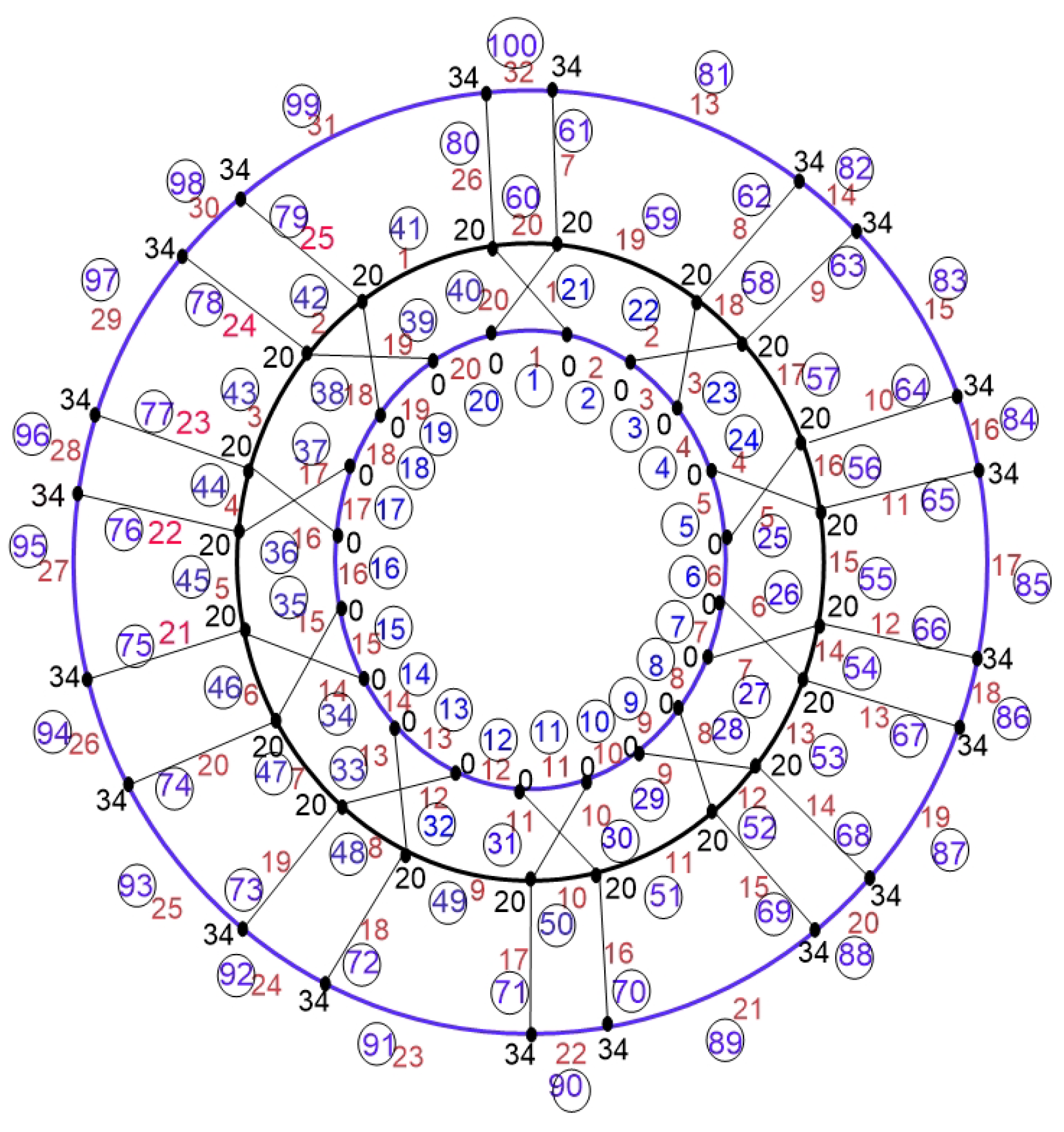
2. Edge-Irregular Reflexive Strength of Cross Prism
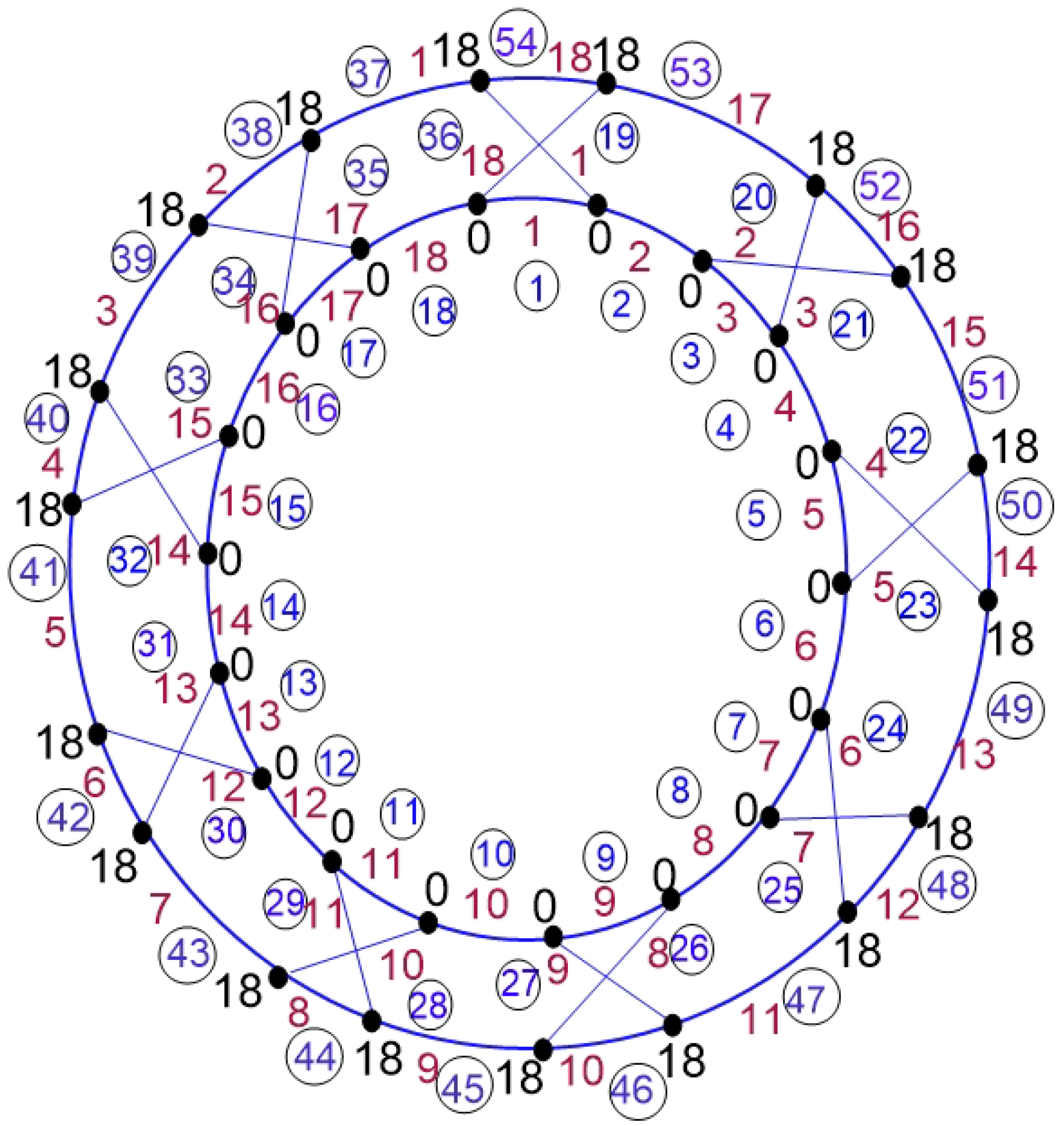
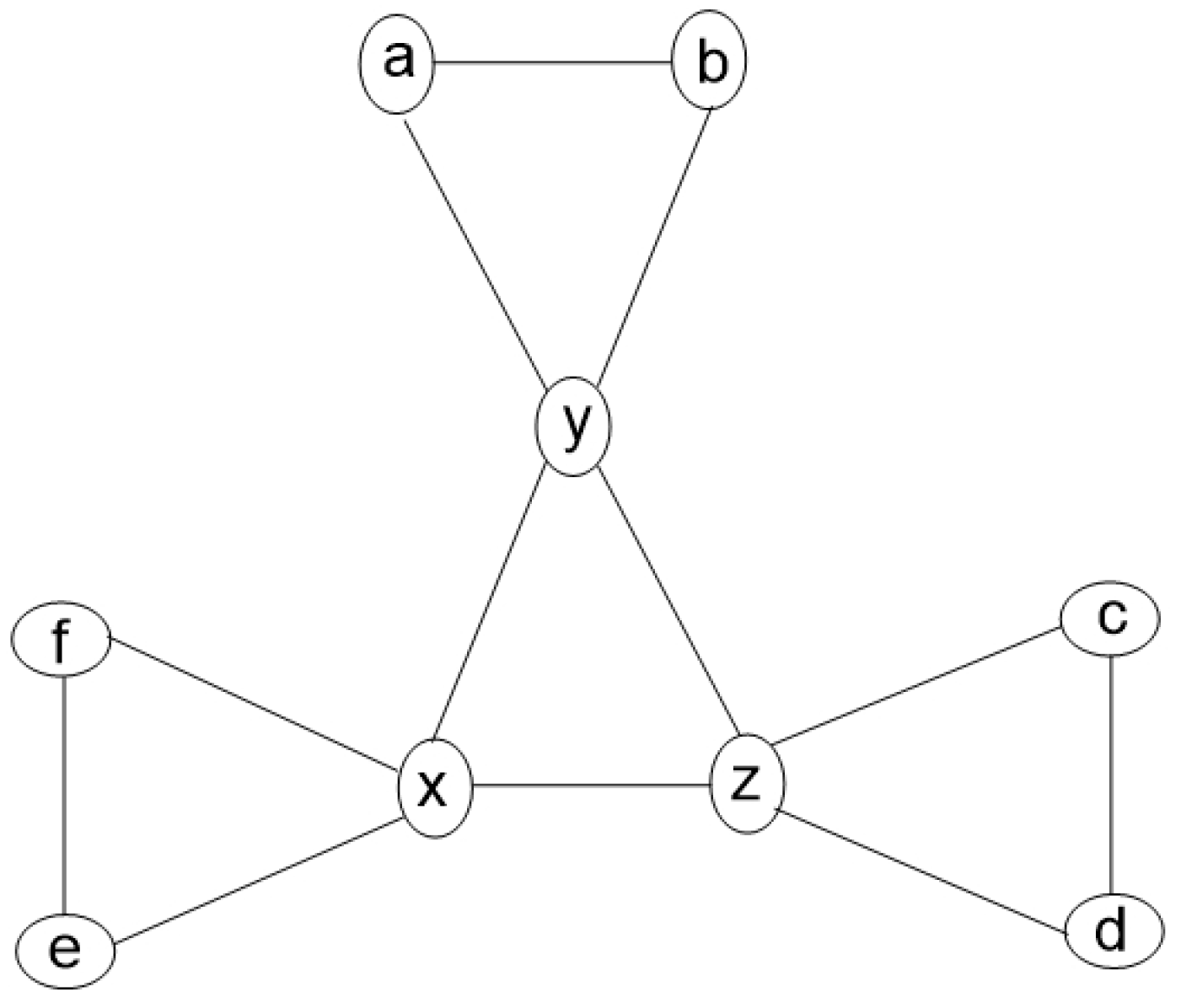
3. Duplication of Graph
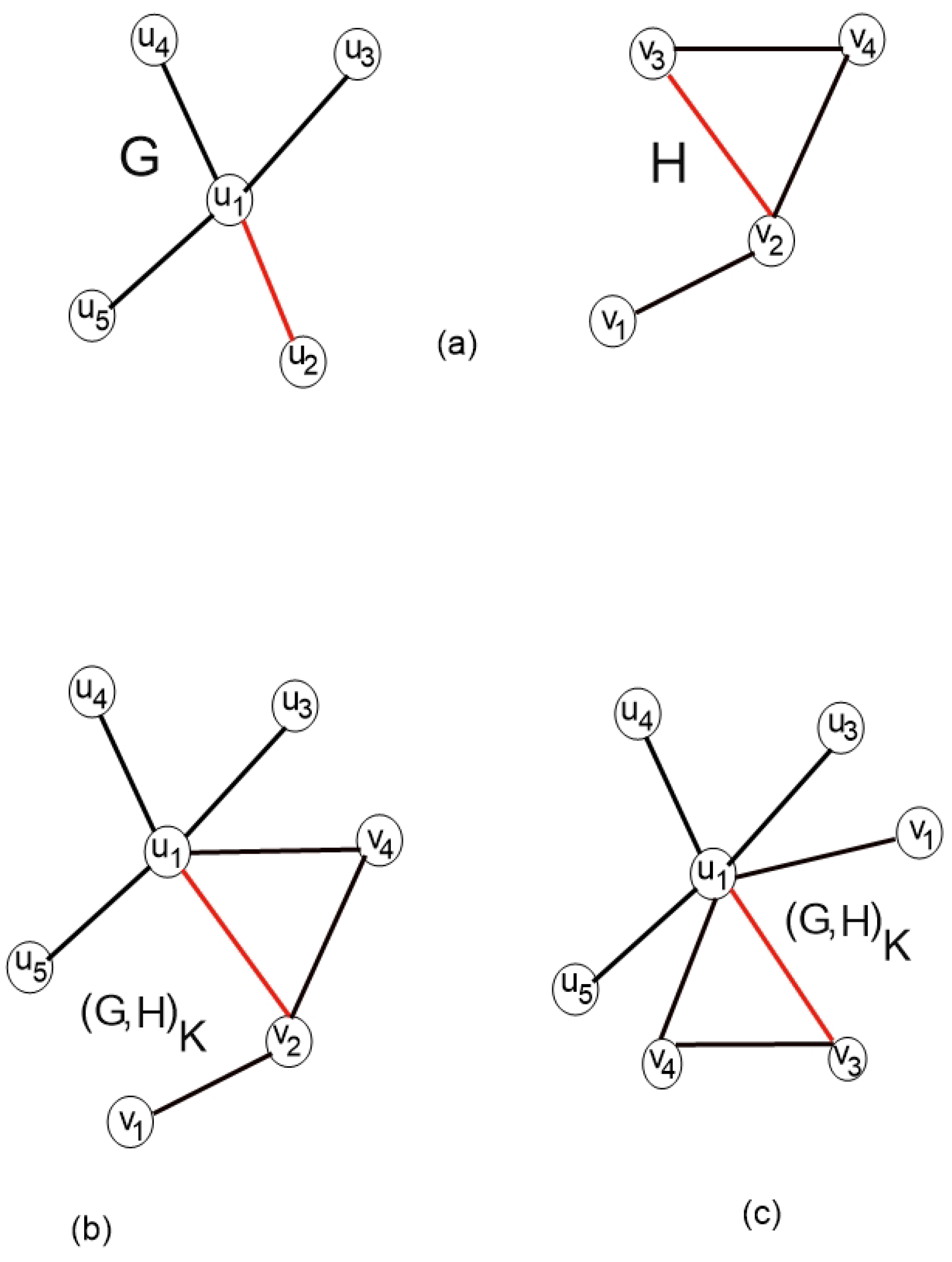
- In a graph S, establishing a new vertex u using produces a new graph . This is known as the duplication of the vertex ς.Vertex duplication by an edge in a graph S provides a new graph with and . Figure 3 depicts an example of edge-induced vertex duplication in .
- When a vertex ς replicates an edge in a graph S, a corresponding graph is produced with .Let be the graph acquired from by duplication of each vertex by an edge. This graph has vertices and edges. Then, the next Theorem 2 describes the edge-irregular reflexive strength of .
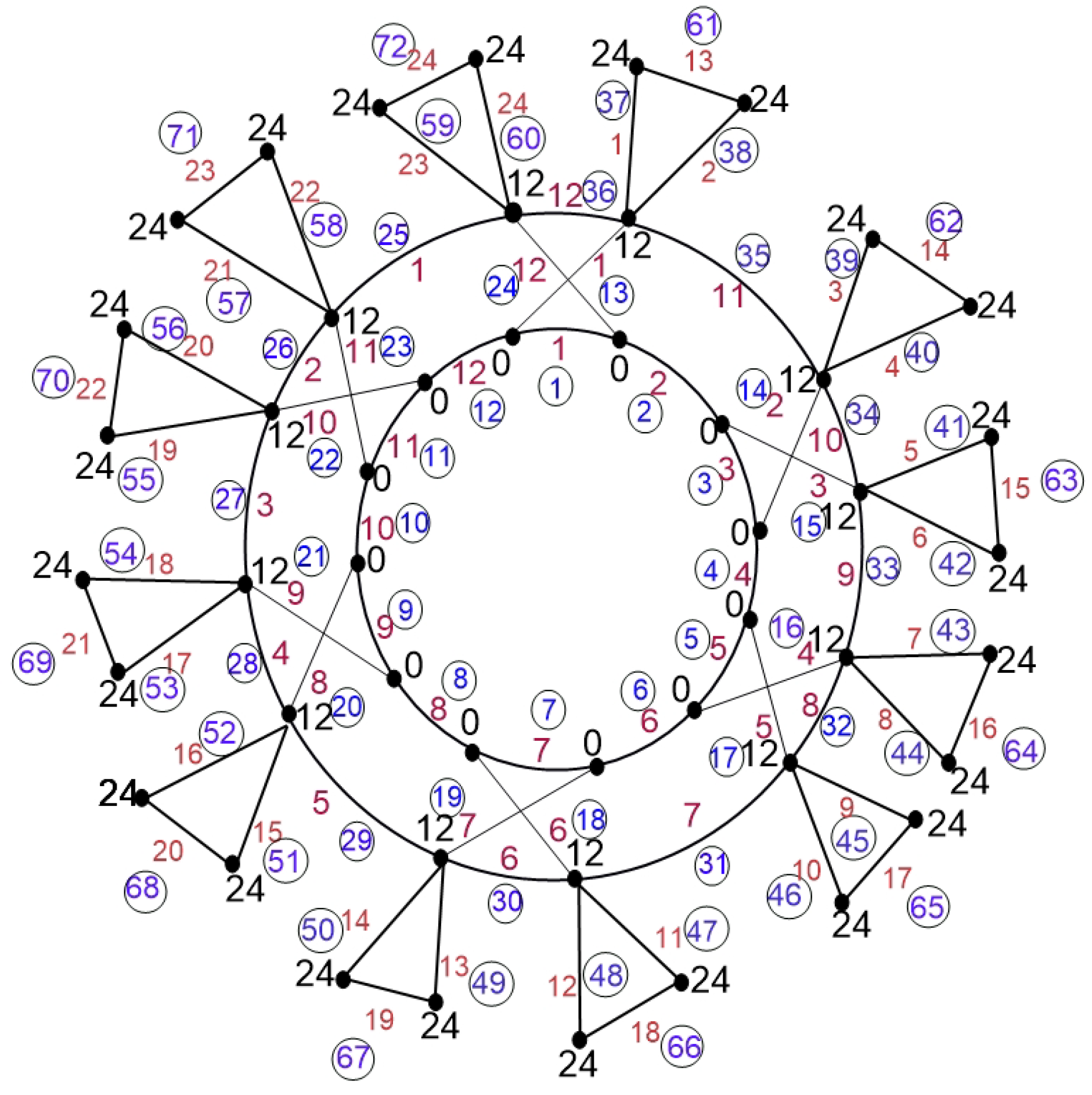
4. Graphs
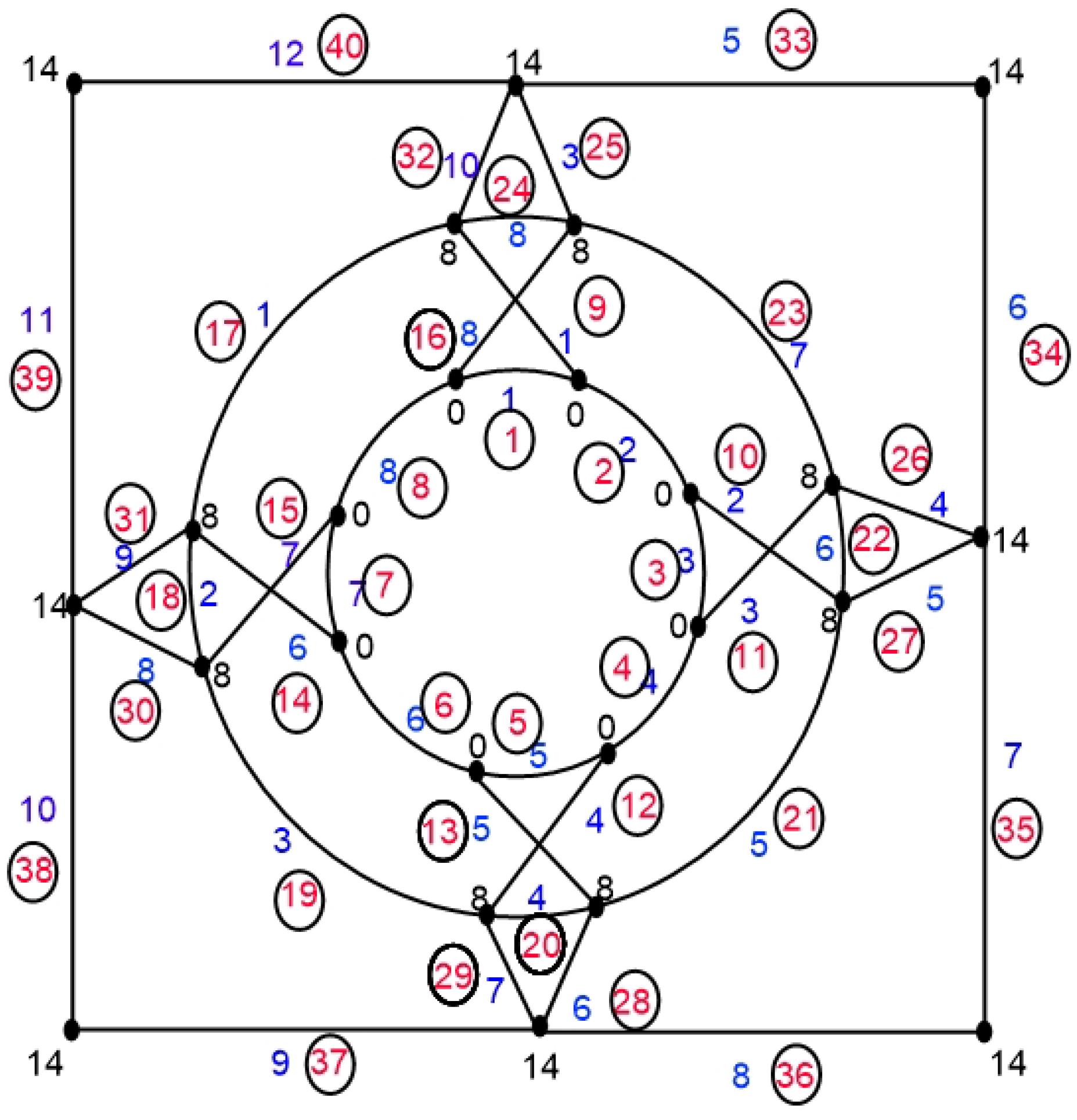
5. Glowing Graph
6. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Bondy, J.A.; Murty, U.S.R. Graph Theory; Springer: Cham, Switzerland, 2008. [Google Scholar]
- Dolev, D.; Dwork, C.; Stockmeyer, L. On the minimal synchronism needed for distributed consensus. J. ACM 1987, 34, 77–97. [Google Scholar] [CrossRef]
- Rucker, C.; Rucker, G. Molecular networks: From graphs to chemical properties. J. Chem. Inf. Model. 2016, 56, 791–804. [Google Scholar]
- Diestel, R. Graph Theory, 5th ed.; Springer: Cham, Switzerland, 2017. [Google Scholar]
- Baker, J. Symmetry in chemical structures. Chem. Rev. 2010, 110, 1565–1590. [Google Scholar]
- Sutherland, B. Symmetry and Structure in Condensed Matter Physics; Cambridge University Press: Cambridge, UK, 2012. [Google Scholar]
- Li, Y.; Liu, J.; Zhang, P. Graph-based optimization in wireless networks. IEEE Trans. Netw. 2020, 28, 1254–1267. [Google Scholar]
- Gallian, J.A. A dynamic survey of graph labeling. Electron. J. Comb. 2022, 25, 4–623. [Google Scholar] [CrossRef] [PubMed]
- Baca, J.; Jendrol, E.; Miller, M.; Ryan, J. Total vertex irregularity strength of graphs. Discret. Appl. Math. 2006, 154, 234–245. [Google Scholar]
- Ahmad, A.; Bača, M. Edge irregular total labeling of certain family of graphs. AKCE Int. J. Graphs Comb. 2009, 1, 9–21. [Google Scholar]
- Wang, M.; Lin, Y.; Wang, S. The nature diagnosability of bubble-sort star graphs under the PMC model and MM* model. Int. J. Eng. Appl. Sci. 2017, 4, 2394–3661. [Google Scholar]
- Xiang, D.; Hsieh, S.Y. G-good-neighbor diagnosability under the modified comparison model for multiprocessor systems. Theor. Comput. Sci. 2025, 1028, 115027. [Google Scholar]
- Vaidya, S.K.; Barasara, C.M. Product Cordial Graphs in the Context of Some Graph Operations. Int. J. Math. Comput. Sci. 2011, 1, 1–6. [Google Scholar]
- Vaidya, S.K.; Dani, N.A. Some New Product Cordial Graphs. J. App. Comp. Sci. Math. 2010, 8, 62–65. [Google Scholar]
- Jeyanthi, M.; Sudha, K. Total edge irregularity strength of wheel graphs. J. Discret. Math. Sci. 2015, 9, 567–578. [Google Scholar]
- Jeyanthi, M.; Sudha, K. Extended results on graph irregular labeling. J. Graph Theory 2017, 11, 120–133. [Google Scholar]
- Mughal, A.; Jamil, N. Total Face Irregularity Strength of Grid and Wheel Graph under K-Labeling of Type (1, 1, 0). J. Math. 2021, 1, 1311269. [Google Scholar] [CrossRef]
- Tanna, D.; Ryan, J.; Semaničová-Feňovčíková, A. Edge irregular reflexive labeling of prisms and wheels. Australas. J. Comb. 2017, 3, 394–401. [Google Scholar]

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Khan, S.; Akram, M.W.; Ishtiaq, U.; Garayev, M.; Popa, I.-L. Edge-Irregular Reflexive Strength of Non-Planar Graphs. Symmetry 2025, 17, 386. https://doi.org/10.3390/sym17030386
Khan S, Akram MW, Ishtiaq U, Garayev M, Popa I-L. Edge-Irregular Reflexive Strength of Non-Planar Graphs. Symmetry. 2025; 17(3):386. https://doi.org/10.3390/sym17030386
Chicago/Turabian StyleKhan, Suleman, Muhammad Waseem Akram, Umar Ishtiaq, Mubariz Garayev, and Ioan-Lucian Popa. 2025. "Edge-Irregular Reflexive Strength of Non-Planar Graphs" Symmetry 17, no. 3: 386. https://doi.org/10.3390/sym17030386
APA StyleKhan, S., Akram, M. W., Ishtiaq, U., Garayev, M., & Popa, I.-L. (2025). Edge-Irregular Reflexive Strength of Non-Planar Graphs. Symmetry, 17(3), 386. https://doi.org/10.3390/sym17030386









