Nuclear Matter Properties and Neutron Star Phenomenology Using the Finite Range Simple Effective Interaction
Abstract
1. Introduction
2. Formalism
The Fitting Procedure of SEI
3. Symmetric and Asymmetric Nuclear Matter Properties
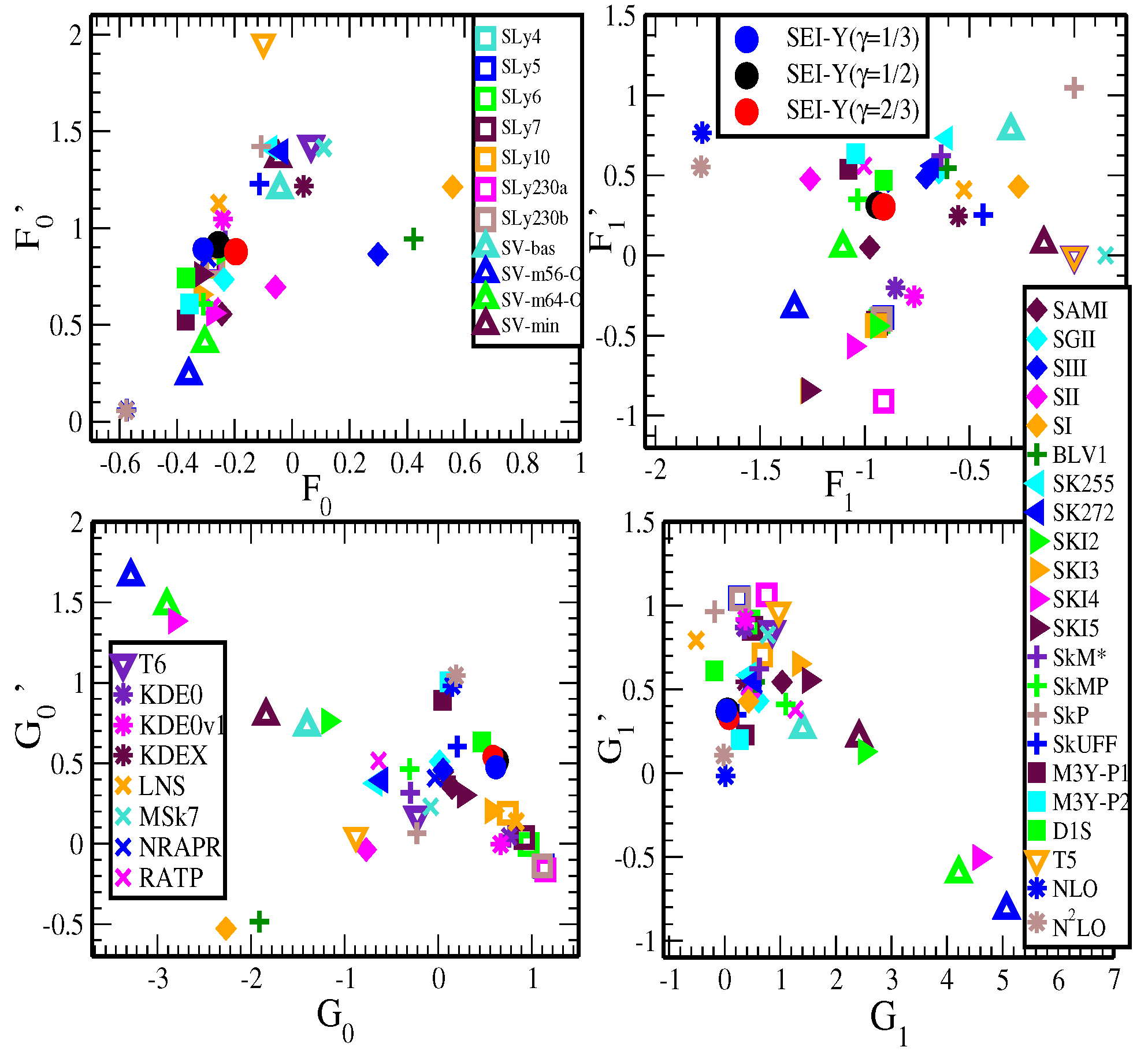
3.1. Landau Parameters
3.2. High-Order Derivatives of the Energy per Particle in Asymmetric Nuclear Matter
| Expt./Observation/Theory | [MeV] |
|---|---|
| Mean-field calculations and Astrophysical Observations | |
| Dutra et al., 2012 [29] | 27–36 |
| B A Li and Han, 2013 [68] | 31.6 ± 0.92 |
| Oertel et al., 2017 [69] | 31.7 ± 3.2 |
| PREX II Experiment Reed et al., 2021 [70] | 38.1 ± 4.7 |
| Charged Pion Spectra at high momenta Estee et al., 2021 [71] | 32.5–38.1 |
| Charge exchange and elastic scattering data Danielewicz et al., 2017 [72] | 33.5–36.4 |
| Expt./Observation/Theory | [MeV] |
| SEI-Y () | 55.74 |
| SEI-Y () | 55.38 |
| SEI-Y () | 54.93 |
| HIC and Transport Calculations | |
| ASY-EoS experiment at GSI [74] | 46–54 |
| UrQMD transport calculation [75] | 55 ± 5 |
| Zhang et al., 2020 [76] | 35–55 |
| Gravitational Waves Zhang and Li, 2019 [77] | |
| Xie and Li, 2019 [73] | |
| Tong et al., 2020 [78] | 60.7 ± 10.9 |
| Chiral Effective Field Theory | |
| Drischler et al., 2020 [84] | 45 ± 3 |
| Lonardoni et al., 2020 [85] | 45 ± 5 |
| Neutron Star Observables B A Li et al., 2021 [79] | 51 ± 13 |
| Nakazato and Suzuki, 2019 [80] | 40–60 |
| Yue et al., 2022 [81] | 62.8 ± 15.9 |
| Xie and Li, 2020 [82] | |
| Zhou et al., 2019 [83] | [] |
| Mean-Field Calculations Chen et al., 2015 [86] | 40.2 ± 12.8 |
4. Neutron Star Phenomenology
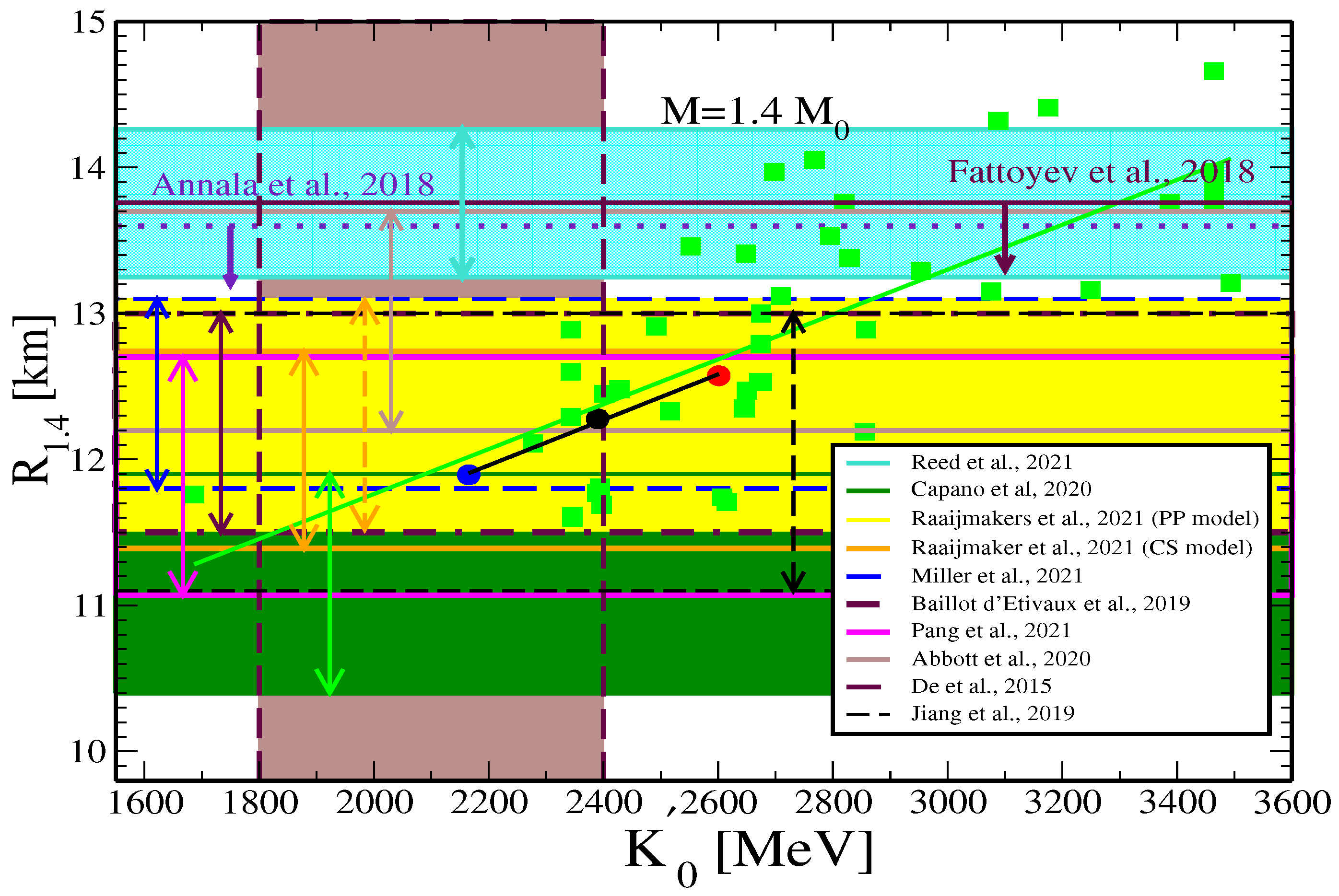
4.1. The Radius of Neutron Stars and the Slope of the Isoscalar Incompressibility
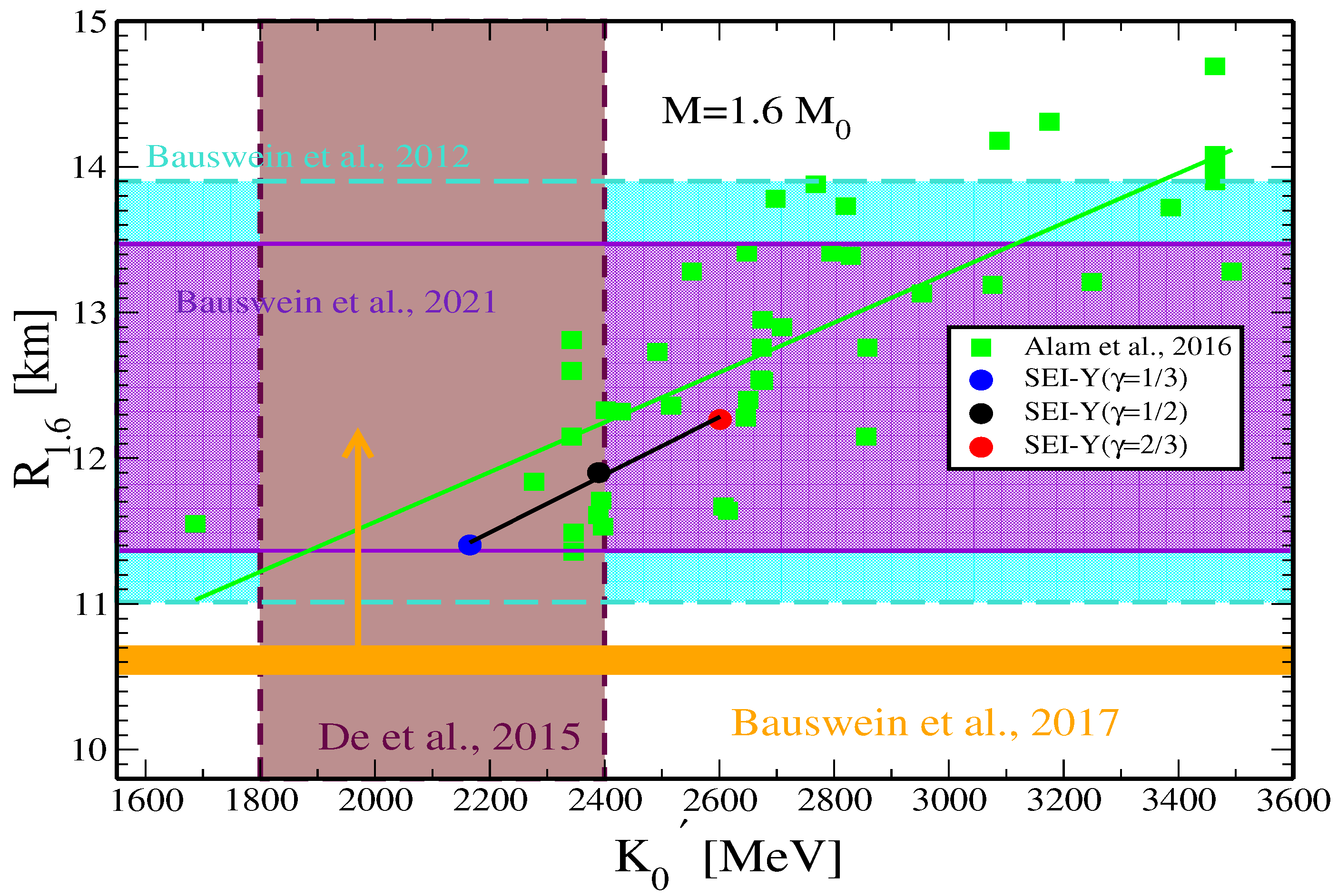
4.2. Neutron Star Merger and Incompressibility of Asymmetric Nuclear Matter
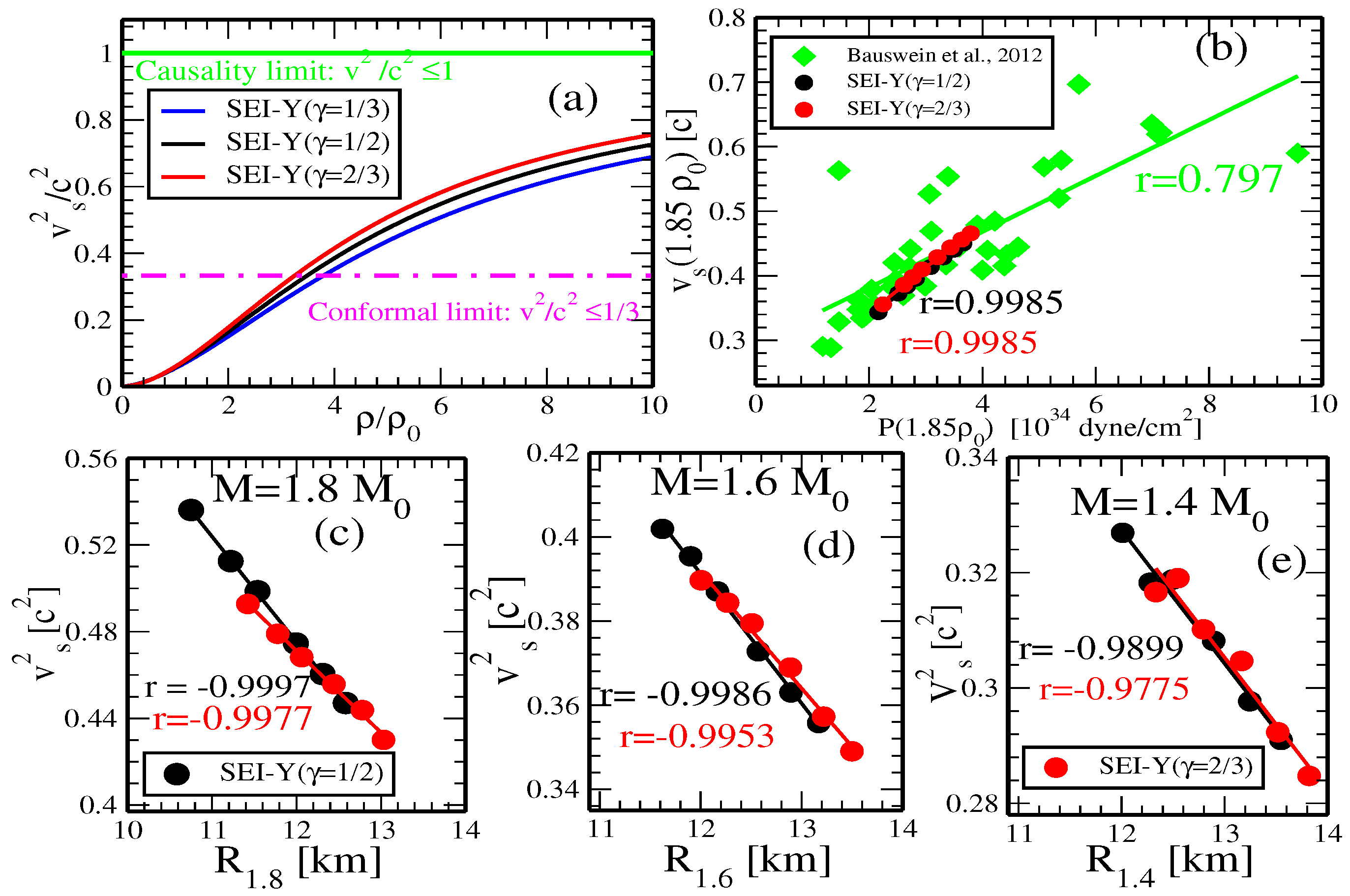
4.3. Sound Speed in Neutron Star Matter
4.4. Gravitational Redshift
4.5. Neutron Star Mass, Radius and Gravitational Redshift at Different Central Densities
5. Summary and Outlook
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A. Relations between the Six Strength Parameters and the Interaction Parameters
| b [] | [fm] | [] | W [MeV] | ||
|---|---|---|---|---|---|
| 1/3 | 0.4161 | 0.4232 | −0.0630 | 9536.129 | −1380.539 |
| 1/2 | 0.5880 | 0.4242 | −0.112 | 9277.281 | −1321.847 |
| 2/3 | 0.7796 | 0.4250 | −0.153 | 10228.257 | −1214.475 |
| B [MeV] | H [MeV] | M [MeV] | [MeV] | ||
| 128.0918 | −630.968 | −808.871 | 333.5 | 1.151 | 119.3 |
| 100.950 | −575.215 | −832.339 | 566.7 | 0.664 | 118.4 |
| 49.094 | −470.284 | −881.144 | 647.4 | 0.520 | 118.2 |
Appendix B. Landau Parameters
References
- Haar, B.T.; Malfliet, R. Nucleons, mesons and deltas in nuclear matter a relativistic Dirac-Brueckner approach. Phys. Rep. 1987, 149, 207. [Google Scholar] [CrossRef]
- Van Dalen, E.N.E.; Fuchs, C.; Faessler, A. The relativistic Dirac–Brueckner approach to asymmetric nuclear matter. Nucl. Phys. A 2004, 744, 227–248. [Google Scholar] [CrossRef]
- de Jong, F.; Lenske, H. Asymmetric nuclear matter in the relativistic Brueckner-Hartree-Fock approach. Phys. Rev. C 1998, 57, 3099. [Google Scholar] [CrossRef]
- Cugnon, J.; Deneye, P.; Lejeune, A.Z. Neutron matter properties in an extended Brueckner approach. Zeitschrift für Physik A-Atomic Nuclei 1987, 328, 409–415. [Google Scholar] [CrossRef]
- Zuo, W.; Lejeune, A.; Lombardo, U.; Mathiot, J.F. Microscopic three-body force for asymmetric nuclear matter. Eur. Phys. J. A 2002, 14, 469–475. [Google Scholar] [CrossRef]
- Bombaci, I.; Lombardo, U. Asymmetric nuclear matter equation of state. Phys. Rev. C 1991, 44, 1892. [Google Scholar] [CrossRef] [PubMed]
- Day, B.D.; Wiringa, R.B. Brueckner-Bethe and variational calculations of nuclear matter. Phys. Rev. C 1985, 32, 1057. [Google Scholar] [CrossRef] [PubMed]
- Baldo, M.; Burgio, G.F.; Schulze, H.-J. Hyperon stars in the Brueckner-Bethe-Goldstone theory. Phys. Rev. C 2000, 61, 055801. [Google Scholar] [CrossRef]
- Vidaña, I.; Polls, A.; Ramos, A.; Engvik, L.; Hjorth-Jensen, M. Hyperon-hyperon interactions and properties of neutron star matter. Phys. Rev. C 2000, 62, 035801. [Google Scholar] [CrossRef]
- Akmal, A.; Pandharipande, V.R.; Ravenhall, D.G. Equation of state of nucleon matter and neutron star structure. Phys. Rev. C 1998, 58, 1804. [Google Scholar] [CrossRef]
- Mukherjee, A.; Pandharipande, V.R. Variational theory of hot nucleon matter. Phys. Rev. C 2007, 75, 035802. [Google Scholar] [CrossRef]
- Hebeler, K.; Schwenk, A. Chiral three-nucleon forces and neutron matter. Phys. Rev. C 2010, 82, 014314. [Google Scholar] [CrossRef]
- Hebeler, K.; Lattimer, J.M.; Pethick, C.J.; Schwenk, A. Constraints on Neutron Star Radii Based on Chiral Effective Field Theory Interactions. Phys. Rev. Lett. 2010, 105, 161102. [Google Scholar] [CrossRef] [PubMed]
- Haensel, P.; Kutschera, M.; Prószyński, M. Uncertainty in the saturation density of nuclear matter and neutron star models. Astron. Astrophys. 1981, 102, 299–302. Available online: http://adsabs.harvard.edu/abs/1981A%26A...102..299H (accessed on 27 January 2024).
- Skyrme, T.H.R. CVII. The Nuclear Surface. Philos. Mag. 1956, 1, 1043. [Google Scholar] [CrossRef]
- Decharge, J.; Gogny, D. Hartree-Fock-Bogolyubov calculations with the D1 effective interaction on spherical nuclei. Phys. Rev. C 1980, 21, 1568. [Google Scholar] [CrossRef]
- Bertsch, G.; Borysowicz, J.; McManus, H.; Love, W.G. Interactions for inelastic scattering derived from realistic potentials. Nucl. Phys. A 1977, 284, 399–419. [Google Scholar] [CrossRef]
- Behera, B.; Routray, T.R.; Satpathy, R.K. Momentum and density dependence of the mean field in nuclear matter. J. Phys. G Nucl. Part. Phys. 1998, 24, 2073. [Google Scholar] [CrossRef]
- Behera, B.; Routray, T.R.; Pradhan, A.; Patra, S.K.; Sahu, P.K. Momentum and density dependence of the isospin part of nuclear mean field and equation of state of asymmetric nuclear matter. Nucl. Phys. A 2005, 753, 367–386. [Google Scholar] [CrossRef]
- Behera, B.; Viñas, X.; Routray, T.R.; Centelles, M. Study of spin polarized nuclear matter and finite nuclei with finite range simple effective interaction. J. Phys. G Nucl. Part. Phys. 2015, 42, 045103. Available online: http://iopscience.iop.org/0954-3899/42/4/045103 (accessed on 27 January 2024). [CrossRef]
- Behera, B.; Viñas, X.; Bhuyan, M.; Routray, T.R.; Sharma, B.K.; Patra, S.K. Simple effective interaction: Infinite nuclear matter and finite nuclei. J. Phys. G Nucl. Part. Phys. 2013, 40, 095105. Available online: http://iopscience.iop.org/0954-3899/40/9/095105 (accessed on 27 January 2024). [CrossRef]
- Bertsch, G.F.; Das Gupta, S. A guide to microscopic models for intermediate energy heavy ion collisions. Phys. Rep. 1988, 160, 189. [Google Scholar] [CrossRef]
- Gale, C.; Bertsch, G.F.; Das Gupta, S. Heavy-ion collision theory with momentum-dependent interactions. Phys. Rev. C 1987, 35, 1666. [Google Scholar] [CrossRef] [PubMed]
- Gale, C.; Welke, G.M.; Prakash, M.; Lee, S.J.; Das Gupta, S. Transverse momenta, nuclear equation of state, and momentum-dependent interactions in heavy-ion collisions. Phys. Rev. C 1990, 41, 1545. [Google Scholar] [CrossRef]
- Csernai, L.P.; Fai, G.; Gale, C.; Osnes, E. Nuclear equation of state with momentum-dependent interactions. Phys. Rev. C 1992, 46, 736. [Google Scholar] [CrossRef]
- Behera, B.; Routray, T.R.; Satpathy, R.K. Causal violation of the speed of sound and the equation of state of nuclear matter. J. Phys. G 1997, 23, 445. Available online: http://iopscience.iop.org/0954-3899/23/4/005 (accessed on 27 January 2024). [CrossRef]
- Danielewicz, P.; Lacey, R.; Lynch, W.G. Determination of the Equation of State of Dense Matter. Science 2002, 298, 1592. [Google Scholar] [CrossRef]
- Behera, B.; Routray, T.R.; Tripathy, S.K. Neutron–proton effective mass splitting and thermal evolution in neutron-rich matter. J. Phys. G Nucl. Part. Phys. 2011, 38, 115104. Available online: http://iopscience.iop.org/0954-3899/38/11/115104 (accessed on 27 January 2024). [CrossRef]
- Dutra, M.; Lourenço, O.; Martins, J.S.S.; Delfino, A.; Stone, J.R.; Stevenson, P.D. Skyrme interaction and nuclear matter constraints. Phys. Rev. C 2012, 85, 035201. [Google Scholar] [CrossRef]
- Behera, B.; Routray, T.R.; Pradhan, A.; Patra, S.K.; Sahu, P.K. Nuclear mean field and equation of state of asymmetric nuclear matter. Nucl. Phys. A 2007, 794, 132–148. [Google Scholar] [CrossRef]
- Wiringa, R.B. Single-particle potential in dense nuclear matter. Phys. Rev. C 1988, 38, 2967. [Google Scholar] [CrossRef]
- Sammarruca, F. The Microscopic Approach to Nuclear Matter and Neutron Star Matter. Int. J. Mod. Phys. E 2010, 19, 1259–1313. [Google Scholar] [CrossRef]
- Behera, B.; Routray, T.R.; Tripathy, S.K. Temperature dependence of the nuclear symmetry energy and equation of state of charge neutral n + p + e + μ matter in beta equilibrium. J. Phys. G Nucl. Part. Phys. 2009, 36, 125105. [Google Scholar] [CrossRef]
- Sammarruca, F.; Krastev, P.G. Spin polarized neutron matter within the Dirac-Brueckner-Hartree-Fock approach. Phys. Rev. C 2007, 75, 034315. [Google Scholar] [CrossRef]
- Behera, B.; Viñas, X.; Routray, T.R.; Robledo, L.M.; Centelles, M.; Pattnaik, S.P. Deformation properties with a finite-range simple effective interaction. J. Phys. G Nucl. Part. Phys. 2016, 43, 045115. Available online: http://iopscience.iop.org/0954-3899/43/4/045115 (accessed on 27 January 2024). [CrossRef]
- Li, B.A.; Ramos, À.; Verde, G.; Vidaña, I. Topical issue on nuclear symmetry energy. Eur. Phys. J. A 2014, 50, 9. [Google Scholar] [CrossRef]
- Chen, L.W.; Cai, B.J.; Ko, C.M.; Li, B.A.; Shen, C.; Xu, J. Higher-order effects on the incompressibility of isospin asymmetric nuclear matter. Phys. Rev. C 2009, 80, 014322. [Google Scholar] [CrossRef]
- Prakash, M.; Bedell, K.S. Incompressibility of neutron-rich nuclear matter. Phys. Rev. C 1985, 32, 1118(R). [Google Scholar] [CrossRef]
- Shlomo, S.; Kolomietz, V.M.; Colò, G. Deducing the nuclear-matter incompressibility coefficient from data on isoscalar compression modes. Eur. Phys. J. A 2006, 30, 23–30. [Google Scholar] [CrossRef]
- Garg, U.; Colò, G. The compression-mode giant resonances and nuclear incompressibility. Prog. Part. Nucl. Phys. 2018, 101, 55. [Google Scholar] [CrossRef]
- Tagami, S.; Wakasa, T.; Yahiro, M. Slope parameters determined from CREX and PREX2. Res. Phys. 2022, 43, 106037. [Google Scholar] [CrossRef]
- Dutra, M.; Lourenço, O.; Avancini, S.S.; Carlson, B.V.; Delfino, A.; Menezes, D.P.; Providência, C.; Typel, S.; Stone, J.R. Relativistic mean-field hadronic models under nuclear matter constraints. Phys. Rev. C 2014, 90, 055203. [Google Scholar] [CrossRef]
- Stone, J.R.; Stone, N.J.; Moszkowski, S.A. Incompressibility in finite nuclei and nuclear matter. Phys. Rev. C 2014, 89, 044316. [Google Scholar] [CrossRef]
- Avogadro, P.; Bertulani, C.A. Role of pairing in the description of giant monopole resonances. Phys. Rev. C 2013, 88, 044319. [Google Scholar] [CrossRef]
- Khan, E.; Margueron, J.; Vidaña, I. Constraining the Nuclear Equation of State at Subsaturation Densities. Phys. Rev. Lett. 2012, 109, 092501. [Google Scholar] [CrossRef]
- Vretenar, D.; Nikšić, T.; Ring, P. A microscopic estimate of the nuclear matter compressibility and symmetry energy in relativistic mean-field models. Phys. Rev. C 2003, 68, 024310. [Google Scholar] [CrossRef]
- Landau, L.D. The Theory of a Fermi Liquid. J. Exp. Theor. Phys. 1957, 3, 920. Available online: http://jetp.ras.ru/cgi-bin/e/index/e/3/6/p920?a=list (accessed on 27 January 2024).
- Landau, L.D. Oscillations in a Fermi Liquid. J. Exp. Theor. Phys. 1957, 5, 101. Available online: http://jetp.ras.ru/cgi-bin/e/index/e/5/1/p101?a=list (accessed on 27 January 2024).
- Landau, L.D. On the theory of the Fermi liquid. J. Exp. Theor. Phys. 1959, 8, 70. Available online: http://jetp.ras.ru/cgi-bin/e/index/e/8/1/p70?a=list (accessed on 27 January 2024).
- Vautherin, D.; Brink, D.M. Hartree-Fock calculations with Skyrme’s interaction. I. Spherical nuclei. Phys. Rev. C 1972, 5, 626. [Google Scholar] [CrossRef]
- Bäckman, S.O.; Källman, C.G.; Sjöberg, O. Calculation of Landau’s fermi-liquid parameters in pure neutron matter. Phys. Lett. B 1973, 43, 263–266. [Google Scholar] [CrossRef]
- Bäckman, S.O.; Jackson, A.D.; Speth, J. Landau parameters for nuclear matter using the Skyrme interaction. Phys. Lett. B 1975, 56, 209–211. [Google Scholar] [CrossRef]
- Cao, L.-G.; Colò, G.; Sagawa, H. Spin and spin-isospin instabilities and Landau parameters of Skyrme interactions with tensor correlations. Phys. Rev. C 2010, 81, 044302. [Google Scholar] [CrossRef]
- Chabanat, E.; Bonche, P.; Haensel, P.; Meyer, J.; Schaeffer, R. A Skyrme parametrization from subnuclear to neutron star densities Part II. Nuclei far from stabilities. Nucl. Phys. A 1998, 635, 231–256. [Google Scholar] [CrossRef]
- Chabanat, E.; Bonche, P.; Haensel, P.; Meyer, J.; Schaeffer, R. A Skyrme parametrization from subnuclear to neutron star densities. Nucl. Phys. A 1997, 627, 710–746. [Google Scholar] [CrossRef]
- Taqi, A.H.; Khidher, E.G. Nuclear multipole excitations in the framework of self-consistent Hartree–Fock random phase approximation for Skyrme forces. Pramana J. Phys. 2019, 93, 60. [Google Scholar] [CrossRef]
- Goriely, S.; Tondeur, F.; Pearson, J.M. A Hartree-Fock nuclear mass table. At. Data Nucl. Data Tables 2001, 77, 311–381. [Google Scholar] [CrossRef]
- Tselyaev, V.; Lyutorovich, N.; Speth, J.; Reinhard, P.-G.; Smirnov, D. Low-energy M1 excitations in 208Pb and the spin channel of the Skyrme energy-density functional. Phys. Rev. C 2019, 99, 064329. [Google Scholar] [CrossRef]
- Agrawal, B.K.; Shlomo, S.; Kim Au, V. Determination of the parameters of a Skyrme type effective interaction using the simulated annealing approach. Phys. Rev. C 2005, 72, 014310. [Google Scholar] [CrossRef]
- Lahiri, J.; Atta, D.; Basu, D.N. Properties of glitching pulsars in the Skyrme-Hartree-Fock framework. arXiv 2022, arXiv:2207.13384v3. [Google Scholar] [CrossRef]
- Keh-Fei, L.; Hongde, L.; Ma, Z.; Shen, Q.; Moszkowski, S.A. Skyrme-Landau parameterization of effective interactions (I). Hartree-Fock ground states. Nucl. Phys. A 1991, 534, 1–24. [Google Scholar] [CrossRef]
- Vuong, A.K. New Skyrme Nucleon-Nucleon Interaction for the Mean-Field Approximation. Doctoral Dissertation, Texas A & M University, College Station, TX, USA, 2003. Available online: http://hdl.handle.net/1969.1/5996 (accessed on 27 January 2024).
- Dutra, M.; Lourenço, O.; Delfino, A. Consistent Skyrme parametrization and its critical parameter values. J. Phys. Conf. Ser. 2019, 1291, 012040. Available online: https://iopscience.iop.org/article/10.1088/1742-6596/1291/1/012040/meta (accessed on 27 January 2024). [CrossRef]
- Nakada, H. Hartree-Fock approach to nuclear matter and finite nuclei with M3Y-type nucleon-nucleon interactions. Phys. Rev. C 2003, 68, 014316. [Google Scholar] [CrossRef]
- Idini, A.; Bennaceur, K.; Dobaczewski, J. Landau parameters for energy density functionals generated by local finite-range pseudopotentials. J. Phys. G Nucl. Part. Phys. 2017, 44, 064004. [Google Scholar] [CrossRef]
- Margueron, J.; Navarro, J.; Van Giai, N. Instabilities of infinite matter with effective Skyrme-type interactions. Phys. Rev. C 2002, 66, 014303. [Google Scholar] [CrossRef]
- Goriely, S.; Chamel, N.; Pearson, J.M. Further explorations of Skyrme-Hartree-Fock-Bogoliubov mass formulas. XII. Stiffness and stability of neutron-star matter. Phys. Rev. C 2010, 82, 035804. [Google Scholar] [CrossRef]
- Li, B.A.; Han, X. Constraining the neutron–proton effective mass splitting using empirical constraints on the density dependence of nuclear symmetry energy around normal density. Phys. Lett. B 2013, 727, 276–281. [Google Scholar] [CrossRef]
- Oertel, M.; Hempel, M.; Klähn, T.; Typel, S. Equations of state for supernovae and compact stars. Rev. Mod. Phys. 2017, 89, 015007. [Google Scholar] [CrossRef]
- Reed, B.T.; Fattoyev, F.J.; Horowitz, C.J.; Piekarewicz, J. Implications of PREX-2 on the Equation of State of Neutron-Rich Matter. Phys. Rev. Lett. 2021, 126, 172503. [Google Scholar] [CrossRef]
- Estee, J.; Lynch, W.G.; Tsang, C.Y.; Barney, J.; Jhang, G.; Tsang, M.B.; Wang, R.; Kaneko, M.; Lee, J.W.; Isobe, T.; et al. Probing the Symmetry Energy with the Spectral Pion Ratio. Phys. Rev. Lett. 2021, 126, 162701. [Google Scholar] [CrossRef]
- Danielewicz, P.; Singh, P.; Lee, J. Symmetry energy III: Isovector skins. Nucl. Phys. A 2017, 958, 147. [Google Scholar] [CrossRef]
- Xie, W.-J.; Li, B.-A. Bayesian Inference of High-density Nuclear Symmetry Energy from Radii of Canonical Neutron Stars. Astrophys. J. 2019, 883, 174. [Google Scholar] [CrossRef]
- Russotto, P.; Gannon, S.; Kupny, S.; Lasko, P.; Acosta, L.; Adamczyk, M.; Al-Ajlan, A.; Al-Garawi, M.; Al-Homaidhi, S.; Amorini, F.; et al. Results of the ASY-EOS experiment at GSI: The symmetry energy at suprasaturation density. Phys. Rev. C 2016, 94, 034608. [Google Scholar] [CrossRef]
- Senger, P. Probing Dense Nuclear Matter in the Laboratory: Experiments at FAIR and NICA. Universe 2021, 7, 171. [Google Scholar] [CrossRef]
- Zhang, Y.; Liu, M.; Xia, C.J.; Li, Z.; Biswal, S.K. Constraints on the symmetry energy and its associated parameters from nuclei to neutron stars. Phys. Rev. C 2020, 101, 034303. [Google Scholar] [CrossRef]
- Zhang, N.B.; Li, B.A. Extracting nuclear symmetry energies at high densities from observations of neutron stars and gravitational waves. Eur. Phys. J. A 2019, 55, 39. [Google Scholar] [CrossRef]
- Tong, H.; Zhao, P.; Meng, J. Symmetry energy at supra-saturation densities via the gravitational waves from GW170817. Phys. Rev. C 2020, 101, 035802. [Google Scholar] [CrossRef]
- Li, B.-A.; Cai, B.-J.; Xie, W.-J.; Zhang, N.-B. Progress in Constraining Nuclear Symmetry Energy Using Neutron Star Observables Since GW170817. Universe 2021, 7, 182. [Google Scholar] [CrossRef]
- Nakazato, K.; Suzuki, H. Cooling Timescale for Protoneutron Stars and Properties of Nuclear Matter: Effective Mass and Symmetry Energy at High Densities. Astrophys. J. 2019, 878, 25. [Google Scholar] [CrossRef]
- Yue, T.-G.; Chen, L.-W.; Zhang, Z.; Zhou, Y. Constraints on the symmetry energy from PREX-II in the multimessenger era. Phys. Rev. Res. 2022, 4, L022054. [Google Scholar] [CrossRef]
- Xie, W.J.; Li, B.A. Bayesian Inference of the Symmetry Energy of Superdense Neutron-rich Matter from Future Radius Measurements of Massive Neutron Stars. Astrophys. J. 2020, 899, 4. [Google Scholar] [CrossRef]
- Zhou, Y.; Chen, L.-W.; Zhang, Z. Equation of state of dense matter in the multimessenger era. Phys. Rev. D 2019, 99, 121301(R). [Google Scholar] [CrossRef]
- Drischler, C.; Furnstahl, R.J.; Melendez, J.A.; Phillips, D.R. How Well Do We Know the Neutron-Matter Equation of State at the Densities Inside Neutron Stars? A Bayesian Approach with Correlated Uncertainties. Phys. Rev. Lett. 2020, 125, 202702. [Google Scholar] [CrossRef]
- Lonardoni, D.; Tews, I.; Gandolfi, S.; Carlson, J. Nuclear and neutron-star matter from local chiral interactions. Phys. Rev. Res. 2020, 2, 022033(R). [Google Scholar] [CrossRef]
- Chen, L.-W. Symmetry energy systematics and its high density behavior. EPJ Web Conf. 2015, 88, 00017. [Google Scholar] [CrossRef]
- Lynch, W.G.; Tsang, M.B. Decoding the density dependence of the nuclear symmetry energy. Phys. Lett. B 2022, 830, 137098. [Google Scholar] [CrossRef]
- Lattimer, J.M.; Lim, Y. Constraining the symmetry parameters of the nuclear interaction. Astrophys. J. 2013, 771, 51. [Google Scholar] [CrossRef]
- Chen, L.W.; Ko, C.M.; Li, B.A. Nuclear matter symmetry energy and the neutron skin thickness of heavy nuclei. Phys. Rev. C 2005, 72, 064309. [Google Scholar] [CrossRef]
- Bano, P.; Pattnaik, S.P.; Centelles, M.; Viñas, X.; Routray, T.R. Correlations between charge radii differences of mirror nuclei and stellar observables. Phys. Rev. C 2023, 108, 015802. [Google Scholar] [CrossRef]
- Li, B.-A.; Magno, M. Curvature-slope correlation of nuclear symmetry energy and its imprints on the crust-core transition, radius, and tidal deformability of canonical neutron stars. Phy. Rev. C 2020, 102, 045807. [Google Scholar] [CrossRef]
- d’Etivaux, N.B.; Guillot, S.; Margueron, J.; Webb, N.; Catelan, M.; Reisenegger, A. New Constraints on the Nuclear Equation of State from the Thermal Emission of Neutron Stars in Quiescent Low-mass X-ray Binaries. Astrophys. J. 2019, 887, 48. [Google Scholar] [CrossRef]
- Carson, Z.; Steiner, A.W.; Yagi, K. Constraining nuclear matter parameters with GW170817. Phys. Rev. D 2019, 99, 043010. [Google Scholar] [CrossRef]
- Choi, S.; Miyatsu, T.; Cheoun, M.; Saito, K. Constraints on Nuclear Saturation Properties from Terrestrial Experiments and Astrophysical Observations of Neutron Stars. Astrophys. J. 2021, 909, 156. [Google Scholar] [CrossRef]
- Drischler, C.; Hebeler, K.; Schwenk, A. Asymmetric nuclear matter based on chiral two- and three-nucleon interactions. Phys. Rev. C 2016, 93, 054314. [Google Scholar] [CrossRef]
- Newton, W.G.; Crocombe, G. Nuclear symmetry energy from neutron skins and pure neutron matter in a Bayesian framework. Phy. Rev. C 2021, 103, 064323. [Google Scholar] [CrossRef]
- Grams, G.; Somasundaram, R.; Margueron, J.; Khan, E. Nuclear incompressibility and speed of sound in uniform matter and finite nuclei. Phys. Rev. C 2022, 106, 044305. [Google Scholar] [CrossRef]
- Sagawa, H.; Yoshida, S.; Cao, L.G. EoS from terrestrial experiments: Static and dynamic polarizations of nuclear density. AIP Conf. Proc. 2019, 2127, 020002. [Google Scholar] [CrossRef]
- Tews, I.; Lattimer, J.M.; Ohnishi, A.; Kolomeitsev, E.E. Symmetry Parameter Constraints from a Lower Bound on Neutron-matter Energy. Astrophys. J. 2017, 848, 105. [Google Scholar] [CrossRef]
- Zhang, N.B.; Cai, B.J.; Li, B.A.; Newton, W.G.; Xu, J. How tightly is the nuclear symmetry energy constrained by a unitary Fermi gas? Nucl. Sci. Tech. 2017, 28, 181. [Google Scholar] [CrossRef]
- Mondal, C.; Agrawal, B.K.; De, J.N.; Samaddar, S.K.; Centelles, M.; Viñas, X. Interdependence of different symmetry energy elements. Phy. Rev C 2017, 96, 021302(R). [Google Scholar] [CrossRef]
- Sagawa, H.; Yoshida, S.; Zeng, G.M.; Gu, J.Z.; Zhang, X.Z. Isospin dependence of incompressibility in relativistic and nonrelativistic mean field calculations. Phys. Rev. C 2007, 76, 034327. [Google Scholar] [CrossRef]
- Li, T.; Garg, U.; Liu, Y.; Marks, R.; Nayak, B.K.; Rao, P.V.M.; Fujiwara, M.; Hashimoto, H.; Kawase, K.; Nakanishi, K.; et al. Isotopic Dependence of the Giant Monopole Resonance in the Even-A 112–124Sn Isotopes and the Asymmetry Term in Nuclear Incompressibility. Phys. Rev. Lett. 2007, 99, 162503. [Google Scholar] [CrossRef]
- Li, T.; Garg, U.; Liu, Y.; Marks, R.; Nayak, B.K.; Rao, P.V.M.; Fujiwara, M.; Hashimoto, H.; Kawase, K.; Nakanishi, K.; et al. Isoscalar giant resonances in the Sn nuclei and implications for the asymmetry term in the nuclear-matter incompressibility. Phys. Rev. C 2010, 81, 034309. [Google Scholar] [CrossRef]
- Cozma, M.D. Feasibility of constraining the curvature parameter of the symmetry energy using elliptic flow data. Eur. Phys. J. A 2018, 54, 40. [Google Scholar] [CrossRef]
- Centelles, M.; Roca-Maza, X.; Viñas, X.; Warda, M. Nuclear Symmetry Energy Probed by Neutron Skin Thickness of Nuclei. Phys. Rev. Lett. 2009, 102, 122502. [Google Scholar] [CrossRef]
- Cai, B.J.; Chen, L.W. Constraints on the skewness coefficient of symmetric nuclear matter within the nonlinear relativistic mean field model. Nucl. Sci. Tech. 2017, 28, 185. [Google Scholar] [CrossRef]
- Farine, M.; Pearson, J.M.; Tondeur, F. Nuclear-matter incompressibility from fits of generalized Skyrme force to breathing-mode energies. Nucl. Phy. A 1997, 615, 135. [Google Scholar] [CrossRef]
- Xie, W.-J.; Li, B.-A. Bayesian inference of the incompressibility, skewness and kurtosis of nuclear matter from empirical pressures in relativistic heavy-ion collisions. J. Phys. G Nucl. Part. Phys. 2021, 48, 025110. [Google Scholar] [CrossRef]
- Steiner, A.W.; Lattimer, J.M.; Brown, E.F. The Equation of State from Observed Masses and Radii of Neutron Stars. Astrophys. J. 2010, 722, 33–54. [Google Scholar] [CrossRef]
- Alam, N.; Agrawal, B.K.; De, J.N.; Samaddar, S.K.; Colò, G. Equation of state of nuclear matter from empirical constraints. Phys. Rev. C 2014, 90, 054317. [Google Scholar] [CrossRef]
- Alam, N.; Agrawal, B.K.; Fortin, M.; Pais, H.; Providência, C.; Raduta, A.R.; Sulaksono, A. Strong correlations of neutron star radii with the slopes of nuclear matter incompressibility and symmetry energy at saturation. Phys. Rev. C 2016, 94, 052801(R). [Google Scholar] [CrossRef]
- Baym, G.; Bethe, H.A.; Pethick, C.J. Neutron star matter. Nucl. Phys. A 1971, 175, 225–271. [Google Scholar] [CrossRef]
- Baym, G.; Pethick, C.J.; Sutherland, P. The Ground State of Matter at High Densities: Equation of State and Stellar Models. Astrophys. J 1971, 170, 299–317. [Google Scholar] [CrossRef]
- De, J.N.; Samaddar, S.K.; Agrawal, B.K. Reassessing nuclear matter incompressibility and its density dependence. Phys. Rev. C 2015, 92, 014304. [Google Scholar] [CrossRef]
- Capano, C.D.; Tews, I.; Brown, S.M.; Margalit, B.; De, S.; Kumar, S.; Brown, D.A.; Krishnan, B.; Reddy, S. Stringent constraints on neutron-star radii from multimessenger observations and nuclear theory. Nat. Astron. 2020, 4, 625–632. [Google Scholar] [CrossRef]
- Raaijmakers, G.; Greif, S.K.; Hebeler, K.; Hinderer, T.; Nissanke, S.; Schwenk, A.; Riley, T.E.; Watts, A.L.; Lattimer, J.M.; Ho, W.C.J. Constraints on the Dense Matter Equation of State and Neutron Star Properties from NICER’s Mass–Radius Estimate of PSR J0740+6620 and Multimessenger Observations. Astrophys. J. Lett. 2021, 918, L29. [Google Scholar] [CrossRef]
- Miller, M.C.; Lamb, F.K.; Dittmann, A.J.; Bogdanov, S.; Arzoumanian, Z.; Gendreau, K.C.; Guillot, S.; Ho, W.C.G.; Lattimer, J.M.; Loewenstein, M.; et al. The Radius of PSR J0740 + 6620 from NICER and XMM-Newton Data. Astrophys. J. Lett. 2021, 918, L28. [Google Scholar] [CrossRef]
- Pang, P.T.H.; Tews, I.; Coughlin, M.W.; Bulla, M.; Broeck, C.V.D.; Dietrich, T. Nuclear Physics Multimessenger Astrophysics Constraints on the Neutron Star Equation of State: Adding NICER’s PSR J0740 + 6620 Measurement. Astrophys. J. 2021, 922, 14. [Google Scholar] [CrossRef]
- Jiang, J.-L.; Tang, S.-P.; Shao, D.-S.; Han, M.-Z.; Li, Y.-J.; Wang, Y.-Z.; Jin, Z.-P.; Fan, Y.-Z.; Wei, D.-M. The Equation of State and Some Key Parameters of Neutron Stars: Constraints from GW170817, the Nuclear Data, and the Low-mass X-Ray Binary Data. Astrophys. J. 2019, 885, 39. [Google Scholar] [CrossRef]
- Abbott, R.; Abbott, T.D.; Abraham, S.; Acernese, F.; Ackley, K.; Adams, C.; Adhikari, R.X.; Adya, V.B.; Affeldt, C.; Agathos, M.; et al. GW190814: Gravitational Waves from the Coalescence of a 23 Solar Mass Black Hole with a 2.6 Solar Mass Compact Object. (LIGO Scientific Collaboration and Virgo Collaboration). Astrophys. J. 2020, 896, L44. [Google Scholar] [CrossRef]
- Bauswein, A.; Blacker, S.; Lioutas, G.; Soultanis, T.; Vijayan, V.; Stergioulas, N. Systematics of prompt black-hole formation in neutron star mergers. Phys. Rev. D 2021, 103, 123004. [Google Scholar] [CrossRef]
- Bauswein, A.; Janka, H.-T. Hebeler, K.; Schwenk, A. Equation-of-state dependence of the gravitational-wave signal from the ring-down phase of neutron-star mergers. Phys. Rev. D 2012, 86, 063001. [Google Scholar] [CrossRef]
- Annala, E.; Gorda, T.; Kurkela, A.; Vuorinen, A. Gravitational-Wave Constraints on the Neutron-Star-Matter Equation of State. Phys. Rev. Lett. 2018, 120, 172703. [Google Scholar] [CrossRef] [PubMed]
- Fattoyev, F.J.; Piekarewicz, J.; Horowitz, C.J. Neutron Skins and Neutron Stars in the Multimessenger Era. Phys. Rev. Lett. 2018, 120, 172702. [Google Scholar] [CrossRef]
- Bauswein, A.; Just, O.; Janka, H.-T.; Stergioulas, N. Neutron-star Radius Constraints from GW170817 and Future Detections. Astrophys. J. Lett. 2017, 850, L34. [Google Scholar] [CrossRef]
- Bauswein, A.; Baumgarte, T.W.; Janka, H.T. Prompt Merger Collapse and the Maximum Mass of Neutron Stars. Phys. Rev. Lett. 2013, 111, 131101. [Google Scholar] [CrossRef]
- Perego, A.; Logoteta, D.; Radice, D.; Bernuzzi, S.; Kashyap, R.; Das, A.; Padamata, S.; Prakash, A. Probing the Incompressibility of Nuclear Matter at Ultrahigh Density through the Prompt Collapse of Asymmetric Neutron Star Binaries. Phys. Rev. Lett. 2022, 129, 032701. [Google Scholar] [CrossRef] [PubMed]
- Shibata, M.; Taniguchi, K.; Uryū, K. Merger of binary neutron stars with realistic equations of state in full general relativity. Phys. Rev. D 2005, 71, 084021. [Google Scholar] [CrossRef]
- Hotokezaka, K.; Kyutoku, K.; Okawa, H.; Shibata, M.; Kiuchi, K. Binary neutron star mergers: Dependence on the nuclear equation of state. Phys. Rev. D 2011, 83, 124008. [Google Scholar] [CrossRef]
- Köppel, S.; Bovard, L.; Rezzolla, L. A General-relativistic Determination of the Threshold Mass to Prompt Collapse in Binary Neutron Star Mergers. Astrophys. J. Lett. 2019, 872, L16. [Google Scholar] [CrossRef]
- Kashyap, R.; Das, A.; Radice, D.; Padamata, S.; Prakash, A.; Logoteta, D.; Perego, A.; Godzieba, D.A.; Bernuzzi, S.; Bombaci, I.; et al. Numerical relativity simulations of prompt collapse mergers: Threshold mass and phenomenological constraints on neutron star properties after GW170817. Phys. Rev. D 2022, 105, 103022. [Google Scholar] [CrossRef]
- Glendenning, N.K. Compact Stars: Nuclear Physics, Particle Physics, and General Relativity; Springer: Berlin/Heidelberg, Germany, 2000. [Google Scholar] [CrossRef]
- Margaritis, C.; Koliogiannis, P.S.; Moustakidis, C.C. Speed of sound constraints on maximally rotating neutron stars. Phys. Rev. D 2020, 101, 043023. [Google Scholar] [CrossRef]
- Koliogiannis, P.S.; Moustakidis, C.C. Constraints on the equation of state from the stability condition of neutron stars. Astrophys. Space Sci. 2019, 364, 52. [Google Scholar] [CrossRef]
- Blaizot, J.P. Nuclear compressibilities. Phys. Rep. 1980, 64, 171–248. [Google Scholar] [CrossRef]
- Zhang, N.B.; Li, B.A. Impact of symmetry energy on sound speed and spinodal decomposition in dense neutron-rich matter. Eur. Phys. J. A 2023, 59, 86. [Google Scholar] [CrossRef]
- Hartle, J.B. Bounds on the mass and moment of inertia of non-rotating neutron stars. Phys. Rep. 1978, 46, 201–247. [Google Scholar] [CrossRef]
- Reed, B.; Horowitz, C.J. Large sound speed in dense matter and the deformability of neutron stars. Phys. Rev. C 2020, 101, 045803. [Google Scholar] [CrossRef]
- Cottam, J.; Paerels, F.; Mendez, M. Gravitationally redshifted absorption lines in the X-ray burst spectra of a neutron star. Nature 2002, 420, 51–54. [Google Scholar] [CrossRef]
- Hambaryan, V.; Neuhäuser, R.; Suleimanov, V.; Werner, K. Observational constraints of the compactness of isolated neutron stars. J. Phys. Conf. Ser. 2014, 496, 012015. [Google Scholar] [CrossRef]
- Hambaryan, V.; Suleimanov, V.; Haberl, F.; Schwope, A.D.; Neuhäuser, R.; Hohle, M.; Werner, K. The compactness of the isolated neutron star RXJ0720.4-3125. Astron. Astrophys. 2017, 601, A108. [Google Scholar] [CrossRef]
- Tang, S.P.; Jiang, J.L.; Gao, W.-H.; Fan, Y.-Z.; Wei, D.-M. The Masses of Isolated Neutron Stars Inferred from the Gravitational Redshift Measurements. Astrophys. J. 2020, 888, 45. [Google Scholar] [CrossRef]
- Sotani, H.; Iida, K.; Oyamatsu, K.; Ohnishi, A. Mass and radius formulas for low-mass neutron stars. Prog. Theor. Exp. Phys. 2014, 2014, 051E01. [Google Scholar] [CrossRef]
- Sotani, H.; Nishimura, N.; Naito, T. New constraints on the neutron-star mass and radius relation from terrestrial nuclear experiments. Prog. Theor. Exp. Phys. 2022, 2022, 041D01. [Google Scholar] [CrossRef]
- Carlson, B.V.; Dutra, M.; Lourenço, O.; Margueron, J. Low-energy nuclear physics and global neutron star properties. Phys. Rev. C 2023, 107, 035805. [Google Scholar] [CrossRef]


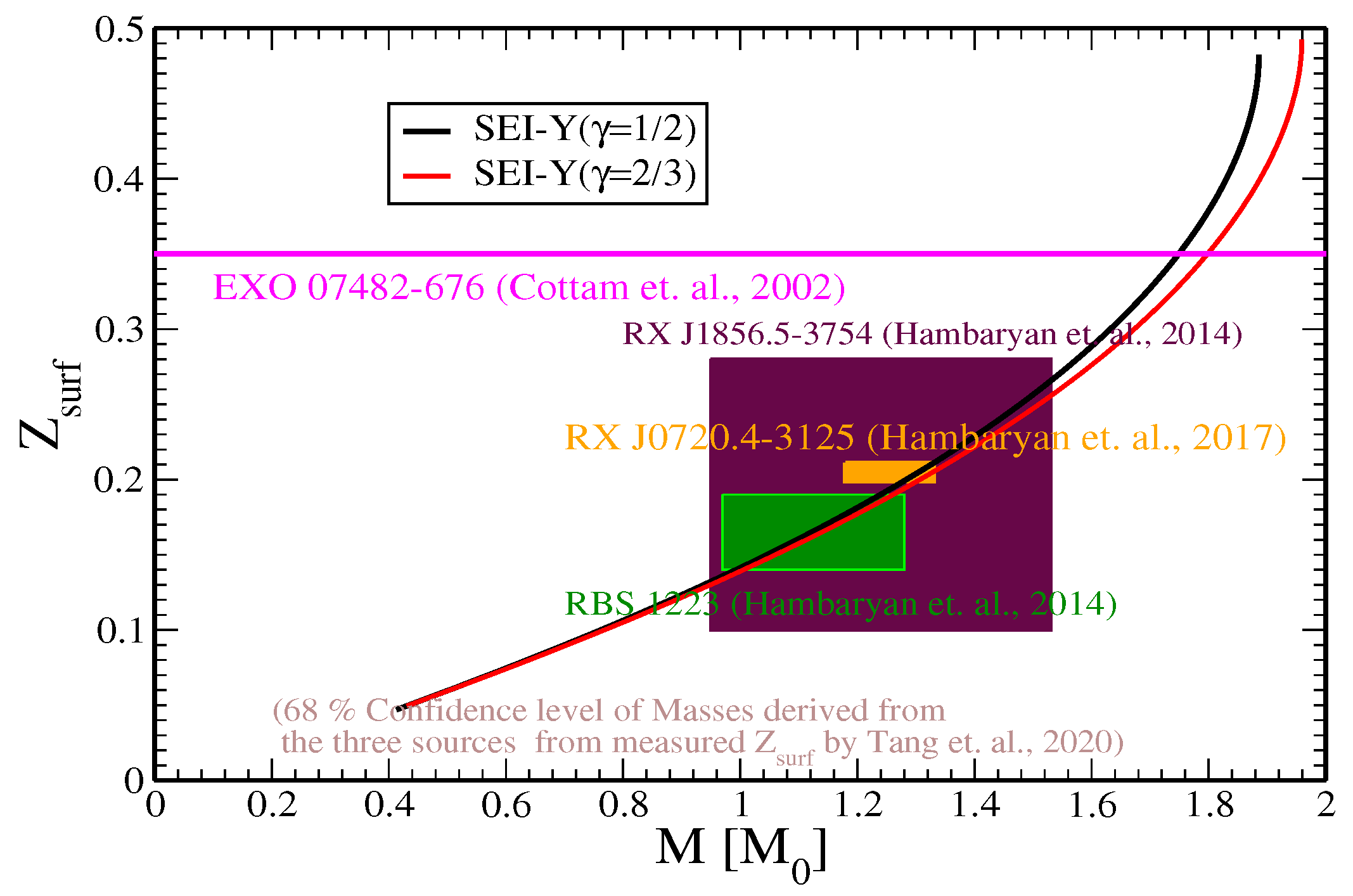
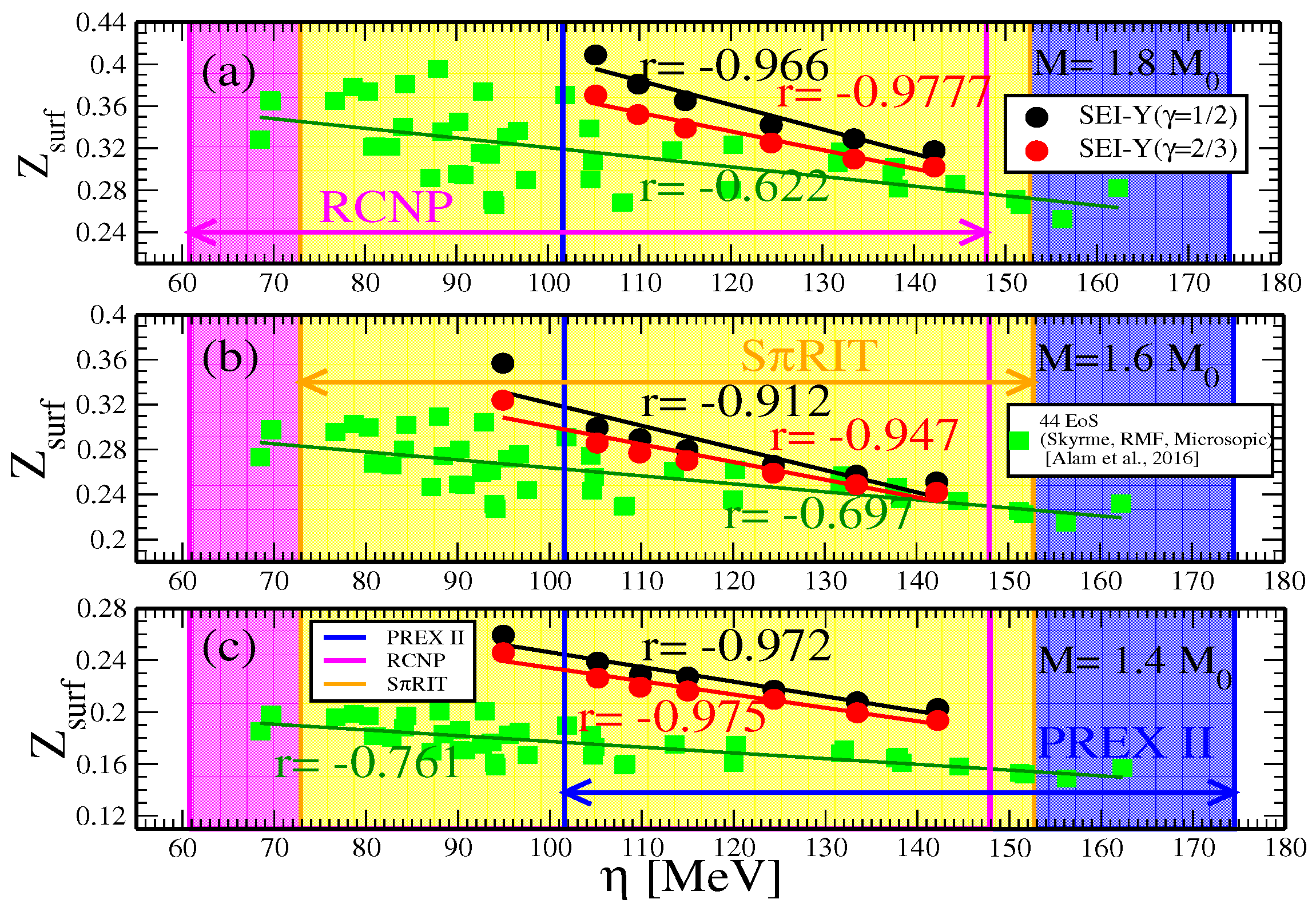
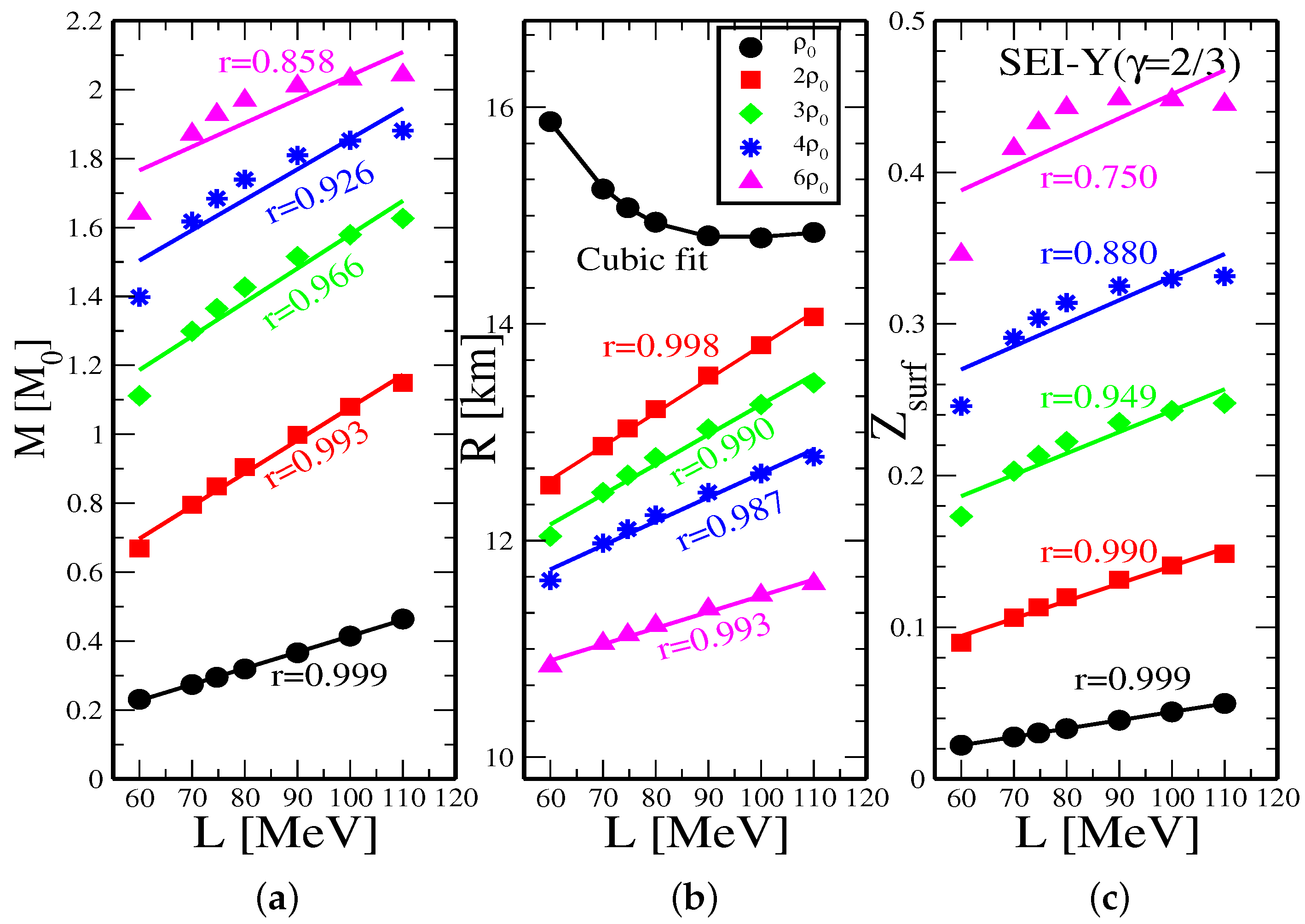
| b [] | [fm] | [MeV] | [MeV] | [MeV] | |
|---|---|---|---|---|---|
| 1/3 | 0.4161 | 0.4232 | −129.344 | −86.229 | −82.245 |
| 1/2 | 0.5880 | 0.4242 | −127.707 | −85.138 | −50.600 |
| 2/3 | 0.7796 | 0.4250 | −126.390 | −84.260 | −34.904 |
| [MeV] | [MeV] | [MeV] | |||
| −47.189 | 104.428 | 74.006 | 333.5 | 1.151 | 119.3 |
| −27.509 | 73.124 | 54.250 | 566.7 | 0.664 | 118.4 |
| −17.859 | 58.095 | 44.690 | 647.4 | 0.520 | 118.2 |
| SEI-Y () | SEI-Y () | SEI-Y () | |
|---|---|---|---|
| [] | 0.161 | 0.158 | 0.156 |
| [MeV] | −16.04 | −16 | −16 |
| 0.664 | 0.686 | 0.666 | |
| 0.685 | 0.621 | 0.622 | |
| [MeV] | 220.346 | 237.643 | 253.219 |
| [MeV] | −478.763 | −461.807 | −437.529 |
| [] | −0.163 | −0.149 | −0.138 |
| [MeV] | 35.5 | 35 | 34.5 |
| L [MeV] | 74.4 | 74.7 | 74.7 |
| [MeV] | −103.487 | −101.471 | −99.252 |
| [MeV] | 273.008 | 252.462 | 234.0525 |
| [MeV] | −388.232 | −404.509 | −418.381 |
| [MeV] | 19.46 | 19 | 18.5 |
| [MeV] | 116.858 | 136.172 | 153.966 |
| [MeV] | −205.754 | −209.345 | −203.476 |
| Expt./Theory | [MeV] |
|---|---|
| Analysis of experimental data of the GMR | |
| S. Shlomo et al. (2006) [39] | |
| U. Garg et al. (2018) [40] | |
| J.R. Stone et al., 2014 [43] | 250–315 |
| P. Avogadro and C.A. Bertulani, 2013 [44] | 200 |
| Relativistic and non-relativistic mean-field calculations of the GMR | |
| E. Khan et al., 2012 [45] | |
| D. Vretenar et al., 2003 [46] | 250–270 |
| M. Dutra et al., 2012 [29] | 246 ± 41 |
| M. Dutra et al., 2014 [42] | 271 ± 86 |
| Analysis of PREX-II and CREX data | |
| S. Tagami et al., 2022 [41] | 210–275 |
| EoS | ||||||
|---|---|---|---|---|---|---|
| SEI-Y () | −0.31 | −0.913 | −0.247 | 0.89 | 0.304 | 0.0826 |
| SEI-Y () | −0.257 | −0.939 | −0.253 | 0.914 | 0.313 | 0.0845 |
| SEI-Y () | −0.195 | −0.9091 | −0.2447 | 0.8771 | 0.303 | 0.0816 |
| EoS | ||||||
| SEI-Y () | 0.617 | 0.0321 | 0.0087 | 0.474 | 0.3739 | 0.1013 |
| SEI-Y () | 0.627 | 0.0479 | 0.0129 | 0.514 | 0.3690 | 0.0998 |
| SEI-Y () | 0.584 | 0.0744 | 0.02001 | 0.5401 | 0.3297 | 0.0887 |
| SEI-Y () | ||||||||
|---|---|---|---|---|---|---|---|---|
| ( ) | [ | [MeV] | [MeV] | [MeV] | [MeV] | [MeV] | ||
| () | 0.161 | 230.59 | 0.695 | 1.101 | 35.10 | 30.02 | 27.38 | 24.47 |
| () | 0.158 | 237.74 | 0.686 | 1.104 | 34.048 | 28.95 | 26.94 | 26.38 |
| () | 0.156 | 263.14 | 0.696 | 1.101 | 34.10 | 28.79 | 27.97 | 27.94 |
| Expt./Observation/Theory | L [MeV] |
|---|---|
| Terrestrial Experiments and Astrophysical Observations | |
| Li and Han, 2013 [68] | 58.9 ± 16.5 |
| Oertel et al., 2017 [69] | 58.7 ± 28.1 |
| Lattimer and Lim, 2013 [88] | 40.5–61.9 |
| Xie et al., 2019, 2020 [73,82] | |
| PREX-II Experiment | |
| Reed et al., 2021 [70] | 106 ± 37 |
| Charge exchange and elastic scattering data Danielewicz et al., 2017 [72] | 70–101 |
| Charged Pion Spectra at high momenta Estee et al., 2021 [71] | 42–117 |
| Isospin Diffusion Data Chen et al., 2005 [89] | 63–113 |
| Charge radii difference in mirror pairs | |
| Bano et al., 2023 [90] | 70–100 |
| Expt./Observation/Theory | [MeV] |
| Analysis of Different Neutron Star Observables Li et al., 2020 [91] | |
| d’Etivaux et al., 2019 [92] | |
| Carson et al., 2019 [93] | −259 to +32 |
| Choi et al., 2021 [94] | −128 to −33 |
| Chiral Effective Field Theory | |
| Drischler et al., 2016 [95] | −240 to −70 |
| Newton and Crocombe, 2021 [96] | |
| Grams et al., 2022 [97] | −200 to +50 |
| Terrestrial Nuclear Experiments and Mean-Field Predictions | |
| Sagawa et al., 2019 [98] | |
| Tews et al., 2017 [99], Zhang et al., 2017 [100] | −400 to +100 |
| Mondal et al., 2017 [101] | |
| Expt./Theory | [MeV] |
| Experimental data of Isoscalar Giant Monopole Resonances | |
| Sagawa et al., 2008 [102] | |
| Li et al., 2010 [103,104] | |
| Stone et al., 2014 [43] | −840 to −350 |
| Theoretical calculations of GMR with MDI interactions Chen et al., 2009 [37] | |
| Cozma, 2018 [105] | |
| Neutron skin sizes across the mass table | |
| Centelles et al., 2009 [106] |
| SEI-Y () | ||||||
|---|---|---|---|---|---|---|
| Ref | a | b | k | |||
| [127] | −3.342 | 2.42 | 10.523 | 0.275 | 1.499 | 2.937 |
| [126] | −3.38 | 2.43 | 10.523 | 0.275 | 1.498 | 2.936 |
| [132] | 10.523 | 0.275 | ||||
| SEI-Y () | ||||||
| Ref | a | b | k | |||
| [127] | −3.342 | 2.42 | 10.243 | 0.272 | 1.5095 | 2.846 |
| [126] | −3.38 | 2.43 | 10.243 | 0.272 | 1.5091 | 2.845 |
| [132] | 10.243 | 0.272 | ||||
| SEI-Y () | ||||||
| Ref | a | b | k | |||
| [127] | −3.342 | 2.42 | 9.943 | 0.267 | 1.5252 | 2.7437 |
| [126] | −3.38 | 2.43 | 9.943 | 0.267 | 1.5250 | 2.7434 |
| [132] | 9.943 | 0.267 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Viñas, X.; Bano, P.; Naik, Z.; Routray, T.R. Nuclear Matter Properties and Neutron Star Phenomenology Using the Finite Range Simple Effective Interaction. Symmetry 2024, 16, 215. https://doi.org/10.3390/sym16020215
Viñas X, Bano P, Naik Z, Routray TR. Nuclear Matter Properties and Neutron Star Phenomenology Using the Finite Range Simple Effective Interaction. Symmetry. 2024; 16(2):215. https://doi.org/10.3390/sym16020215
Chicago/Turabian StyleViñas, Xavier, Parveen Bano, Zashmir Naik, and Tusar Ranjan Routray. 2024. "Nuclear Matter Properties and Neutron Star Phenomenology Using the Finite Range Simple Effective Interaction" Symmetry 16, no. 2: 215. https://doi.org/10.3390/sym16020215
APA StyleViñas, X., Bano, P., Naik, Z., & Routray, T. R. (2024). Nuclear Matter Properties and Neutron Star Phenomenology Using the Finite Range Simple Effective Interaction. Symmetry, 16(2), 215. https://doi.org/10.3390/sym16020215






