Dense Baryonic Matter Predicted in “Pseudo-Conformal Model”
Abstract
1. Introduction
2. GEFT
3. Predictions
3.1. Density Regime
3.2. Density Regime
3.3. Predictions vs. Observables
- Smoothed cusp of at :The bending-over of influences the slope L and induces the “soft-to-stiff” changeover. It also plays a crucial role in giving rise to the pseudo-conformal sound velocity (to be addressed below). Although as stressed the detailed structure and magnitude cannot be precisely pinned down, its simplicity with intricate topology change in the jungle of theories (as depicted in [9]) is a distinctive prediction of the PCM. It is at odds with the PREX-II/Jefferson data, which give generally stiff EoS, although there are some caveats [18,19]. To date, there are no trustworthy experimental data to quantitatively compare with.
- Maximum mass star: :No empirical data are known to be available at present for the central density . The only information on this quantity inferred—not extracted—from PSR J0740 + 6620 is violently at odds with the PCM prediction. I will address this issue below.
- 1.44 star:The stunning agreements between the PCM predictions and the NICER and XMM-Newton measurements—with the exception of the sound velocity to be addressed below—could not be accidental. Not only does the maximum star mass come out the same, but also the radii agree. Furthermore, the difference , in agreement with the data. We will note later that this support of the PCM by the NICER/XMM-Newton has an even more surprising implication on scale-chiral symmetry in nuclear medium so far unsuspected.
- Tidal deformabilityThe predicted in the PCM comes out to be ∼550, to be compared with (GW1700817). This may seem to signal a tension. However, there is a basic difficulty in theoretically pinning down . In the PCM, the density at which is measured is ∼. This density sits very close to where the topology change takes place. It is here the SchiEFT is most likely to start breaking down as the cusp in indicates and the pQCD cannot access. This is an “uncharted wilderness” for theory. As can be seen in [4], a small increase in the central density, say, from to (or increase in corresponding star mass), makes drop to 420 while involving no change at all in radius. This means that the location of the HQC will strongly influence the . One can associate this behavior with the increase in attraction in going from toward in the cusp structure as one can see in the schematic plot Figure 1. This clearly suggests that it would be extremely difficult to theoretically pin down in the vicinity of the crossover regime.As noted below, the sound velocity has a complex “bump” structure in the vicinity of the topology change density. This is due to the interplay, encoding the putative HQC, between the hadronic degrees of freedom and the “dual quark-gluon” degrees of freedom. This would complicate significantly the linking of to the structure of the sound velocity below or near . To give an example, let me quote [23] where the bump structure—“the slope, the hill, the drop, the swoosh, etc.”—associated with the possible phase structure of QCD is proposed to pin down by up-coming measurements. The hope here is to determine the possible phase transition near the HQC density. Given the theoretical wilderness inevitably involved, this seems a far-fetched endeavor.In short, contrary to what is claimed by some workers in the field, ruling out an EoS based on the precise value of would be premature.
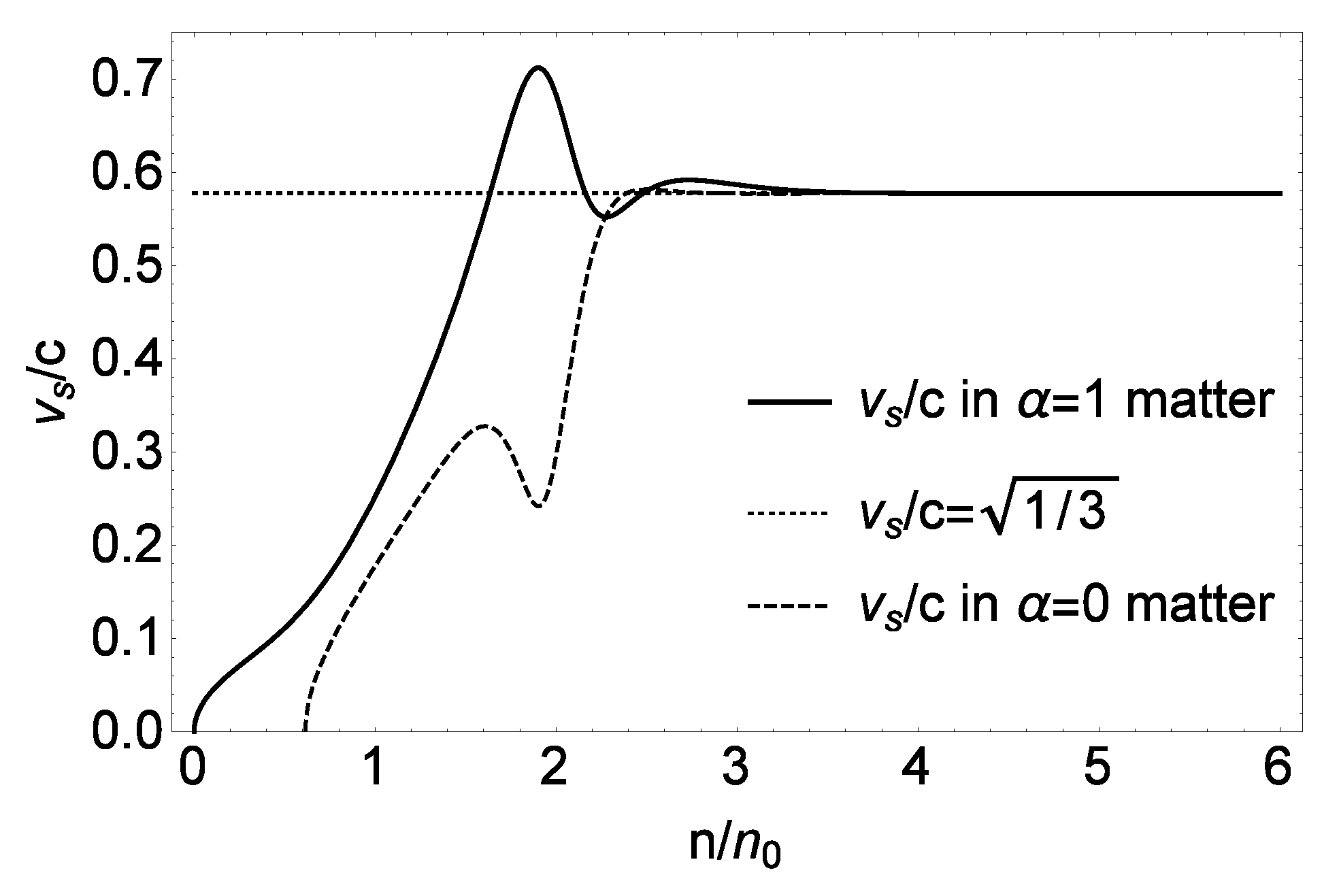
- Sound speedThe most striking prediction of the PCM, so far not shared by other models, is the sound speed for . It predicts the pseudo-conformal sound speedIt is not to be identified with the conformal sound speed because the energy-momentum tensor is not traceless, i.e., scale symmetry is spontaneously broken.This prediction can be understood as follows.As noted above, the quasiparticle mass goes as the density goes above and the dilaton condensate becomes independent of density, reaching . This has to do with a delicate interplay between the attraction associated with the dilaton exchange and the repulsion, which leads to the parity doubling. Where this interplay starts taking place cannot be pinned down precisely but it must be in the density regime where the symmetry energy is involved, going from to the core of massive stars, say, . In this density regime, the Landau fixed-point approximation with can be taken to be reliable. One can then calculate the trace of the energy-momentum tensor in the mean-field approximation of GnEFT, i.e., LFL fixed-point approximation, which will become density-independent as given by (11). In this density range we will havewhere is the energy density and . It is approximate since there can easily be terms that are compounded with EFT and pQCD at the point where the symmetry energy has the cusp structure. Since there is no Lee-Wick-type state, one must havewhich gives the pseudo-conformal sound speedThe “approximate zero” here stands for the fact that it is pseudo-conformal with scale symmetry broken both explicitly and spontaneously, the dilaton mass and the mass balancing so as to lead to parity doubling in the dense system. The true conformal velocity, within the model, should be reached only at a density much higher than that of the core density of the massive stars. Where precisely the conformality sets in is not relevant to the compact star physics.In Figure 3 is shown the sound speed for (nuclear matter) and 1 (neutron matter) calculated in RG for . They are of the same form for except for the slight shift in the density and the height of . This result serves as an illustration of the arguments to follow.What is noticeable is the large bump in in the vicinity of and the rapid convergence to the speed 1/3. The approximation involved on top of the pseudo-conformality would of course give fluctuations on top of but the point here is it is the pseudo-conformality that “controls” the general structure. The large bump signals a complex interplay between hadronic and non-hadronic degrees of freedom manifested through the pseudo-gap structure of the chiral condensates. I will discuss below how the degrees of freedom in the core of the massive stars could masquerade as “deconfined quarks”.
- Quenched in nucleiThough it is not directly connected with the star properties, a relevant and intriguing observation is what I would call “quasibaryon” in nuclear matter. It follows from the possible existence of the IR fixed point associated with the “genuine dilaton (GD)." The effective in the Gamow–Teller transitions in nuclei, , is observed to be from light nuclei to heavy nuclei and even to the dilaton-limit fixed point at . It has been argued that an approximate scale invariance “emerges” in nuclear interactions [24], in a way most likely related to the way sets in precociously.Returning to , is there any indication in recent astrophysical observations for such a precocious onset of the pseudo-conformal sound velocity?To date, there is no known “smoking-gun” signal for the sound velocity from observations. In the literature, however, there are a gigantic number of articles on the structure of sound velocity deduced from the gravity wave data as well as theoretically. Some argue for phase transitions or continuous ones or simply no crossovers, etc. Some extreme cases are discussed in [9]. I will no go into this wilderness here. Let me just describe one case which illustrates most transparently what can very well be involved.Let us take the case of NICER and XMM-Newton observables (NXN for short) discussed, namely (13) and (14). This case brings out how puzzling the problem can be.In [25], the properties of high-density matter were inferred in most detailed analyses of the NXN data. Ruling out essentially all other scenarios, with or without phase changes, the authors arrive at the sound velocity (“H-bump”) plotted in Figure 4.The central density and the maximum sound velocity inferred wereWhile the star properties they took into account are exactly those reproduced by the PCM, i.e., (13) and (14), the central density and the sound velocity are totally different from the PCM predictions. One can understand the low central density accounting for the sound speed overshooting the conformal bound, characteristic of strongly interacting hadronic phase. In fact there are in the literature numerous scenarios anchored on a variety of density-functional approaches giving rise to the wilderness of one form or other in the sound velocity—including bumps similar to the H-bump—but I am not aware of any that can survive the battery of bona fide constraints coming from the current observations both in theory and experiment as claimed by [25].A puzzle immediately raised is this: How can the PCM with an emergent (pseudo-)conformality and the strong H-bump with no hint of conformal symmetry give the almost identical global star properties (13) and (14)? The only statement one can make at this point is (A) either the sound velocity and the global star properties are totally unrelated or (B) there is something wrong either in the strong H-bump scenario or in the simple PCM structure. Option (A) is hard to accept, so perhaps option (B) is a plausible possibility. My bet is option (B) and the H-bump scenario are at odds with nature.
- Conformailty
4. Conclusion: The Duck Story
Funding
Data Availability Statement
Conflicts of Interest
References
- Rho, M. ’Mass without mass’ and nuclear matter. arXiv 2017, arXiv:1703.02277. [Google Scholar]
- Paeng, W.G.; Kuo, T.T.S.; Lee, H.K.; Rho, M. Scale-invariant hidden local symmetry, topology change and dense baryonic matter. Phys. Rev. C 2016, 93, 055203. [Google Scholar] [CrossRef]
- Paeng, W.G.; Kuo, T.T.S.; Lee, H.K.; Ma, Y.L.; Rho, M. Scale-invariant hidden local symmetry, topology change, and dense baryonic matter. II. Phys. Rev. D 2017, 96, 014031. [Google Scholar] [CrossRef]
- Ma, Y.L.; Rho, M. Towards the hadron–quark continuity via a topology change in compact stars. Prog. Part. Nucl. Phys. 2020, 113, 103791. [Google Scholar] [CrossRef]
- Rho, M.; Ma, Y.L. Manifestation of hidden symmetries in baryonic matter: From finite nuclei to neutron stars. Mod. Phys. Lett. A 2021, 36, 2130012. [Google Scholar] [CrossRef]
- Park, B.Y.; Vento, V. Skyrmion approach to finite density and temperature. In The Multifacetted Skyrmion; Brown, G.E., Rho, M., Eds.; World Scientific: Singapore, 2010. [Google Scholar]
- Lee, H.K.; Park, B.Y.; Rho, M. Half-skyrmions, tensor forces and symmetry energy in cold dense matter. Phys. Rev. C 2011, 83, 025206, Erratum in Phys. Rev. C 2011, 84, 059902. [Google Scholar] [CrossRef]
- Adam, C.; García Martín-Caro, A.; Huidobro, M.; Vázquez, R.; Wereszczynski, A. Quantum skyrmion crystals and the symmetry energy of dense matter. Phys. Rev. D 2022, 106, 114031. [Google Scholar] [CrossRef]
- Lee, H.K.; Ma, Y.L.; Paeng, W.G.; Rho, M. Cusp in the symmetry energy, speed of sound in neutron stars and emergent pseudo-conformal symmetry. Mod. Phys. Lett. A 2022, 37, 2230003. [Google Scholar] [CrossRef]
- Garaud, J.; Babaev, E. Effective model and magnetic properties of the resistive electron quadrupling state. Phys. Rev. Lett. 2022, 129, 087602. [Google Scholar] [CrossRef]
- Harada, M.; Yamawaki, K. Hidden local symmetry at loop: A New perspective of composite gauge boson and chiral phase transition. Phys. Rep. 2003, 381, 1. [Google Scholar] [CrossRef]
- Crewther, R.J. Genuine dilatons in gauge theories. Universe 2020, 6, 96. [Google Scholar] [CrossRef]
- Crewther, R.J.; Tunstall, L.C. ΔI = 1/2 rule for kaon decays derived from QCD infrared fixed point. Phys. Rev. D 2015, 91, 034016. [Google Scholar]
- Brown, G.E.; Rho, M. Double decimation and sliding vacua in the nuclear many body system. Phys. Rep. 2004, 396, 1–39. [Google Scholar] [CrossRef]
- Shankar, R. Renormalization group approach to interacting fermions. Rev. Mod. Phys. 1994, 66, 129–192. [Google Scholar] [CrossRef]
- Friman, B.; Rho, M. From chiral Lagrangians to Landau Fermi liquid theory of nuclear matter. Nucl. Phys. A 1996, 606, 303. [Google Scholar] [CrossRef]
- Song, C. Dense nuclear matter: Landau Fermi liquid theory and chiral Lagrangian with scaling. Phys. Rep. 2001, 347, 289–371. [Google Scholar] [CrossRef]
- Piekarewicz, J. The nuclear physics of neutron stars. arXiv 2022, arXiv:2209.14877. [Google Scholar]
- Salinas, M.; Piekarewicz, J. Building an equation of state density ladder. Symmetry 2023, 15, 994. [Google Scholar] [CrossRef]
- He, B.R.; Harada, M. Parity doubling among baryons in a holographic QCD model at finite density. PoS 2013, arXiv:10.22323/1.205.0192, 192. [Google Scholar]
- Zhang, P.; Kimm, K.; Zou, L.; Cho, Y.M. Re-interpretation of Skyrme theory: New topological structures. arXiv 2017, arXiv:1704.05975. [Google Scholar]
- Beane, S.R.; van Kolck, U. The dilated chiral quark model. Phys. Lett. B 1994, 328, 137–142. [Google Scholar] [CrossRef]
- Tan, H.; Dexheimer, V.; Noronha-Hostler, J.; Yunes, N. Finding structure in the speed of sound of supranuclear matter from binary love relations. Phys. Rev. Lett. 2022, 128, 161101. [Google Scholar] [CrossRef]
- Rho, M. Multifarious roles of hidden chiral-scale symmetry: “Quenching” gA in Nuclei. Symmetry 2021, 13, 1388. [Google Scholar] [CrossRef]
- Legred, I.; Chatziioannou, K.; Essick, R.; Han, S.; Landry, P. Impact of the PSR J0740+6620 radius constraint on the properties of high-density matter. Phys. Rev. D 2021, 104, 063003. [Google Scholar] [CrossRef]
- Fujimoto, Y.; Fukushima, K.; McLerran, L.D.; Praszalowicz, M. Trace anomaly as signature of conformality in neutron stars. Phys. Rev. Lett. 2022, 129, 252702. [Google Scholar] [CrossRef] [PubMed]
- Marczenko, M.; McLerran, L.; Redlich, K.; Sasaki, C. Reaching percolation and conformal limits in neutron stars. Phys. Rev. C 2023, 107, 025802. [Google Scholar] [CrossRef]
- Rojas, J.C.; Demircik, T.; Järvinen, M. Popcorn transitions and approach to conformality in homogeneous holographic nuclear matter. Symmetry 2023, 15, 331. [Google Scholar] [CrossRef]
- Annala, E.; Gorda, T.; Hirvonen, J.; Komoltsev, O.; Kurkela, A.; Nättilä, J.; Vuorinen, A. Strongly interacting matter exhibits deconfined behavior in massive neutron stars. arXiv 2023, arXiv:2303.11356. [Google Scholar]
- Ma, Y.L.; Rho, M. The sound speed and core of massive compact stars: A manifestation of hadron-quark duality. arXiv 2021, arXiv:2104.13822. [Google Scholar]
- Fujimoto, Y.; Kojo, T.; McLerran, L.D. Momentum shell in quarkyonic matter from explicit duality: A solvable model nalysis. arXiv 2023, arXiv:2306.04304. [Google Scholar]
- Meng, X.L.; Sun, P.; Alexandru, A.; Horváth, I.; Liu, K.-F.; Wang, G.; Yang, Y.-B. Separation of infrared and bulk in thermal QCD. arXiv 2023, arXiv:2305.09459. [Google Scholar]
- Annala, E.; Gorda, T.; Kurkela, A.; Nättilä, J.; Vuorinen, A. Evidence for quark-matter cores in massive neutron stars. Nat. Phys. 2020, 16, 907–910. [Google Scholar] [CrossRef]
- Ma, Y.L.; Rho, M. What’s in the core of massive neutron stars? arXiv 2020, arXiv:2006.14173. [Google Scholar]
- Rho, M. Fractionalized quasiparticles in dense baryonic matter. arXiv 2020, arXiv:2004.09082. [Google Scholar]
- Sarti, V.M.; Vento, V. The half-skyrmion phase in a chiral-quark model. Phys. Lett. B 2014, 728, 323–327. [Google Scholar] [CrossRef]
- Sulejmanpasic, T.; Shao, H.; Sandvik, A.; Unsal, M. Confinement in the bulk, deconfinement on the wall: Infrared equivalence between compactified QCD and quantum magnets. Phys. Rev. Lett. 2017, 119, 091601. [Google Scholar] [CrossRef] [PubMed]



Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Rho, M. Dense Baryonic Matter Predicted in “Pseudo-Conformal Model”. Symmetry 2023, 15, 1271. https://doi.org/10.3390/sym15061271
Rho M. Dense Baryonic Matter Predicted in “Pseudo-Conformal Model”. Symmetry. 2023; 15(6):1271. https://doi.org/10.3390/sym15061271
Chicago/Turabian StyleRho, Mannque. 2023. "Dense Baryonic Matter Predicted in “Pseudo-Conformal Model”" Symmetry 15, no. 6: 1271. https://doi.org/10.3390/sym15061271
APA StyleRho, M. (2023). Dense Baryonic Matter Predicted in “Pseudo-Conformal Model”. Symmetry, 15(6), 1271. https://doi.org/10.3390/sym15061271





