On λ-Pseudo Bi-Starlike Functions Related to Second Einstein Function

Abstract

A new class ${\mathcal{B}}_{\mathsf{\Sigma }}^{\lambda }(\gamma ,\kappa )$ of bi-starlike $\lambda$-pseudo functions related to the second Einstein function is presented in this paper. $c_2$ and $c_3$ indicate the initial Taylor coefficients of $\varphi \in {\mathcal{B}}_{\mathsf{\Sigma }}^{\lambda }(\gamma ,\kappa ),$ and the bounds for $|c_2|$ and $|c_3|$ are obtained. Additionally, for $\varphi \in {\mathcal{B}}_{\mathsf{\Sigma }}^{\lambda }(\gamma ,\kappa )$, we calculate the Fekete–Szegö functional.

1. Introduction

Consider the set $\mathcal{A}$ of all functions of the series notation

$$\varphi (\eta )=\eta +\sum _{m=2}^{\infty }{c}_m{\eta }^m,$$

(1)

defined in the open unit disc $\mathbb{D}=\{\eta :\eta \in \mathbb{C}\mathrm{and}|\eta |<1\},$ assume these functions are analytic. Additionally, let $\mathcal{S}\subset \mathcal{A}$, and if $\varphi \in \mathcal{S}$, then $\varphi$ is univalent with $\varphi (0)=0={\varphi }^{\prime }(0)-1$.

We say that an analytic function $\varphi$ is subordinate to an analytic function $\psi$ if there exists an analytic function $\omega$ in $\mathbb{D}$ such that $\omega (0)=0,|\omega (\eta )|<1$ for $|\eta |<1$ and $\varphi (\eta )=\psi (\omega (\eta ))$. Specifically, in the event that $\psi$ is univalent in $\mathbb{D},$ the corresponding equivalency is as follows:

$$\varphi (\eta )\prec \psi (\eta )⟺\varphi (0)=\psi (0)\mathrm{and}\varphi (|\eta |<1)\subset \psi (|\eta |<1).$$

For starlike functions of order $\nu$, the class ${\mathcal{S}}^{*}(\nu )$ is a significant and well-studied subclass of $\mathcal{S}$. It is defined by the following condition

$$Re\left({\displaystyle \frac{\eta {\varphi }^{\prime }(\eta )}{\varphi (\eta )}}\right)>\nu ,(0\le \nu <1;\eta \in \mathbb{D}),$$

and the class of convex functions of order $\nu$ in $\mathcal{K}(\nu )\subset \mathcal{S}$, is defined by condition

$$Re\left(1+{\displaystyle \frac{\eta {\varphi }^{″}(\eta )}{{\varphi }^{\prime }(\eta )}}\right)>\nu ,(0\le \nu <1;\eta \in \mathbb{D}).$$

The set ${\mathcal{B}}_{\lambda }(\nu )$ of $\lambda$-pseudo-starlike functions of order $\nu$ were given and studied by Babalola [1]. If $\varphi \in \mathcal{A},$ then $\varphi \in {\mathcal{B}}_{\lambda }(\nu )$ if

$$Re\left({\displaystyle \frac{\eta {({\varphi }^{\prime }(\eta ))}^{\lambda }}{\varphi (\eta )}}\right)>\nu ,(0\le \nu <1;\eta \in \mathbb{D}).$$

It was demonstrated in [1] that all pseudo-starlike functions are univalent in open unit disc $\mathbb{D}$ and Bazilevič of type $\left(1-1/\lambda \right)$ with order ${\nu }^{1/\lambda }$.

We consider the set $\mathcal{P}$ to be the collection of all analytic functions $h:\mathbb{D}\to \mathbb{C}$ satisfying the conditions $h(0)>0$, h is univalent with $Re(h(\eta ))>0$, $h(\mathbb{D})$ is starlike with respect to 1, and $h(\mathbb{D})$ is symmetric about a real axis.

In the field of computation sciences, symmetry is essential, particularly in geometric function theory. We use the expression

$$\mathsf{\Phi }(z)={\displaystyle \frac{1+Az}{1+Bz}},$$

where $-1\le B<A\le 1$, to illustrate this role. Convex in kind, $\mathsf{\Phi }$ transfers the open unit U conformally onto a disc symmetrical with respect to the real axis. The disc has a radius equal to ${\displaystyle \frac{A-B}{1-B^2}}$ $(B\ne \pm 1)$ and is centered at the point ${\displaystyle \frac{1-AB}{1-B^2}}$ $(B\ne \pm 1)$. Additionally, the disc’s boundary circle crosses the real axis at $\frac{1-A}{1-B}$ and $\frac{1+A}{1+B}$, which yields $B\ne \pm 1$. Great research opportunities in the field of geometric function theory were made possible by this symmetric function. We refer the reader to Janowski’s 1973 introduction of the well-known starlike and convex functions criteria (see [2]).

$${\displaystyle \frac{z{f}^{\prime }(z)}{f(z)}}\prec {\displaystyle \frac{1+Az}{1+Bz}},$$

and

$$1+{\displaystyle \frac{z{f}^{″}(z)}{f^{\prime }(z)}}\prec {\displaystyle \frac{1+Az}{1+Bz}}.$$

The cosine function [3], secant function [4], balloon function [5], and many other symmetric functions are the subject of several investigations. In this paper, we applied the symmetry of a certain Einstein function. The preliminaries of Einstein functions are given in this section.

Gradshteyn and Ryzhik [6] gave the formula defining Bernoulli polynomials in 1980. These polynomials have important applications in number theory and classical analysis. They may be found in the integral representation of differentiable periodic functions since they are used to approximate such functions in terms of polynomials. They are also used in the expression of the remainder term of the unified Euler–MacLaurin quadrature rule.

The generating function is typically used to define the Bernoulli polynomials $b_m(y)$ (see, e.g., [7]).

$$G(y,t):={\displaystyle \frac{t{e}^{yt}}{e^t-1}}=\sum _{m=0}^{\infty }{\displaystyle \frac{b_m(y)}{m!}}{t}^m,0<|t|<2\pi ,$$

(2)

Recursion is a simple method for computing the Bernoulli polynomials, since

$$\sum _{j=0}^{m-1}\left({\displaystyle \genfrac{}{}{0pt}{}{m}{j}}\right)b_j(y)=n{y}^{m-1},m=2,3,\cdots$$

The initial Bernoulli polynomials are

$$b_0(y)=1,b_1(y)=y-{\displaystyle \frac{1}{2}},b_2(y)=y^2-y-{\displaystyle \frac{1}{6}},b_3(y)=y^3-{\displaystyle \frac{3}{2}}{y}^2+{\displaystyle \frac{1}{2}}y,\cdots$$

Moreover, by substituting $y=0$ in Bernoulli polynomials, one may directly obtain Bernoulli numbers $b_m:=b_m(0)$. Among the initial Bernoulli numbers are

$$b_0=1,b_1=-{\displaystyle \frac{1}{2}},b_2={\displaystyle \frac{1}{6}},\cdots \mathrm{and}{b}_{2m+1}=0,\forall m=1,2,\cdots .$$

Additionally, the so-called Einstein function $E(\eta )$ may be used to construct Bernoulli numbers $b_m$, as follows:

$$E(\eta ):={\displaystyle \frac{\eta }{e^{\eta }-1}}=\sum _{m=0}^{\infty }{\displaystyle \frac{b_m}{m!}}{\eta }^m.$$

(3)

Every one of the following functions might occasionally be called the Einstein function (see [8,9]):

$$E_1(\eta ):={\displaystyle \frac{\eta }{e^{\eta }-1}},E_2(\eta ):={\displaystyle \frac{{\eta }^2{e}^{\eta }}{{\left(e^{\eta }-1\right)}^2}},E_3(\eta ):=log\left(1-e^{-\eta }\right),E_4(\eta ):={\displaystyle \frac{\eta }{e^{\eta }-1}}-log\left(1-e^{-\eta }\right).$$

The range of $E_2$, is symmetric around the x-axis and starlike w.r.t. $\eta =1$ ($E_2$ is convex) and $Re\left(E_2(\eta )\right)>0$ ∀ $\eta \in \mathbb{D}$. It is evident that both functions $E_1$ and $E_2$ have these properties, but $E_3$ and $E_4$ do not (see Figure 1).

Figure 1. Images of the open unit disc by Einstein functions.

The series forms of $E_1$ and $E_2$ are

$$E_1(\eta )=1+\sum _{m=1}^{\infty }{\displaystyle \frac{b_m}{m!}}{\eta }^m,and{E}_2(\eta )=1+\sum _{m=1}^{\infty }{\displaystyle \frac{(1-m)b_m}{m!}}{\eta }^m,$$

(4)

such that $b_m$ refers to the Bernoulli numbers.

Einstein function $E_1$ has been the subject of various results introduced by El-Qadeem et al. [10]. El-Qadeem et al. [11] have also applied the Einstein function $E_2$ to introduce various results.

Here, we employ $E_2$ to define a novel subclass of pseudo bi-Starlike functions. Note that $E_2^{\prime }(0)\ngtr 0$ (indeed $E_2^{\prime }(0)=0$), i.e., $E_2\notin \mathcal{P}$. Thus, a modified version of the Einstein function ${\mathbb{E}}_{\kappa }$ is defined for this purpose.

$${\mathbb{E}}_{\kappa }(\eta ):=E_2(\eta )+\kappa \eta ,$$

(5)

where $\kappa \in I:=[0.28,0.92]$. Then ${\mathbb{E}}_{\kappa }(\mathbb{D})$ is a convex domain, symmetric about the x-axis and starlike w.r.t. $\eta =1$ and $Re\left({\mathbb{E}}_{\kappa }(\eta )\right)>0$ ∀ $\eta \in \mathbb{D}$, moreover, ${\mathbb{E}}_{\kappa }^{\prime }(0)=\kappa >0$. This illustrates that ${\mathbb{E}}_{\kappa }\in \mathcal{P}.$

Example 1.

(i)

If $\kappa <0.28$, then $E_2(\eta )+\kappa \eta$ is not a convex function, see Figure 2a;

(ii)

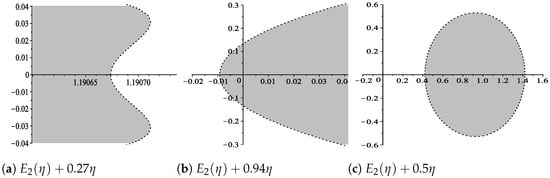
If $\kappa >0.92$, then $\exists \eta \in \mathbb{D}$ s.t. $Re\left(E_2(\eta )+\kappa \eta \right)\ngtr 0$, see Figure 2b;

(iii)

If $0.28\le \kappa \le 0.92$, then $Re\left(E_2(\eta )+\kappa \eta \right)>0$ ∀ $\eta \in \mathbb{D}$, also $E_2(\eta )+\kappa \eta$ is a convex function, see Figure 2c.

Figure 2. ${\mathbb{E}}_{\kappa }(\mathbb{D})$ by three different values of $\kappa$.

Every univalent function $\varphi \in \mathcal{S}$ of the shape (1) is known to have an inverse ${\varphi }^{-1}(w)$ defined in $\left(|w|<r_0(\varphi );r_0(\varphi )\ge {\displaystyle \frac{1}{4}}\right)$ where

$$\psi (w)={\varphi }^{-1}(w)=w-c_2{w}^2+\left(2c_2^2-c_3\right)w^3-\left(5c_2^3-5c_2{c}_3+c_4\right)w^4+\dots$$

(6)

A function $\varphi \in \mathcal{S}$ is bi-univalent in $\mathbb{D}$ if there exists a function $\psi \in \mathcal{S}$ such that $\psi (\eta )$ is a univalent extension of ${\varphi }^{-1}$ to $\mathbb{D}$. $\mathsf{\Sigma }$ is a representation of the class of bi-univalent functions in $\mathbb{D}$. The class $\mathsf{\Sigma }$ includes the functions

$${\displaystyle \frac{\eta }{1-\eta }},-log(1-\eta )and{\displaystyle \frac{1}{2}}log\left({\displaystyle \frac{1+\eta }{1-\eta }}\right),$$

(details in [12]). But the well-known Koebe function is not bi-univalent. A bound $|c_2| \leqq 1.51$ was found by Lewin [13] while studying the class of bi-univalent functions $\sigma$. Inspired by Lewin’s [13] research, Brannan and Clunie [14] hypothesised that $|c_2| \leqq \sqrt{2}.$ The coefficient estimation problem ([12]) for $|c_m|(m\in \mathbb{N},m\geqq 3)$ still requires further effort. Brannan and Taha [15] provided estimates for the initial coefficients of numerous subclasses of the bi-univalent function class $\mathsf{\Sigma }$. Many types of bi-univalent functions have been introduced and studied recently; bi-univalent function study has benefited greatly from the work of Srivastava et al. [12]. Many researchers who recently investigated a large number of important subclasses of the class $\mathsf{\Sigma }$ were motivated by this, as they found non-sharp estimates on the first two Taylor–Maclaurin coefficients (see [12,16,17,18,19], and the references cited therein).

Furthermore, Joshi et al. [20] recently described a unique class of bi-pseudo-starlike functions and discovered bounds for the initial coefficients $|c_2|$ and $|c_3|$. This study uses the second Einstein function. In order to determine the bounds for the initial coefficients of $|c_2|$ and $|c_3|$ for $\varphi \in {\mathcal{B}}_{\mathsf{\Sigma }}^{\lambda }(\gamma ,\kappa )$, we define and study the class ${\mathcal{B}}_{\mathsf{\Sigma }}^{\lambda }(\gamma ,\kappa )$. We also discussed the Fekete–Szegö problem in this article.

Definition 1.

Given $\varphi \in \mathsf{\Sigma }$ where ${({\varphi }^{\prime }(\eta ))}^{\lambda }$ ($\lambda \ge 1$) is analytic in $\mathbb{D}$ such that ${({\varphi }^{\prime }(0))}^{\lambda }=1$. Moreover, let ψ be an extension of ${\varphi }^{-1}$ to $\mathbb{D}$ where ${({\psi }^{\prime }(\eta ))}^{\lambda }$ is analytic in $\mathbb{D}$ with ${({\psi }^{\prime }(0))}^{\lambda }=1$. Then $\varphi \in {\mathcal{B}}_{\mathsf{\Sigma }}^{\lambda }(\gamma ,\kappa )$ of λ-bi-pseudo-starlike functions if each of the below circumstances holds true:

$${\displaystyle \frac{\eta {({\varphi }^{\prime }(\eta ))}^{\lambda }}{(1-\gamma )\eta +\gamma \varphi (\eta )}}\prec {\mathbb{E}}_{\kappa }(\eta )(\eta \in \mathbb{D}),$$

(7)

and

$${\displaystyle \frac{w{({\psi }^{\prime }(w))}^{\lambda }}{(1-\gamma )w+\gamma \psi (w)}}\prec {\mathbb{E}}_{\kappa }(w)(w\in \mathbb{D}),$$

(8)

where $0\le \gamma \le 1$.

Remark 1.

If $\lambda =1,$ then $\varphi \in \mathsf{\Sigma }$ is in the class ${\mathcal{B}}_{\mathsf{\Sigma }}^1(\gamma ,\kappa )\equiv {\mathcal{M}}_{\mathsf{\Sigma }}(\gamma ,\kappa )$ if both of the subsequent two criteria are fulfilled:

$$\begin{array}{c}\hfill {\displaystyle \frac{\eta {\varphi }^{\prime }(\eta )}{(1-\gamma )\eta +\gamma \varphi (\eta )}}\prec {\mathbb{E}}_{\kappa }(\eta ),\end{array}$$

(9)

and

$$\begin{array}{c}\hfill {\displaystyle \frac{w{g}^{\prime }(w)}{(1-\gamma )w+\gamma \psi (w)}}\prec {\mathbb{E}}_{\kappa }(w),\end{array}$$

(10)

where $\eta ,w\in \mathbb{D}$ and ψ is expressed by (6).

Remark 2.

If $\lambda =1;\gamma =1,$ then $\varphi \in \mathsf{\Sigma }$ is in the class ${\mathcal{B}}_{\mathsf{\Sigma }}^1(1,\kappa )\equiv {\mathcal{S}}_{\mathsf{\Sigma },\kappa }^{*}$ if both of the subsequent two criteria are fulfilled:

$${\displaystyle \frac{\eta {\varphi }^{\prime }(\eta )}{\varphi (\eta )}}\prec {\mathbb{E}}_{\kappa }(\eta ),$$

(11)

and

$${\displaystyle \frac{w{g}^{\prime }(w)}{\psi (w)}}\prec {\mathbb{E}}_{\kappa }(w),$$

(12)

where $\eta ,w\in \mathbb{D}$ and ψ is expressed by (6).

Remark 3.

If $\gamma =0,$ then $\varphi \in \mathsf{\Sigma }$ is in the class ${\mathcal{B}}_{\mathsf{\Sigma }}^{\lambda }(0,\kappa )\equiv {\mathcal{R}}_{\mathsf{\Sigma },\kappa }^{\lambda }$ if the following conditions are satisfied:

$${({\varphi }^{\prime }(\eta ))}^{\lambda }\prec {\mathbb{E}}_{\kappa }(\eta )and{({\psi }^{\prime }(w))}^{\lambda }\prec {\mathbb{E}}_{\kappa }(w),$$

(13)

where $\eta ,w\in \mathbb{D}$ and ψ is expressed by (6).

Remark 4.

For $\lambda =1;\gamma =0,$ then $\varphi \in \mathsf{\Sigma }$ is in the class ${\mathcal{B}}_{\mathsf{\Sigma }}^1(0)\equiv {\mathcal{N}}_{\mathsf{\Sigma },\kappa }$ if both of the subsequent two criteria are fulfilled:

$${\varphi }^{\prime }(\eta )\prec {\mathbb{E}}_{\kappa }(\eta )and{\psi }^{\prime }(w)\prec {\mathbb{E}}_{\kappa }(w),$$

(14)

where $\eta ,w\in \mathbb{D}$ and ψ is expressed by (6).

2. Coefficient Estimates for $\mathit{\varphi }\mathbf{\in }{\mathcal{B}}_{\mathsf{\Sigma }}^{\mathit{\lambda }}\mathbf{(}\mathit{\gamma }\mathbf{,}\mathit{\kappa }\mathbf{)}$

Unless otherwise mentioned, we let $\lambda \ge 1$, $0\le \gamma \le 1$, $\kappa \in [0.28,0.92]$, and $\eta ,\omega \in \mathbb{D}$. Also, we recall the following lemmas:

Lemma 1

([21,22]). Let $l_1,l_2\in \mathbb{R}$ and $p_1,p_2\in \mathbb{C}$. If $|p_1|,|p_2|<\zeta$, then

$$\left|(l_1+l_2)p_1+(l_1-l_2)p_2\right|\le \left\{\begin{array}{ccc}2|l_1|\zeta ,& & |l_1|\ge |l_2|,\\ 2|l_2|\zeta ,& & |l_1|\le |l_2|.\end{array}\right.$$

Lemma 2

([23]). Assume that on the unit open disc $\mathbb{D}$, $\chi (\eta )$ is analytic, and $\chi (0)=0$.$|\chi (\eta )|<1$, also

$$\chi (\eta )={\rho }_1\eta +\sum _{m=2}^{\infty }{\rho }_m{\eta }^{m}forall\eta \in \mathbb{D},$$

(15)

then

$$|{\rho }_1|\le 1,\mathrm{and}|{\rho }_m|\le 1-|{\rho }_1{|}^2\left(m\in \mathbb{N}\setminus \{1\}\right).$$

(16)

Theorem 1.

Let $\varphi (\eta )$ given in (1). If $\varphi \in {\mathcal{B}}_{\mathsf{\Sigma }}^{\lambda }(\gamma ,\kappa )$, then

$$|c_2|\le min\{{\displaystyle \frac{\kappa }{2\lambda -\gamma }};\sqrt{{\displaystyle \frac{2\kappa }{|\left[4{\lambda }^2+2\lambda (1-2\gamma )-2\gamma (1-\gamma )\right]+\frac{{(2\lambda -\gamma )}^2}{6{\kappa }^2}|+\frac{2{(2\lambda -\gamma )}^2}{\kappa }}}}\},$$

(17)

$$\begin{array}{ccc}\hfill |c_3|& \le & \left\{\begin{array}{ccc}{\displaystyle \frac{2\kappa }{3\lambda -\gamma }}& ,& {\displaystyle \frac{2(2\lambda -\gamma )}{\kappa (3\lambda -\gamma )}}\ge 1,\\ & & \\ \left[{\displaystyle \frac{2\kappa (3\lambda -\gamma )-4(2\lambda -\gamma )}{\mathsf{\Lambda }(\lambda ,\gamma ,\kappa )(3\lambda -\gamma )}}\right]+{\displaystyle \frac{2\kappa }{3\lambda -\gamma }}& ,& {\displaystyle \frac{2(2\lambda -\gamma )}{\kappa (3\lambda -\gamma )}}<1,\end{array}\right.\hfill \end{array}$$

(18)

where

$$\mathsf{\Lambda }(\lambda ,\gamma ,\kappa )=|\left[4{\lambda }^2+2\lambda (1-2\gamma )-2\gamma (1-\gamma )\right]+{\displaystyle \frac{{(2\lambda -\gamma )}^2}{6{\kappa }^2}}|+{\displaystyle \frac{2{(2\lambda -\gamma )}^2}{\kappa }}.$$

Proof. 

Let $\psi$ be of the form

$$\psi (w)=w-c_2{w}^2+\left(2c_2^2-c_3\right)w^3-\left(5c_2^3-5c_2{c}_3+c_4\right)w^4+\dots .$$

Since $\varphi \in {\mathcal{B}}_{\mathsf{\Sigma }}^{\lambda }(\gamma ,\kappa )$, there exist analytic functions $u,v:\mathbb{D}\to \mathbb{D}$, with $u(0)=0=v(0)$, such that $|u(\eta )|<1$, $|v(\eta )|<1$, given by

$$u(\eta )=\sum _{m=1}^{\infty }{p}_m{\eta }^m,\mathrm{and}v(\omega )=\sum _{m=1}^{\infty }{q}_m{\omega }^m,$$

Through straightforward calculation, we get

$$\begin{array}{}\left(19\right)& \hfill \kappa u(\eta )+E_2(u(\eta ))& =& 1+\kappa u(\eta )-{\displaystyle \frac{{(u(\eta ))}^2}{12}}+{\displaystyle \frac{{(u(\eta ))}^4}{240}}+\cdots \hfill \left(20\right)& & =& 1+\kappa p_1\eta +\left(\kappa p_2-{\displaystyle \frac{p_1^2}{12}}\right){\eta }^2+\cdots ,\hfill \end{array}$$

and

$$\begin{array}{}\left(21\right)& \hfill \kappa v(\omega )+E_2(v(\omega ))& =& 1+\kappa v(\omega )-{\displaystyle \frac{{(v(\omega ))}^2}{12}}+{\displaystyle \frac{{(v(\omega ))}^4}{240}}+\cdots \hfill \left(22\right)& & =& 1+\kappa q_1\omega +\left(\kappa q_2-{\displaystyle \frac{q_1^2}{12}}\right){\omega }^2+\cdots .\hfill \end{array}$$

$$\begin{array}{c}\hfill {\displaystyle \frac{\eta {[{\varphi }^{\prime }(\eta )]}^{\lambda }}{(1-\gamma )\eta +\gamma \varphi (\eta )}}=\kappa u(\eta )+E_2(u(\eta )),\end{array}$$

(23)

$$\begin{array}{c}\hfill {\displaystyle \frac{w{[{\psi }^{\prime }(w)]}^{\lambda }}{(1-\gamma )w+\gamma \psi (w)}}=\kappa v(\omega )+E_2(v(\omega )).\end{array}$$

(24)

However, we have

$${\displaystyle \frac{\eta {[{\varphi }^{\prime }(\eta )]}^{\lambda }}{(1-\gamma )\eta +\gamma \varphi (\eta )}}$$

$$=1+(2\lambda -\gamma )c_2\eta +[\left(2{\lambda }^2-2\lambda (\gamma +1)+{\gamma }^2\right)c_2^2+(3\lambda -\gamma )c_3]{\eta }^2+\cdots ,$$

(25)

and

$${\displaystyle \frac{w{[{\psi }^{\prime }(w)]}^{\lambda }}{(1-\gamma )w+\gamma \psi (w)}}$$

$$=1-(2\lambda -\gamma )c_{2}w+[\left(2{\lambda }^2+(2\lambda -\gamma )(2-\gamma )\right)c_2^2-(3\lambda -\gamma )c_3]w^2+\cdots .$$

(26)

Using (19), (21), (25) and (26) and comparing the like coefficients of $\eta$ and ${\eta }^2$, we get

$$\begin{array}{ccc}\hfill (2\lambda -\gamma )c_2& =& \kappa p_1,\hfill \end{array}$$

(27)

$$\begin{array}{c}\hfill \left(2{\lambda }^2-2\lambda (\gamma +1)+{\gamma }^2\right)c_2^2+(3\lambda -\gamma )c_3=\kappa p_2-{\displaystyle \frac{p_1^2}{12}},\end{array}$$

(28)

$$\begin{array}{ccc}\hfill -(2\lambda -\gamma )c_2& =& \kappa q_1,\hfill \end{array}$$

(29)

$$\begin{array}{c}\hfill \left(2{\lambda }^2+(2\lambda -\gamma )(2-\gamma )\right)c_2^2-(3\lambda -\gamma )c_3=\kappa q_2-{\displaystyle \frac{q_1^2}{12}}.\end{array}$$

(30)

From (27) and (29), we find that

$$c_2={\displaystyle \frac{\kappa p_1}{2\lambda -\gamma }}=-{\displaystyle \frac{\kappa q_1}{2\lambda -\gamma }};$$

it follows that

$$p_1=-q_1,$$

(31)

and

$$2{(2\lambda -\gamma )}^2{c}_2^2={\kappa }^2(p_1^2+q_1^2).$$

(32)

Thus,

$$c_2^2={\displaystyle \frac{{\kappa }^2(p_1^2+q_1^2)}{2{(2\lambda -\gamma )}^2}}(or)p_1^2+q_1^2={\displaystyle \frac{2{(2\lambda -\gamma )}^2}{{\kappa }^2}}{c}_2^2(or)p_1^2={\displaystyle \frac{{(2\lambda -\gamma )}^2}{{\kappa }^2}}{c}_2^2.$$

(33)

Also,

$$|c_2{|}^2\le {\displaystyle \frac{{\kappa }^2}{{(2\lambda -\gamma )}^2}},|c_2|\le {\displaystyle \frac{\kappa }{2\lambda -\gamma }}.$$

(34)

Adding (28) and (30), we have

$$\begin{array}{ccc}& & \left[4{\lambda }^2+2\lambda (1-2\gamma )-2\gamma (1-\gamma )\right]c_2^2=\kappa (p_2+q_2)-{\displaystyle \frac{1}{12}}\left(p_1^2+q_1^2\right).\hfill \end{array}$$

(35)

Substituting (31) and (33) in (35), we get

$$\begin{array}{cc}\hfill & \left[4{\lambda }^2+2\lambda (1-2\gamma )-2\gamma (1-\gamma )\right]c_2^2=\kappa (p_2+q_2)-{\displaystyle \frac{{\kappa }^2}{6}}{(2\lambda -\gamma )}^2{c}_2^2,\hfill \\ \hfill & \left(\left[4{\lambda }^2+2\lambda (1-2\gamma )-2\gamma (1-\gamma )\right]+{\displaystyle \frac{{(2\lambda -\gamma )}^2}{6{\kappa }^2}}\right)c_2^2=\kappa (p_2+q_2),\hfill \\ \hfill & \left|\left[4{\lambda }^2+2\lambda (1-2\gamma )-2\gamma (1-\gamma )\right]+{\displaystyle \frac{{(2\lambda -\gamma )}^2}{6{\kappa }^2}}\right||c_2{|}^2=2\kappa (1-|p_1{|}^2),\hfill \\ \hfill & \{\left|\left[4{\lambda }^2+2\lambda (1-2\gamma )-2\gamma (1-\gamma )\right]+{\displaystyle \frac{{(2\lambda -\gamma )}^2}{6{\kappa }^2}}\right|+{\displaystyle \frac{2{(2\lambda -\gamma )}^2}{\kappa }}\}{|c_2|}^2=2\kappa .\hfill \end{array}$$

(36)

Hence,

$$c_2^2={\displaystyle \frac{2\kappa }{|\left[4{\lambda }^2+2\lambda (1-2\gamma )-2\gamma (1-\gamma )\right]+\frac{{(2\lambda -\gamma )}^2}{6{\kappa }^2}|+\frac{2{(2\lambda -\gamma )}^2}{\kappa }}}.$$

(37)

Applying Lemma 2 in (37),

$$|c_2|\le \sqrt{{\displaystyle \frac{2\kappa }{|\left[4{\lambda }^2+2\lambda (1-2\gamma )-2\gamma (1-\gamma )\right]+\frac{{(2\lambda -\gamma )}^2}{6{\kappa }^2}|+\frac{2{(2\lambda -\gamma )}^2}{\kappa }}}},$$

we get the desired inequality (17).

Subtracting (28) from (30), and using (33), we obtain

$$\begin{array}{}& \hfill c_3& =& c_2^2+{\displaystyle \frac{\kappa (p_2-q_2)}{2(3\lambda -\gamma )}}\hfill \left(38\right)& & =& c_2^2+{\displaystyle \frac{\kappa (p_2-q_2)}{2(3\lambda -\gamma )}},\hfill & \hfill |c_3|& \le & \left[1-{\displaystyle \frac{2(2\lambda -\gamma )}{\kappa (3\lambda -\gamma )}}\right]{|c_2|}^2+{\displaystyle \frac{2\kappa }{3\lambda -\gamma }}\hfill \left(39\right)& & =& \left[{\displaystyle \frac{2\kappa (3\lambda -\gamma )-4(2\lambda -\gamma )}{\mathsf{\Lambda }(\lambda ,\gamma ,\kappa )(3\lambda -\gamma )}}\right]+{\displaystyle \frac{2\kappa }{3\lambda -\gamma }},\hfill \end{array}$$

where

$$\mathsf{\Lambda }(\lambda ,\gamma ,\kappa )=|\left[4{\lambda }^2+2\lambda (1-2\gamma )-2\gamma (1-\gamma )\right]+{\displaystyle \frac{{(2\lambda -\gamma )}^2}{6{\kappa }^2}}|+{\displaystyle \frac{2{(2\lambda -\gamma )}^2}{\kappa }}.$$

By virtue of (37), the result is obtained. Again, by (31), we have $p_1^2=q_1^2$ and applying Lemma 2, the required inequality is obtained by using (38). The proof of Theorem 1 is therefore fulfilled. □

By the restriction $\lambda =1$, then we consider the next corollary:

Corollary 1.

Let ϕ in (1). If $\varphi \in {\mathcal{M}}_{\mathsf{\Sigma }}(\gamma ,\kappa )$, then

$$\begin{array}{ccc}\hfill |c_2|& \le & min\{{\displaystyle \frac{\kappa }{2-\gamma }};\sqrt{{\displaystyle \frac{2\kappa }{|\left[4+2(1-2\gamma )-2\gamma (1-\gamma )\right]+\frac{{(2-\gamma )}^2}{6{\kappa }^2}|+\frac{2{(2-\gamma )}^2}{\kappa }}}}\},\hfill \end{array}$$

(40)

$$\begin{array}{ccc}\hfill |c_3|& \le & \left\{\begin{array}{ccc}{\displaystyle \frac{2\kappa }{3-\gamma }},& & {\displaystyle \frac{2(2-\gamma )}{\kappa (3-\gamma )}}\ge 1,\\ & & \\ \left[{\displaystyle \frac{2\kappa (3-\gamma )-4(2-\gamma )}{\mathsf{\Lambda }(1,\gamma ,\kappa )(3-\gamma )}}\right]+{\displaystyle \frac{2\kappa }{3-\gamma }},& & {\displaystyle \frac{2(2-\gamma )}{\kappa (3-\gamma )}}<1,\end{array}\right.\hfill \end{array}$$

(41)

where

$$\mathsf{\Lambda }(1,\gamma ,\kappa )=|\left[4+2(1-2\gamma )-2\gamma (1-\gamma )\right]+{\displaystyle \frac{{(2-\gamma )}^2}{6{\kappa }^2}}|+{\displaystyle \frac{2{(2-\gamma )}^2}{\kappa }}.$$

By the restriction $\lambda =1=\gamma$, then we consider the next corollary:

Corollary 2.

Let ϕ be the function expressed in (1). If $\varphi \in {\mathcal{B}}_{\mathsf{\Sigma }}^1(1,\kappa )\equiv {\mathcal{S}}_{\mathsf{\Sigma },\kappa }^{*}$, then

$$\begin{array}{ccc}\hfill |c_2|& \le & min\{\kappa ;\sqrt{{\displaystyle \frac{2\kappa }{2+\frac{1}{6{\kappa }^2}+\frac{2}{\kappa }}}}\},\hfill \\ \hfill |c_3|& \le & \left\{\begin{array}{ccc}2\kappa ,& & {\displaystyle \frac{1}{\kappa }}\ge 1,\\ & & \\ {\displaystyle \frac{2(\kappa -1)}{2+\frac{1}{6{\kappa }^2}+\frac{2}{\kappa }}}+\kappa ,& & {\displaystyle \frac{1}{\kappa }}<1,\end{array}\right.\hfill \end{array}$$

By taking $\lambda =1$ and $\gamma =0$, we have the following:

Corollary 3.

Let ϕ expressed in (1). If $\varphi \in {\mathcal{N}}_{\mathsf{\Sigma },\kappa }$, then

$$\begin{array}{ccc}\hfill |c_2|& \le & min\{{\displaystyle \frac{\kappa }{2}};\sqrt{{\displaystyle \frac{2\kappa }{6+\frac{2}{3{\kappa }^2}+\frac{8}{\kappa }}}}\},\hfill \end{array}$$

(42)

$$\begin{array}{ccc}\hfill |c_3|& \le & \left\{\begin{array}{ccc}{\displaystyle \frac{2\kappa }{3}},& & {\displaystyle \frac{4}{3\kappa }}\ge 1,\\ & & \\ {\displaystyle \frac{6\kappa -8}{3\left(6+\frac{2}{3{\kappa }^2}+\frac{8}{\kappa }\right)}}+{\displaystyle \frac{2\kappa }{3}},& & {\displaystyle \frac{4}{3\kappa }}<1.\end{array}\right.\hfill \end{array}$$

(43)

By taking $\gamma =0$, we have the following:

Corollary 4.

Let ϕ expressed in (1). If $\varphi \in {\mathcal{R}}_{\mathsf{\Sigma },\kappa }^{\lambda }$, then

$$\begin{array}{ccc}\hfill |c_2|& \le & min\{{\displaystyle \frac{\kappa }{2\lambda }};\sqrt{{\displaystyle \frac{2\kappa }{4{\lambda }^2+2\lambda +\frac{2{\lambda }^2}{3{\kappa }^2}+\frac{8{\lambda }^2}{\kappa }}}}\},\hfill \end{array}$$

(44)

$$\begin{array}{ccc}\hfill |c_3|& \le & \left\{\begin{array}{ccc}{\displaystyle \frac{2\kappa }{3\lambda }},& & {\displaystyle \frac{4}{3\kappa }}\ge 1,\\ & & \\ {\displaystyle \frac{6\kappa -8}{3\left[4{\lambda }^2+2\lambda +\frac{4{\lambda }^2}{6{\kappa }^2}+\frac{8{\lambda }^2}{\kappa }\right]}}+{\displaystyle \frac{2\kappa }{3\lambda }},& & {\displaystyle \frac{4}{3\kappa }}<1.\end{array}\right.\hfill \end{array}$$

(45)

3. Fekete–Szegö Problem of ${\mathcal{B}}_{\mathsf{\Sigma }}^{\mathit{\lambda }}\mathbf{(}\mathit{\gamma }\mathbf{,}\mathit{\kappa }\mathbf{)}$

For the function class ${\mathcal{B}}_{\mathsf{\Sigma }}^{\lambda }(\gamma ,\kappa )$, we give the Fekete–Szegö functional, by using the values of $c_2^2$ and $c_3$, inspired by the latest research of Zaprawa [21,22].

Theorem 2.

Let $\delta \in \mathbb{C}$. If $\varphi \in {\mathcal{B}}_{\mathsf{\Sigma }}^{\lambda }(\gamma ,\kappa )$, then

$$|c_3-\delta c_2^2|\le \left\{\begin{array}{ccc}2\kappa |\mathsf{\Theta }(\lambda ,\gamma ,\kappa )|,& & |\mathsf{\Theta }(\lambda ,\gamma ,\kappa )|\ge |{\displaystyle \frac{1}{2(3\lambda -\gamma )}}|,\\ {\displaystyle \frac{\kappa }{|3\lambda -\gamma |}},& & |\mathsf{\Theta }(\lambda ,\gamma ,\kappa )|\le |{\displaystyle \frac{1}{2(3\lambda -\gamma )}}|,\end{array}\right.$$

(46)

where

$$\mathsf{\Theta }(\lambda ,\gamma ,\kappa )={\displaystyle \frac{1-\delta }{\left[4{\lambda }^2+2\lambda (1-2\gamma )-2\gamma (1-\gamma )\right]+\frac{{(2\lambda -\gamma )}^2}{6{\kappa }^2}}}.$$

Proof. 

From (38), we have

$$c_3=c_2^2+{\displaystyle \frac{\kappa (p_2-q_2)}{2(3\lambda -\gamma )}}.$$

Using (37), performing some calculations, we obtain

$$\begin{array}{ccc}\hfill c_3-\delta c_2^2& =& {\displaystyle \frac{\kappa (p_2-q_2)}{2(3\lambda -\gamma )}}+(1-\delta )c_2^2\hfill \\ & =& {\displaystyle \frac{\kappa (p_2-q_2)}{2(3\lambda -\gamma )}}+{\displaystyle \frac{(1-\delta )\kappa (p_2+q_2)}{\left(\left[4{\lambda }^2+2\lambda (1-2\gamma )-2\gamma (1-\gamma )\right]+\frac{{(2\lambda -\gamma )}^2}{6{\kappa }^2}\right)}}\hfill \\ & =& \kappa \left[\mathsf{\Theta }(\lambda ,\gamma ,\kappa )+{\displaystyle \frac{1}{2(3\lambda -\gamma )}}\right]p_2+\kappa \left[\mathsf{\Theta }(\lambda ,\gamma ,\kappa )+{\displaystyle \frac{1}{2(3\lambda -\gamma )}}\right]q_2,\hfill \end{array}$$

where

$$\mathsf{\Theta }(\lambda ,\gamma ,\kappa )={\displaystyle \frac{1-\delta }{\left[4{\lambda }^2+2\lambda (1-2\gamma )-2\gamma (1-\gamma )\right]+\frac{{(2\lambda -\gamma )}^2}{6{\kappa }^2}}}.$$

In view of research of Kanas et al. [24], and applying (16), Lemma 1 finishes the proof. □

Remark 5.

Let $\delta =1$. If $\varphi \in {\mathcal{B}}_{\mathsf{\Sigma }}^{\lambda }(\gamma ,\kappa )$, then

$$|c_3-c_2^2|\le {\displaystyle \frac{\kappa }{|3\lambda -\gamma |}}.$$

(47)

4. Conclusions

A novel class ${\mathcal{B}}_{\mathsf{\Sigma }}^{\lambda }(\gamma ,\kappa )$ of $\lambda$-pseudo bi-starlike functions is presented, using the second Einstein function. The bounds for $|c_2|$ and $|c_3|$ are found. Additionally, for $\varphi \in {\mathcal{B}}_{\mathsf{\Sigma }}^{\lambda }(\gamma ,\kappa )$, we estimate the Fekete–Szegö-type inequalities regarding these functions. As future work, specializing the parameters $\lambda ,\gamma$ suitably, as mentioned in Remarks 1–4, we will be able to investigate the Fekete–Szegö functional for the classes stated in Remarks 1–4, which have not yet been studied for the function class associated with Einstein function. Also, interested readers can investigate the corresponding results according to the first Einstein function.